A Comprehensive Review of In Situ Measurement Techniques for Evaluating the Electro-Chemo-Mechanical Behaviors of Battery Electrodes
Abstract
1. Introduction
2. Multi-Beam Optical Stress Sensor (MOSS)
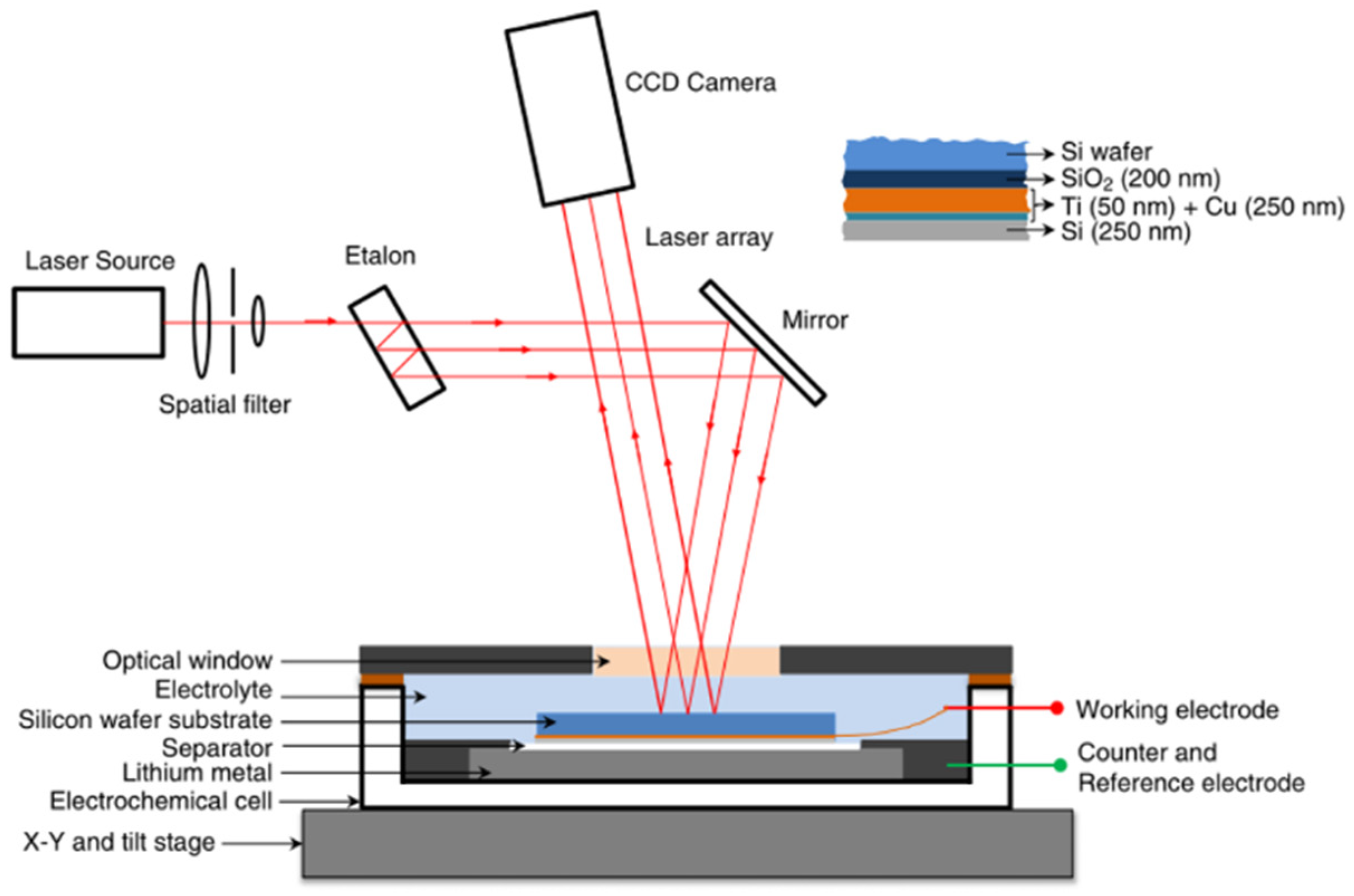
2.1. Experimental Device and Mathematical Model
2.1.1. The Experimental Device and Basic Theory of the MOSS
2.1.2. Mathematical Model for the MOSS
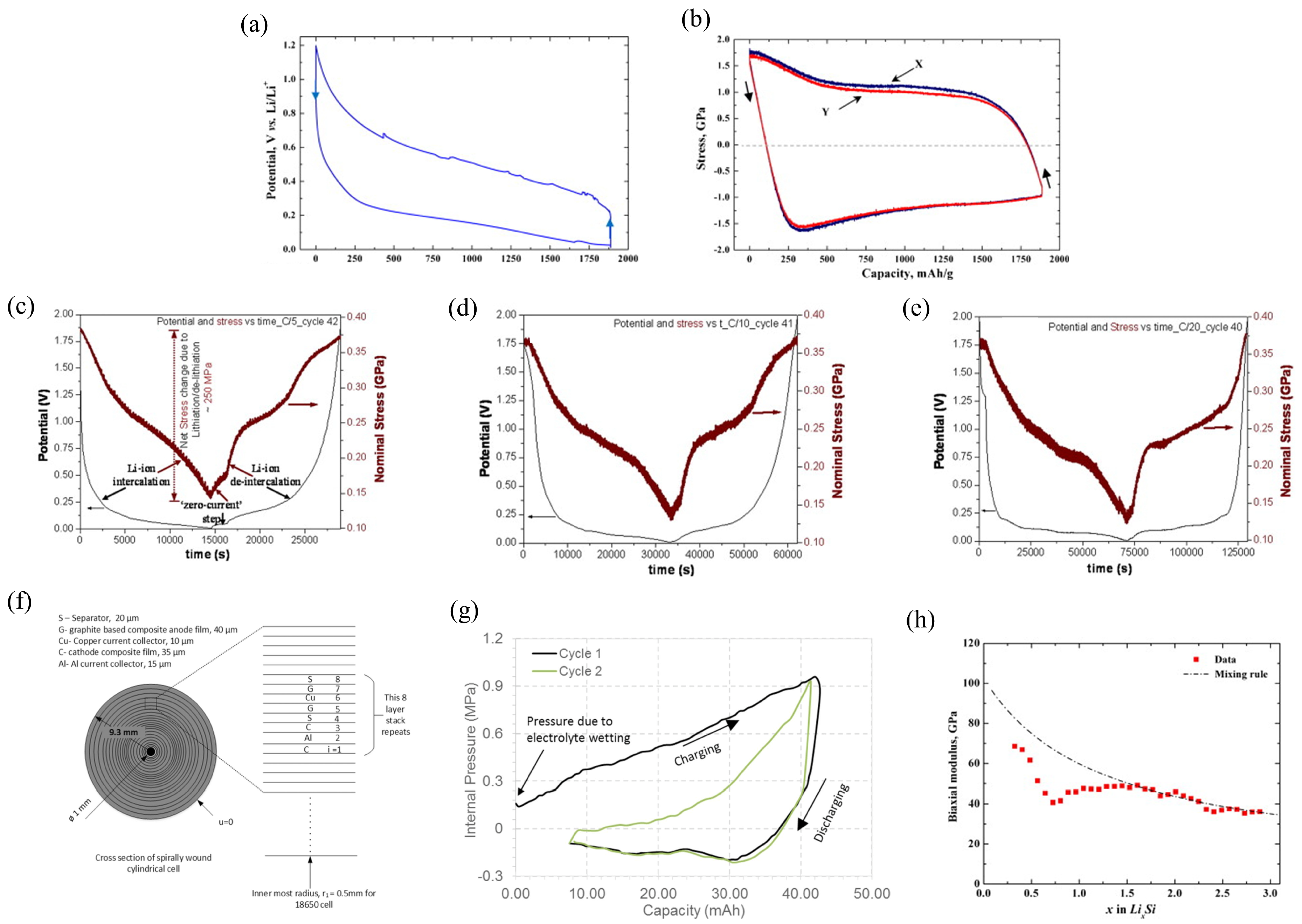
2.2. Stress and the Biaxial Modulus in the Lithium-Ion Battery
2.2.1. Potential Stress of Battery Electrode
2.2.2. Internal Pressure for Spirally Wound Battery
2.2.3. The Biaxial Module of the Silicon Thin-Film Electrode
2.3. Applications of the Moss Measurement System in Battery Materials
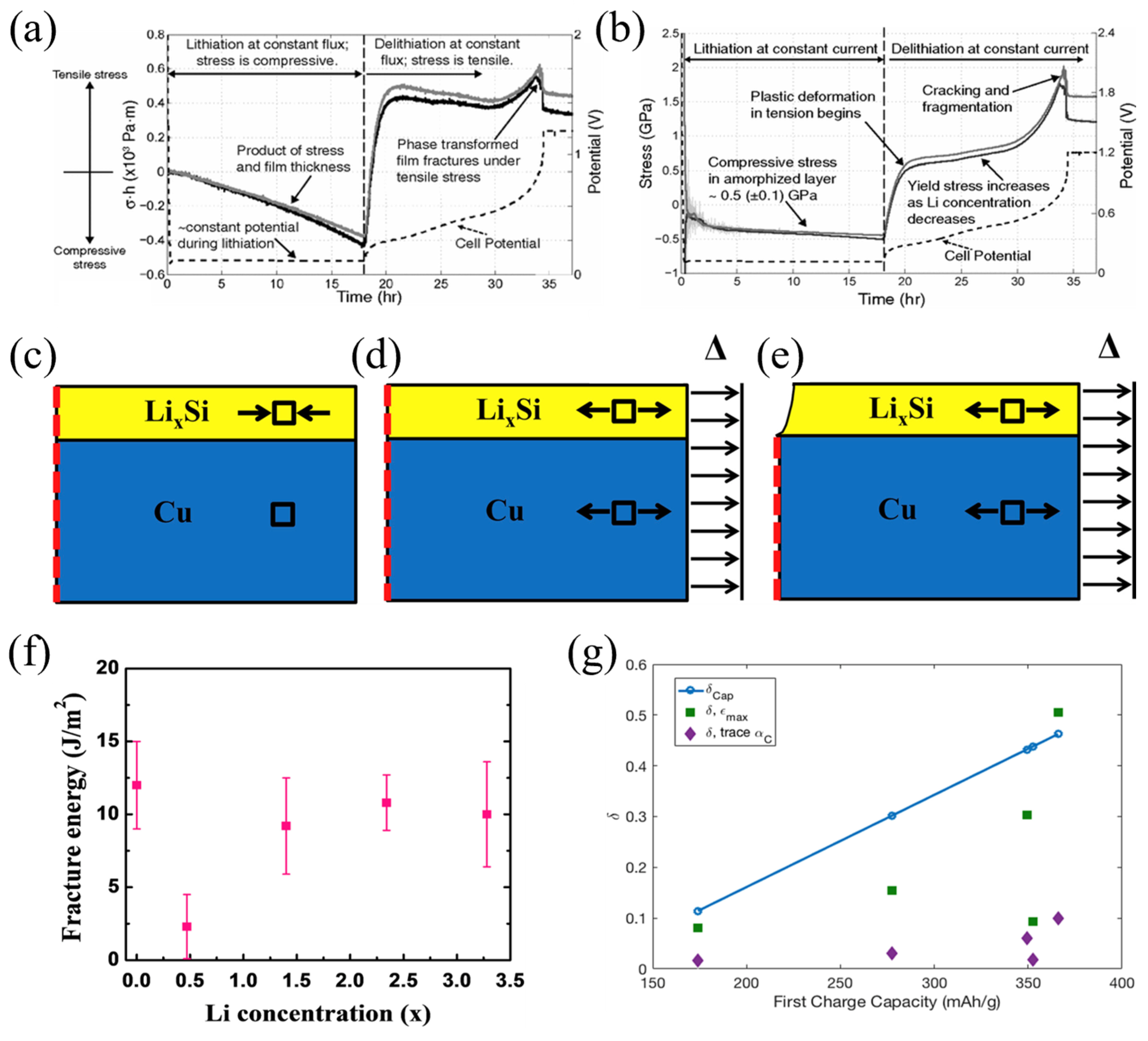
2.3.1. Phase Transformation in Si Wafer
2.3.2. Fracture Energy of the Si Electrode
2.3.3. Oxygen Vacancy Measurement by MOSS
3. Digital Image Correlation (DIC)
3.1. Experimental Device and Mathematical Model
3.2. Strain of the Graphite Electrodes
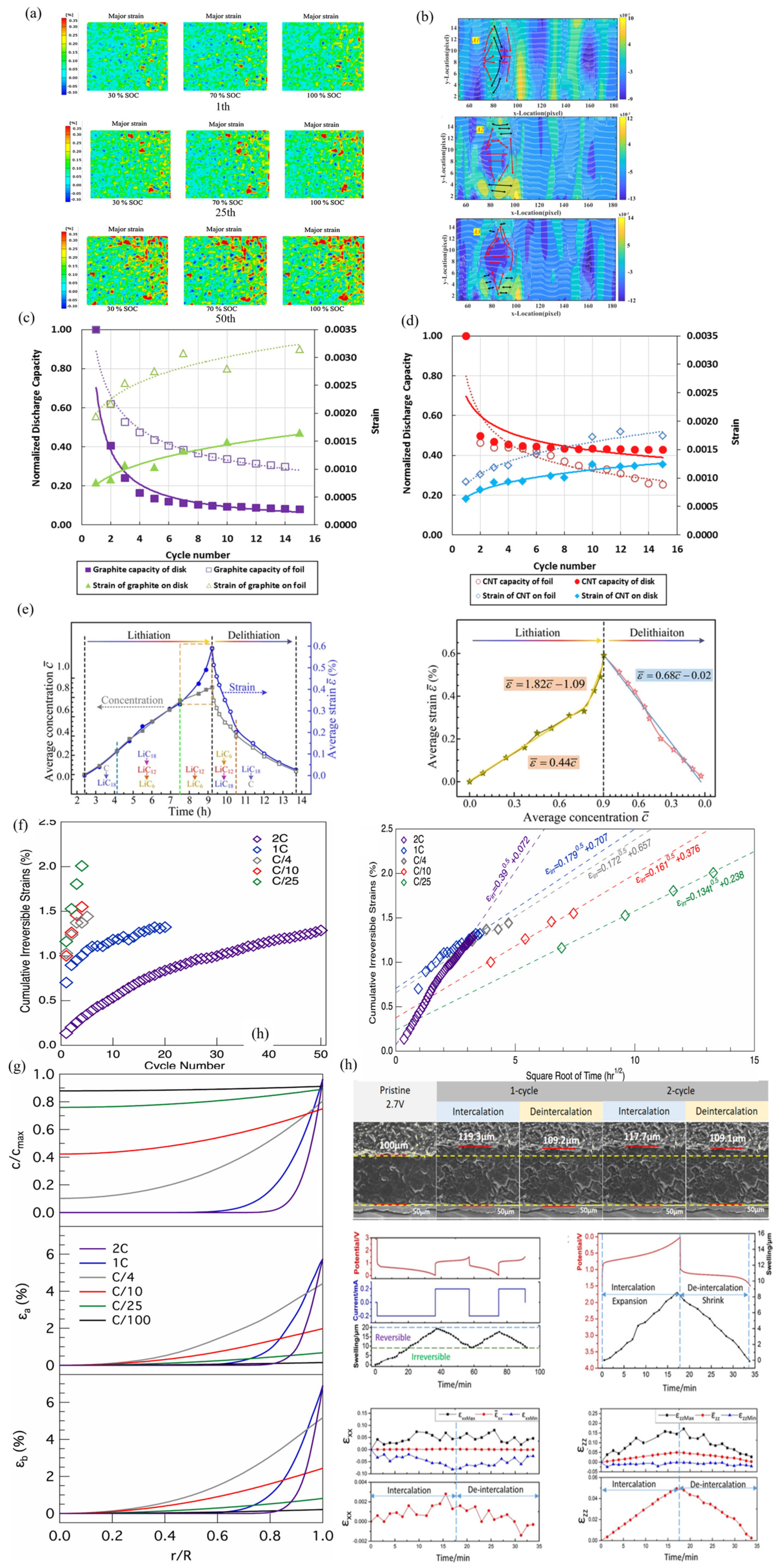
3.3. Lithium-Ion Concentration and Stress
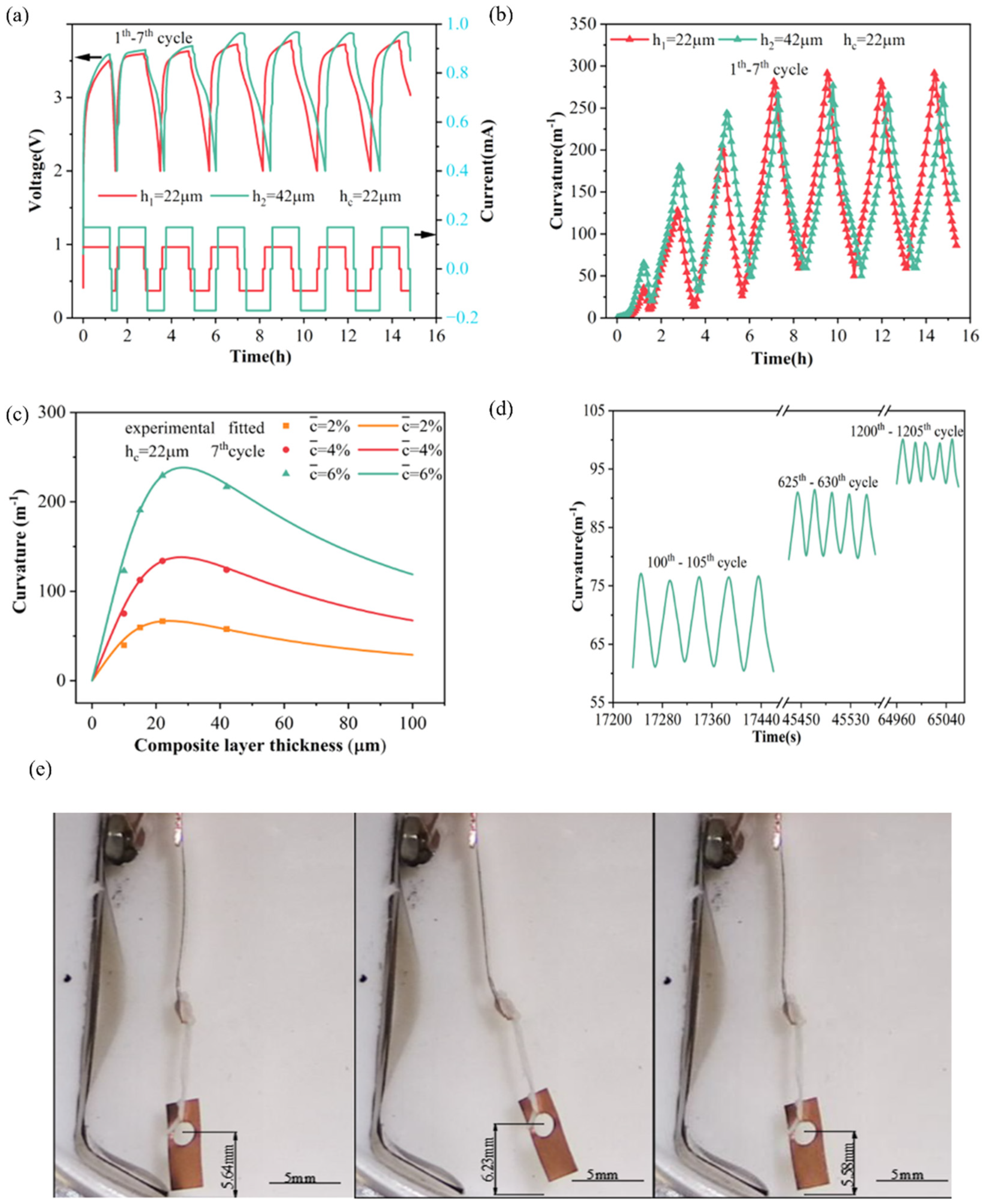
4. Bending Curvature Measurement System (BCMS)
4.1. Experimental Device and Mathematical Model
4.2. Modulus and Stress
4.3. Partial Molar Volume
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dresselhaus, M.S.; Thomas, I. Alternative energy technologies. Nature 2001, 414, 332–337. [Google Scholar] [CrossRef] [PubMed]
- Mukhopadhyay, A.; Sheldon, B.W. Deformation and stress in electrode materials for Li-ion batteries. Prog. Mater. Sci. 2014, 63, 58–116. [Google Scholar] [CrossRef]
- Kang, K.; Meng, Y.S.; Breger, J.; Grey, C.P.; Ceder, G. Electrodes with high power and high capacity for rechargeable lithium batteries. Science 2006, 311, 977–980. [Google Scholar] [CrossRef] [PubMed]
- Tarascon, J.-M.; Armand, M. Issues and challenges facing rechargeable lithium batteries. Nature 2001, 414, 359–367. [Google Scholar] [CrossRef] [PubMed]
- Wachsman, E.D.; Lee, K.T. Lowering the temperature of solid oxide fuel cells. Science 2011, 334, 935–939. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zhang, Q.; Li, D.; Hu, J.; Xu, J.; Dang, D.; Xiao, X.; Cheng, Y.T. Mechanical property evolution of silicon composite electrodes studied by environmental nanoindentation. Adv. Energy Mater. 2018, 8, 1702578. [Google Scholar] [CrossRef]
- Cui, Z.W.; Gao, F.; Qu, J.M. A finite deformation stress-dependent chemical potential and its applications to lithium ion batteries. J. Mech. Phys. Solids 2012, 60, 1280–1295. [Google Scholar] [CrossRef]
- Bower, A.F.; Guduru, P.R.; Sethuraman, V.A. A finite strain model of stress, diffusion, plastic flow, and electrochemical reactions in a lithium-ion half-cell. J. Mech. Phys. Solids 2011, 59, 804–828. [Google Scholar] [CrossRef]
- Harris, S.J.; Timmons, A.; Baker, D.R.; Monroe, C. Direct in situ measurements of Li transport in Li-ion battery negative electrodes. Chem. Phys. Lett. 2010, 485, 265–274. [Google Scholar] [CrossRef]
- He, Y.-L.; Hu, H.; Song, Y.-C.; Guo, Z.-S.; Liu, C.; Zhang, J.-Q. Effects of concentration-dependent elastic modulus on the diffusion of lithium ions and diffusion induced stress in layered battery electrodes. J. Power Sources 2014, 248, 517–523. [Google Scholar] [CrossRef]
- Xiong, R.; Pan, Y.; Shen, W.; Li, H.; Sun, F. Lithium-ion battery aging mechanisms and diagnosis method for automotive applications: Recent advances and perspectives. Renew. Sustain. Energy Rev. 2020, 131, 110048. [Google Scholar] [CrossRef]
- Vykhodtsev, A.V.; Jang, D.; Wang, Q.; Rosehart, W.; Zareipour, H. A review of modelling approaches to characterize lithium-ion battery energy storage systems in techno-economic analyses of power systems. Renew. Sustain. Energy Rev. 2022, 166, 112584. [Google Scholar] [CrossRef]
- Yang, Y.; Zhou, Q.; Zhang, L.; Du, D.; Zheng, M.; Niu, Q.; Gao, L.; Yuan, X. Recent progresses in state estimation of lithium-ion battery energy storage systems: A review. Trans. Inst. Meas. Control 2022, 01423312221124354. [Google Scholar] [CrossRef]
- Zhao, K.J.; Pharr, M.; Vlassak, J.J.; Suo, Z.G. Fracture of electrodes in lithium-ion batteries caused by fast charging. J. Appl. Phys. 2010, 108, 073517. [Google Scholar] [CrossRef]
- Li, A.G.; West, A.C.; Preindl, M. Towards unified machine learning characterization of lithium-ion battery degradation across multiple levels: A critical review. Appl. Energy 2022, 316, 119030. [Google Scholar] [CrossRef]
- O’Kane, S.E.; Ai, W.; Madabattula, G.; Alonso-Alvarez, D.; Timms, R.; Sulzer, V.; Edge, J.S.; Wu, B.; Offer, G.J.; Marinescu, M. Lithium-ion battery degradation: How to model it. Phys. Chem. Chem. Phys. 2022, 24, 7909–7922. [Google Scholar] [CrossRef] [PubMed]
- Zheng, H.; Tan, L.; Liu, G.; Song, X.; Battaglia, V.S. Calendering effects on the physical and electrochemical properties of Li Ni1/3Mn1/3Co1/3 O2 cathode. J. Power Sources 2012, 208, 52–57. [Google Scholar] [CrossRef]
- Song, J.; Han, X.; Gaskell, K.J.; Xu, K.; Lee, S.B.; Hu, L. Enhanced electrochemical stability of high-voltage LiNi0.5Mn1.5O4 cathode by surface modification using atomic layer deposition. J. Nanopart. Res. 2014, 16, 2745. [Google Scholar] [CrossRef]
- Li, J.; Yang, M.; Zhang, X.; Wen, J.; Wang, C.; Huang, G.; Song, W. First-Principles Study of the Effect of Ni-Doped on the Spinel-Type Mn-Based Cathode Discharge. ACS Appl. Mater. Interfaces 2023, 15, 8208–8216. [Google Scholar] [CrossRef] [PubMed]
- Sim, R.; Lee, S.; Li, W.; Manthiram, A. Influence of Calendering on the Electrochemical Performance of LiNi0.9Mn0.05Al0.05O2 Cathodes in Lithium-Ion Cells. ACS Appl. Mater. Interfaces 2021, 13, 42898–42908. [Google Scholar] [CrossRef] [PubMed]
- Stoney, G.G. The tension of metallic films deposited by electrolysis. Proc. R. Soc. Lond. Ser. A Contain. Pap. A Math. Phys. Character 1909, 82, 172–175. [Google Scholar]
- Freund, L.B.; Suresh, S. Thin Film Materials: Stress, Defect Formation and Surface Evolution; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Sethuraman, V.A.; Nguyen, A.; Chon, M.J.; Nadimpalli, S.P.; Wang, H.; Abraham, D.P.; Bower, A.F.; Shenoy, V.B.; Guduru, P.R. Stress evolution in composite silicon electrodes during lithiation/delithiation. J. Electrochem. Soc. 2013, 160, A739. [Google Scholar] [CrossRef]
- Li, H.; Zhu, C.; Xu, G.; Luo, L. Experimental identification of thermal induced warpage in polymer–metal composite films. Microelectron. Reliab. 2016, 62, 141–147. [Google Scholar] [CrossRef]
- Pan, B.; Wu, D.; Xia, Y. An active imaging digital image correlation method for deformation measurement insensitive to ambient light. Opt. Laser Technol. 2012, 44, 204–209. [Google Scholar] [CrossRef]
- Song, H.; Liu, C.; Zhang, H.; Yang, X.; Chen, Y.; Leen, S.B. Experimental investigation on damage evolution in pre-corroded aluminum alloy 7075-T7651 under fatigue loading. Mater. Sci. Eng. A 2021, 799, 140206. [Google Scholar] [CrossRef]
- Jangid, M.K.; Mukhopadhyay, A. Real-time monitoring of stress development during electrochemical cycling of electrode materials for Li-ion batteries: Overview and perspectives. J. Mater. Chem. A 2019, 7, 23679–23726. [Google Scholar] [CrossRef]
- Jones, E.; Silberstein, M.; White, S.R.; Sottos, N.R. In situ measurements of strains in composite battery electrodes during electrochemical cycling. Exp. Mech. 2014, 54, 971–985. [Google Scholar] [CrossRef]
- Chen, J.; Thapa, A.K.; Berfield, T.A. In-situ characterization of strain in lithium battery working electrodes. J. Power Sources 2014, 271, 406–413. [Google Scholar] [CrossRef]
- Li, D.; Wang, Y.; Hu, J.; Lu, B.; Cheng, Y.-T.; Zhang, J. In situ measurement of mechanical property and stress evolution in a composite silicon electrode. J. Power Sources 2017, 366, 80–85. [Google Scholar] [CrossRef]
- Li, D.; Wang, Y. In-situ measurements of mechanical property and stress evolution of commercial graphite electrode. Mater. Des. 2020, 194, 108887. [Google Scholar] [CrossRef]
- Xie, H.; Kang, Y.; Song, H.; Guo, J.; Zhang, Q. In situ method for stress measurements in film-substrate electrodes during electrochemical processes: Key role of softening and stiffening. Acta Mech. Sin. 2020, 36, 1319–1335. [Google Scholar] [CrossRef]
- Guo, Z.; Liu, C.; Lu, B.; Feng, J. Theoretical and experimental study on the interfacial adhesive properties of graphite electrodes in different charging and aging states. Carbon 2019, 150, 32–42. [Google Scholar] [CrossRef]
- Huang, P.; Liu, C.; Guo, Z.; Feng, J. Analytical model and experimental verification of the interfacial peeling strength of electrodes. Exp. Mech. 2021, 61, 321–330. [Google Scholar] [CrossRef]
- Yang, S.-z.; Huang, Y.-f.; Han, X.-c.; Han, G.-h. Enhancing electrochemical performance of SnO2 anode with humic acid modification. Trans. Nonferrous Met. Soc. China 2021, 31, 2062–2073. [Google Scholar] [CrossRef]
- S Bhattacharyya, A.; P Kumar, R.; Acharya, G.; Ranjan, V. Nanoindentation and Scratch test on Thin Film Energy Materials. Curr. Smart Mater. 2017, 2, 39–43. [Google Scholar] [CrossRef]
- Bessette, S.; Hovington, P.; Demers, H.; Golozar, M.; Bouchard, P.; Gauvin, R.; Zaghib, K. In-situ characterization of lithium native passivation layer in a high vacuum scanning electron microscope. Microsc. Microanal. 2019, 25, 866–873. [Google Scholar] [CrossRef] [PubMed]
- Dai, C.; Zhu, X.; Pan, J.; Liao, X.; Pan, Y. Mechanical Abuse Simulation and Effect of Graphene Oxides on Thermal Runaway of Lithium ion Batteries. Int. J. Electrochem. Sci. 2019, 14, 3363–3374. [Google Scholar] [CrossRef]
- Gabbardo, A.D.; Frankel, G. Hydrogen evolution on bare Mg surfaces using the scratched electrode technique. Corros. Sci. 2020, 164, 108321. [Google Scholar] [CrossRef]
- Sharifi, H.; Aliofkhazraei, M.; Darband, G.B.; Shrestha, S. A review on adhesion strength of peo coatings by scratch test method. Surf. Rev. Lett. 2018, 25, 1830004. [Google Scholar] [CrossRef]
- Hovington, P.; Dontigny, M.; Guerfi, A.; Trottier, J.; Lagacé, M.; Mauger, A.; Julien, C.; Zaghib, K. In situ Scanning electron microscope study and microstructural evolution of nano silicon anode for high energy Li-ion batteries. J. Power Sources 2014, 248, 457–464. [Google Scholar] [CrossRef]
- Tsuda, T.; Kanetsuku, T.; Sano, T.; Oshima, Y.; Ui, K.; Yamagata, M.; Ishikawa, M.; Kuwabata, S. In situ SEM observation of the Si negative electrode reaction in an ionic-liquid-based lithium-ion secondary battery. Microscopy 2015, 64, 159–168. [Google Scholar] [CrossRef] [PubMed]
- Gómez-Cámer, J.L.; Bünzli, C.; Hantel, M.M.; Poux, T.; Novák, P. On the correlation between electrode expansion and cycling stability of graphite/Si electrodes for Li-ion batteries. Carbon 2016, 105, 42–51. [Google Scholar] [CrossRef]
- Zhou, X.; Li, T.; Cui, Y.; Fu, Y.; Liu, Y.; Zhu, L. In situ focused ion beam scanning electron microscope study of microstructural evolution of single tin particle anode for Li-ion batteries. ACS Appl. Mater. Interfaces 2019, 11, 1733–1738. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.-Y.; Sano, T.; Tsuda, T.; Ui, K.; Oshima, Y.; Yamagata, M.; Ishikawa, M.; Haruta, M.; Doi, T.; Inaba, M. In situ scanning electron microscopy of silicon anode reactions in lithium-ion batteries during charge/discharge processes. Sci. Rep. 2016, 6, 36153. [Google Scholar] [CrossRef] [PubMed]
- Shao, M. In situ microscopic studies on the structural and chemical behaviors of lithium-ion battery materials. J Power Sources 2014, 270, 475–486. [Google Scholar] [CrossRef]
- Harris, S.J.; Rahani, E.K.; Shenoy, V.B. Direct in situ observation and numerical simulations of non-shrinking-core behavior in an MCMB graphite composite electrode. J. Electrochem. Soc. 2012, 159, A1501. [Google Scholar] [CrossRef]
- Yang, L.; Chen, H.-S.; Jiang, H.; Wei, Y.-J.; Song, W.-L.; Fang, D.-N. Failure mechanisms of 2D silicon film anodes: In situ observations and simulations on crack evolution. Chem. Commun. 2018, 54, 3997–4000. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Zeng, W.; Hong, L.; Xu, W.; Yang, H.; Wang, F.; Duan, H.; Tang, M.; Jiang, H. Stress-driven lithium dendrite growth mechanism and dendrite mitigation by electroplating on soft substrates. Nat. Energy 2018, 3, 227–235. [Google Scholar] [CrossRef]
- Li, N.; Xin, Y.; Chen, H.; Jiao, S.; Jiang, H.; Song, W.-L.; Fang, D. Thickness evolution of graphite-based cathodes in the dual ion batteries via in operando optical observation. J. Energy Chem. 2019, 29, 122–128. [Google Scholar] [CrossRef]
- Breitung, B.; Baumann, P.; Sommer, H.; Janek, J.; Brezesinski, T. In situ and operando atomic force microscopy of high-capacity nano-silicon based electrodes for lithium-ion batteries. Nanoscale 2016, 8, 14048–14056. [Google Scholar] [CrossRef] [PubMed]
- Yoon, I.; Abraham, D.P.; Lucht, B.L.; Bower, A.F.; Guduru, P.R. In situ measurement of solid electrolyte interphase evolution on silicon anodes using atomic force microscopy. Adv. Energy Mater. 2016, 6, 1600099. [Google Scholar] [CrossRef]
- Mahankali, K.; Thangavel, N.K.; Reddy Arava, L.M. In situ electrochemical mapping of lithium–sulfur battery interfaces using AFM–SECM. Nano Lett. 2019, 19, 5229–5236. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Zhang, X.; Li, Z.; Ma, Y.; Ma, L. Recent progress of biomass-derived carbon materials for supercapacitors. J. Power Sources 2020, 451, 227794. [Google Scholar] [CrossRef]
- Hu, J.; Wang, Y.; Li, D.; Cheng, Y.-T. Effects of adhesion and cohesion on the electrochemical performance and durability of silicon composite electrodes. J. Power Sources 2018, 397, 223–230. [Google Scholar] [CrossRef]
- Zou, R.; Cui, Z.; Liu, Q.; Guan, G.; Zhang, W.; He, G.; Yang, J.; Hu, J. In situ transmission electron microscopy study of individual nanostructures during lithiation and delithiation processes. J. Mater. Chem. A 2017, 5, 20072–20094. [Google Scholar] [CrossRef]
- Wheatcroft, L.; Özkaya, D.; Cookson, J.; Inkson, B.J. Towards in-situ TEM for Li-ion Battery Research. Energy Procedia 2018, 151, 163–167. [Google Scholar] [CrossRef]
- Xie, H.; Tan, X.; Luber, E.J.; Olsen, B.C.; Kalisvaart, W.P.; Jungjohann, K.L.; Mitlin, D.; Buriak, J.M. β-SnSb for sodium ion battery anodes: Phase transformations responsible for enhanced cycling stability revealed by in situ TEM. ACS Energy Lett. 2018, 3, 1670–1676. [Google Scholar] [CrossRef]
- Ji, Y.-R.; Weng, S.-T.; Li, X.-Y.; Zhang, Q.-H.; Gu, L. Atomic-scale structural evolution of electrode materials in Li-ion batteries: A review. Rare Met. 2020, 39, 205–217. [Google Scholar] [CrossRef]
- Zheng, H.; Lu, X.; He, K. In situ transmission electron microscopy and artificial intelligence enabled data analytics for energy materials. J. Energy Chem. 2022, 68, 454–493. [Google Scholar] [CrossRef]
- Sun, Z.; Pan, J.; Chen, W.; Chen, H.; Zhou, S.; Wu, X.; Wang, Y.; Kim, K.; Li, J.; Liu, H. Electrochemical Processes and Reactions In Rechargeable Battery Materials Revealed via In Situ Transmission Electron Microscopy. Adv. Energy Mater. 2024, 14, 2303165. [Google Scholar] [CrossRef]
- Cui, J.; Zheng, H.; He, K. In situ TEM study on conversion-type electrodes for rechargeable ion batteries. Adv. Mater. 2021, 33, 2000699. [Google Scholar] [CrossRef] [PubMed]
- Sethuraman, V.A.; Srinivasan, V.; Bower, A.F.; Guduru, P.R. In Situ Measurements of Stress-Potential Coupling in Lithiated Silicon. J. Electrochem. Soc. 2010, 157, A1253–A1261. [Google Scholar] [CrossRef]
- Brantley, W.A. Calculated elastic constants for stress problems associated with semiconductor devices. J. Appl. Phys. 1973, 44, 534–535. [Google Scholar] [CrossRef]
- Beaulieu, L.Y.; Hatchard, T.D.; Bonakdarpour, A.; Fleischauer, M.D.; Dahn, J.R. Reaction of Li with alloy thin films studied by in situ AFM. J. Electrochem. Soc. 2003, 150, A1457–A1464. [Google Scholar] [CrossRef]
- Sethuraman, V.A.; Chon, M.J.; Shimshak, M.; Van Winkle, N.; Guduru, P.R. In situ measurement of biaxial modulus of Si anode for Li-ion batteries. Electrochem. Commun. 2010, 12, 1614–1617. [Google Scholar] [CrossRef]
- Sethuraman, V.A.; Chon, M.J.; Shimshak, M.; Srinivasan, V.; Guduru, P.R. In situ measurements of stress evolution in silicon thin films during electrochemical lithiation and delithiation. J. Power Sources 2010, 195, 5062–5066. [Google Scholar] [CrossRef]
- Sheth, J.; Karan, N.K.; Abraham, D.P.; Nguyen, C.C.; Lucht, B.L.; Sheldon, B.W.; Guduru, P.R. In situ stress evolution in Li1+ xMn2O4 thin films during electrochemical cycling in li-ion cells. J. Electrochem. Soc. 2016, 163, A2524. [Google Scholar] [CrossRef]
- Nadimpalli, S.P.V.; Sethuraman, V.A.; Abraham, D.P.; Bower, A.F.; Guduru, P.R. Stress Evolution in Lithium-Ion Composite Electrodes during Electrochemical Cycling and Resulting Internal Pressures on the Cell Casing. J. Electrochem. Soc. 2015, 162, A2656–A2663. [Google Scholar] [CrossRef]
- Mukhopadhyay, A.; Tokranov, A.; Sena, K.; Xiao, X.; Sheldon, B.W. Thin film graphite electrodes with low stress generation during Li-intercalation. Carbon 2011, 49, 2742–2749. [Google Scholar] [CrossRef]
- Sethuraman, V.A.; Van Winkle, N.; Abraham, D.P.; Bower, A.F.; Guduru, P.R. Real-time stress measurements in lithium-ion battery negative-electrodes. J. Power Sources 2012, 206, 334–342. [Google Scholar] [CrossRef]
- Chon, M.J.; Sethuraman, V.A.; McCormick, A.; Srinivasan, V.; Guduru, P.R. Real-time measurement of stress and damage evolution during initial lithiation of crystalline silicon. Phys. Rev. Lett. 2011, 107, 045503. [Google Scholar] [CrossRef] [PubMed]
- Choi, Y.S.; Pharr, M.; Oh, K.H.; Vlassak, J.J. A simple technique for measuring the fracture energy of lithiated thin-film silicon electrodes at various lithium concentrations. J. Power Sources 2015, 294, 159–166. [Google Scholar] [CrossRef]
- Nation, L.; Li, J.; James, C.; Qi, Y.; Dudney, N.; Sheldon, B.W. In situ stress measurements during electrochemical cycling of lithium-rich cathodes. J. Power Sources 2017, 364, 383–391. [Google Scholar] [CrossRef]
- Koga, H.; Croguennec, L.; Ménétrier, M.; Mannessiez, P.; Weill, F.; Delmas, C. Different oxygen redox participation for bulk and surface: A possible global explanation for the cycling mechanism of Li1.20Mn0.54CO0.13Ni0.13O2. J. Power Sources 2013, 236, 250–258. [Google Scholar] [CrossRef]
- Lee, E.; Persson, K.A. Structural and Chemical Evolution of the Layered Li-Excess LixMnO3 as a Function of Li Content from First-Principles Calculations. Adv. Energy Mater. 2014, 4, 1400498. [Google Scholar] [CrossRef]
- Xie, H.; Yang, W.; Kang, Y.; Zhang, Q.; Han, B.; Qiu, W. In-situ strain field measurement and mechano-electro-chemical analysis of graphite electrodes via fluorescence digital image correlation. Exp. Mech. 2021, 61, 1249–1260. [Google Scholar] [CrossRef]
- Li, C.; Yang, S.; Xin, L.; Wang, Z.; Xu, Q.; Li, L.; Wang, S. In-Situ Characterization for Solid Electrolyte Deformations in a Lithium Metal Solid-State Battery. J. Electrochem. Soc. 2021, 168, 070551. [Google Scholar] [CrossRef]
- Özdogru, B.; Dykes, H.; Gregory, D.; Saurel, D.; Murugesan, V.; Casas-Cabanas, M.; Çapraz, Ö.Ö. Elucidating cycling rate-dependent electrochemical strains in sodium iron phosphate cathodes for Na-ion batteries. J. Power Sources 2021, 507, 230297. [Google Scholar] [CrossRef]
- Tao, R.; Zhu, J.; Zhang, Y.; Song, W.-L.; Chen, H.; Fang, D. Quantifying the 2D anisotropic displacement and strain fields in graphite-based electrode via in situ scanning electron microscopy and digital image correlation. Extrem. Mech. Lett. 2020, 35, 100635. [Google Scholar] [CrossRef]
- Luo, J.; Dai, C.; Wang, Z.; Liu, K.; Mao, W.; Fang, D.; Chen, X. In-situ measurements of mechanical and volume change of LiCoO2 lithium-ion batteries during repeated charge–discharge cycling by using digital image correlation. Measurement 2016, 94, 759–770. [Google Scholar] [CrossRef]
- Yang, W.; Xie, H.; Shi, B.; Song, H.; Qiu, W.; Zhang, Q. In-situ experimental measurements of lithium concentration distribution and strain field of graphite electrodes during electrochemical process. J. Power Sources 2019, 423, 174–182. [Google Scholar] [CrossRef]
- Mao, W.; Wang, Z.; Li, C.; Zhu, X.; Dai, C.; Yang, H.; Chen, X.; Fang, D. In-situ characterizations of chemo-mechanical behavior of free-standing vanadium pentoxide cathode for lithium-ion batteries during discharge-charge cycling using digital image correlation. J. Power Sources 2018, 402, 272–280. [Google Scholar] [CrossRef]
- Dai, C.; Li, C.; Huang, H.; Wang, Z.; Zhu, X.; Liao, X.; Chen, X.; Pan, Y.; Fang, D. In situ strain measurements and stress analysis of SiO@ C composite electrodes during electrochemical cycling by using digital image correlation. Solid State Ion. 2019, 331, 56–65. [Google Scholar] [CrossRef]
- Xie, H.; Han, B.; Song, H.; Li, X.; Kang, Y.; Zhang, Q. In-situ measurements of electrochemical stress/strain fields and stress analysis during an electrochemical process. J. Mech. Phys. Solids 2021, 156, 104602. [Google Scholar] [CrossRef]
- Mukaibo, H.; Momma, T.; Shacham-Diamand, Y.; Osaka, T.; Kodaira, M. In situ stress transition observations of electrodeposited Sn-based anode materials for lithium-ion secondary batteries. Electrochem. Solid-State Lett. 2007, 10, A70. [Google Scholar] [CrossRef]
- Xie, H.; Qiu, W.; Song, H.; Tian, J. In situ measurement of the deformation and elastic modulus evolution in Si composite electrodes during electrochemical lithiation and delithiation. J. Electrochem. Soc. 2016, 163, A2685. [Google Scholar] [CrossRef]
- Xie, H.; Zhang, Q.; Song, H.; Shi, B.; Kang, Y. Modeling and in situ characterization of lithiation-induced stress in electrodes during the coupled mechano-electro-chemical process. J. Power Sources 2017, 342, 896–903. [Google Scholar] [CrossRef]
- Tavassol, H.; Jones, E.M.; Sottos, N.R.; Gewirth, A.A. Electrochemical stiffness in lithium-ion batteries. Nat. Mater. 2016, 15, 1182–1187. [Google Scholar] [CrossRef]
- Özdogru, B.; Dykes, H.; Padwal, S.; Harimkar, S.; Çapraz, Ö.Ö. Electrochemical strain evolution in iron phosphate composite cathodes during lithium and sodium ion intercalation. Electrochim. Acta 2020, 353, 136594. [Google Scholar] [CrossRef]
- Mao, W.; Zhu, X.; Zhang, Z.; Huang, H.; Dai, C.; Pan, J.; Pan, Y.; Chen, X.; Fang, D. Measurements of fracture properties of MWCNTs modified LiNi0.5Mn0.3Co0.2O2 electrodes by a modified shear lag model. Mater. Sci. Eng. A 2020, 781, 139223. [Google Scholar] [CrossRef]
- Zheng, H.; Zhang, L.; Liu, G.; Song, X.; Battaglia, V.S. Correlationship between electrode mechanics and long-term cycling performance for graphite anode in lithium ion cells. J. Power Sources 2012, 217, 530–537. [Google Scholar] [CrossRef]
- Wu, Z.; Yang, X.; Sheng, K.; Li, D. Experimental Investigation and Controllability Study of Electrochemical Actuators Based on Si/CNTs Composite Material. J. Electrochem. Energy Convers. Storage 2024, 21, 021011. [Google Scholar] [CrossRef]
- Zhang, J.; Lu, B.; Song, Y.; Ji, X. Diffusion induced stress in layered Li-ion battery electrode plates. J. Power Sources 2012, 209, 220–227. [Google Scholar] [CrossRef]
- Yu, H.; Li, J.; Jiang, H.; Li, W.; Li, G.; Li, D. Chemo-Mechanical Coupling Measurement of LiMn2O4 Composite Electrode during Electrochemical Cycling. Batteries 2023, 9, 209. [Google Scholar] [CrossRef]
- Li, D.; Wang, Y.; Lu, B.; Zhang, J. Real-time measurements of electro-mechanical coupled deformation and mechanical properties of commercial graphite electrodes. Carbon 2020, 169, 258–263. [Google Scholar] [CrossRef]
- Li, D.; Li, Z.; Song, Y.; Zhang, J. Analysis of diffusion induced elastoplastic bending of bilayer lithium-ion battery electrodes. Appl. Math. Mech. 2016, 37, 659–670. [Google Scholar] [CrossRef]
- Li, D.; Wang, Y.; Hu, J.; Lu, B.; Dang, D.; Zhang, J.; Cheng, Y.-T. Role of polymeric binders on mechanical behavior and cracking resistance of silicon composite electrodes during electrochemical cycling. J. Power Sources 2018, 387, 9–15. [Google Scholar] [CrossRef]
- Li, D.; Liu, H.; Wan, H.; Wang, Y.; Zhang, J. Real time characterization of the current collector’s role on the electro-chemo-mechanical coupling performance of Si composite electrode. J. Energy Storage 2022, 56, 106018. [Google Scholar] [CrossRef]
- Li, D.; Zhu, G.; Liu, H.; Wang, Y. Diffusion-induced stress in commercial graphite electrodes during multiple cycles measured by an in situ method. Micromachines 2022, 13, 142. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Wang, Y. Communication—Controllable Deformation of Composite Graphite Electrodes during Electrochemical Process. J. Electrochem. Soc. 2020, 167, 140511. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, G.; Li, D.; Zhang, J. An Improved Experiment for Measuring Lithium Concentration-Dependent Material Properties of Graphite Composite Electrodes. Nanomaterials 2022, 12, 4448. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Wan, H.; Liu, H.; Wang, Y.; Zhang, J. Experimental measurement of electro-chemo-mechanical properties of a composite silicon electrode in lithium ion batteries. Phys. Chem. Chem. Phys. 2022, 24, 25580–25587. [Google Scholar] [CrossRef] [PubMed]
- Yu, H.; Liu, X.; Li, D. Experimental measurement of stress evolution in silicon carbide composite electrode during electrochemical cycling. Mater. Sci. Semicond. Process. 2022, 138, 106275. [Google Scholar] [CrossRef]
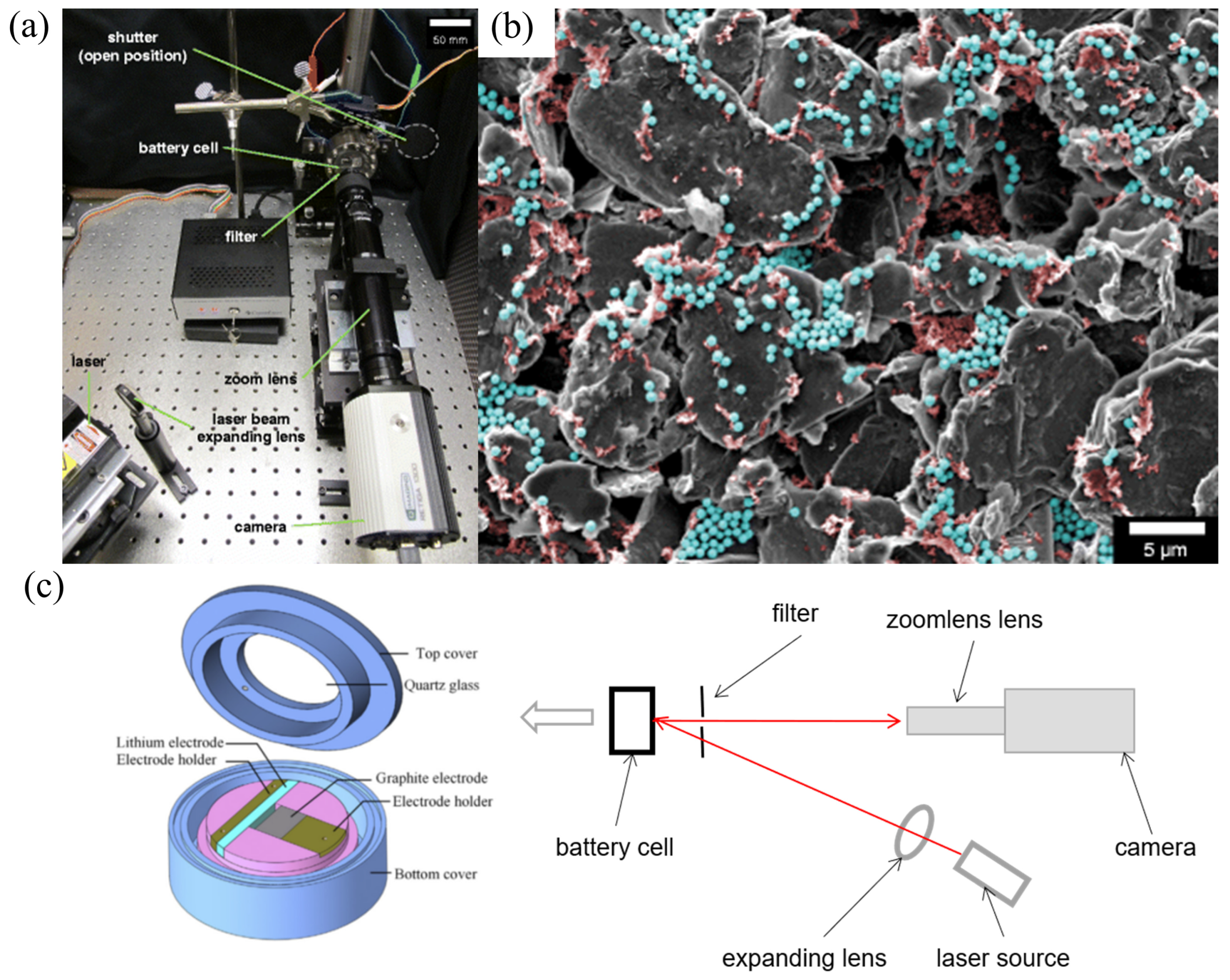


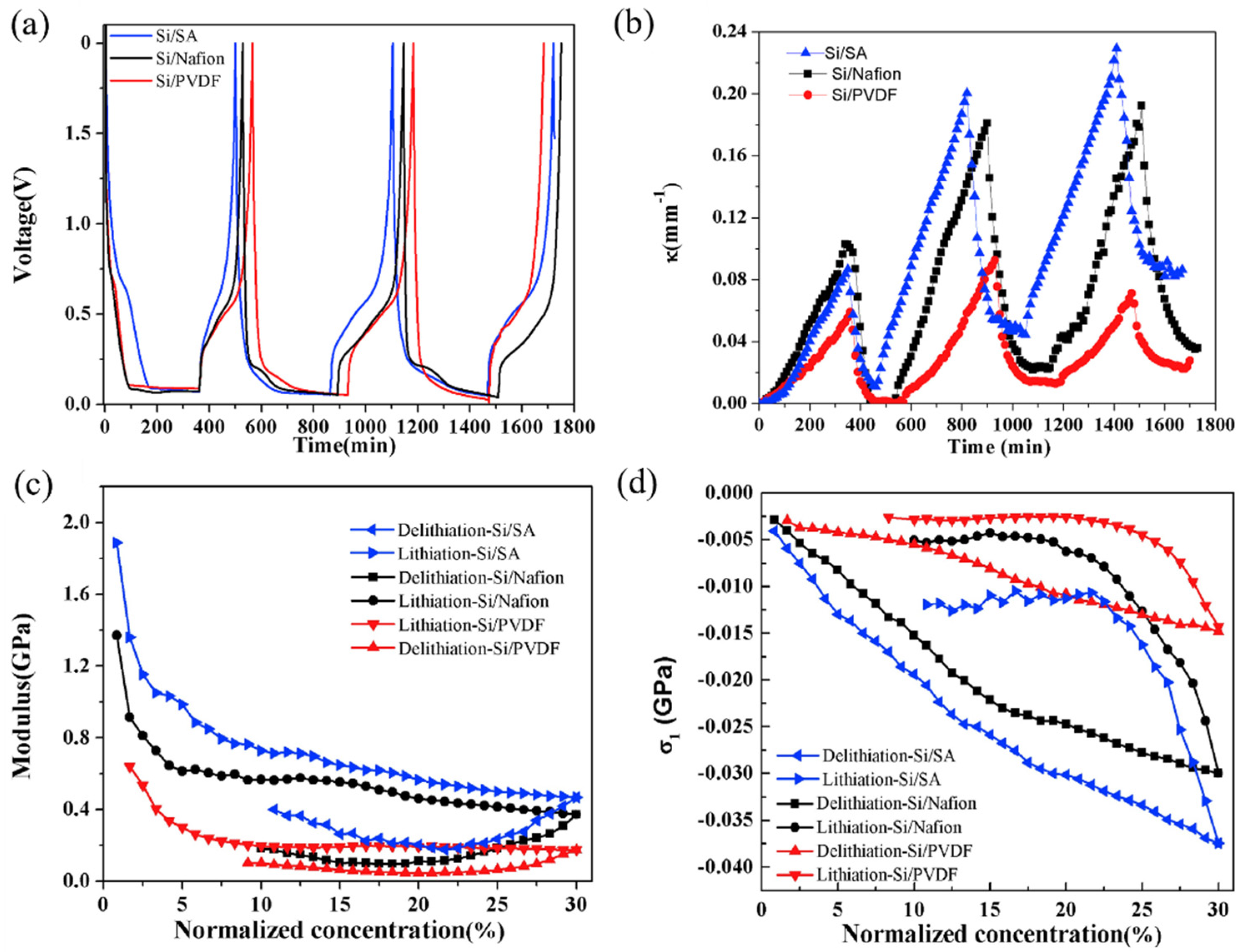
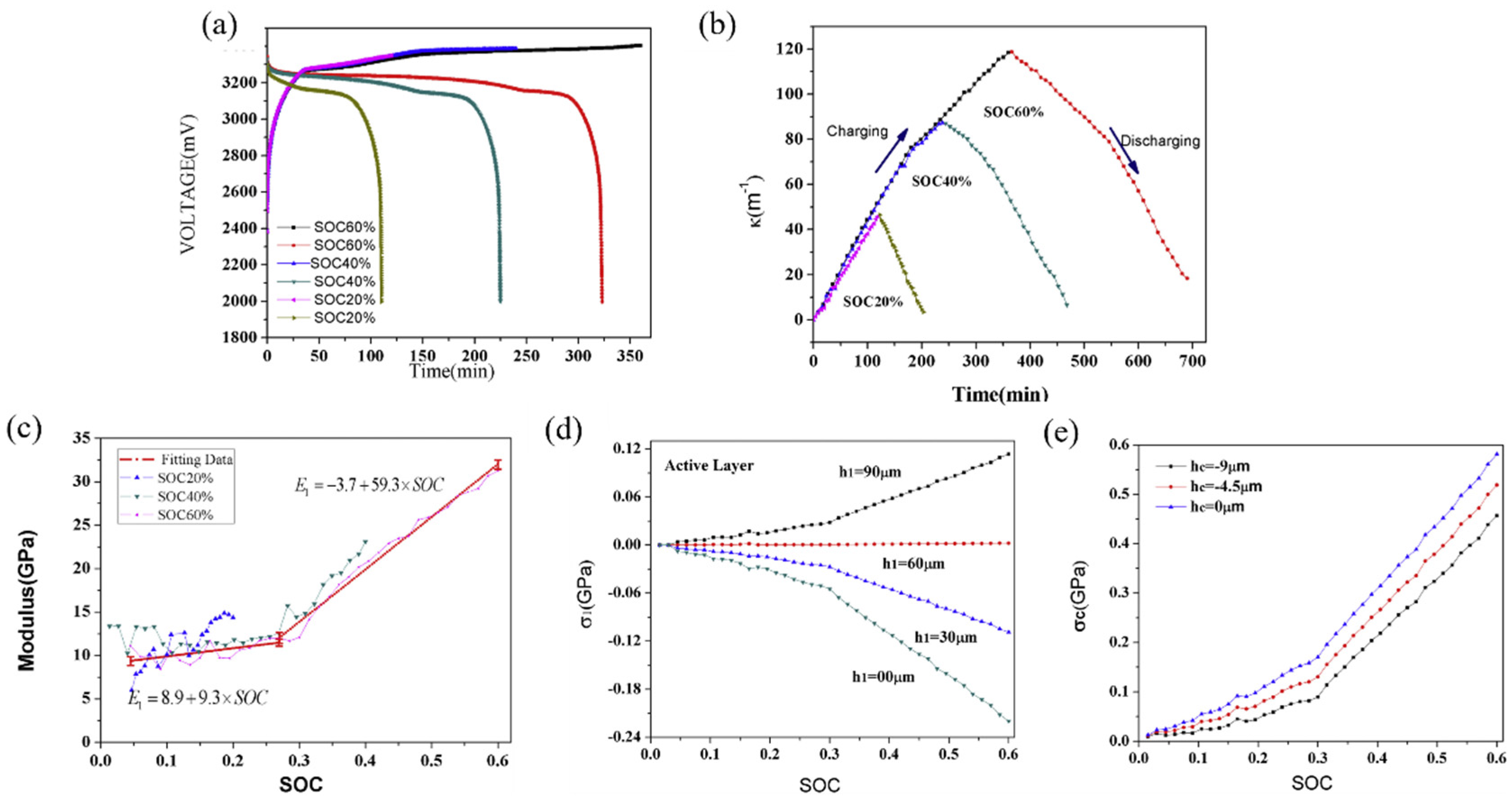

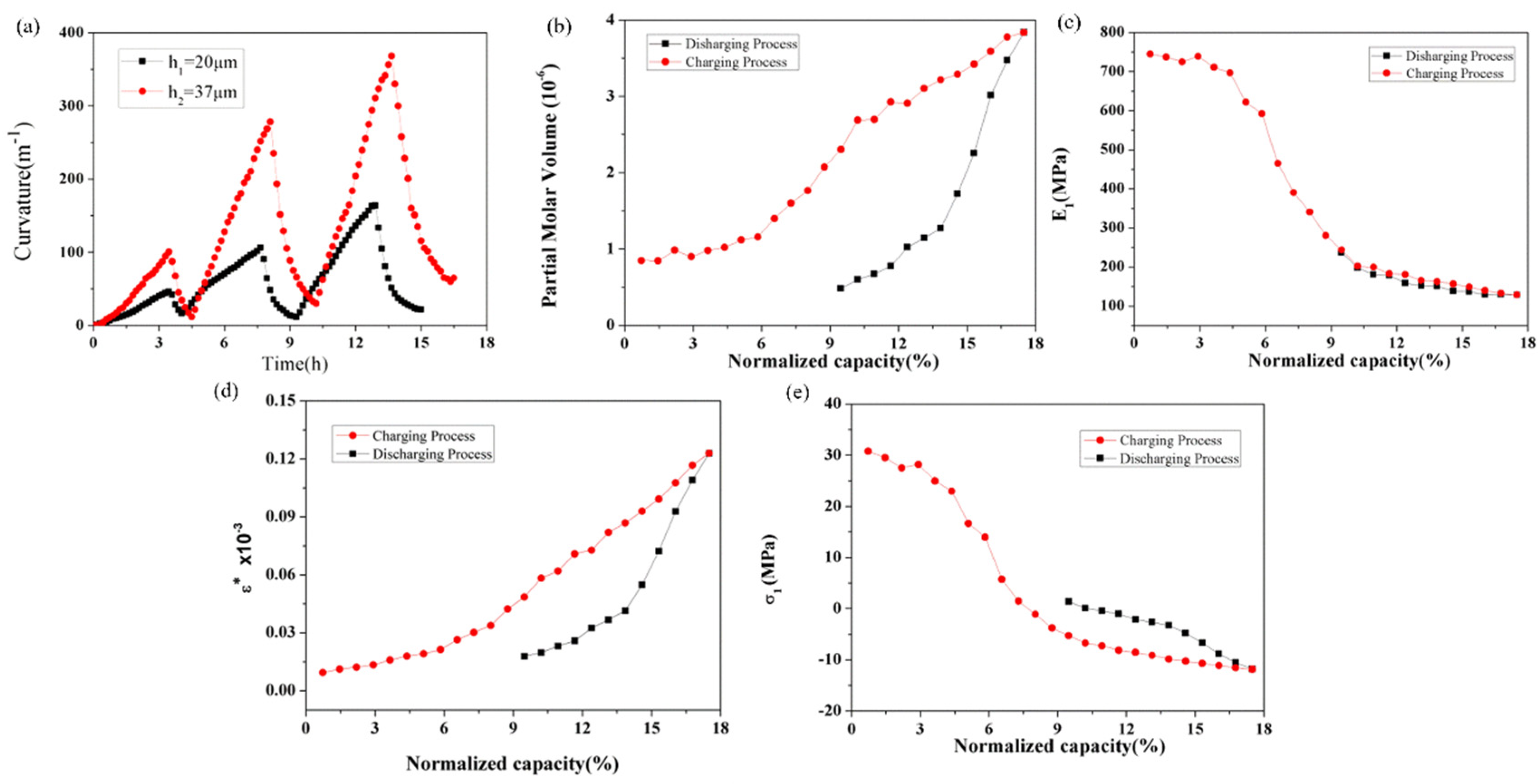

| Characterization Tool | Characteristic Length Scale | Pros | Cons |
|---|---|---|---|
| Multi-beam optical stress sensor (MOSS) | Nanometers to micrometers | Non-contact, high precision | Sensitive to disturbances, expensive |
| Digital image correlation (DIC) | Micrometers to millimeters | High-resolution deformation measurement | Requires high-quality imaging and complex processing |
| Bending curvature measurement system (BCMS) | Micrometers to centimeters | Simple operation, applicable in diverse scenarios | Slightly lower accuracy, indirect method for mechanical properties |
| Scanning electron microscope (SEM) | Nanometers to micrometers | High-resolution imaging | Vacuum operation, may alter native state, not for real-time analysis |
| Atomic force microscopy (AFM) | Nanometers | High spatial resolution, measures nanoscale mechanical properties | Limited sample area, slow, requires careful operation |
| Transmission electron microscopy (TEM) | Atomic to nanometers | Highest spatial resolution, comprehensive material characterization | Complex sample prep, vacuum operation, limited field of view, not for in situ mechanical measurements |
| Material | Parameter | Ref. | ||
|---|---|---|---|---|
| Stress (GPa) | Modulus (GPa) | Strain (%) | ||
| Si | 1.75 | 113 | 1.35 | [67,73] |
| Li0.32Si | 70 | [66] | ||
| Li0.47Si | 0.8 | 58.5 | 1.75 | [73] |
| Li1.4Si | 0.54 | 49.6 | 1.85 | [73] |
| Li2.34Si | 0.46 | 42.9 | 1.91 | [73] |
| Li3.0Si | 35 | [66] | ||
| Li3.5Si | 0.5 | [72] | ||
| Graphite | 0.25 | [70] | ||
| Li1.2Ni0.15Mn0.55Co0.1O2 | 0.006 | [69] | ||
| Battery casing (cylindrical spirally wound configuration) | 0.001 | [69] | ||
| Material | Battery State | Parameter | Ref. | ||
|---|---|---|---|---|---|
| Stress (GPa) | Strain (%) | Modulus (GPa) | |||
| Composite graphite electrodes | 1 cycle Cu | 0.253 | 0.35 | 16.7 | [81] |
| 55 cycle Cu | 0.22 | 0.27 | 10.6 | ||
| SiO@C composite electrodes | Lithiation phase 100% SOC | −0.01189 | 3.00 | 0.12 | [84] |
| Graphite electrode | 75% lithiation | −0.529 | 0.178 | 0.244 | [85] |
| 100% lithiation | −7.08 | 0.203 | 0.272 | ||
| V2O5 | 100% SOC | 0.0282 | 1.3 | 0.61858 | [83] |
| Materials | Battery State | Stress (GPa) | Partial Molar Volume (10−6) | Strain (10−3) | Modulus (GPa) | References |
|---|---|---|---|---|---|---|
| Si | 17% SOC (lithiation) | −0.0112 | 8.2 | 0.11 | 0.1 | [103] |
| 17% SOC (delithiation) | −0.0098 | 0.5 | 0.005 | 0.3 | ||
| Graphite composite electrodes | 40% SOC | 1.88 | 0.007 | 31.9 | [96] | |
| Silicon carbide composite electrodes | 40% SOC (lithiation) | −0.0148 | 0.618 | 0.38 | [104] | |
| Composite silicon electrode | 30% SOC (lithiation) | −0.015 | 0.18 | [30] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, H.; Chen, J.; Li, X.; Jin, Z.; Chen, T.; Liu, J.; Li, D. A Comprehensive Review of In Situ Measurement Techniques for Evaluating the Electro-Chemo-Mechanical Behaviors of Battery Electrodes. Molecules 2024, 29, 1873. https://doi.org/10.3390/molecules29081873
Jiang H, Chen J, Li X, Jin Z, Chen T, Liu J, Li D. A Comprehensive Review of In Situ Measurement Techniques for Evaluating the Electro-Chemo-Mechanical Behaviors of Battery Electrodes. Molecules. 2024; 29(8):1873. https://doi.org/10.3390/molecules29081873
Chicago/Turabian StyleJiang, Hainan, Jie Chen, Xiaolin Li, Zhiyao Jin, Tianjun Chen, Jiahui Liu, and Dawei Li. 2024. "A Comprehensive Review of In Situ Measurement Techniques for Evaluating the Electro-Chemo-Mechanical Behaviors of Battery Electrodes" Molecules 29, no. 8: 1873. https://doi.org/10.3390/molecules29081873
APA StyleJiang, H., Chen, J., Li, X., Jin, Z., Chen, T., Liu, J., & Li, D. (2024). A Comprehensive Review of In Situ Measurement Techniques for Evaluating the Electro-Chemo-Mechanical Behaviors of Battery Electrodes. Molecules, 29(8), 1873. https://doi.org/10.3390/molecules29081873






