Silicon-28-Tetrafluoride as an Educt of Isotope-Engineered Silicon Compounds and Bulk Materials for Quantum Systems
Abstract
1. Introduction
- (i)
- Spin vacuum: The coherence of the electron spin states is disrupted by the interference of nuclear spins, for instance, from 29Si nuclei, which results in the loss of functionality of the qubits [3]. Silicon crystals made of nuclear-spin-free stable isotopes like 28Si and 30Si are ideal for hosting spin quantum bits due to their coherent properties, which are not disrupted by host nuclear spins. The property known as spin vacuum results in sharp ensemble donor resonances and extended spin relaxation times [4]. In pure 28Si material, the theoretical maximum for qubit decoherence times is 10 h [5]. In addition to quantum computing, the 28Si spin vacuum is also employed to stabilize optically active quantum dots in silicon [6].
- (ii)
- High thermal conductivity: The phonon scattering and, consequently, the thermal conductivity are markedly changed at low temperatures in the isotopically pure materials [4,7]. The formation of scattering modes is primarily caused by the presence of impurities, doping agents, defects, and the natural mass distribution of isotopes. It is possible to reduce the impact of the former factors by optimizing the crystal growth and deposition processes. The thermal conductivity of high-purity, monocrystalline, low-defect natSi with a natural isotope distribution is 45 Wcm−1K−1 at a temperature of 21 K. With the use of isotopically pure 28Si, the thermal conductivity at 21 K can be increased to 450 Wcm−1K−1 when the material is 99.99% pure. This value exceeds the previous maximum conductivity of 12C diamond at 104 K of 410 Wcm−1K−1. Consequently, the highest thermal conductivity ever measured for a dielectric was achieved [7].
- (iii)
- Sharp spectroscopic states: The analysis of photoluminescence spectra indicates that the homogeneous mass of the 28Si atoms in the crystal lattice results in a shift of the band gap by 58 meV [8]. Furthermore, high-energy measurements reveal a more concise fine structure of the non-phononic photoluminescence lines of the bound excitons. The optimized fine structure in the photoluminescence spectrum can be used for excitonic processes in isotopically pure materials with high resolution, for example, for quantum sensing.
2. Chemical Properties and Processing of 28SiF4
2.1. Synthesis and Selected Properties of SiF4 (and 28SiF4)
2.1.1. Synthesis Routes and Formation of SiF4
2.1.2. Solid State, Gas Phase, and Electronic Structure of SiF4
2.1.3. Physical and Chemical Properties
| Property | Symbol | Unit | Value | Reference |
|---|---|---|---|---|
| Melting point | mp | °C | −95.0 (sublm.) | [62] |
| Boiling point | bp | K | 177.1 | [63] |
| Critical temperature | TC | K | 259 | [64] |
| Critical pressure | pC | bar | 37.1457 | [64] |
| Vapor pressure | P° | Pa | 3.36 × 10⁵ (at 190 K) | [65] |
| Heat capacity | C | J/molK | 73.621 | [66] |
| Ionization potential | IE | eV | 15.29 ± 0.08 | [67] |
| Bond dissociation energy (homolysis) | D0 | kJ/mol | 565 | [68] |
| Appearance energy (heterolysis, SiF3+) | AE | eV | 16.2 ± 0.1 | [69] |
| Standard enthalpy of formation | ΔHf | kJ/mol | −1615 (at 298 K) | [66] |
| Standard entropy | S | J/molK | 282.76 (at 298 K) | [66] |
| Viscosity | v | m2/s | 0.404 × 106 (at 300 K; 975 kPa) | [70] |
| Density | ρ | g/cm3 | 0.00469 (at 760 mmHg) | [71] |
2.1.4. Isotope-Dependent Physical/Chemical Properties
2.1.5. Further Selected Properties of SiF4
2.2. Important Applications of SiF4
3. Enrichment
- (i)
- The vapor pressure of the substance must exceed 5−10 mmHg (≙ 0.7–1.3 kPa) as the majority of enrichment techniques require gaseous sources;
- (ii)
- The substance must not react chemically with the materials of the enrichment chamber. Enrichment techniques exploit the slight differences in the physical properties of different isotopes. To exploit these properties, the working gas is introduced into enrichment chambers, which are usually made of stainless steel or special high-temperature alloys;
- (iii)
- Most enrichment techniques exploit the mass-dependent inertia of the isotopes. For sufficient enrichment, the molecular mass of the molecules must not be less than 40 u. Higher molecular masses are usually associated with higher enrichment rates and lower energy consumption;
- (iv)
- During the enrichment process, the working gas is usually heated either purposefully or by unwanted dissipative processes. The substance to be enriched must have a sufficiently high thermal stability;
- (v)
- There should be no chemical reaction between the molecules of the working gas. Anion exchange or atom exchange would result in an incomplete enrichment. Any interaction between the molecules of the substance should be minimized.
3.1. Gas Centrifuge
3.2. Laser Separation
3.3. Gas Diffusion
3.4. Other and Discontinued Technologies
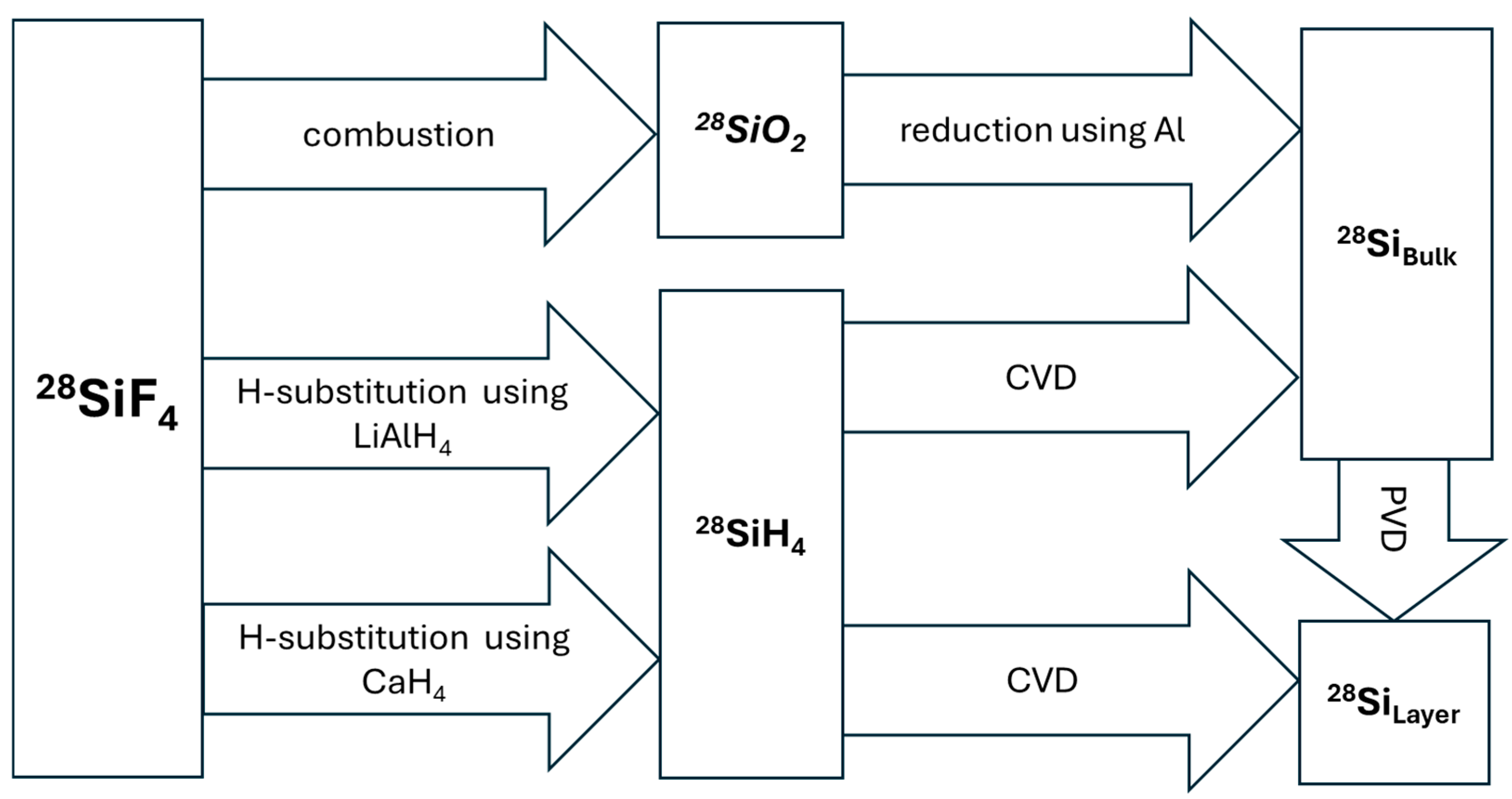
4. Conversion to Silanes and Solid Silicon
4.1. Conversion to Bulk 28Si via 28SiO2
4.2. Conversion to 28SiH4
4.3. Conversion to Bulk 28Si via 28SiH4
4.4. Conversion to Thin 28Si Layers
5. Novel Physical Properties of 28Si Resulting from Isotope Enrichment and Its Applications
5.1. Mass Distribution|Avogadro Project
5.2. Thermal Conductivity|Cryogenics
5.3. Nuclear Spin|Quantum Technologies
5.4. Further Selected Properties and Potential Applications
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Scappucci, G.; Taylor, P.J.; Williams, J.R.; Ginley, T.; Law, S. Crystalline materials for quantum computing: Semiconductor heterostructures and topological insulators exemplars. MRS Bull. 2021, 46, 596–606. [Google Scholar] [CrossRef]
- Zwerver, A.M.J.; Krähenmann, T.; Watson, T.F.; Lampert, L.; George, H.C.; Pillarisetty, R.; Bojarski, S.A.; Amin, P.; Amitonov, S.V.; Boter, J.M.; et al. Qubits made by advanced semiconductor manufacturing. Nat. Electron. 2022, 5, 184–190. [Google Scholar] [CrossRef]
- Itoh, K.M.; Watanabe, H. Isotope engineering of silicon and diamond for quantum computing and sensing applications. MRS Commun. 2014, 4, 143–157. [Google Scholar] [CrossRef]
- Inyushkin, A.V.; Taldenkov, A.N.; Gibin, A.M.; Gusev, A.V.; Pohl, H.-J. On the isotope effect in thermal conductivity of silicon. Phys. Status Solidi 2004, C 1, 2995–2998. [Google Scholar] [CrossRef]
- Kane, B. A Silicon-based nuclear spin quantum computer. Nature 1998, 393, 133–137. [Google Scholar] [CrossRef]
- Liu, Y.; Rinner, S.; Remmele, T.; Ernst, O.; Reiserer, A.; Boeck, T. 28Silicon-on-insulator for optically interfaced quantum emitters. J. Cryst. Growth 2022, 593, 126733. [Google Scholar] [CrossRef]
- Inyushkin, A.V.; Taldenkov, A.N.; Ager, J.W., III; Haller, E.E.; Riemann, H.; Abrosimov, N.V.; Pohl, H.-J.; Becker, P. Ultrahigh thermal conductivity of isotopically enriched silicon. J. Appl. Phys. 2018, 123, 095112. [Google Scholar] [CrossRef]
- Karaiskaj, D.; Thewalt, M.L.W.; Ruf, T.; Cardona, M.; Pohl, H.-J.; Deviatych, G.G.; Sennikov, P.G.; Riemann, H. Photoluminescence of Isotopically Purified Silicon: How Sharp are Bound Exciton Transitions? Phys. Rev. Lett. 2001, 86, 6010–6013. [Google Scholar] [CrossRef]
- Brooks, M. Beyond quantum supremacy: The hunt for useful quantum computers. Nature 2019, 574, 19–21. [Google Scholar] [CrossRef]
- Boudon, V.; Richard, C.; Manceron, L. High-Resolution spectroscopy and analysis of the fundamental modes of 28SiF4. Accurate experimental determination of the Si-F bond length. J. Mol. Spectrosc. 2022, 383, 111549. [Google Scholar] [CrossRef]
- Gordon, J.P.; Bowers, K.D. Microwave spin echoes from donor electrons in Silicon. Phys. Rev. Lett. 1958, 1, 368–370. [Google Scholar] [CrossRef]
- Sennikov, P.G.; Kornev, R.A.; Abrosimov, N.V. Production of stable silicon and germanium isotopes via their enriched volatile compounds. J. Radioanal. Nucl. Chem. 2015, 306, 21–30. [Google Scholar] [CrossRef]
- Kohen, A.; Limbach, H.-H. (Eds.) Isotope Effects in Chemistry and Biology, 1st ed.; CRC Press LLC.: Boca Raton, FL, USA, 2006; p. 1074. ISBN 0-8247-2449-6. [Google Scholar]
- Yu, J. Production of silicon tetrafluoride (translated from Chinese). Wujiyan Gongye 2006, 38, 1–4. [Google Scholar]
- Chen, X.-H.; Li, Y.; Zhang, J.-G.; Gao, W.-L.; Cao, X.; Chen, Y.; Liu, Z.-D. Preparation and purification of silicon tetrafluoride (translated from Chinese). Guisuanyan Tongbao 2015, 34, 1891–1895. [Google Scholar]
- Han, J.; Li, X.; Wang, J. Research and development on process of silicon tetrafluoride and the progress thereof (translated from Chinese). Wujiyan Gongye 2013, 45, 4–7. [Google Scholar]
- Loginov, A.V.; Garbar, A.M. Silicon tetrafluoride. Properties, synthesis, and use (translated from Russian). Vysok. Veshchestva 1989, 5, 27–34. [Google Scholar]
- Gelmboldt, V.O.; Ennan, A.A. Silicon tetrafluoride: Prospects for its processing and use as chemical reagent (translated from Russian). Ekotek. I Resur. 2004, 2, 28–34. [Google Scholar]
- Davy, H. An Account of Some Experiments on Different Combinations of Fluoric Acid. Philos. Trans. R. Soc. Lond. 1812, 102, 352–369. [Google Scholar] [CrossRef]
- Jeffes, J.H.E.; Richardson, F.D.; Pearson, J. The Heats of Formation of Manganous Orthosilicate and Manganous Sulphide. J. Trans. Faraday Soc. 1954, 50, 364. [Google Scholar] [CrossRef]
- Joshi, A.N. A review of processes for separation and utilization of fluorine from phosphoric acid and phosphate fertilizers. Chem. Pap. 2022, 76, 6033–6045. [Google Scholar] [CrossRef]
- Ruscic, B.; Pinzon, R.E.; Morton, M.L.; von Laszewski, G.; Bittner, S.; Nijsure, S.G.; Amin, K.A.; Minkoff, M.; Wagner, A.F. Introduction to Active Thermochemical Tables: Several “Key” Enthalpies of Formation Revisited. J. Phys. Chem. A 2004, 108, 9979–9997. [Google Scholar] [CrossRef]
- Padma, D.K.; Kalbandkeri, R.G.; Suresh, B.S.; Bhat, V.S. Low temperature fluorination of some non-metals and non-metal compounds with fluorine. Indian J. Chem. Sect. A Inorg. Phys. Theor. Anal. 1991, 30, 172–176. [Google Scholar]
- Magomedbekov, E.P.; Chizhevskaya, S.V.; Davydov, A.V.; Zhukov, A.V.; Klimenko, O.M.; Sarychev, G.A.; Kudryavtsev, E.M. Interaction of depleted uranium tetrafluoride with silica. At. Energy 2012, 112, 226–229. [Google Scholar] [CrossRef]
- Biehl, E.; Schubert, U. Reaktionen von Siliciummonoxid mit Münzmetallhalogeniden. Monatsh. Chem. 2000, 131, 813–818. [Google Scholar] [CrossRef]
- Bulanov, A.D.; Pryakhin, D.A.; Balabanov, V.V. Preparation of High-Purity Silicon Tetrafluoride by Thermal Dissociation of Na2SiF6. Russ. J. Appl. Chem. 2003, 76, 1393–1395. [Google Scholar] [CrossRef]
- Cankaya, K.; Pretzer, W.R.; Livingston, W.A.; Kana’an, A.S. Equilibrium decomposition pressures of barium hexafluorosilicate. High Temp. Sci. 1970, 2, 322–330. [Google Scholar]
- Zachara, J.; Wisniewski, W. Electronegativity force of cations and thermal decomposition of complex fluorides. I. Thermal decomposition of fluorosilicates. J. Therm. Anal. 1995, 44, 363–373. [Google Scholar] [CrossRef]
- Krylov, V.A.; Sorochkina, T.G.; Bulanov, A.D.; Lashkov, A.Y. C1-C4 hydrocarbon release in the preparation of SiF4 through Na2SiF6 pyrolysis. Inorg. Mater. 2012, 48, 7–9. [Google Scholar] [CrossRef]
- Padma, D.K.; Kumar, H.P.S. Displacement of Lewis acid gases—Phosphorus pentafluoride, boron fluoride, and silicon fluoride from their ammonium, alkali metal and pyridinium fluoro complexes by sulfur trioxide at room temperature. Synth. React. Inorg. Met.-Org. Chem. 1992, 22, 1533–1549. [Google Scholar] [CrossRef]
- Padma, D.K.; Suresh, B.S.; Vasudevamurthy, A.R. Silicon tetrafluoride: Preparation and reduction with lithium aluminum hydride. J. Fluor. Chem. 1979, 14, 327–329. [Google Scholar] [CrossRef]
- Padma, D.K.; Murthy, A.R. Vasudeva New method for the preparation of silicon tetrafluoride. J. Fluor. Chem. 1974, 4, 241–242. [Google Scholar] [CrossRef]
- Roesky, H.W.; Herzog, A.; Keller, K. Organotin fluorides as fluorinating reagents for chlorides of main group elements—Quantitative recycling of the fluorinating reagent. Z. Naturforsch. B J. Chem. Sci. 1994, 49, 981–982. [Google Scholar] [CrossRef]
- Boehm, H.P. Silicon tetrafluoride preparation. Z. Anorg. Allg. Chem. 1969, 365, 176–179. [Google Scholar] [CrossRef]
- Lieser, K.H.; Rosenbaum, I. Preparation of silicon tetrafluoride from silicon dioxide and lead fluoride. Z. Anorg. Allg. Chem. 1967, 351, 306–308. [Google Scholar] [CrossRef]
- Stapf, A.; Gondek, C.; Kroke, E.; Roewer, G. Wafer Cleaning, Etching, and Texturization. In Handbook of Photovoltaic Silicon, 1st ed.; Yang, D., Ed.; Springer-Verlag GmbH Germany: Berlin/Heidelberg, Germany, 2018; pp. 1–47. [Google Scholar] [CrossRef]
- Gondek, C.; Hanich, R.; Honeit, F.; Lißner, A.; Stapf, A.; Kroke, E. Etching Silicon with Aqueous Acidic Ozone Solutions: Reactivity Studies and Surface Investigations. J. Phys. Chem. C 2016, 120, 4207–4215. [Google Scholar] [CrossRef]
- Gondek, C.; Lippold, M.; Röver, I.; Bohmhammel, K.; Kroke, E. Etching Silicon with HF-H2O2-based Mixtures: Reactivity Studies and Surface Investigations. J. Phys. Chem. C 2014, 118, 2044–2051. [Google Scholar] [CrossRef]
- Patzig-Klein, S.; Roewer, G.; Kroke, E. New insights into acidic wet chemical silicon etching by HF/H2O-NOHSO4-H2SO4 solutions. Mater. Sci. Semicond. Process. 2010, 13, 71–79. [Google Scholar] [CrossRef]
- Stapf, A.; Nattrodt, P.; Kroke, E. On The Mechanism of the Anisotropic Dissolution of Silicon in Chlorine Containing Hydrofluoric Acid Solutions. J. Electrochem. Soc. 2018, 165, H3045–H3050. [Google Scholar] [CrossRef]
- Schubert, N.; Stapf, A.; Lißner, A.; Zomack, N.; Neumann, A.-L.; Kroke, E. Analysis of silicon surfaces etched in aqueous HF-(HBr)–Br2-mixtures. Chem. Inorg. Mater. 2024, 3, 100063. [Google Scholar] [CrossRef]
- Dawei, L.; Xilu, Y.; Xianfeng, Q.; Yanan, P.; Xiaoyan, L. Effects of HF Acid on Dissolution of Elemental Si. Silicon 2023, 15, 7877–7884. [Google Scholar] [CrossRef]
- Rietig, A.; Langner, T.; Acker, J. A revised model of silicon oxidation during the dissolution of silicon in HF/HNO3 mixtures. Phys. Chem. Chem. Phys. 2019, 21, 22002–22013. [Google Scholar] [CrossRef]
- Rietig, A.; Langner, T.; Acker, J. Comprehensive stoichiometric studies on the reaction of silicon in HF/HNO3 and HF/HNO3/H2SiF6 mixtures. Phys. Chem. Chem. Phys. 2022, 24, 3094–3108. [Google Scholar] [CrossRef]
- Haase, M.; Melzer, M.; Lang, N.; Ecke, R.; Zimmermann, S.; van Helden, J.-P.H.; Schulz, S.E. On the relationship between SiF4 plasma species and sample properties in ultra low-k etching processes. AIP Adv. 2020, 10, 065212. [Google Scholar] [CrossRef]
- Osipov, A.A.; Iankevich, G.A.; Speshilova, A.B.; Osipov, A.A.; Endiiarova, E.V.; Berezenko, V.I.; Tyurikova, I.A.; Tyurikov, K.S.; Alexandrov, S.E. High-temperature etching of SiC in SF6/O2 inductively coupled plasma. Sci. Rep. 2020, 10, 19977. [Google Scholar] [CrossRef] [PubMed]
- Tasaka, A.; Takahashi, K.; Tanaka, K.; Shimizu, K.; Mori, K.; Tada, S.; Shimizu, S.; Abe, T.; Inaba, M.; Ogumi, Z.; et al. Plasma etching of SiC surface using NF3. J. Vac. Sci. Technol. 2002, 20, 1254–1260. [Google Scholar] [CrossRef]
- Donnelly, V.M.; Kornblit, A. Plasma Etching: Yesterday, Today, and Tomorrow. J. Vac. Sci. Technol. A 2013, 31, 050825. [Google Scholar] [CrossRef]
- Saito, Y.; Yamaoka, O.; Yoshida, A. Plasmaless cleaning process of silicon surface using chlorine trifluoride. Appl. Phys. Lett. 1990, 56, 1119–1121. [Google Scholar] [CrossRef]
- Carver, C.T.; Plombon, J.J.; Romero, P.E.; Suri, S.; Tronic, T.A.; Turkot, R.B., Jr. Atomic Layer Etching: An Industry Perspective. ECS J. Solid State Sci. Technol. 2015, 4, N5005–N5009. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, J.; Cao, Z.; Liu, Q.; Wei, F.; Zhou, J.; Wang, D.; Shi, S.; Qian, G. Improvement on Fluorine Migration from SF6 to SiF4 by an Efficient Mediator of Fe2O3/Cr2O3 Composites. ACS Appl. Mater. Interfaces 2019, 11, 16538–16545. [Google Scholar] [CrossRef]
- Natta, G. Structure of silicon tetrafluoride. Gazz. Chim. Ital. 1930, 60, 911–922. [Google Scholar]
- Brockway, L.O.; Wall, F.T. The Electron Diffraction Investigation of Some Non-metallic Halides. J. Am. Chem. Soc. 1934, 56, 2373–2379. [Google Scholar] [CrossRef]
- Mootz, D.; Korte, L. Fluoride und Fluorosäuren, VIII—Über eine fehlgeordnete feste Phase des SF4 sowie die Kristallstrukturen von Produkten seiner unbeabsichtigten Hydrolyse in Glasapparaturen, SiF4 (Neubestimmung) und SOF2. Z. Naturforsch. 1984, 39, 1295–1299. [Google Scholar] [CrossRef]
- Schomaker, V.; Stevenson, D.P. Some Revisions of the Covalent Radii and the Additivity Rule for the Lengths of Partially Ionic Single Covalent Bonds. J. Am. Chem. Soc. 1941, 63, 37–40. [Google Scholar] [CrossRef]
- Pauling, L. The Nature of the Chemical Bond, 3rd ed.; Cornell University Press: Ithaca, NY, USA, 1960; pp. 313–314. [Google Scholar]
- Gillespie, R.J. Covalent and Ionic Molecules: Why Are BeF2 and AlF3 High Melting Point Solids whereas BF3 and SiF4 Are Gases? J. Chem. Educ. 1998, 75, 923–925. [Google Scholar] [CrossRef]
- Takami, M.; Kuze, H. Infrared–microwave double resonance spectroscopy of the SiF4 ν3 fundamental using a tunable diode laser. J. Chem. Phys. 1983, 78, 2204–2209. [Google Scholar] [CrossRef]
- Donald, K.J.; Böhm, M.C.; Lindner, H.J. Analysis of competing bonding parameters. Part 2. The structure of halosilanes and halogermanes (MH4−nXn, n = 1–4; M=Si, Ge; X=F, Cl, Br). J. Mol. Struct. Theochem. 2005, 713, 215–226. [Google Scholar] [CrossRef]
- Wang, H.; Wu, P.; Wu, Z.; Shi, L.; Cheng, L. New insight into the electronic structure of SiF4: Synergistic back-donation and the eighteen-electron rule. Phys. Chem. Chem. Phys. 2022, 24, 17679–17685. [Google Scholar] [CrossRef]
- Chamberlin, A. Isotope Effects in Chemical Reactions. Annu. Rev. Phys. Chem. 2016, 67, 63–87. [Google Scholar]
- Otto, R. Some physical constants of SiF4, WF6 and MoF6. Z. Anorg. Allg. Chem. 1931, 196, 413–420. [Google Scholar] [CrossRef]
- Golovanov, I.B. Quantitative Structure-Property Relationship: XXIV. Properties of Halo Derivatives of Methane, Silane, and Methylsilanes. Russ. J. Gen. Chem. 2005, 75, 1899–1903. [Google Scholar] [CrossRef]
- Booth, H.S.; Swinehart, C.F. Critical Constants and Vapor Pressure of Some Gaseous Fluorides of Group IV. J. Am. Chem. Soc. 1935, 57, 1337–1342. [Google Scholar] [CrossRef]
- Hanson, D.E.; Santos, C.M. Silicon Tetrafluoride Vapor Pressure Study. Technical Report. 2022. Available online: https://www.osti.gov/biblio/1908063/ (accessed on 3 September 2024).
- Sukkaew, P. Thermochemical Properties of Halides and Halohydrides of Silicon and Carbon. ECS J. Solid State Sci. Technol. 2016, 5, P27–P35. [Google Scholar] [CrossRef]
- Kickel, B.L.; Fisher, E.R.; Armentrout, P.B. Dissociative charge-transfer reactions of atomic nitrogen (1+) (3P), dinitrogen (1+) (2Σg+), argon(1+) (2P3/2,1/2), and krypton(1+) (2P3/2) with tetrafluorosilane. Thermochemistry of SiF4+ and SiF3+. J. Chem. Phys. 1993, 97, 10198–10203. [Google Scholar] [CrossRef]
- Kolditz, L. Anorganikum, 13th ed.; Johann Ambrosius Bart Deutscher Verlag der Wissenschaften: Berlin, Germany, 1993; p. 519. [Google Scholar]
- McDonald, J.D.; Williams, C.H.; Thompson, J.C.; Margrave, J.L. Appearance potentials, ionization potentials and heats of formation for perfluorosilanes and perfluoroborosilanes. Advan. Chem. Ser. 1968, 72, 261–266. [Google Scholar] [CrossRef]
- Estrada-Alexanders, A.F.; Hurly, J.J. Kinematic viscosity and speed of sound in gaseous CO, CO2, SiF4, SF6, C4F8, and NH3 from 220K to 375K and pressures up to 3.4 MPa. J. Chem. Thermodyn. 2008, 40, 193–202. [Google Scholar] [CrossRef]
- Lide, D.R. (Ed.) CRC Handbook of Chemistry and Physics, 75th ed.; CRC Press Inc.: Boca Raton, FL, USA, 1994; pp. 4–94. [Google Scholar]
- Nakamoto, K. Infrared and Raman Spectra of Inorganic and Coordination Compounds: Part A: Theory and Applications in Inorganic Chemistry, 6th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2009; pp. 1–350. [Google Scholar] [CrossRef]
- Hirota, E. High-Resolution Spectroscopy of Transient Molecules, 40th ed.; Springer: Berlin/Heidelberg, Germany, 1985; pp. 1–236. [Google Scholar] [CrossRef]
- McLafferty, F.W.; Tureček, F. Interpretation of Mass Spectra, 4th ed.; University Science Books: Mill Valley, CA, USA, 1993; p. 371. ISBN 0-935702-25-3. [Google Scholar]
- Levitt, M.H. Spin Dynamics: Basics of Nuclear Magnetic Resonance, 2nd ed.; Wiley: Hoboken, NJ, USA, 2008; p. 752. ISBN 978-0-470-51117-6. [Google Scholar]
- Claridge, T.D.W. High-Resolution NMR Techniques in Organic Chemistry, 3rd ed.; Elsevier Science: Amsterdam, The Netherlands, 2016; p. 552. ISBN 9780080999869. [Google Scholar]
- Keeler, J. Understanding NMR Spectroscopy, 2nd ed.; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2010; p. 526. ISBN 978-0-470-74609-7. [Google Scholar]
- Lakowicz, J.R. Principles of Fluorescence Spectroscopy, 3rd ed.; Springer US: Boston, MA, USA, 2006; p. 954. [Google Scholar] [CrossRef]
- Atkins, P.; de Paula, J. Physical Chemistry, 10th ed.; OUP: Oxford, UK, 2014; p. 1008. ISBN 9780199697403. [Google Scholar]
- Safety Data Sheet Silicon Tetrafluoride. Available online: https://produkte.linde-gas.at/sdb_konform/SiF4_10021730EN.pdf (accessed on 21 July 2024).
- Rom, W.N.; Markowitz, S.B. Environmental and Occupational Medicine, 4th ed.; Lippincott Williams & Wilkins: Baltimore, MD, USA, 2006; pp. 487–496. ISBN 978-0-7817-6299-1. [Google Scholar]
- Furr, A.K. CRC Handbook of Laboratory Safety, 5th ed.; CRC Press: Boca Raton, FL, USA, 2000; p. 802. ISBN 9780849325236. [Google Scholar]
- Greenwood, N.N.; Earnshaw, A. Chemistry of the Elements, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 1997; p. 1600. ISBN 9780750633659. [Google Scholar]
- Dobkin, D.M.; Zuraw, M.K. Principles of Chemical Vapor Deposition, 1st ed.; Kluwer Academic Publishers: New York, NY, USA, 2003; p. 288. ISBN 978-9048162772. [Google Scholar]
- Wolf, S.; Tauber, R.N. Silicon Processing for the VLSI Era. In Process Technology, 2nd ed.; Lattice Press: San Diego, CA, USA, 2000; Volume 1. [Google Scholar]
- Rana, T.; Chandrashekhar, M.V.S.; Sudarshan, T.S. Elimination of silicon gas phase nucleation using tetrafluorosilane (SiF4) precursor for high quality thick silicon carbide (SiC) homoepitaxy. Phys. Status Solidi A 2012, 209, 2455–2462. [Google Scholar] [CrossRef]
- Sennikov, P.; Pryakhin, D.; Andreev, B.; Gavrilenko, L.; Drozdov, Y.; Drozdov, M.; Pohl, H.-J.; Shashkin, V. Plasma-enhanced chemical vapor deposition of 99.95% 28Si in form of nano- and polycrystals using silicon tetrafluoride precursor. Cryst. Res. Technol. 2010, 45, 983–987. [Google Scholar] [CrossRef]
- Ingle, W.M.; Thompson, S.W. Silicon purification process. US Patent US4138509A, 2 June 1979. [Google Scholar]
- Becker, M.; Oles, M. Silicon Tetrafluoride for the Synthesis of Ultra-pure Silicon. J. Cryst. Growth 1990, 104, 284–290. [Google Scholar]
- Ryssel, H.; Ruge, I. Ion Implantation: Equipment and Techniques, 99th ed.; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 1986; p. 478. ISBN 978-0471103110. [Google Scholar]
- Sze, S.M.; Ng, K.K. Physics of Semiconductor Devices, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006; p. 815. [Google Scholar] [CrossRef]
- Smith, J.D. Fluorination Reactions of Organic Compounds, Advances in Fluorine Chemistry, 2nd ed.; Academic Press: Cambridge, MA, USA, 1965; pp. 47–70. [Google Scholar]
- Wallace, R. Dielectric Materials for Microelectronics. In Springer Handbook of Electronic and Photonic Materials, 1st ed.; Kasap, S., Capper, P., Eds.; Springer Handbooks; Springer: Boston, MA, USA, 2006; pp. 625–657. [Google Scholar] [CrossRef]
- Grill, A.; Neumayer, D.A. Structure of low dielectric constant to extreme low dielectric constant SiCOH films: Fourier tra nsform infrared spectroscopy characterization. J. Appl. Phys. 2003, 94, 6697–6707. [Google Scholar] [CrossRef]
- Wolf, S.; Tauber, R.N. Silicon Processing for the VLSI Era: Volume 4—Deep-Submicron Process Technology, 1st ed.; Lattice Press: San Diego, CA, USA, 2002; p. 822. ISBN 978-0961672182. [Google Scholar]
- Collins, K.D.; Smith, D.K. Fluorosilicic Acid and its Industrial Applications. Ind. Eng. Chem. Res. 2008, 47, 2337–2343. [Google Scholar]
- Ullmann, F.; Gerhartz, W.; Yamamoto, Y.S.; Campbell, F.T.; Pfefferkorn, R.; Rounsaville, J.F. Ullmann’s Encyclopedia of Industrial Chemistry, 5th ed.; VCH Verlagsgesellschaft mbH: Hoboken, NJ, USA, 1986; Volume A11, pp. 1–6. [Google Scholar]
- Corey, E.J.; Cheng, X.M. The Logic of Chemical Synthesis, 1st ed.; Wiley: Hoboken, NJ, USA, 1995; p. 464. ISBN 978-0-471-11594-6. [Google Scholar]
- Ojima, I. Catalytic Asymmetric Synthesis, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2010; p. 998. [Google Scholar] [CrossRef]
- Wagler, J.; Böhme, U.; Kroke, E. Higher-Coordinated Molecular Silicon Compounds. Funct. Mol. Silicon Compd. I Regul. Oxid. States 2014, 77, 29–105. [Google Scholar] [CrossRef]
- Elschenbroich, C. Organometallics, 3rd ed.; Wiley-VCH: Hoboken, NJ, USA, 2006; p. 804. ISBN 978-3-527-29390-2. [Google Scholar]
- Hollemann, A.F.; Wiberg, E. Lehrbuch der Anorganischen Chemie, 101st ed.; de Gruyter: Berlin, Germany, 1995; p. 2033. ISBN 978-3-11-012641-9. [Google Scholar]
- Levin, E.V. Separation of multicomponent isotopic mixtures in a gas centrifuge—Approximate method for solving the system of diffusion-transport equations and analysis of some separation characteristics. At. Energy 1994, 77, 760–767. [Google Scholar] [CrossRef]
- Borisevich, V.; Levin, E. Separation of Multicomponent Isotope Mixtures by Gas Centrifuge. Sep. Sci. Technol. 2001, 36, 1697–1735. [Google Scholar] [CrossRef]
- URENCO Limited. Information der Öffentlichkeit nach der Strahlenschutzverordnung und der Störfallverordnung. 2019. Available online: https://www.urenco.com/cdn/uploads/supporting-files/UD_Information_booklet_260623.pdf (accessed on 3 September 2024).
- Available online: https://www.internetchemistry.com (accessed on 25 July 2024).
- Glaser, A. Characteristics of the Gas Centrifuge for Uranium Enrichment and Their Relevance for Nuclear Weapon Proliferation. Sci. Glob. Secur. 2008, 16, 1–25. [Google Scholar] [CrossRef]
- Kushner, D. The Real Story of Stuxnet. IEEE Spectr. 2013, 50, 48–53. [Google Scholar] [CrossRef]
- Bogovalov, S.V.; Borman, V.D. Isotope Separation in Concurrent Gas Centrifuges. Phys. Procedia 2015, 72, 297–304. [Google Scholar] [CrossRef][Green Version]
- Snyder, R. A Proliferation Assessment of Third Generation Laser Uranium Enrichment Technology. Sci. Glob. Secur. 2016, 24, 68–91. [Google Scholar] [CrossRef]
- Bokhan, P.A.; Buchanov, V.V.; Fateev, N.V.; Kalugin, M.M.; Kazaryan, M.A.; Prokhorov, A.M.; Zakrevskii, D.E. Laser Isotope Separation in Atomic Vapor, 1st ed.; Wiley-VCH: Berlin, Germany, 2006; ISBN 3-527-40621-2. [Google Scholar]
- Eerkens, J.W. Spectral considerations in the laser isotope separation of Uranium Hexafluoride. Appl. Phys. 1976, 10, 15–31. [Google Scholar] [CrossRef]
- Baranov, I.Y.; Koptev, A.V. Mode-Locked CO Laser for Isotope Separation of Uranium Employing Condensation Repression. Adv. Opt. Technol. 2010, 2010, 693530. [Google Scholar] [CrossRef][Green Version]
- Nundy, U.; Kumar, M. Generation of tunable 16 μm radiation from CO2 by cascade lasing. Pramana J. Phys. 2012, 79, 1425–1441. [Google Scholar] [CrossRef]
- Li, D.J.; Yang, G.L.; Chen, F.; Xie, J.J.; Zhang, L.M.; Guo, J.; Shao, C.L.; Peng, Z.Q.; Lu, Q.P. Stimulated rotational Raman scattering at multiwavelength under tea CO2 laser pumping with a multiple-pass cell. Laser Phys. 2012, 22, 937–940. [Google Scholar] [CrossRef]
- Available online: https://www.osti.gov/etdeweb/biblio/20073948 (accessed on 25 July 2024).
- Available online: https://www.silex.com.au/silex-technology/silex-zs-si-production-for-quantum-computing/ (accessed on 25 July 2024).
- Available online: https://www.silex.com.au/investors/announcements/ (accessed on 25 July 2024).
- Dargan, J. Quantum Silicon Production Project Awarded $5.1M Funding under the Defence Trailblazer Program. Quantum Insid. 18 August 2023. Available online: https://thequantuminsider.com/2023/08/18/quantum-silicon-production-project-awarded-5-1m-funding-under-the-defence-trailblazer-program/ (accessed on 25 July 2024).
- Miller, D.G. Thermodynamics of Irreversible Processes. The Experimental Verification of the Onsager Reciprocal Relations. Chem. Rev. 1960, 60, 15–37. [Google Scholar] [CrossRef]
- Rowe, D.M. Thermoelectrics Handbook, 1st ed.; CRC Press: Boca Raton, FL, USA, 2018; p. 1008. [Google Scholar] [CrossRef]
- Welty, J.R.; Wicks, C.E.; Wilson, R.E.; Rorrer, G.L. Fundamentals of Momentum, Heat and Mass Transfer, 5th ed.; John Wiley and Sons: Hoboken, NJ, USA, 2008; ISBN 978-0-470-12868-8. [Google Scholar]
- Cotton, S. Lanthanide and actinide chemistry, 1st ed.; John Wiley and Sons, Ltd.: Chichester, UK, 2006; pp. 163–165. ISBN 978-0-470-01006-8. [Google Scholar]
- Liehr, G.; Die AKW-Krokodile. Taz. 1997. Available online: https://taz.de/Die-AKW-Krokodile/!1380786/ (accessed on 3 September 2024).
- Musik, A.; Die Krokodile von Tricastin. Diepresse. 2011. Available online: https://www.diepresse.com/643109/die-krokodile-von-tricastin (accessed on 3 September 2024).
- Hiltzik, M.A. Big Science: Ernest Lawrence and the Invention that Launched the Military-Industrial Complex, 1st ed.; Simon & Schuster: New York, NY, USA, 2015; p. 528. ISBN 978-1-4516-7575-7. [Google Scholar]
- Love, L.O. Electromagnetic Separation of Isotopes at Oak Ridge. Science 1979, 182, 343–352. [Google Scholar] [CrossRef] [PubMed]
- Clarence, L. The Role of Chemistry in the Oak Ridge Electromagnetic Project. Bull. Hist. Chem. 2003, 28, 101–109. [Google Scholar]
- Albright, D.; Hibbs, M. Iraq’s Nuclear Hide-and-Seek. Bull. At. Sci. 1991, 47, 14–23. [Google Scholar] [CrossRef]
- Gsponer, A.; Hurni, J.-P. Iraq’s Calutrons Electromagnetic Isotope Separation, Beam Technology and Nuclear Weapon Proliferation; ISRI: Washington, DC, USA, 1995. [Google Scholar]
- Tang, K.; Kim, H.S.; Ramanayaka, A.N.R.; Simons, D.S.; Pomeroy, J.M. A compact, ultra-high vacuum ion source for isotopically enriching and depositing 28Si thin films. Rev. Sci. Instrum. 2019, 90, 083308. [Google Scholar] [CrossRef]
- Tang, K.; Kim, H.S.; Ramanayaka, A.N.R.; Simons, D.S.; Pomeroy, J.M. Targeted enrichment of 28Si thin films for quantum computing. J. Phys. Commun. 2020, 4, 035006. [Google Scholar] [CrossRef]
- Holmes, D.; Johnson, B.C.; Chua, C.; Voisin, B.; Kocsis, S.; Rubanov, S.; Robson, S.G.; McCallum, J.C.; McCamey, D.R.; Rogge, S.; et al. Isotopic enrichment of silicon by high fluence 28Si− ion implantation. Phys. Rev. Mater. 2021, 5, 014601. [Google Scholar] [CrossRef]
- Acharya, R.; Coke, M.; Adshead, M.; Li, K.; Achinuq, B.; Cai, R.; Gholizadeh, A.B.; Jacobs, J.; Boland, J.L.; Haigh, S.J.; et al. Highly 28Si enriched silicon by localised focused ion beam implantation. Commun. Mater. 2024, 5, 57. [Google Scholar] [CrossRef]
- Becker, E.W.; Bley, P.; Ehrfeld, W.; Lenné, H. Uranisotopentrennung mit einem Gegenstromwirbelrohr. Z. Naturforsch. A Phys. Sci. 1978, 33, 1588–1589. [Google Scholar] [CrossRef]
- Becker, E.W.; Bier, W.; Bley, P.; Ehrfeld, U.; Ehrfeld, W.; Eisenbeiß, G. Das Entwicklungspotential des Trenndüsenverfahrens zur U-235-Anreicherung. Atomwirtschaft 1979, 11, 1–16. [Google Scholar]
- Becker, E.W.; Bier, W.; Ehrfeld, W.; Schubert, K.; Seidel, D. Entwicklung und technische Anwendung des Trenndüsenver-fahrens zur Anreicherung von Uran 235. KfK-Nachrichten 1981, 1–2. Available online: https://publikationen.bibliothek.kit.edu/270017217/3812402 (accessed on 3 September 2024).
- Albright, D. South Africa’s Secret Nuclear Weapons (ISIS Reports). 1994. Available online: https://www.isis-online.org/publications/southafrica/ir0594.html (accessed on 3 September 2024).
- Cochran, T.B. Highly Enriched Uranium Production for South African Nuclear Weapons. Sci. Glob. Secur. 1994, 4, 161–176. [Google Scholar] [CrossRef]
- Becker, P.; Pohl, H.-J.; Riemann, H.; Abrosimov, N. Enrichment of silicon for a better kilogram. Phys. Status Solidi A 2010, 207, 49–66. [Google Scholar] [CrossRef]
- Pfann, W.G. Principles of Zone-Melting. JOM 1952, 4, 747–753. [Google Scholar] [CrossRef]
- Zakharkin, L.I.; Gavrilenko, V.V.; Khorlina, I.M.; Zhigareva, G.G. The reduction of silicon and germanium chlorides and alkoxides by sodium and potassium aluminum hydrides. Bull. Acad. Sci. USSR Div. Chem. Sci. (Engl. Transl.) 1962, 11, 1784–1785. [Google Scholar] [CrossRef]
- Lefrancois, P.A. Production of Silane. EP0052808, 1982. [Google Scholar]
- Devyatykh, G.G.; Dianov, E.M.; Bulanov, A.D.; Troshin, O.Y.; Balabanov, V.V.; Pryakhin, D.A. Preparation of high-purity monoisotopic silane: 28SiH4, 29SiH4, and 30SiH4. Dokl. Chem. 2003, 391, 204–205. [Google Scholar] [CrossRef]
- Bulanov, A.D.; Mikheev, V.S.; Troshin, O.Y.; Lashkov, A.Y. Reaction of Silicon Tetrafluoride with Calcium Hydride as a Propagating Wave. Russ. J. Inorg. Chem. 2008, 53, 6–10. [Google Scholar] [CrossRef]
- Troshin, O.Y.; Bulanov, A.D.; Mikheev, V.S.; Lashkov, A.Y. Mechanically Activated Synthesis of Monosilane by the Reaction of Calcium Hydride with Silicon Tetrafluoride. Russ. J. Inorg. Chem. 2010, 83, 984–988. [Google Scholar] [CrossRef]
- Fadeev, L.L.; Kvaratskheli, J.K.; Zhirkov, M.S.; Ivashin, A.M.; Kudrjavtsev, V.V.; Grishin, A.V.; Filipinov, V.T. Method of preparing monosilane. RU02077483, 20 April 1997. [Google Scholar]
- Churbanov, M.F.; Bulanov, A.D.; Kotkov, A.P.; Potapov, A.M.; Troshin, O.Y.; Lashkov, A.Y.; Grishnova, N.D.; Adamchik, S.A. Production of Silanes 29SiH4 and 30SiH4 of High Chemical and Isotopic Purity. Dokl. Chem. 2010, 432, 126–128. [Google Scholar] [CrossRef]
- Sennikov, P.G.; Kotkov, A.P.; Adamchik, S.A.; Grishnova, N.D.; Chuprov, L.A.; Ignatov, S.A. Impurities in Monosilanes Synthesized by Different Processes. Inorg. Mater. 2010, 46, 358–363. [Google Scholar] [CrossRef]
- Bulanov, A.D.; Balabanov, V.V.; Pryakhin, D.A.; Troshin, O.Y. Preparation and Fine Purification of SiF4 and 28SiH4. Inorg. Mater. 2002, 38, 283–287. [Google Scholar] [CrossRef]
- Ager III, J.W.; Beeman, J.W.; Hansen, W.L.; Haller, E.E.; Sharp, I.D.; Liao, C.; Yang, A.; Thewalt, M.L.W.; Riemann, H. High-Purity, Isotopically Enriched Bulk Silicon. J. Electrochem. Soc. 2005, 152, G448. [Google Scholar] [CrossRef]
- Weerts, W.L.M.; de Croon, M.H.J.M.; Martin, G.B. The Kinetics of the Low-Pressure Chemical Vapor Deposition of Polycrystalline Silicon from Silane. J. Electrochem. Soc. 1998, 145, 1318–1330. [Google Scholar] [CrossRef]
- Becker, P.; Friedrich, H.; Fujii, K.; Giardini, W.; Mana, G.; Picard, A.; Pohl, H.-J.; Riemann, H.; Valkiers, S. The Avogadro constant determination via enriched silicon-28. Meas. Sci. Technol. 2009, 20, 092002. [Google Scholar] [CrossRef]
- Mazzocchi, V.; Sennikov, P.G.; Bulanov, A.D.; Churbanov, M.F.; Bertrand, B.; Hutin, L.; Barnes, J.P.; Drozdov, M.N.; Hartmann, J.M.; Sanquer, M. 99.992% 28Si CVD-grown epilayer on 300 mm substrates for large scale integration of silicon spin qubits. J. Cryst. Growth 2019, 509, 1–7. [Google Scholar] [CrossRef]
- Baptista, A.; Silva, F.; Porteiro, J.; Míguez, J.; Pinto, G. Sputtering Physical Vapor Deposition (PVT) Coatings: A Critical Review on Process Improvement and Market Trend Demands. Coatings 2018, 8, 402. [Google Scholar] [CrossRef]
- Bean, J.C. Silicon MBE: From strained-layer epitaxy to device application. J. Cryst. Growth 1984, 70, 444–451. [Google Scholar] [CrossRef]
- Ramanayaka, A.N.; Tang, K.; Hagmann, J.A.; Kim, H.-S.; Simons, D.S.; Richter, C.A.; Pomeroy, J.M. Use of quantum effects as potential qualifying metrics for “quantum grade silicon”. AIP Adv. 2019, 9, 125153. [Google Scholar] [CrossRef]
- Andreas, B.; Azuma, Y.; Bartl, G.; Becker, P.; Bettin, H.; Borys, M.; Busch, I.; Gray, M.; Fuchs, P.; Fujii, K.; et al. Determination of the Avogadro Constant by Counting the Atoms in a 28Si Crystal. Phys. Rev. Lett. 2011, 106, 030801. [Google Scholar] [CrossRef]
- Available online: https://www.wissenschaft.de/erde-umwelt/kampf-ums-kilo/ (accessed on 25 July 2024).
- Available online: https://www.britannica.com/science/metric-system-measurement#ref258582 (accessed on 25 July 2024).
- Available online: https://www.spiegel.de/wissenschaft/mensch/physikalische-masseinheiten-das-raetselhafte-schrumpfen-des-urkilogramms-a-505526.html (accessed on 25 July 2024).
- Girard, G. The Third Periodic Verification of National Prototypes of the Kilogram (1988–1992). Metrologia 1994, 31, 317. [Google Scholar] [CrossRef]
- Available online: https://leopard.tu-braunschweig.de/receive/dbbs_mods_00032342 (accessed on 25 July 2024).
- Available online: https://www.nytimes.com/2018/11/16/science/kilogram-physics-measurement.html (accessed on 25 July 2024).
- Available online: https://web.archive.org/web/20210402142630/https://www.bipm.org/utils/en/pdf/CGPM/Draft-Resolution-A-EN.pdf (accessed on 25 July 2024).
- Robinson, I.A.; Schlamminger, S. The watt or Kibble balance: A technique for implementing the new SI definition of the unit of mass. Metrologia 2016, 53, A46. [Google Scholar] [CrossRef] [PubMed]
- Azuma, Y.; Barat, P.; Bartl, G.; Bettin, H.; Borys, M.; Busch, I.; Cibik, L.; D’Agostino, G.; Fujii, K.; Fujimoto, H.; et al. Improved measurement results for the Avogadro constant using a 28Si-enriched crystal. Metrologia 2015, 52, 360. [Google Scholar] [CrossRef]
- Andreas, B.; Azuma, Y.; Bartl, G.; Becker, P.; Bettin, H.; Borys, M.; Busch, I.; Fuchs, P.; Fujii, K.; Fujimoto, H.; et al. Counting the atoms in a 28Si crystal for a new kilogram definition. Metrologia 2011, 48, S1. [Google Scholar] [CrossRef]
- Bulska, E.; Drozdov, M.N.; Mana, G.; Pramann, A.; Rienitz, O.; Sennikov, P.; Valkiers, S. The isotopic composition of enriched Si: A data analysis. Metrologia 2011, 48, S32. [Google Scholar] [CrossRef]
- Picard, A.; Barat, P.; Borys, M.; Firlus, M.; Mizushima, S. State-of-the-art mass determination of 28Si spheres for the Avogadro project. Metrologia 2011, 48, S112. [Google Scholar] [CrossRef]
- Pramann, A.; Rienitz, O. Mass Spectrometric Investigation of Silicon Extremely Enriched in 28Si: From 28SiF4 (Gas Phase IRMS) to 28Si Crystals (MC-ICP-MS). Anal. Chem. 2016, 88, 5963–5970. [Google Scholar] [CrossRef] [PubMed]
- Pramann, A.; Lee, K.-S.; Noordmann, J.; Rienitz, O. Probing the homogeneity of the isotopic composition and molar mass of the ‘Avogadro’-crystal. Metrologia 2015, 52, 800. [Google Scholar] [CrossRef]
- Pramann, A.; Rienitz, O.; Noordmann, J.; Güttler, B.; Schiel, D. A More Accurate Molar Mass of Silicon via High Resolution MC-ICP-Mass Spectrometry. Z. Phys. Chem. 2014, 228, 405–419. [Google Scholar] [CrossRef]
- Rienitz, O.; Pramann, A.; Schiel, D. Novel concept for the mass spectrometric determination of absolute isotopic abundances with improved measurement uncertainty: Part 1—Theoretical derivation and feasibility study. Int. J. Mass Spectrom. 2010, 289, 47–53. [Google Scholar] [CrossRef]
- Pramann, A.; Rienitz, O.; Schiel, D.; Güttler, B. Novel concept for the mass spectrometric determination of absolute isotopic abundances with improved measurement uncertainty: Part 2—Development of an experimental procedure for the determination of the molar mass of silicon using MC-ICP-MS. Int. J. Mass Spectrom. 2011, 299, 78–86. [Google Scholar] [CrossRef]
- Pramann, A.; Rienitz, O.; Schiel, D.; Güttler, B.; Valkiers, S. Novel concept for the mass spectrometric determination of absolute isotopic abundances with improved measurement uncertainty: Part 3—Molar mass of silicon highly enriched in 28Si. Int. J. Mass Spectrom. 2011, 305, 58–68. [Google Scholar] [CrossRef]
- Haller, E.E. Isotopically engineered semiconductors. J. Appl. Phys. 1995, 77, 2857–2878. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Riveros, R.E.; Mitsuishi, I.; Takagi, U.; Ezoe, Y.; Yamasaki, N.; Mitsuda, K.; Hashimoto, F. Magnetic field-assisted finishing for micropore X-ray focusing mirrors fabricated by deep reactive ion etching. CIRP Ann. 2010, 59, 351–354. [Google Scholar] [CrossRef]
- Li, Q.; Raimbault, V.; Calmon, P.-F.; Reig, B.; Debernardi, P.; Ottevaere, H.; Doucet, J.-B.; Roul, J.; Bardinal, V. Direct 3D-printing of microlens on single mode polarization-stable VCSEL chip for miniaturized optical spectroscopy. J. Opt. Microsyst. 2023, 3, 033501. [Google Scholar] [CrossRef]
- Shvyd’ko, Y. X-ray Optics: High-Energy-Resolution Applications (Springer Series in Optical Sciences, 98), 1st ed.; Springer: Berlin/Heidelberg, Germany, 2004; p. 420. ISBN 978-3540214847. [Google Scholar]
- Hawking, S.; Hawking, S.W.; Israel, W. Three Hundred Years of Gravitation, 1st ed.; Cambridge University Press: Cambridge, UK, 1987; p. 684. ISBN 978-0521343121. [Google Scholar]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Hild, S.; Chelkowski, S.; Freise, A. Pushing towards the ET sensitivity using ‘conventional’ technology. arXiv 2008, arXiv:0810.0604. [Google Scholar] [CrossRef]
- Available online: https://web.archive.org/web/20170620143851/https://tds.virgo-gw.eu/?call_file=ET-0106C-10.pdf (accessed on 25 July 2024).
- Di Giovanni, M.; Giunchi, C.; Saccorotti, G.; Berbellini, A.; Boschi, L.; Olivieri, M.; De Rosa, R.; Naticchioni, L.; Oggiano, G.; Carpinelli, M.; et al. A Seismological Study of the Sos Enattos Area—The Sardinia Candidate Site for the Einstein Telescope. Seismol. Res. Lett. 2020, 92, 352–364. [Google Scholar] [CrossRef]
- Available online: https://www.einsteintelescope-emr.eu/de/2024/02/21/der-prototyp-des-einstein-teleskops-e-test-besteht-seine-erste-testreihe/ (accessed on 25 July 2024).
- Available online: https://www.ikz-berlin.de/en/cosmology-particle-physics (accessed on 25 July 2024).
- Available online: https://www.einstein-teleskop.de/partner/ (accessed on 25 July 2024).
- Available online: https://www.deutscheszentrumastrophysik.de (accessed on 26 July 2024).
- Available online: https://www.esa.int/Science_Exploration/Space_Science/LISA/Capturing_the_ripples_of_spacetime_LISA_gets_go-ahead (accessed on 25 July 2024).
- Ernst, O.C.; Liu, Y.; Boeck, T. Leveraging dewetting models rather than nucleation models: Current crystallographic challenges in interfacial and nanomaterials research. Z. Kristallogr. Cryst. Mater. 2022, 237, 191–200. [Google Scholar] [CrossRef]
- Schreiber, L.R.; Bluhm, H. Toward a silicon-based quantum computer. Science 2018, 259, 393–394. [Google Scholar] [CrossRef]
- Almudever, C.G.; Lao, L.; Fu, X.; Khammassi, N.; Ashraf, I.; Iorga, D.; Varsamopoulos, S.; Eichler, C.; Wallraff, A.; Geck, L.; et al. The Engineering Challenges in Quantum Computing; Design, Automation & Test in Europe Conference & Exhibition (DATE): Lausanne, Switzerland, 2017; pp. 836–845. [Google Scholar] [CrossRef]
- Loss, D.; DiVincenzo, D.P. Quantum computation with quantum dots. Phys. Rev. A 1998, 57, 120. [Google Scholar] [CrossRef]
- Vandersypen, L.M.K.; Eriksson, M.A. Quantum computing with semiconductor spins. Phys. Today 2019, 72, 38–45. [Google Scholar] [CrossRef]
- Zwanenburg, F.A.; Dzurak, A.S.; Morello, A.; Simmons, M.Y.; Hollenberg, L.C.L.; Klimeck, G.; Rogge, S.; Coppersmith, S.N.; Eriksson, M.A. Silicon quantum electronics. Rev. Mod. Phys. 2013, 85, 961–1019. [Google Scholar] [CrossRef]
- Gritsch, A.; Ulanowski, A.; Reiserer, A. Purcell enhancement of sing le-photon emitters in silicon. Optica 2023, 10, 783–789. [Google Scholar] [CrossRef]
- Johnston, A.; Felix-Rendon, U.; Wong, Y.-E.; Chen, S. Cavity-coupled telecom atomic source in silicon. Nat. Commun. 2024, 15, 2350. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Li, H.-O.; Cao, G.; Xiao, M.; Guo, G.-C.; Guo, G.-P. Semiconductor quantum computation. Natl. Sci. Rev. 2019, 6, 32–54. [Google Scholar] [CrossRef]
- Jia, Z.; Fu, Y.; Cao, Z.; Cheng, W.; Zhao, Y.; Dou, M.; Duan, P.; Kong, W.; Cao, G.; Li, H.; et al. Superconducting and Silicon-Based Semiconductor Quantum Computers: A review. IEEE Nanotechnol. Mag. 2022, 16, 10–19. [Google Scholar] [CrossRef]
- Bluhm, H.; Schreiber, L.R. Semiconductor Spin Qubits—A S calable Platform for Quantum Computing? In Proceedings of the 2019 IEEE International Symposium on Circuits and Systems (ISCAS), Sapporo, Japan, 26–29 May 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Schreiber, L.; Bluhm, H. Silicon comes back. Nat. Nanotech. 2014, 9, 966–968. [Google Scholar] [CrossRef]
- Laucht, A.; Hohls, F.; Ubbelohde, N.; Gonzalez-Zalba, M.F.; Reilly, D.J.; Stobbe, S.; Schröder, T.; Scarlino, P.; Koski, J.V.; Dzurak, A.; et al. Roadmap on quantum nanotechnologies. Nanotechnology 2021, 32, 162003. [Google Scholar] [CrossRef]
- Sabbagh, D.; Thomas, N.; Torres, J.; Pillarisetty, R.; Amin, P.; George, H.; Singh, K.; Budrevich, A.; Robinson, M.; Merrill, D.; et al. Quantum Transport Properties of Industrial 28Si/28SiO2. Phys. Rev. Appl. 2019, 12, 014013. [Google Scholar] [CrossRef]
- Xue, X.; Patra, B.; van Dijk, J.P.G.; Samkharadze, N.; Subramanian, S.; Corna, A.; Wuetz, B.P.; Jeon, C.; Sheikh, F.; Juarez-Hernandez, E.; et al. CMOS-based cryogenic control of silicon quantum circuits. Nature 2021, 593, 205–210. [Google Scholar] [CrossRef]
- Shankar, S.; Tyryshkin, A.M.; He, J.; Lyon, S.A. Spin relaxation and coherence times for electrons at the Si/SiO2 interface. Phys. Rev. B 2010, 82, 195323. [Google Scholar] [CrossRef]
- Schäffler, F. High-mobility Si and Ge structures. Semicond. Sci. Technol. 1997, 12, 1515. [Google Scholar] [CrossRef]
- Watson, T.F.; Philips, S.G.J.; Kawakami, E.; Ward, D.R.; Scarlino, P.; Veldhorst, M.; Savage, D.E.; Lagally, M.G.; Friesen, M.; Coppersmith, S.N.; et al. A programmable two-qubit quantum processor in silicon. Nature 2018, 555, 633–637. [Google Scholar] [CrossRef] [PubMed]
- Hollmann, A.; Struck, T.; Langrock, V.; Schmidbauer, A.; Schauer, F.; Leonhardt, T.; Sawano, K.; Riemann, H.; Abrosimov, N.V.; Bougeard, D.; et al. Large, Tunable Valley Splitting and Single-Spin Relaxation Mechanisms in a Si/SixGe1−x Quantum Dot. Phys. Rev. Appl. 2020, 13, 034068. [Google Scholar] [CrossRef]
- Simmons, S.; Brown, R.M.; Riemann, H.; Abrosimov, N.V.; Becker, P.; Pohl, H.-J.; Thewalt, M.L.W.; Itoh, K.M.; Morton, J.J.L. Entanglement in a solid-state spin ensemble. Nature 2011, 470, 69–72. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Gradwohl, K.-P.; Lu, C.-H.; Yamamoto, Y.; Remmele, T.; Corley-Wiciak, C.; Teubner, T.; Richter, C.; Albrecht, M.; Boeck, T. Growth of 28Si Quantum Well Layers for Qubits by a Hybrid MBE/CVD Technique. ECS J. Solid State Sci. Technol. 2023, 12, 024006. [Google Scholar] [CrossRef]
- Scappucci, G.; Kloeffel, C.; Zwanenburg, F.A.; Loss, D.; Myronov, M.; Zhang, J.-J.; De Franceschi, S.; Katsaros, G.; Veldhorst, M. The germanium quantum information route. Nat. Rev. Mater. 2021, 6, 926–943. [Google Scholar] [CrossRef]
- Hill, C.D.; Peretz, E.; Hile, S.J.; House, M.G.; Fuechsle, M.; Rogge, S.; Simmons, M.Y.; Hollenberg, L.C.L. A surface code quantum computer in silicon. Sci. Adv. 2015, 1, e1500707. [Google Scholar] [CrossRef]
- Morton, J.J.L.; Tyryshkin, A.M.; Brown, R.M.; Shankar, S.; Lovett, B.W.; Ardavan, A.; Schenkel, T.; Haller, E.E.; Ager, J.W.; Lyon, S.A. Solid-state quantum memory using the 31P nuclear spin. Nature 2008, 455, 1085–1088. [Google Scholar] [CrossRef]
- O’Brien, J.L.; Schofield, S.R.; Simmons, M.Y.; Clark, R.G.; Dzurak, A.S.; Curson, N.J.; Kane, B.E.; McAlpine, N.S.; Hawley, M.E.; Brown, G.W. Towards the fabrication of phosphorus qubits for a silicon quantum computer. Phys. Rev. B 2001, 64, 161401. [Google Scholar] [CrossRef]
- Parthasarathy, S.K.; Kallinger, B.; Kaiser, F.; Berwian, P.; Dasari, D.B.R.; Friedrich, J.; Nagy, R. Scalable Quantum Memory Nodes Using Nuclear Spins in Silicon Carbide. Phys. Rev. Appl. 2023, 19, 034026. [Google Scholar] [CrossRef]
- Babin, C.; Stöhr, R.; Morioka, N.; Linkewitz, T.; Steidl, T.; Wörnle, R.; Liu, D.; Hesselmeier, E.; Vorobyov, V.; Denisenko, A.; et al. Fabrication and nanophotonic waveguide integration of silicon carbide colour centres with preserved spin-optical coherence. Nat. Mater. 2022, 21, 67–73. [Google Scholar] [CrossRef] [PubMed]
- Plotnichenko, V.G.; Nazaryants, V.O.; Kryukova, E.B.; Koltashev, V.V.; Sokolov, V.O.; Gusev, A.V.; Gavva, V.A.; Kotereva, T.V.; Churbanov, M.F.; Dianov, E.M. Refractive index spectral dependence, Raman spectra, and transmission spectra of high-purity 28Si, 29Si, 30Si, and natSi single crystals. Appl. Opt. 2011, 50, 4633–4641. [Google Scholar] [CrossRef]
- Moutanabbir, O.; Senz, S.; Zhang, Z.; Gösele, U. Synthesis of isotopically controlled metal-catalyzed silicon nanowires. Nano Today 2009, 4, 393–398. [Google Scholar] [CrossRef]
- Wagner, R.S.; Ellis, W.C. Vapor-Liquid-Solid mechanism of Single-Crystal growth. Appl. Phys. Lett. 1964, 4, 89–90. [Google Scholar] [CrossRef]
- Ernst, O.C.; Uebel, D.; Kayser, S.; Lange, F.; Teubner, T.; Boeck, T. Revealing all states of dewetting of a thin gold layer on a silicon surface by nanosecond laser conditioning. Appl. Surf. Sci. Adv. 2021, 3, 100040. [Google Scholar] [CrossRef]
- Ernst, O.C.; Lange, F.; Uebel, D.; Teubner, T.; Boeck, T. Analysis of catalyst surface wetting: The early stage of epitaxial germanium nanowire growth. Beilstein J. Nanotechnol. 2020, 11, 1371–1380. [Google Scholar] [CrossRef] [PubMed]
- Lange, F.; Ernst, O.; Teubner, T.; Richter, C.; Schmidbauer, M.; Skibitzki, O.; Schroeder, T.; Schmidt, P.; Boeck, T. In-plane growth of germanium nanowires on nanostructured Si(001)/SiO2 substrates. Nano Futures 2020, 4, 035006. [Google Scholar] [CrossRef]
- Shi, L.; Yao, D.; Zhang, G.; Li, B. Size dependent thermoelectric properties of silicon nanowires. Appl. Phys. Lett. 2009, 95, 063102. [Google Scholar] [CrossRef]
- Ci, P.; Sun, M.; Upadhyaya, M.; Song, H.; Jin, L.; Sun, B.; Jones, M.R.; Ager, J.W.; Aksamija, Z.; Wu, J. Giant Isotope Effect of Thermal Conductivity in Silicon Nanowires. Phys. Rev. Lett. 2022, 128, 085901. [Google Scholar] [CrossRef] [PubMed]
- Available online: http://isosilicon.com/wp-content/uploads/2016/01/JO-Odden-Si-28.pdf (accessed on 25 July 2024).
- Nobuyoshi, F.; Satoshi, U.; Hirotoshi, Y. Monoisotopic Silicon-28 (28Si). Japanese Patent JP2005129602, 2005. [Google Scholar]
- Fujimaki, N.; Ushio, S. Solar Cell And Method For Manufacturing The Same. Japanese Patent JP2005142434, 19 May 2005. [Google Scholar]
- Available online: https://gepris.dfg.de/gepris/projekt/221263527?context=projekt&task=showDetail&id=221263527& (accessed on 25 July 2024).
- Available online: https://prosjektbanken.forskningsradet.no/en/project/FORISS/193329?Kilde=FORISS&distribution=Ar&chart=bar&calcType=funding&Sprak=no&sortBy=score&sortOrder=desc&resultCount=30&offset=90&Fritekst=INVENT&source=FORISS&projectId=176869 (accessed on 25 July 2024).

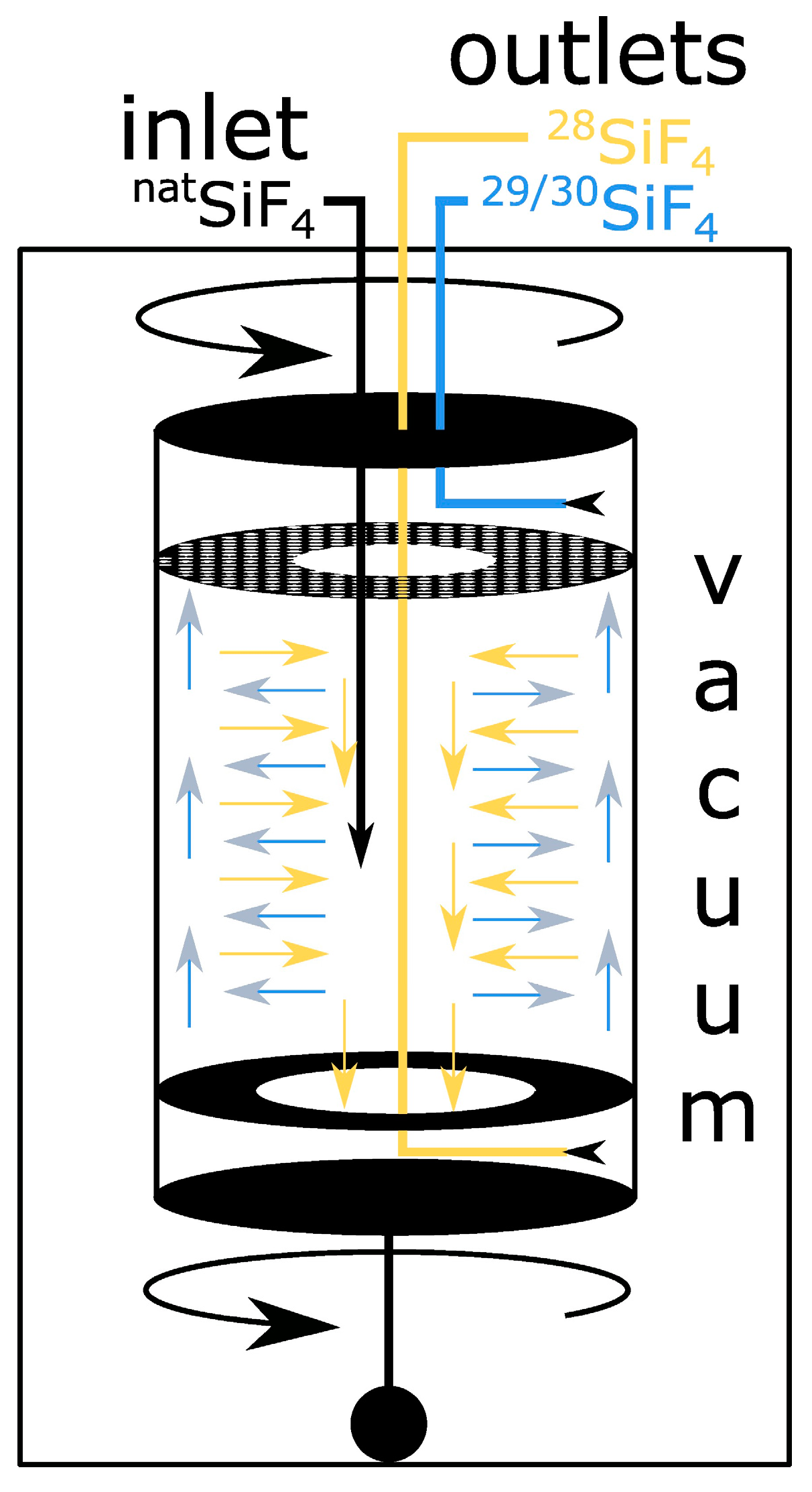


| Nucleon Number Si | Nucleon Number F/H | Isotopic Ratio | Molar Mass | |
|---|---|---|---|---|
| 28SiF4 | 28 | 19 | 92.23% | 103.94 u |
| 29SiF4 | 29 | 19 | 4.67% | 104.94 u |
| 30SiF4 | 30 | 19 | 3.10% | 105.93 u |
| 28Si1H4 | 28 | 1 | 92.219(4)% | 32.01 u |
| 28Si2H4 | 28 | 2 | 0.010(6)% | 36.03 u |
| 29Si1H4 | 29 | 1 | 4.669(5)% | 33.01 u |
| 29Si2H4 | 29 | 2 | 0.000(5)% | 37.03 u |
| 30Si1H4 | 30 | 1 | 3.099(6)% | 34.00 u |
| 30Si2H4 | 30 | 2 | 0.000(4)% | 38.14 u |
| natSi | 28Si | Reference | |
|---|---|---|---|
| Mass distribution | 92.2%—28 u | 100%—28 u | [102] |
| 4.7%—29 u | |||
| 3.1%—30 u | |||
| Thermal conductivity @ 21 K | 45 Wcm−1K−1 | 450 Wcm−1K−1 | [7] |
| Band gap | 1.12 eV | 1.06 eV | [8] |
| Nuclear spin | I = 1/2; Ī = √3/2 ħ | I = 0; Ī = 0 ħ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ernst, O.C.; Uebel, D.; Brendler, R.; Kraushaar, K.; Steudel, M.; Acker, J.; Kroke, E. Silicon-28-Tetrafluoride as an Educt of Isotope-Engineered Silicon Compounds and Bulk Materials for Quantum Systems. Molecules 2024, 29, 4222. https://doi.org/10.3390/molecules29174222
Ernst OC, Uebel D, Brendler R, Kraushaar K, Steudel M, Acker J, Kroke E. Silicon-28-Tetrafluoride as an Educt of Isotope-Engineered Silicon Compounds and Bulk Materials for Quantum Systems. Molecules. 2024; 29(17):4222. https://doi.org/10.3390/molecules29174222
Chicago/Turabian StyleErnst, Owen C., David Uebel, Roman Brendler, Konstantin Kraushaar, Max Steudel, Jörg Acker, and Edwin Kroke. 2024. "Silicon-28-Tetrafluoride as an Educt of Isotope-Engineered Silicon Compounds and Bulk Materials for Quantum Systems" Molecules 29, no. 17: 4222. https://doi.org/10.3390/molecules29174222
APA StyleErnst, O. C., Uebel, D., Brendler, R., Kraushaar, K., Steudel, M., Acker, J., & Kroke, E. (2024). Silicon-28-Tetrafluoride as an Educt of Isotope-Engineered Silicon Compounds and Bulk Materials for Quantum Systems. Molecules, 29(17), 4222. https://doi.org/10.3390/molecules29174222






