Modelling Complex Bimolecular Reactions in a Condensed Phase: The Case of Phosphodiester Hydrolysis
Abstract
1. Introduction
2. Theory
2.1. General Considerations
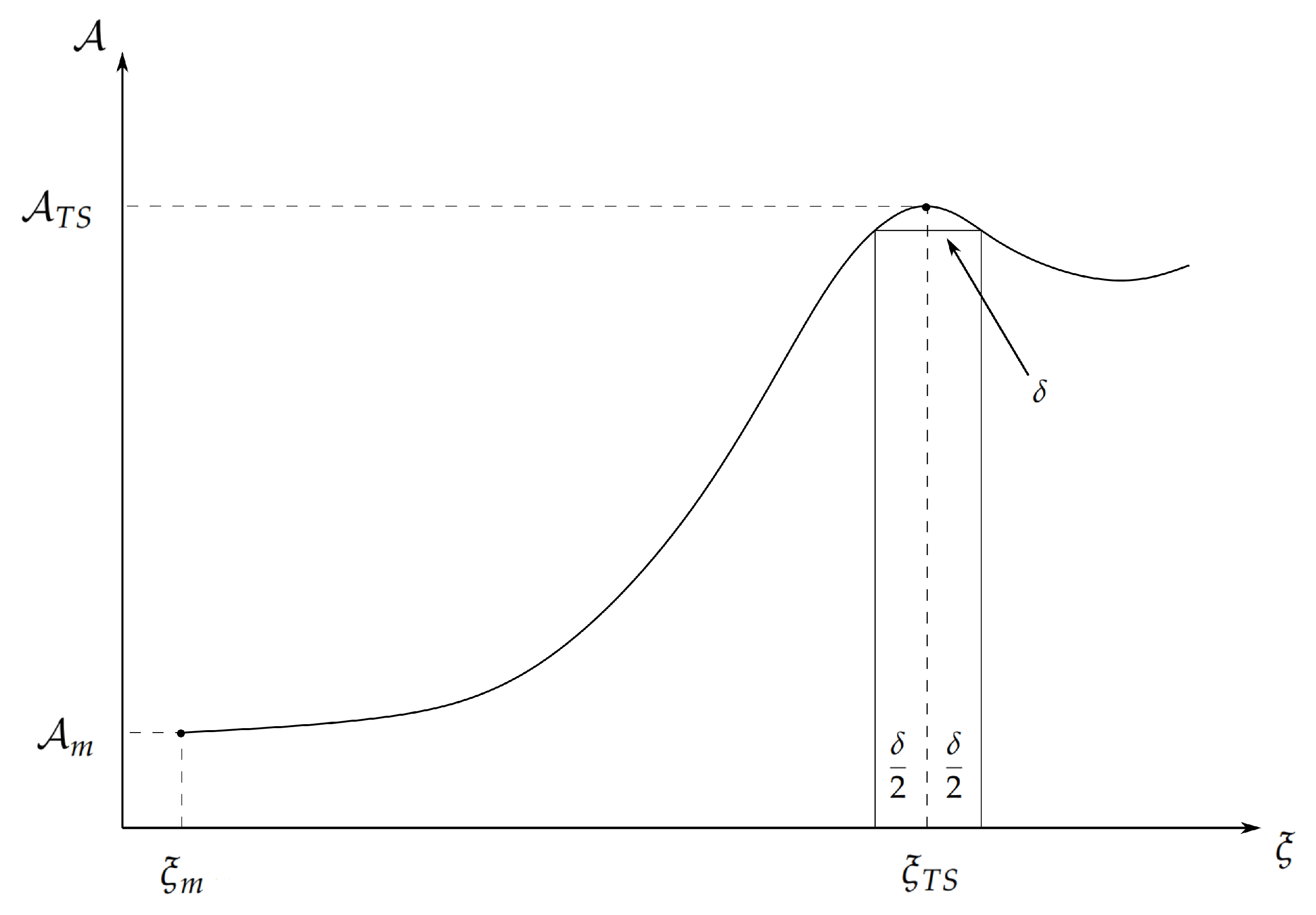
2.2. The Reaction Coordinate, the Landau Free Energy and the Rate Constant
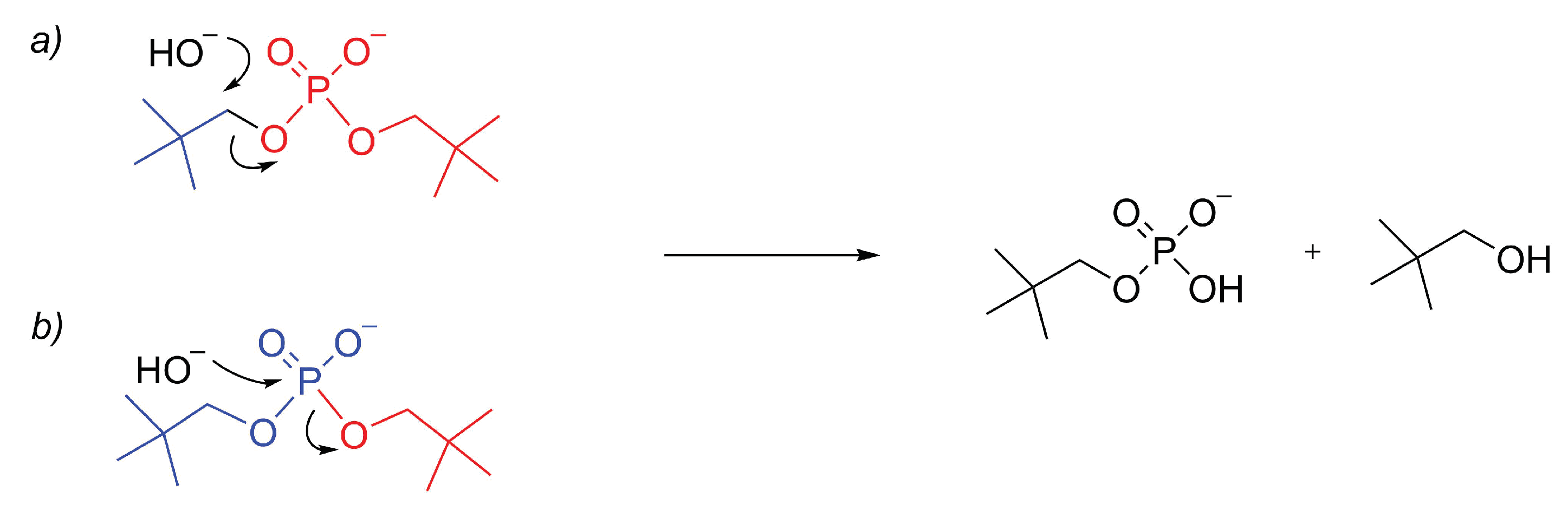
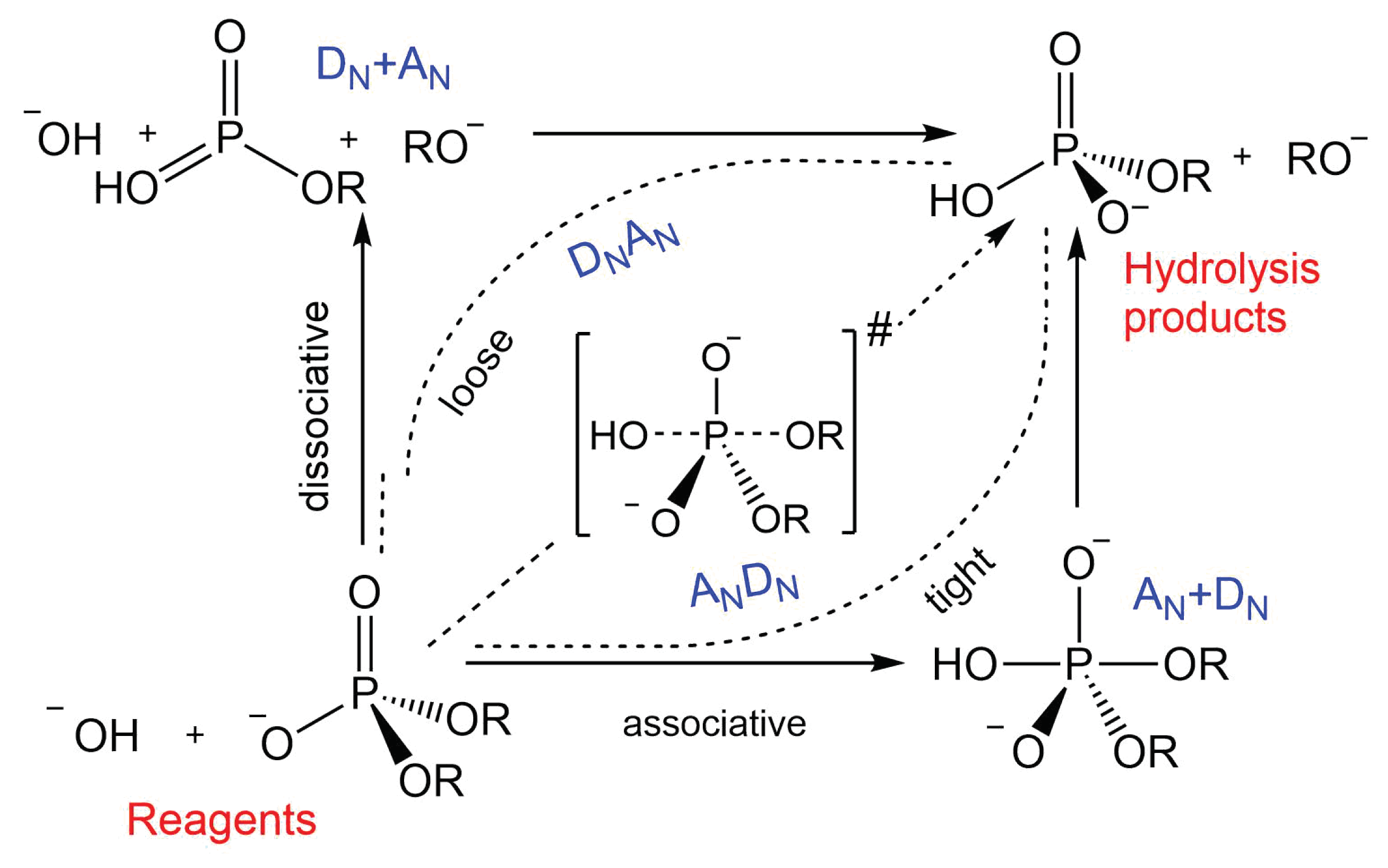
2.3. Application to the Hydrolysis Reaction
3. Methods
3.1. Quantum Chemical Calculations
3.2. Molecular Dynamics Simulations
3.3. Computational Strategy
- Calculation of the unperturbed quantum chemical properties, needed to apply the MD-PMM procedure, for each point along the reaction coordinate (electronic ground state energy, permanent dipole moments and CHELPG charges [40]).
- Simulations of selected representative ensemble along the reaction coordinate (reactants, two intermediate structures between reactants and transition state, corresponding to different nucleophile–phosphorous atom distances, and the transition state) providing the perturbation along the reaction coordinate.
- MD-PMM calculation for each point to obtain the free energy difference from one point to the following along the reaction coordinate, according to Equation (17).
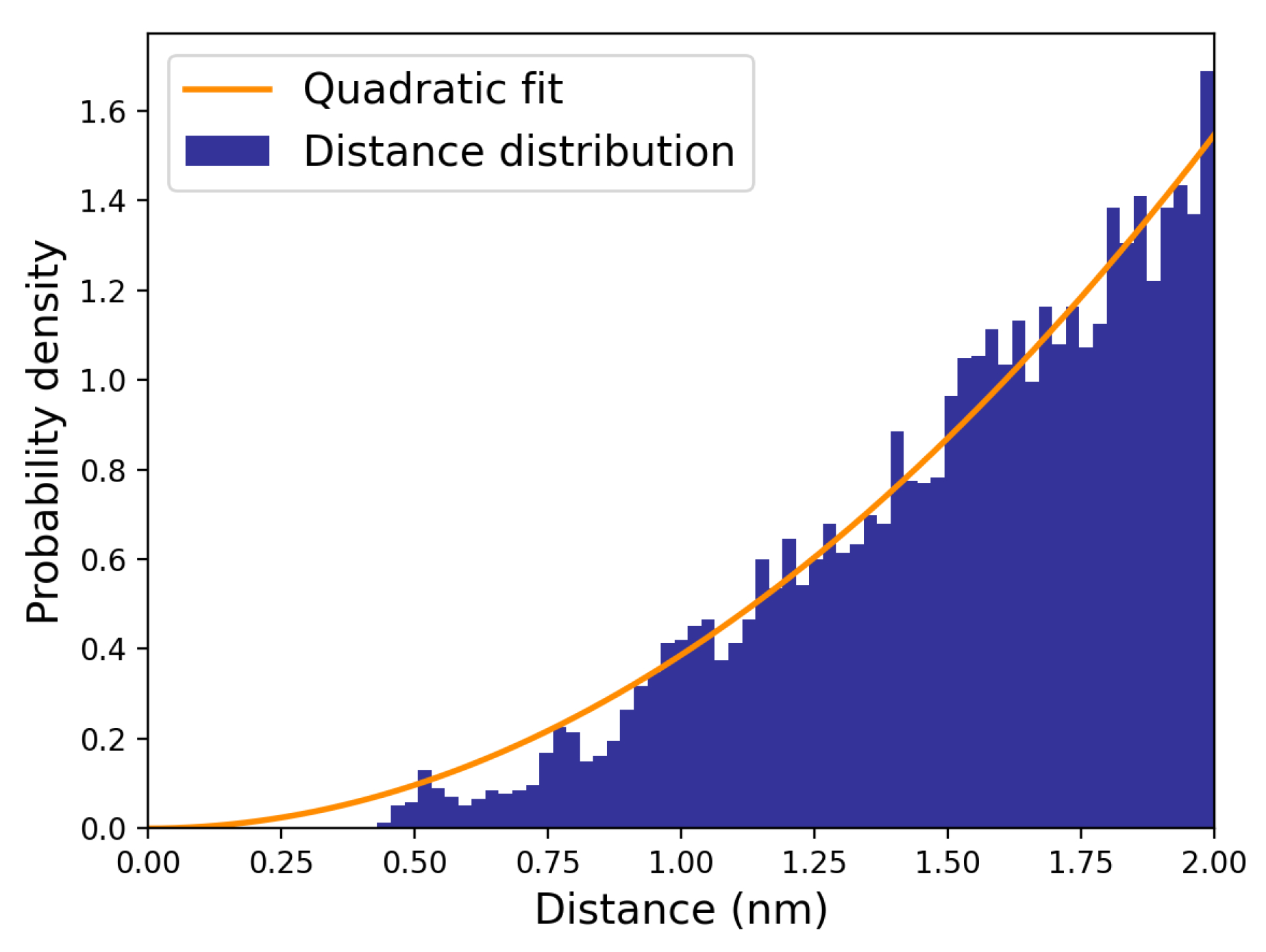
- Calculation of the kinetic rate constants involved in the reactive non-reactive equilibrium in Scheme (21) by performing different sets of MD simulations: (i) a 100 ns long simulation of the free reactants to obtain the hydroxide ion–dineopentyl phosphate distance probability distribution and the equilibrium fraction of the non-reactive complex, ; (ii) one short simulation for the determination of the diffusion coefficient of the dineopentyl phosphate; (iii) a set of 70 simulations in order to reconstruct the reactive and non-reactive complex dissociation kinetics.
4. Results and Discussion
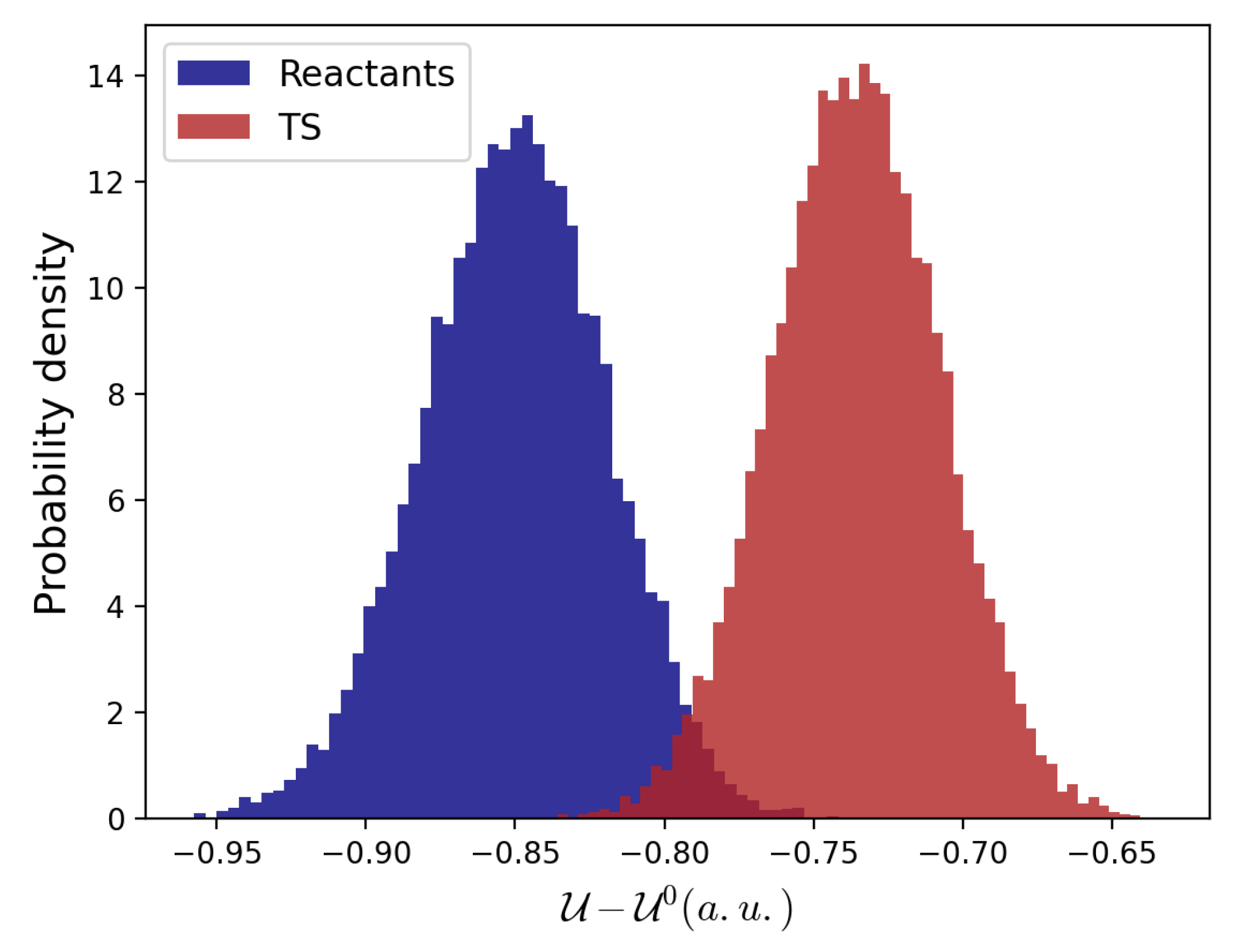
4.1. Evaluation of the Rate Constant of the Dineopentyl Phosphate Hydrolysis
4.2. Evaluation of the Other Kinetic Rate Constants
4.3. Hydrolysis of the Dimethyl Phosphate
4.4. Mechanistic Considerations
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wolfenden, R. Degrees of difficulty of water-consuming reactions in the absence of enzymes. Chem. Rev. 2006, 106, 3379–3396. [Google Scholar] [CrossRef]
- Schroeder, G.K.; Lad, C.; Wyman, P.; Williams, N.H.; Wolfenden, R. The time required for water attack at the phosphorus atom of simple phosphodiesters and of DNA. Proc. Natl. Acad. Sci. USA 2006, 103, 4052–4055. [Google Scholar] [CrossRef]
- Zalatan, J.G.; Herschlag, D. Alkaline phosphatase mono-and diesterase reactions: Comparative transition state analysis. J. Am. Chem. Soc. 2006, 128, 1293–1303. [Google Scholar] [CrossRef]
- Raynal, M.; Ballester, P.; Vidal-Ferran, A.; van Leeuwen, P.W. Supramolecular catalysis. Part 2: Artificial enzyme mimics. Chem. Soc. Rev. 2014, 43, 1734–1787. [Google Scholar] [CrossRef]
- Mikkola, S.; Lönnberg, T.; Lönnberg, H. Phosphodiester models for cleavage of nucleic acids. Beilstein J. Org. Chem. 2018, 14, 803–837. [Google Scholar] [CrossRef]
- Mancin, F.; Scrimin, P.; Tecilla, P. Progress in artificial metallonucleases. Chem. Commun. 2012, 48, 5545–5559. [Google Scholar] [CrossRef]
- Morrow, J.R.; Amyest, T.L.; Richard, J.P. Phosphate Binding Energy and Catalysis by Small and Large Molecules. Acc. Chem. Res. 2008, 41, 539–548. [Google Scholar] [CrossRef]
- Segreto, G.E.; Alba, J.; Salvio, R.; D’Abramo, M. DNA cleavage by endonuclease I-DmoI: A QM/MM study and comparison with experimental data provide indications on the environmental effects. Theor. Chem. Acc. 2020, 139, 1–7. [Google Scholar] [CrossRef]
- Salvio, R.; Casnati, A. Guanidinium Promoted Cleavage of Phosphoric Diesters: Kinetic Investigations and Calculations Provide Indications on the Operating Mechanism. J. Org. Chem. 2017, 82, 10461–10469. [Google Scholar] [CrossRef]
- Salvio, R.; Moliterno, M.; Caramelli, D.; Pisciottani, L.; Antenucci, A.; D’Amico, M.; Bella, M. Kinetic resolution of phosphoric diester by Cinchona alkaloid derivatives provided with a guanidinium unit. Cat. Sci. Technol. 2016, 6, 2280–2288. [Google Scholar] [CrossRef]
- Salvio, R.; Casnati, A.; Mandolini, L.; Sansone, F.; Ungaro, R. ATP cleavage by cone tetraguanidinocalix[4]arene. Org. Biomol. Chem. 2012, 10, 8941–8943. [Google Scholar] [CrossRef]
- Salvio, R.; Volpi, S.; Cacciapaglia, R.; Sansone, F.; Mandolini, L.; Casnati, A. Phosphoryl Transfer Processes Promoted by a Trifunctional Calix[4]arene Inspired by DNA Topoisomerase I. J. Org. Chem. 2016, 81, 9012–9019. [Google Scholar] [CrossRef] [PubMed]
- Cacciapaglia, R.; Di Stefano, S.; Mandolini, L.; Salvio, R. Reactivity of carbonyl and phosphoryl groups at calixarenes. Supramol. Chem. 2013, 25, 537–554. [Google Scholar] [CrossRef]
- Salvio, R.; Volpi, S.; Folcarelli, T.; Casnati, A.; Cacciapaglia, R. A calix[4]arene with acylguanidine units as an efficient catalyst for phosphodiester bond cleavage in RNA and DNA model compounds. Org. Biomol. Chem. 2019, 17, 7482–7492. [Google Scholar] [CrossRef] [PubMed]
- Imhof, P.; Fischer, S.; Krämer, R.; Smith, J.C. Density functional theory analysis of dimethylphosphate hydrolysis: Effect of solvation and nucleophile variation. J. Mol. Struct. Theochem 2005, 713, 1–5. [Google Scholar] [CrossRef]
- Lopez, X.; Dejaegere, A.; Leclerc, F.; York, D.M.; Karplus, M. Nucleophilic attack on phosphate diesters: A density functional study of in- line reactivity in dianionic, monoanionic, and neutral systems. J. Phys. Chem. B 2006, 110, 11525–11539. [Google Scholar] [CrossRef]
- Kirby, A.J.; Younas, M. The Reactivity of Phosphate Esters. Diester Hydrolysis. J. Chem. Soc. B 1970, 510–513. [Google Scholar] [CrossRef]
- Kim, J.H.; Chin, J. Dimethyl Phosphate Hydrolysis at Neutral pH. J. Am. Chem. Soc. 1992, 114, 9792–9795. [Google Scholar] [CrossRef]
- Prieto, J.; Epinat, J.C.; Redondo, P.; Ramos, E.; Padro, D.; Cedrone, F.; Montoya, G.; Paques, F.; Blanco, F. Generation and Analysis of Mesophilic Variants of the Thermostable Archaeal I-DmoI Homing Endonuclease. J. Biol. Chem. 2008, 283, 4364–4374. [Google Scholar] [CrossRef]
- Stoddart, B.L. Homing endonuclease structure and function. Q. Rev. Biophys. 2006, 38, 49–95. [Google Scholar] [CrossRef]
- Amadei, A.; D’Alessandro, M.; Aschi, M. Statistical mechanical modeling of chemical reactions in complex systems: The reaction free energy surface. J. Phys. Chem. B 2004, 108, 16250–16254. [Google Scholar] [CrossRef]
- Zanetti-Polzi, L.; Del Galdo, S.; Daidone, I.; D’Abramo, M.; Barone, V.; Aschi, M.; Amadei, A. Extending the perturbed matrix method beyond the dipolar approximation: Comparison of different levels of theory. Phys. Chem. Chem. Phys. 2018, 20, 24369–24378. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.G.; Nardi, A.N.; Amadei, A.; D’Abramo, M. PyMM: An Open-Source Python Program for QM/MM Simulations Based on the Perturbed Matrix Method. J. Chem. Theory Comput. 2023, 19, 33–41. [Google Scholar] [CrossRef] [PubMed]
- Cantor, C.R.; Schimmel, P.R. Biophysical Chemistry Part II; W.H. Freeman and Co.: San Francisco, CA, USA; New York, NY, USA, 1998. [Google Scholar]
- Del Galdo, S.; Aschi, M.; Amadei, A. In silico characterization of bimolecular electron transfer reactions: The ferrocene–ferrocenium reaction as a test case. Int. J. Quantum Chem. 2016, 116, 1723–1730. [Google Scholar] [CrossRef]
- Neese, F. The ORCA program system. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Adamo, C.; Barone, V. Toward reliable density functional methods without adjustable parameters: The PBE0 model. J. Chem. Phys. 1999, 110, 6158–6170. [Google Scholar] [CrossRef]
- Krishnan, R.; Binkley, J.S.; Seeger, R.; Pople, J.A. Self-consistent molecular orbital methods. XX. A basis set for correlated wave functions. J. Chem. Phys. 1980, 72, 650–654. [Google Scholar] [CrossRef]
- Clark, T.; Chandrasekhar, J.; Spitznagel, G.W.; Schleyer, P.V.R. Efficient diffuse function-augmented basis sets for anion calculations. III. The 3-21+ G basis set for first-row elements, Li–F. J. Comput. Chem. 1983, 4, 294–301. [Google Scholar] [CrossRef]
- Ribeiro, A.J.; Ramos, M.J.; Fernandes, P.A. Benchmarking of DFT functionals for the hydrolysis of phosphodiester bonds. J. Chem. Theory Comput. 2010, 6, 2281–2292. [Google Scholar] [CrossRef]
- Hehre, W.J.; Ditchfield, R.; Pople, J.A. Self—consistent molecular orbital methods. XII. Further extensions of Gaussian—type basis sets for use in molecular orbital studies of organic molecules. J. Chem. Phys. 1972, 56, 2257–2261. [Google Scholar] [CrossRef]
- Abraham, M.J.; Murtola, T.; Schulz, R.; Páll, S.; Smith, J.C.; Hess, B.; Lindahl, E. GROMACS: High performance molecular simulations through multi-level parallelism from laptops to supercomputers. SoftwareX 2015, 1–2, 19–25. [Google Scholar] [CrossRef]
- Mark, P.; Nilsson, L. Structure and dynamics of the TIP3P, SPC, and SPC/E water models at 298 K. J. Phys. Chem. A 2001, 105, 9954–9960. [Google Scholar] [CrossRef]
- Sousa da Silva, A.W.; Vranken, W.F. ACPYPE-Antechamber python parser interface. BMC Res. Notes 2012, 5, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Bussi, G.; Donadio, D.; Parrinello, M. Canonical sampling through velocity rescaling. J. Chem. Phys. 2007, 126, 014101. [Google Scholar] [CrossRef]
- Darden, T.; York, D.; Pedersen, L.G. Particle mesh Ewald: An N-log(N) method for Ewald sums in large systems. J. Chem. Phys. 1993, 98, 10089–10092. [Google Scholar] [CrossRef]
- Essmann, U.; Perera, L.; Berkowitz, M.L.; Darden, T.; Lee, H.; Pedersen, L.G. A smooth particle mesh Ewald method. J. Chem. Phys. 1995, 103, 8577–8593. [Google Scholar] [CrossRef]
- Allen, M. DJ Tildesley Computer Simulation of Liquids; Clarendon: Oxford, UK, 1987. [Google Scholar]
- Light, T.S.; Licht, S.; Bevilacqua, A.C.; Morash, K.R. The fundamental conductivity and resistivity of water. Electrochem. Solid-State Lett. 2004, 8, E16. [Google Scholar] [CrossRef]
- Breneman, C.M.; Wiberg, K.B. Determining atom-centered monopoles from molecular electrostatic potentials. The need for high sampling density in formamide conformational analysis. J. Comput. Chem. 1990, 11, 361–373. [Google Scholar] [CrossRef]
- Pereira, E.S.; Da Silva, J.C.; Brandao, T.A.; Rocha, W.R. Phosphorane lifetime and stereo-electronic effects along the alkaline hydrolysis of phosphate esters. Phys. Chem. Chem. Phys. 2016, 18, 18255–18267. [Google Scholar] [CrossRef]
- Prasad, B.R.; Plotnikov, N.V.; Warshel, A. Addressing open questions about phosphate hydrolysis pathways by careful free energy mapping. J. Phys. Chem. B 2013, 117, 153–163. [Google Scholar] [CrossRef]


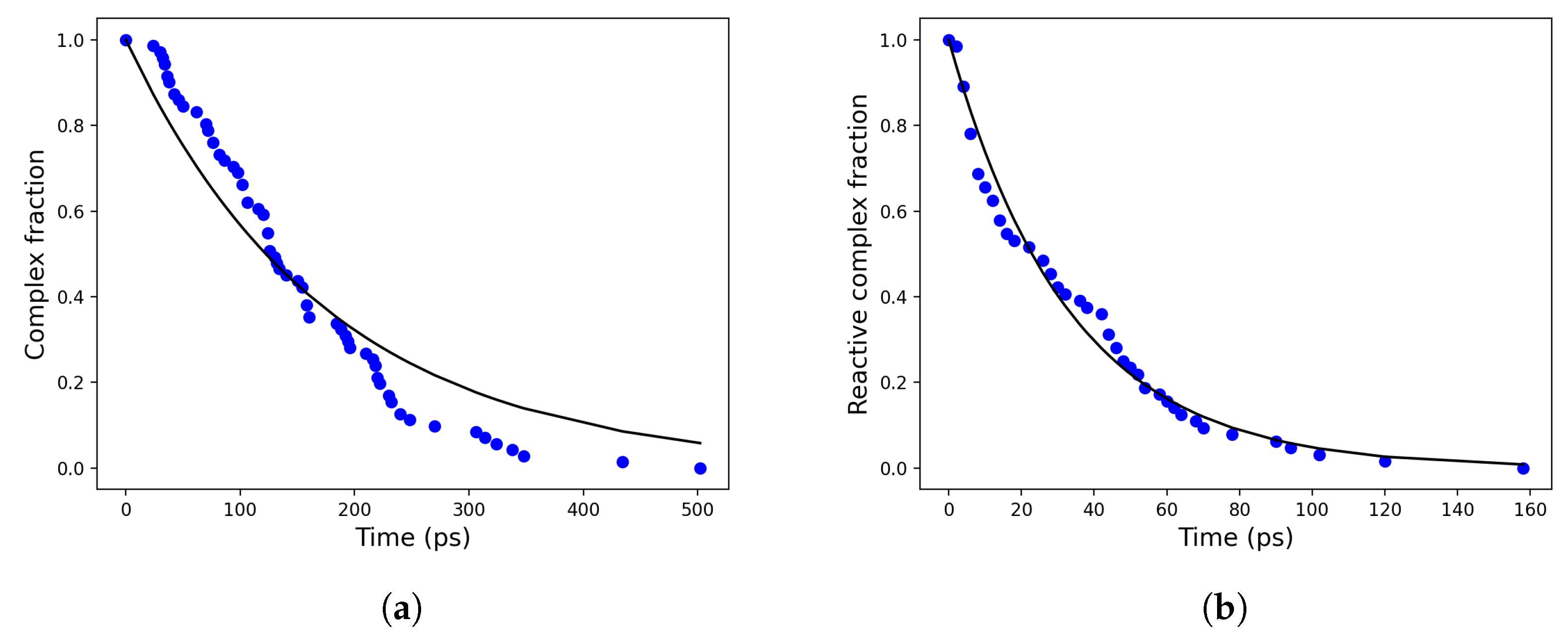
| Calculated Rate Constants | Value | Dimension |
|---|---|---|
| Experimental rate constant | ||
| 1.4 | ||
| 1.4 |
| Bond Cleavage | P–O | C–O | P–O | C–O |
| (kcal/mol) | 27.5 | 28.0 | 28.5 | 36.1 |
| (kcal/mol) | 39.5 | 30.9 | 38.8 | - |
| (s) | 3.75 | 3.17 | 1.85 | - |
| (sM) | NA | 3.0 | 1.4 | NA |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nardi, A.N.; Olivieri, A.; Amadei, A.; Salvio, R.; D’Abramo, M. Modelling Complex Bimolecular Reactions in a Condensed Phase: The Case of Phosphodiester Hydrolysis. Molecules 2023, 28, 2152. https://doi.org/10.3390/molecules28052152
Nardi AN, Olivieri A, Amadei A, Salvio R, D’Abramo M. Modelling Complex Bimolecular Reactions in a Condensed Phase: The Case of Phosphodiester Hydrolysis. Molecules. 2023; 28(5):2152. https://doi.org/10.3390/molecules28052152
Chicago/Turabian StyleNardi, Alessandro Nicola, Alessio Olivieri, Andrea Amadei, Riccardo Salvio, and Marco D’Abramo. 2023. "Modelling Complex Bimolecular Reactions in a Condensed Phase: The Case of Phosphodiester Hydrolysis" Molecules 28, no. 5: 2152. https://doi.org/10.3390/molecules28052152
APA StyleNardi, A. N., Olivieri, A., Amadei, A., Salvio, R., & D’Abramo, M. (2023). Modelling Complex Bimolecular Reactions in a Condensed Phase: The Case of Phosphodiester Hydrolysis. Molecules, 28(5), 2152. https://doi.org/10.3390/molecules28052152







