Nitrogen-Doped Titanium Dioxide as a Hole Transport Layer for High-Efficiency Formamidinium Perovskite Solar Cells
Abstract
1. Introduction
2. Methodology
2.1. SCAPS Simulation
2.2. TiO2:N as a p-Type HTL
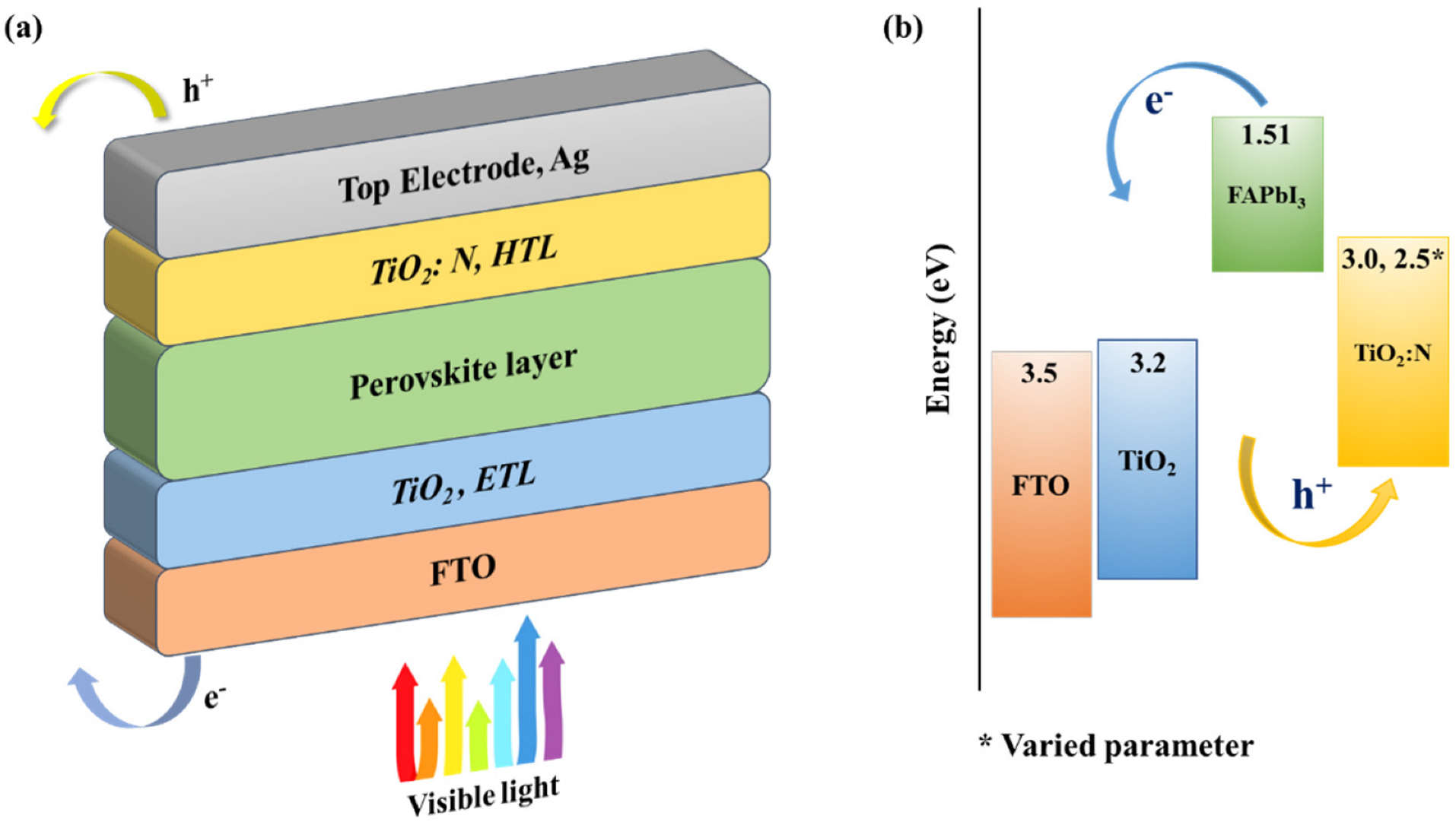
2.3. Recipe of the PSC Structure
- Recipe-1:
- FTO/TiO2/FAPbI3/TiO2:Na/Ag
- Recipe-2:
- FTO/TiO2/FAPbI3/TiO2:Nb/Ag
| Parameter | FTO | TiO2 | FAPbI3 | TiO2:Na | TiO2:Nb |
|---|---|---|---|---|---|
| Thickness ‘t’ (nm) | 400 | 50 | 300 * | 100 | 100 |
| Band gap ‘Eg’ (eV) | 3.5 | 3.2 | 1.51 | 2.5 | 3.0 |
| Electron affinity ‘χ’ (eV) | 4 | 4 | 4 | 2.2 | 2.2 |
| Dielectric Permittivity ‘εr’ | 9 | 9 | 6.6 | 3 | 3 |
| CB EDOS ‘Nc’ (cm−3) | 2.2 × 1018 | 2.1 × 1018 | 1.2 × 1019 | 1.3 × 1018 | 1.3 × 1014 |
| VB EDOS ‘Nv’ (cm−3) | 2.2 × 1018 | 2.2 × 1017 | 1.2 × 1019 | 1.3 × 1019 | 1.3 × 1015 |
| e− thermal velocity (cm·s−1) | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 |
| h+ thermal velocity (cm·s−1) | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 |
| Electron mobility ‘μn’ (cm2/V·s) | 20 | 20 | 2.7 | 1.5 | 2.0 |
| Hole mobility ‘μh’ (cm2/V·s) | 10 | 10 | 1.8 | 1.5 | 2.0 |
| Shallow donor density ‘N.D.’ (cm−3) | 2 × 1019 | 9 × 1016 | 1.3 × 1016 | 0 | 0 |
| Shallow Acceptor density ‘N.A.’ (cm−3) | 0 | 0 | 1.3 × 1016 | 1.3 × 1019 | 1.3 × 1014 |
| Defect density ‘Nt’ (cm−3) | 1015 | 1015 | 1 × 1013 * | 1015 | 1015 |
- (a)
- The phase of the formamidinium crystal structure is stable at the α-phase; there is no drift into the δ-phase.
- (b)
- The temperature coefficient on the perovskite recipes is precluded.
3. Results and Discussion
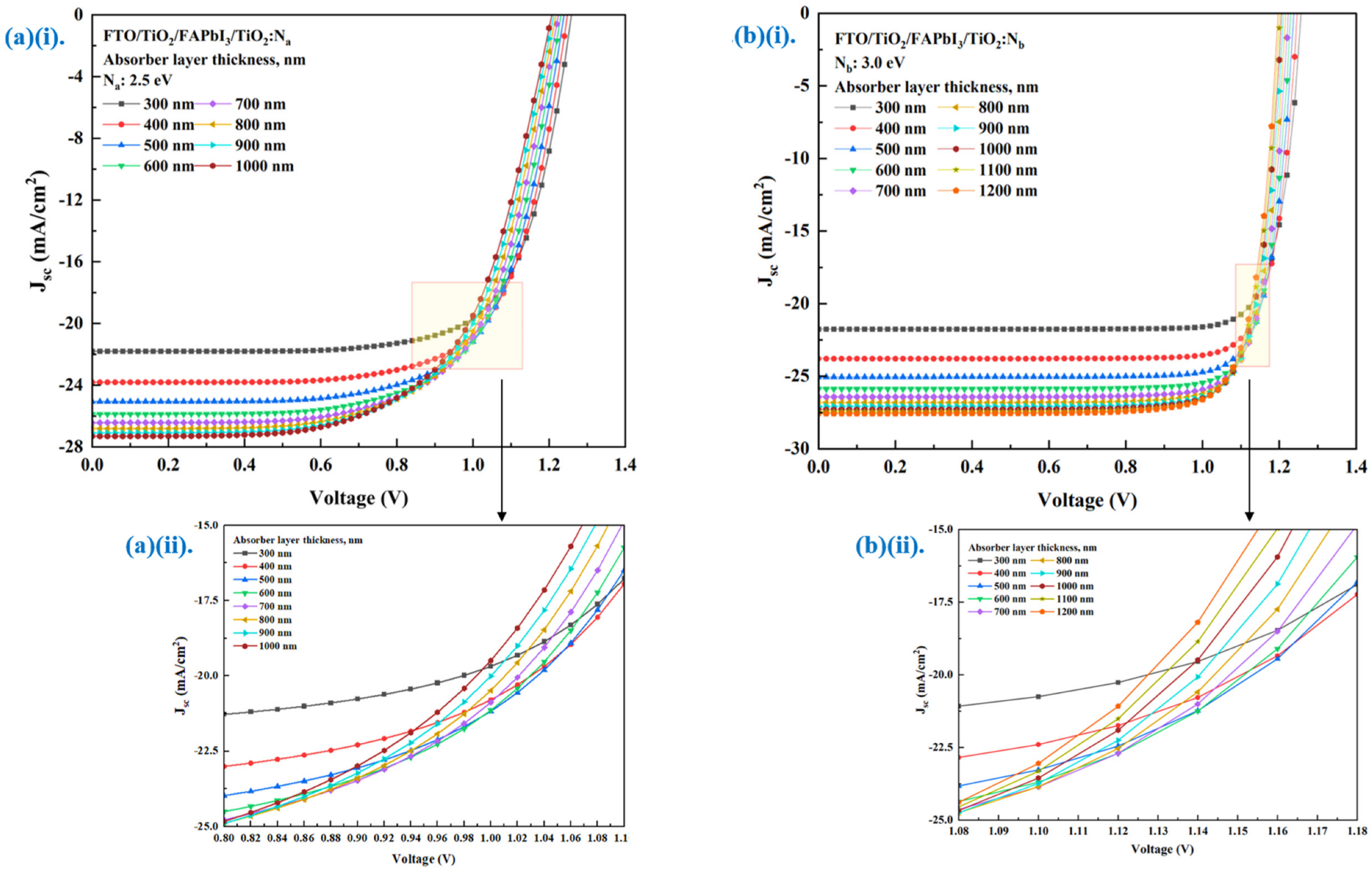
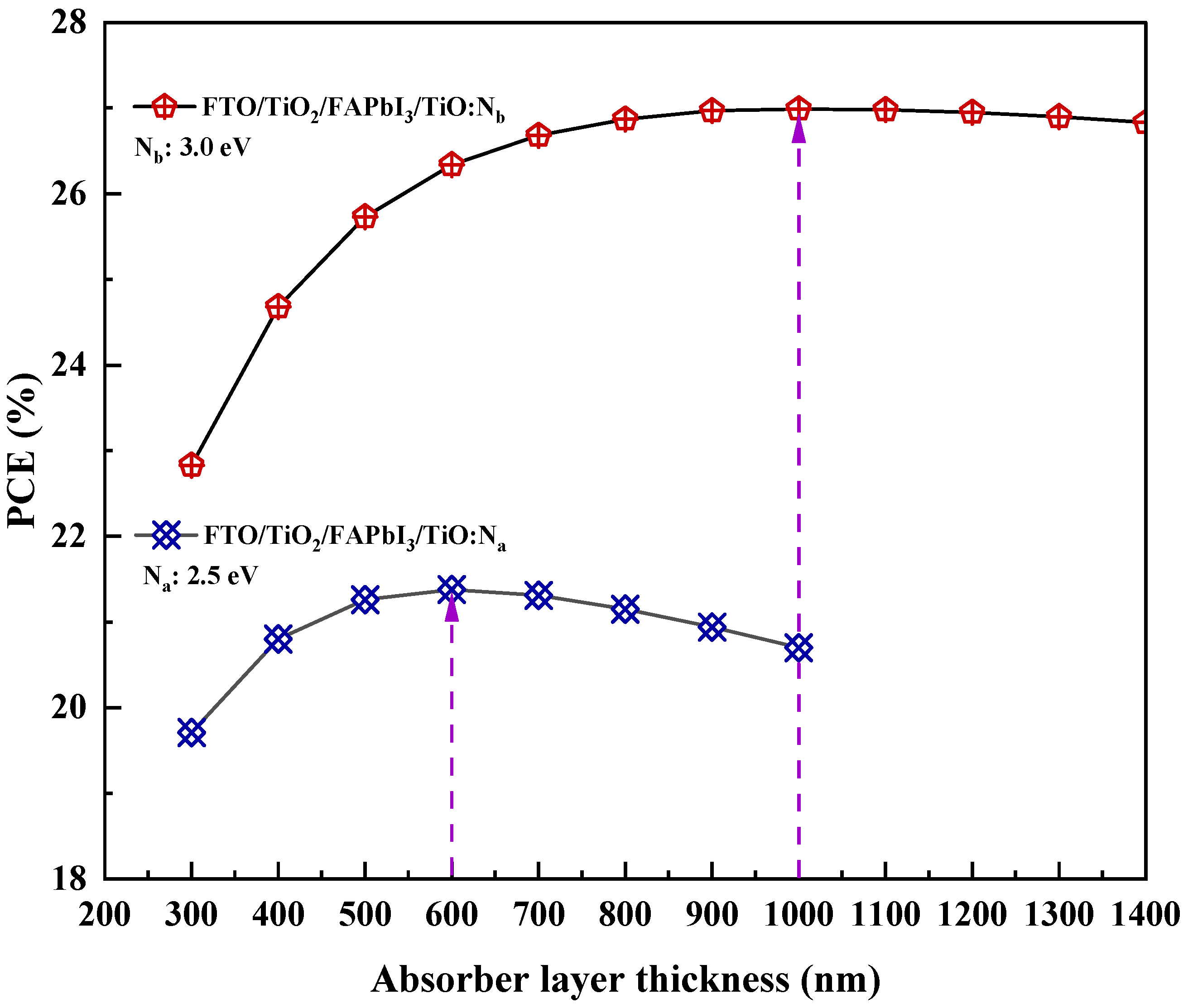
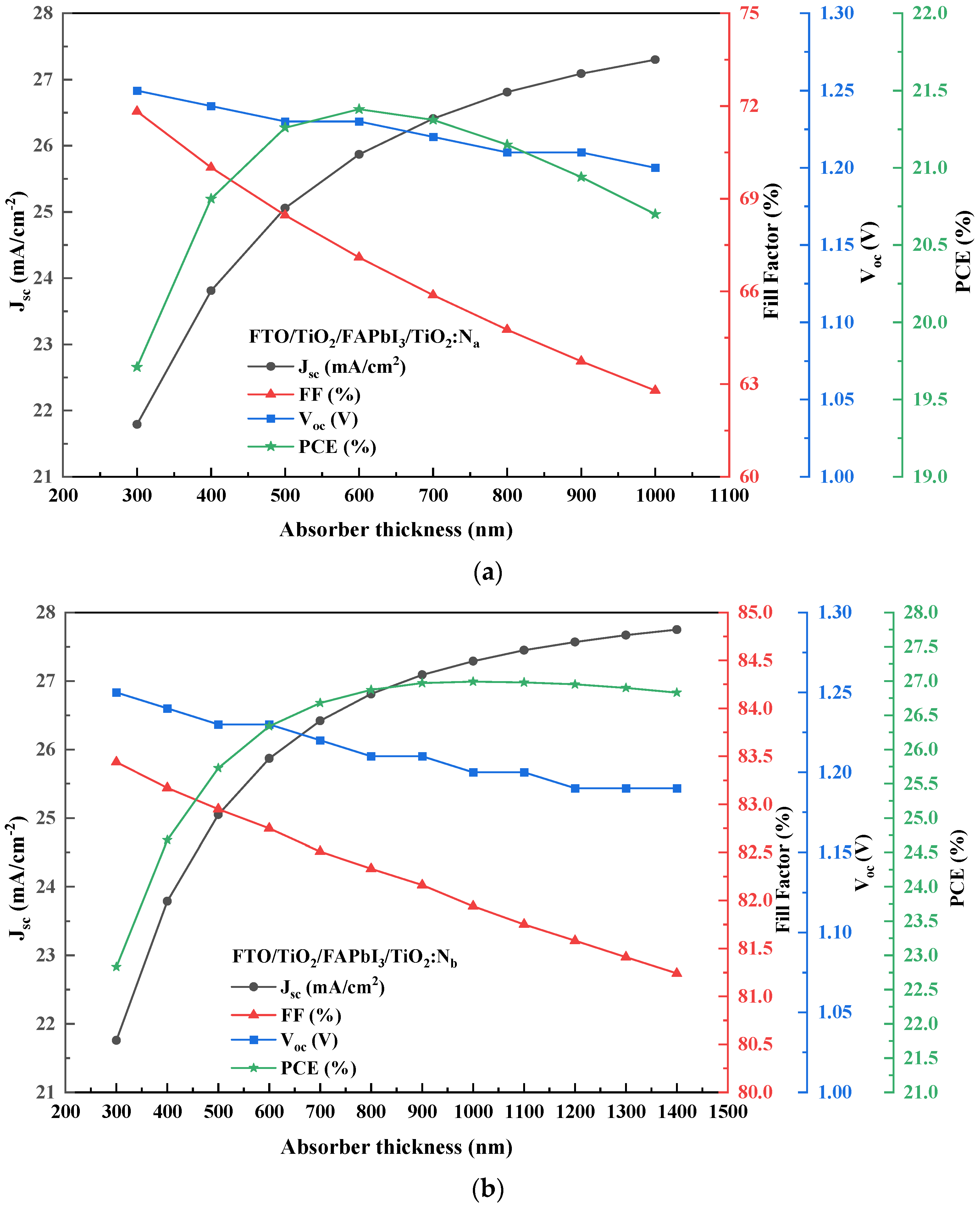
3.1. Effect of Perovskite Absorber Layer Thickness
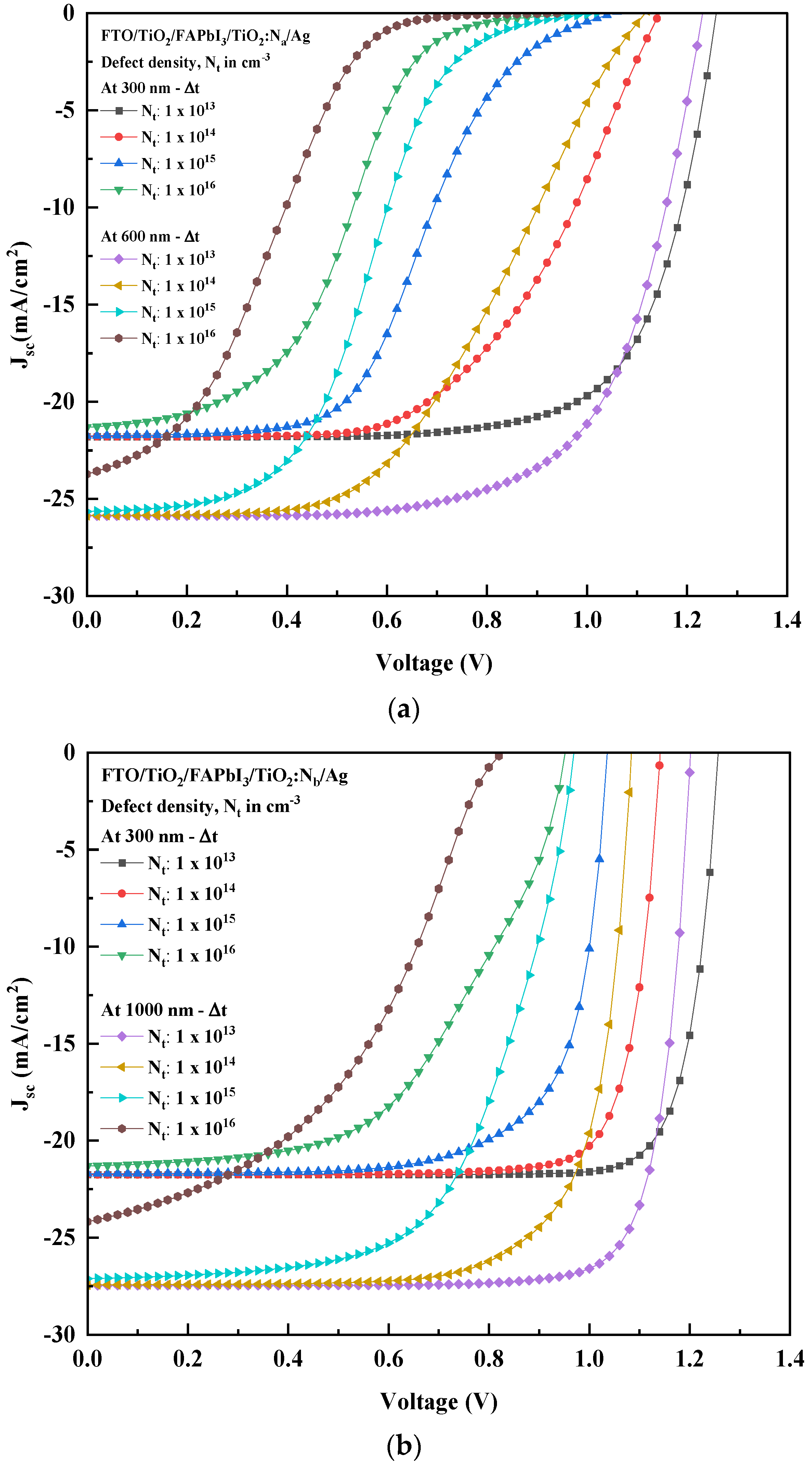
3.2. Effect of Defect Density in the Perovskite Absorber Layer
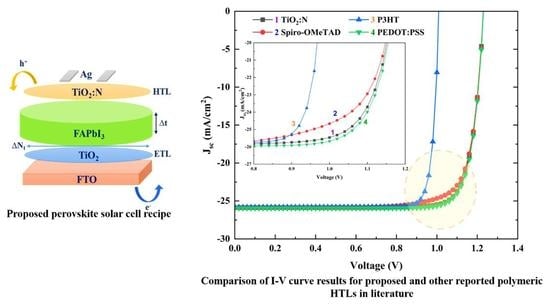
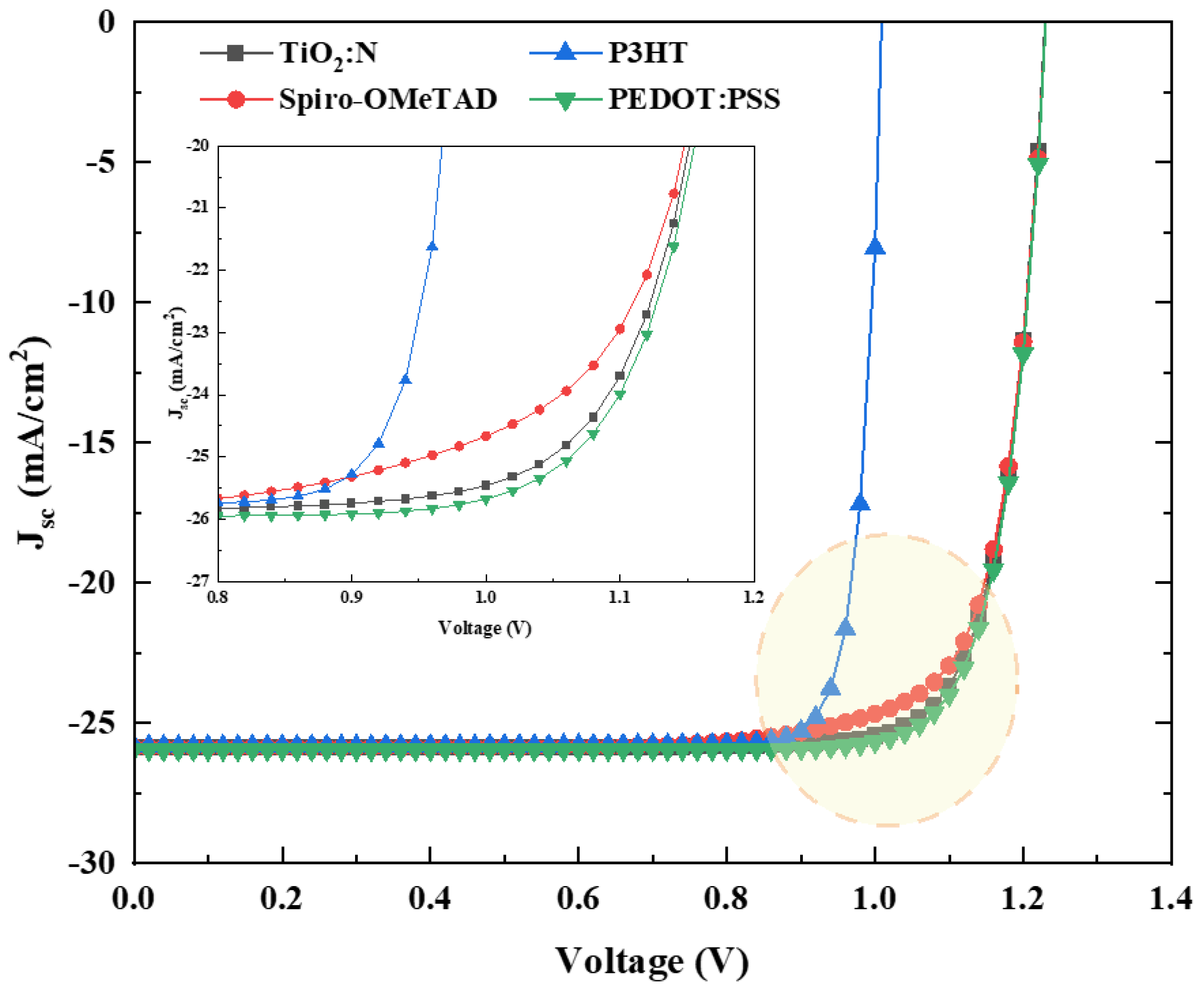
3.3. Comparison of Different Polymeric HTLs Used in an FA-Based PSC Recipe
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xue, L.; Liu, X.; Wang, Q.; Yang, M.; Du, S.; Yang, C.; Tong, J.; Xia, Y.; Li, J. Improved Performance of Organic Solar Cells by Utilizing Green Non-Halogen Additive to Modulate Active-Layer Morphology. Energy Technol. 2022, 10, 2200504. [Google Scholar] [CrossRef]
- Rao, V.T.; Sekhar, Y.R. Comparative analysis on embodied energy and CO2 emissions for stand-alone crystalline silicon photovoltaic thermal (PVT) systems for tropical climatic regions of India. Sustain. Cities Soc. 2022, 78, 103650. [Google Scholar] [CrossRef]
- Yang, D.; Zhang, X.; Hou, Y.; Wang, K.; Ye, T.; Yoon, J.; Wu, C.; Sanghadasa, M.; Liu, S.; Priya, S. 28.3%-efficiency perovskite/silicon tandem solar cell by optimal transparent electrode for high efficient semitransparent top cell. Nano Energy 2021, 84, 105934. [Google Scholar] [CrossRef]
- Rao, V.T.; Sekhar, Y.R. Hybrid Photovoltaic/Thermal (PVT) Collector Systems with Different Absorber Configurations for Thermal Management—A Review. Energy Environ. 2021, 0958305X211065575. [Google Scholar] [CrossRef]
- Ren, Y.; Liu, X.; Li, H.; Qin, J.; Du, S.; Lu, X.; Tong, J.; Yang, C.; Li, J. Utilizing non-conjugated small-molecular tetrasodium iminodisuccinateas electron transport layer enabled improving efficiency of organic solar cells. Opt. Mater. 2022, 129, 112520. [Google Scholar] [CrossRef]
- Ehrler, B.; Alarcón-Lladó, E.; Tabernig, S.W.; Veeken, T.; Garnett, E.C.; Polman, A. Photovoltaics Reaching for the Shockley–Queisser Limit. ACS Energy Lett. 2020, 5, 3029–3033. [Google Scholar] [CrossRef]
- Nalianya, M.A.; Awino, C.; Barasa, H.; Odari, V.; Gaitho, F.; Omogo, B.; Mageto, M. Numerical study of lead free CsSn0.5Ge0.5I3 perovskite solar cell by SCAPS-1D. Optik 2021, 248, 168060. [Google Scholar] [CrossRef]
- Ahmed, S.; Jannat, F.; Khan, A.K.; Alim, M.A. Numerical development of eco-friendly Cs2TiBr6 based perovskite solar cell with all-inorganic charge transport materials via SCAPS-1D. Optik 2020, 225, 165765. [Google Scholar] [CrossRef]
- Liu, G.; Liu, Z.; Wang, L.; Zhang, K.; Xie, X. A combined chrome oxide and titanium oxide based electron-transport layer for high-performance perovskite solar cells. Chem. Phys. Lett. 2021, 771, 138496. [Google Scholar] [CrossRef]
- NREL. Best Research-Cell Efficiency Chart. 2022. Available online: https://www.nrel.gov/pv/cell-efficiency.html (accessed on 5 October 2022).
- Lin, L.; Jiang, L.; Li, P.; Fan, B.; Qiu, Y.; Yan, F. Simulation of optimum band structure of HTM-free perovskite solar cells based on ZnO electron transporting layer. Mater. Sci. Semicond. Process. 2018, 90, 1–6. [Google Scholar] [CrossRef]
- Chen, C.; Zheng, S.; Song, H. Photon management to reduce energy loss in perovskite solar cells. Chem. Soc. Rev. 2021, 50, 7250–7329. [Google Scholar] [CrossRef] [PubMed]
- Chiara, R.; Morana, M.; Malavasi, L. Germanium-Based Halide Perovskites: Materials, Properties, and Applications. ChemPlusChem 2021, 86, 879–888. [Google Scholar] [CrossRef] [PubMed]
- Correa-Baena, J.-P.; Nienhaus, L.; Kurchin, R.C.; Shin, S.S.; Wieghold, S.; Hartono, N.T.P.; Layurova, M.; Klein, N.D.; Poindexter, J.R.; Polizzotti, A.; et al. A-Site Cation in Inorganic A3Sb2I9 Perovskite Influences Structural Dimensionality, Exciton Binding Energy, and Solar Cell Performance. Chem. Mater. 2018, 30, 3734–3742. [Google Scholar] [CrossRef]
- Tai, Q.; Tang, K.-C.; Yan, F. Recent progress of inorganic perovskite solar cells. Energy Environ. Sci. 2019, 12, 2375–2405. [Google Scholar] [CrossRef]
- Nam, J.K.; Chai, S.U.; Cha, W.; Choi, Y.J.; Kim, W.; Jung, M.S.; Kwon, J.; Kim, D.; Park, J.H. Potassium Incorporation for Enhanced Performance and Stability of Fully Inorganic Cesium Lead Halide Perovskite Solar Cells. Nano Lett. 2017, 17, 2028–2033. [Google Scholar] [CrossRef] [PubMed]
- Gkini, K.E.; Antoniadou, M.; Balis, N.; Kaltzoglou, A.; Kontos, A.G.; Falaras, P. Mixing cations and halide anions in perovskite solar cells. Mater. Today Proc. 2019, 19, 73–78. [Google Scholar] [CrossRef]
- Shao, S.; Loi, M.A. The Role of the Interfaces in Perovskite Solar Cells. Adv. Mater. Interfaces 2019, 7, 1901469. [Google Scholar] [CrossRef]
- Zhou, D.; Zhou, T.; Tian, Y.; Zhu, X.; Tu, Y. Perovskite-Based Solar Cells: Materials, Methods, and Future Perspectives. J. Nanomater. 2018, 2018, 8148072. [Google Scholar] [CrossRef]
- Nhari, L.M.; El-Shishtawy, R.M.; Asiri, A.M. Recent progress in organic hole transport materials for energy applications. Dye. Pigment. 2021, 193, 109465. [Google Scholar] [CrossRef]
- Wang, Q.; Lin, Z.; Su, J.; Hu, Z.; Chang, J.; Hao, Y. Recent progress of inorganic hole transport materials for efficient and stable perovskite solar cells. Nano Sel. 2021, 2, 1055–1080. [Google Scholar] [CrossRef]
- Li, S.; Cao, Y.-L.; Li, W.-H.; Bo, Z.-S. A brief review of hole transporting materials commonly used in perovskite solar cells. Rare Met. 2021, 40, 2712–2729. [Google Scholar] [CrossRef]
- Kim, J.; Kim, K.S.; Myung, C.W. Efficient electron extraction of SnO2 electron transport layer for lead halide perovskite solar cell. NPJ Comput. Mater. 2020, 6, 100. [Google Scholar] [CrossRef]
- Bendib, T.; Bencherif, H.; Abdi, M.; Meddour, F.; Dehimi, L.; Chahdi, M. Combined optical-electrical modeling of perovskite solar cell with an optimized design. Opt. Mater. 2020, 109, 110259. [Google Scholar] [CrossRef]
- Hima, A.; Lakhdar, N. Enhancement of efficiency and stability of CH3NH3GeI3 solar cells with CuSbS2. Opt. Mater. 2019, 99, 109607. [Google Scholar] [CrossRef]
- Bhavsar, K.; Lapsiwala, P. Numerical simulation of perovskite solar cell with different material as electron transport layer using SCAPS-1D Software. Semicond. Phys. Quantum Electron. Optoelectron. 2021, 24, 341–347. [Google Scholar] [CrossRef]
- Kim, T.; Lim, J.; Song, S. Recent Progress and Challenges of Electron Transport Layers in Organic–Inorganic Perovskite Solar Cells. Energies 2020, 13, 5572. [Google Scholar] [CrossRef]
- Assi, A.A.; Saleh, W.R.; Mohajerani, E. Investigate of TiO2 and SnO2 as electron transport layer for perovskite solar cells. AIP Conf. Proc. 2020, 2290, 050039. [Google Scholar] [CrossRef]
- Asahi, R.; Morikawa, T.; Irie, H.; Ohwaki, T. Nitrogen-Doped Titanium Dioxide as Visible-Light-Sensitive Photocatalyst: Designs, Developments, and Prospects. Chem. Rev. 2014, 114, 9824–9852. [Google Scholar] [CrossRef]
- Zafar, M.; Yun, J.-Y.; Kim, D.-H. Performance of inverted organic photovoltaic cells with nitrogen doped TiO2 films by atomic layer deposition. Korean J. Chem. Eng. 2018, 35, 567–573. [Google Scholar] [CrossRef]
- Mahmood, K.; Sarwar, S.; Mehran, M.T. Current status of electron transport layers in perovskite solar cells: Materials and properties. RSC Adv. 2017, 7, 17044–17062. [Google Scholar] [CrossRef]
- Ansari, S.A.; Khan, M.M.; Ansari, M.O.; Cho, M.H. Nitrogen-doped titanium dioxide (N-doped TiO2) for visible light photocatalysis. New J. Chem. 2016, 40, 3000–3009. [Google Scholar] [CrossRef]
- Kim, T.H.; Go, G.-M.; Cho, H.-B.; Song, Y.; Lee, C.-G.; Choa, Y.-H. A novel synthetic method for N doped TiO2 nanoparticles through plasma-assisted electrolysis and photocatalytic activity in the visible region. Front. Chem. 2018, 6, 458. [Google Scholar] [CrossRef] [PubMed]
- Panepinto, A.; Dervaux, J.; Cormier, P.A.; Boujtita, M.; Odobel, F.; Snyders, R. Synthesis of p-type N-doped TiO2 thin films by co-reactive magnetron sputtering. Plasma Process. Polym. 2020, 17, 1900203. [Google Scholar] [CrossRef]
- Santos, I.M.D.L.; Cortina-Marrero, H.J.; Ruíz-Sánchez, M.; Hechavarría-Difur, L.; Sánchez-Rodríguez, F.; Courel, M.; Hu, H. Optimization of CH3NH3PbI3 perovskite solar cells: A theoretical and experimental study. Sol. Energy 2020, 199, 198–205. [Google Scholar] [CrossRef]
- Mamta; Maurya, K.; Singh, V. Sb2Se3 versus Sb2S3 solar cell: A numerical simulation. Sol. Energy 2021, 228, 540–549. [Google Scholar] [CrossRef]
- Sebastian, V.; Kurian, J. Simulation and optimization studies on CsPbI3 based inorganic perovskite solar cells. Sol. Energy 2021, 221, 99–108. [Google Scholar] [CrossRef]
- Vasu, K.; Sreedhara, M.B.; Ghatak, J.; Rao, C.N.R. Atomic Layer Deposition of p-Type Epitaxial Thin Films of Undoped and N-Doped Anatase TiO2. ACS Appl. Mater. Interfaces 2016, 8, 7897–7901. [Google Scholar] [CrossRef]
- Lee, M.D.; Lee, G.J.; Nam, I.; Abbas, M.A.; Bang, J.H. Exploring the Effect of Cation Vacancies in TiO2: Lithiation Behavior of n-Type and p-Type TiO2. ACS Appl. Mater. Interfaces 2022, 14, 6560–6569. [Google Scholar] [CrossRef]
- Vasilopoulou, M.; Kelaidis, N.; Polydorou, E.; Soultati, A.; Davazoglou, D.; Argitis, P.; Papadimitropoulos, G.; Tsikritzis, D.; Kennou, S.; Auras, F.; et al. Hydrogen and nitrogen codoping of anatase TiO2 for efficiency enhancement in organic solar cells. Sci. Rep. 2017, 7, 17839. [Google Scholar] [CrossRef]
- Anitha, V.C.; Banerjee, A.N.; Joo, S.W. Recent developments in TiO2 as n- and p-type transparent semiconductors: Synthesis, modification, properties, and energy-related applications. J. Mater. Sci. 2015, 50, 7495–7536. [Google Scholar] [CrossRef]
- Bakar, S.A.; Ribeiro, C. Nitrogen-doped titanium dioxide: An overview of material design and dimensionality effect over modern applications. J. Photochem. Photobiol. C Photochem. Rev. 2016, 27, 1–29. [Google Scholar] [CrossRef]
- Fu, C.; Gu, Z.; Tang, Y.; Xiao, Q.; Zhang, S.; Zhang, Y.; Song, Y. From Structural Design to Functional Construction: Amine Molecules in High-Performance Formamidinium-Based Perovskite Solar Cells. Angew. Chem. 2022, 134, e202117067. [Google Scholar] [CrossRef]
- Zheng, Z.; Wang, S.; Hu, Y.; Rong, Y.; Mei, A.; Han, H. Development of formamidinium lead iodide-based perovskite solar cells: Efficiency and stability. Chem. Sci. 2021, 13, 2167–2183. [Google Scholar] [CrossRef] [PubMed]
- Dong, X.; Chao, L.; Niu, T.; Li, Y.; Guo, P.; Hui, W.; Song, L.; Wu, Z.; Chen, Y. Phase-Pure Engineering for Efficient and Stable Formamidinium-Based Perovskite Solar Cells. Sol. RRL 2022, 6, 2200060. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, X.; Wang, C.; Cheng, R.; Zhao, L.; Wang, X.; Zhang, X.; Shang, J.; Zhang, H.; Zhao, L.; et al. Defect suppression and energy level alignment in formamidinium-based perovskite solar cells. J. Energy Chem. 2021, 67, 65–72. [Google Scholar] [CrossRef]
- Kundu, S.; Zhang, D.; Askar, A.M.; Moloney, E.G.; Adachi, M.M.; Nadeem, A.; Moradi, S.; Yeddu, V.; Abdelhady, A.L.; Voznyy, O.; et al. Bismuth Stabilizes the α-Phase of Formamidinium Lead Iodide Perovskite Single Crystals. ACS Mater. Lett. 2022, 4, 707–712. [Google Scholar] [CrossRef]
- Bu, T.; Ono, L.K.; Li, J.; Su, J.; Tong, G.; Zhang, W.; Liu, Y.; Zhang, J.; Chang, J.; Kazaoui, S.; et al. Modulating crystal growth of formamidinium–caesium perovskites for over 200 cm2 photovoltaic sub-modules. Nat. Energy 2022, 7, 528–536. [Google Scholar] [CrossRef]
- Liu, S.; Chen, R.; Tian, X.; Yang, Z.; Zhou, J.; Ren, F.; Zhang, S.; Zhang, Y.; Guo, M.; Shen, Y.; et al. Boost the efficiency of nickel oxide-based formamidinium-cesium perovskite solar cells to 21% by using coumarin 343 dye as defect passivator. Nano Energy 2022, 94, 106935. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Z.; Yu, B.; Tan, S.; Cui, Y.; Wu, H.; Luo, Y.; Shi, J.; Li, D.; Meng, Q. Efficient, stable formamidinium-cesium perovskite solar cells and minimodules enabled by crystallization regulation. Joule 2022, 6, 676–689. [Google Scholar] [CrossRef]
- Vishnuwaran, M.; Ramachandran, K.; Anand, D.; Ragavendran, V. Using low-cost materials for highly efficient eco-friendly formamidinium tin iodide based solar cell with copper oxide as hole transport material and titanium oxide as electron transport material with different metal contacts. Ceram. Int. 2022, 48, 29314–29321. [Google Scholar] [CrossRef]
- Niloy, A.B.; Al Razy, M.; Ahmed, S.; Jannat, F.; Alim, M.A. Performance investigation of cesium formamidinium lead mixed halide (FA0.83Cs0.17PbI3−xBrx) for different iodine and bromine ratios. Micro Nanostructures 2022, 168, 207305. [Google Scholar] [CrossRef]
- Sabbah, H.; Arayro, J.; Mezher, R. Numerical Simulation and Optimization of Highly Stable and Efficient Lead-Free Perovskite FA1−xCsxSnI3−x Based Solar Cells Using SCAPS. Materials 2022, 15, 4761. [Google Scholar] [CrossRef] [PubMed]
- Vishnuwaran, M.; Ramachandran, K. Novel Approach on Formamidinium Tin Iodide-Based Provskite Solar Cell for the Best Replacement Materials of Hole Transport Layer and Electron Transport Layer by Using Solar Cell Capacitance Simulation; Research Square: Durham, NC, USA, 2022. [Google Scholar] [CrossRef]
- Teimouri, R.; Mehrvarz, S.; Ebrahimi, A.; Kolahdouz, M.; Darab, M. Performance of planar perovskite solar cells based on formamidinium cations: Simulation and fabrication. Int. J. Energy Res. 2022. [Google Scholar] [CrossRef]
- Jannat, F.; Ahmed, S.; Alim, M.A. Performance analysis of cesium formamidinium lead mixed halide based perovskite solar cell with MoOx as hole transport material via SCAPS-1D. Optik 2020, 228, 166202. [Google Scholar] [CrossRef]
- Stanić, D.; Kojić, V.; Čižmar, T.; Juraić, K.; Bagladi, L.; Mangalam, J.; Rath, T.; Gajović, A. Simulating the Performance of a Formamidinium Based Mixed Cation Lead Halide Perovskite Solar Cell. Materials 2021, 14, 6341. [Google Scholar] [CrossRef]
- Alipour, H.; Ghadimi, A. Optimization of lead-free perovskite solar cells in normal-structure with WO3 and water-free PEDOT: PSS composite for hole transport layer by SCAPS-1D simulation. Opt. Mater. 2021, 120, 111432. [Google Scholar] [CrossRef]
- Tara, A.; Bharti, V.; Sharma, S.; Gupta, R. Device simulation of FASnI3 based perovskite solar cell with Zn (O0.3, S0.7) as electron transport layer using SCAPS-1D. Opt. Mater. 2021, 119, 111362. [Google Scholar] [CrossRef]
- Patil, S.V.; Dave, S.; Bhargava, K. Comparative Analysis of MAPbI3 and FAPbI3 based Perovskite Solar Cells: A Numerical Evaluation. In Proceedings of the 28th National Conference on Condensed Matter Physics, NIT Silchar, India, 11–13 December 2021; Springer: Singapore, 2021; pp. 177–185. [Google Scholar] [CrossRef]
- Kanoun, M.B.; Kanoun, A.A.; Merad, A.E.; Goumri-Said, S. Device design optimization with interface engineering for highly efficient mixed cations and halides perovskite solar cells. Results Phys. 2021, 20, 103707. [Google Scholar] [CrossRef]
- Bhardwaj, K.S.; Rai, S.; Sadanand; Lohia, P.; Dwivedi, D.K. Investigating the performance of mixed cation mixed halide-based perovskite solar cells using various hole-transport materials by numerical simulation. Opt. Quantum Electron. 2021, 53, 602. [Google Scholar] [CrossRef]
- Kumar, M.; Raj, A.; Kumar, A.; Anshul, A. An optimized lead-free formamidinium Sn-based perovskite solar cell design for high power conversion efficiency by SCAPS simulation. Opt. Mater. 2020, 108, 110213. [Google Scholar] [CrossRef]
- Karthick, S.; Velumani, S.; Bouclé, J. Experimental and SCAPS simulated formamidinium perovskite solar cells: A comparison of device performance. Sol. Energy 2020, 205, 349–357. [Google Scholar] [CrossRef]
- Hussain, S.S.; Riaz, S.; Nowsherwan, G.A.; Jahangir, K.; Raza, A.; Iqbal, M.J.; Sadiq, I.; Naseem, S. Numerical Modeling and Optimization of Lead-Free Hybrid Double Perovskite Solar Cell by Using SCAPS-1D. J. Renew. Energy 2021, 2021, 6668687. [Google Scholar] [CrossRef]
- Madan, J.; Singh, K.; Pandey, R. Comprehensive device simulation of 23.36% efficient two-terminal perovskite-PbS CQD tandem solar cell for low-cost applications. Sci. Rep. 2021, 11, 19829. [Google Scholar] [CrossRef]
- Kim, G.; Choi, H.; Kim, M.; Lee, J.; Son, S.Y.; Park, T. Hole Transport Materials in Conventional Structural (n–i–p) Perovskite Solar Cells: From Past to the Future. Adv. Energy Mater. 2020, 10, 1903403. [Google Scholar] [CrossRef]
- Rono, N.; Merad, A.E.; Kibet, J.K.; Martincigh, B.S.; Nyamori, V.O. Optimization of Hole Transport Layer Materials for a Lead-Free Perovskite Solar Cell Based on Formamidinium Tin Iodide. Energy Technol. 2021, 9, 2100859. [Google Scholar] [CrossRef]

| S. No. | Year | Author [Reference] | Recipe | Remarks |
|---|---|---|---|---|
| 1 | 2022 | Wang et al. [46] | SnO2/FAPSC/Spiro-OMeTAD | 4-fluorophenylmethylammonium iodide (F-PMAI) was used to modulate surface structure and energy level alignment. |
| 2 | 2022 | Kundu et al. [47] | FAPbI3 crystals | The α-FAPbI3 single crystals are stabilized through Pb-site doping with a heterovalent metal–bismuth (Bi). The optimum concentration of Bi extends the phase change by four orders of magnitude. |
| 3 | 2022 | Bu et al. [48] | (FA-Cs) lead halide | Controlled the formation of intermediate phases during the growth of formamidinium–caesium lead triiodide perovskite films by using methylammonium chloride additives in the co-solvent system of N-methyl-2-pyrrolidone/N, N-dimethylformamide. |
| 4 | 2022 | Liu et al. [49] | FA-Cs + NiOx (HTL) | A molecular additive—the organic dye coumarin 343—was used to reduce Voc loss and improve efficiency. |
| 5 | 2022 | Li et al. [50] | (FA-Cs) lead halide | Additives reduced crystallization and defects of the FA-Cs perovskite film. |
| S. No. | Year | Author | Perovskite Solar Cell Structure (ETL/Absorber/HTL) | Performance Factors (Voc (V), Jsc (mA/cm2), FF (%), PCE (%) | Remarks | Reference |
|---|---|---|---|---|---|---|
| 1 | 2022 | Vishnuwaran et al. | TiO2/FASnI3/CuO2 | Voc: 0.7921, Jsc: 29.61, FF: 78.14, PCE: 18.10 | Varied absorber layer thickness—350 nm yielded the best results | [51] |
| 2 | 2022 | Niloy et al. | SnO2/FA0.83Cs0.17PbI0.5Br2.5/MoOx | PCE up to 22.89% for absorber thickness of 169 nm | Absorber layer thickness influences the PCE | [52] |
| 3 | 2022 | Sabbah et al. | (TiO2, ZnOS)/FA1−xCsxSnI3/CuO2 | Voc: 0.89, Jsc: 31.4, FF: 78.7, PCE: 22 | ZnOS exhibited stable behaviour and was better than TiO2 | [53] |
| 4 | 2022 | Vishnuwaran | ZnOS/FASnI3/CuI | Voc: 6.20, Jsc: 30.77, FF: 12.68, PCE: 24.22 | CuI and ZnOS are considered ideal replacements for Spiro-OMeTAD and TiO2 | [54] |
| 5 | 2022 | Teimouri et al. | Cs0.05 (FAxMA[1−x])0.95Pb(I0.83Br0.17)3 | Attained a PCE of up to 20.98% | Analyzed bandgap ratios between 2.175 eV to 1.5 eV and x factor influenced the power output | [55] |
| 6 | 2021 | Jannat et al. | SnO2/FA0.83Cs0.17 PbI1.5Br1.5/MoOx | Voc: 1.44, Jsc: 17.04, FF: 81.83, PCE: 20.10 | MoOx exhibited a low valence band offset. HTL and absorber thickness was varied | [56] |
| 7 | 2021 | Stanić et al. | TiO2/Rb0.05Cs0.1FA0.85PbI3/Spiro-OMeTAD | Voc: 0.80, Jsc: 20.60, FF: 45.51, PCE: 7.35 | Absorber layer thickness, defect density concentration, and the influence of the resistivity were analyzed | [57] |
| 8 | 2021 | Alipour and Ghadimi | PC61BM/FASnI3/PEDOT: PSS+WO3 | Voc: 1.12, Jsc: 24.65, FF: 86.02, PCE: 23.69 | FA depicted better outputs that MA based structures | [58] |
| 9 | 2021 | Tara et al. | Zn (O0.3, S0.7)/FASnI3/CuSCN | Voc: 1.08, Jsc: 28.12, FF: 84.96, PCE: 25.94 | Variations in electron affinity, CBO, doping density, and thickness of Zn (O0.3, S0.7) were analyzed | [59] |
| 10 | 2021 | Patil et al. | ZnO/FAPbI3/Spiro-OMeTAD | Voc: 0.99, Jsc: 26.75, FF: 79.80, PCE: 21.26 | FA-based PSC structures have depicted higher efficiency than MA | [60] |
| 11 | 2021 | Kanoun et al. | TiO2/FAPbI3/PTAA and Cu2O | PCE of up to 24% for absorber thickness of 400 nm | PTAA and Cu2O as HTLs enhance change carriers | [61] |
| 12 | 2021 | Bhardwaj et al. | SnO2/FA0.85Cs0.15Pb(I0.85Br0.15)3/Spiro-OMeTAD and Cu2O | Spiro-OMeTAD (HTL)—PCE: 15.36% Cuprous oxide (HTL)—PCE: 19.38% | CuO2 delivers the highest efficiency when compared to other inorganic HTLs | [62] |
| PSC Recipe | Jsc (mA/cm−2) | FF (%) | Voc (V) | PCE (%) |
|---|---|---|---|---|
| FTO/TiO2/FAPbI3/TiO2:Na/Ag | 21.798 | 71.83 | 1.25 | 19.71 |
| FTO/TiO2/FAPbI3/TiO2:Nb/Ag | 21.760 | 83.44 | 1.25 | 22.83 |
| Recipe | ∆t (nm) | Jsc (mA/cm−2) | FF (%) | Voc (V) | PCE (%) |
|---|---|---|---|---|---|
| FTO/TiO2/FAPbI3/ TiO2:Na/Ag | 300 | 21.79 | 71.83 | 1.25 | 19.71 |
| 400 | 23.81 | 70.01 | 1.24 | 20.80 | |
| 500 | 25.06 | 68.46 | 1.23 | 21.26 | |
| 600 # | 25.87 | 67.10 | 1.23 | 21.38 | |
| 700 | 26.41 | 65.88 | 1.22 | 21.31 | |
| 800 | 26.81 | 64.76 | 1.21 | 21.15 | |
| 900 | 27.09 | 63.74 | 1.21 | 20.94 | |
| 1000 | 27.30 | 62.79 | 1.20 | 20.70 | |
| FTO/TiO2/FAPbI3/ TiO2:Nb/Ag | 300 | 21.76 | 83.44 | 1.25 | 22.83 |
| 400 | 23.79 | 83.17 | 1.24 | 24.68 | |
| 500 | 25.05 | 82.95 | 1.23 | 25.73 | |
| 600 | 25.87 | 82.75 | 1.23 | 26.34 | |
| 700 | 26.42 | 82.51 | 1.22 | 26.68 | |
| 800 | 26.81 | 82.33 | 1.21 | 26.87 | |
| 900 | 27.09 | 82.16 | 1.21 | 26.97 | |
| 1000 # | 27.29 | 81.94 | 1.20 | 26.99 | |
| 1100 | 27.45 | 81.75 | 1.20 | 26.98 | |
| 1200 | 27.57 | 81.58 | 1.19 | 26.95 | |
| 1300 | 27.67 | 81.41 | 1.19 | 26.90 | |
| 1400 | 27.75 | 81.24 | 1.19 | 26.83 |
| Recipe | Absorber Layer Thickness (∆t) | Defect Density (Nt) | Jsc (mA/cm−2) | FF (%) | Voc (V) | PCE (%) |
|---|---|---|---|---|---|---|
| FTO/TiO2/FAPbI3/ TiO2:Na/Ag | 300 nm | 1 × 1013 | 21.79 | 71.83 | 1.25 | 19.71 |
| 1 × 1014 | 21.79 | 55.15 | 1.14 | 13.92 | ||
| 1 × 1015 | 21.75 | 45.68 | 1.05 | 10.44 | ||
| 1 × 1016 | 21.31 | 33.12 | 0.99 | 7.06 | ||
| 600 nm # | 1 × 1013 | 25.87 | 67.10 | 1.23 | 21.38 | |
| 1 × 1014 | 25.85 | 48.79 | 1.11 | 14.08 | ||
| 1 × 1015 | 26.62 | 37.12 | 1.01 | 9.62 | ||
| 1 × 1016 | 23.72 | 22.17 | 0.93 | 4.94 | ||
| FTO/TiO2/FAPbI3/ TiO2:Nb/Ag | 300 nm | 1 × 1013 | 21.76 | 83.44 | 1.25 | 22.83 |
| 1 × 1014 | 21.75 | 81.76 | 1.14 | 20.31 | ||
| 1 × 1015 | 21.71 | 72.57 | 1.03 | 16.33 | ||
| 1 × 1016 | 21.31 | 54.16 | 0.95 | 11.00 | ||
| 1000 nm # | 1 × 1013 | 27.29 | 81.94 | 1.20 | 26.99 | |
| 1 × 1014 | 27.27 | 74.82 | 1.08 | 22.22 | ||
| 1 × 1015 | 26.99 | 63.02 | 0.97 | 16.59 | ||
| 1 × 1016 | 24.45 | 44.63 | 0.84 | 9.17 |
| Parameters | Substrate | ETL | Perovskite Absorber Layer | Novel HTL | Polymeric HTLs | ||
|---|---|---|---|---|---|---|---|
| FTO | TiO2 | FAPbI3 | TiO2:N | Spiro-OMeTAD [62] | P3HT [68] | PEDOT: PSS [58] | |
| Thickness ‘t’ (nm) | 400 | 50 | 600 | 100 | 200 | 50 | 200 |
| Band gap ‘Eg’ (eV) | 3.5 | 3.2 | 1.51 | 3.0 | 2.88 | 1.1 | 1.8 |
| Electron affinity ‘χ’ (eV) | 4 | 4 | 4 | 2.2 | 2.05 | 4.6 | 3.4 |
| Dielectric Permittivity ‘εr’ | 9 | 9 | 6.6 | 3 | 3 | 13.6 | 18 |
| CB EDOS ‘Nc’ (cm−3) | 2.2 × 1018 | 2.1 × 1018 | 1.2 × 1019 | 1.3 × 1014 | 2.2 × 1018 | 3 × 1018 | 2.2 × 1018 |
| VB EDOS ‘Nv’ (cm−3) | 2.2 × 1018 | 2.2 × 1017 | 1.2 × 1019 | 1.3 × 1015 | 1.8 × 1019 | 2 × 1019 | 1.8 × 1019 |
| e− thermal velocity (cm·s−1) | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 |
| h+ thermal velocity (cm·s−1) | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 | 1 × 107 |
| Electron mobility ‘μn’ (cm2/V·s) | 20 | 20 | 2.7 | 2 | 2 × 10−4 | 25 | 4.5 × 10−2 |
| Hole mobility ‘μh’ (cm2/V·s) | 10 | 10 | 1.8 | 2 | 2 × 10−4 | 25 | 4.5 × 10−2 |
| Shallow donor density ‘N.D.’ (cm−3) | 2 × 1019 | 9 × 1016 | 1.3 × 1016 | 0 | 0 | 0 | 0 |
| Shallow Acceptor density ‘N.A.’ (cm−3) | 0 | 0 | 1.3 × 1016 | 1.3 × 1014 | 2 × 1019 | 3 × 1016 | 1 × 1020 |
| Defect density ‘Nt’ (cm−3) | 1 × 1015 | 1 × 1015 | 1 × 1013 | 1 × 1015 | 1 × 1015 | 1 × 1013 | 1 × 1015 |
| PSC Recipe | Jsc (mA/cm−2) | FF (%) | Voc (V) | PCE (%) |
|---|---|---|---|---|
| FTO/TiO2/FAPbI3/TiO2:N/Ag | 25.87 | 82.75 | 1.23 | 26.34 |
| FTO/TiO2/FAPbI3/Spiro-OMeTAD/Ag | 25.86 | 79.83 | 1.23 | 25.42 |
| FTO/TiO2/FAPbI3/P3HT/Ag | 25.81 | 87.62 | 1.01 | 22.85 |
| FTO/TiO2/FAPbI3/PEDOT: PSS/Ag | 25.95 | 83.30 | 1.23 | 26.61 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pochont, N.R.; Sekhar, Y.R.; Vasu, K.; Jose, R. Nitrogen-Doped Titanium Dioxide as a Hole Transport Layer for High-Efficiency Formamidinium Perovskite Solar Cells. Molecules 2022, 27, 7927. https://doi.org/10.3390/molecules27227927
Pochont NR, Sekhar YR, Vasu K, Jose R. Nitrogen-Doped Titanium Dioxide as a Hole Transport Layer for High-Efficiency Formamidinium Perovskite Solar Cells. Molecules. 2022; 27(22):7927. https://doi.org/10.3390/molecules27227927
Chicago/Turabian StylePochont, Nitin Ralph, Yendaluru Raja Sekhar, Kuraganti Vasu, and Rajan Jose. 2022. "Nitrogen-Doped Titanium Dioxide as a Hole Transport Layer for High-Efficiency Formamidinium Perovskite Solar Cells" Molecules 27, no. 22: 7927. https://doi.org/10.3390/molecules27227927
APA StylePochont, N. R., Sekhar, Y. R., Vasu, K., & Jose, R. (2022). Nitrogen-Doped Titanium Dioxide as a Hole Transport Layer for High-Efficiency Formamidinium Perovskite Solar Cells. Molecules, 27(22), 7927. https://doi.org/10.3390/molecules27227927