Optimization of the Laminate Structure of a Composite Cylinder Based on the Combination of Response Surface Methodology (RSM) and Finite Element Analysis (FEA)
Abstract
:1. Introduction
2. Establishment of FEA Model and Theoretical Verification of Progressive Failure
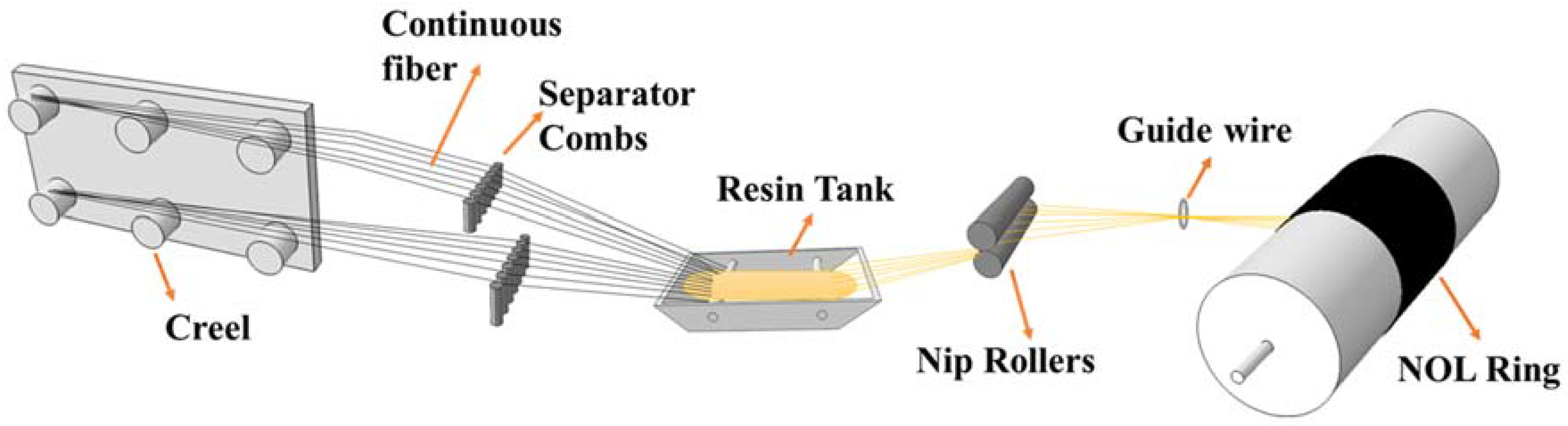
2.1. Establishment of Gas Cylinder FEA Model
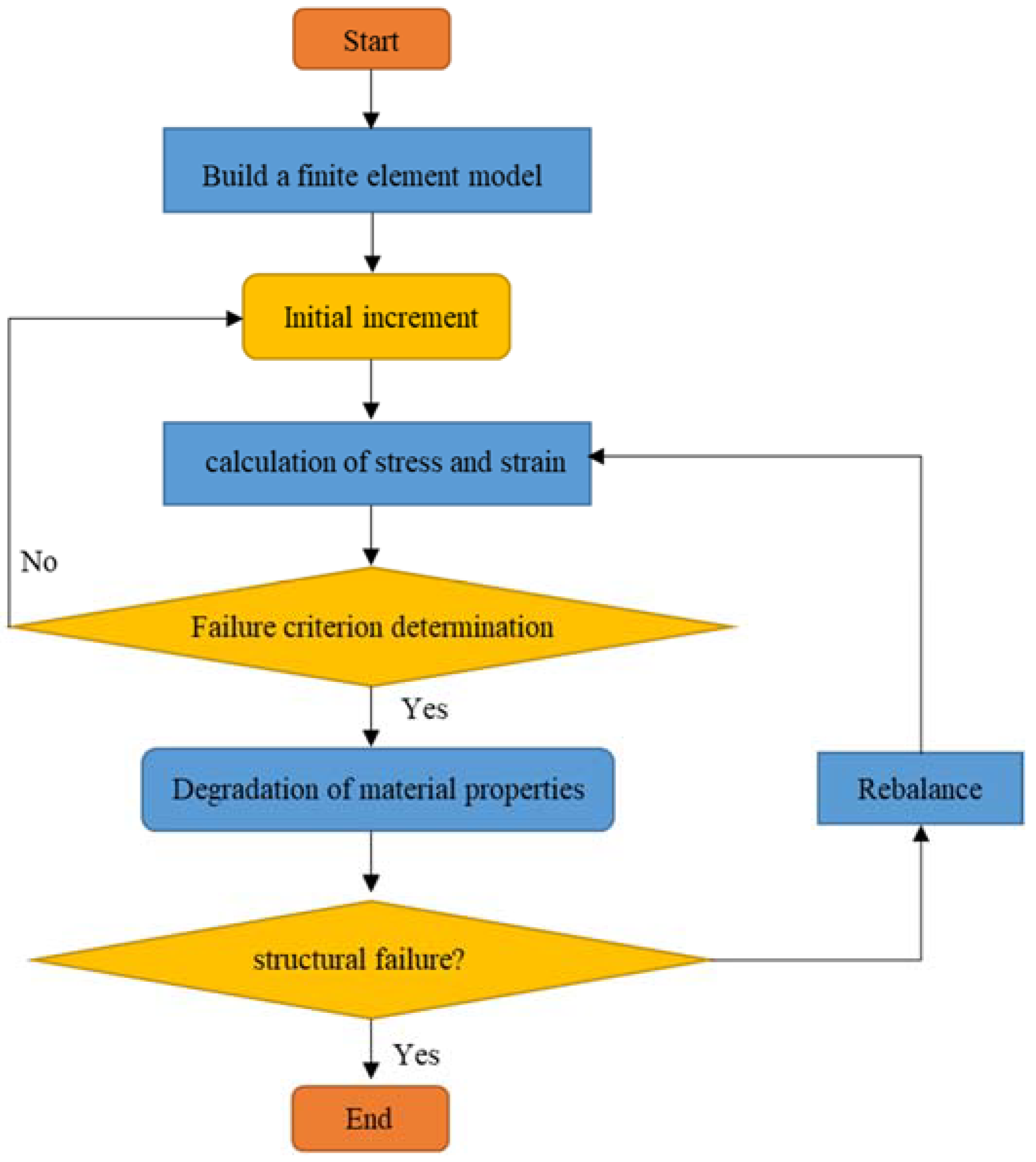
2.2. Progressive Failure Theory
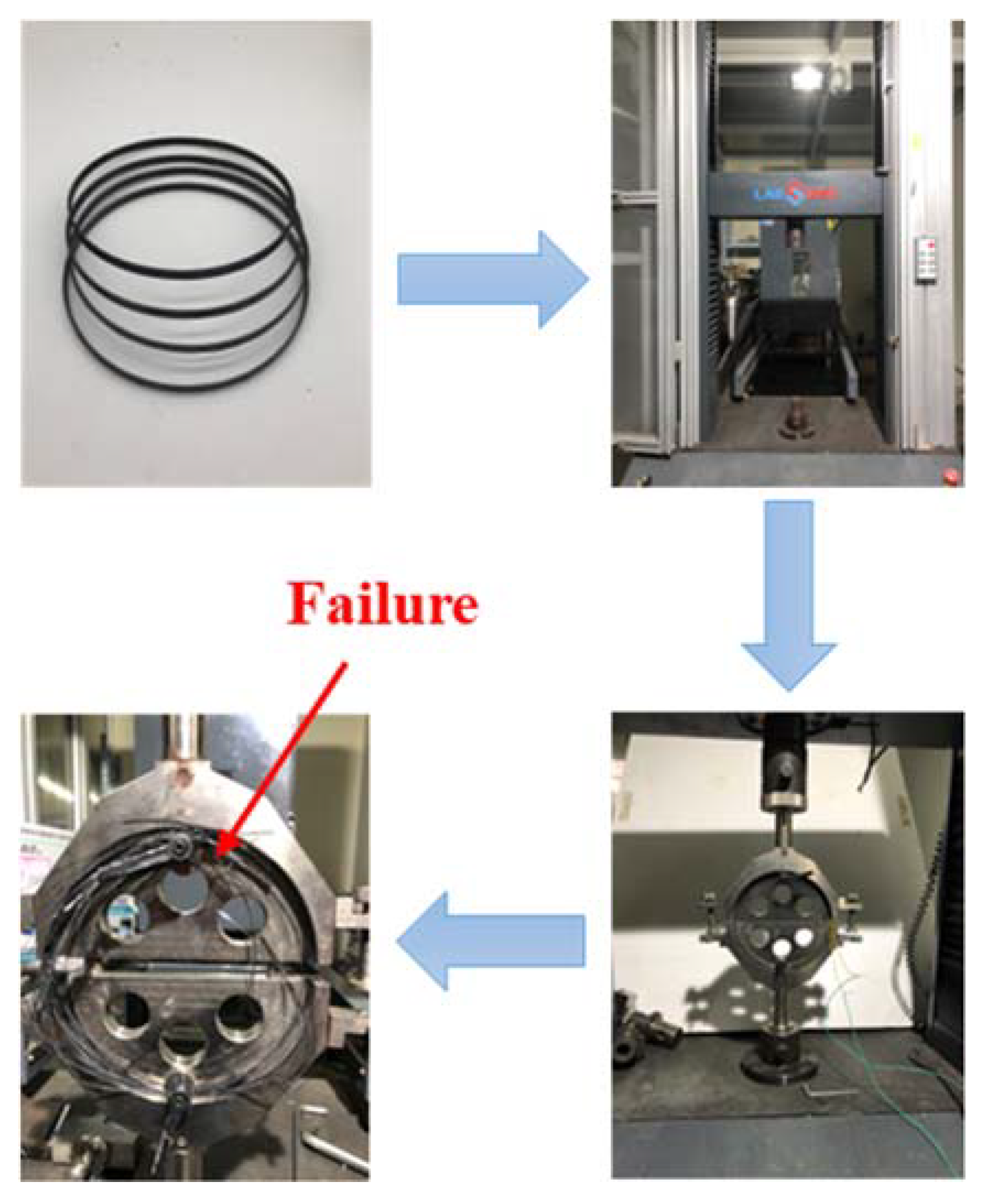
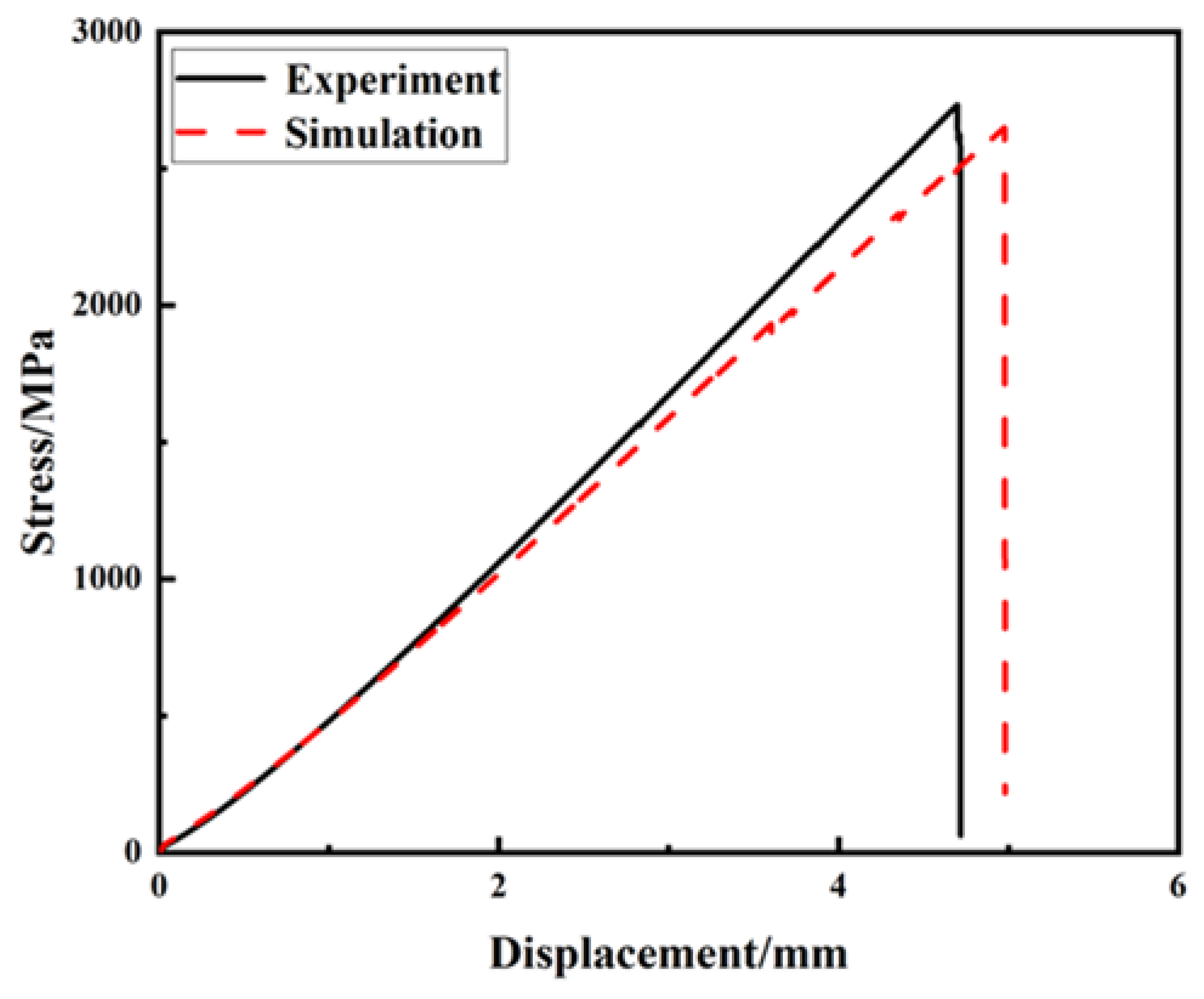
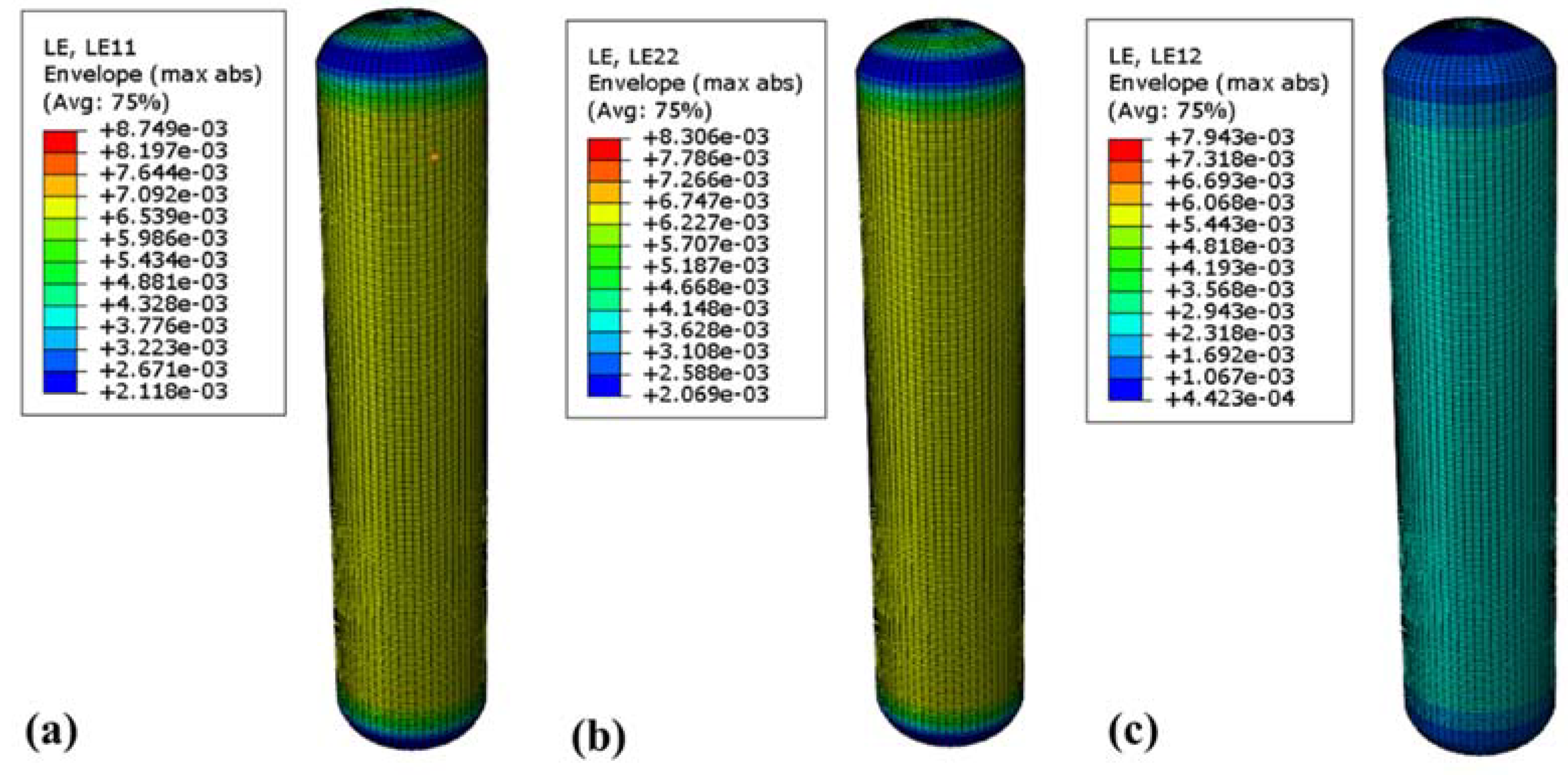
2.3. Experimental Verification of Gradual Failure Theory
3. Response Surface Design Analysis and Finite Element Verification
3.1. Response Surface Design Results
3.2. Model Establishment and Significance Test
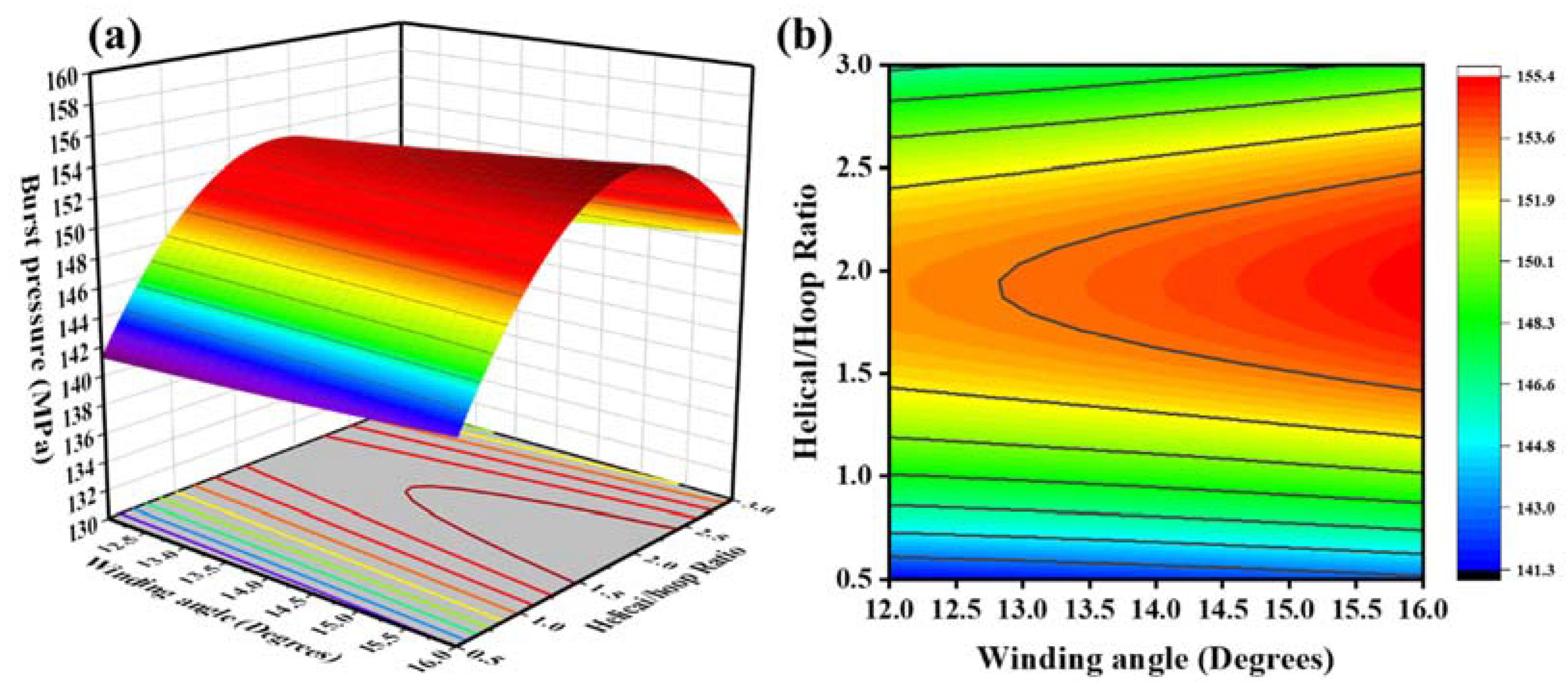
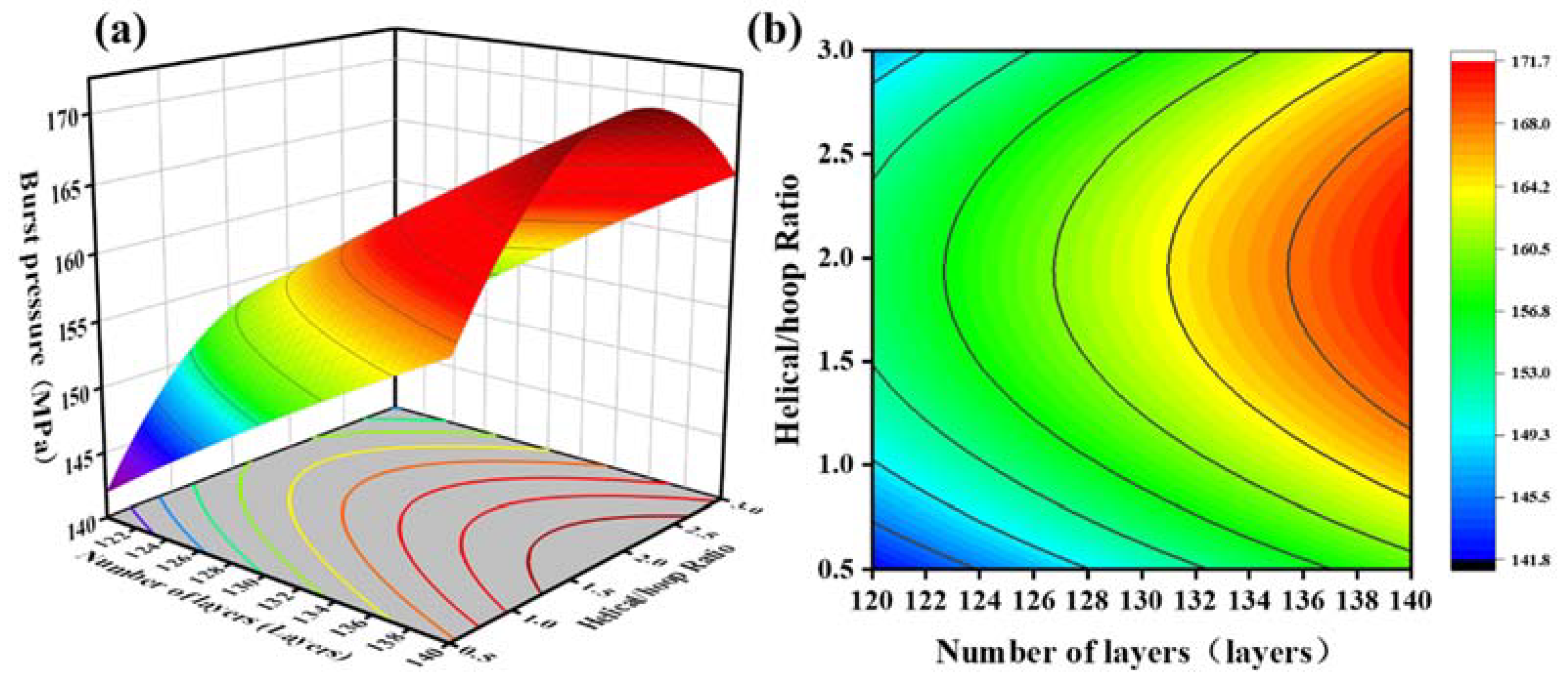
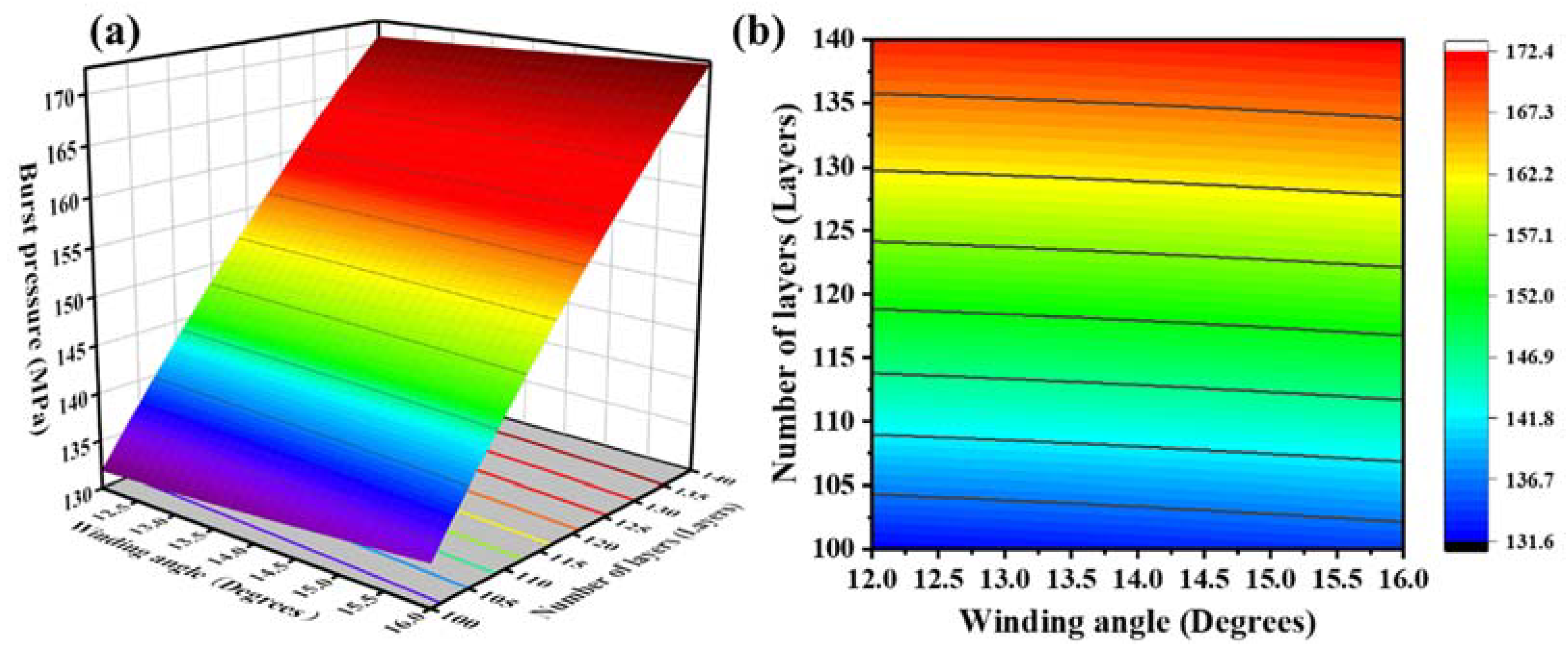
3.3. Response Surface Analysis
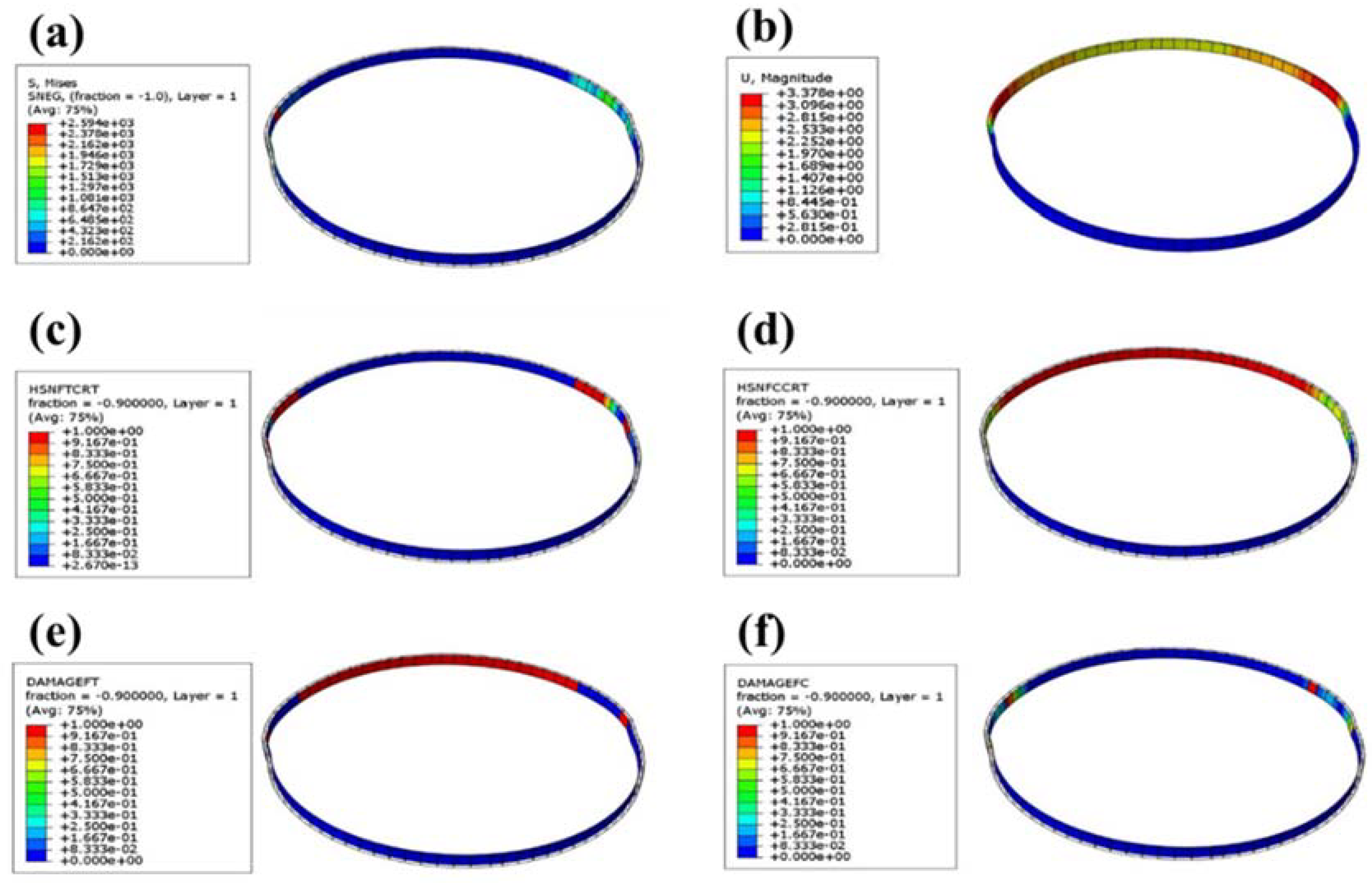
3.4. Finite Element Verification of Gas Cylinders
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Conflicts of Interest
References
- Liu, B.; Xiao, J.; Xu, L.; Yao, Y.; Costa, B.F.; Domingos, V.F.; Ribeiro, E.S.; Shi, F.-N.; Zhou, K.; Su, J.; et al. Gelatin-assisted sol–gel derived TiO2 microspheres for hydrogen storage. Int. J. Hydrogen Energy 2015, 40, 4945–4950. [Google Scholar] [CrossRef]
- Hosseini, S.E.; Wahid, M.A. Hydrogen production from renewable and sustainable energy resources: Promising green energy carrier for clean development. Renew. Sustain. Energy Rev. 2016, 57, 850–866. [Google Scholar] [CrossRef]
- Barthelemy, H.; Weber, M.; Barbier, F. Hydrogen storage: Recent improvements and industrial perspectives. Int. J. Hydrogen Energy 2017, 42, 7254–7262. [Google Scholar] [CrossRef]
- Zhao, G.; Nielsen, E.R.; Troncoso, E.; Hyde, K.; Romeo, J.S.; Diderich, M. Life cycle cost analysis: A case study of hydrogen energy application on the Orkney Islands. Int. J. Hydrogen Energy 2019, 44, 9517–9528. [Google Scholar] [CrossRef]
- Sakintuna, B.; Lamari-Darkrim, F.; Hirscher, M. Metal hydride materials for solid hydrogen storage: A review. Int. J. Hydrogen Energy 2007, 32, 1121–1140. [Google Scholar] [CrossRef]
- Parra, D.; Walker, G.S.; Gillott, M. Modeling of PV generation, battery and hydrogen storage to investigate the benefits of energy storage for single dwelling. Sustain. Cities Soc. 2014, 10, 1–10. [Google Scholar] [CrossRef]
- Ogden, J.; Jaffe, A.M.; Scheitrum, D.; McDonald, Z.; Miller, M. Natural gas as a bridge to hydrogen transportation fuel: Insights from the literature. Energy Policy 2018, 115, 317–329. [Google Scholar] [CrossRef]
- Yamawaki, M.; Nishihara, T.; Inagaki, Y.; Minato, K.; Oigawa, H.; Onuki, K.; Hino, R.; Ogawa, M. Application of nuclear energy for environmentally friendly hydrogen generation. Int. J. Hydrogen Energy 2007, 32, 2719–2725. [Google Scholar] [CrossRef]
- Nikolaidis, P.; Poullikkas, A. A comparative overview of hydrogen production processes. Renew. Sustain. Energy Rev. 2017, 67, 597–611. [Google Scholar] [CrossRef]
- Momirlan, M.; Veziroglu, T. The properties of hydrogen as fuel tomorrow in sustainable energy system for a cleaner planet. Int. J. Hydrogen Energy 2005, 30, 795–802. [Google Scholar] [CrossRef]
- Kaur, M.; Pal, K. Review on hydrogen storage materials and methods from an electrochemical viewpoint. J. Energy Storage 2019, 23, 234–249. [Google Scholar] [CrossRef]
- Parra, D.; Valverde, L.; Pino, F.J.; Patel, M.K. A review on the role, cost and value of hydrogen energy systems for deep decarbonization. Renew. Sustain. Energy Rev. 2019, 101, 279–294. [Google Scholar] [CrossRef]
- Schulz, R.; Huot, J.; Liang, G.X.; Boily, S.; van Neste, A. Structure and Hydrogen Sorption Properties of Ball Milled Mg Dihydride. J. Metastable Nanocryst. Mater. 1999, 2–6, 615–622. [Google Scholar]
- Azeem, M.; Ya, H.H.; Alam, M.A.; Kumar, M.; Stabla, P.; Smolnicki, M.; Gemi, L.; Khan, R.; Ahmed, T.; Ma, Q.; et al. Application of Filament Winding Technology in Composite Pressure Vessels and Challenges: A Review. J. Energy Storage 2022, 49, 103468. [Google Scholar] [CrossRef]
- Nguyen, B.N.; Roh, H.S.; Merkel, D.R.; Simmons, K.L. A predictive modeling tool for damage analysis and design of hydrogen storage composite pressure vessels. Int. J. Hydrogen Energy 2021, 46, 20573–20585. [Google Scholar] [CrossRef]
- Moharrerzadeh, S.; Atai, A. Obtaining an Optimum Weight for a Composite Pressure Vessel. Mater. Sci. Forum 2010, 636–637, 1105–1111. [Google Scholar] [CrossRef]
- Vafaeesefat, A.; Khani, A. Head Shape and Winding Angle Optimization of Composite Pressure Vessels Based on a Multi-level Strategy. Appl. Compos. Mater. 2008, 14, 379–391. [Google Scholar] [CrossRef]
- Statnikov, R.B.; Gavriushin, S.S.; Dang, M.; Statnikov, A. Multicriteria design of composite pressure vessels. Int. J. Multicrit. Decis. Mak. 2014, 4, 252–278. [Google Scholar] [CrossRef]
- Kim, C.-U.; Hong, C.-S.; Kim, C.-G.; Kim, J.-Y. Optimal design of filament wound type 3 tanks under internal pressure using a modified genetic algorithm. Compos. Struct. 2005, 71, 16–25. [Google Scholar] [CrossRef]
- Roh, H.S.; Hua, T.Q.; Ahluwalia, R.K. Optimization of carbon fiber usage in Type 4 hydrogen storage tanks for fuel cell automobiles. Int. J. Hydrogen Energy 2013, 38, 12795–12802. [Google Scholar] [CrossRef]
- Yamashita, A.; Kondo, M.; Goto, S.; Ogami, N. Development of High-Pressure Hydrogen Storage System for the Toyota “Mirai”; SAE Technical Paper Series; SAE: Warrendale, PA, USA, 2015. [Google Scholar]
- Leh, D.; Magneville, B.; Saffré, P.; Francescato, P.; Arrieux, R.; Villalonga, S. Optimisation of 700 bar type IV hydrogen pressure vessel considering composite damage and dome multi-sequencing. Int. J. Hydrogen Energy 2015, 40, 13215–13230. [Google Scholar] [CrossRef]
- Xu, P.; Zheng, J.; Chen, H.; Liu, P. Optimal design of high pressure hydrogen storage vessel using an adaptive genetic algorithm. Int. J. Hydrogen Energy 2010, 35, 2840–2846. [Google Scholar] [CrossRef]
- Donadon, M.; Iannucci, L.; Falzon, B.; Hodgkinson, J.; Almeida, S. A progressive failure model for composite laminates subjected to low velocity impact damage. Comput. Struct. 2008, 86, 1232–1252. [Google Scholar] [CrossRef]
- Tan, S.C.; Perez, J. Progressive Failure of Laminated Composites with a Hole under Compressive Loading. J. Reinf. Plast. Compos. 1993, 12, 1043–1057. [Google Scholar] [CrossRef]



| Property | T700 Composites |
|---|---|
| Tensile modulus in 1-direction () | 134,000 MPa |
| Tensile modulus in 2-direction () | 8800 MPa |
| Tensile modulus in 3-direction () | 8800 MPa |
| Shear modulus () | 4790 MPa |
| Shear modulus () | 4790 MPa |
| Shear modulus () | 5790 MPa |
| Poisson’s ratio () | 0.31 |
| Poisson’s ratio () | 0.31 |
| Poisson’s ratio () | 0.34 |
| Longitudinal tensile strength | 2600 MPa |
| Longitudinal compressive strength | 1300 MPa |
| Transverse tensile strength | 74 MPa |
| Lateral compressive strength | 180 MPa |
| Longitudinal shear strength | 105 MPa |
| Transverse shear strength | 105 MPa |
| Property | Aluminum Alloy 6061 | Nylon 11 |
|---|---|---|
| Elastic modulus, GPa | 74 | 1.3 |
| Yield modulus, MPa | 280 | 40 |
| Poisson’s ratio | 0.28 | 0.4 |
| Tensile strength, MPa | 368 | 50 |
| Density, | 2.75 | 1.04 |
| Materials and Equipment | Specification | Manufacturer |
|---|---|---|
| Epoxy resin | HY 406 | Huayu New Material Technology Co., Ltd, Shanghai, Chin. |
| Carbon fiber | T700SC-12000-50C | Toray, Japan |
| Four-axis CNC winding machine | CRJ-12 | Longde Science and Technology Co., Ltd, Xi’an, China. |
| Acetone | WF 330 | Lingfeng Chemical Reagent Co., Ltd, Shanghai, China. |
| Heat shrink tape | PVC-12MM | Tengda Plastic Products Co., Ltd, Weifang, China. |
| Name | Unit | Low Value | High Value | |
|---|---|---|---|---|
| A | Winding angle | Degrees | 12 | 16 |
| B | Number of layers | Layers | 100 | 140 |
| C | Helical/Hoop Ratio | 1 | 0.5 | 3 |
| Test Number | Winding Angle (Degrees) | Number of Layers (Layers) | Helical/Hoop Ratio | Burst Pressure (MPa) |
|---|---|---|---|---|
| 1 | 12 | 100 | 1.75 | 132 |
| 2 | 12 | 140 | 1.75 | 170 |
| 3 | 14 | 120 | 1.75 | 155 |
| 4 | 14 | 140 | 3 | 165 |
| 5 | 14 | 140 | 0.5 | 160 |
| 6 | 16 | 120 | 3 | 149 |
| 7 | 12 | 120 | 3 | 147 |
| 8 | 14 | 120 | 1.75 | 154 |
| 9 | 14 | 120 | 1.75 | 153 |
| 10 | 14 | 120 | 1.75 | 156 |
| 11 | 14 | 100 | 3 | 125 |
| 12 | 12 | 120 | 0.5 | 141 |
| 13 | 16 | 120 | 0.5 | 142 |
| 14 | 16 | 140 | 1.75 | 172 |
| 15 | 14 | 120 | 1.75 | 152 |
| 16 | 16 | 100 | 1.75 | 135 |
| 17 | 14 | 100 | 0.5 | 121 |
| Source of Variance | Sum of Squares | Degrees of Freedom | Mean Square Sum | F-Value | |
|---|---|---|---|---|---|
| Regression Model | 3428.56 | 9 | 380.95 | 183.91 | |
| Residual | 14.5 | 7 | 2.07 | / | / |
| Misfit | 4.5 | 3 | 1.5 | 0.6 | 0.6483 |
| Pure error | 10 | 4 | 2.5 | / | / |
| Total error | 3443.06 | 16 | / | / | / |
| Coefficient Term | Sum of Squares | Degrees of Freedom | Mean Square Sum | F-Value | |
|---|---|---|---|---|---|
| 8.00 | 1 | 380.95 | 183.91 | <0.0001 | |
| 2964.50 | 1 | 8.00 | 3.86 | 0.0901 | |
| 60.50 | 1 | 2964.50 | 1431.14 | <0.0001 | |
| 0.25 | 1 | 60.50 | 29.21 | 0.0010 | |
| 0.25 | 1 | 0.25 | 0.12 | 0.7385 | |
| 0.25 | 1 | 0.25 | 0.12 | 0.7385 | |
| 0.066 | 1 | 0.25 | 0.12 | 0.7385 | |
| 14.80 | 1 | 0.066 | 0.032 | 0.8636 | |
| 370.07 | 1 | 14.80 | 7.15 | 0.0319 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Liu, Y.; Qi, L.; Mei, Z.; Gao, R.; Yu, M.; Sun, Z.; Wang, M. Optimization of the Laminate Structure of a Composite Cylinder Based on the Combination of Response Surface Methodology (RSM) and Finite Element Analysis (FEA). Molecules 2022, 27, 7361. https://doi.org/10.3390/molecules27217361
Li Z, Liu Y, Qi L, Mei Z, Gao R, Yu M, Sun Z, Wang M. Optimization of the Laminate Structure of a Composite Cylinder Based on the Combination of Response Surface Methodology (RSM) and Finite Element Analysis (FEA). Molecules. 2022; 27(21):7361. https://doi.org/10.3390/molecules27217361
Chicago/Turabian StyleLi, Zhiqi, Yipeng Liu, Liangliang Qi, Zhonghao Mei, Ruize Gao, Muhuo Yu, Zeyu Sun, and Ming Wang. 2022. "Optimization of the Laminate Structure of a Composite Cylinder Based on the Combination of Response Surface Methodology (RSM) and Finite Element Analysis (FEA)" Molecules 27, no. 21: 7361. https://doi.org/10.3390/molecules27217361
APA StyleLi, Z., Liu, Y., Qi, L., Mei, Z., Gao, R., Yu, M., Sun, Z., & Wang, M. (2022). Optimization of the Laminate Structure of a Composite Cylinder Based on the Combination of Response Surface Methodology (RSM) and Finite Element Analysis (FEA). Molecules, 27(21), 7361. https://doi.org/10.3390/molecules27217361






