Improved Chambadal Model with New Optimization Results
Abstract
:1. Introduction
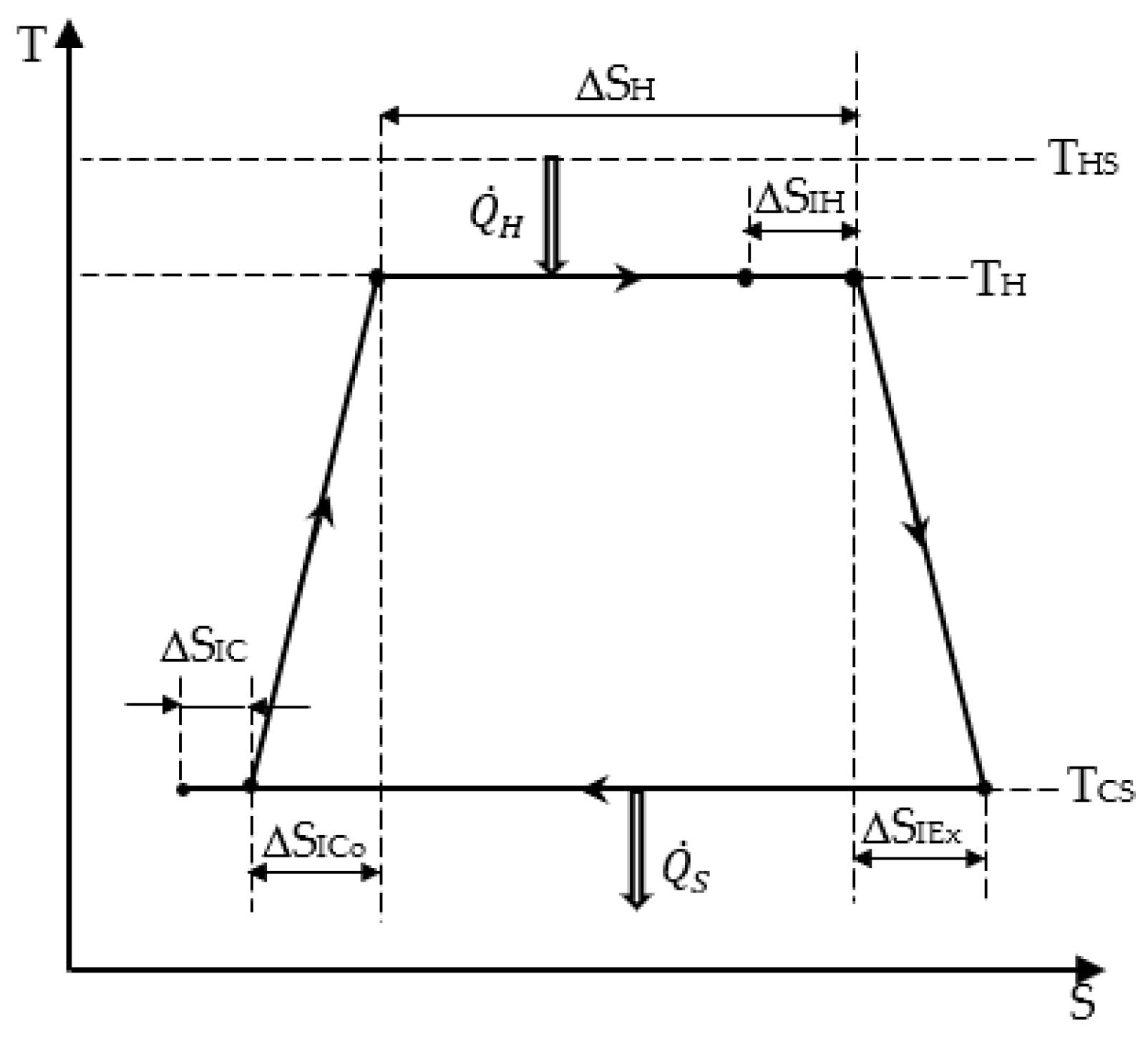
2. Chambadal Model Optimization Based on Entropy Production and with a Coupling Constraint for the Heat Transfer between Source and Converter
- The heat energy expense from the hot source that is expressed as:
- The heat energy converted into mechanical one along the isothermal transformation at TH:
- The entropy balance over the cycle, expressed by:
- The total entropy production of the cycle ΔSI, which is the sum of the four entropy productions during the processes:
- The energy balance over the cycle for the system composed of heat source, converter, and heat sink:
3. Chambadal Model Optimization from the Energy Degradation Point of View
4. Discussion
4.1. Model Considering Heat Transfer Entropy
- -
- The optimum duration of the isothermal process at TH:
- -
- The optimum duration of each process of the cycle:
- -
- The maximum of the mechanical energy:
4.2. Model Considering Energy Degradation
5. Conclusions
- First case, : entropy production method.
- Second case, : energy degradation method.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Carnot, S. Réflexion sur la Puissance Motrice du feu et des Machines Propres à Développer Cette Puissance; Albert Blanchard: Paris, France, 1953. (In French) [Google Scholar]
- Curzon, F.L.; Ahlborn, B. Efficiency of a Carnot Engine at Maximum Power Output. Am. J. Phys. 1975, 43, 22–24. [Google Scholar] [CrossRef]
- Feidt, M.; Costea, M. Progress in Carnot and Chambadal Modeling of Thermomechanical Engine by Considering Entropy Production and Heat Transfer Entropy. Entropy 2019, 21, 1232. [Google Scholar] [CrossRef]
- Feidt, M. The History and Perspectives of Efficiency at Maximum Power of the Carnot Engine. Entropy 2017, 19, 369. [Google Scholar] [CrossRef]
- Tu, Z.C. Recent advance on the efficiency at maximum power of heat engines. Chin. Phys. B 2012, 21, 020513. [Google Scholar] [CrossRef]
- Holubec, V.; Ryabov, A. Maximum efficiency of low-dissipation heat engines at arbitrary power. J. Stat. Mech. Theory Exp. 2016, 2016, 073204. [Google Scholar] [CrossRef]
- Tlili, I. Finite time thermodynamic evaluation of endoreversible Stirling heat engine at maximum power conditions. Renew. Sust. Energ. Rev. 2012, 16, 2234–2241. [Google Scholar] [CrossRef]
- Singh, U.R.; Kumar, A. Review on solar Stirling engine: Development and performance. Therm. Sci. Eng. Prog. 2018, 8, 244–256. [Google Scholar] [CrossRef]
- Kosloff, R.; Rezek, Y. The Quantum Harmonic Otto Cycle. Entropy 2017, 19, 136. [Google Scholar] [CrossRef]
- Birhanu, T.; Bassie, Y.; Abebe, Y.; Bekele, M. Efficiency at Maximum Power in a Parallel Connected Two Quantum Dots Heat Engine. J. Eng. 2023, 2023, 6665740. [Google Scholar] [CrossRef]
- Benenti, G.; Casati, G.; Saito, K.; Whitney, R. Fundamental aspects of steady-state conversion of heat to work at the nanoscale. Phys. Rep. 2017, 649, 1–124. [Google Scholar] [CrossRef]
- Hess, H.; Saper, G. Engineering with Biomolecular Motors. Acc. Chem. Res. 2018, 51, 3015–3022. [Google Scholar] [CrossRef] [PubMed]
- Feidt, M.; Feidt, R. Endo-irreversible thermo-mechanical Carnot engine with new concept of entropy production action coefficient. Eur. Phys. J. Appl. Phys. 2021, 93, 30901. [Google Scholar] [CrossRef]
- Chambadal, P. Les Centrales Nucléaires; Armand Colin: Paris, France, 1957. (In French) [Google Scholar]
- Ibrahim, O.M.; Klein, S.A.; Mitchell, J.W. Optimum heat power cycles for specified boundary conditions. J. Eng. Gas Turb. Power 1991, 113, 514–521. [Google Scholar] [CrossRef]
- Novikov, I.I. The efficiency of atomic power stations. At. Energy 1957, 3, 409–412. [Google Scholar] [CrossRef]
- Esposito, M.; Kawai, R.; Lindenberg, K.; Van den Broeck, C. Efficiency at maximum power of low dissipation Carnot engines. Phys. Rev. Lett. 2010, 105, 150603. [Google Scholar] [CrossRef] [PubMed]
- Feidt, M.; Costea, M. A New Step in the Optimization of the Chambadal Model of the Carnot Engine. Entropy 2022, 24, 84. [Google Scholar] [CrossRef] [PubMed]
- Jourdain, P.E.B. Maupertuis and the principle of least action. Monist 1912, 22, 414–459. Available online: https://www.jstor.org/stable/27900387 (accessed on 29 November 2023). [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feidt, M.; Costea, M. Improved Chambadal Model with New Optimization Results. Entropy 2024, 26, 125. https://doi.org/10.3390/e26020125
Feidt M, Costea M. Improved Chambadal Model with New Optimization Results. Entropy. 2024; 26(2):125. https://doi.org/10.3390/e26020125
Chicago/Turabian StyleFeidt, Michel, and Monica Costea. 2024. "Improved Chambadal Model with New Optimization Results" Entropy 26, no. 2: 125. https://doi.org/10.3390/e26020125
APA StyleFeidt, M., & Costea, M. (2024). Improved Chambadal Model with New Optimization Results. Entropy, 26(2), 125. https://doi.org/10.3390/e26020125







