Entropy of Conduction Electrons from Transport Experiments
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
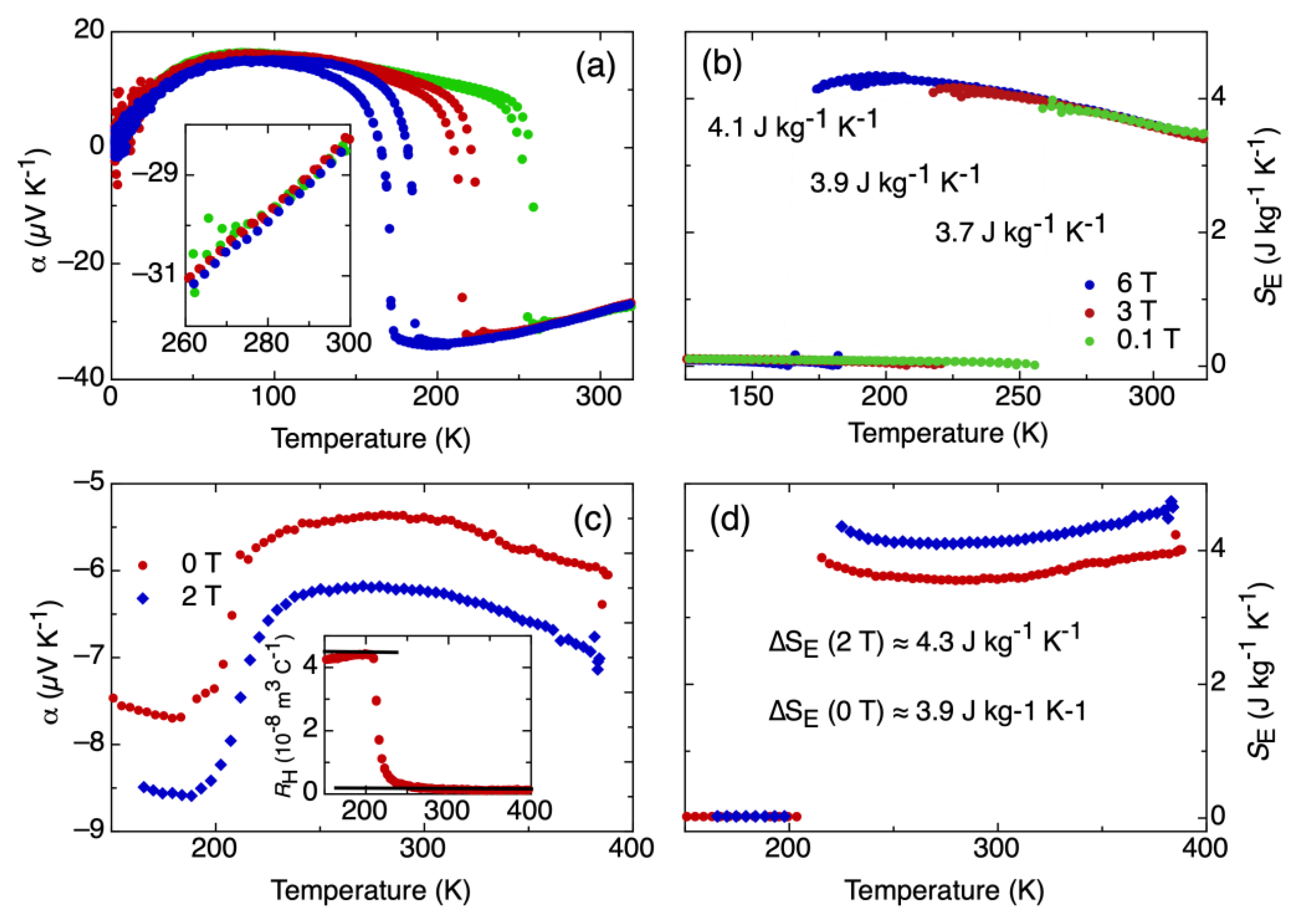
3.1. Magneto-Structural Phase Transition
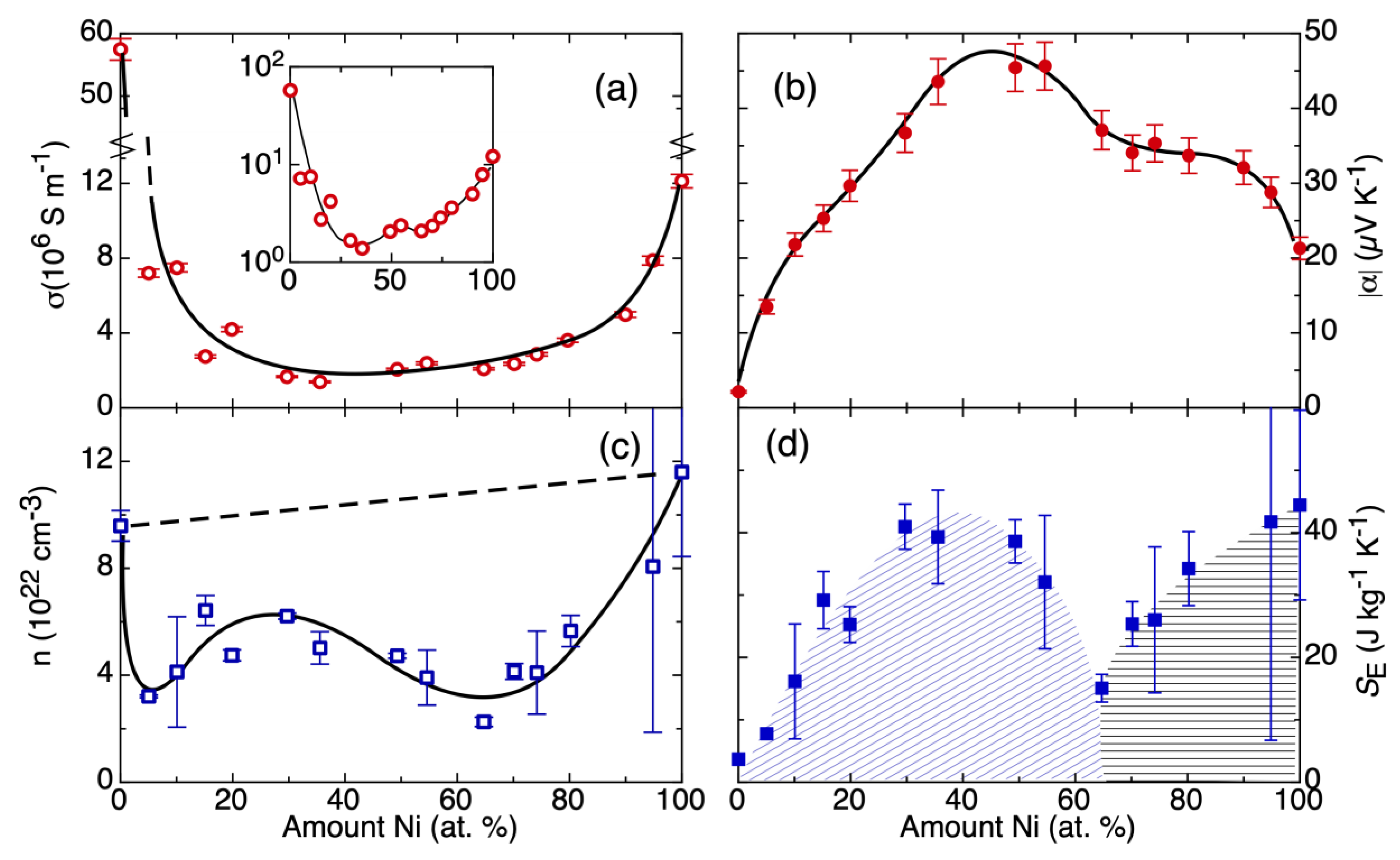
3.2. Alloying
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Brooks/Cole Thomson Learning: South Melbourne, Australia, 2012. [Google Scholar]
- Pérez, N.; Chirkova, A.; Skokov, K.P.; Woodcock, T.G.; Gutfleisch, O.; Baranov, N.V.; Nielsch, K.; Schierning, G. Electronic entropy change in Ni-doped FeRh. Mater. Today Phys. 2019, 9, 100129. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. II. Phys. Rev. 1931, 38, 2265–2279. [Google Scholar] [CrossRef] [Green Version]
- Ioffe, A.F.; Stil’Bans, L.S.; Iordanishvili, E.K.; Stavitskaya, T.S.; Gelbtuch, A.; Vineyard, G. Semiconductor Thermoelements and Thermoelectric Cooling. Phys. Today 1959, 12, 42. [Google Scholar] [CrossRef]
- Rockwood, A.L. Partial Molar Entropy and Partial Molar Heat Capacity of Electrons in Metals and Superconductors. J. Mod. Phys. 2016, 7, 199–218. [Google Scholar] [CrossRef] [Green Version]
- Rockwood, A.L. Partial molar entropy of electrons in a jellium model: Implications for thermodynamics of ions in solution and electrons in metals. Electrochim. Acta 2013, 112, 706–711. [Google Scholar] [CrossRef] [Green Version]
- Peterson, M.; Shastry, B.S. Kelvin formula for thermopower. Phys. Rev. B 2010, 82, 195105. [Google Scholar] [CrossRef] [Green Version]
- Rockwood, A.L. Relationship of thermoelectricity to electronic entropy. Phys. Rev. A 1984, 30, 2843–2844. [Google Scholar] [CrossRef]
- Kleeorin, Y.; Thierschmann, H.; Buhmann, H.; Georges, A.; Molenkamp, L.W.; Meir, Y. How to measure the entropy of a mesoscopic system via thermoelectric transport. Nat. Commun. 2019, 10, 5081. [Google Scholar] [CrossRef]
- Rinzler, C.C.; Allanore, A. Connecting electronic entropy to empirically accessible electronic properties in high temperature systems. Philos. Mag. 2016, 96, 3041–3053. [Google Scholar] [CrossRef] [Green Version]
- Goupil, C.; Seifert, W.; Zabrocki, K.; Müller, E.; Snyder, G.J.; Müller, E. Thermodynamics of Thermoelectric Phenomena and Applications. Entropy 2011, 13, 1481–1517. [Google Scholar] [CrossRef] [Green Version]
- Cutler, M.; Mott, N.F. Observation of Anderson Localization in an Electron Gas. Phys. Rev. 1969, 181, 1336–1340. [Google Scholar] [CrossRef]
- Roberts, R.B. The absolute scale of thermoelectricity. Philos. Mag. 1977, 36, 91–107. [Google Scholar] [CrossRef]
- da Rosa, A. Thermoelectricity. In Fundamentals of Renewable Energy Processes; Elsevier: Amsterdam, The Netherlands, 2013; pp. 149–212. [Google Scholar]
- Nikitin, S.; Myalikgulyev, G.; Tishin, A.; Annaorazov, M.; Asatryan, K.; Tyurin, A. The magnetocaloric effect in Fe49Rh51 compound. Phys. Lett. A 1990, 148, 363–366. [Google Scholar] [CrossRef]
- Fukamichi, K.; Fujita, A.; Fujieda, S. Large magnetocaloric effects and thermal transport properties of La(FeSi)13 and their hydrides. J. Alloys Compd. 2006, 408–412, 307–312. [Google Scholar] [CrossRef]
- Fujieda, S.; Hasegawa, Y.; Fujita, A.; Fukamichi, K. Thermal transport properties of magnetic refrigerants La(FexSi1−x)13 and their hydrides, and Gd5Si2Ge2 and MnAs. J. Appl. Phys. 2004, 95, 2429–2431. [Google Scholar] [CrossRef]
- Shen, B.G.; Sun, J.R.; Hu, F.X.; Zhang, H.W.; Cheng, Z.H. Recent Progress in Exploring Magnetocaloric Materials. Adv. Mater. 2009, 21, 4545–4564. [Google Scholar] [CrossRef] [Green Version]
- Hu, F.-X.; Shen, B.-G.; Sun, J.; Cheng, Z.; Rao, G.-H.; Zhang, X.-X. Influence of negative lattice expansion and metamagnetic transition on magnetic entropy change in the compound LaFe11.4Si1.6. Appl. Phys. Lett. 2001, 78, 3675–3677. [Google Scholar] [CrossRef]
- Hu, F.-X.; Ilyn, M.; Tishin, A.M.; Sun, J.R.; Wang, G.J.; Chen, Y.F.; Wang, F.; Cheng, Z.H.; Shen, B.G. Direct measurements of magnetocaloric effect in the first-order system LaFe11.7Si1.3. J. Appl. Phys. 2003, 93, 5503–5506. [Google Scholar] [CrossRef]
- Baranov, N.; Barabanova, E. Electrical resistivity and magnetic phase transitions in modified FeRh compounds. J. Alloys Compd. 1995, 219, 139–148. [Google Scholar] [CrossRef]
- Glushko, O.; Funk, A.; Maier-Kiener, V.; Kraker, P.; Krautz, M.; Eckert, J.; Waske, A. Mechanical properties of the magnetocaloric intermetallic LaFe11.2Si1.8 alloy at different length scales. Acta Mater. 2019, 165, 40–50. [Google Scholar] [CrossRef] [Green Version]
- Haeusler, J. Die Geometriefunktion vierelektrodiger Hallgeneratoren. Electr. Eng. 1968, 52, 11–19. [Google Scholar] [CrossRef]
- Lu, W.; Nam, N.T.; Suzuki, T. First-order magnetic phase transition in FeRh–Pt thin films. J. Appl. Phys. 2009, 105, 07A904. [Google Scholar] [CrossRef] [Green Version]
- Gercsi, Z.; Fuller, N.; Sandeman, K.G.; Fujita, A. Electronic structure, metamagnetism and thermopower of LaSiFe12and interstitially doped LaSiFe12. J. Phys. D Appl. Phys. 2017, 51, 034003. [Google Scholar] [CrossRef] [Green Version]
- Jia, L.; Liu, G.J.; Sun, J.R.; Zhang, H.W.; Hu, F.-X.; Dong, C.; Rao, G.; Shen, B.G. Entropy changes associated with the first-order magnetic transition in LaFe13−xSix. J. Appl. Phys. 2006, 100, 123904. [Google Scholar] [CrossRef]
- Hannemann, U.; Lyubina, J.; Ryan, M.P.; Alford, N.M.; Cohen, L.F. Thermopower of LaFe 13−x Si x alloys. EPL 2012, 100, 57009. [Google Scholar] [CrossRef]
- Gruner, M.; Keune, W.; Landers, J.; Salamon, S.; Krautz, M.; Zhao, J.; Hu, M.Y.; Toellner, T.; Alp, E.E.; Gutfleisch, O.; et al. Moment-Volume Coupling in La(Fe1−x Si x)13. Phys. Status Solidi (B) 2017, 255, 1700465. [Google Scholar] [CrossRef] [Green Version]
- Landers, J.; Salamon, S.; Keune, W.; Gruner, M.; Krautz, M.; Zhao, J.; Hu, M.Y.; Toellner, T.S.; Alp, E.E.; Gutfleisch, O.; et al. Determining the vibrational entropy change in the giant magnetocaloric material LaFe11.6Si1.4 by nuclear resonant inelastic x-ray scattering. Phys. Rev. B 2018, 98, 024417. [Google Scholar] [CrossRef] [Green Version]
- Madelung, O. Cr-Cs–Cu-Zr; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Gruen, D.M.; Bruno, P.; Xie, M. Configurational, electronic entropies and the thermoelectric properties of nanocarbon ensembles. Appl. Phys. Lett. 2008, 92, 143118. [Google Scholar] [CrossRef] [Green Version]
- Liu, R.; Chen, H.; Zhao, K.; Qin, Y.; Jiang, B.; Zhang, T.; Sha, G.; Shi, X.; Uher, C.; Zhang, W.; et al. Entropy as a Gene-Like Performance Indicator Promoting Thermoelectric Materials. Adv. Mater. 2017, 29, 1702712. [Google Scholar] [CrossRef]
- Hurd, C.M. The Hall Effect in Metals and Alloys; Springer Science and Business Media LLC: Berlin, Germany, 1972. [Google Scholar]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pérez, N.; Wolf, C.; Kunzmann, A.; Freudenberger, J.; Krautz, M.; Weise, B.; Nielsch, K.; Schierning, G. Entropy of Conduction Electrons from Transport Experiments. Entropy 2020, 22, 244. https://doi.org/10.3390/e22020244
Pérez N, Wolf C, Kunzmann A, Freudenberger J, Krautz M, Weise B, Nielsch K, Schierning G. Entropy of Conduction Electrons from Transport Experiments. Entropy. 2020; 22(2):244. https://doi.org/10.3390/e22020244
Chicago/Turabian StylePérez, Nicolás, Constantin Wolf, Alexander Kunzmann, Jens Freudenberger, Maria Krautz, Bruno Weise, Kornelius Nielsch, and Gabi Schierning. 2020. "Entropy of Conduction Electrons from Transport Experiments" Entropy 22, no. 2: 244. https://doi.org/10.3390/e22020244
APA StylePérez, N., Wolf, C., Kunzmann, A., Freudenberger, J., Krautz, M., Weise, B., Nielsch, K., & Schierning, G. (2020). Entropy of Conduction Electrons from Transport Experiments. Entropy, 22(2), 244. https://doi.org/10.3390/e22020244




