Recent Advances in Statistical and Matrix Manifolds: Theory and Applications

Abstract submission deadline

closed (20 July 2023)

Manuscript submission deadline

closed (20 December 2023)

Viewed by

1124

Topic Information

Dear Colleagues,

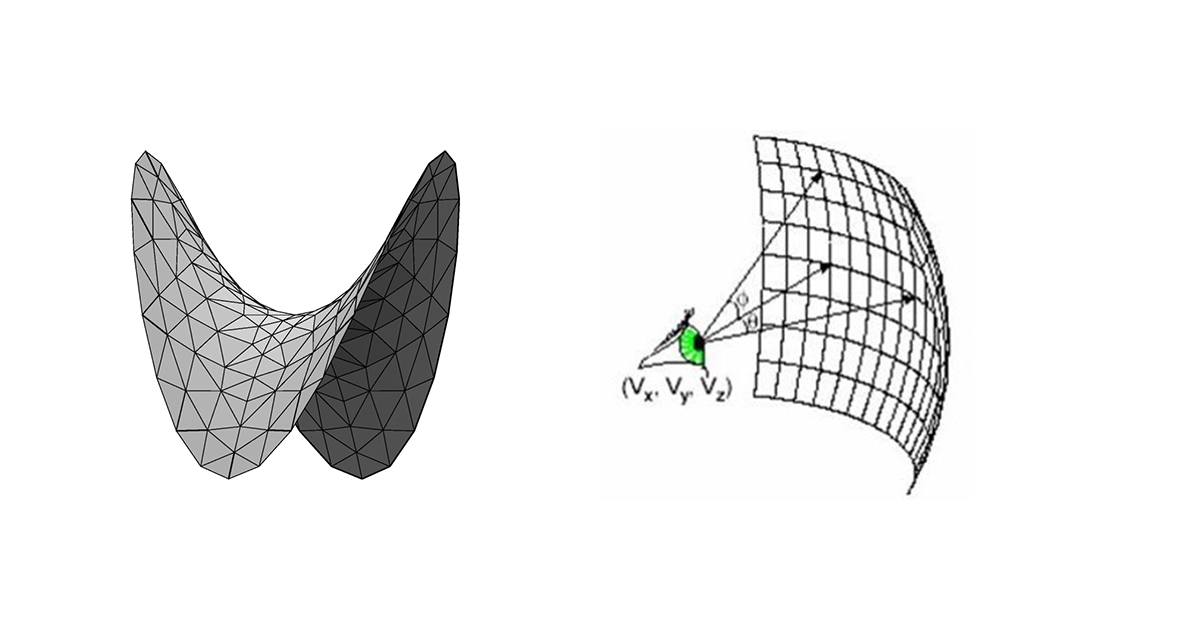
Recent years have seen the uptake of information geometry to study structure of manifolds and optimization problems where probability distributions are central. The impetus has come from a number of engineering applications in signal processing, optimization, control and more recently statistical machine learning in relation to statistical inference problems and properties of statistical estimators. For example, the manifold of a positive-definite Hessian matrix arises in signal processing in the covariance matrix estimation problem of complex normal distributions and the solution of Lyapunov stability and the Riccati equations in control. Central to information geometry is the notion of a divergence measure between any two points on a manifold which endows the manifold with a Riemannian structure and a pair of dually flatness criteria. This allows generalization of the Pythagorean Theorem and the related projection theorem to manifolds. By generalizing the distance measure in the Euclidean space to a divergence, a manifold is endowed with a Riemannian structure. The information geometric approach provides a geometric interpretation for analysis which yields greater insight. Moreover, the geometric structure can be exploited to develop computationally efficient optimization algorithms. To study geometric properties of statistical and matrix manifolds and exploiting them to develop geometric optimization algorithms that will be superior to traditional methods. Homology will provide a mathematically rigorous framework to study the geometry of a general class of manifolds.

In the deterministic setting, the geometry of subgroups and submanifolds of the general linear group, that is, orthogonal group, symplectic group and special Euclidean group is of interest. In particular the left invariant metric is adopted as the Riemannian metric and the geodesic curve between any two points is defined. The exponential and logarithmic maps on these subgroups and submanifolds admit an analytical form which is convenient for computations.

For this Special Issue, we are looking for reviews or original research papers on a wide range of topics related to the information geometry and topology of manifolds and its applications in mathematics and the physical sciences.

Dr. Muhammad Shoaid Arif
Dr. Syed Ahmed Pasha
Dr. kamaleldin Abodayeh
Topic Editors

Keywords

Participating Journals

Journal Name	Impact Factor	CiteScore	Launched Year	First Decision (median)	APC
Algorithms algorithms	1.8	4.1	2008	18.9 Days	CHF 1600
Axioms axioms	1.9	-	2012	22.8 Days	CHF 2400
Entropy entropy	2.1	4.9	1999	22.3 Days	CHF 2600
Mathematics mathematics	2.3	4.0	2013	18.3 Days	CHF 2600
Symmetry symmetry	2.2	5.4	2009	17.3 Days	CHF 2400

Preprints.org is a multidisciplinary platform offering a preprint service designed to facilitate the early sharing of your research. It supports and empowers your research journey from the very beginning.

MDPI Topics is collaborating with Preprints.org and has established a direct connection between MDPI journals and the platform. Authors are encouraged to take advantage of this opportunity by posting their preprints at Preprints.org prior to publication:

1. Share your research immediately: disseminate your ideas prior to publication and establish priority for your work.
2. Safeguard your intellectual contribution: Protect your ideas with a time-stamped preprint that serves as proof of your research timeline.
3. Boost visibility and impact: Increase the reach and influence of your research by making it accessible to a global audience.
4. Gain early feedback: Receive valuable input and insights from peers before submitting to a journal.
5. Ensure broad indexing: Web of Science (Preprint Citation Index), Google Scholar, Crossref, SHARE, PrePubMed, Scilit and Europe PMC.

Published Papers