Mechanical Properties of Al–Mg–Si Alloys (6xxx Series): A DFT-Based Study
Abstract
:1. Introduction
2. Materials and Methods
2.1. Density Functional Theory
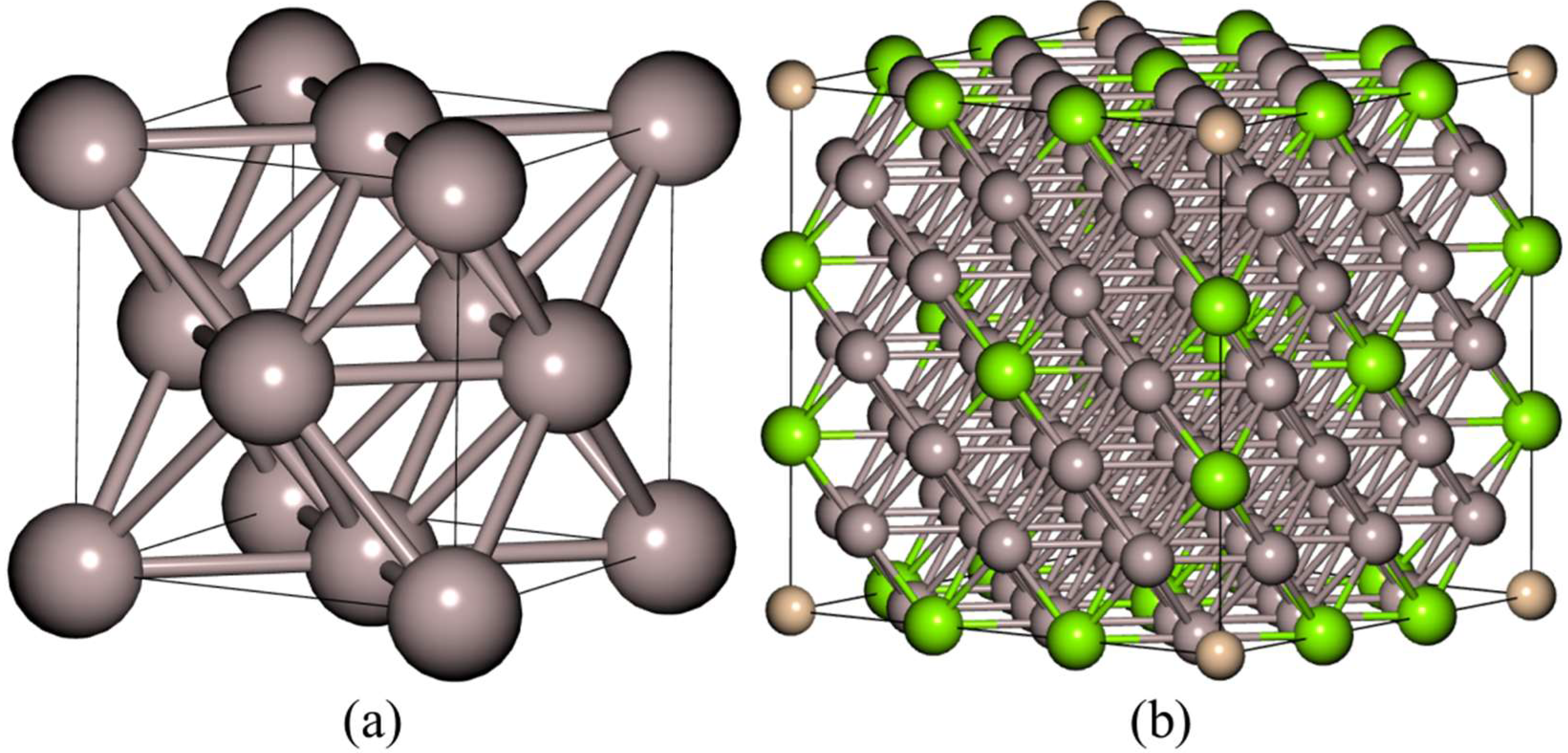
2.2. Modeling the Structures of the Alloys
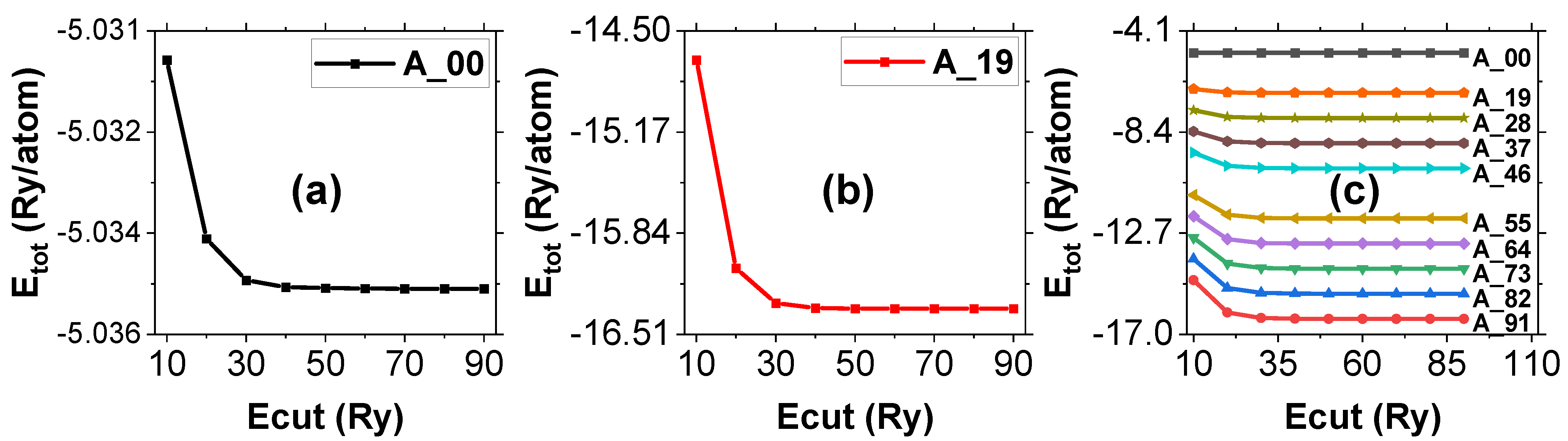
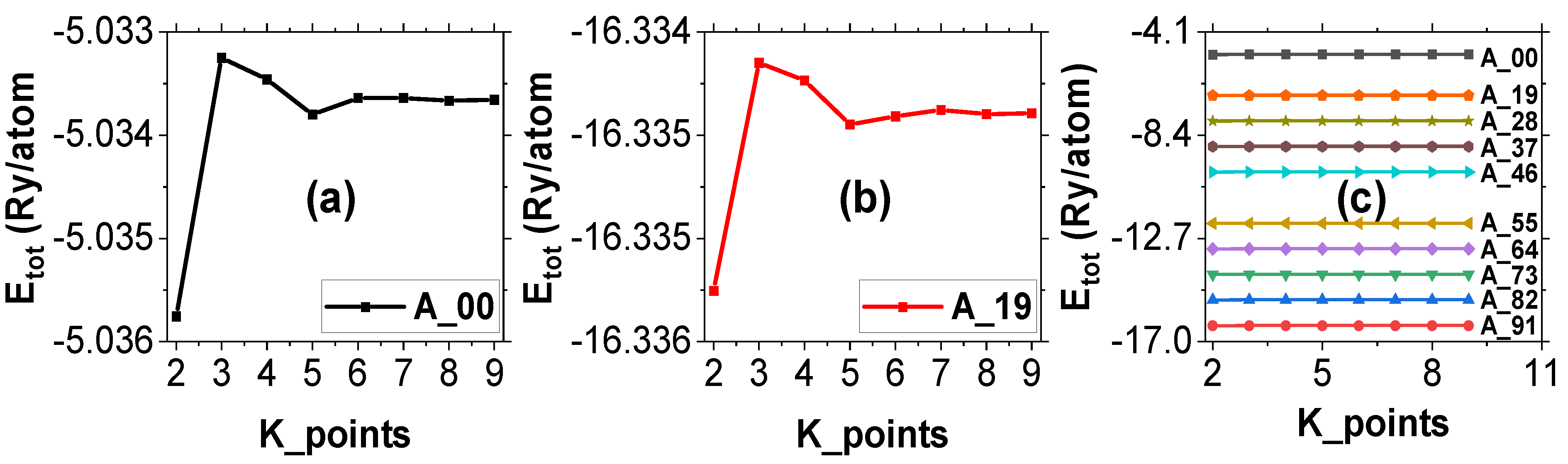
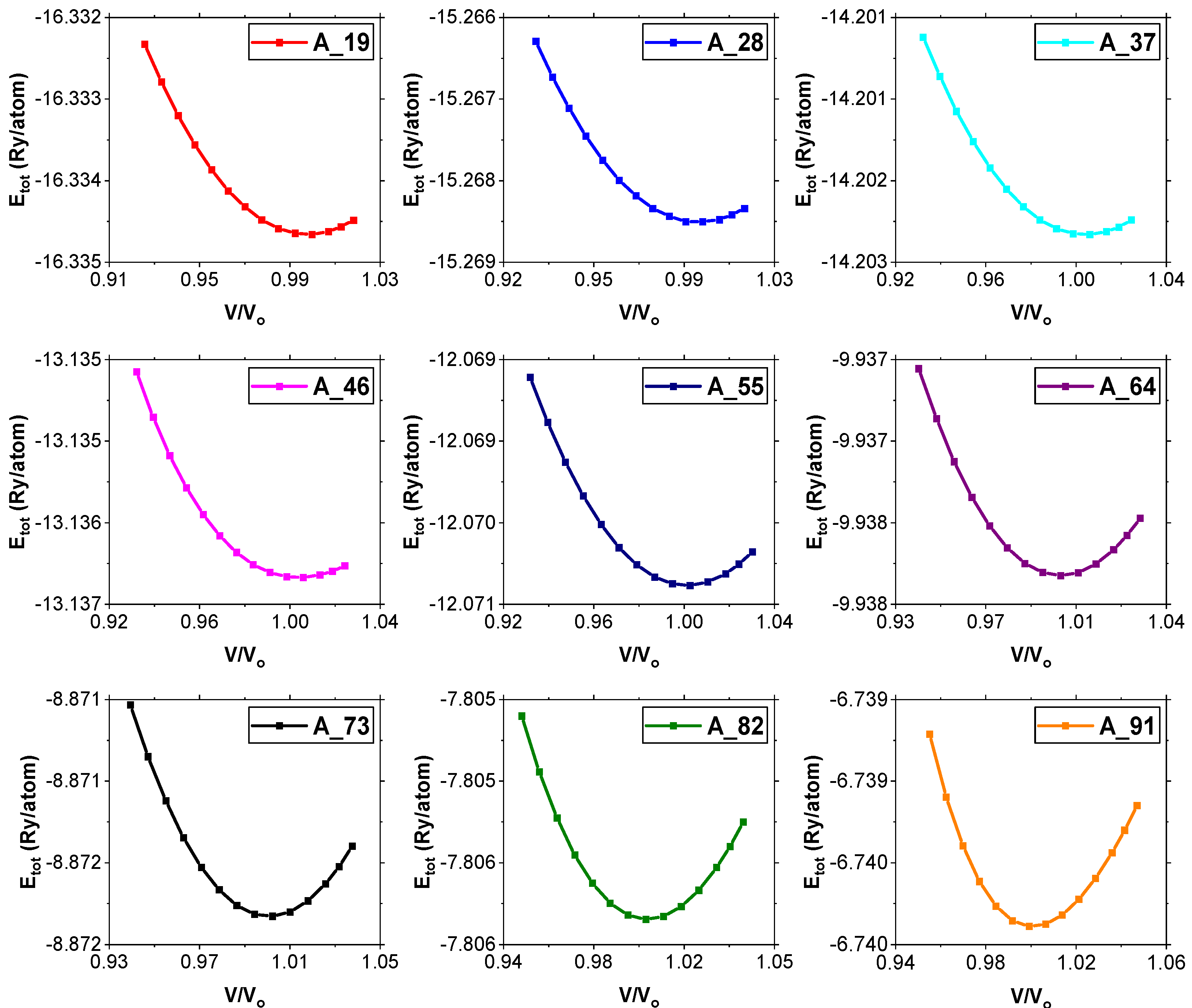
2.3. Structural Optimization
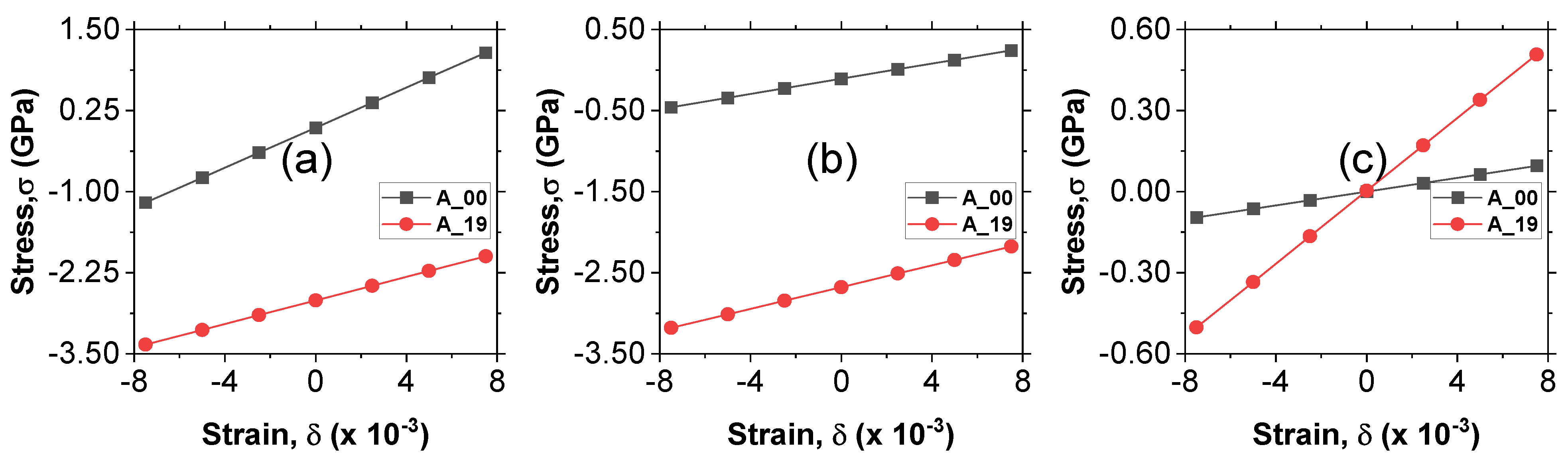
2.4. Calculation of Mechanical Properties
3. Results and Discussion
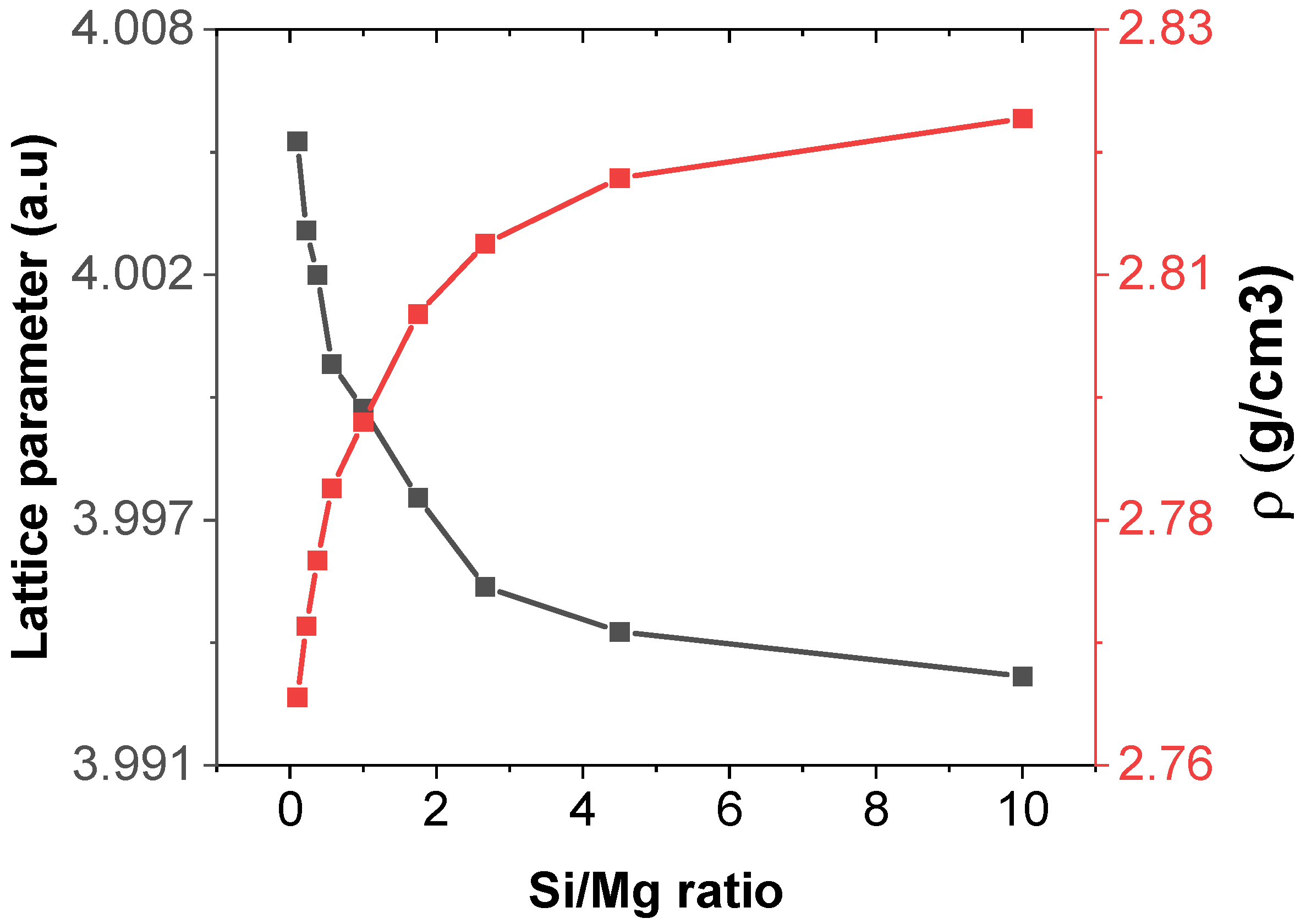
3.1. Structural Properties
3.2. Mechanical Properties
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hirsch, J. Aluminium alloys for automotive application. Mater. Sci. Forum 1997, 242, 33–50. [Google Scholar] [CrossRef]
- Hirsch, J. Recent development in aluminium for automotive applications. Trans. Nonferrous Met. Soc. China 2014, 24, 1995–2002. [Google Scholar] [CrossRef]
- Ambroziak, A.; Solarczyk, M.T. Application and Mechanical Properties of Aluminum Alloys; Gdansk University of Technology, Faculty of Civil and Environmental Engineering: Gdansk, Poland, 2018. [Google Scholar] [CrossRef]
- Heinz, A.; Haszler, A.; Keidel, C.; Moldenhauer, S.; Beneductus, R.; Miller, W.S. Recent development in aluminium alloys for aerospace applications. Mater. Sci. Eng. A 2000, 280, 102–107. [Google Scholar] [CrossRef]
- Hirsch, J. Automotive trends in aluminium—The European perspective. Mater. Sci. Forum 2004, 28, 14–23. [Google Scholar]
- Padmanaban, D.A.; Kurien, G. Silumins: The automotive alloys. AM&P Tech. Artic. 2012, 170, 28–30. [Google Scholar] [CrossRef]
- Derlet, P.M.; Andersen, S.J.; Marioara, C.D.; Froseth, A. A first rinciples study of the B″-phase in Al-Mg-Si alloys. J. Phys. Condens. Matter 2009, 14, 4011. [Google Scholar] [CrossRef]
- Baruah, M.; Borah, A. Processing and precipitation strengthening of 6xxx series aluminium alloys: A review. Int. J. Mater. Sci. 2020, 1, 40–48. [Google Scholar] [CrossRef]
- Mageto, J.M. TEM Study of Microstructure in Relation to Hardness and ductility In Al-Mg-Si Alloys (6xxx Series). Master’s Thesis, Department of Physics, Faculty of Natural Sciences and Technology, Norwegian University of Science and Technology (NTNU), Trondheim, Norway, 2003. [Google Scholar]
- Werinos, M.; Antrekowitsch, H.; Ebner, T.; Prillhofer, R.; Uggowitzer, P.J.; Pogatscher, S. Hardening of Al–Mg–Si alloys: Effect of trace elements and prolonged natural aging. Mater. Des. 2016, 107, 257–268. [Google Scholar] [CrossRef]
- Yuan, W.; Liang, Z.; Zhang, C.; Wei, L. Effects of La addition on the mechanical properties and thermal-resistant properties of Al–Mg–Si–Zr alloys based on AA 6201. Mater. Des. 2012, 34, 788–792. [Google Scholar] [CrossRef]
- Raj, R.J.; Selvam, P.; Pughalendi, M. A review of aluminum alloys in aircraft and aerospace industry. J. Huazhong Univ. Sci. Technol. 2021, 1671, 4512. [Google Scholar]
- Georgantzia, E.; Gkantou, M.; Kamaris, G.S. Aluminium alloys as structural material: A review of research. Eng. Struct. 2021, 227, 111372. [Google Scholar] [CrossRef]
- Lejaeghere, K.; Bihlmayer, G.; Bjorkman, T.; Blaha, P.; Blugel, S. Reproducibility in Density Functional Theory Calculations of Solids. Science 2016, 351, 3000. [Google Scholar] [CrossRef]
- Avery, P.; Wang, X.; Oses, C.; Gossett, E.; Proserpio, D.M.; Toher, C.; Curtalo, S.; Zurek, E. Predicting superhard materials via a machine learning informed evolutionary structure search. Comput. Mater. 2019, 89, 1038. [Google Scholar] [CrossRef]
- Jona, F.P.; Marcus, P.M. Lattice parameters of aluminium in Mbar range by first principles. J. Physiscs Condens. Matter 2006, 18, 10881–10888. [Google Scholar] [CrossRef]
- Vanderbilt, D. Soft self-consistent pseudopotential in generalised eigenvalue formalism. Phys. Rev. B Am. Phys. Soc. 1990, 41, 7892–7895. [Google Scholar] [CrossRef]
- Adllan, A.A.; Corso, A.D. Ultrasoft pseudopotentials and projector augmented-wave data sets: Application to diatomite molecules. J. Phys. Condens. Matter 2011, 23, 425501. [Google Scholar] [CrossRef]
- Ongwen, N.O.; Ogam, E.; Otunga, H. Ab initio study of elastic properties of orthorhombic cadmium stannate as a substrate for manufacture of MEMS devices. Mater. Today Commun. 2020, 26, 101822. [Google Scholar] [CrossRef]
- Bouchenafa, M.; Benmakhlouf, A.; Sidoumou, M.; Bouchemadou, A.; Maabed, S.; Halit, M. Theoretical investigation of the structural, elastic, electronic and optical properties of the ternary tetragonal tellurides KBTe2 (B = Al, In). Mater. Sci. Semicond. Process. 2020, 114, 105085. [Google Scholar] [CrossRef]
- Boucetta, S.; Zegrar, F. Density functional study of elastic, mechanical and thermodynamic properties of MgCu with a CsCl-type structure. J. Magnessium Alloys 2013, 1, 128–133. [Google Scholar] [CrossRef]
- Daoud, S.; Loucif, K.; Bioud, N.; Lebga, N. First-principles study of structural, elastic and mechanical properties of zinc-blende boron nitride (B3-BN). Acta Phys. Pol. A 2012, 122, 109–115. [Google Scholar] [CrossRef]
- Ongwen, N.O.; Ogam, E.; Fellah, Z.E.A.; Otunga, H.O.; Oduor, A.; Mageto, M. Accurate Ab-initio calculation of elastic constants of anisotropic binary alloys: A case of Fe–Al. Solid State Commun. 2022, 353, 114879. [Google Scholar] [CrossRef]
- Wen, Y.; Wang, L.; Liu, H.; Song, L. Ab initio study of elastic and mechanical properties of B19 TiAl. Crystals 2017, 7, 39. [Google Scholar] [CrossRef]
- Hill, R. The elastic behaviour of a crystalline aggregate. Proc. Physcal Soc. A 1952, A65, 349. [Google Scholar] [CrossRef]
- Chen, X.Q.; Niu, H.; Franchini, C.; Li, D.; Li, Y. Hardness of T-carbon: Density functional calculations. Phys. Rev. B 2011, 84, 121405. [Google Scholar] [CrossRef]
- Ongwen, N.; Ogam, E.; Fellah, Z.E.A.; Mageto, M.; Othieno, H.; Otunga, H. Thermal properties and pressure-dependent elastic constants of cadmium stannate as a substrate for MEMS: An ab initio study. Phys. B Condens. Matter 2022, 651, 414599. [Google Scholar] [CrossRef]
- Kiely, E.; Zware, R.; Fox, R.; Reilly, A.; Guerin, S. Density functional theory predictions of the mechanical properties of crystalline materials. R. Soc. Chem. 2021, 23, 5697–5710. [Google Scholar] [CrossRef]
- Froseth, A.G.; Hoier, R.; Derlet, P.M.; Andersen, S.J.; Marioara, C.D. Bonding in MgSi and Al-Mg-Si compounds relevant in Al-Mg-Si alloys. Phys. Rev. B 2003, 67, 224106. [Google Scholar] [CrossRef]
- Nakashima, P.N.H. The Crystallography of Aluminium and Its Alloys. In Encyclopedia of Aluminium and Its Alloys; Totten, G.E., Tiryakioglu, M., Kessler, O., Eds.; CRC Press: Boca Raton, FL, USA, 2018; pp. 488–586. [Google Scholar]
- Hashiguchi, D.; Ashurst, A.N.; Grensing, F.C.; Marder, J.M. Materion beryllium & composites. In Proceedings of the International Symposium Advanced Materials for Lightweight Structures, ESTEC, Noordwijk, The Netherlands, 25–27 March 1992. [Google Scholar]
- Marie, H.A. Retrieved from What Is Shear Modulus? Available online: https://www.thoughtco.com/shear-modulus-4176406 (accessed on 17 February 2021).
- Hadi, M.; Rayhan, M.; Naqib, S.; Chroneous, A.; Islam, A. Structural, elastic, thermal and lattice dynamic propertiesof new 321 MAX phases. Comput. Mater. Sci. 2019, 170, 109144. [Google Scholar] [CrossRef]
- Fleury, E.; Kim, D. Poisson’s ratio and fragility of bulk metallic glasses. J. Mater. Res. 2008, 23, 523–528. [Google Scholar] [CrossRef]
- Pugh, S. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals. Lond. Edinburg Dublin Philos. J. Sci. 1954, 84, 823–843. [Google Scholar] [CrossRef]
- Teter, D. Computational alchemy: The search for new super hard materials. MRS Bull. 1998, 23, 22–27. [Google Scholar] [CrossRef]

| Sample ID | Silicon | Magnesium | Aluminium | Si/Mg Ratio | Si/(Mg+Si) | |||
|---|---|---|---|---|---|---|---|---|
| Conc. (%) | Atoms | Conc. (%) | Atoms | Conc. (%) | Atoms | |||
| A_00 | 0 | 0 | 0 | 0 | 100 | 108 | - | - |
| A_19 | 1 | 1 | 9 | 10 | 90 | 97 | 0.100 | 0.091 |
| A_28 | 2 | 2 | 8 | 9 | 90 | 97 | 0.222 | 0.182 |
| A_37 | 3 | 3 | 7 | 8 | 90 | 97 | 0.375 | 0.270 |
| A_46 | 4 | 4 | 6 | 7 | 90 | 97 | 0.571 | 0.364 |
| A_55 | 5 | 5 | 5 | 5 | 90 | 97 | 1.000 | 0.455 |
| A_64 | 6 | 7 | 4 | 4 | 90 | 97 | 1.750 | 0.545 |
| A_73 | 7 | 8 | 3 | 3 | 90 | 97 | 2.667 | 0.636 |
| A_82 | 8 | 9 | 2 | 2 | 90 | 97 | 4.500 | 0.727 |
| A_91 | 9 | 10 | 1 | 1 | 90 | 97 | 10.00 | 0.818 |
| Alloy Sample | a (Å) | ρ (g/cm3) |
|---|---|---|
| A_00 | 4.020 (4.050 a) (4.032 b) | 2.756 (2.700 c) (2.700 d) |
| A_19 | 4.005 | 2.762 |
| A_28 | 4.003 | 2.770 |
| A_37 | 4.002 | 2.779 |
| A_46 | 4.000 | 2.783 |
| A_55 | 3.999 | 2.790 |
| A_64 | 3.997 | 2.801 |
| A_73 | 3.995 | 2.808 |
| A_82 | 3.994 | 2.815 |
| A_91 | 3.993 | 2.821 |
| Alloy Sample | Si/Mg Ratio | (GPa) | (GPa) | (GPa) |
|---|---|---|---|---|
| A_00 | - | 100.5 | 62.5 | 34.6 |
| A_19 | 0.100 | 90.5 | 68.0 | 33.2 |
| A_28 | 0.222 | 87.8 | 69.5 | 29.5 |
| A_37 | 0.375 | 87.0 | 69.9 | 29.5 |
| A_46 | 0.571 | 82.5 | 71.0 | 24.5 |
| A_55 | 1.000 | 88.6 | 71.5 | 29.6 |
| A_64 | 1.750 | 114.3 | 61.8 | 34.4 |
| A_73 | 2.667 | 118.2 | 59.9 | 34.2 |
| A_82 | 4.500 | 126.3 | 58.7 | 34.8 |
| A_91 | 10.00 | 123.3 | 63.3 | 33.2 |
| Alloy Sample | Si/Mg Ratio | B (GPa) | G (GPa) | E (GPa) | µ | n | Hv (GPa) Chen | Hv (GPa) Tian |
|---|---|---|---|---|---|---|---|---|
| A_00 | - | 74.0 | 27.6 | 73.1 | 0.335 | 2.70 | 1.35 | 3.14 |
| A_19 | 0.100 | 75.5 | 21.5 | 59.0 | 0.370 | 3.51 | −0.22 | 1.94 |
| A_28 | 0.222 | 75.6 | 18.5 | 51.3 | 0.387 | 4.09 | −0.88 | 1.46 |
| A_37 | 0.375 | 75.6 | 18.0 | 50.1 | 0.390 | 4.20 | −0.97 | 1.39 |
| A_46 | 0.571 | 74.8 | 13.8 | 39.0 | 0.413 | 5.42 | −1.71 | 0.86 |
| A_55 | 1.000 | 77.2 | 18.0 | 50.2 | 0.392 | 4.28 | −1.02 | 1.37 |
| A_64 | 1.750 | 79.3 | 30.9 | 82.0 | 0.328 | 2.57 | 1.93 | 3.57 |
| A_73 | 2.667 | 79.3 | 32.1 | 84.6 | 0.322 | 2.48 | 2.27 | 3.82 |
| A_82 | 4.500 | 81.2 | 34.4 | 90.4 | 0.315 | 2.36 | 2.79 | 4.24 |
| A_91 | 10.00 | 83.3 | 31.9 | 84.8 | 0.330 | 2.61 | 1.93 | 3.58 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pius, K.K.; Ongwen, N.O.; Mageto, M.; Odari, V.; Gaitho, F.M. Mechanical Properties of Al–Mg–Si Alloys (6xxx Series): A DFT-Based Study. Alloys 2023, 2, 213-226. https://doi.org/10.3390/alloys2030015
Pius KK, Ongwen NO, Mageto M, Odari V, Gaitho FM. Mechanical Properties of Al–Mg–Si Alloys (6xxx Series): A DFT-Based Study. Alloys. 2023; 2(3):213-226. https://doi.org/10.3390/alloys2030015
Chicago/Turabian StylePius, Kipkorir Kirui, Nicholas O. Ongwen, Maxwell Mageto, Victor Odari, and Francis Magiri Gaitho. 2023. "Mechanical Properties of Al–Mg–Si Alloys (6xxx Series): A DFT-Based Study" Alloys 2, no. 3: 213-226. https://doi.org/10.3390/alloys2030015
APA StylePius, K. K., Ongwen, N. O., Mageto, M., Odari, V., & Gaitho, F. M. (2023). Mechanical Properties of Al–Mg–Si Alloys (6xxx Series): A DFT-Based Study. Alloys, 2(3), 213-226. https://doi.org/10.3390/alloys2030015