A Collection of New Trigonometric- and Hyperbolic-FGM-Type Copulas

Abstract

Copula analysis was created to explain the dependence of two or more quantitative variables. Due to the need for in-depth data analysis involving complex variable relationships, there is always a need for new copula models with original features. As a modern example, for the analysis of circular or periodic data types, trigonometric copulas are particularly attractive and recommended. This is, however, an underexploited topic. In this article, we propose a new collection of eight trigonometric and hyperbolic copulas, four based on the sine function and the others on the tangent function, all derived from the construction of the famous Farlie–Gumbel–Morgenstern copula. In addition to their original trigonometric and hyperbolic functionalities, the proposed copulas have the feature of depending on three parameters with complementary roles: one is a dependence parameter; one is a shape parameter; and the last can be viewed as an angle parameter. In our main findings, for each of the eight copulas, we determine a wide range of admissible values for these parameters. Subsequently, the capabilities, features, and functions of the new copulas are thoroughly examined. The shapes of the main functions of some copulas are illustrated graphically. Theoretically, symmetry in general, stochastic dominance, quadrant dependence, tail dependence, Archimedean nature, correlation measures, and inference on the parameters are investigated. Some copula shapes are illustrated with the help of figures. On the other hand, some two-dimensional inequalities are established and may be of separate interest.

1. Introduction

In many real-world scenarios, modeling the relationship (or dependence) between two or more quantitative variables is crucial. In order to achieve this, the copulas provide effective solutions in the quantitative case. They recently received a lot of attention due to the varying intricacy of dependence that emerges in current data analysis challenges. An overview of the copula theory can be found in [1,2,3,4,5]. Two-dimensional copulas must be used when there are only two quantitative variables of concern. The following mathematical basis provides a definition of such two-dimensional copulas in the absolutely continuous case.

Definition 1.

(Absolutely continuous two-dimensional copulas [5]). Let $\mathcal{S}={[0,1]}^2$. A two-dimensional function $C(x,y)$, $(x,y)\in \mathcal{S}$, is said to be an absolutely continuous two-dimensional copula if and only if the following conditions are met for any $(x,y)\in \mathcal{S}$:

(I)

$C(x,0)=0$ and $C(0,y)=0$,

(II)

$C(x,1)=x$ and $C(1,y)=y$,

(III)

${\displaystyle {\partial }_{x,y}C(x,y)\ge 0}$, where ${\partial }_{x,y}={\partial }^2/(\partial x\partial y)$ represents the standard mixed second order partial derivatives according to x and y.

In the following, the mention “absolutely continuous two-dimensional” is omitted to lighten the comments. Clearly, from a technical viewpoint, the less immediate item in Definition 1 is item (III). It can demand fastidious developments.

Established families of copulas include the elliptical copulas (Gaussian, Student, etc.), Archimedean copulas (Ali–Mikhail–Haq, Clayton, Frank, etc.), extreme value copulas, and Farlie–Gumbel–Morgenstern (FGM)-type copulas. Despite the extensive literature, copula modeling is still a hot topic, and numerous research studies have been conducted to develop the approach from both the theoretical and practical aspects. Significant contemporary references include [6,7,8,9,10,11,12,13,14,15,16,17,18].

In order to motivate the findings of this article, a review of the FGM copula is required. To start, it is mathematically described as

$$\begin{array}{c}\hfill C(x,y;\theta )=xy+\theta xy(1-x)(1-y),(x,y)\in \mathcal{S},\end{array}$$

where $\theta \in [-1,1]$. In this expression, the polynomial function $\theta xy(1-x)(1-y)$ is considered a perturbed function of $xy$, and $xy$ corresponds to the independence copula; when $\theta =0$, $C(x,y;\theta )$ is reduced to the independence copula. The key details about the FGM copula are contained in [1,2,3,4,5,19]. The polynomial form of the perturbation function and the moderate correlation range are two drawbacks of the FGM copula. These factors are crucial, among other situations, when fitting two-dimensional data. The FGM copula has, however, garnered a lot of attention from researchers due to its straightforward algebraic characteristics. It has also motivated new varieties of FGM-type copulas. See, for instance, [12,20,21,22].

On the other hand, recent references have suggested including trigonometric functions in the construction of copulas, making them more suitable in some circumstances. In particular, in order to model the correlations into phenomena that have a periodic, circular, or seasonal nature, it is intended to give the copula some oscillating properties. They are particularly well adapted to the correlation analysis of movement, circular, and environmental data. The references for conventional trigonometric copulas include [7,23,24,25,26,27,28,29]. For their practical aspect, we refer to [16,30,31,32,33]. Additionally, the trigonometric copulas now have a new applied dimension thanks to the R package Cylcop, recently created in [34].

In this article, we create a collection of new three-parameter copulas that have the general form of the FGM copula, but the perturbed function is completely redefined; it involves either trigonometric or hyperbolic functions. More precisely, we focus on special copulas of the following form:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

where $\upsilon =(\alpha ,\beta ,\gamma )$ is the considered three-parameter vector and $\varphi \left(u\right)$ is a specific trigonometric or hyperbolic sine or tangent function. More precisely, the following functions for $\varphi \left(u\right)$ are considered:

$$\varphi \left(u\right)\in \{sin(u),arcsin(u),sinh(u),arcsinh(u),tan(u),tanh(u),arctan(u),arctanh(u\left)\right\}.$$

The main novelties of the resulting copulas are in (i) the inclusion of a shape parameter $\gamma$ that modulates the effect of $x^{\gamma }{y}^{\gamma }$ on the perturbation function, following the spirit of the method in [12] for the extended FGM copula, (ii) the consideration of completely new trigonometric or hyperbolic functions for $\varphi \left(u\right)$ as the main transformation, and (iii) the consideration of the parameter $\beta$, which is major to exploit at the maximum support of $\varphi \left(u\right)$; it can be considered an angle parameter if $\varphi \left(u\right)$ is a trigonometric function (see [7,26]). It is also worth noting that the perturbation function $\varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]$ is not separable in x and y, i.e., we cannot write it as $\psi \left(x\right)\phi \left(y\right)$, where $\psi \left(x\right)$ and $\phi \left(y\right)$ denote two functions. For the common separable case, we may refer to [14], and the references therein.

In this innovative setting, for each of the eight copulas, we establish wide admissible ranges of values for $\alpha$, $\beta$ and $\gamma$, which may be connected in a sophisticated manner. The proofs are based on complex two-dimensional differentiations, factorizations, and various kinds of mathematical inequalities. The features of the suggested copulas are then investigated. The effect of the parameters on the shapes of some of them is illustrated graphically. In addition, the related functions (survival copula and copula density), symmetry (diagonal symmetry and radial symmetry), quadrant dependence, first-order stochastic dominance, tail dependence, medial and Spearman correlations are examined. The inference on the parameters is also briefly discussed. This study serves as the foundation for the development of new multi-dimensional trigonometric and hyperbolic copulas, which continue to be of particular relevance in various applications. On the other hand, a number of two-dimensional inequalities are discovered and might be of independent interest.

Three other sections compose the article. The main copulas are studied and presented in Section 2. Section 3 proposes some graphical analyses and a unified study of their properties. A short conclusion is given in Section 4.

2. A Collection of Eight New Copulas

This section describes the created copulas in detail, as well as the main functions associated with them.

2.1. Sine-FGM Copula

The result below presents a new sine-FGM-type copula.

Proposition 1.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha sin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(1)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$, and

$$\begin{array}{c}\hfill \beta \le \frac{\pi }{2m_{\gamma }^2},\left|\alpha \right|\le \frac{1}{\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)},\end{array}$$

(2)

where $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)$. Then, $C(x,y;\upsilon )$ is a copula.

Proof. 

The proof consists in demonstrating that the proposed function satisfies the items (I), (II) and (III) of Definition 1.

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha sin\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha sin\left(0\right)=0.$$

Using a similar development, for any $y\in [0,1]$, we obtain $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we have

$$C(x,1;\upsilon )=x\times 1+\alpha sin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha sin\left(0\right)=x.$$

Similarly, for any $y\in [0,1]$, it is clear that $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, we find

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )=1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]cos\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]}\hfill \\ \hfill & -\alpha {\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]sin\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Hence, by manipulating the absolute values, the following inequality is obtained:

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\left|cos\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|}\hfill \\ \hfill & -\left|\alpha \right|{\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\left|sin\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|.\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$.

Furthermore, since $\beta \ge 0$, we have

$$0\le \beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\le \beta m_{\gamma }^2,$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

In addition, since $\beta \le \pi /\left(2m_{\gamma }^2\right)$, we have $\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\in [0,\pi /2]$. On the other hand, for any $u\in [0,\pi /2]$, we obviously have $cos\left(u\right)\ge 0$ and $sin\left(u\right)\ge 0$. These results imply that

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\left|\alpha \right|\beta {\gamma }^2{x}^{\gamma -1}{y}^{\gamma -1}cos\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & -\left|\alpha \right|{\beta }^2{\gamma }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}sin\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Owing to the following trigonometric inequalities, $sin\left(u\right)\le u$ and $cos\left(u\right)\le 1$ for any $u\in [0,\pi /2]$, we have

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\beta {\gamma }^2{x}^{\gamma -1}{y}^{\gamma -1}\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right].\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, it is clear that $x^{\gamma -1}{y}^{\gamma -1}\le 1$, and we have

$$1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\le 1+{\beta }^2{m}_{\gamma }^4.$$

By combining the above inequalities, we obtain

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\left|\alpha \right|\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4).\hfill \end{array}$$

Finally, the assumption $\left|\alpha \right|\le 1/\left[\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)\right]$ gives

$$\begin{array}{c}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 0.}\end{array}$$

Item (III) is proved.

The proof of Proposition 1 ends. □

The copula shown in Equation (1) is named the sine-FGM (S) copula.

Remark 1.

Due to the numerous formulas involving the sine function, various equivalent expressions for the S copulas are possible. For instance, by using the formula $sin\left(u\right)=2sin(u/2)cos(u/2)$ for $u\in \mathbb{R}$, an alternative expression is

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+2\alpha sin\left[\frac{\beta }{2}{x}^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]cos\left[\frac{\beta }{2}{x}^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S}.\end{array}$$

The sine and cosine functions are thus involved.

Based on Equation (1), the S copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )=1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]cos\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & -\alpha {\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]sin\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

(3)

Clearly, the parameter $\gamma$ has a strong effect on the function at the extreme points $(0,0)$, $(1,0)$, and $(0,1)$, which modifies the global morphology. We can examine the modeling potential of the S copula as well as the effects of the parameters $\alpha$, $\beta$ and $\gamma$ on its shapes by looking at the forms of this function. A graphical analysis of the S copula and the S copula density is performed in Section 3.

As a complementary copula function, the S survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha sin\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The main difference between the S copula and the S survival copula is the effect of $\gamma$, which is not the same. The S survival copula is also a novel three-parameter copula to be included in the body of the existing literature.

In a similar vein, we can present two new copulas based on the flipping method, thanks to the work of [35]; the x-flipping copula is specified as

$$\begin{array}{cc}\hfill \overline{C}(x,y;\upsilon )& =y-C(1-x,y;\upsilon )\hfill \\ \hfill & =xy-\alpha sin\left[\beta {(1-x)}^{\gamma }{y}^{\gamma }x(1-y)\right],(x,y)\in \mathcal{S},\hfill \end{array}$$

and the y-flipping copula is specified as

$$\begin{array}{cc}\hfill \tilde{C}(x,y;\upsilon )& =x-C(x,1-y;\upsilon )\hfill \\ \hfill & =xy-\alpha sin\left[\beta x^{\gamma }{(1-y)}^{\gamma }(1-x)y\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

For the sake of clarity, only the corresponding survival copulas are described for the remaining trigonometric and hyperbolic copulas.

Remark 2.

The coming proofs associated with the new copulas follow the main structure of the proof of Proposition 1, but the mathematical developments necessitate different approaches in terms of differentiation, factorization, and inequality tools, yielding a wide range of values for the parameters α, β, and γ.

2.2. Arcsine-FGM Copula

A novel copula of the arcsine-FGM type is shown in the result below. We recall that the arcsine of x, denoted as $f\left(x\right)=arcsin\left(x\right)$, is defined as the inverse sine function of x when $x\in [-1,1]$. Moreover, we have $f^{\prime }\left(x\right)=1/\sqrt{1-x^2}$.

Proposition 2.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha arcsin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(4)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \beta \le \frac{1}{m_{\gamma }^2},\left|\alpha \right|\le \frac{{(1-{\beta }^2{m}_{\gamma }^4)}^{3/2}}{\beta {\gamma }^2},\end{array}$$

(5)

where $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)$. Then $C(x,y;\upsilon )$ is a copula.

Proof. 

The proof is based on Definition 1; the goal is to demonstrate that the proposed function satisfies the related items (I), (II) and (III).

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha arcsin\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha arcsin\left(0\right)=0.$$

Using a similar development, for any $y\in [0,1]$, we obtain $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we have

$$C(x,1;\upsilon )=x\times 1+\alpha arcsin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha arcsin\left(0\right)=x.$$

Clearly, for any $y\in [0,1]$, we have $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, we establish that

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{\sqrt{1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }}}\hfill \\ \hfill & +\frac{\alpha {\beta }^3{x}^{3\gamma -1}{y}^{3\gamma -1}{(1-x)}^2{(1-y)}^2[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}}\hfill \\ \hfill & =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}}.\hfill \end{array}$$

Hence, with the use of absolute values, we obtain the following inequality:

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\frac{\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}}.\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. Furthermore, we have $x^{\gamma -1}{y}^{\gamma -1}\le 1$, $\beta \ge 0$, and since $\beta \le 1/m_{\gamma }^2$, we establish that

$$1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\ge 1-{\beta }^2{m}_{\gamma }^4>0,$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

By combining the above inequalities, we obtain

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\frac{\left|\alpha \right|\beta {\gamma }^2}{{(1-{\beta }^2{m}_{\gamma }^4)}^{3/2}}.\hfill \end{array}$$

Hence, under the assumption $\left|\alpha \right|\le {(1-{\beta }^2{m}_{\gamma }^4)}^{3/2}/\left(\beta {\gamma }^2\right)$, we find

$$\begin{array}{c}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 0.}\end{array}$$

Item (III) is proved.

The proof of the proposition ends. □

The copula indicated in Equation (4) is called the arcsine-FGM (AS) copula.

Based on Equation (4), the AS copula density is specified by

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )=1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}},(x,y)\in \mathcal{S}.\hfill \end{array}$$

It is important to note that, in contrast to other new copulas, this copula density has a relatively manageable expression.

The AS survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha arcsin\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

It is another new three-parameter copula to be added to the body of literature.

2.3. Hyperbolic Sine-FGM Copula

An innovative hyperbolic sine-FGM type copula is shown in the result below. We recall that the hyperbolic sine of x, denoted as $f\left(x\right)=sinh\left(x\right)$, is defined as $f\left(x\right)=(e^x-e^{-x})/2$ with $x\in \mathbb{R}$. Furthermore, we have $f^{\prime }\left(x\right)=(e^x+e^{-x})/2$, which corresponds exactly to the hyperbolic cosine function, denoted by $cosh\left(x\right)$.

Proposition 3.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha sinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(6)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \left|\alpha \right|\le \frac{1}{\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)cosh\left(\beta m_{\gamma }^2\right)},\end{array}$$

(7)

where $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)$. Then $C(x,y;\upsilon )$ is a copula.

Proof. 

The proof refers to items (I), (II) and (III) of Definition 1.

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha sinh\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha sinh\left(0\right)=0.$$

Similarly, for any $y\in [0,1]$, we obtain $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we have

$$C(x,1;\upsilon )=x\times 1+\alpha sinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha sinh\left(0\right)=x.$$

Additionally, for any $y\in [0,1]$, we have $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, we obtain

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )=1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]cosh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]}\hfill \\ \hfill & +\alpha {\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]sinh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Hence, with the use of absolute values, we establish the following inequality:

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\left|cosh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|}\hfill \\ \hfill & -\left|\alpha \right|{\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\left|sinh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|.\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. Furthermore, it is clear that, since $\beta \ge 0$, we have $\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\ge 0$, and for $u\ge 0$, we have $cosh\left(u\right)\ge 1>0$ and $sinh\left(u\right)\ge 0$. These results imply that

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\left|\alpha \right|\beta {\gamma }^2{x}^{\gamma -1}{y}^{\gamma -1}cosh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & -\left|\alpha \right|{\beta }^2{\gamma }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}sinh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Owing to the hyperbolic inequality $ucosh\left(u\right)\ge sinh\left(u\right)$ for any $u\in \mathbb{R}$, we find

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )\hfill \\ \hfill \ge & 1-\left|\alpha \right|{\gamma }^2\beta x^{\gamma -1}{y}^{\gamma -1}\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]cosh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, it is clear that $x^{\gamma -1}{y}^{\gamma -1}\le 1$, and, because $cosh\left(u\right)$ is increasing for $u\ge 0$, we obtain

$$1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }cosh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\le (1+{\beta }^2{m}_{\gamma }^4)cosh\left(\beta m_{\gamma }^2\right),$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

By putting the above inequalities together, we obtain

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\left|\alpha \right|\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)cosh\left(\beta m_{\gamma }^2\right).\hfill \end{array}$$

Finally, the assumption $\left|\alpha \right|\le 1/[\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)cosh\left(\beta m_{\gamma }^2\right)]$ gives

$$\begin{array}{c}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 0.}\end{array}$$

Item (III) is proved.

The proof of the proposition ends. □

The copula indicated in Equation (6) is called the hyperbolic sine-FGM (HS) copula.

Remark 3.

An alternative expression of the HS copula involving the exponential function is

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\frac{\alpha }{2}\left(e^{\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}-e^{-\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}\right),(x,y)\in \mathcal{S}.\end{array}$$

To begin, based on Equation (6), the HS copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )=1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]cosh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & +\alpha {\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]sinh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The HS survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha sinh\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The HS survival copula is also a novel three-parameter copula to be included in the body of existing literature.

2.4. Hyperbolic Arcsine-FGM Copula

Below is the result, which displays a brand-new copula of the hyperbolic arcsine-FGM type. We recall that the hyperbolic arcsine of x, denoted as $f\left(x\right)=arcsinh\left(x\right)$, is defined as $f\left(x\right)=log\left(x+\sqrt{1+x^2}\right)$ with $x\in \mathbb{R}$. Moreover, we have $f^{\prime }\left(x\right)=1/\sqrt{1+x^2}$.

Proposition 4.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha arcsinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(8)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \left|\alpha \right|\le \frac{1}{\beta {\gamma }^2}.\end{array}$$

(9)

Then, $C(x,y;\upsilon )$ is a copula.

Proof. 

The proof is based on Definition 1; the objective is to show that the proposed function fulfills the associated items (I), (II) and (III).

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha arcsinh\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha arcsinh\left(0\right)=0.$$

Using a similar development, for any $y\in [0,1]$, we obtain $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we obtain

$$C(x,1;\upsilon )=x\times 1+\alpha arcsinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha arcsinh\left(0\right)=x.$$

Clearly, for any $y\in [0,1]$, we have $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, the following expression is determined:

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{\sqrt{1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }}}\hfill \\ \hfill & -\frac{\alpha {\beta }^3{x}^{3\gamma -1}{y}^{3\gamma -1}{(1-x)}^2{(1-y)}^2[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}}\hfill \\ \hfill & =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}}.\hfill \end{array}$$

Hence, with the use of absolute values, we obtain the following inequality:

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\frac{\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}}.\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. Furthermore, we have $x^{\gamma -1}{y}^{\gamma -1}\le 1$, $\beta \ge 0$, and, obviously, $1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\ge 1$. By combining the above inequalities, along with the assumption $\left|\alpha \right|\le 1/\left(\beta {\gamma }^2\right)$, we obtain

$$\begin{array}{cc}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}& \ge 1-\left|\alpha \right|\beta {\gamma }^2\ge 0.\hfill \end{array}$$

Item (III) is proved.

This completes the proof of Proposition 4. □

The copula shown in Equation (8) is named the hyperbolic arcsine-FGM (HAS) copula.

Remark 4.

An alternative expression of the HAS copula involving the logarithmic function is

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha log\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)+\sqrt{1+{\beta }^2{x}^{2\gamma }{y}^{2\gamma }{(1-x)}^2{(1-y)}^2}\right],(x,y)\in \mathcal{S}.\end{array}$$

Based on Equation (8), the HAS copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )=1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^{3/2}},(x,y)\in \mathcal{S}.\hfill \end{array}$$

(10)

The HAS copula and copula density have the advantages of having weak constraints on the parameters (as indicated in Equation (9)), and manageable analytical expressions. For these reasons, a graphic analysis is devoted to them in Section 3.

The HAS survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha arcsinh\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

Another brand-new three-parameter copula to be added to the corpus of current research is the HAS survival copula.

2.5. Tangent-FGM Copula

The result below exhibits a novel tangent-FGM type copula. We recall that the tangent function of x, denoted as $f\left(x\right)=tan\left(x\right)$, is defined as $f\left(x\right)=sin\left(x\right)/cos\left(x\right)$ with x such that $cos\left(x\right)\ne 0$. Moreover, we have $f^{\prime }\left(x\right)=1+{tan}^2\left(x\right)={sec}^2\left(x\right)$, where $sec\left(x\right)=1/cos\left(x\right)$.

Proposition 5.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha tan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(11)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \beta <\frac{\pi }{2m_{\gamma }^2},\left|\alpha \right|\le \frac{1}{\beta {\gamma }^2\left[1+2\beta m_{\gamma }^{2}tan\left(\beta m_{\gamma }^2\right)\right]{sec}^2\left(\beta m_{\gamma }^2\right)},\end{array}$$

(12)

where $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)$. Then $C(x,y;\upsilon )$ is a copula.

Proof. 

For this proof, it is enough to show that the items (I), (II) and (III) of Definition 1 are fulfilled.

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha tan\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha tan\left(0\right)=0.$$

Using a similar argument, for any $y\in [0,1]$, we have $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, it is clear that

$$C(x,1;\upsilon )=x\times 1+\alpha tan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha tan\left(0\right)=x.$$

In addition, for any $y\in [0,1]$, we have $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, we find

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )=\hfill \\ \hfill & 1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]{sec}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & +2\alpha {\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\times \hfill \\ \hfill & tan\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]{sec}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & =1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\times \hfill \\ \hfill & \left[1+2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }tan\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right]{sec}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Hence, by using the absolute value properties, we obtain

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\times \hfill \\ \hfill & \left[1+2\left|\beta \right|(1-x)(1-y)x^{\gamma }{y}^{\gamma }\left|tan\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|\right]{sec}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. Furthermore, it is clear that $x^{\gamma -1}{y}^{\gamma -1}\le 1$. On the other hand, we have $\beta \ge 0$ and

$$0\le \beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\le \beta m_{\gamma }^2,$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

Since $\beta <\pi /\left(2m_{\gamma }^2\right)$ (strict inequality), we have $\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\in [0,\pi /2)$. Since $\beta \ge 0$, $tan\left(u\right)\ge 0$, and $tan\left(u\right)$ and $sec\left(u\right)$ are increasing for $u\in [0,\pi /2)$, we obtain

$$1+2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\left|tan\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|\le 1+2\beta m_{\gamma }^{2}tan\left(\beta m_{\gamma }^2\right)$$

and

$${sec}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\le {sec}^2\left(\beta m_{\gamma }^2\right).$$

The above inequalities give

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\beta {\gamma }^2\left[1+2\beta m_{\gamma }^{2}tan\left(\beta m_{\gamma }^2\right)\right]{sec}^2\left(\beta m_{\gamma }^2\right)}\hfill \end{array}$$

Finally, the assumption $\left|\alpha \right|\le 1/\left\{\beta {\gamma }^2\left[1+2\beta m_{\gamma }^{2}tan\left(\beta m_{\gamma }^2\right)\right]{sec}^2\left(\beta m_{\gamma }^2\right)\right\}$ implies that

$$\begin{array}{c}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 0.}\end{array}$$

The item (III) is proved.

The proof of the proposition ends. □

The copula shown in Equation (11) is named the tangent-FGM (T) copula.

Based on Equation (11), the T copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )\hfill \\ \hfill & =1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\times \hfill \\ \hfill & \left[1+2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }tan\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right]{sec}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The T survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha tan\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The T survival copula is also a novel three-parameter copula to be included in the body of existing literature.

2.6. Arctangent-FGM Copula

The result shown below exhibits a distinctive copula of the arctangent-FGM type. We recall that the arctangent function of x, denoted as $f\left(x\right)=arctan\left(x\right)$, is defined as the inverse tangent function of x when $x\in \mathbb{R}$. Moreover, we have $f^{\prime }\left(x\right)=1/(1+x^2)$.

Proposition 6.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha arctan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(13)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \beta \le \frac{\sqrt{2}}{m_{\gamma }^2},\left|\alpha \right|\le \frac{1}{\beta {\gamma }^2}.\end{array}$$

(14)

Then $C(x,y;\upsilon )$ is a copula.

Proof. 

The proof is based on Definition 1; we aim to demonstrate that the proposed function satisfies the related items (I), (II) and (III).

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha arctan\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha arctan\left(0\right)=0.$$

Similarly, for any $y\in [0,1]$, we obtain $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we obviously have

$$C(x,1;\upsilon )=x\times 1+\alpha arctan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha arctan\left(0\right)=x.$$

In addition, for any $y\in [0,1]$, we obtain $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, we find

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )=1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }}\hfill \\ \hfill & -\frac{2\alpha {\beta }^3{(1-x)}^2{(1-y)}^2{x}^{3\gamma -1}{y}^{3\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2}\hfill \\ \hfill & =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2}.\hfill \end{array}$$

Hence, with the use of absolute values, we have

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )}\hfill \\ \hfill & \ge 1-\frac{\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\left|1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right|}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2}.\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. In addition, it is clear that $x^{\gamma -1}{y}^{\gamma -1}\le 1$ and $1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\ge 1$. Furthermore, we have

$$1-{\beta }^2{m}_{\gamma }^4\le 1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\le 1,$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

Hence, the assumptions $\beta \ge 0$ and $\beta \le \sqrt{2}/m_{\gamma }^2$ imply that $1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\ge -1$ and

$$\left|1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right|\le 1.$$

The above inequalities and the assumption $\left|\alpha \right|\le 1/\left(\beta {\gamma }^2\right)$ yield

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\beta {\gamma }^2\ge 0.}\hfill \end{array}$$

Item (III) is proved.

The proof of the proposition ends. □

The copula shown in Equation (13) is named the arctangent-FGM (AT) copula.

Based on Equation (13), the AT copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )\hfill \\ \hfill & =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}{{\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2},(x,y)\in \mathcal{S}.\hfill \end{array}$$

The AT survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha arctan\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The AT survival copula is also a novel three-parameter copula to be included in the body of existing literature.

2.7. Hyperbolic Tangent-FGM Copula

A unique hyperbolic tangent-FGM type copula can be seen in the result below. We recall that the hyperbolic tangent function of x, denoted as $f\left(x\right)=tanh\left(x\right)$, is defined as $f\left(x\right)=sinh\left(x\right)/cosh\left(x\right)=(e^x-e^{-x})/(e^x+e^{-x})$ when $x\in \mathbb{R}$. In addition, we have $f^{\prime }\left(x\right)=4/{(e^x+e^{-x})}^2=1/{cosh}^2\left(x\right)$, which corresponds to the square of the hyperbolic secant function, denoted by $sech\left(x\right)$, i.e., $sech\left(x\right)=1/cosh\left(x\right)$.

Proposition 7.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha tanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(15)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \beta \le \frac{x_{☆}}{m_{\gamma }^2},\left|\alpha \right|\le \frac{1}{\beta {\gamma }^2},\end{array}$$

(16)

with $x_{★}$ such that $x_{★}tanh\left(x_{★}\right)=1$, i.e., $x_{★}\approx 1.19968$. Then $C(x,y;\upsilon )$ is a copula.

Proof. 

The objective is to demonstrate that the proposed function satisfies the items (I), (II) and (III) of Definition 1.

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we obtain

$$C(x,0;\upsilon )=x\times 0+\alpha tanh\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha tanh\left(0\right)=0.$$

Using a similar development, for any $y\in [0,1]$, we have $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we have

$$C(x,1;\upsilon )=x\times 1+\alpha tanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha tanh\left(0\right)=x.$$

Additionally, for any $y\in [0,1]$, it is clear that $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, we obtain

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )=\hfill \\ \hfill & 1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]{sech}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & -2\alpha {\beta }^2(1-x)(1-y)x^{2\gamma -1}{y}^{2\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\times \hfill \\ \hfill & tanh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]{sech}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\hfill \\ \hfill & =1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\times \hfill \\ \hfill & \left[1-2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }tanh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right]{sech}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Hence, the following inequalities are established with the use of absolute values:

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\times \hfill \\ \hfill & \left|1-2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }tanh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|{sech}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right].\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. Furthermore, it is clear that $x^{\gamma -1}{y}^{\gamma -1}\le 1$. On the other hand, since $\beta \ge 0$, we have

$$0\le \beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\le \beta m_{\gamma }^2,$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

Owing to the assumption $\beta \le x_{★}/m_{\gamma }^2$, we obtain $\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\in [0,x_{★}]$. Let us now set $f\left(u\right)=1-2utanh\left(u\right)$. Since $f^{\prime }\left(u\right)=-2[tanh\left(u\right)+u{sech}^2\left(u\right)]\le 0$ for $u\in [0,x_{★}]$, $f\left(u\right)$ is decreasing and we have $-1=f\left(x_{★}\right)\le f\left(u\right)\le f\left(0\right)=1$, so $\left|f\right(u\left)\right|\le 1$. This implies that

$$\left|1-2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }tanh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right|\le 1.$$

Since $sech\left(u\right)$ is decreasing for $u\ge 0$, with $sech\left(u\right)\ge 0$, we have ${sech}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\le {sech}^2\left(0\right)=1$.

The above inequalities and the assumption $\left|\alpha \right|\le 1/\left(\beta {\gamma }^2\right)$ give

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\beta {\gamma }^2\ge 0.}\hfill \end{array}$$

Item (III) is proved.

Proposition 7 is proved. □

The copula shown in Equation (15) is named the hyperbolic tangent-FGM (HT) copula.

Remark 5.

An alternative expression of the HT copula involving the exponential function is

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha \frac{e^{\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}-e^{-\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}}{e^{\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}+e^{-\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}},(x,y)\in \mathcal{S}.\end{array}$$

Based on Equation (15), the HT copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )\hfill \\ \hfill & =1+\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\times \hfill \\ \hfill & \left[1-2\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }tanh\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right]\right]{sech}^2\left[\beta (1-x)(1-y)x^{\gamma }{y}^{\gamma }\right],\hfill \\ \hfill & (x,y)\in \mathcal{S}.\hfill \end{array}$$

The HT survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha tanh\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

Another new three-parameter copula to be added to the body of current research is the HT survival copula.

2.8. Hyperbolic Arctangent-FGM Copula

A remarkable copula of the hyperbolic arctangent-FGM type is proposed in the result given below. We recall that the hyperbolic arctangent function of x, denoted as $f\left(x\right)=arctanh\left(x\right)$, is defined as $f\left(x\right)=(1/2)log\left[(1+x)/(1-x)\right]$ when $x\in (-1,1)$. Moreover, we have $f^{\prime }\left(x\right)=1/(1-x^2)$.

Proposition 8.

Let us consider the following two-dimensional function:

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha arctanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

(17)

where $\upsilon =(\alpha ,\beta ,\gamma )$, and α, β and γ are such that $\beta \ge 0$, $\gamma \ge 1$ and

$$\begin{array}{c}\hfill \beta <\frac{1}{m_{\gamma }^2},\left|\alpha \right|\le \frac{{(1-{\beta }^2{m}_{\gamma }^4)}^2}{\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)},\end{array}$$

(18)

where $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)$. Then $C(x,y;\upsilon )$ is a copula.

Proof. 

The proof is based on Definition 1; we need to show that the proposed function satisfies the related items (I), (II) and (III).

(I)

For any $x\in [0,1]$, since $\gamma \ge 1$, we have

$$C(x,0;\upsilon )=x\times 0+\alpha arctanh\left[\beta x^{\gamma }\times 0^{\gamma }\times (1-x)(1-0)\right]=0+\alpha arctanh\left(0\right)=0.$$

Similarly, for any $y\in [0,1]$, we obtain $C(0,y;\upsilon )=0$.

(II)

For any $x\in [0,1]$, we have

$$C(x,1;\upsilon )=x\times 1+\alpha arctanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-1)\right]=x+\alpha arctanh\left(0\right)=x.$$

Moreover, for any $y\in [0,1]$, we have $C(1,y;\upsilon )=y$.

(III)

After differentiation, simplifications and factorizations, for any $(x,y)\in \mathcal{S}$, the following expression is obtained:

$$\begin{array}{cc}\hfill & {\partial }_{x,y}C(x,y;\upsilon )=1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }}\hfill \\ \hfill & +\frac{2\alpha {\beta }^3{(1-x)}^2{(1-y)}^2{x}^{3\gamma -1}{y}^{3\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2}\hfill \\ \hfill & =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2}.\hfill \end{array}$$

Hence, with the use of absolute values, we establish the following inequality:

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge }\hfill \\ \hfill & 1-\frac{\left|\alpha \right|\left|\beta \right|x^{\gamma -1}{y}^{\gamma -1}|\gamma (x-1)+x||\gamma (y-1)+y|\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2}.\hfill \end{array}$$

Since $\gamma \ge 1$ and $(x,y)\in \mathcal{S}$, we have $\gamma (x-1)+x\in [-\gamma ,1]$ and $\gamma (y-1)+y\in [-\gamma ,1]$, so $max\left[\right|\gamma (x-1)+x|,|\gamma (y-1)+y\left|\right]\le max(\gamma ,1)=\gamma$. Furthermore, we have $x^{\gamma -1}{y}^{\gamma -1}\le 1$,

$$1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\le 1+{\beta }^2{m}_{\gamma }^4$$

and

$$1-{\beta }^2{m}_{\gamma }^4\le 1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma },$$

where

$$m_{\gamma }=\underset{x\in [0,1]}{sup}{x}^{\gamma }(1-x)=\frac{1}{\gamma +1}{\left(\frac{\gamma }{\gamma +1}\right)}^{\gamma }.$$

Hence, under the assumption $\beta <1/m_{\gamma }^2$ (strict inequality), we obviously have $1-{\beta }^2{m}_{\gamma }^4>0$. The above inequalities give

$$\begin{array}{cc}\hfill & {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 1-\left|\alpha \right|\beta {\gamma }^2\frac{1+{\beta }^2{m}_{\gamma }^4}{{(1-{\beta }^2{m}_{\gamma }^4)}^2}.}\hfill \end{array}$$

The assumption $\left|\alpha \right|\le {(1-{\beta }^2{m}_{\gamma }^4)}^2/\left[\beta {\gamma }^2(1+{\beta }^2{m}_{\gamma }^4)\right]$ implies that

$$\begin{array}{c}\hfill {\displaystyle {\partial }_{x,y}C(x,y;\upsilon )\ge 0.}\end{array}$$

Item (III) is proved.

The proof of the proposition ends. □

The copula shown in Equation (17) is named the hyperbolic arctangent-FGM (HAT) copula.

Remark 6.

An alternative expression of the HAT copula involving the logarithmic function is

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\frac{\alpha }{2}log\left[\frac{1+\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}{1-\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)}\right],(x,y)\in \mathcal{S}.\end{array}$$

Based on Equation (17), the HAT copula density is calculated as

$$\begin{array}{cc}\hfill & c(x,y;\upsilon )={\partial }_{x,y}C(x,y;\upsilon )\hfill \\ \hfill & =1+\frac{\alpha \beta x^{\gamma -1}{y}^{\gamma -1}[\gamma (x-1)+x][\gamma (y-1)+y]\left[1+{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}{{\left[1-{\beta }^2{(1-x)}^2{(1-y)}^2{x}^{2\gamma }{y}^{2\gamma }\right]}^2},(x,y)\in \mathcal{S}.\hfill \end{array}$$

The HAT survival copula is given by

$$\begin{array}{cc}\hfill \widehat{C}(x,y;\upsilon )& =x+y-1+C(1-x,1-y;\upsilon )\hfill \\ \hfill & =xy+\alpha arctanh\left[\beta {(1-x)}^{\gamma }{(1-y)}^{\gamma }xy\right],(x,y)\in \mathcal{S}.\hfill \end{array}$$

The HAT survival copula is a brand-new three-parameter copula to be added to the corpus of current research.

Some graphical and analytical characteristics of the proposed copulas are the focus of the remaining sections of the article.

3. Graphics and Properties

This section contains a short graphical copula analysis and a unified study of the proposed copulas.

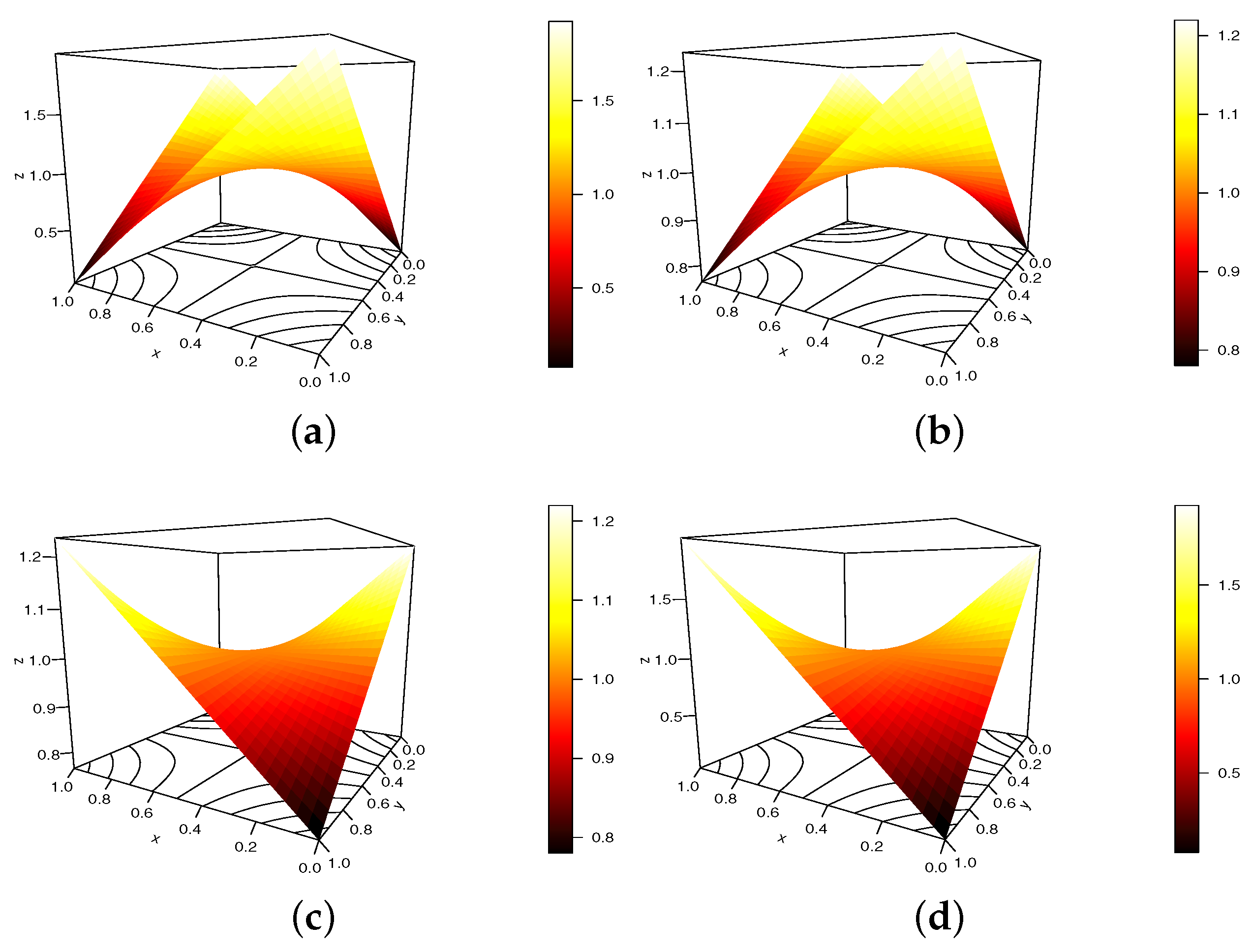
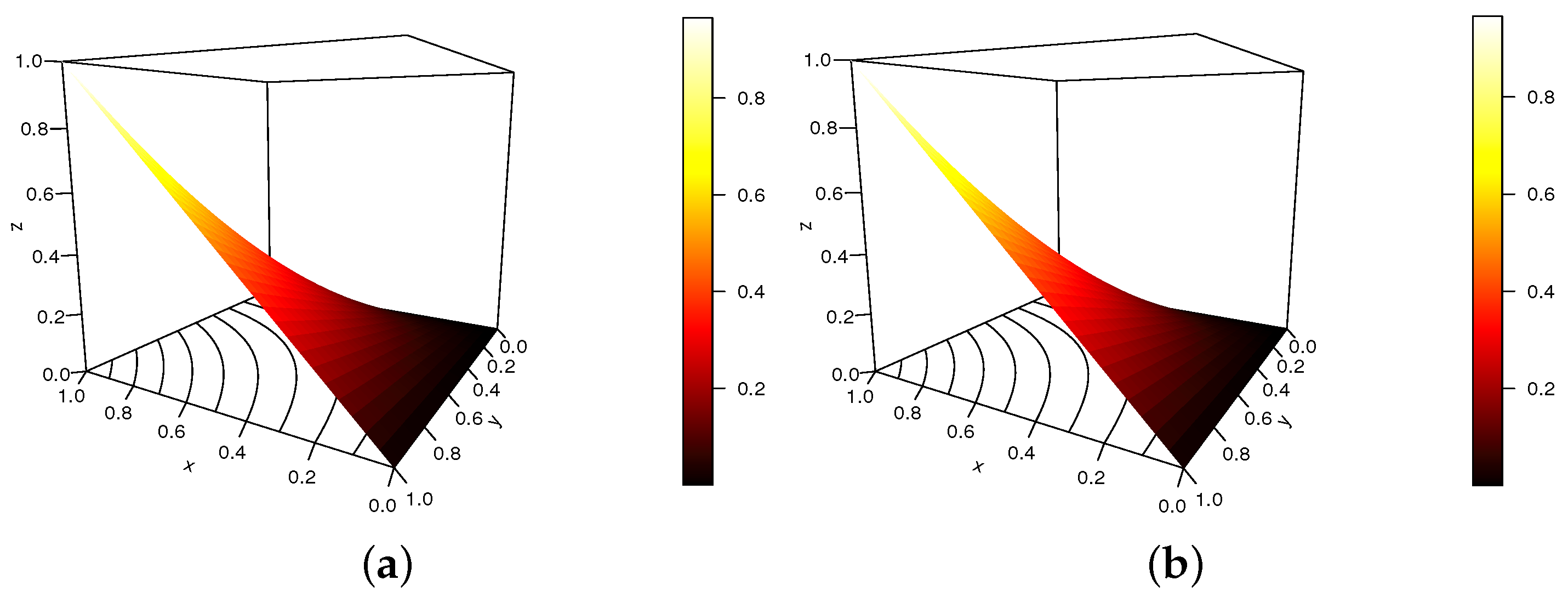
3.1. Graphical Analysis of The S Copula

We examine the shapes of two particular copulas, the S and HAS copulas, in order to emphasize the importance of our findings. After some preliminary tests, certain parameter values will be chosen from the list of acceptable values and according to their impact on the shapes.

We begin with the S copula as defined by (1). First, a simplified configuration is considered: $\gamma =1$, which gives $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)=1/4$. The conditions in Proposition 1, i.e., in Equation (2), yield $\beta \in [0,8\pi ]$ and

$$\begin{array}{c}\hfill \left|\alpha \right|\le \frac{1}{\beta (1+{\beta }^2/256)}.\end{array}$$

Let us now explore the effect of $\alpha$ on the shapes of the S copula and the S copula density, in turn.

For the first analysis, we arbitrarily chose $\beta =\pi /2$. In this case, the condition on $\alpha$ becomes $\left|\alpha \right|\le 0.6305424$.

In Figure 1, we plot the S copula as defined in Equation (1) with $\gamma =1$, $\beta =\pi /2$ and $\alpha \in \{-0.63,-0.15,0.15,0.63\}$, satisfying the required conditions.

We observe that the shapes of the copula are affected by the change in values of $\alpha$, which reveals a certain versatility. Moreover, it is clear that the conditions satisfied by a copula are fulfilled.

Figure 2 completes Figure 1: we plot the S copula density as defined in Equation (3) under the same configuration, i.e., $\gamma =1$, $\beta =\pi /2$ and $\alpha \in \{-0.63,-0.15,0.15,0.63\}$.

Again, we see that the changing values of $\alpha$ have an impact on the morphologies of the shapes. Furthermore, it is evident that the mathematical requirements of a copula density are satisfied, particularly the positivity.

Let us now investigate the effect of $\beta$ on the shapes of the S copula and the S copula density.

We chose $\beta \in [0,4\pi ]$ for numerical convenience. Then, the condition on $\alpha$ becomes $\left|\alpha \right|\le 0.0492$. Hence, we propose to fix $\alpha =0.49$ and vary the values of $\beta$.

In Figure 3, we plot the S copula with $\gamma =1$, $\alpha =0.049$ and $\beta \in \{\pi /2,2\pi ,3\pi ,4\pi \}$, satisfying the required conditions.

The contour plot reveals a difference in lines and a difference in intensity in the red color zone, both of which show that the forms of the S copula are altered; this is evidence that $\beta$ has a shape effect.

In Figure 4, we plot the S copula density under the same configuration, i.e., $\gamma =1$, $\alpha =0.049$ and $\beta \in \{\pi /2,2\pi ,3\pi ,4\pi \}$.

In this figure, the contour plot makes it easier to see the subtle changes in the copula density shapes. However, it is evident that the requirements for a legitimate copula density are met.

We end this graphical analysis with an example of $\gamma =2$. In this case, we have $m_{\gamma }=4/27$, and we can take $\beta$ as $\left|\beta \right|\le 71.5694$. With the arranging choice $\beta =\pi$, we can take $\alpha$ as $\left|\alpha \right|\le 0.07920093$.

In Figure 5, we plot the S copula with $\gamma =2$, $\beta =\pi$ and $\alpha \in \{-0.079,0.05\}$, satisfying the required conditions.

The contour plot and the intensity of the red color zone are the key differences.

Figure 6 completes Figure 5: we plot the S copula density with $\gamma =2$, $\beta =\pi$ and $\alpha \in \{-0.079,0.05\}$.

The shapes completely change in this figure because of the alternated sign. When $\gamma >1$, the restriction $c(0,0;\upsilon )=1$ is applied and may present a challenge for flexible dependence modeling.

3.2. Graphical Analysis of the HAS Copula

One of our most important discoveries is the manageability of the HAS copula as defined in Equation (8), which only requires the following parameter conditions: $\beta \ge 0$, $\gamma \ge 1$ and $\left|\alpha \right|\le 1/\left({\gamma }^2\beta \right)$. For this reason, we propose to study it from a graphical viewpoint.

To begin, let us replace $\beta$ by $\beta =1/\sqrt{\gamma }$ with $\gamma \ge 1$, such that $\left|\alpha \right|\le 1$, to activate all the parameters in a straightforward manner. With this configuration, we define the HAS copula with only two varying parameters, $\gamma$ and $\alpha$, but the values of $\gamma$ have an effect on those of $\beta$.

In Figure 7, we plot the HAS copula with $\gamma =1$, $\beta =1/\sqrt{\gamma }=1$ and $\alpha \in$$\{-0.8,-0.2,0.2,0.8\}$, satisfying the required conditions.

In this figure, especially if we compare Figure 7a,d, we observe a wide change in the shapes of the HAS copula, indicating a significant effect of $\alpha$. This shows the versatility of the HAS copula in terms of shapes.

In Figure 8, we plot the HAS copula density as defined in Equation (10) under the same configuration, i.e., $\gamma =1$, $\beta =1/\sqrt{\gamma }=1$ and $\alpha \in \{-0.8,-0.2,0.2,0.8\}$.

In this figure, positional differences are observed with various intensity values. Once again, the copula density meets the requirement in terms of assumptions, including positivity.

Let us now investigate the effect of $\gamma$ on the shapes of the HAS copula and the HAS copula density, under the configuration $\beta =1/\sqrt{\gamma }$. Let us fix $\alpha =1$ and vary the values of $\gamma$.

In Figure 9, we plot the HAS copula with $\alpha =1$, $\beta =1/\sqrt{\gamma }$ and $\gamma \in \{1,1.5,2,5\}$, satisfying the required conditions.

In this figure, we also observe changes of morphologies, indicating that the copula shapes are dependent on the combined action of $\beta$ and $\gamma$.

In Figure 10, we plot the HAS copula density under the same configuration, i.e., $\alpha =1$, $\beta =1/\sqrt{\gamma }$ and $\gamma \in \{1,1.5,2,5\}$.

In some sense, Figure 10 supports Figure 9; the HAS copula produces a copula density very versatile in shapes.

To wrap up this section, we display figures to show that a few of the proposed copulas have the adaptability to be used in practical settings and produce applicable dependence models. Similar characteristics are seen for the other copulas as well, but for space reasons, we do not include their graphical analysis.

3.3. List of Properties

This section establishes some basic characteristics of the introduced copulas. We work with their general form, which involves the function $\varphi \left(u\right)$, i.e.,

$$\begin{array}{c}\hfill C(x,y;\upsilon )=xy+\alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right],(x,y)\in \mathcal{S},\end{array}$$

with

$$\varphi \left(u\right)\in \{sin(u),arcsin(u),sinh(u),arcsinh(u),tan(u),tanh(u),arctan(u),arctanh(u\left)\right\},$$

and $\beta \ge 0$ and $\gamma \ge 1$ (which are common conditions in all of the preceding propositions). The theoretical details of the coming notions are all contained in [5].

To begin, the independence copula is always obtained by setting $\alpha =0$. The proposed copulas are diagonally symmetric because $C(x,y;\upsilon )=C(y,x;\upsilon )$ for any $(x,y)\in \mathcal{S}$. They are not Archimedean because one can find many examples of triplet values $(a,b,c)$ such that

$$\begin{array}{c}\hfill C\left[a,C\left(b,c;\upsilon \right);\upsilon \right]\ne C\left[C\left(a,b;\upsilon \right),c;\upsilon \right].\end{array}$$

The non-Archimedean property is implied by the non-associativity of $C(x,y;\upsilon )$. Because of the change of the parameter $\gamma$, the considered copulas are not radially symmetric for $\alpha \ne 0$ and $\gamma >1$; there exists $(x,y)$ such that $\widehat{C}(x,y;\upsilon )\ne C(x,y;\upsilon )$. They are radially symmetric for $\alpha \ne 0$ or $\gamma =1$.

Of course, as a well-established copula fact, we have the Fréchet–Hoeffding bounds: for any $(x,y)\in \mathcal{S}$, we have $max(x+y-1,0)\le C(x,y;\upsilon )\le min(x,y)$. As immediate consequences, the following two-dimensional inequalities involving $\alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]$ hold: for any $(x,y)\in \mathcal{S}$, we have

$$\begin{array}{c}\hfill max(x+y-1,0)-xy\le \alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy.\end{array}$$

These inequalities can be of separate interest and serve as mathematical tools for various two-dimensional analyses.

Let us now look at the various quadrant dependence properties in relation to the parameters.

• For $\alpha \ge 0$, it is clear that $\alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\ge 0$, implying that $C(x,y;\upsilon )\ge xy$ for any $(x,y)\in \mathcal{S}$; the considered copulas are positively quadrant dependent.
• For $\alpha \le 0$, it is clear that $\alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le 0$, implying that the reversed inequality holds: $C(x,y;\upsilon )\le xy$ for any $(x,y)\in \mathcal{S}$; the considered copulas are negatively quadrant dependent.

In addition, some first-order copula orders with other copulas can be demonstrated. In particular, if $\varphi \left(u\right)$ satisfies $\varphi \left(u\right)\le u$ for u in the support of $\varphi$, which is the case for $\varphi \left(u\right)\in \{sin(u),arcsinh(u),arctan(u),tanh(u\left)\right\}$, the results below are established.

• For $\alpha \ge 0$, we have $C(x,y;\upsilon )\le C_{†}(x,y;\upsilon )$ for any $(x,y)\in \mathcal{S}$, where

  $$\begin{array}{c}\hfill C_{†}(x,y;\upsilon )=xy+\alpha \beta x^{\gamma }{y}^{\gamma }(1-x)(1-y),(x,y)\in \mathcal{S}.\end{array}$$

  (19)
  It is a copula that is an extended version of the FGM copula with the dependence parameters $\theta =\alpha \beta$ (see [12]).
• For $\alpha \le 0$, the reversed inequality holds: we have $C(x,y;\upsilon )\ge C_{†}(x,y;\upsilon )$ for any $(x,y)\in \mathcal{S}$.

If $\varphi \left(u\right)$ satisfies $\varphi \left(u\right)\ge u$ for u in the support of $\varphi$, which is the case for $\varphi \left(u\right)\in \{arcsin(u),sinh(u),tan(u),arctanh(u\left)\right\}$, the reversed first-order copula orders hold.

The proposed copulas have tractable series expansions based on series expansions of $\varphi \left(u\right)$ with $u\in [0,1)$. Such expansions can express or approximate various crucial correlation measures, which makes them useful in particular situations.

Various kinds of tail dependence for the proposed copulas are examined below. Since all the considered functions $\varphi \left(u\right)$ satisfied $\varphi \left(u\right)\sim u$ when $u\to 0$ and $\gamma \ge 1$, we have

$$\begin{array}{cc}\hfill {\lambda }_{low}& =\underset{x\to 0}{lim}\frac{C(x,x;\upsilon )}{x}=\underset{x\to 0}{lim}\left\{x+\frac{\alpha \varphi \left[\beta x^{2\gamma }{(1-x)}^2\right]}{x}\right\}=\underset{x\to 0}{lim}\left(x+\alpha \beta x^{2\gamma -1}\right)=0.\hfill \end{array}$$

Thus, the considered copulas have no lower tail dependence. Concerning the upper tail dependence, using similar arguments, we have

$$\begin{array}{cc}\hfill {\lambda }_{up}& =\underset{x\to 1}{lim}\frac{1-2x+C(x,x;\upsilon )}{1-x}=\underset{x\to 1}{lim}\frac{1-2x+x^2+\alpha \varphi \left[\beta x^{2\gamma }{(1-x)}^2\right]}{1-x}\hfill \\ \hfill & =\underset{x\to 1}{lim}\left(1-x+\frac{\alpha \varphi \left[\beta x^{2\gamma }{(1-x)}^2\right]}{1-x}\right)=\underset{x\to 1}{lim}\left[1-x+\alpha \beta (1-x)\right]=0.\hfill \end{array}$$

No upper tail dependence is determined. As a result, the suggested copulas are totally tail independent.

There exist many measures of correlation (see [5]). Among them are the medial correlation (or coefficient of Blomqvist) and the Spearman correlation. In our setting, there are investigated below.

The medial correlations of the considered copulas are given by the following general formula:

$$M=4C\left(\frac{1}{2},\frac{1}{2};\upsilon \right)-1=4\alpha \varphi \left(\beta 2^{-2(\gamma +1)}\right).$$

They are expressed for each of the eight copulas in

Table 1. Expressions of the medial correlations and series expansions of the Spearman correlations of the new copulas.

Copulas	Medial Correlations	Spearman Correlations (Series Expansions)
S	${\displaystyle 4\alpha sin\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=0}^{\infty }\frac{{(-1)}^i}{(2i+1)!}{\beta }^i{B}^2\left[\gamma (2i+1)+1,2(i+1)\right]}$
AS	${\displaystyle 4\alpha arcsin\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=0}^{\infty }\frac{\left(2i\right)!}{4^i{(i!)}^2(2i+1)}{\beta }^i{B}^2\left[\gamma (2i+1)+1,2(i+1)\right]}$
HS	${\displaystyle 4\alpha sinh\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=0}^{\infty }\frac{1}{(2i+1)!}{\beta }^i{B}^2\left[\gamma (2i+1)+1,2(i+1)\right]}$
HAS	${\displaystyle 4\alpha arcsinh\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=0}^{\infty }\frac{{(-1)}^i(2i-1)!!}{(2i+1)\left(2i\right)!!}{\beta }^i{B}^2\left[\gamma (2i+1)+1,2(i+1)\right]}$
T	${\displaystyle 4\alpha tan\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=1}^{\infty }\left|{\mathcal{B}}_{2i}\right|\frac{4^i(4^i-1)}{\left(2i\right)!}{\beta }^i{B}^2\left[\gamma (2i-1)+1,2i\right]}$
AT	${\displaystyle 4\alpha arctan\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=0}^{\infty }\frac{{(-1)}^i}{2i+1}{\beta }^i{B}^2\left[\gamma (2i+1)+1,2(i+1)\right]}$
HT	${\displaystyle 4\alpha tanh\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=1}^{\infty }{\mathcal{B}}_{2i}\frac{4^i(4^i-1)}{\left(2i\right)!}{\beta }^i{B}^2\left[\gamma (2i-1)+1,2i\right]}$
HAT	${\displaystyle 4\alpha arctanh\left(\beta 2^{-2(\gamma +1)}\right)}$	${\displaystyle 12\alpha \sum _{i=0}^{\infty }\frac{1}{2i+1}{\beta }^i{B}^2\left[\gamma (2i+1)+1,2(i+1)\right]}$

.

Some medial correlation orders can be established. In particular, if $\varphi \left(u\right)$ satisfies $\varphi \left(u\right)\le u$ for u in the support of $\varphi$, which is the case for $\varphi \left(u\right)\in \{sin(u),arcsinh(u),arctan(u),tanh(u\left)\right\}$, then the results below hold.

• For $\alpha \ge 0$, we have $M\le M_{†}$, where $M_{†}$ denotes the medial correlation of the copula given in Equation (19).
• For $\alpha \le 0$, the reversed inequality holds; we have $M\ge M_{†}$.

If $\varphi \left(u\right)$ satisfies $\varphi \left(u\right)\ge u$ for u in the support of $\varphi$, which is the case for $\varphi \left(u\right)\in \{arcsin(u),sinh(u),tan(u),arctanh(u\left)\right\}$, the reversed medial correlation orders hold.

The Spearman correlations of the considered copulas are specified by the following general formula:

$$\rho =12\int {\int }_{\mathcal{S}}\left[C(x,y;\upsilon )-xy\right]dxdy=12\alpha \int {\int }_{\mathcal{S}}\varphi \left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]dxdy.$$

However, the series expansions for $C(x,y;\upsilon )$ mentioned above can be used to obtain series expansions for $\rho$. These expansions are given in Table 1. The standard beta function is defined in this table as $B(u,v)={\int }_0^1{t}^{u-1}{(1-t)}^{v-1}dt$ with $u>0$ and $v>0$ (implemented in most of the mathematical software), the double factorial as $n!!=n(n-2)(n-4)\dots$ (until 1 or 2, depending on the parity nature of n), and the Bernoulli numbers as ${\mathcal{B}}_{2n}$.

Other well-known copula correlation measures can be expressed in a similar way (Gini gamma, Spearman footrule coefficient, etc.).

Some Spearman correlation orders can be established. In particular, if $\varphi \left(u\right)$ satisfies $\varphi \left(u\right)\le u$ for u in the support of $\varphi$, which is the case for $\varphi \left(u\right)\in \{sin(u),arcsinh(u),arctan(u),tanh(u\left)\right\}$, then the results below follow.

• For $\alpha \ge 0$, we have $\rho \le {\rho }_{†}$, where ${\rho }_{†}$ denotes the Spearman correlation of the copula given in Equation (19).
• For $\alpha \le 0$, the reversed inequality holds: we have $\rho \ge {\rho }_{†}$.

If $\varphi \left(u\right)$ satisfies $\varphi \left(u\right)\ge u$ for u in the support of $\varphi$, which is the case for $\varphi \left(u\right)\in \{arcsin(u),sinh(u),tan(u),arctanh(u\left)\right\}$, the reversed Spearman correlation orders hold. Thus, the values of the Spearman correlations of the proposed copulas can be really versatile and go beyond those of the FGM copula (in the positive or negative sense). This reason, combined with the functionalities of $\varphi \left(u\right)$, justifies the use of these copulas for applications where the FGM copula produces moderate or good results, for possible better modeling.

As a well-known copula fact, the proposed copulas can define new parametric two-dimensional distributions. In fact, by merging two uni-dimensional cumulative distribution functions, say $F\left(x\right)$ and $G\left(x\right)$, we build a new two-dimensional cumulative distribution function as follows:

$$\begin{array}{cc}\hfill H(x,y;\zeta )& =C(F\left(x\right),G\left(y\right);\upsilon )=F\left(x\right)G\left(y\right)+\alpha \varphi \left\{\beta F^{\gamma }\left(x\right)G^{\gamma }\left(y\right)[1-F\left(x\right)][1-G\left(y\right)]\right\},\hfill \\ \hfill & (x,y)\in {\mathbb{R}}^2,\hfill \end{array}$$

where $\zeta$ represents the vector of all the involved parameters, i.e., $\upsilon =(\alpha ,\beta ,\gamma )$ and those in $F\left(x\right)$ and $G\left(x\right)$. In [36], among other topics, the choices for motivated lifetime cumulative distribution functions are covered.

Finally, in a statistical scenario, we often need to estimate the unknown parameter vector $\upsilon =(\alpha ,\beta ,\gamma )$ from data. To this end, as for all copulas, we may think to use the “omnibus estimation method”, which can be described as follows. For a known integer n, let $(x_i,y_i)$, $i=1,\dots ,n$ be data drawn from a continuous random vector, say $(X,Y)$. Then, with the use of the proposed copulas and their respective copula densities, denoted generically here by $c(x,y;\upsilon )$, the omnibus estimate of $\upsilon$ is defined as

$$\widehat{\upsilon }={argmax}_{\upsilon }\sum _{i=1}^{n}log\left\{c\left[\widehat{F}\left(x_i\right),\widehat{G}\left(y_i\right);\upsilon \right]\right\},$$

where

$$\widehat{F}\left(x\right)=\frac{1}{n}\sum _{i=1}^n{1}_{\{x_i\le x\}},\widehat{G}\left(y\right)=\frac{1}{n}\sum _{i=1}^n{1}_{\{y_i\le y\}},$$

and $1_A$ refers to the indicator function over an event denoted as A. Therefore, after substituting the transformed data $(\widehat{F}\left(x_i\right),\widehat{G}\left(y_i\right))$, $i=1,\dots ,n$, the omnibus estimate can be described as a fundamental maximum likelihood estimate of $\upsilon$. This semi-parametric estimation technique aims to prevent potential marginal distribution misspecification, which could lead to an overestimation of the level of dependence in the data. The references [37,38] contain the theoretical guarantees of $\widehat{\upsilon }$. Additional remarks on the perspectives of the applied aspects of the suggested copulas are provided in the section that follows.

4. Conclusions

4.1. Summary

In this article, we produced eight two-dimensional copulas by combining trigonometric and hyperbolic perturbation functions with a FGM copula structure, riding the wave of the emergence of new dependence models. They depend on three parameters, each of which plays an important role in various shape and correlation aspects.

To the best of our knowledge, all of the main copulas described in this article are brand new to the literature. For a summary view, they are listed in

Table 2. Copulas proposed in this article.

Names	Main Copulas	Survival Copulas	Conditions
S	${\displaystyle xy+\alpha sin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha sin\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (2)
AS	${\displaystyle xy+\alpha arcsin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha arcsin\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (5)
HS	${\displaystyle xy+\alpha sinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha sinh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (7)
HAS	${\displaystyle xy+\alpha arcsinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha arcsinh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (9)
T	${\displaystyle xy+\alpha tan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha tan\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (12)
AT	${\displaystyle xy+\alpha arctan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha arctan\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (14)
HT	${\displaystyle xy+\alpha tanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha tanh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (16)
HAT	${\displaystyle xy+\alpha arctanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]}$	${\displaystyle xy+\alpha arctanh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]}$	Equation (18)

.

The trigonometric nature of some new copulas offers interesting perspectives for modeling circular-type dependence (see [31,33,34]). For each of the eight copulas, we identified wide ranges of acceptable parameter values. The proofs were given in full detail. Their capabilities were carefully examined. Figures were used to illustrate the shapes of the S and HAS copulas. Theoretically, the symmetry in general, stochastic dominance, quadrant dependence, tail dependence, Archimedean nature, and correlation measures were investigated. The inference on the parameters was discussed. As a result, the provided collections of copulas enhance the specialized literature and serve as the foundation for fresh dependence models for diverse theoretical or practical uses.

4.2. Limitations

• To obtain a wide range of acceptable values for the three parameters of the suggested copulas, every mathematical effort was made. However, we do not claim that these ranges are optimal in the strict sense. There is probably some (minor) room for improvement, but a solid mathematical basis is given in the article.
• It is worth noting that the article is mainly theory oriented, even though concrete models are provided with all the details necessary to be practically implemented. However, applications to real-world data are missing. This aspect requires experience and skills that go beyond what the author of this study currently have.

4.3. Perspectives

• The applied aspect is the logical sequel of this article. In particular, by their construction, our trigonometric copulas are interesting and logically recommended for the analysis of circular or periodic data types. This is the most recent interpretation of our findings. The creation of R packages for the proposed copula models similar to Cylcop (see [34]) is also a challenging project.
• Based on our two-dimensional copulas, we can consider building copulas of higher dimensions, particularly three-dimensional copulas, given their potential applications in diverse environmental models, etc. (see [39,40,41]). A natural three-dimensional extended form is

  $$\begin{array}{c}\hfill C(x,y,z;\upsilon )=xyz+\alpha \varphi \left[\beta x^{\gamma }{y}^{\gamma }{z}^{\gamma }(1-x)(1-y)(1-z)\right],(x,y,z)\in \mathcal{S}\times [0,1],\end{array}$$

  with

  $$\varphi \left(u\right)\in \{sin(u),arcsin(u),sinh(u),arcsinh(u),tan(u),tanh(u),arctan(u),arctanh(u\left)\right\},$$

  and $\beta \ge 0$ and $\gamma \ge 1$.

These points need further investigation, which we will leave for future work.

4.4. Extra Remark

Finally,

Table 3. Inequalities derived from the proposed copulas (and survival copulas).

Copulas	Inequalities $((\mathit{x},\mathit{y})\in {[0,1]}^2)$	Conditions
S	${\displaystyle max(x+y-1,0)-xy\le \alpha sin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (2)
surv. S	${\displaystyle max(x+y-1,0)-xy\le \alpha sin\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (2)
AS	${\displaystyle max(x+y-1,0)-xy\le \alpha arcsin\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (5)
surv. AS	${\displaystyle max(x+y-1,0)-xy\le \alpha arcsin\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (5)
HS	${\displaystyle max(x+y-1,0)-xy\le \alpha sinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (7)
surv. HS	${\displaystyle max(x+y-1,0)-xy\le \alpha sinh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (7)
HAS	${\displaystyle max(x+y-1,0)-xy\le \alpha arcsinh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (9)
surv. HAS	${\displaystyle max(x+y-1,0)-xy\le \alpha arcsinh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (9)
T	${\displaystyle max(x+y-1,0)-xy\le \alpha tan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (12)
surv. T	${\displaystyle max(x+y-1,0)-xy\le \alpha tan\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (12)
AT	${\displaystyle max(x+y-1,0)-xy\le \alpha arctan\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (14)
surv. AT	${\displaystyle max(x+y-1,0)-xy\le \alpha arctan\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (14)
HT	${\displaystyle max(x+y-1,0)-xy\le \alpha tanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (16)
surv. HT	${\displaystyle max(x+y-1,0)-xy\le \alpha tanh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (16)
HAT	${\displaystyle max(x+y-1,0)-xy\le \alpha arctanh\left[\beta x^{\gamma }{y}^{\gamma }(1-x)(1-y)\right]\le min(x,y)-xy}$	Equation (18)
surv. HAT	${\displaystyle max(x+y-1,0)-xy\le \alpha arctanh\left[\beta xy{(1-x)}^{\gamma }{(1-y)}^{\gamma }\right]\le min(x,y)-xy}$	Equation (18)

shows some inequalities resulting from our copulas and survival copulas, and the associated Fréchet–Hoeffding bounds. To the best of our knowledge, these inequalities are new and might be of independent interest. We recall that, as minimum conditions, $\beta \ge 0$, and $\gamma \ge 1$, and that $m_{\gamma }={\left[\gamma /\left(\gamma +1\right)\right]}^{\gamma }/(\gamma +1)$.