One-Dimensional Matter Waves as a Multi-State Bit
Abstract
1. Introduction
2. Model and Methods
2.1. Optical Potentials
- The potential is employed in order to keep stable over time. To this end, we consider two possibilities: a disodered potential and a periodic potential .The disordered case. The potential is produced by generating an optical speckle , with intensity and autocorrelation length [25,38]. The probability distribution of is . Moreover, it holds that:An optical speckle is obtained by transmitting a laser beam through a medium with a random phase profile, such as a ground glass plate. The resulting complex electric field is a sum of independent random variables and forms a Gaussian process. Atoms experience a random potential proportional to the intensity of the field. can be either positive or negative, the potential resulting in a series of barriers or wells. However, in both cases, it is possible to observe Anderson localization phenomena [26,27]. The autocorrelation length represents a natural scale for the system and is the corresponding energy scale. We define:where is a rescaled dimensionless intensity. The speckle pattern can be generated numerically as discussed in [25] (and references therein).The ordered case. A smooth, periodic potential can be used as well to maintain as stable over time, depending on the considered .where (), , and . Adopting a common notation, stands for the remainder of .In Section 4, we consider as a realistic case.
- can be obtained from by doubling the period and considering a different amplitude , which is a parameter independent from .where the same requirements described above hold. In Section 4, we consider as a realisitic case.
- Additionally, the third potential is smooth and periodic, and in antiphase with :In the following, we will always consider only.
- The initial condition must be localized around such that . This can be achieved by forcing the BEC to the ground state of a properly chosen optical potential . In Section 3, we consider:where is a constant, dimensioned as , and valued as .
2.2. The Instantaneous Potential Switch and Alternate Assumptions
2.3. Measure of System’s State and Stability
2.4. Numerical Methods
- in case the system is strongly self-interacting, the midpoint integration scheme is still applicable, while the standard Crank–Nicolson scheme is replaced by a modified version that is well defined also in the nonlinear case [43].
3. How to Use the System as a Multi-State Bit
3.1. Reading Information from the Position of a Localized Matter Wave
3.2. Writing and Maintaining Information in the System
3.2.1. Definition of
3.2.2. Definition of
3.2.3. Near to the Boundaries
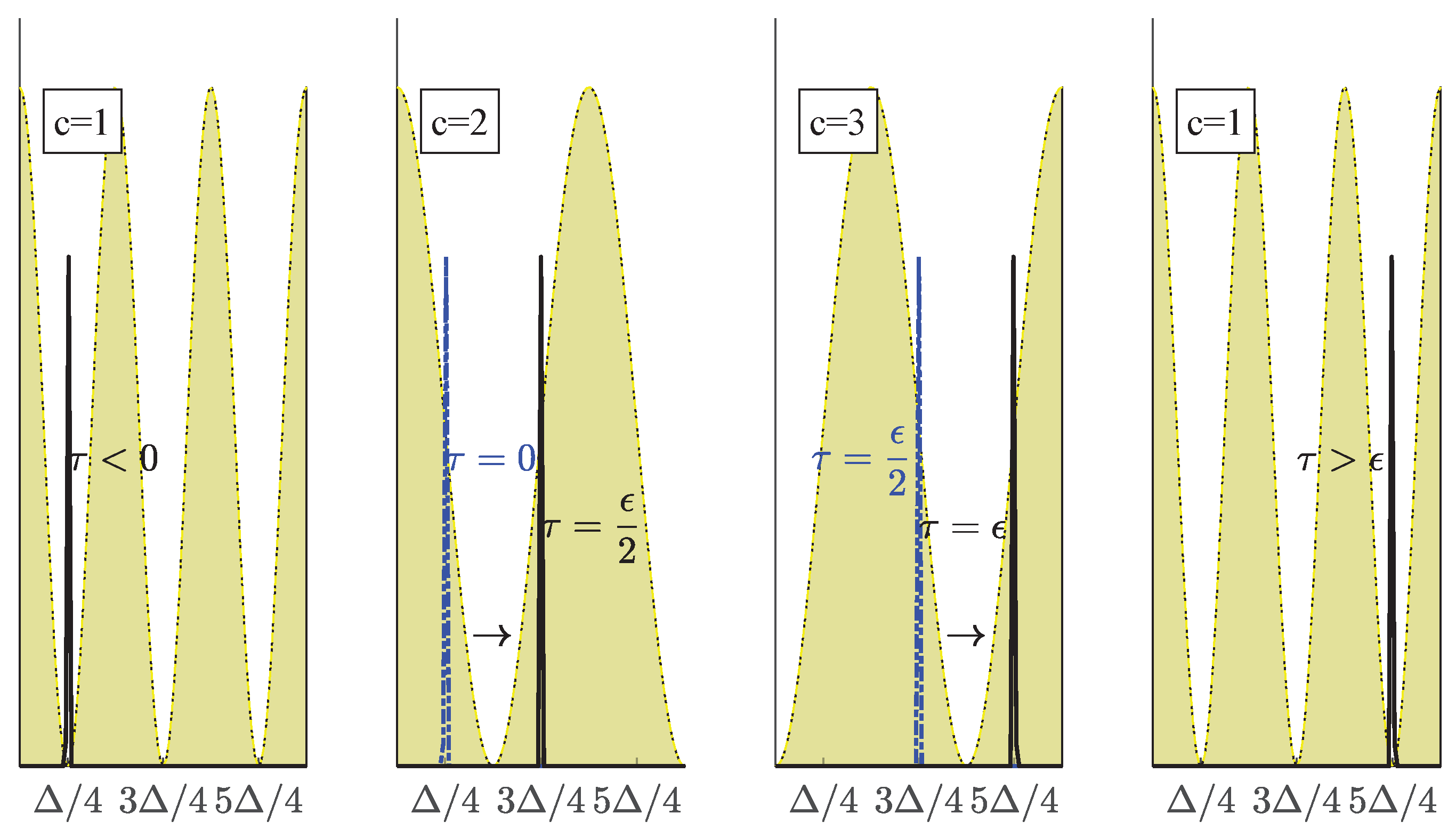

3.2.4. Time Scale of Writing Operations
4. Stability and Robustness of the Multi-State Bit
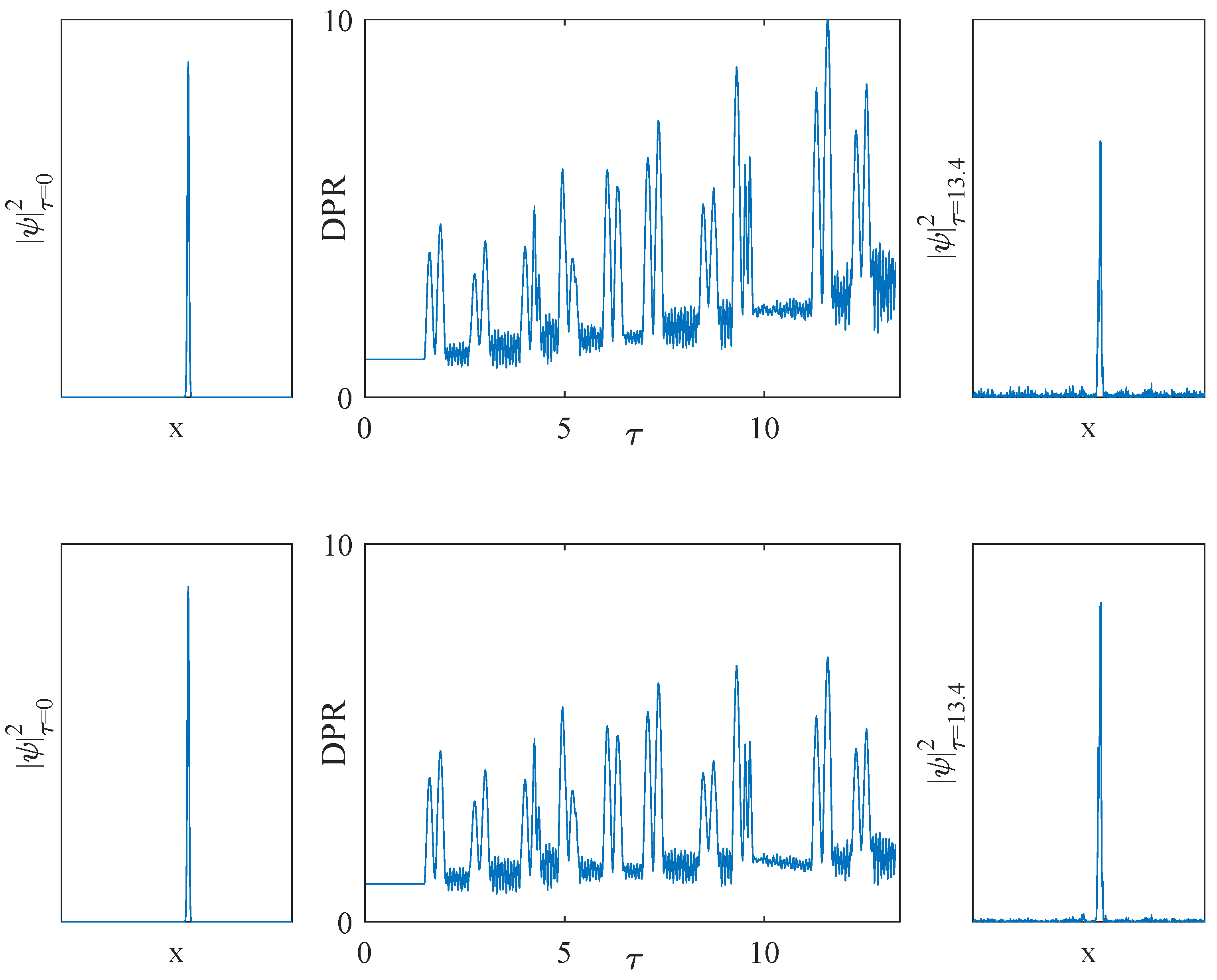
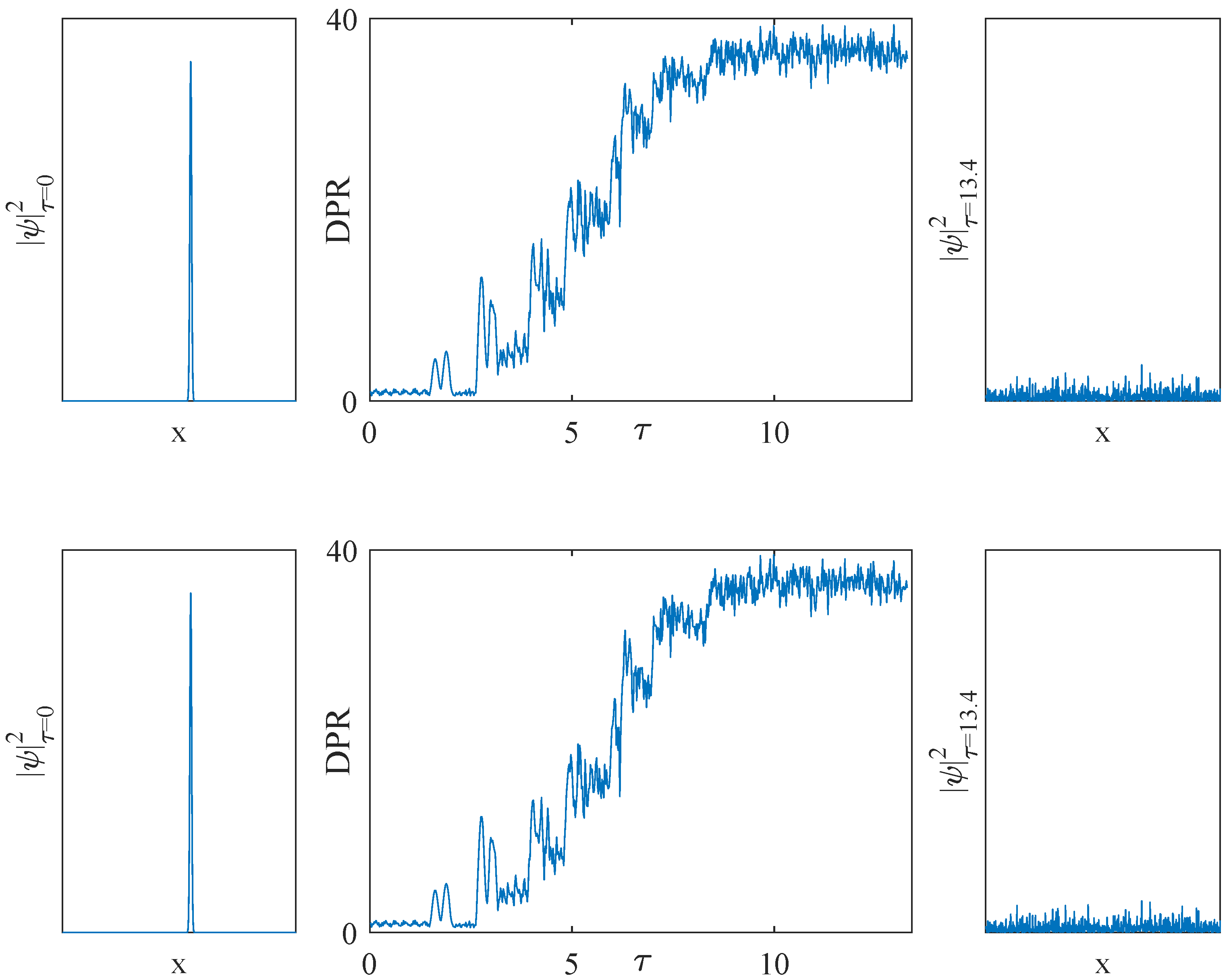
4.1. Multi-State Bit under Various Settings
4.2. Multi-State Bit Stability against Potential Imperfections
5. Summary
Funding
Acknowledgments
Conflicts of Interest
Appendix A. 1D NPSE in Our Units
References
- Anderson, M.H.; Ensher, J.R.; Matthews, M.R.; Weiman, C.E.; Cornell, E.A. Observation of Bose-Einstein condensation in a dilute atomic vapor. Science 1995, 269, 198. [Google Scholar] [CrossRef] [PubMed]
- Davis, K.B.; Mewes, M.O.; Andrews, M.R.; van Druten, N.J.; Durfee, D.S.; Kurn, D.M.; Ketterle, W. Bose-Einstein Condensation in a Gas of Sodium Atoms. Phys. Rev. Lett. 1995, 75, 3969. [Google Scholar] [CrossRef] [PubMed]
- Jin, D.S.; Ensher, J.R.; Matthews, M.R.; Wieman, C.E.; Cornell, E.A. Collective Excitations of a Bose-Einstein Condensate in a Dilute Gas. Phys. Rev. Lett. 1996, 77, 420. [Google Scholar] [CrossRef] [PubMed]
- Inguscio, M. Bose-Einstein condensation. A new trick of the trade. Science 2001, 292, 452. [Google Scholar] [CrossRef]
- Fort, C.; Minardi, F.; Modugno, M.; Inguscio, M. Recent Advances in Metrology and Fundamental Constants; IOS Press: Amsterdam, The Netherlands, 2001; Volume 146, p. 765. [Google Scholar]
- Ferlaino, F.; Maddaloni, P.; Burger, S.; Cataliotti, F.S.; Fort, C.; Modugno, M.; Inguscio, M. Dynamics of a Bose-Einstein condensate at finite temperature in an atomoptical coherence filter. Phys. Rev. A 2002, 66, 011604. [Google Scholar] [CrossRef]
- Henderson, K.; Ryu, C.; MacCormick, C.; Boshier, M.G. Experimental demonstration of painting arbitrary and dynamic potentials for Bose–Einstein condensates. New J. Phys. 2009, 11, 043030. [Google Scholar] [CrossRef]
- Abdullaev, F.K.; Galimzyanov, R.M.; Ismatullaev, K.N. Collective excitations of a BEC under anharmonic trap position jittering. J. Phys. B 2008, 41, 015301. [Google Scholar] [CrossRef]
- Girardeau, M.D.; Wright, E.M.; Triscari, J.M. Ground-state properties of a one-dimensional system of hard-core bosons in a harmonic trap. Phys. Rev. A 2001, 63, 033601. [Google Scholar] [CrossRef]
- Zhang, X.; Yang, Q.; Zhang, J.; Chen, X.Z.; Liu, W.M. Controlling soliton interactions in Bose-Einstein condensates by synchronizing the Feshbach resonance and harmonic trap. Phys. Rev. A 2008, 77, 023613. [Google Scholar] [CrossRef]
- Cataliotti, F.S.; Fallani, L.; Ferlaino, F.; Fort, C.; Maddaloni, P.; Inguscio, M. Dynamics of a trapped Bose–Einstein condensate in the presence of a one-dimensional optical lattice. J. Opt. B 2003, 5, 571. [Google Scholar] [CrossRef]
- Fort, C.; Cataliotti, F.S.; Fallani, L.; Ferlaino, F.; Maddaloni, P.; Inguscio, M. Collective excitations of a trapped Bose-Einstein condensate in the presence of a 1D optical lattice. Phys. Rev. Lett. 2003, 90, 140405. [Google Scholar] [CrossRef] [PubMed]
- Fallani, L.; Sarlo, L.D.; Lye, J.E.; Modugno, M.; Saers, R.; Fort, C.; Inguscio, M. Observation of Dynamical Instability for a Bose-Einstein Condensate in a Moving 1D Optical Lattice. Phys. Rev. Lett. 2004, 93, 140406. [Google Scholar] [CrossRef] [PubMed]
- Ferlaino, F.; Mirandes, E.D.; Heidemann, R.; Roati, G.; Modugno, G.; Inguscio, M. Quasi-2D Fermi-Bose mixture in an optical lattice. J. Phys. IV 2004, 116, 253. [Google Scholar] [CrossRef]
- Meyrath, T.P.; Schreck, F.; Hanssen, J.L.; Chuu, C.S.; Raizen, M.G. Bose-Einstein condensate in a box. Phys. Rev. A 2005, 71, 041604(R). [Google Scholar] [CrossRef]
- Fallani, L.; Fort, C.; Inguscio, M. Bose–Einstein Condensates in Disordered Potentials. Adv. At. Mol. Opt. Phys. 2008, 56, 119. [Google Scholar]
- Shapiro, B. Cold atoms in the presence of disorder. J. Phys. A 2012, 45, 143001. [Google Scholar] [CrossRef]
- Sanchez-Palencia, L.; Lewenstein, M. Disordered quantum gases under control. Nat. Phys. 2010, 6, 87–95. [Google Scholar] [CrossRef]
- Modugno, G. Anderson localization in Bose–Einstein condensates. Rep. Prog. Phys. 2010, 73, 102401. [Google Scholar] [CrossRef]
- Damski, B.; Zakrzewski, J.; Santos, L.; Zoller, P.; Lewenstein, M. Atomic Bose and Anderson glasses in optical lattices. Phys. Rev. Lett. 2003, 91, 080403. [Google Scholar] [CrossRef]
- Lye, J.E.; Fallani, L.; Modugno, M.; Wiersma, D.S.; Fort, C.; Inguscio, M. Bose-Einstein Condensate in a Random Potential. Phys. Rev. Lett. 2005, 95, 070401. [Google Scholar] [CrossRef]
- Fort, C.; Fallani, L.; Guarrera, V.; Lye, J.E.; Modugno, M.; Wiersma, D.S.; Inguscio, M. Effect of Optical Disorder and Single Defects on the Expansion of a Bose-Einstein Condensate in a One-Dimensional Waveguide. Phys. Rev. Lett. 2005, 95, 170410. [Google Scholar] [CrossRef] [PubMed]
- Clement, D.; Varon, A.F.; Retter, J.A.; Sanchez-Palencia, L.; Aspect, A.; Bouyer, P. Experimental study of the transport of coherent interacting matter-waves in a 1D random potential induced by laser speckle. New J. Phys. 2006, 8, 165. [Google Scholar] [CrossRef]
- Ramanathan, A.; Wright, K.C.; Muniz, S.R.; Zelan, M.; Hill, W.T.; Lobb, C.J.; Helmerson, K.; Phillips, W.D.; Campbell, G.K. Superflow in a Toroidal Bose-Einstein Condensate: An Atom Circuit with a Tunable Weak Link. Phys. Rev. Lett. 2011, 106, 130401. [Google Scholar] [CrossRef] [PubMed]
- Modugno, M. Collective dynamics and expansion of a Bose-Einstein condensate in a random potential. Phys. Rev. A 2006, 73, 013606. [Google Scholar] [CrossRef]
- Falco, G.M.; Fedorenko, A.A.; Giacomelli, J.; Modugno, M. Density of states in an optical speckle potential. Phys. Rev. A 2010, 82, 053405. [Google Scholar] [CrossRef]
- Giacomelli, J. Localization properties of one-dimensional speckle potentials in a box. Physica A 2014, 404, 158. [Google Scholar] [CrossRef][Green Version]
- Khaykovich, L.; Schreck, F.; Ferrari, G.; Bourdel, T.; Cubizolles, J.; Carr, L.D.; Castin, Y.; Salomon, C. Formation of a Matter-Wave Bright Soliton. Science 2002, 296, 1290. [Google Scholar] [CrossRef]
- Strecker, K.E.; Partridge, G.B.; Truscott, A.G.; Hulet, R.G. Formation and propagation of matter wave soliton trains. Nature 2002, 417, 150. [Google Scholar] [CrossRef]
- Roati, G.; Zaccanti, M.; D’Errico, C.; Catani, J.; Modugno, M.; Simoni, A.; Inguscio, M.; Modugno, G. 39K Bose-Einstein Condensate with Tunable Interactions. Phys. Rev. Lett. 2007, 99, 010403. [Google Scholar] [CrossRef]
- Calarco, T.; Dorner, U.; Julienne, P.; Williams, C.; Zoller, P. Quantum computations with atoms in optical lattices: Marker qubits and molecular interactions. Phys. Rev. A 2004, 70, 012306. [Google Scholar] [CrossRef]
- Ahufinger, V.; Mebrahtu, A.; Corbalan, R.; Sanpera, A. Quantum switches and quantum memories for matter-wave lattice solitons. New J. Phys. 2007, 9, 4. [Google Scholar] [CrossRef]
- Wang, Z.M.; Wu, L.A.; Modugno, M.; Byrd, M.S.; Yu, T.; You, J.Q. Fault-tolerant breathing pattern in optical lattices as a dynamical quantum memory. Phys. Rev. A 2014, 89, 042326. [Google Scholar] [CrossRef]
- Salasnich, L.; Parola, A.; Reatto, L. Effective wave equations for the dynamics of cigar-shaped and disk-shaped Bose condensates. Phys. Rev. A 2002, 65, 043614. [Google Scholar] [CrossRef]
- Stringari, S.; Pitaevskii, L. Bose-Einstein Condensation; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Wu, B.; Niu, Q. Landau and dynamical instabilities of the superflow of Bose-Einstein condensates in optical lattices. Phys. Rev. A 2001, 64, 061603. [Google Scholar] [CrossRef]
- Smerzi, A.; Trombettoni, A.; Kevrekidis, P.G.; Bishop, A.R. Dynamical Superfluid-Insulator Transition in a Chain of Weakly Coupled Bose-Einstein Condensates. Phys. Rev. Lett. 2002, 89, 170402. [Google Scholar] [CrossRef]
- Goodman, J.W. Speckle Phenomena in Optics: Theory and Applications; Roberts and Company Publishers: Greenwood Village, CO, USA, 2005. [Google Scholar]
- Evers, F.; Mirlin, A.D. Fluctuations of the Inverse Participation Ratio at the Anderson Transition. Phys. Rev. Lett. 2000, 84, 3690. [Google Scholar] [CrossRef]
- Motta, M.; Sun, C.; Tan, A.T.; O′Rourke, M.J.; Ye, E.; Minnich, A.J.; Brandão, F.G.; Chan, G.K.-L. Determining eigenstates and thermal states on a quantum computer using quantum imaginary time evolution. Nat. Phys. 2020, 16, 205. [Google Scholar] [CrossRef]
- Crank, J.; Nicolson, P. A practical method for numerical evaluation of solutions of partial differential equations of the heat conduction type. Math. Proc. Camb. Philos. Soc. 1947, 43, 50. [Google Scholar] [CrossRef]
- Burden, R.L.; Faires, J.D. Numerical Analysis; Brooks/Cole—Cengage Learning: Boston, MA, USA, 2010. [Google Scholar]
- Choy, Y.Y.; Tan, W.N.; Tay, K.G.; Ong, C.T. Crank-Nicolson implicit method for the nonlinear Schrodinger equation with variable coefficient. AIP Conf. Proc. 2014, 1605, 76. [Google Scholar]
- Sackett, C.A.; Kielpinski, D.; King, B.E.; Langer, C.; Meyer, V.; Myatt, C.J.; Rowe, M.; Turchette, Q.A.; Itano, W.M.; Wineland, D.J.; et al. Experimental entanglement of four particles. Nature 2000, 404, 256. [Google Scholar] [CrossRef]
- Rohde, H.; Gulde, S.T.; Roos, C.F.; Barton, P.A.; Leibfried, D.; Eschner, J.; Schmidt-Kaler, F.; Blatt, R. Sympathetic ground state cooling and coherent manipulation with two-ion-crystals. J. Opt. B 2001, 3, 34. [Google Scholar] [CrossRef]
- Paredes, B.; Fedichev, P.; Cirac, J.I.; Zoller, P. ½-Anyons in Small Atomic Bose-Einstein Condensates. Phys. Rev. Lett. 2001, 87, 010402. [Google Scholar] [CrossRef] [PubMed]



| i | Transient | |
|---|---|---|
| 1 |  | |
| 2 | 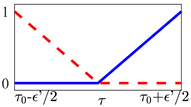 | |
| 3 | 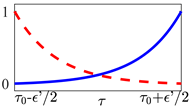 | |
| 4 |  | |
| 5 | 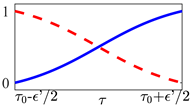 | |
| 6 |  |
| (K Atoms) | [m] | (s) | |
|---|---|---|---|
| 30 | 90 | ∼ | |
| 6 | 200 | ∼ | |
| 3 | 400 | ∼ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giacomelli, J. One-Dimensional Matter Waves as a Multi-State Bit. AppliedMath 2022, 2, 143-158. https://doi.org/10.3390/appliedmath2010008
Giacomelli J. One-Dimensional Matter Waves as a Multi-State Bit. AppliedMath. 2022; 2(1):143-158. https://doi.org/10.3390/appliedmath2010008
Chicago/Turabian StyleGiacomelli, Jacopo. 2022. "One-Dimensional Matter Waves as a Multi-State Bit" AppliedMath 2, no. 1: 143-158. https://doi.org/10.3390/appliedmath2010008
APA StyleGiacomelli, J. (2022). One-Dimensional Matter Waves as a Multi-State Bit. AppliedMath, 2(1), 143-158. https://doi.org/10.3390/appliedmath2010008






