Foundations of Electromagnetism: A Review of Wilhelm Weber’s Electrodynamic Force Law
Abstract
1. Introduction
2. Fundamentals of Weber’s Theory
3. Literature Review
3.1. Two Different Theories of Electrodynamics
3.1.1. Maxwell’s Equations and Field Theory
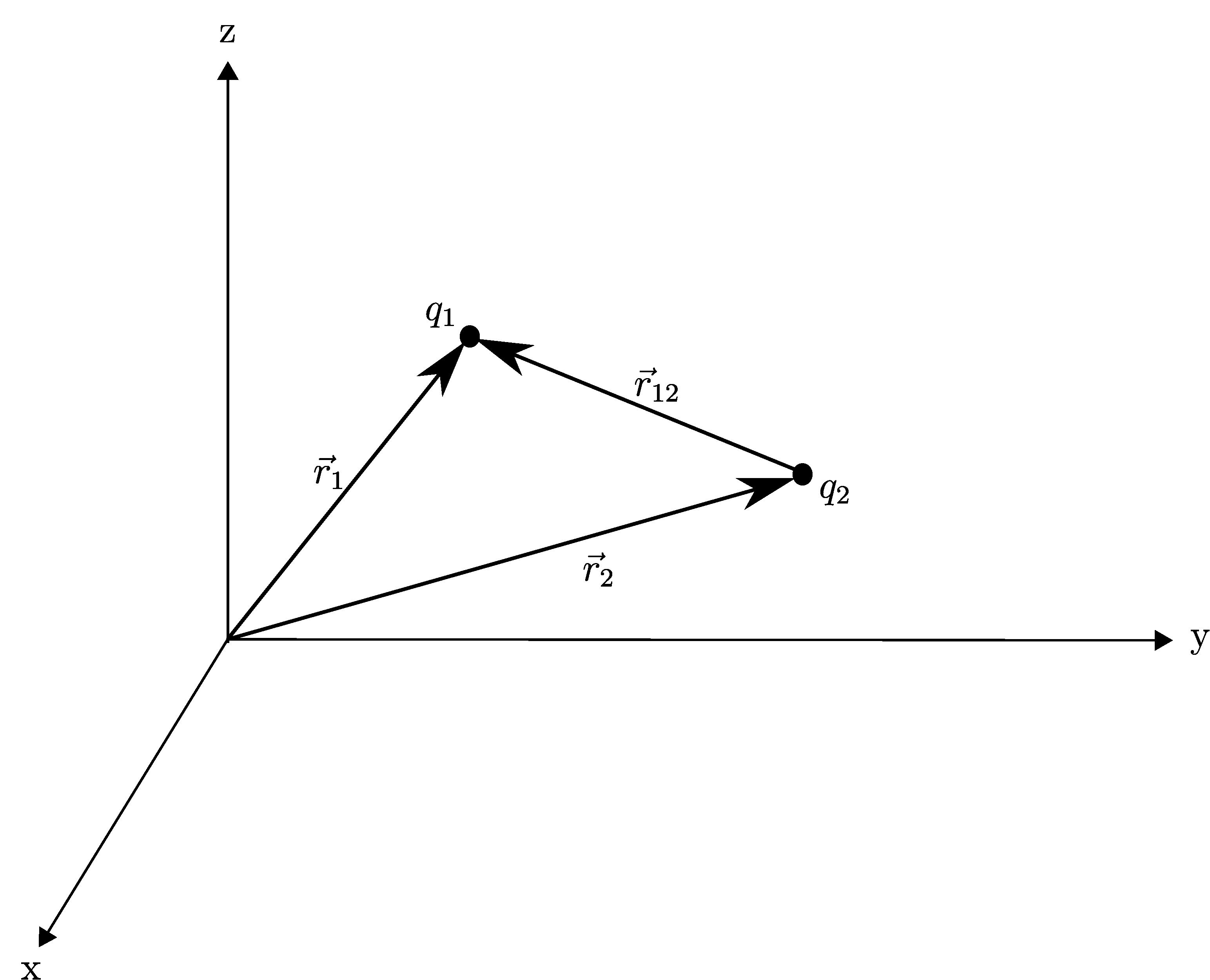
3.1.2. Weber’s Theory of Electrodynamics
3.2. Weber Electrodynamics in the Literature
3.2.1. Electromagnetic Phenomena
3.2.2. Relevance of Weber’s Force Beyond Electromagnetism
3.2.3. Cosmology and Breakthrough Physics
3.3. Criticism of Weber’s Theory
4. Perspective and Future Prospects
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lykken, J.; Spiropulu, M. Supersymmetry and the Crisis in Physics. Sci. Am. 2014, 310, 34–39. [Google Scholar] [CrossRef]
- Crowther, K. Defining a crisis: The roles of principles in the search for a theory of quantum gravity. Synthese 2021, 198, 3489–3516. [Google Scholar] [CrossRef]
- Meucci, S. Physics has Evolved Beyond the Physical. Cosm. Hist. J. Nat. Soc. Philos. 2020, 16, 452–465. [Google Scholar]
- Hossenfelder, S. Lost in Math: How Beauty Leads Physics Astray; Hachette: Paris, France, 2018. [Google Scholar]
- Kinzer, E.; Fukai, J. Weber’s force and Maxwell’s equations. Found. Phys. Lett. 1996, 9, 457–461. [Google Scholar] [CrossRef]
- Assis, A.K.T. Weber’s Electrodynamics; Springer: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Gray, R.W. Candid Remarks On Introductory Classical Electrodynamics and Textbooks. Preprint 2020, 1–27. [Google Scholar] [CrossRef]
- Anonymous. Advances in Weber and Maxwell Electrodynamics; Amazon Fulfillment: Wroclaw, Poland, 2018. [Google Scholar]
- Wesley, J.P. Weber electrodynamics, Part I—General theory, steady current effects. Found. Phys. Lett. 1990, 3, 443–469. [Google Scholar] [CrossRef]
- Holzmüller, G. Über die Anwendung der Jacobi-Hamilton’schen Methode auf den Fall der Anziehung nach dem Electrodynamischen Gesetze von Weber; Universität Halle-Wittenberg: Halle, Germany, 1870. [Google Scholar]
- Torres-Silva, H.; López-Bonilla, J.; López-Vázquez, R.; Rivera-Rebolledo, J. Weber’s electrodynamics for the hydrogen atom. Indones. J. Appl. Phys. 2015, 5, 39–46. [Google Scholar] [CrossRef]
- Frauenfelder, U.; Weber, J. The fine structure of Weber’s hydrogen atom: Bohr–Sommerfeld approach. Z. Angew. Math. Phys. 2019, 70, 105. [Google Scholar] [CrossRef]
- Sokolskii, A.; Sadovnikov, A. Lagrangian Solutions for Weber’s Law of Attraction. Sov. Astron. 1987, 31, 90. [Google Scholar]
- Moon, P.; Spencer, D.E.; Mirchandaney, A.S.; Shama, U.Y.; Mann, P.J. The Electrodynamics of Gauss, Neumann, and Hertz. Phys. Essays 1994, 7, 28–33. [Google Scholar] [CrossRef]
- Lorentz, H.A. La Théorie Électromagnétique de Maxwell et son Application aux Corps Mouvants; EJ Brill: Leiden, The Netherlands, 1892; Volume 25. [Google Scholar]
- McCormmach, R. HA Lorentz and the electromagnetic view of nature. Isis 1970, 61, 459–497. [Google Scholar] [CrossRef]
- Woodruff, A.E. The contributions of Hermann von Helmholtz to electrodynamics. Isis 1968, 59, 300–311. [Google Scholar] [CrossRef]
- Martínez, A.A. Ritz, Einstein, and the emission hypothesis. Phys. Perspect. 2004, 6, 4–28. [Google Scholar] [CrossRef]
- Moon, P.; Spencer, D.E. A new electrodynamics. J. Frankl. Inst. 1954, 257, 369–382. [Google Scholar] [CrossRef]
- Wheeler, J.A.; Feynman, R.P. Interaction with the absorber as the mechanism of radiation. Rev. Mod. Phys. 1945, 17, 157. [Google Scholar] [CrossRef]
- Wheeler, J.A.; Feynman, R.P. Classical electrodynamics in terms of direct interparticle action. Rev. Mod. Phys. 1949, 21, 425. [Google Scholar] [CrossRef]
- Maxwell, J.C. A Treatise on Electricity and Magnetism Unabridged; Dover: New York, NY, USA, 1954. [Google Scholar]
- Yaghjian, A.D. Reflections on Maxwell’s treatise. Prog. Electromagn. Res. 2014, 149, 217–249. [Google Scholar] [CrossRef]
- Assis, A.K.T.; Silva, H.T. Comparison between Weber’s electrodynamics and classical electrodynamics. Pramana 2000, 55, 393–404. [Google Scholar] [CrossRef]
- O’Rahilly, A. Electromagnetic Theory: A Critical Examination of Fundamentals; Dover Publications: New York, NY, USA, 1965; Volumes I and II. [Google Scholar]
- Jackson, J.D. Classical Electrodynamics; Springer Science & Business Media: New York, NY, USA, 1999. [Google Scholar]
- Darwin, C.G. LI. The dynamical motions of charged particles. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1920, 39, 537–551. [Google Scholar] [CrossRef]
- Landau, L.; Lifshitz, E. Electrodynamics of Continuous Media, 2nd ed.; Pergamon: Oxford, UK, 1985. [Google Scholar]
- Page, L.; Adams, N.I., Jr. Action and reaction between moving charges. Am. J. Phys. 1945, 13, 141–147. [Google Scholar] [CrossRef]
- Steane, A.M. Nonexistence of the self-accelerating dipole and related questions. Phys. Rev. D 2014, 89, 125006. [Google Scholar] [CrossRef]
- Cornille, P. The Lorentz force and Newton’s third principle. Can. J. Phys. 1995, 73, 619–625. [Google Scholar] [CrossRef]
- Cornille, P. Review of the application of Newton’s third law in physics. Prog. Energy Combust. Sci. 1999, 25, 161–210. [Google Scholar] [CrossRef]
- Sebens, C.T. Forces on fields. Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 2018, 63, 1–11. [Google Scholar] [CrossRef]
- Assis, A.; Peixoto, F. On the velocity in the Lorentz force law. Phys. Teach. 1992, 30, 480–483. [Google Scholar] [CrossRef][Green Version]
- Tran, M. Evidence for Maxwell’s equations, fields, force laws and alternative theories of classical electrodynamics. Eur. J. Phys. 2018, 39, 063001. [Google Scholar] [CrossRef]
- Pietsch, W. On conceptual issues in classical electrodynamics: Prospects and problems of an action-at-a-distance interpretation. Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 2010, 41, 67–77. [Google Scholar] [CrossRef]
- Lazarovici, D. Against fields. Eur. J. Philos. Sci. 2018, 8, 145–170. [Google Scholar] [CrossRef]
- Muller, F.A. Inconsistency in classical electrodynamics? Philos. Sci. 2007, 74, 253–277. [Google Scholar] [CrossRef]
- Frisch, M. Inconsistency, Asymmetry, and Non-Locality: A Philosophical Investigation of Classical Electrodynamics; Oxford University Press: New York, NY, USA, 2005. [Google Scholar]
- Feynman, R.P. Space-time approach to quantum electrodynamics. In Selected Papers of Richard Feynman; Brown, L.M., Ed.; World Scientific: Singapore, 2000; pp. 99–119. [Google Scholar]
- Dirac, P.A.M. The evolution of the physicist’s picture of nature. Sci. Am. 1963, 208, 45–53. [Google Scholar] [CrossRef]
- Kastner, R.E. Haag’s Theorem as a Reason to Reconsider Direct-Action Theories. arXiv 2015, arXiv:1502.03814. [Google Scholar]
- Griffiths, D.J. Introduction to Electrodynamics; Pearson Prentice Hall: Hoboken, NJ, USA, 2005. [Google Scholar]
- Cullwick, E.G. Electromagnetism and Relativity: With Particular Reference to Moving Media and Electromagnetic Induction; Longmans: London, UK, 1959. [Google Scholar]
- Moon, P.H.; Spencer, D.E. Foundations of Electrodynamics; Boston Technical Publishers Inc: Cambridge, MA, USA, 1965. [Google Scholar]
- Lorrain, P.; Corson, D.R. Electromagnetic Fields and Waves; Courier Corporation: North Chelmsford, MA, USA, 1970. [Google Scholar]
- Maxwell, J.C. XXV. On physical lines of force: Part I—the theory of molecular vortices applied to magnetic phenomena. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1861, 21, 161–175. [Google Scholar] [CrossRef]
- Weber, W.E. Wilhelm Weber’s Werke; First Part; Julius Springer: Berlin, Germany, 1893; Volume 3. [Google Scholar]
- Weber, W.E. Wilhelm Weber’s Werke; Second Part; Julius Springer: Berlin, Germany, 1893; Volume 4. [Google Scholar]
- Weber, W. Determinations of Electrodynamic Measure: Concerning a Universal Law of Electrical Action; 21st Century Science and Technology: Leesburg, VA, USA, 2007. [Google Scholar]
- Weber, W. Electrodynamic Measurements, Especially Measures of Resistance. 2021. Available online: https://www.ifi.unicamp.br/~assis/Weber-EM2.pdf (accessed on 5 July 2022).
- Weber, W. Electrodynamic Measurements, Especially on Diamagnetism. 2021. Available online: https://www.ifi.unicamp.br/~assis/Weber-EM3.pdf (accessed on 5 July 2022).
- Kohlrausch, R.; Weber, W. Electrodynamic Measurements, Especially Attributing Mechanical Units to Measures of Current Intensity. 2021. Available online: http://www.ifi.unicamp.br/~assis/Weber-EM4.pdf (accessed on 5 July 2022).
- Weber, W. Electrodynamic Measurements, Especially on Electric Oscillations. 2021. Available online: http://www.ifi.unicamp.br/~assis/Weber-EM5.pdf (accessed on 5 July 2022).
- Weber, W. Electrodynamic measurements—Sixth memoir, relating specially to the principle of the conservation of energy. Lond. Edinb. Dublin Philos. Mag. J. Sci. Fourth Ser. 1872, 43, 1–20. [Google Scholar]
- Weber, W. Electrodynamic Measurements, Especially on the Energy of Interaction. 2021. Available online: http://www.ifi.unicamp.br/~assis/Weber-EM7.pdf (accessed on 5 July 2022).
- Weber, W. Determinations of Electrodynamic Measure: Particularly in Respect to the Connection of the Fundamental Laws of Electricity with the Law of Gravitation; 21st Century Science and Technology: Leesburg, VA, USA, 2021; Available online: https://www.ifi.unicamp.br/~assis/Weber_EM8.pdf (accessed on 5 July 2022).
- Davies, P. A quantum theory of Wheeler–Feynman electrodynamics. In Proceedings of the Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1970; Volume 68, pp. 751–764. [Google Scholar] [CrossRef]
- Wesley, J. Weber electrodynamics, part II unipolar induction, Z-antenna. Found. Phys. Lett. 1990, 3, 471–490. [Google Scholar] [CrossRef]
- Wesley, J.P. Weber electrodynamics: Part III. mechanics, gravitation. Found. Phys. Lett. 1990, 3, 581–605. [Google Scholar] [CrossRef]
- Assis, A.K.T. Deriving Ampere’s law from Weber’s law. Hadron. J. 1990, 13, 441–451. [Google Scholar]
- Moyssides, P.G. Calculation of the sixfold integrals of the Ampere force law in a closed circuit. IEEE Trans. Magn. 1989, 25, 4307–4312. [Google Scholar] [CrossRef]
- Cavalleri, G.; Bettoni, G.; Tonni, E.; Spavieri, G. Experimental proof of standard electrodynamics by measuring the self-force on a part of a current loop. Phys. Rev. E 1998, 58, 2505. [Google Scholar] [CrossRef]
- Assis, A.K.T. Comment on “Experimental proof of standard electrodynamics by measuring the self-force on a part of a current loop”. Phys. Rev. E 2000, 62, 7544. [Google Scholar] [CrossRef]
- Assis, A.K.T.; Bueno, M.A. Equivalence between Ampere and Grassmann’s forces. IEEE Trans. Magn. 1996, 32, 431–436. [Google Scholar] [CrossRef]
- Bueno, M.; Assis, A.K.T. Proof of the identity between Ampère and Grassmann’s forces. Phys. Scr. 1997, 56, 554. [Google Scholar] [CrossRef]
- Assis, A.K.; Bueno, M. Bootstrap effect in classical electrodynamics. Rev. Fac. Ing. 2000, 7, 49–55. [Google Scholar]
- Hofmann, J.R. André-Marie Ampère: Enlightenment and Electrodynamics; Cambridge University Press: Cambridge, UK, 1996; Volume 7. [Google Scholar]
- Ampère, A.M. Mémoire présenté à l’Académie royale des Sciences, le 2 octobre 1820, où se trouve compris le résumé de ce qui avait été lu à la même Académie les 18 et 25 septembre 1820, sur les effets des courans électriques. In Proceedings of the Annales de Chimie et de Physique, Paris, France, 2 October 1820; Volume 15, pp. 59–76. [Google Scholar]
- Ampère, A. The effects of electric currents. In Early Electrodynamics—The First Law of Circulation; Tricker, R.A.R., Ed.; Pergamon: Oxford, UK, 1965; pp. 140–154. [Google Scholar]
- Assis, A.; Souza Filho, M.; Caluzi, J.; Chaib, J. From Electromagnetism to Electrodynamics: Ampère’s Demonstration of the Interaction between Current Carrying Wires. In Proceedings of the 4th International Conference on Hands-on Science, University of Azores, Ponta Delgada, Portugal, 23 July 2007; pp. 9–16. [Google Scholar]
- Chaib, J.; Lima, F. Resuming Ampère’s experimental investigation of the validity of Newton’s third law in electrodynamics. Ann. Fond. Louis Broglie 2020, 45, 19. [Google Scholar]
- Graneau, P. Electromagnetic jet-propulsion in the direction of current flow. Nature 1982, 295, 311. [Google Scholar] [CrossRef]
- Graneau, P. Application of Ampere’s force law to railgun accelerators. J. Appl. Phys. 1982, 53, 6648–6654. [Google Scholar] [CrossRef]
- Graneau, P. First indication of Ampere tension in solid electric conductors. Phys. Lett. A 1983, 97, 253–255. [Google Scholar] [CrossRef]
- Graneau, P. Ampere tension in electric conductors. IEEE Trans. Magn. 1984, 20, 444–455. [Google Scholar] [CrossRef][Green Version]
- Graneau, P. Ampère-Neumann Electrodynamics of Metals; Hadronic Press, Inc.: Nonantum, MA, USA, 1985. [Google Scholar]
- Graneau, P. The Ampere-Neumann Electrodynamics of Metallic Conductors. Fortschritte Der Phys. 1986, 34, 457–501. [Google Scholar]
- Graneau, P.; Graneau, N. The electromagnetic impulse pendulum and momentum conservation. Il Nuovo Cimento D 1986, 7, 31–45. [Google Scholar] [CrossRef]
- Graneau, P. Amperian recoil and the efficiency of railguns. J. Appl. Phys. 1987, 62, 3006–3009. [Google Scholar] [CrossRef]
- Graneau, P.; Thompson, D.S.; Morrill, S.L. The motionally induced back-emf in railguns. Phys. Lett. A 1990, 145, 396–400. [Google Scholar] [CrossRef]
- Graneau, P.; Graneau, N. The role of Ampere forces in nuclear fusion. Phys. Lett. A 1992, 165, 1–13. [Google Scholar] [CrossRef]
- Assis, A.K.T. On the mechanism of railguns. Galilean Electrodyn. 1992, 3, 93–95. [Google Scholar]
- Nasiłowski, J. A note on longitudinal Ampere forces in gaseous conductors. Phys. Lett. A 1985, 111, 315–316. [Google Scholar] [CrossRef]
- Pappas, P. The original Ampere force and Biot-Savart and Lorentz forces. Il Nuovo Cimento B (1971–1996) 1983, 76, 189–197. [Google Scholar] [CrossRef]
- Phipps, T.; Phipps, T., Jr. Observation of Ampere forces in mercury. Phys. Lett. A 1990, 146, 6–14. [Google Scholar] [CrossRef]
- Phipps, T.E. New evidence for Ampère longitudinal forces. Phys. Essays 1990, 3, 198. [Google Scholar] [CrossRef]
- Wesley, J. Pinch effect and Ampere tension to drive Hering’s pump. Found. Phys. Lett. 1994, 7, 95–104. [Google Scholar] [CrossRef]
- Peoglos, V. Measurement of the magnetostatic force of a current circuit on a part of itself. J. Phys. D Appl. Phys. 1988, 21, 1055. [Google Scholar] [CrossRef]
- Achilles, R. Again on the Guala-Valverde homopolar induction experiments. Spacetime Subst. 2002, 3, 235. [Google Scholar]
- Achilles, R.; Guala-Valverde, J. Action at a Distance: A Key to Homopolar Induction. Apeiron Stud. Infin. Nat. 2007, 14, 169–183. [Google Scholar]
- Guala-Valverde, J.; Achilles, R. A Manifest Failure of Grassmann’s Force. Apeiron Stud. Infin. Nat. 2008, 15, 202–205. [Google Scholar]
- Papageorgiou, C.; Raptis, T. Fragmentation of Thin Wires Under High Power Pulses and Bipolar Fusion. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2010; Volume 1203, pp. 955–960. [Google Scholar] [CrossRef]
- Alfaro, A.D. Ampre’s Longitudinal Forces Revisited; Technical Report; Naval Postgraduate School, United States: Monterey, CA, USA, 2018. [Google Scholar]
- Pappas, P.; Pappas, L.; Pappas, T. Ampère cardinal forces-electrodynamics—Proof and prediction of empirical Faraday induction. Phys. Essays 2014, 27, 570–579. [Google Scholar] [CrossRef]
- Rambaut, M.; Vigier, J. The simultaneous existence of EM Grassmann-Lorentz forces (acting on charged particles) and Ampère forces (acting on charged conducting elements) does not contradict relativity theory. Phys. Lett. A 1989, 142, 447–452. [Google Scholar] [CrossRef]
- Rambaut, M.; Vigier, J. Ampere forces considered as collective non-relativistic limit of the sum of all Lorentz interactions acting on individual current elements: Possible consequences for electromagnetic discharge stability and tokamak behaviour. Phys. Lett. A 1990, 148, 229–238. [Google Scholar] [CrossRef]
- Moyssides, P.G. The anticipated longitudinal forces by the Biot-Savart-Grassmann-Lorentz force law are in complete agreement with the longitudinal Ampère forces. Eur. Phys. J. Plus 2014, 129, 1–17. [Google Scholar] [CrossRef]
- Saumont, R. Ampère Force: Experimental Tests. In Advanced Electromagnetism: Foundations, Theory and Applications; World Scientific: Singapore, 1995; pp. 620–635. [Google Scholar]
- Graneau, N.; Phipps, T., Jr.; Roscoe, D. An experimental confirmation of longitudinal electrodynamic forces. Eur. Phys. J. D At. Mol. Opt. Plasma Phys. 2001, 15, 87–97. [Google Scholar] [CrossRef]
- Kühn, S. Experimental investigation of an unusual induction effect and its interpretation as a necessary consequence of Weber electrodynamics. J. Electr. Eng. 2021, 72, 366–373. [Google Scholar] [CrossRef]
- Einstein, A. Zur elektrodynamik bewegter körper. Ann. Der Phys. 1905, 354, 769–822. [Google Scholar] [CrossRef]
- Frisch, D.H.; Wilets, L. Development of the Maxwell-Lorentz equations from special relativity and Gauss’s law. Am. J. Phys. 1956, 24, 574–579. [Google Scholar] [CrossRef]
- Field, J. Derivation of the Lorentz force law, the magnetic field concept and the Faraday–Lenz and magnetic Gauss laws using an invariant formulation of the Lorentz transformation. Phys. Scr. 2006, 73, 639. [Google Scholar] [CrossRef]
- Dodig, H. Direct derivation of Lienard–Wiechert potentials, Maxwell’s equations and Lorentz force from Coulomb’s law. Mathematics 2021, 9, 237. [Google Scholar] [CrossRef]
- Smith, R.T.; Jjunju, F.P.; Maher, S. Evaluation of Electron Beam Deflections across a Solenoid Using Weber-Ritz and Maxwell-Lorentz Electrodynamics. Prog. Electromagn. Res. 2015, 151, 83–93. [Google Scholar] [CrossRef][Green Version]
- Smith, R.T.; Maher, S. Investigating Electron Beam Deflections by a Long Straight Wire Carrying a Constant Current Using Direct Action, Emission-Based and Field Theory Approaches of Electrodynamics. Prog. Electromagn. Res. 2017, 75, 79–89. [Google Scholar] [CrossRef][Green Version]
- Baumgärtel, C.; Smith, R.T.; Maher, S. Accurately predicting electron beam deflections in fringing fields of a solenoid. Sci. Rep. 2020, 10, 10903. [Google Scholar] [CrossRef] [PubMed]
- Baumgärtel, C.; Maher, S. A Charged Particle Model Based on Weber Electrodynamics for Electron Beam Trajectories in Coil and Solenoid Elements. Prog. Electromagn. Res. C 2022, 123, 151–166. [Google Scholar] [CrossRef]
- Li, Q. Electric Field Theory Based on Weber’s Electrodynamics. Int. J. Magn. Electromagn. 2021, 7, 1–6. [Google Scholar]
- Kühn, S. The Inhomogeneous Wave Equation, Liénard-Wiechert Potentials, and Hertzian Dipole in Weber Electrodynamics. 2022. Available online: https://www.researchgate.net/publication/358747323_The_inhomogeneous_wave_equation_Lienard-Wiechert_potentials_and_Hertzian_dipole_in_Weber_electrodynamics (accessed on 5 July 2022). [CrossRef]
- Rambaut, M. Macroscopic non-relativistic Ampère EM interactions between current elements reflect the conducting electron accelerations by the ion’s electric fields. Phys. Lett. A 1991, 154, 210–214. [Google Scholar] [CrossRef]
- Weber, W.; Kohlrausch, R. Ueber die Elektricitätsmenge, welche bei galvanischen Strömen durch den Querschnitt der Kette fliesst. Ann. Phys. 1856, 175, 10–25. [Google Scholar] [CrossRef]
- Assis, A.K. On the first electromagnetic measurement of the velocity of light by Wilhelm Weber and Rudolf Kohlrausch. In Volta and the History of Electricity; Università degli Studi di Pavia and Editore Ulrico Hoepli: Milano, Italy, 2003; pp. 267–286. [Google Scholar]
- Kirchhoff, G. Ueber die Bewegung der Elektricität in Leitern. Ann. Phys. 1857, 178, 529–544. [Google Scholar] [CrossRef]
- Weber, W. Elektrodynamische Maassbestimmungen Insbesondere über Elektrische Schwingungen; Teubner: Leipzig, Germany, 1864; Volume 6, pp. 105–241. [Google Scholar]
- Assis, A.K.T.; Hernandes, J. Telegraphy equation from Weber’s electrodynamics. IEEE Trans. Circuits Syst. II Express Briefs 2005, 52, 289–292. [Google Scholar] [CrossRef]
- Fukai, J. A Promenade Along Electrodynamics; Vales Lake Publishing: Pueblo West, CO, USA, 2003. [Google Scholar]
- Assis, A.K.T. Circuit theory in Weber electrodynamics. Eur. J. Phys. 1997, 18, 241. [Google Scholar] [CrossRef]
- Bueno, M.; Assis, A.K.T. Equivalence between the formulas for inductance calculation. Can. J. Phys. 1997, 75, 357–362. [Google Scholar] [CrossRef]
- McDonald, K.T. Weber’s Electrodynamics and the Hall Effect. 2020, pp. 1–15. Available online: http://kirkmcd.princeton.edu/examples/weber.pdf (accessed on 5 July 2022).
- Smith, R.T.; Taylor, S.; Maher, S. Modelling electromagnetic induction via accelerated electron motion. Can. J. Phys. 2014, 93, 802–806. [Google Scholar] [CrossRef]
- Smith, R.T.; Jjunju, F.P.; Young, I.S.; Taylor, S.; Maher, S. A physical model for low-frequency electromagnetic induction in the near field based on direct interaction between transmitter and receiver electrons. Proc. R. Soc. A Math. Phys. Eng. Sci. 2016, 472, 20160338. [Google Scholar] [CrossRef]
- Assis, A.K.; Thober, D.S. Unipolar induction and Weber’s electrodynamics. In Frontiers of Fundamental Physics; Springer: Berlin, Germany, 1994; pp. 409–414. [Google Scholar]
- Baumgärtel, C.; Maher, S. A Novel Model of Unipolar Induction Phenomena Based on Direct Interaction Between Conductor Charges. Prog. Electromagn. Res. 2021, 171, 123–135. [Google Scholar] [CrossRef]
- Baumgärtel, C.; Maher, S. Resolving the Paradox of Unipolar Induction—New Experimental Evidence on the Influence of the Test Circuit. Sci. Rep. 2022, 12, 16791. [Google Scholar] [CrossRef]
- Härtel, H. Unipolar Induction-a Messy Corner of Electromagnetism. Eur. J. Phys. Educ. 2020, 11, 47–59. [Google Scholar]
- Assis, A.K.T.; Fukai, J.; Carvalho, H. Weberian induction. Phys. Lett. A 2000, 268, 274–278. [Google Scholar] [CrossRef]
- Feynman, R. Lectures in Physics; Chapter 17 The Laws of Induction; California Institute of Technology: Pasadena, CA, USA, 1963; Volume II. [Google Scholar]
- Ma, T.C.E. Field angular momentum in Feynman’s disk paradox. Am. J. Phys. 1986, 54, 949–950. [Google Scholar] [CrossRef]
- Prytz, K.A. Meissner effect in classical physics. Prog. Electromagn. Res. 2018, 64, 1–7. [Google Scholar] [CrossRef][Green Version]
- Assis, A.; Tajmar, M. Superconductivity with Weber’s electrodynamics: The London moment and the Meissner effect. Ann. Fond. Louis Broglie 2017, 42, 307. [Google Scholar]
- Wesley, J. Induction Produces Aharonov-Bohm Effect. Apeiron 1998, 5, 73–82. [Google Scholar]
- Prytz, K. Antenna Theory—The Loop and the Dipole. In Electrodynamics: The Field-Free Approach; Springer: Berlin, Germany, 2015; pp. 219–238. [Google Scholar]
- Assis, A.K.T.; Wiederkehr, K.H.; Wolfschmidt, G. Weber’s Planetary Model of the Atom; Tredition Science: Hamburg, Germany, 2011. [Google Scholar]
- Weber, W.E. Electrodynamische Maassbestimmungen; S. Hirzel: Leipzig, Germany, 1871; Volume 2. [Google Scholar]
- Bush, V. The force between moving charges. J. Math. Phys. 1926, 5, 129–157. [Google Scholar] [CrossRef]
- Clemente, R.; Assis, A.K.T. Two-body problem for Weber-like interactions. Int. J. Theor. Phys. 1991, 30, 537–545. [Google Scholar] [CrossRef]
- Riecke, E. Ueber Molecularbewegung zweier Theilchen, deren Wechselwirkung durch das webersche Gesetz der elektrischen Kraft bestimmt wird. In Nachrichten von der Königl. Gesellschaft der Wissenschaften und der Georg-Augusts-Universität zu Göttingen; Dieterich: Göttingen, Germany, 1874; p. 665. [Google Scholar]
- Lolling, G. Ueber Bewegungen elektrischer Theilchen nach dem Weber’schen Grundgesetz der Elektrodynamik. In Verhandlungen der Kaiserlichen Leopoldinisch-Carolinischen Deutschen Akademie der Naturforscher; Kaiserlich-Leopoldinisch-Carolinische Deutsche Akademie der Naturforscher: Halle, Germany, 1882; Volume 44, pp. 273–336. [Google Scholar]
- Ritter, E., II. Bewegung eines materiellen mit Eletricität geladenen Theilchens unter der Einwirkung eines ruhenden Centrum bei Giltigkeit des Weber’schen Gesetzes. Z. Math. Und Phys. Organ Angew. Math. 1892, 37, 8–24. [Google Scholar]
- Zöllner, J.F. Principien einer Elektrodynamischen Theorie der Materie; Engelmann: Leipzig, Germany, 1876; Volume 1. [Google Scholar]
- Wesley, J.P. Evidence for Weber–Wesley electrodynamics. In Proceedings of the Conference on Foundations of Mathematics and Physics, Perugia, Italy, 27–29 September 1989; pp. 27–29. [Google Scholar]
- Post, E.J. A Tale of Fine Structure Coincidences. In Quantum Reprogramming; Springer: Berlin, Germany, 1995; pp. 151–168. [Google Scholar]
- Pearson, J.; Kilambi, A. Velocity-dependent nuclear forces and Weber’s electrodynamics. Am. J. Phys. 1974, 42, 971–975. [Google Scholar] [CrossRef]
- Assis, A.K.T. Deriving gravitation from electromagnetism. Can. J. Phys. 1992, 70, 330–340. [Google Scholar] [CrossRef]
- Assis, A.K.T. Gravitation as a fourth order electromagnetic effect. In Advanced Electromagnetism: Foundations, Theory and Applications; Barret, T.W., Gales, D.M., Eds.; World Scientific Publishers: Singapore, 1995; pp. 314–331. [Google Scholar]
- Tajmar, M. Derivation of the Planck and Fine-Structure Constant from Assis’s Gravity Model. J. Adv. Phys. 2015, 4, 219–221. [Google Scholar] [CrossRef]
- Baumgärtel, C.; Tajmar, M. The Planck Constant and the Origin of Mass Due to a Higher Order Casimir Effect. J. Adv. Phys. 2018, 7, 135–140. [Google Scholar] [CrossRef]
- Tisserand, F. Sur le Mouvement des Planètes Autour du Soleil, D’après la loi Électrodynamique de Weber; Gauthier-Villars: Paris, France, 1872. [Google Scholar]
- Gerber, P. Die räumliche und zeitliche Ausbreitung der Gravitation. Z. Math. Phys. 1898, 43, 415–444. [Google Scholar]
- Schrödinger, E. Die Erfüllbarkeit der Relativitätsforderung in der klassischen Mechanik. Ann. Phys. 1925, 382, 325–336. [Google Scholar] [CrossRef]
- Assis, A.K.T. On Mach’s principle. Found. Phys. Lett. 1989, 2, 301–318. [Google Scholar] [CrossRef]
- Tajmar, M.; Assis, A.K.T. Influence of Rotation on the Weight of Gyroscopes as an Explanation for Flyby Anomalies. J. Adv. Phys. 2016, 5, 176–179. [Google Scholar] [CrossRef][Green Version]
- Bunchaft, F.; Carneiro, S. Weber-like interactions and energy conservation. Found. Phys. Lett. 1997, 10, 393–401. [Google Scholar] [CrossRef][Green Version]
- Mendes, R.; Malacarne, L.; Assis, A. Virial Theorem For Weber’s Law. In Has the Last Word Been Said on Classical Electrodynamics? New Horizons; Chubykalo, A., Espinoza, A., Onoochin, V., Smirnov-Rueda, R., Eds.; Rinton Press: Paramus, NJ, USA, 2004; pp. 67–70. [Google Scholar]
- Clemente, R.A.; Cesar, R.G. Cold plasma oscillations according to Weber’s law. An unphysical result. Int. J. Theor. Phys. 1993, 32, 1257–1260. [Google Scholar] [CrossRef]
- Assis, A.K.T. Relational Mechanics and Implementation of Mach’s Principle with Weber’s Gravitational Force; C. Roy Keys Inc.: Montreal, QC, Canada, 2014. [Google Scholar]
- Seegers, C. Über die Bewegung und die Störungen der Planeten, Wenn Dieselben Sich Nach dem Weberschen Elektrodynamischen Gesetz um die Sonne Bewegen; Springer: Berlin, Germany, 2013. [Google Scholar]
- Servus, H. Untersuchungen über die Bahn und die Störungen der Himmelskörper mit Zugrundelegung des Weber’schen Electrodynamischen Gesetzes. Ph.D. Thesis, Universität Halle-Wittenberg, Halle, Germany, 1885. [Google Scholar]
- Zöllner, J.K.F. Über die Natur der Cometen: Beiträge zur Geschichte und Theorie der Erkenntniss; Engelmann: Leipzig, Germany, 1872. [Google Scholar]
- Eby, P. On the perihelion precession as a Machian effect. Nuovo C. Lett. 1977, 18, 93–96. [Google Scholar] [CrossRef]
- Ragusa, S. Gravitation with a modified Weber force. Found. Phys. Lett. 1992, 5, 585–589. [Google Scholar] [CrossRef]
- Lévy, M. Sur l’application des lois électrodynamiques au mouvement des planétes. Comput. Rend. Acad. Sci. 1890, 110, 545. [Google Scholar]
- Giné, J. Is gravitational quantization another consequence of General Relativity? Chaos Solitons Fractals 2009, 42, 1893–1899. [Google Scholar] [CrossRef]
- Kholodenko, A. Newtonian Limit of Einsteinian Gravity and Dynamics of Solar System. In Relativity, Gravitation, Cosmology: Foundations; Nova Science Publishers: Hauppauge, NY, USA, 2015; pp. 101–152. [Google Scholar]
- Tiandho, Y. Weber’s gravitational force as static weak field approximation. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2016; Volume 1708, p. 070012. [Google Scholar]
- Lima, F. Nonzero Gravitational Force Exerted by a Spherical Shell on a Body Moving Inside It, and Cosmological Implications. Gravit. Cosmol. 2020, 26, 387–398. [Google Scholar] [CrossRef]
- Das, S. Mach Principle and a new theory of gravitation. arXiv 2012, arXiv:1206.6755. [Google Scholar]
- Roscoe, D. Mach’S Principle and Instantaneous Action at a Distance. In Instantaneous Action at a Distance in Modern Physics: “Pro” and “Contra”; Andrew E. Chubykalo, V.P., Smirnov-Rueda, R., Eds.; Nova Science Publishers, Inc.: Commack, NY, USA, 1999; pp. 175–188. [Google Scholar]
- Assis, A. Assis, A. A steady-state cosmology. In Progress in New Cosmologies; Springer: Berlin, Germany, 1993; pp. 153–167. [Google Scholar]
- Assis, A.K.; Graneau, P. Nonlocal forces of inertia in cosmology. Found. Phys. 1996, 26, 271–283. [Google Scholar] [CrossRef]
- Graneau, P.; Graneau, N. Machian inertia and the isotropic universe. Gen. Relativ. Gravit. 2003, 35, 751–770. [Google Scholar] [CrossRef]
- Tajmar, M.; Assis, A. Gravitational induction with Weber’s force. Can. J. Phys. 2015, 93, 1571–1573. [Google Scholar] [CrossRef]
- Assis, A.K.T. Changing the inertial mass of a charged particle. J. Phys. Soc. Jpn. 1993, 62, 1418–1422. [Google Scholar] [CrossRef]
- Tajmar, M. Propellantless propulsion with negative matter generated by electric charges. In Proceedings of the AIAA Joint Propulsion Conference, AIAA-2013, San Jose, CA, USA, 14–17 July 2013; Volume 3913. [Google Scholar]
- Tajmar, M.; Assis, A.K.T. Particles with negative mass: Production, properties and applications for nuclear fusion and self-acceleration. J. Adv. Phys. 2015, 4, 77–82. [Google Scholar] [CrossRef][Green Version]
- Tajmar, M. Revolutionary Propulsion Research at TU Dresden. In Proceedings of the Advanced Propulsion Workshop, Estes Park, CO, USA, 19–22 September 2016; pp. 20–22. [Google Scholar]
- Mikhailov, V. The Action of an Electrostatic Potential on the Electron Mass. Ann. Fond. Louis Broglie 1999, 24, 161. [Google Scholar]
- Mikhailov, V.F. Influence of an electrostatic potential on the inertial electron mass. Ann. Fond. Louis Broglie 2001, 26, 633–638. [Google Scholar]
- Mikhailov, V. Influence of a field-less electrostatic potential on the inertial electron mass. Ann. Fond. Louis Broglie 2003, 28, 231. [Google Scholar]
- Junginger, J.E.; Popovic, Z.D. An experimental investigation of the influence of an electrostatic potential on electron mass as predicted by Weber’s force law. Can. J. Phys. 2004, 82, 731–735. [Google Scholar] [CrossRef]
- Lorincz, I.; Tajmar, M. Experimental Investigation of the Influence of Spatially Distributed Charges on the Inertial Mass of Moving Electrons as Predicted by Weber’s Electrodynamics. Can. J. Phys. 2017, 95, 1023. [Google Scholar] [CrossRef]
- Weikert, M.; Tajmar, M. Investigation of the Influence of a field-free electrostatic Potential on the Electron Mass with Barkhausen-Kurz Oscillation. arXiv 2019, arXiv:1902.05419. [Google Scholar]
- Tajmar, M.; Weikert, M. Evaluation of the Influence of a Field-Less Electrostatic Potential on Electron Beam Deflection as Predicted by Weber Electrodynamics. Prog. Electromagn. Res. M 2021, 105, 1–8. [Google Scholar] [CrossRef]
- Assis, A. On the unification of forces of nature. Ann. Fond. Louis Broglie 2002, 27, 149–161. [Google Scholar]
- Fricke, H. Two Rival Programmes in 19th. Century Classical Electrodynamics Action-at-a-Distance versus field Theories. Ph.D. Thesis, The London School of Economics and Political Science (LSE), London, UK, 1982. [Google Scholar]
- Clausius, R. Ueber die Ableitung eines neuen elektrodynamischen Grundgesetzes. J. Reine Angew. Math. 1877, 82, 85. [Google Scholar]
- Woodruff, A.E. Action at a distance in nineteenth century electrodynamics. Isis 1962, 53, 439–459. [Google Scholar] [CrossRef]
- Zöllner, F. Ueber die Einwendungen von Clausius gegen das Weber’sche Gesetz. Ann. Phys. 1877, 236, 514–537. [Google Scholar] [CrossRef]
- Erman, P. Über die electroskopischen Phänomene des Gasapparats an der Voltaischen Säule. Ann. Phys. 1802, 10, 1–23. [Google Scholar] [CrossRef]
- Assis, A.K.T.; Hernandes, J.A. The Electric Force of a Current: Weber and the Surface Charges of Resistive Conductors Carrying Steady Currents; Apeiron, C. Roy Keys Inc.: Montreal, QC, Canada, 2007. [Google Scholar]
- Hernandes, J.; Assis, A.K.T. Electric potential due to an infinite conducting cylinder with internal or external point charge. J. Electrost. 2005, 63, 1115–1131. [Google Scholar] [CrossRef]
- Assis, A.K.T.; Rodrigues, W.; Mania, A. The electric field outside a stationary resistive wire carrying a constant current. Found. Phys. 1999, 29, 729–753. [Google Scholar] [CrossRef]
- Caluzi, J.; Assis, A.K.T. A critical analysis of Helmholtz’s argument against Weber’s electrodynamics. Found. Phys. 1997, 27, 1445–1452. [Google Scholar] [CrossRef]
- Phipps, T.E. Toward modernization of Weber’s force law. Phys. Essays 1990, 3, 414. [Google Scholar] [CrossRef]
- Caluzi, J.; Assis, A.K.T. An analysis of Phipps’s potential energy. J. Frankl. Inst. 1995, 332, 747–753. [Google Scholar] [CrossRef]
- Li, Q. Extending Weber’s Electrodynamics to High Velocity Particles. Int. J. Magn. Electromagn. 2022, 8, 1–9. [Google Scholar]
- Assis, A.K.T. Arguments in favour of action at a distance. In Instantaneous Action at a Distance in Modern Physics: “Pro” and “Contra”; Chubykalo, A.E., Pope, V., Smimov-Rueda, R., Eds.; Nova Science Publishers: Commack, NY, USA, 1999; pp. 45–56. [Google Scholar]
- Haug, E.G. Demonstration that Newtonian gravity moves at the speed of light and not instantaneously (infinite speed) as thought! J. Phys. Commun. 2021, 5, 025005. [Google Scholar] [CrossRef]
- Brown, G.B. Retarded Action-at-a-Distance: The Change of Force with Motion; Cortney Publications: Luton, Bedfordshire, UK, 1982. [Google Scholar]
- Lentze, G. Dialogue concerning magnetic forces. Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 2019, 68, 158–162. [Google Scholar] [CrossRef]
- Assis, A.K.T. Weber’s law and mass variation. Phys. Lett. A 1989, 136, 277–280. [Google Scholar] [CrossRef]
- Wesley, J.P. Empirically correct electrodynamics. Found. Phys. Lett. 1997, 10, 189–204. [Google Scholar] [CrossRef]
- Assis, A.K.T.; Caluzi, J. A limitation of Weber’s law. Phys. Lett. A 1991, 160, 25–30. [Google Scholar] [CrossRef]
- Montes, J. On Limiting Velocity with Weber-like Potentials. Can. J. Phys. 2017, 95, 770–776. [Google Scholar] [CrossRef]
- Assis, A.K.T.; Clemente, R. The ultimate speed implied by theories of Weber’s type. Int. J. Theor. Phys. 1992, 31, 1063–1073. [Google Scholar] [CrossRef]
- Kühn, S. Analysis of a stochastic emission theory regarding its ability to explain the effects of special relativity. J. Electromagn. Anal. Appl. 2020, 12, 169–187. [Google Scholar] [CrossRef]
- Sherwin, C.W. The Weber Radar. Phys. Essays 1991, 4, 417–419. [Google Scholar] [CrossRef]
- Barrett, P.; Jones, H.; Franklin, R. Dispersion of electron plasma waves. Plasma Phys. 1968, 10, 911. [Google Scholar] [CrossRef]
- Wesley, J. Weber electrodynamics extended to include radiation. Specul. Sci. Technol. 1987, 10, 50–53. [Google Scholar]
- Phipps, T. Electromagnetic Force Laws and Hot Fusion. Galilean Electrodyn. 2016, 27, 79–80. [Google Scholar]
- Edwards, W.F.; Kenyon, C.; Lemon, D. Continuing investigation into possible electric fields arising from steady conduction currents. Phys. Rev. D 1976, 14, 922. [Google Scholar] [CrossRef]
- Jefimenko, O. Demonstration of the electric fields of current-carrying conductors. Am. J. Phys. 1962, 30, 19–21. [Google Scholar] [CrossRef]
- Jefimenko, O.D. Electricity and Magnetism: An Introduction to the Theory of Electric and Magnetic Fields, 2nd ed.; Electret Scientific Company: Star City, WV, USA, 1989. [Google Scholar]
- Zhao, Y. A Novel Two-Particle Steady-State Wave Equation. IRanian J. Sci. Technol. Trans. A Sci. 2022, 46, 1019–1026. [Google Scholar] [CrossRef]

| Category | Field Theory | Weber Electrodynamics |
|---|---|---|
| Point Particle Interaction | Liénard-Wiechert-Schwarzschild force | Weber force |
| Current Element Interaction | Grassmann’s force | Ampère force |
| Longitudinal Forces | Can be obtained in various ways [96,97,112] | Intrinsic |
| Magnetic Force | Lorentz force | Weber-Ampère force |
| Conservation laws | Energy is stored or dissipated by the field to restore conservation laws | Follows linear momentum, angular momentum and energy conservation |
| Field Equations | Maxwell equations | Can be obtained in various ways [5,6,8,9,110] |
| Induction | Through changing electric and magnetic fields and flux | Through particle movement (velocity and acceleration) |
| Wave Equations | Arise from moving fields | Can be obtained through retarded time and also predicts telegraph equation |
| Compatibility with other forces of nature | Weak force, strong force, gravity not yet known | Gravity, strong force, weak force not yet known |
| Compatibility with SRT | Compatible through Lorentz transformation | Incompatible with Lorentz transformation |
| Extension to Quantum Mechanics | QED, QFT | Initial connections and some problems exist, but not yet fully known |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baumgärtel, C.; Maher, S. Foundations of Electromagnetism: A Review of Wilhelm Weber’s Electrodynamic Force Law. Foundations 2022, 2, 949-980. https://doi.org/10.3390/foundations2040065
Baumgärtel C, Maher S. Foundations of Electromagnetism: A Review of Wilhelm Weber’s Electrodynamic Force Law. Foundations. 2022; 2(4):949-980. https://doi.org/10.3390/foundations2040065
Chicago/Turabian StyleBaumgärtel, Christof, and Simon Maher. 2022. "Foundations of Electromagnetism: A Review of Wilhelm Weber’s Electrodynamic Force Law" Foundations 2, no. 4: 949-980. https://doi.org/10.3390/foundations2040065
APA StyleBaumgärtel, C., & Maher, S. (2022). Foundations of Electromagnetism: A Review of Wilhelm Weber’s Electrodynamic Force Law. Foundations, 2(4), 949-980. https://doi.org/10.3390/foundations2040065







