The Logarithmic Derivative in Scientific Data Analysis
Abstract
1. Introduction
2. Logarithmic Derivative and a Generalized Decay Constant
3. Examples
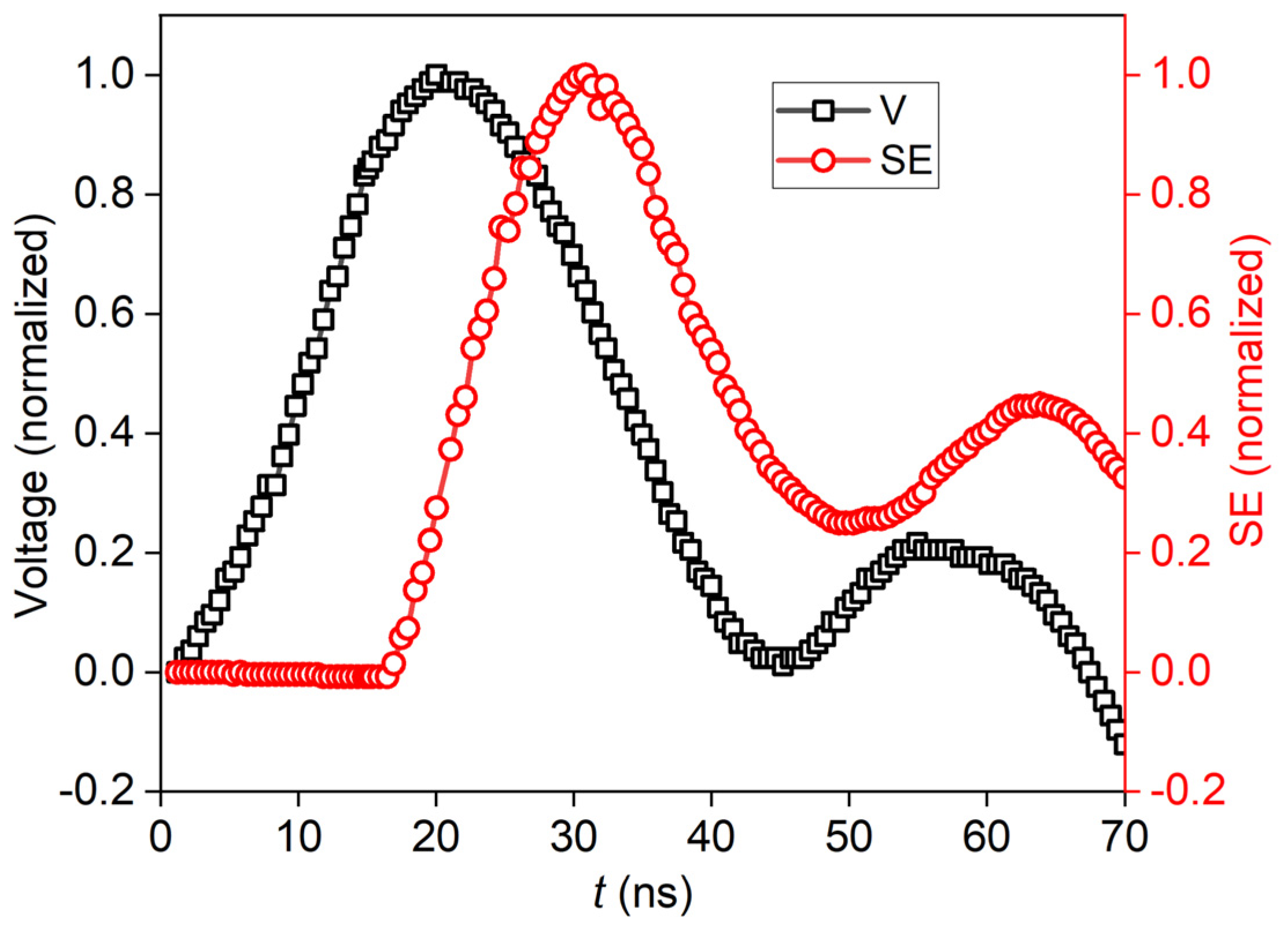
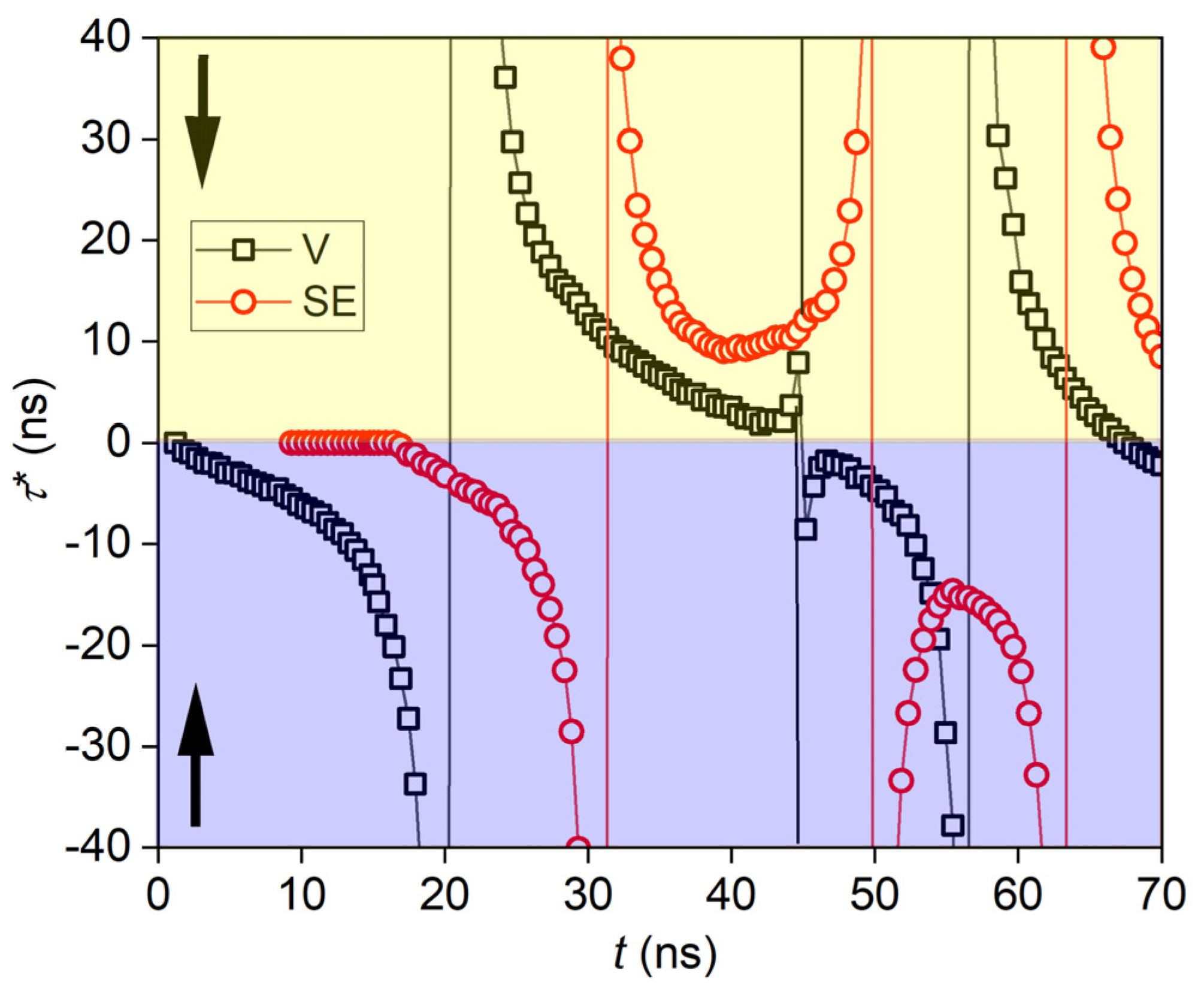
3.1. Time-Dependent Spontaneous Emission of Molecular Nitrogen
3.2. UV-Multiphoton Dissociation of Molybdenum Hexacarbonyl
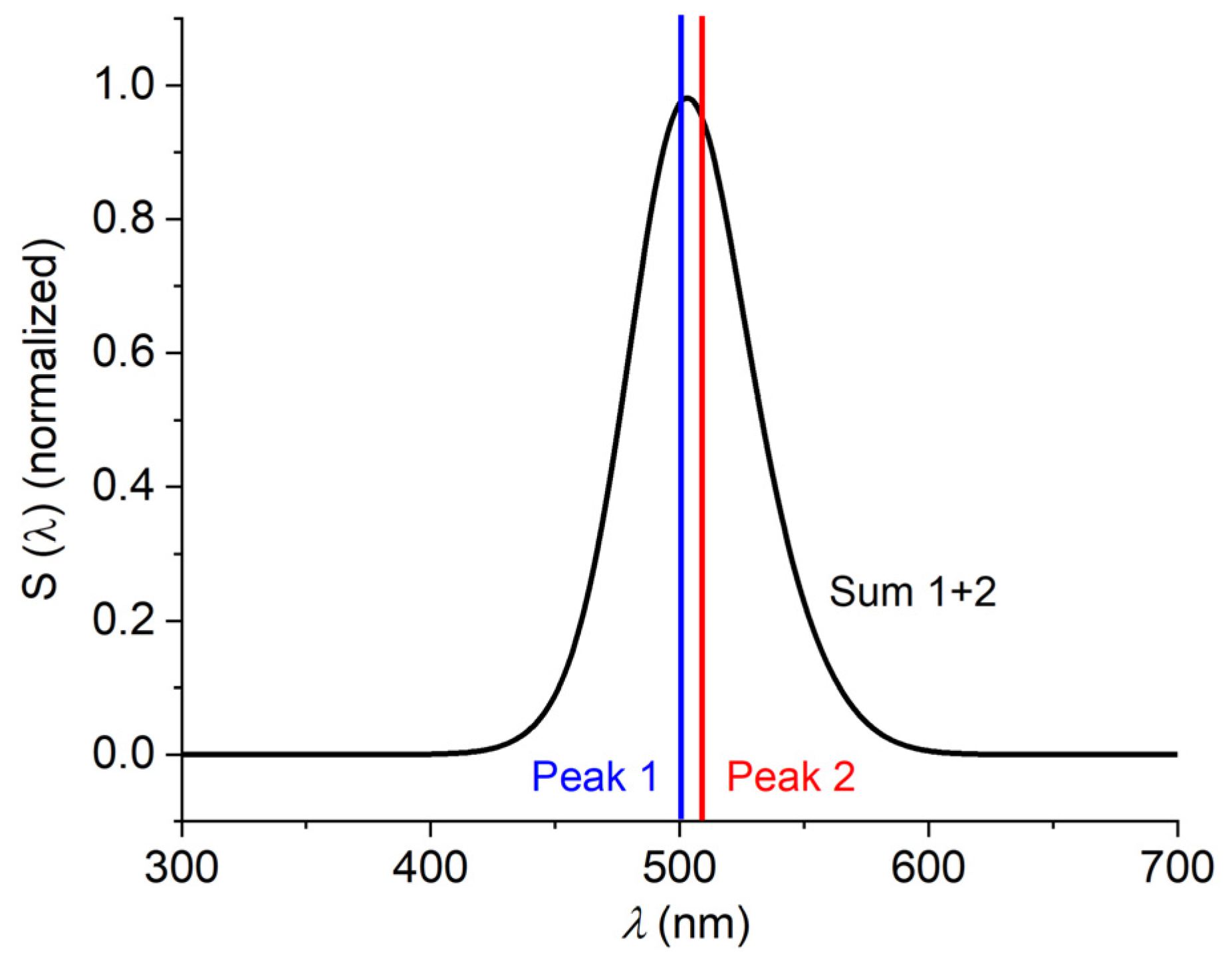
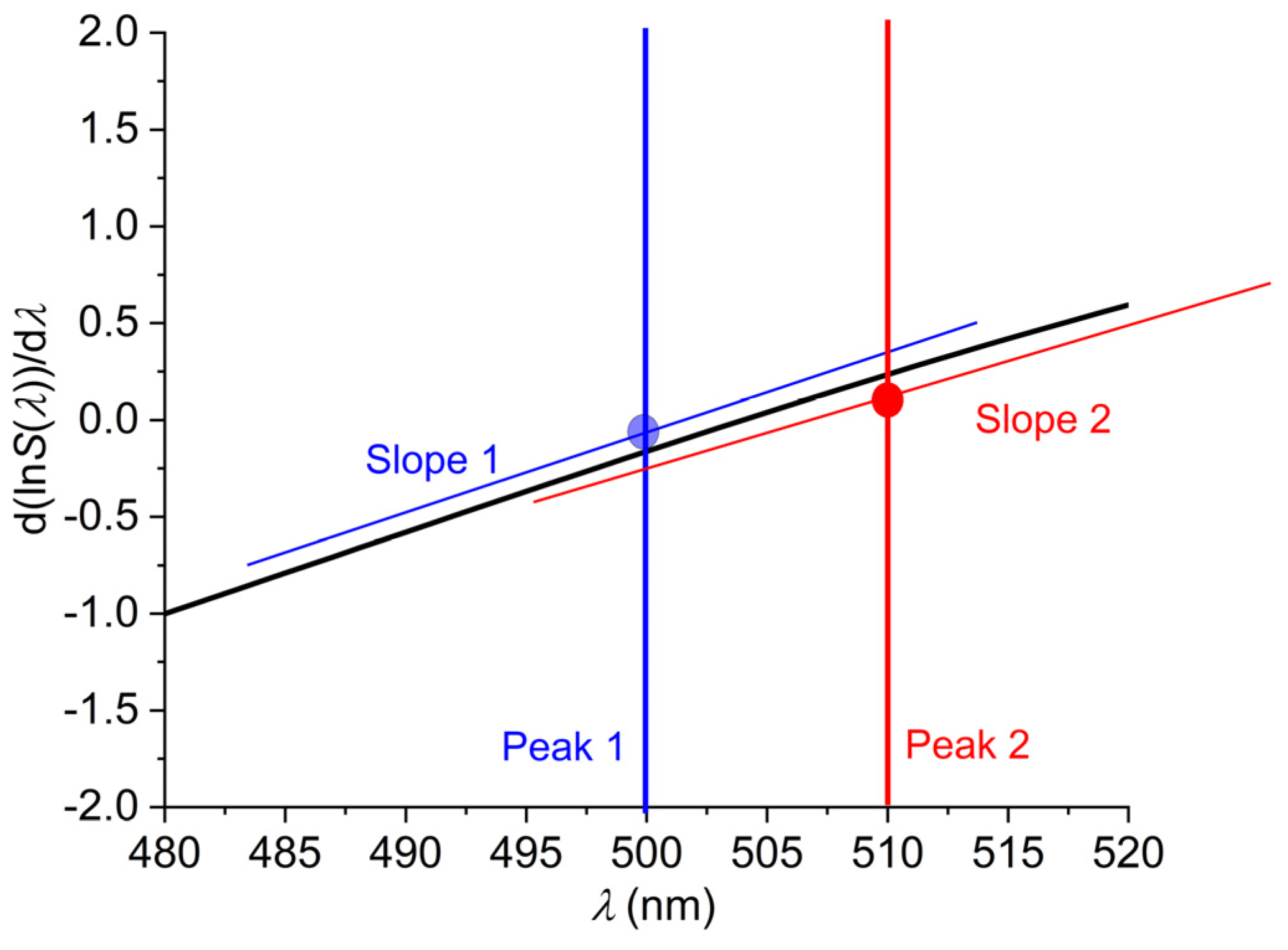
3.3. Separation of Overlapping Spectral Peaks
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ASE | Amplified spontaneous emission |
| HWHM | Half width at half maximum |
| MPD | Multiphoton dissociation |
| MPI | Multiphoton ionization |
References
- Singleton, F.; Collier, G.L. Infra-red analysis by the derivative method. J. Appl. Chem. 1956, 6, 495–510. [Google Scholar] [CrossRef]
- Dehghani, H.; Leblond, F.; Pogue, B.W.; Chauchard, F. Application of spectral derivative data in visible and near-infrared spectroscopy. Phys. Med. Biol. 2010, 55, 3381–3399. [Google Scholar] [CrossRef]
- Vandermeer, J. How populations grow: The exponential and logistic equations. Nat. Educ. Knowl. 2010, 3, 15. [Google Scholar]
- Delfini, L.; Lepri, S.; Livi, R.; Politi, A. Self-consistent mode-coupling approach to one-dimensional heat transport. Phys. Rev. E 2006, 73, 060201. [Google Scholar] [CrossRef]
- Wübbenhorst, M.; van Turnhout, J. Analysis of complex dielectric spectra. I. One-dimensional derivative techniques and three-dimensional modelling. J. Non-Cryst. Solids 2002, 305, 40–49. [Google Scholar] [CrossRef]
- Haspel, H.; Kukovecz, Á.; Kóny, Z.; Kiricsi, I. Numerical differentiation methods for the logarithmic derivative technique used in dielectric spectroscopy. Process. Appl. Ceram. 2010, 4, 87–93. [Google Scholar] [CrossRef]
- Kaatze, U. Logarithmic derivative complex permittivity spectrometry. Meas. Sci. Technol. 2003, 14, N55–N58. [Google Scholar] [CrossRef]
- Jurelo, A.R.; Menegotto Costa, R.; de Andrade, A.V.C.; Júnior, P.R.; da Cruz, G.K.; Lopes, C.S.; dos Santos, M.; de Sousa, W.T.B. Analysis of fluctuation conductivity of polycrystalline Er1-xPrxBa2Cu3O7-δ superconductors. Brazil. J. Phys. 2009, 39, 667–672. [Google Scholar] [CrossRef]
- Siems, U.; Kreuter, C.; Erbe, A.; Schwierz, N.; Sengupta, S.; Leiderer, P.; Nielaba, P. Non-monotonic crossover from single-file to regular diffusion in micro-channels. Sci. Rep. 2015, 1, srep01015. [Google Scholar] [CrossRef]
- Swain, P.S.; Stevenson, K.; Leary, A.; Montano-Gutierrez, L.F.; Clark, I.B.N.; Vogel, J.; Pilizota, T. Inferring time derivatives including cell growth rates using Gaussian processes. Nat. Commun. 2016, 7, 13766. [Google Scholar] [CrossRef]
- Jarosiński, Ł.; Pawlak, J.; Al-Ani, S.K.J. Inverse logarithmic derivative method for determining the energy gap and the type of electron transitions as an alternative to the Tauc method. Opt. Mater. 2019, 88, 667–673. [Google Scholar] [CrossRef]
- Manolopulos, D.E. An improved log derivative method for inelastic scattering. J. Chem. Phys. 1986, 85, 6425–6429. [Google Scholar] [CrossRef]
- Li, B.Q. A logarithmic derivative lemma in several complex variables and its applications. Trans. Am. Math. Soc. 2011, 363, 6257–6267. [Google Scholar] [CrossRef]
- Yousif, H.A.; Melka, R. Bessel function of the first kind with complex argument. Comput. Phys. Commun. 1997, 106, 199–206. [Google Scholar] [CrossRef]
- Fisher, R.A. On the mathematical foundations of theoretical statistics. Philos. Trans. R. Soc. A 1922, 222, 309–368. [Google Scholar] [CrossRef]
- Cox, D.R.; Hinkley, D.V. Theoretical Statistics, 1st ed.; Chapman & Hall: New York, NY, USA, 1974. [Google Scholar] [CrossRef]
- Frieden, B.R. Fisher information as the basis for diffraction optics. Opt. Lett. 1989, 14, 199–201. [Google Scholar] [CrossRef]
- Yang, F.; Nair, R.; Tsang, M.; Simon, C.; Lvovsky, A.I. Fisher information for far-field linear optical superresolution via homodyne or heterodyne detection in a higher-order local oscillator mode. Phys. Rev. A 2017, 96, 063829. [Google Scholar] [CrossRef]
- Chao, J.; Ward, E.S.; Ober, R.J. Fisher information theory for parameter estimation in single molecule microscopy: Tutorial. J. Opt. Soc. Am. A 2016, 33, B36–B57. [Google Scholar] [CrossRef]
- Kohn, W. Variational methods in nuclear collision problems. Phys. Rev. 1948, 74, 1763–1772. [Google Scholar] [CrossRef]
- Gonze, X.; Käckell, P.; Scheffler, M. Ghost states for separable, norm-conserving, ab initio pseudopotentials. Phys. Rev. B 1990, 41, 12264–12267. [Google Scholar] [CrossRef]
- Schreier, P.J.; Scharf, L.L. Statistical Signal Processing of Complex-Valued Data—The Theory of Improper and Noncircular Signals; Cambridge University Press: Cambridge, UK, 2010; pp. 162–164. [Google Scholar] [CrossRef]
- Tsuda, K.; Kawanabe, M.; Müller, K.-R. Clustering with the Fisher Score. In Proceedings of the 16th Annual Conferece on Neural Information Processing Systems (NIPS), Vancouver, BC, Canada, 9–14 December 2002; Paper 2292, Advances in Neural Information Processing Systems 15. Becker, S., Thrun, S., Obermayer, K., Eds.; MIT Press: Cambridge, MA, USA, 2003; pp. 729–736. [Google Scholar] [CrossRef]
- DeNoyer, L.K.; Dodd, J.G. Smoothing and derivatives in Spectroscopy. In Handbook of Vibrational Spectroscopy; Chalmers, J., Griffiths, P.R., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 2006; p. 2173. [Google Scholar] [CrossRef]
- Chechile, R.A. Bayesian Statistics for Experimental Scientists—A General Introduction Using Distribution-Free Methods; MIT Press: Cambridge, MA, USA, 2020. [Google Scholar]
- Ramos, G.; Carrera, J.; Gómez, S.; Minutti, C.; Camacho, R. A stable computation of log-derivatives from noisy drawdown data. Water Resour. Res. 2017, 53, 7904–7916. [Google Scholar] [CrossRef]
- Heard, H.G. Ultra-violet gas laser at room temperature. Nature 1963, 200, 667. [Google Scholar] [CrossRef]
- Stong, C.L. An unusual kind of gas laser that puts out pulses in the ultraviolet. Sci. Am. 1974, 230, 122–127. [Google Scholar]
- Bergmann, A.; Jansen, S.; Christoffel, S.; Zimmermann, A.; Busch, K.; Hofmann, R. A low-cost setup for microstructuring experiments using a homemade UV laser. Am. J. Phys. 2012, 80, 260–265. [Google Scholar] [CrossRef]
- Herzberg, G. Molecular Spectra and Molecular Structure. I. Spectra of Diatomic Molecules; Van Nostrand: Princeton, NJ, USA, 1950. [Google Scholar]
- Subhash, N.; Kartha, S.C.; Sathianandan, K. New vibrational bands in nitrogen laser emission spectra. Appl. Opt. 1983, 22, 3612–3617. [Google Scholar] [CrossRef]
- Luo, Q.; Liu, W.; Chin, S.L. Lasing action in air induced by ultra-fast laser filamentation. Appl. Phys. B 2003, 76, 337–340. [Google Scholar] [CrossRef]
- Bergé, L.; Skupin, S.; Nuter, R.; Kasparian; Wolf, J.-P. Ultrashort filaments of light in weakly Ionized, optically transparent media. Rep. Prog. Phys. 2007, 70, 1633–1713. [Google Scholar] [CrossRef]
- Dogariu, A.; Michael, J.B.; Scully, M.O.; Miles, R.B. High-gain backward lasing in air. Science 2022, 331, 442–445. [Google Scholar] [CrossRef]
- Kartashov, D.; Ališauskas, S.; Andriukaitis, G.; Pugžlys, A.; Schneider, M.; Zheltikov, A.; Chin, S.L.; Baltuška, A. Free-space nitrogen gas laser driven by a femtosecond filament. Phys. Rev. A 2012, 86, 033831. [Google Scholar] [CrossRef]
- Steinmeyer, G. A breakthrough for remote lasing in air. Physics 2014, 7, 129. [Google Scholar] [CrossRef]
- López, S.; Garcia, A.; Rueda, D.; Oliva, E. 3D modeling of cavity-free lasing in nitrogen plasma filaments. Opt. Express 2023, 31, 8479–8493. [Google Scholar] [CrossRef] [PubMed]
- Kosareva, O.G.; Andreeva, V.A.; Shipilo, D.E.; Savel’ev, A.B.; Shkurinov, A.P.; Kandidov, V.P.; Makarov, V.A. Terahertz and mid-infrared radiation from femtosecond filaments in gases. In Progress in Photon Science—Basics and Applications; Yamanouchi, K., Ed.; Springer Series in Chemical Physics; Springer: Berlin/Heidelberg, Germany, 2017; Volume 115, pp. 35–43. [Google Scholar] [CrossRef]
- Rodriguez, M.; Sauerbrey, R.; Wille, H.; Wöste, L.; Fujii, T.; André, Y.-B.; Mysyrowicz, A.; Klingbeil, L.; Rethmeier, K.; Kalkner, W.; et al. Triggering and guiding megavolt discharges by use of laser-induced ionized filaments. Opt. Lett. 2002, 27, 772–774. [Google Scholar] [CrossRef] [PubMed]
- Vrba, P.; Vrbová, M.; Bobrova, N.A.; Sasorov, P.V. Modelling of a nitrogen x-ray laser pumped by capillary discharge. Cent. Eur. J. Phys. (CEJP) 2005, 3, 564–580. [Google Scholar] [CrossRef]
- Xu, H.L.; Azarm, A.; Bernhardt, J.; Kamali, Y.; Chin, S.L. The mechanism of nitrogen fluorescence inside a femtosecond laser filament in air. Chem. Phys. 2009, 360, 171–175. [Google Scholar] [CrossRef]
- Yao, J.; Xie, H.; Zeng, B.; Chu, W.; Li, G.; Ni, J.; Zhang, H.; Jing, C.; Zhang, C.; Xu, H.; et al. Gain dynamics of a free-space nitrogen laser pumped by circularly polarized femtosecond laser pulses. Opt. Express 2014, 22, 19005–19013. [Google Scholar] [CrossRef]
- Kossyi, I.A.; Kostinsky, A.Y.; Matveyev, A.A.; Silakov, V.P. Kinetic scheme of the non-equilibrium discharge in nitrogen-oxygen mixtures. Plasma Sources Sci. Technol. 1992, 1, 207–220. [Google Scholar] [CrossRef]
- Fons, J.T.; Schappe, R.S.; Lin, C.C. Electron-impact excitation of the second positive band system (C3Πu-->B3Πg) and the C3Πu electronic state of the nitrogen molecule. Phys. Rev. A 1996, 53, 2239–2247. [Google Scholar] [CrossRef]
- Persephonis, P.; Giannetas, V.; Ioannou, A.; Parthenios, J.; Georgiades, C. The time dependent resistance and inductance of the electric discharges in pulsed gas lasers. IEEE J. Quant. Electron. 1995, 31, 1779–1784. [Google Scholar]
- Silva, A.V.; Tsui, K.H.; Pimentel, N.P.; Massone, C.A. Plasma electronics in pulsed nitrogen lasers. IEEE J. Quant. Electron. 1992, 28, 1937–1940. [Google Scholar] [CrossRef]
- Panchenko, A.N.; Tarasenko, V.F.; Lomaev, M.I.; Panchenko, N.A.; Suslov, A.I. Efficient N2 laser pumped by nanosecond diffuse discharge. Opt. Commun. 2019, 430, 210–218. [Google Scholar] [CrossRef]
- Castro, M.P.P.; Fellows, C.E.; Massone, C.A. Simultaneous emission of seven bands in the N2 2+ system by current confinement and discharge channel plasma inductance reduction. Opt. Commun. 1993, 102, 53–58. [Google Scholar] [CrossRef]
- Rogowski, W.; Steinhaus, W. Die Messung der magnetischen Spannung. (Messung des Linienintegrals der magnetischen Feldstärke). Arch. Elektrotechnik 1912, 1, 141–150. [Google Scholar] [CrossRef]
- Grunwald, R.; Hertz, J.H. Über die Kleinsignalgewinnmessung nach Ladenburg-Levy (About the small signal gain measurement after Ladenburg and Levy). Ann. Phys. 1986, 498, 201–212. [Google Scholar] [CrossRef]
- Lademann, J.; König, R.; Kudryavtsev, Y.; Albrecht, H.; Fritsch, G.; Grunwald, R.; Winkelmann, G. Ein einfacher Excimerlaser mit automatischer Vorionisation (A simple excimer laser with automatic pre-ionization). Exp. Tech. Phys. 1984, 32, 235–246. [Google Scholar]
- Grunwald, R. Untersuchungen der Spontanen Emission eines Diffusen N2-Laser-Plasmas (Investigations of the Spontaneous Emission of a Diffuse N2-Laser Plasma). Diploma Thesis, Humboldt University Berlin, Berlin, Germany, 1982. (In German). [Google Scholar]
- Grunwald, R.; Hertz, J.H. Messungen der spontanen Emission an einem diffusen N2-Laserplasma (Measurements of the spontaneous emission of a diffuse N2-laser plasma). In Proceedings of the 6th Conference Physik und Technik des Plasmas, Leipzig, Germany, 5–8 July 1982; Volume 133. [Google Scholar]
- Becker, K.H.; Engels, H.; Tatarczyk, T. Lifetime measurements of the C3∏u state of nitrogen by laser-induced fluorescence. Chem. Phys. Lett. 1977, 51, 111–115. [Google Scholar] [CrossRef]
- Shemansky, D.E.; Broadfoot, A.L. Excitation of N2 and N2+ systems by electrons–I Absolute transition probabilities. J. Quant. Spectrosc. Radiat. Transf. 1971, 11, 1385–1400. [Google Scholar] [CrossRef]
- Lofthus, A.; Krupenie, P.H. The spectrum of molecular nitrogen. J. Phys. Chem. Ref. Data 1977, 6, 113–307. [Google Scholar] [CrossRef]
- Valk, F.; Aints, M.; Paris, P.; Plank, T.; Maksimov, J.; Tamm, A. Measurement of collisional quenching rate of nitrogen states N2(C3∏u, υ = 0) and N2+(B2∑+g, υ = 0). J. Phys. D Appl. Phys. 2010, 43, 385202. [Google Scholar] [CrossRef]
- Strak, P.; Koronski, K.; Sakowski, K.; Sobczak, K.; Borysiuk, J.; Korona, K.P.; Suchocki, A.; Monroy, E.; Krukowski, S.; Kaminska, A. Exact method of determination of the recombination mode from time resolved photoluminescence data. arXiv 2017, arXiv:1709.05249v4. [Google Scholar]
- Eberly, J.H. Extended two-level theory of the exponential index of multiphoton processes. Phys. Rev. Lett. 1979, 42, 1049–1052. [Google Scholar] [CrossRef]
- Allen, L.; McMahon, D. The exponential index of multiphoton processes in two-photon absorption. J. Phys. B At. Mol. Phys. 1983, 16, L721–L725. [Google Scholar] [CrossRef]
- Persistent Lines of Neutral Molybdenum (Mo I), Basic Atomic Spectroscopic Data, National Institute of Standards and Technology (NIST). Available online: https://physics.nist.gov/PhysRefData/Handbook/Tables/molybdenumtable3_a.htm (accessed on 23 October 2024).
- Grunwald, R. Experimentelle Untersuchungen zur XeCl-Laser-Induzierten Stoßfreien UV-Mehrphotonendissoziation Ausgewählter Organischer und Metallorganischer Moleküle (Experimental Investigations of the XeCl-Laser Induced Collision-Free UV Multiphoton Dissociation of Selected Organic and Metal-Organic Molecules), Erroneously, an Excitation Wavelength of 337 nm Was Assumed Instead of 308 nm. The Corrected Energetic Scheme Indicates a Dominant 3-Photon Channel in Good Agreement with the Detected Nonlinear Order. Ph.D. Thesis, Humboldt University Berlin, Berlin, Germany, 1986. (In German). [Google Scholar]
- Pilcher, G.; Warec, M.J.; Pittam, D.A. The thermodynamic properties of chromium, molybdenum and tungsten hexacarbonyls in the gaseous state. J. Less-Common Met. 1975, 42, 223. [Google Scholar] [CrossRef]
- Tyndall, G.W.; Jackson, R.L. Single-photon and multiphoton dissociation of molybdenum hexacarbonyl at 248 nm. J. Phys. Chem. 1991, 95, 687–693. [Google Scholar] [CrossRef]
- Radloff, W.; Hohmann, H.; Ritze, H.-H.; Paul, R. Excimer laser photolysis of molybdenum hexacarbonyl with buffer gas. Appl. Phys. B 1989, 49, 301–305. [Google Scholar] [CrossRef]
- Lenz, K.; Grunwald, R.; Weigmann, H.-J. Laser assisted deposition of carbon and polymer layers. In Proceedings of the 5th International Conference on Lasers and Their Applications (ILA 5), Dresden, Germany, 28 October–1 November 1985; p. 208. [Google Scholar]
- Grunwald, R.; Hertz, J.H. Nachweis eines optischen Gewinns nach UV-Mehrphotonen-Dissoziation von Molybden-Hexacarbonyl (Detection of an optical gain after UV-multiphoton dissociation of molybdenum hexacarbonyl). Ann. Phys. 1986, 7, 499–504. (In German) [Google Scholar] [CrossRef]
- Grunwald, R. Intensity dependent geometry of multiple photon dissociation zones. In Proceedings of the Lasers ’88 Conference, Plovdiv, Bulgaria, 10–14 October 1988; pp. 91–92. [Google Scholar]
- Grunwald, R. Verfahren und Anordnung zur Leistungsmessung von Laserstrahlung (Method and Arrangement for Measuring Laser Power). Patent Application Documents D-WPG01J/2909076, 16 September 1987. [Google Scholar]
- McWilliam, I.G. Derivative spectroscopy and its application to the analysis of unresolved bands. Anal. Chem. 1969, 41, 674–676. [Google Scholar] [CrossRef]
- Grushka, E.; Monacelli, G.C. Slope analysis for recognition and characterization of strongly overlapped chromatographic peaks. Anal. Chem. 1972, 44, 484–489. [Google Scholar] [CrossRef]
- Vandeginste, B.G.M.; De Galan, L. Critical evaluation of curve fitting in infrared spectrometry. Anal. Chem. 1975, 47, 2124–2134. [Google Scholar] [CrossRef]
- Fleissner, G.; Hage, W.; Hallbrucker, A.; Mayer, E. Improved curve resolution of highly overlapping bands by comparison of fourth-derivative curves. Appl. Spectrosc. 1996, 50, 1235–1245. [Google Scholar] [CrossRef]
- Morrey, J.R. On Determining spectral peak positions from composite spectra with a digital computer. Anal. Chem. 1968, 40, 905–914. [Google Scholar] [CrossRef]
- Maddams, W.F.; Mead, W.L. The measurement of derivative i.r. spectra—I. Background studies. Spectrochim. Acta 1982, 38, 437–444. [Google Scholar] [CrossRef]
- Hawkes, S.; Maddams, W.F.; Mead, W.L.; Southon, M.J. The measurement of derivative i.r. spectra—II. Experimental measurements. Spectrochim. Acta 1982, 38, 445–457. [Google Scholar] [CrossRef]
- Holler, F.; Burns, D.H.; Callis, J.B. Direct use of second derivatives in curve-fitting procedures. Appl. Spectrosc. 1989, 43, 877–882. [Google Scholar] [CrossRef]
- Griffiths, T.R.; King, K.; Hubbard, H.V.S.A.; Schwing-Weill, M.J.; Meullemeestre, J. Some aspects of the scope and limitations of derivative spectroscopy. Anal. Chim. Acta 1982, 143, 163–176. [Google Scholar] [CrossRef]
- Chen, L.; Garland, M. Computationally efficient curve-fitting procedure for large two-dimensional experimental infrared spectroscopic arrays using the Pearson VII model. Appl. Spectrosc. 2003, 57, 323–330. [Google Scholar] [CrossRef] [PubMed]
- Fernández-González, A.; Montejo-Bernardo, J.M. Natural Logarithm Derivative Method: A novel and easy methodology for finding maximums in overlapping experimental peaks. Spectrochim. Acta Part A 2009, 74, 714–718. [Google Scholar] [CrossRef]
- Goldston, D.A.; Gonek, S.M.; Montgomery, H.L. Mean values of the logarithmic derivative of the Riemann zeta-function with applications to primes in short intervals. J. Reine Angew. Math. 2001, 537, 105–126. [Google Scholar] [CrossRef]
- Farkas, H.M.; Godin, Y. Logarithmic derivatives of theta functions. Isr. J. Math. 2005, 148, 253–265. [Google Scholar] [CrossRef]
- Yamagata, K. Maximum logarithmic derivative bound on quantum state estimation as a dual of the Holevo bound. J. Math. Phys. 2021, 62, 062203. [Google Scholar] [CrossRef]
- Sidhu, J.S.; Kok, P. A geometric perspective on quantum parameter estimation. AVS Quant. Sci. 2020, 2, 014701. [Google Scholar] [CrossRef]
- Haböck, Multivariate Lookups Based on Logarithmic Derivatives, Cryptology ePrint Archive, Paper 2022/1530. Available online: https://ia.cr/2022/1530 (accessed on 27 March 2025).
- Xie, T.; Li, L.; Sun, Z. Comparison of log-derivative and renormalized Numerov methods in low-energy two-body collisions. Phys. Scr. 2023, 98, 125413. [Google Scholar] [CrossRef]
- Nakajima, S.; Utsumi, Y. Symmetric-logarithmic-derivative Fisher information for kinetic uncertainty relations. Phys. Rev. E 2023, 108, 054136. [Google Scholar] [CrossRef] [PubMed]
- Sinha, K.B.; Sengupta, R. The likelihood operator and Fisher information in quantum probability. arXiv 2025, arXiv:2502.20055v1. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grunwald, R. The Logarithmic Derivative in Scientific Data Analysis. Encyclopedia 2025, 5, 44. https://doi.org/10.3390/encyclopedia5020044
Grunwald R. The Logarithmic Derivative in Scientific Data Analysis. Encyclopedia. 2025; 5(2):44. https://doi.org/10.3390/encyclopedia5020044
Chicago/Turabian StyleGrunwald, Ruediger. 2025. "The Logarithmic Derivative in Scientific Data Analysis" Encyclopedia 5, no. 2: 44. https://doi.org/10.3390/encyclopedia5020044
APA StyleGrunwald, R. (2025). The Logarithmic Derivative in Scientific Data Analysis. Encyclopedia, 5(2), 44. https://doi.org/10.3390/encyclopedia5020044





