Approaches to the Development of Advanced Alloys Based on Refractory Metals
Abstract
1. Introduction
2. Features of the Polyphase (Heterophase) Structure in the HRAs
2.1. Dispersion-Strengthened Alloys
2.2. Eutectic Alloys
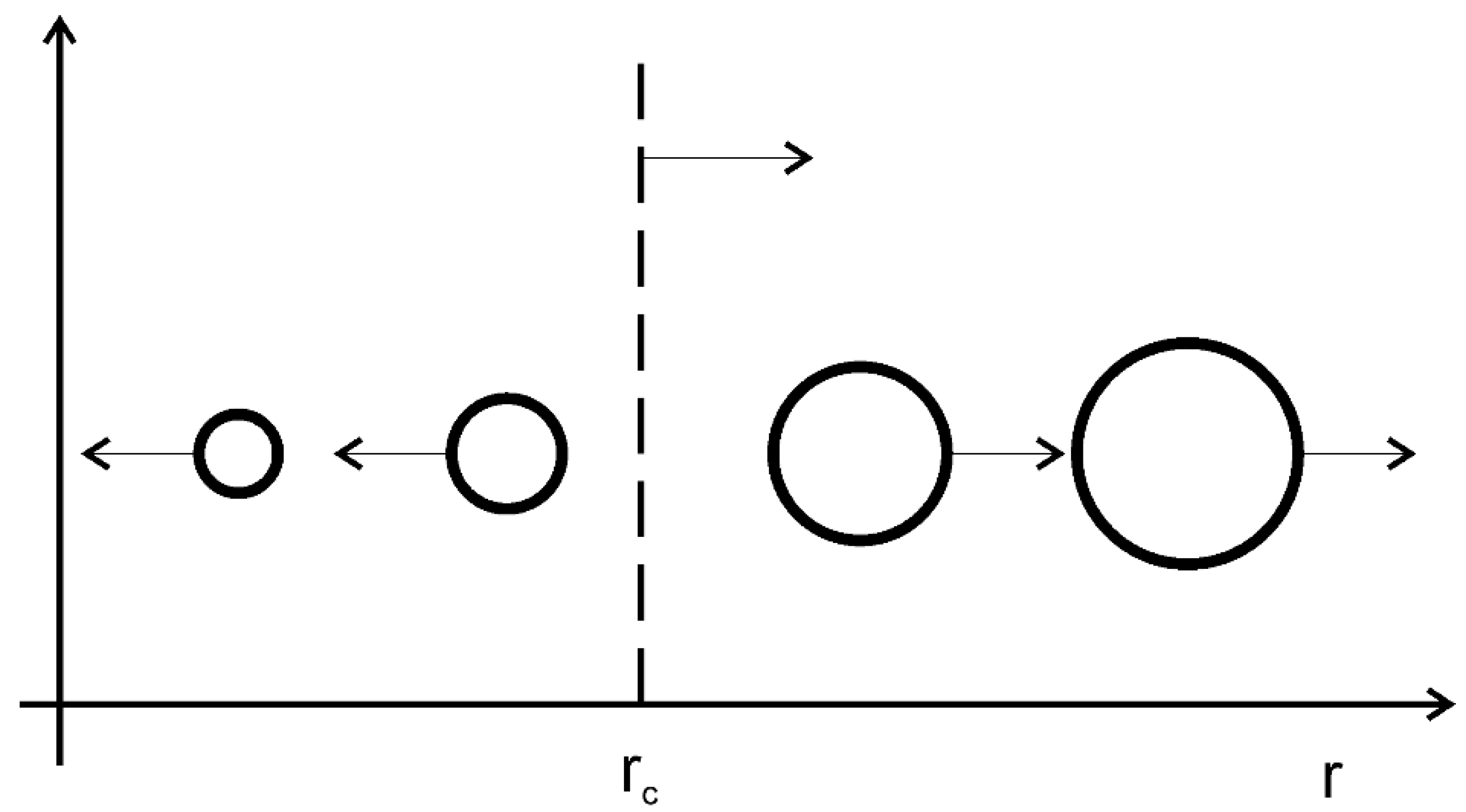
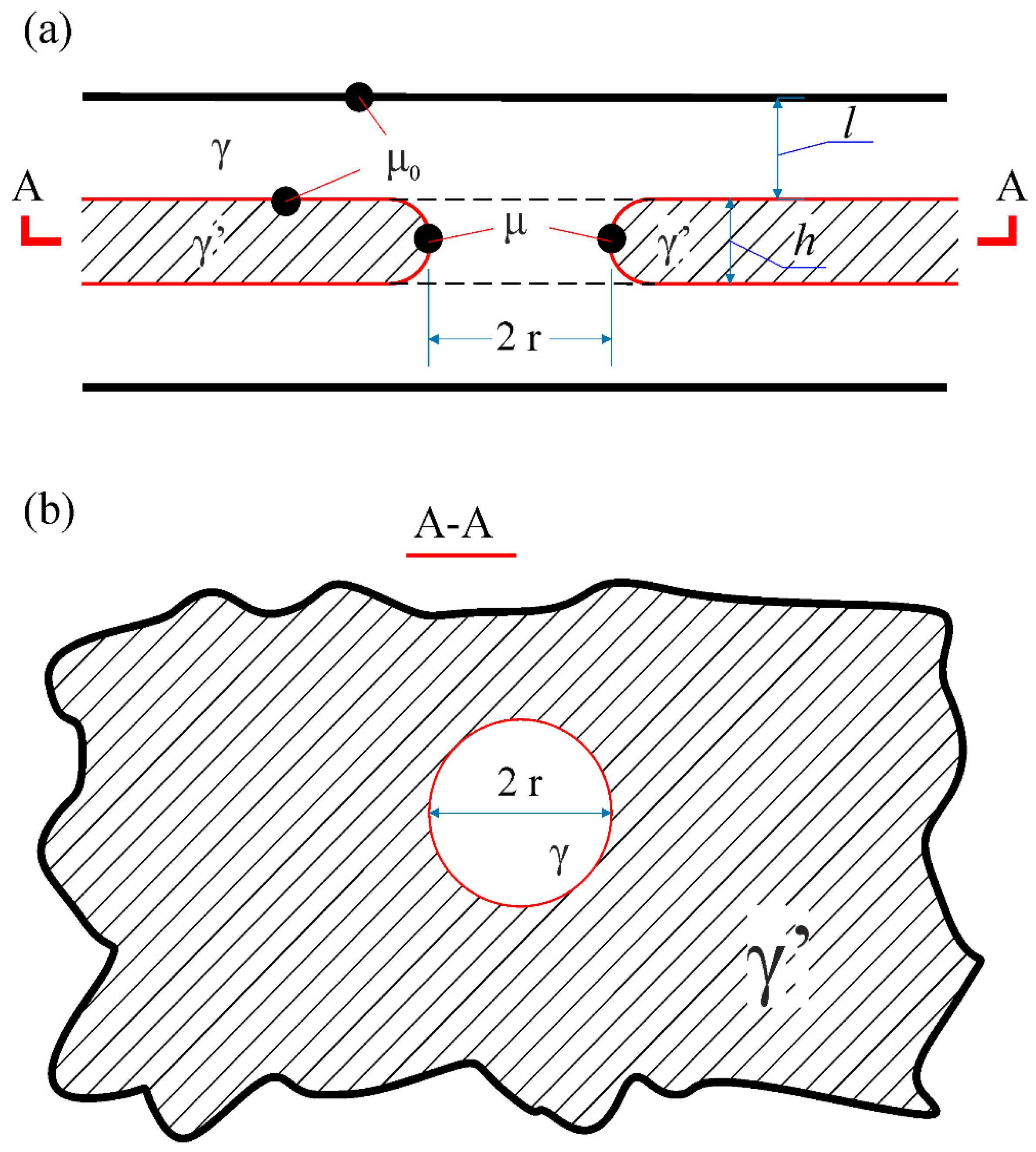
2.3. Stability of the Polyphase Structure of HRAs: Kinetics of the Diffusion Coarsening Process
3. Approaches to the Development of HRAs Based on Refractory Metals
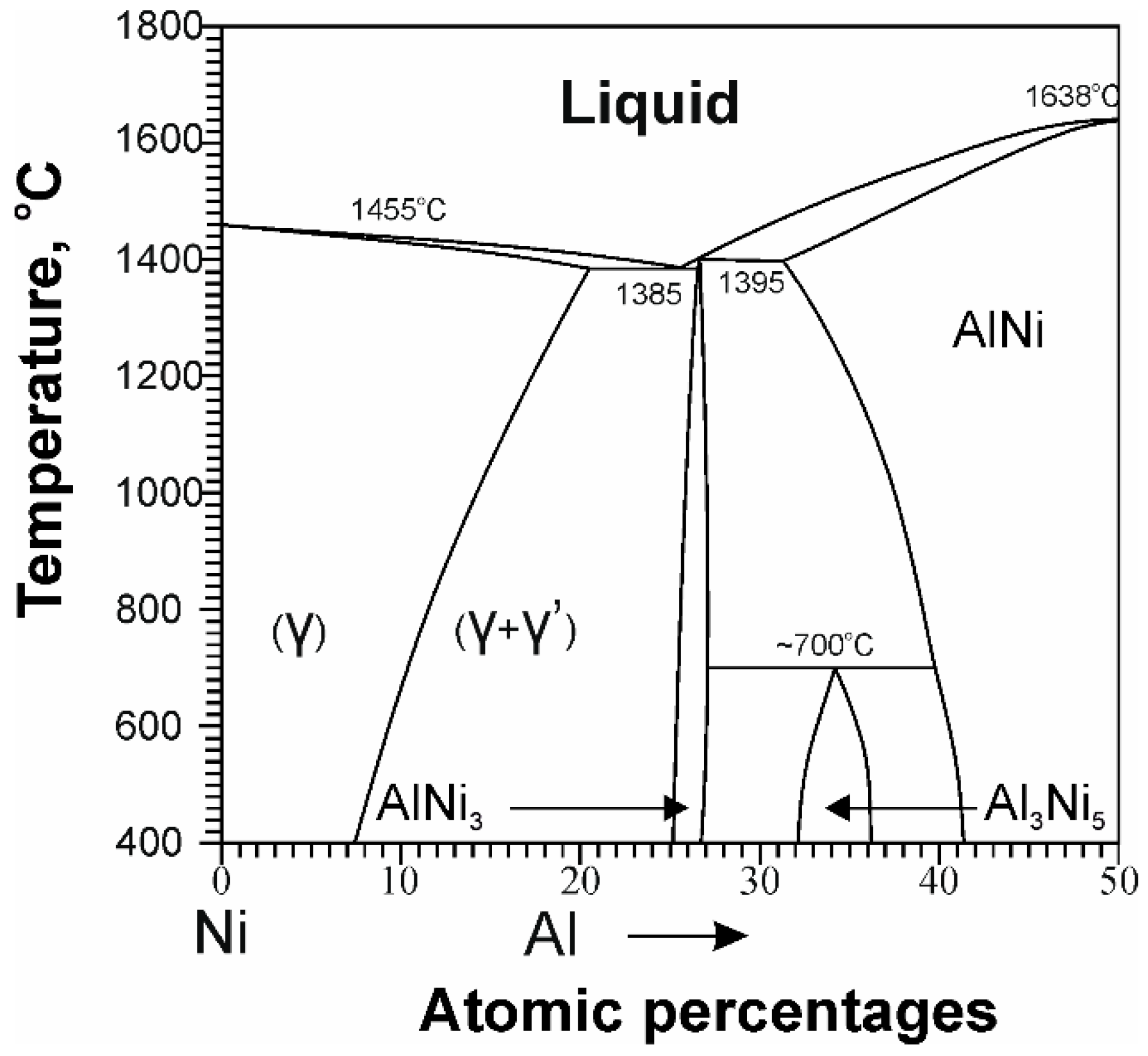
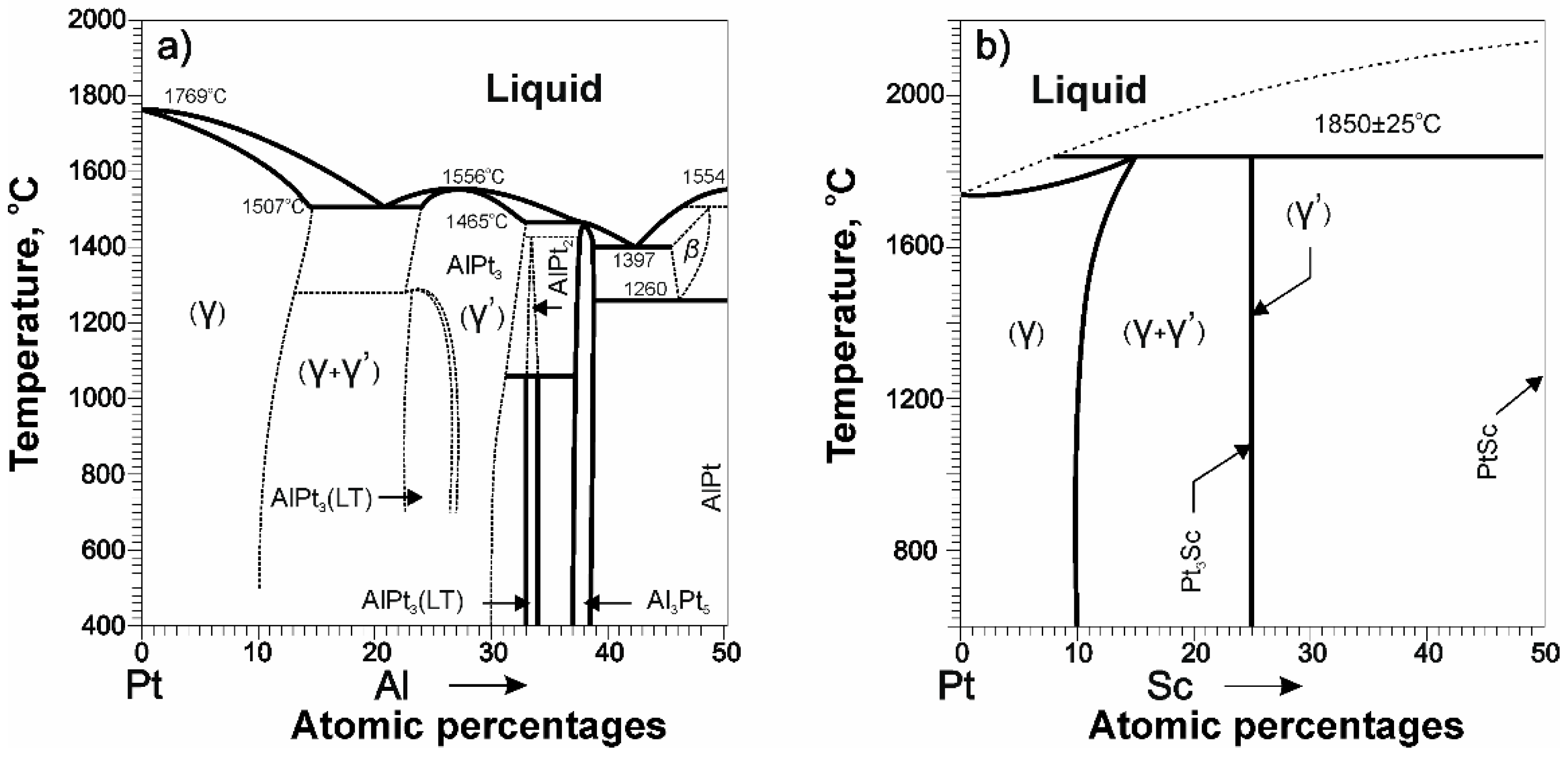
3.1. Alloys Based on Noble Metals
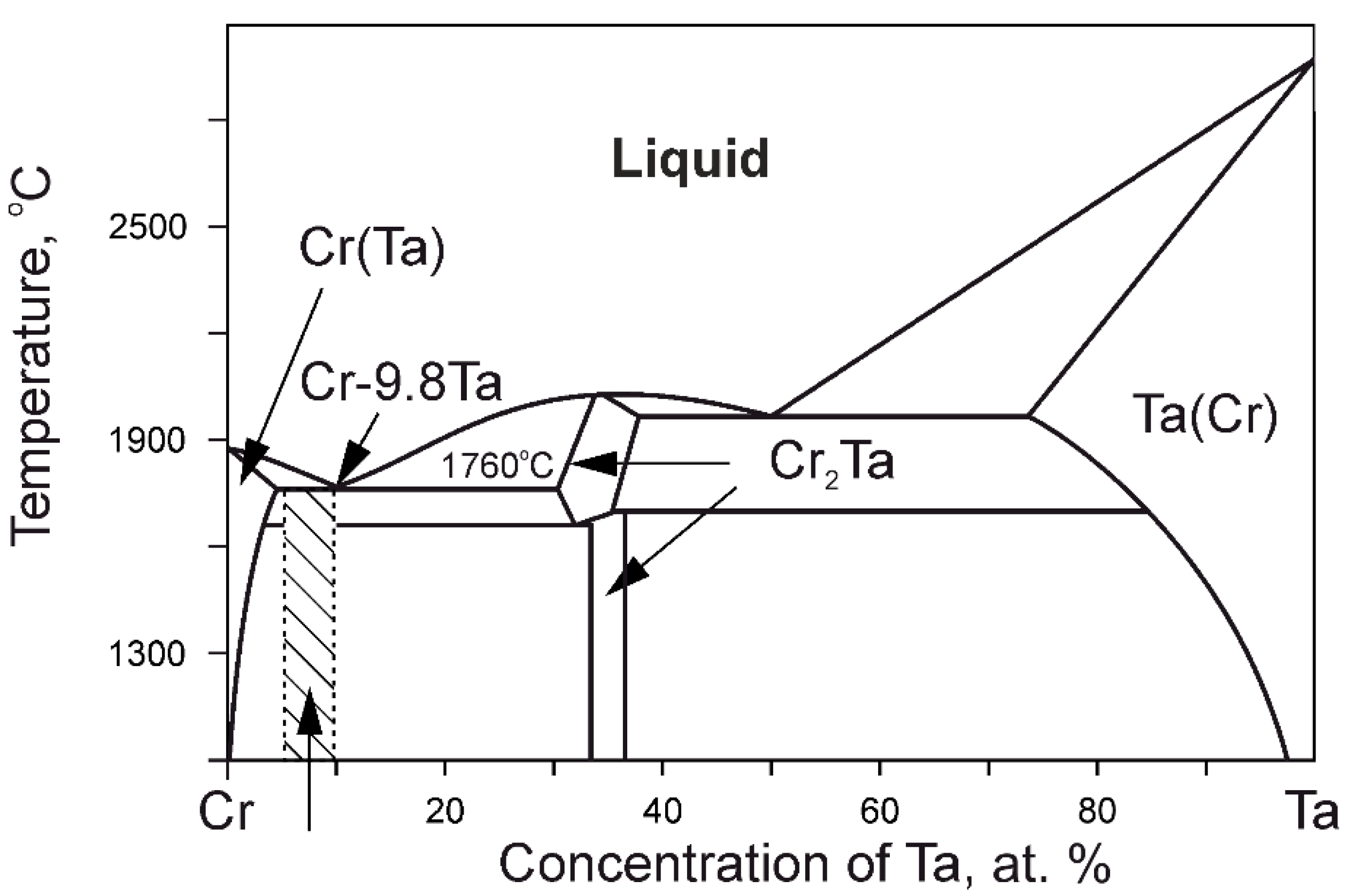
3.2. Alloys Based on Many Refractory Metals
4. Final Notes
Author Contributions
Funding
Conflicts of Interest
References
- Sims, C.T.; Stoloff, N.S.; Hagel, W.C. Superalloys II: High-Temperature Materials for Aerospace and Industrial Power; John Wiley & Sons, Ltd.: New York, NY, USA, 1987. [Google Scholar]
- Kablov, E. Cast Blades of Gas Turbine Engines: Alloys, Technologies, Coatings; MISiS: Moscow, Russia, 2001. (In Russian) [Google Scholar]
- Reed, R.C. The Superalloys: Fundamentals and Applications; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar]
- Logunov, A. Nickel Superalloys for Gas Turbine Blades and Disks; Izd. Dom Gazoturb. Tekhnol.: Rybinsk, Russia, 2017. (In Russian) [Google Scholar]
- Arzamasov, B.N.; Solov’eva, T.V. (Eds.) Handbook of Structural Materials; Publishing House of Bauman Moscow State Technical University: Moscow, Russia, 2005. (In Russian) [Google Scholar]
- Senkov, O.N.; Tsakiropoulos, P.; Couzinié, J.-P. Special Issue “Advanced Refractory Alloys”: Metals, MDPI. Metals 2022, 12, 333. [Google Scholar] [CrossRef]
- Fisher, G.; Datta, P.K.; Burnell-Gray, J.S. An assessment of the oxidation resistance of an iridium and an iridium/platinum low-activity aluminide/MarM002 system at 1100 °C. Surf. Coat. Technol. 1999, 113, 259–267. [Google Scholar] [CrossRef]
- Rytvin, E.I. Heat Resistance of Platinum Alloys; Izd. Metallurgiya: Moscow, Russia, 1987. (In Russian) [Google Scholar]
- Timofeev, N.I.; Ermakov, A.V.; Dmitriev, V.A.; Panfilov, P.E. Fundamentals of Metallurgy and Production Technology of Iridium Products; Ural Branch of the Russian Academy of Sciences: Yekaterinburg, Russia, 1996. (In Russian) [Google Scholar]
- Kablov, E.N. Scientific and Biographical Sketch: S. T. Kishkin. In S. T. Kishkin. Creation, Research and Application of Heat-Resistant Alloys. Selected Works; Nauka Publishing House: Moscow, Russia, 2006. (In Russian) [Google Scholar]
- Chandra Sekhar Tiwary, Prafull Pandey, Suman Sarkar, Rakesh Das, Sumanta Samal, Krishanu Biswas, Kamanio Chattopadhyay. Five decades of research on the development of eutectic as engineering materials. Prog. Mater. Sci. 2022, 123, 100793. [CrossRef]
- Massalski, T.B.; Okamoto, H.; Subramanian, P.R.; Kacprzak, L. Binary Alloy Phase Diagrams, 2nd ed.; ASM International: Materials Park, OH, USA, 1986. [Google Scholar]
- Razumovskii, I.M.; Ruban, A.V.; Razumovskiy, V.I.; Logunov, A.V.; Larionov, V.N.; Ospennikova, O.G.; Poklad, V.A.; Johansson, B. New generation of Ni-based superalloys designed on the basis of first-principles calculations. Mater. Sci. Eng. A 2008, 497, 18–24. [Google Scholar] [CrossRef]
- Bokshtein, S.Z.; Ginsburg, S.S.; Razumovskii, I.M.; Kishkin, S.T.; Stroganov, G.B. Autoradiography of Interfaces and Structural Stability of Alloys; Izd. Metallurgiya: Moscow, Russia, 1987. (In Russian) [Google Scholar]
- Pineau, A. Influence of uniaxial stress on the morphology of coherent precipitates during coarsening. Acta Metall. 1976, 24, 559–564. [Google Scholar] [CrossRef]
- Nathal, M.V.; Ebert, L.J. Elevated temperature creep-rupture behavior of the single crystal nickel-base superalloy NASAIR-100. Met. Trans. 1985, 16, 427–439. [Google Scholar] [CrossRef]
- Matan, N.; Cox, D.C.; Rae, M.F.; Reed, R.C. On the kinetics of rafting in CMSX-4 superalloy single crystals. Acta Mater. 1999, 47, 2031–2045. [Google Scholar] [CrossRef]
- Zhao, J.-C.; Jackson, M.R.; Peluso, L.A. Mapping of the Nb–Ti–Si phase diagram using diffusion multiples. Mater. Sci. Eng. A 2004, 372, 21–27. [Google Scholar] [CrossRef]
- Bewlay, B.P.; Jackson, M.R.; Lipsitt, H.A. The Balance of Mechanical and Environmental Properties of a Multielement Niobium-Niobium Silicide-Based In-Situ Composite. Metall. Mater. Trans. A 1996, 27, 3801–3808. [Google Scholar] [CrossRef]
- Bewlay, B.P.; Jackson, M.R.; Subramanian, P.R. Processing high temperature refractory metal-silicide in situ composites. J. Met. JOM 1999, 51, 32–36. [Google Scholar] [CrossRef]
- Liu, C.T.; Zhu, J.H.; Brady, M.P.; McKamey, C.G.; Pike, L.M. Physical metallurgy and mechanical properties of transition-metal Laves phase alloys. Intermetallics 2000, 8, 1119–1129. [Google Scholar] [CrossRef]
- Brady, M.P.; Zhu, J.H.; Liu, C.T.; Tortorelli, P.F.; Walker, L.R.; McKamey, C.G.; Wright, J.L.; Carmichael, C.A.; Larson, D.J.; Miller, M.K.; et al. Intermetallic reinforced Cr alloys for high-temperature use. Mater. High Temp. 1999, 16, 189–193. [Google Scholar] [CrossRef]
- Somov, A.I.; Tikhonovsky, M.A. Eutectic Compositions; Izd. Metallurgiya: Moscow, Russia, 1975. (In Russian) [Google Scholar]
- Walter, J.L.; Cline, H.E. Structure and properties of cobalt-base—TaC eutectic alloys. Metall. Trans. 1973, 4, 1775–1784. [Google Scholar] [CrossRef]
- Woodford, D.A. Creep and rupture of an advanced fiber strengthened eutectic composite superalloy. Metall. Transaction. 1977, 8, 639–650. [Google Scholar] [CrossRef]
- Kachanov, E.B.; Petrushin, N.V.; Svetlov, I.L. Heat-resistant eutectic alloys with carbide-intermetallic strengthening. Met. Sci. Heat Treat. 1995, 37, 24–29. [Google Scholar] [CrossRef]
- Lifshitz, I.M.; Slyozov, V.V. The kinetics of precipitation from supersaturated solid solutions. J. Phys. Chem. Solids 1961, 19, 35–50. [Google Scholar] [CrossRef]
- Wagner, C. Theorie der Alterung von Niderschlagen durch Umlosen. Zs. Electrochem. 1961, 65, 581–591. [Google Scholar]
- Razumovskii, I.; Bokstein, B.; Logacheva, A.; Logachev, I.; Razumovsky, M. Cohesive Strength and Structural Stability of the Ni-Based Superalloys. Materials 2022, 15, 200. [Google Scholar] [CrossRef]
- Mishin, Y.; Orekhov, N.; Alyoshin, G.; Noat, P.; Razumovskii, I. Model of diffusion coarsening of the raft structure in single crystals of Ni-based superalloys. Mater. Sci. Eng. A 1993, 171, 163–168. [Google Scholar] [CrossRef]
- Cline, H.E. Shape instabilities of eutectic composites at elevated temperatures. Acta Metall. 1971, 19, 481–491. [Google Scholar] [CrossRef]
- Bokstein, B.S. Diffusion in Metals, 2nd ed.; Lenand: Moscow, Russia, 2019. (In Russian) [Google Scholar]
- Grushko, B.; Samuha, S.; Meshi, L. A study of the Al-Pt-Ir phase diagram. J. Alloy. Compd. 2015, 646, 873–878. [Google Scholar] [CrossRef]
- Savitsky, E.; Polyakova, V.; Gorina, N.; Roshan, N. Physical Metallurgy of Platinum Metals; Pergamon Press: Oxford, UK, 1979. [Google Scholar]
- Johanesson, G.H.; Bligaard, T.; Ruban, A.V.; Skriver, H.L.; Jacobsen, K.W.; Norskov, J.K. Combined Electronic Structure and Evolutionary Search Approach to Materials Design. Phys. Rev. Lett. 2002, 88, 255506. [Google Scholar] [CrossRef]
- Kaufman, L.; Ågren, J. CALPHAD, first and second generation—Birth of the materials genome. Scr. Mater. 2014, 70, 3–6. [Google Scholar] [CrossRef]
- Hill, P.J.; Yamabe-Mitarai, Y.; Wolff, I.M. High-temperature compression strengths of precipitation-strengthened ternary Pt-Al-X alloys. Scr. Mater. 2001, 44, 43–48. [Google Scholar] [CrossRef]
- Cornish, L.A.; Suss, R.; Chrown, L.H.; Taylor, S.; Glaner, L.; Douglas, A.; Prins, S.N. Platinum-based alloys for high temperature and special applications. In Proceedings of the International Platinum Conference ‘Platinum Adding Value’; The South African Institute of Mining and Metallurgy: Johannesburg, South Africa, 2004; pp. 329–336. [Google Scholar]
- Yao, X.; Guo, Y.-F.; Tang, X.-Z.; Xiong, K.; Mao, Y. First-principles study on the effects of the alloying elements on the structural stability and mechanical properties of γ-Pt/γ’-Pt3X (X=Al, Hf, Zr) interfaces. Appl. Surf. Sci. 2022, 605, 154744. [Google Scholar] [CrossRef]
- Gornostyrev, Y.N.; Kontsevoi, O.Y.; Maksyutov, A.F.; Freeman, A.J.; Katsnelson, M.I.; Trefilov, A.V.; Lichtenshtein, A.I. Negative yield stress temperature anomaly and structural instability of Pt3Al. Phys. Rev. B 2004, 70, 014102. [Google Scholar] [CrossRef]
- Razumovskiy, V.I.; Isaev, E.I.; Ruban, A.V.; Korzhavyi, P.A. Ab-initio calculations of elastic properties of Pt-Sc alloys. Intermetallics 2008, 16, 982–986. [Google Scholar] [CrossRef]
- Arkhipova, N.T.; Klotsman, S.M.; Polikarpova, I.P.; Timofeev, A.N.; Satkowski, O.P. Volume self-diffusion of Ir-192 in iridium single crystals. Fiz. Met. I Metalloved. 1986, 62, 1181–1185. [Google Scholar]
- Cattaneo, E.; Germagnoli, E.; Grasso, F. Self-diffusion in platinum. Phil. Mag. 1962, 7, 1373–1383. [Google Scholar] [CrossRef]
- Cantor, B. Multicomponent high-entropy Cantor alloys. Prog. Mater. Sci. 2021, 120, 100754. [Google Scholar] [CrossRef]
- Yeh, J.W. Alloy design strategies and future trends in high-entropy alloys. JOM 2013, 65, 1759–1771. [Google Scholar] [CrossRef]
- Pickering, E.J.; Jones, N.G. High-entropy alloys: A critical assessment of their founding principles and future prospects. Int. Mater. Rev. 2016, 61, 183–202. [Google Scholar] [CrossRef]
- Miracle, D.B.; Senkov, O.N. A critical review of high entropy alloys and related concepts. Acta Mater. 2017, 122, 448–511. [Google Scholar] [CrossRef]
- Morgan, D.; Zhang, Y. Comment on “Thermal vacancies in random alloys in the single-site mean-field approximation”. Phys. Rev. B 2020, 101, 136101. [Google Scholar] [CrossRef]
- Divinski, S.V.; Lukianova, O.A.; Wilde, G.; Dash, A.; Esakkiraja, N.; Paul, A. High-Entropy Alloys: Diffusion. Encycl. Mater. Met. Alloys 2022, 2, 402–416. [Google Scholar] [CrossRef]
- Zhang, J.; Gadelmeier, C.; Sen, S.; Wang, R.; Zhang, X.; Zhong, Y.; Glatzel, U.; Grabowski, B.; Wilde, G.; Divinski, S.V. Zr diffusion in BCC refractory high entropy alloys: A case of ‘non-sluggish’ diffusion behavior. Acta Mater. 2022, 233, 117970. [Google Scholar] [CrossRef]
- Savitsky, E.M.; Burkhanov, G.S. Physical Metallurgy of Refractory Metals and Alloys; Moscow, Nauka Publishing House: Moscow, Russia, 1967. (In Russian) [Google Scholar]
- Senkov, O.N.; Scott, J.M.; Senkova, S.V.; Miracle, D.B.; Woodward, C.F. Microstructure and room temperature properties of a high-entropy TaNbHfZrTi alloy. J. Alloys Compd. 2011, 509, 6043–6048. [Google Scholar] [CrossRef]
- Senkov, O.N.; Scott, J.M.; Senkova, S.V.; Meisenkothen, F.; Miracle, D.B.; Woodward, C.F. Microstructure and elevated temperature properties of a refractory TaNbHfZrTi alloy. J. Mater. Sci. 2012, 47, 4062–4074. [Google Scholar] [CrossRef]
- Senkov, O.N.; Semiatin, S.L. Microstructure and Properties of a Refractory High-Entropy Alloy after Cold Working. J. Alloys Compd. 2015, 649, 1110–1123. [Google Scholar] [CrossRef]
- Senkov, O.N.; Pilchak, A.L.; Semiatin, S.L. Effect of Cold Deformation and Annealing on the Microstructure and Tensile Properties of a HfNbTaTiZr Refractory High Entropy Alloy. Metall. Mater. Trans. A 2018, 49A, 2876–2892. [Google Scholar] [CrossRef]
- Portnoi, V.K.; Leonov, A.V.; Gusakov, M.S.; Logachev, I.A.; Fedotov, S.A. Preparation of high-temperature multicomponent alloys by the method of mechanochemical synthesis of refractory elements. Inorg. Mater. 2019, 55, 219–223. [Google Scholar] [CrossRef]
- Málek, J.; Zýka, J.; Luká, F.; Vilémová, M.; Vlasák, T.; Cížek, J.; Melikhova, O.; Machácková, A.; Kim, H.-S. The Effect of Processing Route on Properties of HfNbTaTiZr High Entropy Alloy. Materials 2019, 12, 4022. [Google Scholar] [CrossRef] [PubMed]
- Fazakas, É.; Zadorozhnyy, V.; Varga, L.K.; Inoue, A.; Louzguine-Luzgin, D.V.; Tian, F.; Vitos, L. Experimental and theoretical study of Ti20Zr20Hf20Nb20 × 20 (X = V or Cr) refractory high-entropy alloys. Int. J. Refract. Met. Hard Mater. 2014, 47, 131–138. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, Y.; Li, Y.; Chen, X.; Zhang, H. Microstructure and mechanical properties of a refractory HfNbTiVSi0.5 high entropy alloy composite. Mater. Lett. 2016, 174, 82–85. [Google Scholar] [CrossRef]
- Miracle, D.B.; Tsai, M.-H.; Senkov, O.N.; Soni, V.; Banerjee, R. Refractory high entropy superalloys (RSAs). Scr. Mater. 2020, 187, 445–452. [Google Scholar] [CrossRef]
- Körmann, F.; Kostiuchenko, T.; Shapeev, A.; Neugebauer, J. B2 ordering in body-centered-cubic AlNbTiV refractory high-entropy alloys. Phys. Rev. Mater. 2021, 5, 053803. [Google Scholar] [CrossRef]
- Razumovskiy, V.; Lozovoi, A.; Razumovskii, I. First principles aided design of a new Ni-base superalloy: Influence of transition metal alloying elements on grain boundary and bulk cohesion. Acta Mater. 2015, 82, 369–377. [Google Scholar] [CrossRef]
- Kittel, C. Introduction to Solid State Physics, 7th ed.; John Wiley & Sons, Ltd.: New York, NY, USA, 1996. [Google Scholar]
- Rice, J.; Wang, J.-S. Embrittlement of interfaces by solute segregation. Mater. Sci. Eng. A 1989, 107, 23–40. [Google Scholar] [CrossRef]
- Rice, J.R.; Thompson, R. Ductile versus brittle behaviour of crystals. Phil. Mag. 1974, 29, 73–97. [Google Scholar] [CrossRef]
- Scheiber, D.; Pippan, R.; Puschnig, P.; Romaner, L. Ab initio calculations of grain boundaries in bcc metals. Model. Simul. Mater. Sci. Eng. 2016, 24, 035013. [Google Scholar] [CrossRef]
- Scheiber, D.; Razumovskiy, V.I.; Puschnig, P.; Pippan, R.; Romaner, L. Ab initio description of segregation and cohesion of grain boundaries in W–25 at % Re alloys. Acta Mater. 2015, 88, 180–189. [Google Scholar] [CrossRef]
- Zou, Y.; Maiti, S.; Steurer, W.; Spolenak, R. Sizedependent ductility in an Nb25Mo25Ta25W25 refractory high-entropy alloy. Acta Mater. 2014, 65, 85–97. [Google Scholar] [CrossRef]
- Lejček, P.; Hofmann, S. Entropy-Driven Grain Boundary Segregation: Prediction of the Phenomenon. Metals 2021, 11, 1331. [Google Scholar] [CrossRef]
- Mishin, Y.; Asta, M.; Li, J. Atomistic modeling of interfaces and their impact on microstructure and properties. Acta Mater. 2010, 58, 1117–1151. [Google Scholar] [CrossRef]
- Razumovskii, I.M.; Razumovskiy, V.I.; Logachev, I.A.; Rodin, A.O.; Razumovsky, M.I. Segregation of Refractory Metals at Grain Boundaries in High-Temperature Alloys. Russ. Metall. 2020, 11, 1292–1299. [Google Scholar] [CrossRef]
- Ruban, A.V. On segregation in multicomponent alloys: Surface segregation in austenite and FeCrCoNiMn alloys. Comput. Mater. Sci. 2021, 187, 110080. [Google Scholar] [CrossRef]
- Butrim, V.N. Technological aspects of manufacturing of high-quality chromium alloy for production of critical space craft thruster components. Light Alloy Technol. 2015, 2, 95–104. [Google Scholar]



| T, K | 1523 | 1573 | 1623 | 1670 |
|---|---|---|---|---|
| Ni, D, m2/s | 3.0 × 10−14 | 6.2 × 10−14 | 1.2 × 10−13 | 2.2 × 10−13 |
| Pt, D, m2/s | 5.9 × 10−15 | 1.2 × 10−14 | 2.3 × 10−14 | 4.2 × 10−14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Razumovskii, I.; Bokstein, B.; Razumovsky, M. Approaches to the Development of Advanced Alloys Based on Refractory Metals. Encyclopedia 2023, 3, 311-326. https://doi.org/10.3390/encyclopedia3010019
Razumovskii I, Bokstein B, Razumovsky M. Approaches to the Development of Advanced Alloys Based on Refractory Metals. Encyclopedia. 2023; 3(1):311-326. https://doi.org/10.3390/encyclopedia3010019
Chicago/Turabian StyleRazumovskii, Igor, Boris Bokstein, and Mikhail Razumovsky. 2023. "Approaches to the Development of Advanced Alloys Based on Refractory Metals" Encyclopedia 3, no. 1: 311-326. https://doi.org/10.3390/encyclopedia3010019
APA StyleRazumovskii, I., Bokstein, B., & Razumovsky, M. (2023). Approaches to the Development of Advanced Alloys Based on Refractory Metals. Encyclopedia, 3(1), 311-326. https://doi.org/10.3390/encyclopedia3010019








