The Capital Asset Pricing Model
Definition
1. Introduction
2. Deriving and Defining the Capital Asset Pricing Model
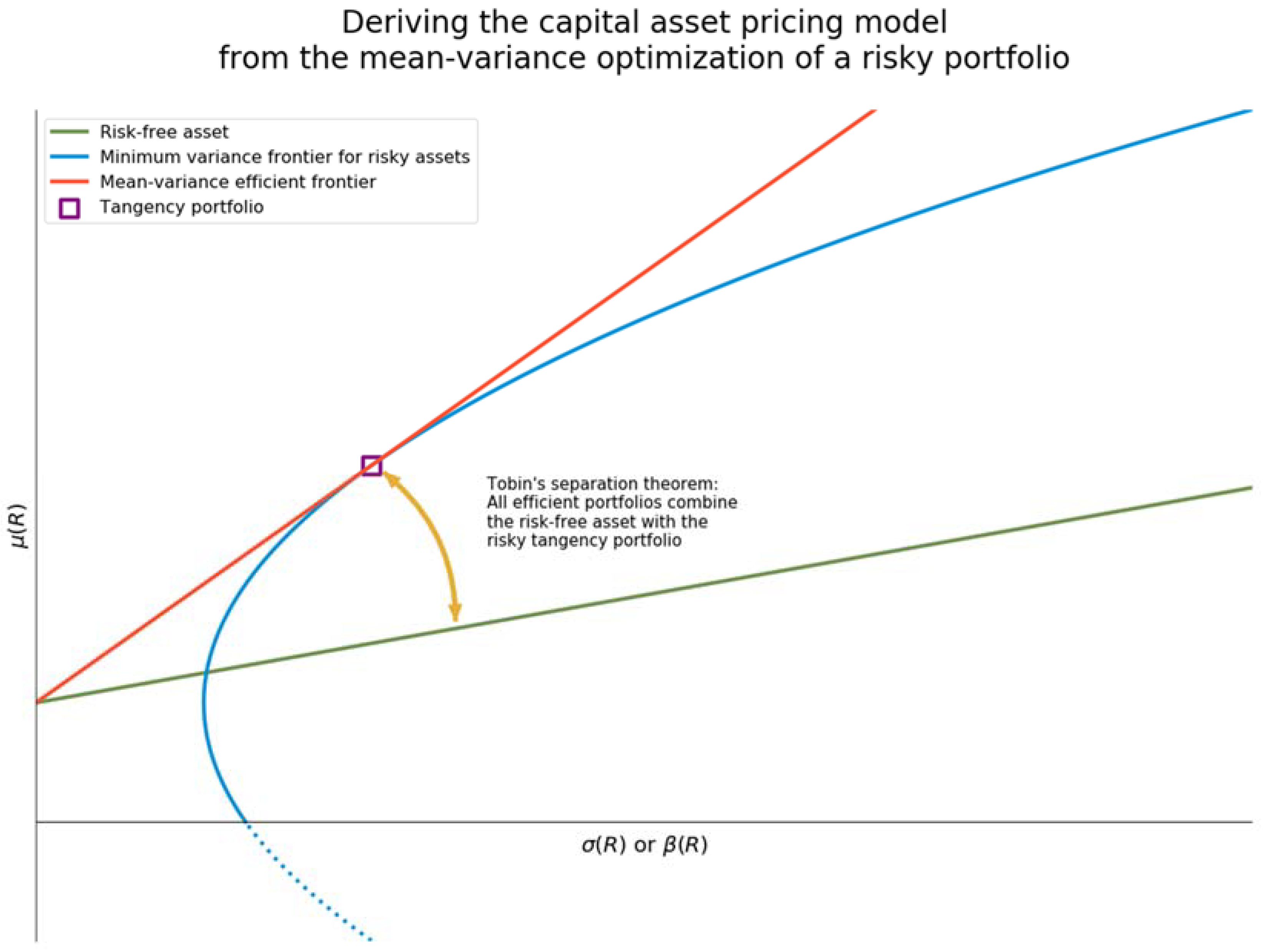
2.1. Mean-Variance Optimization and the Tangency Portfolio
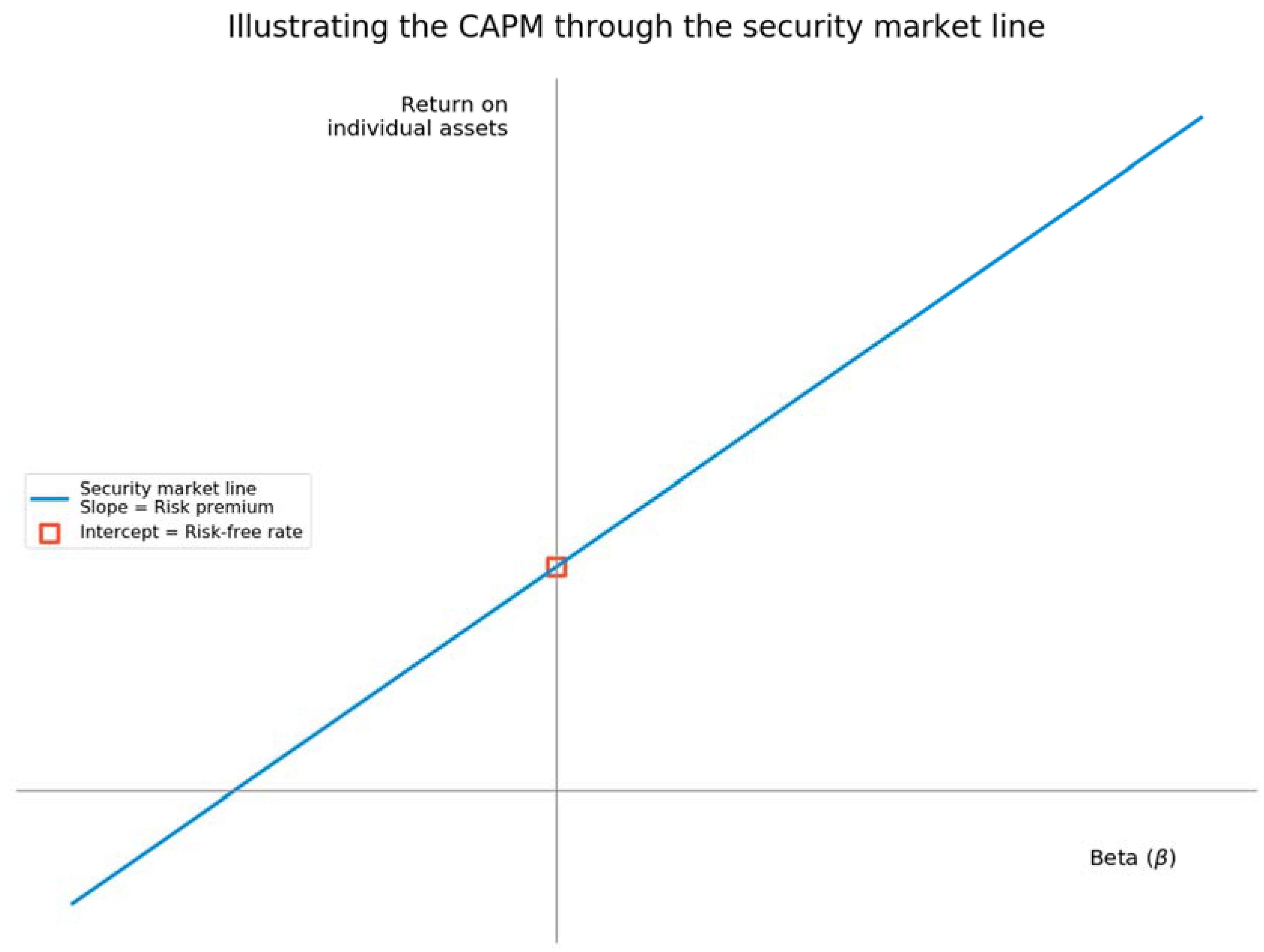
2.2. Defining the Risk Premium and the Risk-Free Rate through the Securty Market Line
2.3. The Treynor and Sharpe Ratios
3. Beta
3.1. Correlated Relative Volatility
3.2. Interpreting Beta: Systematic Versus Idiosyncratic Risk
4. Information Uncertainty and Higher-Moment CAPM
4.1. Risk Versus Uncertainty
4.2. Information Uncertainty
4.3. Generalizing the CAPM to Accommodate Both Risk and Uncertainty
4.4. Higher-Moment CAPM
5. Applications of the CAPM
5.1. The Regulatory CAPM
5.2. Measuring Portfolio and Managerial Performance
5.2.1. The Sharpe Ratio
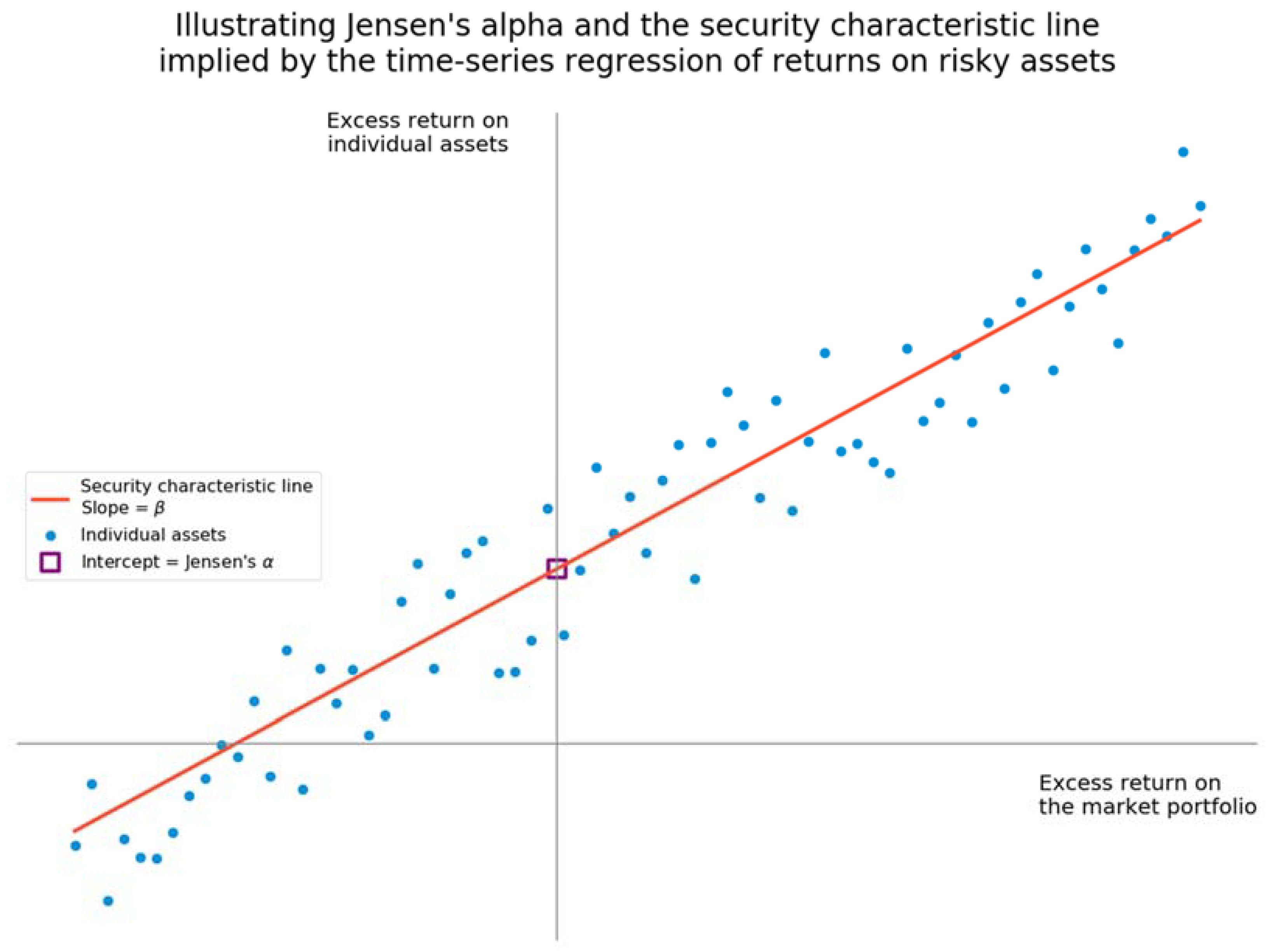
5.2.2. Jensen’s Alpha
5.3. Asset Allocation and Financial Planning
5.3.1. Bond Allocations
5.3.2. Defined Benefit and Defined Contribution Pension Plans
6. Criticisms of the CAPM
6.1. Value, Size, and Momentum: The “Factor Zoo”
6.2. Relaxing Temporal and Spatial Constraints
6.2.1. The Intertemporal CAPM
6.2.2. Roll’s Second Critique
6.3. Consumption-Based CAPM
6.4. Modeling Heterogeneous Agents
6.4.1. From Heterogeneity to Heterodoxy
6.4.2. Behavioral Capital Asset Pricing
7. Multifractality and the Fractal Market Hypothesis
7.1. Beyond Homogeneous Agents and Efficient Markets
7.2. Volatility, Fractality, and the Generalized Hurst Exponent
7.3. Applications and Implications of Multifractal Analysis
8. Conclusions: The CAPM, Dead or Alive
- The evidence against the CAPM remains at best economically ambiguous.
- Alternative models enjoy no greater empirical support.
- Simplicity and interpretive clarity preserve the CAPM’s seductive appeal.
Funding
Acknowledgments
Conflicts of Interest
Entry Link on the Encyclopedia Platform
References
- Fama, E.F. Risk, return, and equilibrium: Some clarifying comments. J. Financ. 1968, 23, 29–40. [Google Scholar] [CrossRef]
- Muth, J.A. Rational expectations and the theory of price movements. Econometrica 1961, 29, 315–335. [Google Scholar] [CrossRef]
- Epstein, L.G.; Wang, T. Intertemporal asset pricing under Knightian uncertainty. Econometrica 1994, 62, 283–322. [Google Scholar] [CrossRef]
- Ross, S.A. The arbitrage theory of capital asset pricing. J. Econ. Theory 1976, 13, 341–360. [Google Scholar] [CrossRef]
- Anderson, E.W.; Ghysels, E.; Juergens, J.L. The impact of risk and uncertainty on expected returns. J. Financ. Econ. 2009, 94, 233–263. [Google Scholar] [CrossRef]
- Supreme Court of the United States. Willcox v. Consolidated Gas Company; U.S. Reports; Supreme Court of the United States: Washington, DC, USA, 1909; Volume 212, pp. 19–55.
- Fama, E.F.; French, K.R. The capital asset pricing model: Theory and evidence. J. Econ. Perspect. 2004, 18, 25–46. [Google Scholar] [CrossRef]
- Markowitz, H. Portfolio selection. J. Financ. 1952, 7, 77–99. [Google Scholar] [CrossRef]
- Markowitz, H.M. Portfolio Selection: Efficient Diversification of Investments; John Wiley & Sons: New York, NY, USA, 1959. [Google Scholar]
- Sharpe, W.F. Capital asset prices: A theory of market equilibrium under conditions of risk. J. Financ. 1964, 19, 425–442. [Google Scholar] [CrossRef]
- Lintner, J. The valuation of risk assets and the selection of risky investments in stock portfolios and capital budgets. Rev. Econ. Stat. 1965, 47, 13–37. [Google Scholar] [CrossRef]
- Treynor, J.L. How to rate management of investment funds. Harv. Bus. Rev. 1965, 43, 63–75. [Google Scholar]
- French, C.W. The Treynor capital asset pricing model. J. Invest. Manag. 2003, 1, 60–72. [Google Scholar]
- Tobin, J. Liquidity preference as behavior towards risk. Rev. Econ. Stud. 1958, 25, 65–86. [Google Scholar] [CrossRef]
- Black, F. Capital market equilibrium with restricted borrowing. J. Bus. 1972, 45, 444–454. [Google Scholar] [CrossRef]
- Korajczyk, R.A. Introduction. In Asset Pricing and Portfolio Performance: Models, Strategy and Performance Metrics; Korajczyk, R.A., Ed.; Risk Books: London, UK, 1999; pp. xiii–xxxi. [Google Scholar]
- Dybvig, P.H.; Ross, S.A. Differential information and performance measurement using a security market line. J. Financ. 1985, 40, 383–399. [Google Scholar] [CrossRef]
- Reilly, F.K.; Brown, K.C. Analysis of Investments and Management of Portfolios, 9th ed.; Cengage Learning: Farmington Hills, MI, USA, 2009. [Google Scholar]
- Sharpe, W.F. Mutual fund performance. J. Bus. 1966, 39, 119–138. [Google Scholar] [CrossRef]
- Sharpe, W.F. Adjusting for risk in portfolio performance measurement. J. Portf. Manag. 1975, 1, 29–34. [Google Scholar] [CrossRef]
- Sharpe, W.F. The Sharpe ratio. J. Portf. Manag. 1994, 21, 49–58. [Google Scholar] [CrossRef]
- Modigliani, F.; Miller, M.H. The cost of capital, corporate finance, and the theory of investment. Am. Econ. Rev. 1958, 48, 261–297. [Google Scholar]
- Sharpe, W.F. A simplified model for portfolio analysis. Manag. Sci. 1963, 9, 277–293. [Google Scholar] [CrossRef]
- Barberis, N.; Shleifer, A.; Wurgler, J. Comovement. J. Financ. Econ. 2005, 75, 283–317. [Google Scholar] [CrossRef]
- Friend, I.; Blume, M. Measure of portfolio performance under uncertainty. Am. Econ. Rev. 1970, 60, 561–575. [Google Scholar] [CrossRef]
- Leibowitz, M.L.; Bova, A.; Hammond, P.B. The Endowment Model of Investing: Return, Risk, and Diversification; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Miller, M.B. Mathematics and Statistics for Financial Risk Management, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Tofallis, C. Investment volatility: A critique of standard beta estimation and a simple way forward. Eur. J. Oper. Res. 2008, 187, 1358–1367. [Google Scholar] [CrossRef]
- Hui, C.-H.; Lo, C.-F.; Chau, P.-H.; Wong, A. Does Bitcoin behave as a currency? A standard monetary model approach. Int. Rev. Financ. Anal. 2020, 70, 101518. [Google Scholar] [CrossRef]
- Farber, D.A. Uncertainty. Georget. Law J. 2011, 99, 901–960. [Google Scholar] [CrossRef]
- Knight, F.H. Risk, Uncertainty, and Profit; Houghton Mifflin Co.: Boston, MA, USA; New York, NY, USA, 1921. [Google Scholar]
- Keynes, J.M. The general theory of employment. Q. J. Econ. 1937, 51, 209–223. [Google Scholar] [CrossRef]
- Zhang, X.F. Information uncertainty and stock returns. J. Financ. 2006, 61, 105–137. [Google Scholar] [CrossRef]
- Bloom, N. Fluctuations in uncertainty. J. Econ. Perspect. 2014, 28, 153–176. [Google Scholar] [CrossRef]
- Epstein, L.G.; Schneider, M. Ambiguity, information quality, and asset pricing. J. Financ. 2008, 43, 197–228. [Google Scholar] [CrossRef]
- Christiano, L.J.; Motto, R.; Rostagno, M. Risk shocks. Am. Econ. Rev. 2014, 104, 27–65. [Google Scholar] [CrossRef]
- Fama, E.F. Mandelbrot and the stable Paretian hypothesis. J. Bus. 1963, 36, 420–429. Available online: https://www.jstor.org/stable/2350971 (accessed on 29 August 2021). [CrossRef]
- Fama, E.F. Portfolio analysis in a stable Paretian market. Manag. Sci. 1965, 11, 404–416. [Google Scholar] [CrossRef]
- de Athayde, G.M.; Flôres, R.G. Finding a maximum skewness portfolio—A general solution to three-moments portfolio choice. J. Econ. Dyn. Control. 2004, 28, 1335–1352. [Google Scholar] [CrossRef]
- Estrada, J. Mean-semivariance behaviour: An alternative behavioural model. J. Emerg. Mark. Financ. 2004, 3, 231–248. [Google Scholar] [CrossRef]
- Harvey, C.R.; Liechty, J.C.; Liechty, M.W.; Müller, P. Portfolio selection with higher moments. Quant. Financ. 2010, 10, 469–485. [Google Scholar] [CrossRef]
- Jurczenko, E.; Maillet, B. The four-moment capital asset pricing model: Between asset pricing and asset allocation. In Multi-Moment Asset Allocation and Pricing Models; Jurczenko, E., Maillet, B., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 2012; pp. 113–163. [Google Scholar] [CrossRef]
- Jondeau, E.; Rockinger, M. Optimal portfolio allocation under higher moments. Eur. J. Financ. Manag. 2006, 12, 29–55. [Google Scholar] [CrossRef]
- Campbell, J.Y.; Lo, A.W.; MacKinlay, C. The Econometrics of Financial Markets; Princeton University Press: Princeton, NJ, USA, 1997. [Google Scholar]
- Harvey, C.R.; Siddique, A. Conditional skewness in asset pricing tests. J. Financ. 2000, 55, 1263–1295. [Google Scholar] [CrossRef]
- Bali, T.G.; Cakici, N.; Whitelaw, R. Maxing out: Stocks as lotteries and the cross-section of expected returns. J. Financ. Econ. 2011, 99, 427–446. [Google Scholar] [CrossRef]
- Brunnermeier, M.K.; Gollier, C.; Parker, J.A. Optimal beliefs, asset prices, and the preference for skewed returns. Am. Econ. Rev. 2007, 97, 159–165. [Google Scholar] [CrossRef]
- Scott, R.C.; Horvath, P.A. On the direction of preference for moments of higher order than the variance. J. Financ. 1980, 35, 915–919. [Google Scholar] [CrossRef]
- Florida Public Service Commission. Returns on Common Equity for Water and Wastewater Utilities; Public Utilities Reports, 4th Series; Florida Public Service Commission: Tallahassee, FL, USA, 1999; Volume 194, pp. 81–91.
- Supreme Court of the United States. Bluefield Water Works v. Public Service Commission; U.S. Reports; Supreme Court of the United States: Washington, DC, USA, 1923; Volume 262, pp. 679–695.
- Lee, C.-F.; Lee, A.C.; Lee, J. (Eds.) Handbook of Quantitative Finance and Risk Management; Springer: New York, NY, USA, 2010; Volume 1. [Google Scholar]
- Jobson, J.D.; Korkie, B.M. Performance hypothesis testing with the Sharpe and Treynor measures. J. Financ. 1981, 36, 888–908. [Google Scholar] [CrossRef]
- Chen, L.; He, S.; Zhang, S. When all risk-adjusted performance measures are the same: In praise of the Sharpe ratio. Quant. Financ. 2011, 11, 1439–1447. [Google Scholar] [CrossRef]
- Sharpe, W.F. The arithmetic of active management. Financ. Anal. J. 1991, 47, 7–9. [Google Scholar] [CrossRef]
- Jensen, M.C. The performance of mutual funds in the period 1945–1964. J. Financ. 1968, 23, 389–416. [Google Scholar] [CrossRef]
- Canner, N.; Mankiw, N.G.; Weil, D.G. An asset allocation puzzle. Am. Econ. Rev. 1997, 87, 181–191. [Google Scholar]
- Kroll, Y.; Levy, H. Further tests of the separation theorem and the capital asset pricing model. Am. Econ. Rev. 1992, 82, 664–670. Available online: https://www.jstor.org/stable/2117330 (accessed on 29 August 2021).
- Zhang, L. The value premium. J. Financ. 2005, 60, 67–103. [Google Scholar] [CrossRef]
- Bernstein, W.J. The Intelligent Asset Investor: How to Build Your Portfolio to Maximize Returns and Minimize Risk; McGraw-Hill Education: New York, NY, USA, 2000. [Google Scholar]
- Graham, B.; Dodd, D.L. Security Analysis, 6th ed.; McGraw-Hill Education: New York, NY, USA, 2008. [Google Scholar]
- Hiller, D.; Grinblatt, M.; Titman, S. Financial Markets and Corporate Strategy, 2nd ed.; McGraw-Hill Education: New York, NY, USA, 2011. [Google Scholar]
- Shalit, H.; Yitzhaki, S. An asset allocation puzzle: Comment. Am. Econ. Rev. 2003, 93, 1002–1008. [Google Scholar] [CrossRef]
- Bajeux-Besnainou, I.; Jordan, J.V.; Portait, R. An asset allocation puzzle: Comment. Am. Econ. Rev. 2001, 91, 1170–1179. [Google Scholar] [CrossRef]
- Gomez, J.-P.; Zapatero, F. Asset pricing implications of benchmarking: A two-factor CAPM. Eur. J. Financ. 2003, 9, 343–357. [Google Scholar] [CrossRef]
- Mulvey, J.M.; Fabozzi, F.; Pauling, W.R.; Simsek, K.D.; Zhang, Z. Modernizing the defined-benefit pension system. J. Portf. Manag. 2005, 31, 73–82. [Google Scholar] [CrossRef]
- Cowling, C.; Fisher, H.; Powe, K.; Sheth, J.; Wright, M. Funding Defined Benefit pension schemes: An integrated risk management approach. Br. Actuar. J. 2019, 24, E7. [Google Scholar] [CrossRef]
- Lally, M. The valuation of GSF’s defined benefit pension entitlements. N. Z. Econ. Pap. 2000, 34, 183–199. [Google Scholar] [CrossRef]
- Menoncin, F.; Vigna, E. Mean-variance dynamic optimality for DC pension schemes. Eur. Actuar. J. 2020, 10, 125–148. [Google Scholar] [CrossRef]
- Pedersen, J.L.; Peskir, G. Optimal mean-variance portfolio selection. Math. Financ. Econ. 2017, 11, 137–160. [Google Scholar] [CrossRef]
- Vigna, E.; Haberman, S. Optimal investment strategy for defined contribution pension schemes. Insur. Math. Econ. 2001, 28, 233–262. [Google Scholar] [CrossRef]
- Basak, S.; Chabkauri, G. Dynamic mean-variance asset allocation. Rev. Financ. Stud. 2010, 23, 2970–3016. [Google Scholar] [CrossRef]
- Zhou, X.Y.; Li, D. Continuous-time mean-variance portfolio selection: A stochastic LQ framework. Appl. Math. Optim. 2000, 42, 19–33. [Google Scholar] [CrossRef]
- Lovelock, J. Gaia: A New Look at Life on Earth; Oxford University Press: Oxford, UK, 1979. [Google Scholar]
- Peters, E.E. Chaos and Order in the Capital Markets—A New View of Cycles, Prices, and Market Volatility; John Wiley & Sons: New York, NY, USA, 1991. [Google Scholar]
- Peters, E.E. Fractal Markte Analysis—Applying Chaos Theory to Investment and Analysis; John Wiley & Sons: New York, NY, USA, 1994. [Google Scholar]
- Fama, E.F.; MacBeth, J.D. Risk, return, and equilibrium: Empirical tests. J. Political Econ. 1973, 81, 607–636. [Google Scholar] [CrossRef]
- Basu, S. Investment performance of common stocks in relation to their price-earnings ratios: A test of the efficient market hypothesis. J. Financ. 1977, 12, 129–156. [Google Scholar] [CrossRef]
- Bhandari, L.C. Debt/equity ratio and expected common stock returns: Empirical evidence. J. Financ. 1988, 43, 507–528. [Google Scholar] [CrossRef]
- Rosenberg, B.; Reid, K.; Lanstein, R. Persuasive evidence of market inefficiency. J. Portf. Manag. 1985, 11, 9–17. [Google Scholar] [CrossRef]
- Ball, R. Anomalies in relationships between securities’ yields and yield surrogates. J. Financ. Econ. 1978, 6, 103–126. [Google Scholar] [CrossRef]
- Banz, R.W. The relationship between return and market value of common stocks. J. Financ. Econ. 1981, 9, 3–18. [Google Scholar] [CrossRef]
- Reinganum, M.R. Misspecification of capital asset pricing: Empirical anomalies based on earnings’ yield and market values. J. Financ. Econ. 1981, 9, 19–46. [Google Scholar] [CrossRef]
- Levy, H.; Levy, M. The small firm effect: A financial mirage? J. Portf. Manag. 2011, 37, 129–138. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. The cross-section of expected stock returns. J. Financ. 1992, 47, 427–465. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. Common risk factors in the returns on stocks and bonds. J. Financ. Econ. 1993, 33, 3–56. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. Size and book-to-market factors in earnings and returns. J. Financ. 1995, 50, 131–155. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. Multifactor explanations of asset pricing anomalies. J. Financ. 1996, 51, 55–84. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. Value versus growth: The international evidence. J. Financ. 1998, 53, 1975–1999. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. Size, value, and momentum in international stock returns. J. Financ. Econ. 2012, 105, 457–472. [Google Scholar] [CrossRef]
- Jegadeesh, N.; Titman, S. Returns to buying winners and selling losers: Implications for stock market efficiency. J. Financ. 1993, 48, 65–91. [Google Scholar] [CrossRef]
- Grinblatt, M.; Titman, S.; Wermers, R. Momentum investment strategies, portfolio performance, and herding: A study of mutual fund behavior. Am. Econ. Rev. 1995, 85, 1088–1105. [Google Scholar]
- Chan, L.K.C.; Jegadeesh, N.; Lakonishok, J. Momentum strategies. J. Financ. 1996, 51, 1681–1713. [Google Scholar] [CrossRef]
- Carhart, M.M. On persistence in mutual fund performance. J. Financ. 1997, 52, 57–82. [Google Scholar] [CrossRef]
- Rath, S.; Durand, R.B. Decomposing the size, value and momentum premia of the Fama–French–Carhart four-factor model. Econ. Lett. 2015, 132, 139–141. [Google Scholar] [CrossRef]
- Fama, E.F.; French, K.R. Dissecting anomalies. J. Financ. 2008, 63, 1653–1678. [Google Scholar] [CrossRef]
- Avramov, D.; Chordia, T. Predicting stock returns. J. Financ. Econ. 2006, 82, 387–415. [Google Scholar] [CrossRef]
- Cochrane, J.H. Discount rates. J. Financ. 2011, 66, 1047–1108. [Google Scholar] [CrossRef]
- Feng, G.; Giglio, S.; Xu, D. Taming the factor zoo: A test of new factors. J. Financ. 2020, 75, 1327–1370. [Google Scholar] [CrossRef]
- Merton, R.C. On estimating the expected return on the market: An exploratory investigation. J. Financ. Econ. 1980, 8, 323–361. [Google Scholar] [CrossRef]
- Merton, R.C. An intertemporal capital asset pricing model. Econometrica 1973, 41, 867–887. [Google Scholar] [CrossRef]
- Chang, B.Y.; Christofferson, P.; Jacobs, K. Market skewness risk and the cross section of stock returns. J. Financ. Econ. 2013, 107, 46–68. [Google Scholar] [CrossRef]
- Kim, H.K.; Kim, T. Capital asset pricing model: A time-varying volatility approach. J. Empir. Financ. 2016, 37, 268–281. [Google Scholar] [CrossRef]
- Koutmos, D. An intertemporal capital asset pricing model with heterogeneous expectations. J. Int. Financ. Mark. Inst. Money 2012, 22, 1176–1187. [Google Scholar] [CrossRef]
- Roll, R. A critique of the asset pricing theory’s tests, part I: On past and potential testability of the theory. J. Financ. Econ. 1977, 4, 129–176. [Google Scholar] [CrossRef]
- Gibbons, M.R.; Ross, S.A.; Shanken, J. A test of the efficiency of a given portfolio. Econometrica 1989, 57, 1121–1152. [Google Scholar] [CrossRef]
- Stambaugh, R.F. On the exclusion of assets from tests of the two-parameter model: A sensitivity analysis. J. Financ. Econ. 1982, 10, 237–268. [Google Scholar] [CrossRef]
- Browning, M.; Deaton, A.; Irish, M. A profitable approach to labor supply and commodity demands over the life-cycle. Econometrica 1985, 53, 503–543. [Google Scholar] [CrossRef]
- Roy, A.D. Safety first and the holding of assets. Econometrica 1952, 20, 431–449. [Google Scholar] [CrossRef]
- Breeden, D.T. An intertemporal asset pricing pricing model with stochastic consumption and investment opportunities. J. Financ. Econ. 1979, 7, 285–296. [Google Scholar] [CrossRef]
- Grossman, S.J.; Shiller, R.J. The determinants of the variability of stock market prices. Am. Econ. Rev. 1981, 71, 222–227. [Google Scholar]
- Lucas, R.E., Jr. Asset prices in an exchange economy. Econometrica 1978, 46, 1429–1446. [Google Scholar] [CrossRef]
- Rubinstein, M. The valuation of uncertain income streams and the pricing of options. Bell J. Econ. 1976, 7, 407–425. [Google Scholar] [CrossRef]
- Cochrane, J.H. Asset Pricing; Princeton University Press: Princeton, NJ, USA, 1981. [Google Scholar]
- Paiella, M. Heterogeneity in financial market participation: Appraising its implications for the C-CAPM. Rev. Financ. 2004, 8, 445–480. [Google Scholar] [CrossRef]
- Campbell, J.Y.; Cochrane, J.H. Explaining the poor performance of consumption-based asset pricing models. J. Financ. 2000, 55, 2863–2878. [Google Scholar] [CrossRef]
- Breeden, D.T.; Gibbons, M.R.; Litzenberger, R.H. Empirical tests of the consumption-oriented CAPM. J. Financ. 1989, 44, 231–262. [Google Scholar] [CrossRef]
- Hansen, L.P.; Singleton, K.J. Generalized instrumental variables estimation of nonlinear rational expectations models. Econometrica 1982, 50, 1269–1288. [Google Scholar] [CrossRef]
- Hansen, L.P.; Singleton, K.J. Stochastic consumption, risk aversion, and the temporal behavior of asset returns. J. Political Econ. 1983, 91, 249–268. [Google Scholar] [CrossRef]
- Wheatley, S. Some tests of international equity integration. J. Financ. Econ. 1988, 21, 177–212. [Google Scholar] [CrossRef]
- Darrat, A.F.; Li, B.; Park, J.C. Consumption-based CAPM models: International evidence. J. Bank. Financ. 2011, 35, 2148–2157. [Google Scholar] [CrossRef]
- Brock, W.A.; Hommes, C.H. Heterogeneous beliefs and routes to chaos in a simple asset pricing model. J. Econ. Dyn. Control 1998, 22, 1235–1274. [Google Scholar] [CrossRef]
- Glosten, L.R.; Milgrom, P.R. Bid, ask, and transaction prices in a specialist market with heterogeneously informed traders. J. Financ. Econ. 1985, 14, 71–100. [Google Scholar] [CrossRef]
- Bhattacharya, U.; Daouk, H. The world price of insider trading. J. Financ. 2002, 57, 75–108. [Google Scholar] [CrossRef]
- Cornell, B.; Sirri, E.R. The reaction of investors and stock prices to insider trading. J. Financ. 1992, 47, 1031–1059. [Google Scholar] [CrossRef]
- Williams, J.T. Capital asset prices with heterogeneous beliefs. J. Financ. Econ. 1977, 5, 219–239. [Google Scholar] [CrossRef]
- Detemple, J.; Murthy, S. Intertemporal asset pricing with heterogeneous beliefs. J. Econ. Theory 1994, 62, 294–320. [Google Scholar] [CrossRef]
- Johnson, T. Forecast dispersion and the cross section of expected returns. J. Financ. 2004, 59, 1957–1978. [Google Scholar] [CrossRef]
- Lintner, J. The aggregation of investor’s diverse judgments and preferences in purely competitive security markets. J. Financ. Quant. Anal. 1969, 4, 347–400. [Google Scholar] [CrossRef]
- Varian, H. Divergence of opinion in complete markets: A note. J. Financ. 1985, 40, 309–317. [Google Scholar] [CrossRef]
- Barberis, N.; Huang, M.; Thaler, R.H. Individual preferences, monetary gambles, and stock market participation: A case for narrow framing. Am. Econ. Rev. 2006, 96, 1069–1090. [Google Scholar] [CrossRef]
- Polkovnichenko, V. Limited stock market participation and the equity premium. Financ. Res. Lett. 2004, 1, 24–34. [Google Scholar] [CrossRef]
- Tversky, A.; Kahneman, D. Advances in prospect theory: Cumulative representation of uncertainty. J. Risk Uncertain. 1992, 5, 297–323. [Google Scholar] [CrossRef]
- Del Vigna, M. Financial market equilibria with heterogeneous agents: CAM and market segmentation. Math. Financ. Econ. 2013, 7, 405–429. [Google Scholar] [CrossRef]
- Chiarella, C.; Dieci, R.; He, X.-Z.; Li, K. An evolutionary CAPM under heterogeneous beliefs. Ann. Financ. 2013, 9, 185–215. [Google Scholar] [CrossRef]
- Black, F. Noise. J. Financ. 1986, 41, 529–543. [Google Scholar] [CrossRef]
- Shefrin, H.; Statman, M. Behavioral capital asset pricing theory. J. Financ. Quant. Anal. 1994, 29, 323–349. [Google Scholar] [CrossRef]
- He, X.-Z.; Li, Y. Heterogeneity, convergence, and autocorrelations. Quant. Financ. 2008, 8, 58–79. [Google Scholar] [CrossRef]
- Lux, T.; Alfarano, S. Financial power laws: Empirical evidence, models, and mechanisms. Chaos Solitons Fractals 2016, 88, 3–18. [Google Scholar] [CrossRef]
- Westerhoff, F.H. Multiasset market dynamics. Macroecon. Dyn. 2004, 8, 591–616. [Google Scholar] [CrossRef][Green Version]
- Westerhoff, F.H.; Dieci, R. The effectiveness of Keynes-Tobin transaction taxes when heterogeneous agents can trade in different markets: A behavioral finance approach. J. Econ. Dyn. Control 2006, 30, 293–322. [Google Scholar] [CrossRef]
- Brown, S.J. The efficient market hypothesis, the Financial Analysts Journal and the professional status of investment management. Financ. Anal. J. 2020, 76, 5–14. [Google Scholar] [CrossRef]
- Fama, E.F. Efficient capital markets: A review of theory and empirical work. J. Financ. 1970, 33, 3–56. [Google Scholar] [CrossRef]
- Fama, E.F. Efficient capital markets: II. J. Financ. 1991, 46, 1575–1617. [Google Scholar] [CrossRef]
- Vasicek, O.A.; McQuown, J.A. The efficient market model. Financ. Anal. J. 1972, 28, 71–84. [Google Scholar] [CrossRef]
- Faber, M.; Manstetten, R.; Petersen, T. Homo Oeconomicus and Homo Politicus. Political economy, constitutional interest and ecological interest. Kyklos 1997, 50, 457–483. [Google Scholar] [CrossRef]
- McMahon, J. Behavioral economics as neoliberalism: Producing and governing homo economicus. Contemp. Political Theory 2015, 14, 137–158. [Google Scholar] [CrossRef]
- Di Matteo, T.; Aste, T.; Dacorogna, M.M. Scaling behavior in differently developed markets. Phys. A Stat. Mech. Its Appl. 2003, 324, 183–188. [Google Scholar] [CrossRef]
- Bianconi, M.; MacLachlan, S.; Sammon, M. Implied volatility and the risk-free rate of return in options markets. N. Am. J. Econ. Financ. 2015, 31, 1–26. [Google Scholar] [CrossRef]
- Deng, Z.-C.; Yu, J.-N.; Yang, L. An inverse problem of determining the implied volatility in option pricing. J. Math. Anal. Appl. 2008, 340, 16–31. [Google Scholar] [CrossRef]
- Pasquini, M.; Serva, M. Multiscaling and clustering of volatility. Phys. A Stat. Mech. Its Appl. 1999, 269, 140–147. [Google Scholar] [CrossRef]
- Alexander, C.; Lazar, E.; Stanescu, S. Analytic moments for GJR-GARCH (1, 1) processes. Int. J. Forecast. 2021, 37, 105–124. [Google Scholar] [CrossRef]
- Bollerslev, T.; Wooldridge, J.M. Quasi-maximum likelihood estimation and inference in dynamic models with time-varying covariance. Econ. Rev. 1992, 11, 143–172. [Google Scholar] [CrossRef]
- Nugroho, D.B.; Kurniawati, D.; Panjaitan, L.P.; Kholil, Z.; Susanto, B.; Sasongko, L.R. Empirical performance of GARCH, GARCH-M, GJR-GARCH and log-GARCH models for returns volatility. J. Phys. Conf. Ser. 2019, 1307, 012003. [Google Scholar] [CrossRef]
- Bacry, E.; Delour, J.; Muzy, J.F. Multifractal random walk. Phys. Rev. E 2001, 64, 026103. [Google Scholar] [CrossRef]
- Morales, R.; Di Matteo, T.; Aste, T. Non-stationary multifractality in stock returns. Phys. A Stat. Mech. Its Appl. 2013, 392, 6470–6483. [Google Scholar] [CrossRef]
- Castiglioni, P.; Lazzeroni, D.; Coruzzi, P.; Faini, A. A multifractal-multiscale analysis of cardiovascular signals: A DFA-based characterization of blood pressure and heart-rate complexity by gender. Complexity 2018, 2018, 4801924. [Google Scholar] [CrossRef]
- Gieraltowski, J.; Żebrowski, J.J.; Baranowski, R. Multiscale multifractal analysis of heart risk variability recordings with a large number of occurrences of arrhythmia. Phys. Rev. E 2012, 85, 021915. [Google Scholar] [CrossRef]
- Barunik, J.; Aste, T.; Di Matteo, T.; Liu, R. Understanding the source of multifracticality in financial markets. Phys. A Stat. Mech. Its Appl. 2012, 391, 4234–4251. [Google Scholar] [CrossRef]
- Carbone, A.; Castelli, G.; Stanley, H.E. Time-dependent Hurst exponent in financial time series. Phys. A Stat. Mech. Its Appl. 2004, 344, 267–271. [Google Scholar] [CrossRef]
- Domino, K. The use of the Hurst exponent to investigate the global maximum of the Warsaw stock exchange WIG20 index. Phys. A Stat. Mech. Its Appl. 2012, 391, 156–159. [Google Scholar] [CrossRef]
- Grech, D.; Pamuła, G. The local Hurst exponent of the financial time series in the vicinity of crashes on the Polish stock exchange. Phys. A Stat. Mech. Its Appl. 2008, 387, 4299–4308. [Google Scholar] [CrossRef]
- Morales, R.; Di Matteo, T.; Gramatica, R.; Aste, T. Dynamical generalized Hurst exponent as a tool to monitor unstable periods in financial time series. Phys. A Stat. Mech. Its Appl. 2012, 391, 3180–3189. [Google Scholar] [CrossRef]
- Gneiting, T.; Schlather, M. Stochastic models that separate fractal dimension and the Hurst effect. SIAM Rev. 2004, 46, 269–282. [Google Scholar] [CrossRef]
- Salat, H.; Murcio, R.; Arcaute, E. Multifractal methodology. Phys. A Stat. Mech. Its Appl. 2017, 473, 467–487. [Google Scholar] [CrossRef]
- Kristoufek, L. Measuring correlations between non-stationary series with DCCA coefficient. Phys. A Stat. Mech. Its Appl. 2014, 402, 291–298. [Google Scholar] [CrossRef]
- Kristoufek, L. Detrending moving-average cross-correlation coefficient: Measuring cross-correlations between non-stationary series. Phys. A Stat. Mech. Its Appl. 2014, 406, 169–175. [Google Scholar] [CrossRef]
- Kristoufek, L. Detrended fluctuation analysis as a regression framework: Estimating dependence at different scales. Phys. Rev. E 2015, 91, 022802. [Google Scholar] [CrossRef]
- Kristoufek, L. Scaling of dependence between foreign exchange rates and stock markets in central Europe. Acta Phys. Pol. 2016, 129, 908–912. [Google Scholar] [CrossRef]
- Kristoufek, L. Fractal market hypothesis and the global financial crisis: Scaling, investment horizons and liquidity. Adv. Complex Syst. 2012, 15, 1250065. [Google Scholar] [CrossRef]
- Kristoufek, L. Fractal market hypothesis and the global financial crisis: Wavelet power evidence. Sci. Rep. 2013, 3, 2857. [Google Scholar] [CrossRef]
- Weron, A.; Weron, R. Fractal market hypothesis and two power-laws. Chaos Solitons Fractals 2000, 11, 289–296. [Google Scholar] [CrossRef]
- Jiang, Z.-Q.; Xie, W.-J.; Zhou, W.-X.; Sornette, D. Multifractal analysis of financial markets: A review. Rep. Prog. Phys. 2019, 82, 82–125901. [Google Scholar] [CrossRef]
- Ihlen, E.A.F.; Vereijken, B. Multifractal formalisms of human behavior. Hum. Mov. Sci. 2013, 32, 633–651. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Choices, values, and frames. Am. Psychol. 1984, 39, 344–350. [Google Scholar] [CrossRef]
- Kristoufek, L.; Ferreira, P. Capital asset pricing model in Portugal: Evidence from fractal regressions. Port. Econ. J. 2018, 17, 173–183. [Google Scholar] [CrossRef]
- Tilfani, O.; Ferreira, P.; El Boukfaoui, M.Y. Multiscale optimal portfolios using CAPM fractal regression: Estimation for emerging stock markets. Post-Communist Econ. 2020, 32, 77–112. [Google Scholar] [CrossRef]
- Ma, F.; Wei, Y.; Huang, D. Multifractal detrended cross-correlation analysis between the Chinese stock market and surrounding stock markets. Phys. A Stat. Mech. Its Appl. 2013, 392, 1659–1670. [Google Scholar] [CrossRef]
- Cao, G.; Xu, L.; Cao, J. Multifractal detrended cross-correlations between the Chinese exchange market and stock market. Phys. A Stat. Mech. Its Appl. 2012, 391, 4855–4866. [Google Scholar] [CrossRef]
- Ferreira, P.; Silva, M.F.D.; Santana, I.S.D. Detrended correlation coefficients between exchange rate (in dollars) and stock markets in the world’s largest economies. Economies 2019, 7, 9. [Google Scholar] [CrossRef]
- Sun, L.; Xiang, M.; Marquez, L. Forecasting the volatility of onshore and offshore USD/RMB exchange rates using a multifractal approach. Phys. A Stat. Mech. Its Appl. 2019, 532, 121787. [Google Scholar] [CrossRef]
- Fan, Q.; Liu, S.; Wang, K. Multiscale multifractal detrended fluctuation analysis of multivariate time series. Phys. A Stat. Mech. Its Appl. 2019, 532, 121864. [Google Scholar] [CrossRef]
- Pagnottoni, P.; Spelta, A.; Pecora, N.; Flori, A.; Pammolli, F. Financial earthquakes: SARS-CoV-2 news shock propagation in stock and sovereign bond markets. Phys. A Stat. Mech. Its Appl. 2021, 582, 126240. [Google Scholar] [CrossRef]
- Kristoufek, L. Fractality in market risk structure: Dow Jones Industrial components case. Chaos Solitons Fractals 2018, 110, 69–75. [Google Scholar] [CrossRef]
- Tilfani, O.; Ferreira, P.; El Boukfaoui, M.Y. Building multi-scale portfolios and efficient market frontiers using fractal regressions. Phys. A Stat. Mech. Its Appl. 2019, 532, 121758. [Google Scholar] [CrossRef]
- Fernández-Martínez, M.; Sánchez-Granero, M.A.; Muñoz Torrecillas, M.J.; McKelvey, B. A comparison of three Hurst exponent approaches to predict nascent bubbles in S&P500 stocks. Fractals 2017, 25, 1750006. [Google Scholar] [CrossRef]
- Preis, T.; Schneider, J.J.; Stanley, H.E. Switching processes in financial markets. Proc. Natl. Acad. Sci. USA 2011, 108, 7674–7678. [Google Scholar] [CrossRef]
- Bekaert, G.; Erb, C.B.; Harvey, C.R.; Viskanta, T.E. Distributional characteristics of emerging market returns and asset allocation. J. Portf. Manag. 1998, 24, 102–116. [Google Scholar] [CrossRef]
- Peiró, A. Skewness in financial returns. J. Bank. Financ. 1999, 23, 847–862. [Google Scholar] [CrossRef]
- Aparicio, F.M.; Estrada, J. Empirical distributions of stock returns: European securities markets, 1990–95. Eur. J. Financ. 2001, 7, 1–21. [Google Scholar] [CrossRef]
- Kon, S.J. Models of stock returns—A comparison. J. Financ. 1984, 19, 147–165. [Google Scholar] [CrossRef]
- Gray, J.B.; French, D.W. Empirical comparisons of distributional models for stock index returns. J. Bus. Financ. Account. 1990, 17, 451–459. [Google Scholar] [CrossRef]
- Mandelbrot, B.B.; Hudson, R.L. The (Mis) Behavior of Markets: A Fractal View of Risk, Ruin, and Reward; Basic Books: New York, NY, USA, 2004. [Google Scholar]
- Fama, E.F.; French, K.R. The CAPM is wanted, dead or alive. J. Financ. 1996, 51, 1947–1958. [Google Scholar] [CrossRef]
- Kaplanski, G. Traditional beta, downside risk beta, and market risk premiums. Q. Rev. Econ. Financ. 2004, 44, 636–653. [Google Scholar] [CrossRef]
- Koller, T.; Goedhart, M.; Wessels, D. Valuation: Measuring and Managing the Value of Companies, 7th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Levy, H. The Capital Asset Pricing Model in the 21st Century: Analytical, Empirical, and Behavioral Perspectives; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Jagannathan, R.; Wang, Z. The conditional CAPM and the cross-section of expected returns. J. Financ. 1996, 51, 3–53. [Google Scholar] [CrossRef]
- Chan, L.K.C.; Lakonishok, J. Are reports of beta’s death premature? J. Portf. Manag. 1993, 19, 51–62. [Google Scholar] [CrossRef]
- Lai, T.-Y.; Stohs, M.H. Yes, CAPM is dead. Int. J. Bus. 2015, 20, 144–158. [Google Scholar]
- Levy, H. The CAPM is alive and well: A review and synthesis. Eur. Financ. Manag. 2009, 16, 43–71. [Google Scholar] [CrossRef]
- Lopes, L.L. Between hope and fear: The psychology of risk. Adv. Exp. Soc. Psychol. 1987, 20, 255–295. [Google Scholar] [CrossRef]
- Preis, T.; Stanley, H.E. Switching phenomena in a system with no switches. J. Stat. Phys. 2010, 138, 431–446. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, J.M. The Capital Asset Pricing Model. Encyclopedia 2021, 1, 915-933. https://doi.org/10.3390/encyclopedia1030070
Chen JM. The Capital Asset Pricing Model. Encyclopedia. 2021; 1(3):915-933. https://doi.org/10.3390/encyclopedia1030070
Chicago/Turabian StyleChen, James Ming. 2021. "The Capital Asset Pricing Model" Encyclopedia 1, no. 3: 915-933. https://doi.org/10.3390/encyclopedia1030070
APA StyleChen, J. M. (2021). The Capital Asset Pricing Model. Encyclopedia, 1(3), 915-933. https://doi.org/10.3390/encyclopedia1030070





