Three-Dimensional Transfer Functions of Interference Microscopes
Abstract
:1. Introduction
2. Results
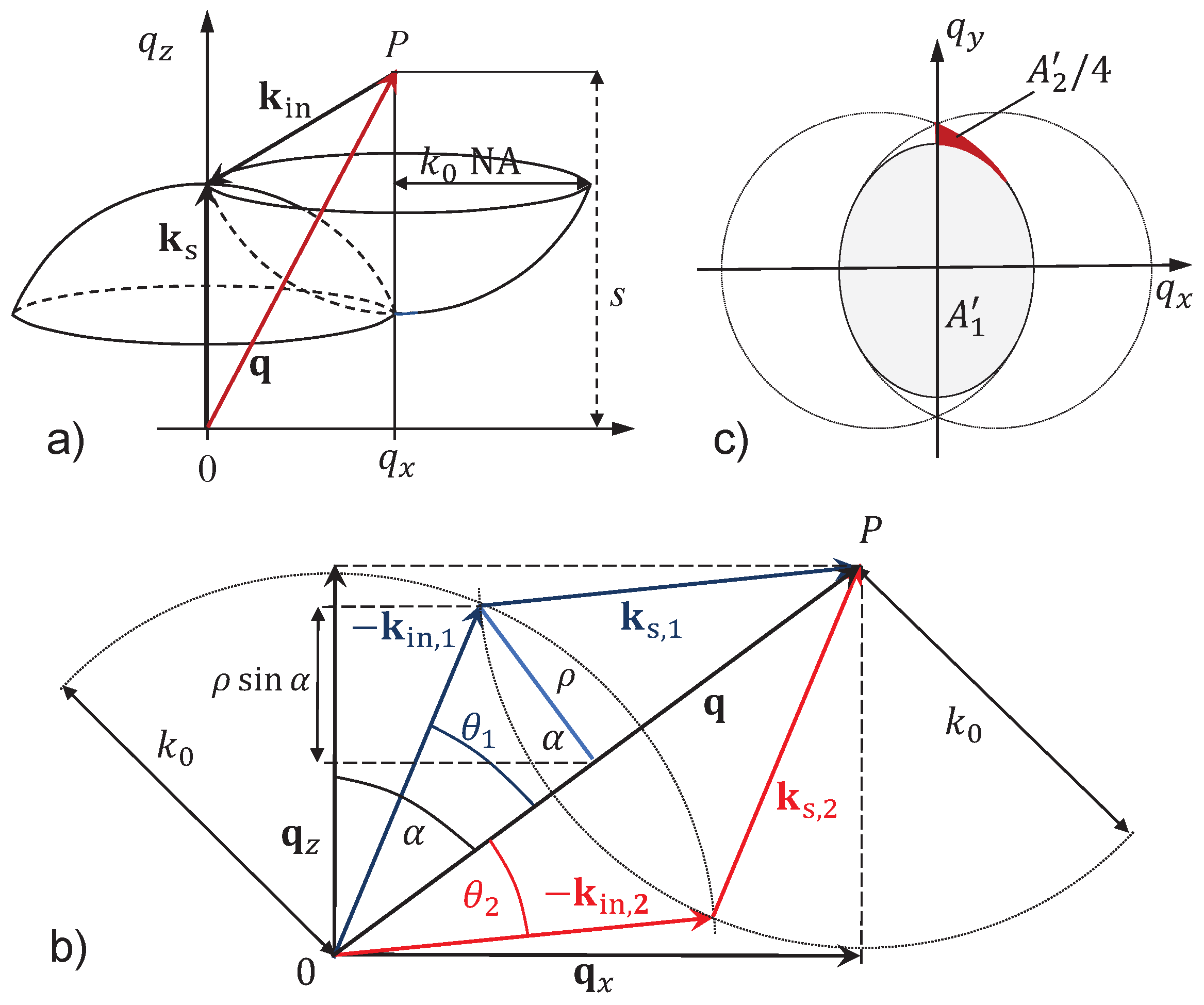
2.1. Derivation of the 3D Transfer Function for Monochromatic Light
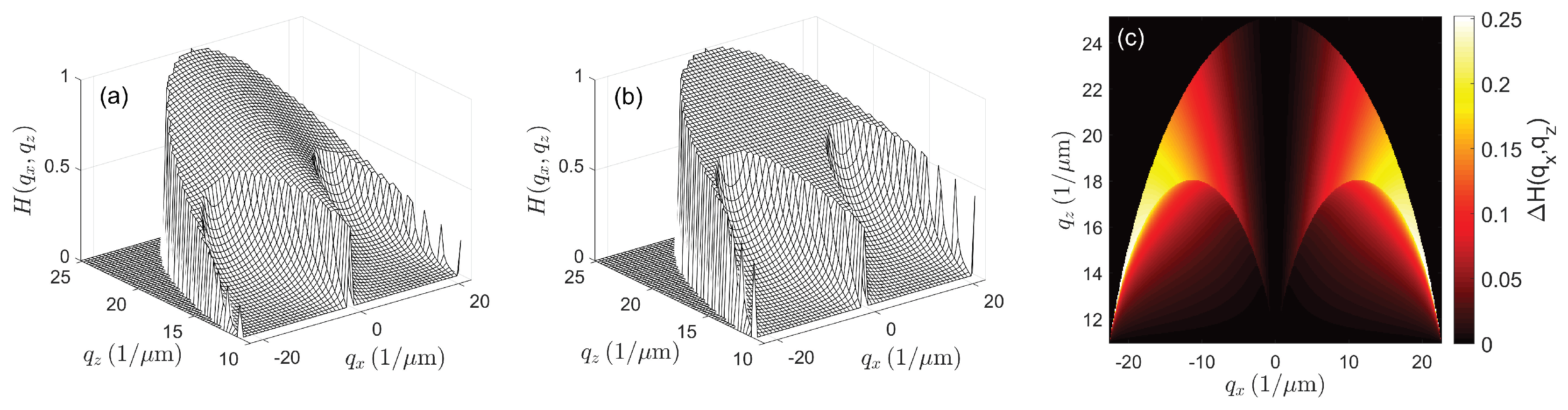
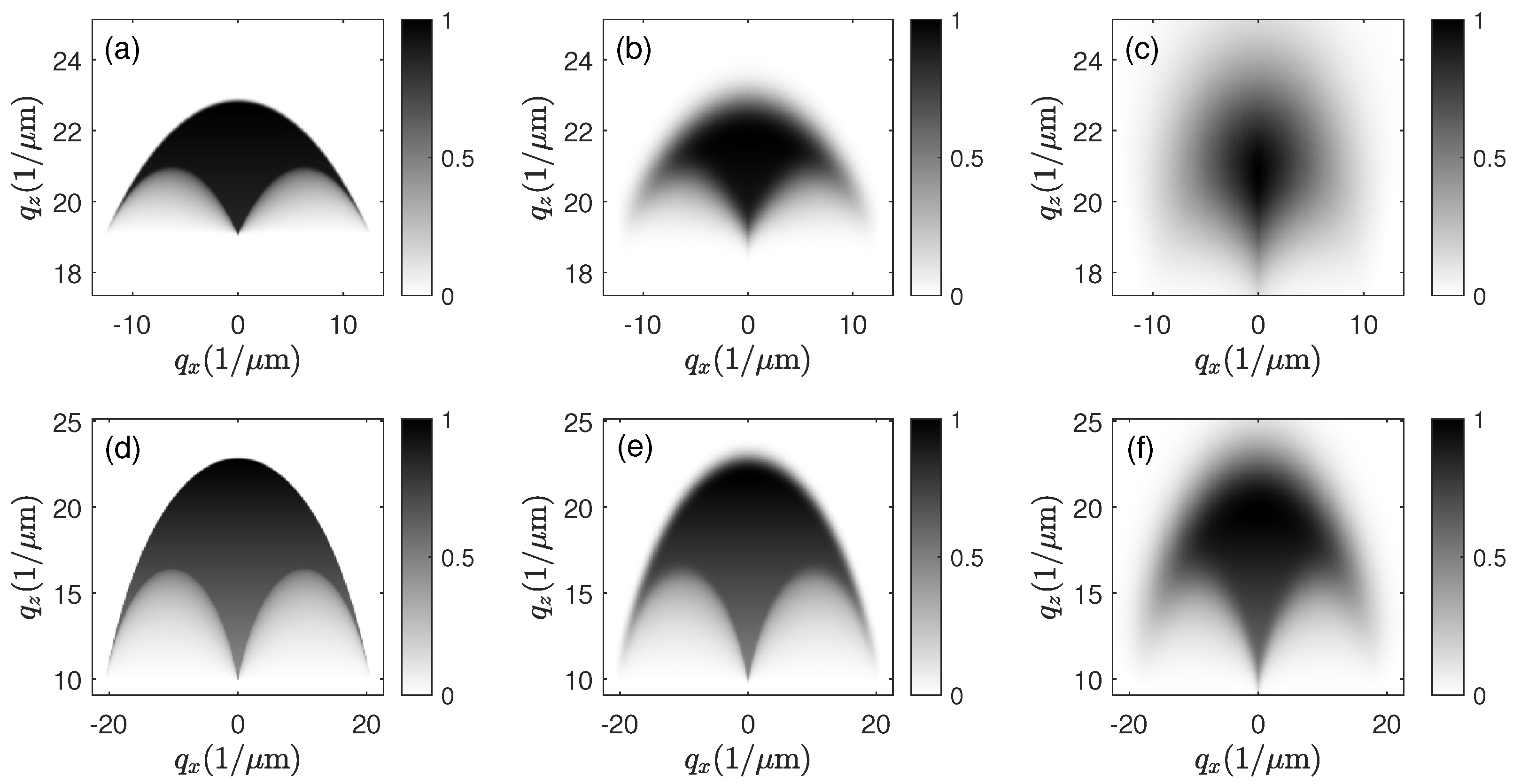
2.2. Dependence of 3D Transfer Functions on Temporal Coherence
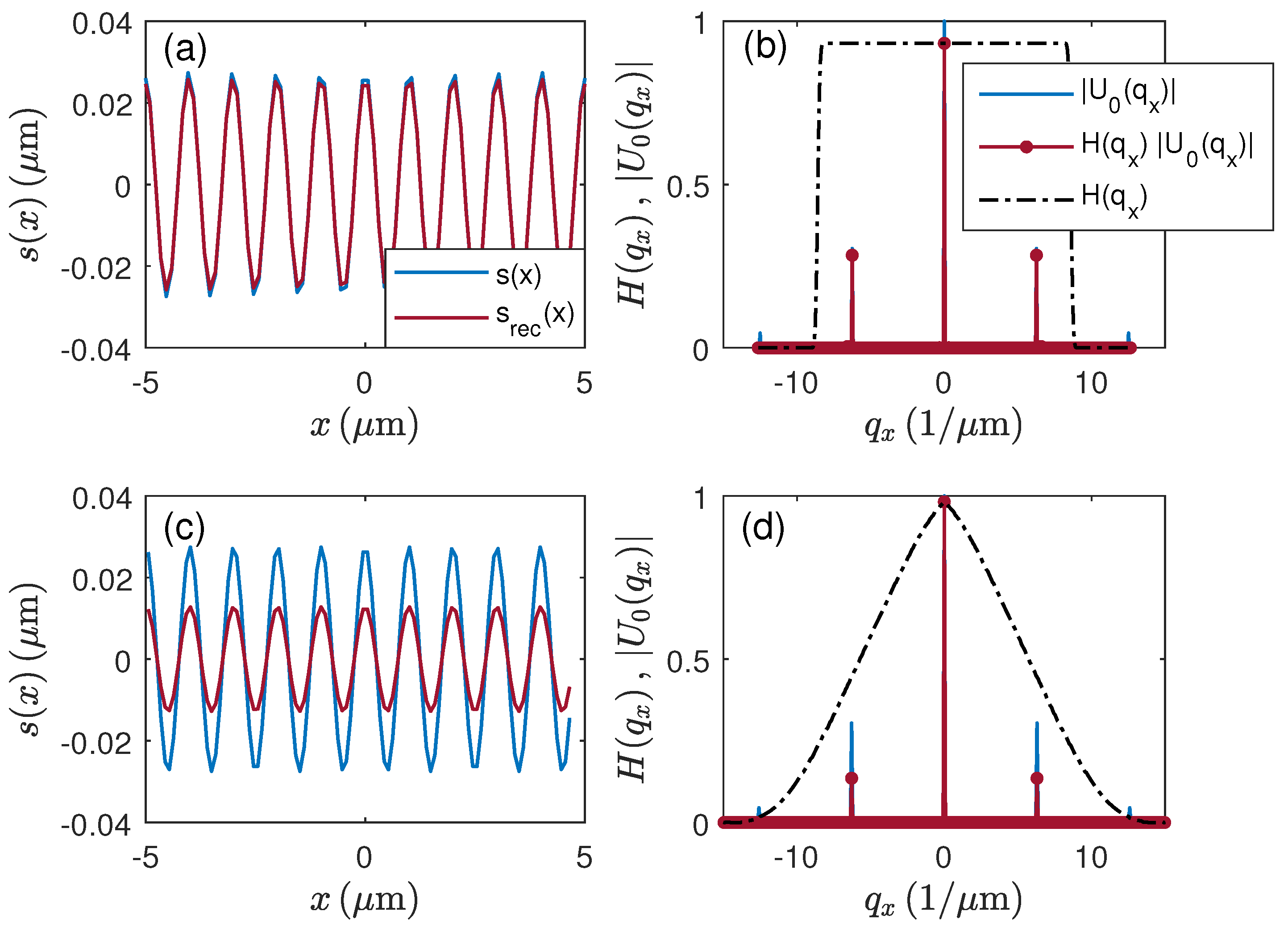
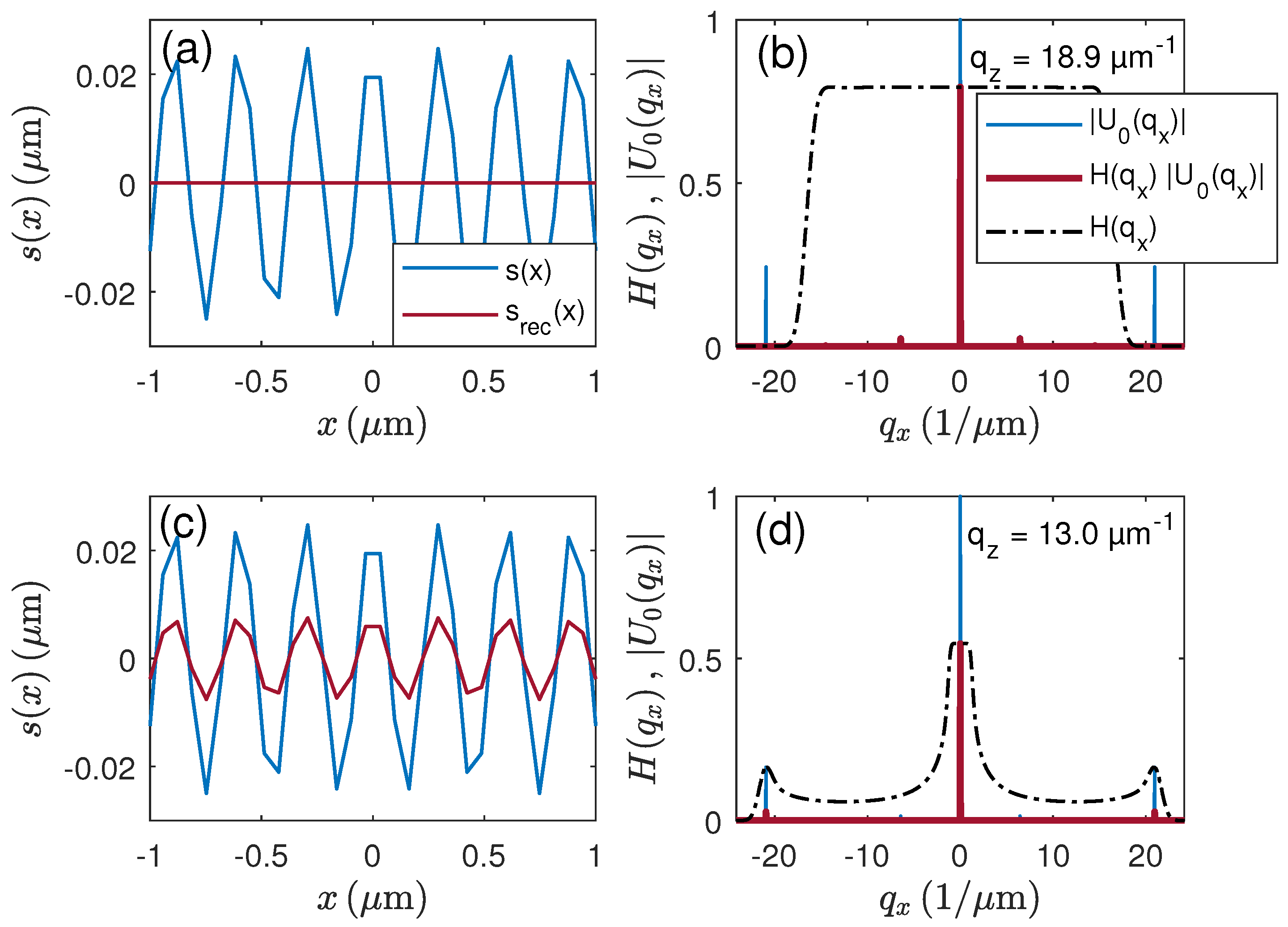
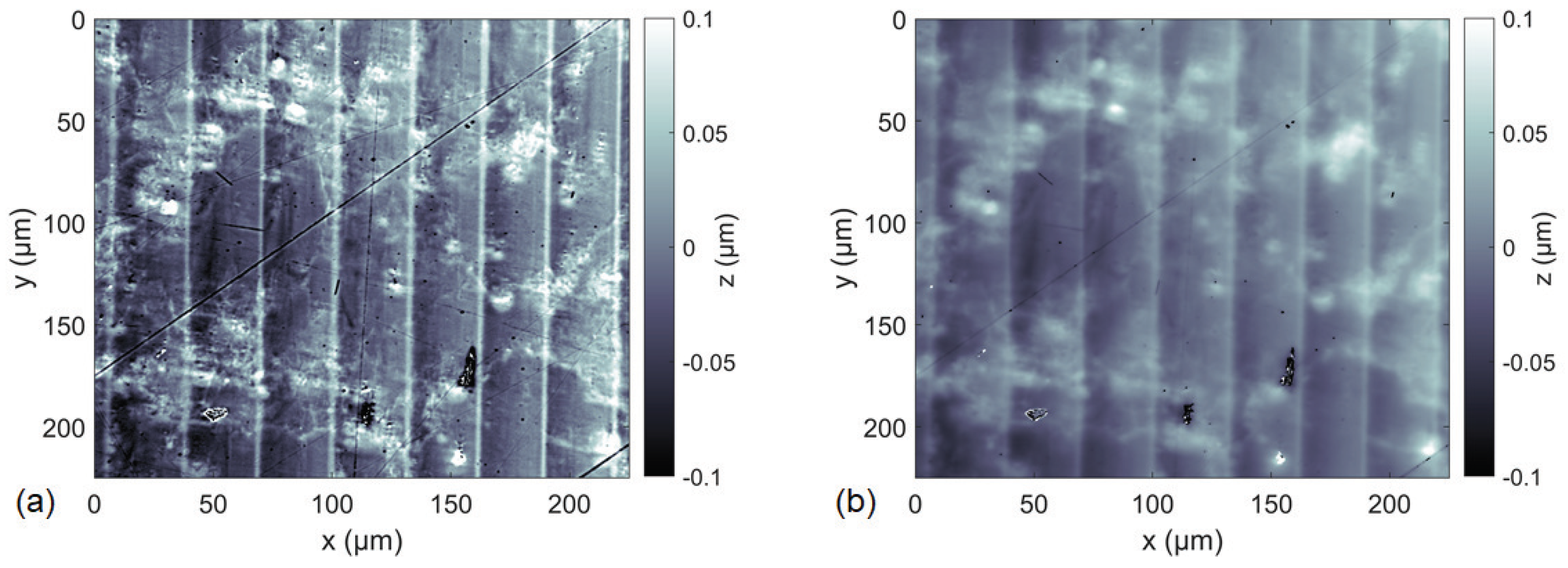
2.3. 3D Transfer Functions and Surface Topography Reconstruction
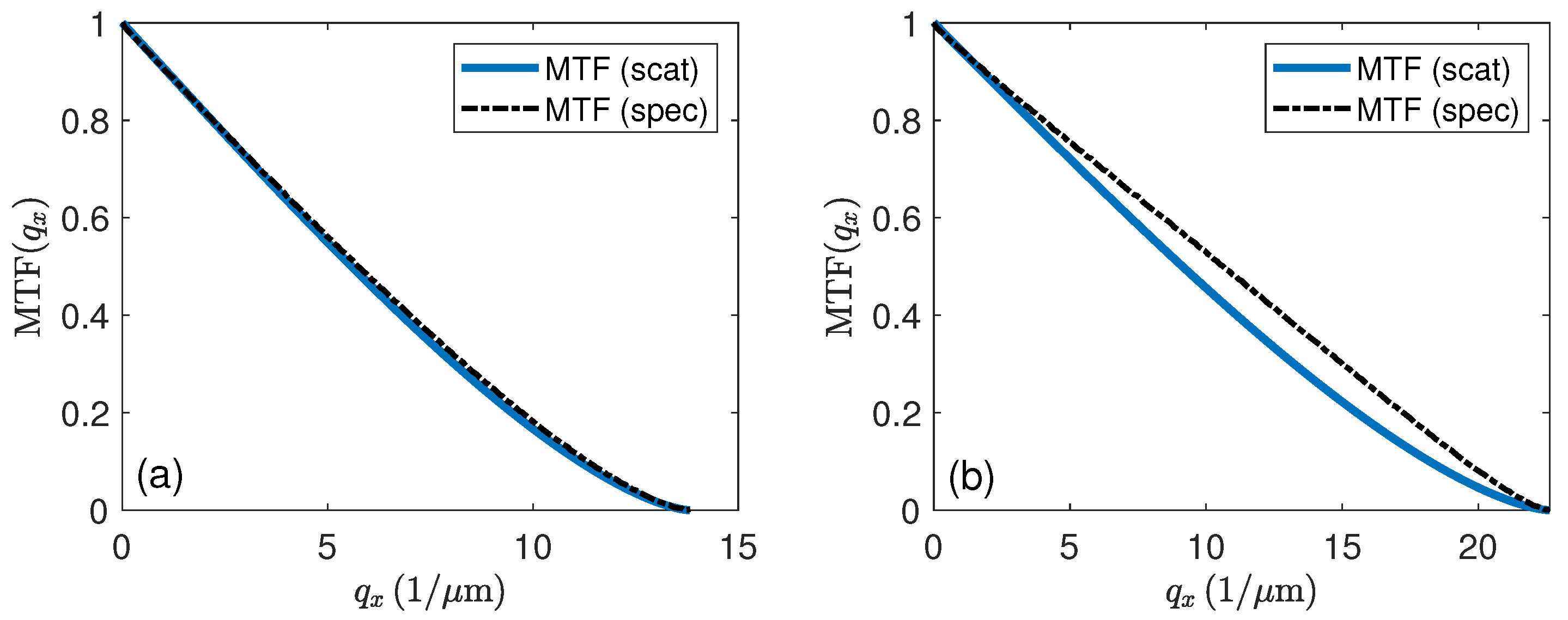
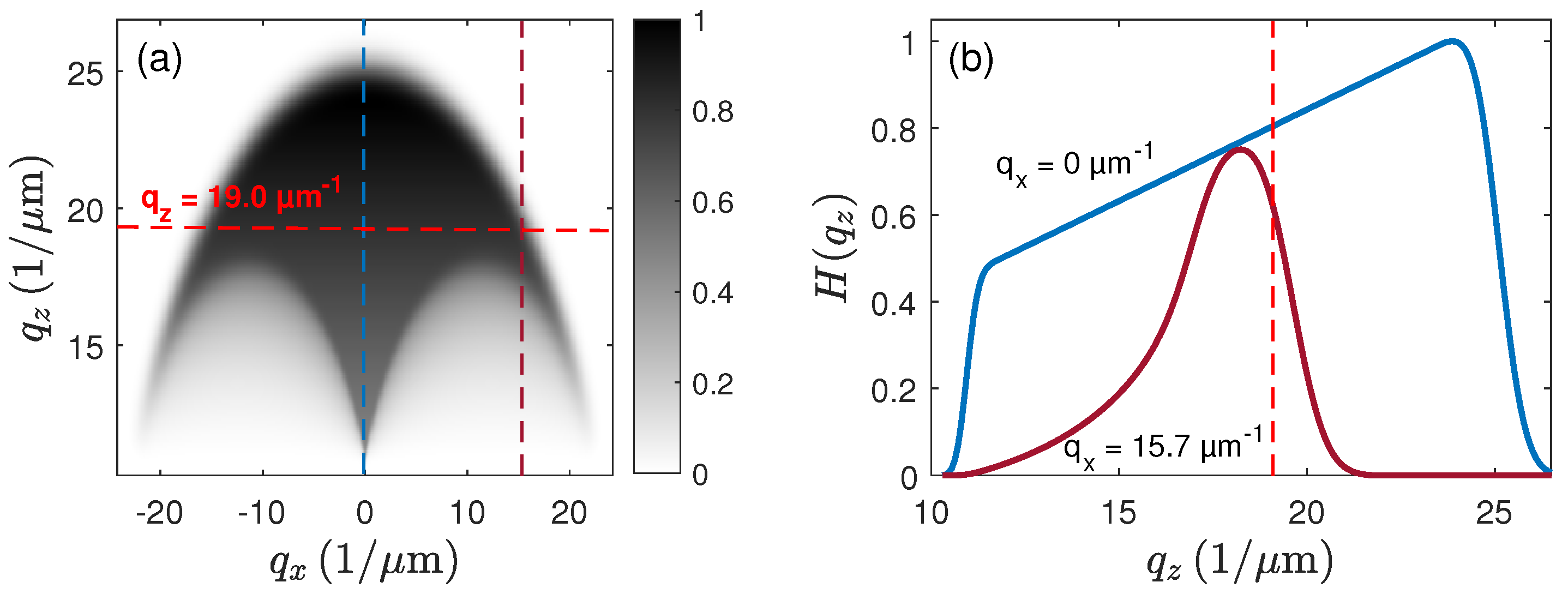
- The 2D MTF can be derived from the 3D TF via integration with respect to the coordinate [19]. Expressing the 2D MTF by an autocorrelation of a uniformly-filled 2D circular pupil function assumes that both the illumination as well as the imaging pupil plane is uniformly-filled. As discussed before, this requires single point scatterers with certain scattering characteristics on the surface under investigation. In contrast, specularly reflecting surfaces such as typical surface standards lead to a non-uniformly filled image pupil function even if the illumination pupil is uniformly-filled (see Figure 5).
- The stack of interference images resulting from a CSI measurement can be analyzed at certain axial spatial frequencies. According to [5,10], this analysis is typically performed for the so-called equivalent wavelength , which corresponds to the axial spatial frequency value . As a consequence, not the integration of with respect to plays a crucial role for surface reconstruction but the coarse of the function for a certain constant -value. This value is related to what we call the ‘evaluation wavelength’ byNote that may equal the equivalent wavelength or not.
3. Discussion
4. Materials and Methods
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Malacara, D. (Ed.) Optical Shop Testing; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Coupland, J.; Lobera, J. Holography, tomography and 3D microscopy as linear filtering operations. Meas. Sci. Technol. 2008, 19, 074012. [Google Scholar] [CrossRef] [Green Version]
- Coupland, J.; Mandal, R.; Palodhi, K.; Leach, R. Coherence scanning interferometry: Linear theory of surface measurement. Appl. Opt. 2013, 52, 3662–3670. [Google Scholar] [CrossRef] [Green Version]
- Su, R.; Thomas, M.; Liu, M.; Drs, J.; Bellouard, Y.; Pruss, C.; Coupland, J.; Leach, R. Lens aberration compensation in interference microscopy. Opt. Lasers Eng. 2020, 128, 106015. [Google Scholar] [CrossRef]
- de Groot, P.; Colonna de Lega, X. Fourier optics modeling of interference microscopes. J. Opt. Soc. Am. A 2020, 37, B1–B10. [Google Scholar] [CrossRef] [PubMed]
- Lehmann, P.; Künne, M.; Pahl, T. Analysis of interference microscopy in the spatial frequency domain. IOP J. Phys. Photonics 2021, 3, 014006. [Google Scholar] [CrossRef]
- Su, R.; Coupland, J.; Sheppard, C.; Leach, R. Scattering and three-dimensional imaging in surface topography measuring interference microscopy. J. Opt. Soc. Am. A 2021, 38, A27–A41. [Google Scholar] [CrossRef]
- Pahl, T.; Hagemeier, S.; Künne, M.; Danzglock, C.; Reinhold, N.; Schulze, R.; Siebert, M.; Lehmann, P. Vectorial 3D modeling of coherence scanning interferometry. Proc. SPIE 2021, 11783, 117830G. [Google Scholar]
- Künne, M.; Pahl, T.; Lehmann, P. Spatial-frequency domain representation of interferogram formation in coherence scanning interferometry. Proc. SPIE 2021, 11782, 117820T. [Google Scholar]
- de Groot, P.; Colonna de Lega, X.; Su, R.; Coupland, J.; Leach, R. Fourier optics modelling of coherence scanning interferometers. Proc. SPIE 2021, 11817, 118170M. [Google Scholar]
- Beckmann, P.; Spizzichino, A. The Scattering of Electromagnetic Waves from Rough Surfaces; Artech House, Inc.: Norwood, MA, USA, 1987. [Google Scholar]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- McCutchen, C.W. Generalized aperture and the three-dimensional diffraction image. J. Opt. Soc. Am. 1964, 54, 240–244. [Google Scholar] [CrossRef]
- Wombell, R.J.; DeSanto, J.A. Reconstruction of rough-surface profiles with the Kirchhoff approximation. J. Opt. Soc. Am. A 1991, 8, 1892–1897. [Google Scholar] [CrossRef]
- Sheppard, C.; Connolly, T.; Gu, M. Imaging and reconstruction for rough surface scattering in the Kirchhoff approximation by confocal microscopy. J. Mod. Opt. 1993, 40, 2407–2421. [Google Scholar] [CrossRef]
- Quartel, J.C.; Sheppard, C.J.R. Surface reconstruction using an algorithm based on confocal imaging. J. Mod. Opt. 1996, 43, 469–486. [Google Scholar] [CrossRef]
- Sheppard, C. Imaging of random surfaces and inverse scattering in the Kirchoff approximation. Waves Random Media 1998, 8, 53–66. [Google Scholar] [CrossRef]
- Xie, W.; Lehmann, P.; Niehues, J. Lateral resolution and transfer characteristics of vertical scanning white-light interferometers. Appl. Opt. 2012, 51, 1795–1803. [Google Scholar] [CrossRef] [PubMed]
- Lehmann, P.; Pahl, T. Three-dimensional transfer function of optical microscopes in reflection mode. J. Microsc. 2021, 284, 45–55. [Google Scholar] [CrossRef]
- Goodman, J.W. Introduction to Fourier Optics; Roberts and Company Publishers: Greenwood Village, CO, USA, 2005. [Google Scholar]
- Wilson, T. (Ed.) Confocal Microscopy; Academic Press, Inc.: London, UK, 1990. [Google Scholar]
- Corle, T.R.; Kino, G.S. Confocal Scanning Optical Microscopy and Related Imaging Systems; Academic Press, Inc.: San Diego, CA, USA, 1996. [Google Scholar]
- Krüger-Sehm, R.; Bakucz, P.; Jung, L.; Wilhelms, H. Chirp Calibration Standards for Surface Measuring Instruments. tm-Tech. Mess. 2007, 74, 572–576. [Google Scholar] [CrossRef]
- Singer, W.; Totzeck, M.; Gross, H. Physical Image Formation. In Handbook of Optical Systems; Wiley-VCH: Weinheim, Germany, 2006; Volume 2. [Google Scholar]
- Garces, D.H.; Rhodes, W.T.; Pena, N.M. Projection-slice theorem: A compact notation. J. Opt. Soc. Am. A 2011, 28, 766–769. [Google Scholar] [CrossRef]
- Sheppard, C.; Larkin, K. Effect of numerical aperture on interference fringe spacing. Appl. Opt. 1995, 34, 4731–4734. [Google Scholar] [CrossRef] [PubMed]
- de Groot, P.; Colonna de Lega, X. Signal modeling for low-coherence height-scanning interference microscopy. Appl. Opt. 2004, 43, 4821–4830. [Google Scholar] [CrossRef] [PubMed]
- de Groot, P.; Colonna de Lega, X.C. Interpreting interferometric height measurements using the instrument transfer function. In Fringe 2005; Springer: Berlin/Heidelberg, Germany, 2006; pp. 30–37. [Google Scholar]
- Lehmann, P.; Tereschenko, S.; Allendorf, B.; Hagemeier, S.; Hüser, L. Spectral composition of low-coherence interferograms at high numerical apertures. J. Eur. Opt. Soc.-Rapid Publ. 2019, 15, 5. [Google Scholar] [CrossRef] [Green Version]
- de Groot, P.; Deck, L. Surface profiling by analysis of white-light interferograms in the spatial frequency domain. J. Mod. Opt. 1995, 42, 389–401. [Google Scholar] [CrossRef]
- Fleischer, M.; Windecker, R.; Tiziani, H. Fast algorithms for data reduction in modern optical three-dimensional profile measurement systems with MMX technology. Appl. Opt. 2000, 39, 1290–1297. [Google Scholar] [CrossRef] [PubMed]
- Tereschenko, S. Digitale Analyse Periodischer und Transienter Messsignale Anhand von Beispielen aus der Optischen Präzisionsmesstechnik. Ph.D. Thesis, University of Kassel, Kassel, Germany, 2018. [Google Scholar]
- Lehmann, P.; Xie, W.; Allendorf, B.; Tereschenko, S. Coherence scanning and phase imaging optical interference microscopy at the lateral resolution limit. Opt. Express 2018, 26, 7376–7389. [Google Scholar] [CrossRef] [PubMed]
- Gao, F.; Leach, R.K.; Petzing, J.; Coupland, J.M. Surface measurement errors using commercial scanning white light interferometers. Meas. Sci. Technol. 2008, 19, 015303. [Google Scholar] [CrossRef] [Green Version]
- Hagemeier, S.; Schake, M.; Lehmann, P. Sensor characterization by comparative measurements using a multi-sensor measuring system. J. Sens. Sens. Syst. 2019, 8, 111–121. [Google Scholar] [CrossRef] [Green Version]
- Künne, M.; Hagemeier, S.; Käkel, E.; Hillmer, H.; Lehmann, P. Investigation of measurement data of low-coherence interferometry at tilted surfaces in the 3D spatial frequency domain. tm-Tech. Mess. 2021, 88, 65–70. [Google Scholar] [CrossRef]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lehmann, P.; Hagemeier, S.; Pahl, T. Three-Dimensional Transfer Functions of Interference Microscopes. Metrology 2021, 1, 122-141. https://doi.org/10.3390/metrology1020009
Lehmann P, Hagemeier S, Pahl T. Three-Dimensional Transfer Functions of Interference Microscopes. Metrology. 2021; 1(2):122-141. https://doi.org/10.3390/metrology1020009
Chicago/Turabian StyleLehmann, Peter, Sebastian Hagemeier, and Tobias Pahl. 2021. "Three-Dimensional Transfer Functions of Interference Microscopes" Metrology 1, no. 2: 122-141. https://doi.org/10.3390/metrology1020009
APA StyleLehmann, P., Hagemeier, S., & Pahl, T. (2021). Three-Dimensional Transfer Functions of Interference Microscopes. Metrology, 1(2), 122-141. https://doi.org/10.3390/metrology1020009





