Efficient and Accurate Modeling of the Surface Deflection of Thin Layers on Composite Substrates with Applications to Piezoelectric Parameter Measurements
Abstract
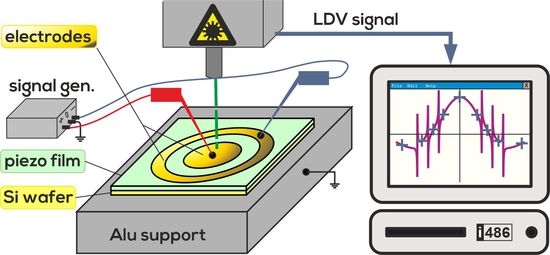
1. Introduction
2. Basic Modeling Approach
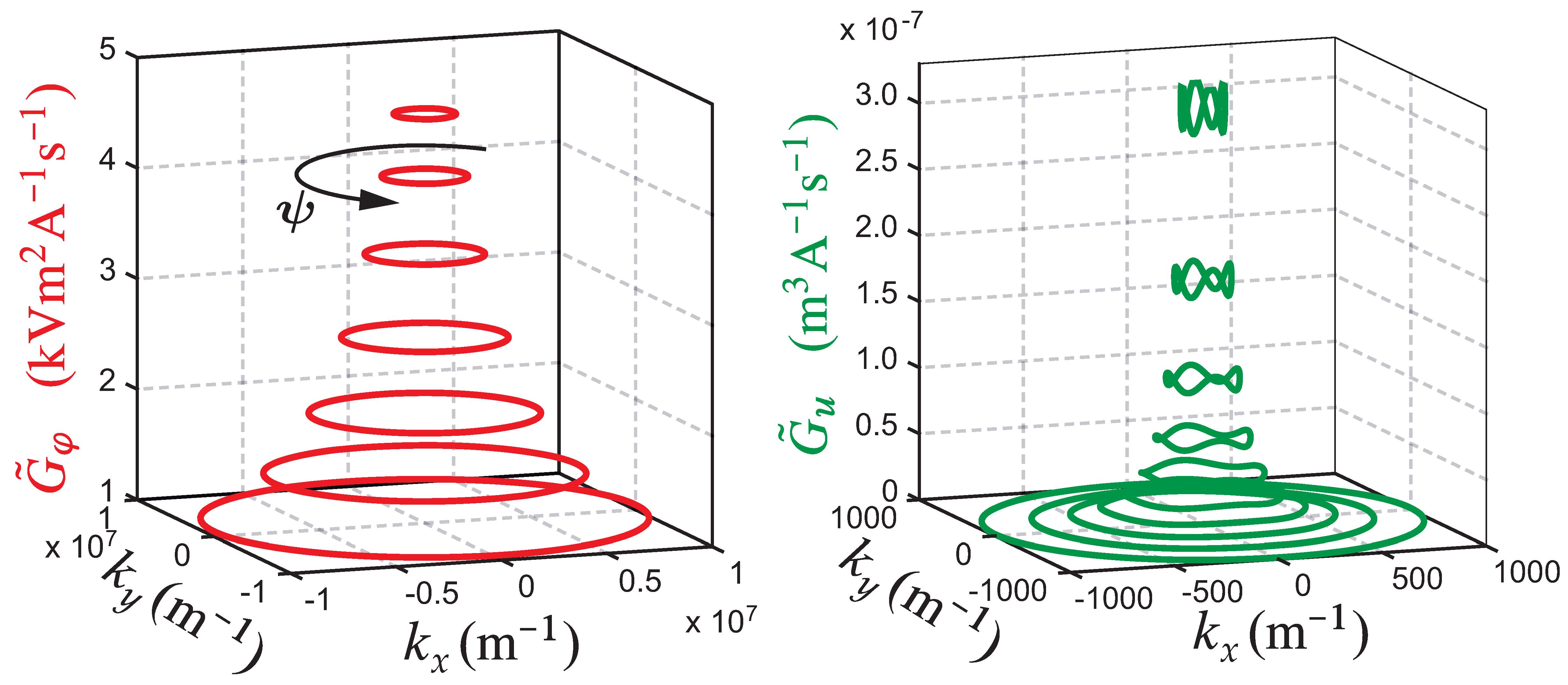
2.1. Green’s Functions
2.1.1. Symmetry Considerations
2.1.2. Dispersion Relations
2.2. Boundary Element Method
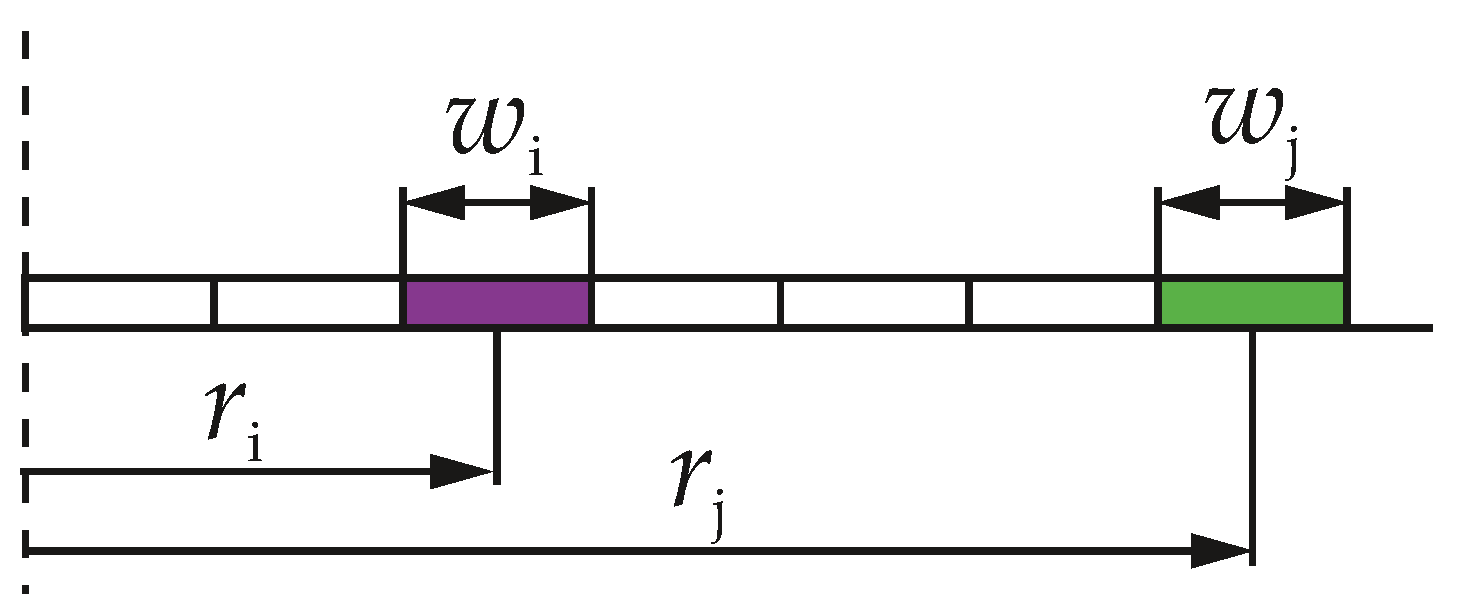
2.2.1. Method of Mean Weighted Residuals (MWR)
2.2.2. Displacement Field Calculation
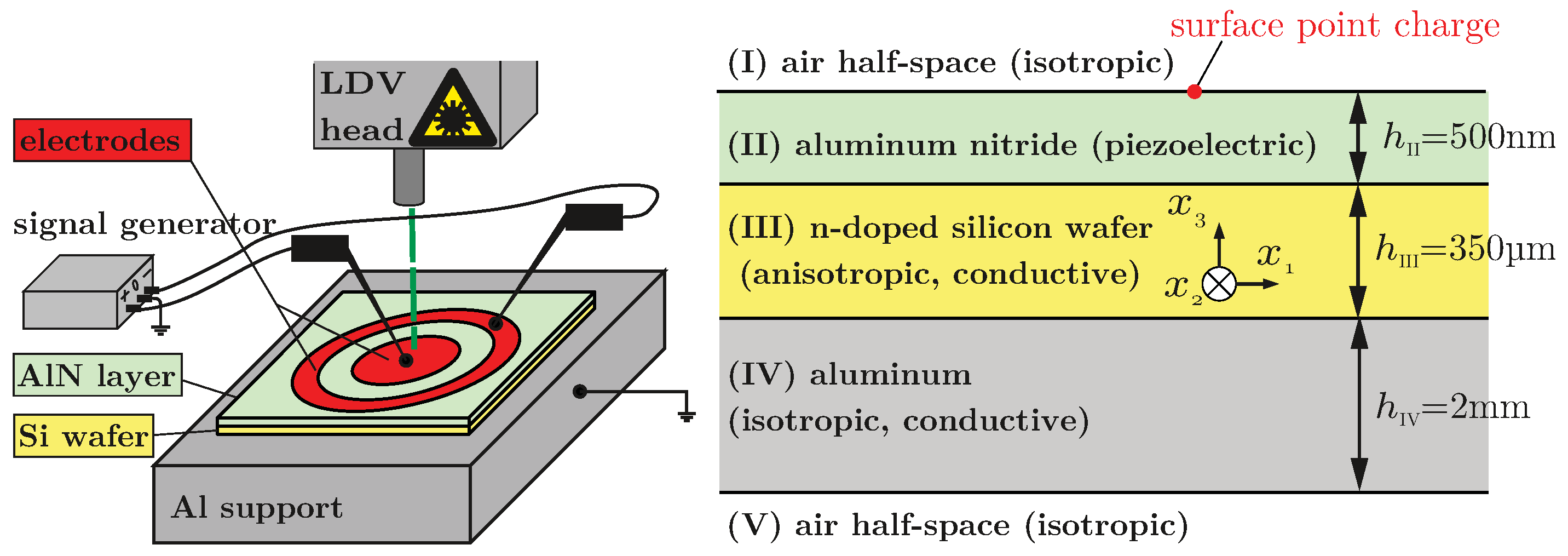
3. Application
3.1. Electrostatic Green’s Function
3.2. Calculation of the Electric Field Using MWR
3.3. Calculation of the Displacement Field
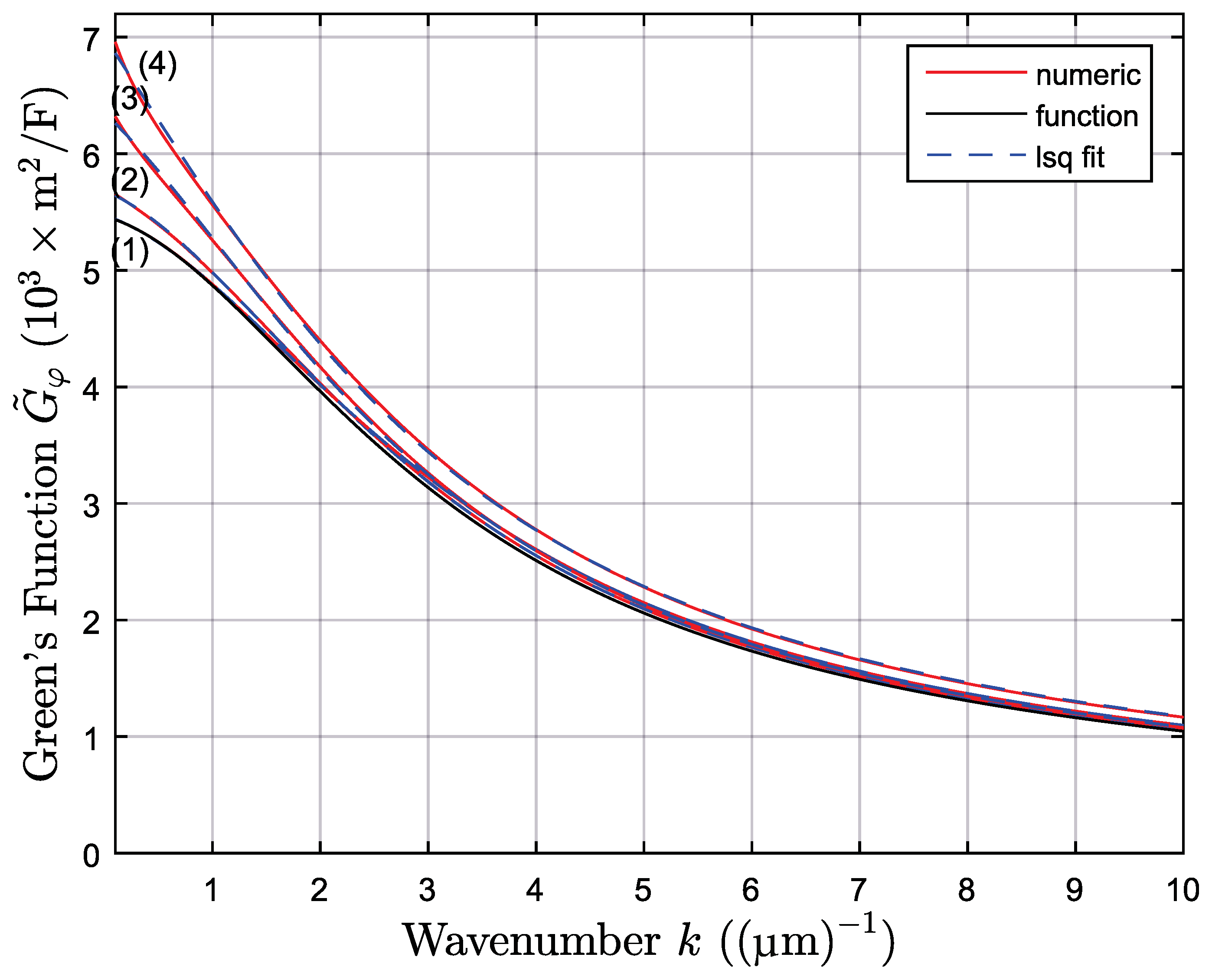
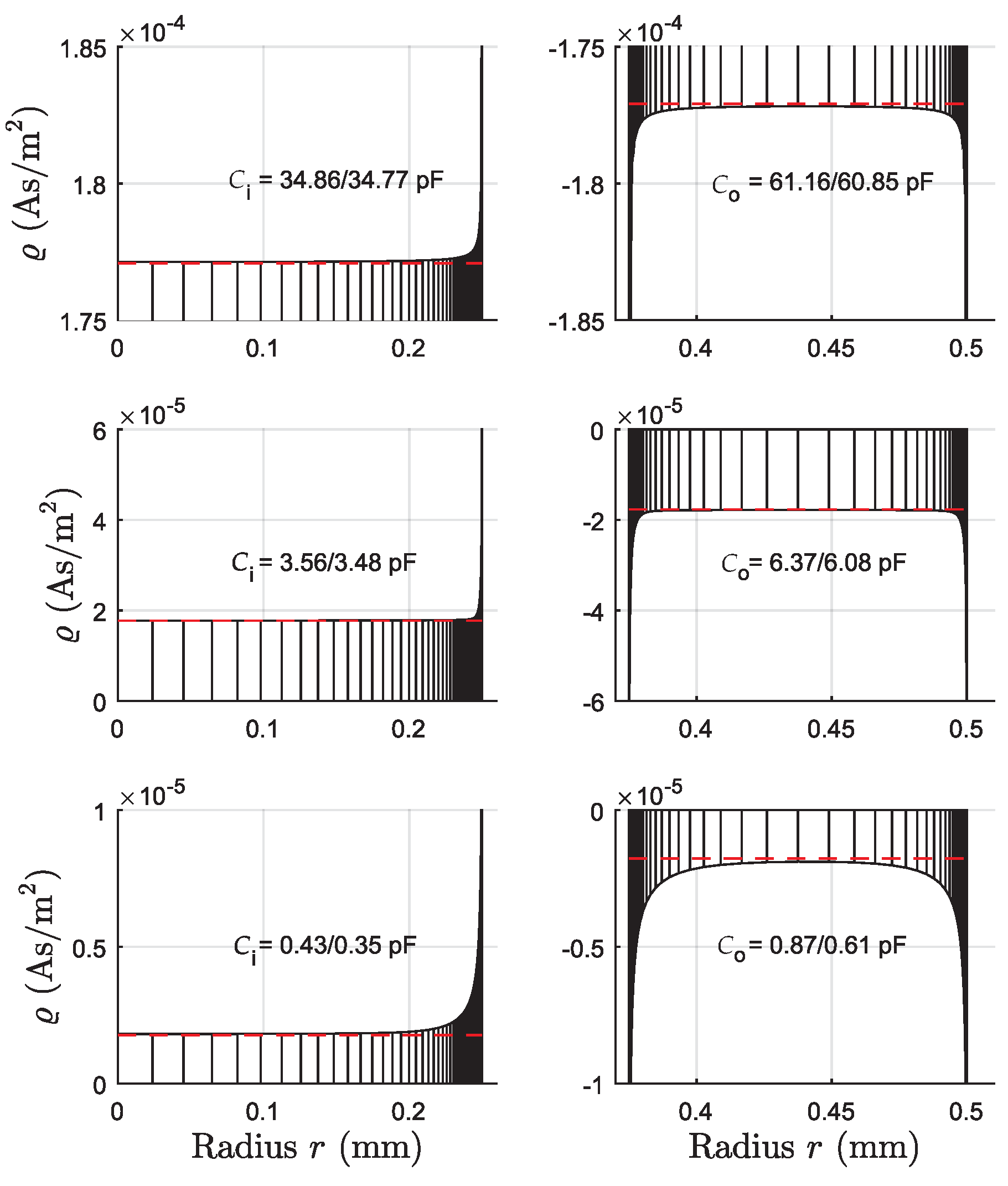
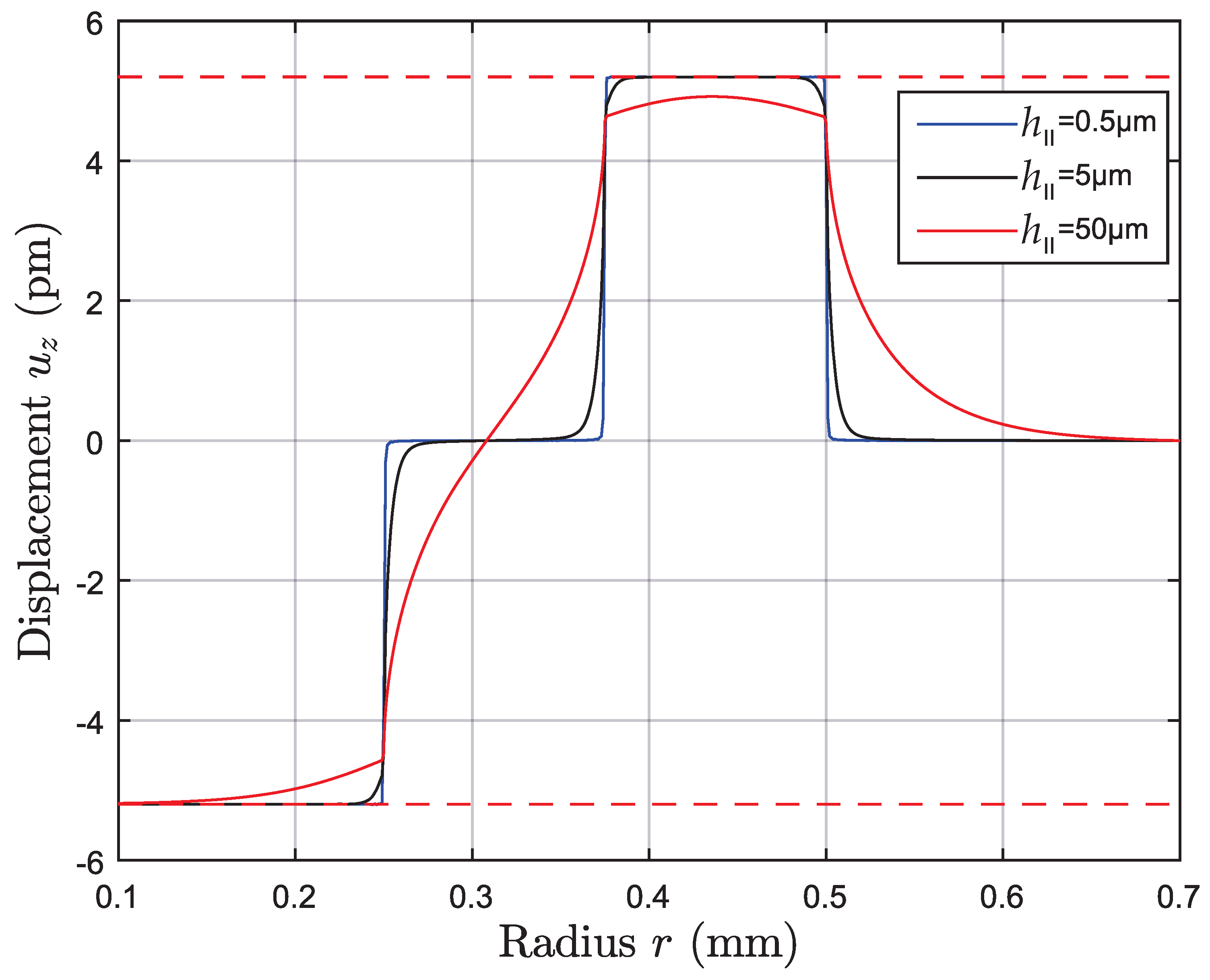
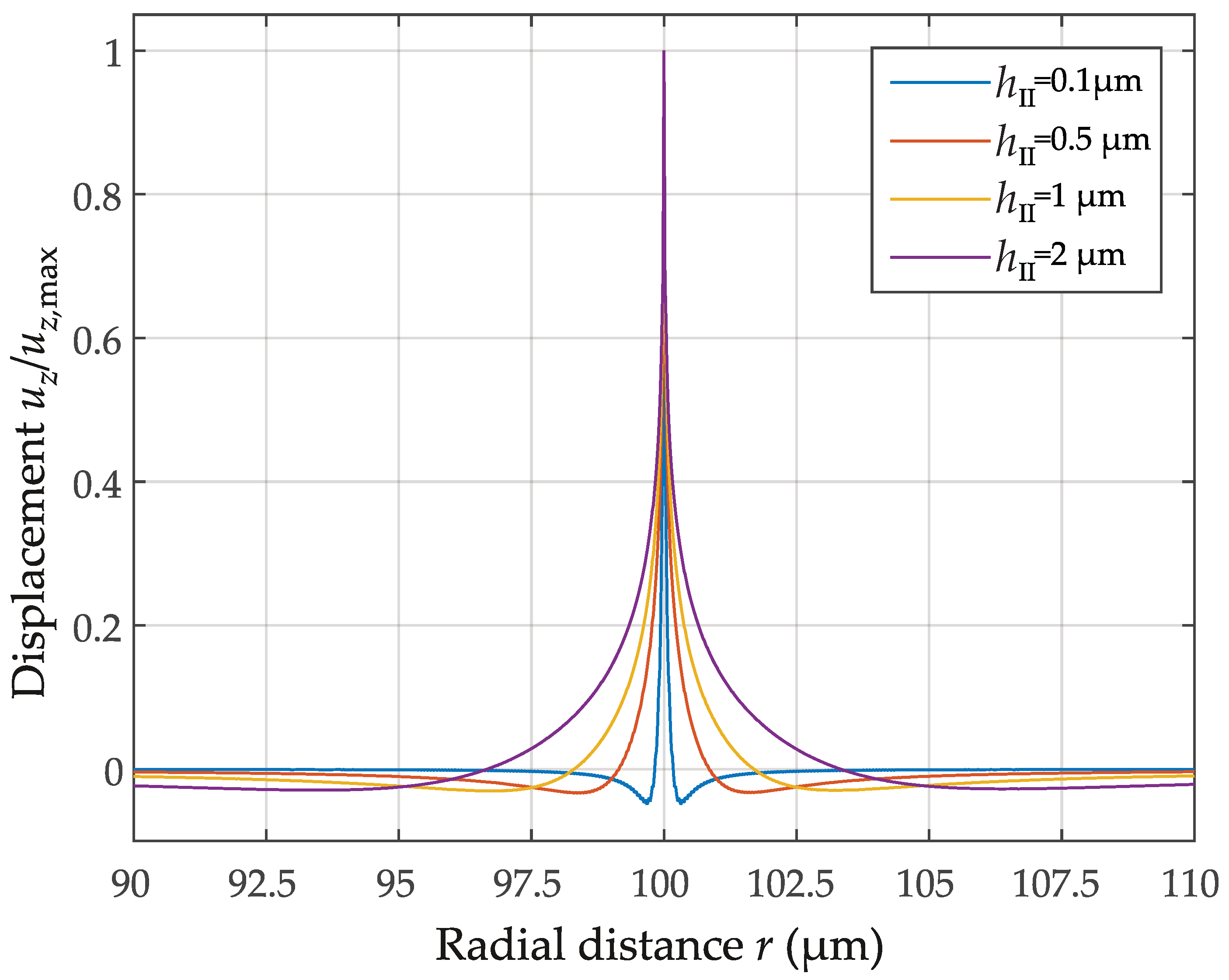
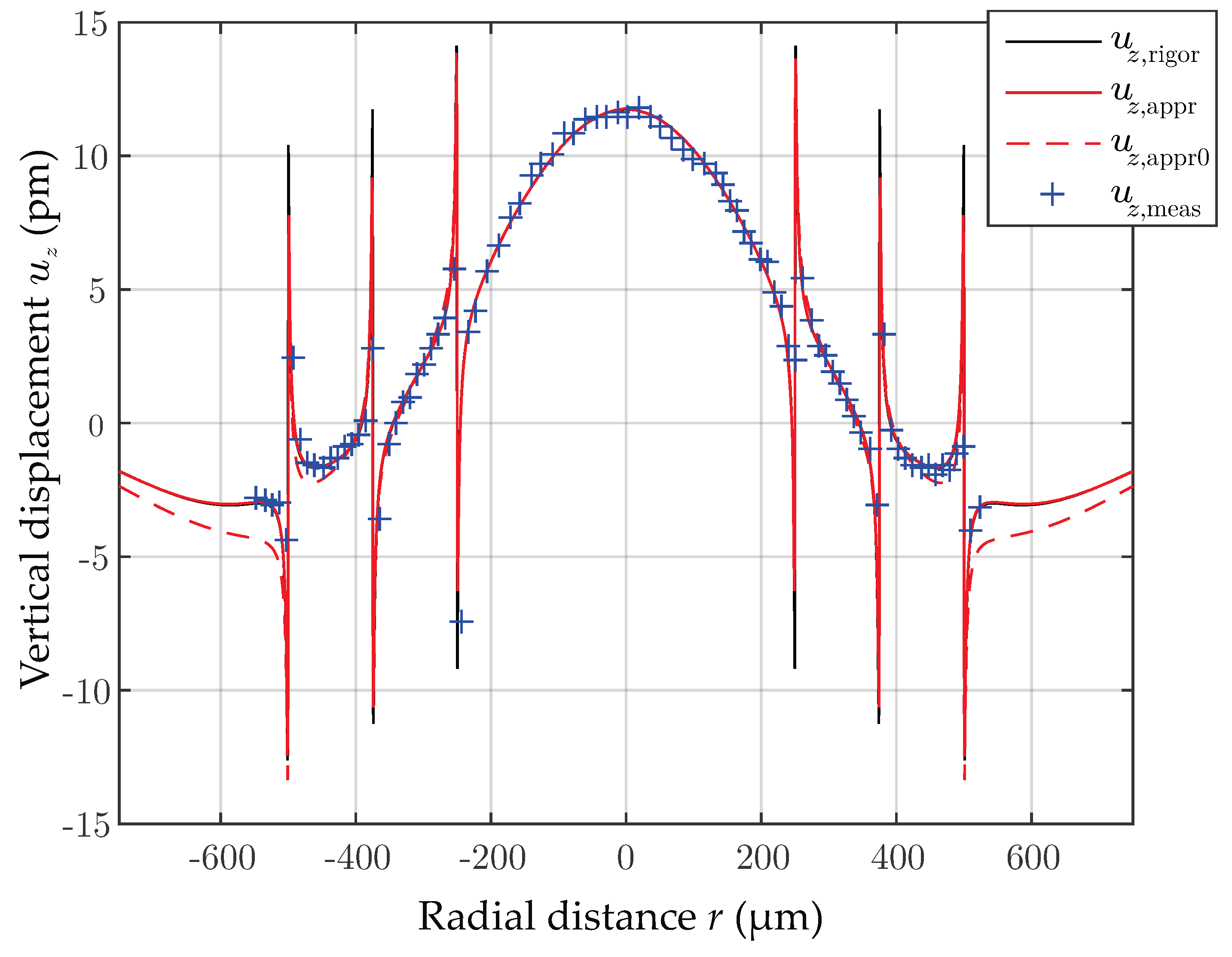
4. Results
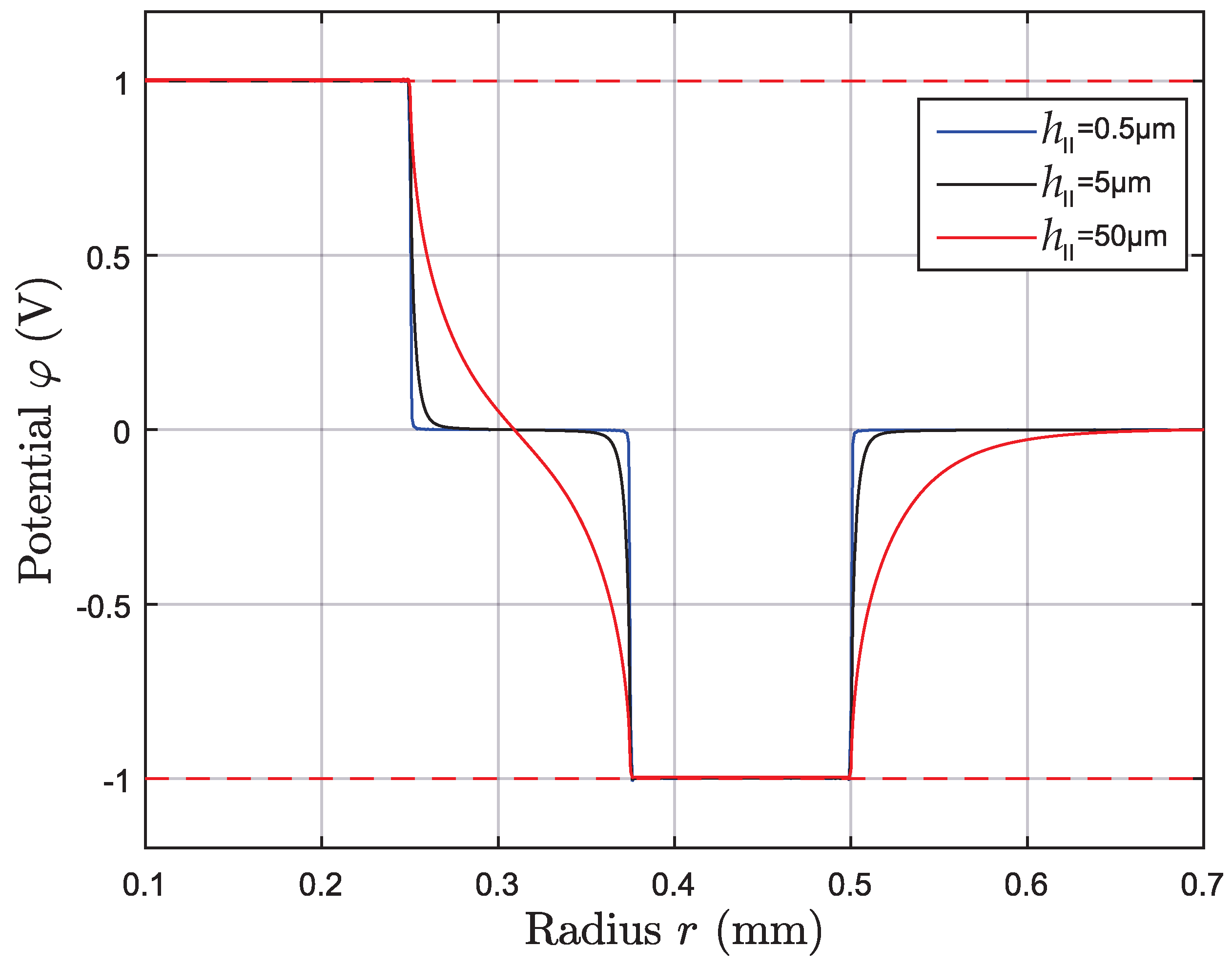
The Influence of the Radial Boundary Condition
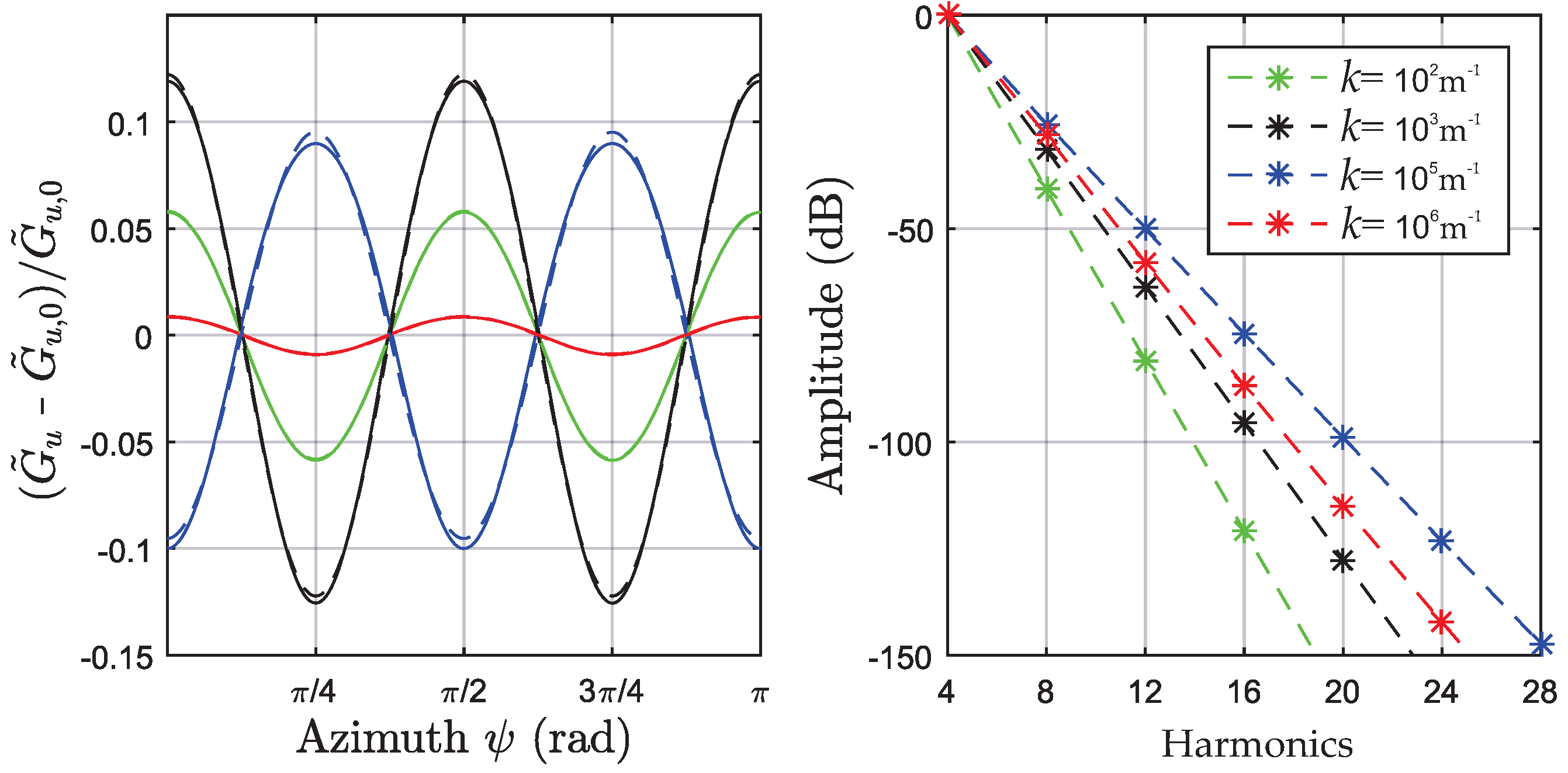
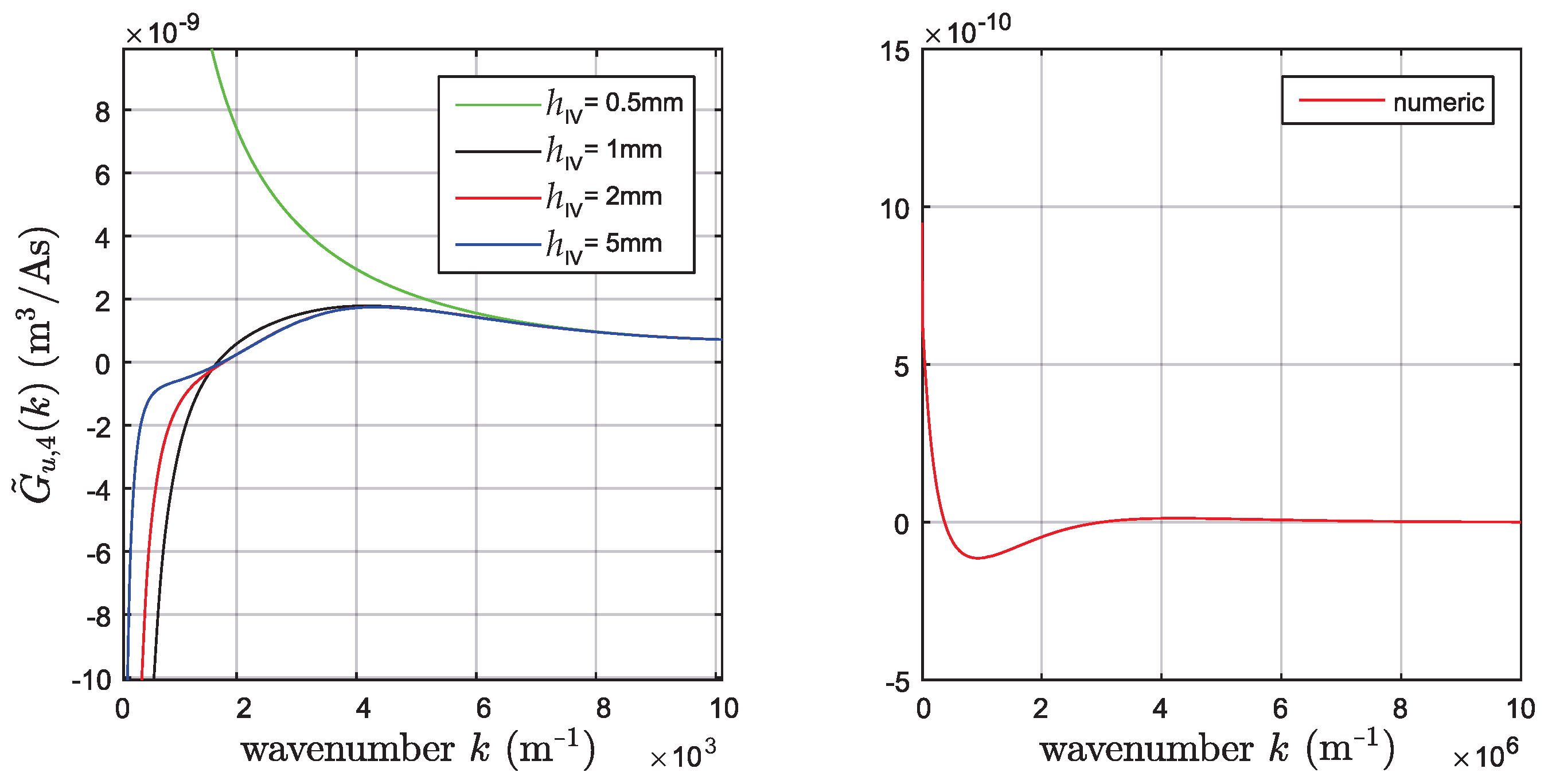
5. Efficient Calculation for Thin Layers
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix A.1. Summary of Applied Material Parameters
Appendix A.1.1. Silicon Substrate
Appendix A.1.2. Aluminum Nitride
Appendix A.1.3. Aluminum (Isotropic Elasticity)
Appendix A.2. Identities for Lipschitz-Hankel Integrals
Appendix A.3. Definitions of Complete Elliptic Integrals
References
- Kholkin, A.; Wütchrich, C.; Taylor, D.; Setter, N. Interferometric measurements of electric field-induced displacements in piezoelectric thin films. Rev. Sci. Instrum. 1996, 67, 1935–1941. [Google Scholar] [CrossRef]
- Hernando, J.; Sánchez-Rojas, J.; González-Castilla, S.; Iborra, E.; Ababneh, A.; Schmid, U. Simulation and laser vibrometry characterization of piezoelectric AlN thin films. J. Appl. Phys. 2008, 104, 053502. [Google Scholar] [CrossRef]
- Mayrhofer, P.; Euchner, H.; Bittner, A.; Schmid, U. Circular test structure for the determination of piezoelectric constants of ScxAl1-xN thin films applying Laser Doppler Vibrometry and FEM simulations. Sens. Actuators Phys. 2015, 222, 301–308. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Hashimoto, K.y.; Watanabe, Y.; Akahane, M.; Yamaguchi, M. Analysis of acoustic properties of multi-layered structures by means of effective acoustic impedance matrix. In Proceedings of the Ultrasonics Symposium, Honolulu, HI, USA, 4–7 December 1990; Volume 2, pp. 937–942. [Google Scholar]
- Peach, R. A general Green function analysis for SAW devices. In Proceedings of the Ultrasonics Symposium, Seattle, WA, USA, 7–10 November 1995; Volume 1, pp. 221–225. [Google Scholar]
- Baghai-Wadji, A.; Penunuri, D. Coordinate-free, frequency-independent universal functions for BAW analysis in SAW devices. In Proceedings of the Ultrasonics Symposium, Seattle, WA, USA, 7–10 November 1995; Volume 1, pp. 287–290. [Google Scholar]
- Laude, V.; Jerez-Hanckes, C.F.; Ballandras, S. Surface Green’s function of a piezoelectric half-space. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2006, 53, 420–428. [Google Scholar] [CrossRef] [PubMed]
- Voglhuber-Brunnmaier, T.; Reichel, E.K.; Jakoby, B.; Beigelbeck, R.; Mayrhofer, P.M.; Schmid, U. Fast method for the calculation of surface bending on circular multilayered piezoelectric structures. In Proceedings of the SENSORS, Orlando, FL, USA, 30 October–3 November 2016; pp. 1–3. [Google Scholar]
- Jakoby, B. Efficient semi-numerical analysis of acoustic sensors using spectral domain methods—A review. Meas. Sci. Technol. 2008, 19, 052001. [Google Scholar] [CrossRef]
- Reichinger, H.; Baghai-Wadji, A.; Seifert, F. Stress pattern on the electrode/substrate interfaces in SAW-devices. In Proceedings of the Ultrasonics Symposium, Baltimore, MD, USA, 31 October–3 November 1993; Volume 1, p. 153. [Google Scholar]
- Worden, K. Rayleigh and Lamb Waves-Basic Principles. Strain 2001, 37, 167–172. [Google Scholar] [CrossRef]
- Voglhuber-Brunnmaier, T.; Beigelbeck, R.; Jakoby, B. Semi-numeric boundary element method for piezoelectric fluid sensors using a fourier spectral approach. In Proceedings of the SENSORS, Valencia, Spain, 2–5 November 2014; pp. 594–597. [Google Scholar]
- Voglhuber-Brunnmaier, T.; Reichel, E.; Jakoby, B. Efficient spectral domain formulation of loading effects in acoustic sensors. Sens. Actuators A-Phys. 2011, 186, 38–47. [Google Scholar] [CrossRef]
- ANSI/IEEE Std 176-1987; IEEE Standard on Piezoelectricity. IEEE: Piscataway, NJ, USA, 1988. [CrossRef]
- Auld, B.A. Acoustic Fields and Waves in Solids: Volume 1; John Wiley: New York, NJ, USA, 1973. [Google Scholar]
- Nye, J. Physical Properties of Crystals: Their Representation by Tensors and Matrices; Oxford University Press: Oxford, UK, 1984. [Google Scholar]
- Davino, D.; Oliviero, C.; Panariello, G.; Verolino, L. Accurate evaluation of the partial capacitance of two coupled microstrips. Electr. Eng. 2000, 82, 207–212. [Google Scholar] [CrossRef]
- Panariello, G.; Schettino, F.; Verolino, L. Capacitance of an annular ring between two conducting plates. Electr. Eng. 1999, 82, 11–15. [Google Scholar] [CrossRef]
- Sneddon, I.N. Mixed Boundary Value Problems in Potential Theory; North-Holland Publishing Company: Amsterdam, The Netherlands, 1966. [Google Scholar]
- Eason, G.; Noble, B.; Sneddon, I. On certain integrals of Lipschitz-Hankel type involving products of Bessel functions. Philos. Trans. R. Soc. Lond. Math. Phys. Eng. Sci. 1955, 247, 529–551. [Google Scholar]
- Watson, G. A Treatise on the Theory of Bessel Functions, 2nd ed.; Cambridge University Press: Cambridge, UK, 1966; Volume 67. [Google Scholar]
- Luke, Y.L. Integrals of Bessel Functions; McGraw-Hill: New York, NY, USA, 1962. [Google Scholar]
- Carlson, B. Computing elliptic integrals by duplication. Numer. Math. 1979, 33, 1–16. [Google Scholar] [CrossRef]
- Hoffend, T. Compute Incomplete Legendre Elliptic Integrals of the First, Second, and Third Kind. 2003. Available online: http://www.mathworks.com/matlabcentral/fileexchange/3705-elliptic-integrals-zip (accessed on 1 June 2022).
- Longman, I. A method for the numerical evaluation of finite integrals of oscillatory functions. Math. Comput. 1960, 14, 53–59. [Google Scholar] [CrossRef]
- Hauer, J.F.; Demeure, C.; Scharf, L. Initial results in Prony analysis of power system response signals. IEEE Trans. Power Syst. 1990, 5, 80–89. [Google Scholar] [CrossRef]
- Lucas, S. Evaluating infinite integrals involving products of Bessel functions of arbitrary order. J. Comput. Appl. Math. 1995, 64, 269–282. [Google Scholar] [CrossRef]
- Magnus, W.; Oberhettinger, F.; Soni, R.P. Formulas and Theorems for the Special Functions of Mathematical Physics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1966; Volume 52. [Google Scholar]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Voglhuber-Brunnmaier, T.; Beigelbeck, R.; Schmid, U.; Sauter, T.; You, T.; Ou, X.; Jakoby, B. Efficient and Accurate Modeling of the Surface Deflection of Thin Layers on Composite Substrates with Applications to Piezoelectric Parameter Measurements. Micro 2022, 2, 369-389. https://doi.org/10.3390/micro2030025
Voglhuber-Brunnmaier T, Beigelbeck R, Schmid U, Sauter T, You T, Ou X, Jakoby B. Efficient and Accurate Modeling of the Surface Deflection of Thin Layers on Composite Substrates with Applications to Piezoelectric Parameter Measurements. Micro. 2022; 2(3):369-389. https://doi.org/10.3390/micro2030025
Chicago/Turabian StyleVoglhuber-Brunnmaier, Thomas, Roman Beigelbeck, Ulrich Schmid, Thilo Sauter, Tiangui You, Xin Ou, and Bernhard Jakoby. 2022. "Efficient and Accurate Modeling of the Surface Deflection of Thin Layers on Composite Substrates with Applications to Piezoelectric Parameter Measurements" Micro 2, no. 3: 369-389. https://doi.org/10.3390/micro2030025
APA StyleVoglhuber-Brunnmaier, T., Beigelbeck, R., Schmid, U., Sauter, T., You, T., Ou, X., & Jakoby, B. (2022). Efficient and Accurate Modeling of the Surface Deflection of Thin Layers on Composite Substrates with Applications to Piezoelectric Parameter Measurements. Micro, 2(3), 369-389. https://doi.org/10.3390/micro2030025