Abraham General Solvation Parameter Model: Predictive Expressions for Solute Transfer into Isobutyl Acetate
Abstract
1. Introduction
2. Chemical Materials and Experimental Methodology
3. Results and Discussion
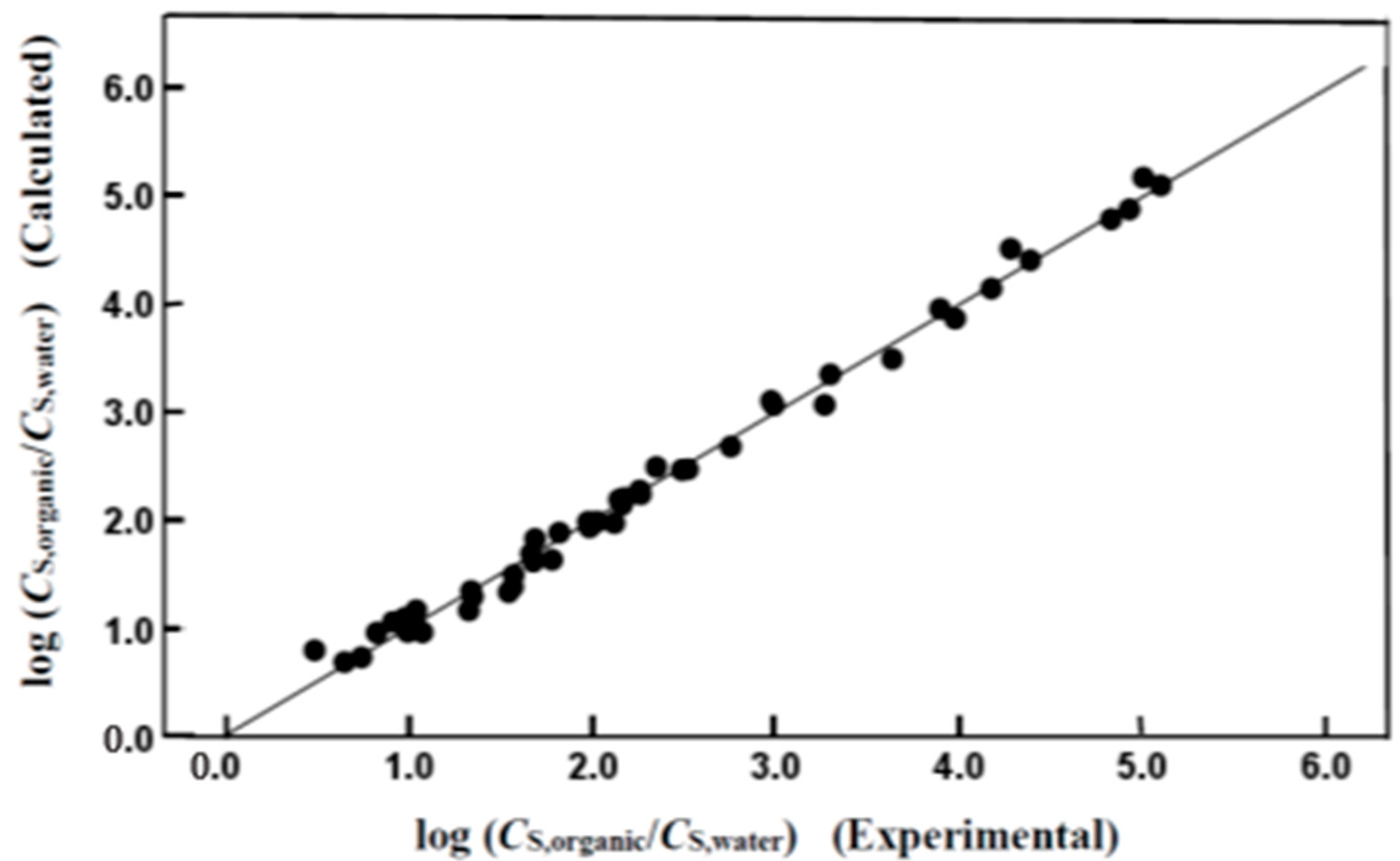
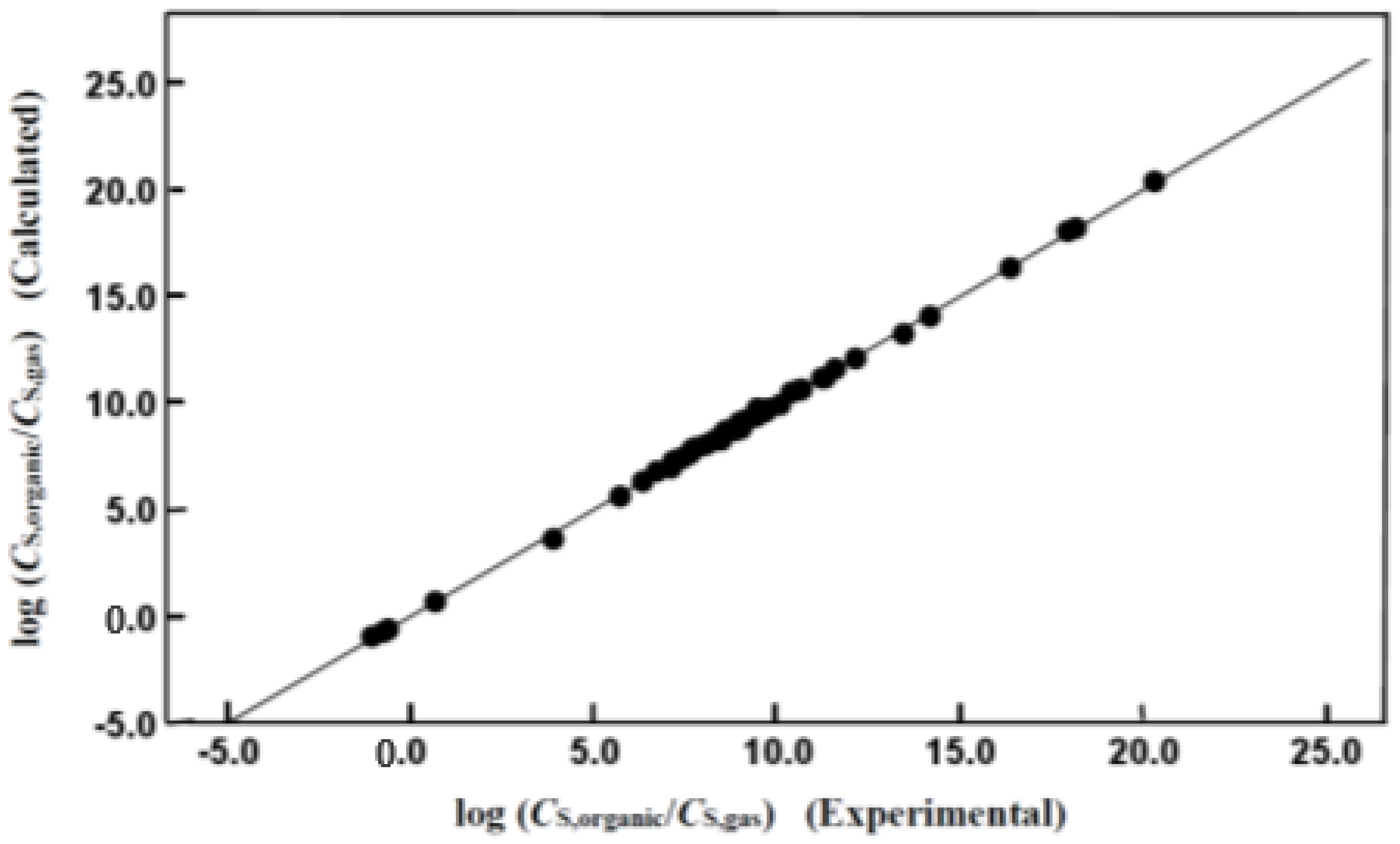
(with N = 49, SD = 0.112, SEE = 0.119; R2 = 0.992, F = 1099)
+ 0.936(0.015) L
(with N = 49, SD = 0.112, SEE = 0.117, R2 = 0.999, F = 15753)
4. Summary
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Abraham, M.H. Scales of solute hydrogen-bonding: Their construction and application to physicochemical and biochemical processes. Chem. Soc. Rev. 1993, 22, 73–83. [Google Scholar] [CrossRef]
- Abraham, M.H.; Ibrahim, A.; Zissimos, A.M. Determination of sets of solute descriptors from chromatographic measurements. J. Chromatogr. A 2004, 1037, 29–47. [Google Scholar] [CrossRef]
- Abraham, M.H.; Smith, R.E.; Luchtefeld, R.; Boorem, A.J.; Luo, R.; Acree, W.E., Jr. Prediction of solubility of drugs and other compounds in organic solvents. J. Pharm. Sci. 2010, 99, 1500–1515. [Google Scholar] [CrossRef] [PubMed]
- Jalan, A.; Ashcraft, R.W.; West, R.H.; Green, W.H. Predicting solvation energies for kinetic modeling. Annu. Rep. Prog. Chem. Sec. C Phys. Chem. 2010, 106, 211–258. [Google Scholar] [CrossRef]
- Clarke, E.D. Beyond physical properties–application of Abraham descriptors and LFER analysis in agrochemical research. Bioorg. Med. Chem. 2009, 17, 4153–4159. [Google Scholar] [CrossRef] [PubMed]
- Poole, C.F.; Ariyasena, T.C.; Lenca, N. Estimation of the environmental properties of compounds from chromatographic properties and the solvation parameter method. J. Chromatogr. A 2013, 1317, 85–104. [Google Scholar] [CrossRef]
- Poole, C.F.; Atapattu, S.N. Recent advances for estimating environmental properties for small molecules from chromato-graphic measurements and the solvation parameter model. J. Chromatogr. A 2022, 1687, 463682. [Google Scholar] [CrossRef]
- Endo, S.; Goss, K.-U. Applications of polyparameter linear free energy relationships in environmental chemistry. Environ. Sci. Technol. 2014, 48, 12477–12491. [Google Scholar] [CrossRef]
- Poole, C.F. Assessment of liquid-liquid partition for the assignment of descriptors for the solvation parameter model. J. Chromatogr. A 2024, 1721, 464850. [Google Scholar] [CrossRef]
- Poole, C.F. Determination of solvation parameter model compound descriptors by gas chromatography. J. Chromatogr. A 2024, 1717, 464711. [Google Scholar] [CrossRef]
- Dayani, M.T.; Ambagaspitiya, A.W.T.D.; Atapattu, S.N.; Ariyasena, T.C. Determination of experimental solute descriptor values for safrole by liquid-liquid partitioning and gas chromatography. Phys. Chem. Liq. 2024, in press. [CrossRef]
- Liu, X.; Acree, W.E., Jr.; Abraham, M.H. Descriptors for some compounds with pharmacological activity; calculation of properties. Int. J. Pharm. 2022, 617, 121597. [Google Scholar] [CrossRef] [PubMed]
- Orlov, A.A.; Demenko, D.Y.; Bignaud, C.; Valtz, A.; Marcou, G.; Horvath, D.; Coquelet, C.; Varnek, A.; de Meyer, F. Chemoinformatics-driven design of new physical solvents for selective CO2 absorption. Environ. Sci. Technol. 2021, 55, 15542–15553. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.-S.; Yi, Z.-M.; Su, M.-G.; Sun, X.-F. Solubility of naphthalene in isobutyl acetate, n-butyric acid, ethyl acetate, N-methyl pyrrolidone, N,N-dimethylformamide, and tetrahydrofuran. J. Chem. Eng. Data 2008, 53, 2701–2703. [Google Scholar] [CrossRef]
- Sun, M.; Xiao, Y.; Zhou, Y.; Zhang, B.; Cui, P.; Zhou, L.; Yin, Q. Solubility measurement and thermodynamic properties of fluorene in 14 pure solvents at temperatures from 278.15 to 318.15 K. J. Chem. Eng. Data 2023, 68, 2491–2499. [Google Scholar] [CrossRef]
- Zhao, S.; Chen, X.; Dai, Q.; Wang, L. Solubilities of benzene carboxylic acids in isobutyl acetate from (299.73 to 353.15) K. J. Chem. Eng. Data 2011, 56, 2399–2402. [Google Scholar] [CrossRef]
- Tully, G.; Hou, G.; Glennon, B. Solubility of benzoic acid and aspirin in pure solvents using focused beam reflective measurement. J. Chem. Eng. Data 2016, 61, 594–601. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, Y.; Li, H.; Li, Y.; Li, T.; Ren, B. Solubility measurement and Hansen solubility parameter of forchlorfenuron in 12 organic pure solvents at various temperatures. J. Chem. Eng. Data 2022, 67, 3278–3289. [Google Scholar] [CrossRef]
- Yu, F.; Wang, F.; Zhang, L.; Zhang, M.; Zhou, L.; Xie, C.; Bao, Y.; Chen, W.; Gong, J. Uncovering dissolution behavior and thermodynamic properties of metronidazole benzoate in twelve mono-solvents by experiments and molecular simulation. J. Mol. Liq. 2024, 393, 123539. [Google Scholar] [CrossRef]
- Wan, Y.; Gao, X.; Wang, R.; Li, F.; Li, Y.; He, H. Research on solubility behavior of iminostilbene in twelve mono-solvents: Measurement, modeling, molecular simulation and thermodynamic properties. J. Chem. Thermodyn. 2024, 188, 107182. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, H.; Dai, J.; Niu, Y.; Yin, Q.; Zhou, L. Solubility determination, model evaluation and solution thermodynamics of isovanillin in 15 pure solvents and 4 binary solvents. J. Mol. Liq. 2021, 340, 116847. [Google Scholar] [CrossRef]
- Hong, M.; Xu, L.; Ren, G.; Chen, J.; Qi, M. Solubility of lansoprazole in different solvents. Fluid Phase Equilib. 2012, 331, 18–25. [Google Scholar] [CrossRef]
- Liu, Y.; Cui, P.; Zhou, L.; Yin, Q. Measurement and correlation of solubility and thermodynamic properties of 2,2′-bis(2-hydroxyethoxy)-1,1′-binaphthalene form B in twelve pure solvents. J. Chem. Eng. Data 2022, 67, 1541–1549. [Google Scholar] [CrossRef]
- Nti-Gyabaah, J.; Chiew, Y.C. Solubility of lovastatin in ethyl acetate, propyl acetate, isopropyl acetate, butyl acetate, sec-butyl acetate, isobutyl acetate, tert-butyl acetate, and 2-butanone, between (285 and 313) K. J. Chem. Eng. Data 2008, 53, 2060–2065. [Google Scholar] [CrossRef]
- Hu, X.; Gong, Y.; Cao, Z.; Huang, Z.; Sha, J.; Li, Y.; Li, T.; Ren, B. Solubility, Hansen solubility parameter and thermodynamic properties of etodolac in twelve organic pure solvents at different temperatures. J. Mol. Liq. 2020, 316, 113779. [Google Scholar] [CrossRef]
- Yan, J.; Yin, Q.; Jiang, C.; Gong, J.; Zhang, M.; Wang, Y.; Hou, B.; Hao, H. Solution thermodynamics of simvastatin in pure solvents and binary solvent mixtures. Fluid Phase Equilib. 2015, 406, 77–90. [Google Scholar] [CrossRef]
- Nti-Gyabaah, J.; Chan, V.; Chiew, Y.C. Solubility and limiting activity coefficient of simvastatin in different organic solvents. Fluid Phase Equilib. 2009, 280, 35–41. [Google Scholar] [CrossRef]
- Shen, Y.; Li, R.; Zhao, P.; Liu, W.; Yang, X.; Zhang, Z. Equilibrium solubility of 18β-glycyrrhetinic acid in 12 pure solvents: Determination, correlation, and Hansen solubility parameter. J. Chem. Eng. Data 2022, 67, 3243–3251. [Google Scholar] [CrossRef]
- He, H.; Wan, Y.; Sha, J.; Sun, R.; Jiang, G.; Li, Y.; Li, T.; Ren, B. Equilibrium solubility of exo-5,6-dehydronorcantharidin in thirteen pure solvents: Determination, correlation, Hansen solubility parameter and thermodynamic properties. J. Mol. Liq. 2020, 312, 113384. [Google Scholar] [CrossRef]
- Monton, J.B.; Munoz, R.; Burguet, M.C.; de la Torre, J. Isobaric vapor-liquid equilibria for the binary systems isobutyl alcohol + isobutyl acetate and tert-butyl alcohol + tert-butyl acetate at 20 and 101.3 kPa. Fluid Phase Equilib. 2005, 227, 19–25. [Google Scholar] [CrossRef]
- Acree, W.E., Jr. IUPAC-NIST Solubility Data Series. Volume 99. Solubility of Benzoic Acid and Substituted Benzoic Acids in Both Neat Organic Solvents and Organic Solvent Mixtures. J. Phys. Chem. Ref. Data 2013, 42, 033103/1–033103/525. [Google Scholar] [CrossRef]
- Fritz, J.S.; Lisicki, N.M. Titration of acids in nonaqueous solvents. Anal. Chem. 1951, 23, 589–591. [Google Scholar] [CrossRef]
- Maiti, A.; Reddy, P.V.N.; Sturdy, M.; Marler, L.; Pegan, S.D.; Mesecar, A.D.; Pezzuto, J.M.; Cushman, M. Synthesis of casimiroin and optimization of its quinone reductase 2 and aromatase inhibitory activities. J. Med. Chem. 2009, 52, 1873–1884. [Google Scholar] [CrossRef]
- Acree, W.; Chickos, J.S. Phase Transition Enthalpy Measurements of Organic and Organometallic Compounds and Ionic Liquids. Sublimation, Vaporization, and Fusion Enthalpies from 1880 to 2015. Part 2. C11-C192. J. Phys. Chem. Ref. Data 2017, 46, 013104/1–013104/532. [Google Scholar] [CrossRef]
- Bai, H.; Fang, X.; Peng, C.; Liu, Q.; Xie, W.; Jia, L.; Song, Z. Dissolution behavior of 2-ethylanthraquinone in various neat solvents: Solubility, correlation and thermodynamic analysis. J. Mol. Liq. 2019, 289, 111122. [Google Scholar] [CrossRef]
- Liu, C.X. Solubility Measurement and Intrinsic Kinetics Study on Hydrogenation of Alkylanthraquinone. Ph.D. Thesis, Tianjin University, Tianjin, China, 2005. [Google Scholar]
- Acree, W.; Chickos, J.S. Phase transition enthalpy measurements of organic and organometallic compounds. sublimation, vaporization and fusion enthalpies from 1880 to 2015. Part 1. C1–C10. J. Phys. Chem. Ref. Data 2016, 45, 033101/1–033101/565. [Google Scholar] [CrossRef]
- Dippy, J.F.J.; Hughes, S.R.C.; Laxton, J.W. Chemical constitution and the dissociation constants of monocarboxylic acids. XV. Steric effects in substituted nitrobenzoic acids. J. Chem. Soc. 1956, 2995–3000. [Google Scholar] [CrossRef]
- Le Fave, G.M. Some reactions of the trifluoromethyl group in the benzotrifluoride series. I. Hydrolysis. J. Am. Chem. Soc. 1949, 71, 4148–4149. Available online: https://pubs.acs.org/doi/epdf/10.1021/ja01180a507 (accessed on 10 May 2024). [CrossRef]
- Wan, Y.; He, H.; Gao, X.; Guo, X.; Li, F.; Li, Y. Solid-liquid equilibrium of 2,3-dimethoxybenzoic acid in fifteen mono-solvents: Determination, correlation, Hansen solubility parameter, molecular dynamic simulation and thermodynamic analysis. J. Mol. Liq. 2022, 348, 118029. [Google Scholar] [CrossRef]
- Monte, M.J.S.; Hillesheim, D.M. Thermodynamic study on the sublimation of six methylnitrobenzoic acids. J. Chem. Thermodyn. 2001, 33, 103–112. [Google Scholar] [CrossRef]
- Li, H.; Wang, Y.; Wu, Y.; Li, Y.; Li, T.; Ren, B. Solubility and thermodynamic properties of 2-naphthoxyacetic acid in twelve mono-solvents. J. Chem. Thermodyn. 2023, 177, 106934. [Google Scholar] [CrossRef]
- Huang, C.-Y.; Tang, M.; Chen, Y.-P. Solid-liquid equilibria for 3-aminophenol + 2-aminophenol, 4-(acetylamino)toluene + 2-(acetylamino)toluene, and 3,4,5-trimethoxybenzoic acid + 2,4,5-trimethoxybenzoic acid. J. Chem. Eng. Data 2005, 50, 40–43. [Google Scholar] [CrossRef]
- Rathi, P.B.; Deshpande, K.V. Extended Hildebrand approach: An empirical model for solubility prediction of etodolac in 1,4-dioxane and water mixtures. J. Solut. Chem. 2014, 43, 1886–1903. [Google Scholar] [CrossRef]
- Acree, W.E., Jr. Commentary on Extended Hildebrand approach: An empirical model for solubility prediction of etodolac in 1,4-dioxane and water mixtures. J. Solut. Chem. 2017, 46, 2130–2133. [Google Scholar] [CrossRef]
- Charlton, A.K.; Daniels, C.R.; Acree, W.E., Jr.; Abraham, M.H. Solubility of crystalline nonelectrolyte solutes in organic solvents: Mathematical correlation of acetylsalicylic acid solubilities with the Abraham general solvation model. J. Solut. Chem. 2003, 32, 1087–1102. [Google Scholar] [CrossRef]
- Hart, E.; Lee, G.; Qian, E.; Jodray, M.; Barrera, M.; Fischer, R.; Che, M.; Liu, Y.; Zha, O.; Woods, D.; et al. Determination of Abraham model solute descriptors for 4-tert-butylbenzoic acid from experimental solubility data in organic mono-solvents. Phys. Chem. Liq. 2019, 57, 445–452. [Google Scholar] [CrossRef]
- ChemSpider Database, The Royal Society of Chemistry. Available online: http://www.chemspider.com/ (accessed on 14 May 2024).
- Sinha, S.; Yang, C.; Wu, E.; Acree, W.E., Jr. Abraham solvation parameter model: Examination of possible intramolecular hydrogen-bonding using calculated solute descriptors. Liquids 2022, 2, 131–146. [Google Scholar] [CrossRef]
- Shanmugam, N.; Zhou, A.; Motati, R.; Yao, E.; Kandi, T.; Longacre, L.; Benavides, D.; Motati, S.; Acree, W.E., Jr. Development of Abraham model correlations for dimethyl adipate from measured solubility data of nonelectrolyte organic compounds. Phys. Chem. Liq. 2023, 61, 328–339. [Google Scholar] [CrossRef]
- Longacre, L.; Wu, E.; Yang, C.; Zhang, M.; Sinha, S.; Varadharajan, A.; Acree, W.E., Jr. Development of Abraham model correlations for solute transfer into the tert-butyl acetate mono-solvent and updated equations for both ethyl acetate and butyl acetate. Liquids 2022, 2, 258–288. [Google Scholar] [CrossRef]
- Sedov, I.A.; Salikov, T.M.; Khaibrakhmanova, D.R.; Wadawadigi, A.; Zha, O.; Qian, E.; Hart, E.; Barrera, M.; Acree, W.E., Jr.; Abraham, M.H. Determination of Abraham model correlations for solute transfer into propyl acetate based on experimental activity coefficient and solubility data. J. Solut. Chem. 2018, 47, 634–653. [Google Scholar] [CrossRef]
- Sprunger, L.M.; Proctor, A.; Acree, W.E., Jr.; Abraham, M.H.; Benjelloun-Dakhama, N. Correlation and prediction of partition coefficient between the gas phase and water, and the solvents dry methyl acetate, dry and wet ethyl acetate, and dry and wet butyl acetate. Fluid Phase Equilib. 2008, 270, 30–44. [Google Scholar] [CrossRef]
| Chemical | Supplier | Purification Method | Purity (Mass Fraction) |
|---|---|---|---|
| Isobutyl acetate | TCI America Chemical Company, Portland, OR, USA | Stored over activated molecular sieves and distilled | 0.998 |
| o-Acetoacetanisidide | Acros Organics, Morris Plains, NJ, USA | Dried for two days at 333 K | 0.996 |
| Anthracene | Aldrich Chemical Company, Milwaukee, WI, USA | Recrystallized from anhydrous acetone | 0.997 |
| Benzoin | Aldrich Chemical Company | Recrystallized from anhydrous methanol | 0.997 |
| Diphenyl sulfone | Aldrich Chemical Company | Recrystallized from anhydrous methanol | 0.996 |
| 2-Ethylanthraquinone | Aldrich Chemical Company | Recrystallized from anhydrous methanol | 0.996 |
| Salicylamide | Aldrich Chemical Company | Recrystallized from anhydrous methanol | 0.997 |
| Thioxanthen-9-one | Aldrich Chemical Company | Recrystallized from anhydrous methanol | 0.997 |
| Xanthene | Aldrich Chemical Company | Recrystallized from anhydrous methanol | 0.996 |
| 4-tert-Butylbenzoic acid | TCI America Chemical Company | Dried for two days at 333 K | 0.998 |
| 3-Chlorobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.997 |
| 4-Chlorobenzoic acid | Acros Organics | Dried for two days at 333 K | 0.996 |
| 2-Chloro-5-nitrobenzoic acid | Acros Organics | Dried for two days at 333 K | 0.998 |
| 4-Chloro-3-nitrobenzoic acid | Acros Organics | Dried for two days at 333 K | 0.998 |
| 3,4-Dichlorobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| 2,3-Dimethoxybenzoic acid | Thermo Scientific, Ward Hill, MA, USA | Dried for two days at 333 K | 0.997 |
| 3,4-Dimethoxybenzoic acid | Across Organics | Dried for two days at 333 K | 0.998 |
| 3,5-Dimethoxybenzoic acid | Across Organics | Dried for two days at 333 K | 0.995 |
| 3,5-Dinitrobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.997 |
| 2-Methoxybenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| 4-Methoxybenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| 2-Methylbenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| 3-Methylbenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| 2-Methyl-3-nitrobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.997 |
| 3-Methyl-4-nitrobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.997 |
| 4-Methyl-3-nitrobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| 2-Naphthoxyacetic acid | Sigma-Aldrich Chemical Company, Milwaukee, WI, USA | Dried for two days at 333 K | 0.995 |
| 3-Nitrobenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.996 |
| 4-Nitrobenzoic acid | Acros Organics | Dried for two days at 333 K | 0.998 |
| 3,4,5-Trimethoxybenzoic acid | Aldrich Chemical Company | Dried for two days at 333 K | 0.998 |
| Toluene | Aldrich Chemical Company | None | 0.998, anhydrous |
| Sodium methoxide, 25 mass % solution in methanol | Aldrich Chemical Company | None | |
| 2-Propanol | Aldrich Chemical Company | None | 0.99 |
| Chemical | Analysis Wavelength | Molar Concentration Range |
|---|---|---|
| o-Acetoacetanisidide | 282 (nm) | 5.81 × 10−5 to 1.94 × 10−4 |
| Anthracene | 356 (nm) | 6.86 × 10−5 to 2.28 × 10−4 |
| Benzoin | 313 (nm) | 1.21 × 10−3 to 4.03 × 10−3 |
| Diphenyl sulfone | 267 (nm) | 2.61 × 10−4 to 8.70 × 10−4 |
| 2-Ethylanthraquinone | 325 (nm) | 1.25 × 10−4 to 4.17 × 10−4 |
| Salicylamide | 300 (nm) | 1.06 × 10−4 to 3.55 × 10−4 |
| Thioxanthen-9-one | 378 (nm) | 6.05 × 10−5 to 2.02 × 10−4 |
| Xanthene | 280 (nm) | 1.79 × 10−4 to 5.95 × 10−4 |
| 4-tert-Butylbenzoic acid | 275 (nm) | 2.81 × 10−4 to 9.37 × 10−4 |
| 3-Chlorobenzoic acid | 280 (nm) | 5.05 × 10−4 to 1.68 × 10−3 |
| 4-Chlorobenzoic acid | 272 (nm) | 4.63 × 10−4 to 1.54 × 10−3 |
| 2-Chloro-5-nitrobenzoic acid | 280 (nm) | 8.69 × 10−5 to 2.90 × 10−4 |
| 4-Chloro-3-nitrobenzoic acid | 292 (nm) | 3.55 × 10−4 to 1.18 × 10−3 |
| 3,4-Dichlorobenzoic acid | 280 (nm) | 4.66 × 10−4 to 1.55 × 10−3 |
| 2,3-Dimethoxybenzoic acid | 293 (nm) | 2.27 × 10−4 to 7.56 × 10−4 |
| 3,4-Dimethoxybenzoic acid | 286 (nm) | 9.25 × 10−5 to 3.08 × 10−4 |
| 3,5-Dimethoxybenzoic acid | 305 (nm) | 2.17 × 10−4 to 7.23 × 10−4 |
| 3,5-Dinitrobenzoic acid | 267 (nm) | 6.35 × 10−5 to 2.12 × 10−4 |
| 2-Methoxybenzoic acid | 295 (nm) | 1.69 × 10−4 to 5.63 × 10−4 |
| 4-Methoxybenzoic acid | 273 (nm) | 9.77 × 10−5 to 3.26 × 10−4 |
| 2-Methylbenzoic acid | 279 (nm) | 4.29 × 10−4 to 1.43 × 10−3 |
| 3-Methylbenzoic acid | 280 (nm) | 4.17 × 10−4 to 1.39 × 10−3 |
| 2-Methyl-3-nitrobenzoic acid | 290 (nm) | 3.36 × 10−4 to 1.12 × 10−3 |
| 3-Methyl-4-nitrobenzoic acid | 295 (nm) | 1.70 × 10−4 to 5.67 × 10−4 |
| 4-Methyl-3-nitrobenzoic acid | 295 (nm) | 3.29 × 10−4 to 1.10 × 10−3 |
| 2-Naphthoxyacetic acid | 284 (nm) | 1.35 × 10−4 to 6.74 × 10−4 |
| 3-Nitrobenzoic acid | 280 (nm) | 1.67 × 10−4 to 5.57 × 10−4 |
| 4-Nitrobenzoic acid | 272 (nm) | 4.75 × 10−5 to 1.58 × 10−4 |
| 3,4,5-Trimethoxybenzoic acid | 289 (nm) | 1.38 × 10−4 to 4.60 × 10−4 |
| Chemical Solute | Tmp,initial/K | Tmp,equilibrated/K | Tmp,literatuare/K |
|---|---|---|---|
| o-Acetoacetanisidide | 359.7 ± 0.5 | 359.4 ± 0.5 | 359.2 b 355.2–357.2 [33] |
| Anthracene | 490.7 ± 0.5 | 491.1 ± 0.4 | 488.9–491.3 [34] |
| Benzoin | 408.6 ± 0.4 | 408.3 ± 0.5 | 408.2 [34] |
| Diphenyl sulfone | 398.2 ± 0.4 | 398.0 ± 0.5 | 398.2 [34] |
| 2-Ethylanthraquinone | 383.8 ± 0.5 | 383.5 ± 0.5 | 382.9 [35] 384.2 [36] |
| Salicylamide | 414.1 ± 0.5 | 413.9 ± 0.4 | 411.9–414.9 [37] |
| Thioxanthen-9-one | 488.2 ± 0.5 | 488.0 ± 0.5 | 486.6–487.9 [34] |
| Xanthene | 374.4 ± 0.5 | 374.1 ± 0.5 | 373.3–374.6 [34] |
| 4-tert-Butylbenzoic acid | 439.3 ± 0.4 | 439.1 ± 0.5 | 440 [34] |
| 3-Chlorobenzoic acid | 429.6 ± 0.5 | 429.2 ± 0.5 | 427.4–429.9 [37] |
| 4-Chlorobenzoic acid | 512.5 ± 0.3 | 512.7 ± 0.4 | 512.3–513.5 [37] |
| 2-Chloro-5-nitrobenzoic acid | 440.1 ± 0.5 | 440.4 ± 0.5 | 437.2–438.2 [38] 438.2–439.2 [39] 437–441 b |
| 4-Chloro-3-nitrobenzoic acid | 456.3 ± 0.5 | 456.5 ± 0.4 | 453.2–455.2 [39] 453–456 b |
| 3,4-Dichlorobenzoic acid | 480.7 ± 0.5 | 480.3 ± 0.5 | 478–481 b |
| 2,3-Dimethoxybenzoic acid | 394.9 ± 0.5 | 395.3 ± 0.5 | 393.1 [40] 394–397 b |
| 3,4-Dimethoxybenzoic acid | 452.9 ± 0.5 | 452.7 ± 0.5 | 453.1 [37] |
| 3,5-Dimethoxybenzoic acid | 455.9 ± 0.5 | 455.6 ± 0.5 | 454–458 b |
| 3,5-Dinitrobenzoic acid | 481.2 ± 0.5 | 481.0 ± 0.5 | 479.2–481.7 [37] |
| 2-Methoxybenzoic acid | 375.1 ± 0.5 | 374.9 ± 0.4 | 374.7 [37] |
| 4-Methoxybenzoic acid | 456.3 ± 0.5 | 456.5 ± 0.5 | 453–455.8 [37] |
| 2-Methylbenzoic acid | 377.2 ± 0.5 | 376.9 ± 0.5 | 376.5–376.9 [37] |
| 3-Methylbenzoic acid | 382.4 ± 0.4 | 382.8 ± 0.4 | 381.9 [36] |
| 2-Methyl-3-nitrobenzoic acid | 455.9 ± 0.5 | 455.7 ± 0.5 | 456.6 [41] |
| 3-Methyl-4-nitrobenzoic acid | 489.7 ± 0.5 | 489.4 ± 0.5 | 489.1 [41] |
| 4-Methyl-3-nitrobenzoic acid | 459.6 ± 0.5 | 459.2 ± 0.5 | 459.8 [41] |
| 2-Naphthoxyacetic acid | 424.6 ± 0.5 | 424.1 ± 0.5 | 424.3 [42] |
| 3-Nitrobenzoic acid | 414.7 ± 0.4 | 414.5 ± 0.4 | 408.7–414.3 [37] |
| 4-Nitrobenzoic acid | 512.2 ± 0.5 | 511.9 ± 0.5 | 512.4 [37] |
| 3,4,5-Trimethoxybenzoic acid | 443.9 ± 0.5 | 444.2 ± 0.5 | 444.5 [43] |
| Chemical Name | XS,organic | Chemical Name | XS,organic |
|---|---|---|---|
| o-Acetoacetanisidide | 0.0464 | 3,4-Dimethoxybenzoic acid | 0.00461 |
| Anthracene | 0.00506 | 3,5-Dimethoxybenzoic acid | 0.00432 |
| Benzoin | 0.00882 | 3,5-Dinitrobenzoic acid | 0.0309 |
| Diphenyl sulfone | 0.0302 | 2-Methoxybenzoic acid | 0.0353 |
| 2-Ethylanthraquinone | 0.0341 | 4-Methoxybenzoic acid | 0.00789 |
| Salicylamide | 0.0500 | 2-Methylbenzoic acid | 0.1222 |
| Thioxanthen-9-one | 0.00306 | 3-Methylbenzoic acid | 0.1265 |
| Xanthene | 0.1057 | 2-Methyl-3-nitrobenzoic acid | 0.0247 |
| 4-tert-Butylbenzoic acid | 0.0750 | 3-Methyl-4-nitrobenzoic acid | 0.0111 |
| 3-Chlorobenzoic acid | 0.0541 | 4-Methyl-3-nitrobenzoic acid | 0.0183 |
| 4-Chlorobenzoic acid | 0.00825 | 2-Naphthoxyacetic acid | 0.0148 |
| 2-Chloro-5-nitrobenzoic acid | 0.0517 | 3-Nitrobenzoic acid | 0.0967 |
| 4-Chloro-3-nitrobenzoic acid | 0.0246 | 4-Nitrobenzoic acid | 0.00682 |
| 3,4-Dichlorobenzoic acid | 0.0133 | 3,4,5-Trimethoxybenzoic acid | 0.00825 |
| 2,3-Dimethoxybenzoic acid | 0.0206 |
| Solute | E | S | A | B | L | V |
|---|---|---|---|---|---|---|
| Hydrogen | 0.000 | 0.000 | 0.000 | 0.000 | −1.200 | 0.1086 |
| Nitrogen | 0.000 | 0.000 | 0.000 | 0.000 | −0.978 | 0.2222 |
| Carbon monoxide | 0.000 | 0.000 | 0.000 | 0.040 | −0.836 | 0.2220 |
| Carbon dioxide | 0.000 | 0.280 | 0.050 | 0.100 | 0.058 | 0.2809 |
| Isobutyl acetate | 0.520 | 0.570 | 0.000 | 0.470 | 3.161 | 1.0284 |
| Naphthalene | 1.340 | 0.920 | 0.000 | 0.200 | 5.161 | 1.0854 |
| Anthracene | 2.290 | 1.340 | 0.000 | 0.280 | 7.568 | 1.4544 |
| Xanthene | 1.502 | 1.070 | 0.000 | 0.230 | 7.153 | 1.4152 |
| Fluorene | 1.588 | 1.060 | 0.000 | 0.250 | 6.922 | 1.3565 |
| Benzoic acid | 0.730 | 0.900 | 0.590 | 0.400 | 4.657 | 0.9317 |
| 4-tert-Butylbenzoic acid | 0.730 | 1.111 | 0.551 | 0.443 | 6.547 | 1.4953 |
| 3-Chlorobenzoic acid | 0.840 | 0.950 | 0.630 | 0.320 | 5.197 | 1.0541 |
| 4-Chlorobenzoic acid | 0.840 | 1.020 | 0.630 | 0.270 | 4.947 | 1.0541 |
| 3,4-Dichlorobenzoic acid | 0.950 | 0.920 | 0.670 | 0.260 | 5.623 | 1.1766 |
| 2,3-Dimethoxybenzoic acid | 0.890 | 1.636 | 0.564 | 0.703 | 6.612 | 1.3309 |
| 3,4-Dimethoxybenzoic acid | 0.950 | 1.646 | 0.570 | 0.755 | 6.746 | 1.3309 |
| 3,5-Dimethoxybenzoic acid | 0.950 | 1.531 | 0.684 | 0.564 | 6.699 | 1.3309 |
| 3,4,5-Trimethoxybenzoic acid | 1.001 | 1.760 | 0.603 | 0.850 | 7.711 | 1.5309 |
| 2-Methoxybenzoic acid | 0.899 | 1.410 | 0.450 | 0.620 | 5.636 | 1.1313 |
| 4-Methoxybenzoic acid | 0.899 | 1.250 | 0.620 | 0.520 | 5.741 | 1.1313 |
| 2-Methylbenzoic acid | 0.730 | 0.840 | 0.420 | 0.440 | 4.677 | 1.0726 |
| 3-Methylbenzoic acid | 0.730 | 0.890 | 0.600 | 0.400 | 4.819 | 1.0726 |
| 2-Methyl-3-nitrobenzoic acid | 1.040 | 1.396 | 0.541 | 0.532 | 6.332 | 1.2468 |
| 3-Methyl-4-nitrobenzoic acid | 1.040 | 1.336 | 0.525 | 0.500 | 6.266 | 1.2468 |
| 4-Methyl-3-nitrobenzoic acid | 1.040 | 1.461 | 0.659 | 0.521 | 6.434 | 1.2468 |
| 3-Nitrobenzoic acid | 0.990 | 1.180 | 0.730 | 0.520 | 5.601 | 1.1059 |
| 4-Nitrobenzoic acid | 0.990 | 1.520 | 0.680 | 0.400 | 5.770 | 1.1059 |
| 3,5-Dinitrobenzoic acid | 1.250 | 1.630 | 0.700 | 0.590 | 6.984 | 1.2801 |
| 2-Chloro-5-nitrobenzoic acid | 1.250 | 1.400 | 0.670 | 0.460 | 6.513 | 1.2283 |
| 4-Chloro-3-nitrobenzoic acid | 1.250 | 1.470 | 0.700 | 0.440 | 6.685 | 1.2283 |
| Isophthalic acid | 1.100 | 1.360 | 1.055 | 0.585 | 6.144 | 1.1470 |
| 2-Naphthoxyacetic acid | 1.610 | 1.940 | 0.690 | 0.764 | 8.553 | 1.5003 |
| 2-Ethylanthraquinone | 1.410 | 1.545 | 0.000 | 0.557 | 8.781 | 1.8106 |
| Thioxanthen-9-one | 1.940 | 1.441 | 0.000 | 0.557 | 8.436 | 1.5357 |
| Diphenylsulfone | 1.570 | 2.150 | 0.000 | 0.700 | 8.902 | 1.6051 |
| Acetylsalicylic acid | 0.781 | 1.690 | 0.710 | 0.670 | 6.279 | 1.2879 |
| o-Acetoacetanisidide | 1.190 | 2.333 | 0.264 | 1.025 | 8.563 | 1.6108 |
| Salicylamide | 1.160 | 1.650 | 0.630 | 0.480 | 5.910 | 1.0315 |
| Benzoin | 1.587 | 2.115 | 0.196 | 0.847 | 9.159 | 1.6804 |
| Forchlorfenuron | 1.870 | 1.928 | 1.186 | 0.702 | 9.986 | 1.7617 |
| Metronidazole benzoate | 1.430 | 2.452 | 0.000 | 1.077 | 10.171 | 1.9563 |
| Iminostilbene | 2.000 | 1.801 | 0.246 | 0.401 | 9.013 | 1.5542 |
| Isovanillin | 1.040 | 1.477 | 0.308 | 0.681 | 5.868 | 1.1313 |
| Lansoprazole | 2.300 | 2.652 | 0.607 | 1.514 | 13.187 | 2.3700 |
| 2,2′-bis(2-Hydroxyethoxy)-1,1′-binaphthalene | 3.610 | 3.377 | 0.491 | 1.633 | 17.398 | 2.8606 |
| Lovastatin | 1.230 | 2.730 | 0.310 | 1.760 | 15.459 | 3.2853 |
| 18β-Glycyrrhetinic acid | 1.800 | 2.809 | 0.823 | 2.220 | 18.246 | 3.8984 |
| Etodolac | 1.610 | 1.860 | 0.455 | 1.170 | 11.019 | 2.2390 |
| Solute | log (CS,organic/CS,gas) | log (CS,organic/CS,water) | log CS,gas | log CS,water |
|---|---|---|---|---|
| Hydrogen | −1.073 a | 0.647 b | ||
| Nitrogen | −0.761 a | 1.039 b | ||
| Carbon monoxide | −0.628 a | 0.992 b | ||
| Carbon dioxide | 0.660 a | 0.740 b | ||
| Isobutyl acetate | 3.880 a | 2.161 b | ||
| Naphthalene | 5.709 | 3.979 | −5.35 | −3.62 |
| Anthracene | 8.036 | 5.006 | −9.46 | −6.43 |
| Xanthene | 7.601 | 5.101 | −7.71 | −5.21 |
| Fluorene | 7.280 | 4.830 | −7.45 | −5.00 |
| Benzoic acid | 6.706 | 1.566 | −6.69 | −1.55 |
| 4-tert-Butylbenzoic acid | 8.862 | 3.638 | −9.123 | −3.899 |
| 3-Chlorobenzoic acid | 7.408 | 2.258 | −7.80 | −2.65 |
| 4-Chlorobenzoic acid | 7.149 | 2.349 | −8.36 | −3.56 |
| 3,4-Dichlorobenzoic acid | 7.715 | 2.975 | −8.72 | −3.98 |
| 2,3-Dimethoxybenzoic acid | 9.432 | 1.347 | −10.245 | −2.16 |
| 3,4-Dimethoxybenzoic acid | 9.478 | 1.031 | −10.942 | −2.495 |
| 3,5-Dimethoxybenzoic acid | 9.678 | 1.984 | −11.170 | −3.476 |
| 3,4,5-Trimethoxybenzoic acid | 10.593 | 1.338 | −11.805 | −2.55 |
| 2-Methoxybenzoic acid | 7.776 | 0.976 | −8.354 | −1.554 |
| 4-Methoxybenzoic acid | 8.270 | 1.570 | −9.50 | −2.80 |
| 2-Methylbenzoic acid | 6.325 | 2.025 | −6.36 | −2.06 |
| 3-Methylbenzoic acid | 7.100 | 2.120 | −7.12 | −2.14 |
| 2-Methyl-3-nitrobenzoic acid | 8.713 | 1.976 | −9.447 | −2.71 |
| 3-Methyl-4-nitrobenzoic acid | 8.510 | 2.146 | −9.594 | −3.23 |
| 4-Methyl-3-nitrobenzoic acid | 9.104 | 1.819 | −9.97 | −2.685 |
| 3-Nitrobenzoic acid | 8.473 | 1.543 | −8.61 | −1.68 |
| 4-Nitrobenzoic acid | 8.587 | 1.687 | −9.88 | −2.98 |
| 3,5-Dinitrobenzoic acid | 10.080 | 1.780 | −10.717 | −2.417 |
| 2-Chloro-5-nitrobenzoic acid | 9.125 | 2.175 | −9.538 | −2.588 |
| 4-Chloro-3-nitrobenzoic acid | 9.474 | 2.264 | −10.21 | −3.00 |
| Isophthalic acid | 9.457 | 0.484 | −12.083 | −3.11 |
| 2-Naphthoxyacetic acid | 11.584 | 1.665 | −12.543 | −2.624 |
| 2-Ethylanthraquinone | 9.744 | 4.930 | −10.344 | −5.530 |
| Thioxanthen-9-one | 8.965 | 3.897 | −10.608 | −5.54 |
| Diphenyl sulfone | 10.380 | 2.990 | −11.03 | −3.64 |
| Acetylsalicylic acid | 9.411 | 0.911 | −10.18 | −1.68 |
| o-Acetoacetanisidide | 11.307 | 1.073 | −11.775 | −1.541 |
| Salicylamide | 9.011 | 1.326 | −9.439 | −1.754 |
| Benzoin | 11.218 | 2.487 | −12.401 | −3.67 |
| Forchlorfenuron | 14.174 | 2.755 | −15.369 | −3.95 |
| Metronidazole benzoate | 12.143 | 2.521 | −12.697 | −3.075 |
| Iminostilbene | 10.670 | 4.389 | −11.592 | −5.311 |
| Isovanillin | 7.661 | 0.823 | −8.390 | −1.552 |
| Lansoprazole | 16.370 | 1.677 | −18.364 | −3.671 |
| 2,2′-bis(2-Hydroxyethoxy)-2,2′-binaphthalene | 20.307 | 3.298 | −21.965 | −4.956 |
| Lovastatin | 18.159 | 4.177 | −19.597 | −5.585 |
| Simvastatin | 17.932 | 4.282 | −18.68 | −5.03 |
| 18β-Glycyrrhetinic acid | 22.179 | 4.349 | −23.917 | −6.087 |
| Etodolac | 13.451 | 3.267 | −13.790 | −3.606 |
| Log (P or CS,org/CS,water) | cp | ep | sp | ap | bp | vp |
| Methyl acetate | 0.351 | 0.223 | −0.150 | −1.035 | −4.527 | 3.972 |
| Ethyl acetate | 0.328 | 0.314 | −0.348 | −0.847 | −4.899 | 4.142 |
| Propyl acetate | 0.362 | 0.280 | −0.390 | −0.975 | −4.928 | 4.183 |
| Isopropyl acetate | 0.307 | 0.314 | −0.481 | −0.952 | −4.779 | 4.159 |
| Butyl acetate | 0.289 | 0.336 | −0.501 | −0.913 | −4.964 | 4.262 |
| Isobutyl acetate | 0.234 | 0.351 | −0.471 | −1.050 | −4.982 | 4.212 |
| tert-Butyl acetate | 0.456 | 0.324 | −0.661 | −1.068 | −4.680 | 4.101 |
| Pentyl acetate | 0.182 | 0.261 | −0.474 | −1.017 | −4.952 | 4.388 |
| Methyl butyrate | 0.238 | 0.368 | −0.538 | −1.031 | −4.623 | 4.253 |
| Isopropyl myristate | −0.605 | 0.930 | −1.153 | −1.682 | −4.093 | 4.249 |
| Dimethyl adipate | 0.128 | 0.546 | −0.404 | −1.001 | −4.481 | 3.987 |
| Log (K or CS,org/CS,gas) | ck | ek | sk | ak | bk | lk |
| Methyl acetate | 0.134 | −0.477 | 1.749 | 2.678 | 0.000 | 0.876 |
| Ethyl acetate | 0.171 | −0.403 | 1.428 | 2.726 | 0.000 | 0.914 |
| Propyl acetate | 0.246 | −0.346 | 1.318 | 2.537 | 0.000 | 0.916 |
| Isopropyl acetate | 0.233 | −0.495 | 1.324 | 2.550 | 0.000 | 0.928 |
| Butyl acetate | 0.154 | −0.439 | 1.223 | 2.586 | 0.000 | 0.953 |
| Isobutyl acetate | 0.173 | −0.353 | 1.183 | 2.463 | 0.000 | 0.936 |
| tert-Butyl acetate | 0.178 | −0.444 | 1.045 | 2.522 | 0.000 | 0.964 |
| Pentyl acetate | 0.154 | −0.424 | 1.172 | 2.506 | 0.000 | 0.962 |
| Methyl butyrate | 0.201 | −0.502 | 1.290 | 2.469 | 0.000 | 0.958 |
| Dimethyl adipate | 0.051 | −0.248 | 1.579 | 2.513 | 0.000 | 0.877 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Motati, R.; Kandi, T.; Francis, J.; Chen, J.; Yao, E.; Motati, S.; Chen, A.; Kumarandurai, D.; Shanmugam, N.; Acree, W.E., Jr. Abraham General Solvation Parameter Model: Predictive Expressions for Solute Transfer into Isobutyl Acetate. Liquids 2024, 4, 470-484. https://doi.org/10.3390/liquids4030026
Motati R, Kandi T, Francis J, Chen J, Yao E, Motati S, Chen A, Kumarandurai D, Shanmugam N, Acree WE Jr. Abraham General Solvation Parameter Model: Predictive Expressions for Solute Transfer into Isobutyl Acetate. Liquids. 2024; 4(3):470-484. https://doi.org/10.3390/liquids4030026
Chicago/Turabian StyleMotati, Ramya, Trisha Kandi, Jilawan Francis, Jocelyn Chen, Emily Yao, Saikiran Motati, Audrey Chen, Dhishithaa Kumarandurai, Nikita Shanmugam, and William E. Acree, Jr. 2024. "Abraham General Solvation Parameter Model: Predictive Expressions for Solute Transfer into Isobutyl Acetate" Liquids 4, no. 3: 470-484. https://doi.org/10.3390/liquids4030026
APA StyleMotati, R., Kandi, T., Francis, J., Chen, J., Yao, E., Motati, S., Chen, A., Kumarandurai, D., Shanmugam, N., & Acree, W. E., Jr. (2024). Abraham General Solvation Parameter Model: Predictive Expressions for Solute Transfer into Isobutyl Acetate. Liquids, 4(3), 470-484. https://doi.org/10.3390/liquids4030026







