Thermodynamic Analysis of the Solubility of Sulfadiazine in (Acetonitrile 1-Propanol) Cosolvent Mixtures from 278.15 K to 318.15 K
Abstract
1. Introduction
2. Materials and Methods
2.1. Reagents
2.2. Preparation of Solvent Mixtures
2.3. Solubility Determination
2.4. Calorimetric Study
3. Results and Discussion
3.1. Experimental Mole Fraction Solubility ()
3.2. Ideal Solubility and Activity Coefficients
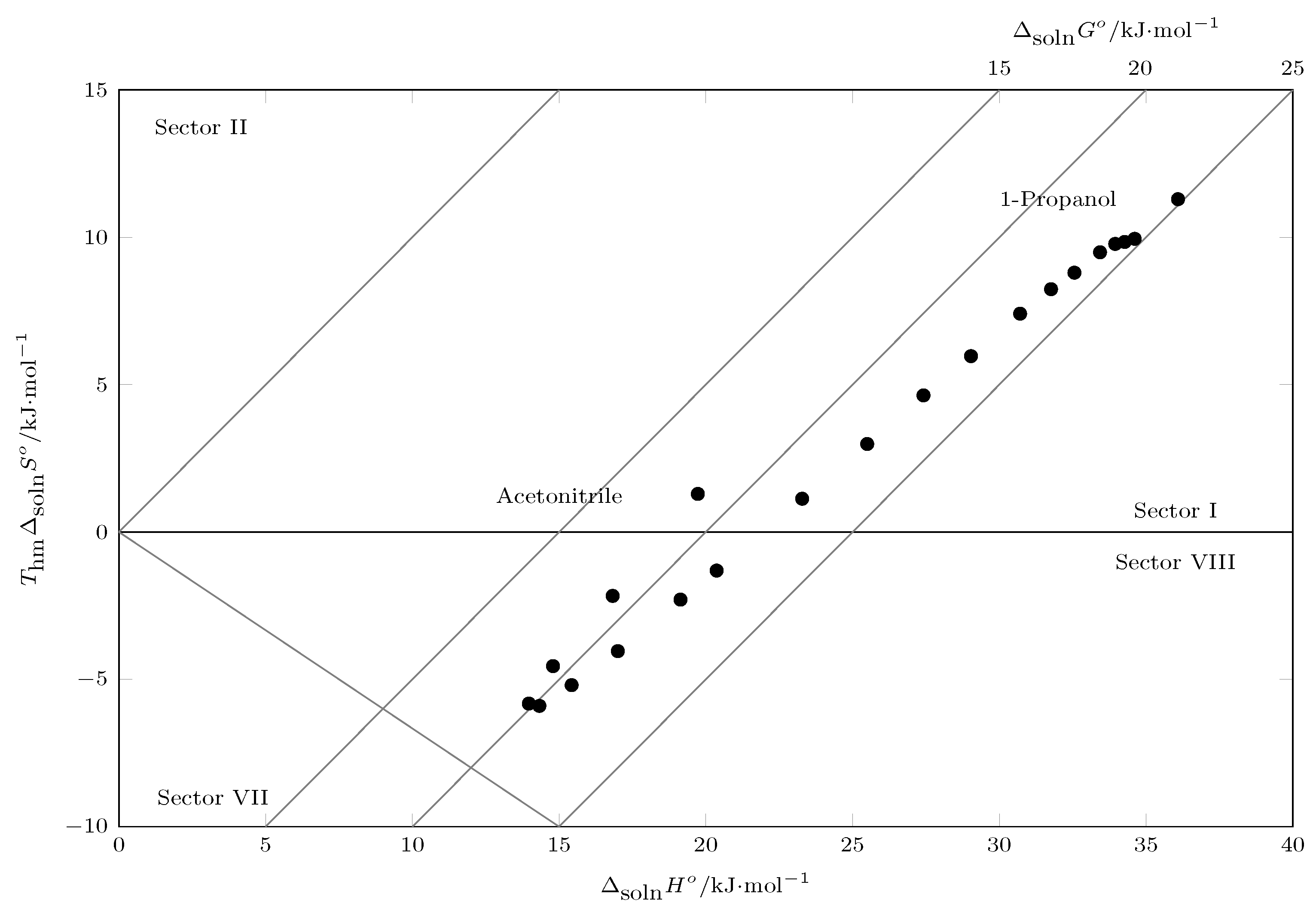
3.3. Thermodynamic Functions of Solution
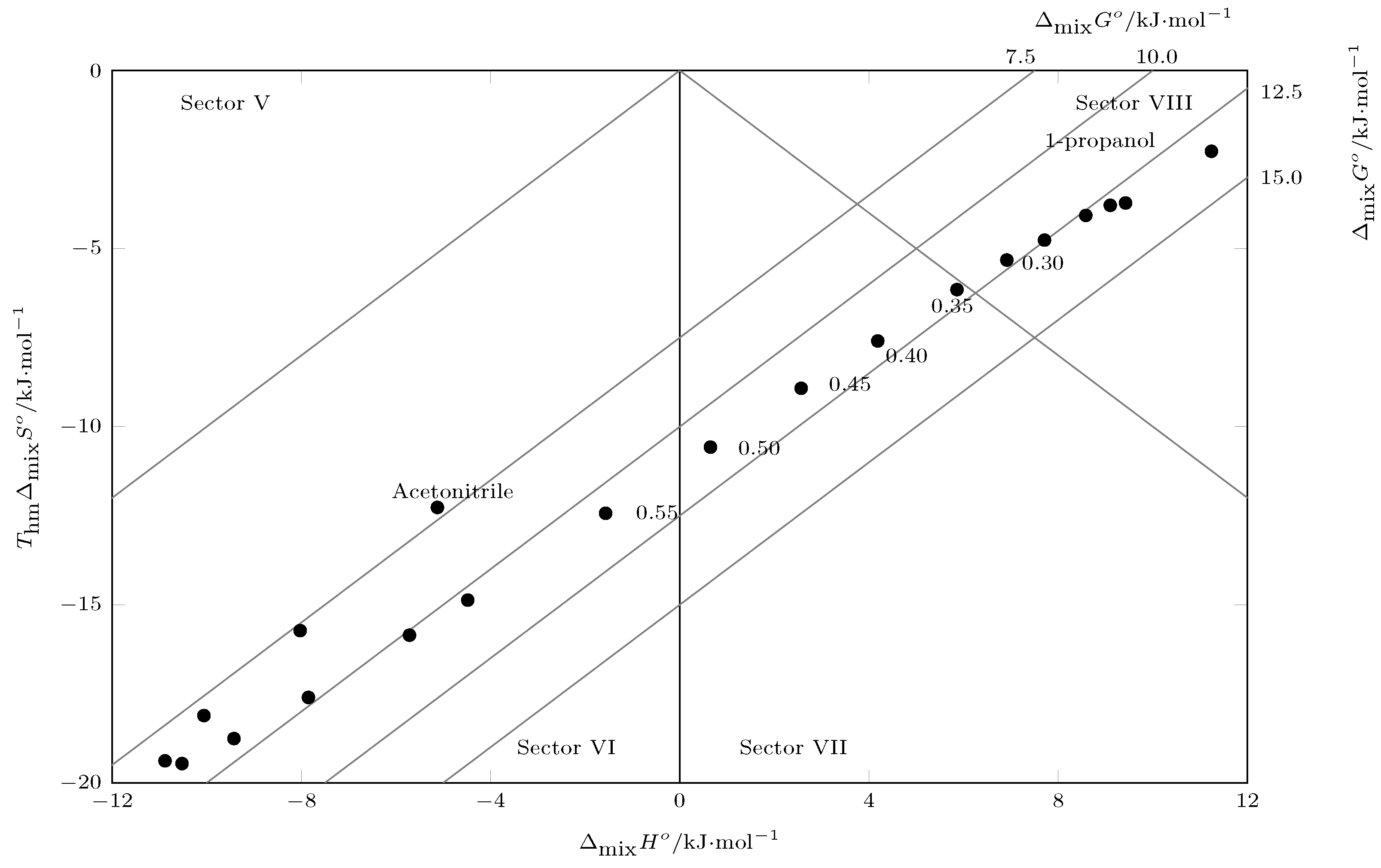
3.4. Thermodynamic Functions of Mixing
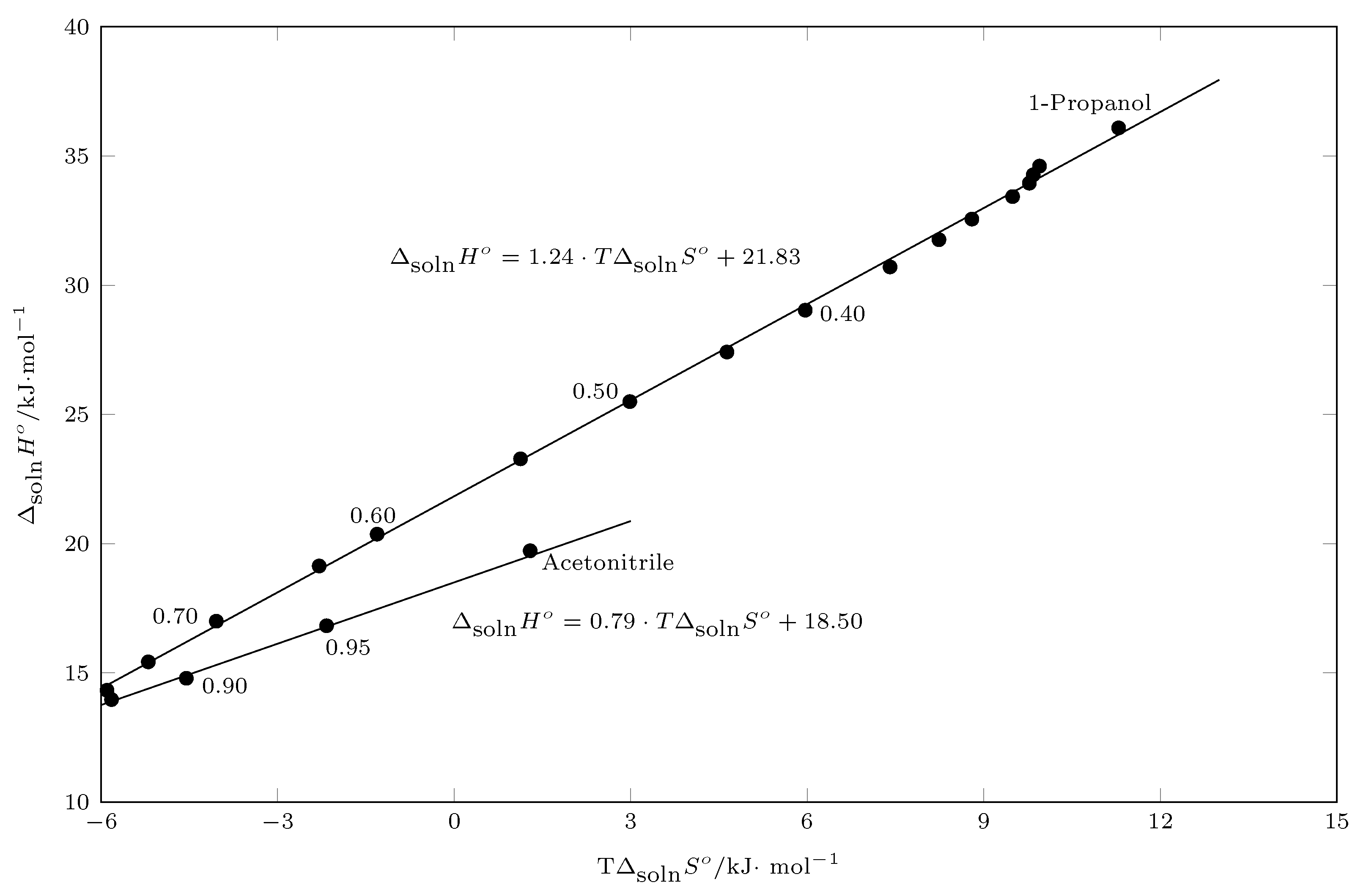
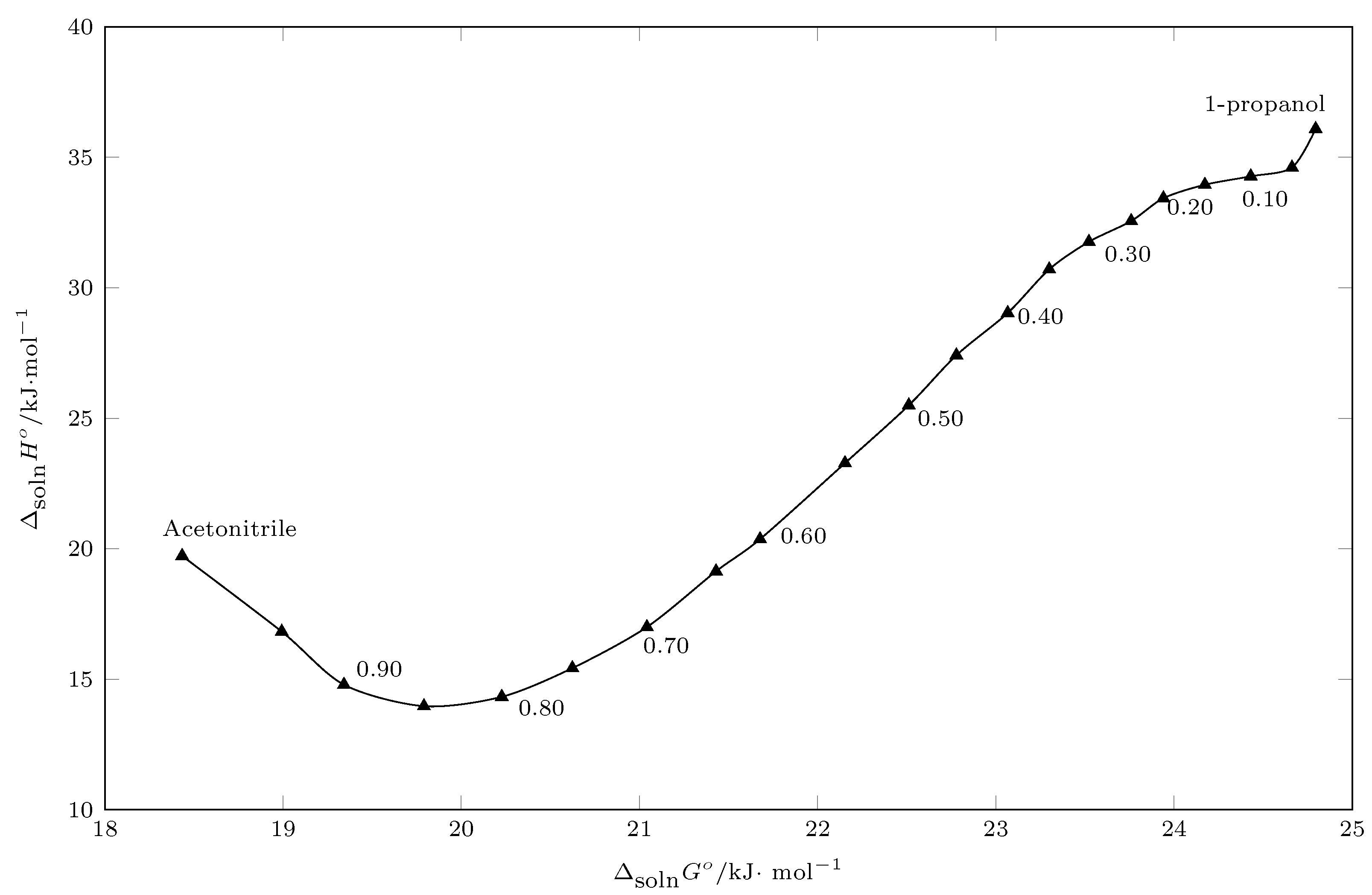
3.5. Enthalpy–Entropy Compensation Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feng, Y.; Wu, D.; Liao, C.; Deng, Y.; Zhang, T.; Shih, K. Red mud powders as low-cost and efficient catalysts for persulfate activation: Pathways and reusability of mineralizing sulfadiazine. Sep. Purif. Technol. 2016, 167, 136–145. [Google Scholar] [CrossRef]
- Delgado, D.R.; Bahamón-Hernandez, O.; Cerquera, N.E.; Ortiz, C.P.; Martínez, F.; Rahimpour, E.; Jouyban, A.; Acree, W.E. Solubility of sulfadiazine in (acetonitrile + methanol) mixtures: Determination, correlation, dissolution thermodynamics and preferential solvation. J. Mol. Liq. 2021, 322, 114979. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Solution thermodynamics of sulfadiazine in some ethanol+water mixtures. J. Mol. Liq. 2013, 187, 99–105. [Google Scholar] [CrossRef]
- Jiménez, D.M.; Cárdenas, Z.J.; Delgado, D.R.; Peña, M.A.; Martínez, F. Solubility temperature dependence and preferential solvation of sulfadiazine in 1,4-dioxane+water co-solvent mixtures. Fluid Phase Equilibria 2015, 397, 26–36. [Google Scholar] [CrossRef]
- Osorio, I.P.; Martínez, F.; Peña, M.A.; Jouyban, A.; Acree, W.E. Solubility, dissolution thermodynamics and preferential solvation of sulfadiazine in (N-methyl-2-pyrrolidone+water) mixtures. J. Mol. Liq. 2021, 330, 115693. [Google Scholar] [CrossRef]
- Bustamante, P.; Escalera, B.; Martin, A.; Selles, E. A modification of the extended Hildebrand approach to predict the solubility of structurally related drugs in solvent mixtures. J. Pharm. Pharmacol. 2011, 45, 253–257. [Google Scholar] [CrossRef] [PubMed]
- Elworthy, P.H.; Worthington, H.E.C. The solubility of sulphadiazine in water-dimethylformamide mixtures. J. Pharm. Pharmacol. 2011, 20, 830–835. [Google Scholar] [CrossRef]
- Zhang, C.L.; Wang, F.A.; Wang, Y. Solubilities of sulfadiazine, sulfamethazine, sulfadimethoxine, sulfamethoxydiazine, sulfamonomethoxine, sulfamethoxazole, and sulfachloropyrazine in water from (298.15 to 333.15) K. J. Chem. Eng. Data 2007, 52, 1563–1566. [Google Scholar] [CrossRef]
- Yalkowsky, S.H. Solubility and Solubilization in Aqueous Media; American Chemical Society: Washington, DC, USA, 1999. [Google Scholar]
- Yalkowsky, S.H.; Wu, M. Estimation of the ideal solubility (crystal-liquid fugacity ratio) of organic compounds. J. Pharm. Sci. 2010, 99, 1100–1106. [Google Scholar] [CrossRef]
- Mauger, J.W.; Paruta, A.N.; Gerraughty, R.J. Solubilities of sulfadiazine, sulfisomidine, and sulfadimethoxine in several normal alcohols. J. Pharm. Sci. 1972, 61, 94–97. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Preferential solvation of sulfadiazine, sulfamerazine and sulfamethazine in ethanol+water solvent mixtures according to the IKBI method. J. Mol. Liq. 2014, 193, 152–159. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Solubility and preferential solvation of sulfadiazine in methanol+water mixtures at several temperatures. Fluid Phase Equilibria 2014, 379, 128–138. [Google Scholar] [CrossRef]
- Muñoz, M.M.; Delgado, D.R.; Peña, M.A.; Jouyban, A.; Martínez, F. Solubility and preferential solvation of sulfadiazine, sulfamerazine and sulfamethazine in propylene glycol+water mixtures at 298.15K. J. Mol. Liq. 2015, 204, 132–136. [Google Scholar] [CrossRef]
- Cruz-González, A.M.; Vargas-Santana, M.S.; Ortiz, C.P.; Cerquera, N.E.; Delgado, D.R.; Martínez, F.; Jouyban, A.; Acree, W.E., Jr. Solubility of sulfadiazine in (ethylene glycol+water) mixtures: Measurement, correlation, thermodynamics and preferential solvation. J. Mol. Liq. 2021, 323, 115058. [Google Scholar] [CrossRef]
- Blanco-Márquez, J.H.; Quigua-Medina, Y.A.; García-Murillo, J.D.; Castro-Camacho, J.K.; Ortiz, C.P.; Cerquera, N.E.; Delgado, D.R. Thermodynamic analysis and applications of the Abraham solvation parameter model in the study of the solubility of some sulfonamides. Rev. Colomb. Cienc. Químico-Farm. 2020, 49, 234–255. [Google Scholar] [CrossRef]
- Delgado, D.R.; Peña, M.; Martínez, F. Extended Hildebrand solubility approach applied to some structurally related sulfonamides in ethanol + water mixtures. Rev. Colomb. Química 2016, 45, 34–43. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Preferential solvation of some structurally related sulfonamides in 1-propanol + water co-solvent mixtures. Phys. Chem. Liq. 2015, 53, 293–306. [Google Scholar] [CrossRef]
- Barton, A.F.M. Handbook of Solubility Parameters and Other Cohesion Parameters, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1991. [Google Scholar]
- Witschi, C.; Doelker, E. Residual solvents in pharmaceutical products: Acceptable limits, influences on physicochemical properties, analytical methods and documented values. Eur. J. Pharm. Biopharm. 1997, 43, 215–242. [Google Scholar] [CrossRef]
- Higuchi, T.; Connors, K. Advances in Analytical Chemistry and Instrumentation; Interscience Publishers, Inc.: New York, NY, USA, 1965. [Google Scholar]
- Dittert, L.W.; Higuchi, T.; Reese, D.R. Phase solubility technique in studying the formation of complex salts of triamterene. J. Pharm. Sci. 1964, 53, 1325–1328. [Google Scholar] [CrossRef]
- Mader, W.J.; Higuchi, T. Phase solubility analysis. Crit. Rev. Anal. Chem. 1970, 1, 193–215. [Google Scholar] [CrossRef]
- Crowley, J.; Teague, G., Jr.; Lowe, J., Jr. A three-dimensional approach to solubility. Chem. Mater. Sci. 1966, 38, 269–280. [Google Scholar]
- Taft, R.W.; Kamlet, M.J. The solvatochromic comparison method. 2. The .alpha.-scale of solvent hydrogen-bond donor (HBD) acidities. J. Am. Chem. Soc. 1976, 98, 2886–2894. [Google Scholar] [CrossRef]
- Hilfiker, R.; Blatter, F.; Raumer, M.V. Relevance of solid-state properties for pharmaceutical products. In Polymorphism; John Wiley & Sons, Ltd.: New York, NY, USA, 2006; Chapter 1; pp. 1–19. [Google Scholar] [CrossRef]
- Martínez, F.; Ávila, C.M.; Gómez, A. Thermodynamic study of the solubility of some sulfonamides in cyclohexane. J. Braz. Chem. Soc. 2003, 14, 803–808. [Google Scholar] [CrossRef]
- Martin, A.; Wu, P.; Velasquez, T. Extended Hildebrand solubility approach: Sulfonamides in binary and ternary solvents. J. Pharm. Sci. 1985, 74, 277–282. [Google Scholar] [CrossRef] [PubMed]
- Sunwoo, C.; Eisen, H. Solubility parameter of selected sulfonamides. J. Pharm. Sci. 1971, 60, 238–244. [Google Scholar] [CrossRef]
- Kofler, L.; Sitte, H.Z. Zur Schmelzpunktbestimmung von Substanzen, die unter Zersetzung schmelzen. Monatshefte Chem. 1950, 81, 619–626. [Google Scholar] [CrossRef]
- Ortiz, C.P.; Cardenas-Torres, R.E.; Martínez, F.; Delgado, D.R. Solubility of sulfamethazine in the binary mixture of acetonitrile + methanol from 278.15 to 318.15 K: Measurement, dissolution thermodynamics, preferential solvation, and correlation. Molecules 2021, 26, 588. [Google Scholar] [CrossRef]
- Hildebrand, J.H.; Prausnitz, J.M.; Scott, R.L. Regular and Related Solutions: The Solubility of Gases, Liquids, and Solids; Van Nostrand Reinhold: New York, NY, USA, 1970. [Google Scholar]
- Neau, S.H.; Flynn, G.L. Solid and liquid heat capacities of n-Alkyl para-aminobenzoates near the melting point. Pharm. Res. 1990, 7, 157–1162. [Google Scholar] [CrossRef]
- Neau, S.H.; Bhandarkar, S.V.; Hellmuth, E.W. Differential molar heat capacities to test ideal solubility estimations. Pharm. Res. 1997, 14, 601–605. [Google Scholar] [CrossRef]
- Opperhuizen, A.; Gobas, F.A.P.C.; Van der Steen, J.M.D.; Hutzinger, O. Aqueous solubility of polychlorinated biphenyls related to molecular structure. Environ. Sci. Technol. 1988, 22, 638–646. [Google Scholar] [CrossRef]
- Holguín, A.R.; Rodríguez, G.A.; Cristancho, D.M.; Delgado, D.R.; Martínez, F. Solution thermodynamics of indomethacin in propylene glycol+water mixtures. Fluid Phase Equilibria 2012, 314, 134–139. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Solubility and solution thermodynamics of sulfamerazine and sulfamethazine in some ethanol+water mixtures. Fluid Phase Equilibria 2013, 360, 88–96. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Solubility and solution thermodynamics of some sulfonamides in 1-propanol + water mixtures. J. Solut. Chem. 2014, 43, 836–852. [Google Scholar] [CrossRef]
- Delgado, D.R.; Almanza, O.A.; Martínez, F.; Peña, M.A.; Jouyban, A.; Acree, W.E. Solution thermodynamics and preferential solvation of sulfamethazine in (methanol+water) mixtures. J. Chem. Thermodyn. 2016, 97, 264–276. [Google Scholar] [CrossRef]
- Delgado, D.R.; Martínez, F. Solution thermodynamics and preferential solvation of sulfamerazine in methanol + water mixtures. J. Solut. Chem. 2015, 44, 360–377. [Google Scholar] [CrossRef]
- Krug, R.R.; Hunter, W.G.; Grieger, R.A. Enthalpy–entropy compensation. 1. Some fundamental statistical problems associated with the analysis of van’t Hoff and Arrhenius data. J. Phys. Chem. 1976, 80, 2335–2341. [Google Scholar] [CrossRef]
- Krug, R.R.; Hunter, W.G.; Grieger, R.A. Enthalpy–entropy compensation. 2. Separation of the chemical from the statistical effect. J. Phys. Chem. 1976, 80, 2341–2351. [Google Scholar] [CrossRef]
- Nagasaka, M.; Yuzawa, H.; Kosugi, N. Microheterogeneity in aqueous acetonitrile solution probed by Soft X-ray absorption spectroscopy. J. Phys. Chem. B 2020, 124, 1259–1265. [Google Scholar] [CrossRef]
- Perlovich, G.L.; Tkachev, V.V.; Strakhova, N.N.; Kazachenko, V.P.; Volkova, T.V.; Surov, O.V.; Schaper, K.; Raevsky, O.A. Thermodynamic and structural aspects of sulfonamide crystals and solutions. J. Pharm. Sci. 2009, 98, 4738–4755. [Google Scholar] [CrossRef]
- Perlovich, G.L.; Strakhova, N.N.; Kazachenko, V.P.; Volkova, T.V.; Tkachev, V.V.; Schaper, K.J.; Raevsky, O.A. Sulfonamides as a subject to study molecular interactions in crystals and solutions: Sublimation, solubility, solvation, distribution and crystal structure. Int. J. Pharm. 2008, 349, 300–313. [Google Scholar] [CrossRef]
- Torres-Cardozo, A.; Cerquera, N.E.; Ortiz, C.P.; Osorio-Gallego, J.; Cardenas-Torres, R.E.; Angarita-Reina, F.; Martinez, F.; Delgado, D.R. Thermodynamic analysis of the solubility of progesterone in 1-octanol+ethanol cosolvent mixtures at different temperatures. Alex. Eng. J. 2022, in press. [Google Scholar] [CrossRef]
- Baracaldo-Santamaría, D.; Calderon-Ospina, C.A.; Ortiz, C.P.; Cardenas-Torres, R.E.; Martinez, F.; Delgado, D.R. Thermodynamic analysis of the solubility of isoniazid in (PEG 200 + water) cosolvent mixtures from 278.15 K to 318.15 K. Int. J. Mol. Sci. 2022, 23, 10190. [Google Scholar] [CrossRef] [PubMed]
- Ryde, U. A fundamental view of enthalpy–entropy compensation. Med. Chem. Commun. 2014, 5, 1324–1336. [Google Scholar] [CrossRef]
- Sharp, K. Entropy–enthalpy compensation: Fact or artifact? Protein Sci. 2001, 10, 661–667. [Google Scholar] [CrossRef] [PubMed]
- Bustamante, P.; Romero, S.; Peña, A.; Escalera, B.; Reillo, A. Enthalpy–entropy compensation for the solubility of drugs in solvent mixtures: Paracetamol, acetanilide, and nalidixic acid in dioxane–water. J. Pharm. Sci. 1998, 87, 1590–1596. [Google Scholar] [CrossRef]
- Peña, M.; Escalera, B.; Reíllo, A.; Sánchez, A.; Bustamante, P. Thermodynamics of cosolvent action: Phenacetin, salicylic acid and probenecid. J. Pharm. Sci. 2009, 98, 1129–1135. [Google Scholar] [CrossRef]
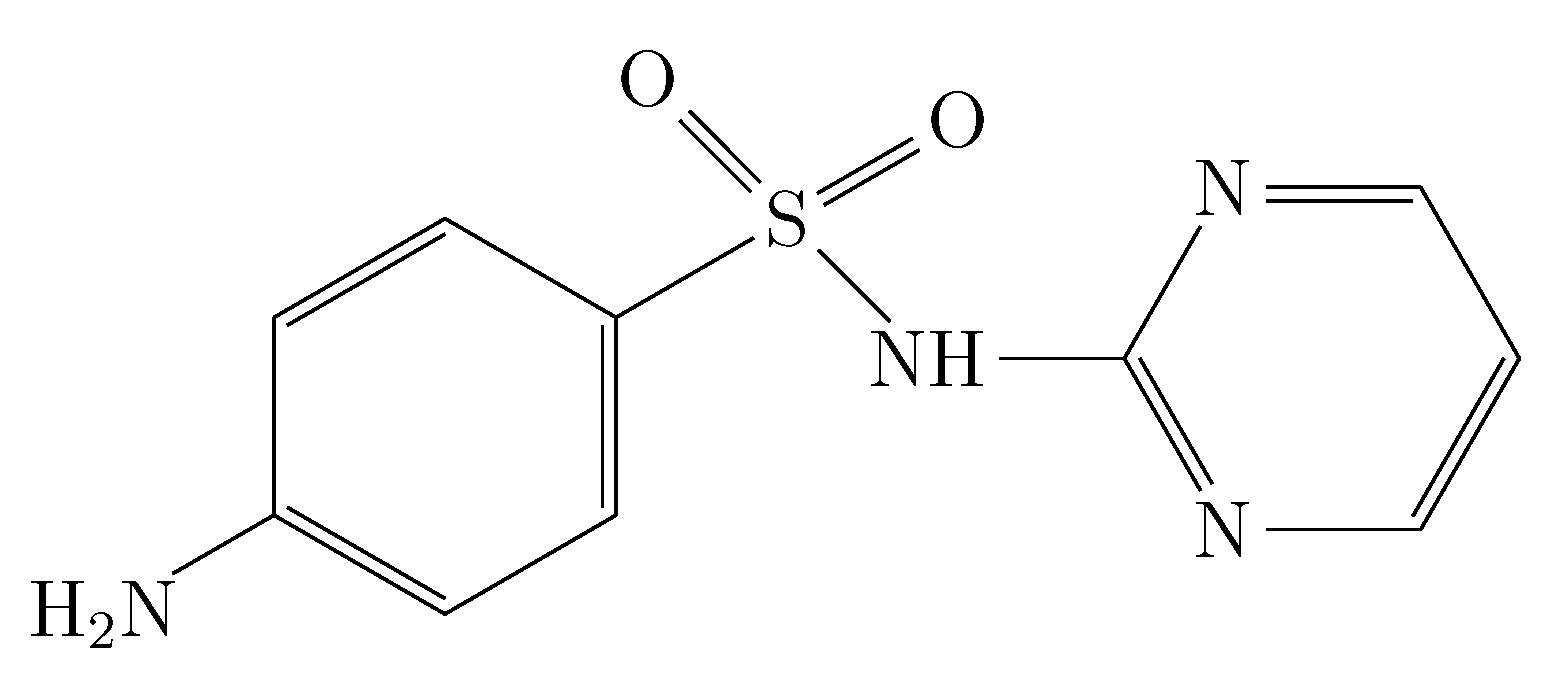

| Chemical Name | CAS | Source | Purity in Mass Fraction | Analytic Technique |
|---|---|---|---|---|
| Sufadiazine | 57-83-0 | Sigma-Aldrich, Burlington, MA, USA | >0.990 | HPLC |
| Acetonitrile | 75-05-8 | Merck Millipore, Burlington, MA, USA | 0.998 | GC |
| 1-Propanol | 71-23-8 | Merck Millipore, Burlington, MA, USA | 0.998 | GC |
| Ethanol | 64-17-5 | Merck Millipore, Burlington, MA, USA | 0.998 | GC |
| Temperature/K | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 278.15 | 283.15 | 288.15 | 293.15 | 298.15 | 303.15 | 308.15 | 313.15 | 318.15 | |
| 0.00 | 0.152 | 0.210 | 0.275 | 0.388 | 0.471 | 0.587 | 0.721 | 0.913 | 1.097 |
| 0.05 | 0.179 | 0.228 | 0.288 | 0.394 | 0.490 | 0.588 | 0.740 | 0.938 | 1.187 |
| 0.10 | 0.203 | 0.254 | 0.326 | 0.407 | 0.518 | 0.650 | 0.821 | 1.038 | 1.303 |
| 0.15 | 0.226 | 0.285 | 0.362 | 0.455 | 0.577 | 0.720 | 0.907 | 1.140 | 1.431 |
| 0.20 | 0.250 | 0.317 | 0.401 | 0.503 | 0.635 | 0.789 | 0.990 | 1.241 | 1.543 |
| 0.25 | 0.281 | 0.346 | 0.435 | 0.536 | 0.675 | 0.843 | 1.055 | 1.310 | 1.642 |
| 0.30 | 0.312 | 0.389 | 0.486 | 0.599 | 0.744 | 0.926 | 1.144 | 1.424 | 1.753 |
| 0.35 | 0.353 | 0.433 | 0.538 | 0.662 | 0.816 | 0.992 | 1.242 | 1.520 | 1.875 |
| 0.40 | 0.407 | 0.495 | 0.603 | 0.731 | 0.890 | 1.093 | 1.324 | 1.614 | 1.974 |
| 0.45 | 0.472 | 0.572 | 0.699 | 0.837 | 1.011 | 1.211 | 1.461 | 1.760 | 2.092 |
| 0.50 | 0.562 | 0.661 | 0.791 | 0.941 | 1.126 | 1.326 | 1.577 | 1.871 | 2.253 |
| 0.55 | 0.679 | 0.804 | 0.949 | 1.106 | 1.292 | 1.523 | 1.783 | 2.085 | 2.402 |
| 0.60 | 0.900 | 1.035 | 1.189 | 1.363 | 1.572 | 1.792 | 2.073 | 2.384 | 2.716 |
| 0.65 | 1.021 | 1.168 | 1.343 | 1.535 | 1.731 | 1.982 | 2.250 | 2.556 | 2.890 |
| 0.70 | 1.269 | 1.440 | 1.611 | 1.795 | 2.032 | 2.279 | 2.560 | 2.859 | 3.208 |
| 0.75 | 1.585 | 1.743 | 1.946 | 2.161 | 2.404 | 2.636 | 2.977 | 3.286 | 3.655 |
| 0.80 | 1.916 | 2.098 | 2.316 | 2.554 | 2.833 | 3.062 | 3.422 | 3.754 | 4.198 |
| 0.85 | 2.306 | 2.525 | 2.783 | 3.024 | 3.382 | 3.684 | 4.055 | 4.446 | 4.946 |
| 0.90 | 2.673 | 2.964 | 3.314 | 3.671 | 4.037 | 4.468 | 4.945 | 5.427 | 5.972 |
| 0.95 | 2.966 | 3.279 | 3.696 | 4.126 | 4.615 | 5.139 | 5.818 | 6.518 | 7.442 |
| 1.00 | 3.162 | 3.849 | 4.653 | 5.323 | 6.022 | 6.663 | 7.748 | 8.649 | 9.352 |
| Sample | Enthalpy of Fusion, /kJ·mol | Melting Point /K | Ref. |
|---|---|---|---|
| Original sample | 44.36 ± 0.5 | 532.6 ± 0.5 | This work |
| 44.352 | 532.7 | [27] | |
| 44.35 | 520.4 | [28] | |
| 31.21 | 538.7 | [29] | |
| 538.8 | [29] | ||
| 534.0 | [30] | ||
| 531.0 | [30] | ||
| 532.4 | [2] | ||
| 532.6 | [15] | ||
| 1-Propanol | 44.23 ± 0.5 | 533.1 ± 0.5 | This work |
| 44.45 ± 0.5 | 531.8 ± 0.5 | This work | |
| Acetonitrile | 44.63 ± 0.5 | 532.4 ± 0.5 | This work |
| Temperature/K | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 278.15 | 283.15 | 288.15 | 293.15 | 298.15 | 303.15 | 308.15 | 313.15 | 318.15 | |
| 0.00 | 99.13 | 85.70 | 77.99 | 65.65 | 63.99 | 60.63 | 58.11 | 53.93 | 52.59 |
| 0.05 | 84.04 | 78.98 | 74.30 | 70.00 | 65.49 | 60.58 | 56.63 | 52.48 | 48.61 |
| 0.10 | 74.19 | 70.73 | 65.77 | 62.60 | 58.24 | 54.78 | 51.08 | 47.46 | 44.28 |
| 0.15 | 66.68 | 63.11 | 59.26 | 55.88 | 52.23 | 49.44 | 46.24 | 43.21 | 40.34 |
| 0.20 | 60.09 | 56.77 | 53.50 | 50.63 | 47.48 | 45.10 | 42.33 | 39.70 | 37.40 |
| 0.25 | 53.51 | 52.02 | 49.28 | 47.50 | 44.62 | 42.21 | 39.74 | 37.59 | 35.15 |
| 0.30 | 48.31 | 46.26 | 44.09 | 42.45 | 40.53 | 38.44 | 36.65 | 34.59 | 32.92 |
| 0.35 | 42.63 | 41.53 | 39.84 | 38.45 | 36.92 | 35.87 | 33.75 | 32.41 | 30.78 |
| 0.40 | 37.01 | 36.33 | 35.54 | 34.82 | 33.87 | 32.58 | 31.66 | 30.51 | 29.23 |
| 0.45 | 31.92 | 31.45 | 30.65 | 30.42 | 29.82 | 29.40 | 28.70 | 27.98 | 27.59 |
| 0.50 | 26.79 | 27.22 | 27.07 | 27.04 | 26.77 | 26.84 | 26.59 | 26.32 | 25.62 |
| 0.55 | 22.16 | 22.36 | 22.57 | 23.00 | 23.33 | 23.37 | 23.51 | 23.62 | 24.03 |
| 0.60 | 16.72 | 17.38 | 18.02 | 18.67 | 19.17 | 19.87 | 20.22 | 20.66 | 21.25 |
| 0.65 | 14.74 | 15.40 | 15.95 | 16.58 | 17.41 | 17.96 | 18.63 | 19.27 | 19.97 |
| 0.70 | 11.86 | 12.49 | 13.30 | 14.18 | 14.83 | 15.62 | 16.38 | 17.23 | 17.99 |
| 0.75 | 9.49 | 10.32 | 11.01 | 11.78 | 12.54 | 13.50 | 14.08 | 14.99 | 15.79 |
| 0.80 | 7.85 | 8.57 | 9.25 | 9.96 | 10.64 | 11.62 | 12.25 | 13.12 | 13.74 |
| 0.85 | 6.53 | 7.12 | 7.70 | 8.42 | 8.91 | 9.66 | 10.34 | 11.08 | 11.67 |
| 0.90 | 5.63 | 6.07 | 6.47 | 6.93 | 7.47 | 7.97 | 8.48 | 9.07 | 9.66 |
| 0.95 | 5.07 | 5.49 | 5.80 | 6.17 | 6.53 | 6.93 | 7.21 | 7.56 | 7.75 |
| 1.00 | 4.76 | 4.67 | 4.60 | 4.78 | 5.00 | 5.34 | 5.41 | 5.69 | 6.17 |
| / | ||||||
|---|---|---|---|---|---|---|
| (kJ·mol) | (kJ·mol) | (J·mol·K | (kJ·mol) | |||
| 0.00 | 24.79 | 36.09 | 37.94 | 11.29 | 0.76 | 0.24 |
| 0.05 | 24.70 | 34.77 | 33.83 | 10.07 | 0.78 | 0.22 |
| 0.10 | 24.43 | 34.27 | 33.07 | 9.84 | 0.78 | 0.22 |
| 0.15 | 24.17 | 33.95 | 32.85 | 9.77 | 0.78 | 0.22 |
| 0.20 | 23.94 | 33.43 | 31.89 | 9.49 | 0.78 | 0.22 |
| 0.25 | 23.76 | 32.56 | 29.56 | 8.80 | 0.79 | 0.21 |
| 0.30 | 23.52 | 31.76 | 27.69 | 8.24 | 0.79 | 0.21 |
| 0.35 | 23.30 | 30.71 | 24.89 | 7.41 | 0.81 | 0.19 |
| 0.40 | 23.07 | 29.03 | 20.05 | 5.97 | 0.83 | 0.17 |
| 0.45 | 22.78 | 27.41 | 15.58 | 4.64 | 0.86 | 0.14 |
| 0.50 | 22.51 | 25.50 | 10.03 | 2.99 | 0.90 | 0.10 |
| 0.55 | 22.15 | 23.28 | 3.79 | 1.13 | 0.95 | 0.05 |
| 0.60 | 21.68 | 20.37 | −4.40 | −1.31 | 0.94 | 0.06 |
| 0.65 | 21.43 | 19.14 | −7.71 | −2.29 | 0.89 | 0.11 |
| 0.70 | 21.04 | 17.00 | −13.58 | −4.04 | 0.81 | 0.19 |
| 0.75 | 20.62 | 15.43 | −17.47 | −5.20 | 0.75 | 0.25 |
| 0.80 | 20.23 | 14.33 | −19.83 | −5.90 | 0.71 | 0.29 |
| 0.85 | 19.79 | 13.97 | −19.56 | −5.82 | 0.71 | 0.29 |
| 0.90 | 19.34 | 14.79 | −15.29 | −4.55 | 0.76 | 0.24 |
| 0.95 | 18.99 | 16.82 | −7.29 | −2.17 | 0.89 | 0.11 |
| 1.00 | 18.43 | 19.73 | 4.34 | 1.29 | 0.94 | 0.06 |
| (kJ·mol) | (kJ·mol) | (J·mol) | (kJ·mol) | |
|---|---|---|---|---|
| 0.00 | 13.51 | 11.24 | −7.62 | −2.27 |
| 0.05 | 13.41 | 9.92 | −11.74 | −3.49 |
| 0.10 | 13.14 | 9.42 | −12.50 | −3.72 |
| 0.15 | 12.88 | 9.10 | −12.72 | −3.78 |
| 0.20 | 12.65 | 8.58 | −13.67 | −4.07 |
| 0.25 | 12.47 | 7.71 | −16.00 | −4.76 |
| 0.30 | 12.23 | 6.91 | −17.88 | −5.32 |
| 0.35 | 12.01 | 5.86 | −20.67 | −6.15 |
| 0.40 | 11.78 | 4.18 | −25.51 | −7.59 |
| 0.45 | 11.49 | 2.57 | −29.99 | −8.92 |
| 0.50 | 11.22 | 0.65 | −35.53 | −10.57 |
| 0.55 | 10.86 | −1.57 | −41.77 | −12.43 |
| 0.60 | 10.39 | −4.48 | −49.96 | −14.87 |
| 0.65 | 10.14 | −5.71 | −53.27 | −15.85 |
| 0.70 | 9.75 | −7.85 | −59.15 | −17.60 |
| 0.75 | 9.33 | −9.42 | −63.03 | −18.76 |
| 0.80 | 8.94 | −10.52 | −65.39 | −19.46 |
| 0.85 | 8.50 | −10.88 | −65.13 | −19.38 |
| 0.90 | 8.05 | −10.06 | −60.86 | −18.11 |
| 0.95 | 7.70 | −8.02 | −52.85 | −15.73 |
| 1.00 | 7.14 | −5.12 | −41.22 | −12.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trujillo-Trujillo, C.F.; Angarita-Reina, F.; Herrera, M.; Ortiz, C.P.; Cardenas-Torres, R.E.; Martinez, F.; Delgado, D.R. Thermodynamic Analysis of the Solubility of Sulfadiazine in (Acetonitrile 1-Propanol) Cosolvent Mixtures from 278.15 K to 318.15 K. Liquids 2023, 3, 7-18. https://doi.org/10.3390/liquids3010002
Trujillo-Trujillo CF, Angarita-Reina F, Herrera M, Ortiz CP, Cardenas-Torres RE, Martinez F, Delgado DR. Thermodynamic Analysis of the Solubility of Sulfadiazine in (Acetonitrile 1-Propanol) Cosolvent Mixtures from 278.15 K to 318.15 K. Liquids. 2023; 3(1):7-18. https://doi.org/10.3390/liquids3010002
Chicago/Turabian StyleTrujillo-Trujillo, Carlos Francisco, Fredy Angarita-Reina, Mauricio Herrera, Claudia Patria Ortiz, Rossember Edén Cardenas-Torres, Fleming Martinez, and Daniel Ricardo Delgado. 2023. "Thermodynamic Analysis of the Solubility of Sulfadiazine in (Acetonitrile 1-Propanol) Cosolvent Mixtures from 278.15 K to 318.15 K" Liquids 3, no. 1: 7-18. https://doi.org/10.3390/liquids3010002
APA StyleTrujillo-Trujillo, C. F., Angarita-Reina, F., Herrera, M., Ortiz, C. P., Cardenas-Torres, R. E., Martinez, F., & Delgado, D. R. (2023). Thermodynamic Analysis of the Solubility of Sulfadiazine in (Acetonitrile 1-Propanol) Cosolvent Mixtures from 278.15 K to 318.15 K. Liquids, 3(1), 7-18. https://doi.org/10.3390/liquids3010002











