Density and Dynamic Viscosity of Perfluorodecalin-Added n-Hexane Mixtures: Deciphering the Role of Fluorous Liquids
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
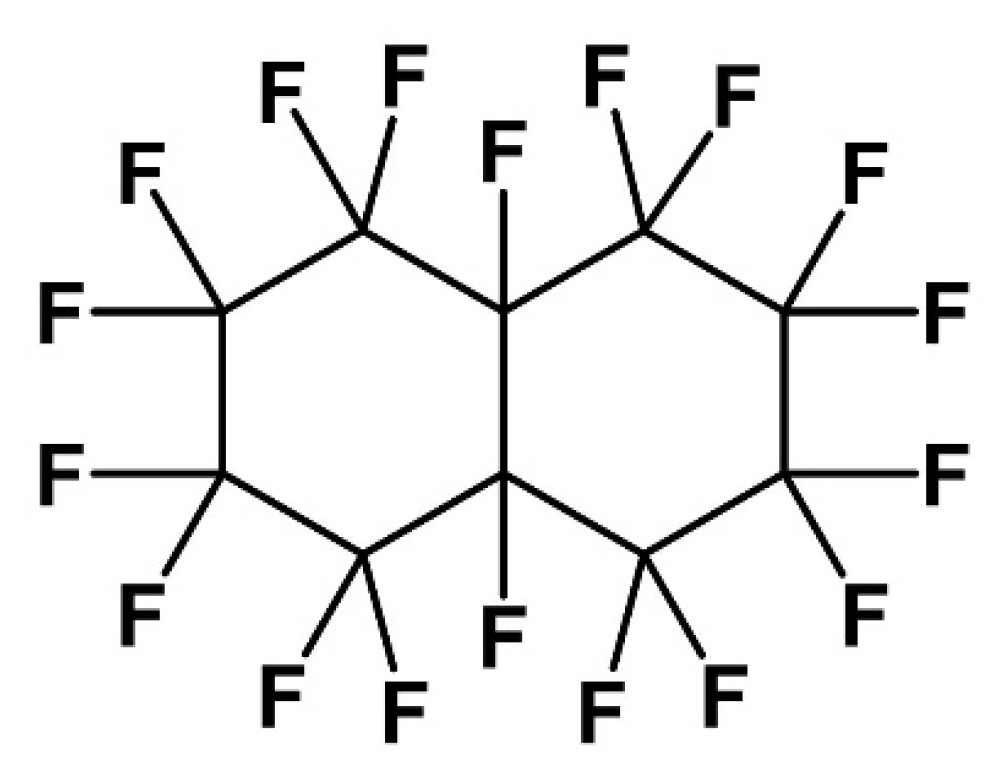
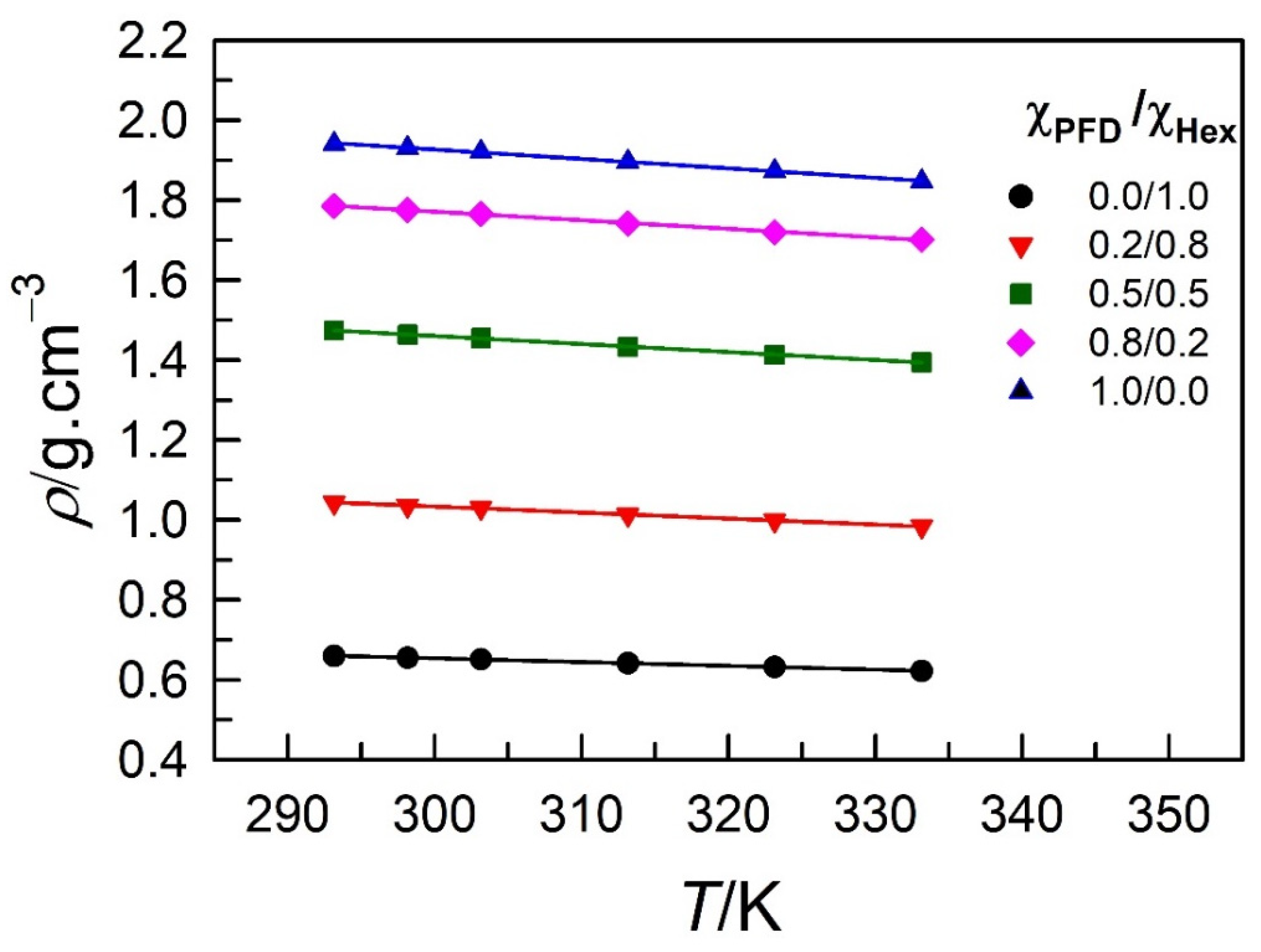
3.1. Density of PFD and (PFD + n-Hexane) Mixtures
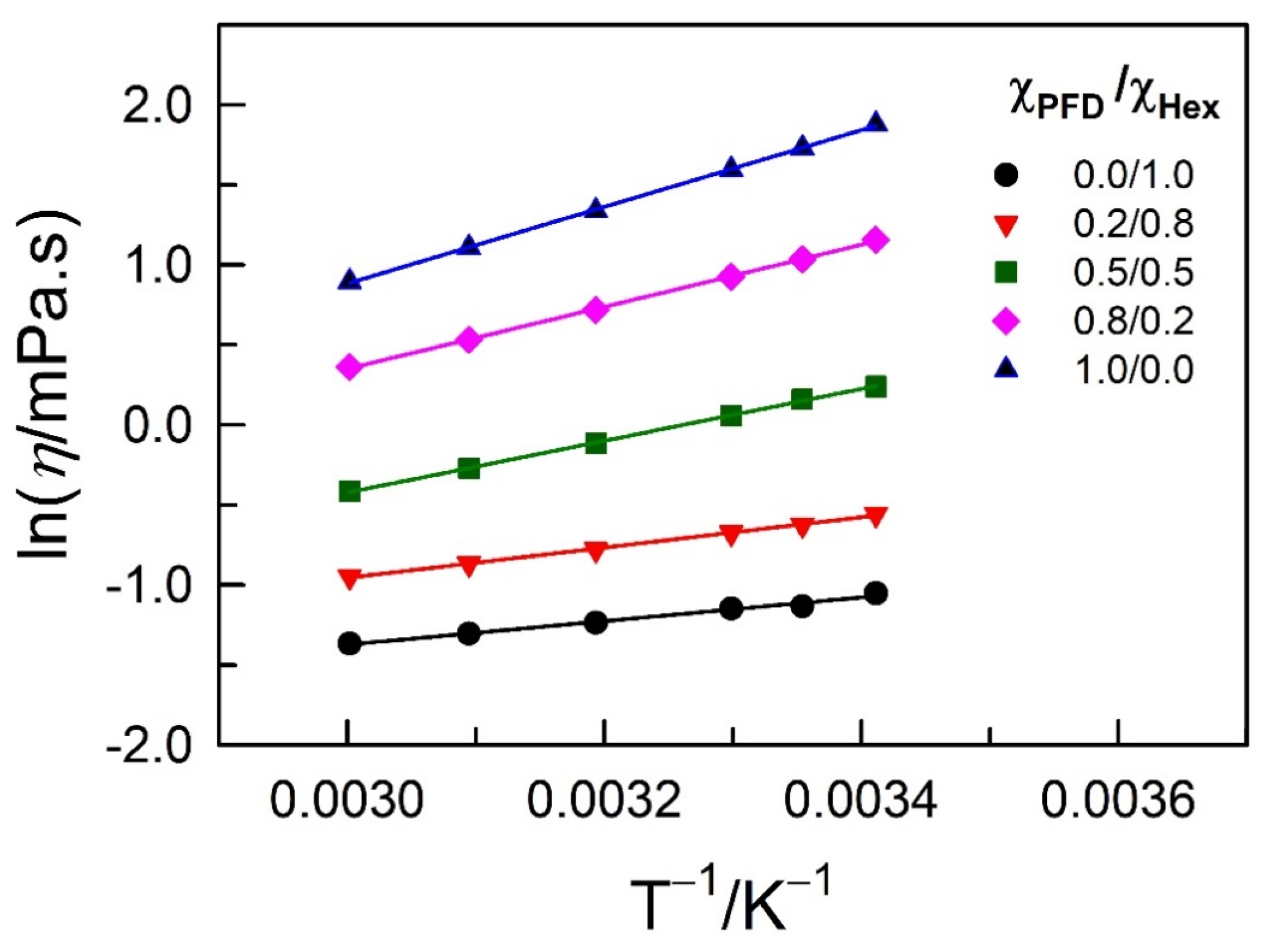
3.2. Dynamic Viscosity of PFD and (PFD + n-Hexane) Mixtures
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sheldon, R.A. Green solvents for sustainable organic synthesis: State of the art. Green Chem. 2005, 7, 267–278. [Google Scholar] [CrossRef]
- Capello, C.; Fischer, U.; Hungerbuhler, K. What is green solvent? A comprehensive framework for the environmental assessment of solvents. Green Chem. 2007, 9, 927–934. [Google Scholar] [CrossRef]
- Clark, J.H.; Tavener, S.J. Alternative solvents: Shades of green. Org. Process Res. Dev. 2007, 11, 149–155. [Google Scholar] [CrossRef]
- Horváth, I.T. Solvents from nature. Green Chem. 2008, 10, 1024–1028. [Google Scholar] [CrossRef]
- Anastas, P.T.; Warner, J.C. Green Chemistry: Theory and Practice; Oxford University Press: Oxford, UK, 1998. [Google Scholar]
- Gladysz, J.A.; Curran, D.P.; Horváth, I.T. Handbook of Fluorous Chemistry; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2004. [Google Scholar]
- Horváth, I.T.; Rábai, J. Facile catalyst separation without water: Fluorous biphase hydroformylation of olefins. Science 1994, 266, 72–75. [Google Scholar] [CrossRef] [PubMed]
- Wolf, E.; van Koten, G.; Deelman, B.-J. Fluorous phase separation techniques in catalysis. Chem. Soc. Rev. 1999, 28, 37–41. [Google Scholar] [CrossRef]
- Barthel Rosa, L.P.; Gladysz, J.A. Chemistry in fluorous media: A user’s guide to practical considerations in the application of fluorous catalysts and reagents. Coord. Chem. Rev. 1999, 190–192, 587–605. [Google Scholar] [CrossRef]
- Zhang, W.; Cai, C. New chemical, and biological applications of fluorous technologies. Chem. Commun. 2008, 5686–5694. [Google Scholar] [CrossRef] [PubMed]
- Horvath, I.T. Fluorous biphase chemistry. Acc. Chem. Res. 1998, 31, 641–650. [Google Scholar] [CrossRef]
- Zhang, W.; Soloshonok, V.A.; Mikami, K.; Yamazaki, T.; Welch, J.T.; Honek, J. Current Fluoroorganic Chemistry: New Synthetic Directions, Technologies, Materials and Biological Applications; Oxford University Press: Oxford, UK, 2006; pp. 207–220. [Google Scholar]
- Lowe, K.C. Engineering blood: Synthetic substitutes from fluorinated compound. Tissue Eng. 2003, 9, 389–399. [Google Scholar] [CrossRef] [PubMed]
- Khalil, M.A.K.; Rasmussen, R.A.; Culbertson, J.A.; Prins, J.M.; Grimsrud, E.P.; Shearer, M.J. Atmospheric perfluorocarbons. Environ. Sci. Technol. 2003, 37, 4358–4361. [Google Scholar] [CrossRef] [PubMed]
- Kennedy, G.L.; Butenhoff, J.L.; Olsen, G.W.; O’Connor, J.C.; Seacat, A.M.; Perkins, R.G.; Biegel, L.B.; Murphy, S.R.; Farrar, D.G. The toxicology of perfluorooctanoate. Crit. Rev. Toxicol. 2004, 34, 351–384. [Google Scholar] [CrossRef] [PubMed]
- Stephan, C.; Schlawne, C.; Grass, S.; Waack, I.N.; Ferenz, K.B.; Bachmann, M.; Barnert, S.; Schubert, R.; Bastmeyer, M.; De Groot, H.; et al. Artificial oxygen carriers based on perfluorodecalin-filled poly(n-butyl-cyanoacrylate) nanocapsules. J. Microencapsul. 2014, 31, 284–292. [Google Scholar] [CrossRef] [PubMed]
- Rumble, J.R.; Bruno, T.J.; Doa, M.J. CRC Handbook of Chemistry and Physics: A Ready Reference Book of Chemical and Physical Data, 102nd ed.; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar]
- Freire, M.G.; Ferreira, A.G.M.; Fonseca, I.M.A.; Marrucho, I.M.; Coutinho, J.A.P. Viscosities of liquid fluorocompounds. J. Chem. Eng. Data 2008, 53, 538–542. [Google Scholar] [CrossRef][Green Version]
- Fehlauer, H.; Wolf, H. Density reference liquids certified by the Physikalisch-Techische Bundesanstalt. Meas. Sci. Technol. 2006, 17, 2588–2592. [Google Scholar] [CrossRef]
- Sulthana, S.P.; Gowrisankar, M.; Babu, S.; Rao, P.V. Binary mixtures of 2-ethyl-1-hexanol with various functional groups (benzyl chloride, 3-methylaniline, 3-methoxyaniline and 2,6-dimethylcyclohexanone. SN Appl. Sci. 2020, 2, 960. [Google Scholar] [CrossRef]
- Heintz, A.; Klasen, D.; Lehmann, J.K. Excess molar volumes and viscosities of binary mixtures of methanol and the ionic l iquid4- Methyl-N-butylpyridinium Tetrafluoroborate at 25, 40, and 50±C. J. Solut. Chem. 2002, 31, 467–476. [Google Scholar] [CrossRef]


| d | T/K | |||||
|---|---|---|---|---|---|---|
| 293 | 298 | 303 | 313 | 323 | 333 | |
| 0.0 | 0.6596 | 0.6553 | 0.6515 | 0.6413 | 0.6320 | 0.6219 |
| 0.2 | 1.0433 | 1.0355 | 1.0298 | 1.0134 | 0.9983 | 0.9835 |
| 0.5 | 1.4735 | 1.4633 | 1.4553 | 1.4332 | 1.4127 | 1.3943 |
| 0.8 | 1.7855 | 1.7750 | 1.7661 | 1.7420 | 1.7199 | 1.7015 |
| 1.0 | 1.9412 | 1.9303 | 1.9212 | 1.8961 | 1.8733 | 1.8468 |
| b | 0,T (g·cm−3) | 10−3 (g·cm−3·K−1) | R2 |
|---|---|---|---|
| 0.0 | 0.9379 ± 0.0048 | −0.9 ± 0.01 | 0.9990 |
| 0.2 | 1.4839 ± 0.0064 | −1.5 ± 0.02 | 0.9993 |
| 0.5 | 2.0610 ± 0.0094 | −2.0 ± 0.03 | 0.9991 |
| 0.8 | 2.4134 ± 0.0134 | −2.1 ± 0.04 | 0.9984 |
| 1.0 | 2.6338 ± 0.0154 | −2.4 ± 0.05 | 0.9982 |
| T/K | 0,xPFD (g·cm−3) | R2 | ||
|---|---|---|---|---|
| 293 | 0.6661 ± 0.0107 | 1.9682 ± 0.0548 | −0.6984 ± 0.0529 | 0.9998 |
| 298 | 0.6616 ± 0.0102 | 1.9521 ± 0.0526 | −0.6883 ± 0.0508 | 0.9998 |
| 303 | 0.6578 ± 0.0103 | 1.9409 ± 0.0527 | −0.6824 ± 0.0509 | 0.9998 |
| 313 | 0.6474 ± 0.0099 | 1.9091 ± 0.0507 | −0.6650 ± 0.0489 | 0.9998 |
| 323 | 0.6378 ± 0.0095 | 1.8789 ± 0.0487 | −0.6478 ± 0.0471 | 0.9998 |
| 333 | 0.6267 ± 0.0076 | 1.8682 ± 0.0392 | −0.6507 ± 0.0379 | 0.9999 |
| d | T/K | |||||
|---|---|---|---|---|---|---|
| 293 | 298 | 303 | 313 | 323 | 333 | |
| 0.0 | 0.349 | 0.322 | 0.317 | 0.291 | 0.271 | 0.255 |
| 0.2 | 0.572 | 0.536 | 0.509 | 0.458 | 0.420 | 0.387 |
| 0.5 | 1.271 | 1.173 | 1.060 | 0.892 | 0.768 | 0.669 |
| 0.8 | 3.179 | 2.816 | 2.524 | 2.050 | 1.703 | 1.435 |
| 1.0 | 6.535 | 5.647 | 4.925 | 3.815 | 3.152 | 2.446 |
| xPFD | /kJ·mol−1 | R2 | |
|---|---|---|---|
| 0.0 | −3.589 ± 0.130 | 6.14 ± 0.33 | 0.9883 |
| 0.2 | −3.794 ± 0.062 | 7.87 ± 0.16 | 0.9983 |
| 0.5 | −5.275 ± 0.055 | 13.45 ± 0.14 | 0.9996 |
| 0.8 | −5.465 ± 0.065 | 16.12 ± 0.17 | 0.9999 |
| 1.0 | −6.303 ± 0.066 | 19.92 ± 0.17 | 0.9999 |
| T/K | 0 (mPa·s) | f | R2 | |
|---|---|---|---|---|
| 293 | 0.238 ± 0.060 | 0.151 ± 0.020 | 3.726 ± 0.130 | 0.9997 |
| 298 | 0.212 ± 0.063 | 0.151 ± 0.023 | 3.583 ± 0.149 | 0.9996 |
| 303 | 0.201 ± 0.041 | 0.145 ± 0.016 | 3.482 ± 0.107 | 0.9998 |
| 313 | 0.174 ± 0.028 | 0.137 ± 0.012 | 3.275 ± 0.084 | 0.9999 |
| 323 | 0.156 ± 0.024 | 0.130 ± 0.012 | 3.093 ± 0.085 | 0.9999 |
| 333 | 0.142 ± 0.031 | 0.124 ± 0.016 | 2.924 ± 0.121 | 0.9997 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deepika; Pandey, S. Density and Dynamic Viscosity of Perfluorodecalin-Added n-Hexane Mixtures: Deciphering the Role of Fluorous Liquids. Liquids 2023, 3, 48-56. https://doi.org/10.3390/liquids3010005
Deepika, Pandey S. Density and Dynamic Viscosity of Perfluorodecalin-Added n-Hexane Mixtures: Deciphering the Role of Fluorous Liquids. Liquids. 2023; 3(1):48-56. https://doi.org/10.3390/liquids3010005
Chicago/Turabian StyleDeepika, and Siddharth Pandey. 2023. "Density and Dynamic Viscosity of Perfluorodecalin-Added n-Hexane Mixtures: Deciphering the Role of Fluorous Liquids" Liquids 3, no. 1: 48-56. https://doi.org/10.3390/liquids3010005
APA StyleDeepika, & Pandey, S. (2023). Density and Dynamic Viscosity of Perfluorodecalin-Added n-Hexane Mixtures: Deciphering the Role of Fluorous Liquids. Liquids, 3(1), 48-56. https://doi.org/10.3390/liquids3010005








