Intermodal Terminal Planning under Decentralized Management: Optimization Model for Rail-Road Terminals and Application to Portugal
Abstract
1. Introduction
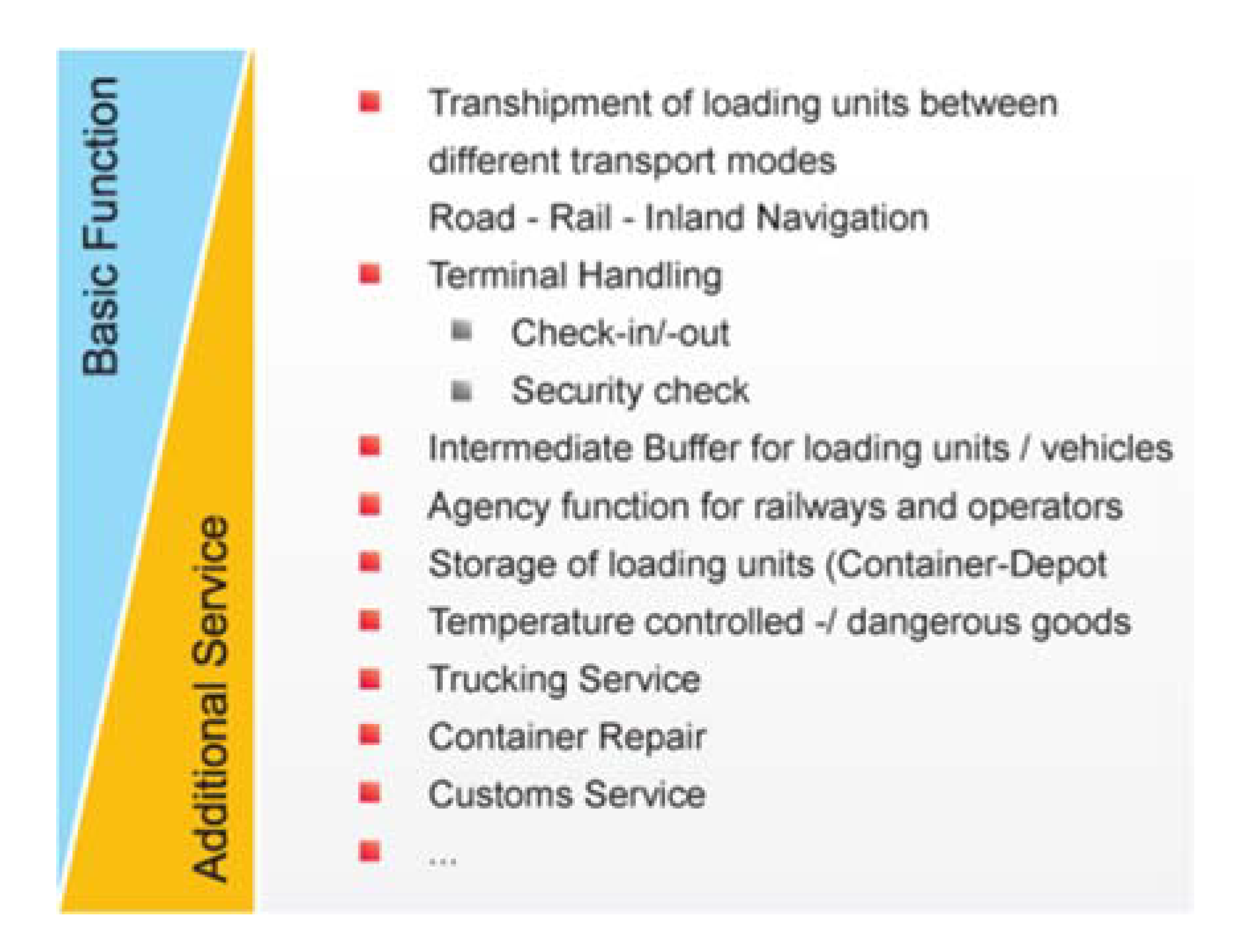
2. Rail-Road Terminals
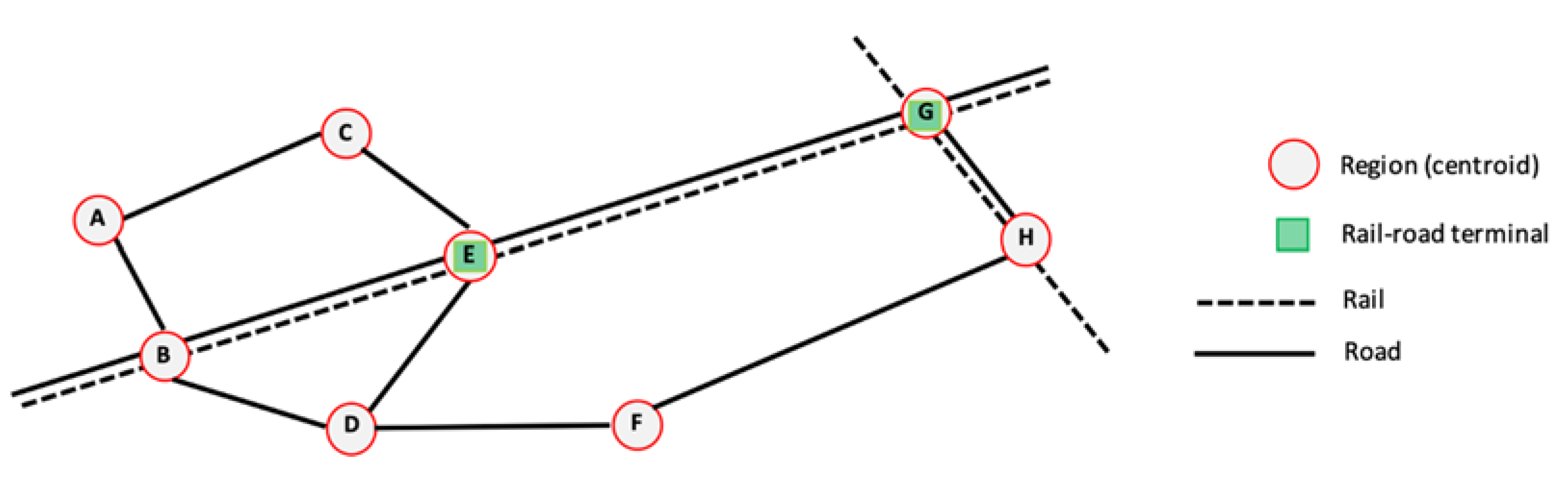
3. Problem Description
4. Related Work
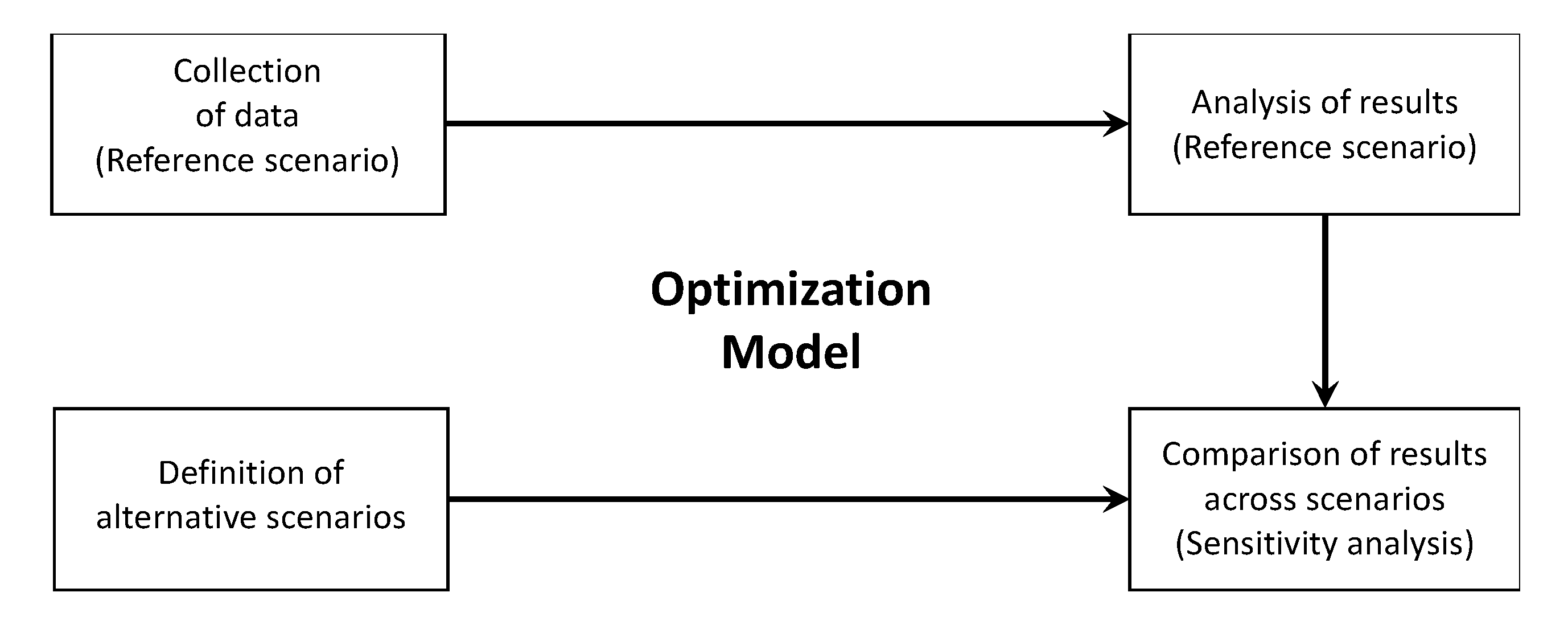
5. Optimization Model
5.1. Centralized Management
5.2. Decentralized Management
6. Case Study
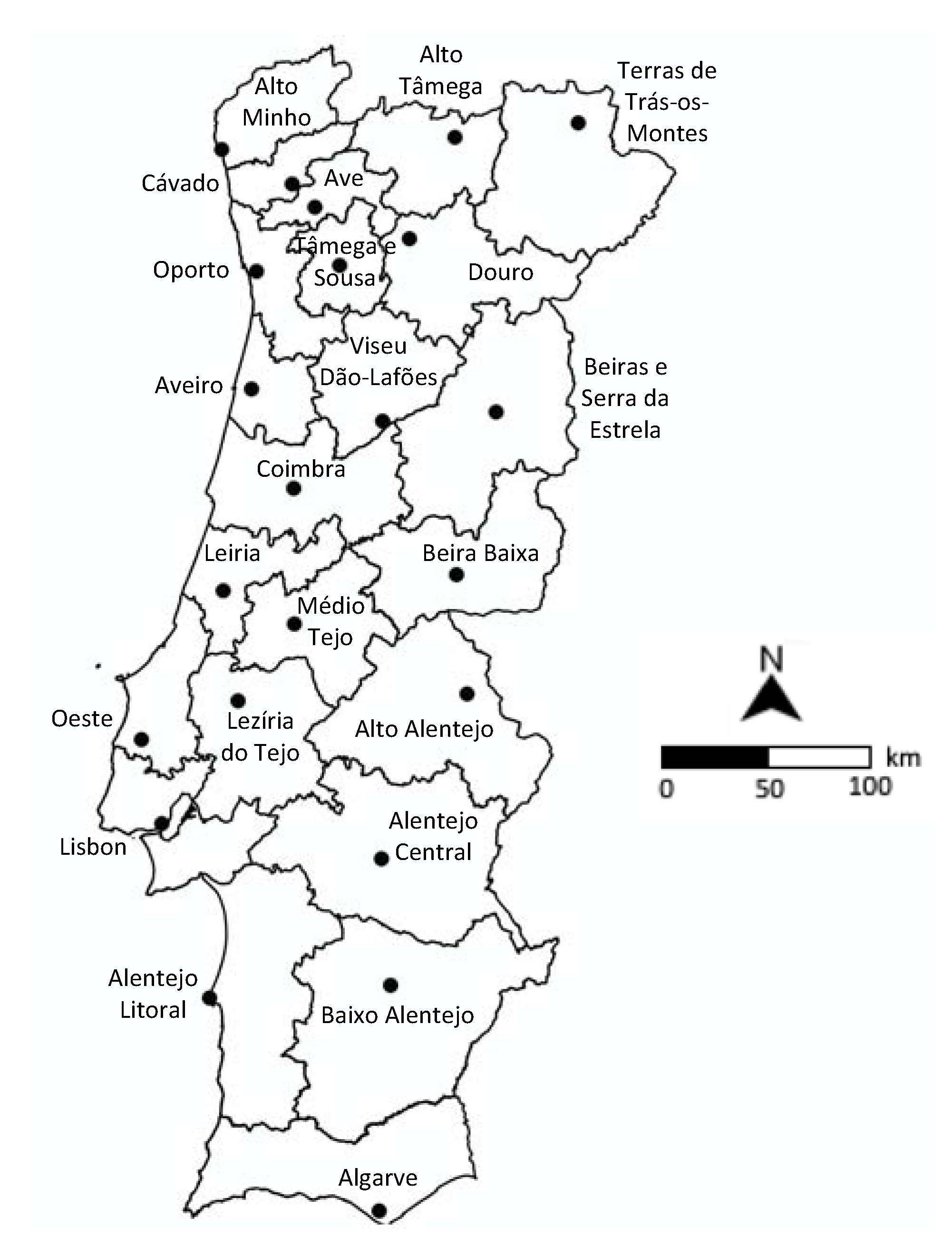
6.1. Study Data
6.2. Study Results
6.2.1. Reference Scenario
6.2.2. Sensitivity Analysis
7. Model Solving
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rodrigue, J.-P. The Geography of Transport Systems; Routledge: New York, NY, USA, 2017. [Google Scholar]
- Agamez-Arias, A.D.M.A.; Moyano-Fuentes, J. Intermodal transport in freight distribution: A literature review. Transp. Rev. 2017, 37, 782–807. [Google Scholar] [CrossRef]
- Donovan, A. Intermodal Transportation in Historical Perspective. Transp. Law J. 2000, 27, 317–344. [Google Scholar]
- Bektas, T. Freight Transport and Distribution: Concepts and Optimisation Models; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Vasconcelos, A.D.; Nassi, C.D.; Lopes, L.A.S. The uncapacitated hub location problem in networks under decentralized management. Comput. Oper. Res. 2011, 38, 1656–1666. [Google Scholar] [CrossRef]
- Caris, A.; Macharis, C.; Janssens, G.K. Planning Problems in Intermodal Freight Transport: Accomplishments and Prospects. Transp. Plan. Technol. 2008, 31, 277–302. [Google Scholar] [CrossRef]
- SteadieSeifi, M.; Dellaert, N.P.; Nuijten, W.; Van Woensel, T.; Raoufi, R. Multimodal freight transportation planning: A literature review. Eur. J. Oper. Res. 2014, 233, 1–15. [Google Scholar] [CrossRef]
- Caris, A.; Macharis, C.; Janssens, G.K. Decision support in intermodal transport: A new research agenda. Comput. Ind. 2013, 64, 105–112. [Google Scholar] [CrossRef]
- Kapros, S.; Panou, K.; Tsamboulas, D.A. Multicriteria Approach to the Evaluation of Intermodal Freight Villages. Transp. Res. Rec. 2005, 1906, 56–63. [Google Scholar] [CrossRef]
- Ballis, A.; Mavrotas, G. Freight village design using the multicriteria method PROMETHEE. Oper. Res. 2007, 7, 213–231. [Google Scholar] [CrossRef]
- Kayikci, Y. A conceptual model for intermodal freight logistics centre location decisions. Procedia Soc. Behav. Sci. 2010, 2, 6297–6311. [Google Scholar] [CrossRef]
- Tadić, S.; Zečević, S.; Krstić, M. A novel hybrid MCDM model based on fuzzy DEMATEL, fuzzy ANP and fuzzy VIKOR for city logistics concept selection. Expert Syst. Appl. 2014, 41, 8112–8128. [Google Scholar] [CrossRef]
- Wiegmans, B.; Behdani, B. A review and analysis of the investment in, and cost structure of, intermodal rail terminals. Transp. Rev. 2018, 38, 33–51. [Google Scholar] [CrossRef]
- Christiansen, M.; Fagerholt, K.; Nygreen, B.; Ronen, D. Maritime Transportation. In Transportation (Handbooks in Operations Research and Management Science Volume 14); Barnhart, C., Laporte, G., Eds.; Elsevier: Amsterdam, The Netherlands, 2007; pp. 189–284. [Google Scholar]
- Wiegmans, B.W.; Masurel, E.; Nijkamp, P. Intermodal freight terminals: An analysis of the freight terminal market. Transp. Plan. Technol. 1999, 63, 105–168. [Google Scholar] [CrossRef][Green Version]
- Tadić, S.; Krstić, M.; Brnjac, N. Selection of efficient types of inland intermodal terminals. J. Transp. Geogr. 2019, 78, 170–180. [Google Scholar] [CrossRef]
- Mostert, M.; Limbourg, S. External Costs as Competitiveness Factors for Freight Transport—A State of the Art. Transp. Rev. 2016, 36, 692–712. [Google Scholar] [CrossRef]
- Alumur, S.A.; Kara, B.Y. Network hub location problems: The state of the art. Eur. J. Oper. Res. 2008, 190, 1–21. [Google Scholar] [CrossRef]
- Campbell, J.F.; O’Kelly, M.E. Twenty-Five Years of Hub Location Research. Transp. Sci. 2012, 46, 153–169. [Google Scholar] [CrossRef]
- Farahani, R.Z.; Hekmatfar, M.; Arabani, A.B.; Nikbakhsh, E. Hub location problems: A review of models, classification, solution techniques, and applications. Comput. Ind. Eng. 2013, 64, 1096–1109. [Google Scholar] [CrossRef]
- Contreras, I. Hub location problems. In Location Science; Laporte, G., Nickel, S., Saldanha da Gama, F., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; pp. 311–344. [Google Scholar]
- Arnold, P.; Thomas, I. Localisation des centres de transbordement dans un système multiréseaux: Essai de formalization. L’Espace Geogr. 1999, 3, 193–204. [Google Scholar] [CrossRef]
- Arnold, P.; Peeters, D.; Thomas, I.; Marchand, H. Pour une localisation optimale des centres de transbordement intermodaux entre réseaux de transport: Formulation et extensions. Can. Geogr. Geogr. Can. 2001, 45, 427–436. [Google Scholar] [CrossRef]
- Arnold, P.; Peeters, D.; Thomas, I. Modelling a rail/road intermodal transportation system. Transp. Res. Part E Logist. Transp. Rev. 2004, 40, 255–270. [Google Scholar] [CrossRef]
- Sörensen, K.; Vanovermeire, C.; Busschaert, S. Efficient metaheuristics to solve the intermodal terminal location problem. Comput. Oper. Res. 2012, 39, 2079–2090. [Google Scholar] [CrossRef]
- Sörensen, K.; Vanovermeire, C. Bi-objective optimization of the intermodal terminal location problem as a policy-support tool. Comput. Ind. 2013, 64, 128–135. [Google Scholar] [CrossRef]
- Lin, C.-C.; Chiang, Y.-I.; Lin, S.-W. Efficient model and heuristic for the intermodal terminal location problem. Comput. Oper. Res. 2014, 51, 41–51. [Google Scholar] [CrossRef]
- Lin, C.-C.; Lin, S.-W. Two-stage approach to the intermodal terminal location problem. Comput. Oper. Res. 2016, 67, 113–119. [Google Scholar] [CrossRef]
- Ishfaq, R.; Cox, C.R. Hub location–allocation in intermodal logistic networks. Eur. J. Oper. Res. 2011, 210, 213–230. [Google Scholar] [CrossRef]
- Santos, B.F.; Limbourg, S.; Carreira, J.S. The impact of transport policies on railroad intermodal freight competitiveness—The case of Belgium. Transp. Res. Part D Transp. Environ. 2015, 34, 230–244. [Google Scholar] [CrossRef]
- Ernst, A.; Krishnamoorthy, M. Efficient algorithms for the uncapacitated single allocation p-hub median problem. Locat. Sci. 1996, 4, 139–154. [Google Scholar] [CrossRef]
- Ghane-Ezabadi, M.; Vergara, H.A. Decomposition approach for integrated intermodal logistics network design. Transp. Res. Part E Logist. Transp. Rev. 2016, 89, 53–69. [Google Scholar] [CrossRef]
- Limbourg, S.; Jourquin, B. Optimal rail-road container terminal locations on the European network. Transp. Res. Part E Logist. Transp. Rev. 2009, 45, 551–563. [Google Scholar] [CrossRef]
- Zhang, M.; Wiegmans, B.; Tavasszy, L. Optimization of multimodal networks including environmental costs: A model and findings for transport policy. Comput. Ind. 2013, 64, 136–145. [Google Scholar] [CrossRef]
- Zhang, M.; Janic, M.; Tavasszy, L.A. A freight transport optimization model for integrated network, service, and policy design. Transp. Res. Part E Logist. Transp. Rev. 2015, 77, 61–76. [Google Scholar] [CrossRef]
- Colson, B.; Marcotte, P.; Savard, G. An overview of bilevel optimization. Ann. Oper. Res. 2007, 153, 235–256. [Google Scholar] [CrossRef]
- Sinha, A.; Malo, P.; Deb, K. A Review on Bilevel Optimization: From Classical to Evolutionary Approaches and Applications. IEEE Trans. Evol. Comput. 2017, 22, 276–295. [Google Scholar] [CrossRef]
- Chow, J.; Yang, C.H.; Regan, A.C. State-of-the art of freight forecast modeling: Lessons learned and the road ahead. Transportation 2010, 37, 1011–1030. [Google Scholar] [CrossRef]
- Tavasszy, L.A.; Ruijgrok, K.; Davydenko, I. Incorporating Logistics in Freight Transport Demand Models: State-of-the-Art and Research Opportunities. Transp. Rev. 2012, 32, 203–219. [Google Scholar] [CrossRef]
- Janić, M. Modelling the full costs of an intermodal and road freight transport network. Transp. Res. Part D Transp. Environ. 2006, 12, 33–44. [Google Scholar] [CrossRef]
- Hanssen, T.-E.S.; Mathisen, T.A.; Jørgensen, F. Generalized Transport Costs in Intermodal Freight Transport. Procedia Soc. Behav. Sci. 2012, 54, 189–200. [Google Scholar] [CrossRef]
- Bína, L.; Bínová, H.; Březina, E.; Kumpošt, P.; Padělek, T. Comparative model of unit costs of road and rail freight transport for selected European countries. Eur. J. Bus. Soc. Sci. 2016, 3, 127–136. [Google Scholar]
- Lodi, A. Mixed Integer Programming Computation. In 50 Years of Integer Programming 1958–2008: From the Early Years to the State-of-the-Art; Jünger, M., Liebling, T.M., Naddef, D., Nemhauser, G.L., Pulleyblank, W.R., Reinelt, G., Rinaldi, G., Wolsey, L.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2010; pp. 619–645. [Google Scholar]




| Terminal Type | Capacity (103 TEU/Year) |
|---|---|
| S | <10 |
| M | 10–30 |
| L | 30–100 |
| XL | 100–500 |
| XXL | >500 |
| Terminal Type | Capacity (103 TEU/Year) | Infrastructure (Rail Tracks) | Area (ha) | Equipment Costs (106 €) | Realization Costs (106 €) |
|---|---|---|---|---|---|
| S | 10 | 1 | 4 | 1.0 | 3.5 |
| M | 20 | 2 | 4 | 1.5 | 5.5 |
| L | 30 | 3 | 6 | 3.0 | 9.5 |
| XL | 100 | 6 | 10 | 13.0 | 47.0 |
| XXL | 500 | 12 | 40 | 23.0 | 138.0 |
| Reference (Chronological Order) | Formal Optimization Model | Model Formulation | Solution Method | Model Application(s) (Number of Possible New Terminals) | ||
|---|---|---|---|---|---|---|
| Fixed Costs | Capacity Constraints | Decentralized Management | ||||
| Arnold and Thomas (1999) | Yes | Yes | Yes | No | No | No |
| Arnold et al., (2001) | Yes | Yes | Yes | No | Exact | Belgium (12) |
| Arnold et al., (2004) | Yes | No | No | No | Heuristic | Iberian Peninsula (13) |
| Limbourg and Jourquin (2009) | Yes | No | No | Yes | Heuristic | Europe (84) |
| Ishfaq and Cox (2011) | Yes | Yes | No | No | Tabu Search | Randomly-generated |
| Vasconcelos et al., (2011) | Yes | Yes | No | Yes | Exact | Brazil (3) |
| Sörensen et al., (2012) | Yes | Yes | Yes | No | GRASP | Randomly-generated |
| Sörensen and Vanovermeire (2013) | Yes | Yes | Yes | No | GRASP | Randomly-generated |
| Lin et al., (2014) | Yes | Yes | Yes | No | Heuristic | Randomly-generated |
| Santos et al., (2015) | Yes | No | No | No | Exact | Belgium (35) |
| Zhang et al., (2015) | No | Yes | No | Yes | Genetic Algorithm | The Netherlands (42) |
| Ghane-Ezabadi and Vergara (2016) | Yes | Yes | Yes | No | Heuristic | Randomly-generated |
| Lin and Lin (2016) | Yes | Yes | No | No | Exact (Decomposition) | Randomly-generated |
| This paper | Yes | Yes | Yes | Yes | Exact | Portugal (16) |
| Solution Features | Intermodal Terminal Network | |||
|---|---|---|---|---|
| Decentralized Management | Centralized Management | |||
| Current | Optimal | Optimal | ||
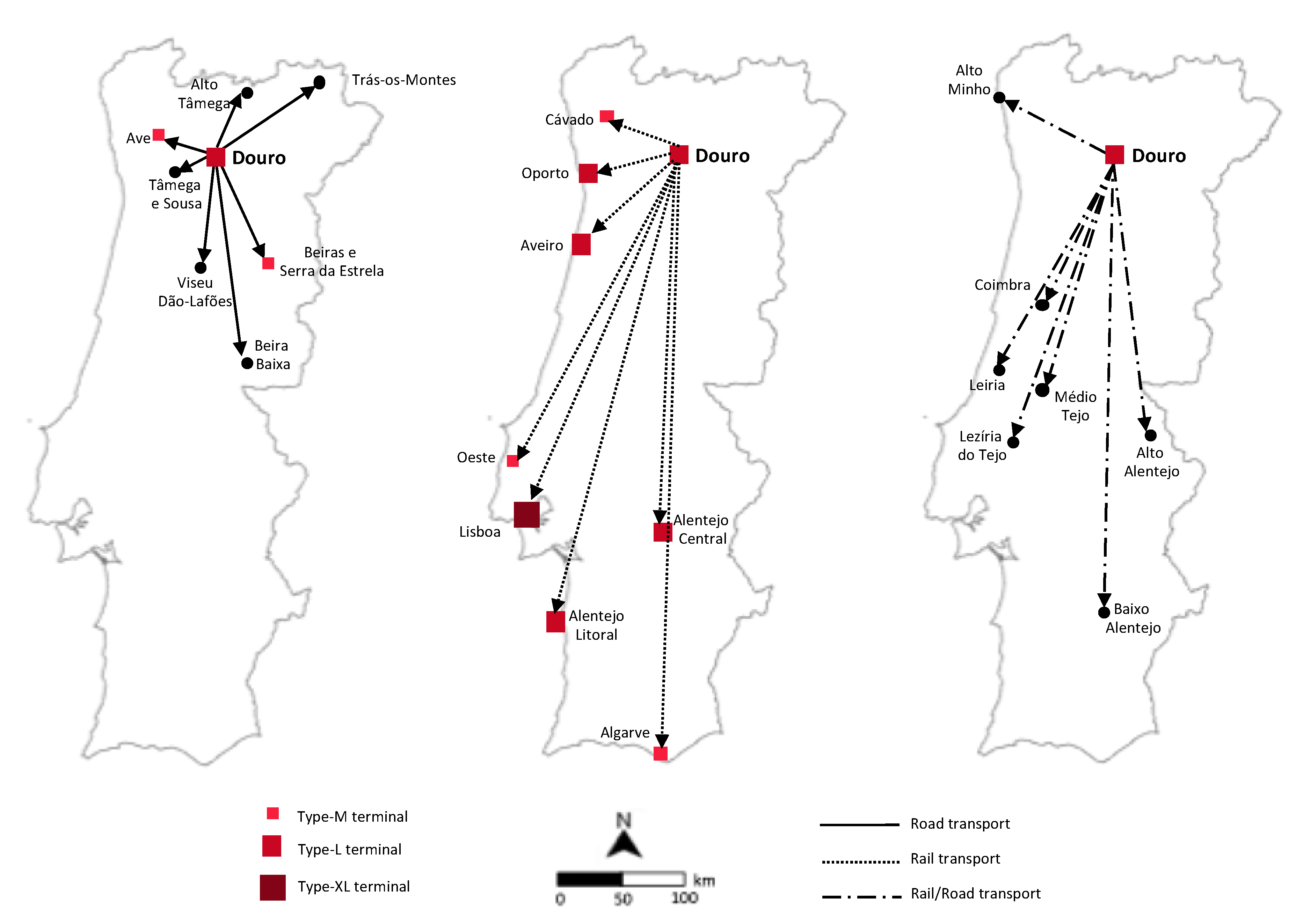
| Number of new terminals | XL | - | 0 | 0 |
| L | - | 2 | 7 | |
| M | - | 4 | 9 | |
| Freight tonnage (106TEU/year) | Intermodal | 0.137 | 0.281 | 0.67 |
| (3.2%) | (6.6%) | (15.8%) | ||
| Road-only | 4.103 | 3.959 | 3.570 | |
| Freight tonnage × km (106TEU×km/year) | Rail | 38.4 | 68.24 | 115.10 |
| (5.0%) | (8.8%) | (14.8%) | ||
| Road | 734.67 | 709.27 | 661.85 | |
| Annual-equivalent terminal investment costs (106 €/year) | - | 8.58 | 26.96 | |
| Total terminal revenues (106 €/year) | 13.66 | 28.12 | 66.97 | |
| Total terminal and transport costs (109 €/year) | 2.722 | 2.698 | 2.632 | |
| Region | Transport Costs (106 €/year) | Transport Cost Savings (%) | |
|---|---|---|---|
| Current Network | Optimal Network under Decentralized Management | ||
| Alentejo Central | 103.7 | 99.9 | 3.66 |
| Alentejo Litoral | 171.7 | 169.3 | 1.40 |
| Algarve | 27.9 | 26.5 | 5.02 |
| Alto Alentejo | 112.7 | 110.4 | 2.04 |
| Alto Minho | 104.7 | 103.8 | 0.86 |
| Alto Tâmega | 92.5 | 91.5 | 1.08 |
| Ave | 83.0 | 82.0 | 1.20 |
| Aveiro | 130.2 | 129.2 | 0.77 |
| Baixo Alentejo | 136.0 | 135.5 | 0.37 |
| Beira Baixa | 153.2 | 152.7 | 0.33 |
| Beiras e Serra da Estrela | 121.9 | 121.6 | 0.25 |
| Cávado | 85.4 | 83.9 | 1.76 |
| Coimbra | 109.0 | 108.6 | 0.37 |
| Douro | 84.9 | 82.3 | 3.06 |
| Leiria | 133.0 | 131.9 | 0.83 |
| Lezíria do Tejo | 85.5 | 85.0 | 0.58 |
| Lisbon | 315.8 | 311.3 | 1.42 |
| Médio Tejo | 110.8 | 110.3 | 0.45 |
| Oeste | 135.9 | 134.6 | 0.96 |
| Oporto | 95.6 | 93.7 | 1.99 |
| Tâmega e Sousa | 64.3 | 64.1 | 0.31 |
| Tras-os-Montes | 146.1 | 144.3 | 1.23 |
| Viseu Dão-Lafões | 117.8 | 117.5 | 0.25 |
| Portugal | 2721.6 | 2689.9 | 1.16 |
| Region | Current Network | Optimal Network under Decentralized Management | ||
|---|---|---|---|---|
| Terminal Type | Freight Handled (103TEU/year) | Terminal Type | Freight Handled (103TEU/Year) | |
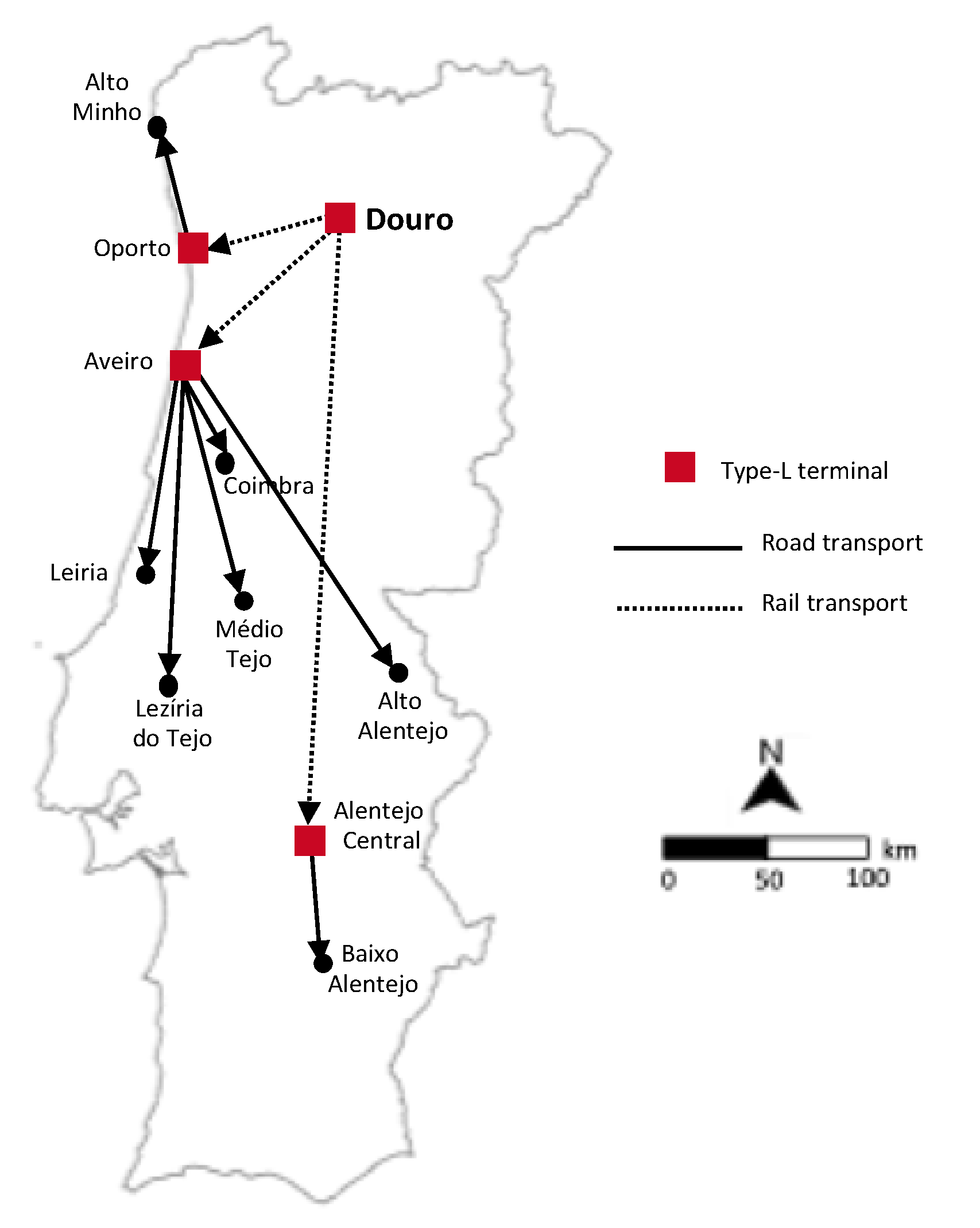
| Alentejo Central | - | - | L | 65.3 |
| Alentejo Litoral | L | 34.6 | L | 53.0 |
| Algarve | - | - | M | 14.5 |
| Ave | - | - | M | 24.8 |
| Aveiro | L | 45.8 | L | 76.0 |
| Beiras e Serra da Estrela | M | 25.5 | M | 25.5 |
| Cávado | - | - | M | 23.9 |
| Douro | - | - | L | 79.3 |
| Lisbon | XL | 99.9 | XL | 110.8 |
| Oeste | - | - | M | 23.9 |
| Oporto | L | 67.4 | L | 65.3 |
| Alternative Scenario | Variation with Respect to Reference Scenario | ||
|---|---|---|---|
| Freight Demand | Rail Transport Unit Costs | Road Transport Unit Costs | |
| S1 | +20% | 0 | 0 |
| S2 | −20% | 0 | 0 |
| S3 | 0 | +20% | 0 |
| S4 | 0 | −20% | 0 |
| S5 | 0 | 0 | +20% |
| S6 | 0 | 0 | −20% |
| Solution Features | Scenario | |||||||
|---|---|---|---|---|---|---|---|---|
| Reference | Freight Demand | Rail Transport Unit Costs | Road Transport Unit Costs | |||||
| S1 | S2 | S3 | S4 | S5 | S6 | |||
| +20% | −20% | +20% | −20% | +20% | −20% | |||
| Number of new terminals | XL | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| L | 2 | 9 | 4 | 3 | 11 | 10 | 2 | |
| M | 4 | 1 | 3 | 8 | 0 | 1 | 8 | |
| Freight tonnage (106TEU/year) | Intermodal | 0.281 | 0.608 | 0.345 | 0.307 | 0.614 | 0.619 | 0.250 |
| (6.6%) | (12.0%) | (10.2%) | (7.2%) | (14.5%) | (14.6%) | (5.9%) | ||
| Road only | 3.959 | 4.479 | 3.047 | 3.933 | 3.626 | 3.621 | 3.990 | |
| Total terminal and transport costs (109 €/year) | 2.698 | 3.190 | 2.141 | 2.703 | 2.605 | 3.122 | 2.175 | |
| Solution Features | Variation in Rail Transport Unit Costs | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| −20% | −15% | −10% | −5% | 0% | 5% | 10% | 15% | 20% | ||
| Number of new terminals | XL | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| L | 11 | 8 | 5 | 5 | 2 | 2 | 4 | 5 | 3 | |
| M | 0 | 2 | 2 | 2 | 4 | 3 | 6 | 7 | 8 | |
| Freight tonnage (106 TEU/year) | Intermodal | 0.614 | 0.534 | 0.431 | 0.418 | 0.281 | 0.269 | 0.376 | 0.422 | 0.307 |
| (14.5%) | (12.6%) | (10.2%) | (9.9%) | (6.6%) | (6.4%) | (8.9%) | (10.0%) | (7.2%) | ||
| Road only | 3.626 | 3.706 | 3.809 | 3.822 | 3.959 | 3.97 | 3.864 | 3.817 | 3.933 | |
| Total terminal and transport costs (109 €/year) | 2.605 | 2.627 | 2.655 | 2.666 | 2.698 | 2.704 | 2.684 | 2.687 | 2.703 | |
| Region | Optimal Terminal Network | |||||||
|---|---|---|---|---|---|---|---|---|
| Reference Scenario | Increase of Rail Transport Unit Costs (∆cra) | |||||||
| 5% | 10% | |||||||
| Type | Capacity | Freight Handled | Type | Capacity | Type | Capacity | ||
| (103 TEU/Year) | ∆cra = 5% | ∆cra = 10% | (103 TEU/Year) | (103 TEU/Year) | ||||
| Alentejo Central | L | 65.3 | 60.7 | 60.3 * | - | - | - | - |
| Alentejo Litoral | L | 53 | 50.8 | 50.8 | L | 56.6 | L | 48.1 |
| Algarve | M | 14.5 | 12.8 | 12.8 | - | - | M | 12.8 |
| Alto Alentejo | - | - | - | - | M | 29.7 | M | 24.5 |
| Ave | M | 24.8 | 24.8 | 23.7 | M | 25.5 | - | - |
| Aveiro | L | 76 | 73.1 | 78 | L | 71.6 | L | 48.7 |
| Beira Baixa | - | - | - | - | - | - | M | 28.9 |
| Beiras e Serra da Estrela | M | 25.5 | 24.6 | 24.6 | M | 25.6 | M | 27.4 |
| Cávado | M | 23.9 | 23.9 | 16.6 | M | 23.9 | M | 17.9 |
| Coimbra | - | - | - | - | - | - | L | 80.9 |
| Douro | L | 79.3 | 70.1 | 51.3 * | L | 71.3 | - | - |
| Leiria | - | - | - | - | - | - | L | 99.1 |
| Lezíria do Tejo | - | - | - | - | L | 71.4 | L | 62.7 |
| Lisbon | XL | 110.8 | 110.8 | 111.2 | XL | 104.5 | XL | 137.9 |
| Oeste | M | 23.9 | 20.1 | 9.3 * | - | - | M | 23.4 |
| Oporto | L | 65.3 | 56.7 | 47.4 | L | 58.6 | L | 37 |
| Tâmega e Sousa | - | - | - | - | - | - | M | 29.6 |
| Viseu Dão-Lafões | - | - | - | - | - | - | L | 73.5 |
| Freight tonnage (106 TEU/year) | Intermodal | 0.281 | 0.269 | 0.349 | ||||
| (6.6%) | (6.4%) | (8.2%) | ||||||
| Road only | 3.959 | 3.971 | 3.863 | |||||
| Total terminal and transport costs (109 €/year) | 2.698 | 2.707 | 2.704 | 2.684 | ||||
| Instance Size (# Regions) | Centralized Management | Decentralized Management | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Computation Time (Minutes) | Optimality Gap (%) | Computation Time (Minutes) | Optimality Gap (%) | |||||||||
| Min | Avg | Max | Min | Avg | Max | Min | Avg | Max | Min | Avg | Max | |
| 15 | <1 | <1 | <1 | 0 | 0 | 0 | 35 | 71 | 149 | 0 | 0 | 0 |
| 20 | <1 | 2 | 7 | 0 | 0 | 0 | 15 | 136 | >180 | 0 | 12.7 | 21.9 |
| 25 | <1 | 1 | 2 | 0 | 0 | 0 | >180 | >180 | >180 | 24.7 | 25.7 | 27.6 |
| 30 | <1 | 5 | 13 | 0 | 0 | 0 | >180 | >180 | >180 | 25.1 | 27.5 | 29.2 |
| 35 | <1 | 77 | >180 | 0 | 0.3 | 0.8 | >180 | >180 | >180 | 31.2 | 31.7 | 32.5 |
| 40 | <1 | 73 | >180 | 0 | 0.5 | 1.3 | >180 | >180 | >180 | 32.2 | 33.4 | 34.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Delgado, E.J.; Barbosa-Póvoa, A.P.; Antunes, A.P. Intermodal Terminal Planning under Decentralized Management: Optimization Model for Rail-Road Terminals and Application to Portugal. Future Transp. 2021, 1, 533-558. https://doi.org/10.3390/futuretransp1030028
Delgado EJ, Barbosa-Póvoa AP, Antunes AP. Intermodal Terminal Planning under Decentralized Management: Optimization Model for Rail-Road Terminals and Application to Portugal. Future Transportation. 2021; 1(3):533-558. https://doi.org/10.3390/futuretransp1030028
Chicago/Turabian StyleDelgado, Erwin J., Ana Paula Barbosa-Póvoa, and António Pais Antunes. 2021. "Intermodal Terminal Planning under Decentralized Management: Optimization Model for Rail-Road Terminals and Application to Portugal" Future Transportation 1, no. 3: 533-558. https://doi.org/10.3390/futuretransp1030028
APA StyleDelgado, E. J., Barbosa-Póvoa, A. P., & Antunes, A. P. (2021). Intermodal Terminal Planning under Decentralized Management: Optimization Model for Rail-Road Terminals and Application to Portugal. Future Transportation, 1(3), 533-558. https://doi.org/10.3390/futuretransp1030028






