Numerical Investigations on Heat and Mass Transport in Passive Solar Evaporators with Non-Uniform Surface Temperature
Abstract
1. Introduction
2. Mathematical Formulation
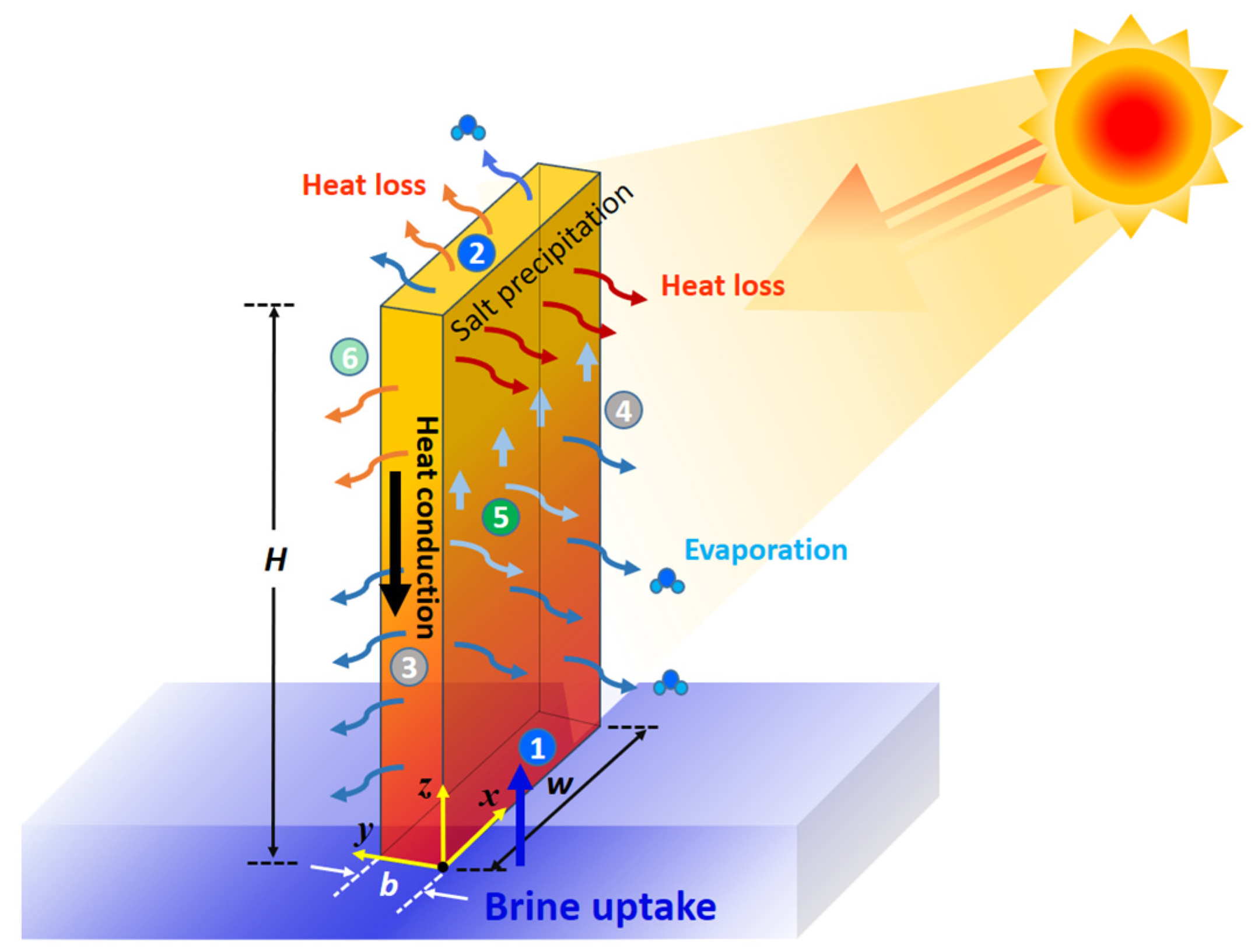
2.1. Physical Model and Simulation Domain
2.2. Evaporative Crystallisation
2.3. Governing Equations
2.4. Initial and Boundary Conditions
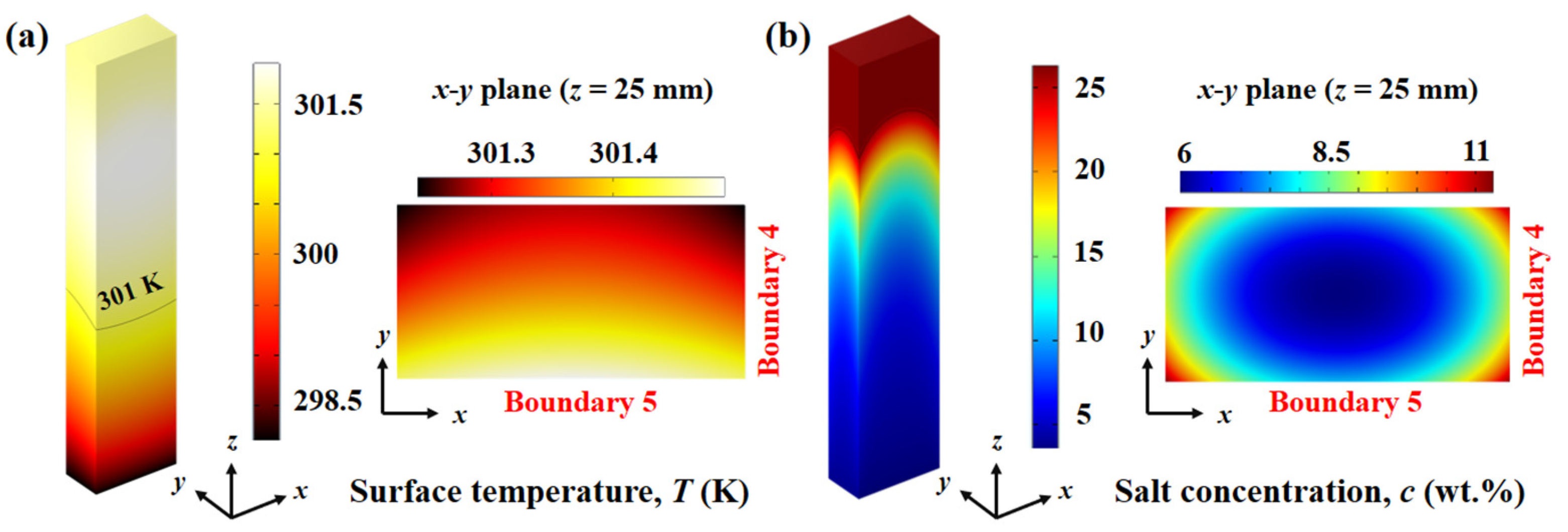
2.5. Model Validation
3. Results and Discussion
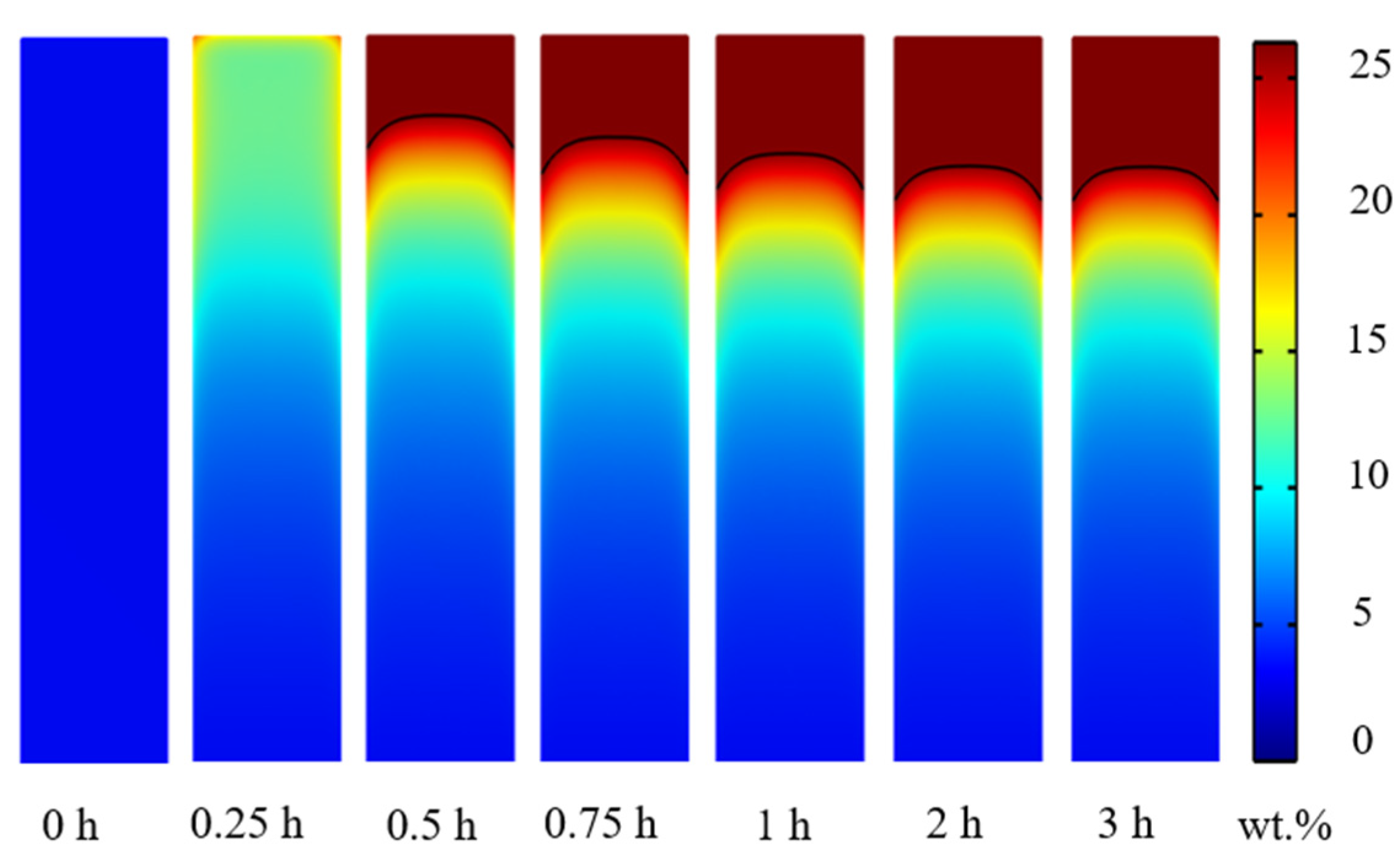
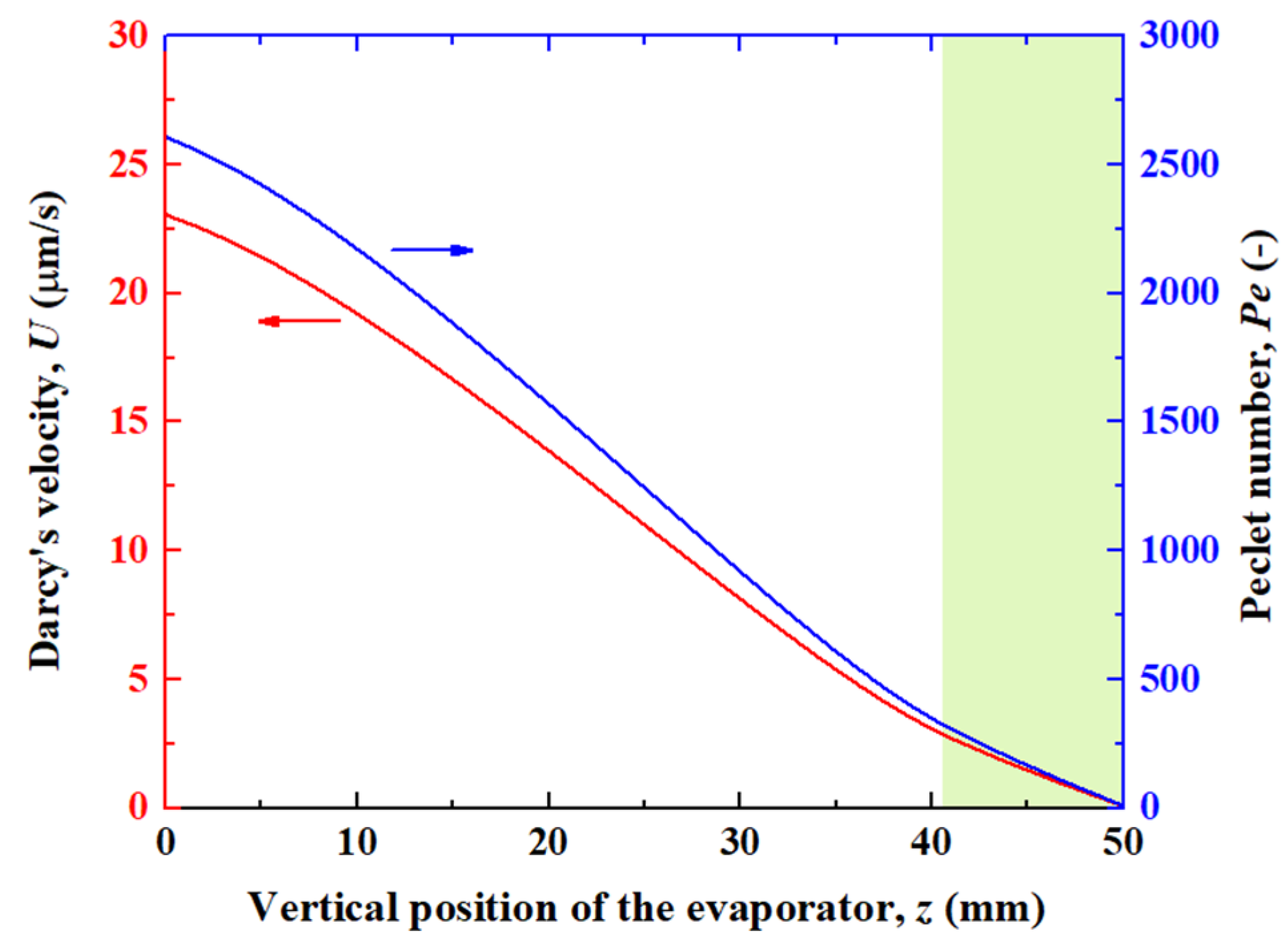
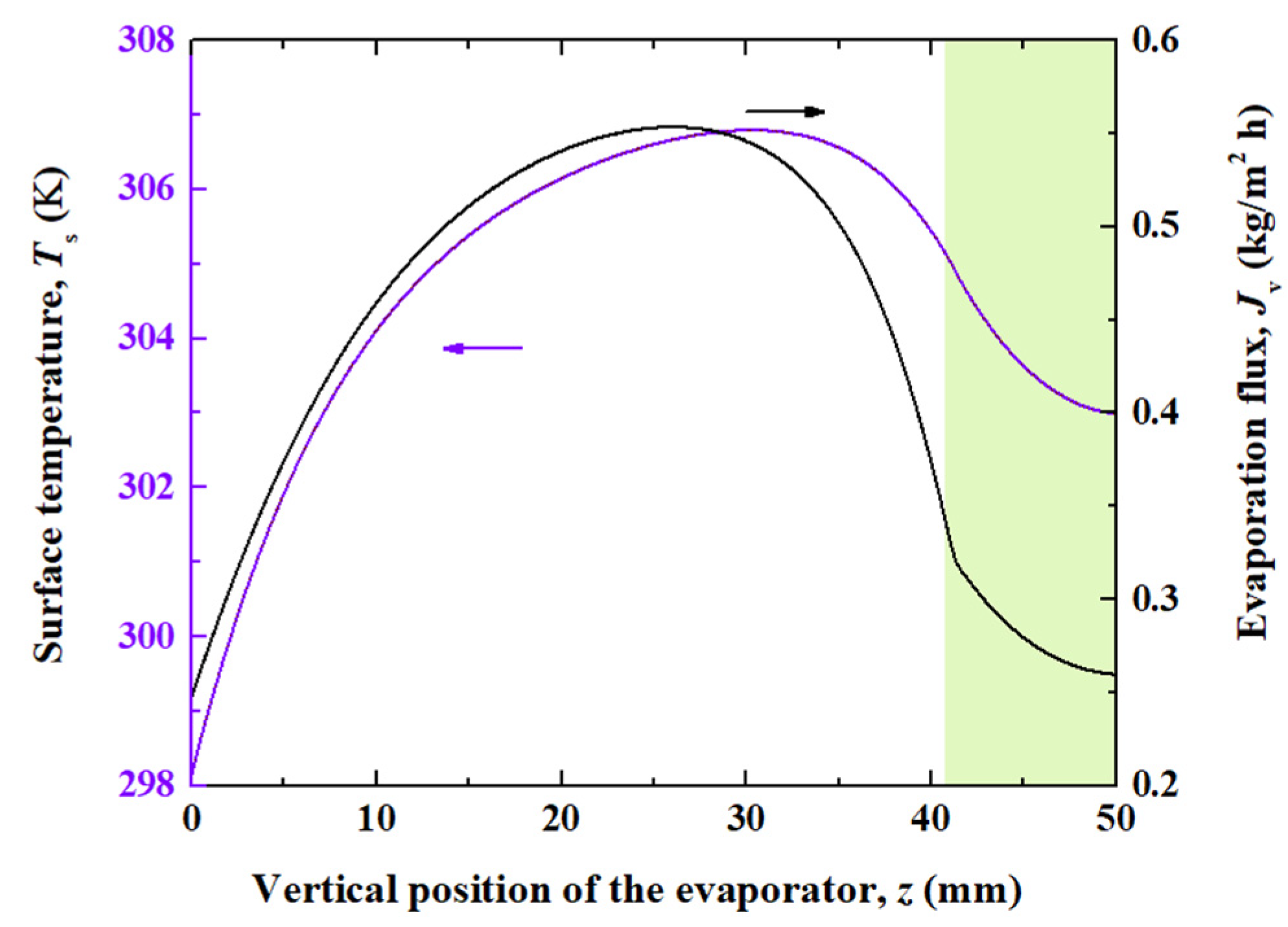
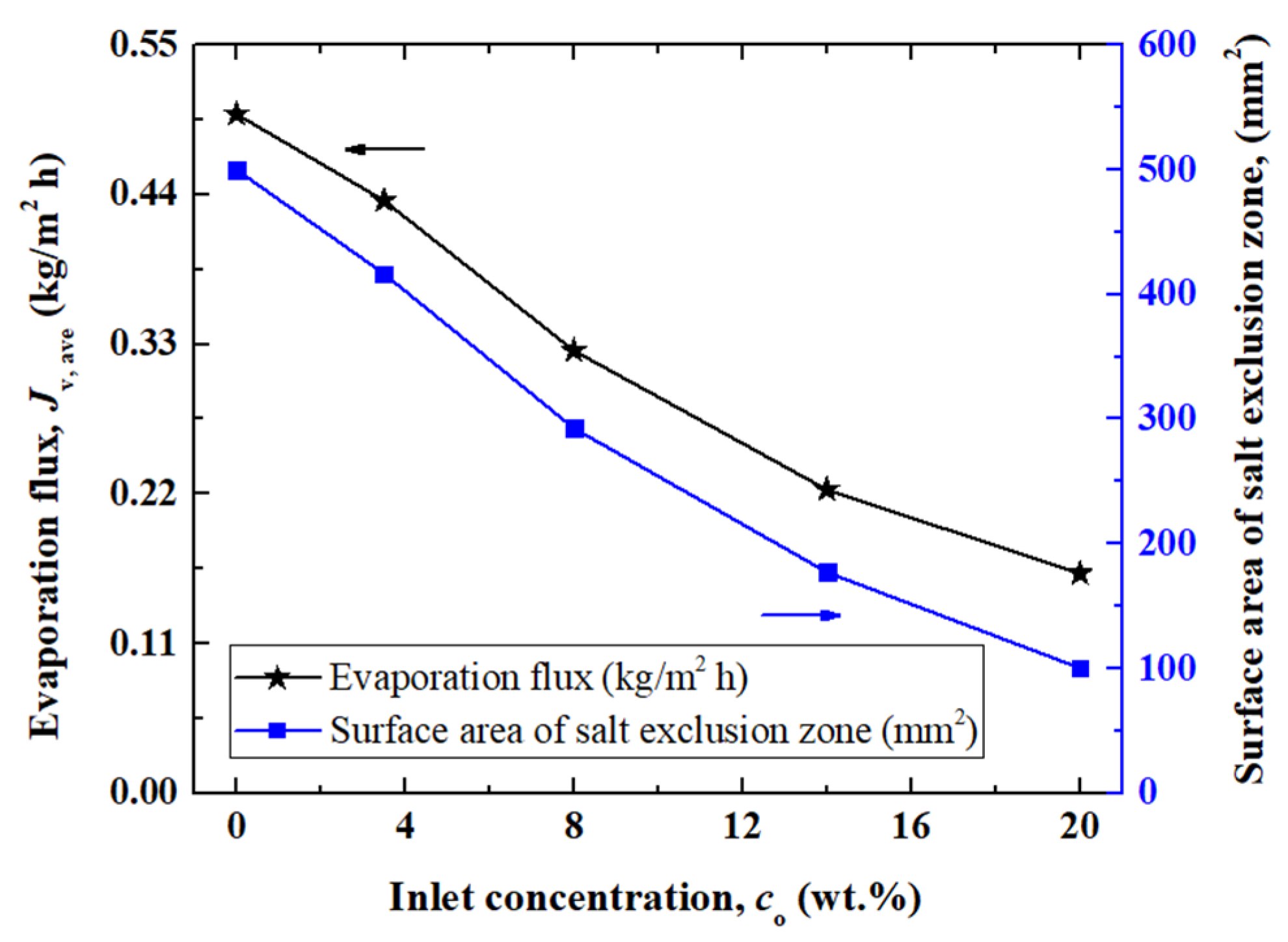
3.1. Effect of the Salt Concentration on Evaporation Performance
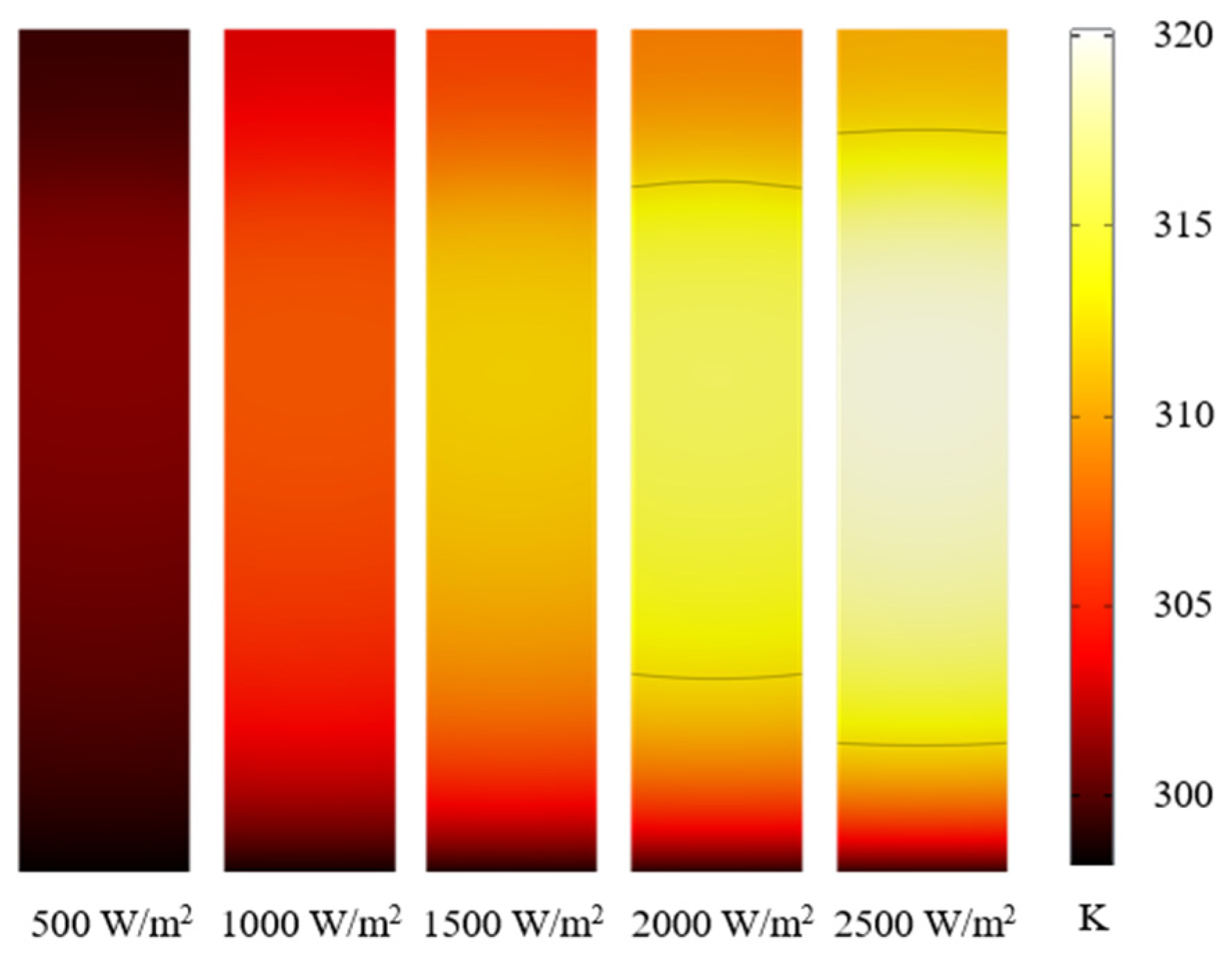
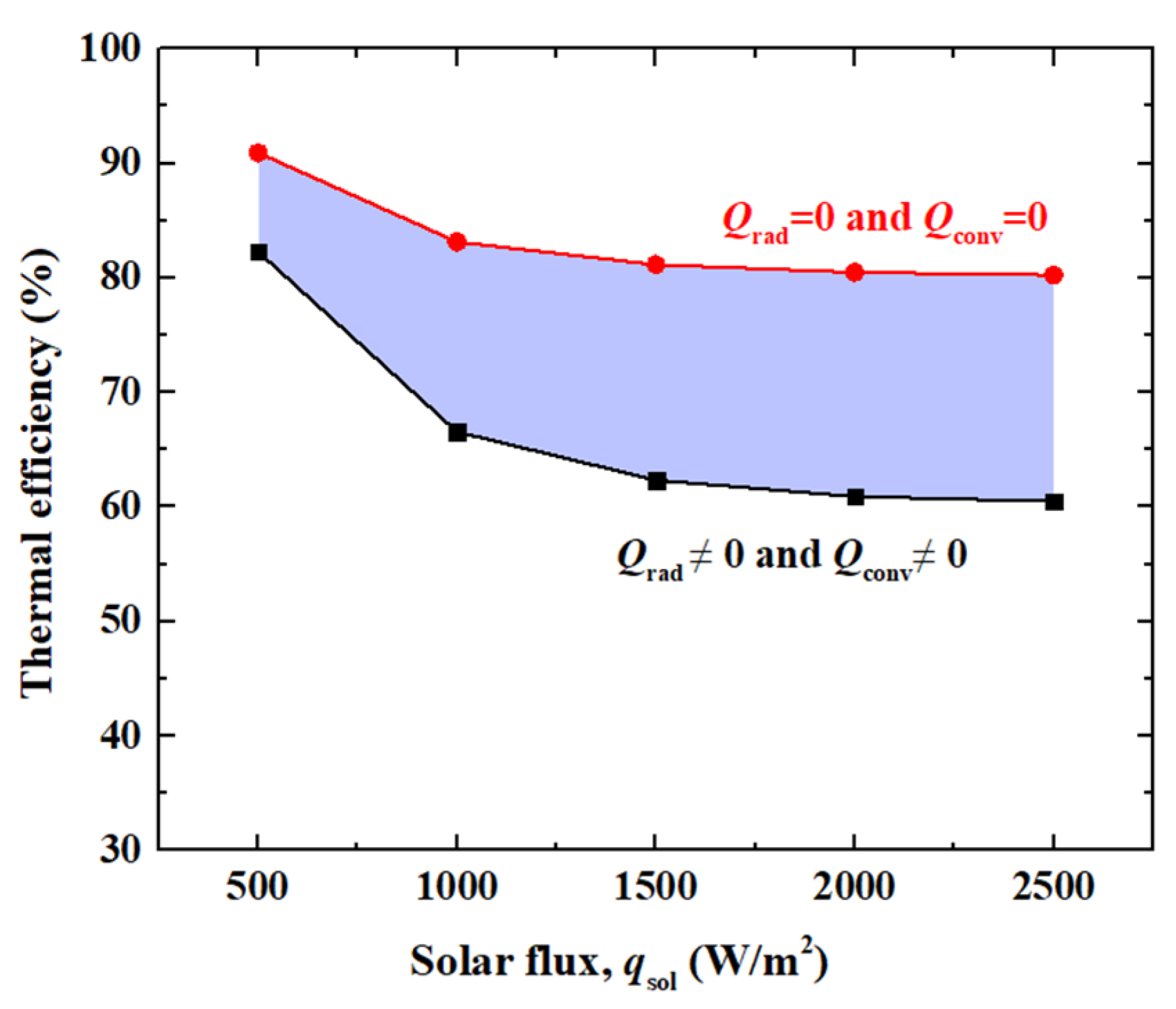
3.2. Effect of Solar Flux on Evaporation Performance
3.3. Effect of Evaporator’s Shape on Evaporation Performance
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hayes, O.R.; Ibrahim, A.A.; Adly, M.S.; Samra, S.E.; Ouf, A.M.A.; El-Hakam, S.A.; Ahmed, A.I. Solar-driven seawater desalination via plasmonic hybrid MOF/polymer and its antibacterial activity. RSC Adv. 2023, 13, 18525–18537. [Google Scholar] [CrossRef]
- Liu, J.; Yang, H.; Gosling, S.N.; Kummu, M.; Flörke, M.; Pfister, S.; Hanasaki, N.; Wada, Y.; Zhang, X.; Zheng, C.; et al. Water scarcity assessments in the past, present, and future. Earth’s Future 2017, 5, 545–559. [Google Scholar] [CrossRef]
- Abdelsalam, M.A.; Sajjad, M.; Raza, A.; AlMarzooqi, F.; Zhang, T. Sustainable biomimetic solar distillation with edge crystallization for passive salt collection and zero brine discharge. Nat. Commun. 2024, 15, 874. [Google Scholar] [CrossRef] [PubMed]
- Panagopoulos, A.; Haralambous, K.-J. Environmental impacts of desalination and brine treatment—Challenges and mitigation measures. Mar. Pollut. Bull. 2020, 161, 111773. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Xu, Z.; Zhao, L.; Bhatia, B.; Zhong, Y.; Gong, S.; Wang, E.N. Passive, high-efficiency thermally-localized solar desalination. Energy Environ. Sci. 2021, 14, 1771–1793. [Google Scholar] [CrossRef]
- Alzaben, H.; Fraser, R. Energy and Exergy Analyses Applied to a Crop Plant System. Thermo 2025, 5, 3. [Google Scholar] [CrossRef]
- Chen, F.; Cheng, Z.; Jiang, L.; Dong, Z. Capillary Wicking on Heliamphora minor-Mimicking Mesoscopic Trichomes Array. Biomimetics 2024, 9, 102. [Google Scholar] [CrossRef]
- Hu, X.; Hu, C.; Chen, S.; He, Y.; Li, L.; Tang, D. Biomimetic porous wick with efficient fluid transport and excellent heat transfer for loop heat pipes. Appl. Therm. Eng. 2024, 249, 123377. [Google Scholar] [CrossRef]
- Meo, R.R.; Morciano, M. Investigating the potentials and limitations of capillary-fed vapor generators: A heat and mass transfer study. Int. Commun. Heat Mass Transf. 2022, 137, 106309. [Google Scholar] [CrossRef]
- Haile, N.; Sajjad, M.; Zhang, Y.; AlAmoodi, N.; AlMarzooqi, F.; Zhang, T. Pore-scale physics of ice melting within unconsolidated porous media revealed by non-destructive magnetic resonance characterization. Sci. Rep. 2024, 14, 5635. [Google Scholar] [CrossRef]
- Sibiryakov, B.; Leite, L.W.B.; Sibiriakov, E. Porosity, specific surface area and permeability in porous media. J. Appl. Geophys. 2021, 186, 104261. [Google Scholar] [CrossRef]
- Rabi’, A.M.; Radulovic, J.; Buick, J.M. Packed Bed Thermal Energy Storage System: Parametric Study. Thermo 2024, 4, 295–314. [Google Scholar] [CrossRef]
- Liu, K.; Zhang, W.; Cheng, H.; Luo, L.; Wang, B.; Mao, Z.; Sui, X.; Feng, X. A Nature-Inspired Monolithic Integrated Cellulose Aerogel-Based Evaporator for Efficient Solar Desalination. ACS Appl. Mater. Interfaces 2021, 13, 10612–10622. [Google Scholar] [CrossRef]
- Khajevand, M.; Azizian, S.; Jaleh, B. A bio-based 3D evaporator nanocomposite for highly efficient solar desalination. Sep. Purif. Technol. 2022, 284, 120278. [Google Scholar] [CrossRef]
- Liu, M.; Jin, X.-T.; Sun, S.-W.; Zhao, J.; Xue, C.; Luo, Y.-H. Modulating hydration chemistry of GO/CSH antibacterial hydrogel evaporator toward high-efficiency solar-driven interfacial desalination. Desalination 2025, 604, 118731. [Google Scholar] [CrossRef]
- Dong, Y.; Tan, Y.; Wang, K.; Cai, Y.; Li, J.; Sonne, C.; Li, C. Reviewing wood-based solar-driven interfacial evaporators for desalination. Water Res. 2022, 223, 119011. [Google Scholar] [CrossRef] [PubMed]
- Cheng, S.; Yu, Z.; Lin, Z.; Li, L.; Li, Y.; Mao, Z. A lotus leaf like vertical hierarchical solar vapor generator for stable and efficient evaporation of high-salinity brine. Chem. Eng. J. 2020, 401, 126108. [Google Scholar] [CrossRef]
- Ma, Z.; Jiang, L.; Cao, Y. 3D printing technology for the design of eco-friendly palisade solar evaporators for enhanced desalination and wastewater treatment. Desalination 2024, 586, 117880. [Google Scholar] [CrossRef]
- Sun, S.; Shi, C.; Kuang, Y.; Li, M.; Li, S.; Chan, H.; Zhang, S.; Chen, G.; Nilghaz, A.; Cao, R.; et al. 3D-printed solar evaporator with seashell ornamentation-inspired structure for zero liquid discharge desalination. Water Res. 2022, 226, 119279. [Google Scholar] [CrossRef]
- Jia, X.; Niu, Y.; Zhu, S.; He, H.; Yan, X. Recent Advances in Carbon-Based Interfacial Photothermal Converters for Seawater Desalination: A Review. C 2024, 10, 86. [Google Scholar] [CrossRef]
- Su, J.; Chen, G.; Liu, D.; Ma, C.; Xie, P.; Zhou, S.; Wang, S.; Li, X.; Luo, H. Novel Ultrathin Quasi-Optical Microcavity-Selective Absorber Based on Ti@a-C Cermet for Solar-Thermal Conversion. ACS Photonics 2024, 11, 2637–2649. [Google Scholar] [CrossRef]
- Wang, Z.; Horseman, T.; Straub, A.P.; Yip, N.Y.; Li, D.; Elimelech, M.; Lin, S. Pathways and challenges for efficient solar-thermal desalination. Sci. Adv. 2019, 5, eaax0763. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Ye, Q.; Liang, X.; Xu, J.; Chang, C.; Song, C.; Shang, W.; Wu, J.; Tao, P.; Deng, T. Paper-based membranes on silicone floaters for efficient and fast solar-driven interfacial evaporation under one sun. J. Mater. Chem. A 2017, 5, 16359–16368. [Google Scholar] [CrossRef]
- Li, S.; Xi, Z.; Yu, L.; Yan, H.; Chen, M. Thermal Management of the Solar Evaporation Process. Langmuir 2023, 39, 8900–8907. [Google Scholar] [CrossRef]
- Yao, J.; Zhong, Q.; Zhang, J.; Zhao, J.; Wang, Z. Interfacial solar evaporation for zero liquid discharge desalination. Commun. Mater. 2024, 5, 103. [Google Scholar] [CrossRef]
- Wu, X.; Lu, Y.; Ren, X.; Wu, P.; Chu, D.; Yang, X.; Xu, H. Interfacial Solar Evaporation: From Fundamental Research to Applications. Adv. Mater. 2024, 36, 2313090. [Google Scholar] [CrossRef]
- Kim, J.; Hwang, J.; Kim, S.; Cho, S.H.; Choi, H.; Kim, H.-Y.; Lee, Y.S. Interfacial Solar Evaporator-Physical Principles and Fabrication Methods. Int. J. Precis. Eng. Manuf.-Green Technol. 2021, 8, 1347–1367. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, S.; Wang, J.; Lan, Q. Recent progress in solar-driven interfacial evaporation: Evaporators, condensers, applications and prospects. Desalination 2025, 597, 118356. [Google Scholar] [CrossRef]
- Fries, N.; Odic, K.; Conrath, M.; Dreyer, M. The effect of evaporation on the wicking of liquids into a metallic weave. J. Colloid Interface Sci. 2008, 321, 118–129. [Google Scholar] [CrossRef]
- Lazhar, R.; Najjari, M.; Prat, M. Combined wicking and evaporation of NaCl solution with efflorescence formation: The efflorescence exclusion zone. Phys. Fluids 2020, 32, 067106. [Google Scholar] [CrossRef]
- Meo, R.R.; Provenzano, M.; Morciano, M. Investigating the solute concentration in capillary-fed vapor generators: A heat and mass transfer study. Int. Commun. Heat Mass Transf. 2023, 148, 106998. [Google Scholar] [CrossRef]
- Sajjad, M.; Li, H.; Raza, A.; AlMarzooqi, F.; Zhang, T. Insights into capillary-driven motion of micro-particles interacting with advancing meniscus on a substrate. Soft Matter 2022, 18, 8894–8905. [Google Scholar] [CrossRef]
- Kestin, J.; Khalifa, H.E.; Correia, R.J. Tables of the dynamic and kinematic viscosity of aqueous NaCl solutions in the temperature range 20–150 °C and the pressure range 0.1–35 MPa. J. Phys. Chem. Ref. Data 1981, 10, 71–88. [Google Scholar] [CrossRef]
- Lewis, A.; Seckler, M.; Kramer, H.; Van Rosmalen, G. Industrial Crystallization: Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Hong, B.; Li, X.a.; Wang, L.; Li, L.; Xue, Q.; Meng, J. Using the Effective Void Ratio and Specific Surface Area in the Kozeny–Carman Equation to Predict the Hydraulic Conductivity of Loess. Water 2020, 12, 24. [Google Scholar] [CrossRef]
- Argo, T.F.; Guild, M.D.; Wilson, P.S.; Schröter, M.; Radin, C.; Swinney, H.L. Sound speed in water-saturated glass beads as a function of frequency and porosity. J. Acoust. Soc. Am. 2011, 129, EL101–EL107. [Google Scholar] [CrossRef] [PubMed]
- Partridge, G.P.; Lehman, D.M.; Huebner, R.S. Modeling the Reduction of Vapor Phase Emissions from Surface Soils Due to Soil Matrix Effects: Porosity/Tortuosity Concepts. J. Air Waste Manag. Assoc. 1999, 49, 412–423. [Google Scholar] [CrossRef] [PubMed]
- Millington, R.J.; Quirk, J.P. Permeability of porous solids. Trans. Faraday Soc. 1961, 57, 1200–1207. [Google Scholar] [CrossRef]
- Ma, X.; Jia, X.; Yao, G.; Wen, D. Double-Sided Suspending Evaporator with Top Water Supply for Concurrent Solar Evaporation and Salt Harvesting. ACS Sustain. Chem. Eng. 2022, 10, 12843–12851. [Google Scholar] [CrossRef]
- Xia, Y.; Hou, Q.; Jubaer, H.; Li, Y.; Kang, Y.; Yuan, S.; Liu, H.; Woo, M.W.; Zhang, L.; Gao, L.; et al. Spatially isolating salt crystallisation from water evaporation for continuous solar steam generation and salt harvesting. Energy Environ. Sci. 2019, 12, 1840–1847. [Google Scholar] [CrossRef]
- Thoai, D.N.; Hoai Ta, Q.T.; Truong, T.T.; Van Nam, H.; Van Vo, G. Review on the recent development and applications of three dimensional (3D) photothermal materials for solar evaporators. J. Clean. Prod. 2021, 293, 126122. [Google Scholar] [CrossRef]
- Försth, M.; Roos, A. Absorptivity and its dependence on heat source temperature and degree of thermal breakdown. Fire Mater. 2011, 35, 285–301. [Google Scholar] [CrossRef]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; Wiley: New York, NY, USA, 1996; Volume 6. [Google Scholar]
- Sharqawy, M.H.; Zubair, S.M. Thermophysical properties of seawater: A review of existing correlations and data. Desalination Water Treat. 2010, 16, 354–380. [Google Scholar] [CrossRef]
- Sajjad, M.; Abdelsalam, M.A.; Raza, A.; AlMarzooqi, F.; Zhang, T. Capillary pumping-evaporation modeling and experimental characterization of saline water transport for passive solar desalination. Int. J. Heat Mass Transf. 2024, 223, 125172. [Google Scholar] [CrossRef]
- Vaartstra, G.; Zhang, L.; Lu, Z.; Díaz-Marín, C.D.; Grossman, J.C.; Wang, E.N. Capillary-fed, thin film evaporation devices. J. Appl. Phys. 2020, 128, 130901. [Google Scholar] [CrossRef]
- Zhang, L.; Li, X.; Zhong, Y.; Leroy, A.; Xu, Z.; Zhao, L.; Wang, E.N. Highly efficient and salt rejecting solar evaporation via a wick-free confined water layer. Nat. Commun. 2022, 13, 849. [Google Scholar] [CrossRef]
- Shi, Y.; Zhang, C.; Li, R.; Zhuo, S.; Jin, Y.; Shi, L.; Hong, S.; Chang, J.; Ong, C.; Wang, P. Solar Evaporator with Controlled Salt Precipitation for Zero Liquid Discharge Desalination. Environ. Sci. Technol. 2018, 52, 11822–11830. [Google Scholar] [CrossRef]
- Li, Y.; Gao, T.; Yang, Z.; Chen, C.; Luo, W.; Song, J.; Hitz, E.; Jia, C.; Zhou, Y.; Liu, B.; et al. 3D-Printed, All-in-One Evaporator for High-Efficiency Solar Steam Generation under 1 Sun Illumination. Adv. Mater. 2017, 29, 1700981. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.; Bhatia, B.; Zhang, L.; Strobach, E.; Leroy, A.; Yadav, M.K.; Yang, S.; Cooper, T.A.; Weinstein, L.A.; Modi, A.; et al. A Passive High-Temperature High-Pressure Solar Steam Generator for Medical Sterilization. Joule 2020, 4, 2733–2745. [Google Scholar] [CrossRef]
- Liu, Z.; Song, H.; Ji, D.; Li, C.; Cheney, A.; Liu, Y.; Zhang, N.; Zeng, X.; Chen, B.; Gao, J.; et al. Extremely Cost-Effective and Efficient Solar Vapor Generation under Nonconcentrated Illumination Using Thermally Isolated Black Paper. Glob. Chall. 2017, 1, 1600003. [Google Scholar] [CrossRef]




| Parameter | Symbol/ Equation | Value(s) |
|---|---|---|
| Width of the evaporator | w | 10 mm |
| Thickness of the evaporator | b | 0.5, 5 mm |
| Height of the evaporator | H | 50 mm |
| Solar area of the evaporator | Asol = wH | 500 mm2 |
| Cross-sectional area of the evaporator | Ac = bw | 5, 50 mm2 |
| Total surface area of the evaporator | Asurf = (2wH) + (2bH) + (bw) | 1055, 1550 mm2 |
| Evaporation area of the evaporator | Aevap = Asurf | 1055, 1550 mm2 |
| Permeability of the evaporator | 30 µm2 | |
| Volumetric porosity of the evaporator | ϕv | 0.4 |
| Surface porosity of the evaporator | ϕs | 1 |
| Diameter of the spherical particles | 175 µm | |
| Empirical parameter | δ | 0.004 m |
| Standard diffusion coefficient of NaCl | D | 1.5 × m2/s |
| Absorption coefficient of the evaporator | 0.97 | |
| Emissivity of the evaporator | ε | 0.97 |
| Stefan–Boltzmann constant | σ | 5.67 × W/m2 K4 |
| Thermal conductivity of porous matrix | 11.4 W/m K | |
| Specific heat capacity of porous matrix | 523 J/kg K | |
| Density of porous matrix | 4500 kg/m3 | |
| Gravitational acceleration | g | −9.81 m/s2 |
| Molar mass of NaCl | 58.44 g/mol | |
| Relative humidity of air | φ | 0.5 |
| Ambient temperature | Tamb | 298.15 K |
| Inlet temperature of the brine | Tin | 298.15 K |
| Inlet Concentration, co | zsat (Theoretical) | zsat (Simulation) |
|---|---|---|
| 3.5 wt.% | 44.9 mm | 43.7 mm |
| 10 wt.% | 34.4 mm | 33.5 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sajjad, M.; Zahid, M.; Qaisrani, M.A. Numerical Investigations on Heat and Mass Transport in Passive Solar Evaporators with Non-Uniform Surface Temperature. Thermo 2025, 5, 15. https://doi.org/10.3390/thermo5020015
Sajjad M, Zahid M, Qaisrani MA. Numerical Investigations on Heat and Mass Transport in Passive Solar Evaporators with Non-Uniform Surface Temperature. Thermo. 2025; 5(2):15. https://doi.org/10.3390/thermo5020015
Chicago/Turabian StyleSajjad, Muhammad, Muhammad Zahid, and Mumtaz A. Qaisrani. 2025. "Numerical Investigations on Heat and Mass Transport in Passive Solar Evaporators with Non-Uniform Surface Temperature" Thermo 5, no. 2: 15. https://doi.org/10.3390/thermo5020015
APA StyleSajjad, M., Zahid, M., & Qaisrani, M. A. (2025). Numerical Investigations on Heat and Mass Transport in Passive Solar Evaporators with Non-Uniform Surface Temperature. Thermo, 5(2), 15. https://doi.org/10.3390/thermo5020015






