Dynamic Character of Thermal Analysis Where Thermal Inertia Is a Real and Not Negligible Effect Influencing the Evaluation of Non-Isothermal Kinetics: A Review
Abstract
1. Introduction
2. Thermal Inertia and Newton’s Law of Cooling
3. Historical Kinetics by Thermal Analysis
4. Physical Meaning of the Phenomenon Called Thermal Inertia and Reaction Kinetics by Thermal Analysis
5. Impact of Thermal Inertia in Thermal Analysis and Calorimetry
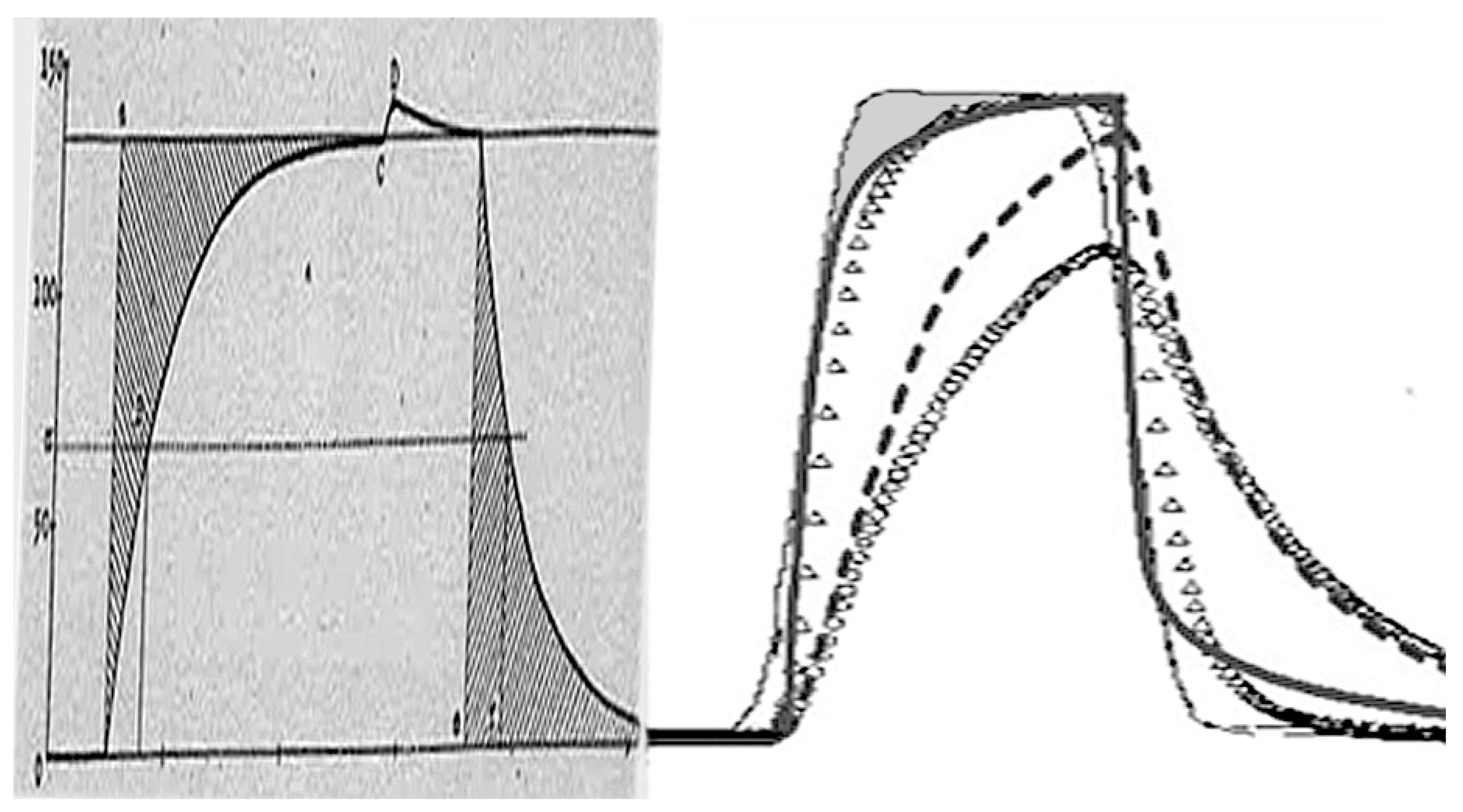
6. DTA Equation and Thermal Inertia Effect in Kinetics
or ΔT = Rt [CS(dΔT/dt) − ΔH(dα/dt)]
7. Discussion and Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Šesták, J.J.; Mackenzie, R.C. Heat/fire concept and its journey from prehistoric time into the third millennium. J. Therm. Anal. Calorim. 2001, 64, 129–147. [Google Scholar] [CrossRef]
- Mackenzie, R.C. History of thermal analysis. Thermochim. Acta 1984, 73, 251. [Google Scholar] [CrossRef]
- Lombardi, G.; Šesták, J. Ten years since Robert C. Mackenzie’s death: A tribute to the thermal analysis founder. J. Therm. Anal. Calorim. 2011, 105, 783–791. [Google Scholar] [CrossRef]
- Šesták, J. Thermodynamic basis for the theoretical description and correct interpretation of thermoanalytical experiments. Thermochim. Acta 1979, 28, 197–227. [Google Scholar] [CrossRef]
- Šesták, J.; Hubík, P.; Mareš, J.J. Historical roots and development of thermal analysis and calorimetry. In Glassy, Amorphous and Nano-Crystalline Materials; Šesták, J., Mareš, J.J., Hubík, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Šesták, J. Thermal science and analysis: History, terminology, development and the role of personalities. J. Therm. Anal. Calorim. 2013, 113, 1049–1054. [Google Scholar] [CrossRef]
- Šesták, J.; Mareš, J.J. From caloric to statmograph and polarography. J. Therm. Anal. Calorim. 2007, 88, 763–771. [Google Scholar] [CrossRef]
- Wang, J.; Bras, L.; Sivandran, G.; Knox, R.G. A simple method for the estimation of thermal inertia. Geophys. Res. Lett. 2010, 37, L05404. [Google Scholar] [CrossRef]
- Verbeke, S.; Audenaert, A. Thermal inertia in buildings: A review. Renew. Sustain. Energy Rev. 2018, 82, 2300–2318. [Google Scholar] [CrossRef]
- Danley, R.L. New heat flux DSC measurement technique. Thermochim. Acta 2003, 395, 201–208. [Google Scholar] [CrossRef]
- Šesták, J. Ignoring heat inertia impairs accuracy of determination of activation energy in thermal analysis. Int. J. Chem. Kinet. 2019, 51, 74–80. [Google Scholar] [CrossRef]
- Vyazovkin, S. How much is the accuracy of activation energy selected by ignoring thermal inertia? Int. J. Chem. Kinet. 2020, 52, 23–28. [Google Scholar] [CrossRef]
- Šesták, J. The evaluation of non-isothermal thermoanalytical kinetics is simplified without the description of heat transfers, such as thermal inertia, which is not negligible, as indicated by Vyazovkin. Int. J. Chem. Kinet. 2021, 53, 1050–1057. [Google Scholar] [CrossRef]
- Vyazovkin, S. When can the effect of thermal inertia be considered negligible? Int. J. Chem. Kinet. 2021. in print. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Burnham, A.K.; Favergeon, L.; Koga, N.; Moukhina, E.; Perez-Maqueda, L.A.; Sbirrazzuoli, N. ICTAC Kinetics Committee recommendations for analysis of multi-step kinetics: Review. Thermochim. Acta 2020, 689, 178597. [Google Scholar] [CrossRef]
- Piloyan, G.O. Introduction to the Theory of Thermal Analysis; Izd. Nauka: Moskva, Russia, 1964. (In Russian) [Google Scholar]
- Garn, P.D. Thermal Analysis of Investigation; Academic Press: New York, NY, USA, 1965. [Google Scholar]
- Sørensen, O.T.; Rouquerol, J. Sample Controlled Thermal Analysis; Kluwer: Dordrecht, The Netherlands, 2003. [Google Scholar]
- Málek, J.; Šesták, J.; Rouquerol, F.; Rouquerol, J.; Criado, J.M.; Ortega, A. Reaction Kinetics by Method of Constant Rate Thermal Analysis. J. Therm. Anal. 1992, 35, 111. [Google Scholar]
- Criado, J.M.; Pérez-Maqueda, L.A.; Koga, N. Sample Controlled Thermal Analysis (SCTA) as a Promising Tool for Kinetic Characterization of Solid-State Reaction and Controlled Material Synthesis. In Thermal Physics and Thermal Analysis; Šesták, J., Mareš, J.J., Eds.; Springer: Berlin/Heidelberg, Germany, 2017; Chapter 2; pp. 11–43. [Google Scholar]
- Gray, A.P. Simple Generalized Theory for Analysis of Dynamic Thermal Measurements. In Analytical Calorimetry; Porter, R.S., Johnson, J.F., Eds.; Plenum Press: New York, NY, NSA, 1968; Volume 1, pp. 210–218. [Google Scholar]
- Winterton, R.H.S. Early study of heat transfer: Newton and Fourier. Heat Trans. Eng. 2001, 22, 3–11. [Google Scholar] [CrossRef]
- Vyazovkin, S. A unified approach to kinetic processing of nonisothermal data. Int. J. Chem. Kinet. 1996, 28, 95–101. [Google Scholar] [CrossRef]
- Šesták, J. Thermophysical Properties of Solids: Theoretical Thermal Analysis; Elsevier: Amsterdam, The Netherlands, 1984; Russian translation by Mir, Moscow 1988. [Google Scholar]
- Šesták, J. Rationale and fallacy of thermoanalytical kinetic patterns. J. Therm. Anal. Calorim. 2012, 110, 5–16. [Google Scholar] [CrossRef]
- Koga, N.; Šesták, J.; Šimon, P. Some fundamental and historical aspects of phenomenological kinetics in solid-state studied by thermal analysis. In Thermal Analysis of Micro-, Nano- and Non-Crystalline Materials; Šesták, J., Šimon, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; Chapter 1; pp. 1–45. [Google Scholar]
- Vyazovkin, S.; Koga, N.; Schick, C. (Eds.) Handbook of Thermal Analysis and Calorimetry, Volume 6: Recent Advances, Techniques and Applications; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar]
- Šesták, J. Thermal Analysis and Thermodynamic Properties of Solids; Elsevier: Amsterdam, The Netherlands, 2021; ISBN 9780323855372. [Google Scholar]
- Holba, P.; Šesták, J. Heat inertia and its role in thermal analysis. J. Therm. Anal. Calorim. 2015, 121, 303–307. [Google Scholar] [CrossRef]
- Šesták, J. Are nonisothermal kinetics fearing historical Newton’s cooling law, or are just afraid of inbuilt complications due to undesirable thermal inertia? J. Therm. Anal. Calorim. 2018, 134, 1385–1393. [Google Scholar] [CrossRef]
- Davidzon, M.J. Newton’s cooling law and its interpretation. Int. J. Heat Mass Trans. 2012, 55, 5397–5402. [Google Scholar] [CrossRef]
- Šesták, J. The quandary aspects of non-isothermal kinetics beyond the ICTAC kinetic committee recommendations. Thermochim. Acta 2015, 611, 26–35. [Google Scholar] [CrossRef]
- Wang, Y.Z.; Song, X.N.; Liu, D. Asymptotic analysis for effect of thermal inertia on thermal behaviors. Acta Phys. Sin. 1013, 62, 2300–2330. [Google Scholar]
- Rubi, M.J.; Perez-Madrid, A. Inertial effects in non-equilibrium thermodynamics. Physica A 1999, 264, 492–502. [Google Scholar] [CrossRef][Green Version]
- Zemansky, M.V. Heat and Thermodynamics; McGraw-Hill/Kogakuscha: Tokyo, Japan, 1968. [Google Scholar]
- Cengel, Y.A. Introduction to Thermodynamics and Heat Transfer; McGraw-Hill: New York, NY, USA, 2009. [Google Scholar]
- Bejan, A. Advanced Engineering Thermodynamics; Wiley: New York, NY, USA, 2016. [Google Scholar]
- Vold, M.J. Differential Thermal Analysis. Anal. Chem. 1949, 21, 683–688. [Google Scholar] [CrossRef]
- Lerchner, J.A.; Wolf, G.; Wolf, J. Recent developments in integrated circuit calorimetry. J. Therm. Anal. Calorim. 1999, 57, 241. [Google Scholar] [CrossRef]
- Minakov, A.A.; Schick, C. Ultrafast thermal processing and nanocalorimetry at heating and cooling rates up to 1 MK/s. Rev. Sci. Instr. 2007, 78, 073902e10. [Google Scholar] [CrossRef] [PubMed]
- Minakov, A.A.; Schick, C. Dynamics of the temperature distribution in ultra-fast thin-film calorimeter sensors. Thermochim. Acta 2015, 603, 205–217. [Google Scholar] [CrossRef]
- Ding, J.; Yu, L.; Wang, X.; Xu, Q.; Yang, S.; Ye, S.; Jiang, J. A kinetic-based approach in accelerating rate calorimetry with the varying thermal inertia consideration. J. Therm. Anal. Calorim. 2020, 141, 783–796. [Google Scholar] [CrossRef]
- Šesták, J. Measuring “hotness”, should the sensor’s readings for rapid temperature changes be named “tempericity”? J. Therm. Anal. Calorim. 2016, 125, 991–999. [Google Scholar] [CrossRef]
- Holba, P. The Šesták’s proposal of term “tempericity” for non-equilibrium temperature and modified Tykodi’s thermal science classification with regards to the methods of thermal analysis. J. Therm. Anal. Calorim. 2017, 127, 2553–2559. [Google Scholar] [CrossRef]
- Šesták, J. Do we really know what temperature is: From Newton’s cooling law to an improved understanding of thermal analysis. J. Therm. Anal. Calorim. 2020, 142, 913–926. [Google Scholar] [CrossRef]
- Holman, S.W. Calorimetry: Methods of Cooling Correction. Proc. Am. Acad. Arts Sci. 1895, 31, 245–254. [Google Scholar] [CrossRef]
- He, H.; Dyck, M.F.; Horton, R.; Ren, T.; Bristow, K.L.; Si, B. Development and Application of the Heat Pulse Method for Physical Measurements. Rew. Geophys. 2017, 56, 567–620. [Google Scholar] [CrossRef]
- Svoboda, H.; Šesták, J. A new approach to DTA calibration by predetermined amount of Joule heat. In Proceedings of the 4th ICTA, Thermal Analysis, Budapest, Hungary, 8–13 July 1974; pp. 726–731. [Google Scholar]
- Kaisersberger, E.; Moukhina, E. Temperature dependence of the time constants for deconvolution of heat flow curves. Thermochim. Acta 2009, 492, 101–109. [Google Scholar]
- Barale, S.; Vincent, L.; Sauder, G.; Sbirrazzuoli, N. Deconvolution of calorimeter response from electrical signals for extracting kinetic data. Thermochim. Acta 2015, 615, 30–37. [Google Scholar] [CrossRef]
- Watanabe, H.; Morimoto, K.; Watanabe, M.; Kato, M.; Konashi, K. Multi-stepwise pulse calorimetry for accurate, efficient measurements of thermophysical properties over a wide temperature range. Thermochim. Acta 2020, 693, 178763. [Google Scholar] [CrossRef]
- Righini, F.; Bussolino, C.; Spišiak, J. Pulse calorimetry at high temperatures. Thermochim. Acta 2000, 347, 93–102. [Google Scholar] [CrossRef]
- Kočí, V.; Kočí, J.; Maděra, J.; Černý, R. Assessment of fast heat evolving processes using inverse analysis of calorimetric data. J. Heat Mass Trans. 2017, 115, 831–838. [Google Scholar] [CrossRef]
- Kossoy, A. Effect of thermal inertia-induced distortions of DSC data on the correctness of the kinetics evaluated. J. Therm. Anal. Calorim. 2021, 143, 599–608. [Google Scholar] [CrossRef]
- Borchardt, H.J.; Danniels, F. The Application of Differential Thermal Analysis to the Study of Reaction Kinetics. J. Am. Chem. Soc. 1957, 79, 41–46. [Google Scholar] [CrossRef]
- Kirsh, Y. On the kinetic analysis of DTA curves. Thermochim. Acta. 1988, 135, 97–101. [Google Scholar] [CrossRef]
- Holba, P.; Šesták, J.; Sedmidubský, D. Heat transfer and phase transition at DTA experiments. In Thermal Analysis of Micro-, Nano- and Non-Crystalline Materials; Šesták, J., Šimon, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; Chapter 5; pp. 99–134. [Google Scholar]
- Holba, P.; Šesták, J. The role of heat transfer and analysis ensuing heat inertia in thermal measurements and its impact to non-isothermal kinetics. In Thermal Analysis and Thermal Physics; Šesták, J., Hubík, P., Mareš, J.J., Eds.; Springer: Berlin/Heidelberg, Germany, 2017; Chapter 15; pp. 319–344. [Google Scholar]
- Kissinger, H.E. Reaction kinetics in differential thermal analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Holba, P.; Šesták, J. Imperfections of Kissinger evaluation method and crystallization kinetics. Glass Phys. Chem. 2014, 40, 486–495. [Google Scholar] [CrossRef]
- Šesták, J.; JHolba, P. Imperfections of Kissinger Evaluation Method and the Explanation of Crystallization Kinetics of Glasses and Melts. In Thermal Analysis and Thermal Physics; Šesták, J., Mareš, J.J., Eds.; Springer: Berlin/Heidelberg, Germany, 2017; Chapter 10; pp. 213–236. [Google Scholar]
- Farjas, J.; Sánchez-Rodriguez, D.; Eloussifi, H.; Roura, P. Thermal Gradients in Thermal Analysis Experiments. In Thermal Physics and Thermal Analysis; Šesták, J., Hubík, P., Mareš, J.J., Eds.; Springer: Berlin/Heidelberg, Germany, 2017; Chapter 16; pp. 345–362. [Google Scholar]
- Avramov, I.; Šesták, J. Generalized Kinetics of Overall Phase Transition Useful for Crystallization When Assuming Heat Inertia. Cond-mat.stat-mech 2019. Available online: https://arXiv.org &>physics&> arXiv1510.02250 (accessed on 8 October 2015).
- Šesták, J. Doubts about the popular Kissinger method of kinetic evaluation and its applicability for crystallization of cooling melts requiring equilibrium temperatures. J. Therm. Anal. Calorim. 2020, 142, 2095–2098. [Google Scholar] [CrossRef]
- Šimon, P. Single-step kinetics approximation employing nonarrhenian temperature function. J. Therm. Anal. Calorim. 2005, 79, 703–709. [Google Scholar]
- Šimon, P.; Thomas, P.; Dubaj, T.; Cibulková, Z. The mathematical incorrectness of the integral isoconversional methods in case of variable activation energy and the consequences. J. Therm. Anal. Calorim. 2014, 115, 853–859. [Google Scholar] [CrossRef]
- Galway, A.K. What theoretical and/or chemical significance is to be attached to the magnitude of an activation energy determined for solid-state reactions? J. Therm. Anal. Calorim. 2006, 86, 267–286. [Google Scholar] [CrossRef]
- Galwey, A.K.; Brown, M.E. Application of the Arrhenius equation to solid-state kinetics:can this be justified? Thermochim. Acta 2002, 386, 91–98. [Google Scholar] [CrossRef]
- Vyazovkin, S. On the phenomenon of variable activation energy for condensed phase reactions. New J. Chem. 2000, 24, 913–917. [Google Scholar] [CrossRef]
- Vyazovkin, S. A time to search: Finding the meaning of variable activation energy. Phys. Chem. Chem. Phys. 2016, 18, 18643–18656. [Google Scholar] [CrossRef]
- Vyazovkin, S. Kinetic effects of pressure on decomposition of solids. Inter. Rew. Phys. Chem. 2020, 39, 35–66. [Google Scholar] [CrossRef]
- Koga, N.; Sakai, Y.; Fukuda, M.; Hara, D.; Tanaka, Y. Universal Kinetics of the Thermal Decomposition of Synthetic Smithsonite over Different Atmospheric Conditions. J. Phys. Chem. C 2021, 125, 1384–1402. [Google Scholar] [CrossRef]
- Wu, X.; Chen, L.; Rao, G.; Chen, W.; Yin, R. Impact of inertia factors on the adiabatic decomposition of ethyl benzene. J. Chem. 2020, 2020, 9845894. [Google Scholar] [CrossRef]
- Heitman, J.L.; Horton, R.; Ren, T.; Nassar, I.N.; Davis, D.D. A test of coupled soil heat and water transfer prediction under transient boundary temperatures. Soil Sci. Soc. Am. J. 2008, 72, 1197–1207. [Google Scholar] [CrossRef]
- Smits, K.M.; Cihan, A.; Sakaki, T.; Illangasekare, T.H. Evaporation from soils under thermal boundary conditions: Experimental and modeling investigation to compare equilibrium- and nonequilibrium-based approaches. Water Resour. Res. 2011, 47, W05540. [Google Scholar] [CrossRef]
- Mianowski, A. Analysis of the Thermokinetics Under Dynamic Conditions by Relative Rate of Thermal Decomposition. J. Therm. Anal. Calorim. 2001, 63, 765–776. [Google Scholar] [CrossRef]
- Ferkl, P.; Hrma, P.; Kloužek, J.; Vernerova, M.; Kruger, A.; Pokorný, R. Model for batch-to-glass conversion: Coupling the heat transfer with conversion kinetics. J. Assian. Ceram. Soc. 2021. in print. [Google Scholar] [CrossRef]
- Patisson, F.; Galant, M.; Francois, T.; Ablitzer, D. A nonisothermal nonequilibrium and nonequimolar transient kinetic model for gas-solid reactions. Chem. Eng. Sci. 1998, 53, 697–708. [Google Scholar] [CrossRef][Green Version]
- Lamberti, G.; De Santis, F. Heat transfer and crystallization kinetics during fast cooling of polymer films. Heat Mass Transf. 2007, 43, 1143–1150. [Google Scholar] [CrossRef]
- Misyura, S.Y. Different modes of heat transfer and crystallization in a drop of NaCl solution: The influence of key factors on the crystallization rate and the heat transfer coefficient. Int. J. Therm. Sci. 2021, 159, 106602. [Google Scholar] [CrossRef]
- Yasnó, J.P.; Conconi, S.; Visintin, A. Non-isothermal reaction mechanism and kinetic analysis for the synthesis of monoclinic lithium zirconate during solid-state reaction. J. Anal. Sci. Technol. 2021, 12, 15–19. [Google Scholar] [CrossRef]
- Skrdla, P.J. Critical Review: Can we trust kinetic methods of thermal analysis? Analyst 2020, 145, 745–749. [Google Scholar] [CrossRef]
- Vyazovkin, S. Kissinger Method in Kinetics of Materials: Things to Beware and Be Aware of. Molecules 2020, 25, 2813. [Google Scholar] [CrossRef]
- Sapunov, V.N.; Saveljev, E.A.; Voronov Valtiner, M.; Linert, W. The basic theorem of temperature-dependent processes. Thermo 2021, 1, 4. [Google Scholar] [CrossRef]
- Zhang, X. Applications of Kinetic Methods in Thermal Analysis: A Review. Eng. Sci. 2021, 14, 1–13. [Google Scholar]
- Smarlak, M. On the inertia of heat. Eur. Phys. J. Plus. 2012, 127, 72–79. [Google Scholar] [CrossRef][Green Version]
- Mareš, J.J.; Šesták, J.; Hubík, P. Transport Constitutive Relations, Quantum Diffusion and Periodic Reactions. In Glassy, Amorphous and Nano-Crystalline Materials: Thermal Physics, Analysis, Structure and Properties; Šesták, J., Mareš, J., Hubík, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; Chapter 14; pp. 227–245. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Šesták, J. Dynamic Character of Thermal Analysis Where Thermal Inertia Is a Real and Not Negligible Effect Influencing the Evaluation of Non-Isothermal Kinetics: A Review. Thermo 2021, 1, 220-231. https://doi.org/10.3390/thermo1020015
Šesták J. Dynamic Character of Thermal Analysis Where Thermal Inertia Is a Real and Not Negligible Effect Influencing the Evaluation of Non-Isothermal Kinetics: A Review. Thermo. 2021; 1(2):220-231. https://doi.org/10.3390/thermo1020015
Chicago/Turabian StyleŠesták, Jaroslav. 2021. "Dynamic Character of Thermal Analysis Where Thermal Inertia Is a Real and Not Negligible Effect Influencing the Evaluation of Non-Isothermal Kinetics: A Review" Thermo 1, no. 2: 220-231. https://doi.org/10.3390/thermo1020015
APA StyleŠesták, J. (2021). Dynamic Character of Thermal Analysis Where Thermal Inertia Is a Real and Not Negligible Effect Influencing the Evaluation of Non-Isothermal Kinetics: A Review. Thermo, 1(2), 220-231. https://doi.org/10.3390/thermo1020015




