1. Introduction
In the Generation IV initiative, six advanced nuclear reactors were selected as promising candidates for a future nuclear fleet deployment. Among them, the Molten Salt Reactor (MSR) concept was chosen because of its high safety, reliability, and efficiency [
1]. These favourable properties are largely due to the use of a homogenous salt mixture that serves as fuel and primary coolant. In the decision process used to select which matrix salt serves the best, important factors including neutronics, melting point, redox potentials, reprocessing scheme, physicochemical properties and economics [
2] need to be evaluated carefully.
Many early studies focused on thermal breeder reactors, which included the necessity to use the lithium fluoride based MSR fuel matrix [
3]. However, recent developments raised the interest in reactor concepts that enable the use of alternative alkali and earth alkali salts [
4]. With those salt mixtures, it is possible to avoid the costly
7Li enrichment and also the in-reactor production of tritium from
6Li residuals (always present in small quantities after enrichment) by neutron capture [
5]. The next higher alkali fluorides, NaF and KF, might serve as alternative key matrix components of the MSR fuel to replace LiF. For this reason, the main focus of this study was to extend the existing JRCMSD thermodynamic database [
6] by the full assessment of the NaF-KF-UF
4 system. With this thermodynamic description, it is possible to predict relevant properties of a multi-component fuel. This includes the melting behaviour, which plays a key role for the safety assessment, but also in economic aspects of MSRs. It is possible to lower the melting temperature of a possible fuel salt mixture, by using a multicomponent fluoride salt [
7,
8,
9,
10]. These systems are complex and need a well-reviewed comprehensive database to be able to model their physico-chemical behaviour. To obtain the full thermodynamic description of the NaF-KF-UF
4 system, thermodynamic optimisations of all related subsystems are necessary.
The NaF-KF pseudo-binary phase diagram was investigated in detail by Kurnakow and Żmcżużnyj [
11], Dombrovskaya and Koloskova [
12], and Holm [
13]. Holm reported an eutectic point of X(KF) = 0.62 mol% [
13]. The thermodynamic assessment of the NaF-KF subsystem was presented in our earlier study [
14] and the data were further used in this work.
NaF-UF
4 phase transitions were first determined from thermal analysis while cooling by Barton et al. [
15]. Thoma et al. [
16] investigated the phase boundaries in the KF-UF
4 system by quenching after equilibration and identification of the phases by powder X-ray diffraction and optical microscopy. Thermal analysis and visual observations were used as supplementary methods.
A first study of the NaF-KF-UF
4 system was presented by Thoma et al. [
17] based on unpublished thermal analysis data from the period 1950–1958. From their data, the authors derived a preliminary phase diagram but stated that they could not list invariant points because their data did not define the phase relationships [
17].
In the current paper, an extensive experimental investigation of the two pseudo-binary phase diagrams for NaF-UF4 and KF-UF4, and a pseudo-ternary investigation of the NaF-KF-UF4 system were performed using Differential Scanning Calorimetry (DSC) and based on the obtained novel phase equilibrium data the full thermodynamic assessment of the NaF-KF-UF4 system was completed. For the NaF-UF4 system, 20 intermediate compositions were synthesised and measured, while for the KF-UF4 system, 21 intermediate compositions were evaluated. Furthermore, 12 different compositions from the pseudo-ternary field were measured for liquidus point determination and used to further optimise the calculated phase diagram.
4. Results
4.1. Phase Equilibria Determination of the Pseudo-Binary NaF-UF4 and KF-UF4 Mixtures
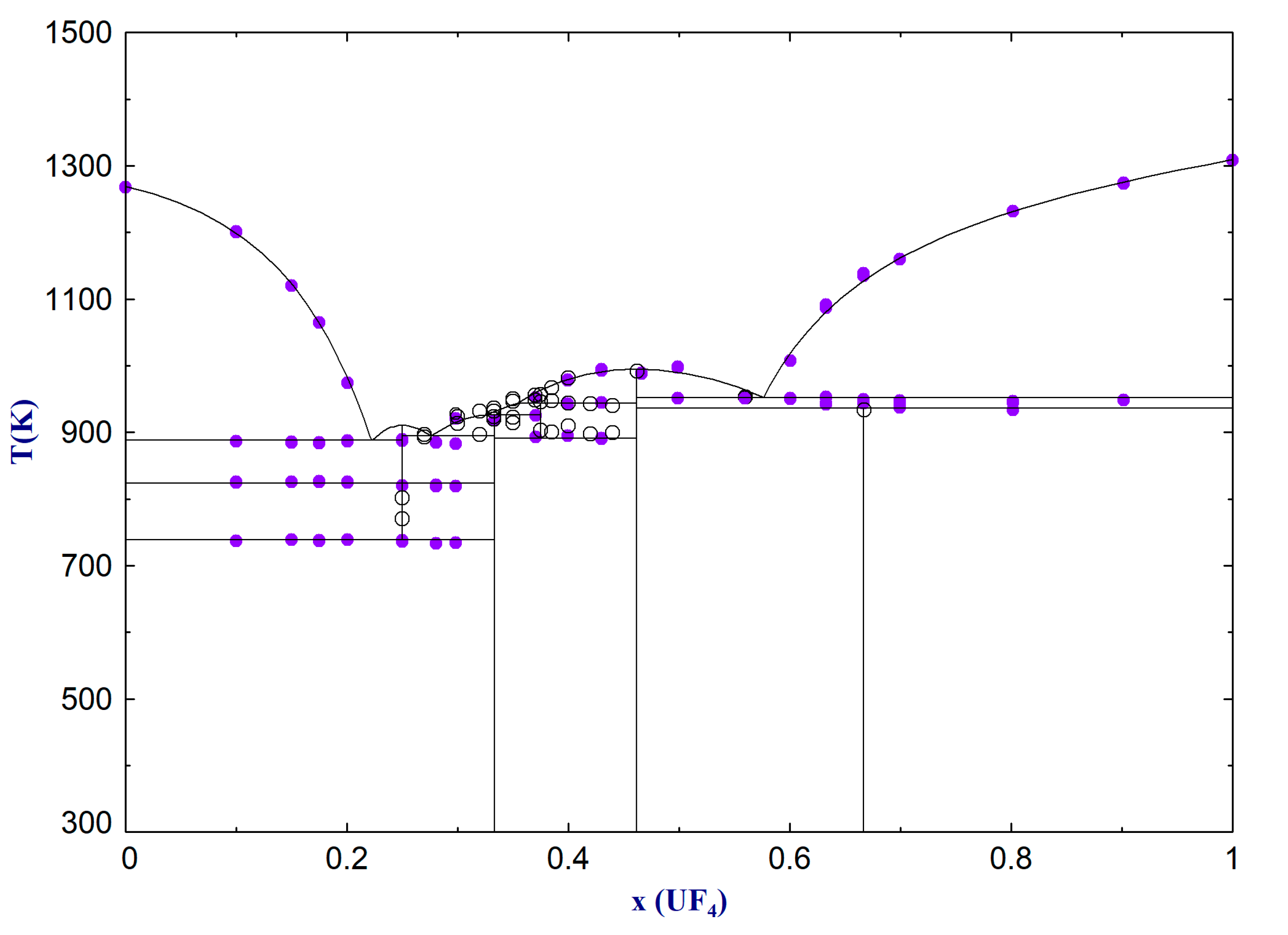
The phase equilibria temperatures experimentally obtained in this study by DSC for the NaF-UF
4 and KF-UF
4 system are presented in
Table 3 and
Table 4 and in
Figure 2 and
Figure 3, respectively. The type of equilibria were identified by the phase diagram assessment and comparison to earlier studies [
15,
16]. For comparison, the equilibrium temperatures calculated from the thermodynamic assessment performed in this study are shown in
Table 3 and
Table 4 as well. They demonstrate good agreement with the experimental data for the whole composition range.
4.2. Phase Equilibria Determination of the Pseudo-Ternary NaF-KF-UF4 Mixtures
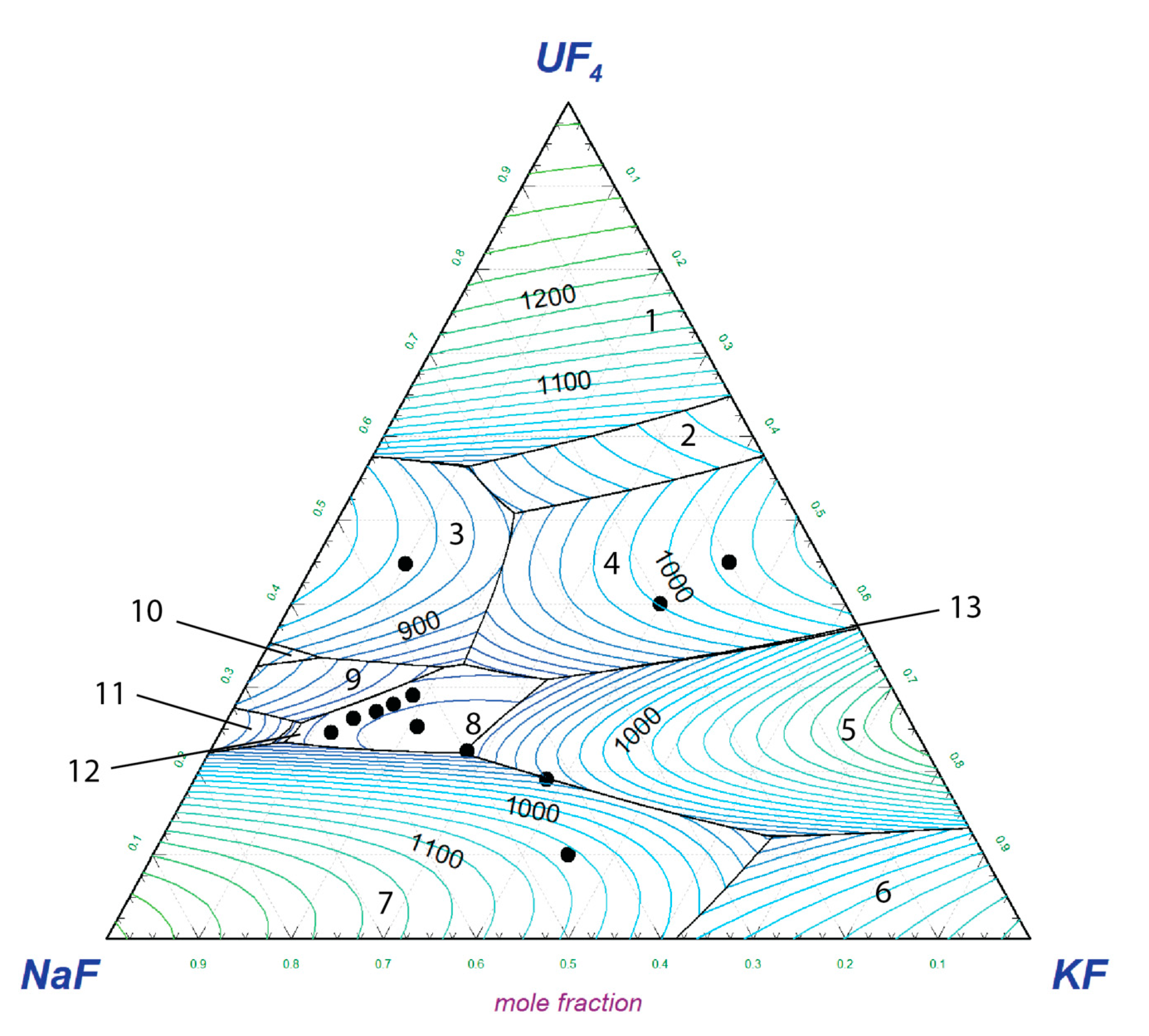
Twelve pseudo-ternary compositions were measured with DSC to evaluate the liquidus behaviour of the NaF-KF-UF
4 system. The obtained data of the liquidus points of all measured compositions are reported in
Table 5 and graphically represented in
Figure 4. Similar to the two studied pseudo-binary systems the calculated temperatures based on our thermodynamic assessment are provided for comparison. Although other types of equilibria (other than the here presented liquidus equilibria) were detected during DSC measurements, additional complementary analyses would be required to clearly identify the type of equilibria. The confirmation of the nature of the invariant equilibria for most of the measured transitions was identified for potential future work. As this study focuses on the melting point behaviour of the pseudo-ternary system, which is of great importance for the MSR systems, the obtained experimental data on the liquidus behaviour provides the right benchmark. Only for one selected composition (close to the suggested lowest pseudo-ternary eutectic point) all equilibria were investigated to clarify the solidus temperature. More details are given in the discussion section.
4.3. Phase Diagram Assessments of the NaF-UF4 and KF-UF4 Systems
Using the experimental equilibrium data obtained in this study and the data from the literature [
15,
16], the pseudo-binary NaF-UF
4 and KF-UF
4 phase diagrams were thermodynamically assessed. They are presented in
Figure 2 and
Figure 3, respectively. Overall, our experimental data agree well with most of the data presented in the literature, only a slight discrepancy was found for the equilibrium related to the formation of the K
2UF
6 compound. We decided to use our obtained data for the modelling of the KF-UF
4 system but to verify this decision, independent measurement of different research group would be useful.
Good agreement between the measured experimental data (purple solid circles in the figures) and the model is evident, indicating a maximum discrepancy of about 15 K on the liquidus, but with most of the liquidus points within the ±5 K range.
The NaF-UF4 system is characterised by 3 eutectic points and 2 peritectic points. The lowest eutectic is at a temperature of T = 888 K and a composition of about X(UF4) = 22.2 mol%. The other two eutectic points are found at T = 894 K with X(UF4) = 27.6 mol% and T = 952 K with X(UF4) = 57.6 mol%. In total, 5 intermediate phases stabilise in the system. Na7[U6F31] and the high temperature phase Na3[UF7] melt congruently at T = 995 K and T = 910 K, respectively. Na2[UF6] and the second high temperature phase Na5[U3F17] exhibit peritectic melting at T = 926 K and T = 943 K, respectively. Na[U2F9] decomposes into UF4 and Na7[U6F31] at 936 K.
In the KF-UF4 system, three eutectic and two peritectic points were identified. The lowest eutectic was found at T = 988 K with X(UF4)= 13.2 mol%, while the other eutectic points were calculated at T = 1026 K with X(UF4)= 37.4 mol% and T = 1019 K with X(UF4) = 57.7 mol%. Of four total intermediate compounds, K3[UF6] and K7[U6F31] show congruent melting points at T = 1217 K and T = 1068 K, respectively. The high temperature stoichiometric phase K2[UF6] decomposes at the peritectic point at 1028 K. The second peritectic point is at 1039 K where K[U2F9] decomposes into UF4 and liquid.
All calculated pseudo-binary phase equilibria of the NaF-UF
4 and KF-UF
4 systems and their exact compositions are summarised in
Table 6 and
Table 7, respectively.
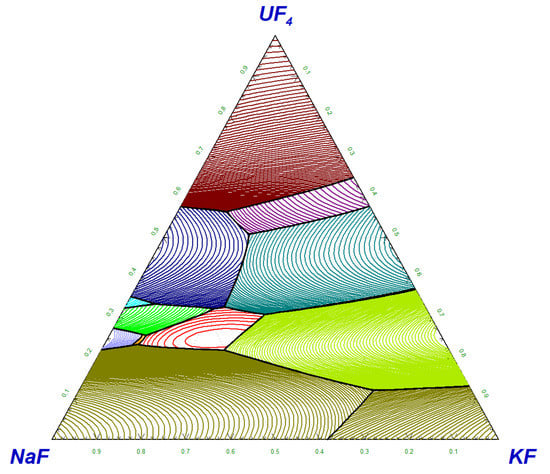
4.4. Phase Diagram Assessment of the NaF-KF-UF4 System
The pseudo-ternary NaF-KF-UF
4 system was thermodynamically assessed based on the thermodynamic assessments of the pseudo-binary sub-systems (NaF-KF, NaF-UF
4, and KF-UF
4) and the experimental data measured in this study. The liquidus projection, together with the indicated measured data, is shown in
Figure 5.
The calculated pseudo-ternary phase diagram contains 13 invariant equilibria and 13 phase fields of primary crystallisation. Two pseudo-ternary intermediate solid phases stabilise in the pseudo-ternary field; the equi-molar NaKUF4 phase and the Na2KUF7 phase. The latter phase was revealed by a XRD analysis performed in the current study.
The calculated lowest pseudo-ternary eutectic was found at 807 K and NaF-KF-UF
4 (68.9-7.6-23.5 mol%) composition. The temperature is in good agreement with the experimentally determined eutectic temperature of 811 K, illustrated below in
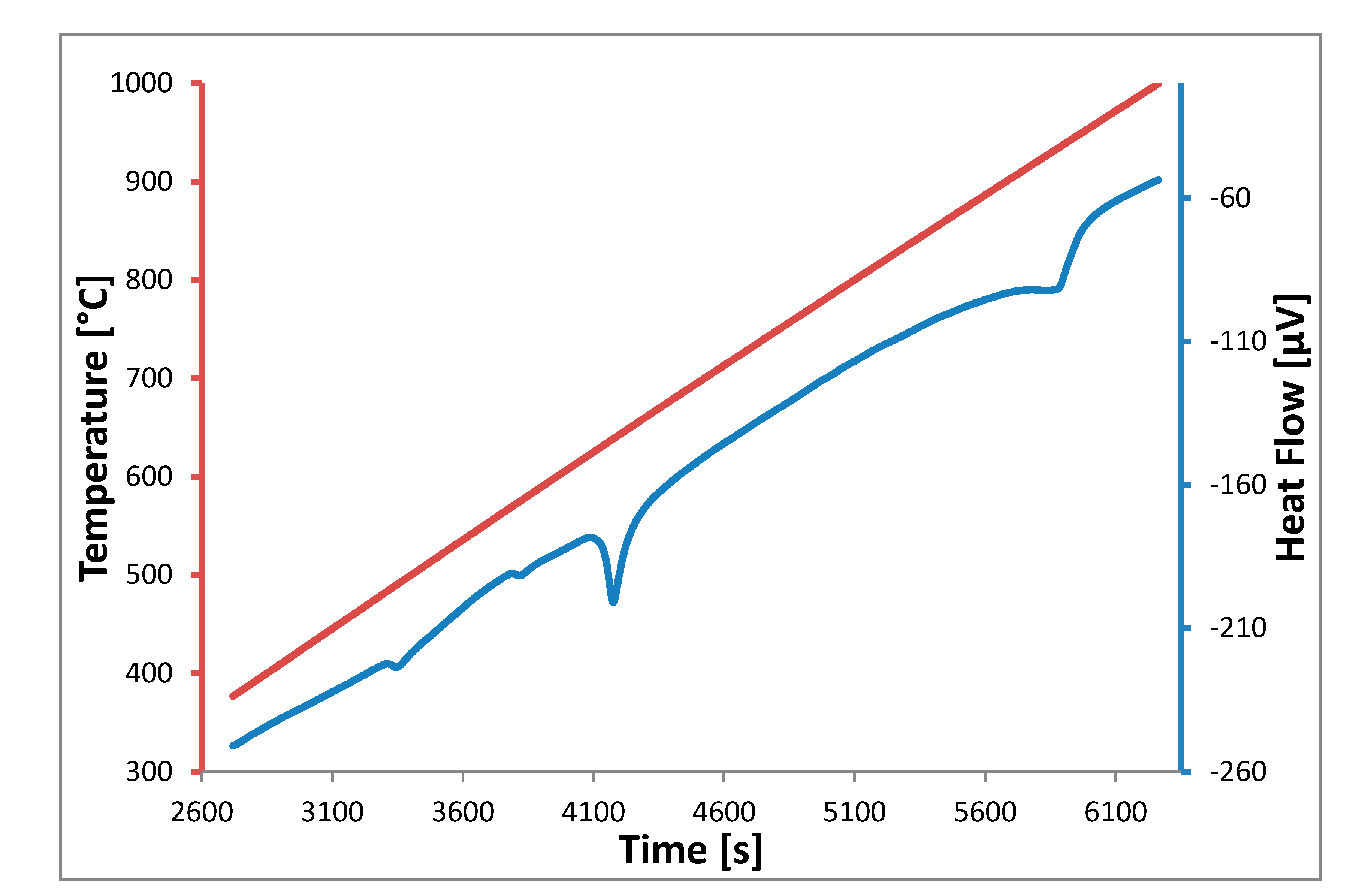
Figure 4. Although the shown example does not represent the exact composition of the eutectic point, the eutectic temperature corresponds to the onset point of the melting peak of the heat flow signal.
All calculated pseudo-ternary invariant equilibria are given in
Table 8, while the primary crystallisation phases are listed in the caption of
Figure 5.
5. Discussion
The experimental re-investigation and thermodynamic modelling of the pseudo-binary NaF-UF
4 and KF-UF
4 systems provided the basis for extrapolation to the NaF-KF-UF
4 system. Only small ternary excess Gibbs parameters of the liquid solution were used for the thermodynamic assessment. The calculated liquidus projection was correlated with 12 experimentally measured data points (highlighted in
Figure 5), which were further used for the optimisation of the pseudo-ternary system. The direct comparison of experimental and calculated liquidus temperatures is shown in
Table 5. It is clear that seven points agree within a 10 K discrepancy, four points are within 45 K error, and one point has a discrepancy of 60 K. When the point with the highest discrepancy is plotted against the liquidus projection of the phase diagram, it is revealed that it belongs to a phase field with a steep liquidus increase. Hence, the composition margin for both experimental and modelling results can likely be the main cause. In this region, a small change of composition has significant impact on the melting temperature. With this note, we conclude that the preliminary assessed pseudo-ternary system provides a good estimate on the liquidus propagation.
Although fairly good general agreement between the calculated phase diagram and the measured data has been achieved, still a few items were identified for potential future investigations. First of all, as already mentioned in
Section 3, we had no experimental evidence for the solid solution behaviour in the pseudo-ternary field, and therefore it was not considered in the current assessment. The presence of a solid solution might affect the liquidus surface of the pseudo-ternary field. More experimental data of the solid solution and its composition extension, with a primary focus on the phase analysis, is needed and we suggest this as a possible topic for future studies.
The same is true for the definition of stability limits of the pseudo-ternary intermediate compounds. Thoma et al. suggested that the NaKUF
6 intermediate compound was stable, but not many details are available on its stability range [
17]. The phase diagram suggested by Thoma et al. [
17] indicates a sub-liquidus stability limit (i.e., no congruent melting was observed); however the exact range is unknown. In the current study, we followed these suggestions and with novel experimental data measured in this study, we optimised the thermodynamic data of this intermediate compound leading to a stability limit of 714 K. Similarly to the solid solution, the need for more data on stability of this phase (or its presence) is suggested for future investigations.
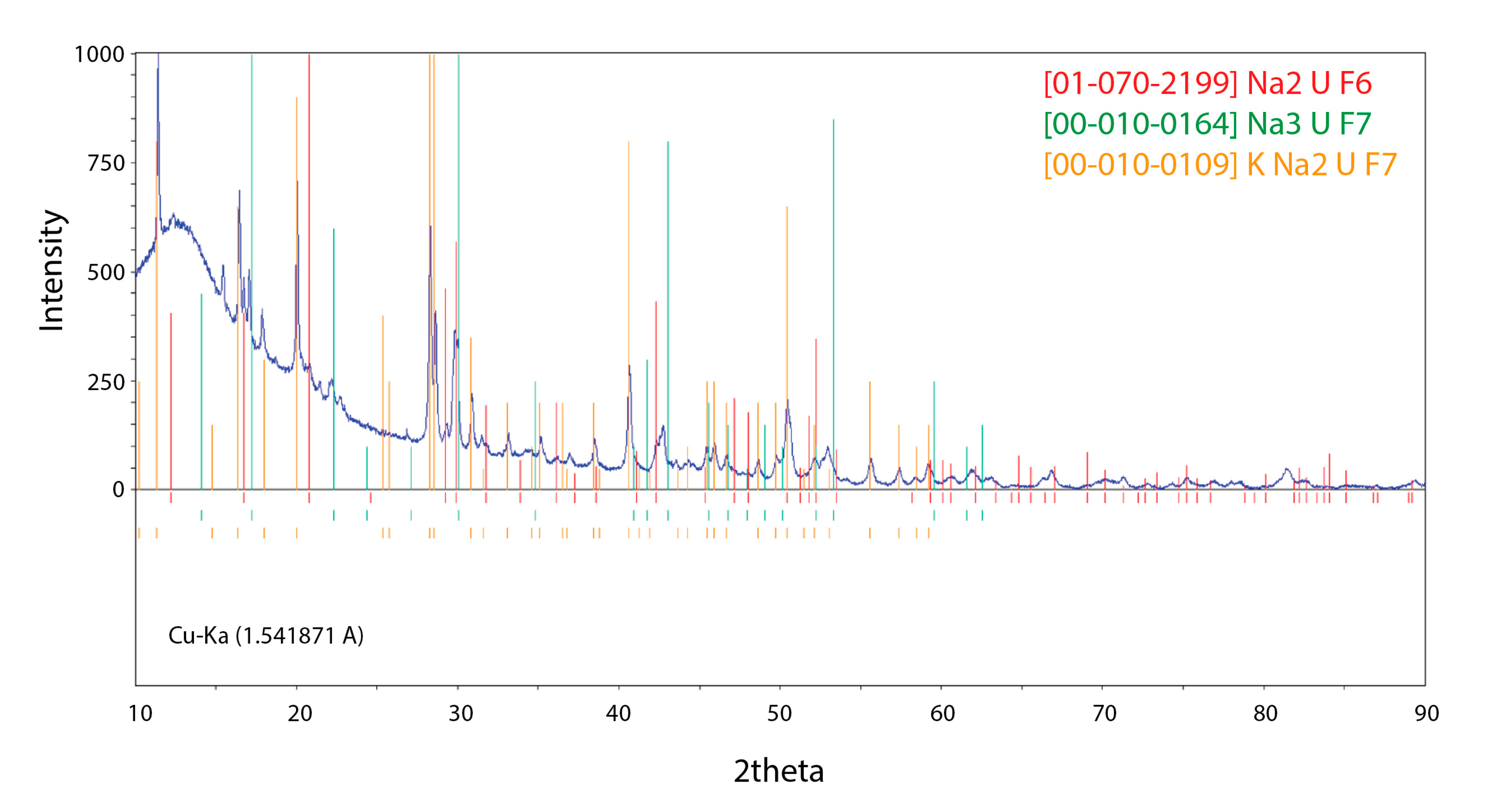
Furthermore, we performed XRD phase analyses of one selected composition from the mid-range region (namely the NaF-KF-UF
4 (52.3-18.7-29.0 mol%) mixture) using a sample that has been analysed by DSC earlier and cooled down to room temperature. The obtained diffractogram is shown in
Figure 6 and clearly indicates ternary equilibrium between Na
2UF
6, Na
3UF
7 and KNa
2UF
7. We took the evidence of the presence of the KNa
2UF
7 pseudo-ternary phase into consideration and optimised its thermodynamic parameters to fit the obtained DSC data of the measured composition from that crystallisation domain. This was a key point for the NaF-KF-UF
4 pseudo-ternary assessment, as only with the presence of the KNa
2UF
7 pseudo-ternary compound we could reach good agreement to the measured lowest pseudo-ternary eutectic temperature.
Comparing the currently assessed pseudo-ternary phase diagram with the one suggested by Thoma et al. [
17], generally good agreement among the phase field domains is obtained, but a slight discrepancy between the lowest melting point (the lowest pseudo-ternary eutectic) is observed. To explain the possible source of this discrepancy, we performed careful analyses of one of the DSC runs obtained for the close-to eutectic composition, in particular the NaF-KF-UF
4 (52.3-18.7-29.0 mol%) composition. As shown on the heat flow signal in
Figure 4, at 744.15 K (471 °C), a first transition occurs, and we consider it is due to the formation of the UNa
3F
7 compound. The thermodynamic model supports this statement, and the temperature is in close agreement with the UNa
3F
7 formation temperature measured at 738 K, as depicted in the NaF-UF
4 pseudo-binary phase diagram. We consider that this could be the source of the disagreement for the lowest melting temperature between the calculated pseudo-ternary phase diagram presented in this study (i.e.,
Figure 5) and the earlier suggested phase diagram by Thoma et al. [
17] in which the lowest pseudo-ternary eutectic was estimated to be between 450 and 500 °C (i.e., 723–773 K, deduced from their figure). Since only few details were given by the authors and all data were based solely on thermal analysis, we think that the lowest melting point identified earlier could be the detected solid phase transition instead. Therefore, we suggest the eutectic temperature as the onset point of the second peak on the heat flow signal represented in
Figure 4, thus 811 K (538 °C).
6. Conclusions
In this work, a substantial amount of novel experimental data on the phase equilibria of the NaF-UF4, KF-UF4 and NaF-KF-UF4 systems was obtained. Based on these data, new pseudo-binary thermodynamic assessments of the NaF-UF4 and KF-UF4 systems were performed, and the optimised Gibbs energy models of the relevant phases were presented. Excellent agreement between the pseudo-binary phase equilibria data and the calculated phase diagram was achieved for both systems.
Furthermore, the full NaF-KF-UF4 pseudo-ternary system was thermodynamically described. The lowest pseudo-ternary eutectic was found at 807 K (experimentally confirmed at 811 ± 5 K). Although we could not experimentally identify the exact lowest eutectic composition, the thermodynamic assessment points towards a composition of 68.9-7.6-23.5 mol% NaF-KF-UF4. This temperature is fairly low, thus NaF and KF might serve as alternative components to LiF. Further research on this particular system is recommended to confirm the composition of the lowest eutectic point and to fully determine the stability range of pseudo-ternary solid solutions and pseudo-ternary intermediate phases.
With the few identified issues left for further research, the thermodynamic assessment of the full NaF-KF-UF4 system presented in this study is a valuable contribution for further extension of the JRCMSD thermodynamic database. It describes well most of the phase field regions and provides a detailed experimental investigation of the surrounding region of the lowest melting point. The JRCMSD thermodynamic database, with this extension, can serve, e.g., nuclear regulators for their thermodynamic properties predictions needed for safety assessments. At the same time, it may be consulted by companies developing molten salt reactor technologies for the fuel composition optimisation of their multi-component fuel mixture.