A Review of the Electrical Conductivity Test Methods for Conductive Fabrics
Abstract
1. Introduction
2. Electrical Properties of Fabrics
2.1. Key Concepts about Electrical Conductivity
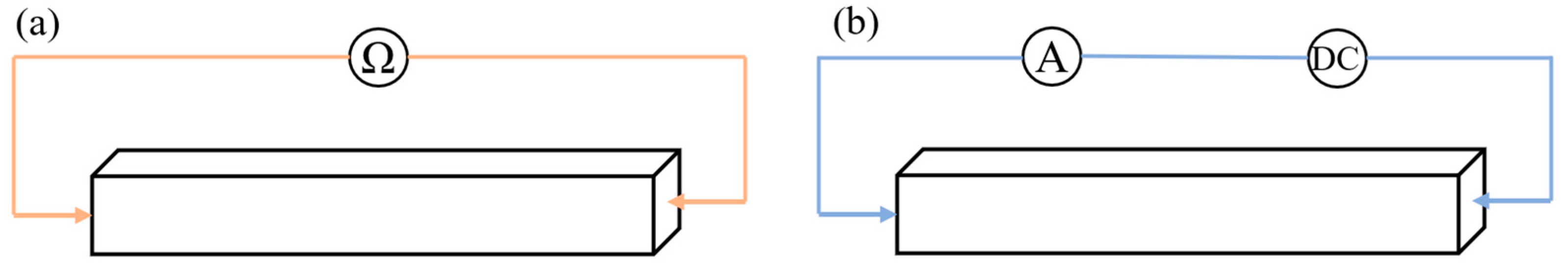
2.2. Resistance and Resistivity in Conductive Textiles
3. Revision of Current Methods Used to Test Electrical Properties of Fabrics
3.1. Contact Methods
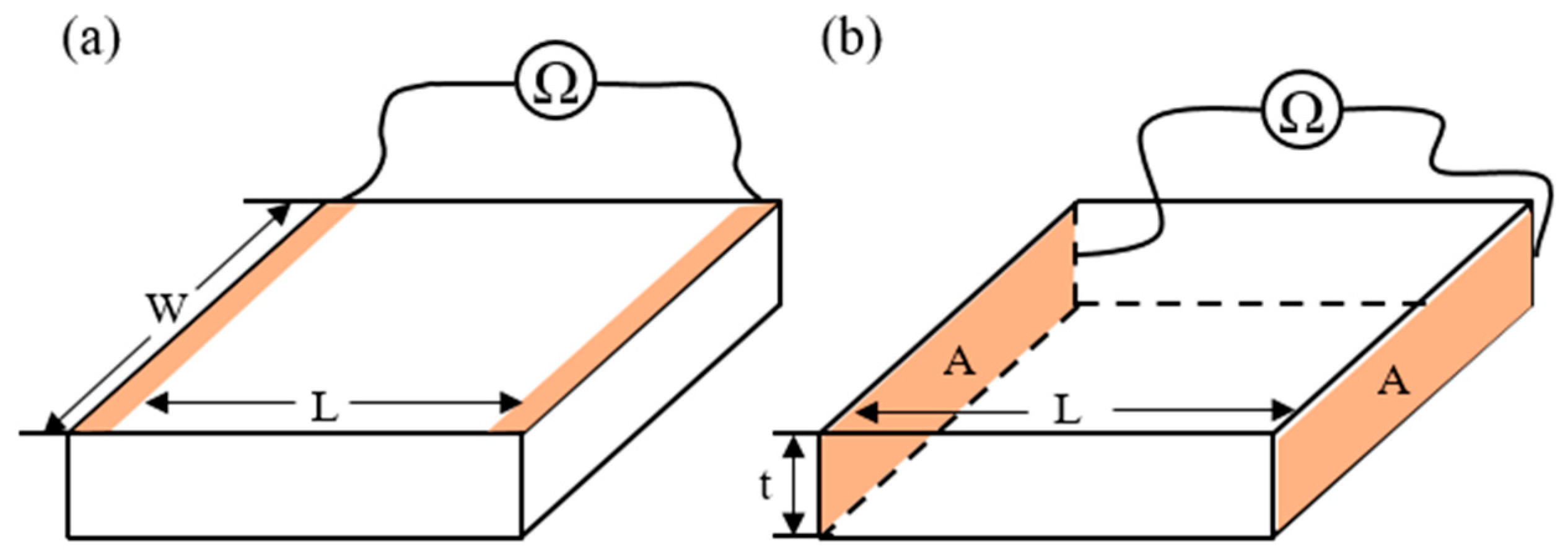
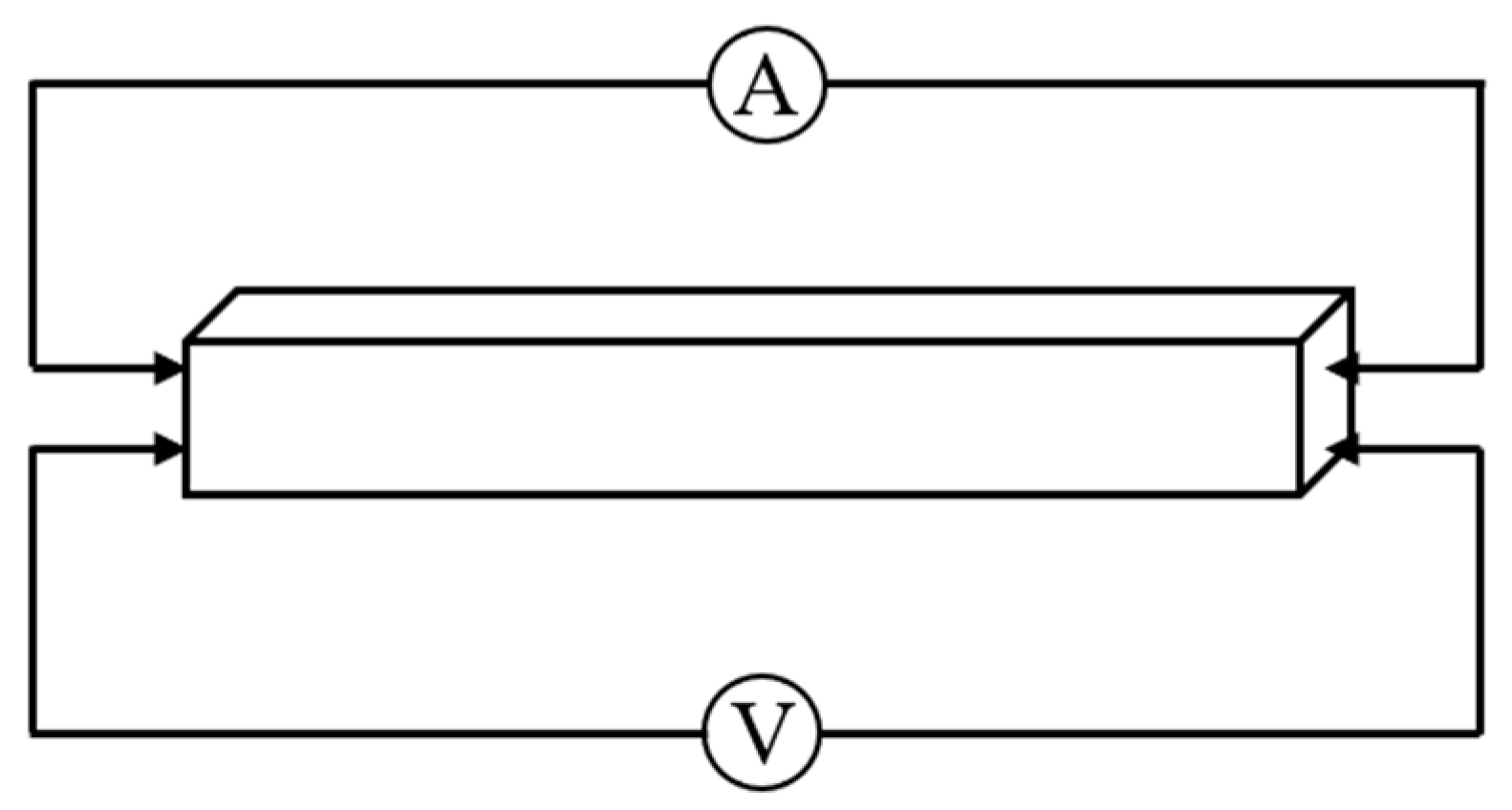
3.1.1. Two-Point Probe Method
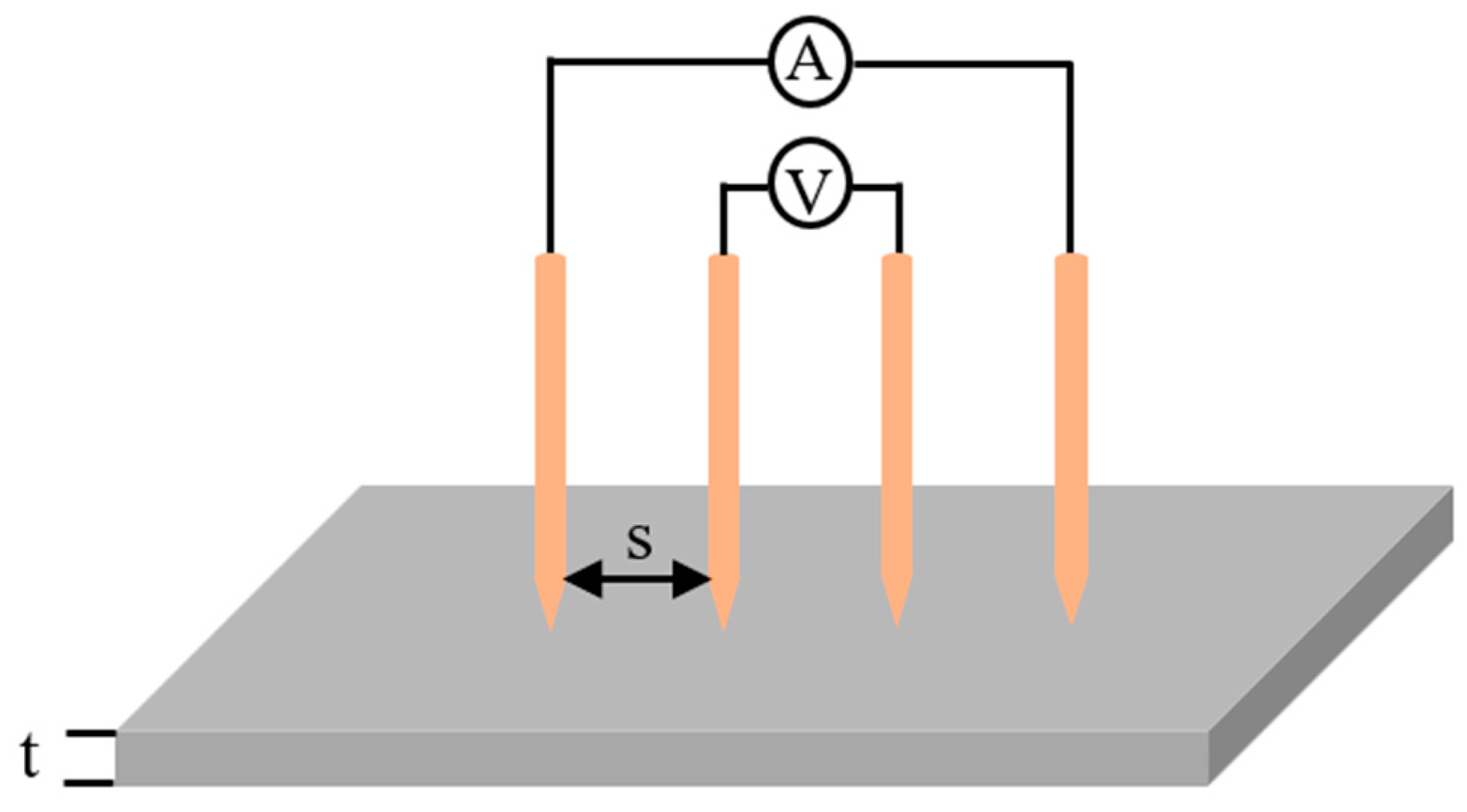
3.1.2. Four-Point Probe Method
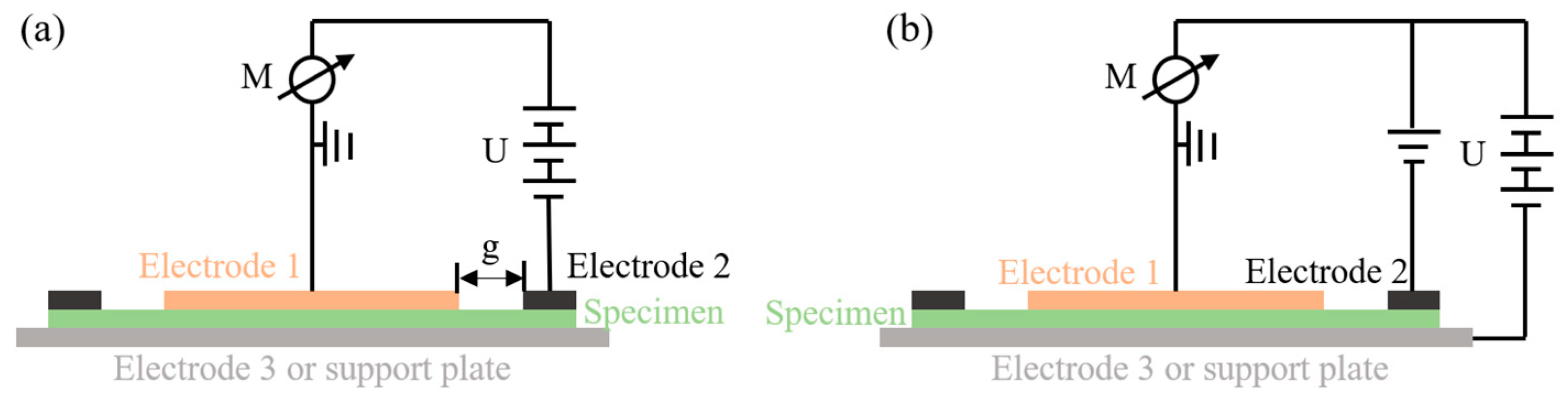
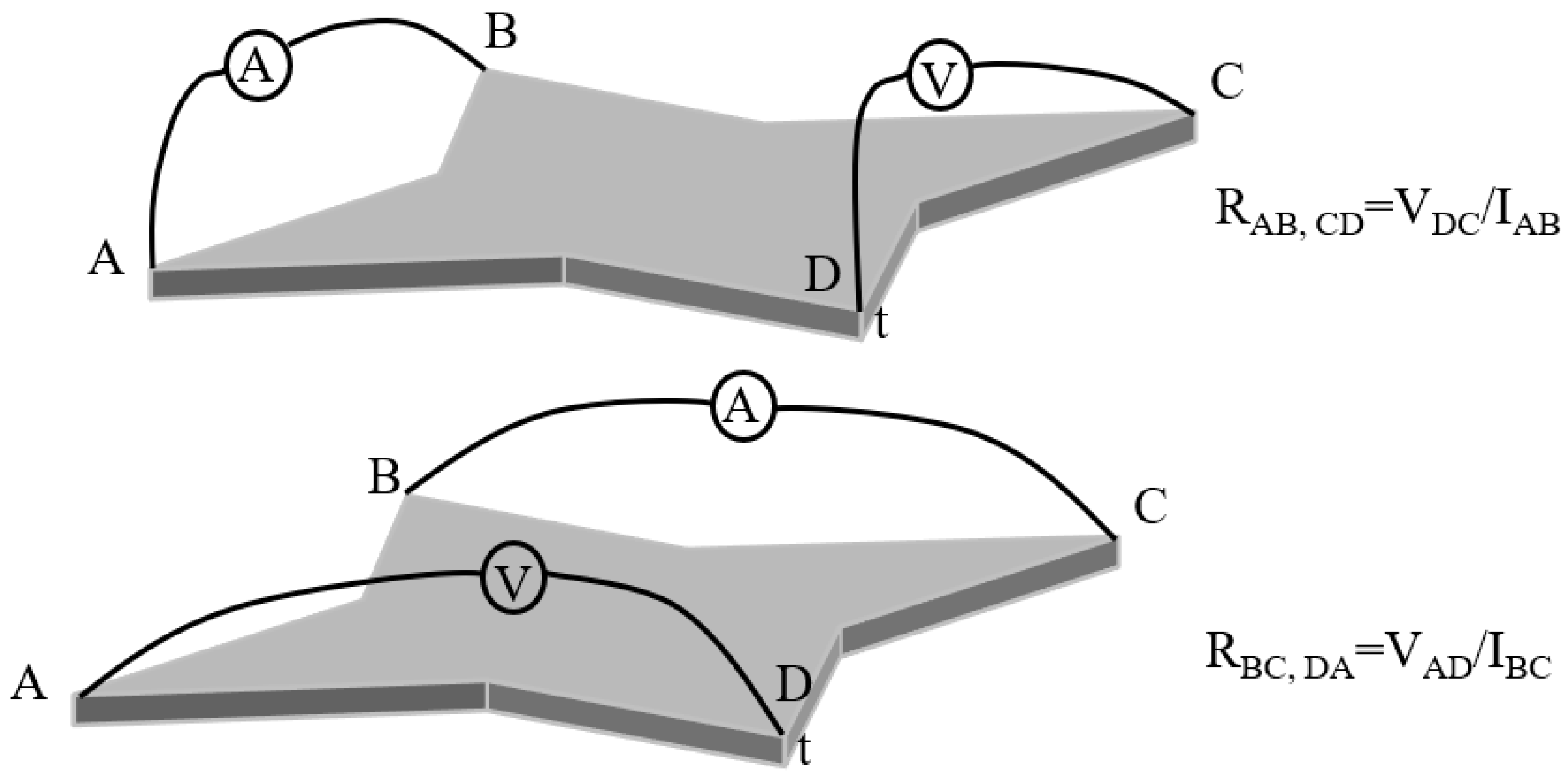
3.1.3. Van der Pauw Method
3.2. Noncontact Methods
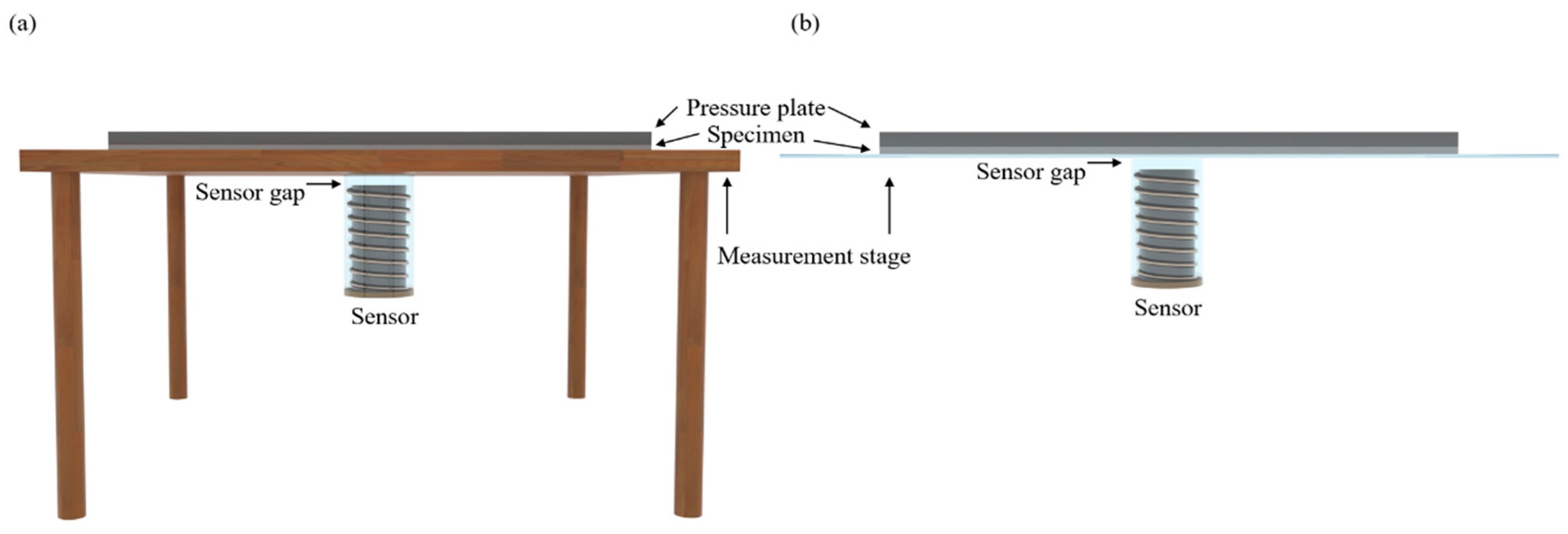
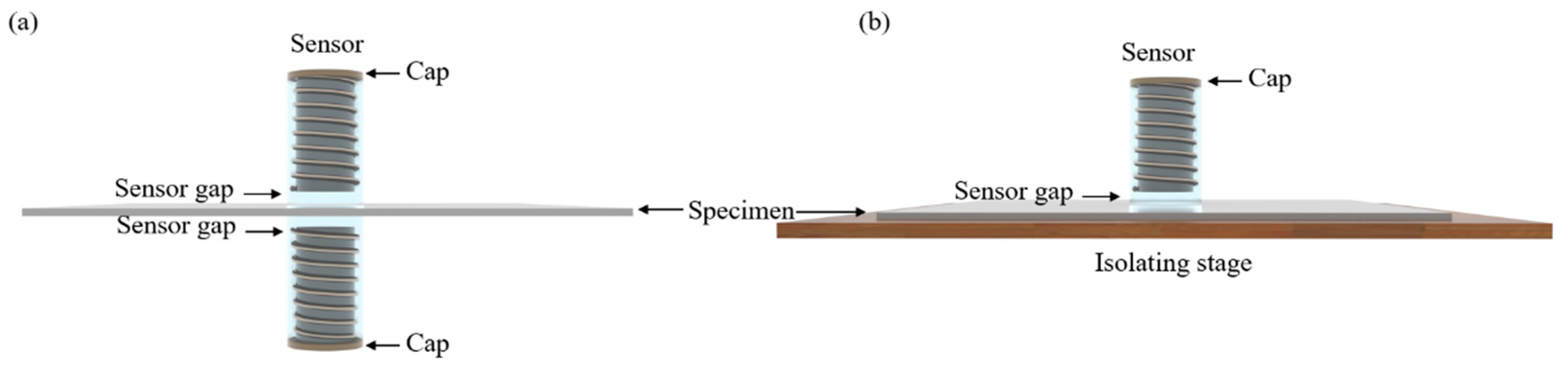
Eddy Current Method
4. Revision of the Test Methods for Different Types of Fabrics
4.1. Intrinsically Conductive Fabrics
4.1.1. Woven Fabrics
4.1.2. Knitted Fabrics
4.1.3. Nonwoven Fabrics
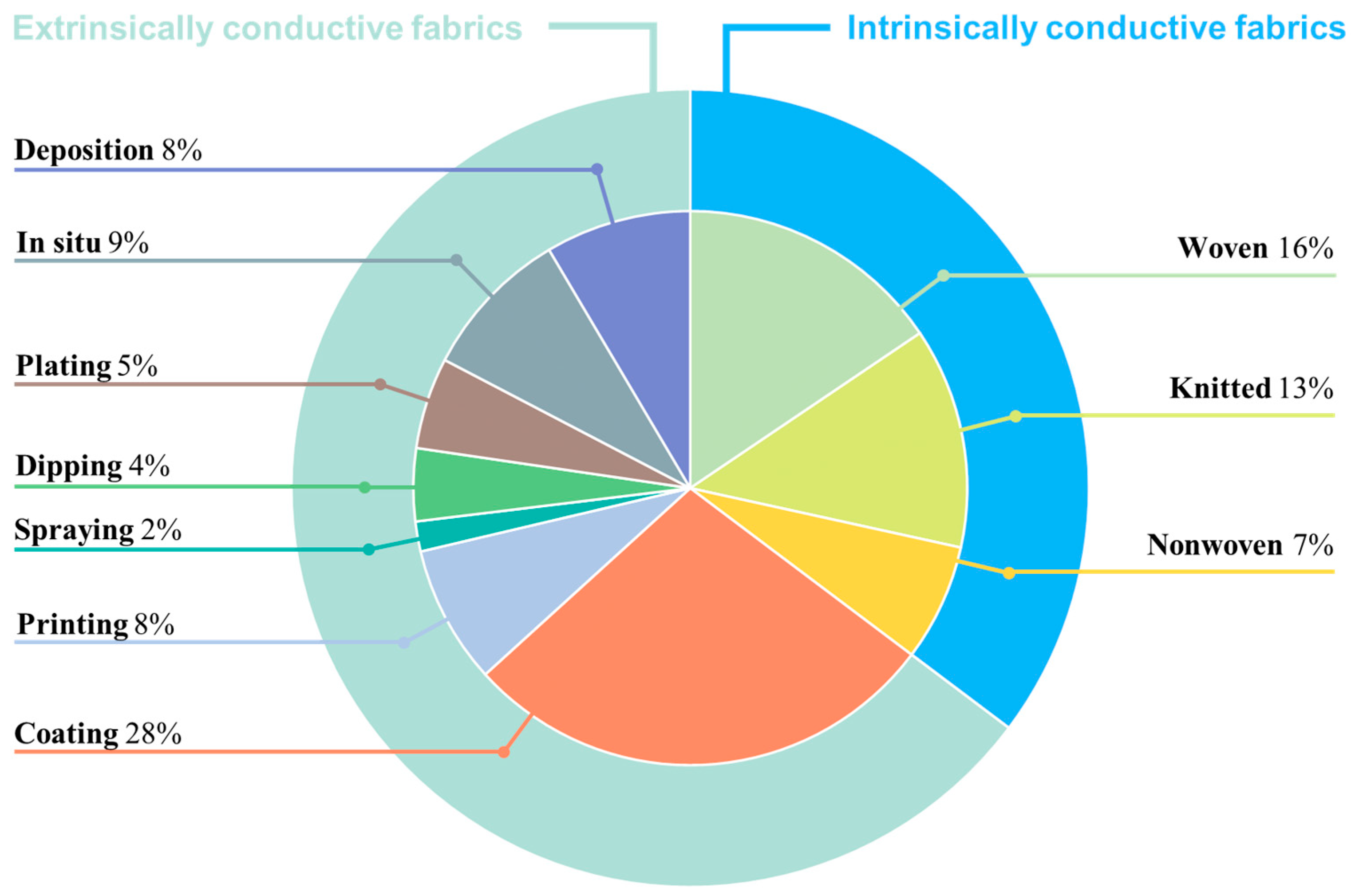
4.2. Extrinsically Conductive Fabrics
5. Conclusions and Outlooks
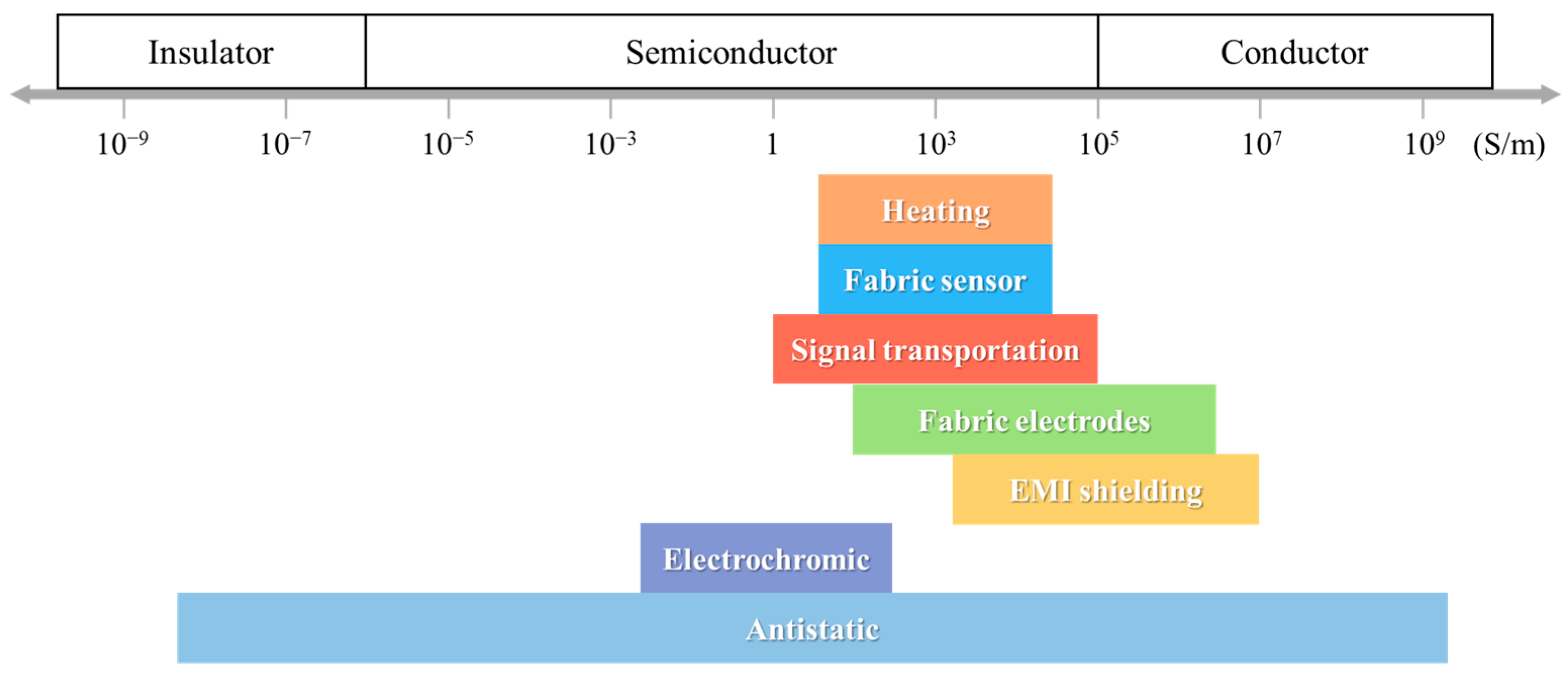
- The parameters used to evaluate the electrical properties of smart textiles (fabric) include surface and volume resistance, sheet resistance, surface and volume resistivity, and conductivity. Depending on the final requirements, different parameters should be measured.
- The electrical resistance test method includes contact (two-point probe, four-point probe, and Van der Pauw) and noncontact (eddy current) methods. Each method has its own advantages and disadvantages.
- Multiple factors affect the conductivity, including the materials’ intrinsic properties, physical strain such as stretching, compression, and bending, and environmental factors such as humidity, temperature, and magnetic fields.
- The method used to test intrinsically conductive fabrics should depend on the properties of the fabric and the final applications. Based on the structure of the fabrics, for woven fabrics, both contact and noncontact resistance test methods are the most suitable; for knitted fabrics, two-point probe, Van der Pauw, and eddy current methods are the most appropriate; and for nonwoven fabrics, both two-point and four-point probe methods can provide more accurate measures. For the applications, it depends on the range of resistance; if the tested specimen has a low resistance, to ensure accuracy, methods that can estimate the contact resistance should be employed.
- The method used to test extrinsically conductive fabric was highly affected by the fabric substrate and conductive layers. The suitable method should be selected based on the properties of the fabrics. If the conductive layers are fragile, the noncontact method should be applied to avoid damage to the specimen.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, X.X. Smart textiles (1): Passive smart. Text. Asia 2001, 32, 45–48. [Google Scholar]
- Zhang, X.X. Smart textiles (2): Active smart. Text. Asia 2001, 32, 49–52. [Google Scholar]
- Zhang, X.X. Smart textiles (3): Very smart. Text. Asia 2001, 32, 35–37. [Google Scholar]
- Van Langenhove, L.; Hertleer, C.; Westbroek, P.; Priniotakis, J. Textile sensors for healthcare. In Smart Textiles for Medicine and Healthcare: Materials, Systems and Applications; Woodhead Publishing: Cambridge, UK, 2007; pp. 106–122. [Google Scholar] [CrossRef]
- Bonaldi, R.R. Electronics used in high-performance apparel-part 1/2. In High-Performance Apparel: Materials, Development, and Applications; Elsevier: Amsterdam, The Netherlands, 2017; pp. 245–284. [Google Scholar] [CrossRef]
- Cho, S.; Chang, T.; Yu, T.; Lee, C.H. Smart Electronic Textiles for Wearable Sensing and Display. Biosensors 2022, 12, 222. [Google Scholar] [CrossRef] [PubMed]
- Naseer, S.; Jabeen, U.; Aamir, M.; Ahmed, S.; Akhtar, J. Smart electronic textiles. In Smart Polymer Nanocomposites: Design, Synthesis, Functionalization, Properties, and Applications; Elsevier: Amsterdam, The Netherlands, 2023; pp. 395–412. [Google Scholar] [CrossRef]
- Ye, C.; Zhao, L.; Yang, S.; Li, X. Recent Research on Preparation and Application of Smart Joule Heating Fabrics. Small 2023, 20, 2309027. [Google Scholar] [CrossRef]
- Fang, S.; Wang, R.; Ni, H.; Liu, H.; Liu, L. A review of flexible electric heating element and electric heating garments. J. Ind. Text. 2022, 51, 101S–136S. [Google Scholar] [CrossRef]
- Tao, X.; Tian, D.; Liang, S.; Jiang, P.; Zhang, L.; Fu, F. Research Progress on the Preparation of Flexible and Green Cellulose-Based Electrothermal Composites for Joule Heating Applications. ACS Appl. Energy Mater. 2022, 5, 13096–13112. [Google Scholar] [CrossRef]
- Kline, W.M.; Lorenzini, R.G.; Sotzing, G.A. A review of organic electrochromic fabric devices. Color. Technol. 2014, 130, 73–80. [Google Scholar] [CrossRef]
- Verma, S.; Dhangar, M.; Paul, S.; Chaturvedi, K.; Khan, M.A.; Srivastava, A.K. Recent Advances for Fabricating Smart Electromagnetic Interference Shielding Textile: A Comprehensive Review. Electron. Mater. Lett. 2022, 18, 331–344. [Google Scholar] [CrossRef]
- Chen, Z.; Yan, T.; Pan, Z. Review of flexible strain sensors based on cellulose composites for multi-faceted applications. Cellulose 2021, 28, 615–645. [Google Scholar] [CrossRef]
- Yu, R.; Zhu, C.; Wan, J.; Li, Y.; Hong, X. Review of Graphene-Based Textile Strain Sensors, with Emphasis on Structure Activity Relationship. Polymers 2021, 13, 151. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Tang, J.; Ma, S.; Zhang, K.; Yan, T.; Pan, Z. A Review of Flexible Strain Sensors Based on Natural Fiber Materials. Adv. Mater. Technol. 2023, 8, 2201503. [Google Scholar] [CrossRef]
- Ahmed, A.; Sharma, S.; Adak, B.; Hossain, M.M.; LaChance, A.M.; Mukhopadhyay, S.; Sun, L. Two-dimensional MXenes: New frontier of wearable and flexible electronics. InfoMat 2022, 4, e12295. [Google Scholar] [CrossRef]
- Liman, M.L.R.; Islam, M.T.; Hossain, M.M. Mapping the Progress in Flexible Electrodes for Wearable Electronic Textiles: Materials, Durability, and Applications. Adv. Electron. Mater. 2022, 8, e578. [Google Scholar] [CrossRef]
- Ding, Y.; Invernale, M.A.; Sotzing, G.A. Conductivity Trends of PEDOT-PSS Impregnated Fabric and the Effect of Conductivity on Electrochromic Textile. ACS Appl. Mater. Interfaces 2010, 2, 1588–1593. [Google Scholar] [CrossRef] [PubMed]
- Conductive, Static Dissipative and Antistatic Foams. Available online: https://palzivna.com/?s=Conductive%2C+Static+Dissipative+and+Antistatic+Foams (accessed on 20 April 2024).
- Difference Between Conductive, Dissipative, Insulative and Antistatic. Available online: https://transforming-technologies.com/esd-fyi/difference-between-conductive-dissipative-and-insulative/ (accessed on 23 April 2024).
- A Brief Guide to Antistatic Fabrics. Available online: https://www.herculite.com/blog/antistatic-fabric-applications (accessed on 17 April 2024).
- Tokarska, M. Characterization of electro-conductive textile materials by its biaxial anisotropy coefficient and resistivity. J. Mater. Sci. Mater. Electron. 2019, 30, 4093–4103. [Google Scholar] [CrossRef]
- Shabani, A.; Hylli, M.; Kazani, I. Investigating Properties of Electrically Conductive Textiles: A Review. Tekstilec 2022, 65, 194–217. [Google Scholar] [CrossRef]
- Rubežienė, V.; Varnaitė-Žuravliova, S. EMI shielding textile materials. In Materials for Potential EMI Shielding Applications: Processing, Properties and Current Trends; Elsevier: Amsterdam, The Netherlands, 2019; pp. 357–378. [Google Scholar] [CrossRef]
- Kacprzyk, R. Measurements of the Volume and Surface Resistance of Textile Materials. FIBRES & TEXTILES in Eastern Europe. 2011. Available online: http://www.fibtex.lodz.pl/pliki/Fibtex_(stsqzuwyu8qs0eb0).pdf (accessed on 21 April 2024).
- Yi, G.; Ko, B.; Jee, M.H. A Study on Changes of Sheet Resistance in Conductive Knit Fabrics Using Non-contact Measurement Method. Fibers. Polym. 2023, 24, 1873–1878. [Google Scholar] [CrossRef]
- Tokarska, M.; Orpel, M. Study of anisotropic electrical resistance of knitted fabrics. Text. Res. J. 2019, 89, 1073–1083. [Google Scholar] [CrossRef]
- Kazani, I.; De Mey, G.; Hertleer, C.; Banaszczyk, J.; Schwarz, A.; Guxho, G.; Van Langenhove, L. Van Der Pauw method for measuring resistivities of anisotropic layers printed on textile substrates. Text. Res. J. 2011, 81, 2117–2124. [Google Scholar] [CrossRef]
- Kazani, I.; De Mey, G.; Hertleer, C.; Banaszczyk, J.; Schwarz, A.; Guxho, G.; Van Langenhove, L. About the collinear four-point probe technique’s inability to measure the resistivity of anisotropic electroconductive fabrics. Text. Res. J. 2013, 83, 1587–1593. [Google Scholar] [CrossRef]
- Banaszczyk, J.; Schwarz, A.; De Mey, G.; Van Langenhove, L. The Van der Pauw method for sheet resistance measurements of polypyrrole-coated para-aramide woven fabrics. J. Appl. Polym. Sci. 2010, 117, 2553–2558. [Google Scholar] [CrossRef]
- Dos Santos, C.A.M.; De Campos, A.; Da Luz, M.S.; White, B.D.; Neumeier, J.J.; De Lima, B.S.; Shigue, C.Y. Procedure for measuring electrical resistivity of anisotropic materials: A revision of the Montgomery method. J. Appl. Phys. 2011, 110, 083703. [Google Scholar] [CrossRef]
- Kazani, I.; Hertleer, C.; De Mey, G.; Schwarz, A.; Guxho, G.; Van Langenhove, L. Electrical Conductive Textiles Obtained by Screen Printing. Available online: http://www.fibtex.lodz.pl/2012/1/57.pdf (accessed on 20 April 2024).
- Tyurin, I.N.; Getmantseva, V.V.; Andreeva, E.G. Van der Pauw Method for Measuring the Electrical Conductivity of Smart Textiles. Fibre. Chem. 2019, 51, 139–146. [Google Scholar] [CrossRef]
- Tokarska, M. Determination of fabric surface resistance by van der pauw method in case of contacts distant from the sample edge. Autex. Res. J. 2014, 14, 55–60. [Google Scholar] [CrossRef]
- Tokarska, M. Evaluation of measurement uncertainty of fabric surface resistance implied by the Van der Pauw Equation. IEEE Trans. Instrum. Meas. 2014, 63, 1593–1599. [Google Scholar] [CrossRef]
- Zhao, M.; Chen, S.; Wang, K.; Liu, G. Analysis of Electrical Resistivity Characteristics and Damage Evolution of Soil-Rock Mixture under Triaxial Shear. Materials 2023, 16, 3698. [Google Scholar] [CrossRef] [PubMed]
- Das, N.C.; Chaki, T.K.; Khastgir, D. Effect of processing parameters, applied pressure and temperature on the electrical resistivity of rubber-based conductive composites. Carbon 2002, 40, 807–816. [Google Scholar] [CrossRef]
- Sawaoka, A.; Mlyahara, S.; Naga, H.; Minomura, S. Effect of Pressure on the Electrical Resistivity of Some Vanadium Spinels. Solid State Commun. 1965, 3, 155–158. [Google Scholar] [CrossRef]
- Raji, R.K.; Miao, X.; Zhang, S.; Li, Y.; Wan, A.; Boakye, A. Knitted piezoresistive strain sensor performance, impact of conductive area and profile design. J. Ind. Text. 2020, 50, 616–634. [Google Scholar] [CrossRef]
- Wijesiriwardana, R. Inductive fiber-meshed strain and displacement transducers for respiratory measuring systems and motion capturing systems. IEEE Sens. J. 2006, 6, 571–579. [Google Scholar] [CrossRef]
- Kim, H.; Lee, S. Effect of Relative Humidity Condition on Electrical Heating Textile Coated with Graphene-based on Cotton Fabric. Fibers Polym. 2021, 22, 276–284. [Google Scholar] [CrossRef]
- Cerovic, D.D.; Asanovic, K.A.; Maletic, S.B.; Dojcilovic, J.R. Comparative study of the electrical and structural properties of woven fabrics. Compos. B Eng. 2013, 49, 65–70. [Google Scholar] [CrossRef]
- Penava, Ž.; Penava, D.Š.; Knezić, Ž. Heat as a Conductivity Factor of Electrically Conductive Yarns Woven into Fabric. Materials 2022, 15, 1202. [Google Scholar] [CrossRef] [PubMed]
- Bica, I.; Iacobescu, G.-E. The Influence of Magnetic Fields on the Electrical Conductivity of Membranes based on Cotton Fabric, Honey, and Microparticles of Carbonyl Iron and Silver. Materials 2023, 16, 1995. [Google Scholar] [CrossRef] [PubMed]
- Ghorbani, M.M.; Taherian, R. Methods of measuring electrical properties of material. In Electrical Conductivity in Polymer-Based Composites: Experiments, Modelling, and Applications; Elsevier: Amsterdam, The Netherlands, 2018; pp. 365–394. [Google Scholar] [CrossRef]
- Miller, G.L.; Robinson, D.A.H.; Wiley, J.D. Contactless measurement of semiconductor conductivity by radio frequency-free-carrier power absorption. Rev. Sci. Instrum. 1976, 47, 799–805. [Google Scholar] [CrossRef]
- Maryniak, W.A.; Uehara, T.; Noras, M.A. Surface Resistivity and Surface Resistance Measurements Using a Concentric Ring Probe Technique. Available online: https://www.advancedenergy.com/getmedia/779e77c3-a07e-4c26-be3b-424bacc6622b/en-esd-surface-resistivity-application-note.pdf (accessed on 5 May 2024).
- ASTM D257-14; Standard Test Methods for DC Resistance or Conductance of Insulating Materials. ASTM: West Conshohocken, PA, USA, 2021.
- Heaney, M.B. Electrical Conductivity and Resistivity. In Measurement Instrumentation Sensors; Springer: Berlin/Heidelberg, Germany, 2003; Volume 7–1, ISBN 9783540648307. [Google Scholar]
- Wang, J.; Zhuang, J.; Jin, W.; Yu, Q.; Yu, J.; He, L.; Wang, Q.; Cheng, D.; Cai, G.; Wang, X. Liquid metal-based on cotton/lycra elastic fabric surface for flexible antenna and wearable strain sensor. Cellulose 2023, 30, 11261–11272. [Google Scholar] [CrossRef]
- Gao, X.; Wang, Y.; Wu, M.; Zhi, C.; Meng, J.; Zhang, L. Multicolor electrochromic fabric with a simple structure of PEDOT:PSS/DMSO. Dye Pigment. 2023, 219, 111642. [Google Scholar] [CrossRef]
- Aileni, R.M.; Chiriac, L. Conductive Membranes Based on Cotton Fabric Coated with Polymers for Electrode Applications. Materials 2022, 15, 7286. [Google Scholar] [CrossRef]
- Ge, F.; Yu, W.; Tan, J.; Sun, J.; Yin, Y.; Wang, C. Gradient Response Color-Changing Joule Heating Fabric for Visual Temperature Detection. Adv. Mater. Interfaces 2022, 9, 2201013. [Google Scholar] [CrossRef]
- Tang, X.; Zhao, X.; Lu, Y.; Li, S.; Zhang, Z.; Zhu, M.; Yao, K.; Zheng, J.; Chen, H.; Duan, Y. Flexible metalized polyimide nonwoven fabrics for efficient electromagnetic interference shielding. Chem. Eng. J. 2024, 480, 148000. [Google Scholar] [CrossRef]
- Wang, C.X.; Lv, J.C.; Ren, Y.; Zhi, T.; Chen, J.Y.; Zhou, Q.Q.; Lu, Z.Q.; Gao, D.W.; Jin, L.M. Surface modification of polyester fabric with plasma pretreatment and carbon nanotube coating for antistatic property improvement. Appl. Surf. Sci. 2015, 359, 196–203. [Google Scholar] [CrossRef]
- Silvestre, R.; Llopis, R.L.; Rodrigo, L.C.; Martinez, V.S.; Ferri, J.; Garcia-Breijo, E. Low-Temperature Soldering of Surface Mount Devices on Screen-Printed Silver Tracks on Fabrics for Flexible Textile Hybrid Electronics. Sensors 2022, 22, 5766. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Yu, Z.; He, Y.; Li, C.; Wang, L.; Wu, M. A fabric-based electrode for wearable piezoelectric nanogenerators to distinguish sense human motions. Appl. Surf. Sci. 2023, 610, 155451. [Google Scholar] [CrossRef]
- Alamer, F.A.; Aldeih, A.; Alsalmi, O.; Althagafy, K.; Al-Dossari, M. Construction of an Electrical Conductor, Strain Sensor, Electrical Connection and Cycle Switch Using Conductive Graphite Cotton Fabrics. Polymers 2022, 14, 4767. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Zhang, X.; Li, G.; Yang, X.; Ding, X. Dependence of sheet resistance of nickel-plated PANi/PTT composite fabric on electroless plating conditions. J. Text. Inst. 2016, 107, 1169–1174. [Google Scholar] [CrossRef]
- Zheng, X.; Tang, J.; Cheng, L.; Yang, H.; Zou, L.; Li, C. Superhydrophobic hollow magnetized Fe3O4 nanospheres/MXene fabrics for electromagnetic interference shielding. J. Alloys Compd. 2023, 934, 167964. [Google Scholar] [CrossRef]
- Kowalczyk, D.; Fortuniak, W.; Mizerska, U.; Kaminska, I.; Makowski, T.; Brzezinski, S.; Piorkowska, E. Modification of cotton fabric with graphene and reduced graphene oxide using sol–gel method. Cellulose 2017, 24, 4057–4068. [Google Scholar] [CrossRef]
- Li, M.; Wang, Y.; Fan, X.; Huang, H.; Wan, Y.; Li, Y.; Fang, J.; Gao, J.; Yang, Y.; Liu, J. A Conductive Bamboo Fabric with Controllable Resistance for Tailoring Wearable Sensors. ACS Appl. Mater. Interfaces 2022, 14, 26958–26969. [Google Scholar] [CrossRef] [PubMed]
- Petru, M.; Ali, A.; Khan, A.S.; Srb, P.; Kucera, L.; Militky, J. Flexible Coated Conductive Textiles as Ohmic Heaters in Car Seats. Appl. Sci. 2023, 13, 6874. [Google Scholar] [CrossRef]
- Singh, Y. Electrical resistivity measurements: A review. Int. J. Mod. Phys. Conf. Ser. 2013, 22, 745–756. [Google Scholar] [CrossRef]
- AATCC TM76-2000e3(2018)e; Test Method for Electrical Surface Resistivity of Fabrics. AATCC: Research Triangle Park, NC, USA, 2018.
- AATCC EP13; Evaluation Procedure of Electrical Resistance of Electronically-Integrated Textiles. AATCC: Research Triangle Park, NC, USA, 2018.
- BS EN 1149-1:2006; Protective Clothing. Electrostatic Properties Test Method for Measurement of Surface Resistivity. BSI: London, UK, 2006.
- BS EN 1149-2:1997; Protective Clothing Electrostatic Properties Part 2: Test Method for Measurement of the Electrical Resistance through a Material (Vertical Resistance). BSI: London, UK, 1997.
- IEC 62899-201:2016+AMD1:2018; Amendment 1—Printed Electronics—Part 201: Materials—Substrates. IEC: Geneva, Switzerland, 2018.
- IEC 62631-3-1:2023; Dielectric and Resistive Properties of Solid Insulating Materials—Part 3-1: Determination of Resistive Properties (DC Methods)—Volume Resistance and Volume Resistivity—General Method. IEC: Geneva, Switzerland, 2023.
- IEC 62631-3-2:2023; Dielectric and Resistive Properties of Solid Insulating Materials—Part 3-2: Determination of Resistive Properties (DC Methods)—Surface Resistance and Surface Resistivity. IEC: Geneva, Switzerland, 2023.
- SEMI MF43; Test Method for Resistivity of Semiconductor Materials. SEMI: Milpitas, CA, USA, 2005.
- SEMI MF84; Test Method for Measuring Resistivity of Silicon Wafers With an In-Line Four-Point Probe. SEMI: Milpitas, CA, USA, 2005.
- ASTM F390-98; Standard Test Method for Sheet Resistance of Thin Metallic Films with a Collinear Four-Probe Array. ASTM: West Conshohocken, PA, USA, 1998.
- IEC 63203-201-2:2022; Wearable Electronic Devices and Technologies—Part 201-2: Electronic Textile—Measurement Methods for Basic Properties of Conductive Fabrics and Insulation Materials. IEC: Geneva, Switzerland, 2022.
- IEC 62899-202:2023; Printed Electronics—Part 202: Materials—Conductive Ink. IEC: Geneva, Switzerland, 2023.
- Rashidi, M.; Mottaghitalab, V.; Haghi, A.K. Nanotextiles-based sensing devices to acquire biomechanical signals. Part I. J. Balk. Tribol. Assoc. 2010, 16, 258–266. [Google Scholar]
- Landsiedel, J.; Tschannett, J.; Lenninger, M.; Stroj, S.; Domke, M.; Bechtold, T.; Pham, T.; Aguiló-Aguayo, N. A siloxane interlayer approach to enhance surface metallization on polyamide fabrics via electroless copper deposition. Surf. Interfaces 2023, 42, 103434. [Google Scholar] [CrossRef]
- Zhou, W.; Tang, Y.; Song, R.; Jiang, L.; Hui, K.S.; Hui, K.N. Characterization of electrical conductivity of porous metal fiber sintered sheet using four-point probe method. Mater. Des. 2012, 37, 161–165. [Google Scholar] [CrossRef]
- BS EN 16812:2016; Textiles and Textile Products. Electrically Conductive Textiles. Determination of the Linear Electrical Resistance of Conductive Tracks. BSI: London, UK, 2016.
- Smits, F.M. Measurement the of Sheet Resistivities Four-Point Probe. Bell Syst. Tech. J. 1958, 37, 711–718. [Google Scholar] [CrossRef]
- Matsumura, T.; Sato, Y. A Theoretical Study on Van Der Pauw Measurement Values of Inhomogeneous Compound Semiconductor Thin Films. J. Mod. Phys. 2010, 01, 340–347. [Google Scholar] [CrossRef][Green Version]
- Van Der Pauw, L.J. A Method of Measuring Specific Resistivity and Hall Effect of Discs of Arbitrary Shape. In Semiconductor Devices: Pioneering Papers; World Scientific eBooks: College Park, MD, USA, 1991; pp. 174–182. [Google Scholar] [CrossRef]
- Tokarska, M. Measuring resistance of textile materials based on Van der Pauw method. Indian J. Fibre. Text. Res. 2013, 38, 198–201. [Google Scholar]
- ISO 24584; Test Method for Sheet Resistance of Conductive Textiles Using Non-Contact Type. ISO: Vernier, Switzerland, 2022.
- IEC 62899-202-3:2019; Printed Electronics—Part 202-3: Materials—Conductive ink—Measurement of Sheet Resistance of Conductive Films—Contactless Method. IEC: Geneva, Switzerland, 2019.
- Gandhi, K.L.; Sondhelm, W.S. Technical fabric structures—1. Woven fabrics. In Handbook of Technical Textiles: Technical Textile Processes; Elsevier: Amsterdam, The Netherlands, 2015; pp. 63–106. [Google Scholar] [CrossRef]
- Stankard, S. Yarn to Fabric: Weaving. In Textiles and Fashion: Materials, Design and Technology; Elsevier Inc.: Amsterdam, The Netherlands, 2015; pp. 255–287. [Google Scholar] [CrossRef]
- Thomas, L. Woven structures and their impact on the function and performance of smart clothing. In Smart Clothes and Wearable Technology; Elsevier Ltd.: Amsterdam, The Netherlands, 2009; pp. 131–155. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, L. Impact of Different Conductive Path Design and Fabrication on Temperature Variation of Thermal Stainless Steel Woven Fabric. J. Text. Inst. 2022, 113, 1240–1247. [Google Scholar] [CrossRef]
- Peng, H.-K.; Wang, Y.; Li, T.-T.; Lou, C.-W.; Lin, J.-H. Conductive woven fabrics made of metallic/modal core yarns: Manufacturing techniques and electromagnetic shielding effectiveness. J. Text. Inst. 2023, 115, 1–10. [Google Scholar] [CrossRef]
- Lou, C.-W.; Liu, Y.-L.; Shiu, B.-C.; Peng, H.-K.; Lin, J.-H. Preparation and Evaluation of Polyester-Cotton/Wire Blended Conductive Woven Fabrics for Electromagnetic Shielding. J. Ind. Text. 2022, 51, 7104S–7118S. [Google Scholar] [CrossRef]
- Lai, M.-F.; Lou, C.-W.; Lin, T.A.; Wang, C.-H.; Lin, J.-H. High-Strength Conductive Yarns and Fabrics: Mechanical Properties, Electromagnetic Interference Shielding Effectiveness, and Manufacturing Techniques. J. Text. Inst. 2021, 112, 347–357. [Google Scholar] [CrossRef]
- Tunakova, V.; Technikova, L.; Militky, J. Influence of washing/drying cycles on fundamental properties of metal fiber-containing fabrics designed for electromagnetic shielding purposes. Text. Res. J. 2017, 87, 175–192. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, J.; Liu, Z.; Wang, Z. A new study on the influencing factors and mechanism of shielding effectiveness of woven fabrics containing stainless steel fibers. J. Ind. Text. 2021, 50, 830–846. [Google Scholar] [CrossRef]
- Su, C.; Chern, J. Effect of Stainless Steel-Containing Fabrics on Electromagnetic Shielding Effectiveness. Text. Res. J. 2004, 74, 51–54. [Google Scholar] [CrossRef]
- Ray, S.C. Classification of Knitting; Woodhead: Cambridge, UK, 2011; ISBN 9780857095558. [Google Scholar]
- Knezic, Z.; Penava, Z.; Penava, D.S.; Rogale, D. The Impact of Elongation on Change in Electrical Resistance of Electrically Conductive Yarns Woven into Fabric. Materials 2021, 14, 3390. [Google Scholar] [CrossRef] [PubMed]
- Teyeme, Y.; Malengier, B.; Tesfaye, T.; Van Langenhove, L. A Fabric-Based Textile Stretch Sensor for Optimized Measurement of Strain in Clothing. Sensors 2020, 20, 7323. [Google Scholar] [CrossRef] [PubMed]
- Büscher, G.H.; Kõiva, R.; Schürmann, C.; Haschke, R.; Ritter, H.J. Flexible and stretchable fabric-based tactile sensor. Rob. Auton. Syst. 2015, 63, 244–252. [Google Scholar] [CrossRef]
- Lin, X.; Seet, B.-C. Battery-Free Smart Sock for Abnormal Relative Plantar Pressure Monitoring. IEEE Trans. Biomed. Circuits. Syst. 2017, 11, 464–473. [Google Scholar] [CrossRef] [PubMed]
- Lee, S. Analysis of electrical and comfort properties of conductive knitted fabrics based on blending ratio of silver-coated yarns for smart clothing. J. Eng. Fiber. Fabr. 2022, 17, 155892502211044. [Google Scholar] [CrossRef]
- Repon, M.R.; Mikucioniene, D.; Baltina, I.; Blums, J.; Laureckiene, G. Ag Coated Pa-Based Electro-Conductive Knitted Fabrics for Heat Generation in Compression Supports. Autex Res. J. 2022, 22, 55–63. [Google Scholar] [CrossRef]
- Liu, S.; Fu, S.; Wu, J.; Li, S. Development and characterization of electrical heating garment based on the weft knitted jacquard pattern for back pain disease. J. Text. Inst. 2022, 113, 2428–2434. [Google Scholar] [CrossRef]
- Sun, K.; Liu, S.; Long, H. Structural Parameters Affecting Electrothermal Properties of Woolen Knitted Fabrics Integrated with Silver-Coated Yarns. Polymers 2019, 11, 1709. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Liu, Y.; Li, L. The impact of different proportions of knitting elements on the resistive properties of conductive fabrics. Text. Res. J. 2019, 89, 881–890. [Google Scholar] [CrossRef]
- Yu, Z.-C.; Zhang, J.-F.; Lou, C.-W.; Lin, J.-H. Wicking behavior and antibacterial properties of multifunctional knitted fabrics made from metal commingled yarns. J. Text. Inst. 2015, 106, 862–871. [Google Scholar] [CrossRef]
- Kaya, D.D.; Oglakcioglu, N.; Sari, B.; Yilmaz, H.A. Electromagnetic Shielding and Comfort Properties of Knitted Fabrics Produced by Electrically Conductive Fibers. Fibers. Polym. 2023, 24, 2451–2468. [Google Scholar] [CrossRef]
- Sancak, E.; Akalin, M.; Usta, I.; Yuksek, M.; Ozen, M.S. The Effects of Fabric and Conductive Wire Properties on Electromagnetic Shielding Effectiveness and Surface Resistivity of Interlock Knitted Fabrics. Fibers Polym. 2018, 19, 843–853. [Google Scholar] [CrossRef]
- Albrecht, W.; Fuchs, H.; Kittelmann, W. Nonwoven Fabrics; Wiley-VCH: Weinheim, Germany, 2003; ISBN 3527304061. [Google Scholar]
- Kim, J.Y.; Lee, S.R.; Lee, G.W.; Park, D.H.; An, K.H.; Kim, W.S. Electrical, mechanical, and thermal characteristics of nonwoven fabric heating sheets containing chopped carbon fibers. SN Appl. Sci. 2020, 2, 1397. [Google Scholar] [CrossRef]
- Lu, L.; Xing, D.; Xie, Y.; Teh, K.S.; Zhang, B.; Chen, S.; Tang, Y. Electrical conductivity investigation of a nonwoven fabric composed of carbon fibers and polypropylene/polyethylene core/sheath bicomponent fibers. Mater. Des. 2016, 112, 383–391. [Google Scholar] [CrossRef]
- Ozen, M.S.; Sancak, E. Investigation of electromagnetic shielding effectiveness of needle punched nonwoven fabrics with staple polypropylene and carbon fibres. J. Text. Inst. 2016, 107, 249–257. [Google Scholar] [CrossRef]
- Ozen, M.S.; Sancak, E.; Soin, N.; Shah, T.H.; Siores, E. Investigation of electromagnetic shielding effectiveness of needle punched nonwoven fabric produced from conductive silver coated staple polyamide fibre. J. Text. Inst. 2016, 107, 912–922. [Google Scholar] [CrossRef]
- Pakdel, E.; Kashi, S.; Baum, T.; Usman, K.A.S.; Razal, J.M.; Varley, R.; Wang, X. Carbon fibre waste recycling into hybrid nonwovens for electromagnetic interference shielding and sound absorption. J. Clean. Prod. 2021, 315, 128196. [Google Scholar] [CrossRef]
- Wang, L.; He, D.; Li, J.; He, B.; Qian, L. Conductive cotton fabrics with ultrahigh washability by electroless silver plating after silane modification. Cellulose 2021, 28, 5881–5893. [Google Scholar] [CrossRef]
- Moazzenchi, B.; Montazer, M. Click Electroless Plating and Sonoplating of Polyester with Copper Nanoparticles Producing Conductive Fabric. Fibers. Polym. 2020, 21, 522–531. [Google Scholar] [CrossRef]
- Yotprayoonsak, P.; Anusak, N.; Virtanen, J.; Kangas, V.; Promarak, V. Facile fabrication of flexible and conductive AuNP/DWCNT fabric with enhanced Joule heating efficiency via spray coating route. Microelectron. Eng. 2022, 255, 111718. [Google Scholar] [CrossRef]
- Jin, Y.; Ma, Z.; Wu, M.; Wang, L. Preparation of MXene with high conductivity and its application on conductive fabrics. Appl. Nanosci. 2022, 12, 2317–2329. [Google Scholar] [CrossRef]
- Ma, H.; Li, J.; Zhou, J.; Luo, Q.; Wu, W.; Mao, Z.; Ma, W. Screen-Printed Carbon Black/Recycled Sericin@Fabrics for Wearable Sensors to Monitor Sweat Loss. ACS Appl. Mater. Interfaces 2022, 14, 11813–11819. [Google Scholar] [CrossRef] [PubMed]
- Shao, Y.; Song, Q.; Chen, W.; Ifra, M.; Jiang, J.; Liu, Y. Characteristics of Electrical Heating and Sensing Properties for CNTs/GNs Polyester-Knitted Fabrics Based on Network Structure. Fibers. Polym. 2023, 24, 1139–1148. [Google Scholar] [CrossRef]
- Makowski, T.; Svyntkivska, M.; Piorkowska, E.; Kregiel, D. Multifunctional polylactide nonwovens with 3D network of multiwall carbon nanotubes. Appl. Surf. Sci. 2020, 527, 146898. [Google Scholar] [CrossRef]
- Yang, T.; Zuo, L.; Lu, D.; Zheng, C.; Chen, Y. Free-standing N-doped composite carbon nanofibers anode with high SiO loading for flexible lithium-ion battery. Mater. Lett. 2023, 333, 133554. [Google Scholar] [CrossRef]
- Jain, V.K.; Chatterjee, A. Graphene Functionalized Cotton Nonwoven for Thermotherapy. J. Nat. Fibers 2022, 19, 12883–12895. [Google Scholar] [CrossRef]
- Moriche, R.; Moreno-Aviles, M.A.; Jimenez-Suarez, A.; Prolongo, S.G.; Urena, A. Graphene nanoplatelets electrical networks as highly efficient self-heating materials for glass fiber fabrics. J. Ind. Text. 2022, 51, 4410S–4423S. [Google Scholar] [CrossRef]
- Wang, B.; Cheng, H.; Zhu, J.; Yuan, Y.; Wang, C. A flexible and stretchable polypyrrole/knitted cotton for electrothermal heater. Org. Electron. 2020, 85, 105819. [Google Scholar] [CrossRef]
- Pragya, A.; Deogaonkar-Baride, S. Effect of yarn interlacement pattern on the surface electrical conductivity of intrinsically conductive fabrics. Synth. Met. 2020, 268, 116512. [Google Scholar] [CrossRef]
- Tseghai, G.B.; Mengistie, D.A.; Malengier, B.; Fante, K.A.; Van Langenhove, L. PEDOT:PSS-Based Conductive Textiles and Their Applications. Sensors 2020, 20, 1881. [Google Scholar] [CrossRef] [PubMed]
- Xie, X.; Xin, B.; Chen, Z.; Xu, Y. Preparation and characterization of PANI-PPY/PET fabric conductive composite for supercapacitors. J. Text. Inst. 2022, 113, 2443–2450. [Google Scholar] [CrossRef]
- Tang, L.; Lyu, B.; Gao, D.; Jia, Z.; Ma, J. A wearable textile with superb thermal functionalities and durability towards personal thermal management. Chem. Eng. J. 2023, 465, 142829. [Google Scholar] [CrossRef]
- Horita, Y.; Kuromatsu, S.; Watanabe, T.; Suga, R.; Koh, S. Polydopamine-assisted dip-and-dry fabrication of highly conductive cotton fabrics using single-wall carbon nanotubes inks for flexible devices. Cellulose 2023, 30, 1971–1980. [Google Scholar] [CrossRef]
- Wang, X.; Wan, A.; Jiang, G.; Raji, R.K.; Yu, D. Preparation of Polypyrrole/Silver Conductive Polyester Fabric by UV Exposure. Autex. Res. J. 2021, 21, 231–237. [Google Scholar] [CrossRef]
- Li, L.; Sun, B.; Li, W.; Jiang, L.; Zhou, Y.; Ma, J.; Chen, S.; Ning, X.; Zhou, F.L. Flexible and Highly Conductive AgNWs/PEDOT:PSS Functionalized Aramid Nonwoven Fabric for High-Performance Electromagnetic Interference Shielding and Joule Heating. Macromol. Mater. Eng. 2021, 306, 2100365. [Google Scholar] [CrossRef]
- He, G.; Wang, L.; Bao, X.; Lei, Z.; Ning, F.; Li, M.; Zhang, X.; Qu, L. Synergistic flame retardant weft-knitted alginate/viscose fabrics with MXene coating for multifunctional wearable heaters. Composites 2022, 232, 109618. [Google Scholar] [CrossRef]
- Lu, Z.; Lv, J.; Qiu, J.; Zhang, L. Polypyrrole-coated recycled polyphenylene sulfite nonwoven fabric with high electrical conductivity for heat generation. J. Ind. Text. 2022, 51, 6968S–6982S. [Google Scholar] [CrossRef]
- Wang, X.; Lei, Z.; Ma, X.; He, G.; Xu, T.; Tan, J.; Wang, L.; Zhang, X.; Qu, L.; Zhang, X. A lightweight MXene-Coated nonwoven fabric with excellent flame Retardancy, EMI Shielding, and Electrothermal/Photothermal conversion for wearable heater. Chem. Eng. J. 2022, 430, 132605. [Google Scholar] [CrossRef]
- Zhao, Y.; Guo, X.; Sun, H.; Tao, L. Recent Advances in Flexible Wearable Technology: From Textile Fibers to Devices. Chem. Rec. 2024, 24, e202300361. [Google Scholar] [CrossRef] [PubMed]
- Ismar, E.; Bahadir, S.K.; Kalaoglu, F.; Koncar, V. Futuristic Clothes: Electronic Textiles and Wearable Technologies. Glob. Chall. 2020, 4, 1900092. [Google Scholar] [CrossRef] [PubMed]
- Islam, M.R.; Afroj, S.; Yin, J.; Novoselov, K.S.; Chen, J.; Karim, N. Advances in Printed Electronic Textiles. Adv. Sci. 2024, 11, e2304140. [Google Scholar] [CrossRef] [PubMed]



| Weave Structure | Fabric Composition | Application (Function) | Testing Method | Electrical Properties | Ref. | |
|---|---|---|---|---|---|---|
| Nonconductive Yarns | Conductive Yarns | |||||
| Plain | PES | SS yarn | Heating | 4-P probe | R = 4.42 Ω | [90] |
| Plain | Modal roving | Metallic/modal core yarns | EMI shielding | 2-P probe (parallel electrodes) | ρs = 0.34 × 103 Ω/sq | [91] |
| Plain | PES/CO roving | Cu wires or SS wires (core) | EMI shielding | 2-P probe (parallel electrodes) | ρs = 3.15 or 4.11 log Ω/sq | [92] |
| Plain | High-strength PES/Bamboo charcoal | SS | EMI shielding | 2-P probe (concentric rings) | ρs = 107 Ω/sq | [93] |
| twill 2/2 | PP | SS | EMI shielding | 2-P probe | ρs = 105–107 Ω ρv = 104–107 Ω·cm | [94] |
| Plain | CO/PES | SS | EMI shielding | 2-P probe (parallel electrodes) | ρs = 103–106 Ω/sq | [95] |
| Twill | ρs = 103 Ω/sq | |||||
| Stain | ρs = 103–106 Ω/sq | |||||
| Hopsack | ρs = 103–106 Ω/sq | |||||
| Unspecified | \ | (tin, nickel, silver) metalized | Conveying electrical signals | Van der Pauw | Rs = 10−3–10−2 Ω | [22] |
| Structure | Fabric Composition | Application (Function) | Testing Method | Electrical Properties | Ref. | |
|---|---|---|---|---|---|---|
| Nonconductive Yarns | Conductive Yarns | |||||
| Interlock stitch | PES | Ag-coated yarn | Heating | 2-P probe (parallel electrodes) | ρs = 0.15 Ω/sq | [102] |
| Half-Milano rib | PA6.6 PU | Ag-coated yarn | Heating | 2-P probe (parallel electrodes) | Rs = 0.14 Ω | [103] |
| Weft-knitted jacquard | Merino WO | Ag-coated yarn | Heating | Two-point probe (parallel electrodes) | Rs = 1.91 Ω | [104] |
| Knit, knit-and-float, and knit-and-tuck stitches | WO yarn/PES yarn | Ag-coated yarn | Heating | 2-P probe | Rs = 5.7–25.5 Ω | [105] |
| Knit, tuck, and float | WO yarn | Ag-coated yarn | Conductive | 2-P probe | Rs = 1–1.5 Ω | [106] |
| Unspecified | Unspecified | Ag-coated yarn | Conductive | Eddy current | Rsh = 0.40 Ω/sq | [26] |
| Plain weft knitted | None | Ag-coated yarn | Conductive | Van der Pauw | Rs = 0.03–0.5 Ω | [27] |
| Unspecified | None | Ag metalized | Conveying electrical signals | Van der Pauw | Rs = 10−1 Ω | [22] |
| Unspecified | antibacterial PA/crisscross-section PES | SS | Conductive/Antimicrobial | 2-P probe (concentric ring) | ρs = 109–1010 Ω/sq | [107] |
| 1 × 1 rib | CO/Bamboo/PES | Ag-coated yarn | EMI shielding | 2-P probe | ρs = 107–1010 Ω/sq ρv = 107–109 Ω·cm | [108] |
| Unspecified | Bamboo charcoal | SS | EMI shielding | 2-P probe (concentric ring) | ρs = 107 Ω/sq | [93] |
| Interlock | CO | SS, Cu, and Ag-coated Cu wires (core) | EMI shielding | 2-P probe (concentric ring) | ρs = 104–105 Ω | [109] |
| Plain jersey | PP | SS | EMI shielding | 2-P probe | ρs = 107–108 Ω ρv = 106–108 Ω·cm | [94] |
| Nonwoven Fabrication Technique | Fabric Composition | Application (Function) | Testing Method | Electrical Properties | Ref. | |
|---|---|---|---|---|---|---|
| Nonconductive Elements | Conductive Fibers | |||||
| Wet laid | Xanthan gum | CF | Heating | 4-P probe | ρs = 10–27 Ω/sq | [111] |
| Needle punching | PP/PE core/sheath bicomponent fiber | CF | Unspecified | 4-P probe | σ = 13 S/cm | [112] |
| Needle punching | PP fiber | CF | EMI shielding | 2-P probe | ρs = 3.35 kΩ | [113] |
| Needle punching | None | Ag-coated fibers | EMI shielding | 2-P probe | ρs = 1.03 kΩ | [114] |
| Needle punching | PA6 fiber | Recycled CF | EMI shielding and sound absorption | 4-P probe | σ = 33.83 S/m | [115] |
| Substrate | Fabric Composition | Application (Function) | Testing Method | Electrical Properties | Ref. | |
|---|---|---|---|---|---|---|
| Conductive Treatment Method | Conductive Material | |||||
| PES woven fabric | In situ polymerization | Aniline and Py | Supercapacitors | 4-P probe | σ = 1.44 × 10−2 S/cm | [129] |
| CO woven fabric | Electroless plating | Ag | Ultrahigh washability | 4-P probe | Rsh = 0.33–2.49 Ω/sq | [116] |
| CO woven fabric | Dip-coating | MXene (Ti3C2Tx) | Strain sensors | 4-P probe | ρv = 1.52 × 10−2 Ω m | [119] |
| CO woven fabrics | Dip-and-dry | PDA and SWCNTs | EMI shielding | Van der Pauw | Rs = 9 ± 2 Ω/sq | [131] |
| CO woven fabric | In situ polymerization | PPy/AgNWs | Joule heating/ Photothermal | 4-P probe | σ = 1.97 × 104 S/m | [130] |
| PES warp-knit | In situ polymerization | PPy/Ag | Conductive and antimicrobial | 4-P probe | Rsh = 61.54 Ω/sq | [132] |
| CO knit | In situ polymerization | PPy | Heating | 4-P probe | ρs = 59.9 ± 13.6 Ω/sq | [126] |
| Plain, basket & twill woven Single jersey weft-knit | In situ polymerization | PPy | Conductive | 2-P probe | σ = 10−3–10−6 S/sq | [127] |
| Rib stitch knitted | Spray-drying coating | CNTs and GNs | Heating/strain sensor | 2-P probe | σ = 36.5 S/m | [121] |
| SA/FRV weft-knit | Spray-drying | MXene | EMI shielding/ Joule heating/ Photothermal | 4-P probe | Rsh = 2 Ω/sq | [137] |
| PLA nonwoven | Padding | MWCNTs | Conductive/antimicrobial | 4-P probe | Rsh = 77–681 Ω/sq | [122] |
| PPS needle punching nonwoven | In situ polymerization | PPy | Heating | 4-P probe | ρs = 4.5–8.8 Ω/sq | [134] |
| Aramid nonwoven | Dip-coating | PEDOT: PSS/AgNWs | EMI shielding/Heating | 4-P probe | Rsh = 0.92 ± 0.06 Ω/sq | [133] |
| CO nonwoven | Dipping | Graphene | Heating | 2-P probe | ρs = 727.57 Ω/sq | [124] |
| Aramid spunlaced nonwoven | Spraying–drying | MXene | EMI shielding/ Joule heating/ Photothermal | 4-P probe | Rsh = 4.36 Ω/sq | [135] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, Z.; Ventura, H.; Ardanuy, M. A Review of the Electrical Conductivity Test Methods for Conductive Fabrics. Textiles 2024, 4, 284-308. https://doi.org/10.3390/textiles4030017
Xie Z, Ventura H, Ardanuy M. A Review of the Electrical Conductivity Test Methods for Conductive Fabrics. Textiles. 2024; 4(3):284-308. https://doi.org/10.3390/textiles4030017
Chicago/Turabian StyleXie, Zeyue, Heura Ventura, and Monica Ardanuy. 2024. "A Review of the Electrical Conductivity Test Methods for Conductive Fabrics" Textiles 4, no. 3: 284-308. https://doi.org/10.3390/textiles4030017
APA StyleXie, Z., Ventura, H., & Ardanuy, M. (2024). A Review of the Electrical Conductivity Test Methods for Conductive Fabrics. Textiles, 4(3), 284-308. https://doi.org/10.3390/textiles4030017







