Contrasting Toxicity of a Fomesafen-Based Herbicide on Three Freshwater Phytoplanktonic Species
Abstract
1. Introduction
2. Results
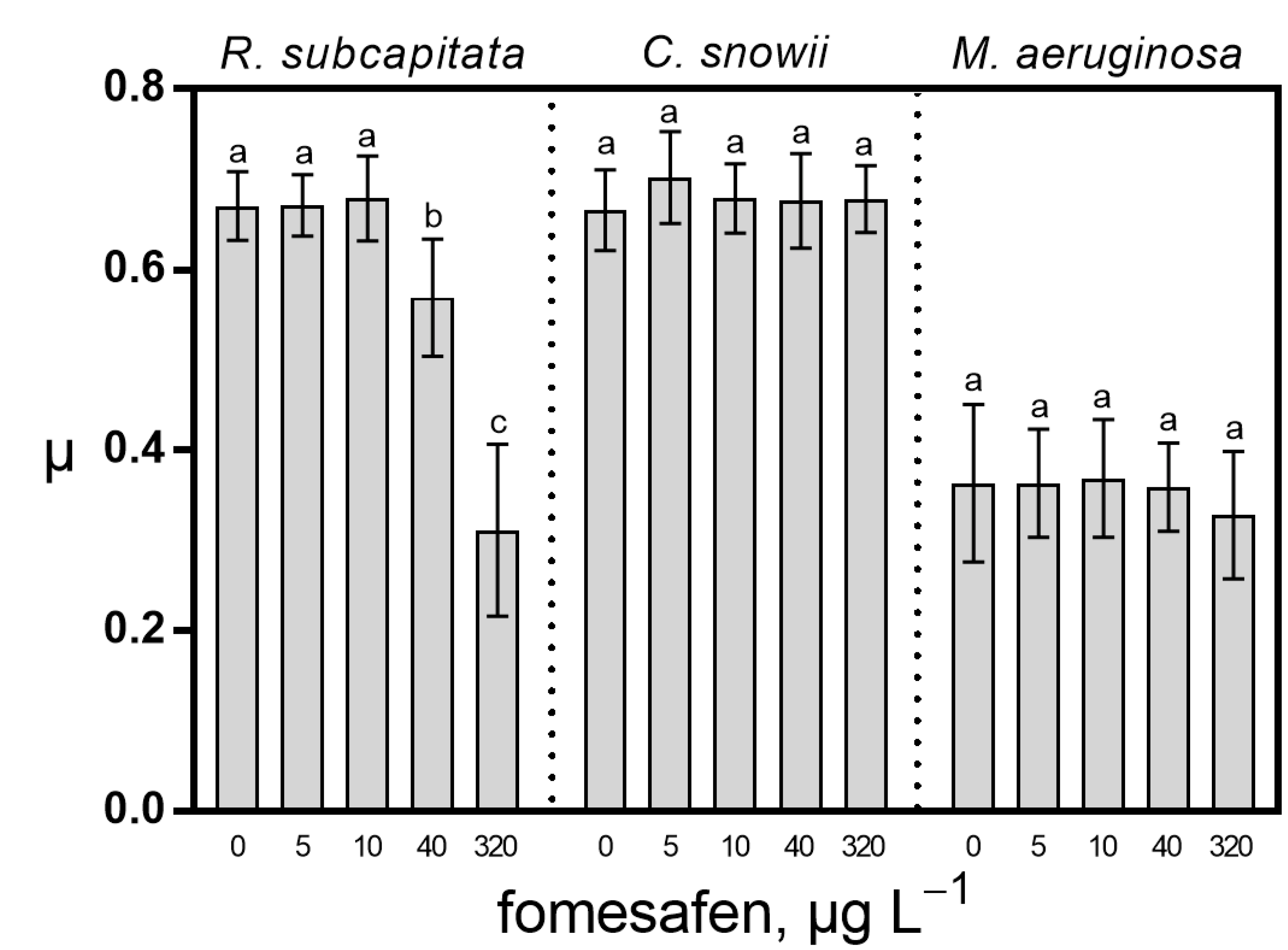
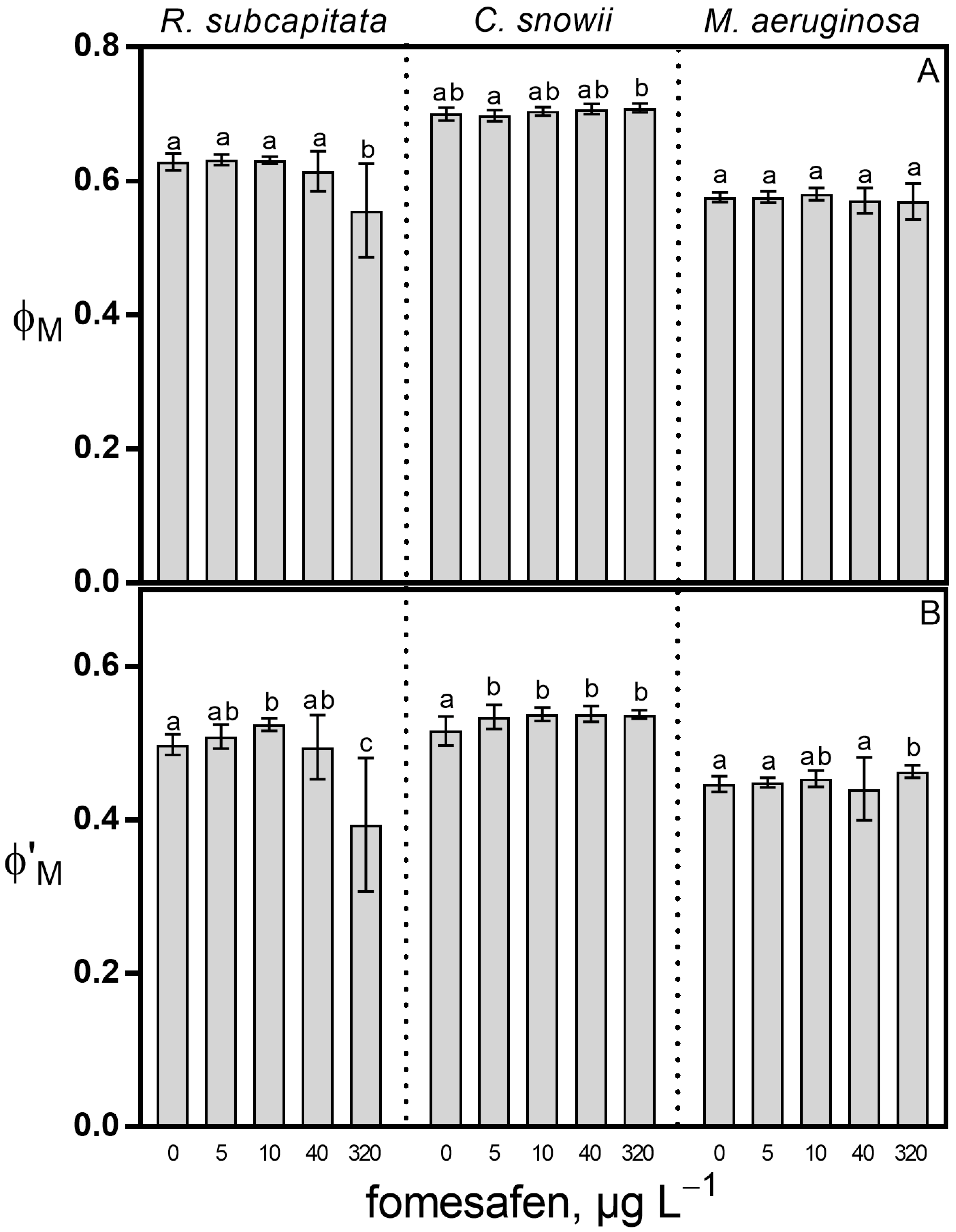
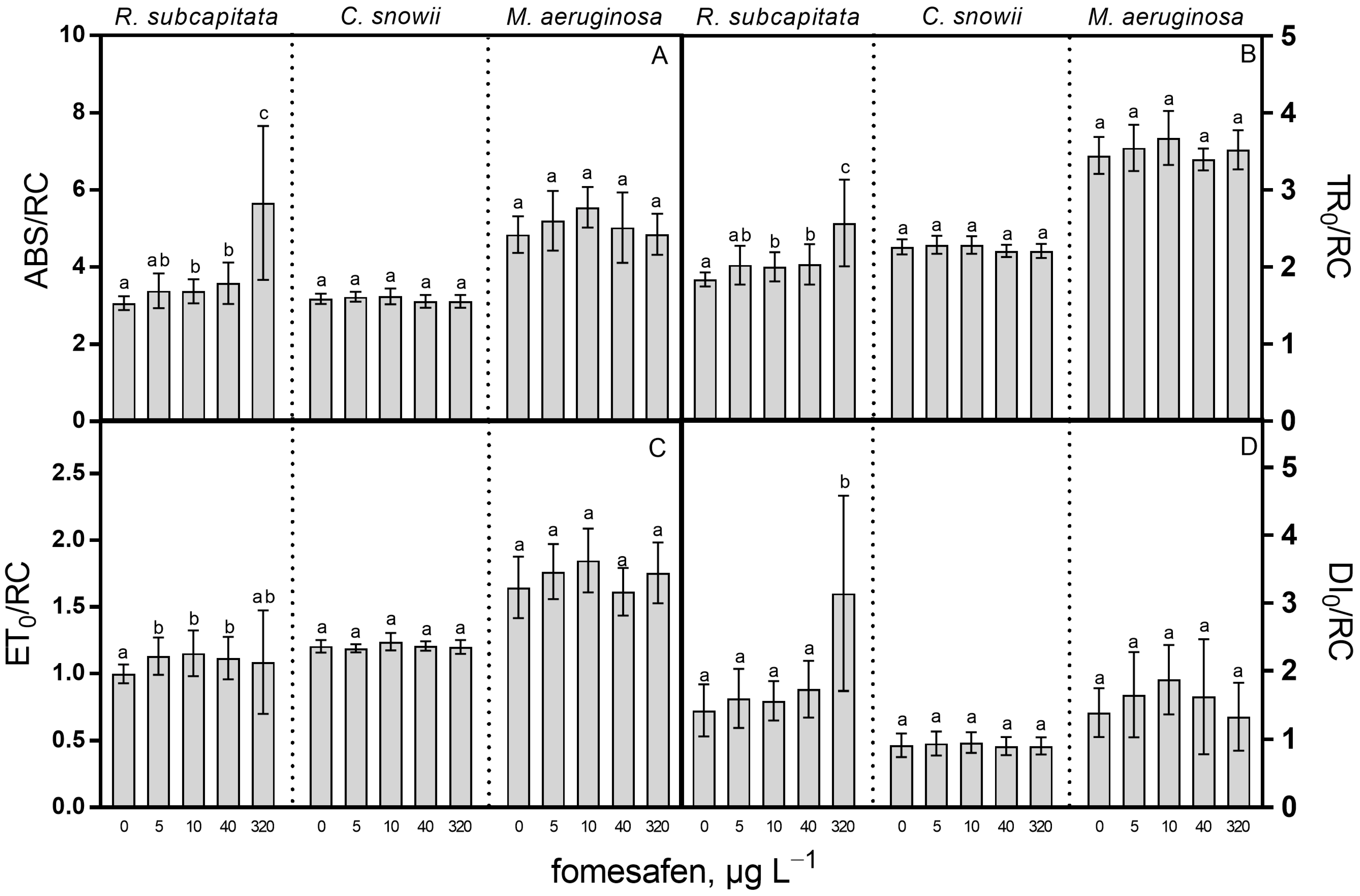
2.1. Growth Rate and Photosynthesis
2.2. Pigment Content
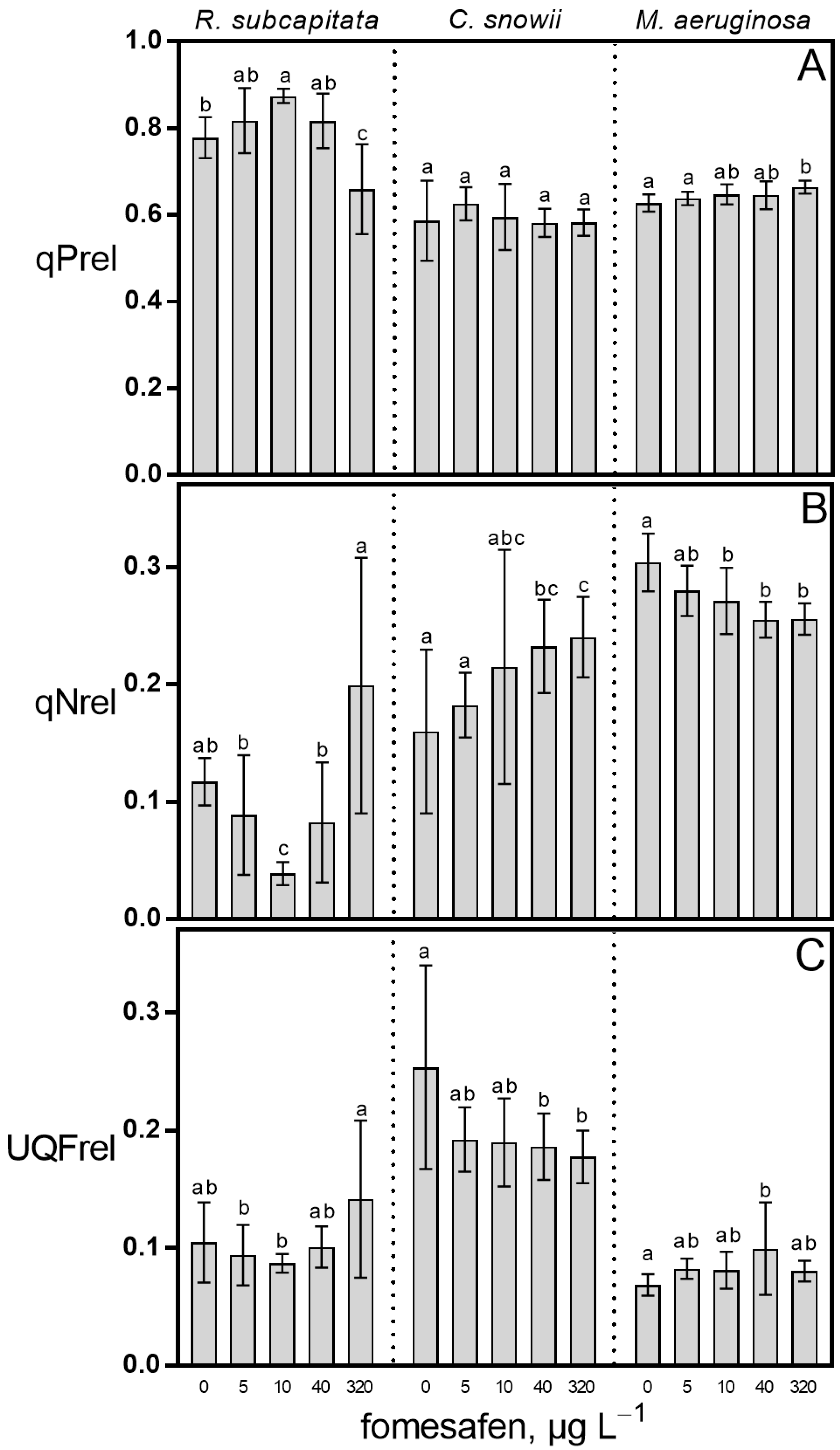
2.3. Oxidative Stress and Cell Complexity
2.4. Cell Biovolume
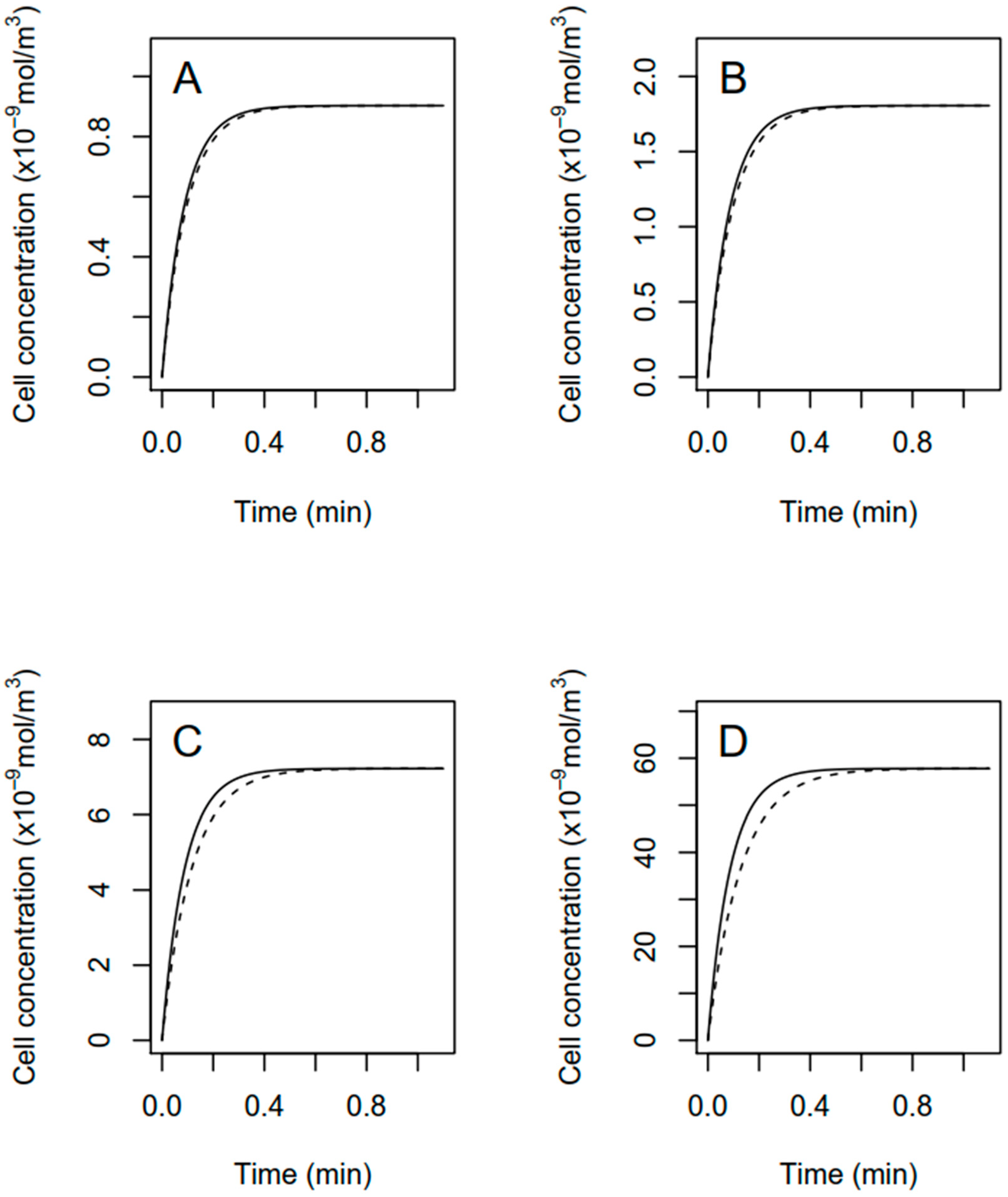
2.5. Modeling of Fomesafen Uptake
3. Discussion
3.1. Fomesafen-Based Herbicide Toxicity to Phytoplankton Physiology
3.2. Modeling of Fomesafen Uptake in R. subcapitata
4. Material and Methods
4.1. Phytoplanktonic Cultures and Growth Conditions
4.2. Herbicide Solutions and Fomesafen Exposure
4.3. Growth and Cell Biovolume Assessments
4.4. Photosystem II Energy Flux Analysis
4.5. Photosynthetic Electron Transport and Fluorescence Quenching Analysis
4.6. Photosystem I Cyclic Electron Flow Measurement
4.7. Pigment Content Analysis
4.8. Reactive Oxygen Species Content and Cell Complexity Determination
4.9. Modeling Fomesafen Uptake in Phytoplankton
4.10. Statistical Analysis
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bond, J.A.; Oliver, L.R.; Stephenson, D.O. Response of Palmer Amaranth (Amaranthus palmeri) Accessions to Glyphosate, Fomesafen, and Pyrithiobac. Weed Technol. 2006, 20, 885–892. [Google Scholar] [CrossRef]
- Chahal, P.S.; Varanasi, V.K.; Jugulam, M.; Jhala, A.J. Glyphosate-Resistant Palmer Amaranth (Amaranthus palmeri) in Nebraska: Confirmation, EPSPS Gene Amplification, and Response to POST Corn and Soybean Herbicides. Weed Technol. 2017, 31, 80–93. [Google Scholar] [CrossRef]
- Sarangi, D.; Sandell, L.D.; Kruger, G.R.; Knezevic, S.Z.; Irmak, S.; Jhala, A.J. Comparison of Herbicide Programs for Season-Long Control of Glyphosate-Resistant Common Waterhemp (Amaranthus rudis) in Soybean. Weed Technol. 2017, 31, 53–66. [Google Scholar] [CrossRef]
- Matringe, M.; Camadro, J.M.; Labbe, P.; Scalla, R. Protoporphyrinogen oxidase as a molecular target for diphenyl ether herbicides. Biochem. J. 1989, 260, 231–235. [Google Scholar] [CrossRef] [PubMed]
- Bridges, D.; Stephenson, M. Weed Control and Tobacco (Nicotiana tabacum) Tolerance with Fomesafen. Weed Technol. 1991, 5, 868–872. [Google Scholar] [CrossRef]
- Kleifeld, Y.; Blumenfeld, T.; Herzlinger, G.; Graph, S.; Buxbaum, H.; Bargutti, A. The use of fomesafen for pre-emergence weed control in cotton. Phytoparasitica 1988, 16, 133–144. [Google Scholar] [CrossRef]
- Kurtz, M.E. Kenaf tolerance to acifluorfen, cyanazine, diuron, fluometuron, fomesafen, lactofen, or prometryn applied postemergence-directed. Ind. Crops Prod. 1996, 5, 119–124. [Google Scholar] [CrossRef]
- Soltani, N.; Shropshire, C.; Sikkema, P.H. Effects of post-emergence application of bentazon and fomesafen on eight market classes of dry beans (Phaseolus vulgaris L.). Crop Prot. 2006, 25, 826–830. [Google Scholar] [CrossRef]
- Caquet, T. Use of carbon and nitrogen stable isotope ratios to assess the effects of environmental contaminants on aquatic food webs. Environ. Pollut. 2006, 141, 54–59. [Google Scholar] [CrossRef]
- Caquet, T.; Deydier-Stephan, L.; Lacroix, G.; Le Rouzic, B.; Lescher-Moutoué, F. Effects of fomesafen, alone and in combination with an adjuvant, on plankton communities in freshwater outdoor pond mesocosms. Environ. Toxicol. Chem. 2005, 24, 1116–1124. [Google Scholar] [CrossRef]
- Perschbacher, P.W.; Stone, N.; Ludwig, G.M.; Guy, C.B., Jr. Evaluation of effects of common aerially-applied soybean herbicides and propanil on the plankton communities of aquaculture ponds. Aquaculture 1997, 157, 117–122. [Google Scholar] [CrossRef]
- USEPA. Environmental fate, ecological risk and endangered species assessment in support of the registration review of fomesafen sodium (PC123802). In Environmental Fate and Effects Division Environmental Risk Branch I; United States Environmental Protection Agency: Washington, DC, USA, 2008. [Google Scholar]
- Minnesota Department of Agriculture (MDA). Water Quality Monitoring Report; MDA: St. Paul, MN, USA, 2020. Available online: https://www.mda.state.mn.us/pesticide-fertilizer/water-monitoring-reports-and-resources (accessed on 15 March 2022).
- Yu, X.B.; Hao, K.; Ling, F.; Wang, G.X. Aquatic environmental safety assessment and inhibition mechanism of chemicals for targeting Microcystis aeruginosa. Ecotoxicology 2014, 23, 1638–1647. [Google Scholar] [CrossRef] [PubMed]
- Boger, P.; Wakabayashi, K. Peroxidizing Herbicides (I): Mechanism of Action. Z. Nat.-Sect. C J. Biosci. 1995, 50, 159–166. [Google Scholar] [CrossRef][Green Version]
- Ledford, H.K.; Niyogi, K.K. Singlet oxygen and photo-oxidative stress management in plants and algae. Plant Cell Environ. 2005, 28, 1037–1045. [Google Scholar] [CrossRef]
- Niyogi, K.K. Photoprotection revisited: Genetic and molecular approaches. Annu. Rev. Plant Biol. 1999, 50, 333–359. [Google Scholar] [CrossRef]
- Niyogi, K.K. Safety valves for photosynthesis. Curr. Opin. Plant Biol. 2000, 3, 455–460. [Google Scholar] [CrossRef] [PubMed]
- Stachowski-Haberkorn, S.; Jérôme, M.; Rouxel, J.; Khelifi, C.; Rincé, M.; Burgeot, T. Multigenerational exposure of the microalga Tetraselmis suecica to diuron leads to spontaneous long-term strain adaptation. Aquat. Toxicol. 2013, 140–141, 380–388. [Google Scholar] [CrossRef]
- Force, L.; Critchley, C.; van Rensen, J.J. New fluorescence parameters for monitoring photosynthesis in plants. Photosynth. Res. 2003, 78, 17–33. [Google Scholar] [CrossRef]
- Buschmann, C. Variation of the Quenching of Chlorophyll Fluorescence under Different Intensities of the Actinic Light in Wildtype Plants of Tobacco and in an Aurea Mutant Deficient of LightHarvesting-Complex. J. Plant Physiol. 1995, 145, 245–252. [Google Scholar] [CrossRef]
- Juneau, P.; Green, B.R.; Harrison, P.J. Simulation of Pulse-Amplitude-Modulated (PAM) fluorescence: Limitations of some PAM-parameters in studying environmental stress effects. Photosynthetica 2005, 43, 75–83. [Google Scholar] [CrossRef]
- Allen, J.F.; Forsberg, J. Molecular recognition in thylakoid structure and function. Trends Plant Sci. 2001, 6, 317–326. [Google Scholar] [CrossRef]
- Govindjee, G. A Role for a Light-Harvesting Antenna Complex of Photosystem II in Photoprotection. Plant Cell 2002, 14, 1663–1668. [Google Scholar] [CrossRef]
- Alric, J. Cyclic electron flow around photosystem I in unicellular green algae. Photosynth. Res. 2010, 106, 47–56. [Google Scholar] [CrossRef] [PubMed]
- Bendall, D.S.; Manasse, R.S. Cyclic photophosphorylation and electron transport. Biochim. Biophys. Acta 1995, 1229, 23–38. [Google Scholar] [CrossRef]
- Alric, J.; Lavergne, J.; Rappaport, F. Redox and ATP control of photosynthetic cyclic electron flow in Chlamydomonas reinhardtii (I) aerobic conditions. Biochim. Biophys. Acta 2010, 1797, 44–51. [Google Scholar] [CrossRef] [PubMed]
- Finazzi, G.; Furia, A.; Barbagallo, R.P.; Forti, G. State transitions, cyclic and linear electron transport and photophosphorylation in Chlamydomonas reinhardtii. Biochim. Biophys. Acta Bioenerg. 1999, 1413, 117–129. [Google Scholar] [CrossRef]
- Munekage, Y.; Hojo, M.; Meurer, J.; Endo, T.; Tasaka, M.; Shikanai, T. PGR5 is involved in cyclic electron flow around photosystem I and is essential for photoprotection in Arabidopsis. Cell 2002, 110, 361–371. [Google Scholar] [CrossRef]
- Huang, W.; Yang, Y.-J.; Zhang, S.-B.; Liu, T. Cyclic Electron Flow around Photosystem I Promotes ATP Synthesis Possibly Helping the Rapid Repair of Photodamaged Photosystem II at Low Light. Front. Plant Sci. 2018, 9, 239. [Google Scholar] [CrossRef]
- Joliot, P.; Joliot, A. Cyclic electron transfer in plant leaf. Proc. Natl. Acad. Sci. USA 2002, 99, 10209–10214. [Google Scholar] [CrossRef]
- Franqueira, D.; Cid, A.; Torres, E.; Orosa, M.; Herrero, C. A comparison of the relative sensitivity of structural and functional cellular responses in the alga chlamydomonas eugametos exposed to the herbicide paraquat. Arch. Environ. Contam. Toxicol. 1999, 36, 264–269. [Google Scholar] [CrossRef]
- Asselborn, V.; Fernández, C.; Zalocar, Y.; Parodi, E.R. Effects of chlorpyrifos on the growth and ultrastructure of green algae, Ankistrodesmus gracilis. Ecotoxicol. Environ. Saf. 2015, 120, 334–341. [Google Scholar] [CrossRef] [PubMed]
- DeLorenzo, M.E.; Leatherbury, M.; Weiner, J.A.; Lewitus, A.J.; Fulton, M.H. Physiological factors contributing to the species-specific sensitivity of four estuarine microalgal species exposed to the herbicide atrazine. Aquat. Ecosyst. Health Manag. 2004, 7, 137–146. [Google Scholar] [CrossRef]
- Tang, J.; Hoagland, K.D.; Siegfried, B.D. Uptake and bioconcentration of atrazine by selected freshwater algae. Environ. Toxicol. Chem. 1998, 17, 1085–1090. [Google Scholar] [CrossRef]
- Kent, R.A.; Currie, D. Predicting algal sensitivity to a pesticide stress. Environ. Toxicol. Chem. 1995, 14, 983–991. [Google Scholar] [CrossRef]
- Kato, K.; Tanaka, R.; Sano, S.; Tanaka, A.; Hosaka, H. Identification of a gene essential for protoporphyrinogen IX oxidase activity in the cyanobacterium Synechocystis sp. PCC6803. Proc. Natl. Acad. Sci. USA 2010, 107, 16649–16654. [Google Scholar] [CrossRef]
- Deblois, C.P.; Marchand, A.; Juneau, P. Comparison of photoacclimation in twelve freshwater photoautotrophs (chlorophyte, bacillaryophyte, cryptophyte and cyanophyte) isolated from a natural community. PLoS ONE 2013, 8, e57139. [Google Scholar] [CrossRef]
- Stein, J. Handbook of Phycological Methods: Culture Methods and Growth Measurements; Cambridge University Press: London, UK, 1973; p. 448. [Google Scholar]
- Wood, A.; Everroad, R.C.; Wingard, L.M. Measuring Growth Rates in Microalgal Cultures. In Algal Culturing Techniques; Andersen, R.A., Ed.; Academic Press: Cambridge, MA, USA, 2005. [Google Scholar]
- Schreiber, U.; Schliwa, U.; Bilger, W. Continuous recording of photochemical and non-photochemical chlorophyll fluorescence quenching with a new type of modulation fluorometer. Photosynth. Res. 1986, 10, 51–62. [Google Scholar] [CrossRef]
- Kitajima, M.; Butler, W. Quenching of chlorophyll fluorescence and primary photochemistry in chloroplasts by dibromothymoquinone. Biochim. Biophys. Acta BBA Bioenerg. 1975, 376, 105–115. [Google Scholar] [CrossRef]
- Genty, B.; Briantais, J.-M.; Baker, N.R. The relationship between the quantum yield of photosynthetic electron transport and quenching of chlorophyll fluorescence. Biochim. Biophys. Acta BBA Gen. Subj. 1989, 990, 87–92. [Google Scholar] [CrossRef]
- Joliot, P.; Joliot, A. Quantification of cyclic and linear flows in plants. Proc. Natl. Acad. Sci. USA 2005, 102, 4913–4918. [Google Scholar] [CrossRef]
- Du, J.; Qiu, B.; Gomes, M.P.; Juneau, P.; Dai, G. Influence of light intensity on cadmium uptake and toxicity in the cyanobacteria Synechocystis sp. PCC6803. Aquat. Toxicol. 2019, 211, 163–172. [Google Scholar] [CrossRef]
- Lichtenthaler, H.K. Chlorophylls and Carotenoids: Pigments of Photosynthetic Biomembranes. In Methods in Enzymology; Academic Press: Cambridge, MA, USA, 1987; Volume 148, pp. 350–382. [Google Scholar]
- Weber, J.B. Ionization and sorption of fomesafen and atrazine by soils and soil constituents. Pestic. Sci. 1993, 39, 31–38. [Google Scholar] [CrossRef]
- Boullemant, A.; Lavoie, M.; Fortin, C.; Campbell, P.G. Uptake of Hydrophobic Metal Complexes by Three Freshwater Algae: Unexpected Influence of pH. Environ. Sci. Technol. 2009, 43, 3308–3314. [Google Scholar] [CrossRef] [PubMed]
- Lavoie, M.; Duval, J.F.L.; Raven, J.A.; Maps, F.; Béjaoui, B.; Kieber, D.J.; Vincent, W.F. Carbonate Disequilibrium in the External Boundary Layer of Freshwater Chrysophytes: Implications for Contaminant Uptake. Environ. Sci. Technol. 2018, 52, 9403–9411. [Google Scholar] [CrossRef] [PubMed]
- Lavoie, M.; Galí, M.; Sévigny, C.; Kieber, D.J.; Sunda, W.G.; Spiese, C.E.; Maps, F.; Levasseur, M. Modelling dimethylsulfide diffusion in the algal external boundary layer: Implications for mutualistic and signalling roles. Environ. Microbiol. 2018, 20, 4157–4169. [Google Scholar] [CrossRef]
- Walter, A.; Gutknecht, J. Permeability of small nonelectrolytes through lipid bilayer membranes. J. Membr. Biol. 1986, 90, 207–217. [Google Scholar] [CrossRef]
- Tomlin, C. Entry 571. In The Pesticide Manual: A World Compendium; The British Crop Protection Council: Farnham, UK; The Royal Society of Chemistry: London, UK, 1994; pp. 832–833. [Google Scholar]
- Hayduk, W.; Laudie, H. Prediction of diffusion coefficients for nonelectrolytes in dilute aqueous solutions. AIChE J. 1974, 20, 611–615. [Google Scholar] [CrossRef]
- Wolf-Gladrow, D.; Riebesell, U. Diffusion and reactions in the vicinity of plankton: A refined model for inorganic carbon transport. Mar. Chem. 1997, 59, 17–34. [Google Scholar] [CrossRef]
- Rawat, M.; Moroney, J.V. The regulation of carbonic anhydrase and ribulose-1,5-bisphosphate carboxylase/oxygenase activase by light and CO2 in Chlamydomonas reinhardtii. Plant Physiol. 1995, 109, 937–944. [Google Scholar] [CrossRef][Green Version]
- R Core Team: A Language and Environment for Statistical Computing. Available online: https://www.R-project.org/ (accessed on 27 November 2022).
- Soetaert, K.; Petzoldt, T.; Setzer, R.W. Solving differential equations in R: Package deSolve. J. Stat. Softw. 2010, 33, 1–25. [Google Scholar] [CrossRef]
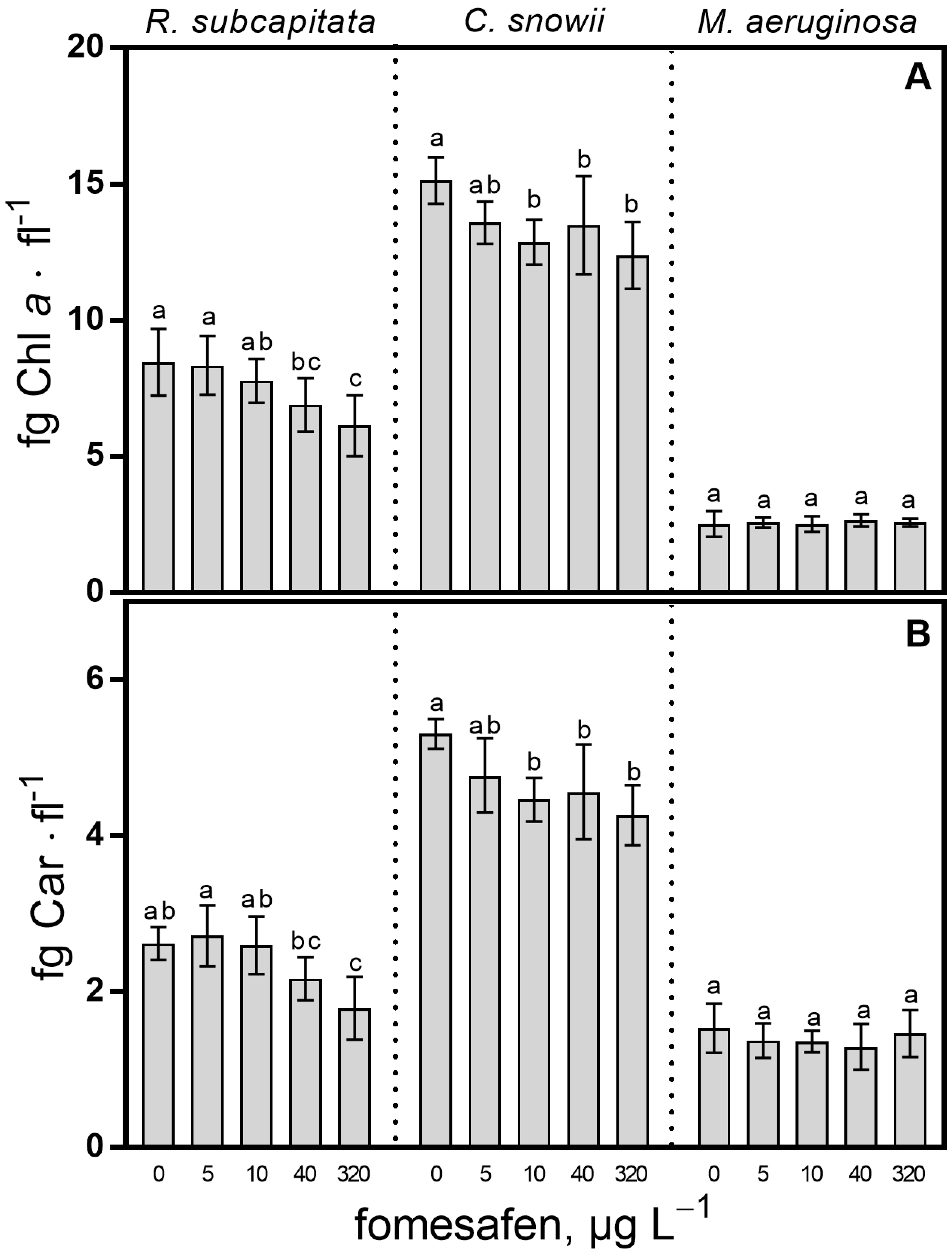
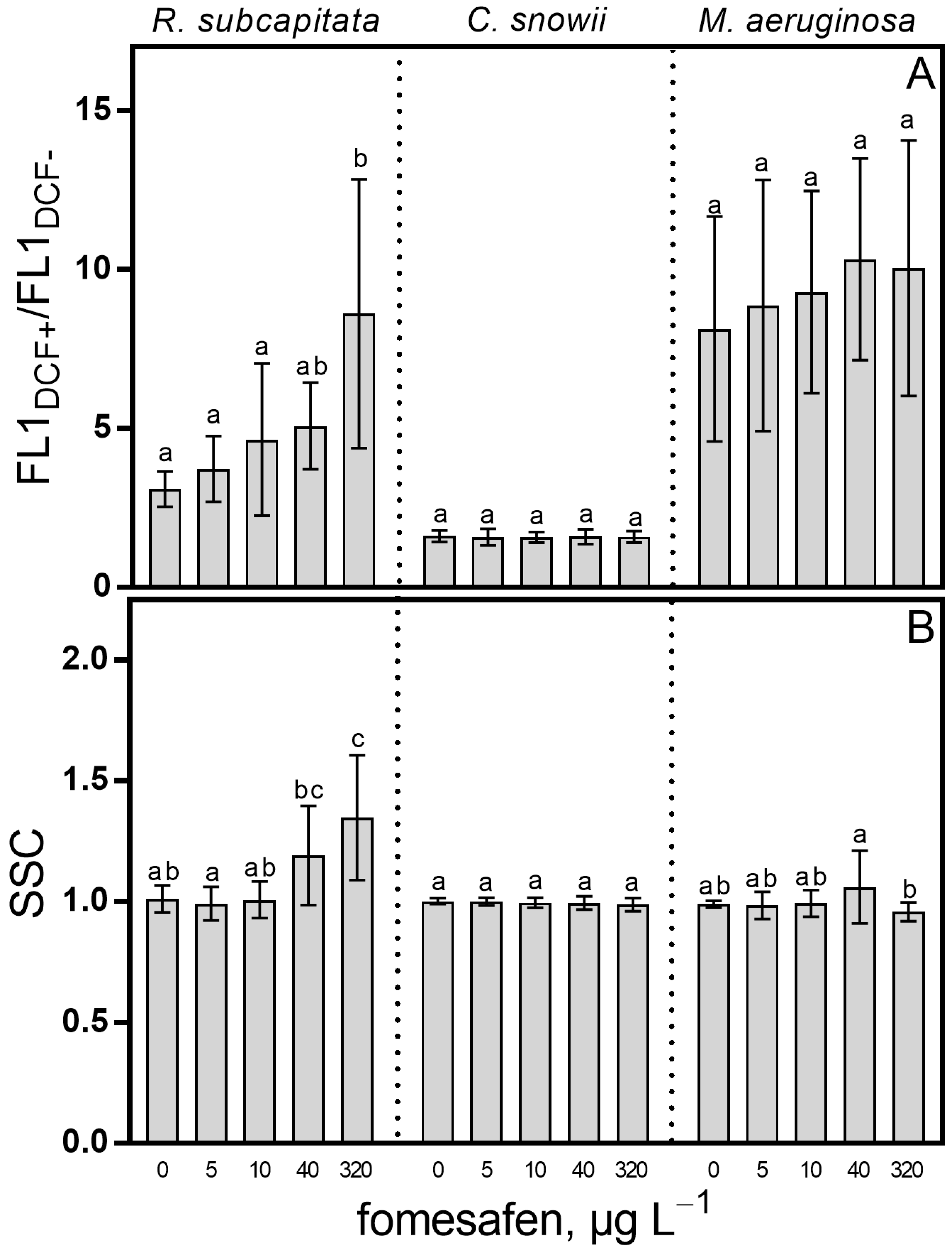
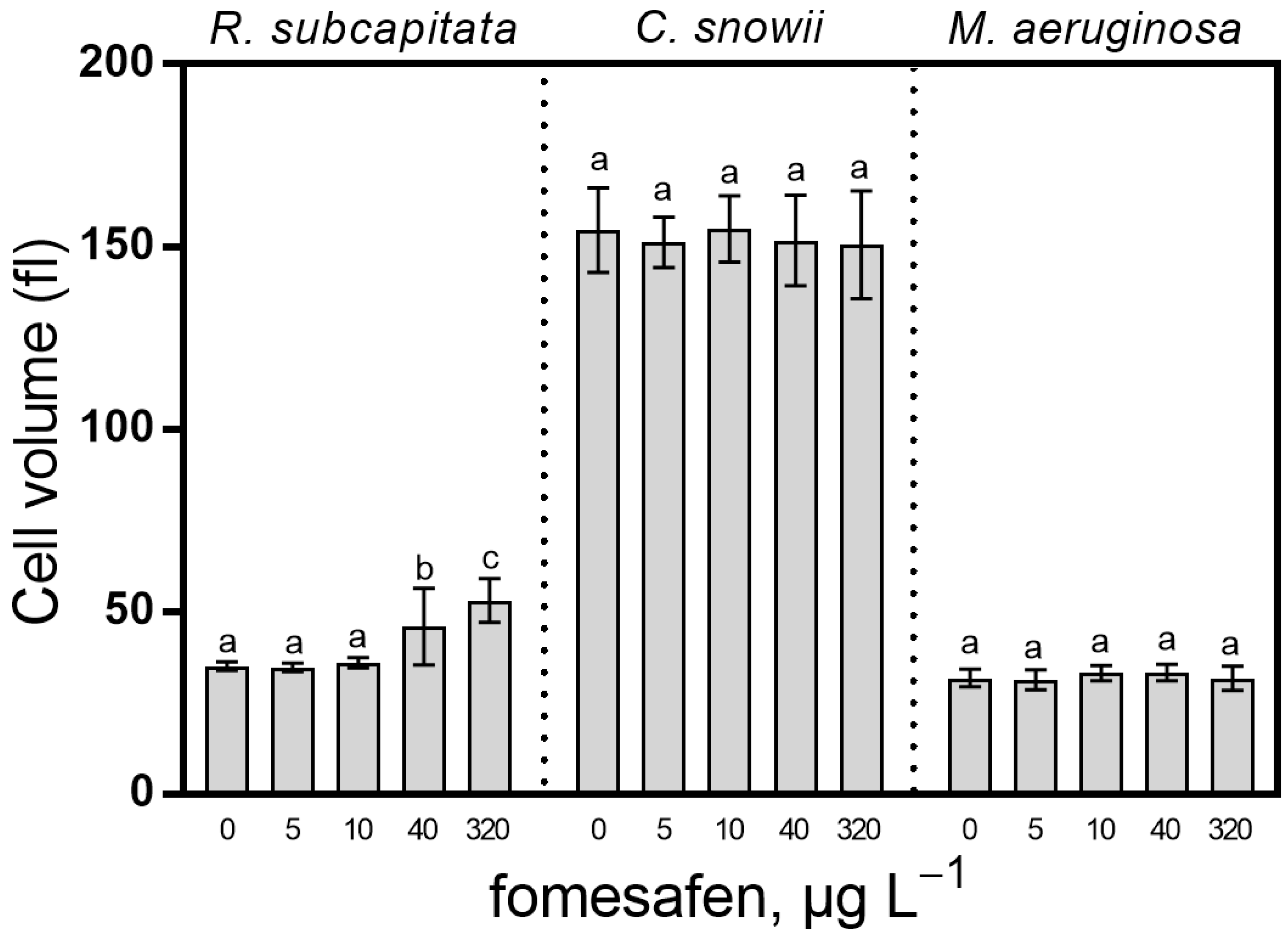
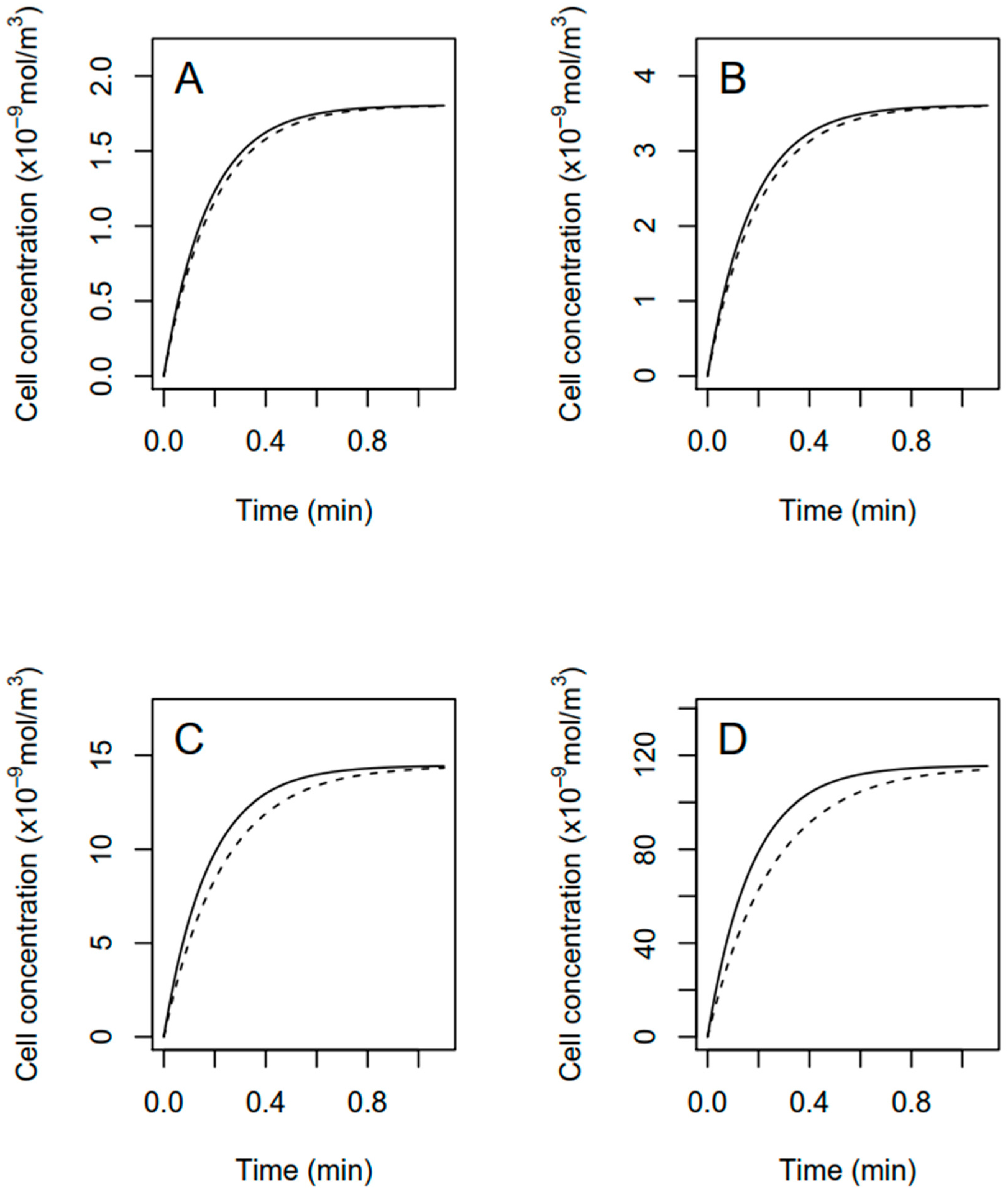

| Fomesafen a.i. (µg · L−1) | % Cyclic Electron Transport around PSI |
|---|---|
| 0 | 6.7 (4.9) a |
| 5 | 8.2 (3.4) a,b |
| 10 | 14.1 (3.0) b |
| 40 | 23.2 (7.9) c |
| 320 | N.d. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naoum, J.; Lavoie, M.; Gomes, M.P.; Juneau, P. Contrasting Toxicity of a Fomesafen-Based Herbicide on Three Freshwater Phytoplanktonic Species. Stresses 2023, 3, 102-124. https://doi.org/10.3390/stresses3010009
Naoum J, Lavoie M, Gomes MP, Juneau P. Contrasting Toxicity of a Fomesafen-Based Herbicide on Three Freshwater Phytoplanktonic Species. Stresses. 2023; 3(1):102-124. https://doi.org/10.3390/stresses3010009
Chicago/Turabian StyleNaoum, Jonathan, Michel Lavoie, Marcelo Pedrosa Gomes, and Philippe Juneau. 2023. "Contrasting Toxicity of a Fomesafen-Based Herbicide on Three Freshwater Phytoplanktonic Species" Stresses 3, no. 1: 102-124. https://doi.org/10.3390/stresses3010009
APA StyleNaoum, J., Lavoie, M., Gomes, M. P., & Juneau, P. (2023). Contrasting Toxicity of a Fomesafen-Based Herbicide on Three Freshwater Phytoplanktonic Species. Stresses, 3(1), 102-124. https://doi.org/10.3390/stresses3010009










