Equations for the Electron Density of the Two-Dimensional Electron Gas in Realistic AlGaN/GaN Heterostructures

Abstract

This paper presents equations for the electron density of the two-dimensional electron gas (2DEG) in AlGaN/GaN heterostructures in three realistic scenarios: (1) AlGaN/GaN heterostructure with surface exposed to ambient with mobile ions, (2) metal gate deposited on the AlGaN surface, and (3) a thick dielectric passivation layer on the AlGaN surface. To derive the equations, we analyzed these scenarios by applying Gauss’s law. In contrast to the idealistic models, our analysis shows that the 2DEG charge density is proportional to the difference between spontaneous polarization of AlGaN and GaN, whereas surprisingly, it is independent of the piezoelectric polarization.

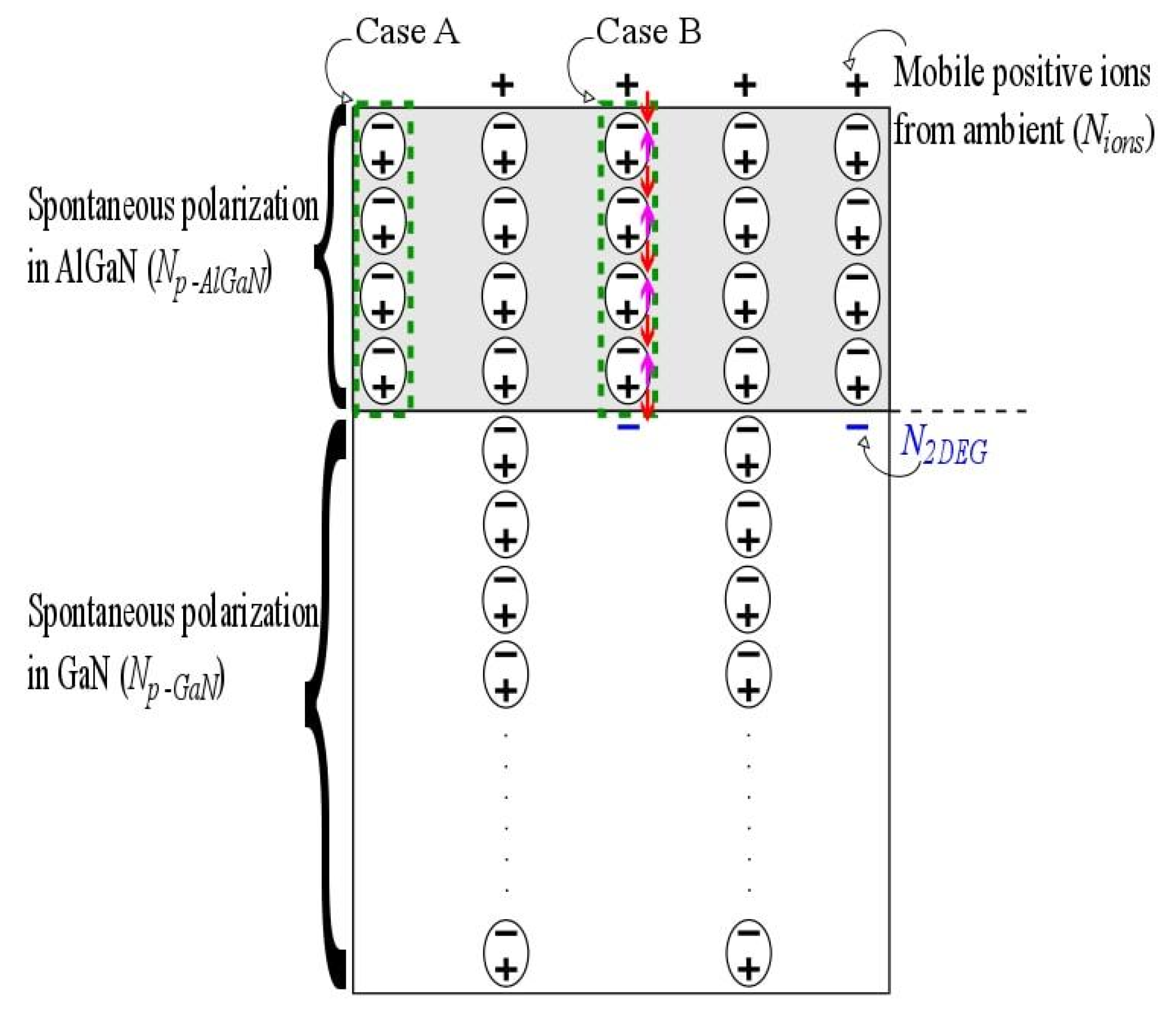
1. Introduction

Aluminium Gallium Nitride/Gallium Nitride (AlGaN/GaN) heterostructures are widely used in devices for high power and radio frequency applications because they enable higher current and faster switching speed [1,2,3,4,5]. Both capabilities are the results of high electron mobility and high electron density of the two-dimensional electron gas (2DEG), which is formed at the AlGaN/GaN heterojunction even in the absence of intentional doping [6]. The formation of 2DEG is considered to be due to the spontaneous and piezoelectric polarizations in the AlGaN/GaN heterostructure [7,8], however, the existing equations for the electron density in the 2DEG, N_2DEG, are inconsistent and confusing [6,9]. For example, the prevalent equations for N_2DEG do not include its dependence on the polarization charges [10,11]. This confusing result corresponds to the implicit assumption that the polarization charges at the AlGaN/GaN heterojunction and AlGaN surface balance each other [10,11]. It should be noted that there have been follow-up works to revise the original equations. In our approach presented herein, we analyze the charge balance for the following three realistic scenarios:

(1)

AlGaN surface is unpassivated and accessible by free ions from the ambient;

(2)

Metal gate is deposited on the AlGaN surface;

(3)

The AlGaN surface is passivated by a thick dielectric layer.

The results of this analysis are realistic equations for N_2DEG.

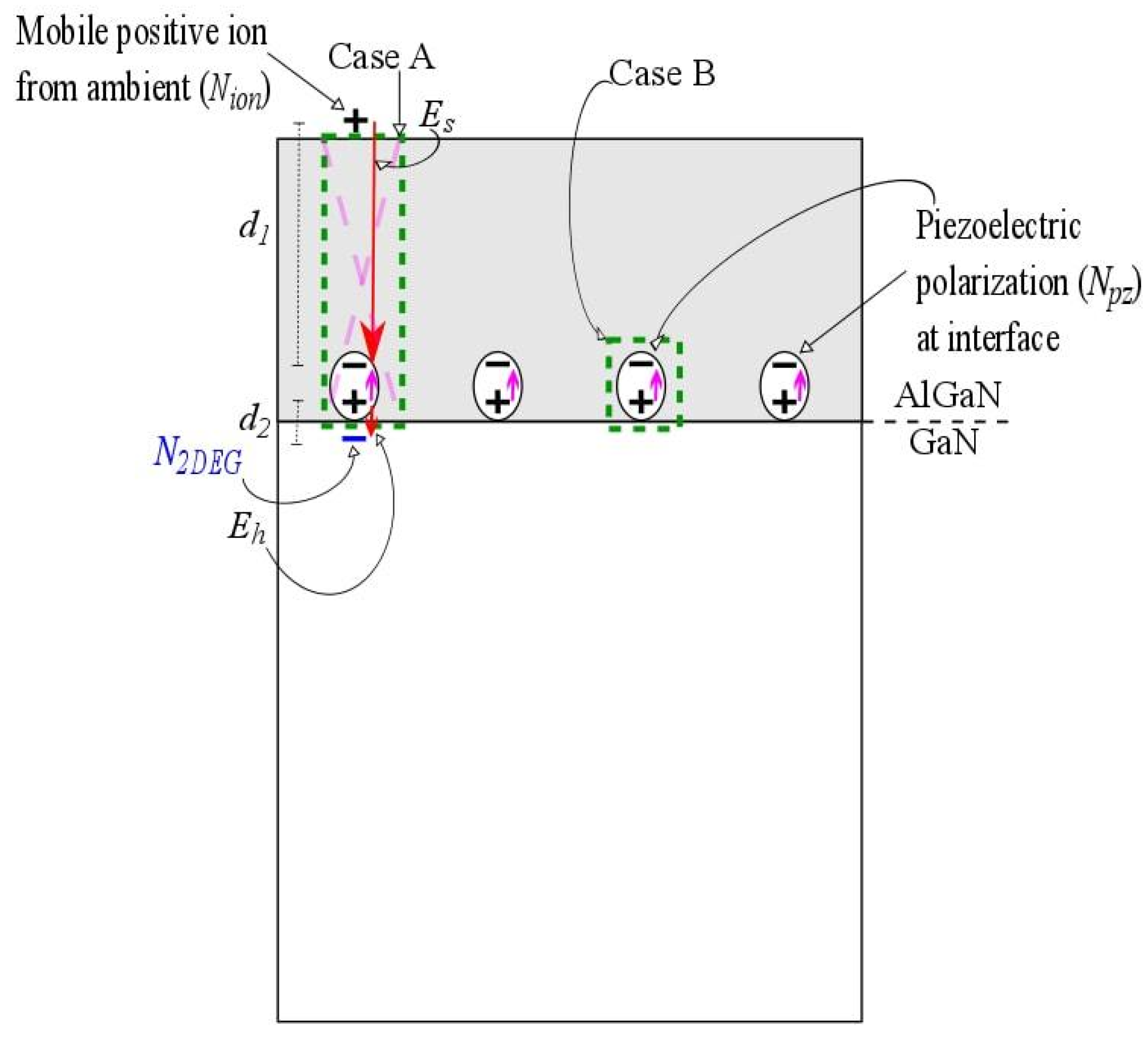
2. Equations for 2DEG in AlGan/GaN Heterostructures

2.1. Unpassivated AlGaN Surface Accessible to Free Ions from Air

GaN is a polar crystal because the formation of the interatomic bonds creates asymmetric electron clouds of Ga and N atoms [11], which is illustrated by the dipole symbols in Figure 1. This effect leads to the spontaneous polarization in GaN, which is quantified by the effective spontaneous polarization charge per unit area, N_p-GaN. In AlGaN, the polarization effect is analogous, with the difference that the spontaneous polarization charge per unit area (N_p-AlGaN) is larger [9,12,13]. The fact that N_p-AlGaN > N_p-GaN is illustrated in Figure 1 by showing five columns of dipoles in AlGaN and three columns of dipoles in GaN. The arrows in Figure 1 show electric field lines.

The statement by Ibbetson et al. that “polarization charge in AlGaN is balanced” [10] implies that there is no field outside the AlGaN layer and that there is no impact on N_2DEG from the spontaneous polarization charge. This result can be obtained by applying Gauss’s theorem in a way that is illustrated by the dashed rectangle labeled as Case A in Figure 1. However, this case excludes the possibility that the positive polarization charge at the heterojunction can attract mobile electrons to the 2DEG and that, likewise, the negative polarization charge at the AlGaN surface can attract positive mobile ions from the ambient. The dashed rectangle in case B shows the application of Gauss’s law that includes electrons next to the heterojunction and positive ions at the AlGaN surface. The total charge inside the Gauss’s rectangle is equal to zero in both cases, which means that the following Gauss’s equation applies to each of them:

$${\mathsf{\epsilon }}_{\mathrm{AlGaN}}{\mathrm{E}}_{\mathrm{s}}-{\mathsf{\epsilon }}_{\mathrm{AlGaN}}{\mathrm{E}}_{\mathrm{h}}=0$$

(1)

In (1), E_s is the electric field at the AlGaN surface, E_h is the electric field at the AlGaN/GaN heterojunction, and ${\mathsf{\epsilon }}_{\mathrm{AlGaN}}$ is the permittivity of AlGaN. Case A, which excludes both the electrons next to the heterojunction and the positive ions at the surface, corresponds to E_s = 0 and E_h = 0. In case B, the heterojunction and the surface fields are equal to each other, E_s = E_h, but they are not equal to zero. The field E_s attracts mobile positive ions from the ambient, whereas the field E_h attracts mobile electrons to the 2DEG. This means that the density of 2DEG electrons balances the difference between the spontaneous polarizations of AlGaN and GaN:

$${\mathrm{N}}_{2\mathrm{DEG}}={\mathrm{N}}_{\mathrm{p}-\mathrm{AlGaN}}-{\mathrm{N}}_{\mathrm{p}-\mathrm{GaN}}$$

(2)

It should be noted that the potential impact of the piezoelectric polarization charge, N_pz, is not included by the analysis based on Figure 1. However, the AlGaN bonds near the heterojunction are strained, which distorts the electron-cloud asymmetry of the nonstrained AlGaN bonds and changes the spontaneous polarization charge, N_p-AlGaN, by the value of N_pz. Therefore, the piezoelectric polarization charge can be modelled by additional dipoles next to the heterojunction, as illustrated in Figure 2. Case A shows that a mobile electron cannot be attracted to the heterojunction, and likewise, a mobile positive charge cannot be attracted to AlGaN surface due to the piezoelectric dipole. The charge enclosed by the dashed rectangle in this case is zero, which means E_s = E_h. If we assume that E_s = E_h is not equal to zero, we violate Kirchhoff’s voltage law because E_sd₁ > E_hd₂. This is not possible as the potential difference between the AlGaN surface and AlGaN/GaN heterojunction has to be equal to zero because there is no electric field above the AlGaN surface and no electric field below the AlGaN/GaN heterojunction. Case B shows a dashed rectangle around a piezoelectric dipole with no mobile charges attracted towards it. This case satisfies both the Gauss’s law and Kirchhoff’s voltage law and shows that piezoelectric polarization near the heterojunction does not impact N_2DEG. Thus, as presented in (2), spontaneous polarization is the only reason for N_2DEG at the AlGaN/GaN heterojunction when the surface is exposed to ambient ions.

2.2. Metal Gate Deposited on AlGaN Surface

The electron density at the AlGaN/GaN heterojunction with a metal gate deposited on the AlGaN surface, N_2DEG−M can also be stated in terms of spontaneous polarization in AlGaN and GaN, with the difference that this parameter also depends on the metal–semiconductor work function difference,$\mathrm{q}{\mathsf{\varphi }}_{\mathrm{ms}}$, between the metal and the GaN acting as the semiconductor. This structure is analogous to metal–oxide–semiconductor (MOS) structures, the only difference being that the dielectric role of the oxide between the metal and the semiconductor is played by the AlGaN layer. Adopting the dependence of electron density on the work–function difference from MOS theory [14,15], we obtain the following equation for N_2DEG−M:

$${\mathrm{N}}_{2\mathrm{DEG}-\mathrm{M}}={\mathrm{N}}_{\mathrm{p}-\mathrm{AlGaN}}-{\mathrm{N}}_{\mathrm{p}-\mathrm{GaN}}+{\mathrm{C}}_{\mathrm{AlGaN}}{\mathsf{\varphi }}_{\mathrm{ms}}$$

(3)

In (3), C_AlGaN is the AlGaN capacitance per unit area.

2.3. Thick Passivating Dielectric on AlGaN Surface

In the case of thick passivating dielectric, there are ions at the AlGaN surface, but they are fixed. This means that the electron density in the passivated case, N_2DEG-P, can be given in terms of the charge density at the passivation interface, N_pass, and the spontaneous polarizations in AlGaN and GaN layers by the following equation:

$${\mathrm{N}}_{2\mathrm{DEG}-\mathrm{P}}={\mathrm{N}}_{\mathrm{p}-\mathrm{AlGaN}}-{\mathrm{N}}_{\mathrm{p}-\mathrm{GaN}}+{\mathrm{N}}_{\mathrm{pass}}$$

(4)

3. Comparison with Other Models

For the first scenario elucidated in Section 2.1, our model using Gauss’s law indicates that 2DEG electron density is proportional to the difference between spontaneous polarization of AlGaN and GaN, whereas it is independent of the piezoelectric polarization. The simplified model by Ibbetson et al. [10] does not consider these factors. The other well-known model is developed by Ambacher et al. [12]. Their model states the dependence of 2DEG on both polarization and piezoelectric charges. Later on, several groups came up with more accurate models [9,16,17,18].

For the second scenario, as explained in Section 2.2, our model using MOS theory shows that 2DEG electron density is dependent on the difference between spontaneous polarization of AlGaN and GaN, as well as the work–function difference due to the metal-semiconductor junction. We have not seen other published model for the same scenario e.g. Morkoc [11] that employ the same approach.

For the third scenario, as documented in Section 2.3, our model indicates that 2DEG electron density is dependent on the difference between spontaneous polarization of AlGaN and GaN, as well as the charge density due to fixed ions at the insulator/AlGaN interface. There are several groups that have investigated this scenario, for example Shealy et al. [19] and Maeda et al. [20]. Both groups conducted experiments with variety of passivation dielectrics before deriving their equations. Despite differing complexities, all models agree that charge density of the passivation interface majorly influences the electron density of 2DEG.

4. Conclusions

We show that N_2DEG depends on the difference between spontaneous polarizations of AlGaN and GaN, but surprisingly, it is independent of the piezoelectric polarization in all realistic scenarios: AlGaN surface exposed to free air with mobile ions, metal gate deposited on the AlGaN surface, and the AlGaN surface passivated by a thick dielectric layer. When a metal gate is deposited on the AlGaN surface, the work–function difference $\left(q{\mathsf{\varphi }}_{ms}\right)$ impacts N_2DEG in analogous way to the well-established dependence of the semiconductor-charge density on the work–function difference in MOS capacitors. Finally, the fixed charge at the interface between AlGaN and a thick passivating layer, which is usually positive, attracts equivalent density of electrons to the 2DEG. The resulting equations from this new analysis provide the ability to distinguish between these differences and realistic scenarios, which is a specific addition to the existing body of literature.