Finite Element Analysis of Stress Distribution in Monolithic High-Translucency Zirconia Dental Prostheses
Abstract
1. Introduction
2. Materials and Methods
2.1. Processing
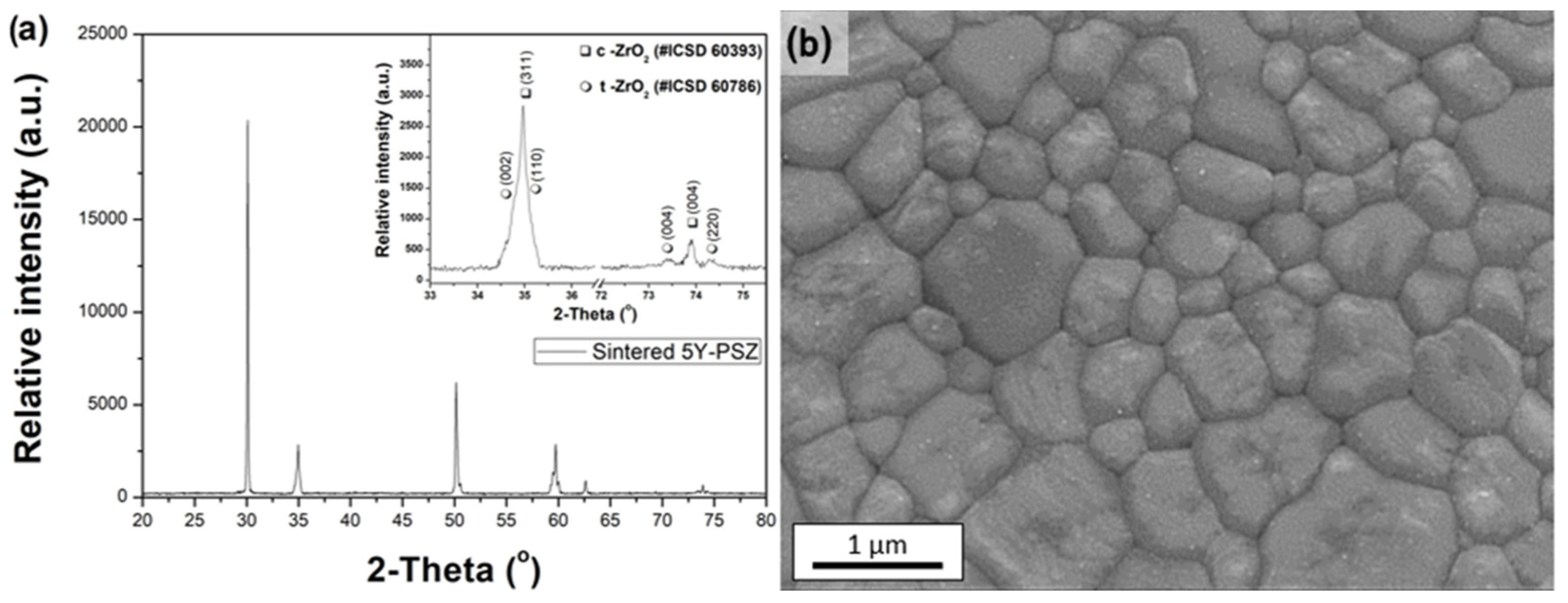
2.2. Characterizations
2.3. Mechanical Properties
2.4. Finite Element Modeling
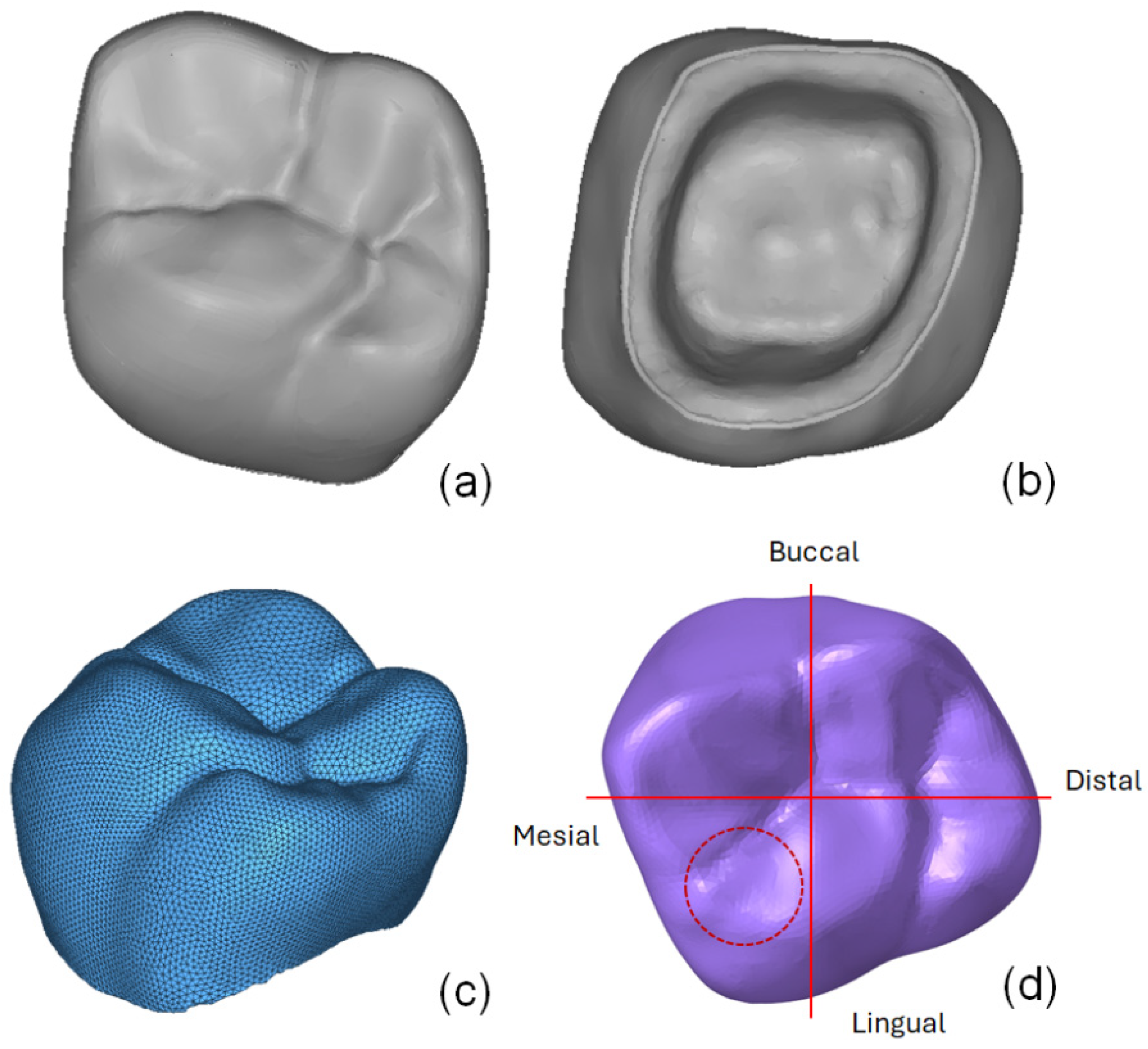
2.4.1. Geometrical Model and Meshing Generation
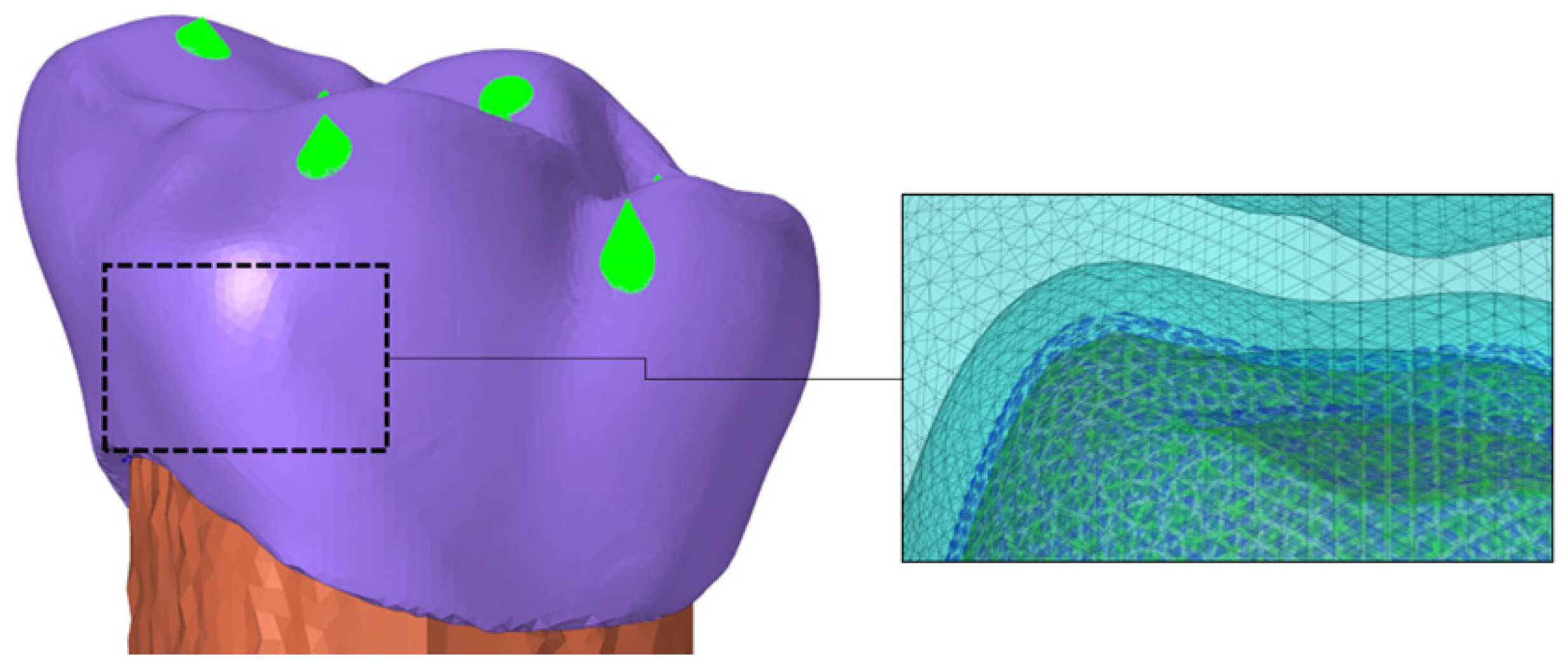
2.4.2. Model Assembly
2.4.3. Mohr–Coulomb Criterion
2.4.4. Numerical Simulations
3. Results and Discussion
3.1. Observations
3.2. Numerical Predictions
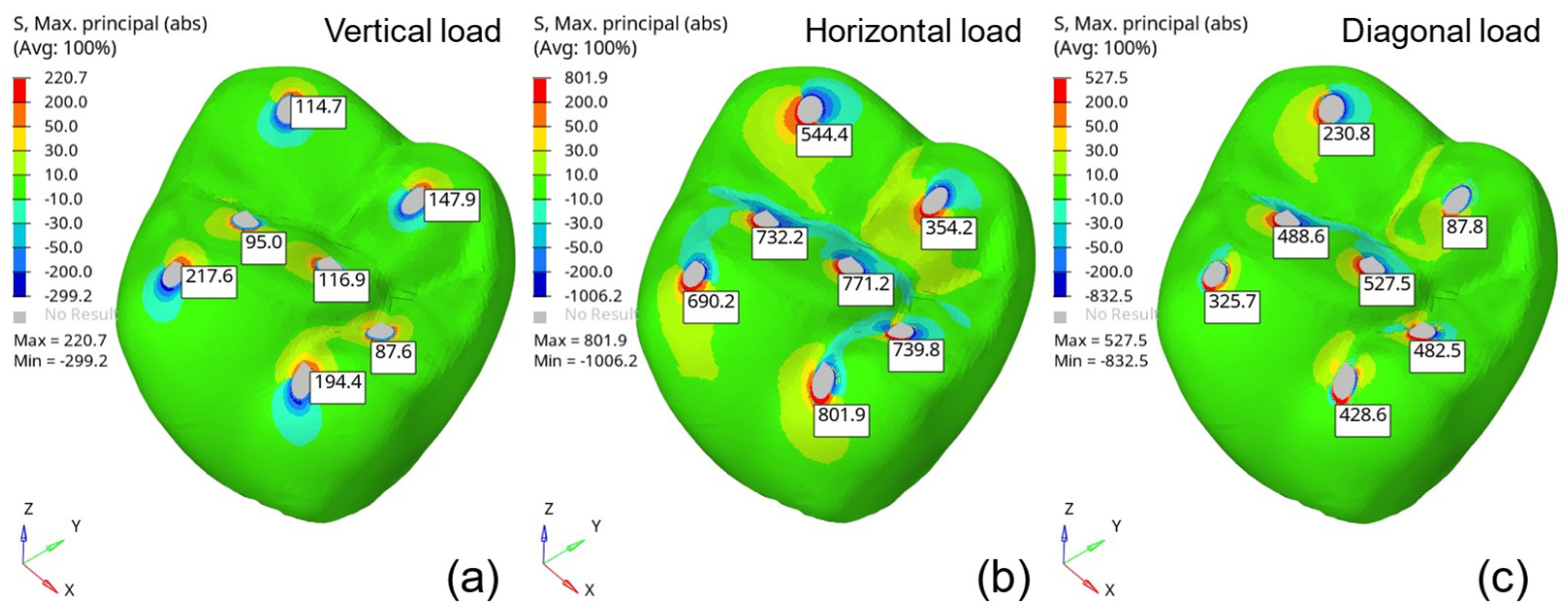
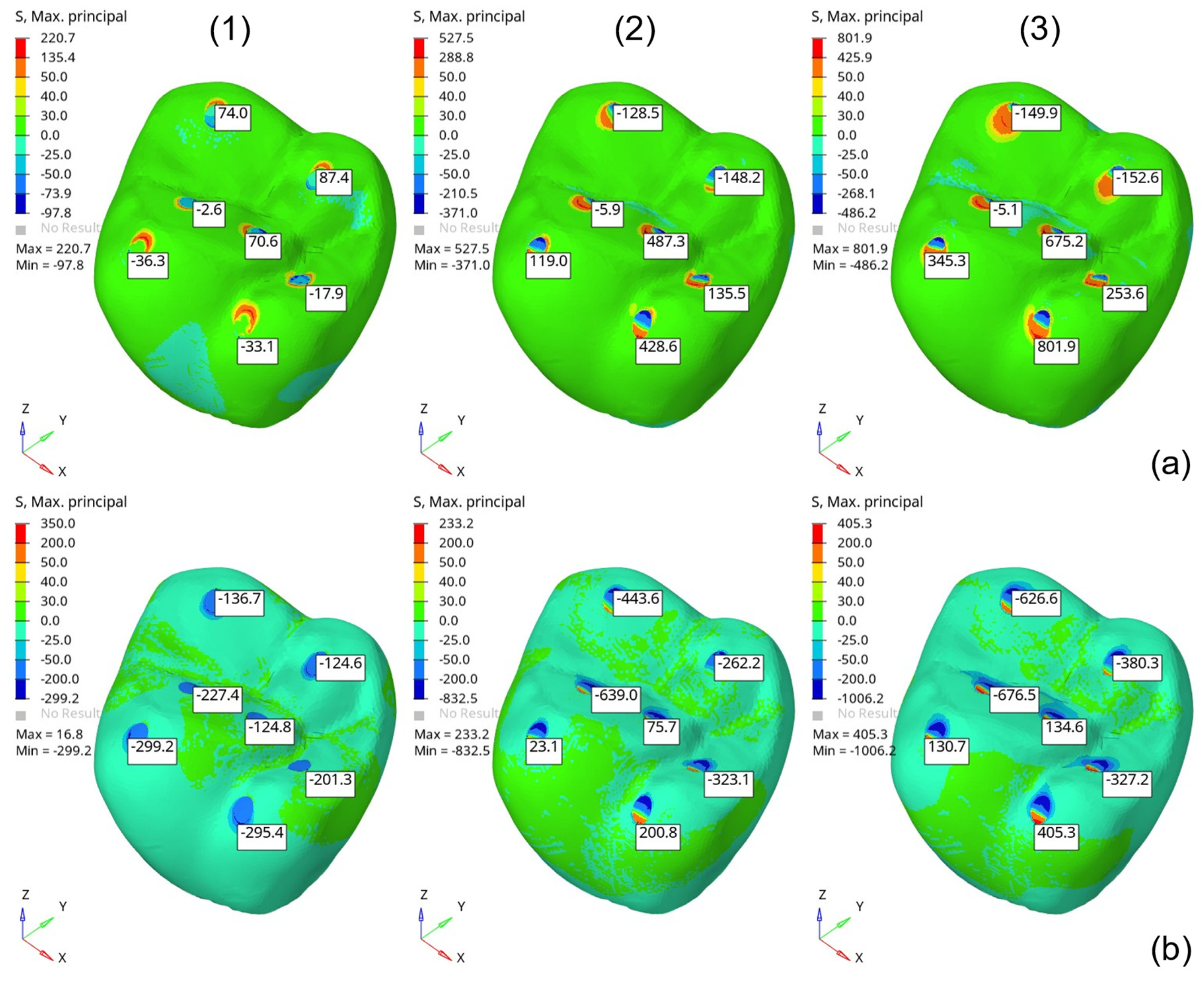
3.2.1. Absolute Maximum Principal Stress
3.2.2. Fixed-Base Prosthesis Assembly
3.2.3. Stress Components
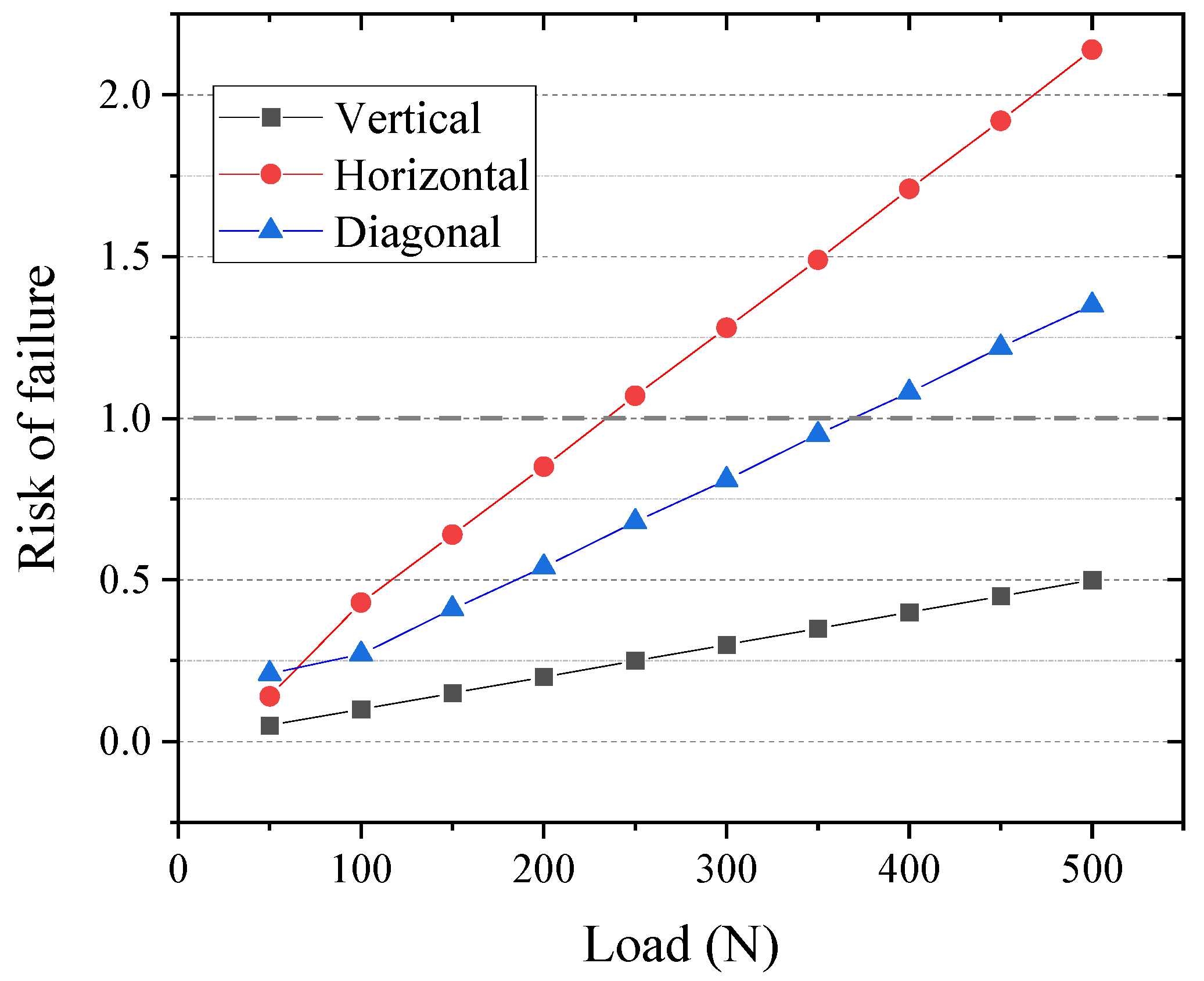
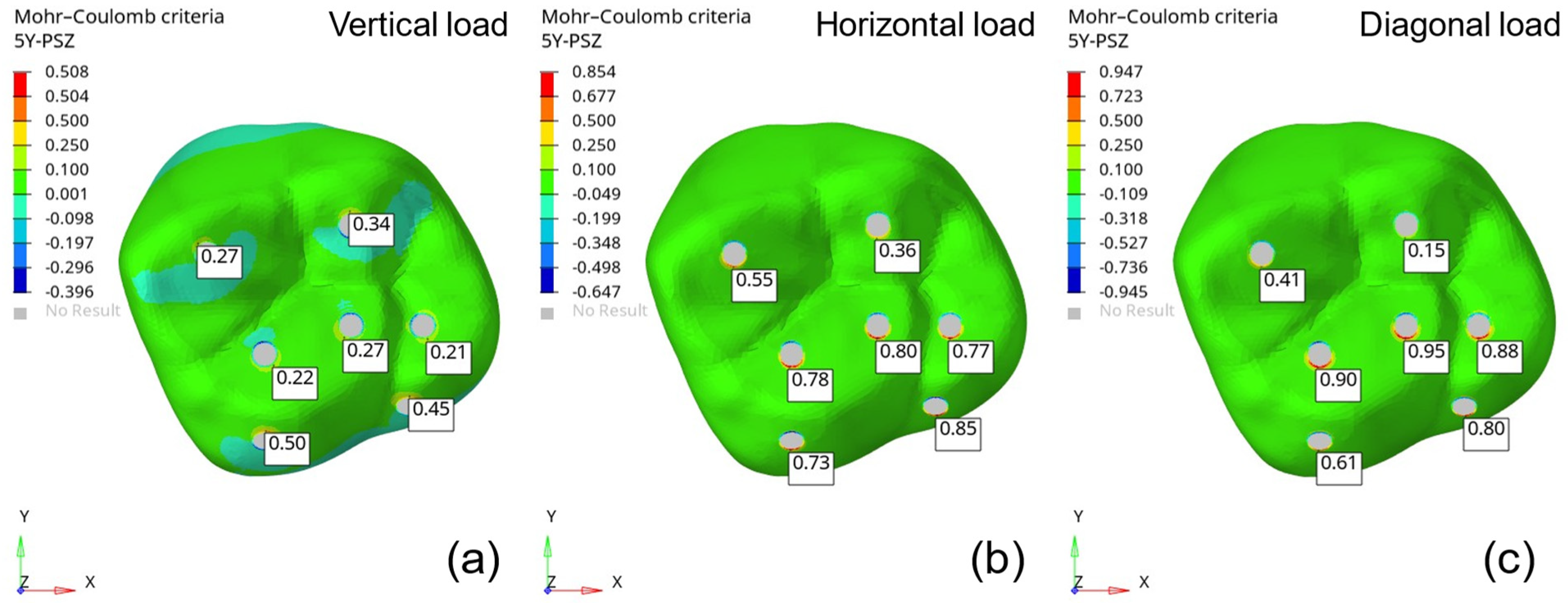
3.2.4. Risk of Failure
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, R.W.K.; Chow, T.W.; Matinlinna, J.P. Review: Ceramic dental biomaterials and CAD/CAM technology: State of the art. J. Prosthod. Res. 2014, 58, 208–216. [Google Scholar] [CrossRef] [PubMed]
- van Noort, R. The future of dental devices is digital. Dent. Mater. 2021, 28, 3–12. [Google Scholar] [CrossRef] [PubMed]
- Zandparsa, R. Digital Imaging and Fabrication. Dent. Clin. N. Am. 2014, 58, 135–158. [Google Scholar] [CrossRef]
- Guazzato, M.; Albakry, M.; Ringer, S.P.; Swain, M.V. Strength, fracture toughness and microstructure of a selection of all-ceramic materials. Part II. Zirconia-based dental ceramics. Dent. Mater. 2004, 20, 449–456. [Google Scholar] [CrossRef] [PubMed]
- Denry, I.; Kelly, J.R. State of the art of zirconia for dental applications. Dent. Mater. 2008, 24, 299–307. [Google Scholar] [CrossRef]
- Camposilvan, E.; Leone, R.; Gremillard, L.; Sorrentino, R.; Zarone, F.; Ferrari, M.; Chevalier, J. Aging resistance, mechanical properties and translucency of different yttria-stabilized zirconia ceramics for monolithic dental crown applications. Dent. Mater. 2018, 34, 879–890. [Google Scholar] [CrossRef]
- Kelly, P.M.; Rose, L.F. The martensitic transformation in ceramics—Its role in transformation toughening. Prog. Mater. Sci. 2002, 47, 463–557. [Google Scholar] [CrossRef]
- Basu, B. Toughening of yttria-stabilised tetragonal zirconia ceramics. Int. Mater. Rev. 2005, 50, 239–256. [Google Scholar] [CrossRef]
- Kelly, J.R.; Denry, I. Stabilized zirconia as a structural ceramic: An overview. Dent. Mater. 2008, 24, 289–298. [Google Scholar] [CrossRef]
- Chevalier, J. What future for zirconia as a biomaterial? Biomaterials 2006, 27, 535–543. [Google Scholar] [CrossRef]
- Abreu, L.G.; Quintino, M.N.; Alves, M.F.; Habibe, C.H.; Ramos, A.S.; Santos, C. Impact of the microstructure on the life prediction of hydrothermal degraded 3Y-TZP bioceramics. J. Mater. Res. Technol. 2020, 9, 10830–10840. [Google Scholar] [CrossRef]
- Ramesh, S.; Lee, K.S.; Tan, C.Y. A review on the hydrothermal ageing behaviour of Y-TZP ceramics. Ceram. Int. 2018, 44, 20620–20634. [Google Scholar] [CrossRef]
- Zhang, F.; Inokoshi, M.; Batuk, M.; Hadermann, J.; Naert, I.; van Meerbeek, B.; Vleugels, J. Strength toughness and aging stability of highly-translucent Y-TZP ceramics for dental restorations. Dent. Mater. 2016, 32, 327–337. [Google Scholar] [CrossRef] [PubMed]
- Alves, M.F.R.P.; Ribeiro, S.; Suzuki, P.A.; Strecker, K.; Santos, C. Effect of Fe2O3 addition and sintering temperature on mechanical properties and translucence of zirconia dental ceramics with different Y2O3 content. Mater. Res. 2021, 24, e20200402. [Google Scholar] [CrossRef]
- Santos, C.; Rosa, G.O.; Quintino, M.N.; Alves, M.F.R.P.; Ribeiro, S.; Melo-Silva, C.L. Effect of surface finishing and thickness on the translucency of zirconia dental ceramics. Ceram. Int. 2020, 46, 7748–7755. [Google Scholar] [CrossRef]
- ISO 6872-15; Dentistry—Dental Materials. International Standard Organization: Geneva, Switzerland, 2015.
- Dittmer, M.P.; Kohorst, P.; Borchers, L.; Stiesch, M. Influence of the supporting structure on stress distribution in all-ceramic FPDs. Int. J. Prosthodont. 2010, 23, 63–68. [Google Scholar]
- Guess, P.C.; Zavanelli, R.A.; Silva, N.R.; Bonfante, E.A.; Coelho, P.G.; Thompson, V.P. Monolithic CAD/CAM lithium disilicate versus veneered Y-TZP crowns: Comparison of failure modes and reliability after fatigue. Int. J. Prosthodont. 2010, 23, 434–442. [Google Scholar] [PubMed]
- Lin, J.; Shinya, A.; GomiI, H.; Shinya, A. Finite element analysis to compare stress distribution of connector of lithia-disilicate reinforced glass ceramic and zirconia based fixed partial denture. Odontology 2012, 100, 96–99. [Google Scholar] [CrossRef]
- ASTM E1876-15; Standard Test Method for Dynamic Young’s Modulus, Shear Modulus, and Poisson’s Ratio by Impulse Excitation of Vibration. ASTM International: West Conshohocken, PA, USA, 2015; 17p.
- ASTM C1327-15; Standard Test Method for Vickers Indentation Hardness of Advanced Ceramics. ASTM International: West Conshohocken, PA, USA, 2015; 10p.
- Niihara, K.A. Fracture mechanics analysis of indentation induced Palmqvist crack in ceramics. J. Mater. Sci. Lett. 1983, 2, 221–223. [Google Scholar] [CrossRef]
- Madeira, M.C.; Rpelf, J.; Rizzolo, C. Capítulo 2, Anatomia individual dos dentes. In Anatomia do Dente; Sarvier: Mount Morris, IL, USA, 2014. [Google Scholar]
- Ha, S.-R.; Kim, S.-H.; Han, J.-S.; Yoo, S.-H.; Jeong, S.-C.; Lee, J.-B.; Yeo, I.-S. The influence of various core designs on stress distribution in the veneered zirconia crown: A finite element analysis study. J. Adv. Prosthodont. 2013, 5, 187–197. [Google Scholar] [CrossRef]
- Labuz, J.F.; Zang, A. Mohr–Coulomb Failure Criterion. Rock Mech. Rock Eng. 2012, 45, 975–979. [Google Scholar] [CrossRef]
- Zhang, Y. Making yttria-stabilized tetragonal zirconia translucent. Dent. Mater. 2014, 30, 1195–1203. [Google Scholar] [CrossRef]
- Santos, C.; Baltazar, J.; Alves, M.F.R.P.; Olhero, S.M. Development of translucent zirconia by robocasting. Mater. Lett. 2022, 325, 132785. [Google Scholar] [CrossRef]
- Santos, C.; Baltazar, J.; Alves, M.F.R.P.; Olhero, S.M. Translucent zirconia dental prosthesis processed by Direct Ink Writing: Updates and challenges. J. Manuf. Process 2024, 131, 1656–1666. [Google Scholar] [CrossRef]
- Amarante, J.E.V.; Pereira, M.V.S.; Souza, G.M.; Alves, M.F.R.P.; Simba, B.G.; Santos, C. Effect of hydrothermal aging on the properties of zirconia with different levels of translucency. J. Mech. Behav. Biomed. Mater. 2020, 100, 103847. [Google Scholar] [CrossRef] [PubMed]
- de Araújo-Júnior, E.N.; Bergamo, E.T.; Bastos, T.M.; Jalkh, E.B.B.; Lopes, A.C.; Monteiro, K.N.; Cesar, P.F.; Tognolo, F.C.; Migliati, R.; Tanaka, R.; et al. Ultra-translucent zirconia processing and aging effect on microstructural, optical, and mechanical properties. Dent. Mater. 2022, 38, 587–600. [Google Scholar] [CrossRef]
- Mavriqi, L.; Traini, T. Mechanical Properties of Translucent Zirconia: An In Vitro Study. Prosthesis 2023, 5, 48–59. [Google Scholar] [CrossRef]
- Shelar, P.; Abdolvand, H.; Butler, S. On the behaviour of zirconia-based dental materials: A review. J. Mech. Behav. Biomed. Mater. 2021, 124, 104861. [Google Scholar] [CrossRef]
- Waltimo, A.; Kononen, M. A novel bite force recorder and maximal isometric bite force values for healthy young adults. Scand. J. Dent. Res. 1993, 101, 171–175. [Google Scholar] [CrossRef]
- Laurell, L.; Lundgren, D. Periodontal ligament areas and occlusal forces in dentitions restored with cross-arch bilateral end abutment bridges. J. Clin. Periodontol. 1985, 12, 850–860. [Google Scholar] [CrossRef]
- Gibbs, C.H.; Mahan, P.E.; Lundeen, H.C.; Brehnan, K.; Walsh, E.K.; Holbrook, W.B. Occlusal forces during chewing and swallowing as measured by sound transmission. J. Prosthet. Dent. 1981, 46, 443–449. [Google Scholar] [CrossRef] [PubMed]
- Ohlmann, B.; Marienburg, K.; Gabbert, O.; Hassel, A.; Gilde, H.; Rammelsberg, P. Fracture-load values of all-ceramic cantilevered FPDs with different framework designs. Int. J. Prosthodont. 2009, 22, 49–52. [Google Scholar] [PubMed]
- Ulusoy, M.; Toksavul, S. Fracture resistance of five different metal framework designs for metal-ceramic restorations. Int. J. Prosthodont. 2002, 15, 571–574. [Google Scholar] [PubMed]
- Morikawa, A. Investigation of occlusal force on lower first molar in function. Kokubyo Gakkai Zasshi 1994, 61, 250–274. [Google Scholar] [CrossRef]
- Pileicikene, G.; Surna, A. The human masticatory system from a biomechanical perspective: A review. Stomatol. Balt. Dent. Maxillofac. J. 2004, 6, 81–84. [Google Scholar]



| Lattice Parameters | 5Y-PSZ |
|---|---|
| ZrO2 –Tetragonal (ZrO2-t), space group P42/nmc | a = 3.610(8) Å/c = 5.162(8) Å/V = 67.3104 A3 |
| Tetragonality (c/a√2) | 1.011(0) |
| Y2O3 in ZrO2-t phase (mol.%) | 4.57 |
| ZrO2 –Cubic (ZrO2-c), space group-Fm-3 m | a = 5.134(1) Å/V = 135.33 A3 |
| Y2O3 in ZrO2-c phase (mol.%) | 5.33 |
| Phase composition | 42.3% ZrO2-t/57.7% ZrO2-c |
| Young’s Modulus (GPa) | Vickers Hardness (HV) | Fracture Toughness (MPa.m1/2) | Poisson’s Ratio | Flexural Strength (MPa) | Characteristic Strength (MPa) | Weibull Modulus |
|---|---|---|---|---|---|---|
| 192.2 ± 4.8 | 1315 ± 48 | 3.7 ± 0.2 | 0.31 ± 0.05 | 433.7 ± 67 | 463.5 | 6.7 |
| Loading Condition | Region | ||||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | |
| Vertical | 217.6 | 194.4 | 95.0 | 116.9 | 87.6 | 114.7 | 147.9 |
| Horizontal | 690.2 | 801.9 | 732.2 | 771.2 | 739.8 | 544.4 | 354.2 |
| Angular (45°) | 325.7 | 428.6 | 488.6 | 527.5 | 482.5 | 230.8 | 87.8 |
| Region | σ11 | σ22 | σ33 | σ12 | σ23 | σ31 | σ1 | σ2 | σ3 | σmises |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | −95.9 | −51.3 | −267.2 | −27.0 | −49.0 | −61.4 | −36.3 | −78.9 | −299.2 | 244.4 |
| 2 | −89.1 | −41.0 | −289.6 | −10.3 | −38.4 | 4.1 | −33.1 | −91.2 | −295.4 | 238.7 |
| 3 | −71.9 | −110.3 | −120.4 | 3.2 | 109.3 | −25.1 | −2.6 | −72.6 | −227.4 | 199.3 |
| 4 | −1.8 | 17.3 | −115.2 | 52.5 | −31.3 | −28.3 | 70.6 | −45.6 | −124.8 | 170.3 |
| 5 | −34.8 | −88.3 | −183.7 | −13.9 | 11.7 | 50.5 | −17.9 | −87.6 | −201.3 | 160.4 |
| 6 | −10.1 | 20.5 | −122.3 | −52.2 | 42.4 | −32.2 | 74.0 | −49.2 | −136.7 | 183.3 |
| 7 | −58.6 | 58.5 | −85.4 | −19.3 | 59.6 | −41.9 | 87.4 | −48.3 | −124.6 | 186.0 |
| Region | σ11 | σ22 | σ33 | σ12 | σ23 | σ31 | σ1 | σ2 | σ3 | σmises |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 156.9 | 196.1 | 320.1 | 9.6 | 1.5 | 68.7 | 345.3 | 197.1 | 130.7 | 190.3 |
| 2 | 414.3 | 489.2 | 759.1 | 8.7 | −104.1 | −53.2 | 801.9 | 455.3 | 405.3 | 374.1 |
| 3 | −197.9 | −311.6 | −383.5 | −9.9 | 321.2 | −79.2 | −5.1 | −211.3 | −676.5 | 595.7 |
| 4 | 140.7 | 651.2 | 415.4 | 46.0 | 73.6 | −16.7 | 675.2 | 397.5 | 134.6 | 468.3 |
| 5 | −16.1 | −41.8 | −16.0 | −261.6 | 125.3 | 20.4 | 253.6 | −0.2 | −327.2 | 504.3 |
| 6 | −172.7 | −611.6 | −305.8 | 70.2 | 42.0 | 36.6 | −149.9 | −313.7 | −626.6 | 419.5 |
| 7 | −186.1 | −356.6 | −178.6 | 67.9 | 4.2 | 15.4 | −152.6 | −188.4 | −380.3 | 212.1 |
| Region | σ11 | σ22 | σ33 | σ12 | σ23 | σ31 | σ1 | σ2 | σ3 | σmises |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 43.1 | 102.4 | 37.4 | −12.3 | −33.6 | 5.1 | 119.0 | 40.8 | 23.1 | 88.4 |
| 2 | 229.9 | 317.0 | 331.9 | −1.1 | −100.8 | −34.8 | 428.6 | 249.4 | 200.8 | 207.8 |
| 3 | −190.8 | −298.3 | −356.3 | −4.7 | 304.4 | −73.8 | −5.9 | −200.4 | −639.0 | 561.7 |
| 4 | 98.2 | 472.7 | 212.3 | 69.6 | 29.9 | −31.8 | 487.3 | 220.1 | 75.7 | 361.7 |
| 5 | −36.0 | −92.0 | −141.2 | −194.8 | 96.9 | 50.1 | 135.5 | −81.6 | −323.1 | 397.3 |
| 6 | −129.3 | −417.9 | −302.7 | 12.7 | 59.6 | 3.2 | −128.5 | −277.8 | −443.6 | 273.0 |
| 7 | −173.1 | −210.8 | −186.7 | 34.4 | 45.1 | −18.7 | −148.2 | −160.1 | −262.2 | 108.6 |
| Vertical Load Maximum Bite (500 N) | Horizontal Load Chewing (200 N) | Angular Load Chewing (350 N) | |||
|---|---|---|---|---|---|
| Critical Stress (MPa) | Risk of Failure | Critical Stress (MPa) | Risk of Failure | Critical Stress (MPa) | Risk of Failure |
| 299.2 | 0.55 | 320.8 | 0.85 | 447.3 | 0.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ward, F.A.d.C.; Moreira, L.P.; Ward, P.A.d.C.; Silva, P.C.d.; Alves, M.F.R.P.; Santos, C. Finite Element Analysis of Stress Distribution in Monolithic High-Translucency Zirconia Dental Prostheses. Oral 2025, 5, 15. https://doi.org/10.3390/oral5010015
Ward FAdC, Moreira LP, Ward PAdC, Silva PCd, Alves MFRP, Santos C. Finite Element Analysis of Stress Distribution in Monolithic High-Translucency Zirconia Dental Prostheses. Oral. 2025; 5(1):15. https://doi.org/10.3390/oral5010015
Chicago/Turabian StyleWard, Fernando Araújo da Costa, Luciano Pessanha Moreira, Pedro Araújo da Costa Ward, Paula Cipriano da Silva, Manuel Fellipe Rodrigues Pais Alves, and Claudinei Santos. 2025. "Finite Element Analysis of Stress Distribution in Monolithic High-Translucency Zirconia Dental Prostheses" Oral 5, no. 1: 15. https://doi.org/10.3390/oral5010015
APA StyleWard, F. A. d. C., Moreira, L. P., Ward, P. A. d. C., Silva, P. C. d., Alves, M. F. R. P., & Santos, C. (2025). Finite Element Analysis of Stress Distribution in Monolithic High-Translucency Zirconia Dental Prostheses. Oral, 5(1), 15. https://doi.org/10.3390/oral5010015






