Revisiting the Management of Water–Energy Systems under the Umbrella of Resilience Optimization ^†

Abstract

The optimal management of sociotechnical systems across the water–energy nexus is a critical issue for the overall goal of sustainable development. However, the new challenges induced by global crises and sudden changes require a paradigm shift in order to ensure tolerance against such kinds of disturbance that are beyond their “normal” operational standards. This may be achieved by incorporating the concept of resilience within the procedure for extracting optimal management policies and assessing their performance by means of well-designed stress tests. The proposed approach is investigated by using as proof of concept the complex and highly extended water resource system of Athens, Greece.

1. Introduction

The long-term management of water–energy systems is subject to multiple and conflicting objectives, aiming at balancing competitive uses, socioeconomic constraints, and environmental requirements. This challenging issue is typically formalized in a multicriteria context, where the optimal management of critical control components (reservoirs, hydropower stations, pumps, etc.) is dictated by assuming steady-state conditions. For instance, we consider a specific scenario of water demands and run the underlying optimization problem to extract the associated set of operational rules that ensures an acceptable tradeoff between the performance metrics of interest (reliability, cost/benefit, etc.).

However, in our era of global crises (environmental, financial, health) and violent changes, where all sociotechnical systems are pushed beyond “normality”, the conventional (i.e., steady-state) paradigm of water–energy management seems to be rather insufficient. In this vein, we introduce an alternative approach to handle the long-term management issue under the prism of resilience optimization. Although the concept of resilience is not new, there has recently been a global interest in embedding this concept within the design and management of complex engineering systems. Herein, after presenting a brief review of the definition and use of resilience in practice, we investigate its implementation in the water–energy nexus by means of a stress-testing protocol.

The proposed framework is demonstrated in the raw water resource system of Athens, Greece. The long-term management of this system is determined by means of parametric operational rules of its storage components, while the need for ensuring a low-cost operational policy (which is associated with energy consumption due to pumping) conflicts with the key objective of reliability. As a proof of concept, we stress the system under various pressures that reflect the population growth, the degradation of infrastructures, and the energy crisis by applying two alternative operational rules, i.e., the optimal rule for the baseline scenario and an alternative rule. The second rule, although sub-optimal for the first scenario, is eventually preferred in terms of resilience.

2. The Concept of Resilience across the Water–Energy Nexus

As the water–energy nexus is becoming even more important for the overall goal of sustainable development [1], the concept of resilience across these systems is expected to be the key quest for their operation.

Resilience has been deeply investigated across different research fields (e.g., economy, energy, water, agriculture), where the different disciplines involved address this issue from their own perspectives. Overall, resilience is the degree to which a system continues to perform with tolerant reliability under progressively increasing disturbance [2]. On the other hand, Grafton et al. [3] pose resilience management as the planning, adaptation, and transformation actions intended to influence the resistance, recovery, and robustness (the so-called three Rs) of the socioecological system under consideration. In the literature, these are defined as follows: (a) resistance is a system’s ability to actively change, while retaining its identity, or to passively maintain its performance following one or more adverse events; (b) recovery is a time measure, where a higher value indicates a shorter recovery time; and (c) robustness is the level of pressure that the system can take without failing [4]. Finally, Pizzol [5] highlights that resilience depends on the system’s elements and the way these elements are connected. Specifically, a specific architecture and design of a system, which may include less efficient components, can better manage stresses.

The concept of resilience provides the essential background for the assessment and evaluation of an a priori determined design of engineering systems under emerging threats [6]. These may include health and economic crises, population growth, and sudden large-scale changes (also referred to as “black swan” events), as well as cyber-physical attacks [7], which are a new type of threat. In the context of water systems that are highly affected by such events, Butler et al. [8] provide a “roadmap” to sustainability, consisting of a set of basic definitions and concepts of reliability and resilience and, eventually, an associated evaluation framework.

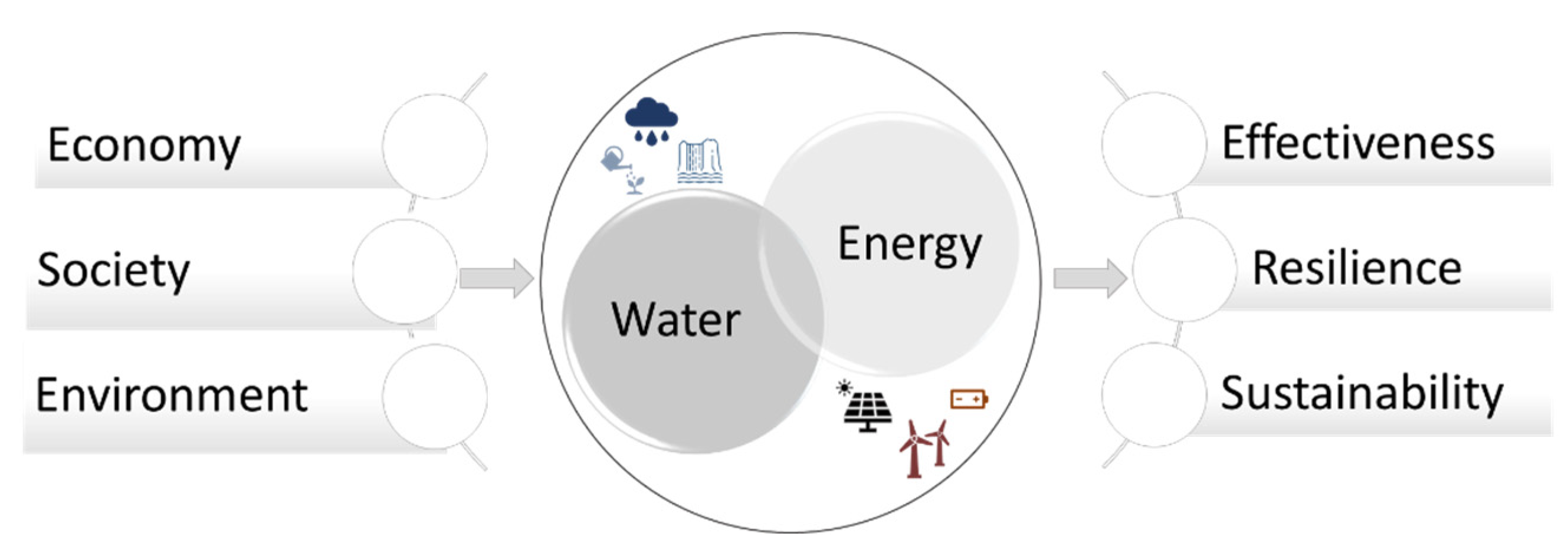
However, in the water–energy nexus, this road is even more challenging since the complementarities and dependencies of the two components tread a fine line. This nexus, as a complex engineering system, should be simultaneously effective, sustainable, and resilient (Figure 1). The first two targets depend both on the structure and the operation of the system, which are outcomes of their design and management, respectively. In particular, the tradeoffs and synergies of the water and energy elements across a well-defined nexus can enrich policy design frameworks, with perspectives from beside and beyond the resilience rationale [9].

In the context of management, this is usually expressed by means of operational rules, which can be conventionally derived from an optimization procedure that regards the successful interplay of the water and energy components under a specific set of assumptions. The two elements are highly interconnected and conflicting since water is the critical ingredient of energy production. On the other hand, energy is needed for the complete water cycle, from water abstraction (through pumping) to water treatment, as well as for recycled water collection and treatment. Following this, we agree that this optimization context is in fact a multicriteria problem, thus leading to multiple rules that are equivalent, from the Pareto optimality perspective [10]. In this vein, the incorporation of resilience as an overall performance metric may be the turning point for supporting decision-making. In particular, this allows for mining the management rules that remain robust across increasing pressures of the system, and finally detecting the best-compromise one.

3. The Water Resource System of Athens and Its Management

3.1. Brief Description of the Hydrosystem

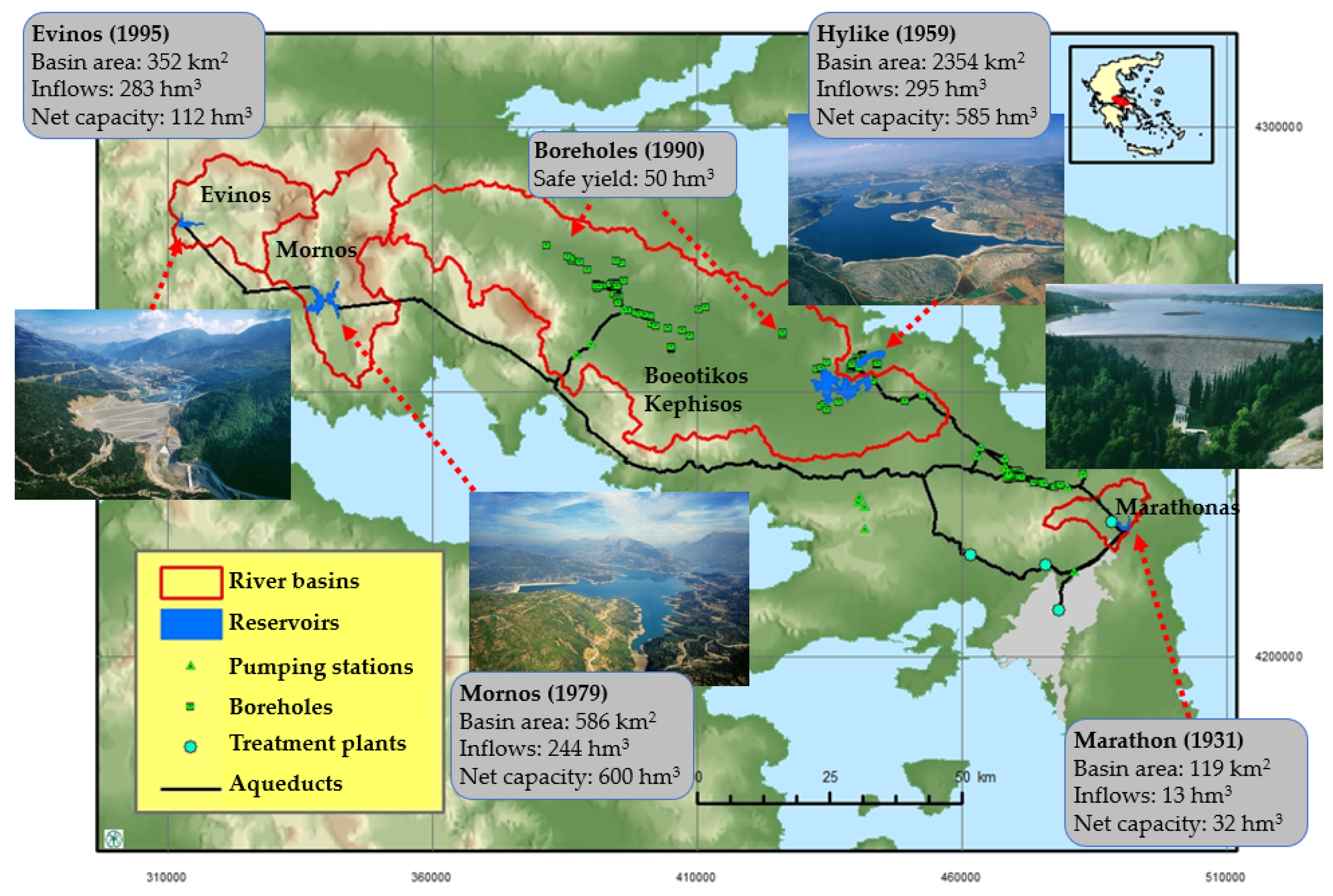
The raw water supply system of Athens is a highly complex and challenging water–energy system that extends over an area of around 4000 km². It comprises four reservoirs, 350 km of aqueducts, 15 pumping stations, several dozens of boreholes (mainly used as emergent resources), and four water treatment plants. The layout of the system and its key hydrological (river basin areas and associated inflows) and technical properties (first year of operation and net storage capacity of reservoirs) are shown in Figure 2. We remark that Hylike is a natural lake, lying in a karstic background, a key peculiarity of which is the significant losses due to leakages that may reach half of its storage capacity in one year.

The system’s main target is to provide raw water to the Athens metropolitan area; last decade, as a result of the broader financial crisis, the annual consumption did not exceed 400 hm³, while in the past it has exceeded 430 hm³. Several other uses are also fulfilled across the hydrosystem, for domestic and industrial water supply, irrigation, and environmental preservation downstream of Evinos and Marathon dams. These withdrawals sum up to 80–85 hm³. On the other hand, the mean annual inflow to the four reservoirs is 825 hm³, almost equally distributed to the three main reservoirs (Mornos, Evinos, Hylike).

The everyday operation of the hydrosystem relies upon several decisions, involving the allocation of abstractions from the different sources and their conveyance through the two main aqueduct branches. The southern branch (Mornos aqueduct) carries water via gravity from the interconnected reservoirs of Evinos and Mornos, while the northern one transfers water from Hylike and the boreholes through pumping, with considerable cost. In this context, the strategic management policy of the system is subject to two conflicting objectives, namely maximizing its reliability and minimizing its operational cost due to pumping. In practice, both objectives are strongly associated with the use of Hylike. For instance, when Hylike is out of operation, the system’s cost is negligible, but almost half of its storage is lost due to leakage. Thus, the reliability of the system highly depends on the inflows to the Evinos–Mornos complex, which may be too risky in the case of prolonged drought periods. We highlight that the desirable reliability for the water supply of Athens is set as high as 99% on an annual basis (indicating one failure per 100 years), while the minimum acceptable value is 95%.

3.2. Modeling Framework for Optimizing the System’s Management Policy

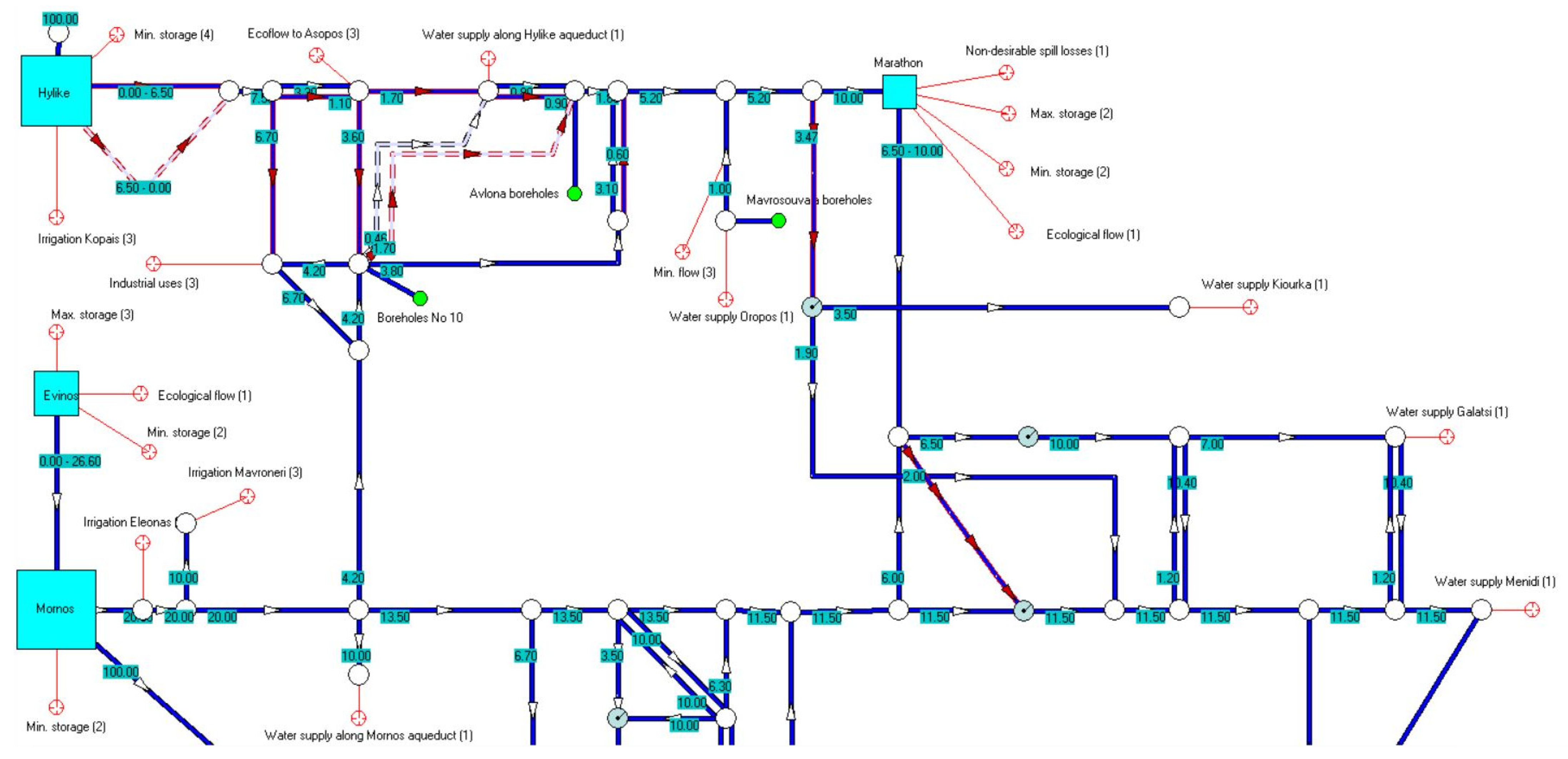
The exploration of the management options and, eventually, the detection of the best-compromise one, is performed through the use of Hydronomeas software, developed by the ITIA research team of the National Technical University of Athens. Hydronomeas is the cornerstone of a broader decision support system for the supervision and the management of the water resource system of Athens [11]. The representation of the physical system as a network model within its graphical interface is demonstrated in Figure 3.

The methodological framework of the model is based on the following triptych:

• Parameterization of the operational policy of the system;
• Stochastic simulation of the system’s dynamics;
• Optimization of the long-term performance of the system.

More specifically, the mathematical expression of the operation rules is an extension of the rationale by Nalbantis and Koutsoyiannis [12] and Koutsoyiannis and Economou [13]. These rules determine the desirable allocation of abstractions from the system’s sources (reservoirs and boreholes), according to its current state (storage, demand), by using only a few control variables. In addition, the simulation module comprises two components. The first aims at representing the system’s drivers (inflows, demands) as stochastic processes, by means of synthetically generated time series that reproduce the probabilistic and stochastic regime (auto- and cross-dependencies) of the parent historical data. The data synthesis is performed using the newly introduced anySim package [14]. For given inflows and demands, the simulation of the system’s operation is formalized as a stepwise allocation of the unknown water and energy fluxes, which are represented as control variables of a network linear programming problem. This aims at minimizing the total transportation cost across the hydrosystem by preserving the pre-specified hierarchy of water uses and constraints [15]. Finally, the overall optimization of the system’s performance is generally expressed as a multicriteria problem. Its components are probabilistic metrics, such as the failure probability (or its complementary metric, i.e., reliability), the mean annual energy production or consumption, and the water deficits and their costs.

4. Resilience-Based Optimization of the System’s Management

4.1. Baseline Scenario Setting

Based on the schematization of Figure 3, we seek the strategic management policy of the water resource system of Athens, for which we set a plethora of targets and operational constraints, classified into three priority levels. The targets that are set in the highest priority are the water supply of broader Athens. In particular, we consider a total annual demand of 400 hm³, i.e., close to the current consumption, which is split into five demand zones. Furthermore, we assume all minor water supply uses across the aqueduct network, which are merged as point demands at three nodes, and the two environmental flow demands downstream of Evinos and Marathon dams. In the second hierarchy level, we set the minimum and maximum storage constraints that are assigned to the four reservoirs as the major components of their operational rules. Finally, the lowest priority is assigned to the three irrigation targets. The system is driven by monthly synthetic rainfall, runoff, and evaporation time series of 2000 years in length, generated via the anySim model.

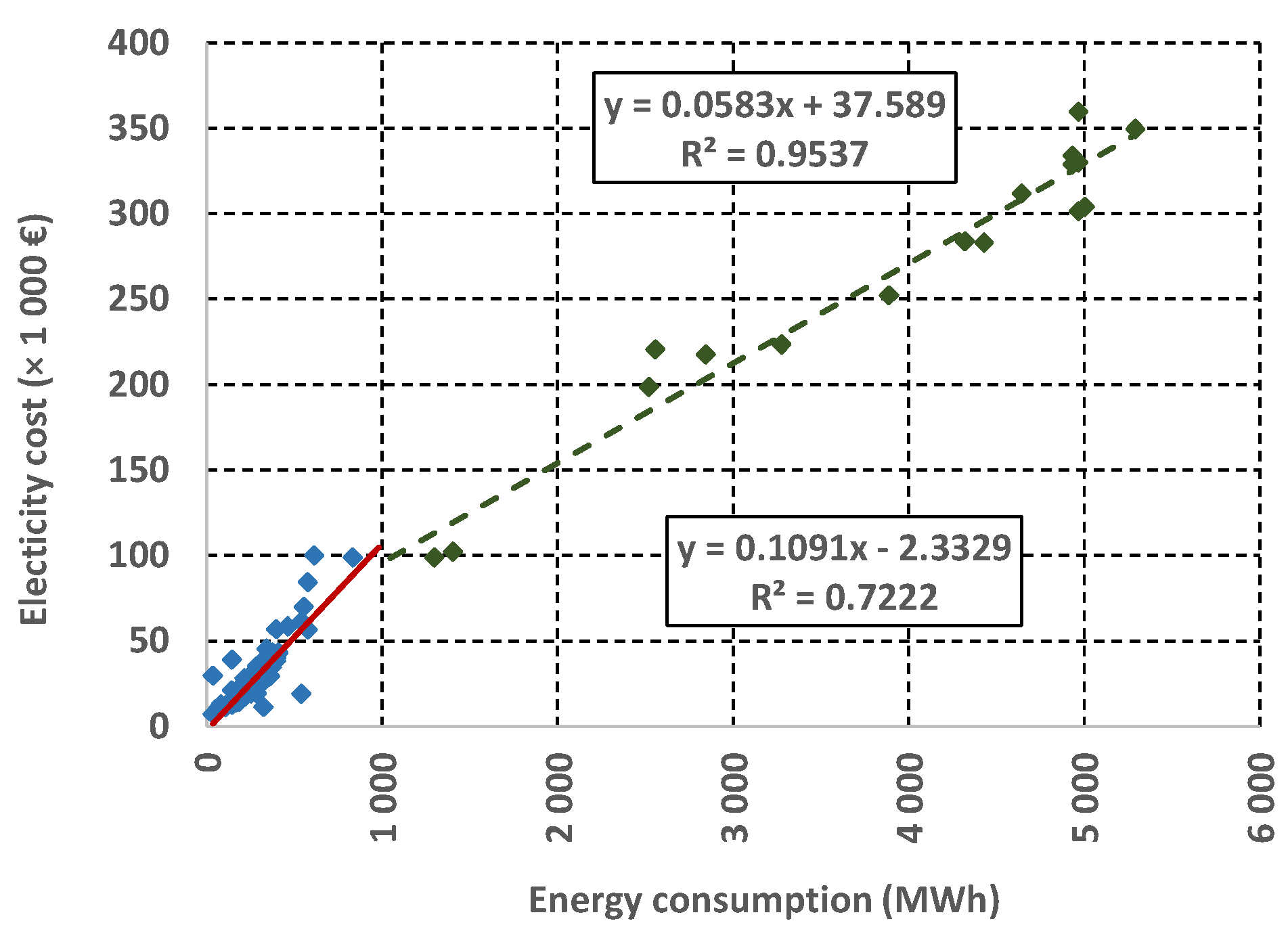
Initially, we consider the aforementioned system’s state as the baseline scenario, for which we extract the optimal operational rules of the four reservoirs. The optimization problem aims at balancing the two key objectives of the water–energy nexus, namely the fulfillment of water supply uses with very high reliability (preferably 99% on a mean annual basis) and the minimization of pumping cost. In this respect, the performance measure is formalized as a cost function, comprising two elements. The first expresses the mean annual deficit cost of all consumptive water uses, for which we apply different unit penalties, namely 1.0 EUR/m³ for water supply and 0.2 EUR/m³ for irrigation. The second element is the mean annual cost of electrical energy due to the use of pumps and boreholes. In order to estimate this cost, we apply piecewise linear functions that are fitted to historical energy consumption and associated cost data, as shown in the example of Figure 4.

4.2. Operation Rules

The optimized operational rules for the baseline scenario are illustrated in Figure 5a. These specify the desirable storage of each reservoir as a function of the expected total storage capacity of the system, which is estimated by accounting for the total storage at the end of the previous time step (month), the expected inflows, and the total water demand. The optimized control variables that are embedded in these rules are two dimensionless parameters for each reservoir, as explained by Koutsoyiannis and Economou [13], and the two operational constraints, by means of minimum and maximum desirable storage.

This rule is contrasted to a more conservative one (Figure 5b), which is adjusted in order to impose a more frequent use of Hylike. As shown in

Table 1. Key results for the baseline scenario when applying the two alternative management policies. All water, energy, and cost quantities are expressed on a mean annual basis.

	Baseline–Optimal	Resilient–Optimal
Reliability of Athens’s water supply (%)	99.0	99.7
Abstraction from Mornos (hm3)	442.92	442.03
Abstraction from Hylike (hm3)	25.22	29.74
Abstraction from boreholes (hm3)	10.21	7.26
Energy consumed in pumping stations (GWh)	24.18	30.04
Energy consumed in boreholes (GWh)	9.88	6.84
Total energy consumption (GWh)	34.06	36.88
Total energy cost (EUR 1 million)	2.73	2.90
Water supply deficit (hm3)	0.26	0.11
Irrigation deficit (hm3)	0.76	1.36

, from the sustainability perception, both rules are in a safe place, since they guarantee the desired reliability level of 99%. However, the second rule is sub-optimal in terms of economy. The question arising is whether this more conservative yet more expensive rule indicates a more resilient management policy. This question is investigated by means of stress scenarios in the next section.

4.3. Stress Scenarios

The water resource system of Athens is stressed against six scenarios that reflect different aspects of potential disturbance (socioeconomic, hydroclimatic, technical). A brief summary of them is given in

Table 2. Summary of stress scenarios.

ID	Description	Driver of Change
1	Baseline scenario (cf. Section 3.1)
2	Setting of irrigation targets at a higher priority level	Social
3	50% decrease in available groundwater resources	Hydroclimatic
4	20% increase in pumping cost	Economic
5	Increase in leakage losses across aqueducts from 5 to 10%	Technical
6	Increase in Athens’s demand to 430 hm3 (max. observed value)	Socioeconomic
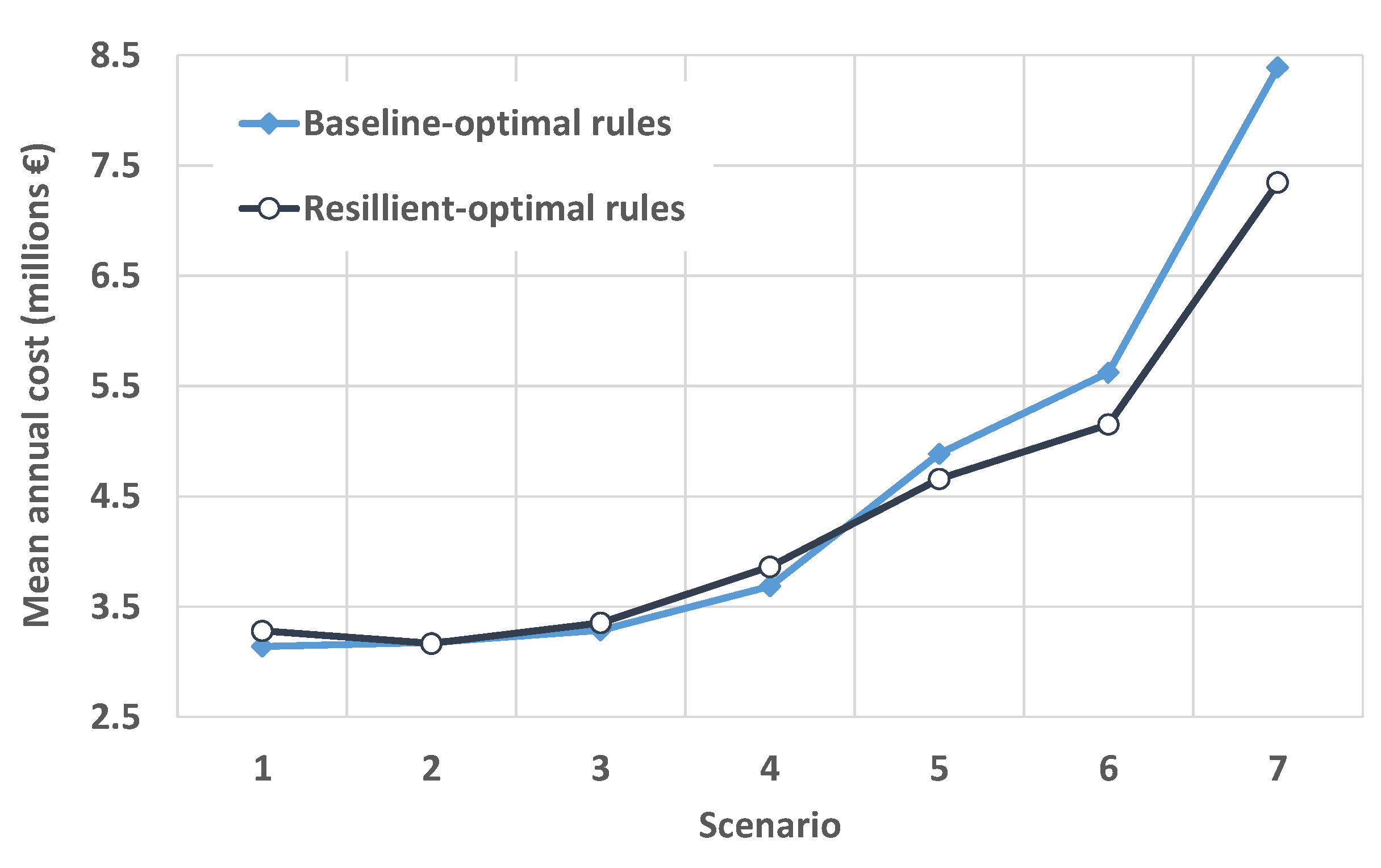
7	Increase in Athens’s demand to 450 hm3 (long-term projection)	Socioeconomic

, while in Figure 6 we contrast the performance of the two operational rules, in terms of mean annual cost. We remind the reader that this includes the energy cost and the cost of water deficits.

For the first three stress scenarios (numbered 2, 3, and 4), the optimal rule so far, according to the baseline state (scenario 1), is equivalent to or slightly outperforms the conservative rule. However, the other three scenarios highlight that the conventional definition of “optimality” does not promise resilience against situations where the system is pushed beyond its standards. Using the concept of resilience proposed by Makropoulos et al. [2], the area below the two curves represents an overall cost metric. Herein, the smaller this area is, the more resilient the operational rule is. Under this assumption, the second rule should be preferred as more robust. It is worth mentioning that the conventionally optimal rule for the last scenario ensures an unacceptably low level of reliability, i.e., 91.3%, while the mean annual energy cost is EUR 4.33 million. On the other hand, the resilient rule still achieves an acceptable reliability level (96.2%), with a relatively small increase in mean energy cost (EUR 4.77 million).

5. Conclusions

Triggered by the violent changes that span all aspects of sociotechnical systems, it is essential to reconsider the far-reaching quest for optimality under the concept of resilience. Taking as an example the challenging water–energy system of Athens, we revisit its long-term management policy, which has been conventionally handled as a typical optimization problem under steady-state conditions. By stressing this under a number of plausible disturbances, caused by social, economic, hydroclimatic, and technical changes, we manifest the necessity for adopting more conservative (in terms of reliability) although more expensive operation rules than the ones optimized against the baseline scenario.

Forthcoming research steps aim at enhancing the proposed protocol by designing a procedure for the automatic generation of stress scenarios, ideally formalized in a stochastic setting. In this procedure, the optimization will be carried out by setting as an objective function a resilience metric that accounts for the global system’s response against all stress scenarios.

Nevertheless, the incorporation of resilience within the configuration of management policies within the water–energy nexus is a crucial presupposition towards the road to sustainability.