Engineering Biomedical Problems to Detect Carcinomas: A Tomographic Impedance Approach
Abstract
1. Introduction
2. Related Works
3. Materials and Methods
3.1. EIT Mathematical Model
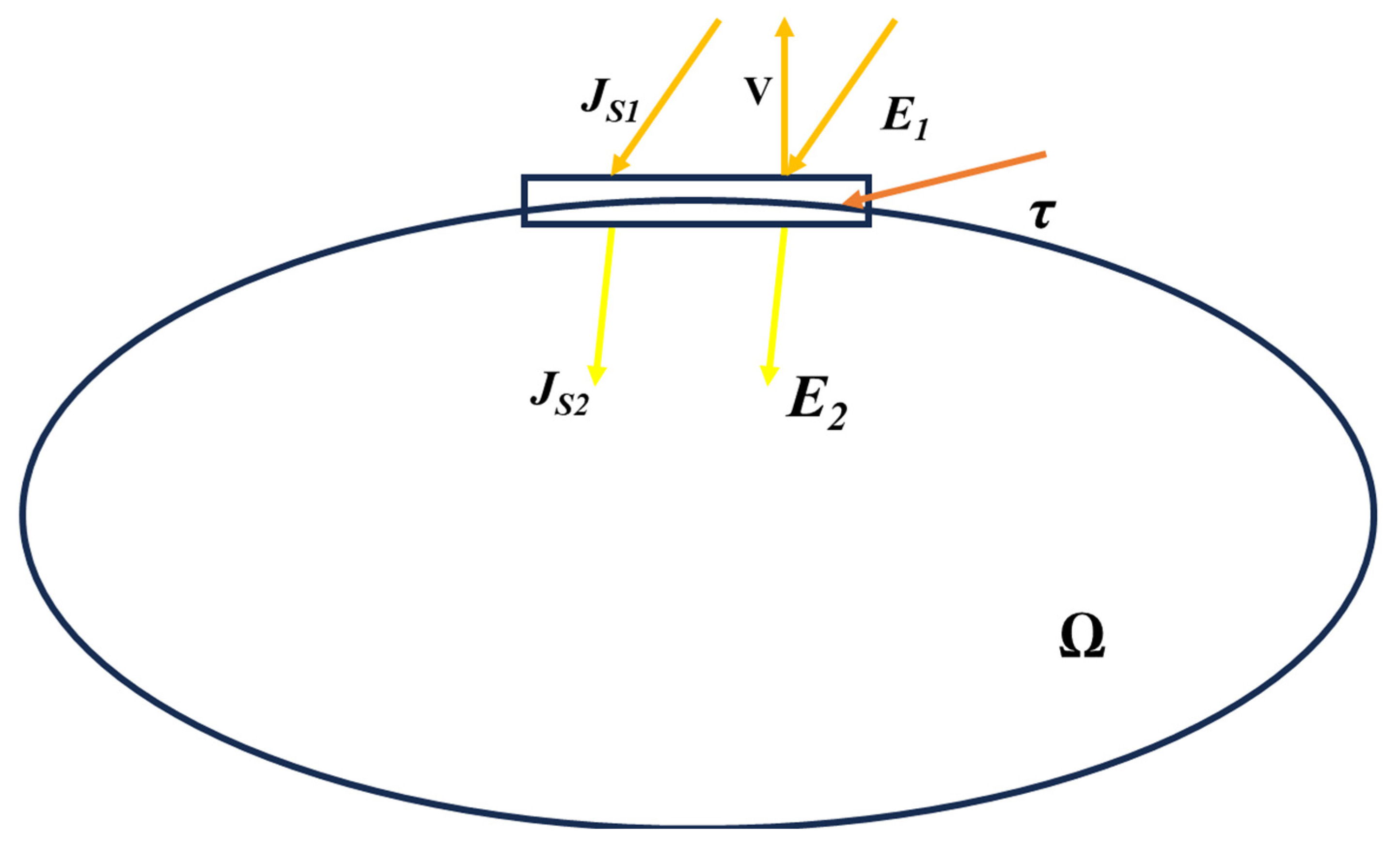
3.2. Boundary Conditions
3.3. Epithelial Tissue
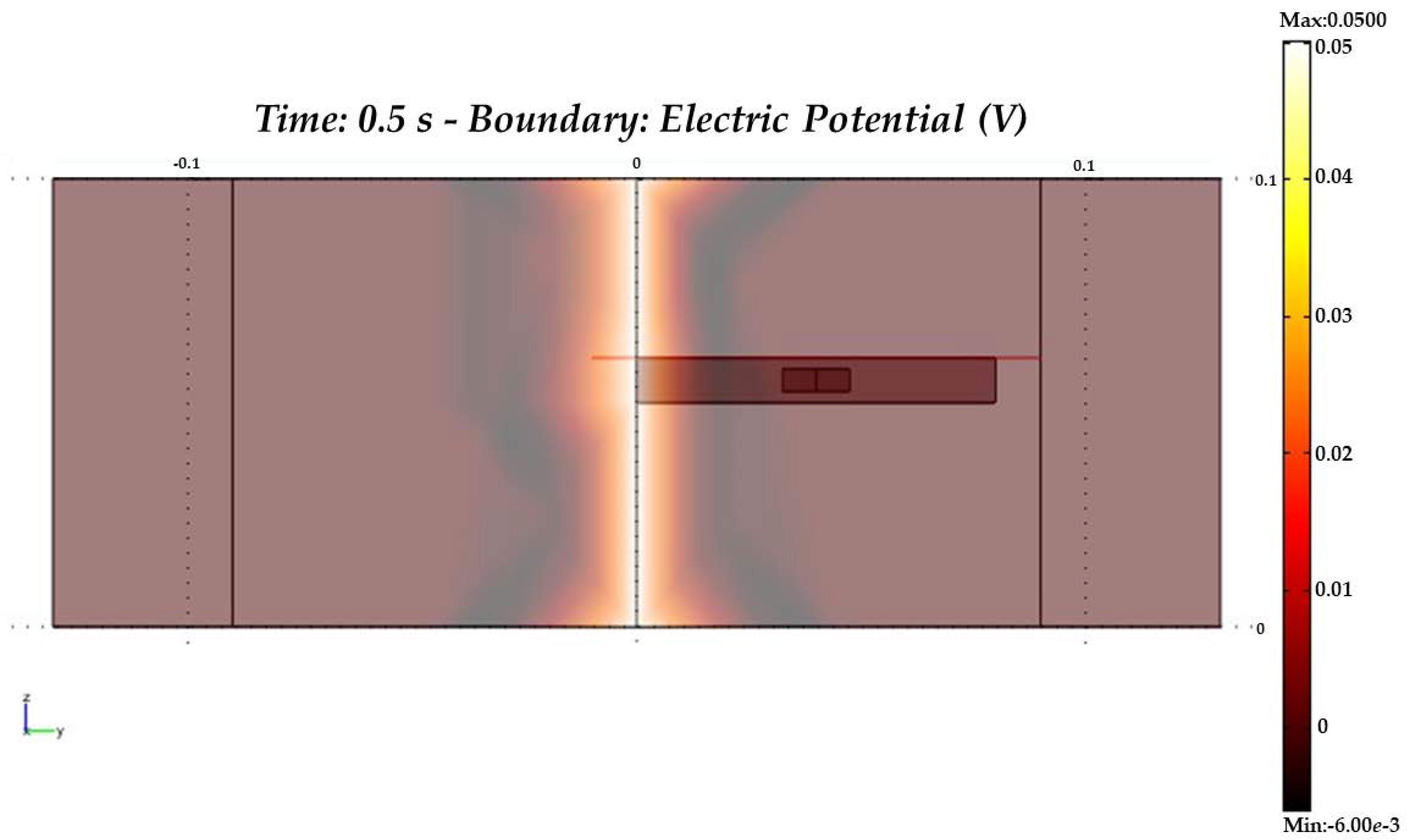
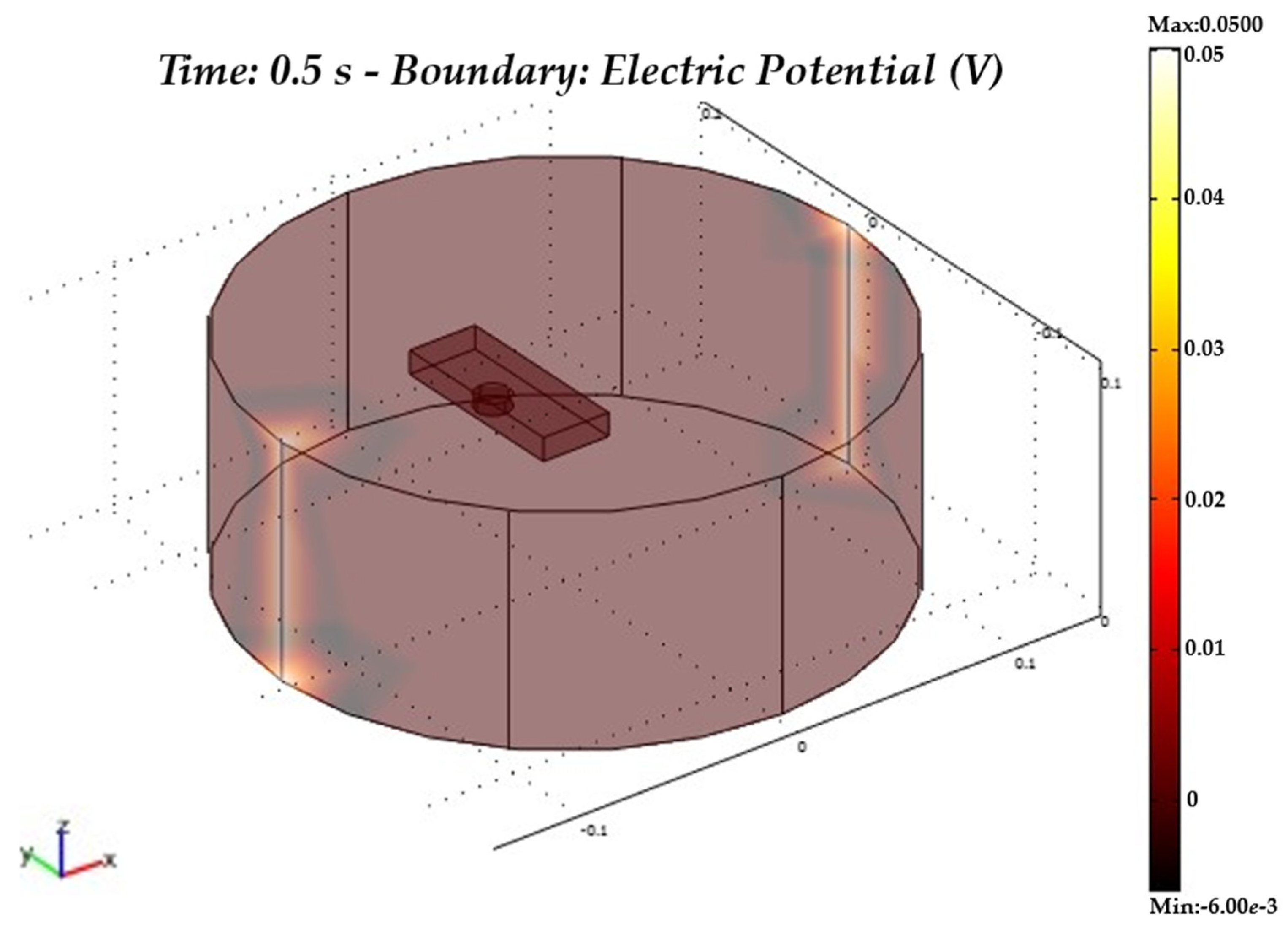
4. Model Realisation in COMSOL-Multiphysics®
- •
- 2D tomograph model analysing a tissue sample without carcinoma;
- •
- 2D tomograph model analysing a tissue sample with the presence of carcinoma;
- •
- 3D tomograph model analysing a tissue sample without carcinoma;
- •
- 3D tomograph model analysing a tissue sample with the presence of carcinoma.
- •
- Adjacent potential configuration;
- •
- Opposite potential configuration.
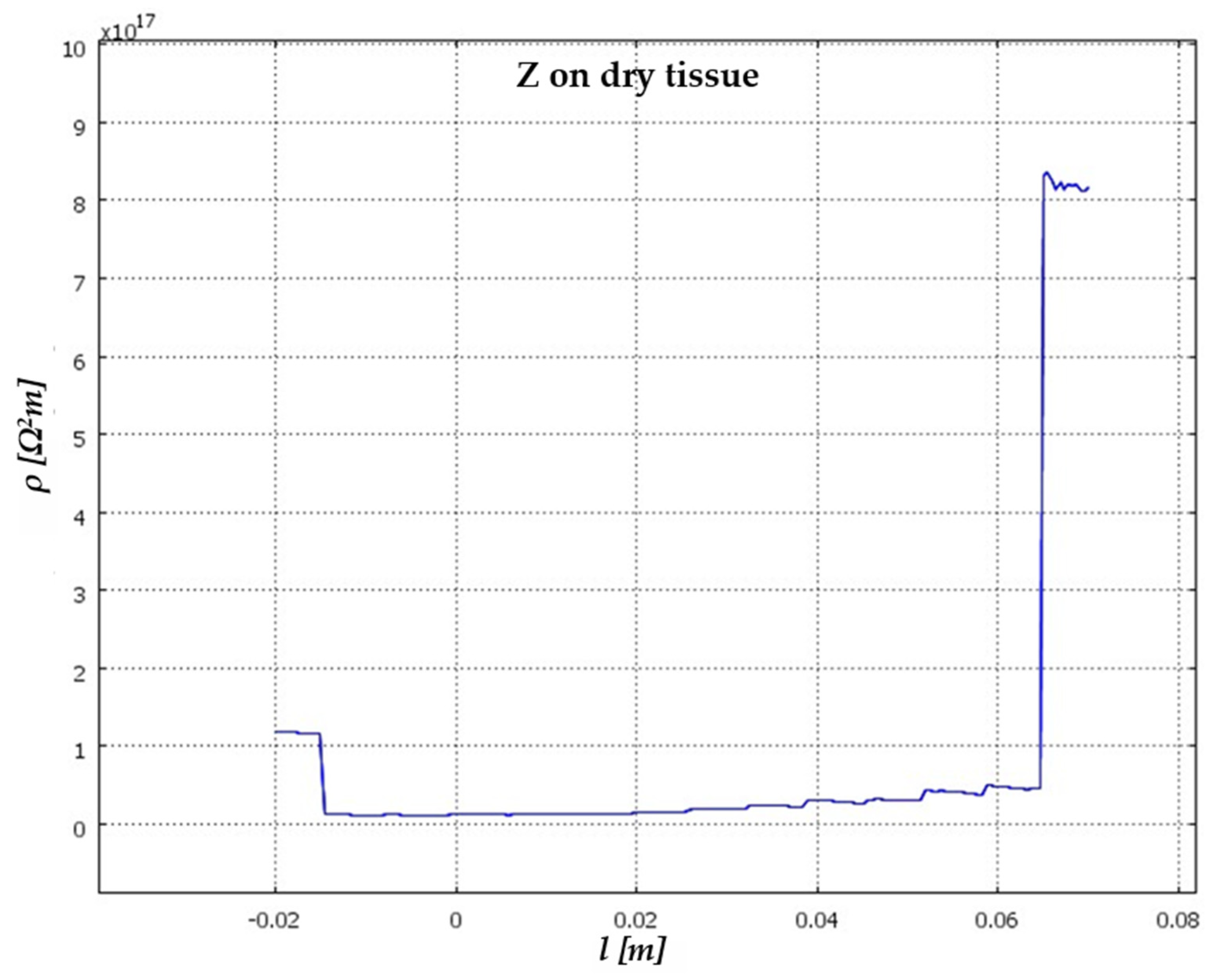
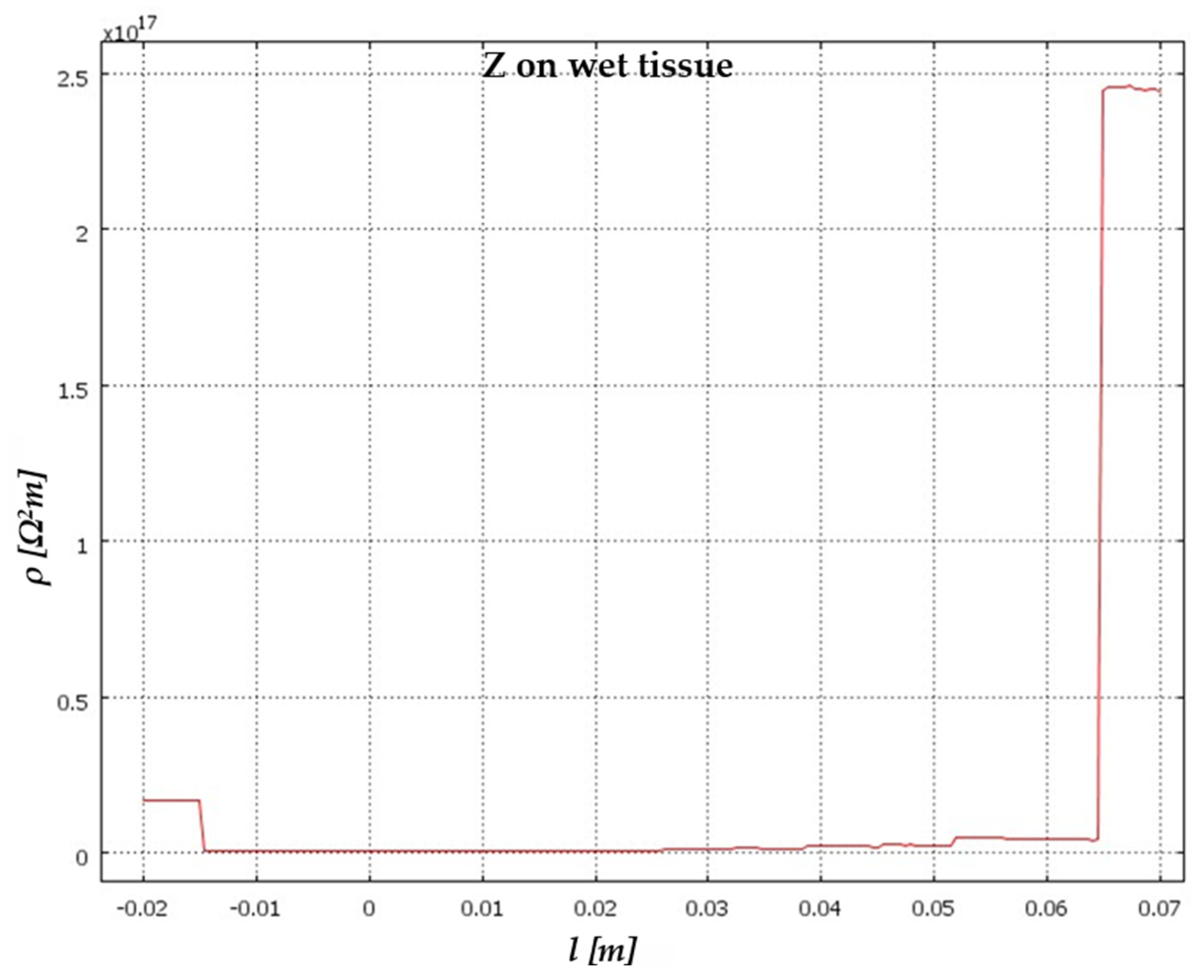
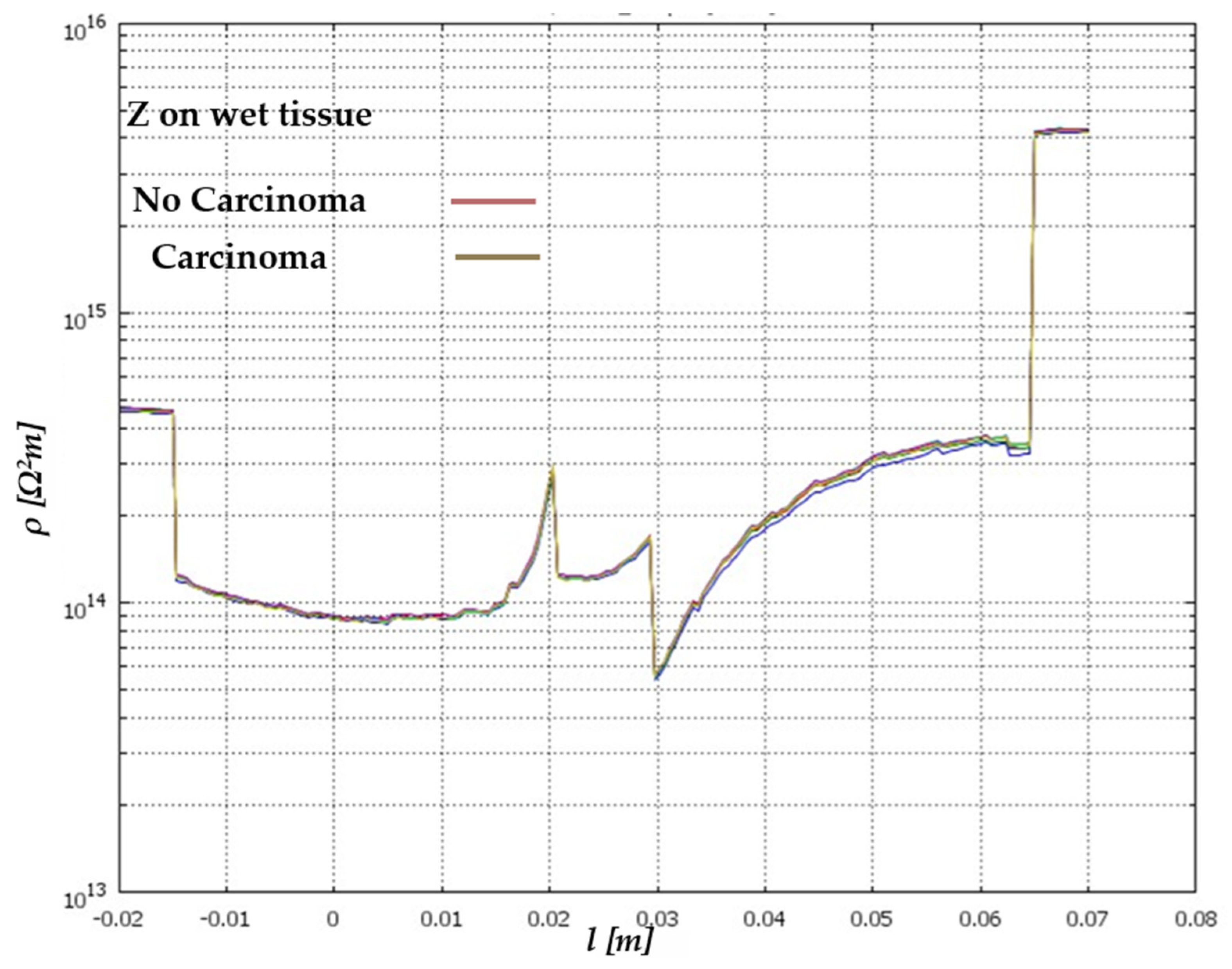
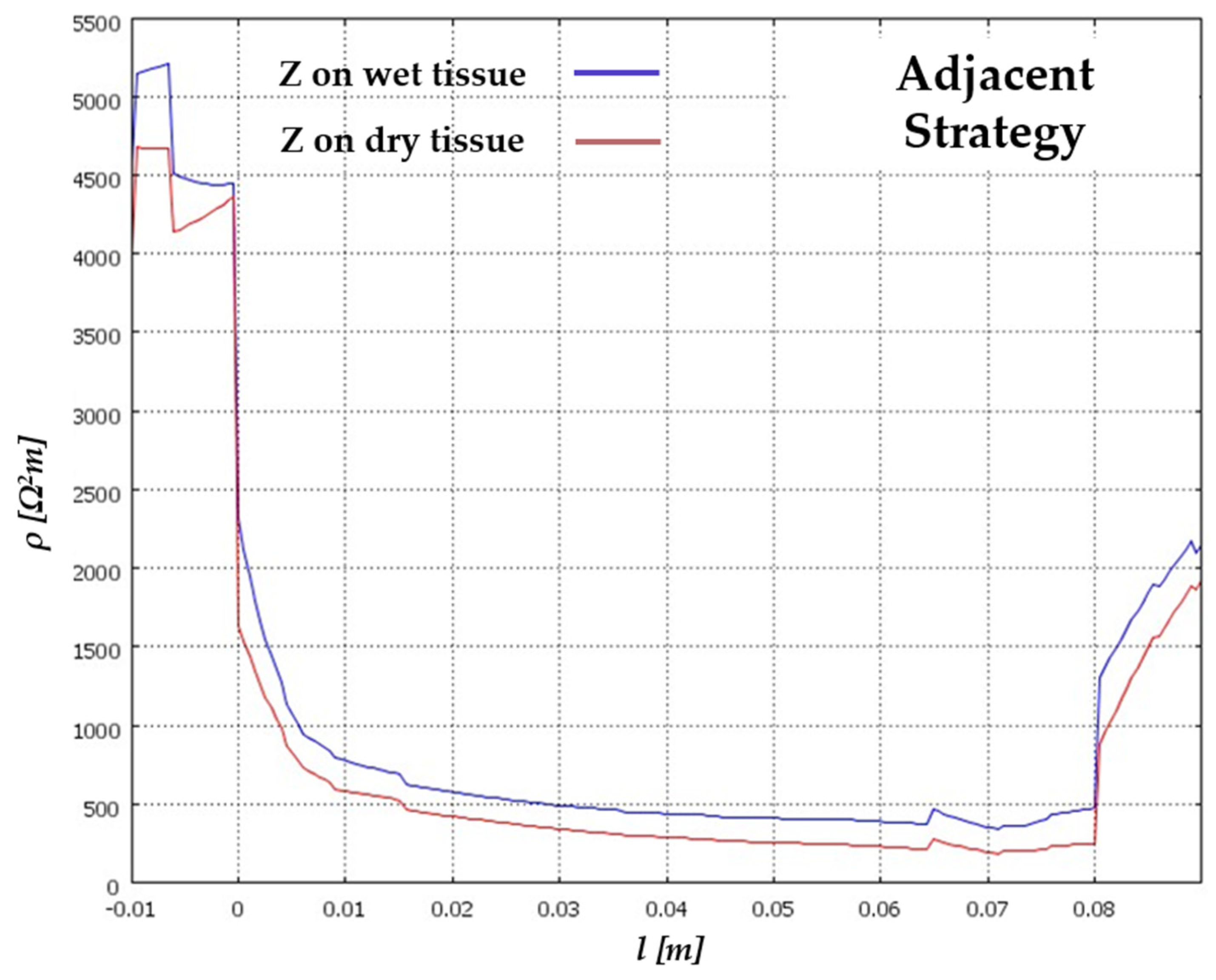
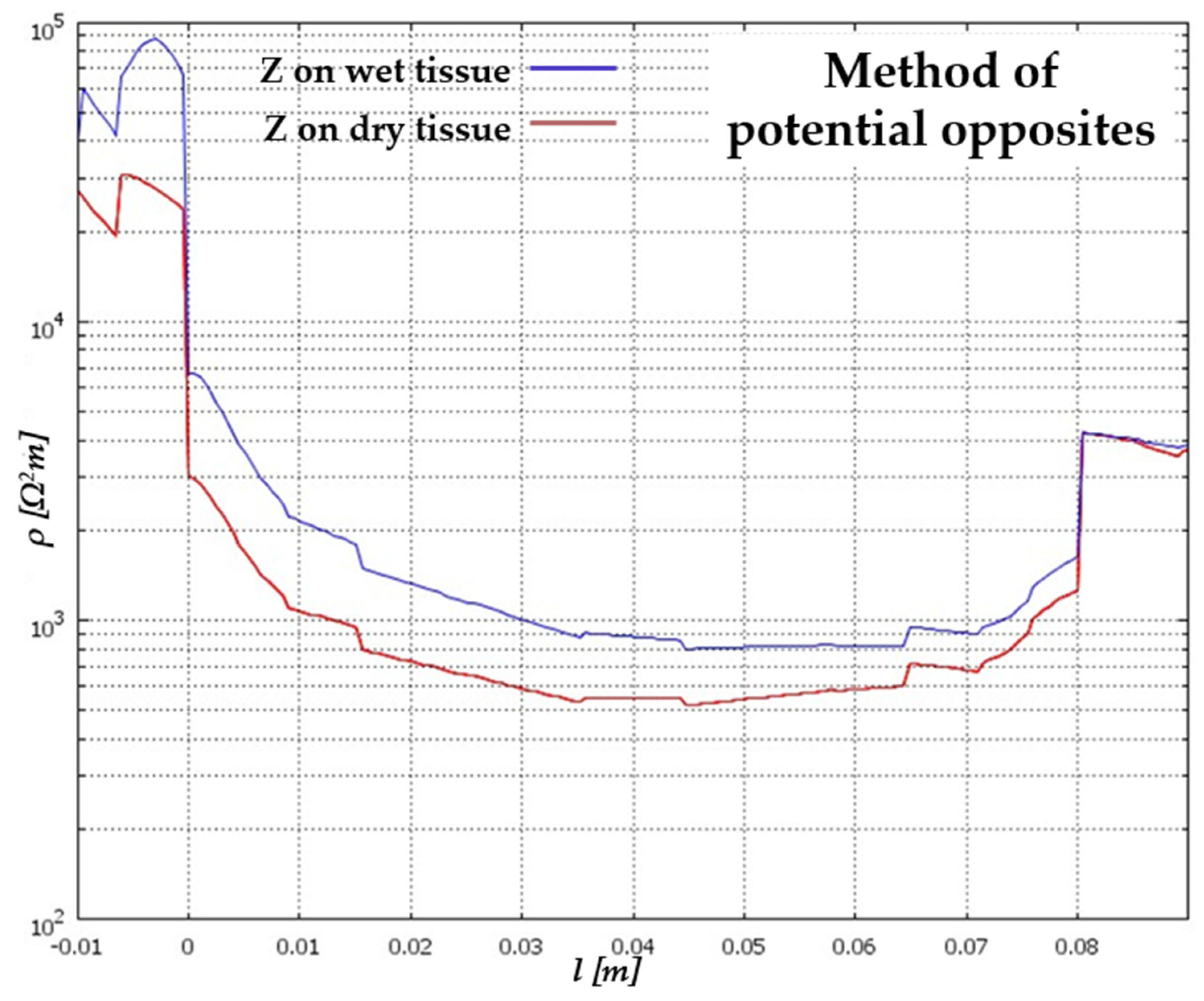
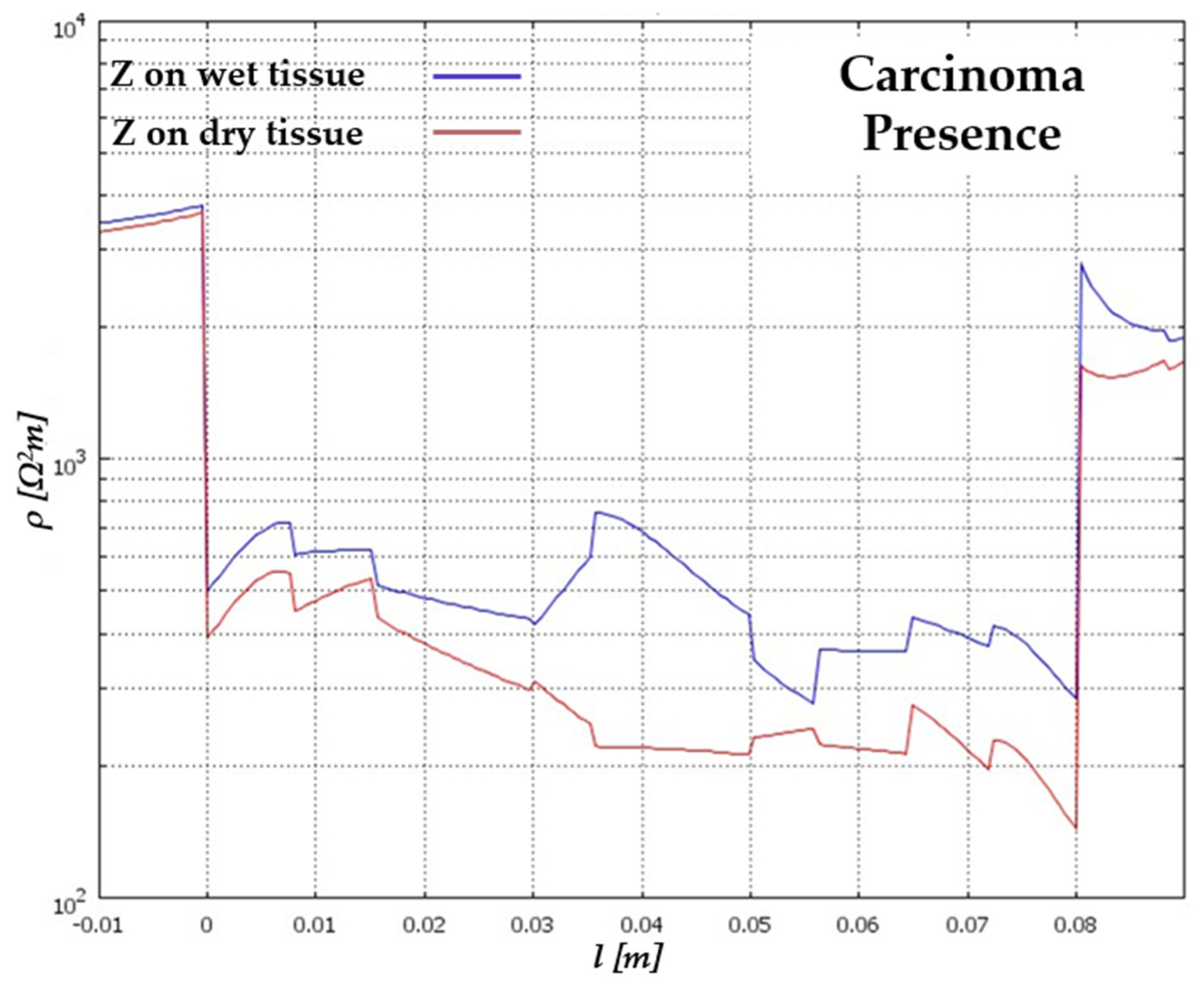
5. Results
- •
- Simplified implementation of the instrumentation due to the accessibility and cost-effectiveness of materials utilised;
- •
- Immediate detection of carcinoma presence and concurrent localization within the examined tissue;
- •
- Non-invasive analysis, ensuring no harm to the tissue being scrutinised;
- •
- Convenient electrical measurement facilitated by sensors or other cost-effective devices.
6. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, Y.; Hanzaee, F.F.; Jiang, D.; Bayford, R.H.; Demosthenous, A. Electrical Impedance Tomography for Biomedical Applications: Circuits and Systems Review. IEEE Open J. Circuits Syst. 2021, 2, 380–397. [Google Scholar] [CrossRef]
- Padilha Leitzke, J.; Zangl, H. A review on electrical impedance tomography spectroscopy. Sensors 2020, 20, 5160. [Google Scholar] [CrossRef] [PubMed]
- Seçkin, A.Ç.; Ateş, B.; Seçkin, M. Review on Wearable Technology in sports: Concepts, Challenges and opportunities. Appl. Sci. 2023, 13, 10399. [Google Scholar] [CrossRef]
- Ke, X.Y.; Hou, W.; Huang, Q. Advances in electrical impedance tomography-based brain imaging. Mil. Med. Res. 2022, 9, 10. [Google Scholar] [CrossRef] [PubMed]
- Adam, E.E.B.; Babikir, E. Survey on medical imaging of electrical impedance tomography (EIT) by variable current pattern methods. J. IoT Soc. Mob. Anal. Cloud 2021, 3, 82–95. [Google Scholar]
- Zhang, H.; Kalra, A.; Lowe, A.; Yu, Y.; Anand, G. A hydrogel-based electronic skin for touch detection using electrical impedance tomography. Sensors 2023, 23, 1571. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Wang, N.; Fan, L.F.; Wang, Y.Q.; Zhao, P.F.; Huang, L.; Wang, Z.Y. Imaging spatial-temporal distribution of moisture in intact maize ear. Measurement 2022, 202, 111809. [Google Scholar] [CrossRef]
- Khan, T.A.; Ling, S.H.; Rizvi, A.A. Optimisation of electrical Impedance tomography image reconstruction error using heuristic algorithms. Artif. Intell. Rev. 2023, 56, 15079–15099. [Google Scholar] [CrossRef]
- Tyni, T.; Stinchcombe, A.R.; Alexakis, S. A boundary integral equation method for the complete electrode model in electrical impedance tomography with tests on experimental data. SIAM J. Imaging Sci. 2024, 17, 672–705. [Google Scholar] [CrossRef]
- Angiulli, G.; Versaci, M.; Calcagno, S. Computation of the cutoff wavenumbers of metallic waveguides with symmetries by using a nonlinear eigenproblem formulation: A group theoretical approach. Mathematics 2020, 8, 489. [Google Scholar] [CrossRef]
- Jain, H.; Isaacson, D.; Edic, P.M.; Newell, J.C. Electrical impedance tomography of complex conductivity distributions with noncircular boundary. IEEE Trans. Biomed. Eng. 1997, 44, 1051–1060. [Google Scholar] [CrossRef] [PubMed]
- Angiulli, G.; Cacciola, M.; Calcagno, S.; De Carlo, D.; Morabito, C.F.; Sgró, A.; Versaci, M. A numerical study on the performances of the flexible BiCGStab to solve the discretized E-field integral equation. Int. J. Appl. Electromagn. Mech. 2014, 46, 547–553. [Google Scholar] [CrossRef]
- Kulkarni, R.; Boverman, G.; Isaacson, D.; Saulnier, G.; Newell, J.C. Layered model for breasts in electrical impedance tomography. In Proceedings of the 2007 29th Annual International Conference of the IEEE Engineering in Medicine and Biology Society, Lyon, France, 22–26 August 2007; pp. 4150–4153. [Google Scholar]
- Cappellini, I.; Campagnola, L.; Consales, G. Electrical Impedance Tomography, Artificial Intelligence, and Variable Ventilation: Transforming Respiratory Monitoring and Treatment in Critical Care. J. Pers. Med. 2024, 14, 677. [Google Scholar] [CrossRef]
- Pennati, F.; Angelucci, A.; Morelli, L.; Bardini, S.; Barzanti, E.; Cavallini, F.; Conelli, A.; Di Federico, G.; Paganelli, C.; Aliverti, A. Electrical Impedance Tomography: From the Traditional Design to the Novel Frontier of Wearables. Sensors 2023, 23, 1182. [Google Scholar] [CrossRef] [PubMed]
- Singh, R.K.; Nayak, N.P.; Behl, T.; Arora, R.; Anwer, M.K.; Gulati, M.; Bungau, S.G.; Brisc, M.C. Exploring the Intersection of Geophysics and Diagnostic Imaging in the Health Sciences. Diagnostics 2024, 14, 139. [Google Scholar] [CrossRef]
- Bianco, M.G.; Quattrone, A.; Sarica, A.; Vescio, B.; Buonocore, J.; Vaccaro, M.G.; Aracri, F.; Calomino, C.; Gramigna, V.; Quattrone, A. Cortical atrophy distinguishes idiopathic normal pressure hydrocephalus from progressive supranuclear palsy: A machine learning approach. Park. Relat. Disord. 2022, 103, 7–14. [Google Scholar]
- Tang, M.; Wang, W.; Wheeler, J.; McCormick, M.; Dong, X. The number of electrodes and basis functions in EIT image reconstruction. Physiol. Meas. 2002, 23, 129–140. [Google Scholar] [CrossRef]
- Sarac, E.; Meiwes, A.; Eigentler, T.K.; Forchhammer, S.; Kofler, L.; Häfner, H.M.; Garbe, C. Diagnostic accuracy of electrical impedance spectroscopy in non-melanoma skin cancer. Acta Derm. Venereol. 2020, 100, adv00328. [Google Scholar] [CrossRef]
- Braun, R.P.; Mangana, J.; Goldinger, S.; French, L.; Dummer, R.; Marghoob, A.A. Electrical Impedance Spectroscopy in Skin Cancer Diagnosis. Dermatol. Clin. 2017, 35, 489–493. [Google Scholar] [CrossRef]
- Becher, T.; Buchholz, V.; Hassel, D.; Meinel, T.; Schädler, D.; Frerichs, I.; Weiler, N. Individualization of PEEP and tidal in ARDS patients with electrical impedance tomography: A pilot feasibility study. Ann. Intensive Care 2021, 11, 89. [Google Scholar] [CrossRef]
- Zhao, Z.; Steinmann, D.; Frerichs, I.; Guttmann, J.; Möller, K. PEEP titration guided by ventilation homogeneity: A feasibility study using electrical impedance tomography. Crit. Care 2010, 14, R8. [Google Scholar] [CrossRef]
- Glickman, Y.A.; Filo, O.; David, M.; Yayon, A.; Topaz, M.; Zamir, B.; Kenan, G. Electrical impedance scanning: A new approach to skin cancer diagnosis. Ski. Res. Technol. 2003, 9, 262–268. [Google Scholar] [CrossRef]
- Aberg, P.; Nicander, I.; Hansson, J.; Geladi, P.; Holmgren, U.; Ollmar, S. Skin cancer identification using multifrequency electrical impedance—A potential screening tool. IEEE Trans. Biomed. Eng. 2004, 51, 2097–2102. [Google Scholar] [CrossRef]
- Åberg, P.; Birgersson, U.; Elsner, P.; Mohr, P.; Ollmar, S. Electrical impedance spectroscopy and the diagnostic accuracy for malignant melanoma. Exp. Dermatol. 2011, 20, 648–652. [Google Scholar] [CrossRef]
- Mohr, P.; Birgersson, U.; Berking, C.; Henderson, C.; Trefzer, U.; Kemeny, L.; Paoli, J. Electrical impedance spectroscopy as a potential adjunct diagnostic tool for cutaneous melanoma. Ski. Res. Technol. 2013, 19, 75–83. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, J.; Yu, G.; Niu, F.; He, P. Design and preliminary evaluation of a portable device for the measurement of bioimpedance spectroscopy. Physiol. Meas. 2006, 27, 1293. [Google Scholar] [CrossRef]
- Åberg, P.; Geladi, P.; Nicander, I.; Hansson, J.; Holmgren, U.; Ollmar, S. Non-invasive and microinvasive electrical impedance spectra of skin cancer—A comparison between two techniques. Ski. Res. Technol. 2005, 11, 281–286. [Google Scholar] [CrossRef]
- Narayanamurthy, V.; Padmapriya, P.; Noorasafrin, A.; Pooja, B.; Hema, K.; Nithyakalyani, K.; Samsuri, F. Skin cancer detection using non-invasive techniques. RSC Adv. 2018, 8, 28095–28130. [Google Scholar] [CrossRef]
- Kamat, D.K.; Chavan, A.P.; Patil, P.M. Bio-impedance measurement system for analysis of skin diseases. Int. J. Appl. Innov. Eng. Manag. 2014, 3, 92–96. [Google Scholar]
- Zhao, Z.; Zhu, H.; Li, X.; Sun, L.; He, F.; Chung, J.E.; Xie, C. Ultraflexible electrode arrays for months-long high-density electrophysiological mapping of thousands of neurons in rodents. Nat. Biomed. Eng. 2023, 7, 520–532. [Google Scholar] [CrossRef] [PubMed]
- Eisenberg, R.S. Maxwell equations without a polarization field, Using a paradigm from biophysics. Entropy 2021, 23, 172. [Google Scholar] [CrossRef]
- Abdul-Al, M.; Amar, A.S.; Elfergani, I.; Littlehales, R.; Ojaroudi Parchin, N.; Al-Yasir, Y.; Abd-Alhameed, R.A. Wireless electromagnetic radiation assessment based on the specific absorption rate (SAR): A review case study. Electronics 2022, 11, 511. [Google Scholar] [CrossRef]
- Wust, P.; Stein, U.; Ghadjar, P. Non-thermal membrane effects of electromagnetic fields and therapeutic applications in oncology. Int. J. Hyperth. 2021, 38, 715–731. [Google Scholar] [CrossRef]
- Mazuir, E.; Fricker, D.; Sol-Foulon, N. Neuron–oligodendrocyte communication in myelination of cortical GABAergic cells. Life 2021, 11, 216. [Google Scholar] [CrossRef]
- Wu, H.; Zhou, W.; Yang, Y.; Jia, J.; Bagnaninchi, P. Exploring the potential of electrical impedance tomography for tissue engineering applications. Materials 2018, 11, 930. [Google Scholar] [CrossRef]
- Yilmaz, T.; Ates Alkan, F. In vivo dielectric properties of healthy and benign rat mammary tissues from 500 MHz to 18 GHz. Sensors 2020, 20, 2214. [Google Scholar] [CrossRef]
- Laganà, F.; Britti, D.; Fiorillo, A.S.; Pullano, S.A. New Surface Electrical Charge Detection System for Ecology and Insect Monitoring. In Proceedings of the 2023 IEEE International Workshop on Biomedical Applications, Technologies and Sensors, BATS 2023—Proceedings, Catanzaro, Italy, 28–29 September 2023. [Google Scholar]
- Di Meo, S.; Bonello, J.; Farhat, I.; Farrugia, L.; Pasian, M.; Camilleri Podesta, M.T.; Sammut, C.V. The variability of dielectric permittivity of biological tissues with water content. J. Electromagn. Waves Appl. 2022, 36, 48–68. [Google Scholar] [CrossRef]
- Seweryn, A. Interactions between surfactants and the skin—Theory and practice. Adv. Colloid Interface Sci. 2018, 256, 242–255. [Google Scholar] [CrossRef]
- Pullano, S.A.; Greco, M.; Islam, S.K.; Fiorillo, A.S. Thermoelectrical Characterization of Cells Using a Pyroelectric Sensor. In Proceedings of the 2023 IEEE SENSORS, Vienna, Austria, 29 October–1 November 2023. [Google Scholar]
- Tang, J.; Lu, M.; Xie, Y.; Yin, W. A Novel Efficient FEM Thin shell model for bio-impedance analysis. Biosensors 2020, 10, 69. [Google Scholar] [CrossRef]
- Pullano, S.A.; Greco, M.; Foti, D.P.; Brunetti, A.; Fiorillo, A.S. Dual Pyroelectric Sensor for Thermal Characterization of Cell Lines. In Proceedings of the 2021 IEEE Sensors Applications Symposium (SAS), Virtual Conference, Sundsvall, Sweden, 23–25 August 2021. [Google Scholar]
- Laganà, F.; De Carlo, D.; Calcagno, S.; Pullano, S.A.; Critello, D.; Falcone, F.; Fiorillo, A.S. Computational model of cell deformation under fluid flow-based rolling. In Proceedings of the 7th IEEE International Conference on E-Health and Bioengineering, EHB 2019, Iasi, Romania, 21–23 November 2019. [Google Scholar]
- Lee, S.; Ozkavukcu, S.; Ku, S.Y. Current and future perspectives for improving ovarian tissue cryopreservation and transplantation outcomes for cancer patients. Reprod. Sci. 2021, 28, 1746–1758. [Google Scholar] [CrossRef]
- Laganà, F.; De Carlo, D.; Calcagno, S.; Oliva, G.; Pullano, S.A.; Fiorillo, A.S. Modeling of Electrical Impedance Tomography for Carcinoma Detection. In Proceedings of the 10th E-Health and Bioengineering Conference EHB 2022, Virtual Conference, Iasi, Romania, 17–18 November 2022. [Google Scholar] [CrossRef]
- Menniti, M.; Oliva, G.; Laganà, F.; Bianco, M.G.; Fiorillo, A.S.; Pullano, S.A. Portable Non-Invasive Ventilator for Homecare and Patients Monitoring System. In Proceedings of the 2023 IEEE International Symposium on Medical Measurements and Applications (MeMeA), Jeju, Republic of Korea, 14–16 June 2023; pp. 1–5. [Google Scholar] [CrossRef]
- Menniti, M.; Laganà, F.; Oliva, G.; Bianco, M.; Fiorillo, A.S.; Pullano, S.A. Development of Non-Invasive Ventilator for Homecare and Patient Monitoring System. Electronics 2024, 13, 790. [Google Scholar] [CrossRef]
- Vaupel, P.; Piazena, H. Hyperhydration of cancers: A characteristic biophysical trait strongly increasing O2, CO2, glucose and lactate diffusivities, and improving thermophysical properties of solid malignancies. In International Society on Oxygen Transport to Tissue; Springer International Publishing: Cham, Switzerland, 2022; pp. 135–145. [Google Scholar]




| Signal Used | Description | Merits | Demerits | Reference |
|---|---|---|---|---|
| 1 kHz and 1000 kHz | Distinguishes the skin cancer from the benign lesions using multi-frequency impedance spectra | The result obtained is more accurate than conventional methods | Distinguishing the tumours takes more time, and false results may also be obtained | [24] |
| 1–1000 kHz | Compares the detection of skin cancer by a non-invasive probe and micro-invasive electrode system, whose surface is furnished with tiny spikes which get penetrated to the stratum corneum | The electrode system produces a better result | Minimally invasive technique | [28] |
| 1 kHz and 1 MHz | Describes the method for detecting skin cancer using electric impedance. The electric impedance of the biological system decreases with the increase in frequency | High resolution | Multivariate and the impedance is complex | [29] |
| 1 kHz to 2.5 MHz | Accuracy of electrical impedance to classify malignant melanoma from benign tumour by automated classification algorithm | Accuracy is high | Various algorithm is needed for the classification of skin cancer | [25] |
| 1–100 kHz | Non-invasive approach for detecting the presence of skin lesions by measuring the impedance change | Low-cost and portable | Electrodes are used, which cause discomfort | [30] |
| 1 kHz to 2.5 MHz | EIS algorithm is used on lesions to differentiate normal skin from abnormal lesions | High resolution | An experienced physician is required | [20] |
| 20 kHz to 1 MHz | A portable bio-impedance system is used to diagnose skin cancer based on the magnitude ratio and phase detection method | Act as a great tool for monitoring the physiological conditions of the biological system | High cost | [27] |
| Thickness [m] | Applied Potential [V] | Conductivity [S/m] | Permittivity | Frequency [Hz] |
|---|---|---|---|---|
| 0.005 | 0.05 | 0.0002 | 1136 | 50 |
| Thickness [m] | Applied Potential [V] | Conductivity [S/m] | Permittivity | Frequency [Hz] |
|---|---|---|---|---|
| 0.005 | 0.05 | 0.0002 | 1136 | 50 |
| Type of Tissue | Thickness [m] | Applied Potential [V] | Conductivity [S/m] | Permittivity | Frequency [Hz] |
|---|---|---|---|---|---|
| Wet | 0.03 | 0.05 | 0.0042719 | 51,274 | 50 |
| Cancer | 0.0001 | 0.05 | 0.0013 | 1 | 50 |
| Type of Tissue | Dimensions XYZ [m] | Applied Potential [V] | Conductivity [S/m] | Permittivity | Frequency [Hz] |
|---|---|---|---|---|---|
| Dry | 0.08 × 0.03 × 0.01 | 0.05 | 0.0002 | 1136 | 50 |
| Wet | 0.08 × 0.03 × 0.01 | 0.05 | 0.0042719 | 51,274 | 50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Laganà, F.; Prattico, D.; De Carlo, D.; Oliva, G.; Pullano, S.A.; Calcagno, S. Engineering Biomedical Problems to Detect Carcinomas: A Tomographic Impedance Approach. Eng 2024, 5, 1594-1614. https://doi.org/10.3390/eng5030084
Laganà F, Prattico D, De Carlo D, Oliva G, Pullano SA, Calcagno S. Engineering Biomedical Problems to Detect Carcinomas: A Tomographic Impedance Approach. Eng. 2024; 5(3):1594-1614. https://doi.org/10.3390/eng5030084
Chicago/Turabian StyleLaganà, Filippo, Danilo Prattico, Domenico De Carlo, Giuseppe Oliva, Salvatore A. Pullano, and Salvatore Calcagno. 2024. "Engineering Biomedical Problems to Detect Carcinomas: A Tomographic Impedance Approach" Eng 5, no. 3: 1594-1614. https://doi.org/10.3390/eng5030084
APA StyleLaganà, F., Prattico, D., De Carlo, D., Oliva, G., Pullano, S. A., & Calcagno, S. (2024). Engineering Biomedical Problems to Detect Carcinomas: A Tomographic Impedance Approach. Eng, 5(3), 1594-1614. https://doi.org/10.3390/eng5030084









