NACA 2412 Drag Reduction Using V-Shaped Riblets
Abstract
1. Introduction
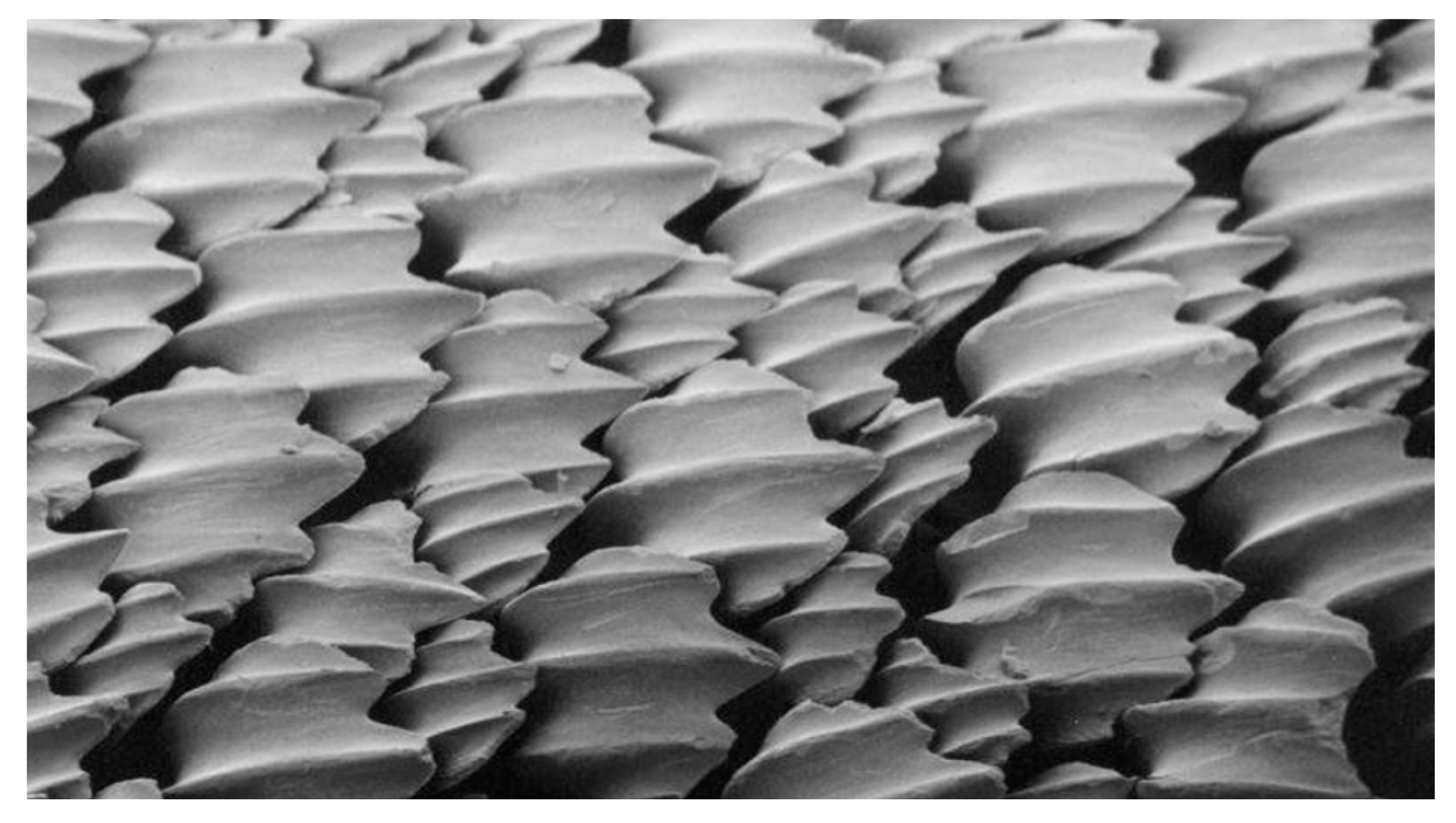
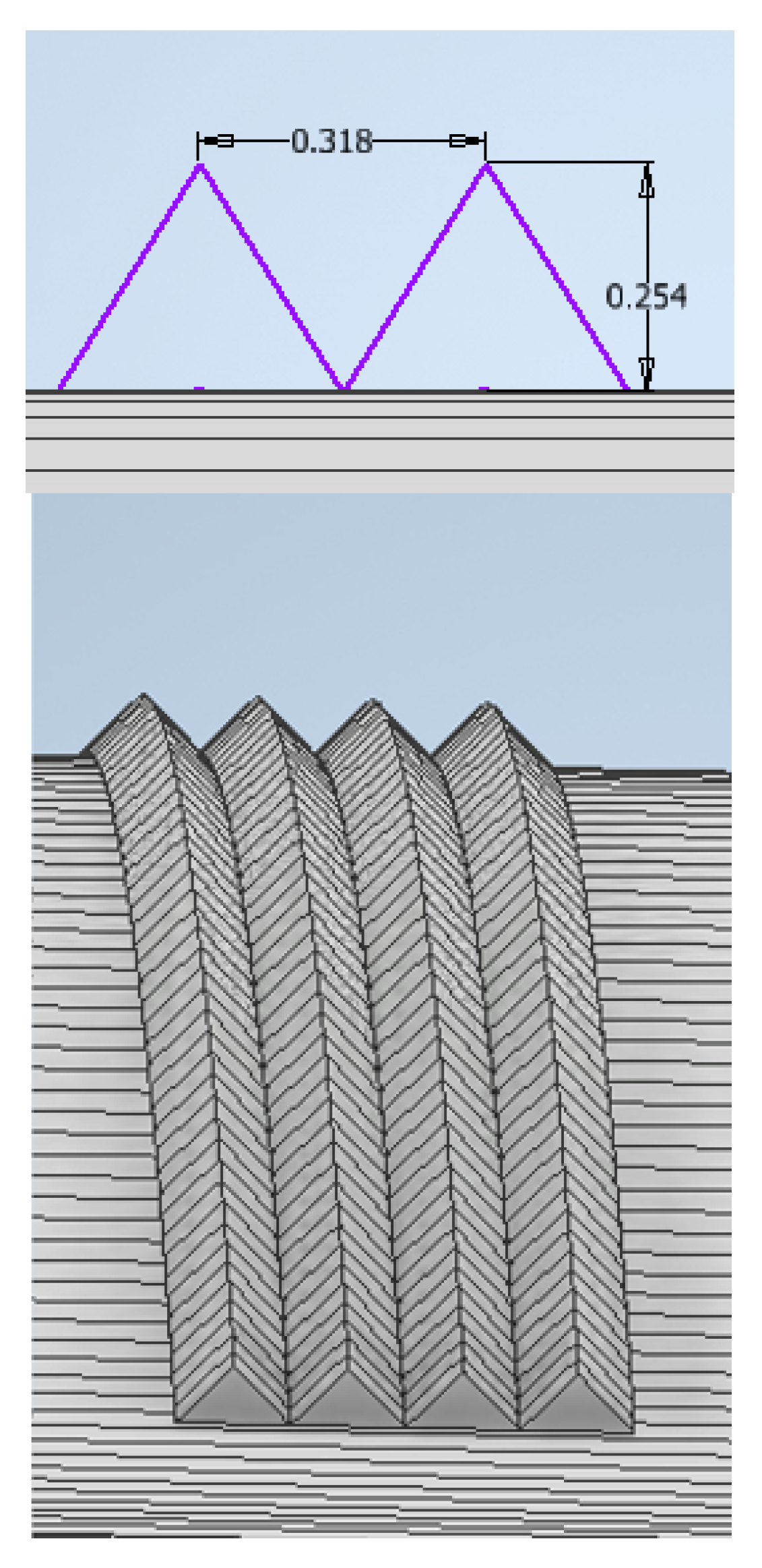
2. Computational Design
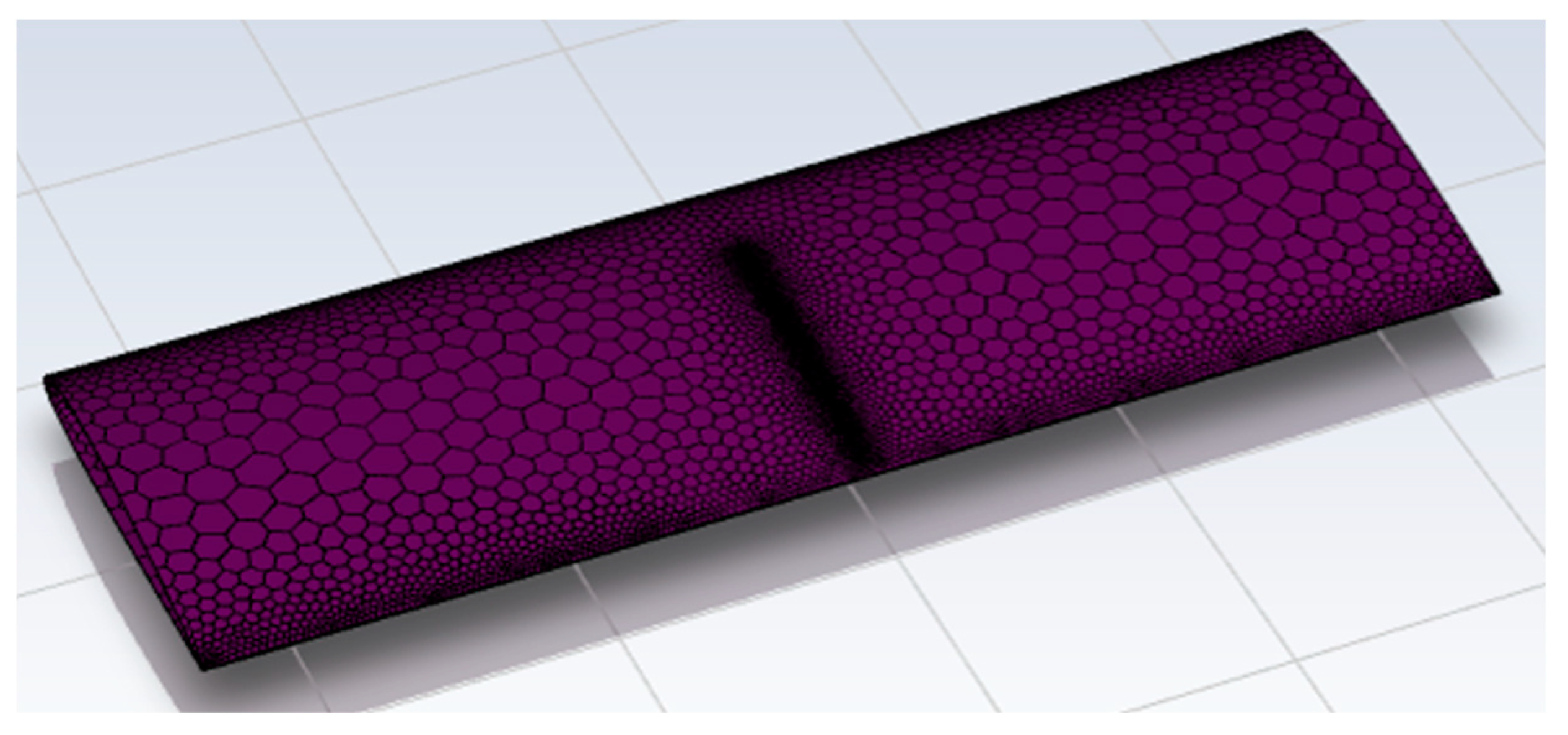
3. Computational Mesh
4. Computational Analysis
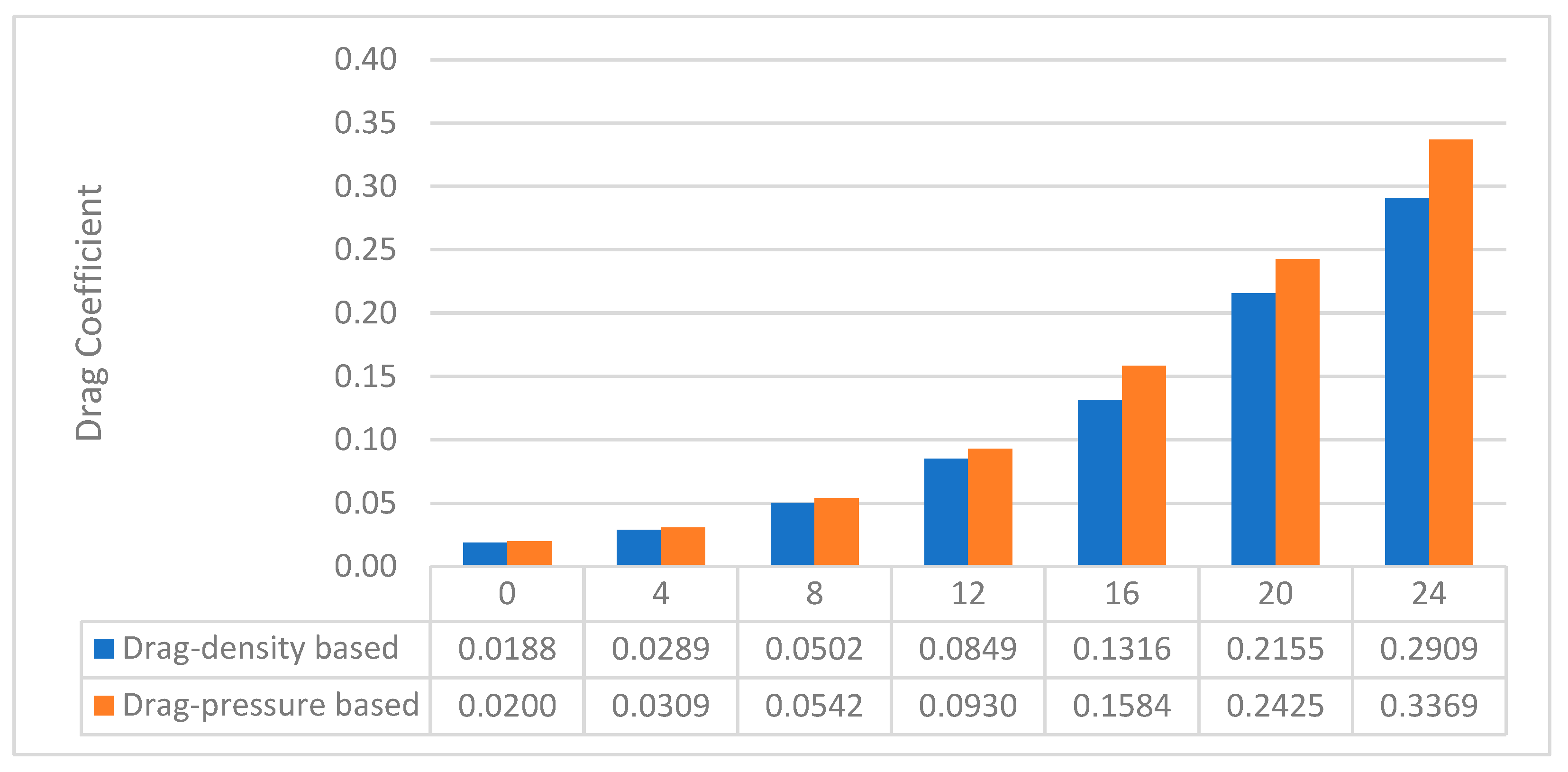
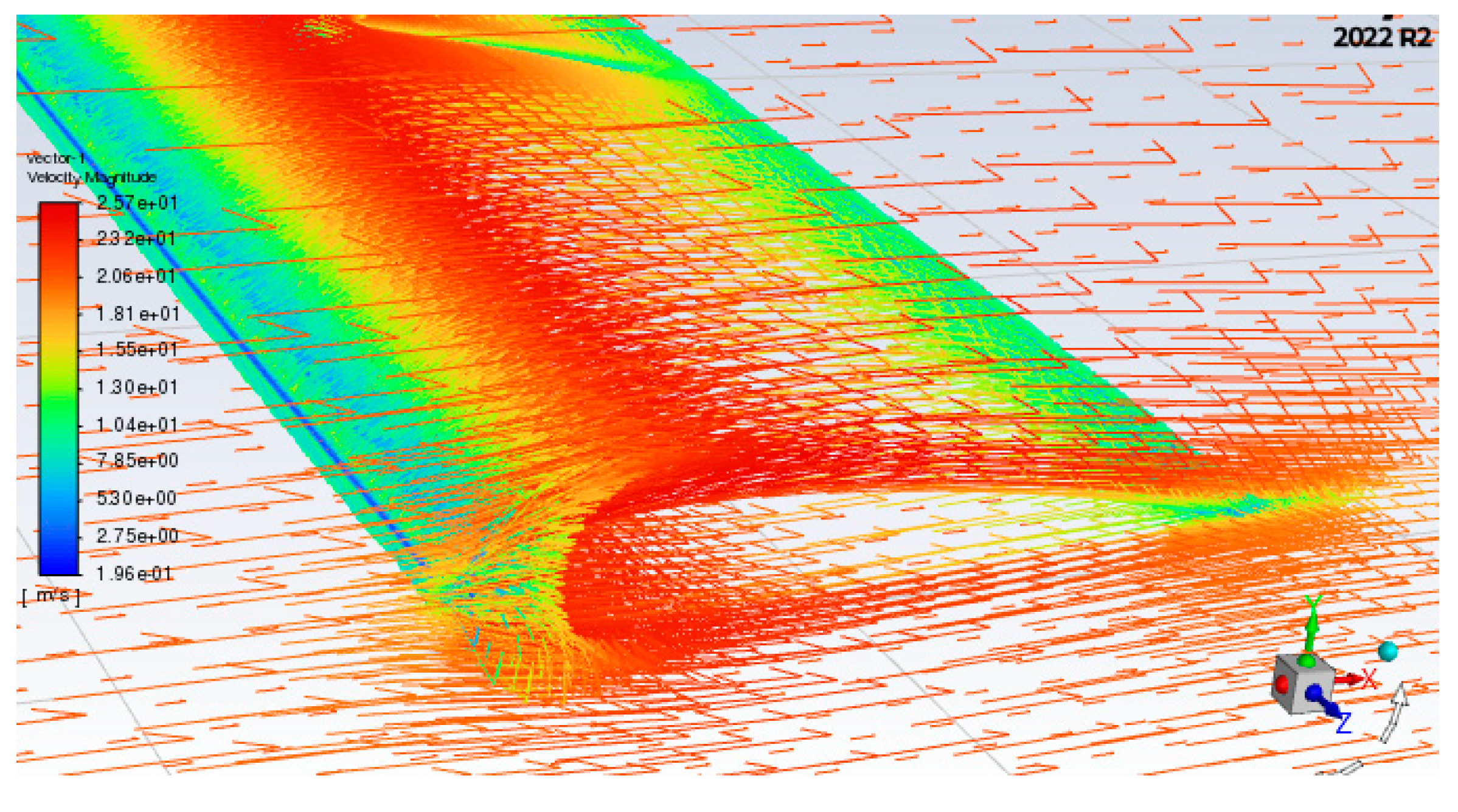
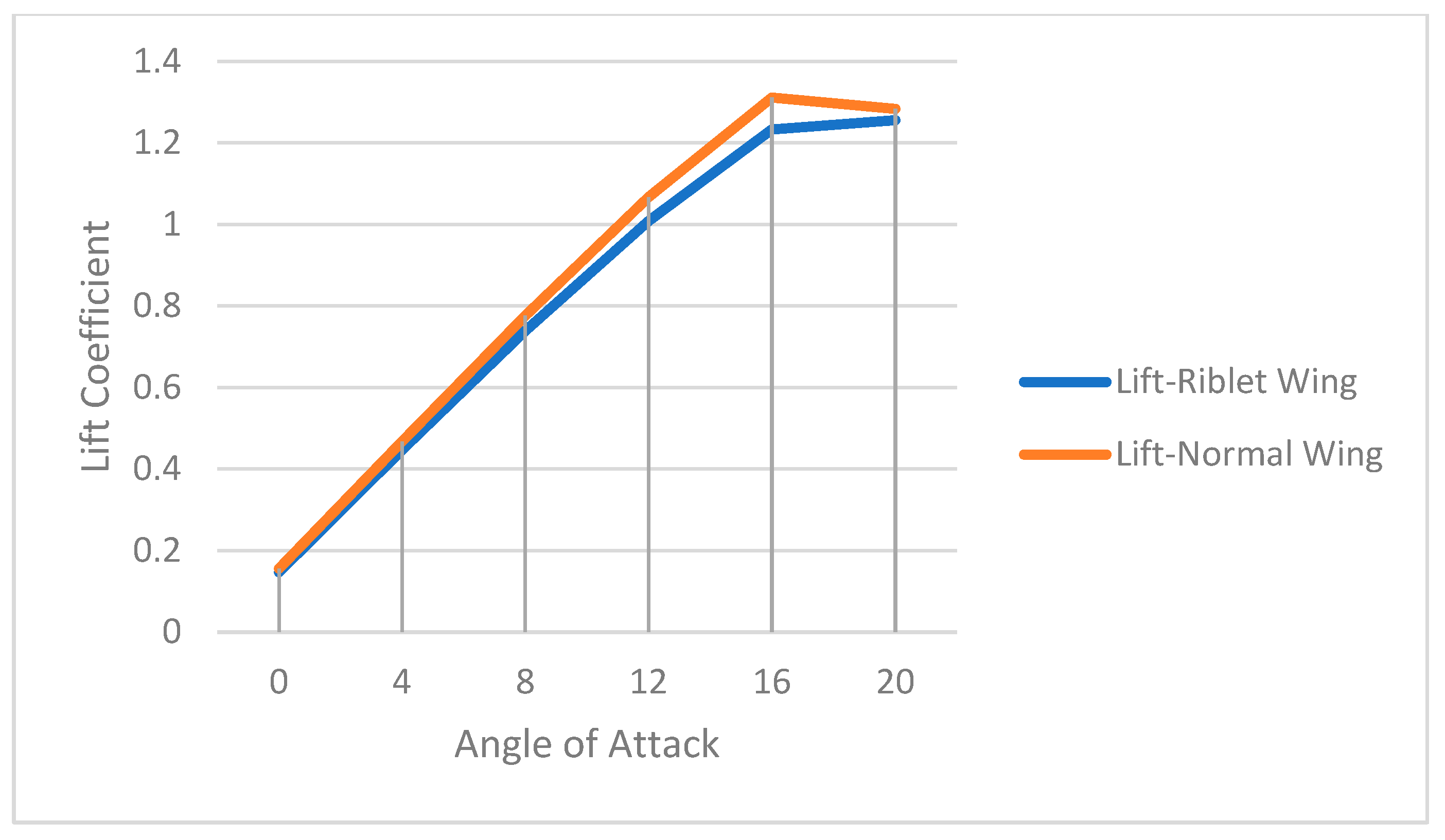
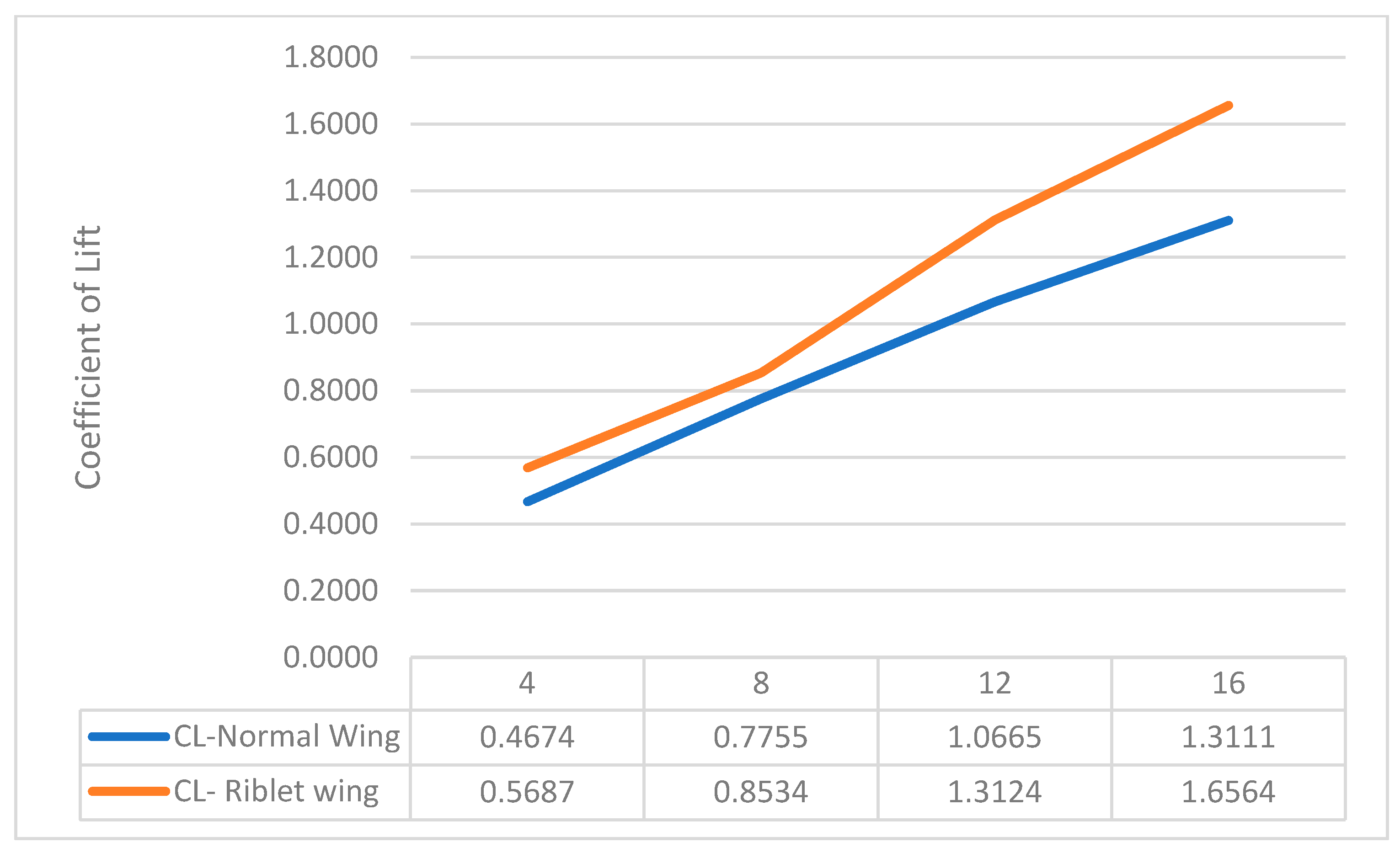
5. Computational Result Analysis

6. Validation
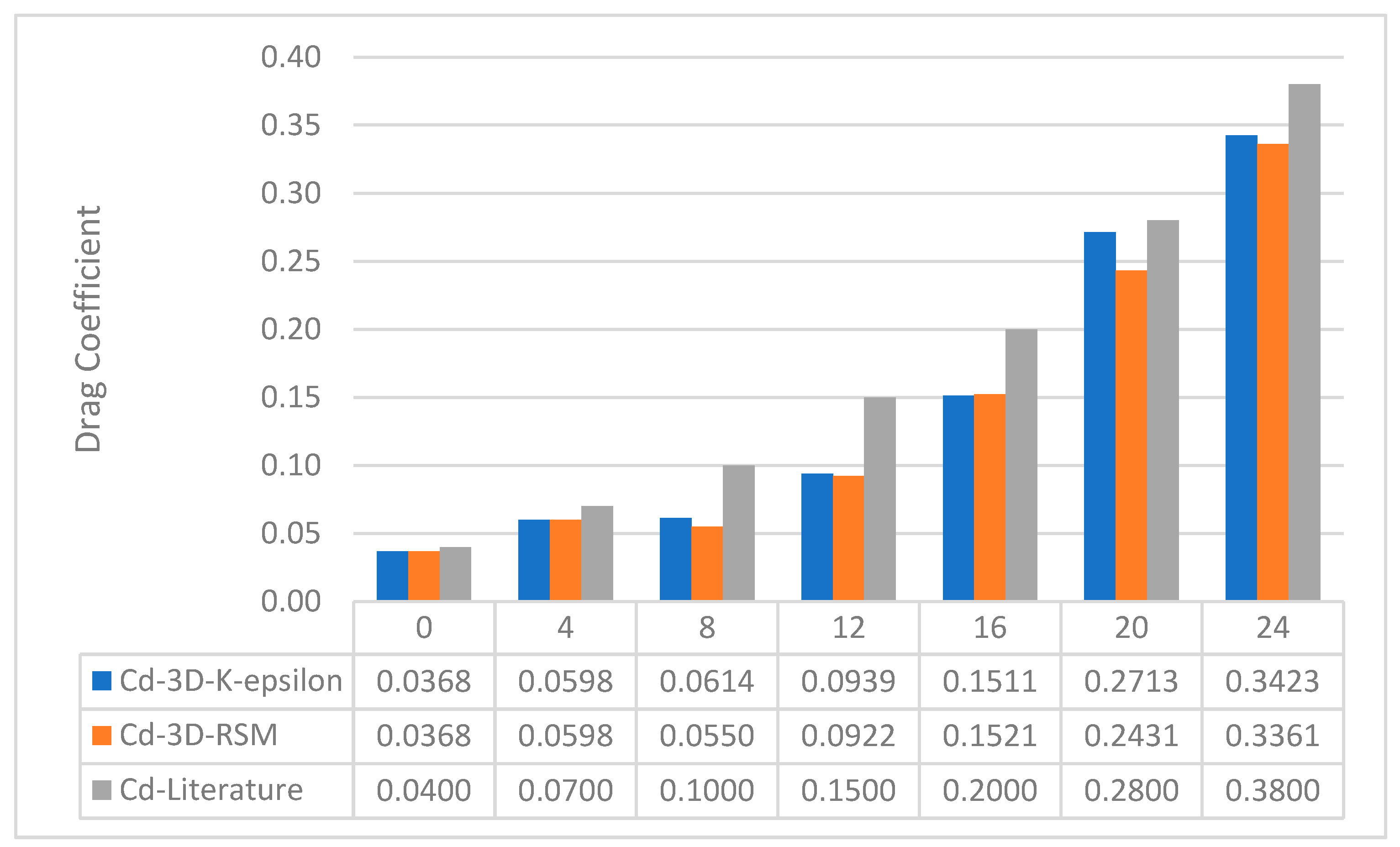
6.1. Method Validation
6.2. Mesh Validation
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Babikian, R.; Lukachko, S.P.; Waitz, I.A. The historical fuel efficiency characteristics of regional aircraft from technological, operational, and cost perspectives. J. Air Transp. Manag. 2002, 8, 389–400. [Google Scholar] [CrossRef]
- Kuhn, H.; Falter, C.; Sizmann, A. Renewable Energy Perspectives for Aviation [Preprint]. In Proceedings of the 3rd CEAS Air & Space Conference, Venice, Italy, 24–28 October 2011. [Google Scholar]
- Hendrickson, R.; Grumman; Roman, D.; Rajkovic, D. 5. Drag: An Introduction—Virginia Tech, 5. Drag: An Introduction. 1997; Available online: https://archive.aoe.vt.edu/mason/Mason_f/CAtxtChap5.pdf (accessed on 14 August 2023).
- Timmer, W.A.; Bak, C. Aerodynamic characteristics of wind turbine blade airfoils. In Advances in Wind Turbine Blade Design and Materials; Book Chapter; Elsevier: Amsterdam, The Netherlands, 2023; pp. 129–167. [Google Scholar] [CrossRef]
- Belligoli, Z.; Koers, A.J.; Dwight, R.P.; Eitelberg, G. Using an anti-fairing to reduce drag at wing/body junctions. AIAA J. 2019, 57, 1468–1480. [Google Scholar] [CrossRef]
- Beratlis, N.; Squires, K.D.; Balaras, E. Separation control and drag reduction using roughness elements. In Proceedings of the Tenth International Symposium on Turbulence and Shear Flow Phenomena, Chicago, IL, USA, 7–9 July 2017. [Google Scholar] [CrossRef]
- Rajasai, B.; Tej, R.; Srinath, S. Aerodynamic effects of dimples on Aircraft Wings. In Proceedings of the Fourth International Conference on Advances in Mechanical, Aeronautical and Production Techniques—MAPT, Kuala Lumpur, Malaysia, 27 September 2015. [Google Scholar] [CrossRef]
- Reneaux, J. Overview on drag reduction technologies for civil transport aircraft. In Proceedings of the European Congress on Computational Methods in Applied Sciences and Engineering (ECCOMAS), Jyväskylä, Finland, 24–28 July 2004; Neittaanmäki, P., Rossi, T., Korotov, S., Oñate, E., Périaux, J., Knörzer, D., Eds.; [Google Scholar]
- Merryisha, S.; Rajendran, P. Experimental and CFD Analysis of Surface Modifiers on Aircraft Wing: A Review. CFD Lett. 2019, 11, 46–56. [Google Scholar]
- Zhou, Y.; Wang, Z.J. Effects of surface roughness on separated and transitional flows over a wing. AIAA J. 2012, 50, 593–609. [Google Scholar] [CrossRef]
- Hövelmann, A.; Knoth, F.; Breitsamter, C. AVT-183 diamond wing flow field characteristics part 1: Varying leading-edge roughness and the effects on flow separation onset. Aerosp. Sci. Technol. 2016, 57, 18–30. [Google Scholar] [CrossRef]
- Kurz, H.B.E.; Kloker, M.J. Discrete-roughness effects in a three-dimensional boundary layer on an airfoil by means of DNS. Procedia IUTAM 2015, 14, 163–172. [Google Scholar] [CrossRef]
- Bari, A.A.; Mashud, M.; Ali, H. Role of partially bumpy surface to control the flow separation of an airfoil. ARPN J. Eng. Appl. Sci. 2012, 7, 584–587. [Google Scholar]
- Lang, A.; Habegger, M.L.; Motta, P. Shark skin drag reduction. In Encyclopedia of Nanotechnology; Springer: Dordrecht, The Netherlands, 2016; pp. 3632–3639. [Google Scholar] [CrossRef]
- Marimuthu, S.; Chinnathambi, D. Computational analysis of biomimetic butterfly valve. Bioinspired Biomim. Nanobiomater. 2020, 9, 223–232. [Google Scholar] [CrossRef]
- Marimuthu, S.; Chinnathambi, D. Computational analysis to enhance the compressible flow over an aerofoil surface. Aircr. Eng. Aerosp. Technol. 2021, 93, 925–934. [Google Scholar] [CrossRef]
- Marimuthu, S.; Al-Rabeei, S.; Boha, H.A. Three-dimensional analysis of biomimetic aerofoil in transonic flow. Biomimetics 2022, 7, 20. [Google Scholar] [CrossRef]
- Marimuthu, S.; Natarajan, M.; Ramesh, R.; Murugesan, R. Hollow three-dimensional model for fuel reduction in aviation industry. Int. J. Interact. Des. Manuf. 2022, 1–11. [Google Scholar] [CrossRef]
- Marimuthu, S.; Murugan, M.A.; Sivasathya, U.; Dharmalingham, S. Biomimetic in Turbulence reduction-Recent Developments. J. Appl. Sci. Res. 2015, 11, 123–134. Available online: https://ssrn.com/abstract=3196017 (accessed on 22 August 2023).
- Fraser, G.J. Shark Study Reveals Taste Buds Were Key to Evolution of Teeth, The Conversation. 2022. Available online: https://theconversation.com/shark-study-reveals-taste-buds-were-key-to-evolution-of-teeth-71376 (accessed on 3 November 2023).
- Kumaar, R.K.; Maniiarasan, P. Reduction of skin friction drag in wings by employing riblets. Int. J. Eng. Res. Technol. 2015, 4, 46–51. [Google Scholar] [CrossRef]
- Bixler, G.D.; Bhushan, B. Biofouling: Lessons from nature. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2012, 370, 2381–2417. [Google Scholar] [CrossRef]
- Tullis, S.; Pollard, A. Modelling the time dependent flow over riblets in the Viscous Wall Region. Appl. Sci. Res. 1993, 50, 299–314. [Google Scholar] [CrossRef]
- Ives, R.; Keir, S.; Bassey, E.; Hamad, F.A. Investigation of the flow around an aircraft wing of section NACA 2412 utilising ANSYS fluent. INCAS Bull. 2018, 10, 95–104. [Google Scholar] [CrossRef]
- Jacobs, E.N.; Ward, K.E.; Pinkerton, R.M. The Characteristics of 78 Related Aerofoil Sections from Tests in the Variable-Density Wind Tunnel. In Report National Advisory Committee for Aeronautics; National Advisory Committee for Aeronautics: Washington, DC, USA, 1933; pp. 299–354. [Google Scholar]
- Mukesh, M.; Arun, M.D.; Divyasharada, N.S.; Prakash, K.; Ram Rakshith, V. Experimental and Numerical Analysis of Rectangular, Tapered and Tapered Swept Back wings. Int. Res. J. Eng. Technol. 2019, 6, 7245–7348. [Google Scholar]
- Chakroun, W.; Al-Mesri, I.; Al-Fahad, S. Effect of surface roughness on the aerodynamic characteristics of a symmetrical aerofoil. Wind Eng. 2004, 28, 547–564. [Google Scholar] [CrossRef]
- Heidarian, A.; Ghassemi, H.; Liu, P. Numerical Analysis of the effects of riblets on drag reduction of a flat plate. J. Appl. Fluid Mech. 2018, 11, 679–688. [Google Scholar] [CrossRef]
- Zhang, Y.; Yan, C.; Chen, H.; Yin, Y. Study of riblet drag reduction for an infinite span wing with different sweep angles. Chin. J. Aeronaut. 2020, 33, 3125–3137. [Google Scholar] [CrossRef]
- Soleimani, S.; Eckels, S. A review of drag reduction and heat transfer enhancement by Riblet surfaces in closed and open channel flow. Int. J. Thermofluids 2021, 9, 100053. [Google Scholar] [CrossRef]
- Sijal. [Ansys Meshing] c or O Domain for Aerofoil Analysis? CFDonline.com. 2012. Available online: https://www.cfd-online.com/Forums/ansys-meshing/108595-c-o-domain-aerofoil-analysis.html (accessed on 16 August 2023).
- LEAP CFD Team. Tips & Tricks: Size Controls in Ansys, Computational Fluid Dynamics CFD Blog LEAP Australia New Zealand. 2020. Available online: https://www.computationalfluiddynamics.com.au/size-controls/ (accessed on 21 August 2023).
- Sosnowski, M.; Krzywanski, J.; Grabowska, K.; Gnatowska, R. Polyhedral meshing in numerical analysis of conjugate heat transfer. EPJ Web Conf. 2018, 180, 02096. [Google Scholar] [CrossRef]
- ANSYS. Near-Wall Mesh Guidelines, Ansys Fluent 12.0 Theory Guide—12.3.1 Near-Wall Mesh Guidelines. 2009. Available online: https://www.afs.enea.it/project/neptunius/docs/fluent/html/ug/node410.htm (accessed on 18 August 2023).
- ANSYS. Standard Wall Functions, Ansys Fluent 12.0 Theory Guide—4.12.2 Standard Wall Functions. 2009. Available online: https://www.afs.enea.it/project/neptunius/docs/fluent/html/th/node99.htm (accessed on 18 August 2023).
- Simscale. Mesh Quality, Simscale. 2022. Available online: https://www.simscale.com/docs/simulation-setup/meshing/mesh-quality/ (accessed on 18 August 2023).
- PRACE. Partnership for Advanced Computing in Europe, Express Introductory Training in ANSYS Fluent. 2012. Available online: https://events.prace-ri.eu/event/156/contributions/14/attachments/70/102/Fluent-Intro_14.5_WS01_Mixing_Tee.pdf (accessed on 18 August 2023).
- Andrade, S.d.S. Understanding and Applying Boundary Conditions in CFD: Diabatix, Heat Sink Generative Design Software. 2023. Available online: https://www.diabatix.com/blog/understanding-and-applying-boundary-conditions-in-cfd (accessed on 22 August 2023).
- Zawawi, M.H.; Saleha, A.; Salwa, A.; Hassan, N.H.; Zahari, N.M.; Ramli, M.Z.; Muda, Z.C. A review: Fundamentals of Computational Fluid Dynamics (CFD). AIP Conf. Proc. 2018, 2030, 020252. [Google Scholar] [CrossRef]
- Bihs, H. What Is Advantage of SIMPLE Algorithm? Cfdonline. 2010. Available online: https://www.cfd-online.com/Forums/main/76202-what-advantage-simple-algorithm.html (accessed on 21 August 2023).
- Telenta, M.; Šubelj, M.; Tavčar, J.; Duhovnik, J. Detached Eddy Simulation of the flow around a simplified vehicle sheltered by wind barrier in transient yaw crosswind. Mechanika 2015, 21, 193–200. [Google Scholar] [CrossRef]
- Goyal, S.; Kulshreshtha, A.; Singh, S. Selection of Turbulence Model for Analysis of Aerofoil Wing using CFD. Int. Res. J. Eng. Technol. (IRJET) 2021, 8, 2608–2614. [Google Scholar]
- Chaudhry, A.U. Fundamentals of Reservoir Oil Flow Analysis. In Oil Well Testing Handbook; Elsevier: Burlington, VT, USA, 2004; pp. 13–43. [Google Scholar] [CrossRef]
- LearnCAx. Basics of Y Plus Boundary Layer and Wall Function in Turbulent Flows, LearnCAx. 2022. Available online: https://www.learncax.com/knowledge-base/blog/by-category/cfd/basics-of-y-plus-boundary-layer-and-wall-function-in-turbulent-flows (accessed on 22 August 2023).
- SimScale. K-Epsilon Turbulence Model: Global Settings, SimScale. 2023. Available online: https://www.simscale.com/docs/simulation-setup/global-settings/k-epsilon/#:~:text=It%20is%20a%20two%2Dequation,and%20diffusion%20of%20turbulent%20energy (accessed on 4 October 2023).
- Crocker, M.J. Handbook of Acoustics; Wiley: New York, NY, USA, 1998. [Google Scholar]
- Lissaman, P.B.S. Low-Reynolds-Number Aerofoils [Preprint]; Annual Reviews: San Mateo, CA, USA, 1983. [Google Scholar]
- Saarlas, M. Aircraft Performance; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- SimScale, S.D. What Is Drag Coefficient?: SimWiki, SimScale. 2023. Available online: https://www.simscale.com/docs/simwiki/lift-drag-pitch/what-is-drag-coefficient/ (accessed on 2 May 2024).
- Konovalov, S.F.; Lashkov, Y.A.; Mikhailov, V.V. Effect of riblets on the drag of a body of revolution with trailing-edge flow separation. Fluid Dyn. 1998, 33, 135–139. [Google Scholar] [CrossRef]
- Geng, Z.; Cai, J.; Jiang, Y.; Zhang, W. On aircraft lift and drag reduction using V shaped riblets. Fluid Dyn. Mater. Process. 2021, 17, 899–915. [Google Scholar] [CrossRef]
- Lee, M.; Park, G.; Park, C.; Kim, C. Improvement of grid independence test for computational fluid dynamics model of building based on Grid Resolution. Adv. Civ. Eng. 2020, 2020, 8827936. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Selvanose, S.M.; Marimuthu, S.; Awan, A.W.; Daniel, K. NACA 2412 Drag Reduction Using V-Shaped Riblets. Eng 2024, 5, 944-957. https://doi.org/10.3390/eng5020051
Selvanose SM, Marimuthu S, Awan AW, Daniel K. NACA 2412 Drag Reduction Using V-Shaped Riblets. Eng. 2024; 5(2):944-957. https://doi.org/10.3390/eng5020051
Chicago/Turabian StyleSelvanose, Smitha Mol, Siva Marimuthu, Abdul Waheed Awan, and Kamran Daniel. 2024. "NACA 2412 Drag Reduction Using V-Shaped Riblets" Eng 5, no. 2: 944-957. https://doi.org/10.3390/eng5020051
APA StyleSelvanose, S. M., Marimuthu, S., Awan, A. W., & Daniel, K. (2024). NACA 2412 Drag Reduction Using V-Shaped Riblets. Eng, 5(2), 944-957. https://doi.org/10.3390/eng5020051






