Inertial Propulsion Devices: A Review
Abstract
1. Introduction
- Class 1: those that have been designed to generate external ratcheting reaction forces, usually (but not restrictively) opposed to the frictional environment.
- Class 2: those that have been designed to operate in a vacuum, in which reaction forces cannot be generated, but motion control is possible.
2. Ancient Times and Fictional References
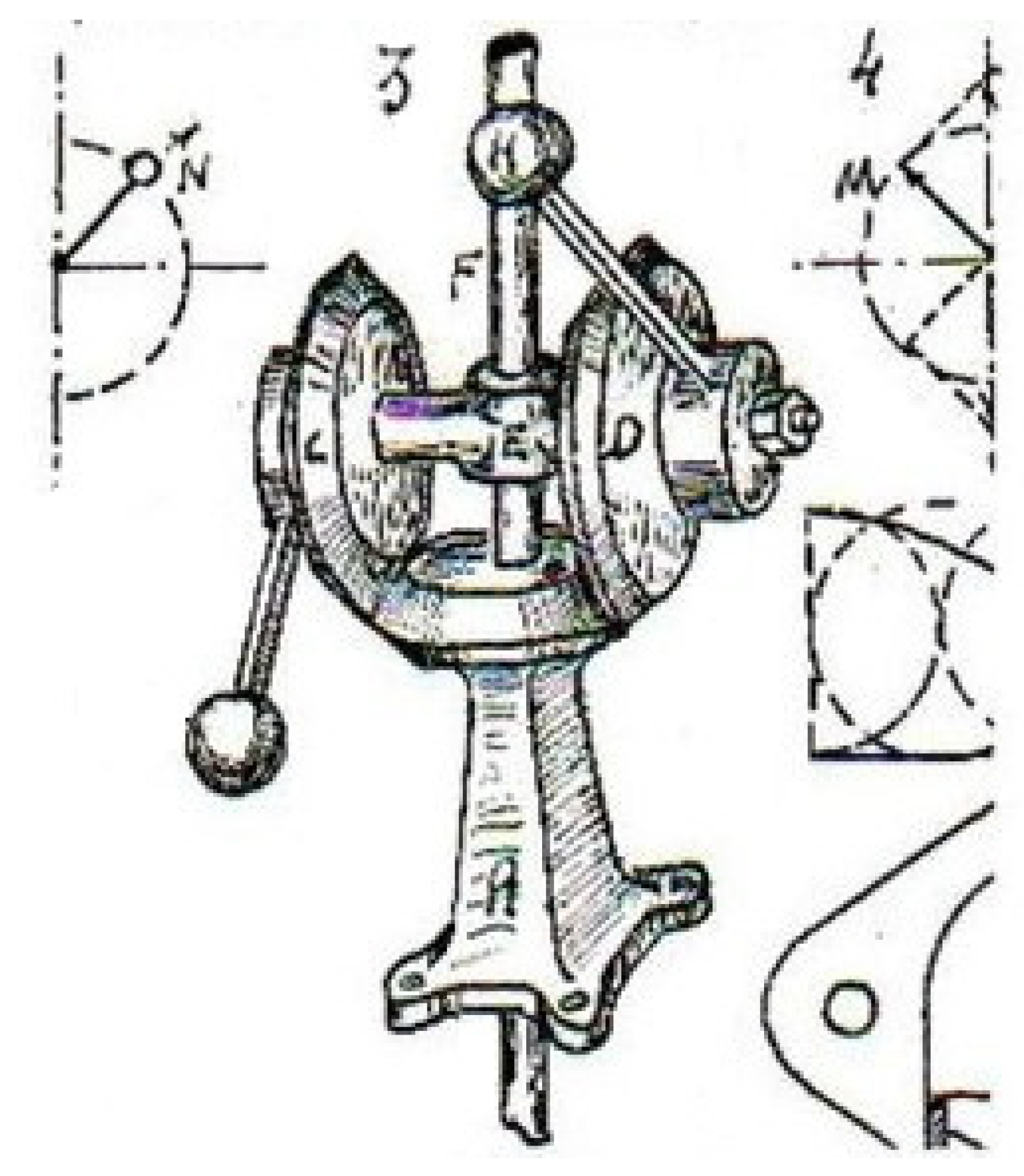
3. Inventions in the Twentieth Century
3.1. Rotating Masses
3.2. Gyroscopes and Spinning Wheels
“… the differential acceleration between a rotating mechanical gyroscope and a nonrotating one was directly measured by using a double free-fall interferometer, and no apparent differential acceleration has been observed at the relative level of 2 × 10−6. It means that the equivalence principle is still valid for rotating extended bodies, i.e., the spin-gravity interaction between the extended bodies has not been observed at this level. Also, to the limit of our experimental sensitivity, there is no observed asymmetrical effect or antigravity of the rotating gyroscopes as reported by Hayasaka et al. [48]”.
4. Progress in the Twenty-First Century
4.1. Theoretical Contributions
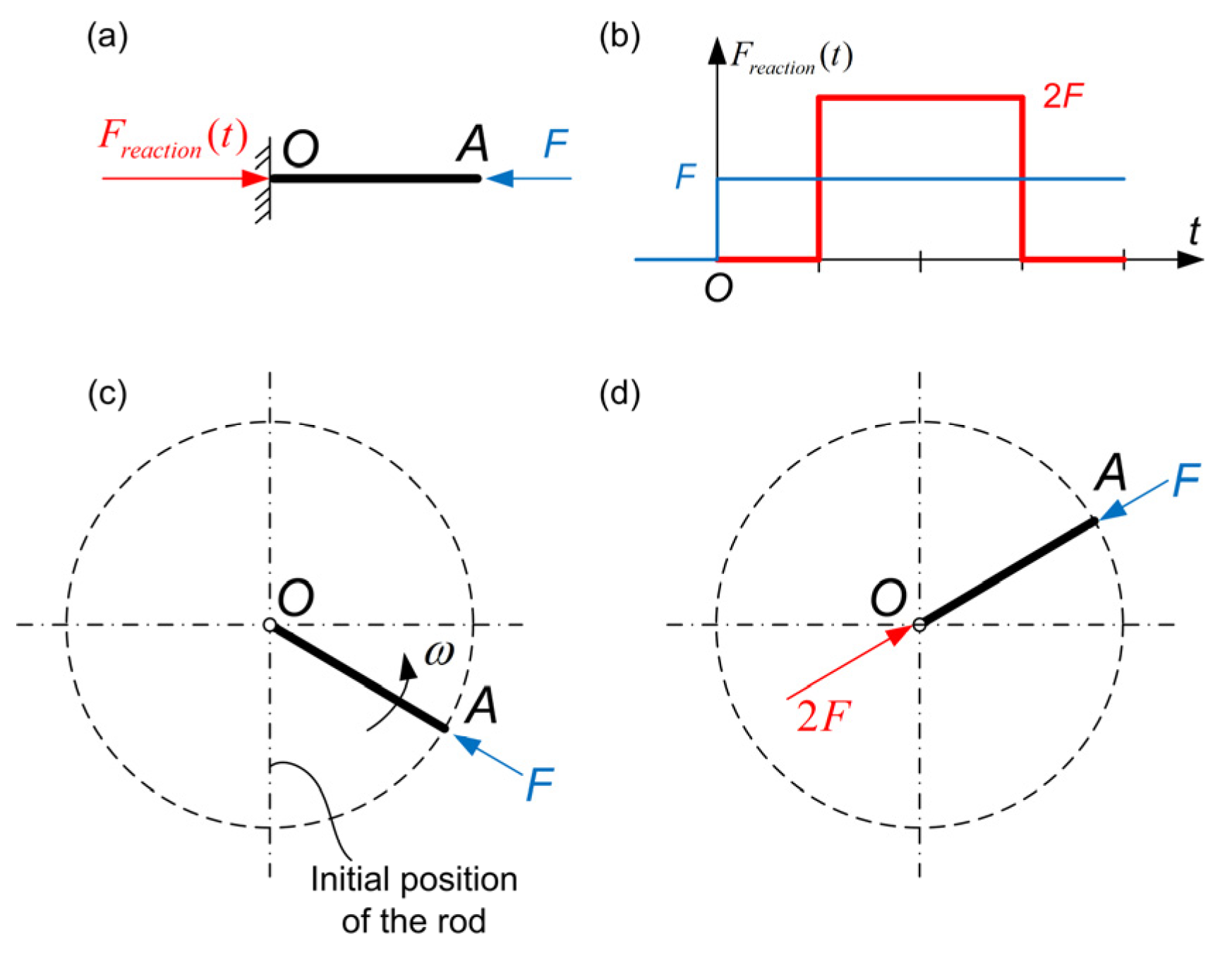
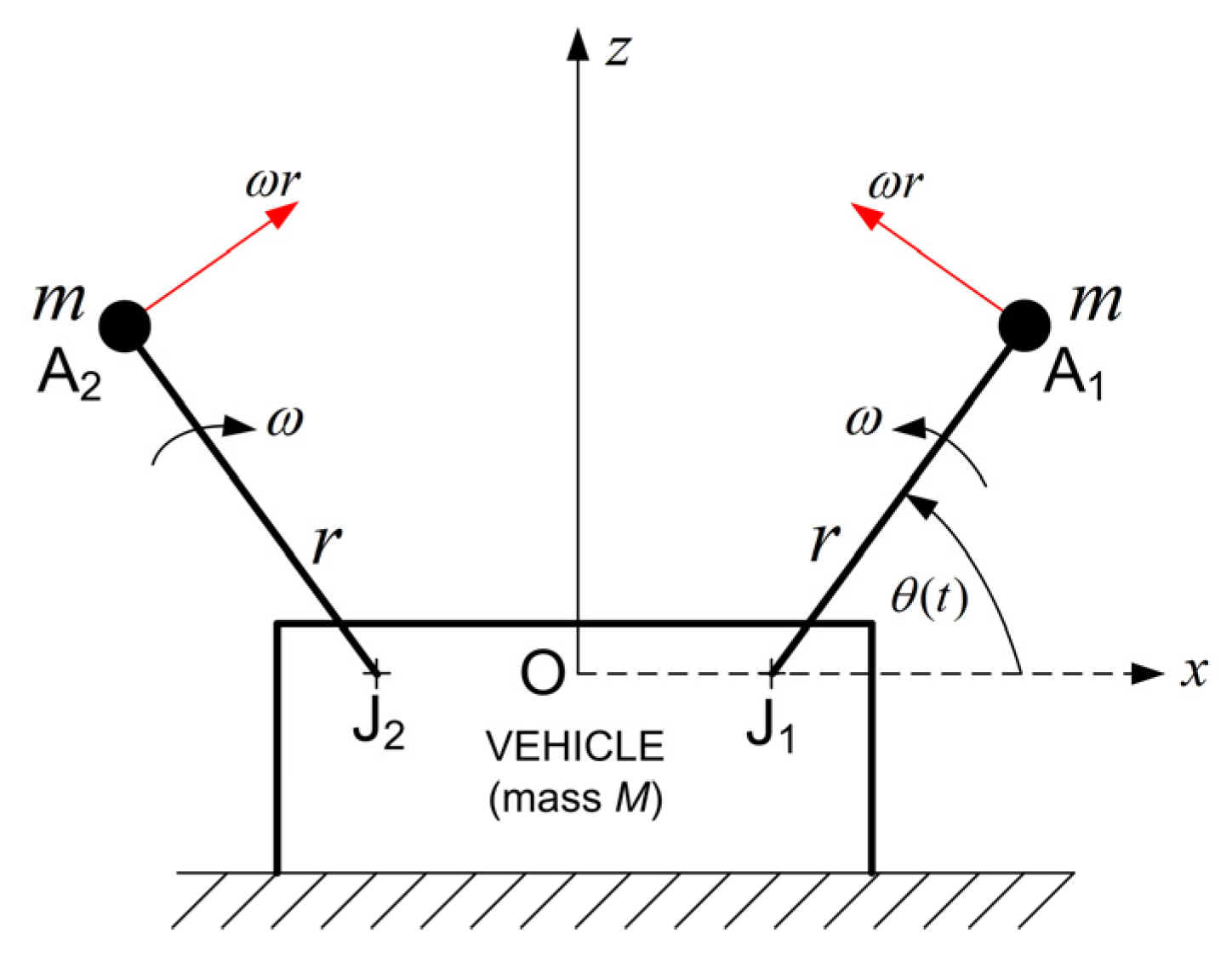
- An inertial drive attached to a vehicle or cart, which initially lies on the ground, causes alternating (sinusoidal) support forces on it. For an immobilized vehicle, the total linear momentum of the contra-rotating masses varies in time and its derivative with respect to time equals the vertical support force (ground reaction exerted on the vehicle or cart). For the continuous motion of the contra-rotating masses at a constant angular velocity , external energy is generally required to withstand the friction losses [96].
- When the magnitude of the constant angular velocity, , is adequately high, the vehicle (cart) can perform a vertical jump. This happens because in the upward motion of the rotating masses (i) the reaction force is higher than the weight, and (ii) the center of mass of the system (cart + rotating masses) has an adequately large initial velocity which allows for a vertical shot.
- An alternative explanation for the motion of the vehicle due to the attached inertial drive is as follows. In the beginning, the rotating masses of the inertial drive possess a certain linear momentum towards the vertical -axis. When the orientation of the connecting rods (radii of out-of-balance masses) becomes vertical, the velocity vectors of these masses become horizontal, and thus the linear momentum of the rotating masses vanishes. If, for example, the angular velocity is high, the change in linear momentum per revolution () will be a small percentage of the total initial value, and thus practically, the linear momentum of the system is preserved. Due to the said conservation of linear momentum in the vertical -axis, the lost momentum is undertaken by the vehicle. But after 90 degrees, the connecting rods will become horizontal with peak velocities, and thus the instantaneous velocity of the vehicle vanishes, and so on [97].
- Obviously, if no extra energy is transmitted to the inertial drive, the initial angular velocity of the rotating masses cannot be preserved at a constant value ; however, again, the vehicle can jump [97].
- The maximum height that the mechanical system “vehicle + drive” can reach depends on the initial velocity of the center of mass of this system.
- The initial velocity of the center of mass occurs when the two connecting rods to which the masses are attached are found on a horizontal position and at the same time the ground suddenly opens like the cover of a well. Then, the conservation of the linear momentum toward the vertical axis is practically ensured (almost closed system by ignoring the gravitational effect) [97]. Again, we recall that while the vehicle stands on the ground the linear momentum is not preserved, because the system is open.
- Depending on the level of the initial velocity at the zero level, the vehicle may elevate following an oscillating mode, with the rods usually having performed many revolutions, until the center of mass takes a zero value (due to the gravitation). Then, the vehicle starts falling, following an oscillating mode, until it returns to the zero level, having its initial velocity but in the opposite direction.
- During an extremely short time interval, it is possible to keep the vehicle immobile in the air by varying the angular velocity according to Equation (A8), shown in Appendix A. Unfortunately, this phase ends when the rods which carry the rotating masses become vertical, and thus the denominator of the closed-form expression (A8) vanishes and the fraction becomes infinite [96].
- In some sense, the sinusoidal support forces are very similar to those exerted on the ground by a spring-mass system [99]. To better understand this issue, note that when the topic of oscillations is presented in high-schools or colleges, teachers say that oscillation is the projection of a moving material point on a circle determined by the extreme positions of that oscillation.
- Overall, the center of mass of the system performs a vertical shot, but an oblique shot is also possible [98].
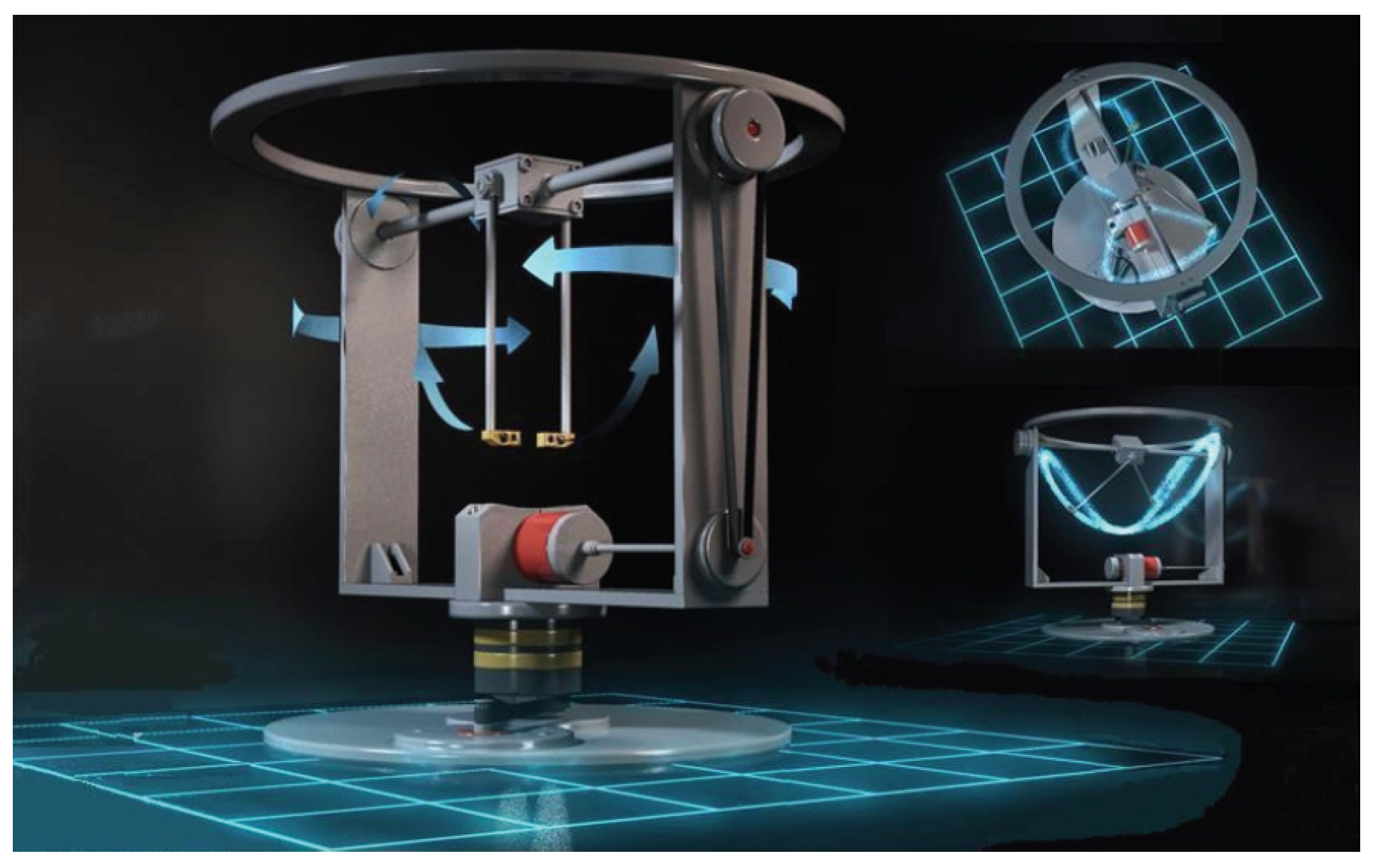
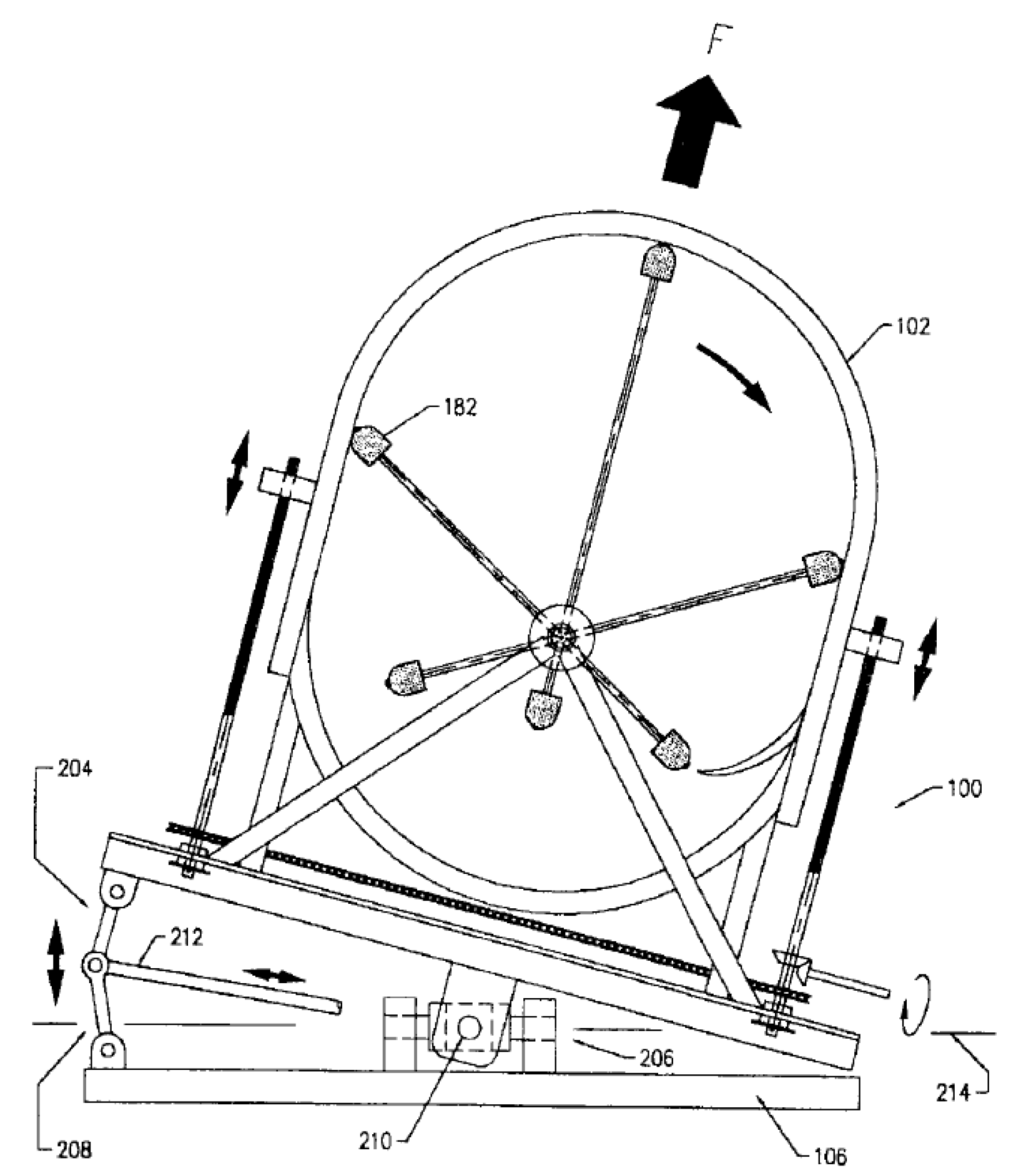
4.2. Practical Applications
4.3. Other Patents and Broadcasts
5. A Critical Note on the Mechanics Involved in Inertial Drives
6. Discussion
7. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. The Mechanics of Contra-Rotating Masses on the Vertical Plane
- Application of Newton’s second and third laws on the five involved rigid bodies in the powertrain (two masses, each of them , two massless beams, and the cart of mass ).
- Application of Newton’s second law to the center of mass of the system.
- Conservation of Linear Momentum.
- Lagrange equations (analytical mechanics).
- The maximum upward travel length of the mass into vacuum is highly influenced by the second term in Equation (A4), and thus is achieved when the connecting beams are horizontally oriented () and at the same time the ground suddenly disappears. For example, this may happen when the cart is standing above a deep well, and then the two halves of the cover open rapidly [96,97,98].
- It is possible to control the angular velocity so that the cart remains immobilized into a vacuum for a while, but this can be undertaken only for almost half a rotation (less than 180 degrees) bounded by the vertical axis through the middle of the cart [96].
- An analogous instantaneous immobilization of the cart into vacuum, between two saddle points, occurs for rotating masses moving along a figure-eight-shaped path [111].
- As a virtual exaggerated example, in the very hypothetical case in which two hydrogen atoms had nuclei in contact and the two associated electrons were moving in synchronization (contra-rotating) according to the Rutherford–Bohr’s model for the harmonic , the maximum height at which the hydrogen compound could fly when it was left free to move would be about 72 km [98].
Appendix B. Repeated Vibrational Motion
Appendix C. Contra-Rotating Wipers
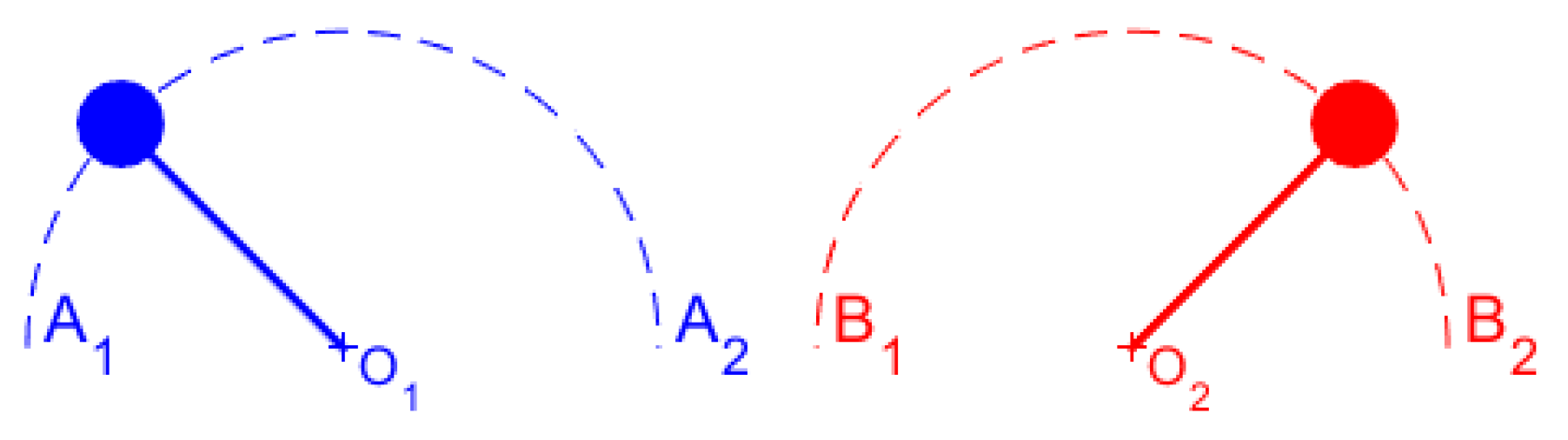
Appendix D. Radial Displacement of a Satellite
References
- Potier, O.N.R. The cancellation of gravity. Electron. Power 1965, 11, 111. [Google Scholar] [CrossRef]
- Farrall, A.W. An inertial propulsion system. Trans. ASAE 1966, 9, 586–587. [Google Scholar] [CrossRef]
- Hancock, R. (Ed.) Entry: “PROPULSION inertial”. In The Illustrated Encyclopedia of Science and Technology: HOW IT WORKS; Marshall Cavendish Ltd.: New York, NY, USA; London, UK, 1977; Volume 14, pp. 1874–1877. Available online: https://archive.org/details/howitworksillust14ralp/page/1874/mode/2up?q=inertial (accessed on 23 January 2024).
- Walgate, R. Eric Laithwaite defies Newton. New Sci. 1974, 14, 470. [Google Scholar]
- Engel, A.B.; Stiebitz, P.H. The cybernetics of inertial propulsion. Kybernetes 2009, 38, 141–157. [Google Scholar] [CrossRef]
- Fiala, H.E.; Fiala, J.E.; Fiala, J.-A. Inertial Propulsion Device to Move an Object Up and Down. U.S. Patent 8.066,226 B2, 29 November 2011. [Google Scholar]
- Goncharevich, I.F. Dynamics of Vibrational Transportation; Nauka: Moscow, Russia, 1972; p. 244. (In Russian) [Google Scholar]
- Blekhman, I.I. Vibrational Mechanics: Nonlinear Dynamic Effects, General Approach, Applications; World Scientific: Singapore, 2000; pp. 15+19. [Google Scholar]
- Kononenko, V.O. Vibrating Systems with a Limited Power Supply; Iliffe Books Ltd.: London, UK, 1969; p. 24. (In English) [Google Scholar]
- Blekhman, I.I. Synchronization in Science and Technology; ASME Press: New York, NY, USA, 1988; (In English, translated from Russian 1981). [Google Scholar]
- Majewski, T. Vibratory forces and synchronization in physical systems. Ing. Mecánica Tecnol. Desarro. 2013, 4, 119–128. [Google Scholar]
- Chen, L.; Pei, Z.; Tang, Z. Maximum power control of gyroscopic wave energy converter. E3S Web Conf. 2019, 118, 2022. [Google Scholar] [CrossRef]
- Townsend, N.; Shenoi, A. A gyroscopic wave energy recovery system for marine vessels. IEEE J. Ocean. Eng. 2012, 37, 271–280. [Google Scholar] [CrossRef]
- Tajmar, M. Advanced Space Propulsion Systems; Springer: Wien, NY, USA, 2002. [Google Scholar]
- Bruno, C.; Accettura, A.G. (Eds.) Advanced Propulsion Systems and Technologies: Today to 2020; Volume 223 Progress in Astronautics and Aeronautics; American Institute of Aeronautics and Astronautics: Reston, VI, USA, 2008. [Google Scholar]
- Millis, M.G.; Thomas, N.E. Responding to Mechanical Antigravity, NASA/TM-2006-214390, AIAA-2006-4913, December 2006. Available online: http://gltrs.grc.nasa.gov/reports/2006/TM-2006-214390.pdf (accessed on 23 January 2024).
- ElSheimy, N.; Youssef, A. Inertial sensors technologies for navigation applications: State of the art and future trends. Satell. Navig. 2020, 1, 2. [Google Scholar] [CrossRef]
- Lawerance, A. Modern Inertial Technologies: Navigation, Guidance and Control; Springer: New York, NY, USA, 1992. [Google Scholar]
- Rimrott, F.P.J. Introductory Attitude Dynamics; Springer: New York, NY, USA, 1989. [Google Scholar]
- Minetti, A.E.; Ardigó, L.P. Halteres used in ancient Olympic long jump. Nature 2002, 420, 141–142. [Google Scholar] [CrossRef]
- Provatidis, C.G. Simplified biomechanics for a possible explanation of the ancient Greek long jump using halteres. Univers. J. Eng. Sci. 2013, 1, 5–16. [Google Scholar] [CrossRef]
- Provatidis, C.G. On the maximum length of standing long jump using halters. Int. J. Multidiscip. Res. Stud. 2018, 1, 153–169. [Google Scholar]
- Citizendium. Available online: http://en.citizendium.org/wiki/Reactionless_propulsion (accessed on 23 January 2024).
- Todeschini, M. Motore a Forza Propulsive Centrifuga Alimentato ad Acqua Dissociate con Variazione di Velocita Graduale Automatic. Regno d’Italia, Ministero Delle Corporazioni, Brevetto Industriale No. 312496. 17 November 1933; See the First Two Pages of the Patent at the Website. Available online: www.circolotodeschini.com (accessed on 23 January 2024).
- Dean, N.L. System for Converting Rotary Motion into Unidirectional Motion. U.S. Patent 2,886,976, 19 May 1959. [Google Scholar]
- Dean, N.L. Variable Oscillator System. U.S. Patent 3,182,517, 11 May 1965. [Google Scholar]
- Campbell, J., Jr. The space drive problem. Astounding Sci. Fact Fict. 1960, 98, 83–106. [Google Scholar]
- Dempewolff, R.F. Engine with built-in wings. Pop. Mech. 1961, 116, 131–134, 264–266. [Google Scholar]
- Burridge, G. Toward antigravity. Rosicrucian Dig. 1962, XL, 136–139. [Google Scholar]
- Davis, W.O. The fourth law of motion. Analog 1962, 69, 83–104. Available online: http://www.rexresearch.com/dean/davis4.htm (accessed on 23 January 2024).
- Stine, G.H. Detesters, Phasers and Dean drives. Analog 1976, 96, 60–80. [Google Scholar]
- Campbell, J.W. (1962)-Interview by Fred Lerner. Available online: https://www.youtube.com/watch?v=SC-o9z-E010&ab_channel=FANACFanHistory (accessed on 23 January 2024).
- Stepanov, G.Y. Why is it impossible to have ‘Dean’s apparatus’? J. Priroda 1963, 7, 85–91. (In Russian) [Google Scholar]
- Di Bella, A. Apparatus for Imparting Motion to a Body. U.S. Patent 3,404,854, 8 October 1968. [Google Scholar]
- Di Bella, A. On propulsive effects of a rotating mass. In Proceedings of the Seventh Symposium Naval Hydrodynamics, Rome, Italy, 25–30 August 1968; Office of Naval Research-Department of The Navy, Arlington, VI, USA. Cooper, R.D., Doroff, S.W., Eds.; pp. 1373–1396. [Google Scholar]
- Available online: https://en.wikipedia.org/wiki/Viviani%27s_curve (accessed on 23 January 2024).
- Therriault, C. Wing Movement for Ornithopters and Apparatus of the Like. U.S. Patent 6,227,483 B1, 8 May 2001. [Google Scholar]
- Kuchinov, E. Quesalid’s Knots: A Guide to the Soviet Union’s Esoteric Research Institutes. Available online: https://sreda.v-a-c.org/en/read-01 (accessed on 23 January 2024).
- Tolchin, V.N. Sily Inertsii Kak Istochnik Postupatel’nogo Dvizheniia; Translation: Forces of Inertia as a Source of Translational Motion; Permskoe Knizhnoe Izdatel’stvo: Perm, Russia, 1977. [Google Scholar]
- Tolchin Drive-Short Original Film Copy. Available online: https://www.youtube.com/watch?v=DOXoaYs790M&ab_channel=stclairtechrd (accessed on 23 January 2024).
- Laithwaite, E.R. Propulsion Without Wheels, 2nd ed.; English Universities Press: London, UK, 1970. [Google Scholar]
- Wikipedia. Available online: https://en.wikipedia.org/wiki/Eric_Laithwaite (accessed on 23 January 2024).
- Laithwaite, E.R. The Engineer through the Looking Glass|The Royal Institution: Science Lives Here. Available online: https://www.rigb.org/explore-science/explore/video/engineer-through-looking-glass-looking-glass-house-1974 (accessed on 7 January 2024).
- Bruce De Palma. Available online: https://en-academic.com/dic.nsf/enwiki/1876830 (accessed on 23 January 2024).
- DePalma, B. The Tuning Fork Experiment, In: R.L. Dione, 1976 (ISBN 553-02723-150). Available online: http://www.brucedepalma.com/n-machine/spinning-ball-experiment/ (accessed on 23 January 2024).
- Available online: http://rexresearch.com/depalma2/depalm.htm (accessed on 23 January 2024).
- Available online: https://depalma.pairsite.com/gyrodrop.html (accessed on 23 January 2024).
- Hayasaka, H.; Takeuchi, S. Anomalous weight reduction on a gyroscope’s right rotations around the vertical axis on the earth. Phys. Rev. Lett. 1989, 63, 2701–2704. [Google Scholar] [CrossRef] [PubMed]
- Adelberger, E.G. Science correspondence on paper by Hayasaka & Takeuchi. Nature 1990, 345, 121. [Google Scholar]
- Faller, J.E.; Hollander, W.J.; Nelson, P.G.; McHugh, M.P. Gyroscope-weighing experiment with a null result. Phys. Rev. Lett. 1990, 64, 825–826. [Google Scholar] [CrossRef]
- MacCallum, M. Does a spinning mass really lose weight? New Sci. 1990, 1704, 30. [Google Scholar]
- Nitschke, J.M.; Wilmarth, P.A. Null result for the weight change of a spinning gyroscope. Phys. Rev. Lett. 1990, 64, 2115–2116. [Google Scholar] [CrossRef] [PubMed]
- Quinn, T.J.; Picard, A. The mass of spinning rotors: No dependence on speed or sense of rotation. Nature 1990, 343, 732–735. [Google Scholar] [CrossRef]
- Salter, S.H. Good vibrations for physics. Nature 1990, 343, 509–510. [Google Scholar] [CrossRef]
- Imanishi, A.; Maruyama, K.; Midorikawa, S.; Morimoto, T. Observation against the weight-reduction of spinning gyroscopes. J. Phys. Soc. Jpn. 1991, 60, 1150–1152. [Google Scholar] [CrossRef]
- Hayasaka, H.; Tanaka, H.; Hashida, T.; Chubachi, T.; Sugiyama, T. Possibility for the existence of anti-gravity: Evidence from a free-fall experiment using a spinning gyro. Specul. Sci. Technol. 1997, 20, 173–181. [Google Scholar]
- Luo, J.; Nie, Y.X.; Zhang, Y.Z.; Zhou, Z.B. Null result for violation of the equivalence principle with the free-fall rotating gyroscopes. Phys. Rev. D 2002, 65, 042005. [Google Scholar] [CrossRef]
- Labeysh, V.G. Experiments on asymmetrical mechanics. Galilean Electrodyn. 2000, 11, 8–11. [Google Scholar]
- Dmitriev, A.L.; Snegov, V.S. Measurements of mass: The weighing of a mechanical gyroscope with horizontal and vertical orientation of the spin axis. Meas. Tech. 2001, 44, 831–833. [Google Scholar] [CrossRef]
- Dmitriev, A.L. Frequency dependence of rotor’s free falling acceleration and inequality of inertial and gravity masses. arXiv 2011, arXiv:1101.4678. [Google Scholar]
- Dmitriev, A.L. Physical substantiation of an opportunity of artificial change of body weight. Phys. Procedia 2012, 38, 150–163. [Google Scholar] [CrossRef][Green Version]
- Dmitriev, A.L. Prospects of high-frequency gravimetry. Int. J. Circuits Syst. Signal Process. 2015, 9, 275–280. Available online: https://www.inase.org/library/2015/vienna/bypaper/APNE/APNE-37.pdf (accessed on 23 January 2024).
- Provatidis, C.G. Free fall of a symmetrical gyroscope in vacuum. Eur. J. Phys. 2021, 42, 065011. [Google Scholar] [CrossRef]
- Wayte, R. The phenomenon of weight-reduction of a spinning wheel. Meccanica 2007, 42, 359–364. [Google Scholar] [CrossRef]
- Lőrincz, I.; Tajmar, M. Identification of error sources in high precision weight measurements of gyroscopes. Measurement 2015, 73, 453–461. [Google Scholar] [CrossRef]
- Provatidis, C.G. Forced precession in a spinning wheel supported on a rotating pivot. Mech. Res. Commun. 2013, 52, 46–51. [Google Scholar] [CrossRef]
- Provatidis, C.G. Forced precession of a gyroscope with application to the Laithwaite’s engine. Eur. J. Phys. 2021, 42, 035003. [Google Scholar] [CrossRef]
- Allen, J.E. Quest for a novel force: A possible revolution in aerospace. Prog. Aerosp. Sci. 2003, 39, 1–60. [Google Scholar] [CrossRef]
- Meek, J. Bae’s Anti-Gravity Research Braves X-Files Ridicule. The Guardian, Mon 27 Mar 2000. Available online: https://www.theguardian.com/science/2000/mar/27/uknews (accessed on 23 January 2024).
- Anonymous. Project Greenglow and the Battle with Gravity. News, 23 March 2016. Available online: https://www.bbc.com/news/magazine-35861334 (accessed on 23 January 2024).
- Interview from Ron Evans. Available online: https://www.youtube.com/watch?v=BwI7Ij-5cMA&ab_channel=TimVentura (accessed on 23 January 2024).
- Anonymous. Science Does the Impossible: February 2003 Cover Story. Popular Mechanics. Available online: https://archive.ph/20070930015205/http://www.popularmechanics.com/science/research/1282281.html?page=3 (accessed on 23 January 2024).
- Anonymous. Boeing Internal Report Looks at Propellentless Propulsion. Available online: https://www.spacedaily.com/news/rocketscience-02t.html (accessed on 23 January 2024).
- BBC-News, Boeing Tries to Defy Gravity. Monday. 29 July 2002. Available online: http://news.bbc.co.uk/2/hi/science/nature/2157975.stm (accessed on 23 January 2024).
- Wikipedia: Eugene Podkletnov. Available online: https://en.wikipedia.org/wiki/Eugene_Podkletnov#cite_note-8 (accessed on 23 January 2024).
- Millis, M.G. Breakthrough Propulsion Physics Research Program. AIP Conf. Proc. 1997, 387, 1297–1302. [Google Scholar]
- Millis, M.G. NASA breakthrough propulsion physics program. Acta Astronaut. 1999, 44, 175–182. [Google Scholar] [CrossRef]
- Millis, M.G. Assessing potential propulsion breakthroughs. Annu. N. Y. Acad. Sci. 2005, 1065, 441–461. [Google Scholar] [CrossRef] [PubMed]
- Millis, M.G.; Davis, E.W. Frontiers of Propulsion Science; American Institute of Aeronautics and Astronautics Inc.: Reston, VA, USA, 2009. [Google Scholar]
- Millis, M.G. Progress in revolutionary propulsion physics, Paper IAC-10-C4.8.7. In Proceedings of the 61st International Astronautical Congress, Prague, Czech Republic, 27 September–1 October 2010. [Google Scholar]
- Robertson, D.B. Propulsion Method and Apparatus Utilizing Centrifugal Force; Number WO 01/46584 A3; World Intellectual Property Organization (WIPO): Karlsruhe, Germany, 2001. [Google Scholar]
- Hampton, S.M. From Dean Drives and Davis Mechanics: Inertial Propulsion and the Manipulation of Time in Symmetrical Systems©; Ebook; Apple Communications: London Mills, IL, USA, 2011. [Google Scholar]
- Hampton, S.M. Asymmetric Impulse Drive. AIAA 2022-4391. ASCEND 2022 (24–26 October 2022, Las Vegas, Nevada & Online). Session. In Frontiers in Deep Space Propulsion; View Video Presentation. Available online: https://video.aiaa.org/title/b3dbbfb4-564b-4cb4-bbd3-8965c3a40be4 (accessed on 23 January 2024).
- Tsiriggakis, V.T.; Provatidis, C.G.; Frigas, S. U.S. Patent Application for an “Antigravity Mechanism”. U.S. Patent 61/110,307, 31 October 2008. [Google Scholar]
- Provatidis, C.G.; Tsiriggakis, V.T. A new concept and design aspects of an ‘antigravity’ propulsion mechanism based on inertial forces. In Proceedings of the 46th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Nashville, TN, USA, 25–28 July 2010. Paper AIAA 2010-6684. [Google Scholar]
- Provatidis, C.G. A device that can produce net impulse using rotating masses. Engineering 2020, 2, 648–657. [Google Scholar] [CrossRef][Green Version]
- Pipes, L.A.; Harville, L.R. Applied Mathematics for Engineers and Physicists, 3rd ed.; McGraw-Hill: New York, NY, USA, 1981; pp. 494–496. [Google Scholar]
- Provatidis, C.G.; Gamble, M.A. Support forces in a synchronized rotating spring-mass system and its electromagnetic equivalent. Int. J. Appl. Electromagn. Mech. 2013, 41, 313–333. [Google Scholar] [CrossRef]
- Manning, J. Space, Propulsion & Energy Sciences International Forum-A Journalist’s Notes. Infin. Energy 2012, 103, 18–23. [Google Scholar]
- Valone, T. Inertial Propulsion: Concept and experiment, Part I. In Proceedings of the Intersociety Energy Conversion Engineering Conference, Monterey, CA, USA, 7–12 August 1994. [Google Scholar]
- Valone, T. Inertial Propulsion: Concept and experiment, Part II. In Proceedings of the Intersociety Energy Conversion Engineering Conference, Monterey, CA, USA, 7–12 August 1994; AIAA-94-4167-CP. pp. 1484–1489. [Google Scholar] [CrossRef]
- Valone, T.; Cox, J. Inertial Propulsion: Patent Collection; Integrity Research Institute: Washington, DC, USA, 2008. [Google Scholar]
- Valone, T.F. Eric Laithwaite Report: Gyromagnetic Engineering Genius–Publications, Inventions & News Clips; IRI PR-02-005; Integrity Research Institute: Beltsville, MD, USA, 2006. [Google Scholar]
- Almesallmy, M. Experimental and Analytical Investigation of Inertial Propulsion Mechanisms and Motion Simulation of Rigid-Multi-Body Mechanical Systems. Ph.D. Thesis, University of Rhode Island, Kingston, RI, USA, 2006. [Google Scholar]
- Guiad, D.; Nilvetti, F.; Pappalardo, C.M. Dry friction influence on cart pendulum dynamics. Int. J. Mech. 2009, 3, 31–38. [Google Scholar]
- Provatidis, C.G. Some issues on inertia propulsion mechanisms using two contra-rotating masses. Theory Mech. Mach. 2010, 8, 34–41. Available online: http://tmm.spbstu.ru/15/Provatidis_15.pdf (accessed on 23 January 2024).
- Provatidis, C.G. A study of the mechanics of an oscillating mechanism. Int. J. Mech. 2011, 5, 263–274. Available online: https://www.naun.org/main/NAUN/mechanics/17-093.pdf (accessed on 23 January 2024).
- Provatidis, C.G. An overview of the mechanics of oscillating mechanisms. Am. J. Mech. Eng. 2013, 1, 58–65. [Google Scholar] [CrossRef][Green Version]
- Provatidis, C.G. Repeated vibrational motion using an inertial drive. Vib. Acoust. Res. J. 2019, 1, 27–43. Available online: https://www.cansrg.com/journals/varj/2019/70.pdf (accessed on 23 January 2024).
- Provatidis, C.G. On the inertial propulsion of floating objects using contra-rotating masses. Mech. Res. Commun. 2014, 62, 117–122. [Google Scholar] [CrossRef]
- Ikuta, K.; Aritomi, S.; Kabashima, T. Tiny silent linear cybernetic actuator driven by piezoelectric device with electromagnetic clamp. In Proceedings of the IEEE Micro Electro Mechanical Systems, Travemunde, Germany, 4–7 February 1992; pp. 232–237. [Google Scholar] [CrossRef]
- Aoyama, H.; Iwata, F.; Sasaki, A. Desktop flexible manufacturing system by movable miniature robots-miniature robots with micro tool and sensor. In Proceedings of the 1995 IEEE International Conference on Robotics and Automation, Nagoya, Japan, 21–27 May 1995; Volume 1, pp. 660–665. [Google Scholar] [CrossRef]
- Zesch, W.; Buchi, R.; Codourey, A.; Siegwart, R. Inertial drives for micro- and nanorobots: Two novel mechanisms. In Proceedings of the Microrobotics and Micromechanical Systems, Philadelphia, PA, USA, 18 October 1995; pp. 80–88. [Google Scholar] [CrossRef]
- Buchi, R.; Zesch, W.; Codourey, A.; Siegwart, R. Inertial drives for micro- and nanorobots: Analytical study. Microrobotics Micromechanical Syst. 1995, 2593, 89–97. [Google Scholar] [CrossRef]
- Yoshikawa, H.; Kagiwada, T.; Harada, H.; Mimura, M. Improvement of propulsion mechanism based on the inertial force. In Towards Synthesis of Micro-/Nano-Systems; Kimura, F., Horio, K., Eds.; Springer: London, UK, 2007; pp. 333–334. [Google Scholar]
- Ju, J.; Wang, Q.; Zhang, K. Design and analysis of a novel micro-robot driving platform. Proc IMechE-Part C J. Mech. Eng. Sci. 2019, 233, 3849–3857. [Google Scholar] [CrossRef]
- Vatholomeos, P.; Papadopoulos, E. Dynamics, design and simulation of a novel micro-robotic platform employing vibration micro-actuators. ASME J. Dyn. Syst. Meas. Control 2006, 128, 122–133. [Google Scholar] [CrossRef]
- Vartholomeos, P.; Papadopoulos, E. Analysis and experiments on the force capabilities of centripetal-force-actuated microrobotic platforms. IEEE Trans. Robot. 2008, 24, 588–599. [Google Scholar] [CrossRef]
- Provatidis, C.G. Design of a propulsion cycle for endless sliding on frictional ground using rotating masses. Univers. J. Mech. Eng. 2014, 2, 35–43. [Google Scholar] [CrossRef]
- Provatidis, C.G. Mechanics of Dean drive on frictional ground. J. Mech. Des. Vib. 2014, 1, 10–19. Available online: https://pubs.sciepub.com/jmdv/1/1/3/index.html (accessed on 23 January 2024). [CrossRef]
- Provatidis, C.G. Unidirectional motion using rotating masses along figure-eight-shaped trajectories. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 397–409. [Google Scholar] [CrossRef]
- Provatidis, C.G. Progress in an antigravity mechanism using inertial masses. In Space, Propulsion & Energy Sciences International Forum (SPESIF-2011); [CR-ROM]; University of Maryland: College Park, MD, USA, 2011. [Google Scholar]
- Provatidis, C.G. Older and contemporary attempts for inertial propulsion. Gen. Sci. J. 2011, 14. Available online: http://gsjournal.net/Science-Journals/Essays/View/3772 (accessed on 23 January 2024).
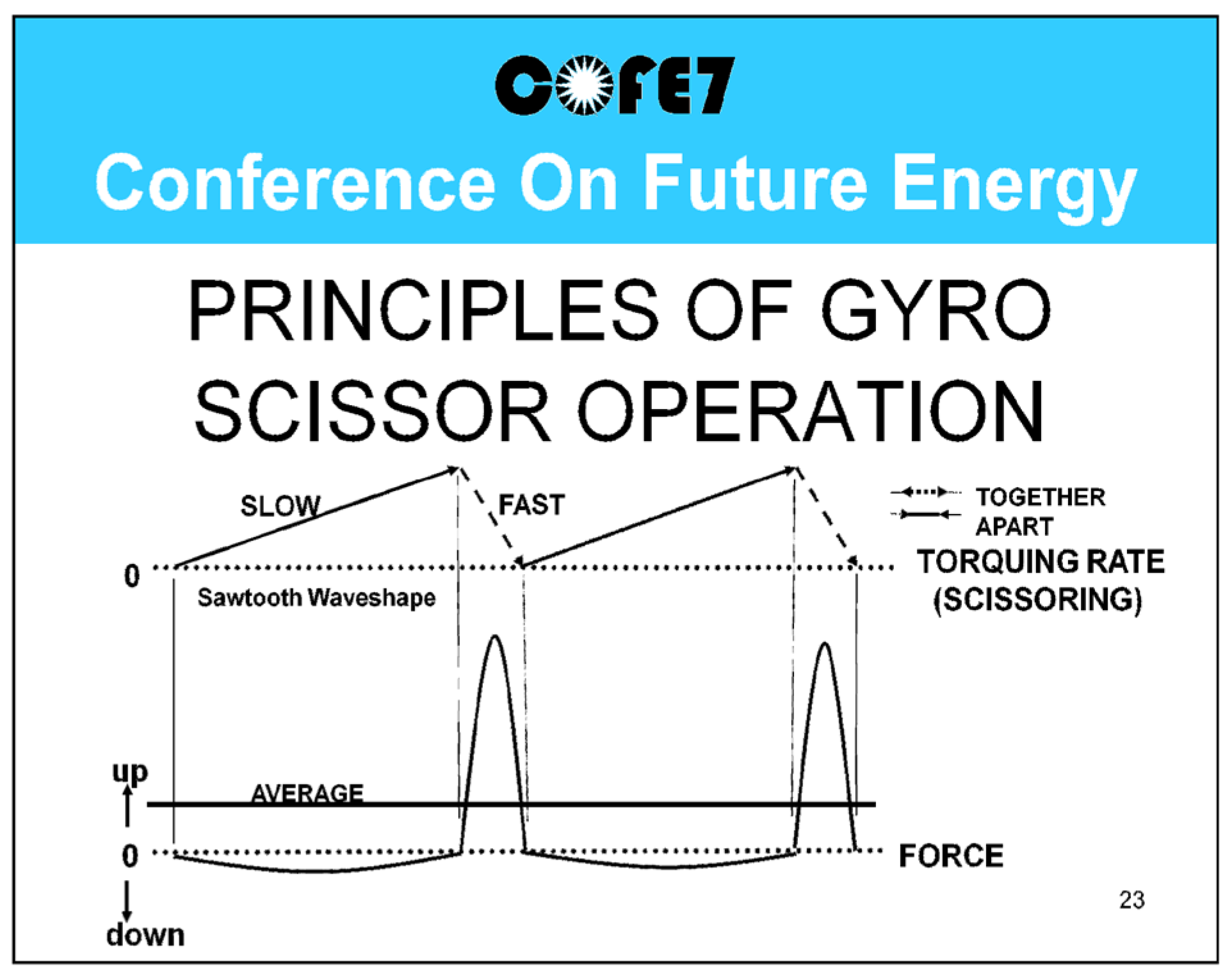
- Gamble, M. History of Boeing control moment gyros (CMG). In Proceedings of the Presentation in Seventh International Conference On Future Energy (COFE7), Embassy Suites, Albuquerque, NM, USA, 30 July–1 August 2015. Boeing 15-00051-EOT. [Google Scholar]
- Gamble, M. Linear propulsion. In Proceedings of the Presentation in Seventh International Conference On Future Energy (COFE7), Embassy Suites, Albuquerque, NM, USA, 30 July–1 August 2015. [Google Scholar]
- Samokhvalov, V.N. Research on, and measurement of, force effects during mass-dynamic interaction. Galilean Electrodyn. GED East 2015, 26, 3–11. [Google Scholar]
- Samokhvalov, V.N. Non-electromagnetic force interaction in presence of rotating masses in vacuum. Int. J. Unconv. Sci. 2016, 1, 68–80. Available online: http://www.unconv-science.org/en/e1/samokhvalov/ (accessed on 23 January 2024).
- Allen, D.P., Jr.; Dunning-Davies, J. Neo-Newtonian Mechanics with Extension to Relativistic Velocities, 18th ed.; Part 1: Non-Radiative Effects; Kindle Direct Publishing: Seattle, WA, USA, 2023. [Google Scholar]
- Milković, V. Gravitational Machines: From Leonardo da Vinci to the Latest Discoveries; Veljko Milkovic Research & Development Center: Novi Sad, Serbia, 2013; ISBN 978-86-88883-03-0. [Google Scholar]
- Allen, D.P.; Provatidis, C.G. Inclined large-angle pendulum may produce endless linear motion of a cart when friction is negligible. WSEAS Trans. Appl. Theor. Mech. 2022, 17, 184–197. Available online: https://wseas.com/journals/mechanics/2022/a465111-016(2022).pdf (accessed on 23 January 2024). [CrossRef]
- Provatidis, C. Teaching the fixed spinning top using four alternative formulations. WSEAS Trans. Adv. Eng. Educ. 2021, 18, 80–95. [Google Scholar] [CrossRef]
- Provatidis, C.G. Revisiting the spinning top. Int. J. Mater. Mech. Eng. 2012, 1, 71–88. Available online: https://www.academia.edu/27516500/Revisiting_the_Spinning_Top (accessed on 23 January 2024).
- Loukanov, I.A. Vibration propulsion of a mobile robot. IOSR J. Mech. Civ. Eng. 2015, 12, 23–33. Available online: https://www.academia.edu/65248904/Vibration_Propulsion_of_a_Mobile_Robot (accessed on 23 January 2024).
- Loukanov, I.A. Application of inertial forces for generating unidirectional motion. Proc. Sci. Conf. Univ. Rousse 2014, 53. Available online: https://www.researchgate.net/publication/314067949_Application_of_Inertial_Forces_for_Generating_Unidirectional_Motion (accessed on 23 January 2024).
- Loukanov, I.A.; Uziak, J. Upon improving the prediction of the flow rate of sonic pump. Int. J. Eng. Res. Appl. 2013, 3, 393–398. Available online: https://www.ijera.com/papers/Vol3_issue1/BF31393398.pdf (accessed on 23 January 2024).
- Lukanov, I.A.; Vitliemov, V.G.; Ivanov, I.V. Dynamics of a mobile mechanical system with vibration propulsion (VibroBot). Int. J. Res. Eng. Sci. (IJRES) 2016, 4, 44–51. [Google Scholar]
- Cook, R.L. Device for Conversion of Centrifugal Force to Linear Force and Motion. U.S. Patent 4,238,968, 16 December 1980. [Google Scholar]
- Thornson, B.R. Apparatus for Developing a Propulsion Force. U.S. Patent No. 4,631,971, 30 December 1986. [Google Scholar]
- Foster, R.E. Converting Rotary Motion into Unidirectional Motion. U.S. Patent 3653269, 4 April 1972. [Google Scholar]
- Hoshino, M. Propulsion Apparatus Using Centrifugal Force. U.S. Patent 20050139022, 30 June 2005. [Google Scholar]
- Chung, T.B. Internal Propulsion Apparatus of Closed System Using a Coriolis Force. International Patent WO 03/087574 A1, 23 October 2003. [Google Scholar]
- Tanner, M.A. Resonant Maglev Centrifugal Propulsion. U.S. Patent 2007/0079663 A1, 12 April 2007. [Google Scholar]
- Farrall, A.W. Inertial Propulsion Device. U.S. Patent US3266233A, 16 August 1966. [Google Scholar]
- Robertson, G.A.; Murad, P.A.; Davis, E. New frontiers in space propulsion sciences. Energy Convers. Manag. 2008, 49, 436–452. [Google Scholar] [CrossRef]
- Robertson, G.A.; Webb, D.W. The death of rocket science in the 21st century. Phys. Procedia 2011, 20, 319–330. [Google Scholar] [CrossRef]
- MrEcodynamic, ZDF Planet e-Die Schwung Maschine. Online in 2 Parts at. Available online: http://www.youtube.com/watch?v=pfk6uykIrUs (accessed on 23 January 2024).
- Laithwaite, E.; Dawson, W. Propulsion System. U.S. Patent 5,860,317, 19 January 1999. [Google Scholar]
- Hilscher, G. Trägheit der Masse mobilisiert die Energietechnik: Mit dem findigen Johann Klimpfinger auf Entdeckungstour durch Archive und seine experimentelle Welt. NET-J. 2011, 16, 46–50. Available online: http://www.borderlands.de/net_pdf/NET0311S46-50.pdf (accessed on 23 January 2024).
- Anonymous. Kreisel Präzessions Antrieb (Gyro Precession Drive). Available online: https://www.youtube.com/watch?v=nstIIZZadAM&ab_channel=gyro2012 (accessed on 23 January 2024).
- Stoney, W. Method of Propulsion. Irish Patent Application IE 20190160 A2, 3 August 2022. [Google Scholar]
- Myshkin, N.P. Dvizheniye tela, nakhod yashegosya v potoke luchistoi energii. Zhyrnal Rus. Fiz.-Him. Obs. 1906, 3, 149. (In Russian) [Google Scholar]
- Kozyrev, N.A. Prichinnaya ili nesimmetrichnaya mekhanika v lineinom priblizhenii, Pulkovo. GAO AN SSSR 1958, 90. (In Russian) [Google Scholar]
- Kozyrev, N.A. On the possibility of experimental investigation of the properties of time. In Time in Science and Philosophy; Elsevier: Prague, Czech Republic, 1971; pp. 111–132. [Google Scholar]
- Available online: http://shipov.com/gennady-i-shipov/ (accessed on 23 January 2024).
- Wikipedia. Available online: https://en.wikipedia.org/wiki/Torsion_field_(pseudoscience) (accessed on 23 January 2024).
- Linevich, E.I.; Ezshov, A.F. The inertial motor. New Energy Technol. 2004, 3, 12–15. [Google Scholar]
- Kouznetsov, D. Support of non-traditional concepts. Far East J. Mech. Eng. Phys. 2010, 1, 1–6. Available online: https://www.pphmj.com/abstract/5076.htm (accessed on 23 January 2024).
- Shipov, G. Inertial Propulsion in Russia: Dr. Gennady Shipov on Torsion Physics & Inertial Propulsion. Interview to American Antigravity.Com. 2012, pp. 1–8. Available online: http://shipov.com/files/200506_news09.pdf (accessed on 23 January 2024).
- Sorokin, V. Available online: http://www.ntv.ru/novosti/84720/video (accessed on 23 January 2024).
- Naumov, A. Russian Scientists Test Perpetual Motion Machine in Space. PRAVDA Newspaper, 14 April 2009. Available online: http://english.pravda.ru/science/tech/14-04-2009/107399-russian_scientists-0 (accessed on 22 January 2024).
- Wikipedia. Available online: https://en.citizendium.org/wiki/Valery_Menshikov (accessed on 23 January 2024).
- Wikipedia. Available online: http://en.wikipedia.org/wiki/Yubileiny (accessed on 23 January 2024).
- Zhao, C.; Zhu, H.; Zhang, Y. Synchronization of two coupled exciters in a vibrating system of spatial motion. Acta Mech. Sin. 2010, 26, 477–493. [Google Scholar] [CrossRef]
- Zhao, C.; Zhang, Y.; Wen, B. Synchronisation and general dynamic symmetry of a vibrating system with two exciters rotating in opposite directions. Chin. Phys. B 2010, 19, 030301. [Google Scholar] [CrossRef]
- Chen, X.; Liu, J.; Li, L. Dynamics of the vibration system driven by three homodromy eccentric rotors using control synchronization. Appl. Sci. 2021, 11, 7691. [Google Scholar] [CrossRef]
- Hambling, D. EmDrive: China’s Radical New Space Drive, Wired 6 February 2013. Available online: https://www.wired.co.uk/article/emdrive-and-cold-fusion (accessed on 23 January 2024).
- Halliday, D.; Resnick, R. Physics; International Edition; Wiley: New York, NY, USA, 1966. [Google Scholar]
- Casey, J. Geometrical derivation of Lagrange’s equations for a system of particles. Am. J. Phys. 1994, 62, 836–847. [Google Scholar] [CrossRef]
- Casey, J. Applying the principle of angular momentum to constrained systems of point masses. Am. J. Phys. 2014, 82, 165–168. [Google Scholar] [CrossRef]
- Laithwaite E 1994 Heretics BBC2 Television London; Plus Private Demonstrations and Imperial College Christmas Lectures. Available online: http://youtube.com/watch?v&tnqx3d;KnNUTOxHoto (accessed on 4 April 2020).
- Pinheiro, M.J. On Newton’s third law and its symmetry-breaking effects. Phys. Scr. 2010, 84, 055004. [Google Scholar] [CrossRef][Green Version]
- Gutsche, G.J. Inertial Propulsion; and You Thought You Knew Everything about Physics; CreateSpace Independent Publishing Platform: Toronto, ON, Canada, 2018. [Google Scholar]
- Gutsche, G.J. Device for Efficient Self-Contained Inertial Vehicular Propulsion. U.S. Patent 9,995,284 B1, 12 January 2018. [Google Scholar]
- Allen, D.P., Jr. Foundations of Gutschian Mechanics: Part 1: Basics, 6th ed.; Kindle Direct Publishing: Seattle, WA, USA, 2019. [Google Scholar]
- Provatidis, C.G. A Review on antigravity, levitation, resonance, and devices inspired by the ouroboros serpent. Rose+Croix J. 2012, 9, 18–47. Available online: https://www.academia.edu/download/50150834/vol9_18_47_provatidis.pdf (accessed on 23 January 2024).
- Manning, J. Escape from Gravity: Is the ancient promise of freedom from the pull of earth alive and well at NASA and elsewhere? In Forbidden Science: From Ancient Technologies to Free Energy; Douglas Kenyon, J., Ed.; Bear & Co: Rochester, VT, USA, 2008; Chapter 13. [Google Scholar]
- Gerjuoy, E. On Newton’s third law and the conservation of momentum. Am. J. Phys. 1949, 17, 477–482. [Google Scholar] [CrossRef]
- Martins, A.A.; Pinheiro, M.J. On the nature of the propulsive force of asymmetric capacitors in the atmosphere. Phys. Procedia 2011, 20, 103–111. [Google Scholar] [CrossRef]
- Martins, A.A.; Pinheiro, M.J. On the nature of the propulsive force of asymmetric capacitors in a vacuum. Phys. Procedia 2011, 20, 112–119. [Google Scholar] [CrossRef]
- Stephenson, A. XX. On induced stability. Lond. Edinb. Dublin Phil. Mag. J. Sci. 1908, 15, 233–236. [Google Scholar] [CrossRef]
- Kapitsa, P.L. Dynamic stability of the pendulum when the point of suspension is oscillating. J. Exp. Theor. Phys. 1951, 21, 588–597. (In Russian) [Google Scholar]
- Blekhman, I.I.; Dresig, H.; Shishkina, E. On the theory of the Indian magic rope. In Selected Topics in Vibrational Mechanics; Blekhman, I.I., Ed.; World Scientific: Singapore, 2004; pp. 139–149. [Google Scholar]
- Cross, R. Laithwaite’s heavy spinning disk demonstration. Phys. Teach. 2014, 52, 349–350. [Google Scholar] [CrossRef]
- Davidowsky, P.; Rogers, M. Debunking a video on YouTube as an authentic research experience. Phys. Teach. 2015, 53, 304–306. [Google Scholar] [CrossRef]
- Sheheitli, H. On the dynamics of a spinning top under high-frequency excitation: Part I—Pivot point under vertical harmonic vibration. Nonlinear Dyn. 2017, 90, 765–779. [Google Scholar] [CrossRef]
- Sheheitli, H. On the dynamics of a spinning top under high-frequency excitation. Part II: Pivot point under horizontal harmonic vibration. Nonlinear Dyn. 2017, 90, 2269–2276. [Google Scholar] [CrossRef]
- LaViolette, P.A. Secrets of Antigravity Propulsion: Tesla, UFOs, and Classified Aerospace Technology; Bear & Company: Rochester, VT, USA, 2008. [Google Scholar]
- Childress, D.H. The Antigravity Handbook, 3rd ed.; Adventures Unlimited Press: Kampton, IL, USA, 2010. [Google Scholar]
- Passaro, V.M.N.; Cuccovillo, A.; Vaiani, L.; De Carlo, M.; Campanella, C.E. Gyroscope technology and applications: A review in the industrial perspective. Sensors 2017, 17, 2284. [Google Scholar] [CrossRef] [PubMed]
- Usubamatov, R. Theory of Gyroscopic Effects for Rotating Objects: Gyroscopic Effects and Applications; Springer: Cham, Switzerland, 2020. [Google Scholar] [CrossRef]
- Shipov, G. Four-Dimensional Gyroscope, Demonstrating the Violation of the Momentum Conservation Law during the Absolute Electic Collision with the Wall. U.S. Patent US20040166482A1, 15 February 2001. [Google Scholar]
- Solomon, B.T. An Introduction to Gravity Modification: A guide to Using Laithwaite’s and Podkletnov’s Experiments and the Physics of Forces for Empirical Results, 2nd ed.; Universal-Publishers: Boca Raton, FL, USA, 2012; pp. 67–81, 309–326. [Google Scholar]
- Solomon, B.T. Gravitational acceleration without mass and noninertia fields. Phys. Essays 2011, 24, 327–337. [Google Scholar] [CrossRef]
- Bjerke, E.; Pehrsson, B. Development of a Nonlinear Mechatronic Cube: The Jumping and Balancing Cube. Master’s Thesis, Chalmers University of Technology, Gothenburg, Sweden, 2016. [Google Scholar]
- Elliott, D.S. Momentum Control Systems and Their Application in Robotic Systems. Ph.D. Thesis, Cornell University, Ithaca, NY, USA, 2019. [Google Scholar]
- Elliott, D.S.; Peck, M.; Nesnas, I.A.D. Optimal solution for torque capability of control moment gyroscopes. In Proceedings of the 2019 IEEE Aerospace Conference, Big Sky, MT, USA, 2–9 March 2019; pp. 1–17. [Google Scholar] [CrossRef]
- Elliott, D.S.; Peck, M.A. Dynamics and control of polyhedral surface robots with control moment gyroscopes. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, Grapevine, TX, USA, 9–13 January 2017. AIAA 2017-1050, Session: Spacecraft Robotics. [Google Scholar] [CrossRef]
- CMG-Rover: NASA Has Supported Researchers in Cornell University to Develop Technologies to Use It for Locomotion Purposes (Say) in Mars. Available online: https://www.spacecraftresearch.com/cmg-rover (accessed on 23 January 2024).
- Gajamohan, M.; Merz, M.; Thommen, I.; D’Andrea, R. The Cubli: A cube that can jump up, balance, and ‘walk’. In Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems, Algavre, Portugal, 7–12 October 2012; pp. 3722–3727. Available online: https://www.youtube.com/watch?v=n_6p-1J551Y&ab_channel=GajamohanMohanarajah (accessed on 23 January 2024).
- NASA’s Helical Engine and Other Reactionless Drives. Available online: https://www.youtube.com/watch?v=yNro9yiVZno&t=3s&ab_channel=PhysicsUnsimplified (accessed on 23 January 2024).
- Gyroscopic Precession Powered Car. Available online: https://www.youtube.com/watch?v=zOGlHC56Brs&t=4s&ab_channel=anisotropicplus (accompanied by 12 more movies) (accessed on 23 January 2024).
- YouTube: Falcon Space Demonstrates Gyroscopic Propellentless Propulsion (Part 2). Available online: https://www.youtube.com/watch?v=OPPnmAFcPiw&ab_channel=CharlieC (accessed on 23 January 2024).
- Raptis, Y.; Kourouklis, G.A. A tribute to the work of the late Professor Evangelos Anastassakis (1938–2000). Phys. Status Solidi (B) 2001, 223, 1–13. [Google Scholar] [CrossRef]
- Ünker, F.; Çuvalci, O. Gyroscopic inertial thruster (GIT). In Proceedings of the 9th International Automotive Technologies Congress, Bursa, Turkey, 7–8 May 2018; pp. 686–695. [Google Scholar]
- Gerocs, A.; Korka, Z.-I.; Biró, I.; Cojocaru, V. Analytical investigation of an inertial propulsion system using rotating masses. J. Phys. Conf. Ser. 2020, 1426, 012031. [Google Scholar] [CrossRef]
- Gerocs, A.; Gillich, G.-R.; Nedelcu, D.; Korka, Z.-I. A multibody inertial propulsion drive with symmetrically placed balls rotating on eccentric trajectories. Symmetry 2020, 12, 1422. [Google Scholar] [CrossRef]
- Timofte, S.; Miclosina, C.-O.; Cojocaru, V.; Gerocs, A.; Korka, Z.-I. Inertial propulsion of a mobile platform driven by two eccentric bodies. Appl. Sci. 2023, 13, 9511. [Google Scholar] [CrossRef]
- Sprenger, A.R.; Scholz, C.; Ldov, A.; Wittkowski, R.; Löwen, H. Inertial self-propelled particles in anisotropic environments. Commun. Phys. 2023, 6, 301. [Google Scholar] [CrossRef]
- Frolov, A.V. New Aerospace Technologies; Independent Publisher: St.-Petersburg, Russia, 2017; ISBN 978-5-7679-3883-4. [Google Scholar]
- McCulloch, M.E. Low-acceleration dwarf galaxies as tests of quantised inertia. Astrophys Space Sci. 2017, 362, 57. [Google Scholar] [CrossRef][Green Version]
- McCulloch, M.E. Physics from the Edge: A New Cosmological Model for Inertia; World Scientific: Singapore, 2014. [Google Scholar]
- McCulloch, M.E. Can the Podkletnov effect be explained by quantised inertia? Phys. Procedia 2011, 20, 134–139. [Google Scholar] [CrossRef]
- Allan Williams, University Research Inspires New Commercial Advances in Satellite Propulsion, 27 April 2022. Available online: https://www.plymouth.ac.uk/news/university-research-inspires-new-commercial-advances-in-satellite-propulsion (accessed on 23 January 2024).
- Mike McCulloch, Using New Physics to Get to Alpha Centauri in a Human Lifetime (19 January 2022). Available online: https://www.youtube.com/watch?v=45E822mP59Q (accessed on 23 January 2024).
- Orf, D. The ‘Impossible’ Quantum Drive Supposedly Defies Newton’s Laws of Motion, Popular Mechanics, 16 November 2023. Available online: https://www.popularmechanics.com/space/satellites/a45850635/quantum-drive-space-test (accessed on 23 January 2024).
- Plain, C. Breaking: Satellite Failure Scuttles First of Its Kind in Space Test of Physics Defying Quantum Drive. The Debrief, 12 February 2024. Available online: https://thedebrief.org/breaking-satellite-failure-scuttles-first-of-its-kind-in-space-test-of-physics-defying-quantum-drive/ (accessed on 23 January 2024).
- Anonymous. Rogue Space Systems Operations Team is Announcing the Suspension of The active Phase of a Partially Successful Mission. 9 February 2024. Available online: https://rogue.space/suspension_of_barry1_operations (accessed on 23 January 2024).
- Aurigema, A.N.; Buhler, C.R. System and Method for Generating Forces Using Asymmetrical Electrostatic Pressure. U.S. Patent 11,511,891 B2, 29 November 2022. [Google Scholar]
- Buhler, C. Propellantless Propulsion Device. Available online: http://www.youtube.com/watch?v=WhsKMWOYuYo (accessed on 23 January 2024).
- Goebel, D.M.; Katz, I. Fundamentals of Electric Propulsion: Ion and Hall Thrusters; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Forward, R.L. Guidelines to antigravity. Am. J. Phys. 1963, 31, 166–170. [Google Scholar] [CrossRef]
- Mashhoon, B.; Hehl, F.W.; Theiss, D.S. On the gravitational effects of rotating masses: The Thirring-Lense papers. Gen. Relativ. Gravit. 1984, 16, 711–750. [Google Scholar] [CrossRef]
- Wikipedia, Entry: Mach’s Principle. Available online: https://en.wikipedia.org/wiki/Mach%27s_principle (accessed on 23 January 2024).
- Tajmar, M. Homopolar artificial gravity generator based on frame-dragging. Acta Astronaut. 2010, 66, 1297–1301. [Google Scholar] [CrossRef]
- Millis, M.G. Inertial frames and breakthrough propulsion physics. Acta Astronaut. 2017, 138, 85–94. [Google Scholar] [CrossRef]
- Wisdom, J. Swimming in spacetime: Motion by cyclic changes in body shape. Science 2003, 299, 1865–1869. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Provatidis, C.G. Inertial Propulsion Devices: A Review. Eng 2024, 5, 851-880. https://doi.org/10.3390/eng5020046
Provatidis CG. Inertial Propulsion Devices: A Review. Eng. 2024; 5(2):851-880. https://doi.org/10.3390/eng5020046
Chicago/Turabian StyleProvatidis, Christopher G. 2024. "Inertial Propulsion Devices: A Review" Eng 5, no. 2: 851-880. https://doi.org/10.3390/eng5020046
APA StyleProvatidis, C. G. (2024). Inertial Propulsion Devices: A Review. Eng, 5(2), 851-880. https://doi.org/10.3390/eng5020046