Development and Applications of 3D Printing-Processed Auxetic Structures for High-Velocity Impact Protection: A Review
Abstract
1. Introduction
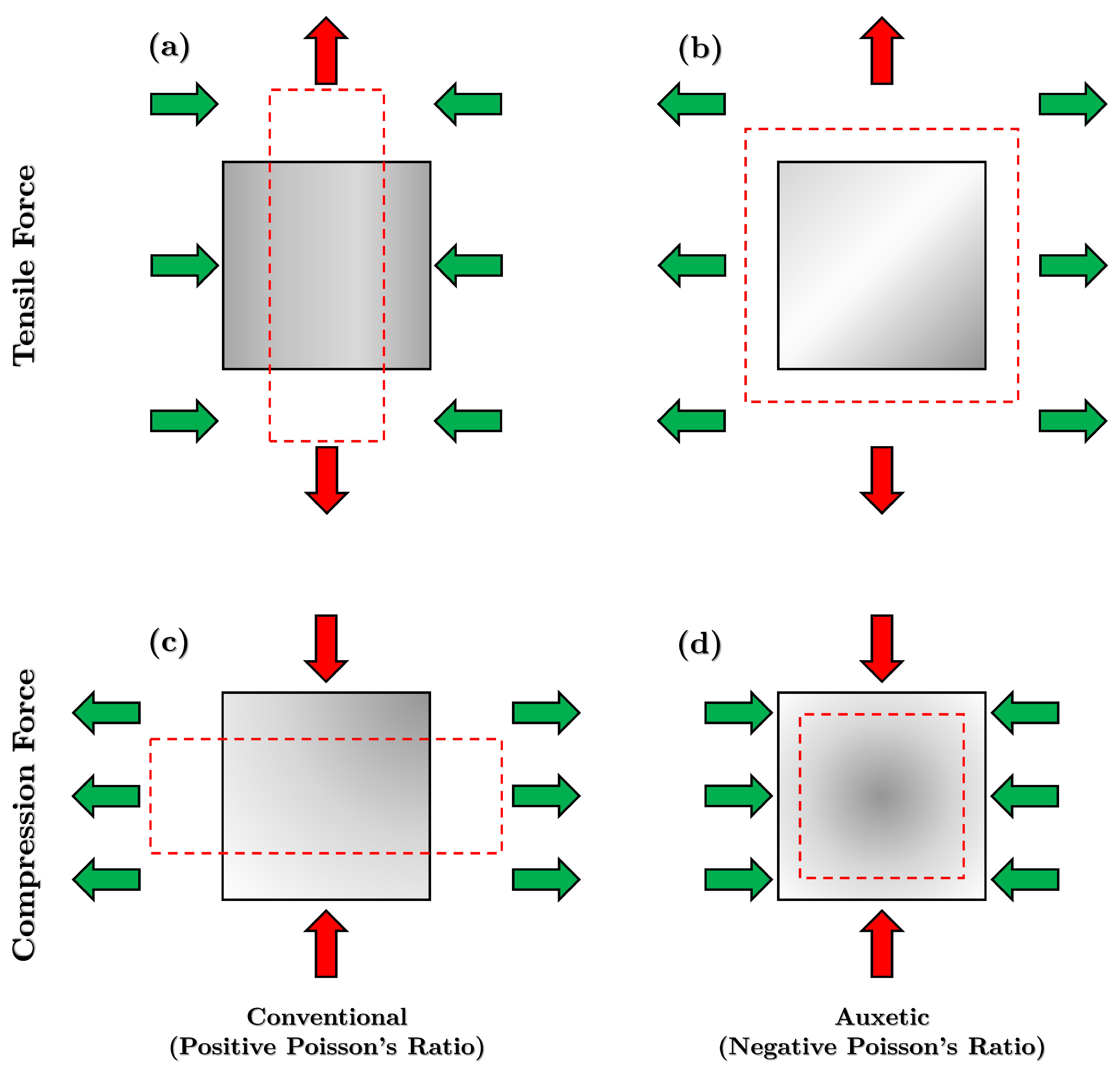
2. Properties of Auxetic Materiais
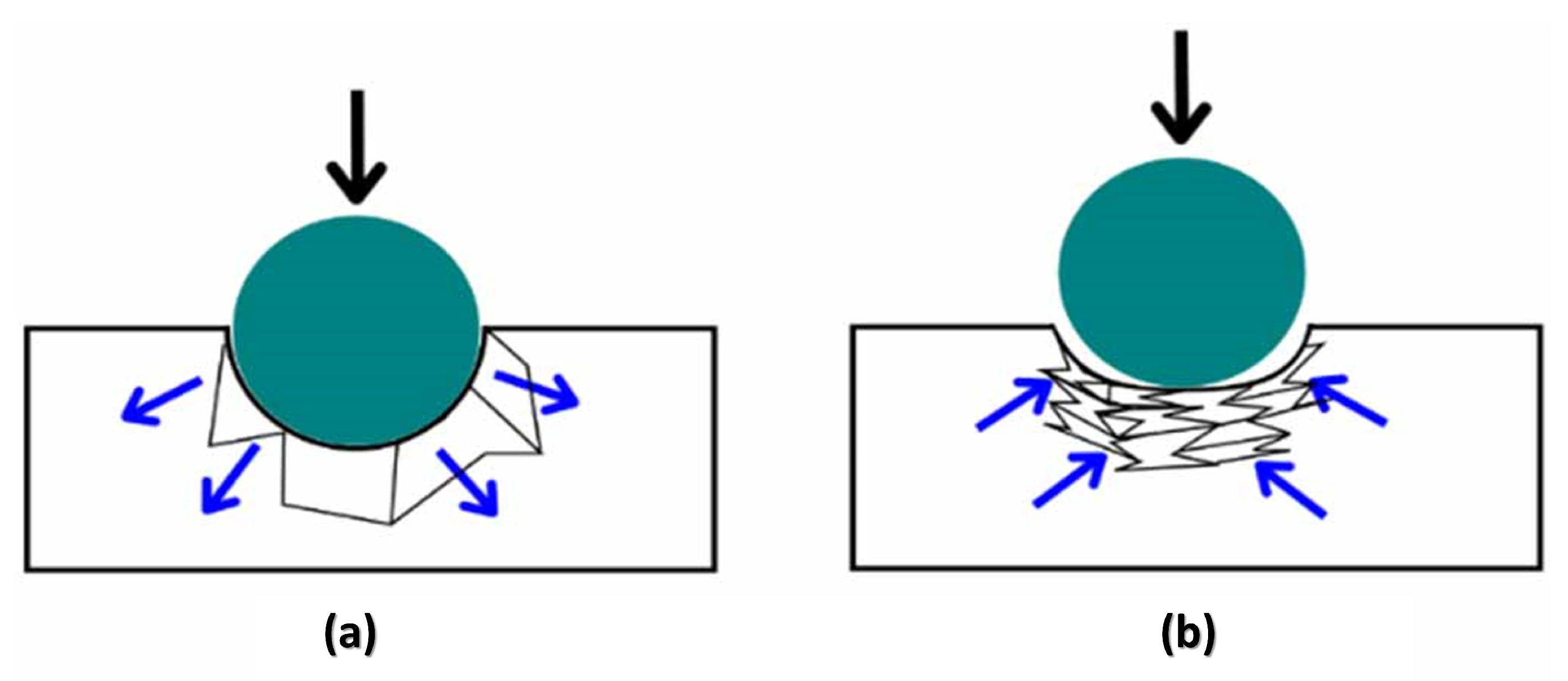
2.1. Resistance to Indentation
2.2. Shear Resistance
2.3. Fracture Resistance
2.4. Energy Absorption
2.5. Synclastic Behavior
2.6. Variable Permeability
2.7. Shape Memory Auxetics
2.8. AXSs for Ballistic Protection
3. Auxetic Structures
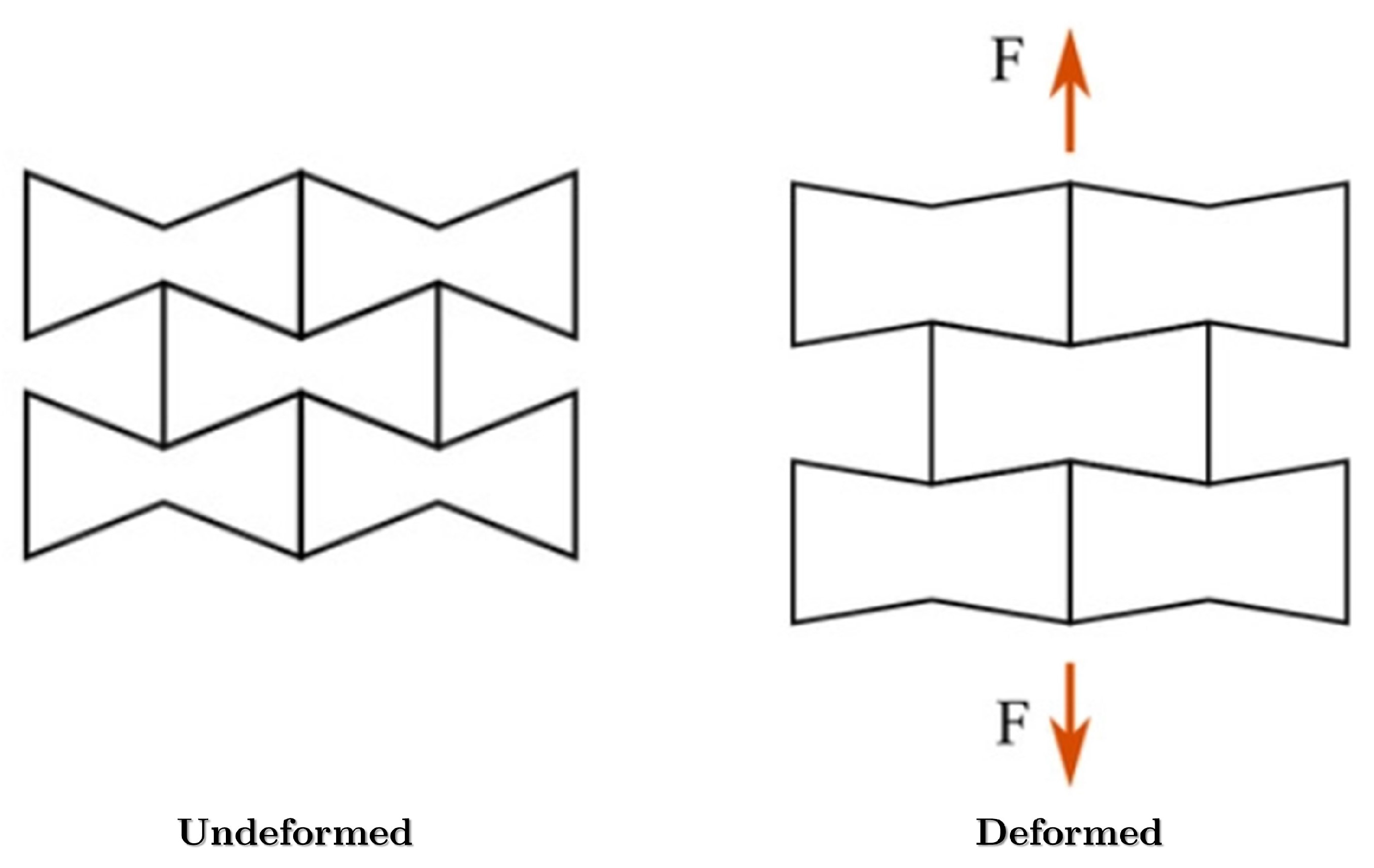
3.1. Re-Entrant Structures
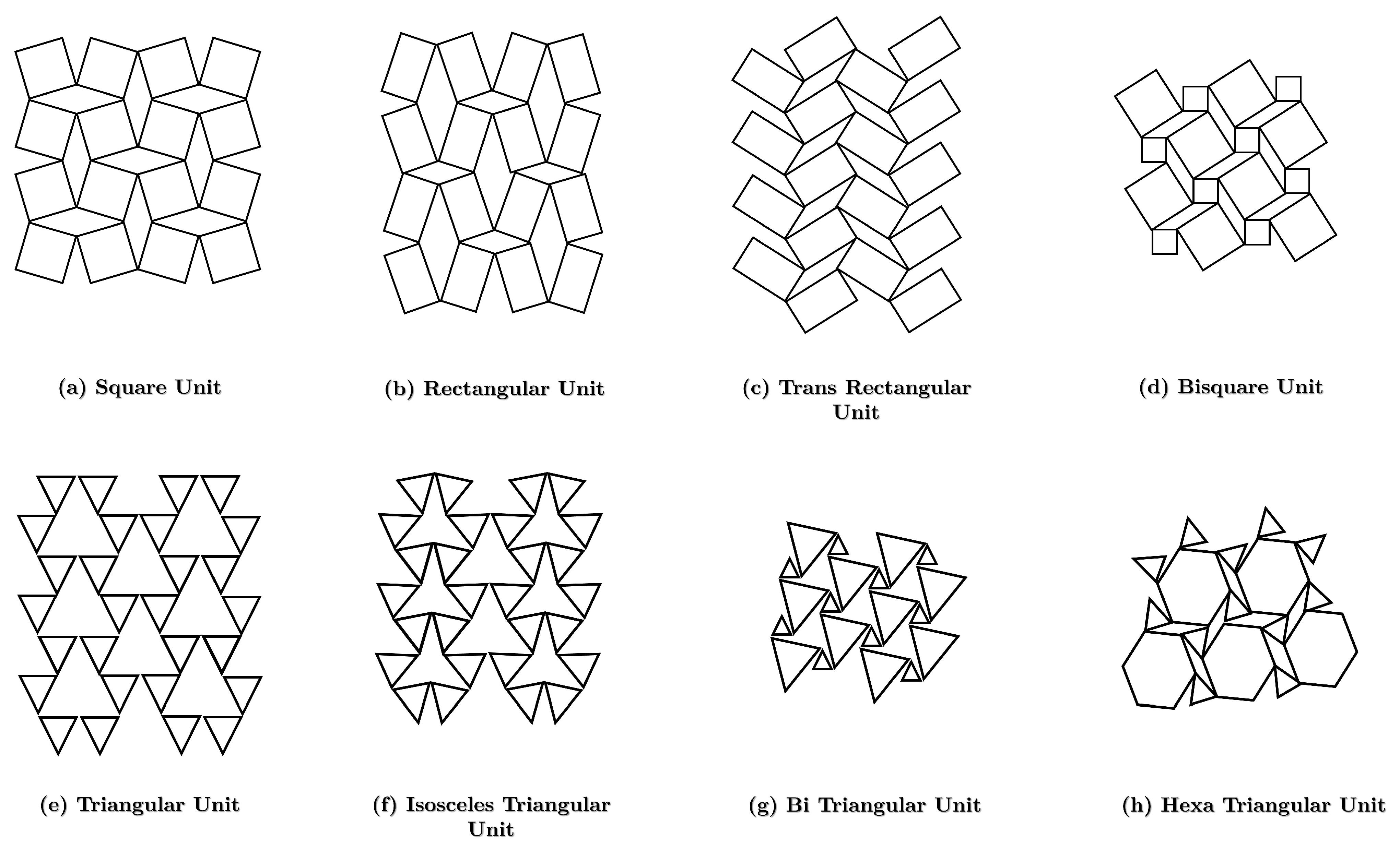
3.2. Rotating Unit Structures
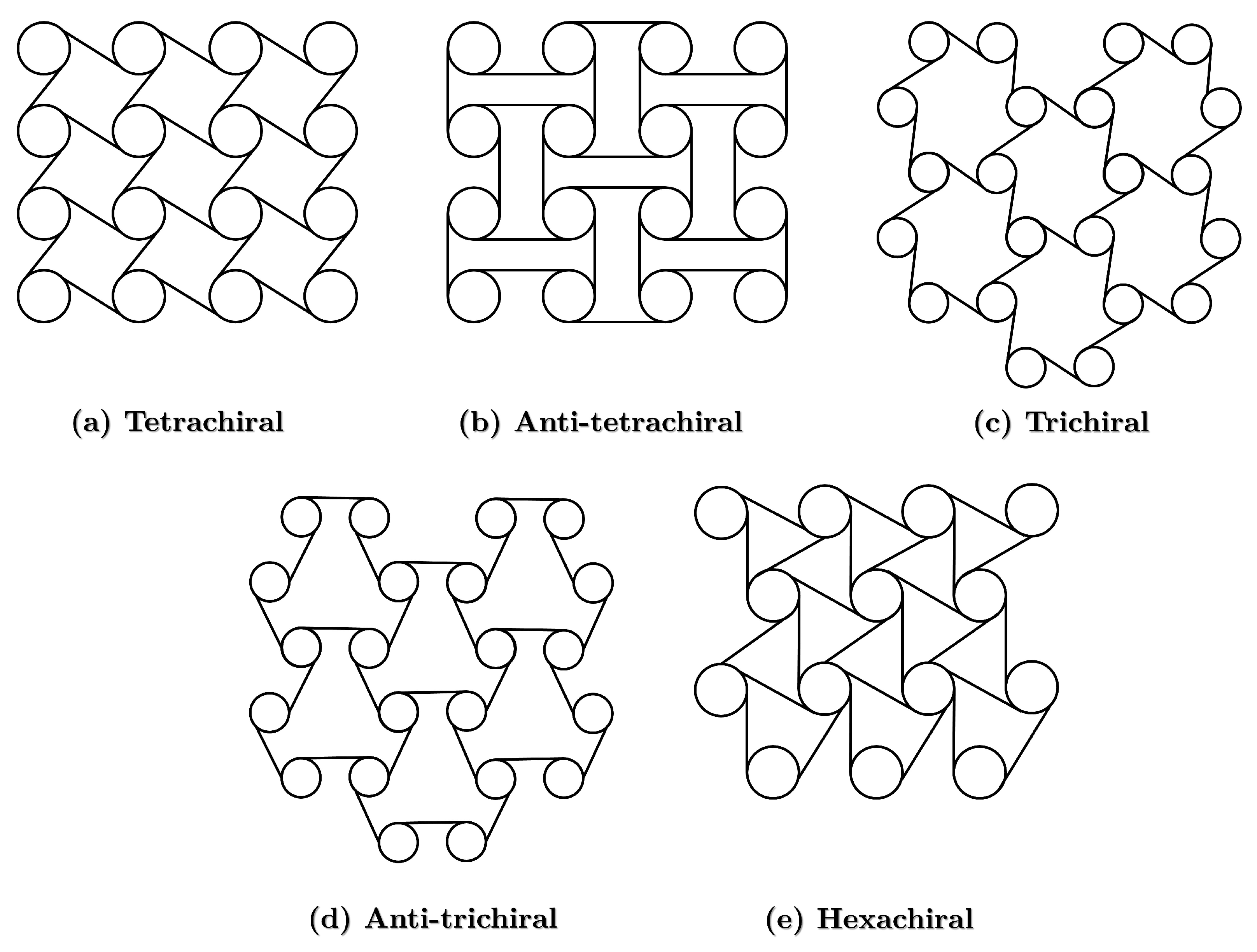
3.3. Chiral Structures
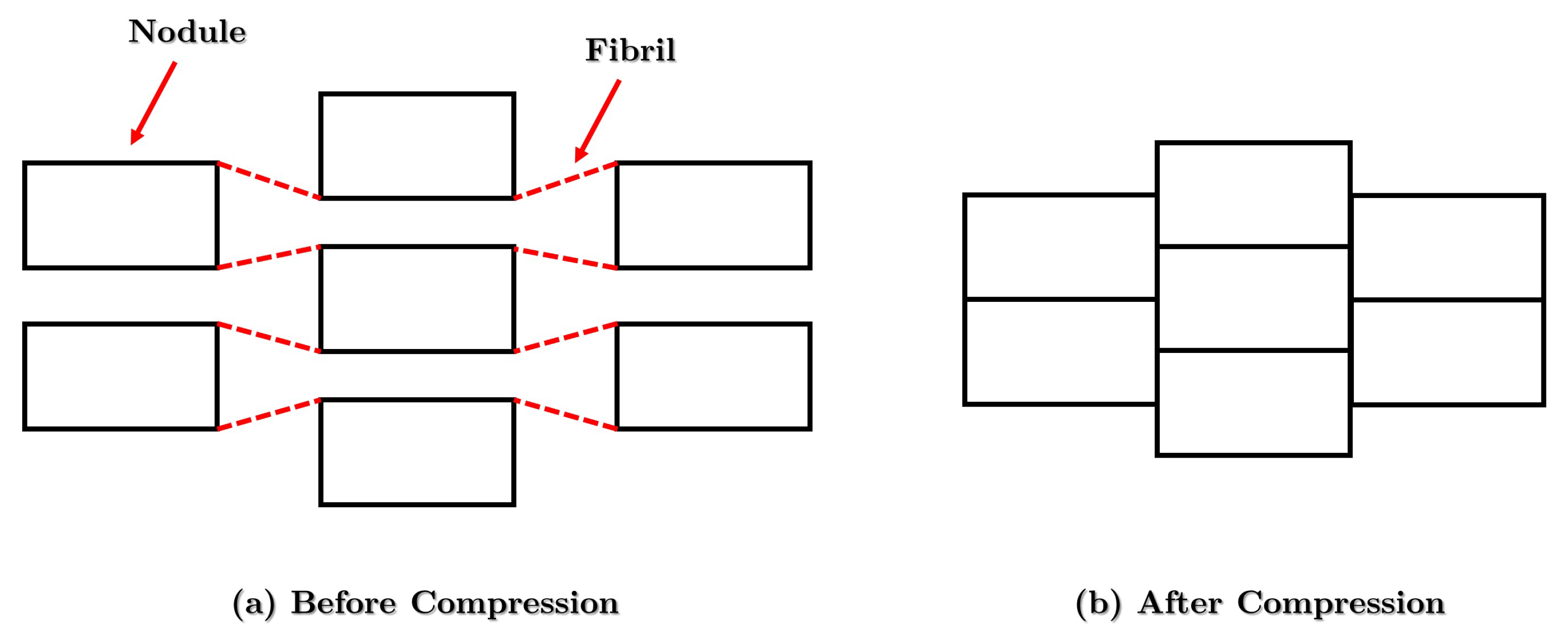
3.4. Nodule and Fibril Structures
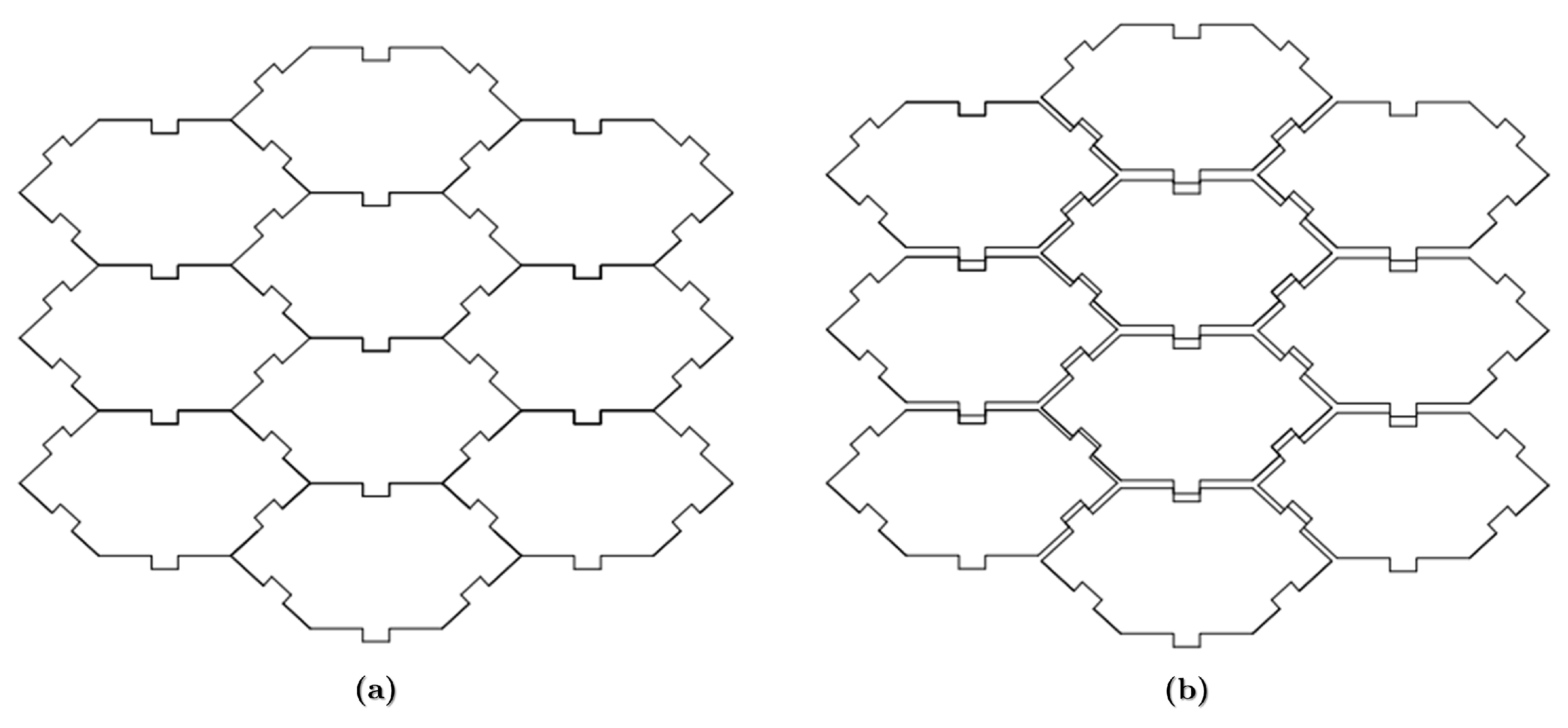
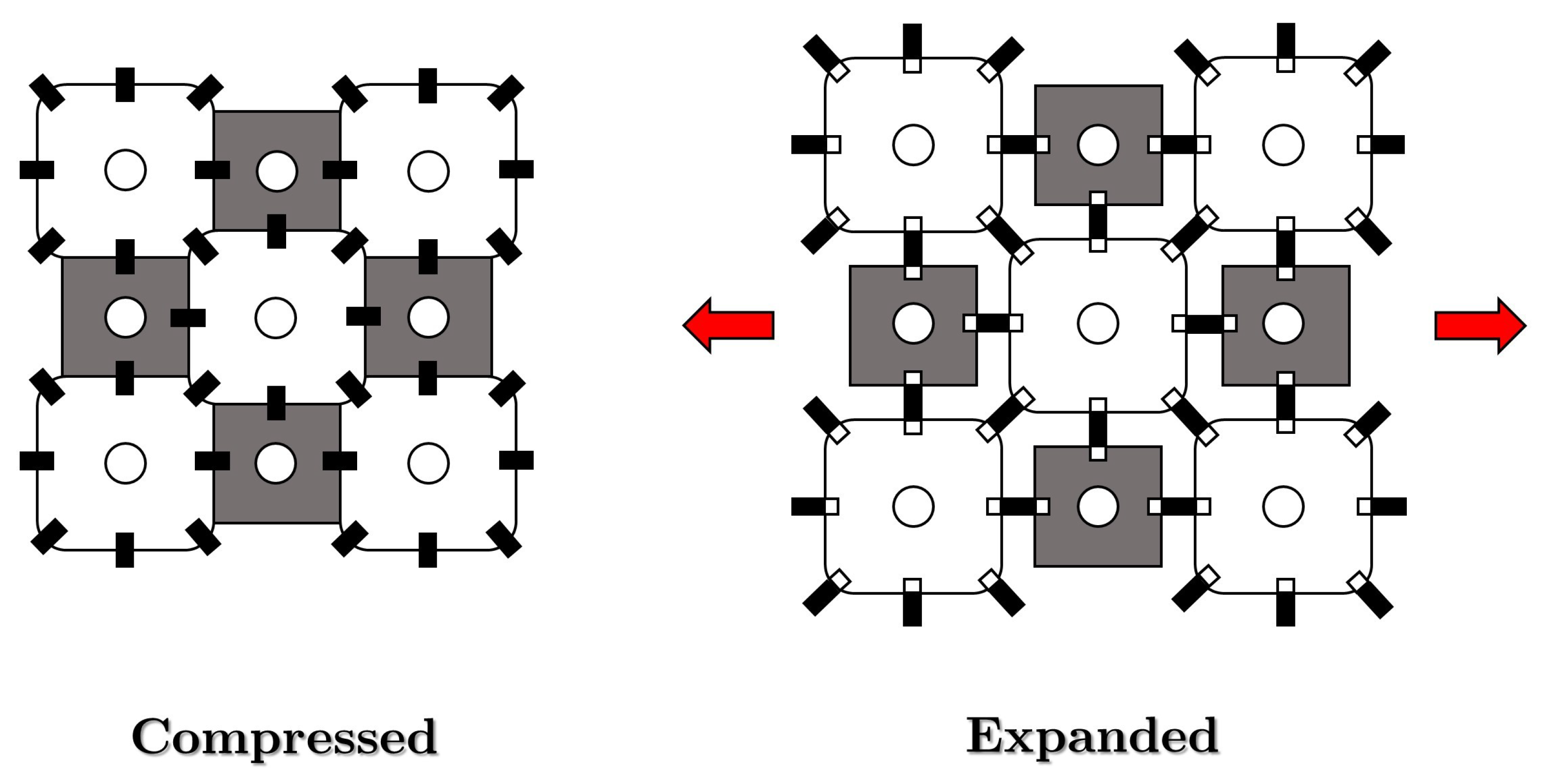
3.5. Interlocking Structure
3.6. Keyed Brick Structure
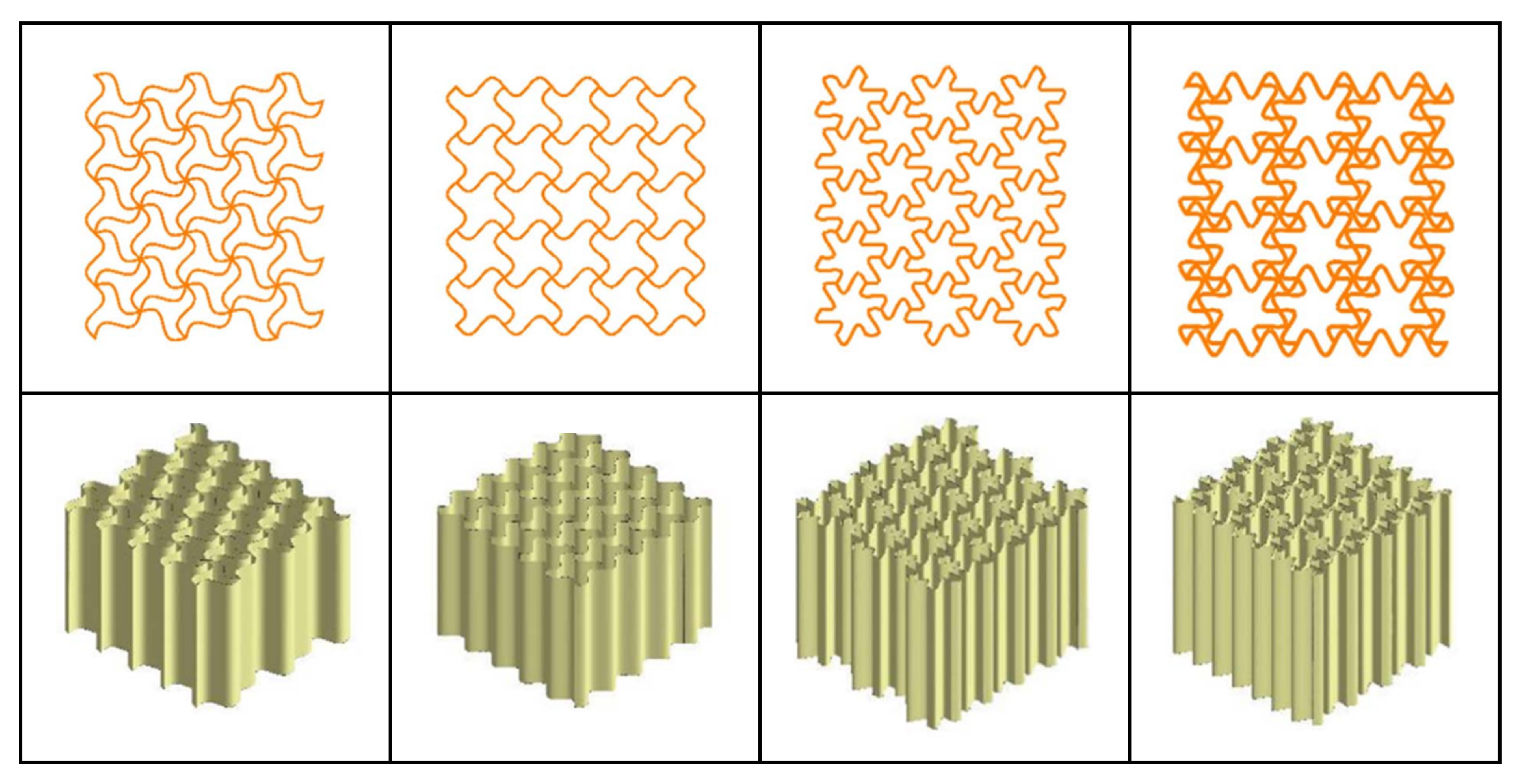
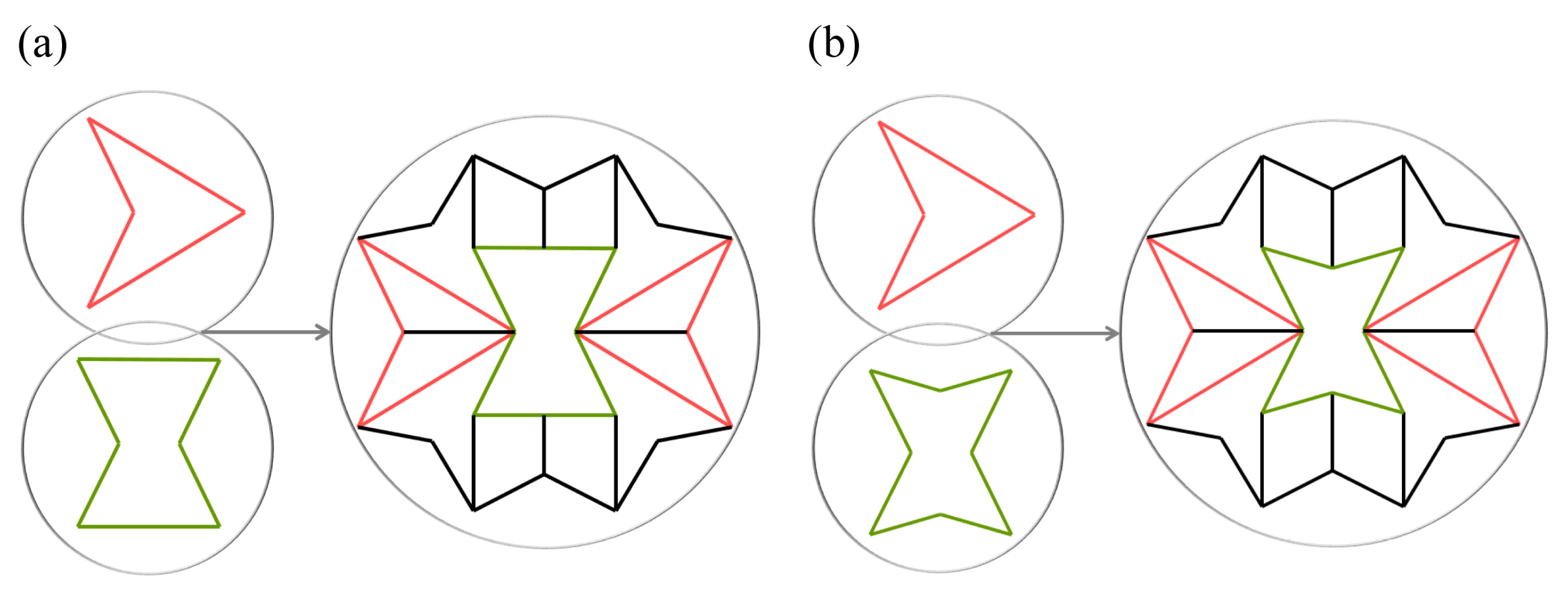
3.7. Other Geometries
3.8. Brief Summary
4. Manufacturing Techniques of Auxetic Structures
- Self-Propagating Photopolymer Waveguide (SPPW);
- Microstereolitography (SLA);
- Direct Laser Writing (DLW);
- Self-Assembly
- Selective Laser Melting (SLM).
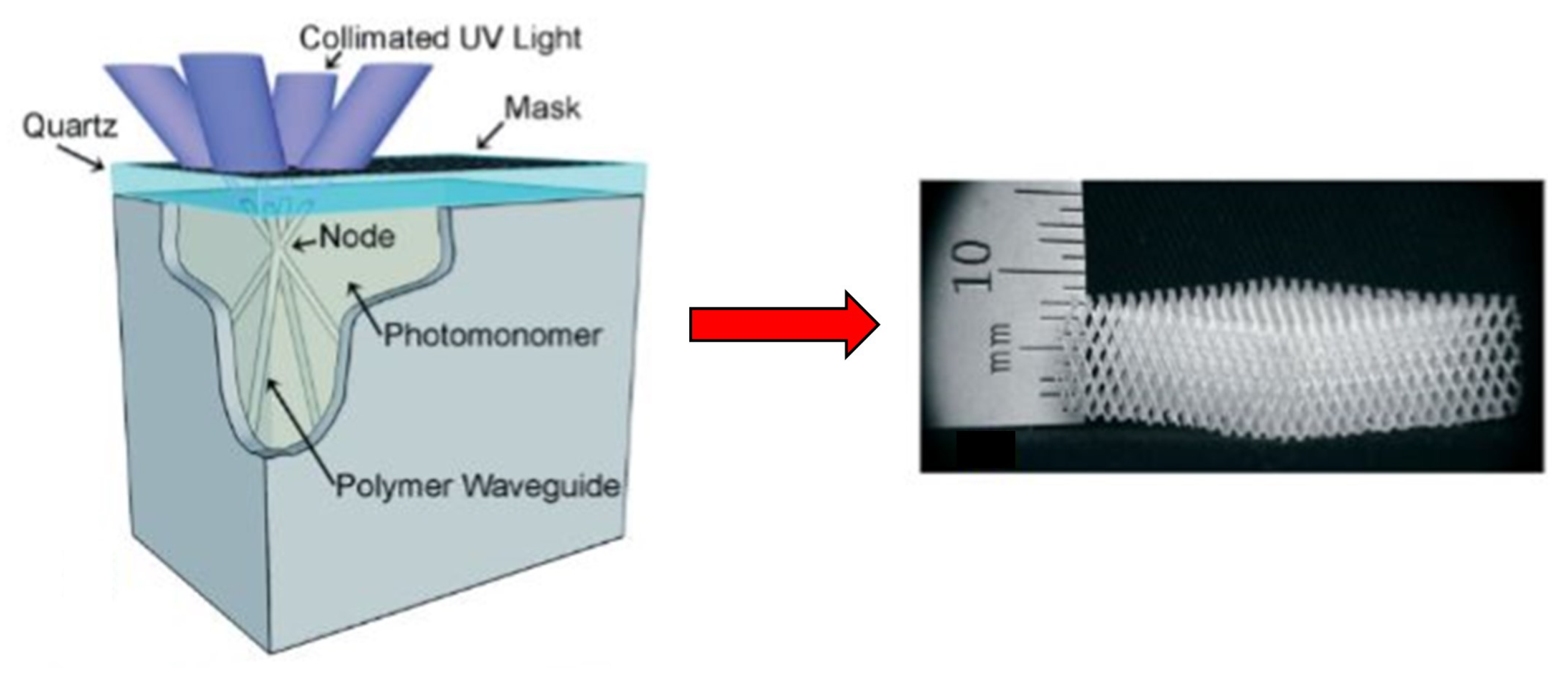
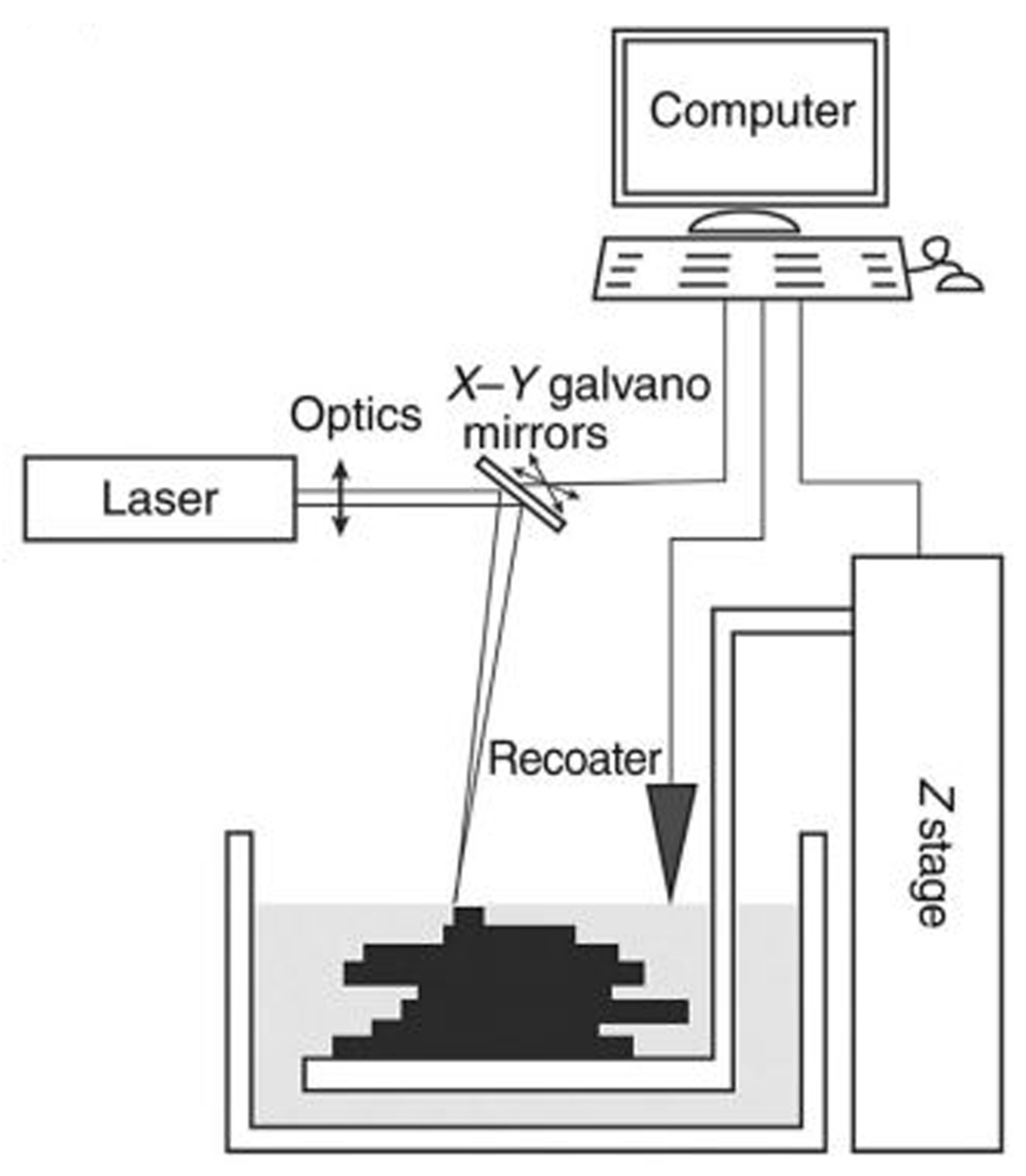
4.1. Self-Propagating Photopolymer Waveguides (SPPW)
4.2. Microstereolithography (SLA)
4.3. Direct Laser Writing (DLW)
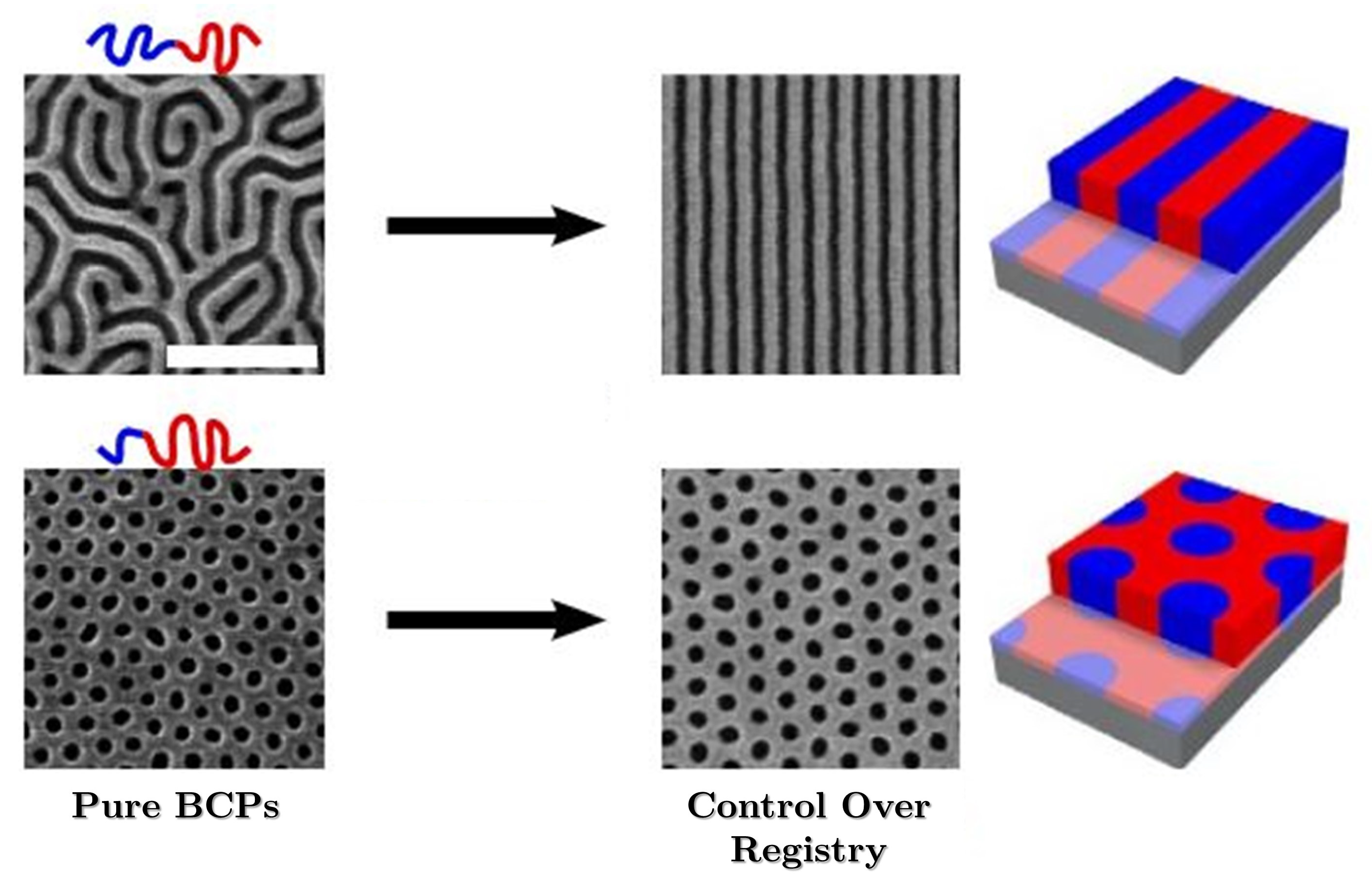
4.4. Self-Assembly
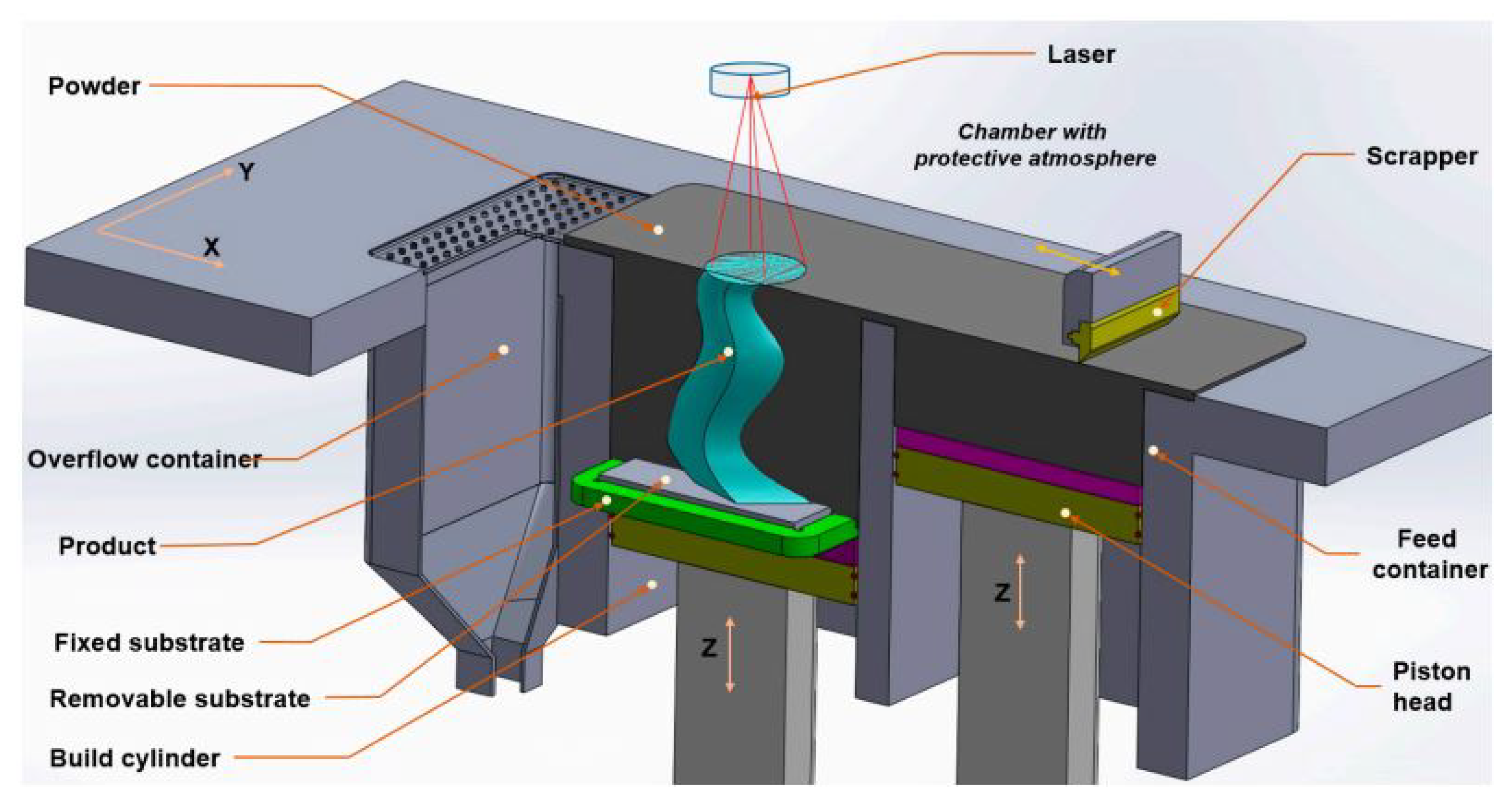
4.5. Selective Laser Melting (SLM)
4.6. Other Techniques
4.7. Brief Summary
5. Applications
Brief Summary
6. Conclusions
7. Final Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Poisson’s Ratio | |
| Wavelength | |
| ABS | Acrylonitrile Butadiene Styrene |
| ACC | Auxetic Contacted Cube |
| Ag | Silver |
| AM | Additive Manufacturing |
| Au | Gold |
| AXS | Auxetic Structures |
| BCP | Block Copolymers |
| BCPS | Braced Cross-Petal Structure |
| CFRP | Carbon Fiber Reinforced Polymer |
| CM | Conventional Material |
| CNC | Computer Numeric Control |
| Cu | Copper |
| CPS | Cross-Petal Structure |
| CVD | Chemical Vapor Deposition |
| DLW | Direct Laser Writing |
| E | Young’s Modulus |
| FDM | Fused Deposition Modeling |
| FEM | Finite Elements Method |
| Fe3O4 | Magnetite |
| G | Shear Modulus |
| GIC | Fracture Initiation Toughness |
| GNP | Graphene Nanoplatelets |
| GPa | Gigapascal |
| H | Hardness |
| HGS | Hourglass Structure |
| K | Bulk Modulus |
| KIC* | Fracture Toughness |
| LDPE | Low Density Polyethylene |
| LRP | Laser Rapid Prototyping |
| MPP | Multi-Photon Polymerization |
| NIJ | National Institute of Justice |
| NiTi | Nickel Titanium |
| NPR | Negative Poisson’s Ratio |
| PDA | Polydiacetylene |
| PLA | Polylactic Acid |
| PP | Polypropylene |
| PU | Polyurethane |
| RAH | Re-entrant Arrowhead Structure |
| RCA | Re-entrant Chiral Auxetic |
| REC | Re-entrant Circular |
| SAH | Star Arrowhead Structure |
| SEA | Specific Energy Absorbed |
| SEBM | Selective ELectron Beam Melting |
| SLA | Microstereolithography |
| SLM | Selective Melting Laser |
| SLS | Selective Laser Sintering |
| SMEP | Shape Memory Epoxy Polymer |
| SMP | Shape Memory Polymers |
| SPPW | Self-propagationg Photopolymer Waveguides |
| SSG | Shear Stiffening Gel |
| STF | Shear Thickening Fluid |
| TNT | Trinitrotoluene |
| TPU | Thermoplastic Polyurethane |
| UV | Ultraviolet |
| VPP | Vat Photopolymerization |
| ZrO2 | Zirconium Oxide |
References
- Callister, W.D., Jr.; Rethwisch, D.G. Fundamentals of Materials Science and Engineering: An Integrated Approach; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Li, X.; Zhang, P.; Wang, Z.; Wu, G.; Zhao, L. Dynamic behavior of aluminum honeycomb sandwich panels under air blast: Experiment and numerical analysis. Compos. Struct. 2014, 108, 1001–1008. [Google Scholar] [CrossRef]
- Han, X.; Cai, H.; Sun, J.; Wei, Z.; Huang, Y.; Wang, A. Numerical Studies on Failure Mechanisms of All-Composite Sandwich Structure with Honeycomb Core under Compression and Impact Loading Conditions. Polymers 2022, 14, 4047. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Yang, X.; Li, P.; Huang, G.; Feng, S.; Shen, C.; Han, B.; Zhang, X.; Jin, F.; Xu, F.; et al. Bioinspired engineering of honeycomb structure—Using nature to inspire human innovation. Prog. Mater. Sci. 2015, 74, 332–400. [Google Scholar] [CrossRef]
- Wahl, L.; Maas, S.; Waldmann, D.; Zürbes, A.; Frères, P. Shear stresses in honeycomb sandwich plates: Analytical solution, finite element method and experimental verification. J. Sandw. Struct. Mater. 2012, 14, 449–468. [Google Scholar] [CrossRef]
- Bitzer, T. Honeycomb Technology: Materials, Design, Manufacturing, Applications and Testing; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1997. [Google Scholar]
- Yang, X.; Sun, Y.; Yang, J.; Pan, Q. Out-of-plane crashworthiness analysis of bio-inspired aluminum honeycomb patterned with horseshoe mesostructure. Thin-Walled Struct. 2018, 125, 1–11. [Google Scholar] [CrossRef]
- He, Q.; Feng, J.; Chen, Y.; Zhou, H. Mechanical properties of spider-web hierarchical honeycombs subjected to out-of-plane impact loading. J. Sandw. Struct. Mater. 2020, 22, 771–796. [Google Scholar] [CrossRef]
- Ha, N.S.; Lu, G. A review of recent research on bio-inspired structures and materials for energy absorption applications. Compos. Part B Eng. 2020, 181, 107496. [Google Scholar] [CrossRef]
- Haq, A.U.; Reddy, N.S.K. A brief review on various high energy absorbing materials. Mater. Today Proc. 2021, 38, 3198–3204. [Google Scholar] [CrossRef]
- Yan, J.; Liu, Y.; Yan, Z.; Bai, F.; Shi, Z.; Si, P.; Huang, F. Ballistic characteristics of 3D-printed auxetic honeycomb sandwich panel using CFRP face sheet. Int. J. Impact Eng. 2022, 164, 104186. [Google Scholar] [CrossRef]
- Yang, C.; Vora, H.D.; Chang, Y. Behavior of auxetic structures under compression and impact forces. Smart Mater. Struct. 2018, 27, 025012. [Google Scholar] [CrossRef]
- Hou, S.; Li, T.; Jia, Z.; Wang, L. Mechanical properties of sandwich composites with 3d-printed auxetic and non-auxetic lattice cores under low velocity impact. Mater. Des. 2018, 160, 1305–1321. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Z.; Xiao, D.; Wu, W.; Fang, D. The Dynamic response of shallow sandwich arch with auxetic metallic honeycomb core under localized impulsive loading. Int. J. Impact Eng. 2020, 137, 103442. [Google Scholar] [CrossRef]
- Usta, F.; Türkmen, H.S.; Scarpa, F. Low-velocity impact resistance of composite sandwich panels with various types of auxetic and non-auxetic core structures. Thin-Walled Struct. 2021, 163, 107738. [Google Scholar] [CrossRef]
- Jin, X.; Wang, Z.; Ning, J.; Xiao, G.; Liu, E.; Shu, X. Dynamic response of sandwich structures with graded auxetic honeycomb cores under blast loading. Compos. Part B Eng. 2016, 106, 206–217. [Google Scholar] [CrossRef]
- Qi, C.; Remennikov, A.; Pei, L.Z.; Yang, S.; Yu, Z.H.; Ngo, T.D. Impact and close-in blast response of auxetic honeycomb-cored sandwich panels: Experimental tests and numerical simulations. Compos. Struct. 2017, 180, 161–178. [Google Scholar] [CrossRef]
- Imbalzano, G.; Linforth, S.; Ngo, T.D.; Lee, P.V.S.; Tran, P. Blast resistance of auxetic and honeycomb sandwich panels: Comparisons and parametric designs. Compos. Struct. 2018, 183, 242–261. [Google Scholar] [CrossRef]
- Qi, C.; Yang, S.; Wang, D.; Yang, L.J. Ballistic resistance of honeycomb sandwich panels under in-plane high-velocity impact. Sci. World J. 2013, 2013, 892781. [Google Scholar] [CrossRef]
- Wang, Y.; Yu, Y.; Wang, C.; Zhou, G.; Karamoozian, A.; Zhao, W. On the out-of-plane ballistic performances of hexagonal, reentrant, square, triangular and circular honeycomb panels. Int. J. Mech. Sci. 2020, 173, 105402. [Google Scholar] [CrossRef]
- Bohara, R.P.; Linforth, S.; Nguyen, T.; Ghazlan, A.; Ngo, T. Anti-blast and-impact performances of auxetic structures: A review of structures, materials, methods, and fabrications. Eng. Struct. 2023, 276, 115377. [Google Scholar] [CrossRef]
- Lakes, R. Foam structures with a negative Poisson’s ratio. Science 1987, 235, 1038–1040. [Google Scholar] [CrossRef]
- Mardling, P.; Alderson, A.; Jordan-Mahy, N.; Le Maitre, C.L. The use of auxetic materials in tissue engineering. Biomater. Sci. 2020, 8, 2074–2083. [Google Scholar] [CrossRef] [PubMed]
- Critchley, R.; Corni, I.; Wharton, J.A.; Walsh, F.C.; Wood, R.J.; Stokes, K.R. A review of the manufacture, mechanical properties and potential applications of auxetic foams. Phys. Status Solidi 2013, 250, 1963–1982. [Google Scholar] [CrossRef]
- Love, A. A Treatise on the Mathematical Theory of Elasticity; Cambridge University Press: Cambridge, UK, 1892. [Google Scholar]
- Greenhill, A. A treatise on the mathematical theory of elasticity. Nature 1893, 47, 529–530. [Google Scholar] [CrossRef]
- Voigt, W. Bestimmung der Elasticitätsconstanten für das chlorsaure Natron. Ann. Phys. 1893, 285, 719–723. [Google Scholar] [CrossRef]
- Gunton, D.; Saunders, G. The Young’s modulus and Poisson’s ratio of arsenic, antimony and bismuth. J. Mater. Sci. 1972, 7, 1061–1068. [Google Scholar] [CrossRef]
- Li, Y. The anisotropic behavior of Poisson’s ratio, Young’s modulus, and shear modulus in hexagonal materials. Phys. Status Solidi 1976, 38, 171–175. [Google Scholar] [CrossRef]
- Yeganeh-Haeri, A.; Weidner, D.J.; Parise, J.B. Elasticity of α-cristobalite: A silicon dioxide with a negative Poisson’s ratio. Science 1992, 257, 650–652. [Google Scholar] [CrossRef]
- Baughman, R.H.; Shacklette, J.M.; Zakhidov, A.A.; Stafström, S. Negative Poisson’s ratios as a common feature of cubic metals. Nature 1998, 392, 362–365. [Google Scholar] [CrossRef]
- Veronda, D.; Westmann, R. Mechanical characterization of skin—Finite deformations. J. Biomech. 1970, 3, 111–124. [Google Scholar] [CrossRef]
- Lees, C.; Vincent, J.F.; Hillerton, J.E. Poisson’s ratio in skin. Bio-Med. Mater. Eng. 1991, 1, 19–23. [Google Scholar] [CrossRef]
- Frolich, L.; LaBarbera, M.; Stevens, W. Poisson’s ratio of a crossed fibre sheath: The skin of aquatic salamanders. J. Zool. 1994, 232, 231–252. [Google Scholar] [CrossRef]
- Williams, J.; Lewis, J. Properties and an anisotropic model of cancellous bone from the proximal tibial epiphysis. J. Biomech. Eng. 1982, 104, 50–56. [Google Scholar] [CrossRef]
- Sanami, M.; Ravirala, N.; Alderson, K.; Alderson, A. Auxetic materials for sports applications. Procedia Eng. 2014, 72, 453–458. [Google Scholar] [CrossRef]
- Duncan, O.; Shepherd, T.; Moroney, C.; Foster, L.; Venkatraman, P.D.; Winwood, K.; Allen, T.; Alderson, A. Review of auxetic materials for sports applications: Expanding options in comfort and protection. Appl. Sci. 2018, 8, 941. [Google Scholar] [CrossRef]
- Du, L.; Luo, S.; Xu, Y. Understanding nonlinear behaviors of auxetic foams using X-ray tomography and pore structure analysis. Mech. Mater. 2022, 165, 104196. [Google Scholar] [CrossRef]
- Zhang, Q.; Scarpa, F.; Barton, D.; Zhu, Y.; Lang, Z.Q.; Zhang, D.; Peng, H.X. Impact properties of uniaxially thermoformed auxetic foams. Int. J. Impact Eng. 2022, 163, 104176. [Google Scholar] [CrossRef]
- Nazari, Z.; Ahmadi, H.; Liaghat, G.; Vahid, S. Investigation on the compressive properties of auxetic foams under different loading rates. Polym. Eng. Sci. 2022, 62, 1720–1730. [Google Scholar] [CrossRef]
- Shah, I.A.; Khan, R.; Koloor, S.S.R.; Petru, M.; Badshah, S.; Ahmad, S.; Amjad, M. Finite Element Analysis of the Ballistic Impact on Auxetic Sandwich Composite Human Body Armor. Materials 2022, 15, 2064. [Google Scholar] [CrossRef]
- Hassanin, H.; Abena, A.; Elsayed, M.A.; Essa, K. 4D printing of NiTi auxetic structure with improved ballistic performance. Micromachines 2020, 11, 745. [Google Scholar] [CrossRef]
- Imbalzano, G.; Tran, P.; Ngo, T.D.; Lee, P.V. Three-dimensional modelling of auxetic sandwich panels for localised impact resistance. J. Sandw. Struct. Mater. 2017, 19, 291–316. [Google Scholar] [CrossRef]
- Scarpa, F. Auxetic materials for bioprostheses [In the Spotlight]. IEEE Signal Process. Mag. 2008, 25. [Google Scholar] [CrossRef]
- Kolken, H.M.; Janbaz, S.; Leeflang, S.M.; Lietaert, K.; Weinans, H.H.; Zadpoor, A.A. Rationally designed meta-implants: A combination of auxetic and conventional meta-biomaterials. Mater. Horizons 2018, 5, 28–35. [Google Scholar] [CrossRef]
- Zadpoor, A.A. Mechanical performance of additively manufactured meta-biomaterials. Acta Biomater. 2019, 85, 41–59. [Google Scholar] [CrossRef] [PubMed]
- Dusfour, G.; LeFloc’h, S.; Cañadas, P.; Ambard, D. Heterogeneous mechanical hyperelastic behavior in the porcine annulus fibrosus explained by fiber orientation: An experimental and numerical approach. J. Mech. Behav. Biomed. Mater. 2020, 104, 103672. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Yu, W.R. Development of a textile structure for multi-directional auxetic deformation. Mater. Des. 2022, 223, 111237. [Google Scholar] [CrossRef]
- Shah, A.A.; Shahid, M.; Siddiqui, N.A.; Nawab, Y.; Iqbal, M. Effect of Geometric Arrangement on Mechanical Properties of 2D Woven Auxetic Fabrics. Textiles 2022, 2, 606–623. [Google Scholar] [CrossRef]
- Yuping, C.; Liu, Y.; Hong, H. Deformation behavior of auxetic laminated fabrics with rotating square geometry. Text. Res. J. 2022, 92, 4652–4665. [Google Scholar] [CrossRef]
- Shen, H.S.; Xiang, Y. Assessment of negative Poisson’s ratio effect on thermal post-buckling of FG-GRMMC laminated cylindrical panels. Adv. Nano Res. 2021, 10, 423–435. [Google Scholar]
- Kolken, H.; De Jonge, C.; Van der Sloten, T.; Garcia, A.F.; Pouran, B.; Willemsen, K.; Weinans, H.; Zadpoor, A. Additively manufactured space-filling meta-implants. Acta Biomater. 2021, 125, 345–357. [Google Scholar] [CrossRef]
- Muslov, S. Characteristics of elastic anisotropy of Ti-Ni crystals with shape memory and their relation to extreme values of the Poisson’s ratio. Lett. Mater. 2021, 11, 28–32. [Google Scholar] [CrossRef]
- Guo, L.; Zhao, S.; Guo, Y.; Yang, J.; Kitipornchai, S. Bandgaps in functionally graded phononic crystals containing graphene origami-enabled metamaterials. Int. J. Mech. Sci. 2023, 240, 107956. [Google Scholar] [CrossRef]
- Liao, M.E.; Li, C.; Shah, N.; Hsiao, Y.H.; Bauchy, M.; Sant, G.; Goorsky, M.S. Experimental evidence of auxeticity in ion implanted single crystal calcite. Sci. Rep. 2022, 12, 6071. [Google Scholar] [CrossRef]
- Jiang, Z.; Xu, N. Emergent Physics on the Route from Crystals to Amorphous Solids. Chin. J. Chem. 2022, 40, 1091–1098. [Google Scholar] [CrossRef]
- Amaya-Amaya, V.; de Icaza-Herrera, M.; Martínez-Hernández, A.L.; Martínez-Barrera, G.; Velasco-Santos, C. Experimental approximation of the sound absorption coefficient (∝) for 3D printed reentrant auxetic structures of poly lactic acid reinforced with chicken keratin materials. Mater. Lett. 2021, 283, 128757. [Google Scholar] [CrossRef]
- Magesh, M.; Jawahar, P. Examination of shape memory polymer-auxetic landing gears on landing approach for quadcopter. Mater. Today Proc. 2021, 47, 471–479. [Google Scholar] [CrossRef]
- Verma, P.; He, C.; Griffin, A.C. Implications for Auxetic Response in Liquid Crystalline Polymers: X-Ray Scattering and Space-Filling Molecular Modeling. Phys. Status Solidi 2020, 257, 2000261. [Google Scholar] [CrossRef]
- Zhang, J.; Dong, B.; Zhang, W. Dynamic Crushing of Gradient Auxetic Honeycombs. J. Vib. Eng. Technol. 2021, 9, 421–431. [Google Scholar] [CrossRef]
- Tang, H.; Jiang, X.; Li, L.; Ling, L.; Hu, Y. Electromechanical properties of ultra-low porous auxetic piezocomposite: From the perspective of Poisson’s ratio. J. Am. Ceram. Soc. 2021, 104, 2628–2645. [Google Scholar] [CrossRef]
- Lantada, A.D.; de Blas Romero, A.; Schwentenwein, M.; Jellinek, C.; Homa, J. Lithography-based ceramic manufacture (LCM) of auxetic structures: Present capabilities and challenges. Smart Mater. Struct. 2016, 25, 054015. [Google Scholar] [CrossRef]
- Zhang, X.G.; Jiang, W.; Zhang, Y.; Luo, C.; Zhang, X.Y.; Han, D.; Hao, J.; Teng, X.C.; Xie, Y.M.; Ren, X. Energy absorption properties of composite tubes with hexagonal and re-entrant honeycomb fillers. Constr. Build. Mater. 2022, 356, 129298. [Google Scholar] [CrossRef]
- Wu, L.; Zhao, F.; Lu, Z.; Lin, J.H.; Jiang, Q. Impact energy absorption composites with shear stiffening gel-filled negative poisson’s ratio skeleton by Kirigami method. Compos. Struct. 2022, 298, 116009. [Google Scholar] [CrossRef]
- Li, Z.Y.; Wang, X.T.; Ma, L.; Wu, L.Z. Study on the mechanical properties of CFRP composite auxetic structures consist of corrugated sheets and tubes. Compos. Struct. 2022, 292, 115655. [Google Scholar] [CrossRef]
- Jiang, F.; Yang, S.; Zhang, Y.; Qi, C.; Chen, S. Fabrication and crushing response of graded re-entrant circular auxetic honeycomb. Int. J. Mech. Sci. 2023, 242, 107999. [Google Scholar] [CrossRef]
- Reyes, G.; Ajdary, R.; Kankuri, E.; Kaschuk, J.J.; Kosonen, H.; Rojas, O.J. Cellulose gelation in NaOH (aq) by CO2 absorption: Effects of holding time and concentration on biomaterial development. Carbohydr. Polym. 2023, 302, 120355. [Google Scholar] [CrossRef] [PubMed]
- Zhao, C.; Goh, K.L.; Lee, H.P.; Yin, C.; Zhang, K.; Zhong, J. Experimental study and finite element analysis on energy absorption of carbon fiber reinforced composite auxetic structures filled with aluminum foam. Compos. Struct. 2023, 303, 116319. [Google Scholar] [CrossRef]
- Chen, X.Y.; Hamdi, O.; Rodrigue, D. Conversion of low density polyethylene foams into auxetic metamaterials. Polym. Adv. Technol. 2022, 34, 228–237. [Google Scholar] [CrossRef]
- Xue, B.Z.; Li, J.W.; Huang, R.; Yang, Y.Z.; Gong, H.S.; Zhang, Q.M.; Kong, L.H.; Li, D. A Study of Negative Poisson’s Ratio of 3D Printed Auxetic Structures. Mech. Solids 2022, 57, 1–10. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, Y.; Jiang, D.; Chen, Y.; Xie, Y.M.; Jia, L.J. In-plane impact behavior of 3D-printed auxetic stainless honeycombs. Eng. Struct. 2022, 266, 114656. [Google Scholar] [CrossRef]
- Fan, Y.; Shen, H.S. Non-symmetric stiffness of origami-graphene metamaterial plates. Compos. Struct. 2022, 297, 115974. [Google Scholar] [CrossRef]
- Köllner, D.; Simon, S.; Niedermeyer, S.; Spath, I.; Wolf, E.; Kakimoto, K.i.; Fey, T. Relation between Structure, Mechanical and Piezoelectric Properties in Cellular Ceramic Auxetic and Honeycomb Structures. Adv. Eng. Mater. 2022, 25, 2201387. [Google Scholar] [CrossRef]
- Fan, G.Z.; Ren, X.; Wang, S.L.; Luo, C.; Xie, Y.M. A novel cement-based auxetic foam composite: Experimental study. Case Stud. Constr. Mater. 2022, 17, e01159. [Google Scholar] [CrossRef]
- Yolcu, D.A.; Baba, B.O. Measurement of Poisson’s ratio of the auxetic structure. Measurement 2022, 204, 112040. [Google Scholar] [CrossRef]
- Li, P.; Xu, Y.; Liang, C.; Zeng, X.C. MgXN2 (X = Hf/Zr) Monolayers: Auxetic Semiconductor with Highly Anisotropic Optical/Mechanical Properties and Carrier Mobility. J. Phys. Chem. Lett. 2022, 13, 10534–10542. [Google Scholar] [CrossRef]
- Fan, D.; Li, N.; Li, M.; Wang, S.; Li, S.; Tang, T. Polyurethane/polydopamine/graphene auxetic composite foam with high-efficient and tunable electromagnetic interference shielding performance. Chem. Eng. J. 2022, 427, 131635. [Google Scholar] [CrossRef]
- Choi, J.; Lakes, R. Nonlinear properties of metallic cellular materials with a negative Poisson’s ratio. J. Mater. Sci. 1992, 27, 5375–5381. [Google Scholar] [CrossRef]
- Argatov, I.I.; Guinovart-Díaz, R.; Sabina, F.J. On local indentation and impact compliance of isotropic auxetic materials from the continuum mechanics viewpoint. Int. J. Eng. Sci. 2012, 54, 42–57. [Google Scholar] [CrossRef]
- Coenen, V.; Alderson, K. Mechanisms of failure in the static indentation resistance of auxetic carbon fibre laminates. Phys. Status Solidi 2011, 248, 66–72. [Google Scholar] [CrossRef]
- Choi, J.; Lakes, R. Fracture toughness of re-entrant foam materials with a negative Poisson’s ratio: Experiment and analysis. Int. J. Fract. 1996, 80, 73–83. [Google Scholar] [CrossRef]
- Alderson, A.; Rasburn, J.; Ameer-Beg, S.; Mullarkey, P.G.; Perrie, W.; Evans, K.E. An auxetic filter: A tuneable filter displaying enhanced size selectivity or defouling properties. Ind. Eng. Chem. Res. 2000, 39, 654–665. [Google Scholar] [CrossRef]
- Francisco, M.B.; Pereira, J.L.J.; Oliver, G.A.; Roque da Silva, L.R.; Cunha, S.S., Jr.; Gomes, G.F. A review on the energy absorption response and structural applications of auxetic structures. Mech. Adv. Mater. Struct. 2022, 29, 5823–5842. [Google Scholar] [CrossRef]
- Gao, Q.; Liao, W.H. Energy absorption of thin walled tube filled with gradient auxetic structures-theory and simulation. Int. J. Mech. Sci. 2021, 201, 106475. [Google Scholar] [CrossRef]
- Bohara, R.P.; Linforth, S.; Nguyen, T.; Ghazlan, A.; Ngo, T. Novel lightweight high-energy absorbing auxetic structures guided by topology optimisation. Int. J. Mech. Sci. 2021, 211, 106793. [Google Scholar] [CrossRef]
- Evans, K.E.; Alderson, A. Auxetic materials: Functional materials and structures from lateral thinking! Adv. Mater. 2000, 12, 617–628. [Google Scholar] [CrossRef]
- Prawoto, Y. Seeing auxetic materials from the mechanics point of view: A structural review on the negative Poisson’s ratio. Comput. Mater. Sci. 2012, 58, 140–153. [Google Scholar] [CrossRef]
- Carneiro, V.H.; Meireles, J.; Puga, H. Auxetic materials—A review. Mater. Sci. Poland 2013, 31, 561–571. [Google Scholar] [CrossRef]
- Wallbanks, M.; Khan, M.F.; Bodaghi, M.; Triantaphyllou, A.; Serjouei, A. On the design workflow of auxetic metamaterials for structural applications. Smart Mater. Struct. 2021, 31, 023002. [Google Scholar] [CrossRef]
- Liu, Q. Literature Review: Materials with Negative Poisson’s Ratios and Potential Applications to Aerospace and Defence; Defence Science and Technology Organisation: Victoria, Australia, 2006. [Google Scholar]
- Tretiakov, K.V.; Wojciechowski, K.W. Elasticity of two-dimensional crystals of polydisperse hard disks near close packing: Surprising behavior of the Poisson’s ratio. J. Chem. Phys. 2012, 136, 204506. [Google Scholar] [CrossRef]
- Narojczyk, J.; Alderson, A.; Imre, A.; Scarpa, F.; Wojciechowski, K. Negative Poisson’s ratio behavior in the planar model of asymmetric trimers at zero temperature. J. Non-Cryst. Solids 2008, 354, 4242–4248. [Google Scholar] [CrossRef]
- Yang, W.; Li, Z.M.; Shi, W.; Xie, B.H.; Yang, M.B. Review on auxetic materials. J. Mater. Sci. 2004, 39, 3269–3279. [Google Scholar] [CrossRef]
- Xinchun, S.; Lakes, R.S. Stability of elastic material with negative stiffness and negative Poisson’s ratio. Phys. Status Solidi 2007, 244, 1008–1026. [Google Scholar] [CrossRef]
- Donoghue, J.; Alderson, K.; Evans, K. The fracture toughness of composite laminates with a negative Poisson’s ratio. Phys. Status Solidi 2009, 246, 2011–2017. [Google Scholar] [CrossRef]
- Maiti, S.; Ashby, M.; Gibson, L. Fracture toughness of brittle cellular solids. Scr. Metall. 1984, 18, 213–217. [Google Scholar] [CrossRef]
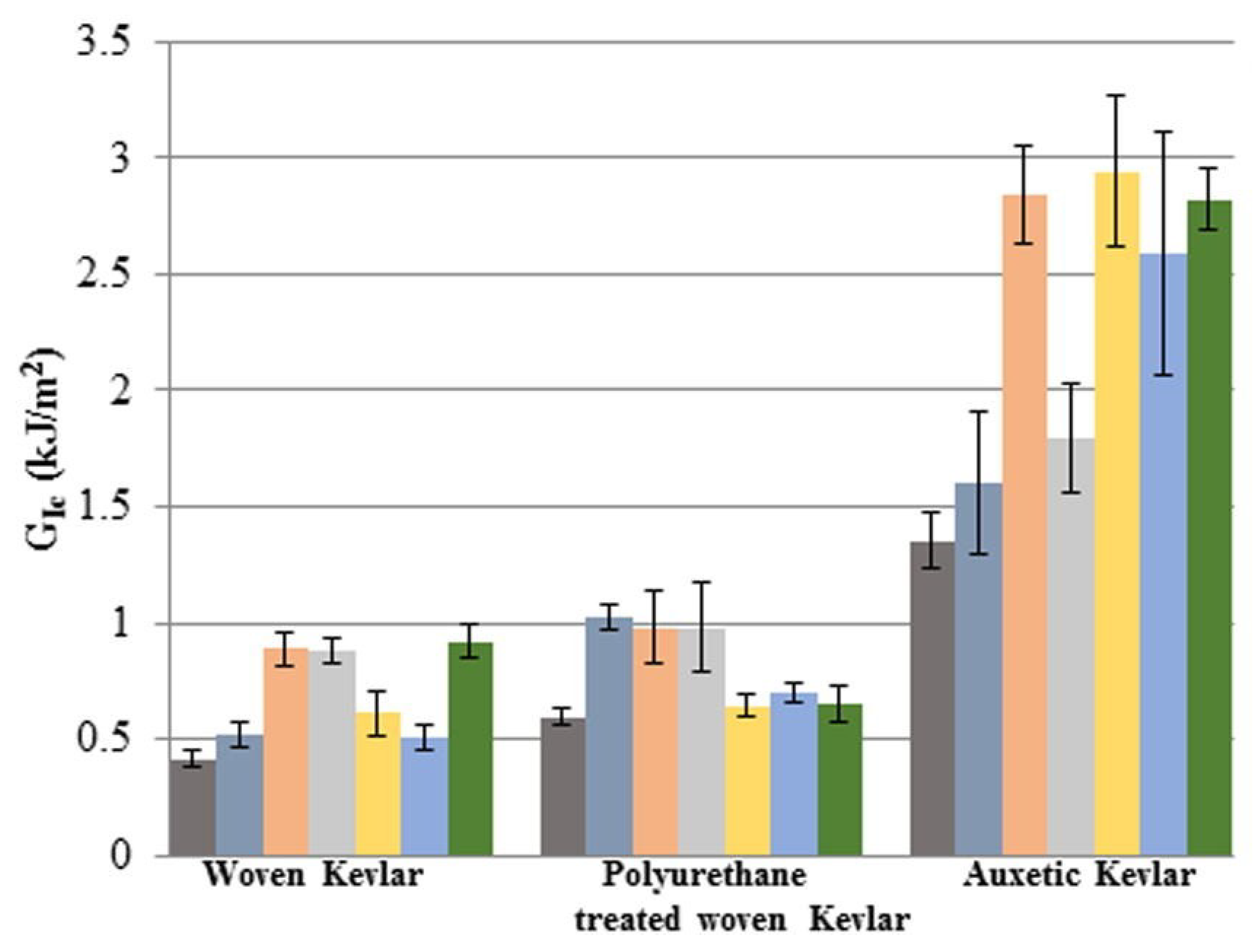
- Yang, S.; Chalivendra, V.B.; Kim, Y.K. Fracture and impact characterization of novel auxetic Kevlar®/Epoxy laminated composites. Compos. Struct. 2017, 168, 120–129. [Google Scholar] [CrossRef]
- Scarpa, F.; Yates, J.; Ciffo, L.; Patsias, S. Dynamic crushing of auxetic open-cell polyurethane foam. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2002, 216, 1153–1156. [Google Scholar] [CrossRef]
- Oh, J.H.; Kim, J.S.; Oh, I.K. Auxetic graphene oxide-porous foam for acoustic wave and shock energy dissipation. Compos. Part B Eng. 2020, 186, 107817. [Google Scholar] [CrossRef]
- Najafi, M.; Ahmadi, H.; Liaghat, G. Experimental investigation on energy absorption of auxetic structures. Mater. Today Proc. 2021, 34, 350–355. [Google Scholar] [CrossRef]
- Grima, J. Modelling Auxetic Materials. In Proceedings of the 2009 Computational Nanotechnology Intensive Programme (CoNan 2009, EU Life-Long Learning Programme), Gdansk, Poland, 2–14 August 2009. [Google Scholar]
- Chen, Y.; Scarpa, F.; Farrow, I.R.; Liu, Y.; Leng, J. Composite flexible skin with large negative Poisson’s ratio range: Numerical and experimental analysis. Smart Mater. Struct. 2013, 22, 045005. [Google Scholar] [CrossRef]
- Veerabagu, U.; Palza, H.; Quero, F. Auxetic polymer-based mechanical metamaterials for biomedical applications. ACS Biomater. Sci. Eng. 2022, 8, 2798–2824. [Google Scholar] [CrossRef]
- Kim, Y.; Son, K.H.; Lee, J.W. Auxetic structures for tissue engineering scaffolds and biomedical devices. Materials 2021, 14, 6821. [Google Scholar] [CrossRef]
- Xue, H.; Luo, Z.; Brown, T.; Beier, S. Design of self-expanding auxetic stents using topology optimization. Front. Bioeng. Biotechnol. 2020, 8, 736. [Google Scholar] [CrossRef]
- Asadi, A.; Hedayat, D.; Ghofrani, S.; Abouei Mehrizi, A.; Shadalooyi, G.; Kadkhodapour, J.; Pourkamali Anaraki, A. Modification of hexachiral unit cell to enhance auxetic stent performance. Mech. Adv. Mater. Struct. 2022, 1–15. [Google Scholar] [CrossRef]
- Alderson, A.; Rasburn, J.; Evans, K.; Grima, J. Auxetic polymeric filters display enhanced de-fouling and pressure compensation properties. Membr. Technol. 2001, 2001, 6–8. [Google Scholar] [CrossRef]
- Dolla, W.J.S.; Fricke, B.A.; Becker, B.R. Structural and drug diffusion models of conventional and auxetic drug-eluting stents. J. Med. Devices. 2007, 1, 47–55. [Google Scholar] [CrossRef]
- Shirdel, M.; Ghofrani, S.; Mehrizi, A.A. Effect of increasing porosity as drug reservoirs for local drug delivery in an auxetic stent. In Proceedings of the 2021 28th National and 6th International Iranian Conference on Biomedical Engineering (ICBME), Tehran, Iran, 25–26 November 2021; pp. 65–69. [Google Scholar]
- Wang, Z.; Luan, C.; Liao, G.; Liu, J.; Yao, X.; Fu, J. Progress in auxetic mechanical metamaterials: Structures, characteristics, manufacturing methods, and applications. Adv. Eng. Mater. 2020, 22, 2000312. [Google Scholar]
- Otsuka, K.; Wayman, C.M. Shape Memory Materials; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Huang, W.; Ding, Z.; Wang, C.; Wei, J.; Zhao, Y.; Purnawali, H. Shape memory materials. Mater. Today 2010, 13, 54–61. [Google Scholar] [CrossRef]
- Le Sénéchal, N.V.; Teixeira, R.; Rodrigues, P.F.; Ribeiro, S.B.; dos Santos Paula, A. The influence of aging treatment on the microstructural and mechanical behavior by ultra-micro hardness tester in Ni-rich NiTi alloy. Cadernos UniFOA 2021, 16, 3810. [Google Scholar] [CrossRef]
- Freitas Rodrigues, P.; Teixeira, R.S.; Le Sénéchal, N.V.; Braz Fernandes, F.M.; Paula, A.S. The Influence of the Soaking Temperature Rotary Forging and Solution Heat Treatment on the Structural and Mechanical Behavior in Ni-Rich NiTi Alloy. Materials 2021, 15, 63. [Google Scholar] [CrossRef]
- Song, D.; Yu, C.; Zhang, C.; Kang, G. Superelasticity degradation of NiTi shape memory alloy in wide ranges of temperature and loading level: Experimental observation and micromechanical constitutive model. Int. J. Plast. 2023, 161, 103487. [Google Scholar] [CrossRef]
- Liu, H.; Wang, F.; Wu, W.; Dong, X.; Sang, L. 4D printing of mechanically robust PLA/TPU/Fe3O4 magneto-responsive shape memory polymers for smart structures. Compos. Part B Eng. 2023, 248, 110382. [Google Scholar] [CrossRef]
- Idowu, A.; Thomas, T.; Boesl, B.; Agarwal, A. Cryo-Assisted Extrusion Three-Dimensional Printing of Shape Memory Polymer–Graphene Composites. J. Manuf. Sci. Eng. 2023, 145, 041003. [Google Scholar]
- Lotfolahpour, A.; Huber, W.; Zaeem, M.A. A phase-field model for interactive evolution of phase transformation and cracking in superelastic shape memory ceramics. Comput. Mater. Sci. 2023, 216, 111844. [Google Scholar] [CrossRef]
- Bianchi, M.; Scarpa, F.; Smith, C.; Whittell, G.R. Physical and thermal effects on the shape memory behaviour of auxetic open cell foams. J. Mater. Sci. 2010, 45, 341–347. [Google Scholar] [CrossRef]
- Kamyab, A.; Ghasemi-Ghalebahman, A.; Fereidoon, A.; Khonakdar, H.A. Investigation into the shape memory behavior of peanut-pattern auxetic structures. Express Polym. Lett. 2022, 16, 679–693. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F.; Schajer, G.; Robertson, C. The mechanics of two-dimensional cellular materials. Proc. R. Soc. Lond. A Math. Phys. Sci. 1982, 382, 25–42. [Google Scholar]
- Bronder, S.; Herter, F.; Röhrig, A.; Bähre, D.; Jung, A. Design Study for Multifunctional 3D Re-entrant Auxetics. Adv. Eng. Mater. 2022, 24, 2100816. [Google Scholar] [CrossRef]
- Grima, J.N.; Evans, K.E. Auxetic Behavior from Rotating Squares; Kluwer Academic Publishers: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Grima, J.N.; Evans, K.E. Auxetic behavior from rotating triangles. J. Mater. Sci. 2006, 41, 3193–3196. [Google Scholar] [CrossRef]
- Alderson, A.; Evans, K.E. Molecular origin of auxetic behavior in tetrahedral framework silicates. Phys. Rev. Lett. 2002, 89, 225503. [Google Scholar] [CrossRef]
- Grima, J.N.; Alderson, A.; Evans, K. Auxetic behaviour from rotating rigid units. Phys. Status Solidi 2005, 242, 561–575. [Google Scholar] [CrossRef]
- Wang, Z.; Hu, H. Auxetic materials and their potential applications in textiles. Text. Res. J. 2014, 84, 1600–1611. [Google Scholar] [CrossRef]
- Grima, J.N.; Gatt, R.; Alderson, A.; Evans, K.E. On the auxetic properties of ‘rotating rectangles’ with different connectivity. J. Phys. Soc. Jpn. 2005, 74, 2866–2867. [Google Scholar] [CrossRef]
- Grima, J.N.; Farrugia, P.S.; Gatt, R.; Attard, D. On the auxetic properties of rotating rhombi and parallelograms: A preliminary investigation. Phys. Status Solidi 2008, 245, 521–529. [Google Scholar] [CrossRef]
- Prall, D.; Lakes, R. Properties of a chiral honeycomb with a Poisson’s ratio of—1. Int. J. Mech. Sci. 1997, 39, 305–314. [Google Scholar] [CrossRef]
- Grima, J.N.; Gatt, R.; Farrugia, P.S. On the properties of auxetic meta-tetrachiral structures. Phys. Status Solidi 2008, 245, 511–520. [Google Scholar] [CrossRef]
- Alderson, A.; Evans, K. Microstructural modelling of auxetic microporous polymers. J. Mater. Sci. 1995, 30, 3319–3332. [Google Scholar] [CrossRef]
- Cho, H.; Seo, D.; Kim, D.N. Mechanics of Auxetic Materials. In Handbook of Mechanics of Materials; Schmauder, S., Chen, C.S., Chawla, K.K., Chawla, N., Chen, W., Kagawa, Y., Eds.; Springer: Singapore, 2019; pp. 733–757. [Google Scholar] [CrossRef]
- He, C.; Liu, P.; Griffin, A.C. Toward negative Poisson ratio polymers through molecular design. Macromolecules 1998, 31, 3145–3147. [Google Scholar] [CrossRef]
- Ravirala, N.; Alderson, A.; Alderson, K. Interlocking hexagons model for auxetic behaviour. J. Mater. Sci. 2007, 42, 7433–7445. [Google Scholar] [CrossRef]
- Etemadi, E.; Gholikord, M.; Zeeshan, M.; Hu, H. Improved mechanical characteristics of new auxetic structures based on stretch-dominated-mechanism deformation under compressive and tensile loadings. Thin-Walled Struct. 2023, 184, 110491. [Google Scholar] [CrossRef]
- Lu, C.; Hsieh, M.; Huang, Z.; Zhang, C.; Lin, Y.; Shen, Q.; Chen, F.; Zhang, L. Architectural Design and Additive Manufacturing of Mechanical Metamaterials: A Review. Engineering 2022, 17, 44–63. [Google Scholar] [CrossRef]
- Jacobsen, A.J.; Barvosa-Carter, W.; Nutt, S. Micro-scale truss structures formed from self-propagating photopolymer waveguides. Adv. Mater. 2007, 19, 3892–3896. [Google Scholar] [CrossRef]
- Erdeniz, D.; Schaedler, T.A.; Dunand, D.C. Deposition-based synthesis of nickel-based superalloy microlattices. Scr. Mater. 2017, 138, 28–31. [Google Scholar] [CrossRef]
- Bauer, J.; Meza, L.R.; Schaedler, T.A.; Schwaiger, R.; Zheng, X.; Valdevit, L. Nanolattices: An emerging class of mechanical metamaterials. Adv. Mater. 2017, 29, 1701850. [Google Scholar] [CrossRef]
- Jacobsen, A.; Kolodziejska, J.; Doty, R.; Fink, K.; Zhou, C.; Roper, C.; Carter, W. Interconnected self-propagating photopolymer waveguides: An alternative to stereolithography for rapid formation of lattice-based open-cellular materials. In Proceedings of the 2010 International Solid Freeform Fabrication Symposium, Austin, TX, USA, 9–11 August 2010. [Google Scholar]
- ISO/ASTM 52900; Additive Manufacturing—General Principles—Fundamentals and Vocabulary. International Organization for Standardization: Geneva, Switzeland, 2021.
- Gibson, I.; Rosen, D.W.; Stucker, B.; Khorasani, M.; Rosen, D.; Stucker, B.; Khorasani, M. Additive Manufacturing Technologies; Springer: Berlin/Heidelberg, Germany, 2021; Volume 17. [Google Scholar]
- Choi, J.W.; MacDonald, E.; Wicker, R. Multi-material microstereolithography. Int. J. Adv. Manuf. Technol. 2010, 49, 543–551. [Google Scholar] [CrossRef]
- Bertsch, A.; Renaud, P. Microstereolithography. In Three-Dimensional Microfabrication Using Two-Photon Polymerization; Elsevier: Amsterdam, The Netherlands, 2020; pp. 25–56. [Google Scholar]
- Sun, Q.; Fang, F.; Wang, W.; Yin, J.; Liu, Q.; Hao, L.; Peng, Y. Stereolithography 3D printing of transparent resin lens for high-power phosphor-coated WLEDs packaging. J. Manuf. Process. 2023, 85, 756–763. [Google Scholar] [CrossRef]
- Kim, K.; Yeatts, A.; Dean, D.; Fisher, J.P. Stereolithographic bone scaffold design parameters: Osteogenic differentiation and signal expression. Tissue Eng. Part B Rev. 2010, 16, 523–539. [Google Scholar] [CrossRef]
- Mondschein, R.J.; Kanitkar, A.; Williams, C.B.; Verbridge, S.S.; Long, T.E. Polymer structure-property requirements for stereolithographic 3D printing of soft tissue engineering scaffolds. Biomaterials 2017, 140, 170–188. [Google Scholar] [CrossRef]
- Deng, X.; Zhang, G.; Yu, Z.; Shao, G.; Li, L. Manufacturing of mesoscale non-assembly mechanism with water-soluble support in projection stereolithography process. J. Manuf. Process. 2023, 85, 658–665. [Google Scholar] [CrossRef]
- Alomarah, A.; Ruan, D.; Masood, S.; Sbarski, I.; Faisal, B. An investigation of in-plane tensile properties of re-entrant chiral auxetic structure. Int. J. Adv. Manuf. Technol. 2018, 96, 2013–2029. [Google Scholar] [CrossRef]
- Varas, D.; Pernas-Sánchez, J.; Fjeldberg, N.; Martín-Montal, J. Experimental analysis at different loading rates of 3D printed polymeric auxetic structure based on cylindrical elements. Polym. Test. 2023, 119, 107930. [Google Scholar] [CrossRef]
- Von Freymann, G.; Ledermann, A.; Thiel, M.; Staude, I.; Essig, S.; Busch, K.; Wegener, M. Three-dimensional nanostructures for photonics. Adv. Funct. Mater. 2010, 20, 1038–1052. [Google Scholar] [CrossRef]
- Kunwar, P.; Soman, P. Direct laser writing of fluorescent silver nanoclusters: A review of methods and applications. ACS Appl. Nano Mater. 2020, 3, 7325–7342. [Google Scholar] [CrossRef]
- Wang, R.; Wei, J.; Fan, Y. Chalcogenide phase-change thin films used as grayscale photolithography materials. Opt. Express 2014, 22, 4973–4984. [Google Scholar] [CrossRef]
- Fischer, J.; Wegener, M. Three-dimensional direct laser writing inspired by stimulated-emission-depletion microscopy. Opt. Mater. Express 2011, 1, 614–624. [Google Scholar] [CrossRef]
- Fanyaeu, I.; Mizeikis, V. Realisation of 3D metamaterial perfect absorber structures by direct laser writing. In Proceedings of the Advanced Fabrication Technologies for Micro/Nano Optics and Photonics X, San Francisco, CA, USA, 29 January–1 February 2017; Volume 10115, pp. 177–185. [Google Scholar]
- Debnath, S.; Zhang, X.; Guney, D.; Soukoulis, C. Two-dimensionally isotropic optical metamaterial feasible for stimulated emission depletion microscopy inspired direct laser writing. In Proceedings of the 2013 7th International Congress on Advanced Electromagnetic Materials in Microwaves and Optics, Bordeaux, France, 16–21 September 2013; pp. 427–429. [Google Scholar]
- Melissinaki, V.; Gill, A.; Ortega, I.; Vamvakaki, M.; Ranella, A.; Haycock, J.; Fotakis, C.; Farsari, M.; Claeyssens, F. Direct laser writing of 3D scaffolds for neural tissue engineering applications. Biofabrication 2011, 3, 045005. [Google Scholar] [CrossRef] [PubMed]
- Selimis, A.; Mironov, V.; Farsari, M. Direct laser writing: Principles and materials for scaffold 3D printing. Microelectron. Eng. 2015, 132, 83–89. [Google Scholar] [CrossRef]
- Srikanth, S.; Dudala, S.; Raut, S.; Dubey, S.K.; Ishii, I.; Javed, A.; Goel, S. Optimization and characterization of direct UV laser writing system for microscale applications. J. Micromech. Microeng. 2020, 30, 095003. [Google Scholar] [CrossRef]
- Somers, N.; Jean, F.; Lasgorceix, M.; Preux, N.; Delmotte, C.; Boilet, L.; Petit, F.; Leriche, A. Fabrication of doped β-tricalcium phosphate bioceramics by Direct Ink Writing for bone repair applications. J. Eur. Ceram. Soc. 2023, 43, 629–638. [Google Scholar] [CrossRef]
- Samartzis, N.; Bhorkar, K.; Athanasiou, M.; Sygellou, L.; Dracopoulos, V.; Ioannides, T.; Yannopoulos, S.N. Direct laser-assisted fabrication of turbostratic graphene electrodes: Comparing symmetric and zinc-ion hybrid supercapacitors. Carbon 2023, 201, 941–951. [Google Scholar] [CrossRef]
- Zhan, T.; Gao, S.; Xu, B. Microfluidic cloth-based analytical devices through simple correction pen direct-writing. Sensors Actuators B Chem. 2023, 375, 132947. [Google Scholar] [CrossRef]
- Schmid, M.; Thiele, S.; Herkommer, A.; Giessen, H. Adjustment-free two-sided 3D direct laser writing for aligned micro-optics on both substrate sides. Opt. Lett. 2023, 48, 131–134. [Google Scholar] [CrossRef]
- Waller, E.H.; von Freymann, G. From photoinduced electron transfer to 3D metal microstructures via direct laser writing. Nanophotonics 2018, 7, 1259–1277. [Google Scholar] [CrossRef]
- Mao, M.; He, J.; Li, X.; Zhang, B.; Lei, Q.; Liu, Y.; Li, D. The emerging frontiers and applications of high-resolution 3D printing. Micromachines 2017, 8, 113. [Google Scholar] [CrossRef]
- Sola, A.; Trinchi, A.; Hill, A.J. Self-assembly meets additive manufacturing: Bridging the gap between nanoscale arrangement of matter and macroscale fabrication. Smart Mater. Manuf. 2023, 1, 100013. [Google Scholar] [CrossRef]
- Tareq, M.S.; Rahman, T.; Hossain, M.; Dorrington, P. Additive manufacturing and the COVID-19 challenges: An in-depth study. J. Manuf. Syst. 2021, 60, 787–798. [Google Scholar] [CrossRef]
- Choong, Y.Y.C.; Tan, H.W.; Patel, D.C.; Choong, W.T.N.; Chen, C.H.; Low, H.Y.; Tan, M.J.; Patel, C.D.; Chua, C.K. The global rise of 3D printing during the COVID-19 pandemic. Nat. Rev. Mater. 2020, 5, 637–639. [Google Scholar] [CrossRef]
- Colorado, H.A.; Mendoza, D.E.; Lin, H.T.; Gutierrez-Velasquez, E. Additive manufacturing against the Covid-19 pandemic: A technological model for the adaptability and networking. J. Mater. Res. Technol. 2022, 16, 1150–1164. [Google Scholar] [CrossRef]
- Soukoulis, C.M.; Wegener, M. Optical metamaterials—More bulky and less lossy. Science 2010, 330, 1633–1634. [Google Scholar] [CrossRef]
- Whitesides, G.M.; Grzybowski, B. Self-assembly at all scales. Science 2002, 295, 2418–2421. [Google Scholar] [CrossRef]
- Li, L.; Sun, R.; Zheng, R. Tunable morphology and functionality of multicomponent self-assembly: A review. Mater. Des. 2021, 197, 109209. [Google Scholar] [CrossRef]
- Kriksin, Y.A.; Neratova, I.V.; Khalatur, P.G.; Khokhlov, A.R. Pattern multiplication by template-guided self-assembly of cylinder-forming copolymers: Field-theoretic and particle-based simulations. Chem. Phys. Lett. 2010, 492, 103–108. [Google Scholar] [CrossRef]
- Li, L.; Wang, Q. Spontaneous self-assembly of silver nanoparticles into lamellar structured silver nanoleaves. Acs Nano 2013, 7, 3053–3060. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.H.; Wu, S.J.; Ho, C.C.; Su, W.F. Rational design of versatile self-assembly morphology of rod–coil block copolymer. Macromolecules 2013, 46, 2725–2732. [Google Scholar] [CrossRef]
- Stein, A.; Wright, G.; Yager, K.G.; Doerk, G.S.; Black, C.T. Selective directed self-assembly of coexisting morphologies using block copolymer blends. Nat. Commun. 2016, 7, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Suzuki, Y.; Cardone, G.; Restrepo, D.; Zavattieri, P.D.; Baker, T.S.; Tezcan, F.A. Self-assembly of coherently dynamic, auxetic, two-dimensional protein crystals. Nature 2016, 533, 369–373. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Qin, M.; Wang, C.; Yan, T.; Zhen, Y.; Sun, X.; Wang, J.; Fu, F. CeO2/MnOx@ C hollow cathode derived from self-assembly of Ce-Mn-MOFs for high-performance aqueous zinc-ion batteries. J. Colloid Interface Sci. 2023, 629, 733–743. [Google Scholar] [CrossRef]
- Wang, H.; Zhao, J.; Yu, J.; Wang, Z. Metal organic framework-derived hierarchical 0D/1D CoPC/CNTs architecture interlaminated in 2D MXene layers for superior absorption of electromagnetic waves. Synth. Met. 2023, 292, 117215. [Google Scholar] [CrossRef]
- Liu, C.; Liu, S.; Feng, X.; Zhu, K.; Lin, G.; Bai, Z.; Wang, L.; Liu, X. Phthalocyanine-mediated interfacial self-assembly of magnetic graphene nanocomposites toward low-frequency electromagnetic wave absorption. Chem. Eng. J. 2023, 452, 139483. [Google Scholar] [CrossRef]
- Waqar, S.; Guo, K.; Sun, J. FEM analysis of thermal and residual stress profile in selective laser melting of 316L stainless steel. J. Manuf. Process. 2021, 66, 81–100. [Google Scholar] [CrossRef]
- Bremen, S.; Meiners, W.; Diatlov, A. Selective laser melting: A manufacturing technology for the future? Laser Techn. J. 2012, 9, 33–38. [Google Scholar] [CrossRef]
- Korkmaz, M.E.; Gupta, M.K.; Robak, G.; Moj, K.; Krolczyk, G.M.; Kuntoğlu, M. Development of lattice structure with selective laser melting process: A state of the art on properties, future trends and challenges. J. Manuf. Process. 2022, 81, 1040–1063. [Google Scholar] [CrossRef]
- Tan, J.H.; Wong, W.L.E.; Dalgarno, K.W. An overview of powder granulometry on feedstock and part performance in the selective laser melting process. Addit. Manuf. 2017, 18, 228–255. [Google Scholar] [CrossRef]
- Vieira de Oliveira, R.; Pereira de Lima, Y.; Hoisler Sallet, E.; Abílio Corrêa Gonçalves, D.; Vieira Le Sénèchal, N.; Alves Oliveira Melo, E.; Teixeira, R.; Freitas Rodrigues, P.; Inforçatti Neto, P.; Vicente Lopes da Silva, J.; et al. Production of Cylindrical Specimens Based on the Ni-Ti System by Selective Laser Melting from Elementary Powders. J. Mater. Eng. Perform. 2021, 30, 5477–5490. [Google Scholar] [CrossRef]
- Park, H.S.; Nguyen, D.S. Study on flaking behavior in selective laser melting process. Procedia CIRP 2017, 63, 569–572. [Google Scholar] [CrossRef]
- Singh, D.; Tobin, D.; Dowling, L.; Trimble, D. Optimization of Cobalt Chrome 3D re-entrant Auxetics fabricated using Selective Laser Melting. Eng. Struct. 2023, 278, 115542. [Google Scholar] [CrossRef]
- Gao, M.; He, D.; Guo, X.; Wu, H.; Tan, Z.; Wu, X.; Shao, W.; Wang, G. Design, preparation and characterization of a 3D hierarchical auxetic lattice structure produced by selective laser melting. In Proceedings of the Structures; Elsevier: Amsterdam, The Netherlands, 2022; Volume 44, pp. 1219–1231. [Google Scholar]
- Alderson, K.; Nazaré, S.; Alderson, A. Large-scale extrusion of auxetic polypropylene fibre. Phys. Status Solidi 2016, 253, 1279–1287. [Google Scholar] [CrossRef]
- Vyavahare, S.; Teraiya, S.; Kumar, S. Auxetic structures fabricated by material extrusion: An experimental investigation of gradient parameters. Rapid Prototyp. J. 2021, 27, 1041–1058. [Google Scholar] [CrossRef]
- Chikkanna, N.; Logakannan, K.P.; Krishnapillai, S.; Ramachandran, V. Quasi-static compression performance of material extrusion enabled re-entrant diamond auxetic metamaterial: Fabrication, tuning the geometrical parameters and fibre reinforcements. Thin-Walled Struct. 2022, 179, 109550. [Google Scholar] [CrossRef]
- Khadem-Reza, L.; Etemadi, E.; Abbaslou, M.; Hu, H. Design of novel 3D auxetic structures based on S-shaped unit-cells. Smart Mater. Struct. 2022, 31, 075024. [Google Scholar] [CrossRef]
- Li, B.; Liang, W.; Zhang, L.; Ren, F.; Xuan, F. TPU/CNTs flexible strain sensor with auxetic structure via a novel hybrid manufacturing process of fused deposition modeling 3D printing and ultrasonic cavitation-enabled treatment. Sens. Actuators A Phys. 2022, 340, 113526. [Google Scholar] [CrossRef]
- Shin, E.J.; Park, Y.; Jung, Y.S.; Choi, H.Y.; Lee, S. Fabrication and characteristics of flexible thermoplastic polyurethane filament for fused deposition modeling three-dimensional printing. Polym. Eng. Sci. 2022, 62, 2947–2957. [Google Scholar] [CrossRef]
- Walther, M.; Ortner, A.; Meier, H.; Löffelmann, U.; Smith, P.J.; Korvink, J.G. Terahertz metamaterials fabricated by inkjet printing. Appl. Phys. Lett. 2009, 95, 251107. [Google Scholar] [CrossRef]
- Kashiwagi, K.; Xie, L.; Li, X.; Kageyama, T.; Miura, M.; Miyashita, H.; Kono, J.; Lee, S.S. Inkjet-printed silver-nanoparticle THz metamaterial. In Proceedings of the 2016 41st International Conference on Infrared, Millimeter, and Terahertz waves (IRMMW-THz), Copenhagen, Denmark, 25–30 September 2016; pp. 1–2. [Google Scholar]
- Schwerdtfeger, J.; Schury, F.; Stingl, M.; Wein, F.; Singer, R.; Körner, C. Mechanical characterisation of a periodic auxetic structure produced by SEBM. Phys. Status Solidi 2012, 249, 1347–1352. [Google Scholar] [CrossRef]
- Schwerdtfeger, J.; Heinl, P.; Singer, R.; Körner, C. Auxetic cellular structures through selective electron-beam melting. Phys. Status Solidi 2010, 247, 269–272. [Google Scholar] [CrossRef]
- Schwerdtfeger, J.; Wein, F.; Leugering, G.; Singer, R.; Körner, C.; Stingl, M.; Schury, F. Design of auxetic structures via mathematical optimization. Adv. Mater. 2011, 23, 2650–2654. [Google Scholar] [CrossRef] [PubMed]
- Mitschke, H.; Schwerdtfeger, J.; Schury, F.; Stingl, M.; Körner, C.; Singer, R.F.; Robins, V.; Mecke, K.; Schröder-Turk, G.E. Finding auxetic frameworks in periodic tessellations. Adv. Mater. 2011, 23, 2669–2674. [Google Scholar] [CrossRef]
- Pan, Y.; Zhang, X.G.; Han, D.; Li, W.; Xu, L.F.; Zhang, Y.; Jiang, W.; Bao, S.; Teng, X.C.; Lai, T.; et al. The out-of-plane compressive behavior of auxetic chiral lattice with circular nodes. Thin-Walled Struct. 2023, 182, 110152. [Google Scholar] [CrossRef]
- Jiang, Y.; Shi, K.; Zhou, L.; He, M.; Zhu, C.; Wang, J.; Li, J.; Li, Y.; Liu, L.; Sun, D.; et al. 3D-printed auxetic-structured intervertebral disc implant for potential treatment of lumbar herniated disc. Bioact. Mater. 2023, 20, 528–538. [Google Scholar] [CrossRef]
- Li, Q.; Wu, L.; Hu, L.; Miao, X.; Liu, X.; Zou, T. A sinusoidal beam lattice structure with negative Poisson’s ratio property. Aerosp. Sci. Technol. 2023, 133, 108103. [Google Scholar] [CrossRef]
- Zhang, M.; Zhao, C.; Li, G.; Luo, K. Mechanical properties of the composite lattice structure with variable density and multi-configuration. Compos. Struct. 2023, 304, 116405. [Google Scholar] [CrossRef]
- Gamba, L.; Johnson, Z.T.; Atterberg, J.; Diaz-Arauzo, S.; Downing, J.R.; Claussen, J.C.; Hersam, M.C.; Secor, E.B. Systematic Design of a Graphene Ink Formulation for Aerosol Jet Printing. ACS Appl. Mater. Interfaces 2023, 15, 3325–3335. [Google Scholar] [CrossRef]
- Konstantinou, X.; Lan, X.; Nguyen, K.; Escorcia, A.; Sandhu, R.; Tice, J.; Spain, W.; Albrecht, J.D.; Papapolymerou, J. Fully Aerosol Jet-Printed Passive Components for 140–220-GHz Operation. IEEE Microw. Wirel. Technol. Lett. 2023, 1–4. [Google Scholar] [CrossRef]
- Ratnayake, D.; Curry, A.; Qu, C.; Wei, D.; Gerber, E.; Walsh, K. Optimizing the Conductivity of a New Nano-particle Silver Ink for Aerosol Jet Printing and Demonstrating its use as a Strain Gauge. IEEE J. Flex. Electron. 2023, 1. [Google Scholar] [CrossRef]
- Chen, Z.; Li, Z.; Wang, H. Two-dimensional auxetic GeSe2 material with ferroelasticity and flexoelectricity. J. Phys. Chem. C 2021, 125, 19666–19672. [Google Scholar] [CrossRef]
- Coluci, V.R.; Hall, L.J.; Kozlov, M.E.; Zhang, M.; Dantas, S.O.; Galvao, D.S.; Baughman, R.H. Modeling the auxetic transition for carbon nanotube sheets. Phys. Rev. B 2008, 78, 115408. [Google Scholar] [CrossRef]
- Yuan, J.H.; Xue, K.H.; Wang, J.F.; Miao, X.S. Gallium thiophosphate: An emerging bidirectional auxetic two-dimensional crystal with wide direct band gap. J. Phys. Chem. Lett. 2019, 10, 4455–4462. [Google Scholar] [CrossRef]
- Ren, X.; Das, R.; Tran, P.; Ngo, T.D.; Xie, Y.M. Auxetic metamaterials and structures: A review. Smart Mater. Struct. 2018, 27, 023001. [Google Scholar] [CrossRef]
- Ahmed, H.I.; Umair, M.; Nawab, Y.; Hamdani, S.T.A. Development of 3D auxetic structures using para-aramid and ultra-high molecular weight polyethylene yarns. J. Text. Inst. 2021, 112, 1417–1427. [Google Scholar] [CrossRef]
- Steffens, F.; Rana, S.; Fangueiro, R. Development of novel auxetic textile structures using high performance fibres. Mater. Des. 2016, 106, 81–89. [Google Scholar] [CrossRef]
- Khoshgoftar, M.; Abbaszadeh, H. Experimental and finite element analysis of the effect of geometrical parameters on the mechanical behavior of auxetic cellular structure under static load. J. Strain Anal. Eng. Des. 2021, 56, 131–138. [Google Scholar] [CrossRef]
- Janus-Michalska, M.; Jasińska, D.; Smardzewski, J. Comparison of contact stress distribution for foam seat and seat of auxetic spring skeleton. Int. J. Appl. Mech. Eng. 2013, 18, 55–72. [Google Scholar] [CrossRef]
- Dehaghani, M.R.; Nourani, A.; Arjmand, N. Effects of auxetic shoe on lumbar spine kinematics and kinetics during gait and drop vertical jump by a combined in vivo and modeling investigation. Sci. Rep. 2022, 12, 18326. [Google Scholar] [CrossRef]
- NIJ 0101.06; Ballistic Resistance of Body Armor. National Institute of Justice: Washington, DC, USA, 2008.
- Nayak, R.; Crouch, I.; Kanesalingam, S.; Ding, J.; Tan, P.; Lee, B.; Miao, M.; Ganga, D.; Wang, L. Body armor for stab and spike protection, Part 1: Scientific literature review. Text. Res. J. 2018, 88, 812–832. [Google Scholar] [CrossRef]
- Mawkhlieng, U.; Majumdar, A.; Laha, A. A review of fibrous materials for soft body armour applications. RSC Adv. 2020, 10, 1066–1086. [Google Scholar] [CrossRef]
- Liu, J.; Yang, W.; Liu, J.; Liu, J.; Huang, W. Ballistic impact analyses of foam-filled double-arrow auxetic structure. Thin-Walled Struct. 2023, 182, 110173. [Google Scholar] [CrossRef]
- Jiang, F.; Yang, S.; Qi, C.; Liu, H.T.; Remennikov, A.; Pei, L.Z. Blast response and multi-objective optimization of graded re-entrant circular auxetic cored sandwich panels. Compos. Struct. 2023, 305, 116494. [Google Scholar] [CrossRef]
- Lan, X.; Huang, G.; Bian, X.; Liu, Y. Impact resistance of foam-filled hybrid-chiral honeycomb beam under localized impulse loading. Int. J. Impact Eng. 2023, 173, 104477. [Google Scholar] [CrossRef]
- Ul Haq, A.; Gunashekar, G.; Narala, S.K.R. The Dynamic Response of AuxHex and Star-Reentrant Honeycomb Cored Sandwich Panels Subject to Blast Loading. Arab. J. Sci. Eng. 2023, 1–17. [Google Scholar] [CrossRef]
- Yan, Z.; Liu, Y.; Yan, J.; Wang, B.; Bai, F.; Shi, Z.; Huang, F. Anti-blast performance of 3D-printed sandwich panels with auxetic hexagonal and regular hexagonal honeycomb cores. Eng. Struct. 2022, 272, 114996. [Google Scholar] [CrossRef]
- Critchley, R.; Hazael, R.; Bhatti, K.; Wood, D.; Peare, A.; Johnson, S.; Temple, T. Blast mitigation using polymeric 3D printed auxetic re-entrant honeycomb structures: A preliminary study. Int. J. Prot. Struct. 2021, 13, 469–486. [Google Scholar] [CrossRef]
- Usta, F.; Türkmen, H.S.; Scarpa, F. High-velocity impact resistance of doubly curved sandwich panels with re-entrant honeycomb and foam core. Int. J. Impact Eng. 2022, 165, 104230. [Google Scholar] [CrossRef]
- Zhao, C.; Wang, Y.; Cao, S.; Xuan, S.; Jiang, W.; Gong, X. Conductive shear thickening gel/Kevlar wearable fabrics: A flexible body armor with mechano-electric coupling ballistic performance. Compos. Sci. Technol. 2019, 182, 107782. [Google Scholar] [CrossRef]
- Zhang, Q.; Qin, Z.; Yan, R.; Wei, S.; Zhang, W.; Lu, S.; Jia, L. Processing technology and ballistic-resistant mechanism of shear thickening fluid/high-performance fiber-reinforced composites: A review. Compos. Struct. 2021, 266, 113806. [Google Scholar] [CrossRef]
- Zhao, C.; Gong, X.; Wang, S.; Jiang, W.; Xuan, S. Shear stiffening gels for intelligent anti-impact applications. Cell Rep. Phys. Sci. 2020, 1, 100266. [Google Scholar] [CrossRef]
- Ribeiro, M.P.; da Silveira, P.H.P.M.; de Oliveira Braga, F.; Monteiro, S.N. Fabric Impregnation with Shear Thickening Fluid for Ballistic Armor Polymer Composites: An Updated Overview. Polymers 2022, 14, 4357. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Y.; Zhou, J.; Wu, J.; Liu, S.; Sang, M.; Liu, B.; Pan, Y.; Gong, X. Multi-functional STF-based yarn for human protection and wearable systems. Chem. Eng. J. 2023, 453, 139869. [Google Scholar] [CrossRef]
- Sheikhi, M.R.; Gürgen, S. Deceleration behavior of multi-layer cork composites intercalated with a non-Newtonian material. Arch. Civ. Mech. Eng. 2023, 23, 1–11. [Google Scholar] [CrossRef]


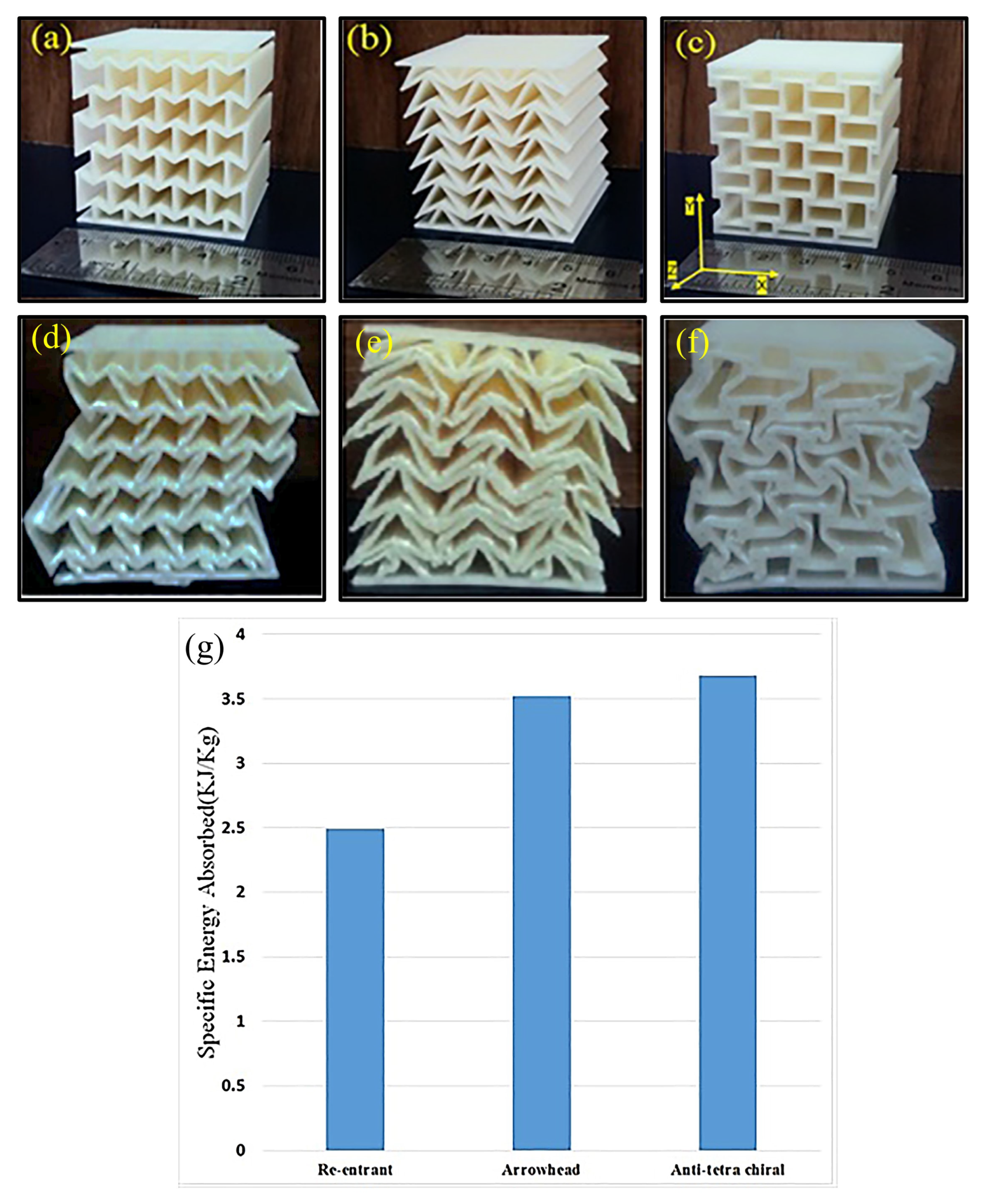
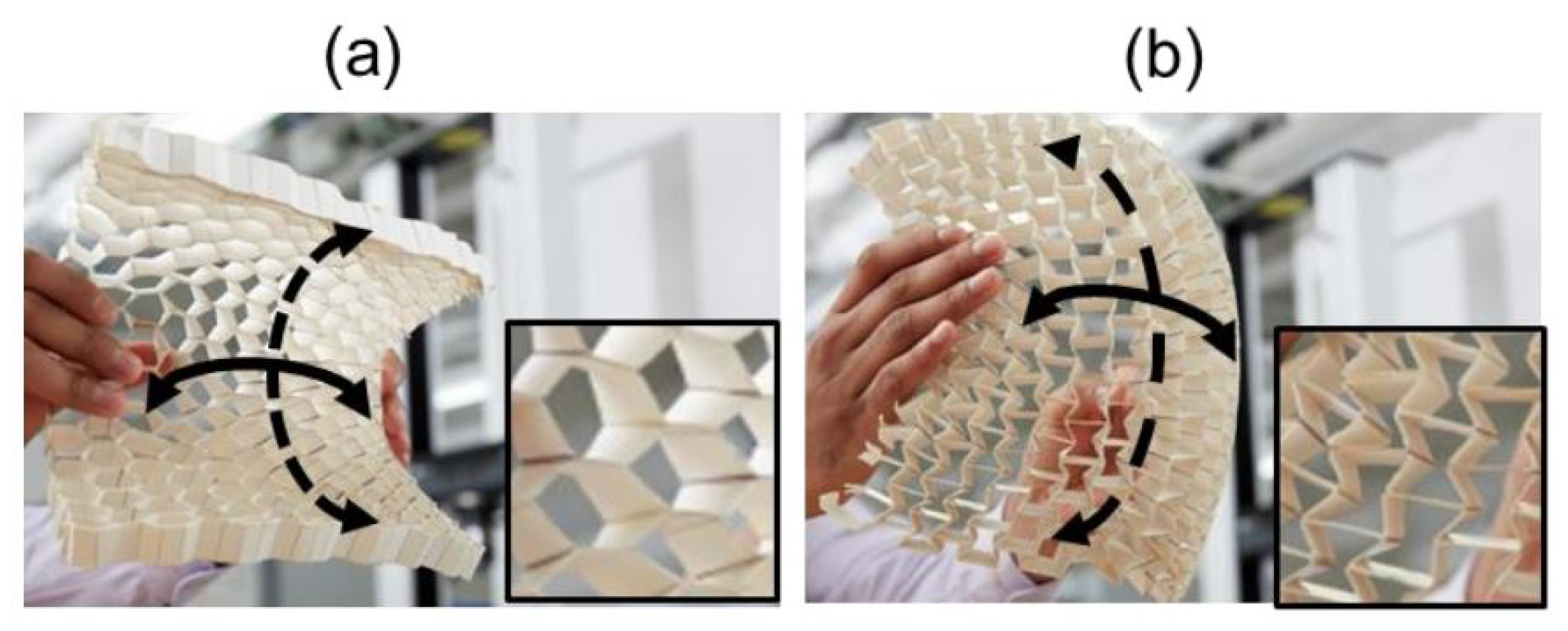

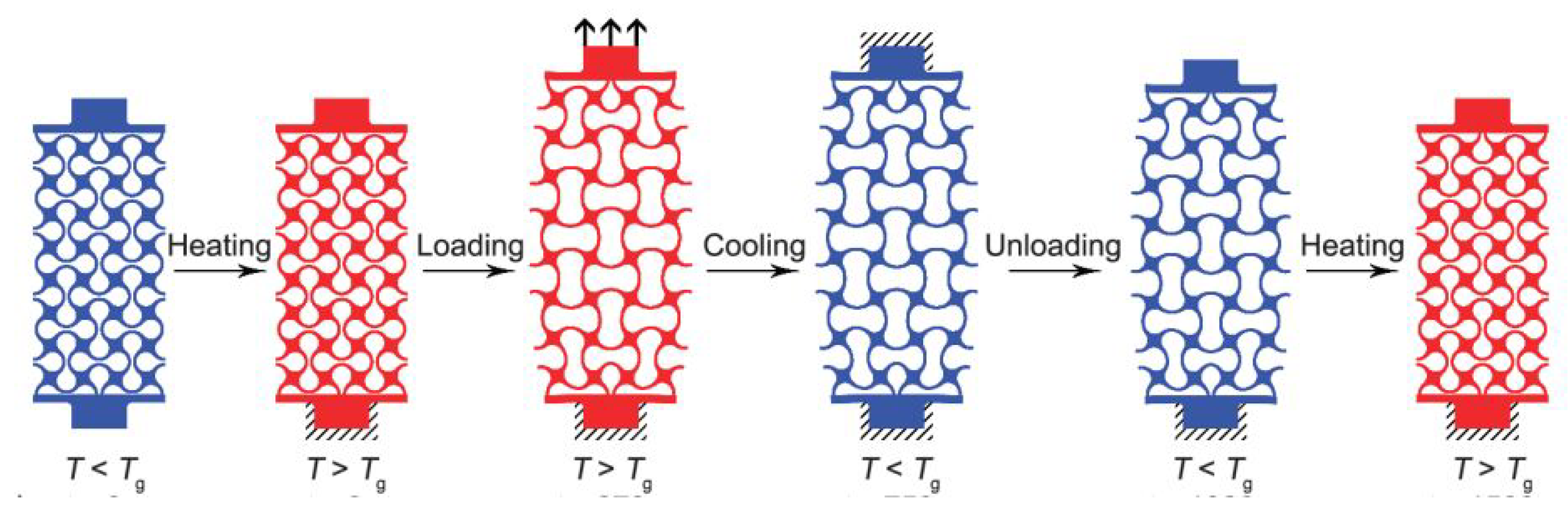
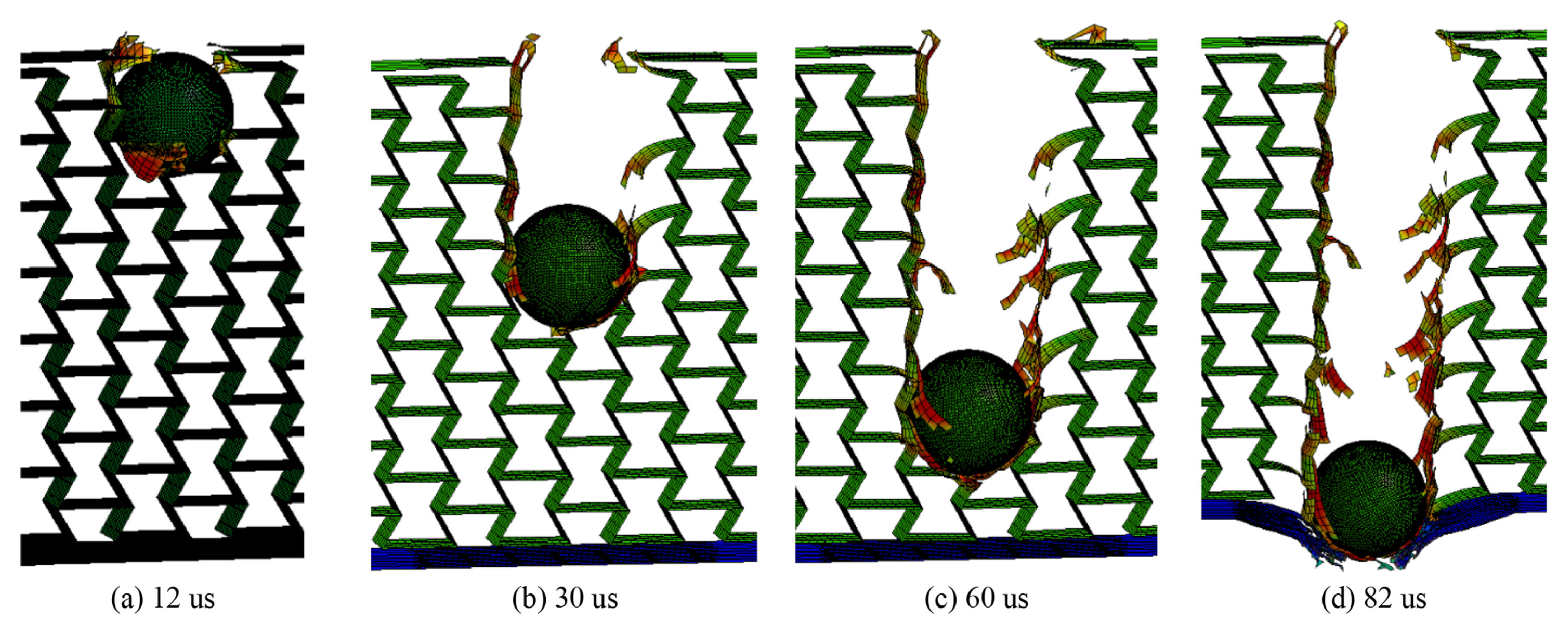


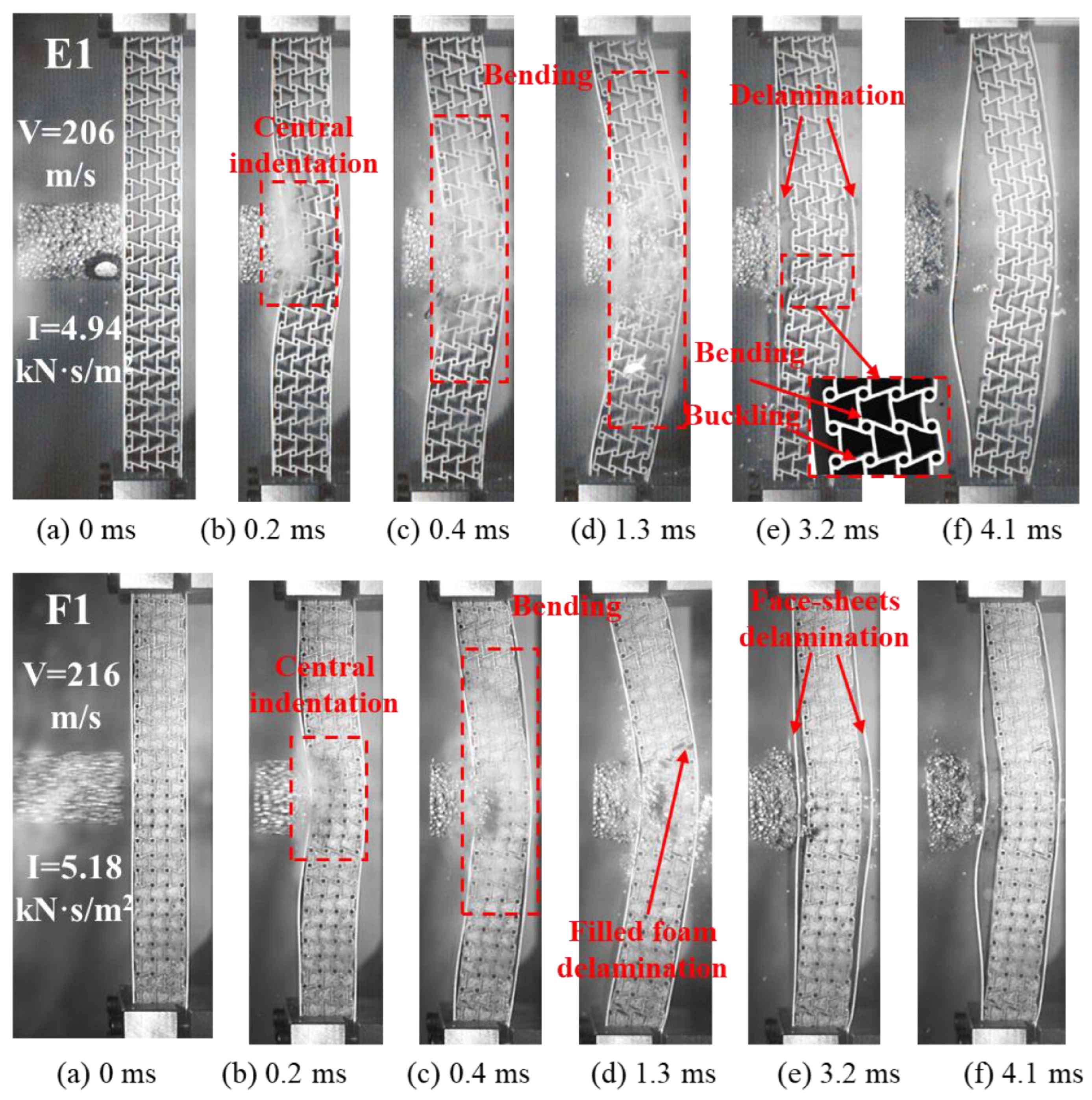
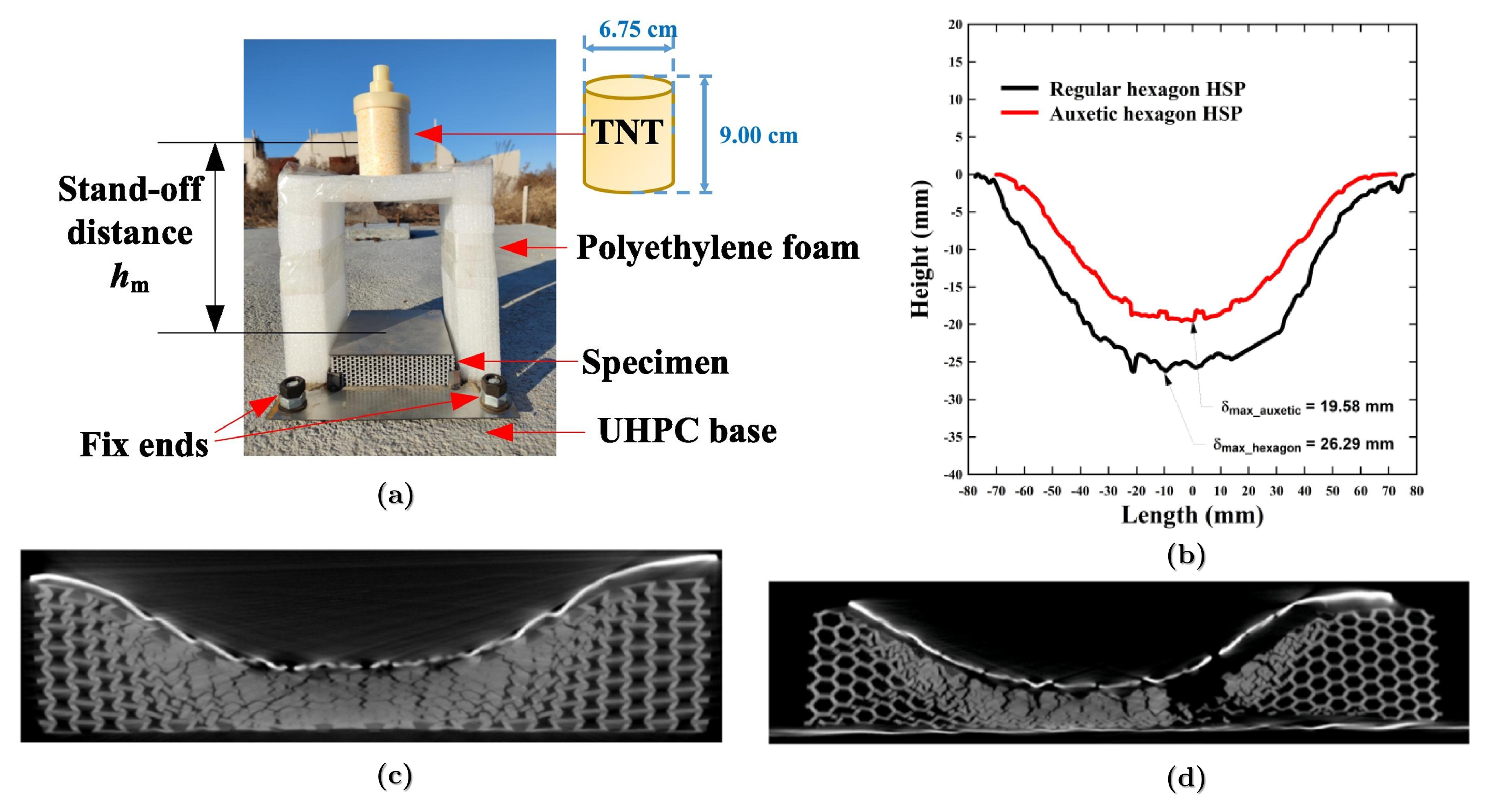
| Material | Poisson’s Ratio | Year | Reference |
|---|---|---|---|
| AA1060 aluminum | −0.82~−1.01 | 2023 | [66] |
| Cellulose | −0.2 | 2023 | [67] |
| Carbon Fiber/Aluminum | −0.13 | 2023 | [68] |
| LDPE | −1.5~−3.5 | 2022 | [69] |
| ABS | −0.65~−1.56 | 2022 | [70] |
| 316L stainless steel | −0.4~−0.8 | 2022 | [71] |
| Graphene/Cu | −0.19~−0.25 | 2022 | [72] |
| Ba0.85Ca0.15Zr0.1Ti0.9O3 | −1~−2 | 2022 | [73] |
| Cement/PU | −0.21~−0.47 | 2022 | [74] |
| PLA | −0.50~−0.78 | 2022 | [75] |
| MgHfN2 and MgZrN2 | −0.2 | 2022 | [76] |
| PU/PDA/Graphene | −2.36 | 2022 | [77] |
| Field | Application |
|---|---|
| Aerospace | Vanes for engine, thermal protection, aircraft nose-cones, wing panel, vibration absorber |
| Automotive | Bumper, cushion, thermal protection, sounds and vibration absorber parts, fastener |
| Biomedical | Bandage, wound pressure pad, dental floss, artificial blood vessel, artificial skin, drug-release unit, ligament anchors, surgical implants |
| Composite | Fiber reinforcement |
| Military | Helmet, bulletproof vest, knee pad, glove, protective gear |
| Sensors/Actuators | Hydrophone, piezoelectric devices |
| Textile | Fibers, functional fabric, color-change straps or fabrics, threads |
| Armor Type | Test Bullets | Bullet Mass (g) | Armor Test Velocity (m/s) |
|---|---|---|---|
| IIA | 9 mm FMJ RN 0.40 S & W FMJ RN | 8.0 11.7 | 373 352 |
| II | 9 mm FMJ RN 0.357 Magnum JSP | 8.0 10.0 | 398 436 |
| IIIA | 0.357 SIG FMJ FN 0.44 Magnum SJHP | 8.1 15.6 | 448 436 |
| III | 7.62 mm NATO FMJ | 9.6 | 847 |
| IV | 0.30 Calibre M2 AP | 10.8 | 878 |
| Author | Year | Material of AXS | Structure | Application |
|---|---|---|---|---|
| Liu et al. [221] | 2023 | Carbon Fiber/PU | Double-Arrow | Ballistic Protection |
| Jiang et al. [222] | 2023 | Aluminum AA6061/Grade 800 HSS | Re-entrant Circular (REC) | Blast Protection |
| Lan et al. [223] | 2023 | AlSi10Mg aluminum alloy/5052 aluminum alloy | Hybrid Chiral | Ballistic Protection |
| Haq et al. [224] | 2023 | Aluminum AA2024/Armor Steel | Re-Entrant/Hexagonal Star/Re-Entrant | Ballistic Protection |
| Yan et al. [225] | 2022 | AlSi10Mg aluminum alloy/Q345 steel | Hexagonal and Re-Entrant | Blast Protection |
| Critchley et al. [226] | 2022 | TPU | Re-entrant | Blast Protection |
| Usta et al. [227] | 2022 | CFRP/PLA | Re-entrant | Ballistic Protection |
| Wu et al. [64] | 2022 | Kevlar | Hexagonal and Re-entrant | Impact Resistance |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Junio, R.F.P.; da Silveira, P.H.P.M.; Neuba, L.d.M.; Monteiro, S.N.; Nascimento, L.F.C. Development and Applications of 3D Printing-Processed Auxetic Structures for High-Velocity Impact Protection: A Review. Eng 2023, 4, 903-940. https://doi.org/10.3390/eng4010054
Junio RFP, da Silveira PHPM, Neuba LdM, Monteiro SN, Nascimento LFC. Development and Applications of 3D Printing-Processed Auxetic Structures for High-Velocity Impact Protection: A Review. Eng. 2023; 4(1):903-940. https://doi.org/10.3390/eng4010054
Chicago/Turabian StyleJunio, Raí Felipe Pereira, Pedro Henrique Poubel Mendonça da Silveira, Lucas de Mendonça Neuba, Sergio Neves Monteiro, and Lucio Fabio Cassiano Nascimento. 2023. "Development and Applications of 3D Printing-Processed Auxetic Structures for High-Velocity Impact Protection: A Review" Eng 4, no. 1: 903-940. https://doi.org/10.3390/eng4010054
APA StyleJunio, R. F. P., da Silveira, P. H. P. M., Neuba, L. d. M., Monteiro, S. N., & Nascimento, L. F. C. (2023). Development and Applications of 3D Printing-Processed Auxetic Structures for High-Velocity Impact Protection: A Review. Eng, 4(1), 903-940. https://doi.org/10.3390/eng4010054








