Numerical and Experimental Investigation of Anchor Channels Subjected to Tension Load in Composite Slabs with Profiled Steel Decking
Abstract
:1. Introduction
Design of Tension-Loaded Anchor Channels
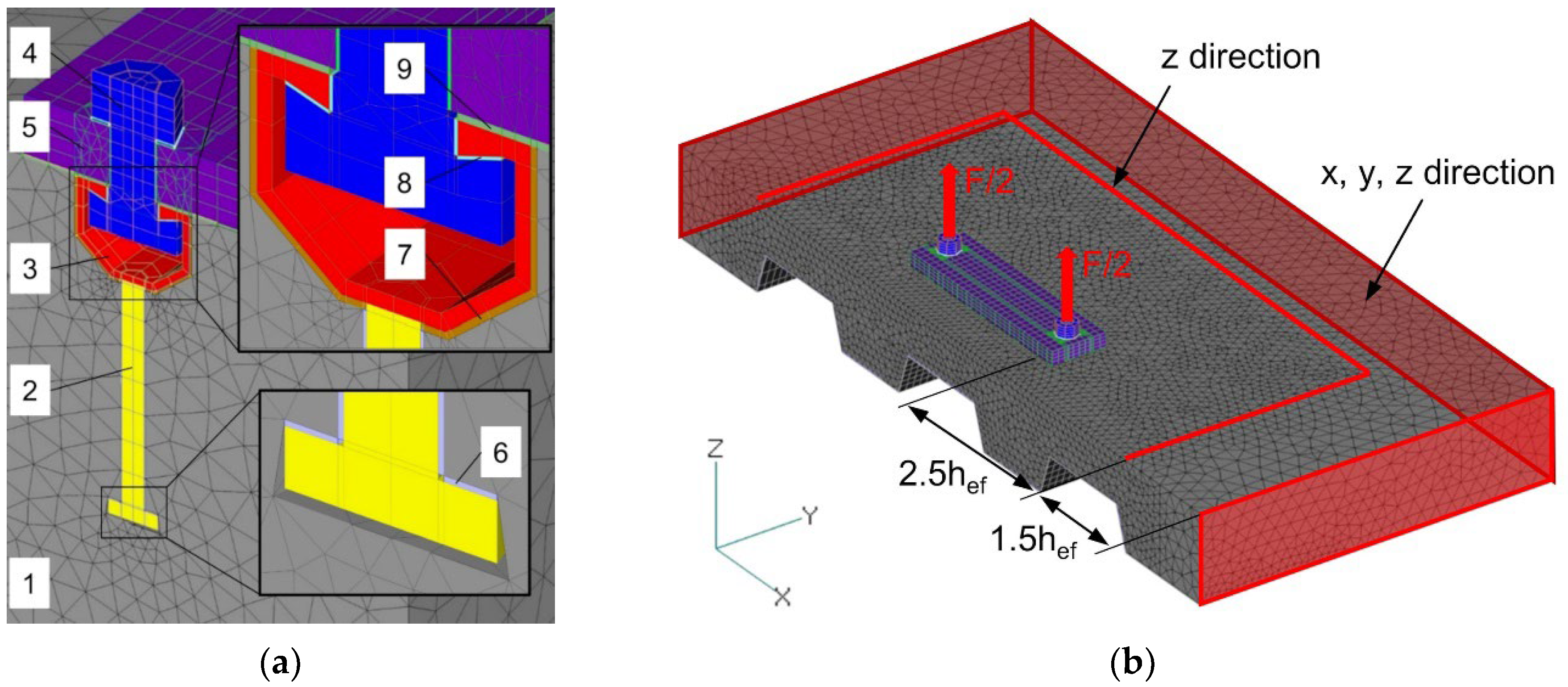
2. FE Model and Experimental Validation
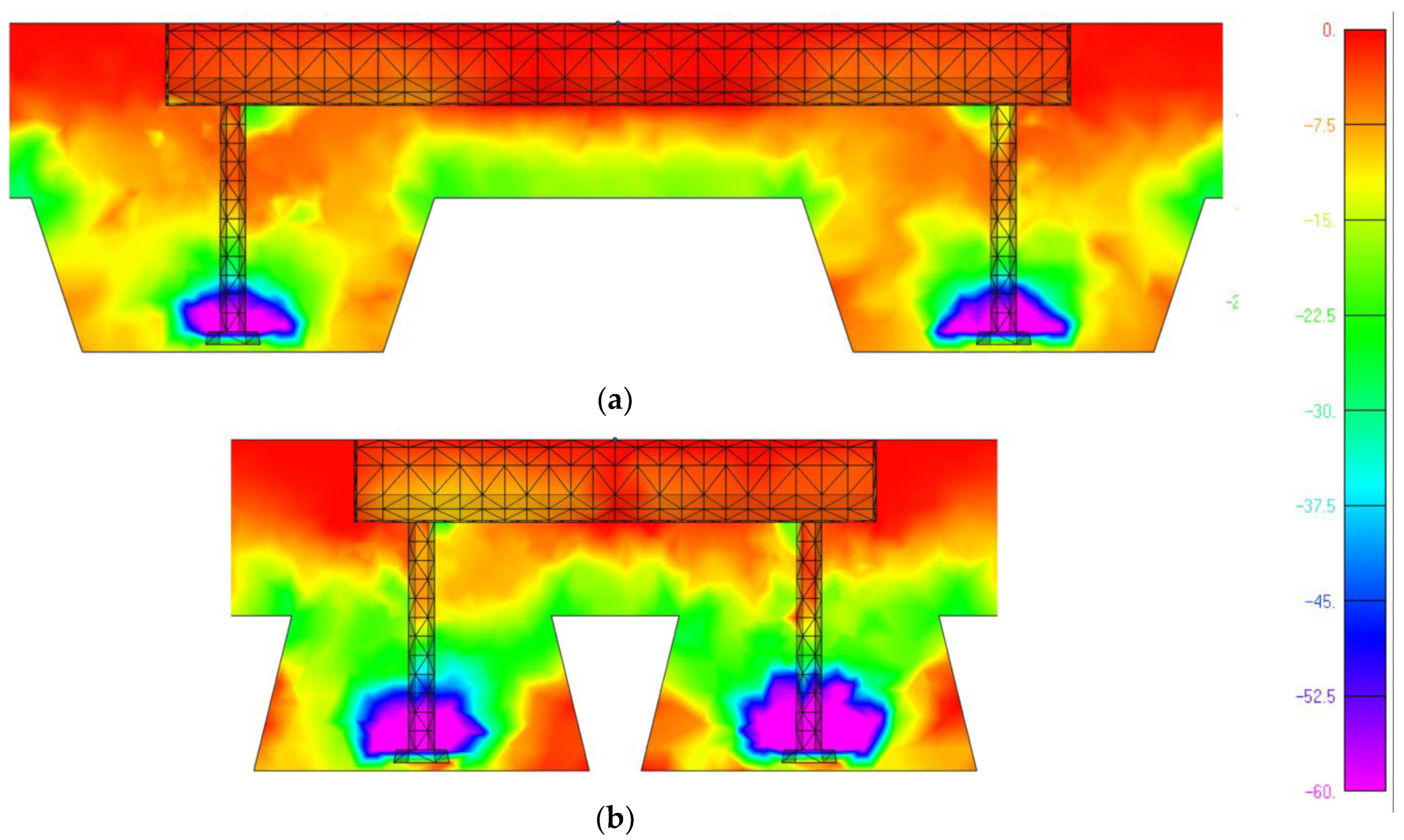
Definition of Boundary Conditions
3. Numerical Parametric Study
- 0.2% of the cross-sectional area of the concrete above the ribs for unpropped construction.
- 0.4% of the cross-sectional area of the concrete above the ribs for propped construction.
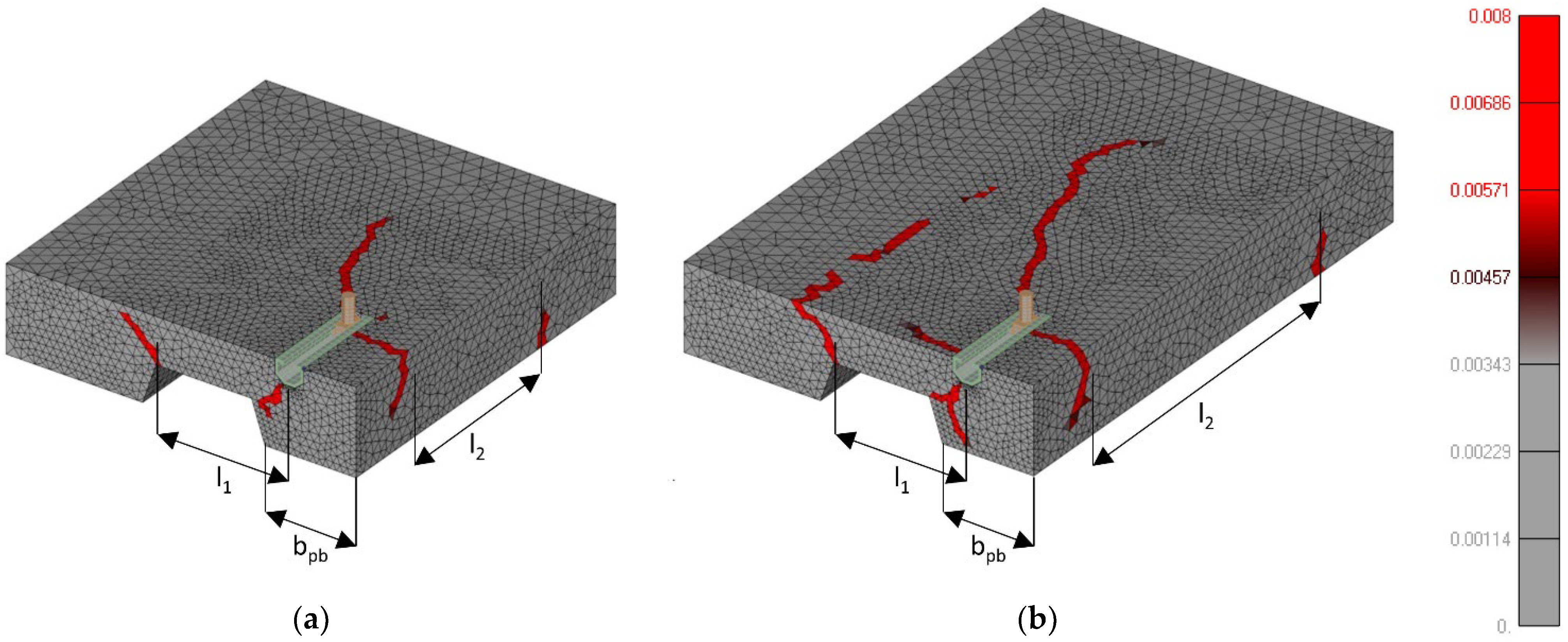
4. Numerical Results
4.1. Series 1
4.2. Series 2
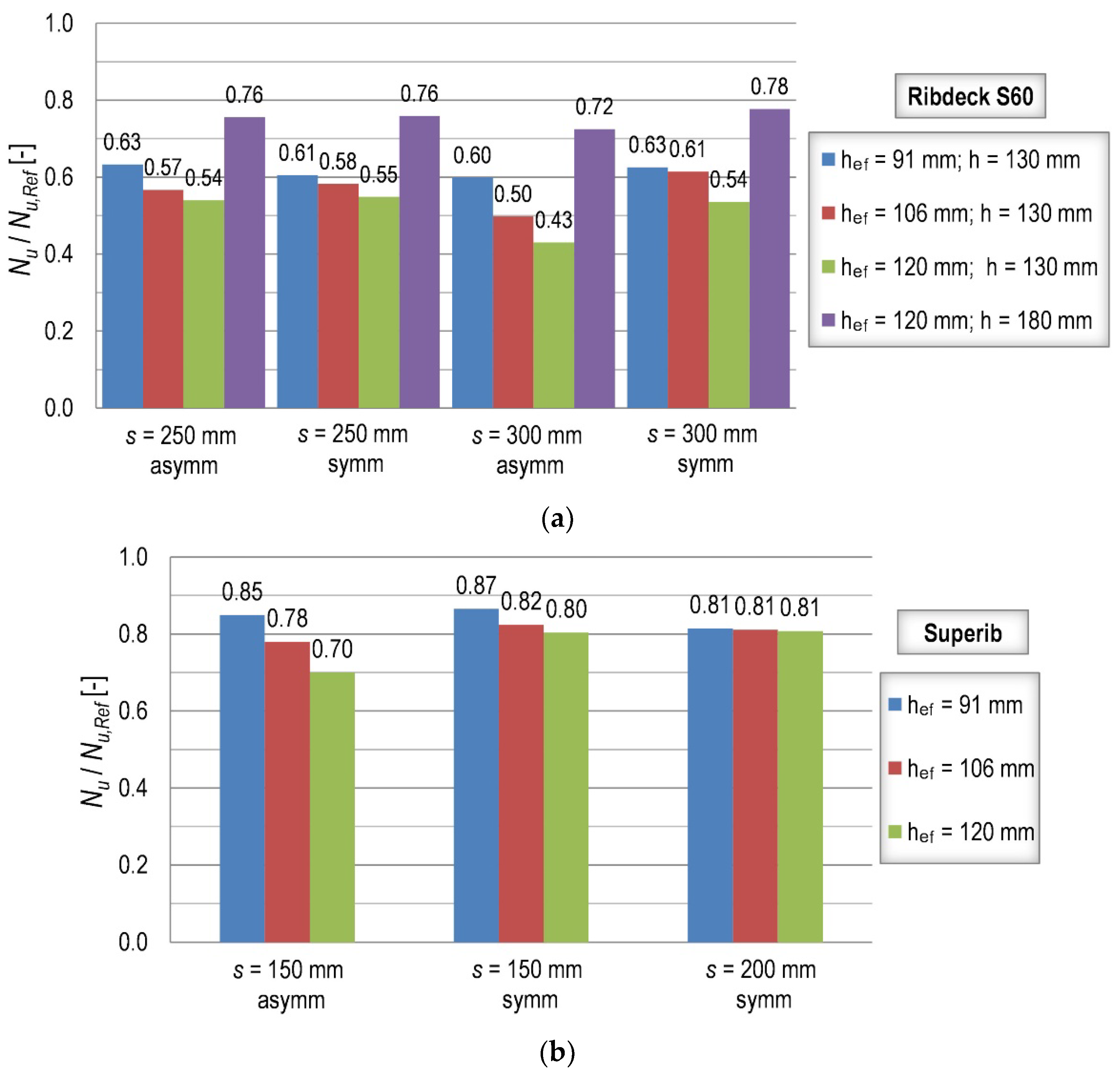
4.3. Series 3
5. Experimental Results
6. Design Recommendations
7. Conclusions
- The influence of bending affects the concrete capacity of tension-loaded fasteners in thin concrete members. It was shown that the influence of support span is not so pronounced when installed in plain concrete slabs. However, for composite slabs, the influence is significant, and the interaction between the global stresses and the local stresses caused by the fastening system should be considered for further discussion.
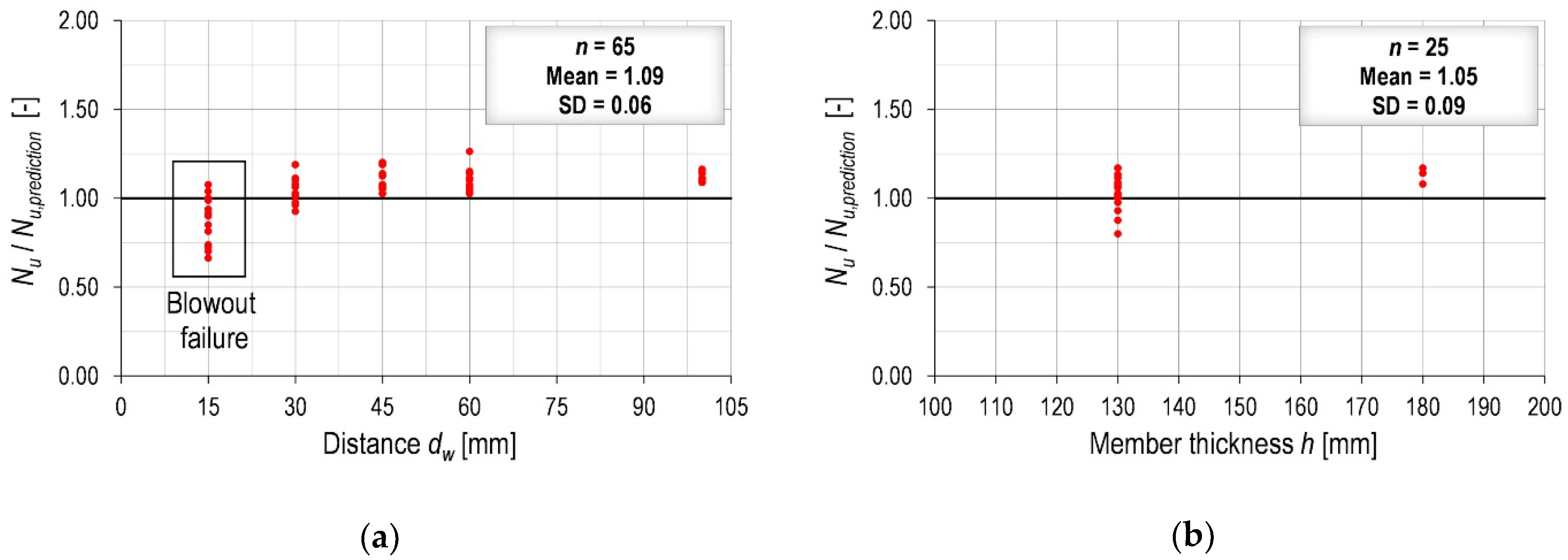
- When anchor channels are installed in perimeter beams, the orientation of the profiled steel decking does not have a pronounced influence. If anchors are placed sufficiently far from the steel decking, a reduction of up to 20% can be expected for the studied geometry, regardless of the width of the perimeter beam, embedment depth, edge distance or member thickness. Therefore, a constant reduction factor of 0.8 is proposed if the distance between the anchor and the steel decking is sufficient to avoid blowout failure. According to the obtained results, this distance should be dw > 30 mm.
- Installation over the steel decking is the most critical position, especially in the case of composite slabs with trapezoidal profiles. For a common thickness of 130 mm, capacity reductions of up to approximately 50% compared to a plain concrete slab are possible for trapezoidal profiles. The influence of the anchor position is minor, as well as the influence of embedment depth. However, the influence of member thickness cannot be neglected in this case. Therefore, a modification factor based on the thickness of the concrete layer above the steel decking and the overall member thickness is proposed. For re-entrant profiles, a constant factor of 0.8 can be adopted as their shape enhances concrete capacity.
- Given the complexity of the topic, the obtained test results can be considered as the first evidence. In order to optimize the design method, further experimental investigations are recommended in the future.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| c1 (mm) | hef (mm) | h (mm) | s (mm) | Nu (kN) |
|---|---|---|---|---|
| 100 | 91 | 130 | 300 | 73.96 |
| 100 | 91 | 130 | 250 | 67.98 |
| 100 | 91 | 130 | 200 | 64.51 |
| 100 | 91 | 130 | 150 | 59.34 |
| 100 | 91 | 180 | 250 | 75.09 |
| 100 | 106 | 130 | 300 | 79.08 |
| 100 | 106 | 130 | 250 | 73.45 |
| 100 | 106 | 130 | 200 | 73.19 |
| 100 | 106 | 130 | 150 | 68.21 |
| 100 | 106 | 180 | 250 | 87.29 |
| 200 | 106 | 130 | 250 | 87.72 |
| 100 | 120 | 130 | 300 | 86.79 |
| 100 | 120 | 130 | 250 | 80.20 |
| 100 | 120 | 130 | 200 | 78.46 |
| 100 | 120 | 130 | 150 | 76.13 |
| 100 | 120 | 180 | 300 | 107.42 |
| 100 | 120 | 180 | 250 | 98.72 |
| c1 (mm) | hef (mm) | h (mm) | s (mm) | Steel Decking | dw (mm) | Nu (kN) | Nu/Nu,Ref (kN) |
|---|---|---|---|---|---|---|---|
| 100 | 91 | 130 | 250 | Ribdeck S60 | 15 | 50.73 | 0.75 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 30 | 53.27 | 0.78 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 45 | 57.82 | 0.85 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 60 | 55.68 | 0.82 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 100 | 59.30 | 0.87 |
| 100 | 91 | 180 | 250 | Ribdeck S60 | 15 | 62.39 | 0.83 |
| 100 | 91 | 180 | 250 | Ribdeck S60 | 30 | 64.20 | 0.86 |
| 100 | 91 | 180 | 250 | Ribdeck S60 | 45 | 64.20 | 0.86 |
| 100 | 91 | 180 | 250 | Ribdeck S60 | 60 | 66.77 | 0.89 |
| 100 | 91 | 180 | 250 | Ribdeck S60 | 100 | 69.09 | 0.92 |
| 100 | 91 | 130 | 250 | Superib | 15 | 44.17 | 0.65 |
| 100 | 91 | 130 | 250 | Superib | 30 | 52.29 | 0.77 |
| 100 | 91 | 130 | 250 | Superib | 45 | 57.73 | 0.85 |
| 100 | 91 | 130 | 250 | Superib | 60 | 56.23 | 0.83 |
| 100 | 91 | 130 | 250 | Superib | 100 | 59.62 | 0.88 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 15 | 53.05 | 0.72 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 30 | 69.56 | 0.95 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 45 | 66.95 | 0.91 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 60 | 64.42 | 0.88 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 100 | 65.69 | 0.89 |
| 100 | 106 | 180 | 250 | Ribdeck S60 | 15 | 69.18 | 0.79 |
| 100 | 106 | 180 | 250 | Ribdeck S60 | 30 | 70.99 | 0.81 |
| 100 | 106 | 180 | 250 | Ribdeck S60 | 45 | 72.95 | 0.84 |
| 100 | 106 | 180 | 250 | Ribdeck S60 | 60 | 74.54 | 0.85 |
| 100 | 106 | 180 | 250 | Ribdeck S60 | 100 | 79.08 | 0.91 |
| 200 | 106 | 130 | 250 | Ribdeck S60 | 15 | 64.45 | 0.73 |
| 200 | 106 | 130 | 250 | Ribdeck S60 | 30 | 75.31 | 0.86 |
| 200 | 106 | 130 | 250 | Ribdeck S60 | 45 | 75.00 | 0.86 |
| 200 | 106 | 130 | 250 | Ribdeck S60 | 60 | 73.32 | 0.84 |
| 200 | 106 | 130 | 250 | Ribdeck S60 | 100 | 76.22 | 0.87 |
| 100 | 106 | 130 | 250 | Superib | 15 | 43.49 | 0.59 |
| 100 | 106 | 130 | 250 | Superib | 30 | 65.19 | 0.89 |
| 100 | 106 | 130 | 250 | Superib | 45 | 70.03 | 0.95 |
| 100 | 106 | 130 | 250 | Superib | 60 | 67.09 | 0.91 |
| 100 | 106 | 130 | 250 | Superib | 100 | 64.06 | 0.87 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 15 | 68.84 | 0.86 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 30 | 76.49 | 0.95 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 45 | 77.25 | 0.96 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 60 | 73.16 | 0.91 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 100 | 70.51 | 0.88 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | 15 | 79.04 | 0.80 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | 30 | 80.76 | 0.82 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | 45 | 81.07 | 0.82 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | 60 | 87.41 | 0.89 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | 100 | 87.41 | 0.89 |
| 100 | 120 | 130 | 250 | Superib | 15 | 44.56 | 0.56 |
| 100 | 120 | 130 | 250 | Superib | 30 | 59.64 | 0.74 |
| 100 | 120 | 130 | 250 | Superib | 45 | 71.92 | 0.90 |
| 100 | 120 | 130 | 250 | Superib | 60 | 81.22 | 1.01 |
| 100 | 120 | 130 | 250 | Superib | 100 | 69.58 | 0.87 |
| c1 (mm) | hef (mm) | h (mm) | s (mm) | Steel Decking | dw (mm) | Nu (kN) | Nu/Nu,Ref (kN) |
|---|---|---|---|---|---|---|---|
| 100 | 91 | 130 | 250 | Ribdeck S60 | 15 | 46.46 | 0.68 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 30 | 55.90 | 0.82 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 45 | 55.44 | 0.82 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 60 | 58.73 | 0.86 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | 100 | 60.41 | 0.89 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 15 | 42.41 | 0.58 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 30 | 64.61 | 0.88 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 45 | 70.03 | 0.95 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 60 | 66.63 | 0.91 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | 100 | 65.05 | 0.89 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 15 | 42.46 | 0.53 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 30 | 67.77 | 0.85 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 45 | 77.02 | 0.96 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 60 | 73.55 | 0.92 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | 100 | 74.35 | 0.93 |
| c1 (mm) | hef (mm) | h (mm) | s (mm) | Steel Decking | Configuration | Nu (kN) | Nu/Nu,Ref (kN) |
|---|---|---|---|---|---|---|---|
| 100 | 91 | 130 | 250 | Ribdeck S60 | Asymmetric | 43.06 | 0.63 |
| 100 | 91 | 130 | 250 | Ribdeck S60 | Symmetric | 41.14 | 0.61 |
| 100 | 91 | 130 | 300 | Ribdeck S60 | Asymmetric | 44.31 | 0.60 |
| 100 | 91 | 130 | 300 | Ribdeck S60 | Symmetric | 46.30 | 0.63 |
| 100 | 91 | 130 | 150 | Superib | Asymmetric | 50.38 | 0.85 |
| 100 | 91 | 130 | 150 | Superib | Symmetric | 51.35 | 0.87 |
| 100 | 91 | 130 | 200 | Superib | Symmetric | 52.56 | 0.81 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | Asymmetric | 41.66 | 0.57 |
| 100 | 106 | 130 | 250 | Ribdeck S60 | Symmetric | 42.82 | 0.58 |
| 100 | 106 | 130 | 300 | Ribdeck S60 | Asymmetric | 39.38 | 0.50 |
| 100 | 106 | 130 | 300 | Ribdeck S60 | Symmetric | 48.60 | 0.61 |
| 100 | 106 | 130 | 150 | Superib | Asymmetric | 53.19 | 0.78 |
| 100 | 106 | 130 | 150 | Superib | Symmetric | 56.18 | 0.82 |
| 100 | 106 | 130 | 200 | Superib | Symmetric | 59.39 | 0.81 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | Asymmetric | 43.30 | 0.54 |
| 100 | 120 | 130 | 250 | Ribdeck S60 | Symmetric | 44.00 | 0.55 |
| 100 | 120 | 130 | 300 | Ribdeck S60 | Asymmetric | 37.34 | 0.43 |
| 100 | 120 | 130 | 300 | Ribdeck S60 | Symmetric | 46.50 | 0.54 |
| 100 | 120 | 130 | 150 | Superib | Asymmetric | 53.32 | 0.70 |
| 100 | 120 | 130 | 150 | Superib | Symmetric | 61.21 | 0.80 |
| 100 | 120 | 130 | 200 | Superib | Symmetric | 63.39 | 0.81 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | Asymmetric | 74.64 | 0.76 |
| 100 | 120 | 180 | 250 | Ribdeck S60 | Symmetric | 74.92 | 0.76 |
| 100 | 120 | 180 | 300 | Ribdeck S60 | Asymmetric | 77.87 | 0.72 |
| 100 | 120 | 180 | 300 | Ribdeck S60 | Symmetric | 83.49 | 0.78 |
References
- Bogdanić, A.; Casucci, D.; Ožbolt, J. Numerical and Experimental Investigation of Anchor Channels Subjected to Shear Load in Composite Slabs with Profiled Steel Decking. Eng. Struct. 2021, 240, 112347. [Google Scholar] [CrossRef]
- Kraus, J. Tragverhalten und Bemessung von Ankerschienen unter Zentrischer Zugbelastung (Load-Bearing Behaviour and Design of Anchor Channels under Axial Tension). Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2003. (In German). [Google Scholar]
- EN 1992-4; Eurocode 2—Design of Concrete Structures—Part 4: Design of Fastenings for Use in Concrete (EN 1992-4:2018). European Committee for Standardization (CEN): Brussels, Belgium, 2018.
- ACI 318; Building Code Requirements for Structural Concrete (ACI 318-14) and Commentary (ACI 318R-14). American Concrete Institute: Farmington Hills, MI, USA, 2014.
- AC232; Acceptance Criteria for Anchor Channels in Concrete Elements. ICC-ES: Whittier, CA, USA, 2019.
- Bogdanić, A.; Casucci, D.; Ožbolt, J. Numerical and Experimental Investigation on Concrete Splitting Failure of Anchor Channels. CivilEng 2021, 2, 502–522. [Google Scholar] [CrossRef]
- Hüer, T. Tragverhalten von Randnahen Zugbeanspruchten Befestigungen bei der Versagensart “Spalten des Betons”, (Behavior of Tension Loaded Fasteners near the Edge for the Concrete Splitting Failure Mode). Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2014. (In German). [Google Scholar]
- Nilforoush, R. Numerical and Experimental Evaluations of Load-Carrying Capacity of Cast-in-Place Headed Anchors and Post-Installed Adhesive Anchors. Ph.D. Thesis, University of Lulea, Lulea, Sweden, 2017. [Google Scholar]
- Ožbolt, J.; Li, Y.; Kožar, I. Microplane model for concrete with relaxed kinematic constraints. Int. J. Solids Struct. 2001, 38, 2683–2711. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Oh, B. Crack band theory for fracture of concrete. Mater. Struct. 1983, 16, 155–177. [Google Scholar] [CrossRef] [Green Version]
- Ožbolt, J.; Gambarelli, S. Microplane model with relaxed kinematic constraint in the framework of micro polar Cosserat continuum. Eng. Fract. Mech. 2018, 199, 476–488. [Google Scholar] [CrossRef]
- Jirásek, M.; Bauer, M. Numerical aspects of the crack band approach. Comput. Struct. 2012, 110, 60–78. [Google Scholar] [CrossRef]
- Ožbolt, J.; Sharma, A.; Reinhardt, H.W. Dynamic fracture of concrete compact tension specimen. Int. J. Solids Struct. 2011, 48, 1534–1543. [Google Scholar] [CrossRef]
- Ožbolt, J.; Bošnjak, J.; Sola, E. Dynamic Fracture of Concrete Compact Tension Specimen: Experimental and Numerical Study. Int. J. Solids Struct. 2013, 50, 4270–4278. [Google Scholar] [CrossRef] [Green Version]
- Ožbolt, J.; Oršanić, F.; Balabanić, G. Modeling pull-out resistance of corroded reinforcement in concrete: Coupled three-dimensional finite element model. Cem. Concr. Compos. 2014, 46, 41–55. [Google Scholar] [CrossRef]
- FEMAP®, version 11.2.2; Siemens PLM Software: Plano, TX, USA, 2015.
- EAD 330008-03-0601; Anchor Channels, European Assessment Document. EOTA: Brussels, Belgium, 2018.
- Jebara, K.; Ožbolt, J.; Hoffman, J. Pryout failure of single headed stud anchor: 3D numerical FE analysis. Mater. Struct. 2016, 49, 4551–4563. [Google Scholar] [CrossRef]
- Odenbreit, C.; Vigneri, V.; Amadio, C.; Bedon, C.; Braun, M. New mechanical model to predict the load bearing resistance of shear connectors with modern forms of profiled sheeting. In Proceedings of the 13th International Conference on Steel, Space and Composite Structures, Perth, Australia, 31 January–2 February 2018. [Google Scholar]
- Hilti Anchor Channels (HAC) with Channel Bolts (HBC); DIBt: Berlin, Germany, 2016.
- CEB-FIP Model Code 1990; Comité Euro-International du Béton and Fédération Internationale de la Précontrainte. Tomas Telford: London, UK, 1993.
- EN 1994-1-1; Eurocode 4–Design of Composite Steel and Concrete Structures–Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization (CEN): Brussels, Belgium, 2004.







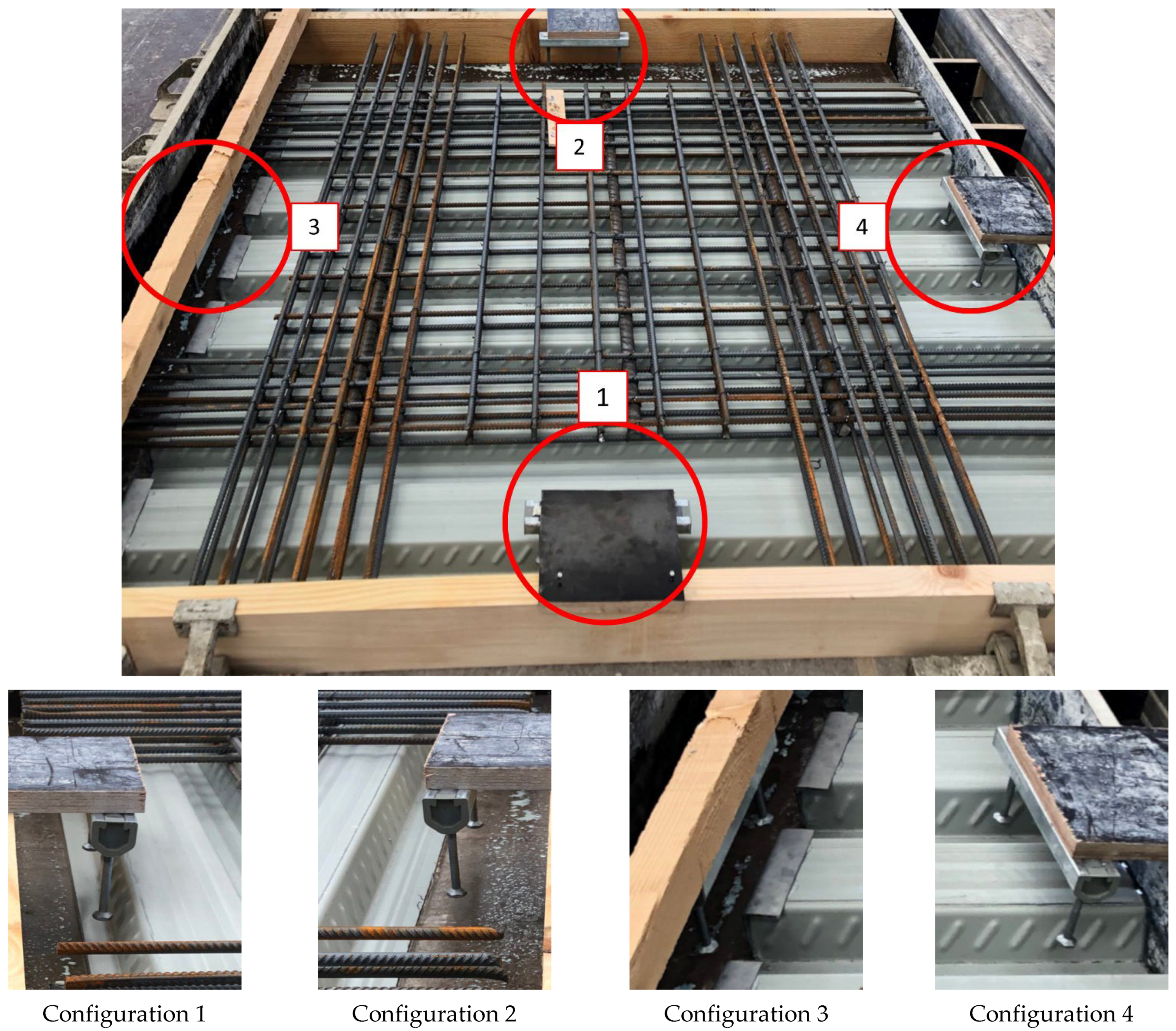


| Slab Type | c1 (mm) | hef (mm) | h (mm) | Nu,m (kN) | σ (kN) | CoV (%) | Nu,sim (kN) | Nu,sim/Nu,m (-) |
|---|---|---|---|---|---|---|---|---|
| Plain | 100 | 106 | 130 | 79.00 | 0.90 | 1.14 | 74.48 | 0.94 |
| Composite | 100 | 106 | 130 | 52.22 | 2.95 | 5.66 | 51.08 | 0.98 |
| Support Span | Nu (kN) | Nu/Nu,ref (-) |
|---|---|---|
| 2.5hef | 66.95 | 1.00 |
| 3.5hef | 58.82 | 0.88 |
| 4.5hef | 47.33 | 0.71 |
| 10hef | 26.57 | 0.40 |
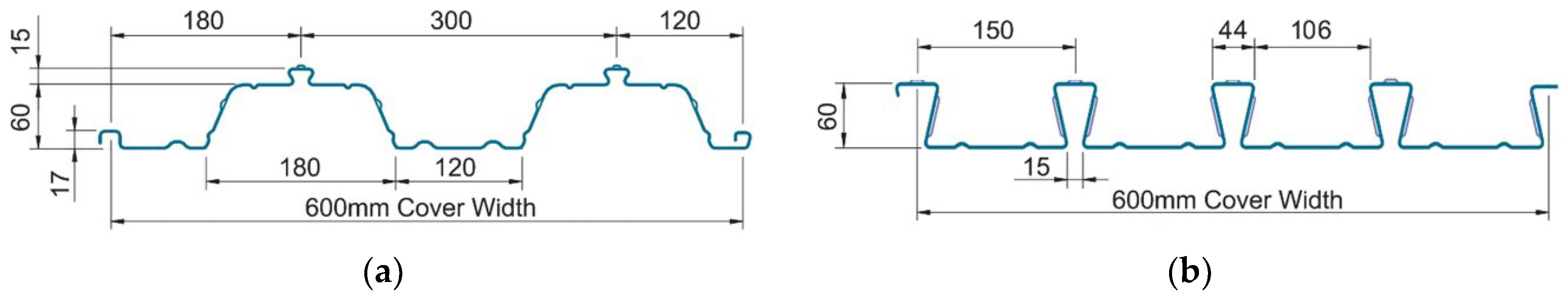
| Orientation | Installation | Profile Type | c1 (mm) | h (mm) | hef (mm) | dw (mm) | s (mm) |
|---|---|---|---|---|---|---|---|
| Parallel | Perimeter beam | Ribdeck S60 | 100 | 130 | 91, 106, 120 | 15, 30, 45, 60, 100 | 250 |
| 100 | 180 | 91, 106, 120 | 15, 30, 45, 60, 100 | 250 | |||
| 200 | 130 | 106 | 15, 30, 45, 60, 100 | 250 | |||
| Superib | 100 | 130 | 91, 106, 120 | 15, 30, 45, 60, 100 | 250 |
| Orientation | Installation | Profile Type | c1 (mm) | H (mm) | hef (mm) | dw (mm) | s (mm) |
|---|---|---|---|---|---|---|---|
| Perpendicular | Perimeter beam | Ribdeck S60 | 100 | 130 | 91, 106, 120 | 15, 30, 45, 60, 100 | 250 |
| Orientation | Installation | Profile Type | c1 (mm) | h (mm) | hef (mm) | dw (mm) | s (mm) |
|---|---|---|---|---|---|---|---|
| Perpendicular | Over the profile | Ribdeck S60 | 100 | 130 | 91, 106, 120 | Depends on configuration | 250, 300 |
| 100 | 180 | 120 | 250, 300 | ||||
| Superib | 100 | 130 | 91, 106, 120 | Depends on configuration | 150, 200 |
| Configuration | s = 250 mm | s = 300 mm |
|---|---|---|
| Symmetric |  |  |
| Asymmetric | 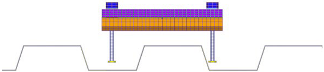 | 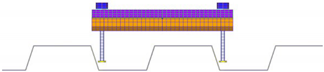 |
| Configuration | Nu,m (kN) | ntest (-) | σ (kN) | CoV (%) | Nu,m/Nu,m,ref (-) |
|---|---|---|---|---|---|
| Reference | 65.18 | 4 | 1.87 | 2.86 | 1.00 |
| 1 | 46.30 | 3 | 2.28 | 4.93 | 0.71 |
| 2 | 58.40 | 3 | 2.39 | 4.09 | 0.90 |
| 3 | 43.21 | 3 | 1.54 | 3.56 | 0.66 |
| 4 | 56.59 | 3 | 3.60 | 6.35 | 0.87 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bogdanić, A.; Casucci, D.; Ožbolt, J. Numerical and Experimental Investigation of Anchor Channels Subjected to Tension Load in Composite Slabs with Profiled Steel Decking. CivilEng 2022, 3, 296-315. https://doi.org/10.3390/civileng3020018
Bogdanić A, Casucci D, Ožbolt J. Numerical and Experimental Investigation of Anchor Channels Subjected to Tension Load in Composite Slabs with Profiled Steel Decking. CivilEng. 2022; 3(2):296-315. https://doi.org/10.3390/civileng3020018
Chicago/Turabian StyleBogdanić, Anton, Daniele Casucci, and Joško Ožbolt. 2022. "Numerical and Experimental Investigation of Anchor Channels Subjected to Tension Load in Composite Slabs with Profiled Steel Decking" CivilEng 3, no. 2: 296-315. https://doi.org/10.3390/civileng3020018
APA StyleBogdanić, A., Casucci, D., & Ožbolt, J. (2022). Numerical and Experimental Investigation of Anchor Channels Subjected to Tension Load in Composite Slabs with Profiled Steel Decking. CivilEng, 3(2), 296-315. https://doi.org/10.3390/civileng3020018






