Thermal Analysis of Mass Concrete Containing Ground Granulated Blast Furnace Slag
Abstract
1. Introduction
2. Thermal Analysis
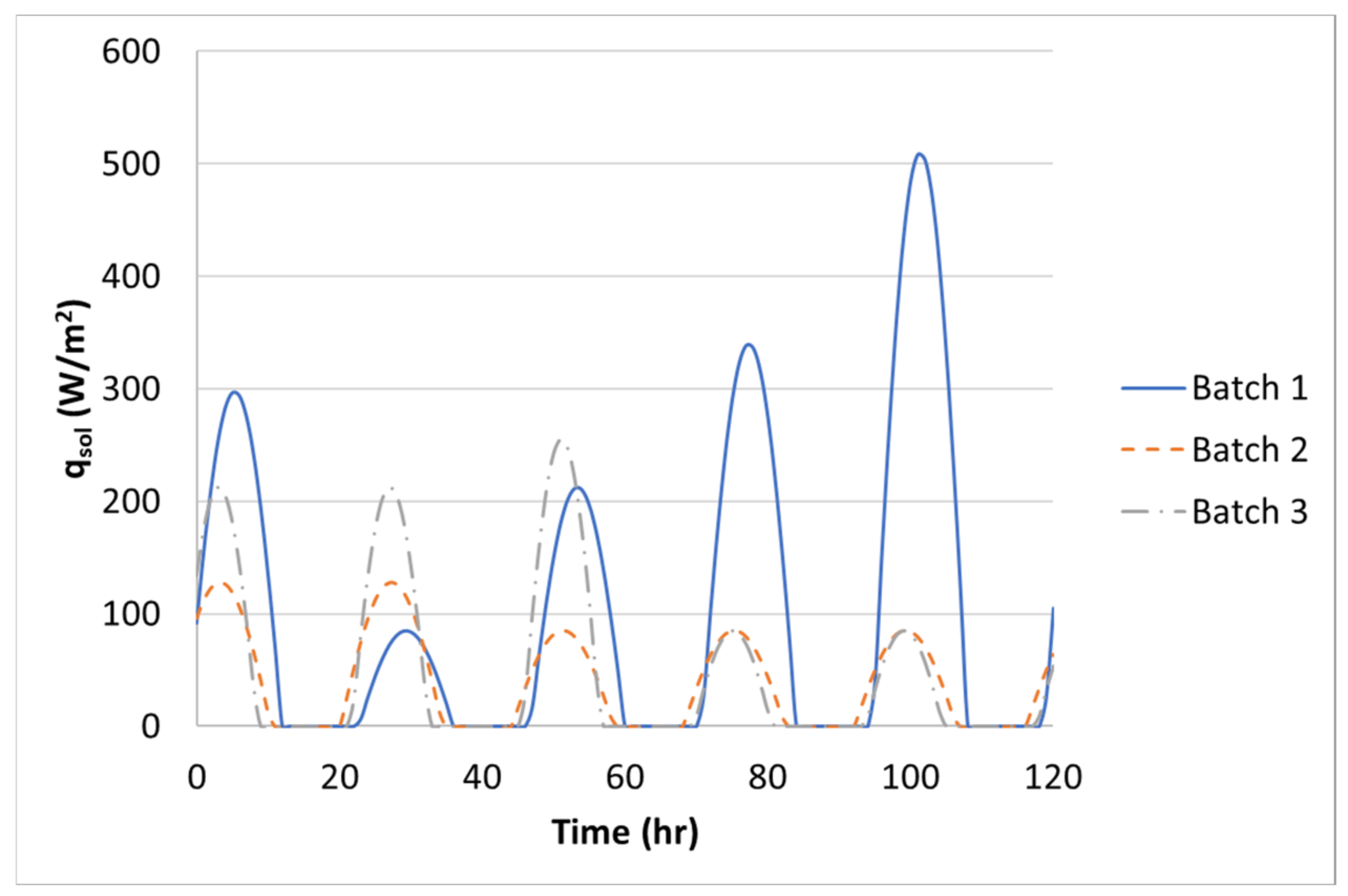
3. Experiments
3.1. Thermal Properties
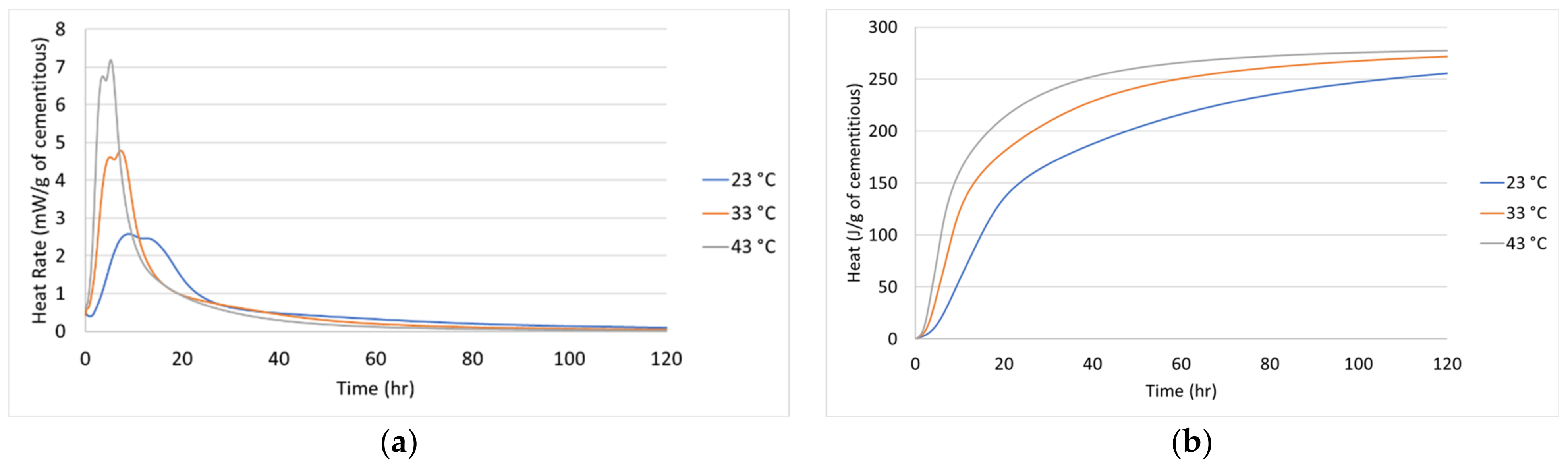
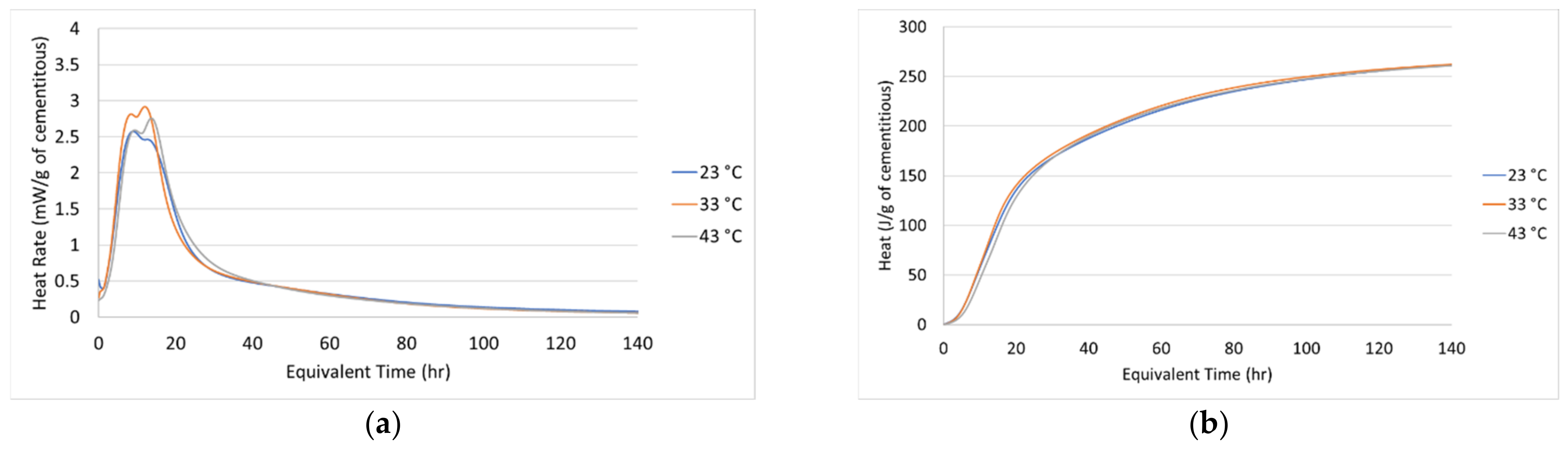
3.1.1. Activation Energy
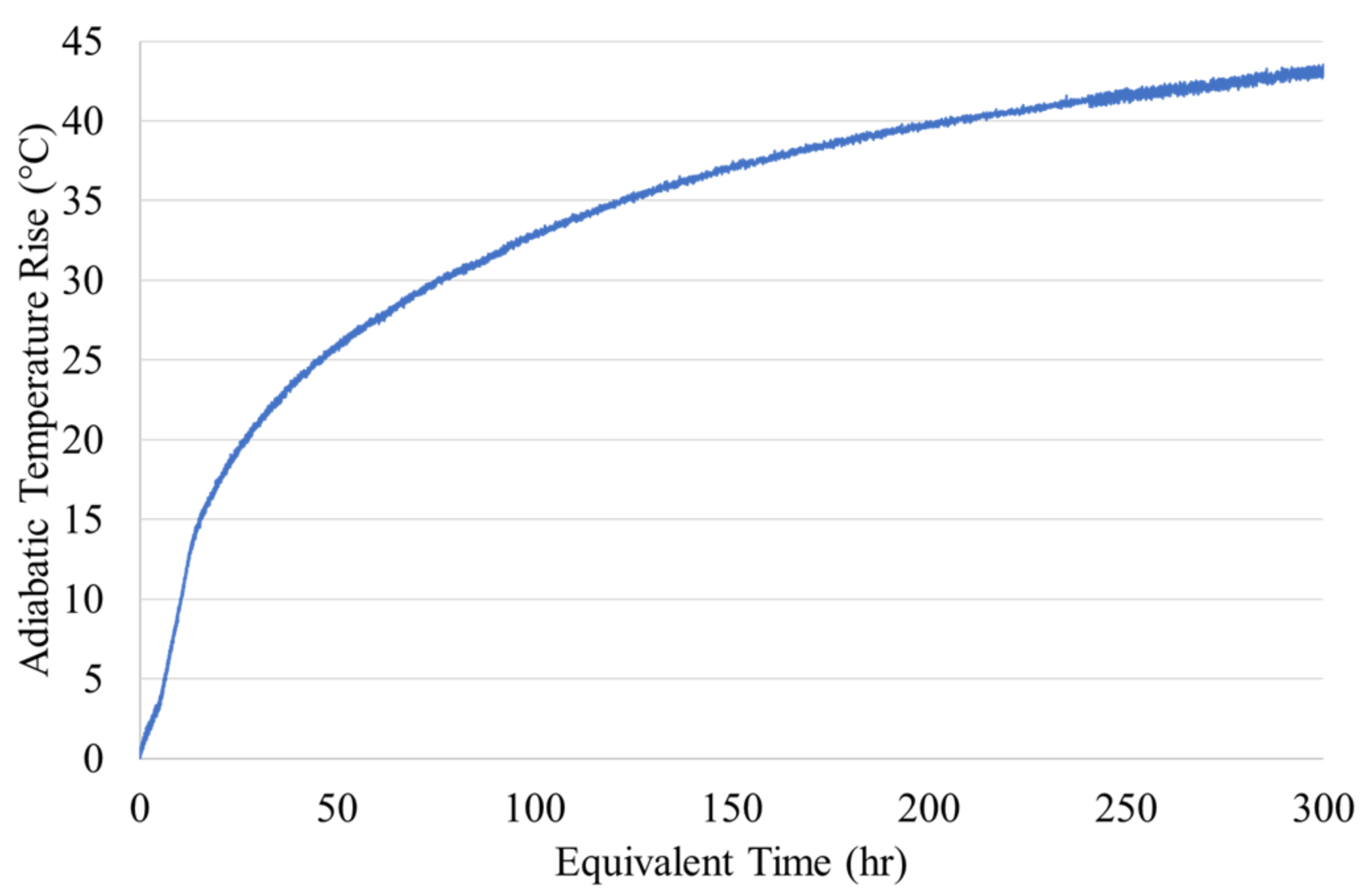
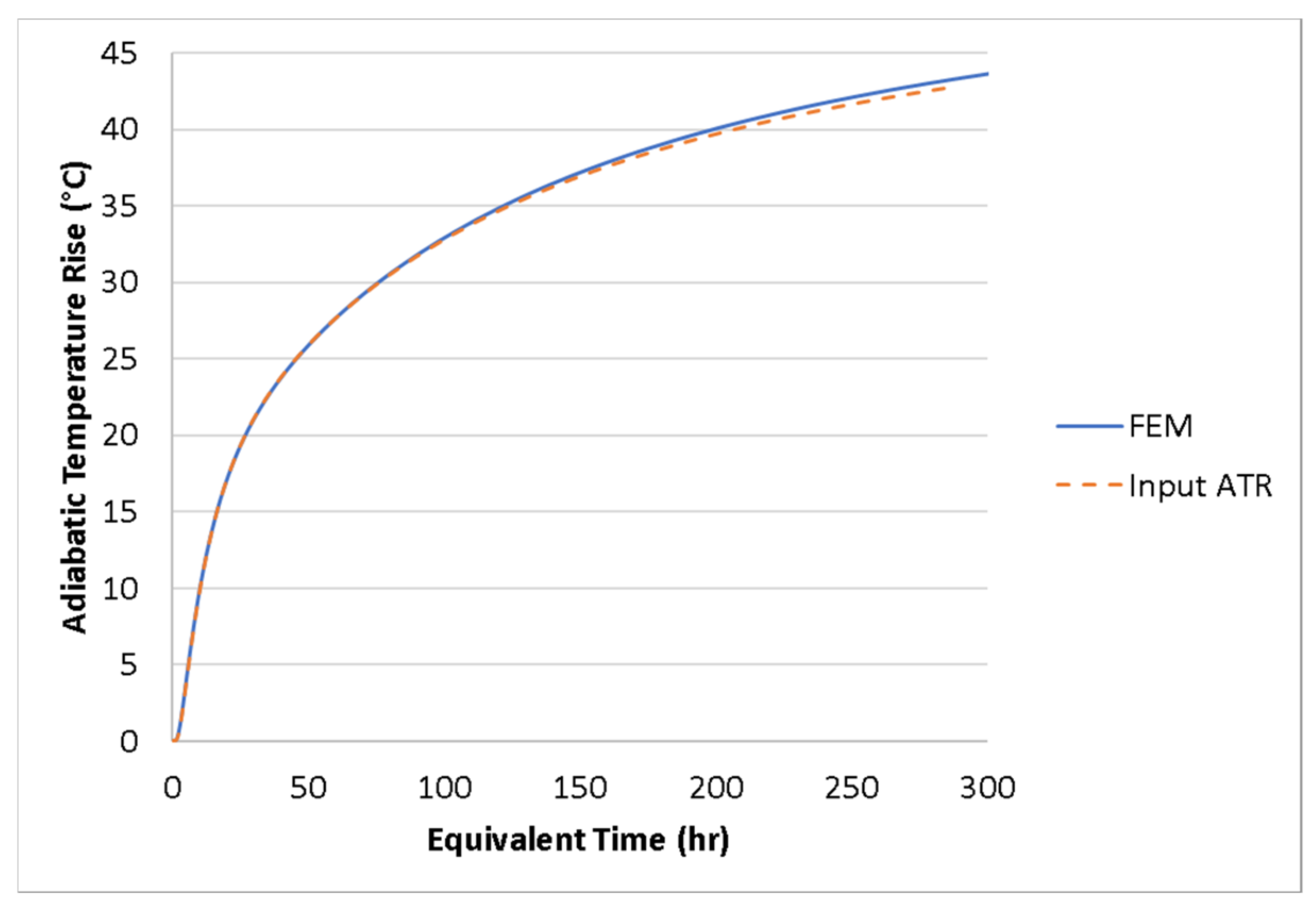
3.1.2. Adiabatic Temperature Rise
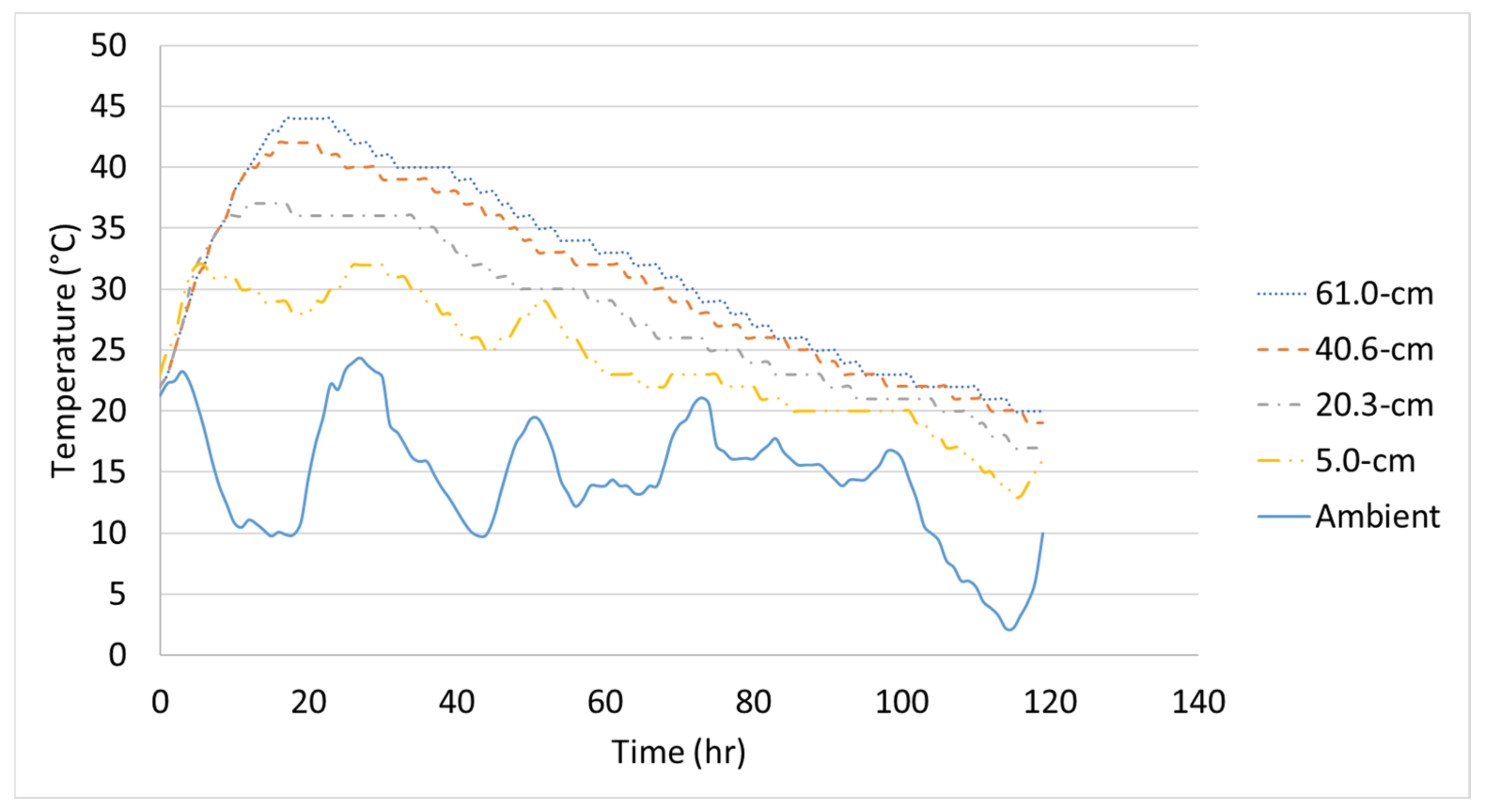
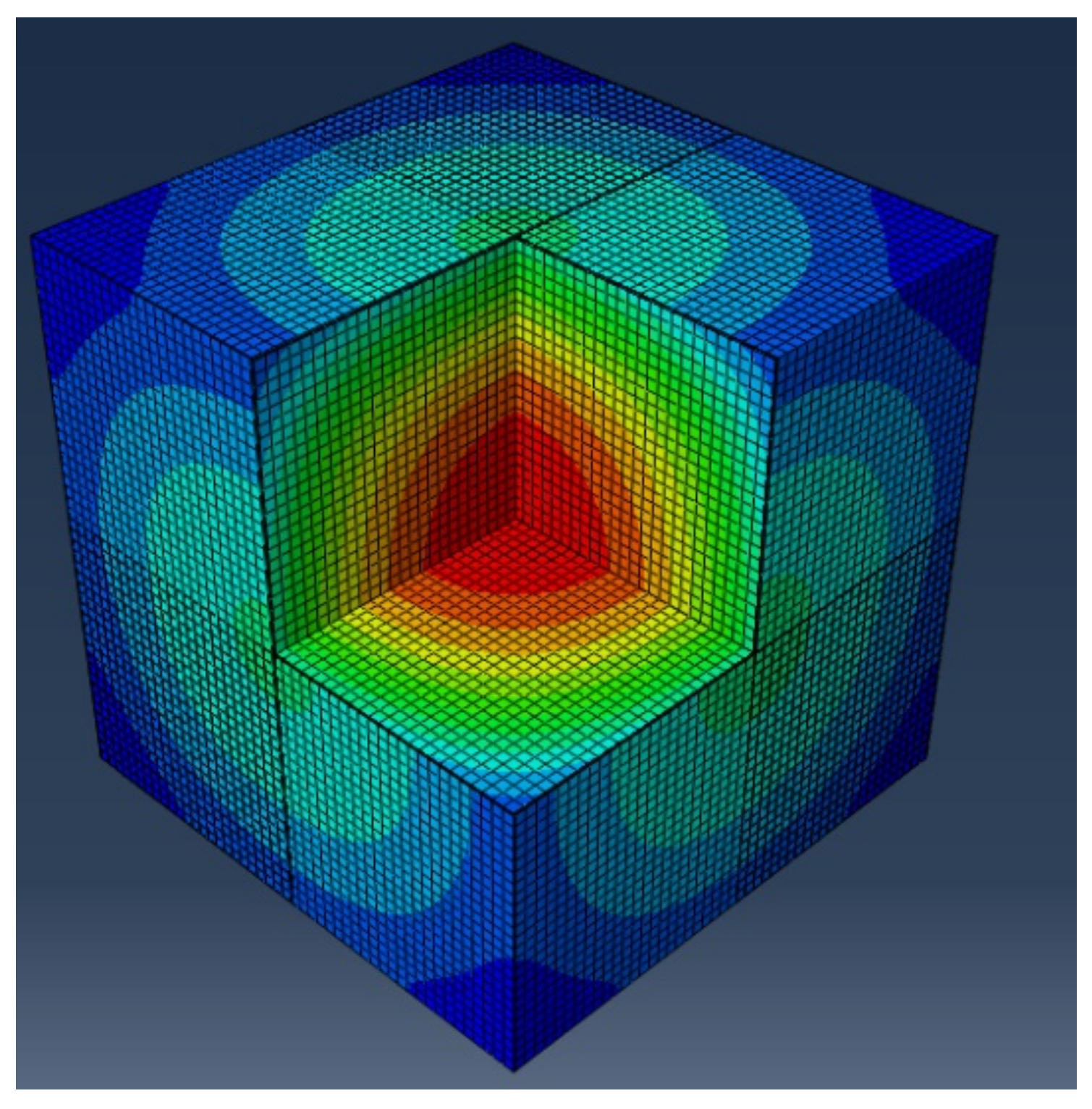
3.1.3. Cube Testing (1.2-m)
4. Finite Element Analysis
4.1. Verification of User Subroutines (DFLUX and USDFLD)
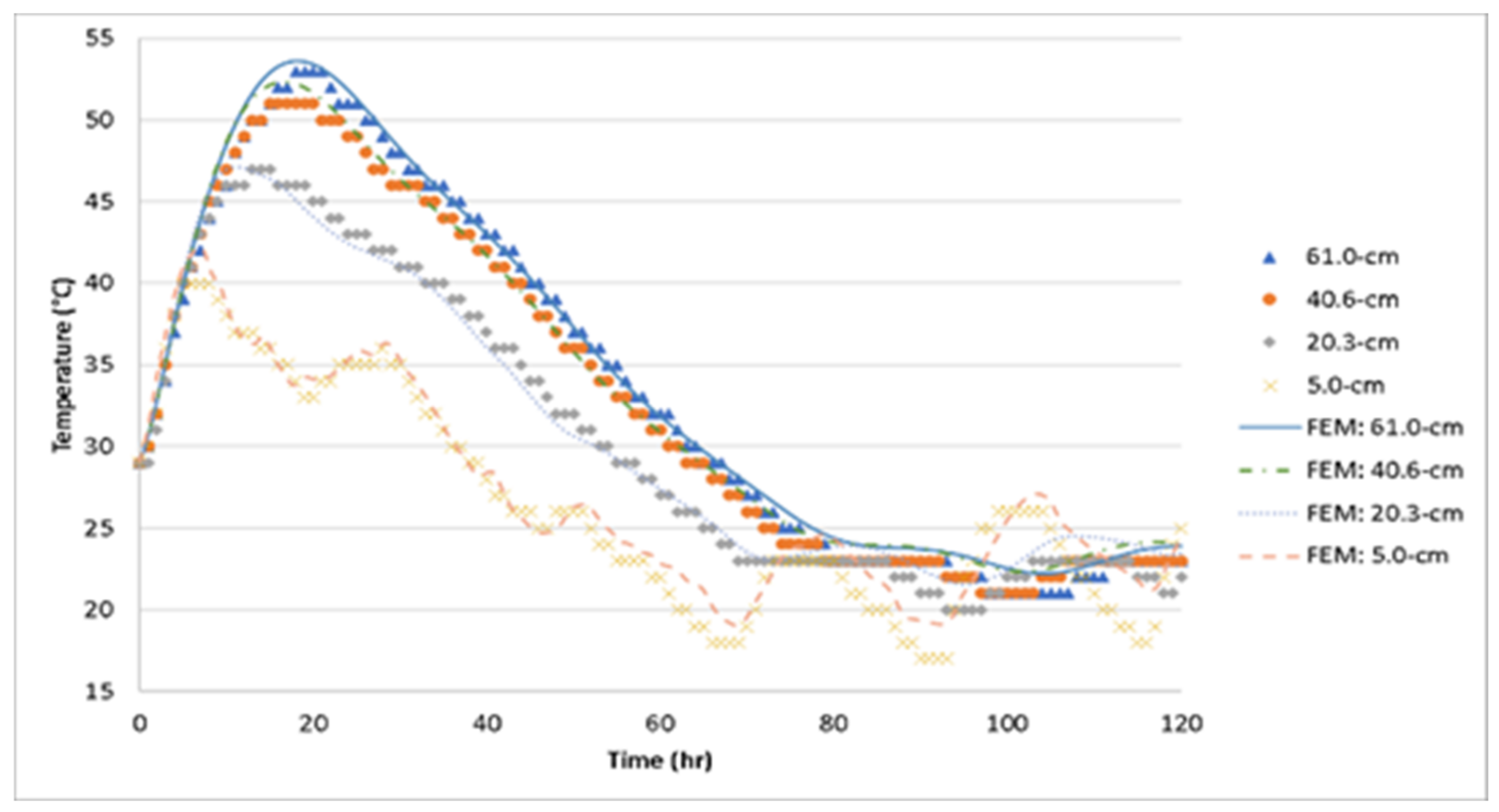
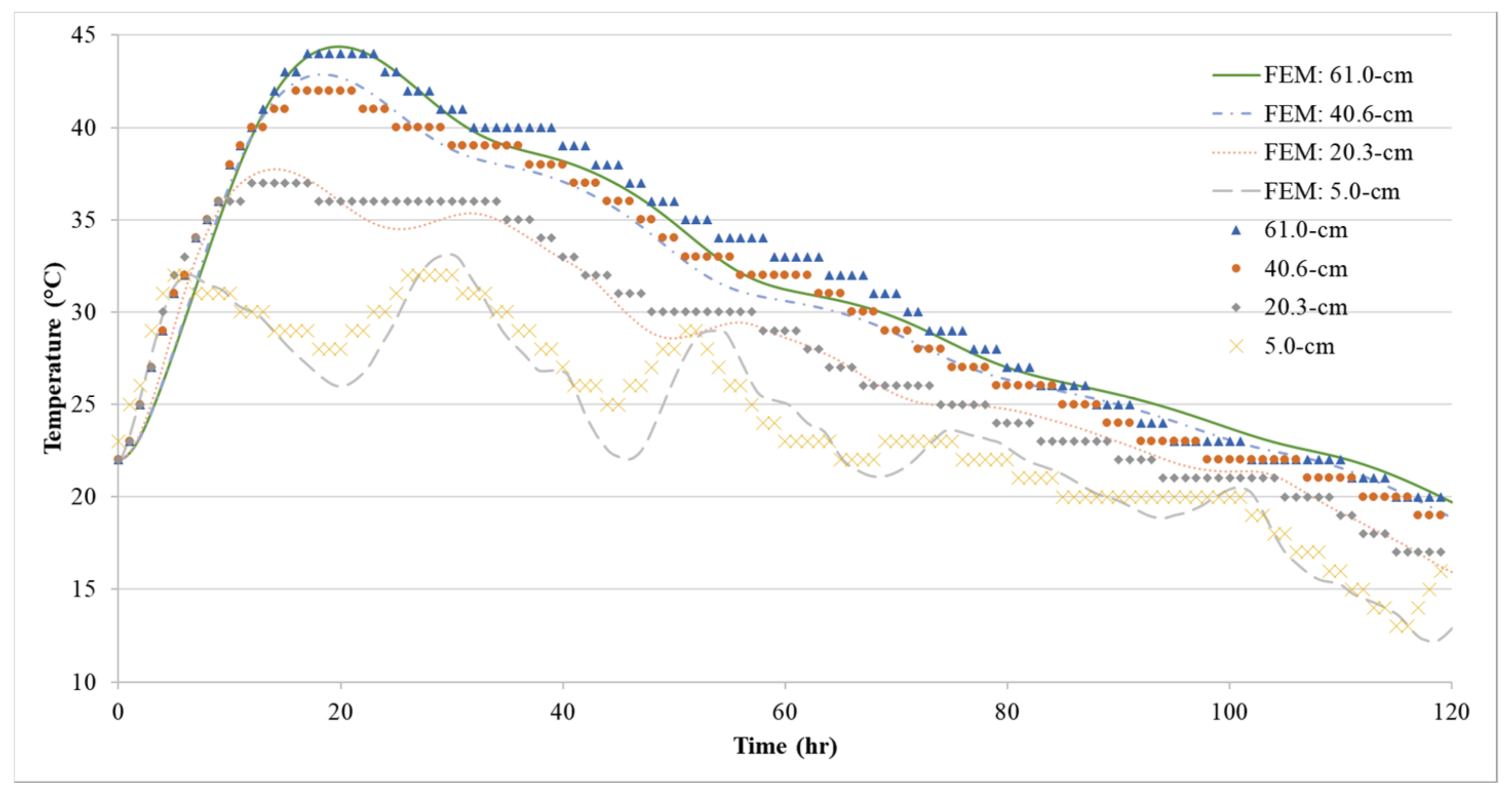
4.2. Temperature Analysis
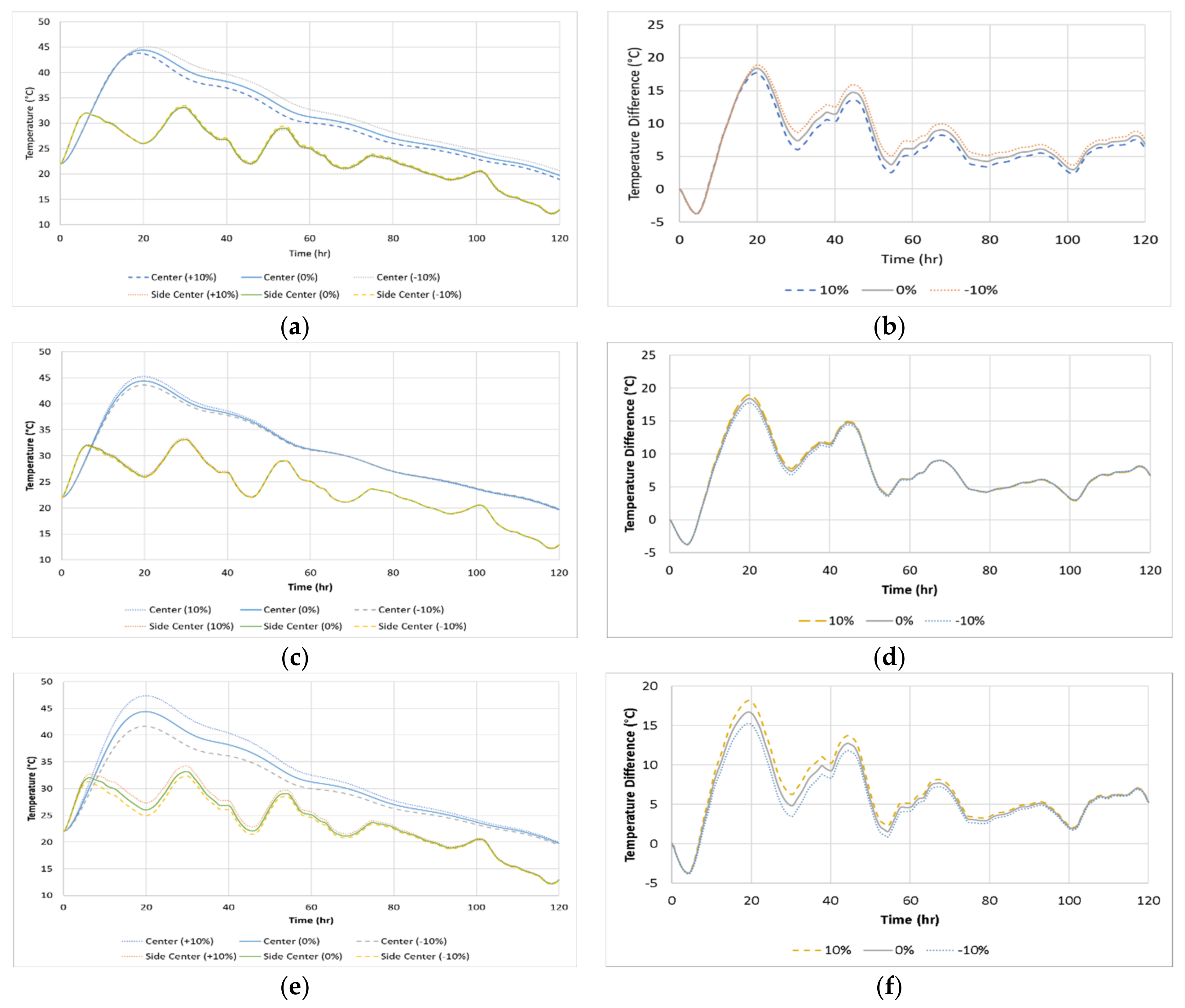
5. Sensitivity Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Riding, K.A.; Poole, J.L.; Schindler, A.K.; Juenger, M.C.; Folliard, K.J. Evaluation of Temperature Prediction Methods for Mass Concrete Members. ACI Mater. J. 2006, 103, 357–365. [Google Scholar] [CrossRef]
- Amin, M.N.; Kim, J.-S.; Lee, Y.; Kim, J.-K. Simulation of the thermal stress in mass concrete using a thermal stress measuring device. Cem. Concr. Res. 2009, 39, 154–164. [Google Scholar] [CrossRef]
- Ballim, Y. A numerical model and associated calorimeter for predicting temperature profiles in mass concrete. Cem. Concr. Compos. 2004, 26, 695–703. [Google Scholar] [CrossRef]
- Bobko, C.P.; Seracino, R.; Zia, P.; Edwards, A. Crack Free Mass Concrete Footings on Bridges in Coastal Environments. Master’s Thesis, North Carolina State University, Raleigh, NC, USA, 2013. [Google Scholar]
- De Borst, R.; Boogaard, A.H.V.D. Finite-Element Modeling of Deformation and Cracking in Early-Age Concrete. J. Eng. Mech. 1994, 120, 2519–2534. [Google Scholar] [CrossRef]
- Do, T.A. Influence of Footing Dimensions on Early-Age Temperature Development and Cracking in Concrete Footings. J. Bridg. Eng. 2015, 20, 06014007. [Google Scholar] [CrossRef]
- Do, T.; Chen, H.; Leon, G.; Nguyen, T. A combined finite difference and finite element model for temperature and stress predictions of cast-in-place cap beam on precast columns. Constr. Build. Mater. 2019, 217, 172–184. [Google Scholar] [CrossRef]
- Lawrence, A.M.; Tia, M.; Ferraro, C.C.; Bergin, M. Effect of Early Age Strength on Cracking in Mass Concrete Containing Different Supplementary Cementitious Materials: Experimental and Finite-Element Investigation. J. Mater. Civ. Eng. 2012, 24, 362–372. [Google Scholar] [CrossRef]
- Lin, Y.; Chen, H.-L. Thermal analysis and adiabatic calorimetry for early-age concrete members. J. Therm. Anal. Calorim. 2015, 122, 937–945. [Google Scholar] [CrossRef]
- Saeed, M.K.; Rahman, M.K.; Baluch, M.H. Early age thermal cracking of mass concrete blocks with Portland cement and ground granulated blast-furnace slag. Mag. Concr. Res. 2016, 68, 1–17. [Google Scholar] [CrossRef]
- Sargam, Y.; Faytarouni, M.; Riding, K.; Wang, K.; Jahren, C.; Shen, J. Predicting thermal performance of a mass concrete foundation—A field monitoring case study. Case Stud. Constr. Mater. 2019, 11, e00289. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Cho, H.-K.; Lee, H.-S. Prediction of temperature distribution in concrete incorporating fly ash or slag using a hydration model. Compos. Part B Eng. 2011, 42, 27–40. [Google Scholar] [CrossRef]
- Wu, S.; Huang, D.; Lin, F.-B.; Zhao, H.; Wang, P. Estimation of cracking risk of concrete at early age based on thermal stress analysis. J. Therm. Anal. Calorim. 2011, 105, 171–186. [Google Scholar] [CrossRef]
- Wang, X.Y.; Lee, H.S. Prediction of compressive strength of slag concrete using a blended cement hydration model. Comput. Concr. 2014, 14, 247–262. [Google Scholar] [CrossRef]
- Yikici, T.A.; Chen, H.L. Numerical Prediction Model for Temperature Development in Mass Concrete Structures. J. Transp. Res. Board 2015, 2508, 102–110. [Google Scholar] [CrossRef]
- Klemczak, B.; Batog, M.; Giergiczny, Z.; Żmij, A. Complex Effect of Concrete Composition on the Thermo-Mechanical Behaviour of Mass Concrete. Materials 2018, 11, 2207. [Google Scholar] [CrossRef] [PubMed]
- Moon, H.; Ramanathan, S.; Suraneni, P.; Shon, C.-S.; Lee, C.-J.; Chung, C.-W. Revisiting the Effect of Slag in Reducing Heat of Hydration in Concrete in Comparison to Other Supplementary Cementitious Materials. Materials 2018, 11, 1847. [Google Scholar] [CrossRef] [PubMed]
- De Schutter, G. Applicability of degree of hydration concept and maturity method for thermo-visco-elastic behaviour of early age concrete. Cem. Concr. Compos. 2004, 26, 437–443. [Google Scholar] [CrossRef]
- Freiesleben, H.P.; Pedersen, E.J. Måleinstrument til kontrol af betons haerdning. J. Nord. Concr. 1977, 1, 21–25. [Google Scholar]
- Poole, J.L.; Riding, K.A.; Folliard, K.J.; Juenger, M.C.G.; Schindler, A.K. Methods for Calculating Activation Energy for Portland Cement. ACI Mater. J. 2007, 104, 303–311. [Google Scholar]
- Robbins, M.E. Predicting the Early Age Temperature Response of Concrete Using Isothermal Calorimetry. Master Thesis, University of Toronto, Toronto, ON, Canada, 2007. [Google Scholar]
- Heat of Hydration Models for Cementitious Materials. ACI Mater. J. 2005, 102, 24–33. [CrossRef]
- Bougara, A.; Lynsdale, C.; Milestone, N. Reactivity and performance of blast furnace slags of differing origin. Cem. Concr. Compos. 2010, 32, 319–324. [Google Scholar] [CrossRef]
- Gruyaert, E.; Robeyst, N.; De Belie, N. Study of the hydration of Portland cement blended with blast-furnace slag by calorimetry and thermogravimetry. J. Therm. Anal. Calorim. 2010, 102, 941–951. [Google Scholar] [CrossRef]
- Woo, H.-M.; Kim, C.-Y.; Yeon, J.H. Heat of hydration and mechanical properties of mass concrete with high-volume GGBFS replacements. J. Therm. Anal. Calorim. 2018, 132, 599–609. [Google Scholar] [CrossRef]
- Mills, R.H. Factors Influencing Cessation of Hydration in Water Cured Cement Pastes, Highway Research Board Special Report. 1966. Available online: https://trid.trb.org/view/101449 (accessed on 2 August 2019).
- Maekawa, K.; Ishida, T.; Kishi, T. Multi-Scale Modeling of Structural Concrete; Routledge: London, UK, 2008. [Google Scholar]
- Van Breugel, K. Prediction of temperature development in hardening concrete. In Prevention of Thermal Cracking in Concrete at Early Ages; CRC Press: Boca Raton, FL, USA, 1998; Volume 51, pp. 51–75. [Google Scholar]
- Chen, H.-L.R.; Mardmomen, S.; Leon, G. On-site measurement of heat of hydration of delivered mass concrete. Constr. Build. Mater. 2021, 269, 121246. [Google Scholar] [CrossRef]
- Krishnaiah, S.; Singh, D. Determination of thermal properties of some supplementary cementing materials used in cement and concrete. Constr. Build. Mater. 2006, 20, 193–198. [Google Scholar] [CrossRef]
- Aloia, L.D. Early Age Kinetics: Activation Energy, Maturity and Equivalent Age. In Early Age Cracking in Cementitious System-Report of RILEM Technical Committe 181-EAS—Early Age Shrinkage Induced Stress and Cracking in Cementitious Systems; RILEM Publications SARL: Paris, France, 2003; pp. 127–148. Available online: https://www.rilem.net/publication/publication/89?id_papier=56 (accessed on 28 May 2020).
- Xu, Q.; Ruiz, J.M.; Hu, J.; Wang, K.; Rasmussen, R.O. Modeling hydration properties and temperature developments of early-age concrete pavement using calorimetry tests. Thermochim. Acta 2011, 512, 76–85. [Google Scholar] [CrossRef]
- Kim, J.K.; Han, S.H.; Lee, K.M. Estimation of compressive strength by a new apparent activation energy function. Cem. Concr. Res. 2001, 31, 217–225. [Google Scholar] [CrossRef]
- Kada-Benameur, H.; Wirquin, E.; Duthoit, B. Determination of apparent activation energy of concrete by isothermal calorimetry. Cem. Concr. Res. 2000, 30, 301–305. [Google Scholar] [CrossRef]
- Riding, K.A.; Poole, J.L.; Folliard, K.J.; Juenger, M.C.; Schindler, A.K. New Model for Estimating Apparent Activation Energy of Cementitious Systems. ACI Mater. J. 2011, 108, 550–557. [Google Scholar] [CrossRef]
- Brooks, A.G.; Schindler, A.K.; Barnes, R.W. Maturity Method Evaluated for Various Cementitious Materials. J. Mater. Civ. Eng. 2007, 19, 1017–1025. [Google Scholar] [CrossRef]
- Riding, K.A.; Poole, J.L.; Schindler, A.K.; Juenger, M.C.G.; Folliard, K.J. Statistical Determination of Cracking Probability for Mass Concrete. J. Mater. Civ. Eng. 2014, 26, 04014058. [Google Scholar] [CrossRef]
- US Army Corps of Engineers. Method of Test for Thermal Diffusivity of Concrete. 1973. Available online: https://www.wbdg.org/ffc/army-coe/standards/crd-c36 (accessed on 2 August 2019).
- Ge, Z. Predicting Temperature and Strength Development of the Field Concrete. Ph.D. Thesis, Iowa State University, Ames, IA, USA, 2005. [Google Scholar]
- Schindler, A.K. Concrete Hydration, Temperature Development and Setting at Early-Ages. Ph.D. Thesis, The University of Texas, Austin, TX, USA, 2002. [Google Scholar]
- Henninger, J.H. Solar Absorptance and Thermal Emittance of Some Common Spacecraft Thermal Control Coatings; NASA: Greenbelt, MD, USA, 1984.








| Material | Quantity (kg/m3) |
|---|---|
| Cement | 150.7 |
| GGBFS | 150.7 |
| Water | 126.6 |
| #57 Limestone Aggregate | 1064.9 |
| Fine Aggregate | 809.2 |
| Air entrainer (oz/cwt) | 0.65 |
| Type B/D Water reducer/retarder (oz/cwt) | 3.0 |
| w/cem | 0.42 |
| Batch | Air Content, % | Slump, cm | Initial Temperature, °C |
|---|---|---|---|
| In-Lab | 6.0 | 13.3 | 21.66 |
| Batch 1 | 5.5 | 12.7 | 26.0 |
| Batch 2 | 6.5 | 20.3 | 29.0 |
| Batch 3 | 6.8 | 15.2 | 22.0 |
| Chemical Component | Portland Cement | Grade 100 GGBFS |
|---|---|---|
| CaO | 63.86% | 47.48% |
| SiO2 | 20.34% | 28.89% |
| Al2O3 | 4.78% | 8.27% |
| Fe2O3 | 3.19% | 1.93% |
| SO3 | 3.01% | 0.73% |
| MgO | 2.41% | 8.34% |
| Na2O | 0.06% | - |
| K2O | 0.65% | 0.66% |
| Blaine fineness | 372 | 325 |
| Hydration Parameters | Two-Term | One-Term |
|---|---|---|
| 0.56685 | 0.8552 | |
| 14.1090 | 28.62 | |
| 0.78485 | 0.609 | |
| 0.28839 | - | |
| 166.985 | - | |
| 0.97925 | - | |
| 0.8552 | 0.8552 |
| Thermal Properties | ||||||
|---|---|---|---|---|---|---|
| Value | 301.4 | 473,050 | 39,778 | 1.65 | 0.8552 | 0.42 |
| Batch | Maximum Temperature (°C) | Maximum Temperature Difference (°C) | ||||
|---|---|---|---|---|---|---|
| Experiment | FEM Model | % Error | Experiment | FEM Model | % Error | |
| 1 | 50 at 20 h | 50.2 at 21.75 h | 0.4% | 18 at 21 h | 17.1 at 23.5 h | 0.56% |
| 2 | 53 at 20 h | 53.6 at 18.25 h | 1.13% | 20 at 20 h | 19.86 at 18 h | 0.7% |
| 3 | 44 at 20 h | 44.4 at 19.75 h | 0.91% | 16 at 19 h | 18.41 at 20 h | 15.1% |
| Thermal Property | Maximum Temperature (°C) | Time of Maximum Temperature (hr) | Maximum Temperature Difference (°C) | Time of Maximum Temperature Difference (hr) |
|---|---|---|---|---|
| Thermal conductivity (±10%) | 44.4 ± 0.85 | 19.75 ± 1.0 | 18.41 ± 0.70 | 20.0 ± 0.25 |
| Activation energy (±10%) | 44.4 ± 0.85 | 19.75 ± 0.0 | 18.41 ± 0.65 | 20.0 ± 0.00 |
| ATR (±10%) | 44.4 ± 2.95 | 19.75 ± 0.25 | 18.41 ± 1.69 | 20.0 ± 0.00 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leon, G.; Chen, H.-L. Thermal Analysis of Mass Concrete Containing Ground Granulated Blast Furnace Slag. CivilEng 2021, 2, 254-270. https://doi.org/10.3390/civileng2010014
Leon G, Chen H-L. Thermal Analysis of Mass Concrete Containing Ground Granulated Blast Furnace Slag. CivilEng. 2021; 2(1):254-270. https://doi.org/10.3390/civileng2010014
Chicago/Turabian StyleLeon, Guadalupe, and Hung-Liang (Roger) Chen. 2021. "Thermal Analysis of Mass Concrete Containing Ground Granulated Blast Furnace Slag" CivilEng 2, no. 1: 254-270. https://doi.org/10.3390/civileng2010014
APA StyleLeon, G., & Chen, H.-L. (2021). Thermal Analysis of Mass Concrete Containing Ground Granulated Blast Furnace Slag. CivilEng, 2(1), 254-270. https://doi.org/10.3390/civileng2010014







