The Effect of Increasing Thread Depth on the Initial Stability of Dental Implants: An In Vitro Study
Abstract
1. Introduction
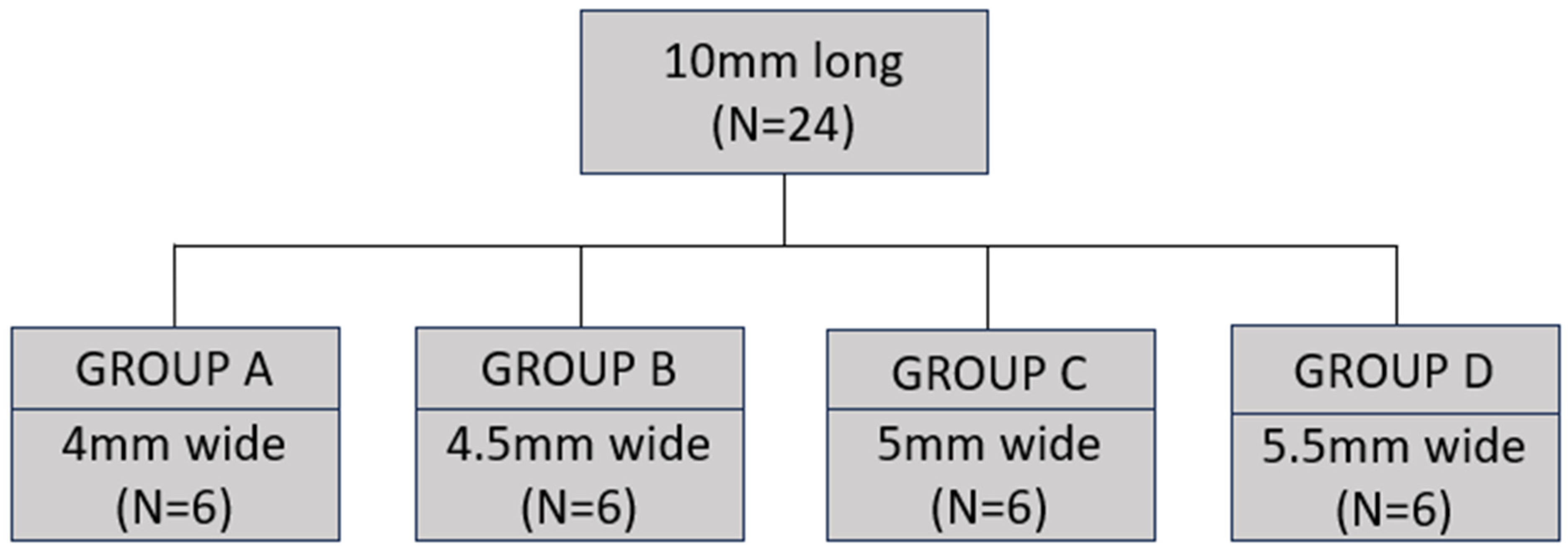
2. Materials and Methods
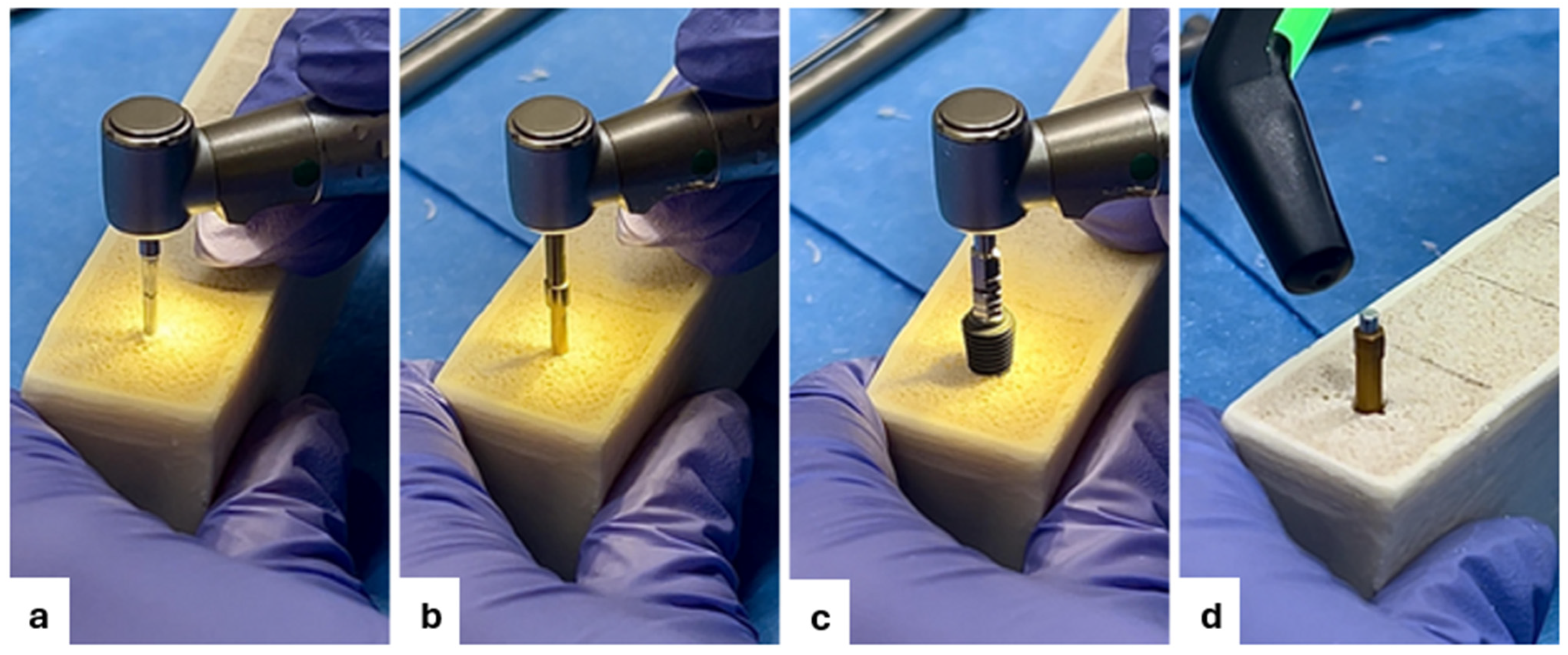
2.1. In Vitro Implant Placement Procedure
2.2. Implant Bed Preparation Sequence
2.3. Statistical Analysis
3. Results
3.1. IT Results
3.2. ISQ Results
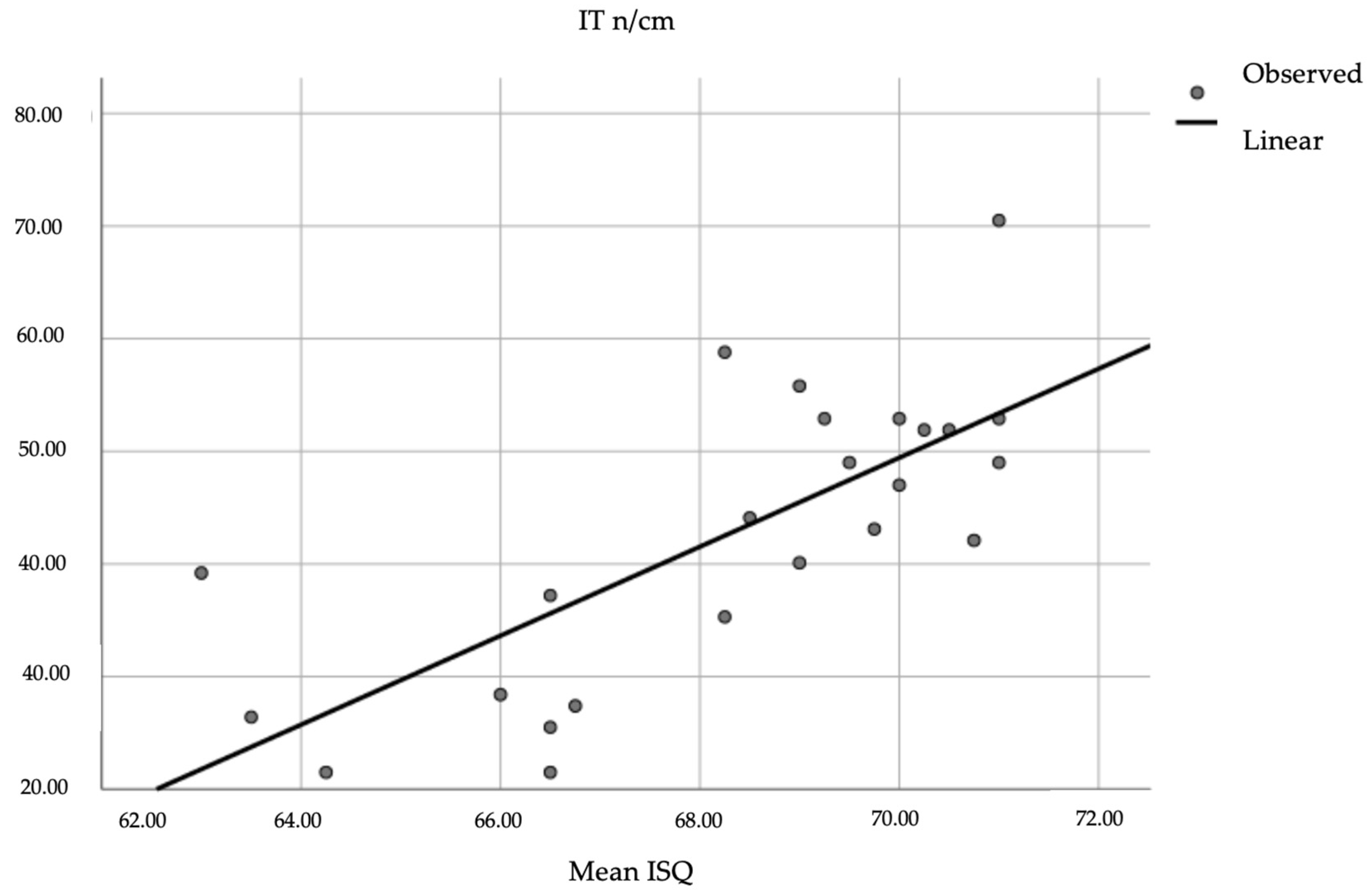
3.3. IT and ISQ Correlation Results
4. Discussion
4.1. Discussion of the IT Results
4.2. Discussion of the ISQ Results
4.3. Discussion of the IT and ISQ Correlation Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lozano-Carrascal, N.; Salomo-Coll, O.; Gilabert-Cerda, M.; Farre-Pages, N.; Gargallo-Albiol, J.; Hernandez-Alfaro, F. Effect of implant macro-design on primary stability: A prospective clinical study. Med. Oral Patol. Oral Cir. Bucal 2016, 21, e214–e221. [Google Scholar] [CrossRef] [PubMed]
- Makary, C.; Menhall, A.; Zammarie, C.; Lombardi, T.; Lee, S.Y.; Stacchi, C.; Park, K.B. Primary stability optimization by using fixtures with different thread depth according to bone density: A clinical prospective study on early loaded implants. Materials 2019, 12, 2398. [Google Scholar] [CrossRef]
- Stoilov, M.; Shafaghi, R.; Stark, H.; Marder, M.; Kraus, D.; Enkling, N. Influence of implant macro-design, -length, and -diameter on primary implant stability depending on different bone qualities using standard drilling protocols—An in vitro analysis. J. Funct. Biomater. 2023, 14, 469. [Google Scholar] [CrossRef] [PubMed]
- Bilhan, H.; Geckili, O.; Mumcu, E.; Bozdag, E.; Sünbüloğlu, E.; Kutay, O. Influence of surgical technique, implant shape and diameter on the primary stability in cancellous bone. J. Oral Rehabil. 2010, 37, 900–907. [Google Scholar] [CrossRef]
- Atieh, M.A.; Alsabeeha, N.; Duncan, W.J. Stability of tapered and parallel-walled dental implants: A systematic review and meta-analysis. Clin. Implant. Dent. Relat. Res. 2018, 20, 634–645. [Google Scholar] [CrossRef]
- Lee, S.-Y.; Kim, S.-J.; An, H.-W.; Kim, H.-S.; Ha, D.-G.; Ryo, K.-H.; Park, K.-B. The effect of the thread depth on the mechanical properties of the dental implant. J. Adv. Prosthodont. 2015, 7, 115–121. [Google Scholar] [CrossRef] [PubMed]
- Sarfaraz, H.; Johri, S.; Sucheta, P.; Rao, S. Study to assess the relationship between insertion torque value and implant stability quotient and its influence on timing of functional implant loading. J. Indian Prosthodont. Soc. 2018, 18, 139–146. [Google Scholar] [CrossRef]
- Mavrogenis, A.F.; Dimitriou, R.; Parvizi, J.; Babis, G.C. Biology of implant osseointegration. J. Musculoskelet. Neuronal Interact. 2009, 9, 61–71. [Google Scholar]
- Smeets, R.; Stadlinger, B.; Schwarz, F.; Beck-Broichsitter, B.; Jung, O.; Precht, C.; Kloss, F.; Gröbe, A.; Heiland, M.; Ebker, T. Impact of dental implant surface modifications on osseointegration. Biomed Res. Int. 2016, 2016, 6285620. [Google Scholar] [CrossRef]
- Falco, A.; Berardini, M.; Trisi, P. Correlation between implant geometry, implant surface, insertion torque, and primary stability: In vitro biomechanical analysis. Int. J. Oral Maxillofac. Implant. 2018, 33, 824–830. [Google Scholar] [CrossRef]
- Dos Santos, M.V.; Elias, C.N.; Cavalcanti Lima, J.H. The effects of superficial roughness and design on the primary stability of dental implants. Clin. Implant. Dent. Relat. Res. 2011, 13, 215–223. [Google Scholar] [CrossRef] [PubMed]
- do Vale Souza, J.P.; de Moraes Melo Neto, C.L.; Piacenza, L.T.; Freitas da Silva, E.V.; de Melo Moreno, A.L.; Penitente, P.A.; Brunetto, J.L.; dos Santos, D.M.; Goiato, M.C. Relation between insertion torque and implant stability quotient: A clinical study. Eur. J. Dent. 2021, 15, 618–623. [Google Scholar] [CrossRef]
- Heimes, D.; Becker, P.; Pabst, A.; Smeets, R.; Kraus, A.; Hartmann, A.; Sagheb, K.; Kämmerer, P.W. How does dental implant macrogeometry affect primary implant stability? A narrative review. Int. J. Implant. Dent. 2023, 9, 20. [Google Scholar] [CrossRef] [PubMed]
- Möhlhenrich, S.C.; Heussen, N.; Elvers, D.; Steiner, T.; Hölzle, F.; Modabber, A. Compensating for poor primary implant stability in different bone densities by varying implant geometry: A laboratory study. Int. J. Oral Maxillofac. Surg. 2015, 44, 1514–1520. [Google Scholar] [CrossRef] [PubMed]
- Huang, H.; Wu, G.; Hunziker, E. The clinical significance of implant stability quotient (ISQ) measurements: A literature review. J. Oral Biol. Craniofacial Res. 2020, 10, 629–638. [Google Scholar]
- Javed, F.; Ahmed, H.B.; Crespi, R.; Romanos, G.E. Role of primary stability for successful osseointegration of dental implants: Factors of influence and evaluation. Interv. Med. Appl. Sci. 2013, 5, 162–167. [Google Scholar] [CrossRef]
- Herrero-Climent, M.; Lemos, B.F.; Herrero-Climent, F.; Falcao, C.; Oliveira, H.; Herrera, M.; Gil, F.J.; Ríos-Carrasco, B.; Ríos-Santos, J.V. Influence of implant design and under-preparation of the implant site on implant primary stability. An in vitro study. Int. J. Environ. Res. Public Health 2020, 17, 4436. [Google Scholar] [CrossRef]
- Gaviria, L.; Salcido, J.P.; Guda, T.; Ong, J.L. Current trends in dental implants. J. Korean Assoc. Oral Maxillofac. Surg. 2014, 40, 50–60. [Google Scholar] [CrossRef]
- Molly, L. Bone density and primary stability in implant therapy. Clin. Oral Implant. Res. 2006, 17, 124–135. [Google Scholar] [CrossRef]
- Farronato, D.; Manfredini, M.; Stocchero, M.; Caccia, M.; Azzi, L.; Farronato, M. Influence of bone quality, drilling protocol, implant diameter/length on primary stability: An in vitro comparative study on insertion torque and resonance frequency analysis. J. Oral Implant. 2020, 46, 182–189. [Google Scholar] [CrossRef]
- Fischer, K.R.; Büchel, J.; Gubler, A.; Liu, C.C.; Sahrmann, P.; Schmidlin, P.R. Nonsurgical cleaning potential of deep-threaded implants and titanium particle release: A novel in vitro tissue model. Clin. Oral Implant. Res. 2023, 34, 416–425. [Google Scholar] [CrossRef] [PubMed]
- Makary, C.; Rebaudi, A.; Sammartino, G.; Naaman, N. Implant primary stability determined by resonance frequency analysis: Correlation with insertion torque, histologic bone volume, and torsional stability at 6 weeks. Implant. Dent. 2012, 21, 474–480. [Google Scholar] [CrossRef] [PubMed]
- Tözüm, T.F.; Turkyilmaz, I.; McGlumphy, E.A. Relationship between dental implant stability determined by resonance frequency analysis measurements and peri-implant vertical defects: An in vitro study. J. Oral Rehabil. 2008, 35, 739–744. [Google Scholar] [CrossRef] [PubMed]
- Ohta, K.; Takechi, M.; Minami, M.; Shigeishi, H.; Hiraoka, M.; Nishimura, M.; Kamata, N. Influence of factors related to implant stability detected by wireless resonance frequency analysis device. J. Oral Rehabil. 2010, 37, 131–137. [Google Scholar] [CrossRef]
- Lages, F.S.; Douglas-de Oliveira, D.W.; Costa, F.O. Relationship between implant stability measurements obtained by insertion torque and resonance frequency analysis: A systematic review. Clin. Implant Dent. Relat. Res. 2018, 20, 26–33. [Google Scholar] [CrossRef]
- Ito, Y.; Sato, D.; Yoneda, S.; Ito, D.; Kondo, H.; Kasugai, S. Relevance of resonance frequency analysis to evaluate dental implant stability: Simulation and histomorphometrical animal experiments. Clin. Oral Implant. Res. 2008, 19, 9–14. [Google Scholar] [CrossRef]
- Turkyilmaz, I.; Tumer, C.; Ozbek, E.N.; Tözüm, T.F. Relations between the bone density values from computerized tomography, and implant stability parameters: A clinical study of 230 regular platform implants. J. Clin. Periodontol. 2007, 34, 716–722. [Google Scholar] [CrossRef]
- Bandela, V.; Munagapati, B.; Komala, J.; Basany, R.B.; Patil, S.R.; Kanaparthi, S. Comparison of primary stability of implants installed by two different methods in D3 and D4 bone types: An in vitro study. J. Int. Soc. Prev. Community Dent. 2020, 10, 620–626. [Google Scholar] [CrossRef]
- Degidi, M.; Daprile, G.; Piattelli, A. Influence of underpreparation on primary stability of implants inserted in poor quality bone sites: An in vitro study. J. Oral Maxillofac. Surg. 2015, 73, 1084–1088. [Google Scholar] [CrossRef]

| Group | Kruskal-Wallis Test | ||||
|---|---|---|---|---|---|
| A | B | C | D | ||
| IT | H = 17.901 (0.000) a,b,c,d,e | ||||
| ± s | 25.12 ± 2.96 | 40.80 ± 4.22 | 50.78 ± 6.51 | 54.03 ± 8.99 | |
| ISQ V | H = 7.970 (0.036) c | ||||
| ± s | 67.50 ± 2.51 | 69.33 ± 2.16 | 68.33 ± 3.14 | 71.00 ± 1.26 | |
| ISQ P | H = 7.045 (0.061) | ||||
| ± s | 67.67 ± 2.34 | 69.67 ± 2.66 | 68.50 ± 3.02 | 71.00 ± 1.10 | |
| ISQ M | H = 12.284 (0.002) a,b,c | ||||
| ± s | 63.50 ± 0.84 | 68.67 ± 1.21 | 68.50 ± 2.74 | 69.17 ± 1.33 | |
| ISQ D | H = 12.316 (0.002) a,b,c | ||||
| ± s | 63.67 ± 0.82 | 68.50 ± 1.52 | 68.33 ± 2.74 | 69.33 ± 1.21 | |
| Mean ISQ | H = 11.751 (0.002) a,c | ||||
| ± s | 65.58 ± 1.37 | 69.04 ± 1.51 | 68.42 ± 2.76 | 70.13 ± 1.12 | |
| ISQ V | ISQ P | ISQ M | ISQ D | Mean ISQ | |
|---|---|---|---|---|---|
| IT | 0.499 * | 0.479 * | 0.795 ** | 0.803 ** | 0.716 ** |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cucinelli, C.; Pereira, M.S.; Borges, T.; Figueiredo, R.; Leitão-Almeida, B. The Effect of Increasing Thread Depth on the Initial Stability of Dental Implants: An In Vitro Study. Surgeries 2024, 5, 817-825. https://doi.org/10.3390/surgeries5030065
Cucinelli C, Pereira MS, Borges T, Figueiredo R, Leitão-Almeida B. The Effect of Increasing Thread Depth on the Initial Stability of Dental Implants: An In Vitro Study. Surgeries. 2024; 5(3):817-825. https://doi.org/10.3390/surgeries5030065
Chicago/Turabian StyleCucinelli, Chiara, Miguel Silva Pereira, Tiago Borges, Rui Figueiredo, and Bruno Leitão-Almeida. 2024. "The Effect of Increasing Thread Depth on the Initial Stability of Dental Implants: An In Vitro Study" Surgeries 5, no. 3: 817-825. https://doi.org/10.3390/surgeries5030065
APA StyleCucinelli, C., Pereira, M. S., Borges, T., Figueiredo, R., & Leitão-Almeida, B. (2024). The Effect of Increasing Thread Depth on the Initial Stability of Dental Implants: An In Vitro Study. Surgeries, 5(3), 817-825. https://doi.org/10.3390/surgeries5030065






