Control of PMSM Based on Switched Systems and Field-Oriented Control Strategy
Abstract
1. Introduction
- PMSM model linearization at a static operating point;
- Basic elements and concept summary of switched-systems stability;
- Application of FOC control strategy and control switched systems for the control of a PMSM under significant variation of parameters that usually change value during operation (stator resistance Rs, stator inductances Ld and Lq, but also combined inertia of PMSM rotor and load J);
- Matlab/Simulink program implementation for calculation of the control system characteristic matrices under parametric variations, calculation of the positive definite matrices Pi from Lyapunov–Metzler inequalities to demonstrate system stability;
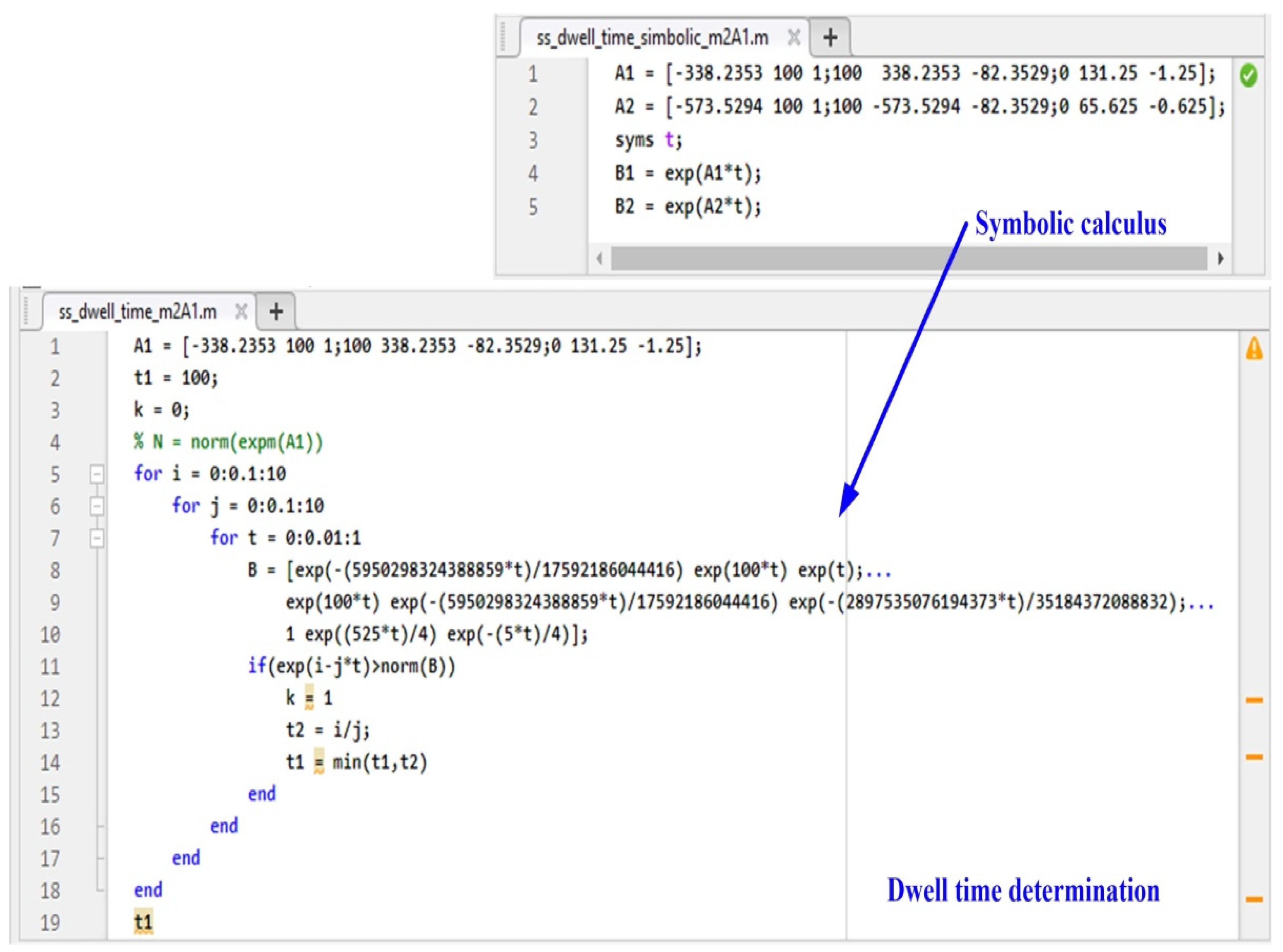
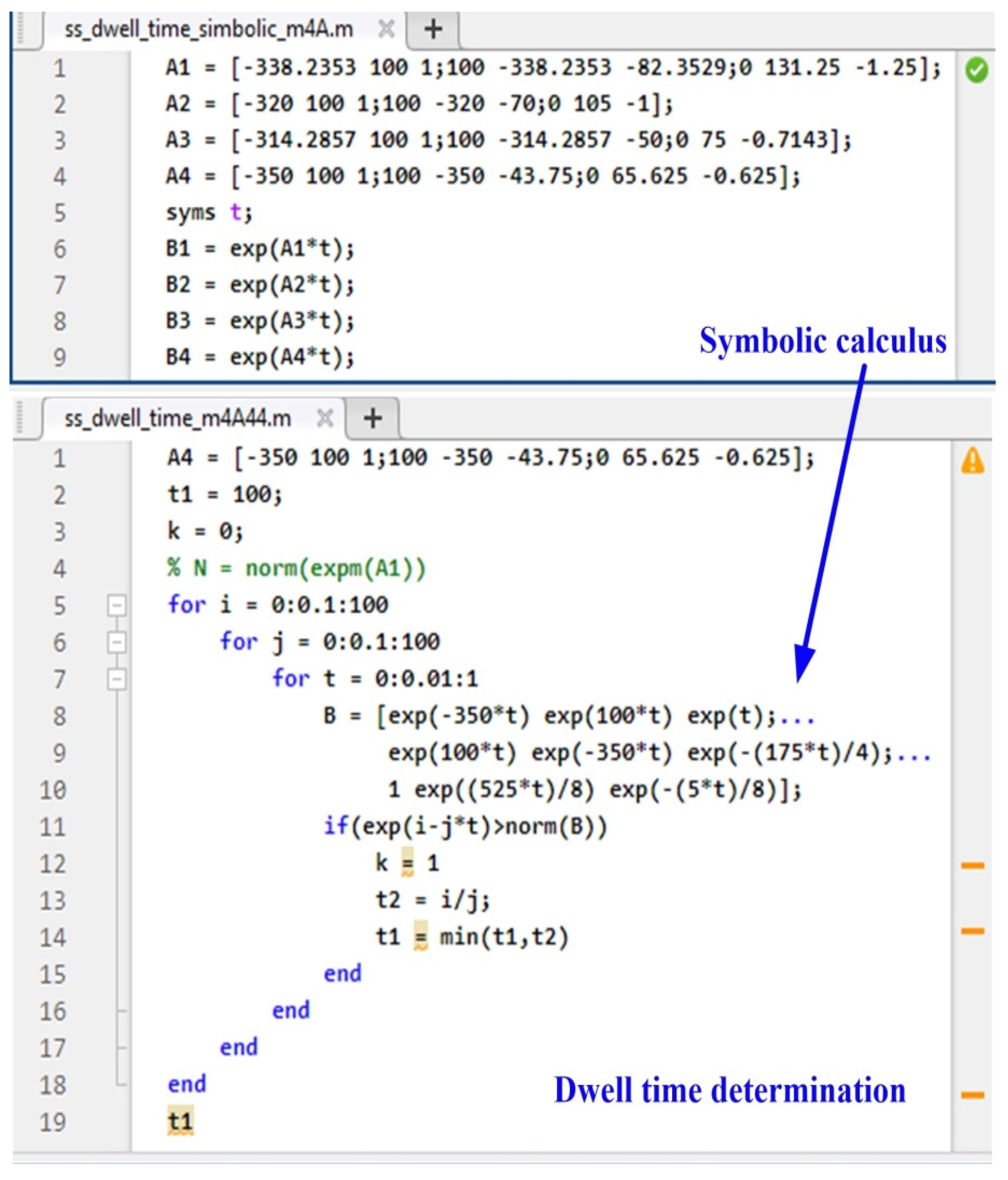
- Matlab program implementation for calculation of the dwell time;
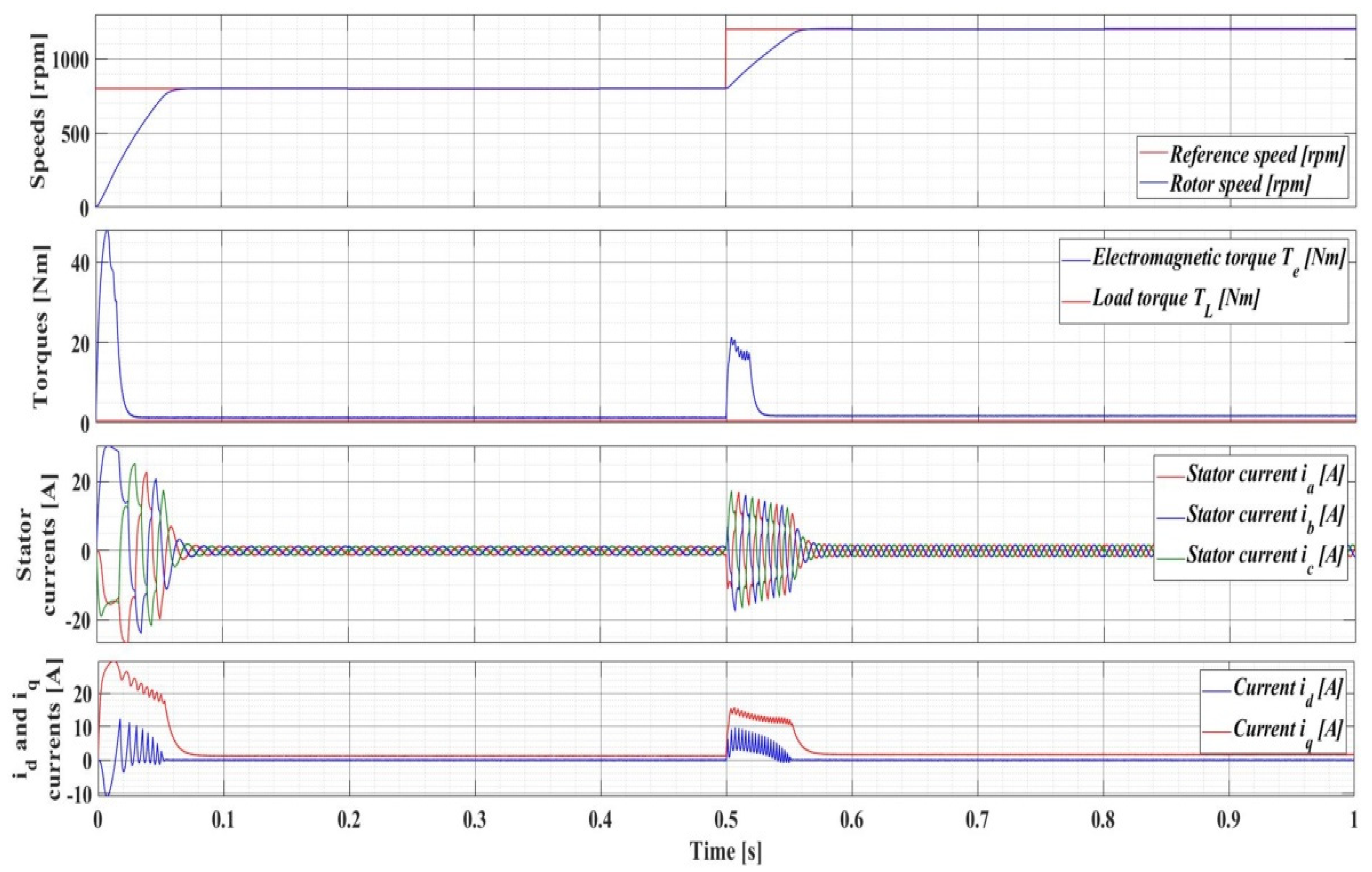
- Numerical simulations development for the PMSM control switched systems using a switching signal with frequency lower than the one corresponding to the dwell time;
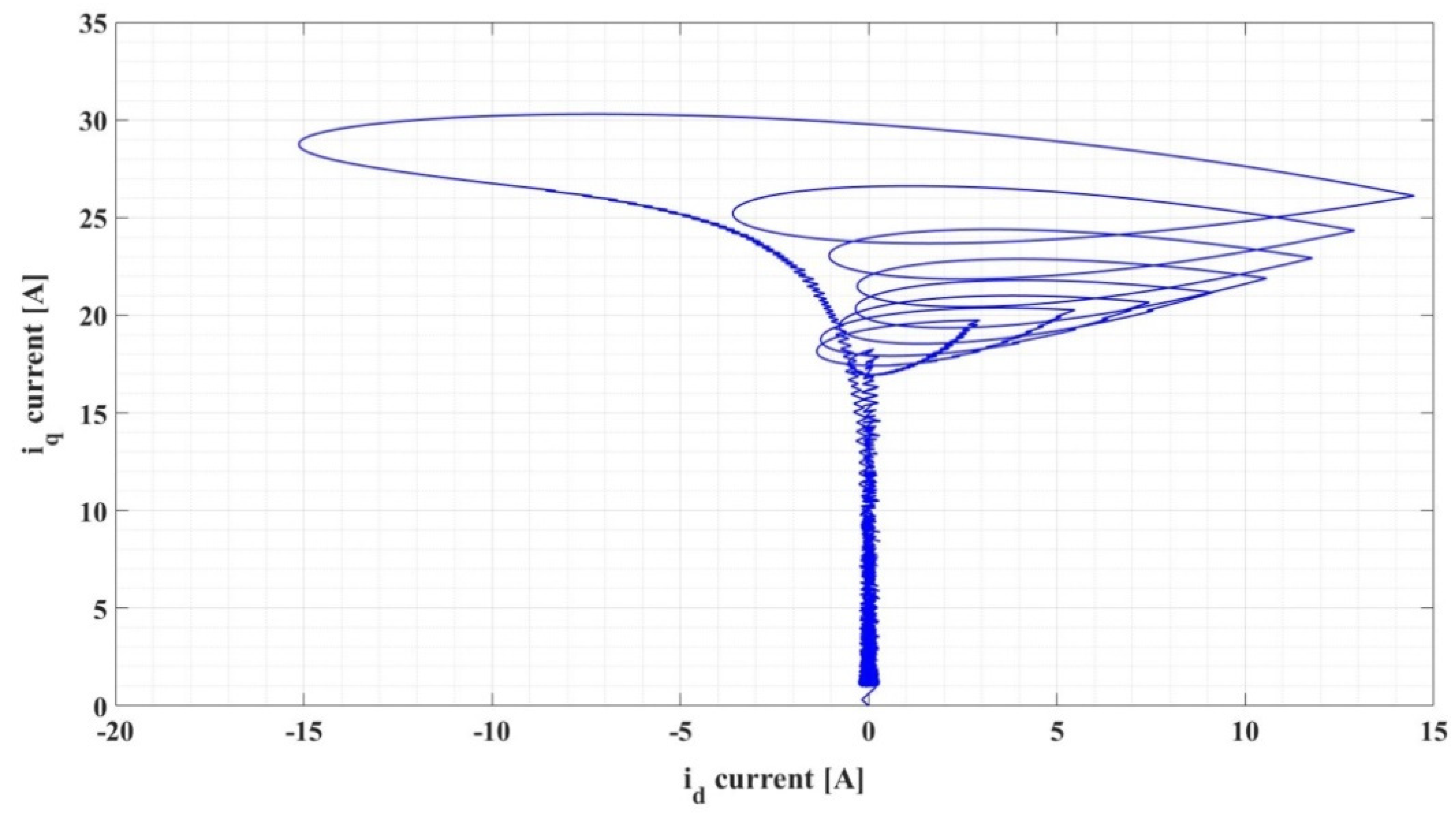
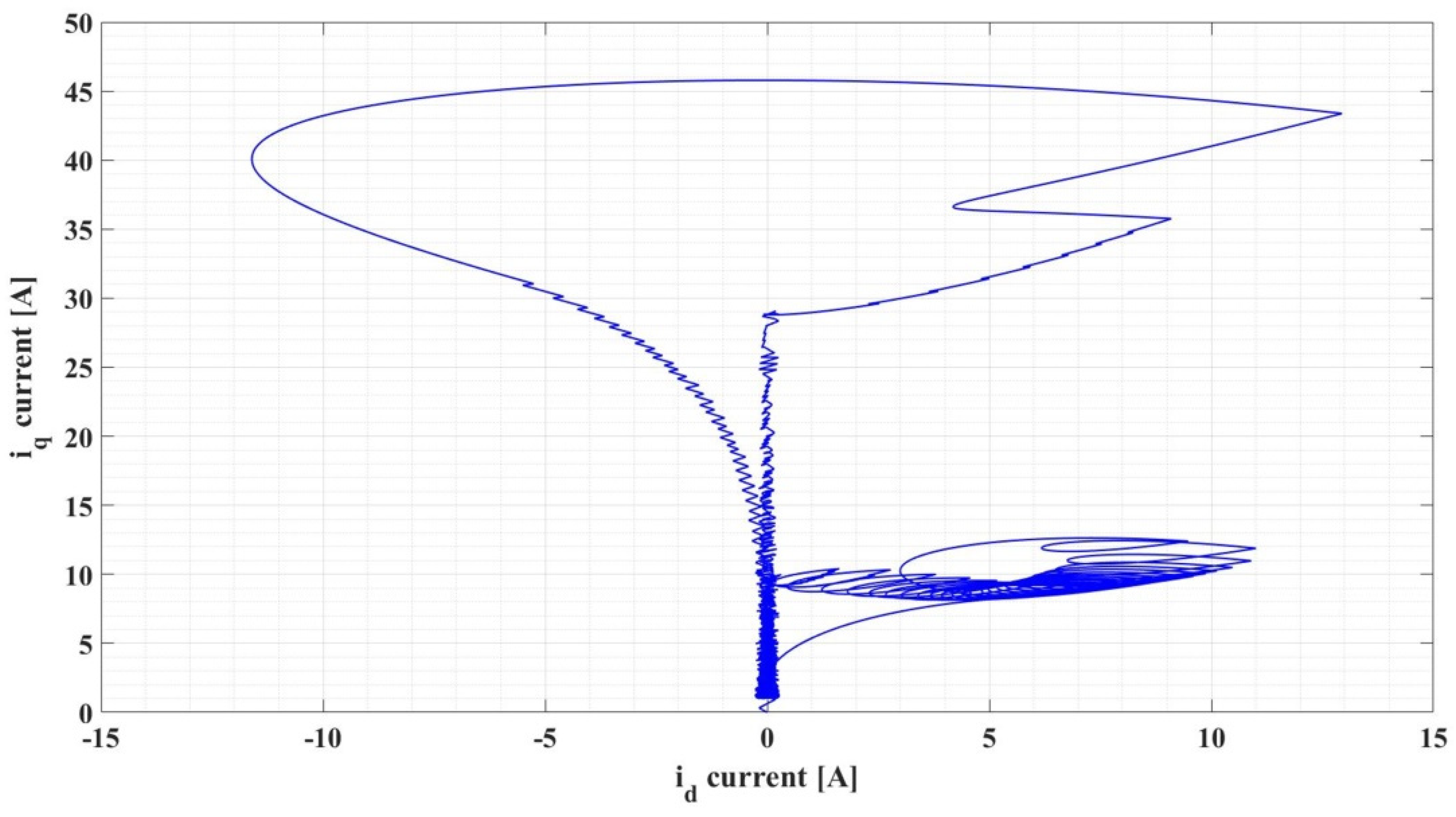
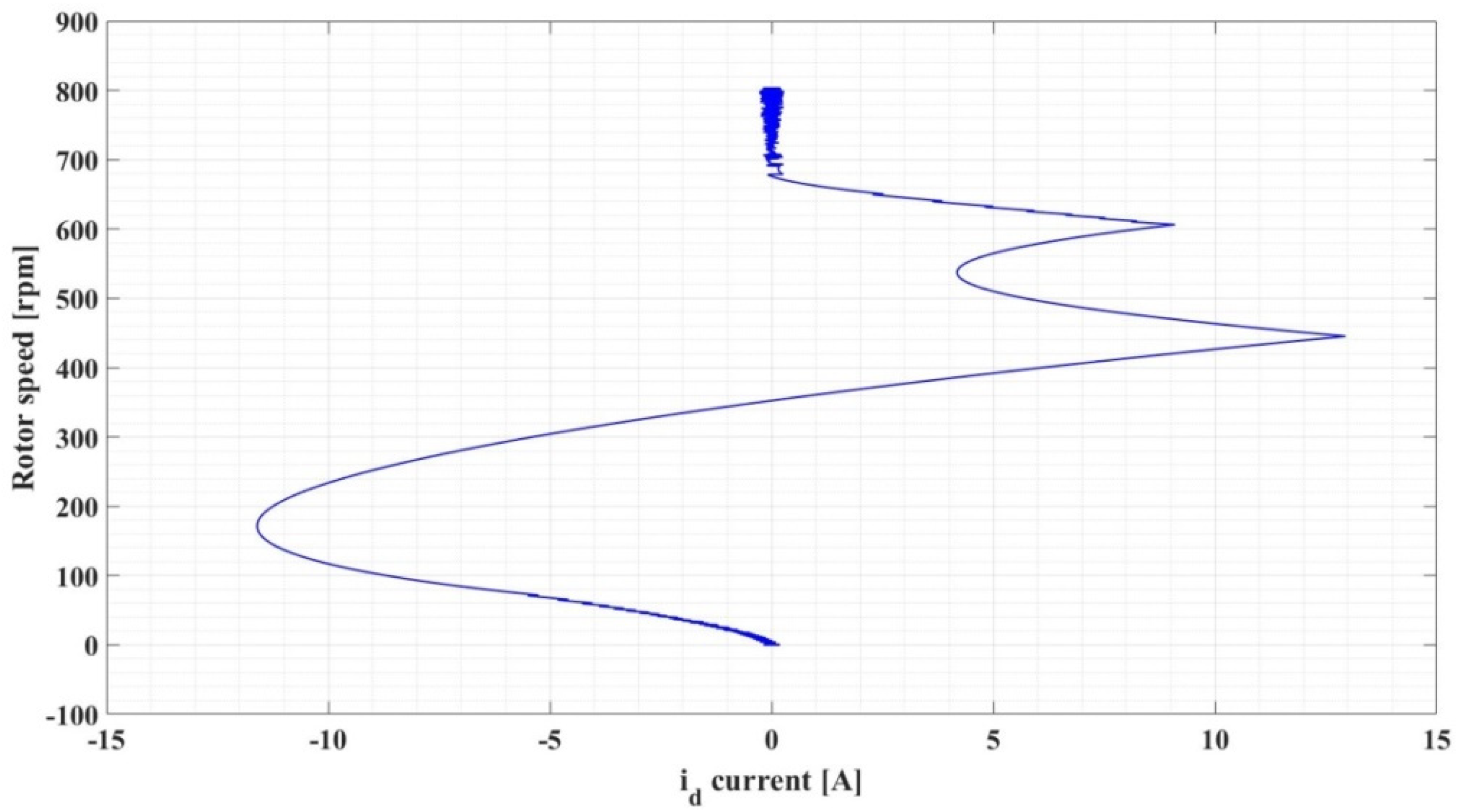
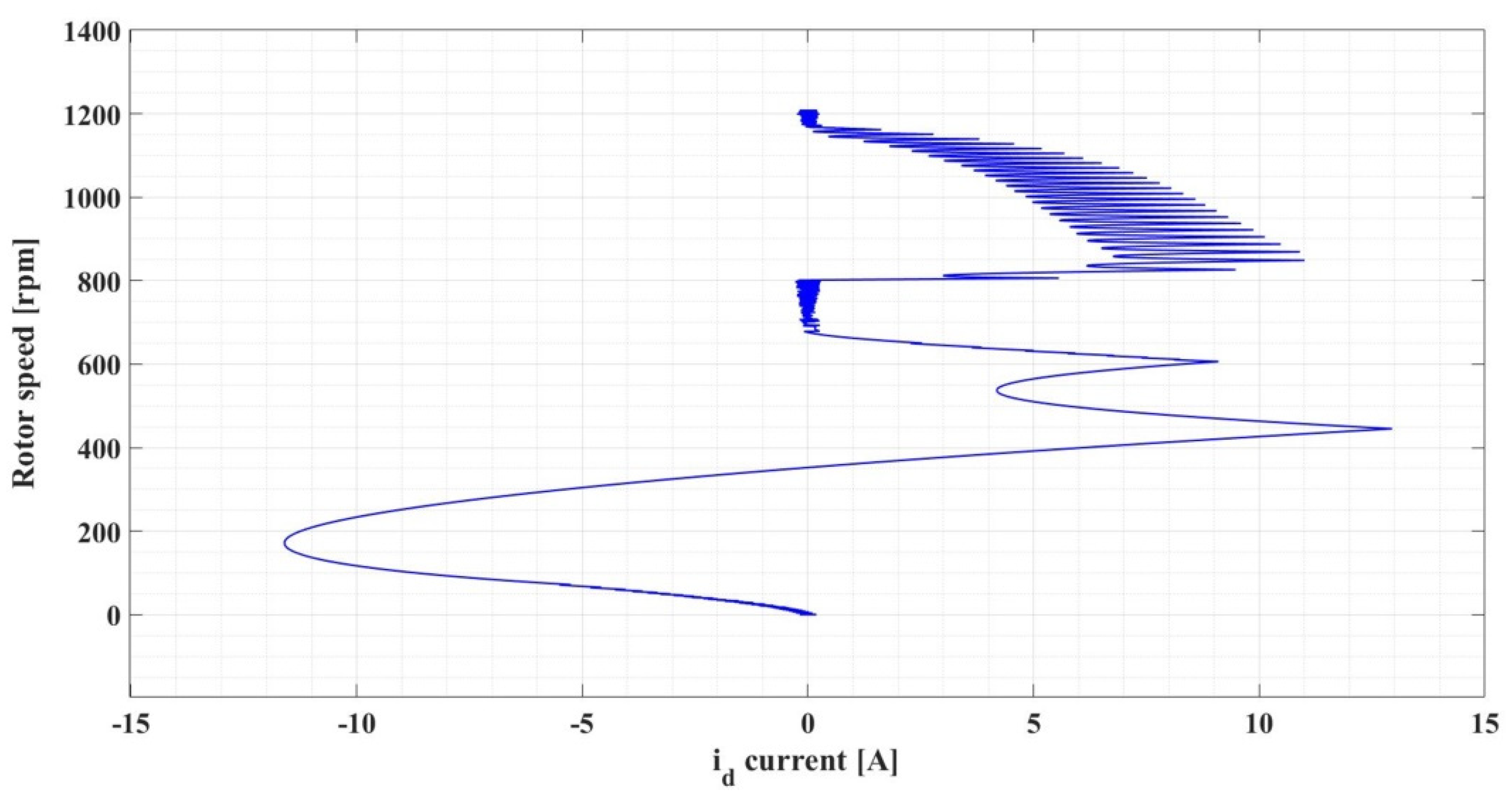
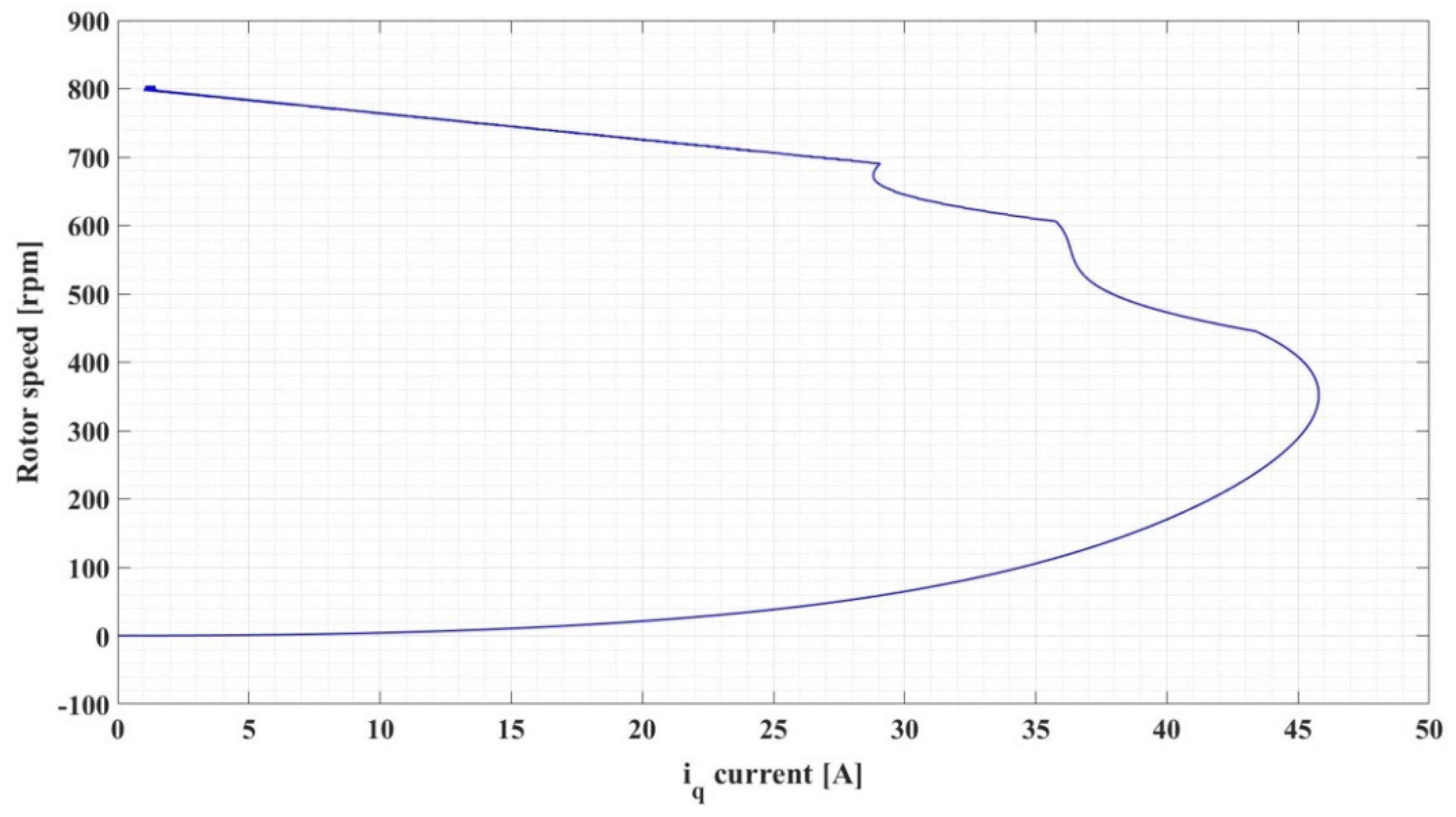
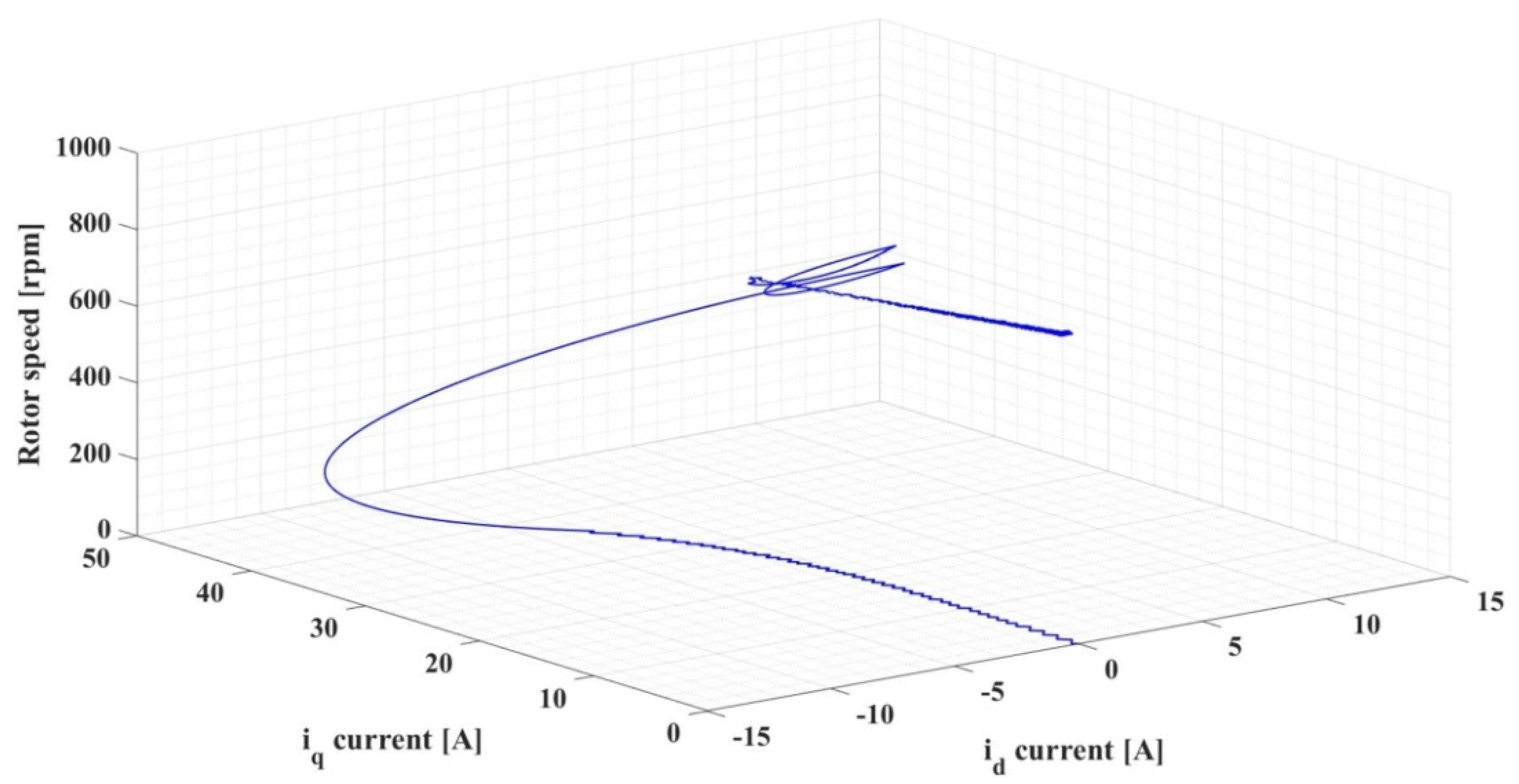
- Qualitative study of the PMSM control system performance by presenting in phase plane and state space the evolution of state vectors: ω PMSM rotor speed, iq current, and id current.
2. PMSM Mathematical Model and FOC-Type Strategy
3. Switched Systems—A General Description
3.1. Example 1
3.2. Example 2
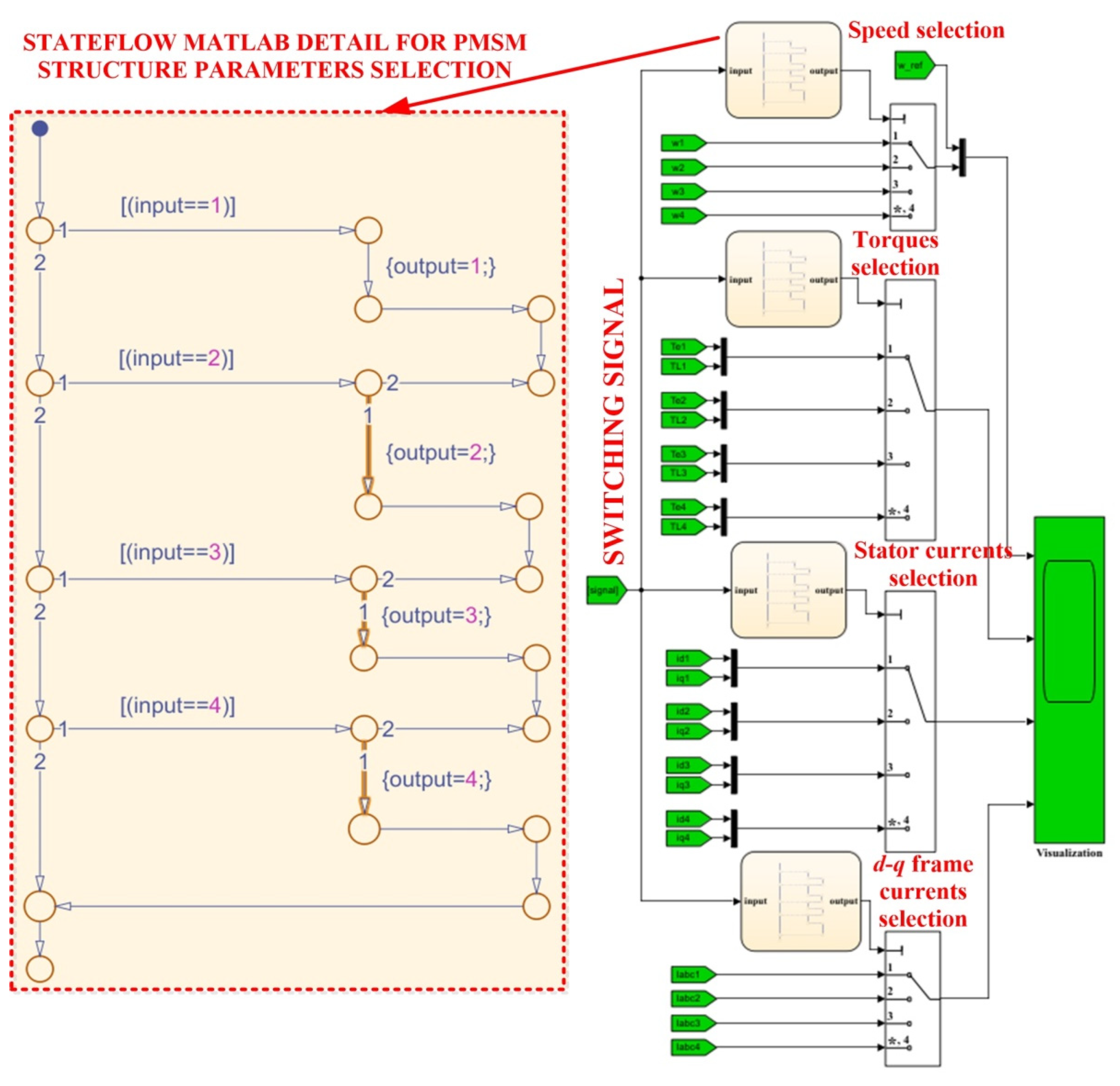
4. Numerical Simulations for PMSM Control Switched Systems
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| PMSM | Permanent Magnet Synchronous Motor |
| FOC | Field Oriented Control |
| DTC | Direct Torque Control |
| YALMIP | A toolbox for modeling and optimization in MATLAB |
| Rs | Stator resistance of the PMSM |
| Rd and Rq | Stator resistances on d-q axis |
| Ld and Lq | Stator inductances on d-q axis |
| ud and uq | Stator voltages on d-q axis |
| id and iq | Stator currents on d-q axis |
| TL | Load torque |
| J | Combined inertia of PMSM rotor and load |
| B | Combined viscous friction of PMSM rotor and load |
| λ0 | Flux induced by the permanent magnets of the rotor in the stator phases |
| np | Pole pairs number |
| ω | PMSM rotor speed |
References
- Wang, S.-C.; Nien, Y.-C.; Huang, S.-M. Multi-Objective Optimization Design and Analysis of V-Shape Permanent Magnet Synchronous Motor. Energies 2022, 15, 3496. [Google Scholar] [CrossRef]
- You, Y.-M. Optimal Design of PMSM Based on Automated Finite Element Analysis and Metamodeling. Energies 2019, 12, 4673. [Google Scholar] [CrossRef]
- Furmanik, M.; Gorel, L.; Konvičný, D.; Rafajdus, P. Comparative Study and Overview of Field-Oriented Control Techniques for Six-Phase PMSMs. Appl. Sci. 2021, 11, 7841. [Google Scholar] [CrossRef]
- Feng, S.; Jiang, W.; Zhang, Z.; Zhang, J.; Zhang, Z. Study of efficiency characteristics of Interior Permanent Magnet Synchronous Motors. IEEE Trans. Magn. 2018, 54, 8108005. [Google Scholar]
- Zakharov, V.; Minav, T. Analysis of Field Oriented Control of Permanent Magnet Synchronous Motor for a Valveless Pump-Controlled Actuator. Multidiscip. Digit. Publ. Inst. Proc. 2020, 64, 19. [Google Scholar]
- Jiang, W.; Han, W.; Wang, L.; Liu, Z.; Du, W. Linear Golden Section Speed Adaptive Control of Permanent Magnet Synchronous Motor Based on Model Design. Processes 2022, 10, 1010. [Google Scholar] [CrossRef]
- Wang, D.; Yuan, T.; Wang, X.; Wang, X.; Wang, S.; Ni, Y. Performance Improvement for PMSM Driven by DTC Based on Discrete Duty Ratio Determination Method. Appl. Sci. 2019, 9, 2924. [Google Scholar] [CrossRef]
- Amin, F.; Sulaiman, E.B.; Utomo, W.M.; Soomro, H.A.; Jenal, M.; Kumar, R. Modelling and Simulation of Field Oriented Control based Permanent Magnet Synchronous Motor Drive System. Indones. J. Electr. Eng. Comput. Sci. 2017, 6, 387. [Google Scholar] [CrossRef]
- Qiu, H.; Zhang, H.; Min, L.; Ma, T.; Zhang, Z. Adaptive Control Method of Sensorless Permanent Magnet Synchronous Motor Based on Super-Twisting Sliding Mode Algorithm. Electronics 2022, 11, 3046. [Google Scholar] [CrossRef]
- Chen, H.; Yao, Z.; Liu, Y.; Lin, J.; Wang, P.; Gao, J.; Zhu, S.; Zhou, R. PMSM Adaptive Sliding Mode Controller Based on Improved Linear Dead Time Compensation. Actuators 2022, 11, 267. [Google Scholar] [CrossRef]
- Tang, M.; Zhuang, S. On Speed Control of a Permanent Magnet Synchronous Motor with Current Predictive Compensation. Energies 2019, 12, 65. [Google Scholar] [CrossRef]
- Nicola, M.; Nicola, C.-I. Sensorless Predictive Control for PMSM Using MRAS Observer. In Proceedings of the International Conference on Electromechanical and Energy Systems (SIELMEN), Craiova, Romania, 9−11 October 2019; pp. 1–7. [Google Scholar]
- Mayilsamy, G.; Natesan, B.; Joo, Y.H.; Lee, S.R. Fast Terminal Synergetic Control of PMVG-Based Wind Energy Conversion System for Enhancing the Power Extraction Efficiency. Energies 2022, 15, 2774. [Google Scholar] [CrossRef]
- Nicola, M.; Nicola, C.-I. Sensorless Fractional Order Control of PMSM Based on Synergetic and Sliding Mode Controllers. Electronics 2020, 9, 1494. [Google Scholar] [CrossRef]
- Nicola, M.; Duta, M.; Nitu, M.; Aciu, A.; Nicola, C.-I. Improved System Based on ANFIS for Determining the Degree of Polymerization. Adv. Sci. Technol. Eng. Syst. J. 2022, 5, 664–675. [Google Scholar] [CrossRef]
- Hoai, H.-K.; Chen, S.-C.; Chang, C.-F. Realization of the Neural Fuzzy Controller for the Sensorless PMSM Drive Control System. Electronics 2020, 9, 1371. [Google Scholar] [CrossRef]
- Nicola, M.; Nicola, C.-I. Tuning of PI Speed Controller for PMSM Control System Using Computational Intelligence. In Proceedings of the 21st International Symposium on Power Electronics (Ee), Novi Sad, Serbia, 27−30 October 2021; pp. 1–6. [Google Scholar]
- Nicola, M.; Nicola, C.-I.; Selișteanu, D. Improvement of PMSM Sensorless Control Based on Synergetic and Sliding Mode Controllers Using a Reinforcement Learning Deep Deterministic Policy Gradient Agent. Energies 2022, 15, 2208. [Google Scholar] [CrossRef]
- Ullah, K.; Guzinski, J.; Mirza, A.F. Critical Review on Robust Speed Control Techniques for Permanent Magnet Synchronous Motor (PMSM) Speed Regulation. Energies 2022, 15, 1235. [Google Scholar] [CrossRef]
- Zhang, Q.; Yu, R.; Li, C.; Chen, Y.-H.; Gu, J. Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System. Actuators 2022, 11, 42. [Google Scholar] [CrossRef]
- Ma, Y.; Li, Y. Active Disturbance Compensation Based Robust Control for Speed Regulation System of Permanent Magnet Synchronous Motor. Appl. Sci. 2020, 10, 709. [Google Scholar] [CrossRef]
- Liu, C.; Liu, X. Stability of Switched Systems with Time-Varying Delays under State-Dependent Switching. Mathematics 2022, 10, 2722. [Google Scholar] [CrossRef]
- Halanay, A.; Samuel, J. Differential Equations, Discrete Systems and Control: Economic Models (Mathematical Modelling: Theory and Applications; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1997; pp. 15–123. [Google Scholar]
- Colaneri, P. Analysis and Control of Linear Switched Systems; The Polytechnic University of Milano: Milan, Italy, 2009; Available online: https://colaneri.faculty.polimi.it/Lucidi-Bertinoro-2009.pdf (accessed on 15 February 2022).
- Ren, W.; Xiong, J. Robust Filtering for 2-D Discrete-Time Switched Systems. IEEE Trans. Autom. Control 2021, 66, 4747–4760. [Google Scholar] [CrossRef]
- Ligang, W.; Rongni, Y.; Peng, S.; Xiaojie, S. Stability analysis and stabilization of 2-D switched systems under arbitrary and restricted switchings. Automatica 2015, 59, 206–215. [Google Scholar]
- Meng, F.; Shen, X.; Li, X. Stability Analysis and Synthesis for 2-D Switched Systems with Random Disturbance. Mathematics 2022, 10, 810. [Google Scholar] [CrossRef]
- Krok, M.; Hunek, W.P.; Feliks, T. Switching Perfect Control Algorithm. Symmetry 2020, 12, 816. [Google Scholar] [CrossRef]
- Nicola, C.-I.; Nicola, M. Real Time Implementation of the PMSM Sensorless Control Based on FOC Strategy. In Proceedings of the 4th Global Power, Energy and Communication Conference (GPECOM), Nevsehir, Turkey, 14−17 June 2022; pp. 179–183. [Google Scholar]
- Löfberg, J. Automatic robust convex programming. Optim. Methods Softw. 2022, 27, 115–129. [Google Scholar] [CrossRef]
- Besselmann, T.; Lofberg, J.; Morari, M. Explicit MPC for LPV Systems: Stability and Optimality. IEEE Trans. Autom. Control 2012, 57, 2322–2332. [Google Scholar] [CrossRef]
- Chandrasekaran, V.; Shah, P. Relative entropy optimization and its applications. Math. Program. Ser. A 2016, 161, 1–32. [Google Scholar] [CrossRef]
- Löfberg, J. YALMIP: A Toolbox for Modeling and Optimization in MATLAB. In Proceedings of the IEEE International Conference on Robotics and Automation (IEEE Cat. No.04CH37508), Taipei, Taiwan, 2−4 September 2004; pp. 284–289. [Google Scholar]
- Nicola, M.; Nicola, C.-I.; Ionete, C.; Şendrescu, D.; Roman, M. Improved Performance for PMSM Control Based on Robust Controller and Reinforcement Learning. In Proceedings of the 26th International Conference on System Theory, Control and Computing (ICSTCC), Sinaia, Romania, 19−21 October 2022; pp. 207–212. [Google Scholar]
- Vijayakumar, V.; Nisar, K.S.; Chalishajar, D.; Shukla, A.; Malik, M.; Alsaadi, A.; Aldosary, S.F. A Note on Approximate Controllability of Fractional Semilinear Integrodifferential Control Systems via Resolvent Operators. Fractal Fract. 2022, 6, 73. [Google Scholar] [CrossRef]
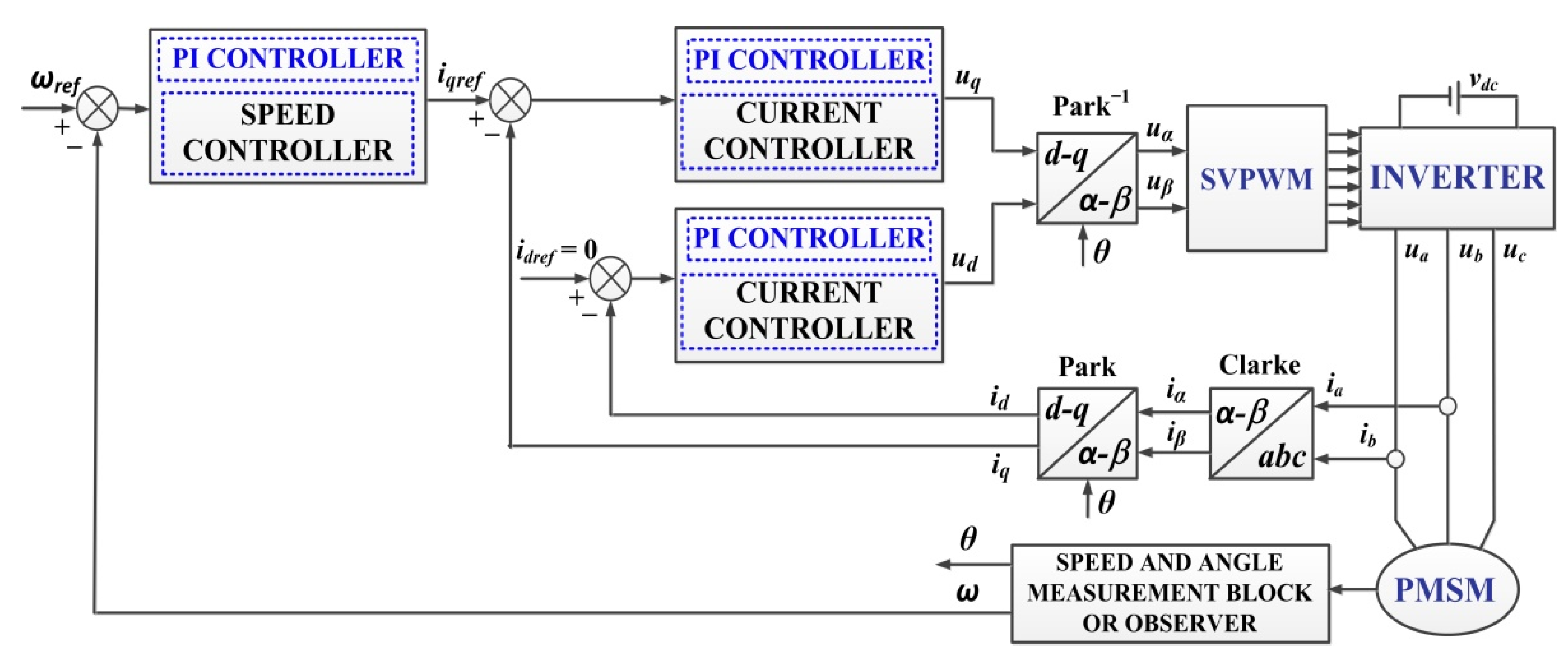
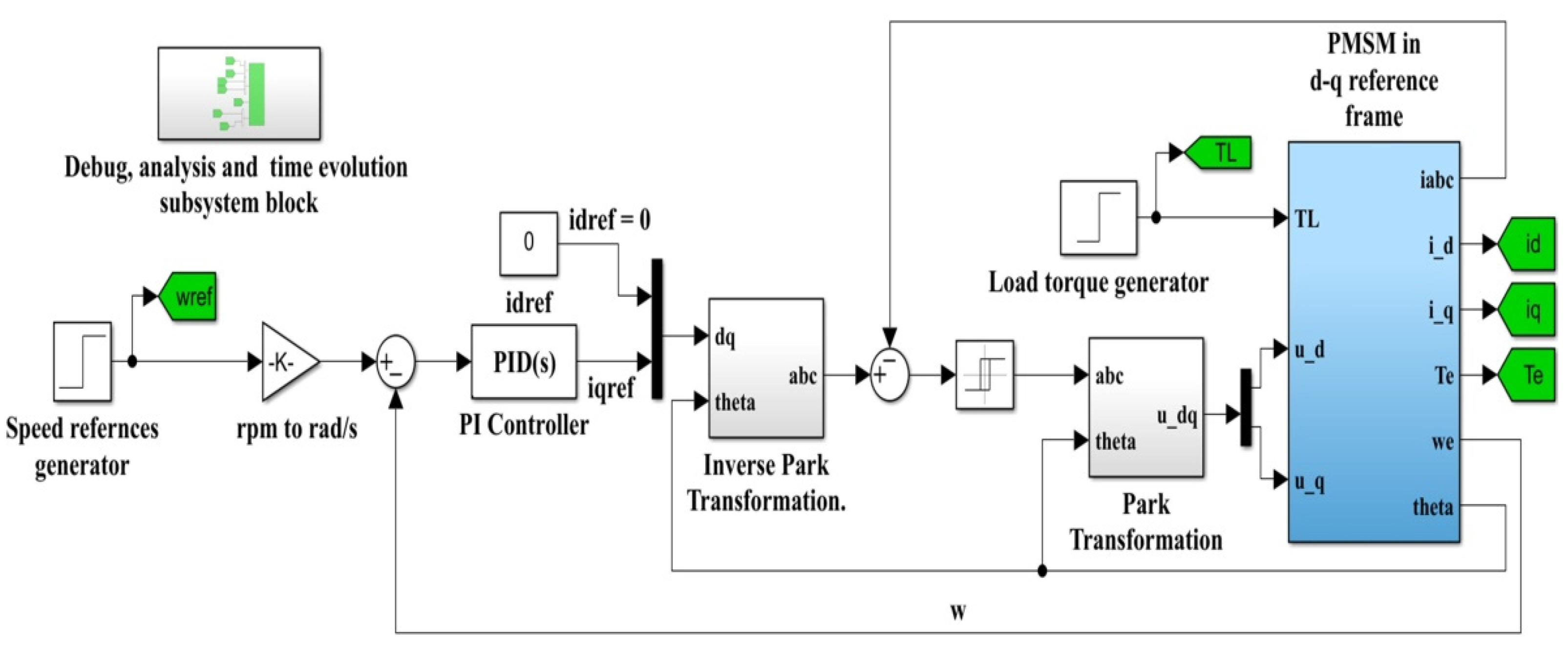






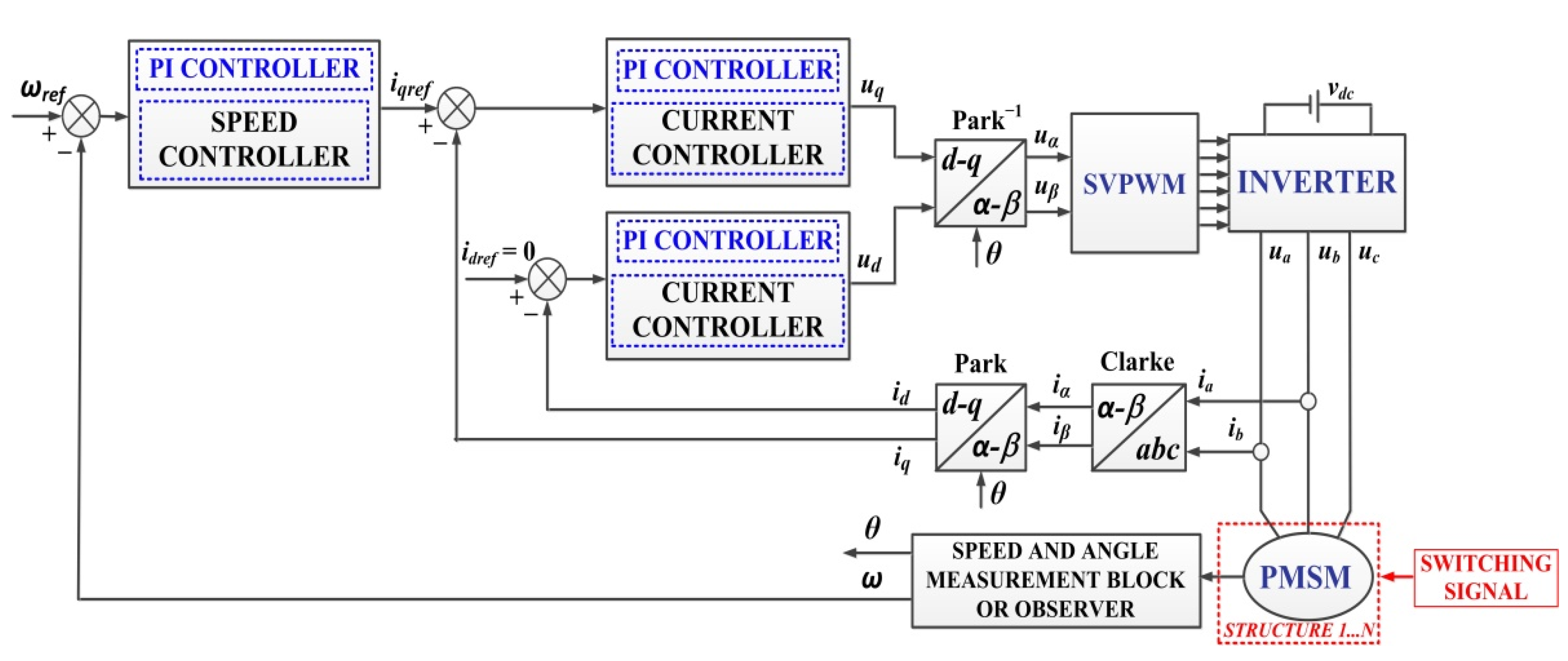
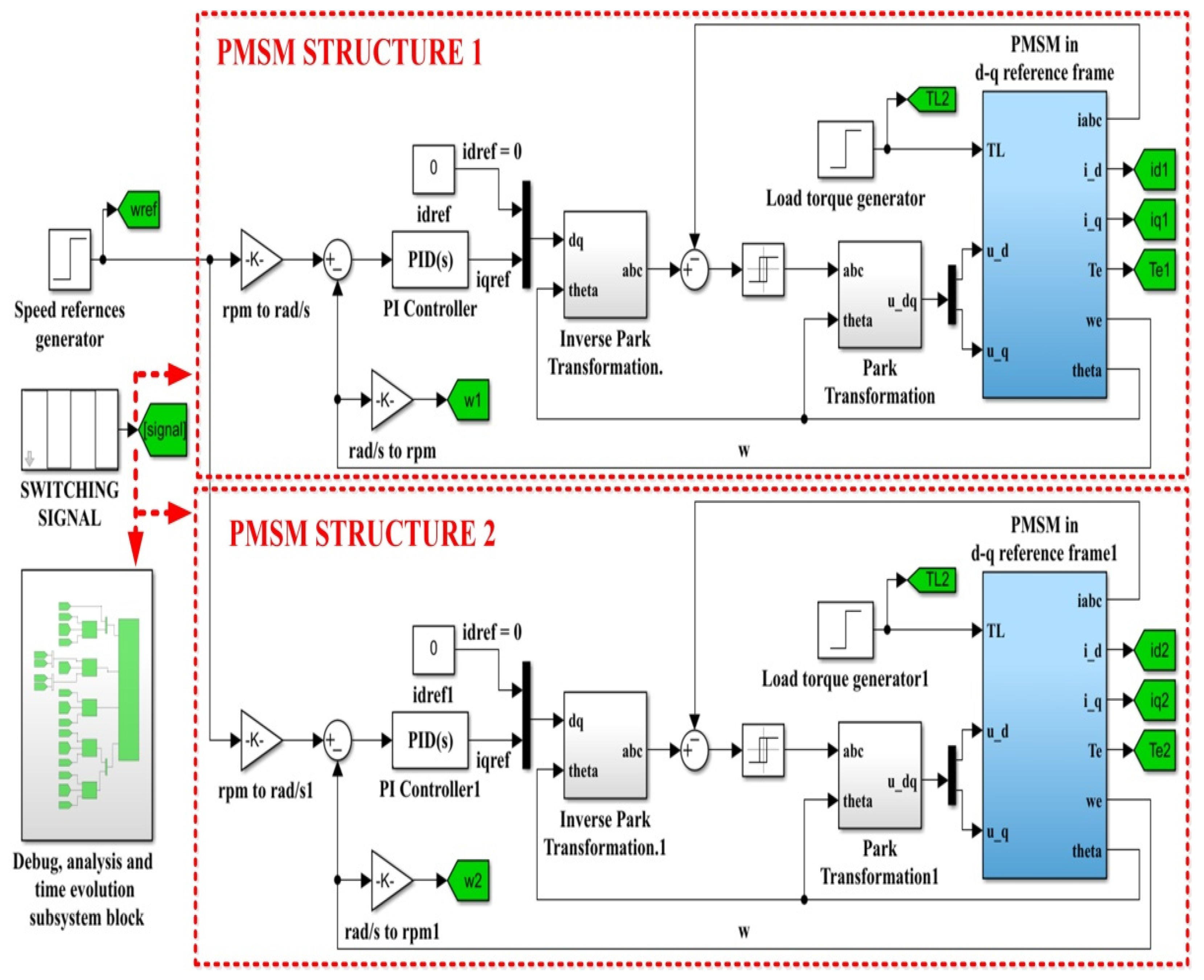













| Parameter | Value | Unit |
|---|---|---|
| Stator resistance—Rs | 2.875 | Ω |
| Inductances on d-q axis—Ld, Lq | 0.0085 | H |
| Combined inertia of PMSM rotor and load—J | 0.008 | kg·m2 |
| Combined viscous friction of PMSM rotor and load—B | 0.01 | N·m·s/rad |
| Flux induced by the permanent magnets of the PMSM rotor in the stator phases—λ0 | 0.175 | Wb |
| Pole pairs number—np | 4 | − |
| Parameter | Value 1 | Value 2 | Unit |
|---|---|---|---|
| Stator resistance—Rs | 2.875 | 4.875 | Ω |
| Combined inertia of PMSM rotor and load—J | 0.008 | 0.016 | kg·m2 |
| Parameter | Value 1 | Value 2 | Value 3 | Value 4 | Unit |
|---|---|---|---|---|---|
| Rs | 2.875 | 3.2 | 4.4 | 5.6 | Ω |
| Ld and Lq | 0.0085 | 0.01 | 0.014 | 0.016 | H |
| J | 0.008 | 0.01 | 0.014 | 0.016 | kg·m2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nicola, M.; Nicola, C.-I.; Selișteanu, D.; Ionete, C. Control of PMSM Based on Switched Systems and Field-Oriented Control Strategy. Automation 2022, 3, 646-673. https://doi.org/10.3390/automation3040033
Nicola M, Nicola C-I, Selișteanu D, Ionete C. Control of PMSM Based on Switched Systems and Field-Oriented Control Strategy. Automation. 2022; 3(4):646-673. https://doi.org/10.3390/automation3040033
Chicago/Turabian StyleNicola, Marcel, Claudiu-Ionel Nicola, Dan Selișteanu, and Cosmin Ionete. 2022. "Control of PMSM Based on Switched Systems and Field-Oriented Control Strategy" Automation 3, no. 4: 646-673. https://doi.org/10.3390/automation3040033
APA StyleNicola, M., Nicola, C.-I., Selișteanu, D., & Ionete, C. (2022). Control of PMSM Based on Switched Systems and Field-Oriented Control Strategy. Automation, 3(4), 646-673. https://doi.org/10.3390/automation3040033








