Electrodiffusion Phenomena in Neuroscience and the Nernst–Planck–Poisson Equations
Abstract
:1. Introduction
2. The Nernst–Planck–Poisson Model
3. The NPP Description of Neurons
- The voltage inside the spine saturates as the injected current increases.
- When the spine head is isolated and the current leak through the neck is slow, ions concentrate at the surface of the spine.
- A large electric field forms at the spine neck–head junction.
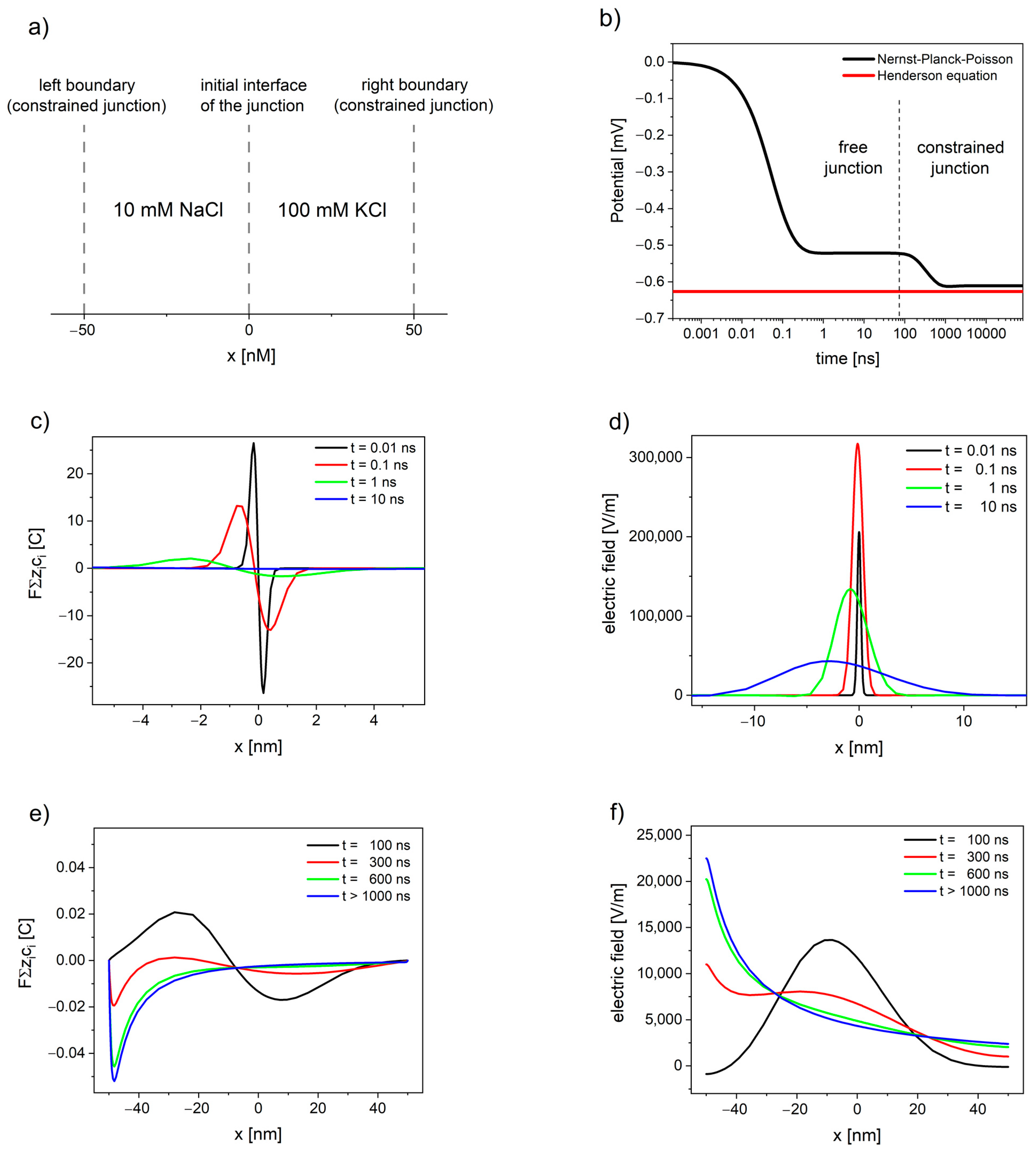
4. Non-Stationary Liquid Junction Potential
5. The NPP Description of Ion Channels
6. Potential Distortions Invisible to Patch-Clamp
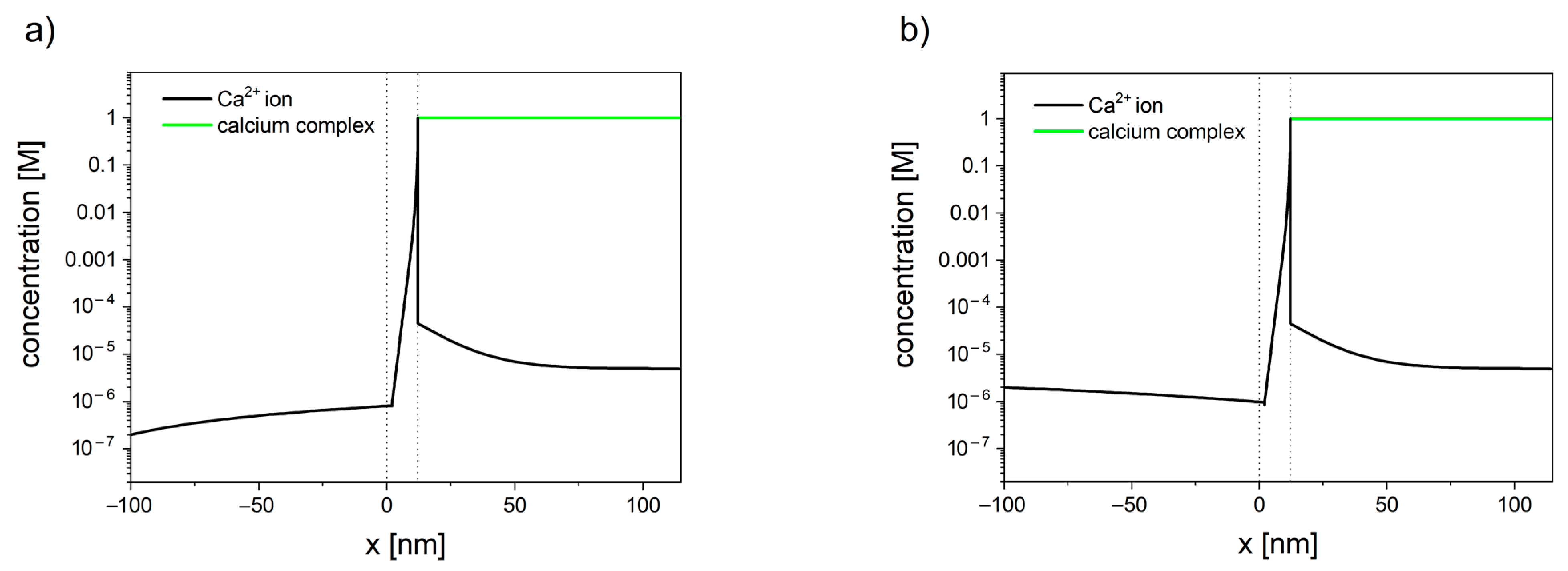
7. Mitochondrial Calcium Transport
8. Limitations and Extensions of NPP Model
9. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Savtchenko, L.P.; Poo, M.M.; Rusakov, D.A. Electrodiffusion phenomena in neuroscience: A neglected companion. Nature 2017, 18, 598–612. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sylantyev, S.; Savtchenko, L.P.; Niu, Y.-P.; Ivanov, A.I.; Jensen, T.P.; Kullmann, D.M.; Xiao, M.-Y.; Rusakov, D.A. Electric Fields Due to Synaptic Currents Sharpen Excitatory Transmission. Science 2008, 319, 1845–1849. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nicholson, C.; Chen, K.; Hrabětová, S.; Tao, L. Diffusion of molecules in brain extracellular space: Theory and experiment. Prog. Brain Res. 2000, 125, 129–154. [Google Scholar] [PubMed]
- Syková, E.; Nicholson, C. Diffusion in Brain extracellular space. Physiol. Rev. 2008, 88, 1277–1340. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Qian, N.; Sejnowski, T.J. An electro-diffusion model for computing membrane-potentials and ionic concentrations in branching dendrites, spines and axons. Biol. Cybern. 1989, 62, 1–15. [Google Scholar] [CrossRef]
- Leopre, C.L.; Bartol, T.M.; Coggan, J.S.; Keller, D.X.; Sosinsky, G.E.; Ellisman, M.H.; Sejnowski, T.J. Computational modelling of three dimensional electrodiffusion in biological systems: Application to the node of Ranvier. Biophys. J. 2008, 95, 2624–2635. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nanninga, P. A computational neuron model based on Poisson-Nernst-Planck theory. ANZIAM J. 2008, 50, 46–59. [Google Scholar] [CrossRef] [Green Version]
- Mori, Y.; Peskin, C.S.A. A numerical method for cellular electrophysiology based on the electrodiffusion equations with internal boundary conditions at membranes. Commun. Appl. Math. Comput. Sci. 2009, 4, 85–134. [Google Scholar] [CrossRef]
- Pods, J.; Schönke, J.; Bastian, P. Electrodiffusion model of neurons and extracellular space using the Poisson-Nernst-Planck equations—Numerical simnulation of intra- and extracellular potential for an axon model. Biophys. J. 2013, 105, 242–254. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cohen, H.; Cooley, J. The numerical solution of the time-dependent Nernst-Planck equations. Biophys. J. 1965, 5, 145–162. [Google Scholar] [CrossRef] [Green Version]
- Van Roosbroeck, W. Theory of flows of electrons and holes in germanium and other semiconductors. Bell Sys. Tech. J. 1950, 29, 560–607. [Google Scholar] [CrossRef]
- Selberherr, S. Analysis and Simulation of Semiconductor Devices; Springer: New York, NY, USA, 1984; pp. 1–293. [Google Scholar]
- Brumleve, T.R.; Buck, R.P. Numerical solution of the Nernst-Planck and Poisson equation system with applications to membrane electrochemistry and solid state physics. J. Electroanal. Chem. 1978, 90, 1–31. [Google Scholar] [CrossRef]
- Ivaska, A.; Bobacka, J.; Lewenstam, A. Potentiometric Ion Sensors. Chem. Rev. 2008, 108, 329–351. [Google Scholar]
- Eisenberg, B. Interacting Ions in Biophysics: Real is not Ideal. Biophys. J. 2013, 104, 1849–1866. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Planck, M. Über die Potenzialdifferenz zwischen zwei werdünnten Lösungen binärer Electrolyte. Ann. Phys. Chem. 1890, 40, 561–576. [Google Scholar] [CrossRef] [Green Version]
- Schlögl, R. Elektrodiffusion i freier Lösung und geladenen membranen. Z. Phys. Chem. 1954, 1, 305–339. [Google Scholar] [CrossRef]
- Helfferich, F.G. Ion. Exchange; McGraw-Hill: New York, NY, USA, 1962; pp. 1–624. [Google Scholar]
- Teorell, T. Transport process and electrical phenomena in ionic membranes. In Progress in Biophysics and Biophysical Chemistry; Butler, J.A.V., Randall, R.T., Eds.; Academic Press: New York, NY, USA, 2016; Volume 3, pp. 305–369. [Google Scholar]
- Conti, F.; Eisenman, G. The non-steady state membrane potential of ion exchangers with fixed sites. Biophys. J. 1965, 5, 247–256. [Google Scholar] [CrossRef] [Green Version]
- Mafé, S.; Pellicer, J.; Aguilella, V.M. The Goldman constant field assumption: significance and applicability conditions. Ber. Bunsenges. Phys. Chem. 1986, 90, 476–479. [Google Scholar] [CrossRef]
- MacGillivray, A.D. Nernst-Planck equations and the electroneutrality and Donnan equilibrium assumptions. J. Chem. Phys. 1968, 48, 2903–2907. [Google Scholar] [CrossRef]
- MacGillivray, A.D.; Hare, D. Applicability of Goldman’s constant field assumption to biological systems. J. Theor. Biol. 1969, 25, 113–126. [Google Scholar] [CrossRef]
- Kato, M. Numerical analysis of the Nernst-Planck-Poisson system. J. Theor. Biol. 1995, 177, 299–304. [Google Scholar] [CrossRef]
- Dickinson, E.J.F.; Limon-Petersen, J.G.; Compton, R.G. The electroneutrality approximation in electrochemistry. J. Solid State Electrochem. 2011, 15, 1335–1345. [Google Scholar] [CrossRef]
- Perram, J.W.; Stiles, P.J. On the nature of liquid junction and membrane potentials. Phys. Chem. Chem. Phys. 2006, 8, 4200–4213. [Google Scholar] [CrossRef] [PubMed]
- Mafé, S.; Pellicer, J.; Aguilella, V.M. Ionic transport and space charge density in electrolytic solutions as described by Nernst-Planck and Poisson equations. J. Phys. Chem. 1986, 90, 6045–6050. [Google Scholar] [CrossRef]
- Manzanares, J.A.; Mafé, S.; Pellicer, J. Transport phenomena and asymmetry effects in membranes with asymmetric fixed charge distributions. J. Phys. Chem. 1991, 95, 5620–5624. [Google Scholar] [CrossRef]
- Kontturi, A.K.; Kontturi, K.; Mafé, S.; Manzanares, J.A.; Niinikoski, P.; Vouristo, M. Convective diffusion in porous membranes with adsorbed charges. Langmuir 1994, 10, 949–954. [Google Scholar] [CrossRef]
- Kontturi, K.; Mafé, S.; Manzanares, J.A.; Pellicer, J.A. New Method for Determining Transport Numbers of Charged Membranes from Convective Diffusion Experiments. J. Electroanal. Chem. Interfacial Electrochem. 1994, 378, 111–116. [Google Scholar] [CrossRef]
- Sokalski, T.; Lewenstam, A. Application of Nernst-Planck and Poisson equations for interpretation of liquid junction and membrane potentials in real-time and space domains. Electrochem. Comm. 2001, 3, 107–112. [Google Scholar] [CrossRef]
- Sokalski, T.; Lingenfelter, P.; Lewenstam, A. Numerical solution of coupled Nernst-Planck and Poisson equations for liquid junction and ion selective membrane potentials. J. Phys. Chem. B 2003, 107, 2443–2452. [Google Scholar] [CrossRef]
- Lingenfelter, P.; Bedlechowicz-Śliwakowska, I.; Sokalski, T.; Maj-Żurawska, M.; Lewenstam, A. Time-dependent phenomena in the potential response of ion-selective electrodes treated by the Nernst-Planck-Poisson model. 1: Intramembrane processes and selectivity. Anal. Chem. 2006, 78, 6783–6791. [Google Scholar] [CrossRef]
- Lewenstam, A.; Sokalski, T.; Jasielec, J.; Kucza, W.; Filipek, R.; Wierzba, B.; Danielewski, M. Modelling Non Equilibrium Potentiometry to Understand and Control Selectivity and Detection Limit. ECS Trans. 2009, 19, 219–224. [Google Scholar] [CrossRef]
- Jasielec, J.J.; Sokalski, T.; Filipek, R.; Lewenstam, A. Comparison of different approaches to the description of the detection limit of ion-selective electrodes. Electrochim. Acta 2010, 55, 6836–6848. [Google Scholar] [CrossRef]
- Szyszkiewicz, K.; Danielewski, M.; Fausek, J.; Jasielec, J.J.; Kucza, W.; Lewenstam, A.; Sokalski, T.; Filipek, R. Breakthrough in modeling of electrodiffusion processes: Continuation and extensions of the classical work of Richard Buck. ECS Trans. 2014, 61, 21–30. [Google Scholar] [CrossRef]
- Jasielec, J.J.; Mousavi, Z.; Granholm, K.; Sokalski, T.; Lewenstam, A. Sensitivity and Selectivity of Ion-Selective Electrodes interpreted using the Nernst-Planck-Poisson model (NPP). Anal. Chem. 2018, 90, 9644–9649. [Google Scholar] [CrossRef] [PubMed]
- Rudolph, M.J. Digital simulations with the fast implicit finite-difference (FIFD) algorithm. Part 4. Simulation of electrical migration and diffuse double-layer effects. Electroanal. Chem. 1994, 375, 89–99. [Google Scholar] [CrossRef]
- Samson, E.; Marchand, J. Numerical solution of the extended Nernst-Planck model. J. Colloid Interface Sci. 1999, 215, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Samson, E.; Marchand, J.; Robert, J.-L.; Bournazel, J.-P. Modelling ion diffusion mechanisms in porous media. Int. J. Numer. Meth. Engng. 1999, 46, 2043–2060. [Google Scholar] [CrossRef]
- Zhou, Y.C.; Lu, B.Z.; Huber, G.A.; Holst, M.J.; McCammon, J.A. Continuum simulations of acetylcholine consumption by acetylcholinesterase—A Poisson-Nernst-Planck approach. J. Phys. Chem. B 2008, 112, 270–275. [Google Scholar] [CrossRef] [Green Version]
- Lu, B.; Holst, M.J.; McCammon, J.A.; Zhou, Y.C. Poisson-Nernst-Planck equations for simulating biomolecular diffusion-reaction processes I: Finite element solutions. J. Comput. Phys. 2010, 229, 6979–6994. [Google Scholar] [CrossRef] [Green Version]
- Moya, A.A.; Horno, J. Application of the network simulation method to ionic transport in ion-exchange membranes including diffuse double-layer effects. J. Phys. Chem. B 1999, 103, 10791. [Google Scholar] [CrossRef]
- Moleón, J.A.; Moya, A.A. Network simulation of the electrical response of ion-exchange membranes with fixed charge varying linearly with position. J. Electroanal. Chem. 2008, 613, 23–34. [Google Scholar] [CrossRef]
- Moleón, J.A.; Moya, A.A. Transient electrical response of ion-exchange membranes with fixed-charge due to ion adsorption. A network simulation approach. J. Electroanal. Chem. 2009, 633, 306–313. [Google Scholar] [CrossRef]
- MacGillivray, A.D. Asymptotic Solutions of the Time-Dependent Nernst-Planck Equations. J. Chem. Phys. 1970, 52, 3126–3132. [Google Scholar] [CrossRef]
- Jasielec, J.J.; Filipek, R.; Szyszkiewicz, K.; Fausek, J.; Danielewski, M.; Lewenstam, A. Computer simulations of electrodiffusion problems based on Nernst-Planck and Poisson equations. Comp. Mat. Sci. 2012, 63, 75–90. [Google Scholar] [CrossRef]
- Grysakowski, B.; Bożek, B.; Danielewski, M. Electro-Diffusion in Multicomponent Ion-Selective Membranes; Numerical Solution of the Coupled Nernst–Planck–Poisson Equations. Defect Diffus. Forum 2008, 273–276, 113–118. [Google Scholar]
- Grysakowski, B.; Jasielec, J.J.; Wierzba, B.; Sokalski, T.; Lewenstam, A.; Danielewski, M. Electrochemical Impedance Spectroscopy (EIS) of Ion Sensors. Direct modelling and inverse problem solving using the Nernst-Planck-Poisson (NPP) model and the HGS(FP) optimization strategy. J. Electroanal. Chem. 2011, 662, 143–149. [Google Scholar] [CrossRef]
- Jasielec, J.J.; Sokalski, T.; Filipek, R.; Lewenstam, A. Neutral-Carrier Ion-Selective Electrodes Assessed by Nernst-Planck-Poisson Model. Anal. Chem. 2015, 87, 8665–8672. [Google Scholar] [CrossRef] [PubMed]
- Jasielec, J.J.; Lisak, G.; Wagner, M.; Sokalski, T.; Lewenstam, A. Nernst-Planck-Poisson Model for the Qualitative Description of the Behaviour of Solid-Contact Ion-Selective-Electrodes at Low Analyte Concentration. Electroanalysis 2013, 25, 133–140. [Google Scholar] [CrossRef]
- Sanders, T.M.; Myers, M.; Asadnia, M.; Umana-Membreno, G.A.; Baker, M.; Fowkes, N.; Parish, G.; Nener, B. Description of ionophore-doped membranes with a blocked interface. Sens. Actuators B 2017, 250, 499–508. [Google Scholar] [CrossRef] [Green Version]
- Paczosa-Bator, B.; Stępień, M.; Maj-Żurawska, M.; Lewenstam, A. Biomimetic study of the Ca2+-Mg2+ and K+-Li+ antagonism on biologically active sites: New methodology to study potential dependent ion exchange. Magnes. Res. 2009, 22, 10–20. [Google Scholar] [CrossRef]
- Nowak, L.; Bregestovski, P.; Ascher, P.; Herbet, A.; Prochiantz, A. Magnesium gates glutamate-activated channels in mouse central neurons. Nature 1984, 307, 462–465. [Google Scholar] [CrossRef]
- Vargas-Caballero, M.; Robinson, H.P.C. Fast and slow voltage-dependent dynamics of magnesium block inthe NMDA receptor: The asymmetric trapping block model. J. Neurosci. 2004, 24, 6171–6180. [Google Scholar] [CrossRef]
- Chen, D.P.; Eisenberg, R.S. Poisson-Nernst-Planck (PNP) theory of open ionic channels. Biophys. J. 1993, 64, A22. [Google Scholar]
- Harden, J.L.; Viovy, J.L. Numerical studies of pulsed iontophoresis through model membranes. J. Control. Released 1996, 38, 129–139. [Google Scholar] [CrossRef]
- Kurnikova, M.G.; Coalson, R.D.; Graf, P.; Nitzan, A. A lattice relaxation algorithm for 3D Poisson-Nernst-Planck with application to ion transport through the gramicidin A channel. Biophys. J. 1999, 76, 642–656. [Google Scholar] [CrossRef] [Green Version]
- McKelvey, J.P. Solid State and Semiconductor Physics; Krieger: Malabar, FL, USA, 1982; pp. 1–504. [Google Scholar]
- Marchand, J.; Gérard, B.; Delagrave, A. Ion transport mechanism in cement-based materials. In Materials Science of Concrete; Skalny, J.P., Ed.; American Ceramic Society: Westerville, OH, USA, 1998; Volume V, pp. 307–400. [Google Scholar]
- Krabbenhøft, K.; Krabbenhøft, J. Application of the Poisson–Nernst–Planck equations to the migration test. Cem. Concr. Res. 2008, 38, 77–88. [Google Scholar] [CrossRef]
- Szyszkiewicz, K.; Jasielec, J.J.; Danielewski, M.; Lewenstam, A.; Filipek, R. Modeling of electrodiffusion processes from nano to macro scale. J. Electrochem. Soc. 2017, 164, E3559–E3568. [Google Scholar] [CrossRef]
- Lakshminarayanaiah, N. Equations of Membrane Biophysics; Academic Press: New York, NY, USA, 1984. [Google Scholar]
- Probstein, R.F. Physicochemical Hydrodynamics; Butterworth: Stoneham, NA, USA, 1989. [Google Scholar]
- Critchlow, D.L. MOSFET scaling—The driver of VLSI technology. Proc. IEEE 1999, 87, 659–667. [Google Scholar] [CrossRef]
- Dennard, R.H.; Gaensslen, F.H.; LeBlanc, A.R. Design of non-implanted MOSFET’s with very small physical dimensions. Proc. IEEE 1999, 87, 668–678. [Google Scholar] [CrossRef] [Green Version]
- Samson, E.; Marchand, J. Modeling the transport of ions in unsaturated cement-based materials. Comput. Struct. 2007, 85, 1740–1756. [Google Scholar] [CrossRef]
- Samson, E.; Marchand, J. Multiionic approaches to model chloride binding in cementious materials. In Proceedings of the 2nd International Symposium on Advances in Concrete through Science and Engineering, Quebec City, QC, Canada, 11–13 September 2006. [Google Scholar]
- Hof, P.R.; de Vellis, J.; Nimchinsky, E.A.; Kidd, G.; Claudio, L.; Trapp, B.D. Cellular Components of Nervous Tissue. In Fundamental Neuroscience, 3rd ed.; Squire, L., Berg, D., Bloom, F., du Lac, S., Gosh, A., Spitzer, N., Eds.; Elsevier Science Publishing Co. Inc.: San Diego, CA, USA, 2012; pp. 41–58. [Google Scholar]
- Savtchenko, L.P.; Kulahin, N.; Korogod, S.M.; Rusakov, D.A. Electric fields of synaptic currents could influence diffusion of charged neurotransmitter molecules. Synapse 2004, 51, 270–278. [Google Scholar] [CrossRef]
- Ercius, P.; Alaidi, O.; Rames, M.J.; Ren, G. Electron Tomography: A Three-Dimensional Analytic Tool for Hard and Soft Materials Research. Adv. Mater. 2015, 27, 5638–5663. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cartailler, J.; Kwon, T.; Yuste, R.; Holcman, D. Deconvolution of voltage sensor time series and electro-diffusion modeling reveal the role of spine geometry in controlling synaptic strength. Neuron 2018, 97, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Araya, R.; Jiang, J.; Eisenthal, K.B.; Yuste, R. The spine neck filters membrane potentials. Proc. Natl. Acad. Sci. USA 2006, 103, 17961–17966. [Google Scholar] [CrossRef] [Green Version]
- Araya, R.; Vogels, T.P.; Yuste, R. Activity-dependent dendritic spine neck changes are correlated with synaptic strength. Proc. Natl. Acad. Sci. USA 2014, 111, E2895–E2904. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goldman, D.E. Potential, impedance, and rectification in membranes. J. Gen. Physiol. 1943, 27, 37–60. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hodgkin, A.L.; Huxley, A.F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 1952, 117, 500–544. [Google Scholar] [CrossRef] [PubMed]
- Holcman, D.; Yuste, R. The new nanophysiology: Regulation of ionic flow in neuronal subcompartments. Nat. Rev. Neurosci. 2015, 16, 685–692. [Google Scholar] [CrossRef]
- Gardner, C.L.; Jones, J.R.; Baer, S.M.; Chang, S. Simulation of the ephaptic effect in the cone-horizontal cell synapse of the retina. SIAM J. Appl. Math. 2013, 73, 636–648. [Google Scholar] [CrossRef]
- Gardner, C.L.; Jones, J.R.; Baer, S.M.; Crook, S.M. Drift-diffusion simulation of the ephaptic effect in the triad synapse of the retina. J. Comput. Neurosci. 2015, 38, 129–142. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Halnes, G.; Mäki-Marttunen, T.; Keller, D.; Pettersen, K.H.; Andreassen, O.A.; Einevoll, G.T. Effect of Ionic Diffusion on Extracellular Potentials in Neural Tissue. PLoS Comput. Biol. 2016, 12, e1005193. [Google Scholar] [CrossRef] [Green Version]
- Neher, E. Correction for liquid junction potentials in patch clamp experiments. Method Enzymol. 1992, 207, 123–131. [Google Scholar]
- Lewenstam, A. Design and pitfalls of ion selective electrodes. Scand. J. Clin. Lab. Investig. Suppl. 1994, 54, 11–19. [Google Scholar] [CrossRef]
- Lewenstam, A. Ion-selective electrodes in clinical chemistry: State of the art. Anal. Proc. 1991, 28, 106–109. [Google Scholar]
- Lewenstam, A.; Maj-Żurawska, M.; Hulanicki, A. Application of ion-selective electrodes in clinical analysis. Electroanalysis 1991, 3, 727–734. [Google Scholar] [CrossRef]
- Henderson, P. Zur thermodynamik der flüssigkeitsketten. Z. Phys. Chem. 1907, 59, 118–127. [Google Scholar] [CrossRef]
- Henderson, P. Zur thermodynamik der flüssigkeitsketten. Z. Phys. Chem. 1908, 63, 325–345. [Google Scholar] [CrossRef]
- Jerzy, J. Jasielec Website. Available online: http://home.agh.edu.pl/~jasielec/software/ljp (accessed on 9 December 2020).
- Hickman, H.J. The liquid junction potential—The free diffusion junction. Chem. Eng. Sci. 1970, 25, 381–398. [Google Scholar] [CrossRef]
- Lingane, J.J. Electroanalytical Chemistry, 2nd ed.; Wiley: New York, NY, USA, 1998. [Google Scholar]
- MacInnes, D.A. The Principles of Electrochemistry, 2nd ed.; Dover Publications: New York, NY, USA, 1961. [Google Scholar]
- Nernst, W.H. Die elektromotorische wirksamkeit der jonen. Z. Phys. Chem. 1889, 4, 129–181. [Google Scholar] [CrossRef]
- Guggenheim, E.A. A study of cells with liquid-liquid junctions. J. Am. Chem. Soc. 1930, 52, 1315–1337. [Google Scholar] [CrossRef]
- Hafemann, D.R. Charge Separation in Liquid Junctions. J. Phys. Chem. 1965, 69, 4226–4231. [Google Scholar] [CrossRef]
- Dickinson, E.J.F.; Freitag, L.; Compton, R.G. Dynamic theory of liquid junction potentials. J. Phys. Chem. B 2010, 114, 187–197. [Google Scholar] [CrossRef]
- Ward, K.R.; Dickinson, E.J.F.; Compton, R.G. Dynamic theory of type 3 liquid junctions potentials: Formation of multilayer liquid junctions. J. Phys. Chem. B 2010, 114, 4521–4528. [Google Scholar] [CrossRef]
- Nelson, D.L.; Cox, M.M. Lehninger Principles of Biochemistry, 6th ed.; Freeman/Worth: New York, NY, USA, 2012; pp. 690–748. [Google Scholar]
- Chung, S.-H.; Tieleman, D.P. Computational and theoretical approaches to Unraveling the Permeation Dynamics in Biological Nanotubes. In Handbook of Theoretical and Computational Nanotechnology; Rieth, M., Schommers, W., Eds.; American Scientific Publishers: Stevenson Ranch, CA, USA, 2006; Volume 10, pp. 1–54. [Google Scholar]
- Århem, P.; Klement, G.; Blomberg, C. Channel Density Regulation of Firing Patterns in a Cortical Neuron Model. Biophys. J. 2006, 90, 4392–4404. [Google Scholar] [CrossRef] [Green Version]
- Zeberg, H.; Blomberg, C.; Århem, P. Ion Channel Density Regulates Switches between Regular and Fast Spiking in Soma but Not in Axons. PLoS Comput. Biol. 2010, 6, e1000753. [Google Scholar] [CrossRef]
- Migliore, R.; Lupascu, C.A.; Bologna, L.L.; Romani, A.; Courcol, J.-D.; Antonel, S.; Van Geit, W.A.H.; Thomson, A.M.; Mercer, A.; Lange, S.; et al. The physiological variability of channel density in hippocampal CA1 pyramidal cells and interneurons explored using a unified data-driven modeling workflow. PLoS Comput. Biol. 2018, 14, e1006423. [Google Scholar] [CrossRef]
- Motipally, S.I.; Allen, K.M.; Williamson, D.K.; Marsat, G. Differences in Sodium Channel Densities in the Apical Dendrites of Pyramidal Cells of the Electrosensory Lateral Line Lobe. Front. Neural Circuits 2019, 13, 41. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eisenberg, R.S. Channels are enzymes. J. Memb. Biol. 1990, 115, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Schuss, Z.; Nadler, B.; Eisenberg, R.S. Derivation of Poisson and Nernst-Planck equations in a bath and channel from a molecular model. Phys. Rev. E 2001, 64, 036116. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, D.; Lear, J.; Eisenberg, B. Permeation through an open channel: Poisson-Nernst-Planck theory of a synthetic ionic channel. Biophys. J. 1997, 72, 97–116. [Google Scholar] [CrossRef] [Green Version]
- Woolley, G.A.; Biggin, P.C.; Schultz, A.; Lien, L.; Jaikaran, D.; Breed, J.; Crowhurst, K.; Sansom, M.S. Intrinsic rectifiction of ion flux in alamethicin channels: Studies with an alamethicin dimer. Biophys. J. 1997, 73, 770–778. [Google Scholar] [CrossRef] [Green Version]
- Nonner, W.; Chen, D.; Eisenberg, B. Anomalous mole fraction effect, electrostatics and binding in ionic channels. Biophys. J. 1998, 74, 2327–2334. [Google Scholar] [CrossRef] [Green Version]
- Aquilella-Arzo, M.; Aguilella, V.M.; Eisenberg, R.S. Computing numerically the access resistance of a pore. Eur. Biophys. J. 2005, 34, 314–322. [Google Scholar] [CrossRef]
- Hall, J.E. Access resistance of a small circular pore. J. Gen. Phys. 1975, 66, 531–532. [Google Scholar] [CrossRef]
- Nonner, W.; Eisenberg, B. Ion Permeation and glutamate residues linked by Poisson-Nernst-Planck theory in L-type calcium channels. Biophys. J. 1998, 75, 1287–1305. [Google Scholar]
- Nonner, W.; Catacuzzeno, L.; Eisenberg, B. Binding and selectivity in L-type Ca channels. Biophys. J. 2000, 79, 1976–1992. [Google Scholar] [CrossRef] [Green Version]
- Eisenberg, B. Crowded Charges in Ion Channels. In Advances in Chemical Physics; Rice, S., Ed.; John Wiley & Sons: New York, NY, USA, 2011; pp. 77–223. [Google Scholar]
- Eisenberg, B. Ionic interactions are everywhere. Physiology 2013, 28, 28–38. [Google Scholar] [CrossRef] [Green Version]
- Maffeo, C.; Bhattacharya, S.; Yoo, J.; Wells, D.; Aksimentiev, A. Modelling and simulation of Ion Channels. Chem. Rev. 2012, 112, 6250–6284. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hollerbach, U.; Chen, D.-P.; Eisenberg, R.S. Two- and three- dimensional Poisson-Nernst-Planck simulations of current flow through Gramicidin A channel. J. Sci. Comp. 2001, 16, 373–409. [Google Scholar] [CrossRef]
- Valent, I.; Petrovič, P.; Neogrády, P.; Schreiber, I.; Marek, M. Electrodiffusion kinetics of ionic transport in a siple membrane channel. J. Phys. Chem. 2013, 117, 14283–14293. [Google Scholar] [CrossRef]
- Cárdenas, A.E.; Coalson, R.D.; Kurnikova, M.G. Three-dimensional Poisson-Nernst-Planck theory studies: Influence of membrane electrostatics on gramicidin A channel conductance. Biophys. J. 2000, 79, 80–93. [Google Scholar] [CrossRef] [Green Version]
- Koumanov, A.; Zachariae, U.; Engelhardt, H.; Karshikoff, A. Improved 3D continuum calculations of ion flux through membrane channels. Eur. Biophys. J. 2003, 32, 689–702. [Google Scholar] [CrossRef]
- Van der Straaten, T.A.; Tang, J.M.; Ravaioli, U.; Eisenberg, R.S.; Aluru, N.R. Simulating ion permeation through the ompF porin ion channel using three-dimensional drift-diffusion theory. J. Comput. Electron. 2003, 2, 29–47. [Google Scholar] [CrossRef]
- Roux, B. The membrane potential and its representation by a constant electric field in computer simulations. Biophys. J. 2008, 95, 4205–4216. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kutzner, C.; Grubmuller, H.; de Groot, B.L.; Zachariae, U. Computational electrophysiology: The molecular dynamics of ion channel permeation and selectivity in atomistic detail. Biophys. J. 2011, 101, 809–817. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jensen, M.; Borhani, W.; Lindorff-Larsen, K.; Maragakis, P.; Jogini, V.; Eastwood, M.P.; Dror, R.O.; Shaw, D.E. Principles of conduction and hydrophobic gating in K+ channels. Proc. Natl. Acad. Sci. USA 2010, 107, 5833–5838. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bidon-Chanal, A.; Krammer, E.-M.; Blot, D.; Pebay-Peyroula, E.; Chipot, C.; Ravaud, S.; Dehez, F. How do membrane transporters sense pH? The case of mitochondrial ADP-ATP carrier. J. Phys. Chem. Lett. 2013, 4, 3787–3791. [Google Scholar] [CrossRef]
- Roux, B.; Allen, T.; Berneche, S.; Im, W. Theoretical and computational models of biological ion channels. Q. Rev. Biophys. 2004, 37, 15–103. [Google Scholar] [CrossRef] [Green Version]
- Krammer, E.-M.; Homblé, F.; Prévost, M. Molecular origin of VDAC selectivity towards inorganic ions: A combined molecular and Brownian dynamics study. Biochem. Biophys. Acta 2013, 1828, 1284–1292. [Google Scholar] [CrossRef] [PubMed]
- Boda, D.; Valiskó, M.; Gillespie, D. Modeling the Device Behavior of Biological and Synthetic Nanopores with Reduced Models. Entropy 2020, 22, 1259. [Google Scholar] [CrossRef]
- Miękisz, J.; Gomułkiewicz, J.; Miękisz, S. Mathematical models of ion transport through cell membrane channels. Math. Appl. 2014, 42, 39–62. [Google Scholar] [CrossRef] [Green Version]
- Kuyucak, S.; Andersen, O.S.; Chung, S.-H. Models of permeation in ion channels. Rep. Prog. Phys. 2001, 64, 1427–1472. [Google Scholar] [CrossRef]
- Corry, B.; Chung, S.-H. Mechanism of valence selectivity in biological ion channels. Cell Mol. Life Sci. 2006, 63, 301–315. [Google Scholar] [CrossRef]
- Liu, J.-L.; Eisenberg, B. Molecular Mean-Field Theory of Ionic Solutions: A Poisson-Nernst-Planck-Bikerman Model. Entropy 2020, 22, 550. [Google Scholar] [CrossRef]
- Bezanilla, F. The voltage sensor in voltage-dependent ion channels. Physiol. Rev. 2000, 80, 555–592. [Google Scholar] [CrossRef] [PubMed]
- Catteral, W.A. Ion channel voltage sensors: Structure, function, and pathophysiology. Neuron 2010, 67, 915–928. [Google Scholar] [CrossRef] [Green Version]
- Tombola, F.; Pathak, M.M.; Isacoff, E.Y. How does voltage open an ion channel? Annu. Rev. Cell. Dev. Biol. 2006, 22, 23–52. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Clarke, D.D.; Sokoloff, L. Circulation and energy metabolism of the brain. In Basic Neurochemistry: Molecular, Cellular, and Medical Aspects, 6th ed.; Siegel, G.J., Agranoff, B.W., Albers, R.W., Fisher, S.K., Uhler, M.D., Eds.; Lippincott-Raven: New York, NY, USA, 1999; pp. 637–669. [Google Scholar]
- Jasielec, J.J.; Filipek, R.; Dołowy, K.; Lewenstam, A. Precipitation of Inorganic Salts in Mitochondrial Matrix. Membranes 2020, 10, 81. [Google Scholar] [CrossRef] [PubMed]
- Griffiths, T.; Evans, M.C.; Meldrum, B.S. Intracellular sites of early calcium accumulation in the rat hippocampus during status epilepticus. Neurosci. Lett. 1982, 30, 329–334. [Google Scholar] [CrossRef]
- Erecińska, M.; Silver, I.A. Relationships between ions and energy metabolism: Celebral calcium movements during ischemia and subsequent recovery. Can. J. Physiol. Pharmacol. 1992, 70, S190–S193. [Google Scholar] [CrossRef]
- Zaidan, E.; Sims, N.R. The calcium content of mitochondria from brain subregions following short-term forebrain ischemia and recirculation in the rat. J. Neurochem. 1994, 63, 1812–1819. [Google Scholar] [CrossRef] [PubMed]
- Fineman, I.; Hovda, D.A.; Smith, M.; Yoshino, A.; Becker, D.P. Concussive brain injury is associated with a prolonged accumulation of calcium: A 45Ca autoradiographic study. Brain Res. 1993, 624, 94–102. [Google Scholar] [CrossRef]
- Sparagna, G.C.; Gunter, K.K.; Sheu, S.S.; Gunter, T.E. Mitochondrial calcium uptake from physiological-type pulses of calcium. A description of the rapid uptake mode. J. Biol. Chem. 1995, 270, 27510–27515. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Williams, G.S.; Boyman, L.; Chikando, A.C.; Khairallah, R.J.; Lederer, W.J. Mitochondrial calcium uptake. Proc. Natl. Acad. Sci. USA 2013, 110, 10479–10486. [Google Scholar] [CrossRef] [Green Version]
- McCormack, J.G.; Denton, R.M. The role of mitochondrial Ca2+ transport and matrix Ca2+ in signal transduction in mammalian tissues. Biochim. Biophys. Acta 1990, 1018, 287–291. [Google Scholar] [CrossRef]
- Kannurpatti, S.S.; Sanganahalli, B.G.; Herman, P.; Hyder, F. Role of mitochondrial calcium uptake homeostasis on resting-state fMRI brain networks. NMR Biomed. 2015, 28, 1579–1588. [Google Scholar] [CrossRef]
- Jasielec, J.J.; Filipek, R.; Szyszkiewicz, K.; Sokalski, T.; Lewenstam, A. Continuous Modeling of Calcium Transport through Biological Membranes. J. Mater. Eng. Perform. 2016, 25, 3285–3290. [Google Scholar] [CrossRef]
- Lehninger, A.L.; Rossi, C.S.; Greenawalt, J.W. Respiration-dependent accumulation of inorganic phosphate and Ca ions by rat liver mitochondria. Biochem. Biophys. Res. Commun. 1963, 10, 444–448. [Google Scholar] [CrossRef]
- Lehninger, A.L. Mitochondria and calcium ion transport. Biochem. J. 1970, 119, 129–138. [Google Scholar] [CrossRef] [Green Version]
- Rossi, C.S.; Lehninger, A.L. Stoichiometric relationships between accumulation of ions by mitochondria and the energy-coupling sites in the respiratory chain. Biochem. Z. 1963, 338, 698–713. [Google Scholar]
- Rossi, C.S.; Lehninger, A.L. Stoichiometry of respiratory stimulation, accumulation of Ca2+ and phosphate and oxidative phosphorylation in rat liver mitochondria. J. Biol. Chem. 1964, 239, 3971–3980. [Google Scholar] [CrossRef]
- Lehninger, A.L.; Carafoli, E.; Rossi, C.S. Energy-linked ion movements in mitochondrial systems. Adv. Enzymol. Relat. Areas Mol. Biol. 1967, 29, 259–320. [Google Scholar] [PubMed]
- Arnaudeau, S.; Kelley, W.L.; Walsh, J.V.; Demaurex, N. Mitochondria recycle Ca2+ to the endoplasmic reticulum and prevent the depletion of neighboring endoplasmic reticulum regions. J. Biol. Chem. 2001, 276, 29430–29439. [Google Scholar] [CrossRef] [Green Version]
- Brandenburger, Y.; Kennedy, E.D.; Python, C.P.; Rossier, M.F.; Vallotton, M.B.; Wollheim, C.B.; Capponi, A.M. Possible role for mitochondrial calcium in angiotensin II- and potassium-stimulated steroidogenesis in bovine adrenal glomerulosa cells. Endocrinology 1996, 137, 5544–5551. [Google Scholar] [CrossRef]
- Schreur, J.H.; Figueredo, V.M.; Miyamae, M.; Shames, D.M.; Baker, A.J.; Camacho, S.A. Cytosolic and mitochondrial [Ca2+] in whole hearts using indo-1 acetoxymethyl ester: Effects of high extracellular Ca2+. Biophys. J. 1996, 70, 2571–2580. [Google Scholar] [CrossRef] [Green Version]
- Miyata, H.; Silverman, H.S.; Sollott, S.J.; Lakatta, E.G.; Stern, M.D.; Hansford, R.G. Measurement of mitochondrial free Ca2+ concentration in living single rat cardiac myocytes. Am. J. Physiol. 1991, 261, H1123–H1134. [Google Scholar] [CrossRef] [PubMed]
- Moreno-Sanchez, R.; Hansford, R.G. Dependence of cardiac mitochondrial pyruvate dehydrogenase activity on intramitochondrial free Ca2+ concentration. Biochem. J. 1988, 256, 403–412. [Google Scholar] [CrossRef] [Green Version]
- Allen, S.P.; Stone, D.; McCormack, J.G. The loading of fura-2 into mitochondria in the intact perfused rat heart and its use to estimate matrix Ca2+ under various conditions. J. Mol. Cell. Cardiol. 1992, 24, 765–773. [Google Scholar] [CrossRef]
- Lukács, G.L.; Kapus, A. Measurement of the matrix free Ca2+ concentration in heart mitochondria by entrapped fura-2 and quin2. Biochem. J. 1987, 248, 609–613. [Google Scholar] [CrossRef] [Green Version]
- Davis, M.H.; Altschuld, R.A.; Jung, D.W.; Brierley, G.P. Estimation of intramitochondrial pCa and pH by fura-2 and 2,7 biscarboxyethyl-5(6)-carboxyfluorescein (BCECF) fluorescence. Biochem. Biophys. Res. Commun. 1987, 149, 40–45. [Google Scholar] [CrossRef]
- Ivannikov, M.V.; Macleod, G.T. Mitochondrial free Ca2+ levels and their effects on energy metabolism in Drosophila motor nerve terminals. Biophys. J. 2013, 104, 2353–2361. [Google Scholar] [CrossRef] [Green Version]
- Dołowy, K. Calcium phosphate buffer formed in the mitochondrial matrix during preconditioning supports ∆pH formation and ischemic ATP production and prolongs cell survival—A hypothesis. Mitochondrion 2019, 47, 210–217. [Google Scholar] [CrossRef]
- Liou, G.Y.; Storz, P. Reactive oxygen species in cancer. Free Radic. Res. 2010, 44, 479–496. [Google Scholar] [CrossRef] [Green Version]
- Simonian, N.A.; Coyle, J.T. Oxidative Stress in Neurodegenerative Diseases. Ann. Rev. Pharmacol. Toxicol. 1996, 36, 83–106. [Google Scholar] [CrossRef] [PubMed]
- Barnham, K.J.; Masters, C.L.; Bush, A.I. Neurodegenerative diseases and oxidatives stress. Nat. Rev. Drug Discov. 2004, 3, 205–214. [Google Scholar] [CrossRef] [PubMed]
- Rao, A.V.; Balachandran, B. Role of oxidative stress and antioxidants in neurodegenerative diseases. Nutr. Neurosci. 2002, 5, 291–309. [Google Scholar] [CrossRef]
- Corry, B.; Kuyucak, S.; Chung, S.H. Invalidity of continuum theories of electrolytes in nanopores. Chem. Phys. Lett. 2000, 320, 35–41. [Google Scholar] [CrossRef]
- Collins, K.D. Why continuum electrostatics theories cannot explain biological structure, polyelectrolytes or ionic strength effects in ion–protein interactions. Biophys. Chem. 2012, 167, 43–59. [Google Scholar] [CrossRef] [Green Version]
- Willems, K.; Ruić, D.; Lucas, F.L.R.; Barman, U.; Verellen, N.; Hofkens, J.; Maglia, G.; Van Dorpe, P. Accurate modeling of a biological nanopore with an extended continuum framework. Nanoscale 2020, 12, 16775–16795. [Google Scholar] [CrossRef]
- Moy, G.; Corry, B.; Kuyucak, S.; Chung, S.-H. Tests of continuum theories as models of ion channels. I. Poisson-Boltzmann theory versus Brownian dynamics. Biophys. J. 2000, 78, 2349–2363. [Google Scholar] [CrossRef] [Green Version]
- Kuyucak, S.; Bastug, T. Physics of Ion Channels. J. Biol. Phys. 2003, 29, 429–446. [Google Scholar] [CrossRef]
- Corry, B.; Kuyucak, S.; Chung, S.-H. Dielectric self-energy in Poisson-Boltzmann and Poisson-Nernst-Planck models of ion channels. Biophys. J. 2003, 84, 3594–3606. [Google Scholar] [CrossRef] [Green Version]
- Im, W.; Roux, B. Ion permeation and selectivity of OmpF porin: A theoretical study based on molecular dynamics, Brownian dynamics, and continuum electrodiffusion theory. J. Mol. Biol. 2002, 322, 851–869. [Google Scholar] [CrossRef]
- Graf, P.; Kurnikova, M.G.; Coalson, R.D.; Nitzan, A. Comparison of dynamic lattice Monte-Carlo simulations and dielectric self energy Poisson-Nernst-Planck continuum theory for model ion channels. J. Phys. Chem. B 2004, 108, 2006–2015. [Google Scholar] [CrossRef]
- Nadler, B.; Hollerbach, U.; Eisenberg, R.S. Dielectric boundary force and its crucial role in gramicidin. Phys. Rev. E 2003, 68, 021905. [Google Scholar] [CrossRef] [PubMed]
- Gillespie, D.; Nonner, W.; Eisenberg, R.S. Coupling Poisson-Nernst-Planck and density functional theory to calculate ion flux. J. Phys. Condens. Matter 2002, 14, 12129–12145. [Google Scholar] [CrossRef]
- Gillespie, D.; Nonner, W.; Eisenberg, R.S. Density functional theory of charged, hard-sphere fluids. Phys. Rev. E 2003, 68, 0313503. [Google Scholar] [CrossRef] [Green Version]
- Roth, R. Fundamental measure theory for hard-sphere mixtures: A review. J. Phys. Condens. Matter 2010, 22, 063102. [Google Scholar] [CrossRef] [Green Version]
- Gillespie, D.; Xu, L.; Wang, Y.; Meissner, G. (De)constructing the ryanodine receptor: Modelling ion permeation and selectivity of the calcium release channel. J. Phys. Chem. B 2005, 109, 15598–15610. [Google Scholar] [CrossRef]
- Borukhov, I.; Andelman, D.; Orland, H. Steric Effects in Electrolytes: A Modified Poisson-Boltzmann Equation. Phys. Rev. Lett. 1997, 79, 435–438. [Google Scholar] [CrossRef] [Green Version]
- Kilic, M.S.; Bazant, M.Z.; Ajdari, A. Steric effects in the dynamics of electrolytes at large applied voltages. I. Double-layer charging. Phys. Rev. E 2007, 75, 021502. [Google Scholar] [CrossRef] [Green Version]
- Lu, B.; Zhou, Y.C. Poisson-Nernst-Planck equations for simulating biomolecular diffusion-reaction processes II: Size effects of ionic distribution and diffusion-reaction rates. Biophys. J. 2011, 100, 2475–2485. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, J.-L.; Eisenberg, B. Analytical Models of Calcium Binding in a Calcium Channel. J. Chem. Phys. 2014, 141, 075102. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.-L. Numerical Methods for the Poisson-Fermi Equation in Electrolytes. J. Comput. Phys. 2013, 247, 88–99. [Google Scholar] [CrossRef]
- Liu, J.-L.; Eisenberg, B. Correlated Ions in a Calcium Channel Model: A Poisson-Fermi Theory. J. Phys. Chem. B 2013, 117, 12051–12058. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.-L.; Eisenberg, B. Poisson-Fermi Model of Single Ion Activities in Aqueous Solutions Chem. Phys. Lett. 2015, 637, 1–6. [Google Scholar]
- Liu, J.-L.; Eisenberg, B. Poisson-Nernst-Planck-Fermi Theory for Modeling Biological Ion Channels. J. Chem. Phys. 2014, 141, 22D532. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.-L.; Eisenberg, B. Numerical Methods for a Poisson-Nernst-Planck-Fermi Model of Biological Ion Channels. Phys. Rev. E 2015, 92, 012711. [Google Scholar] [CrossRef]
- Lu, B.; Hoogerheide, D.P.; Zhao, Q.; Yu, D. Effective driving force applied on DNA inside a solid-state nanopore. Phys. Rev. E 2012, 86, 011921. [Google Scholar] [CrossRef] [Green Version]
- Pederson, E.D.; Barbalas, J.; Drown, B.S.; Culbertson, M.J.; Keranen Burden, L.M.; Kasianowicz, J.J.; Burden, D.L. Proximal Capture Dynamics for a Single Biological Nanopore Sensor. J. Phys. Chem. B 2015, 119, 10448–10455. [Google Scholar] [CrossRef] [PubMed]
- Burger, M.; Schlake, B.; Wolfram, M.-T. Nonlinear Poisson–Nernst–Planck equations for ion flux through confined geometries. Nonlinearity 2012, 25, 961–990. [Google Scholar] [CrossRef] [Green Version]
- Simakov, N.A.; Kurnikova, M.G. Soft Wall Ion Channel in Continuum Representation with Application to Modeling Ion Currents in α-Hemolysin. J. Phys. Chem. B 2010, 114, 15180–15190. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Simakov, N.A.; Kurnikova, M.G. Membrane Position Dependency of the pK a and Conductivity of the Protein Ion Channel. J. Membr. Biol. 2018, 251, 393–404. [Google Scholar] [CrossRef]
- Furini, S.; Zerbetto, F.; Cavalcanti, S. Application of the Poisson-Nernst-Planck Theory with Space-Dependent Diffusion Coefficients to KcsA. Biophys. J. 2006, 91, 3162–3169. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, D. A New Poisson–Nernst–Planck Model with Ion–Water Interactions for Charge Transport in Ion Channels. Bull. Math. Biol. 2016, 78, 1703–1726. [Google Scholar] [CrossRef] [PubMed]
- Eisenberg, B.; Hyon, Y.; Liu, C. Energy variational analysis of ions in water and channels: Field theory for primitive models of complex ionic fluids. J. Chem. Phys. 2010, 133, 104104. [Google Scholar] [CrossRef] [Green Version]
- Vlassiouk, I.; Smirnov, S.; Siwy, Z. Ionic Selectivity of Single Nanochannels. Nano Lett. 2008, 8, 1978–1985. [Google Scholar] [CrossRef]
- Zhang, M.; Yeh, L.-H.; Qian, S.; Hsu, J.-P.; Joo, S.W. DNA electrokinetic translocation through a nanopore: Local permittivity environment effect. J. Phys. Chem. C 2012, 116, 4793–4801. [Google Scholar] [CrossRef]
- Yeh, L.-H.; Zhang, M.; Qian, S.; Hsu, J.-P.; Tseng, S. Ion Concentration Polarization in polyelectrolyte-modified nanopores. J. Phys. Chem. C 2012, 116, 8672–8677. [Google Scholar] [CrossRef]
- Gracheva, M.; Xiong, A.; Aksimentiev, A.; Schulten, K.; Timp, G.; Leburton, J.-P. Simulation of the electric response of DNA translocation through a semiconductor nanopore-capacitor. Nanotechnology 2006, 17, 662. [Google Scholar] [CrossRef] [Green Version]
- Gracheva, M.E.; Xiong, A.; Aksimentiev, A.; Leburton, J.-P. Electrical signatures of single-stranded DNA with single base mutations in a nanopore capacitor. Nanotechnology 2006, 17, 3160–3165. [Google Scholar] [CrossRef]
- Gracheva, M.E.; Leburton, J.-P. Electrolytic charge inversion at the liquid-solid interface in a nanopore in a doped semiconductor membrane. Nanotechnology 2007, 18, 145704. [Google Scholar] [CrossRef] [Green Version]
- Halnes, G.; Østby, I.; Pettersen, K.H.; Omholt, S.W.; Einevoll, G.T. Electrodiffusive model for astrocytic and neuronal ion concentration dynamics. PLoS Comput. Biol. 2013, 9, e1003386. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Halnes, G.; Østby, I.; Pettersen, K.H.; Omholt, S.W.; Einevoll, G.T. An Electrodiffusive Formalism for Ion Concentration Dynamics in Excitable Cells and the Extracellular Space Surrounding Them. In Advances in Cognitive Neurodynamics (IV); Liljenström, H., Ed.; Springer: Dordrecht, The Netherlands, 2015; pp. 353–360. [Google Scholar]
- Solbrå, A.; Bergersen, A.W.; van den Brink, J.; Malthe-Sørenssen, A.; Einevoll, G.T.; Halnes, G. A Kirchhoff-Nernst-Planck framework for modeling large scale extracellular electrodiffusion surrounding morphologically detailed neurons. PLoS Comput. Biol. 2018, 14, e1006510. [Google Scholar] [CrossRef] [PubMed] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jasielec, J.J. Electrodiffusion Phenomena in Neuroscience and the Nernst–Planck–Poisson Equations. Electrochem 2021, 2, 197-215. https://doi.org/10.3390/electrochem2020014
Jasielec JJ. Electrodiffusion Phenomena in Neuroscience and the Nernst–Planck–Poisson Equations. Electrochem. 2021; 2(2):197-215. https://doi.org/10.3390/electrochem2020014
Chicago/Turabian StyleJasielec, Jerzy J. 2021. "Electrodiffusion Phenomena in Neuroscience and the Nernst–Planck–Poisson Equations" Electrochem 2, no. 2: 197-215. https://doi.org/10.3390/electrochem2020014
APA StyleJasielec, J. J. (2021). Electrodiffusion Phenomena in Neuroscience and the Nernst–Planck–Poisson Equations. Electrochem, 2(2), 197-215. https://doi.org/10.3390/electrochem2020014





