The Influence of Mass on Dynamic Response of Cracked Timoshenko Beam with Restrained End Conditions: The Truncated Theory
Abstract
1. Introduction
2. Free Vibrations Analysis of a Timoshenko Cracked Beam: Truncated Theory
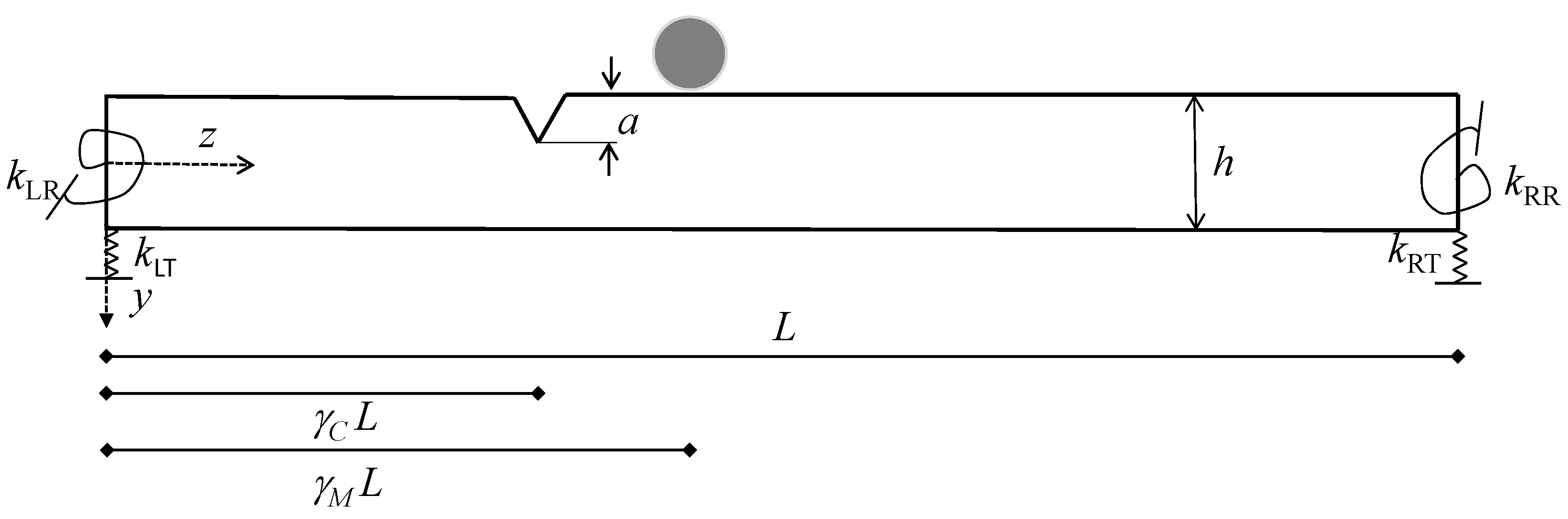
2.1. Variational Formulation of a Timoshenko Beam in the Presence of a Crack and Mass: The Truncated Theory
2.2. Crack Effect Theory for Beam Analysis
3. Numerical Results and Discussion
3.1. Beam with a Crack: Comparison of Results
3.2. Beam with a Crack: Variation of the Position Parameter
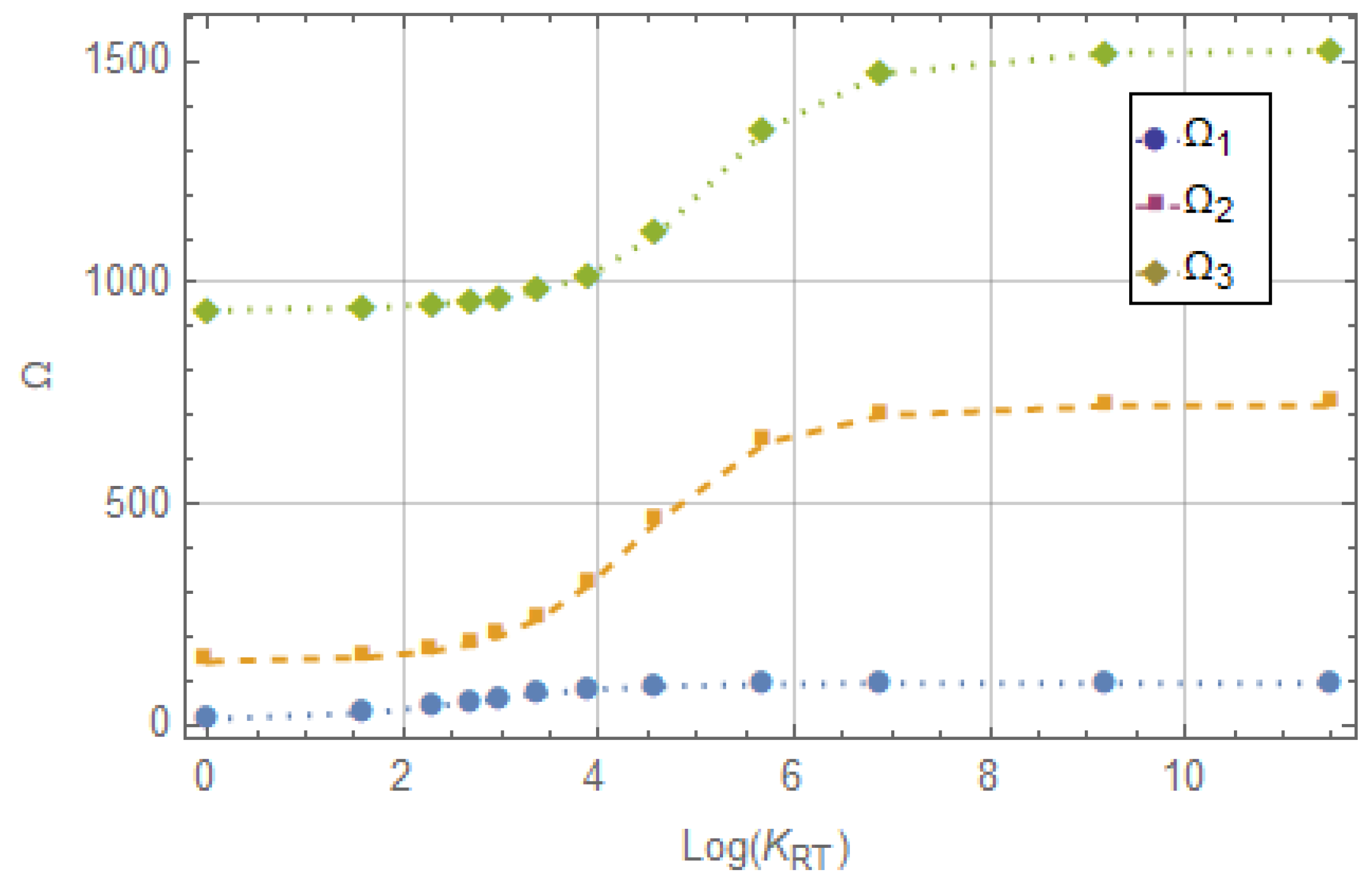
3.3. Beam with a Crack: Clamped–Clamped Beam with Flexible Restraints at the Free End
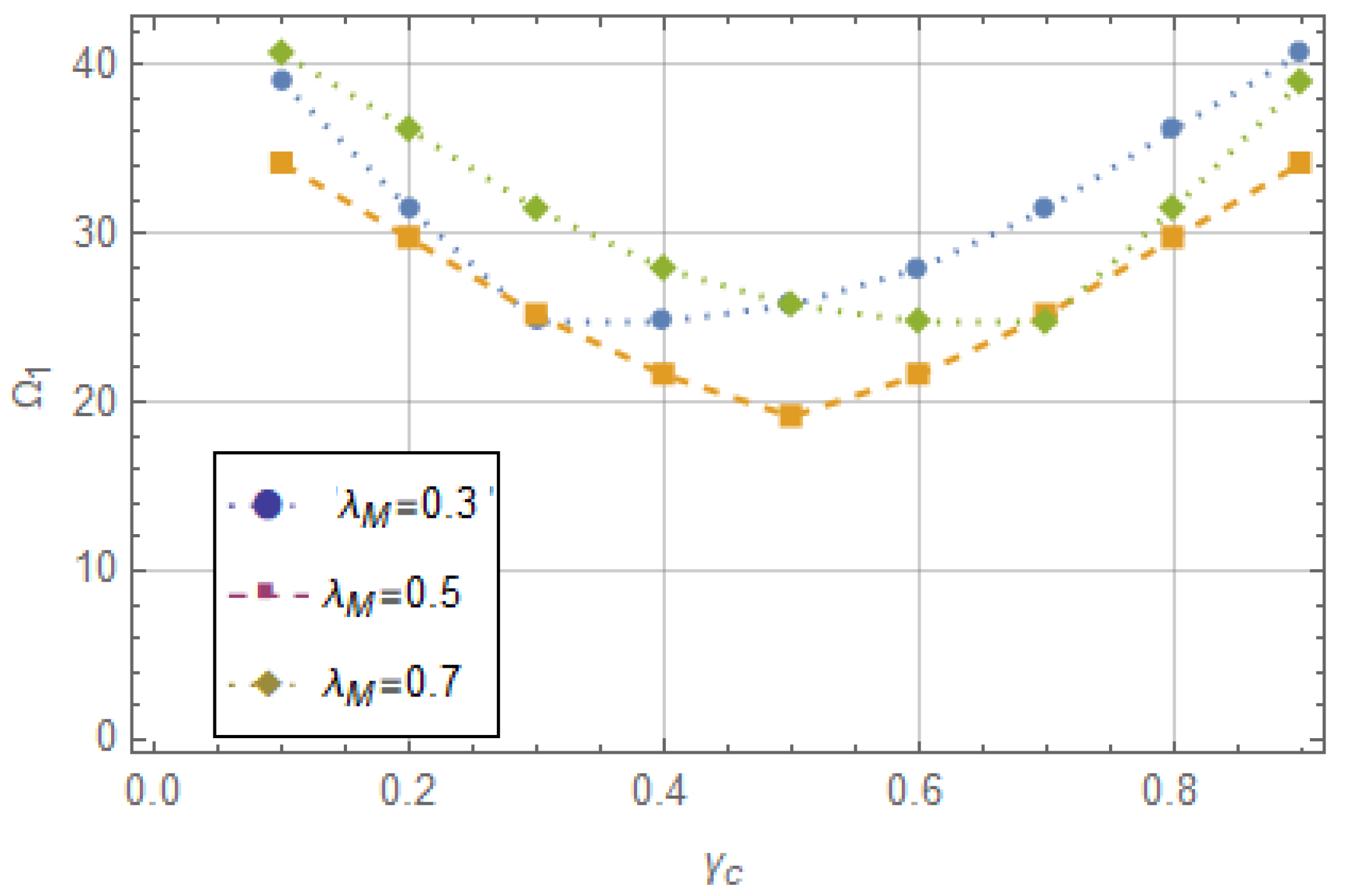
3.4. Dynamic Problem of a Timoshenko Beam in the Presence of a Concentrated Mass and Variable Crack
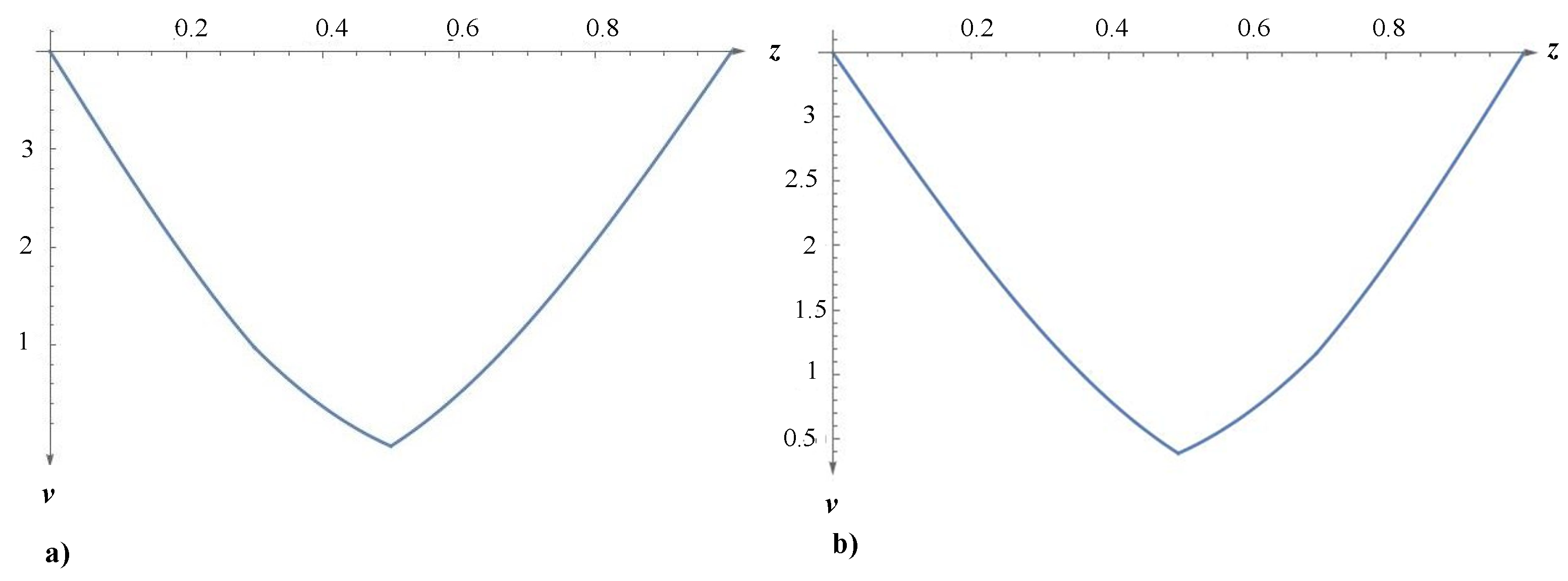
3.4.1. Dynamic Problem of a Timoshenko Simply Supported Beam in the Presence of a Concentrated Mass and Variable Crack
3.4.2. Dynamic Problem of a Timoshenko Clamped–Free Beam in the Presence of a Concentrated Mass and Crack
4. Concluding Remarks
- The change in natural frequencies depends largely on the boundary conditions and the length/depth ratios of the beam.Boundary conditions (such as simply supported, clamped, free, or a combination of these) influence the constraints on the deflection and rotation of the beam, which in turn affect the stiffness and modal properties. The eigenvalue problem, which governs the beam’s natural frequencies, changes with each set of boundary conditions. The aspect ratio of the beam has a direct impact on stiffness and slenderness ratio , which in turn influences the natural frequency value. In general, if the length-to-depth ratio increases, the stiffness relative to mass decreases, resulting in lower natural frequencies. In contrast, a smaller ratio enhances structural stiffness while increasing natural frequencies. Simply supported beams have lower fundamental frequencies than clamped beams due to the smaller value of (the first mode constant). Similarly, increasing reduces frequency when decreases relative to .
- The crack plays a key role in the dynamic behavior of the beam: the values of the natural frequencies increase when the dimensionless transverse and rotational stiffness parameters in the flexible supports increase.The crack introduces a localized reduction in stiffness, causing an uneven distribution of bending rigidity along the beam. As a result, the effective stiffness in the natural frequency equation is altered. The depth, location, and orientation of the crack affect the resistance to deformation of the beam, which results in variations in the modal shapes and frequencies.
- The first non-dimensional natural frequency of the beam is sensitive to added mass and its location.Adding mass alters the system’s overall inertial properties, specifically the effective mass involved in vibration for various modes. The placement of the added mass affects the mass distribution and coupling with mode shapes, influencing how the beam vibrates. The natural frequency is determined by the equilibrium of stiffness and inertia. Adding bulk increases inertia without changing stiffness directly, lowering the natural frequency. The first mode of frequency is particularly sensitive because it often involves the greatest relative displacement along the beam, making it more susceptible to changes in the mass distribution.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, Y.; Zhang, Y.; Tan, X. Review on Vibration-Based Structural Health Monitoring Techniques and Technical Codes. Symmetry 2021, 13, 1998. [Google Scholar] [CrossRef]
- Meirovitch, L. Fundamentals of Vibrations; McGraw-Hill: Boston, MA, USA, 2001; ISBN 978-0-07-041345-0. [Google Scholar]
- Doebling, S.W.; Farrar, C.R.; Prime, M.B.; Shevitz, D.W. Damage Identification and Health Monitoring of Structural and Mechanical Systems from Changes in Their Vibration Characteristics: A Literature Review; SAGE Publications: New York, NY, USA, 1996. [Google Scholar]
- Chondros, T.G.; Dimarogonas, A.D.; Yao, J. A continuous cracked beam vibration theory. J. Sound Vibr. 1998, 215, 17–34. [Google Scholar] [CrossRef]
- Adams, R.D.; Cawley, P.; Pye, C.J.; Stone, B.J. A vibration technique for non-destructively assessing the integrity of structures. J. Mech. Eng. Sci. 1978, 20, 93–100. [Google Scholar] [CrossRef]
- Sinha, J.K.; Friswell, M.I.; Edwards, S. Simplified models for the location of cracks in beam structures using measured vibration data. J. Sound Vibr. 2002, 251, 13–38. [Google Scholar] [CrossRef]
- Thatoi, D.; Jena, P.; Acharya, A.; Mohapatra, S.; Nanda, J. Analysis of the dynamic response of a cracked cantilever beam. In Proceedings of the AICTE Sponsored National Conference on Emerging Trend and its Application in Engineering (NCETAE), Shillong, India, 4–5 March 2011. [Google Scholar]
- Narkis, Y. Identification of crack location in vibrating simply supported beams. J. Sound Vibr. 1994, 172, 549–558. [Google Scholar] [CrossRef]
- Khiem, N.; Lien, T. A simplified method for natural frequency analysis of a multiple cracked beam. J. Sound Vibr. 2001, 245, 737–751. [Google Scholar] [CrossRef]
- Caddemi, S.; Calio, I. Exact closed-form solution for the vibration modes of the Euler- Bernoulli beam with multiple open cracks. J. Sound Vibr. 2009, 327, 473–489. [Google Scholar] [CrossRef]
- Khiem, N.; Hai, T. A closed-form solution for free vibration of beams with arbitrary number of cracks. In Proceedings of the Scientific Conference Dedicated to 35th Anniversary of Vietnam Academy of Science and Technology, Hanoi, Vietnam, 26 October 2010; Volume 1, pp. 30–42. [Google Scholar]
- Timoshenko, S. On the correction for shear of the differential equation for transverse vibrations of prismatic bars. Philos. Mag. 1921, 41, 744–746. [Google Scholar] [CrossRef]
- Li, Q. Vibratory characteristics of Timoshenko beams with arbitrary number of cracks. J. Eng. Mech. 2003, 129, 1355–1359. [Google Scholar] [CrossRef]
- Loya, J.; Rubio, L.; Fernández-Sáez, J. Natural frequencies for bending vibrations of Timoshenko cracked beams. J. Sound Vibr. 2006, 290, 640–653. [Google Scholar] [CrossRef]
- Khaji, N.; Shafiei, M.; Jalalpour, M. Closed-form solutions for crack detection problem of Timoshenko beams with various boundary conditions. Int. J. Mech. Sci. 2009, 51, 667–681. [Google Scholar] [CrossRef]
- Elishakoff, I.; Hache, F.; Challamel, N. Critical contrasting of three versions of vibrating Bresse- Timoshenko beam with a crack. Int. J. Sol. Struct. 2017, 109, 143–151. [Google Scholar] [CrossRef]
- Khiem, N.; Hung, D. A closed-form solution for free vibration of multiple cracked Timoshenko beam and application. Vietnam J. Mech. 2017, 39, 315–328. [Google Scholar] [CrossRef] [PubMed]
- De Rosa, M.; Lippiello, M. Closed-form solutions for vibrations analysis of cracked Timoshenko beams on elastic medium: An analytical approach. Eng. Struc. 2021, 236, 111946. [Google Scholar] [CrossRef]
- De Rosa, M.; Lippiello, M.; Elishakoff, I. Variational Derivation of Truncated Timoshenko- Ehrenfest Beam Theory. J. Appl. Comput. Mech. 2022, 8, 996–1004. [Google Scholar]
- De Rosa, M.; Lippiello, M.; Elishakoff, I. Reduced Theories for Thick Shells. In Proceedings of the ASME 2022 International Mechanical Engineering Congress and Exposition, Columbus, OH, USA, 30 October–3 November 2022. IMECE2022-96660, V005T07A003. [Google Scholar]
- De Rosa, M.A.; Elishakoff, I.; Onorato, A.; Lippiello, M. Dynamic Analysis of a Timoshenko–Ehrenfest Single-Walled Carbon Nanotube in the Presence of Surface Effects: The Truncated Theory. Appl. Mech. 2023, 4, 1100–1113. [Google Scholar] [CrossRef]
- Ostachowitz, W.M.; Krawczuk, M. Analysis of the effect of cracks on the natural frequencies of a cantilever beam. J. Sound Vibr. 1991, 150, 91–201. [Google Scholar] [CrossRef]
- Wolfram, S. The Mathematica 8; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Khoa, N.V.; Quang, N.V. Free vibration of a cracked double-beam carrying a concentrated mass. Vietnam J. Mech. VAST 2016, 38, 279–293. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Shafiei, M.; Khaji, N. Analytical solutions for free and forced vibrations of a multiple cracked Timoshenko beam subject to a concentrated moving load. Act. Mech. 2011, 221, 79–97. [Google Scholar] [CrossRef]
- Murat, R.; Quang, N.V. Vibration of a cracked cantilever beam under moving mass load. J. Civil Eng. Manag. 2012, 18, 106–113. [Google Scholar]

| BC | TCT [18] | TTT | |||||
|---|---|---|---|---|---|---|---|
| S-S | 3 | 6781.8 | 27,316.2 | 42,718.8 | 6751.6 | 26,321.6 | 40,125.5 |
| 5 | 2877.3 | 12,222.2 | 21,006.4 | 2874.7 | 12,079.3 | 20,439.8 | |
| 7 | 1580.3 | 6803.3 | 12,430.6 | 1580.0 | 6773.6 | 12,278.2 | |
| 9 | 996.9 | 4293.3 | 8177.0 | 996.7 | 4285.1 | 8127.3 | |
| S-S-C | 3 | 10,343.4 | 29,333.0 | 43,315.6 | 10,265.6 | 28,392.6 | 41,219.1 |
| 5 | 4544.2 | 13,922.1 | 22,439.0 | 4535.3 | 13,743.7 | 21,881.8 | |
| 7 | 2517.8 | 8010.8 | 13,729.6 | 2516.14 | 7967.4 | 13,621.1 | |
| 9 | 1590.8 | 5155.7 | 9217.4 | 1590.38 | 5142.63 | 9153.78 | |
| C-C | 3 | 13,628.4 | 31,009 | 43,865.0 | 13,482.0 | 30,243.5 | 42,172.0 |
| 5 | 6205.3 | 15,650.9 | 23,599.0 | 6185.27 | 15,451.0 | 23,053.8 | |
| 7 | 3509.1 | 9335.6 | 14,796.1 | 3504.9 | 9276.83 | 14,601.8 | |
| 9 | 2243.2 | 6134.7 | 10,098.1 | 2242.0 | 6114.9 | 10,021.7 | |
| C-F | 3 | 3134.9 | 12,664.4 | 33,381.4 | 3131.7 | 12,541.7 | 32,301.4 |
| 5 | 1222.9 | 5888.2 | 16,504.4 | 1222.7 | 5870.16 | 16,245.0 | |
| 7 | 642.9 | 3352.9 | 9683.4 | 642.9 | 3349.09 | 9612.9 | |
| 9 | 394.9 | 2153.2 | 6295.6 | 394.9 | 2151.2 | 6273.1 |
| 0.5 | 6751.60 | 26,321.60 | 40,125.50 |
| 0.4 | 6878.80 | 25,001.90 | 42,959.40 |
| 0.3 | 7266.16 | 23,029.50 | 44,312.70 |
| 0.2 | 7894.47 | 22,268.80 | 41,018.40 |
| 0.1 | 8575.90 | 24,029.50 | 39,869.30 |
| 0 | 8.8037 | 141.195 | 936.586 |
| 0.1 | 6.2534 | 123.249 | 853.069 |
| 0.2 | 4.8352 | 115.16 | 815.17 |
| 0.3 | 3.9375 | 110.603 | 794.171 |
| 0.4 | 3.3194 | 107.689 | 780.933 |
| 0.5 | 2.8685 | 105.669 | 771.851 |
| 0.6 | 2.5251 | 104.187 | 765.242 |
| 0.7 | 2.2549 | 103.054 | 760.22 |
| 0.8 | 2.0369 | 102.16 | 756.277 |
| 0.9 | 1.8573 | 101.437 | 753.099 |
| 1 | 1.7067 | 100.839 | 750.484 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Rosa, M.A.; Ceraldi, C.; Martin, H.D.; Onorato, A.; Piovan, M.T.; Lippiello, M. The Influence of Mass on Dynamic Response of Cracked Timoshenko Beam with Restrained End Conditions: The Truncated Theory. Appl. Mech. 2025, 6, 11. https://doi.org/10.3390/applmech6010011
De Rosa MA, Ceraldi C, Martin HD, Onorato A, Piovan MT, Lippiello M. The Influence of Mass on Dynamic Response of Cracked Timoshenko Beam with Restrained End Conditions: The Truncated Theory. Applied Mechanics. 2025; 6(1):11. https://doi.org/10.3390/applmech6010011
Chicago/Turabian StyleDe Rosa, Maria Anna, Carla Ceraldi, Hector D. Martin, Antonella Onorato, Marcelo Tulio Piovan, and Maria Lippiello. 2025. "The Influence of Mass on Dynamic Response of Cracked Timoshenko Beam with Restrained End Conditions: The Truncated Theory" Applied Mechanics 6, no. 1: 11. https://doi.org/10.3390/applmech6010011
APA StyleDe Rosa, M. A., Ceraldi, C., Martin, H. D., Onorato, A., Piovan, M. T., & Lippiello, M. (2025). The Influence of Mass on Dynamic Response of Cracked Timoshenko Beam with Restrained End Conditions: The Truncated Theory. Applied Mechanics, 6(1), 11. https://doi.org/10.3390/applmech6010011







