Design Considerations for a Compressed Stiffened Plate with Skin–Stringer Debonding Based on the Evaluation of Adhesive Layer Stress Distribution
Abstract
1. Introduction
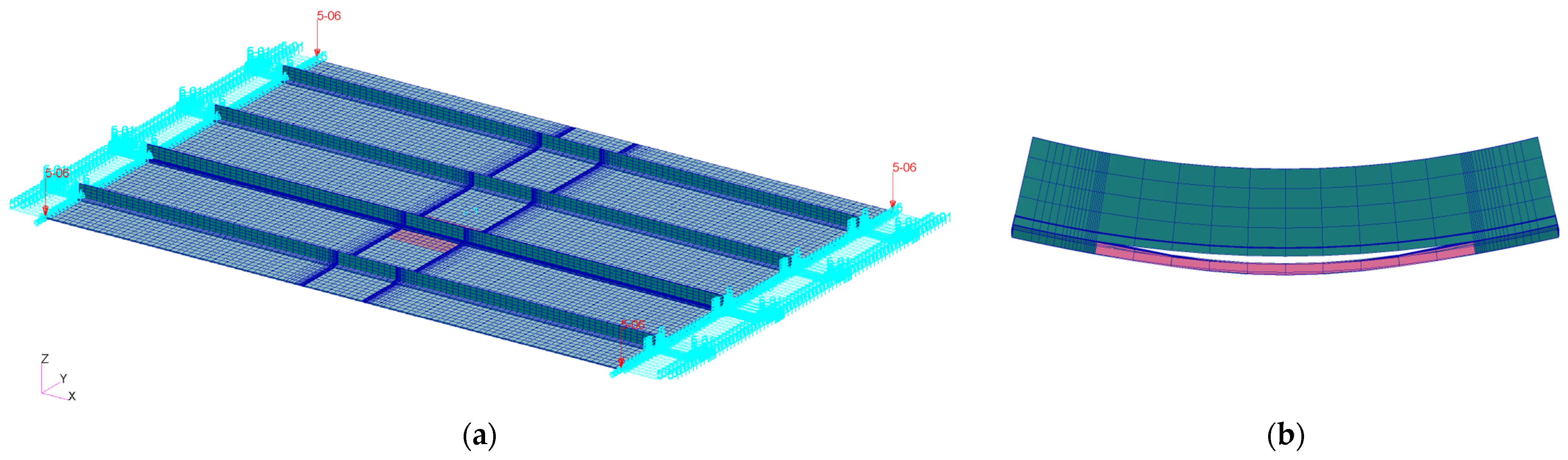
2. Development of FEM
2.1. Model Geometry
2.2. Material Properties
2.3. Mesh
2.3.1. Skin Mesh Size
- Mesh size of 10 × 2 × 2 mm for the intact stiffeners;
- Mesh size of 10 × 1 × 2 mm for the stiffener with the debonding defect.
- Mesh size of 10 mm from the panel edge to 37 mm from the defect tip;
- Four elements with a length of 5 mm;
- Four elements with a length of 2 mm;
- Six elements with a length of 1 mm;
- Six elements with a length of 0.5 mm;
- Mesh size of approximately 10 mm up to the centre of the structure.
2.3.2. Adhesive Layer Mesh Size
2.3.3. Stiffener Mesh Size
2.3.4. Mesh Convergence
2.4. Boundary and Loading Conditions
2.4.1. Boundary Conditions
- The central node of the plate is constrained in the longitudinal direction to prevent unwanted translations along the main axis (x). While not entirely accurate for the damaged structure, this condition is necessary to ensure the analysis can be performed.
- Two nodes of the plate, located at the central region of the end sections, are constrained in the lateral direction (y) to fix transverse rigid displacement.
- The nodes along the four edges of the plate are constrained to prevent vertical displacement (z), simulating a simple lateral support.
2.4.2. Loading Conditions
- A total displacement of 1 mm is imposed on the nodes at the end sections, evenly distributed as 0.5 mm at each end, to apply a uniform compressive load.
- A perturbation of 5 Pa is applied (only for static nonlinear analyses) to the lower surface of the plate, oriented downward.
2.5. FEM Analysis
2.5.1. Linear Buckling Analysis
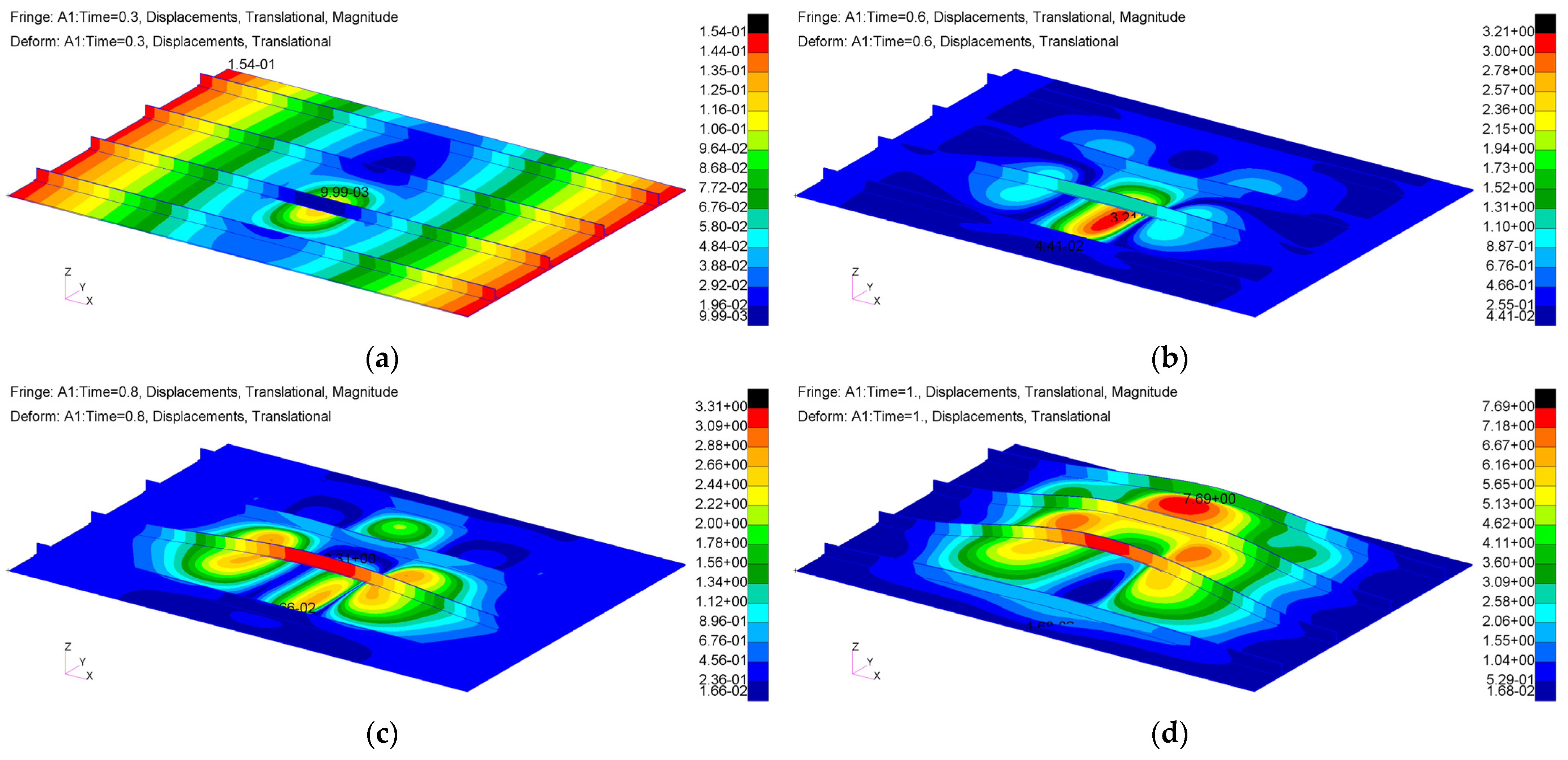
2.5.2. Nonlinear Static Analysis
3. Results
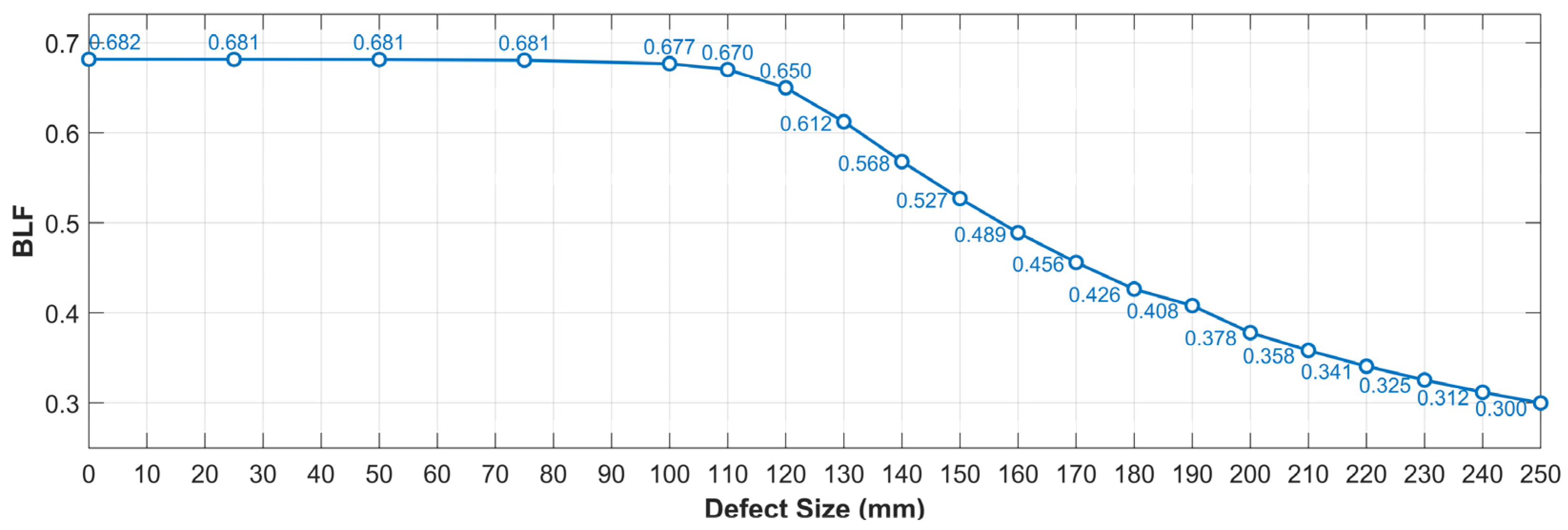
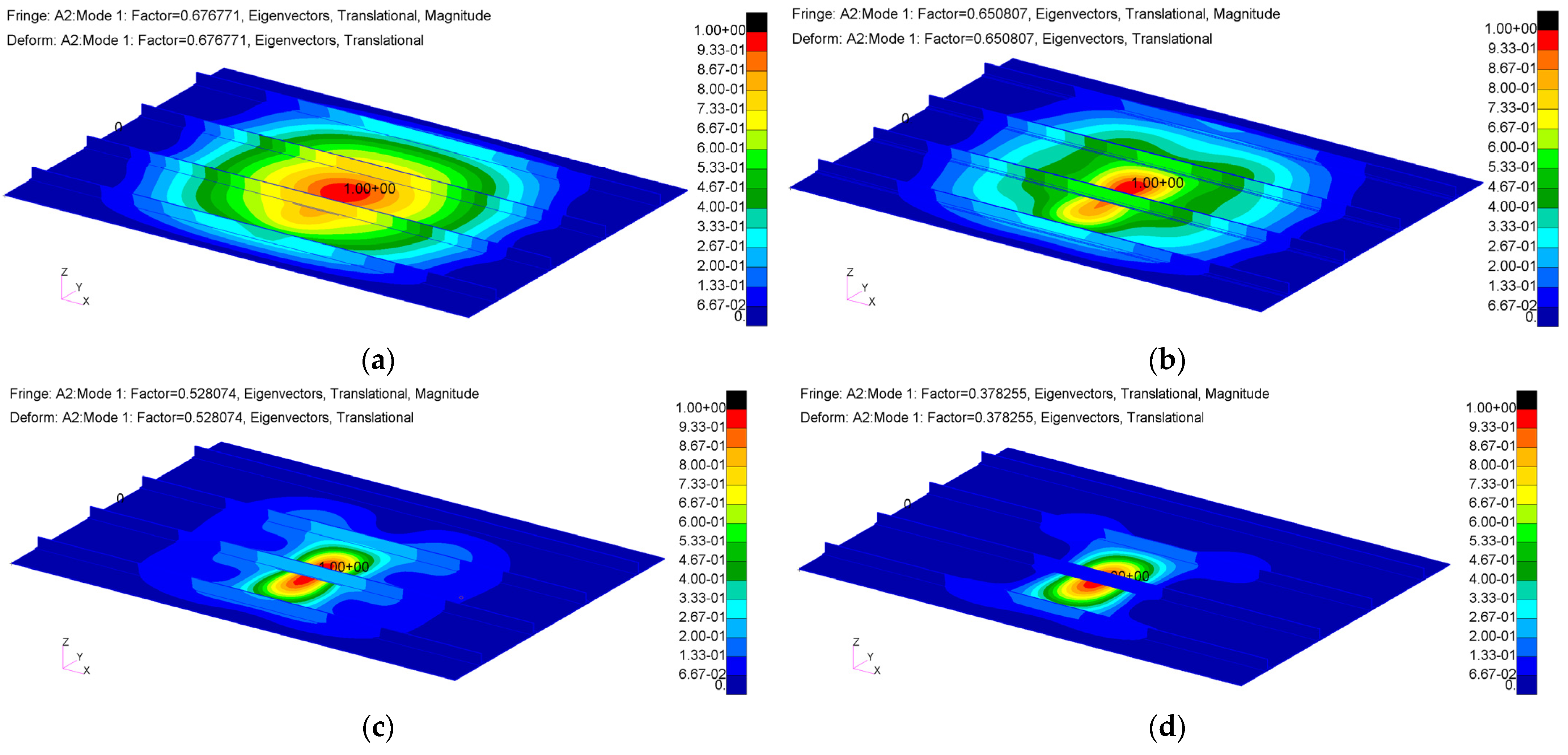
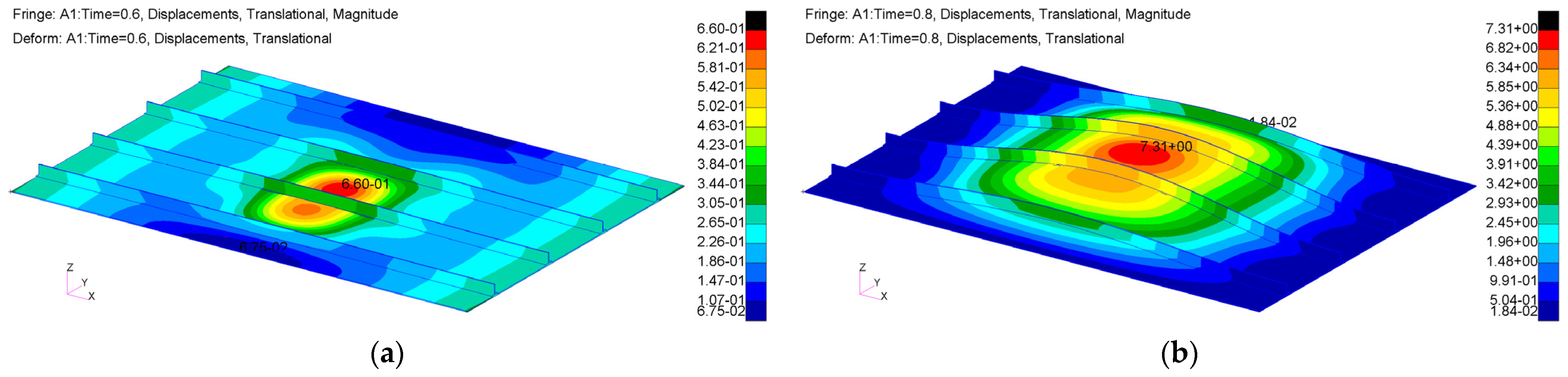
3.1. Critical Conditions
3.2. Stress State at Tip of Defect
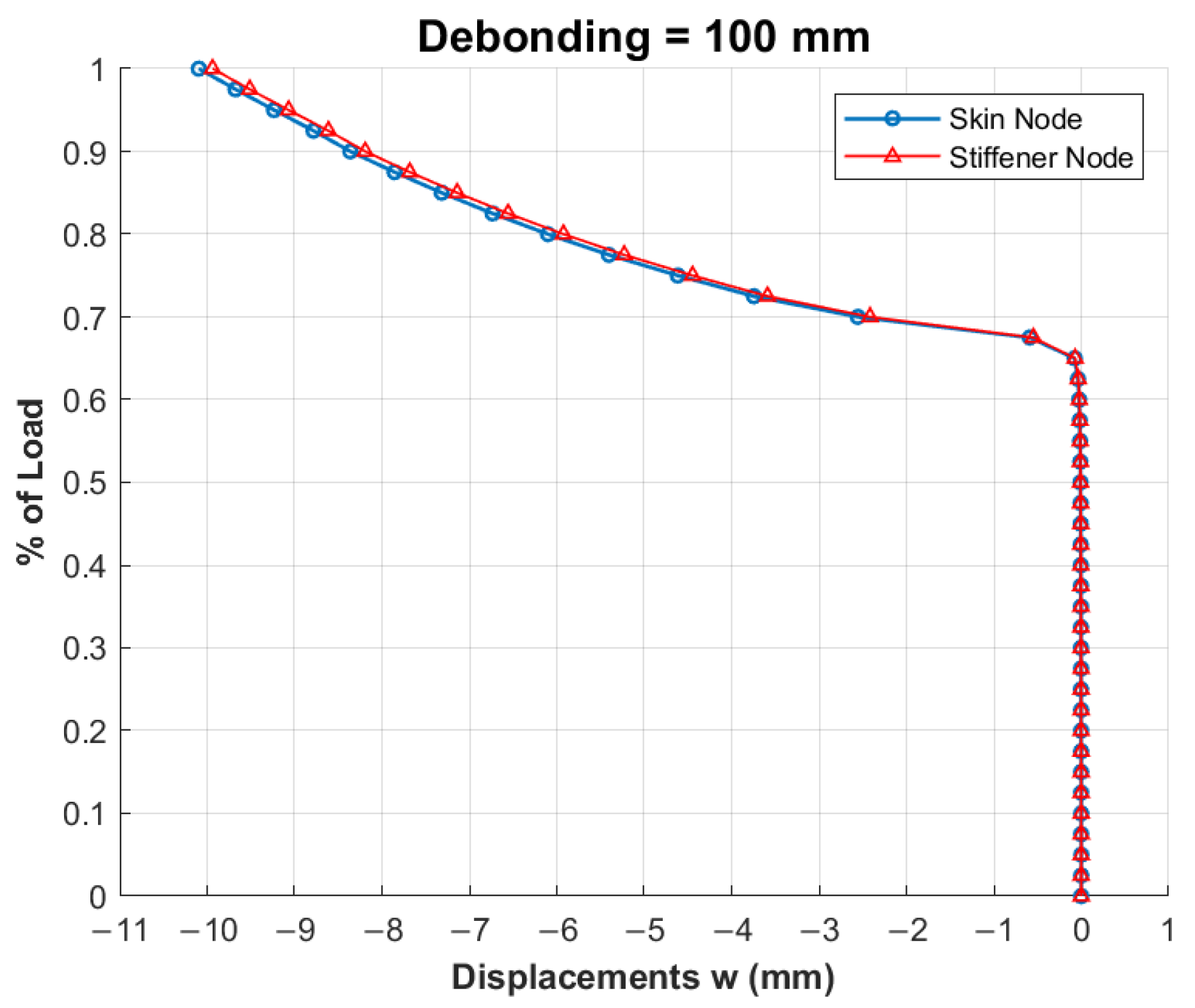
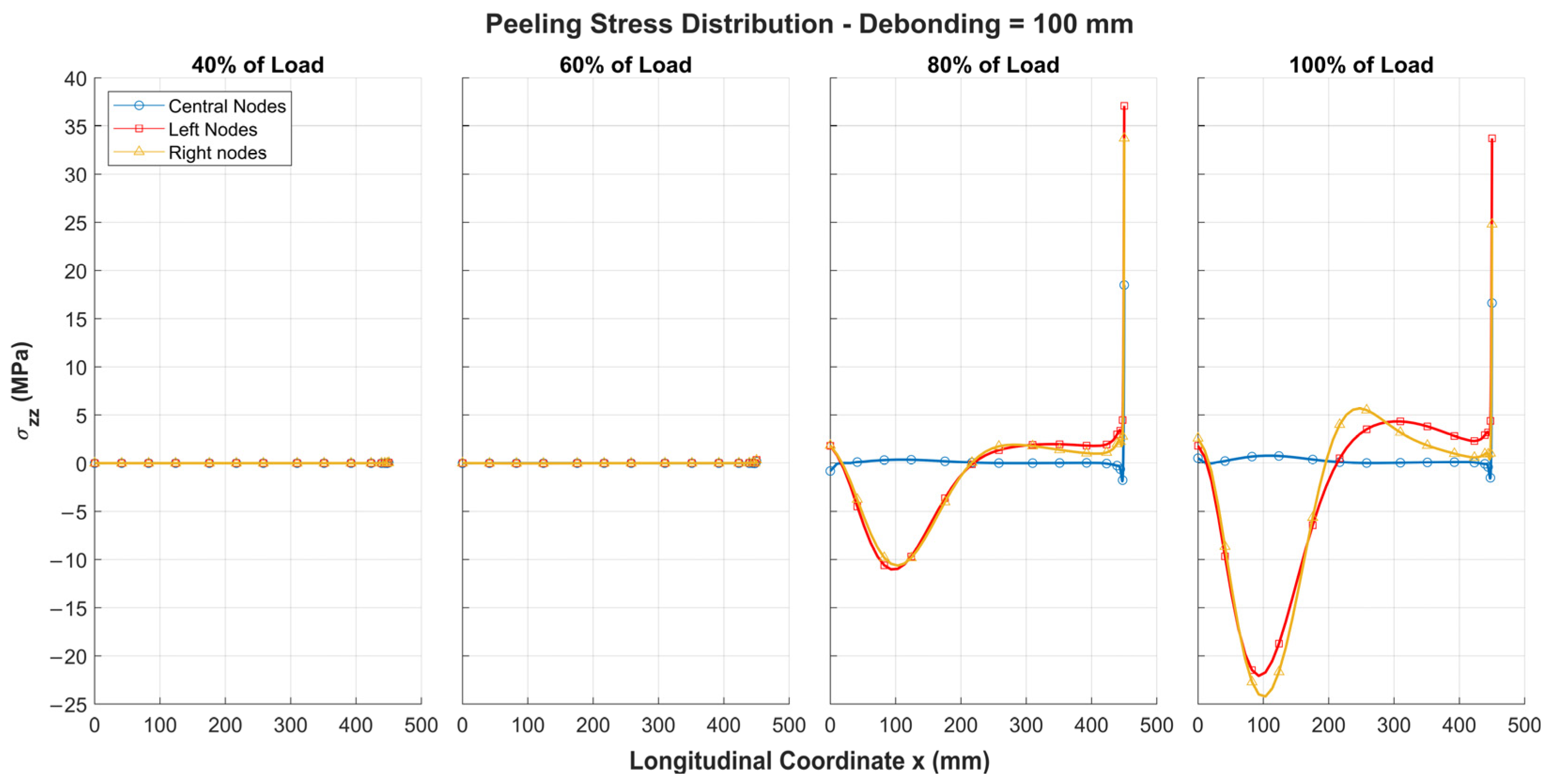
3.2.1. Configuration with 100 mm Debonding
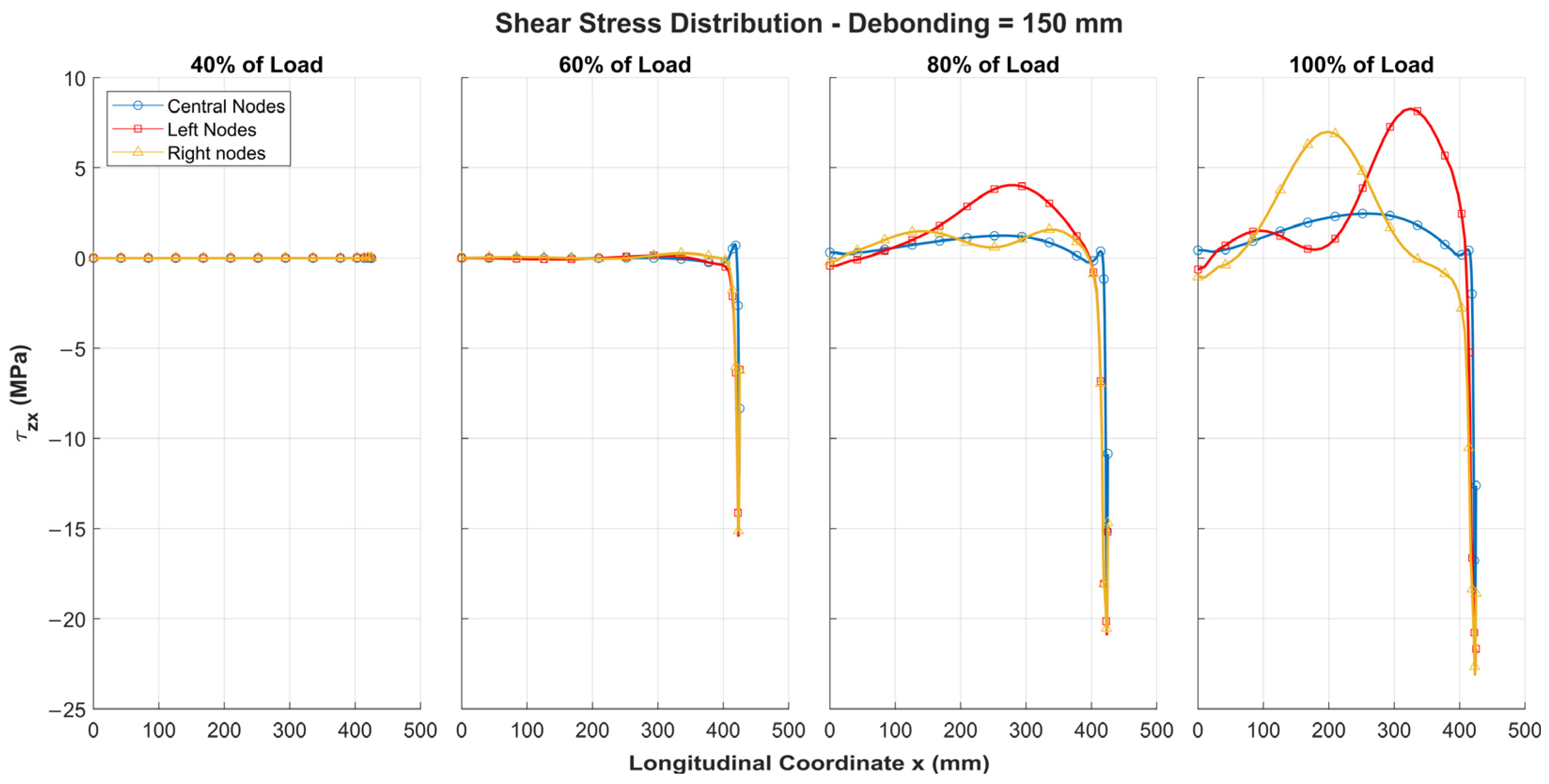
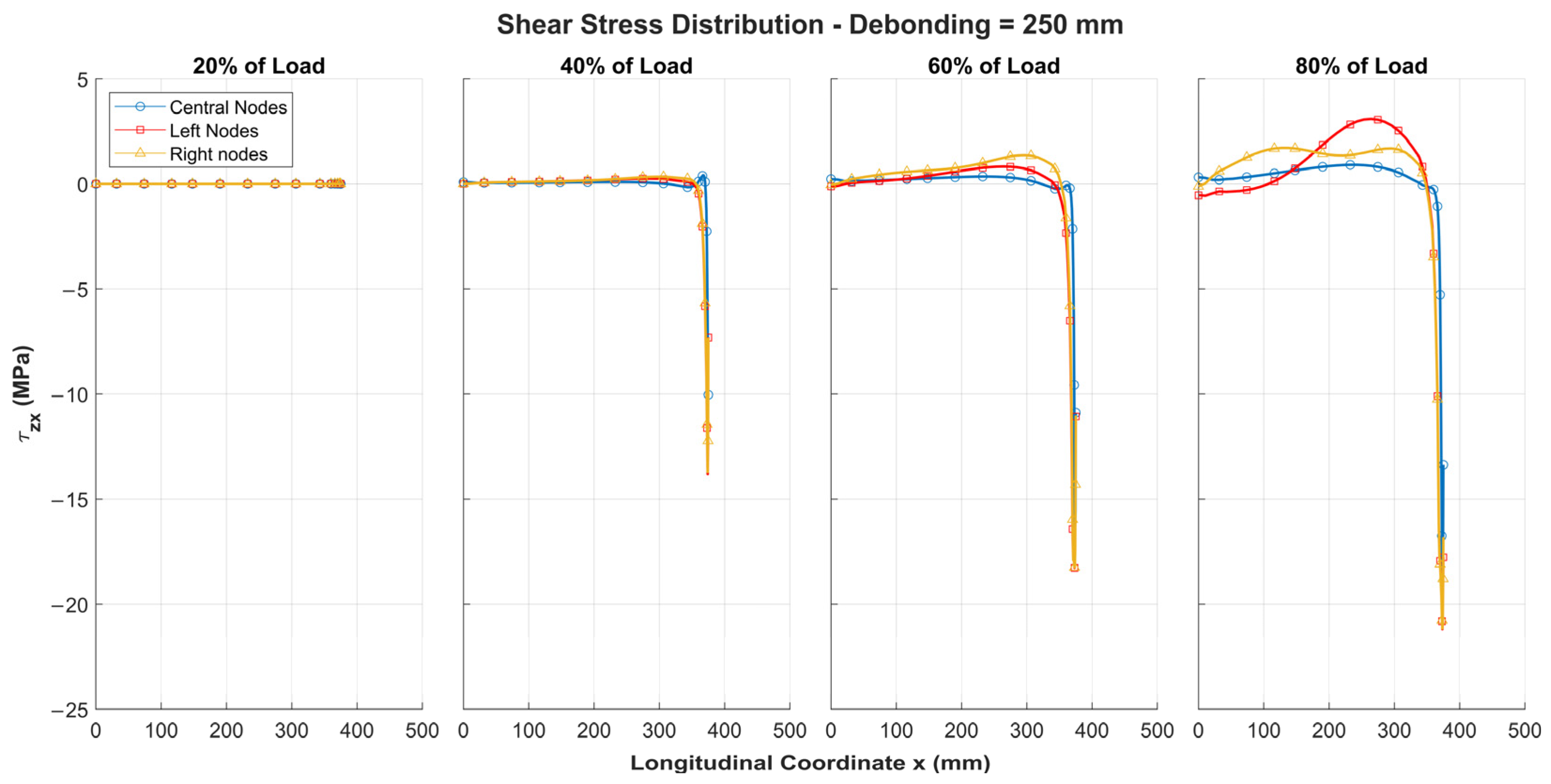
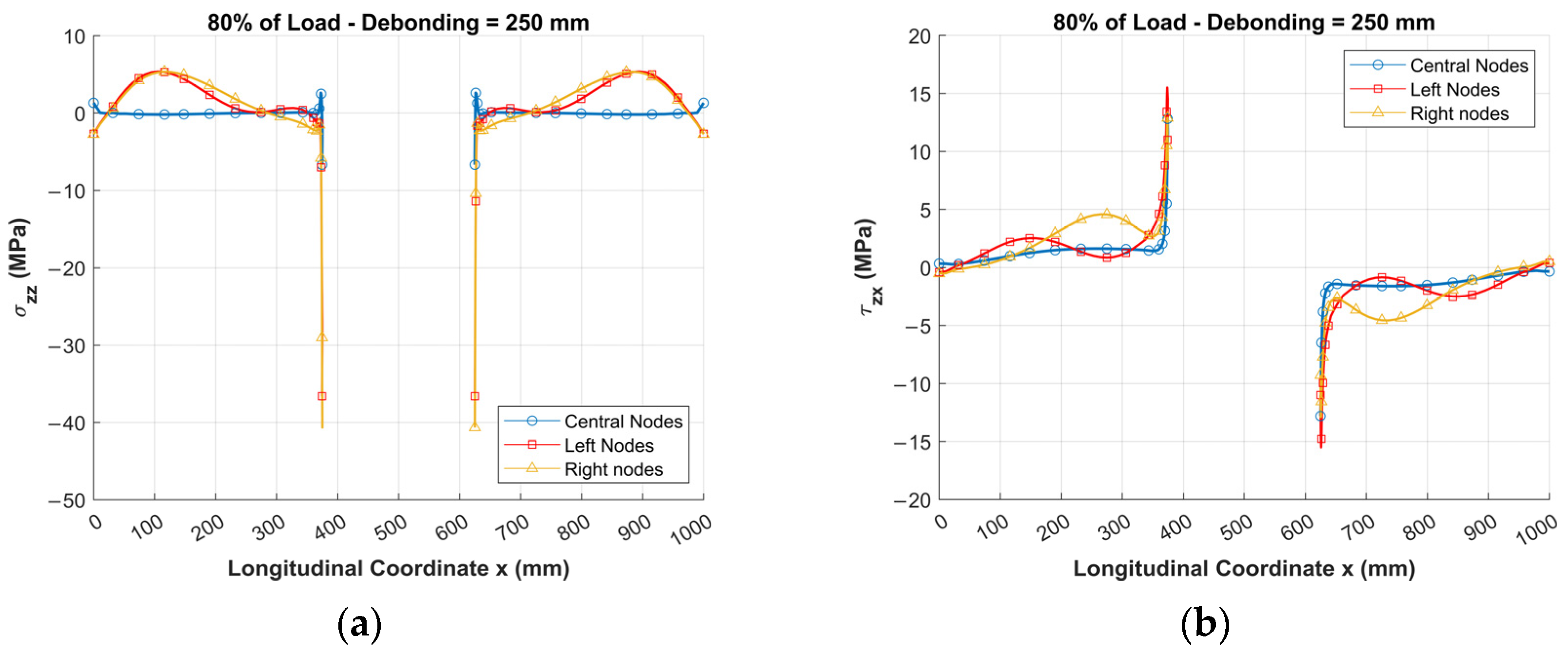
- Central nodes: Positioned in the central area of the adhesive layer;
- Left nodes: Oriented toward the centre of the structure;
- Right nodes: Oriented toward the edge of the panel.
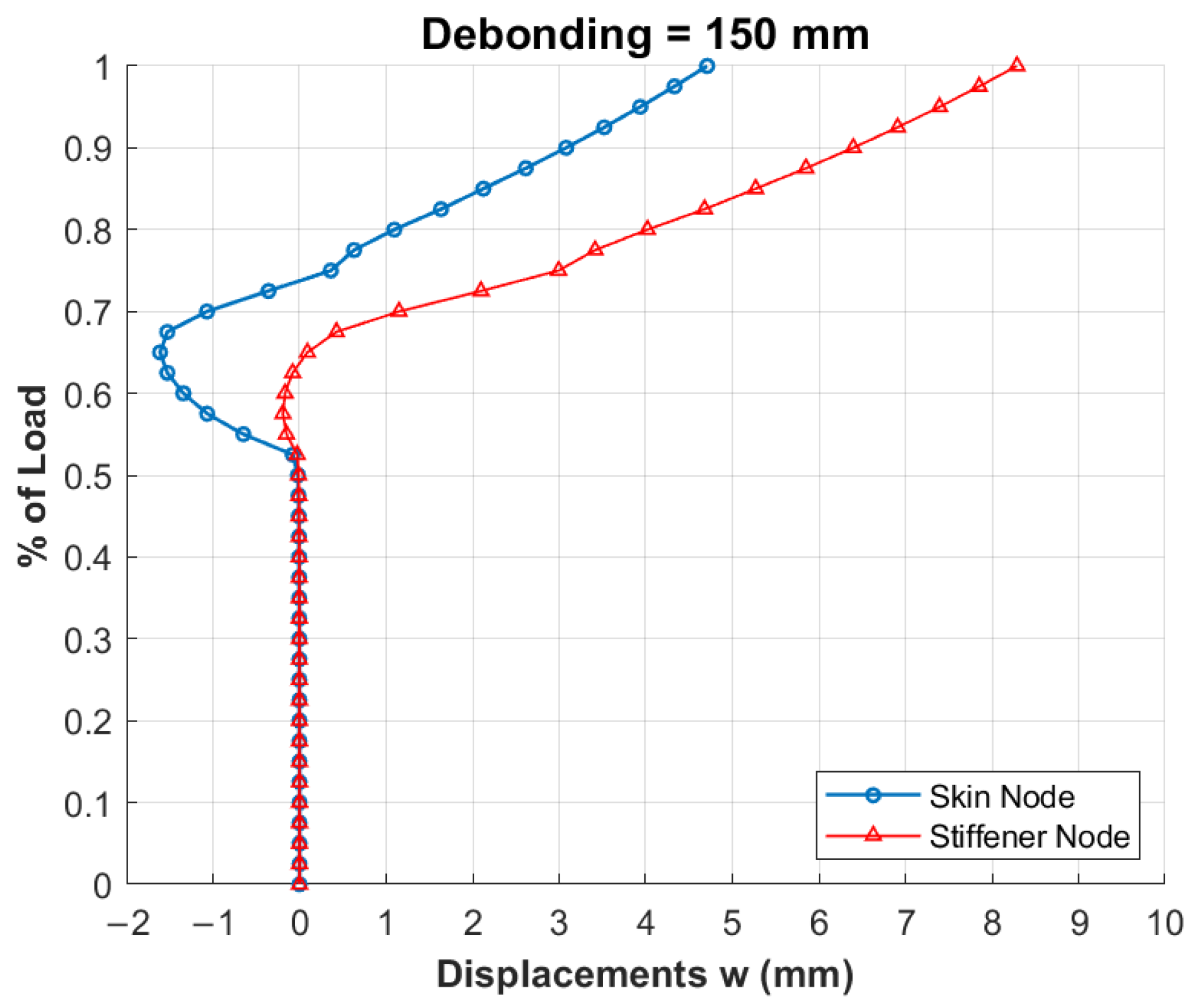
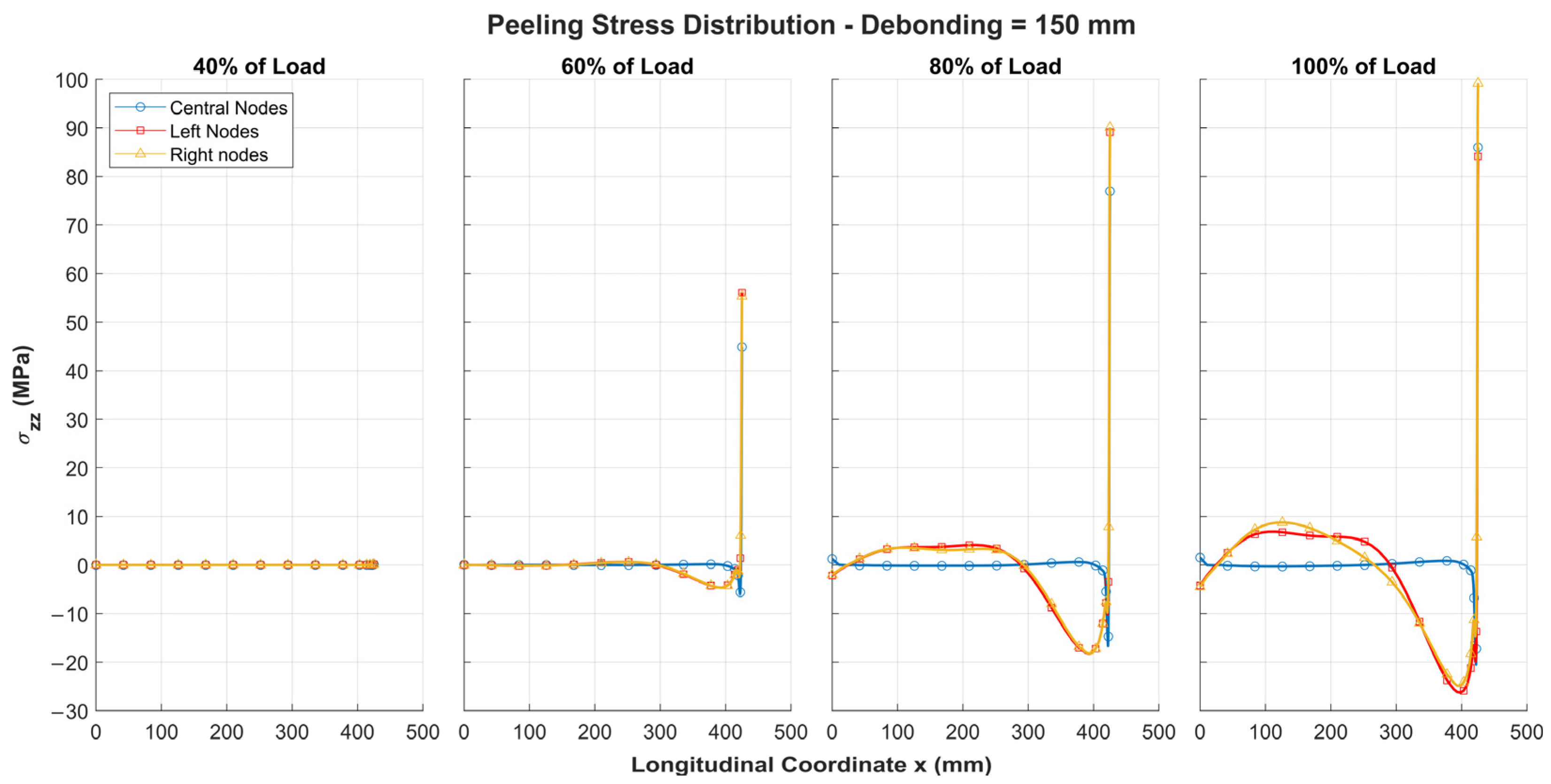
3.2.2. Configuration with 150 mm Debonding
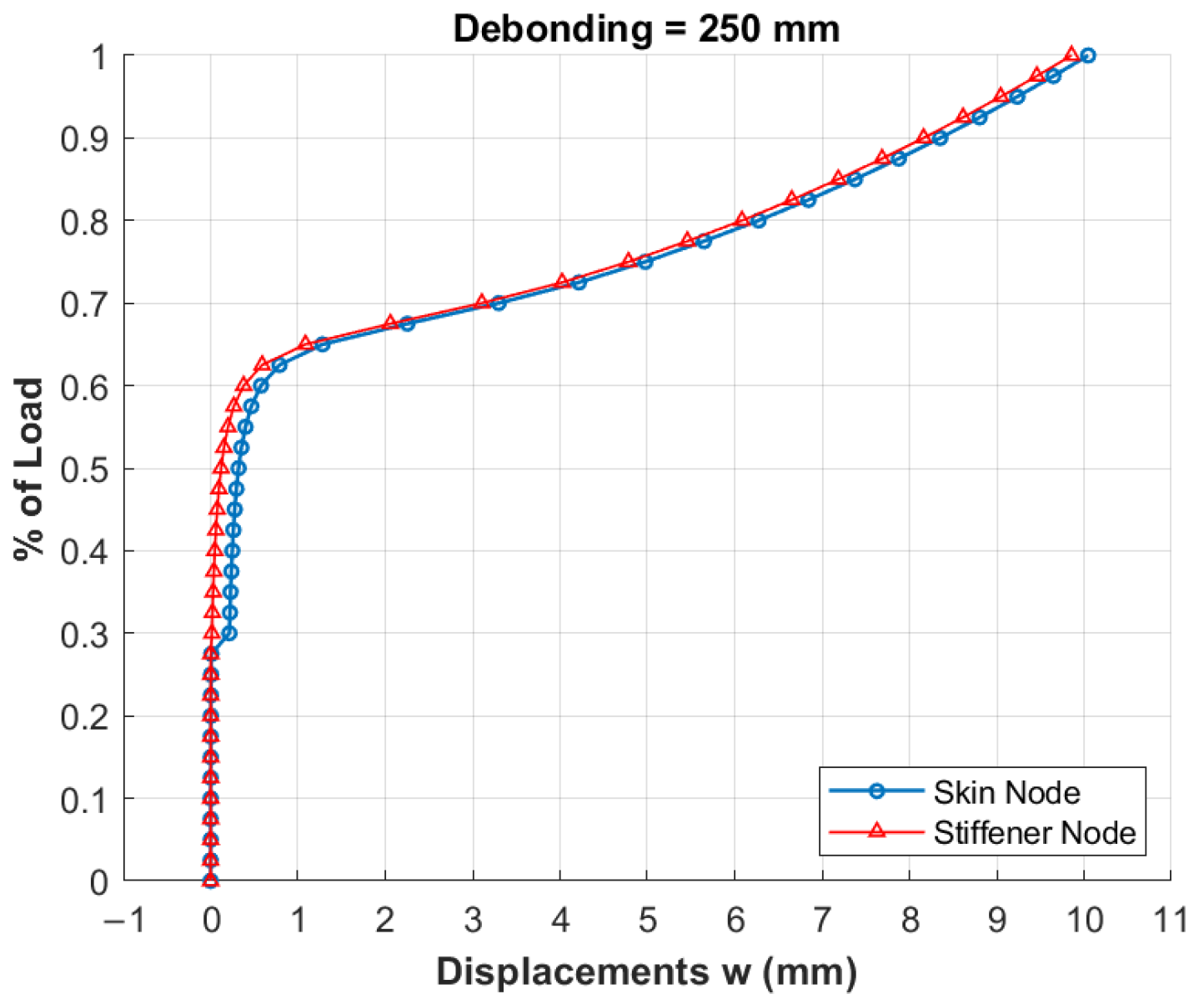
3.2.3. Configuration with 250 mm Debonding
3.3. Stress State for Different Mechanical Properties of Adhesive
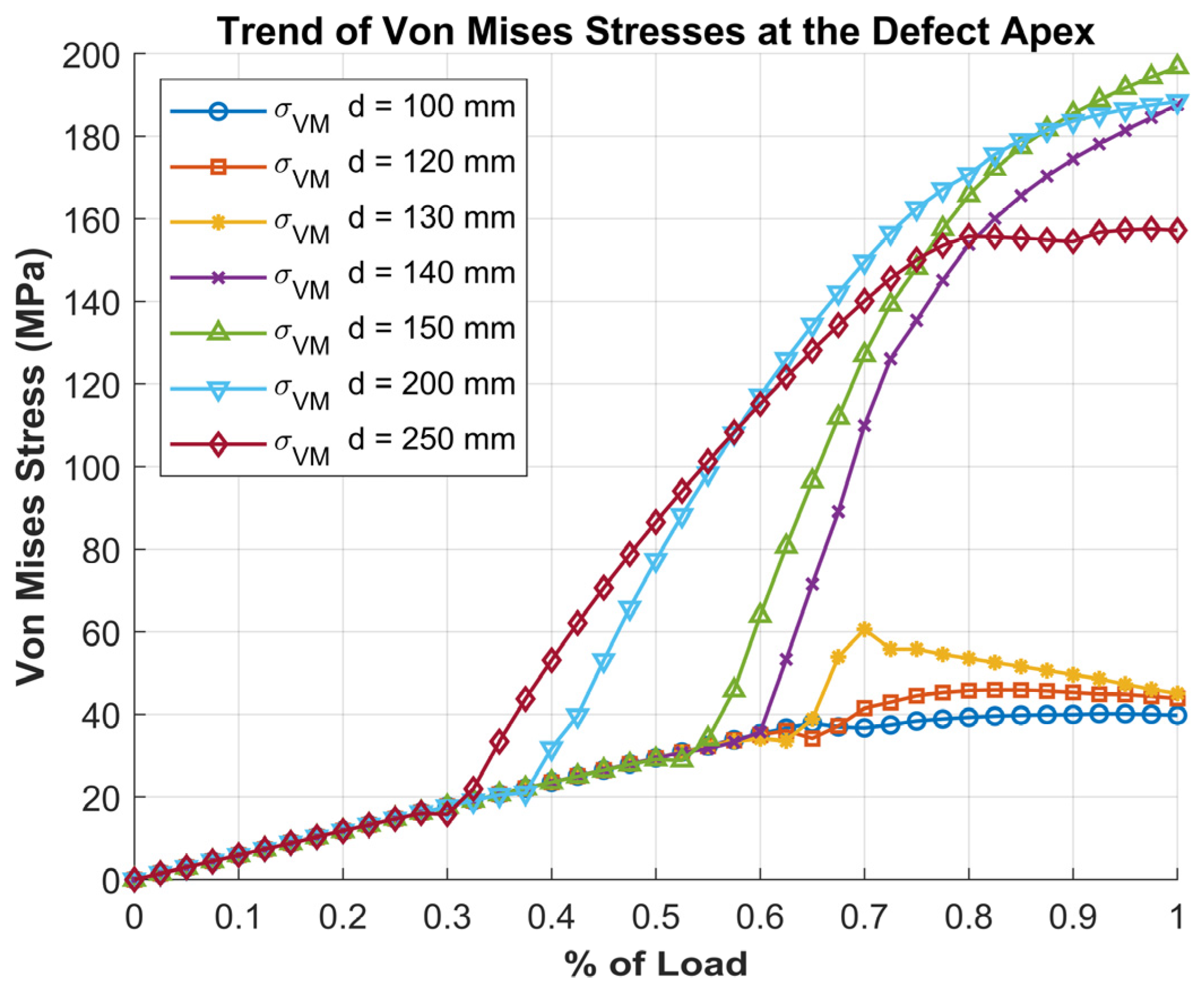
3.4. Von Mises Stress
3.5. Influence of Direction of Perturbation Application
4. Discussion
- (a)
- The defect length significantly influences both global and local buckling modes, which may interact at specific damage dimensions once the defect extends beyond certain thresholds.
- (b)
- Under predominantly global buckling, shear and peel stresses remain relatively low. However, if local buckling occurs, these stresses increase significantly due to greater localized deflection in the damaged area.
- (c)
- In the post-buckling regime, maximum stress values can rise considerably, increasing the likelihood of brittle adhesive fracture just beyond the critical load. A specific debonding length emerges in this context, representing a critical point where local buckling drives a dangerous stress increase in the adhesive interface. Moreover, these maximum stress values are directly proportional to the debonding length, as the local buckling load decreases with larger defect dimensions.
- (d)
- The same trend is observed across various adhesive properties, indicating a robust and general behaviour. However, the direction of the perturbation can affect the buckling shapes and stress distribution, potentially reducing or even preventing the onset of critical structural conditions.
- (e)
- The stress peaks can be directly connected to the load imbalance between the post-buckled, damaged region and the rest of the structure, highlighting the importance of identifying a critical configuration during preliminary design.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bisagni, C.; Frulla, G.; Giavotto, V.; Romeo, G.; Fanteria, D.; Lanciotti, A. Research Activities on Buckling of Composite Structures in Italy. In Proceedings of the 50th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference (AIAA 2009-2349), Palm Springs, CA, USA, 17 April–7 May 2009. [Google Scholar] [CrossRef]
- Frulla, G. Static/Fatigue Structural Behaviour of Damaged Stiffened Composite Plates for UAS Applications. SAE 2013 AeroTech Congress & Exibition, 2013. [Google Scholar] [CrossRef]
- Junior, M.C.B.P.; Pinto, V.T.; Rocha, L.A.O.; Dos Santos, E.D.; Isoldi, L.A. Analysis through constructal design of the influence of spacing between stiffeners in the deflection of plates. Rev. Mundi Eng. Tecnol. Gestão. 2020, 5, 275-01–275-21. [Google Scholar] [CrossRef]
- Wang, X.; Xie, F. Tensile strength and failure behavior of T-stiffened panels with embedded delamination: Experimental investigation. Iran. Polym. J. 2021, 30, 897–905. [Google Scholar] [CrossRef]
- Frulla, G. Structural behavior of Damaged Anisotropic Stiffened Panels under compressive loads. In Proceedings of the 25th ICAS Congress, Hamburg, Germany, 3–8 September 2006; Springer: Berlin/Heidelberg, Germany; ISBN 0953399176.
- Torres, M.; Franco-Urquiza, E.A.; Hernández-Moreno, H.; González-Villa, M.A. Mechanical Behavior of a Fuselage Stiffened Carbon-Epoxy Panel under Debonding Load. J. Aeronaut. Aerosp. Eng. 2018, 7, 1000280. [Google Scholar] [CrossRef]
- Armanios, E.A. Delamination analysis for laminated composites. I: Fundamentals. J. Aerosp. Eng. 1991, 4, 194–215. [Google Scholar] [CrossRef]
- Yap, J.W.; Scott, M.L.; Thomson, R.S.; Hachenberg, D. The analysis of skin-to-stiffener debonding in composite aerospace structures. Compos. Struct. 2002, 57, 425–435. [Google Scholar] [CrossRef]
- Yap, J.W.; Thomson, R.S.; Scott, M.L.; Hachenberg, D. Influence of post-buckling behaviour of composite stiffened panels on the damage criticality. Compos. Struct. 2004, 66, 197–206. [Google Scholar] [CrossRef]
- Ye, Y.; Zhu, W.; Jiang, J.; Xu, Q.; Ke, Y. Computational modelling of postbuckling behavior of composite T-stiffened panels with different bonding methods. Compos. Part B. 2019, 166, 247–256. [Google Scholar] [CrossRef]
- Coudor, P.; Van der Veen, S.; Beakou, A. Global-Local Analysis of Bonded Skin-to-Stiffener Joints in Post-Buckled Panels. In Proceedings of the 25th ICAS Congress, Hamburg, Germany, 3–8 September 2006; Springer: Berlin/Heidelberg, Germany; ISBN 0953399176.
- Greenhalgh, E.; Meeks, C.; Clarke, A.; Thatcher, J. The effect of defects on the performance of post-buckled CFRP stringer-stiffened panels. Compos. Part A. 2003, 34, 623–633. [Google Scholar] [CrossRef]
- Anyfantis, K.N.; Tsouvalis, N.G. Post Buckling Progressive Failure Analysis of Composite Laminated Stiffened Panels. Appl. Compos. Mater. 2012, 19, 219–236. [Google Scholar] [CrossRef]
- Zarouchas, D.S.; Alderliesten, R.C. The effect of disbonds on stability aspects of adhesively bonded aluminum panels during compression loading. Thin-Walled Struct. 2015, 96, 372–382. [Google Scholar] [CrossRef]
- Ji, R.; Zhao, L.; Wang, K.; Liu, F.; Gong, Y.; Zhang, J. Effects of debonding defects on the postbuckling and failure behaviors of composite stiffened panel under uniaxial compression. Compos. Struct. 2021, 256, 113–121. [Google Scholar] [CrossRef]
- Ausili, F. Critical Load of A Stiffened Panel by FEM: Effect of the Presence of a Debonding and Analysis of the Tensional Status at the Apex of the Defect. Master’s Thesis, Politecnico di Torino, Turin, Italy, 5 April 2022. [Google Scholar]
- Parente, G. Critical Conditions and Stress State of a Stiffened Panel with Defect. Master’s Thesis, Politecnico di Torino, Turin, Italy, 29 October 2024. [Google Scholar]
- Frulla, G. Simplified procedure for damage-oriented evaluation of a stiffened panel with skin-stringer de-bonding in pre-liminary design stage. Aeronaut. Aerosp. Open Access J. (AAOAJ) 2021, 5, 95–101. [Google Scholar] [CrossRef]
- Hexagon, A.B. MSC Patran 2023.1 User’s Guide; Hexagon AB: Irvine, CA, USA, 2023. [Google Scholar]
- Hexagon, A.B. MSC Nastran 2023.1. Quick Reference Guide; Hexagon AB: Irvine, CA, USA, 2023. [Google Scholar]
- Timoshenko, S.P.; Gere, J.M. Theory of Elastic Stability; McGraw-Hill: New York, NY, USA, 1961; ISBN 978-0-486-47207-2. [Google Scholar]
- Singer, J.; Arbocz, J.; Weller, T. Buckling Experiments: Experimental Methods in Buckling of Thin-Walled Structures: Basic Concepts, Columns, Beams and Plates; John Wiley & Sons: Hoboken, NJ, USA, 2000; ISBN 978-0471956617. [Google Scholar]
- Bazant, Z.P.; Cedolin, L. Stability of Structures: Elastic, Inelastic, Fracture, and Damage Theories; World Scientific: Singapore, 2010; ISBN 978-9814317023. [Google Scholar]
- Ibeabuchi, V.T.; Ibearugbulem, M.O.; Njoku, K.O.; Ihemegbulem, E.O.; Okorie, P.O. A Contribution to Analytical Solutions for Buckling Analysis of Axially Compressed Rectangular Stiffened Panels. Rev. Compos. Matér. Av. 2021, 31, 301–306. [Google Scholar] [CrossRef]
- ESDU 80023; Buckling of Rectangular Specially Orthotropic Plates. Engineering Sciences Data Unit: London, UK, 2000; ISBN 978-0-85679-306-6.
- Zou, D.; Bisagni, C. Skin-Stiffener Separation in T-Stiffened Composite Specimens in Postbuckling Condition. J. Aerosp. Eng. 2018, 31, 04018027. [Google Scholar] [CrossRef]
- Orifici, A.C.; Lauterbach, S.; Abramovich, H.; Thomson, R.S.; Wagner, W.; Balzani, C. Analysis of damage sensitivity and collapse in postbuckling fibre-reinforced multi-stiffener panels. In Proceedings of the 2nd International Conference on Buckling and Postbuckling Behaviour of Composite Laminated Shell Structures, Braunschweig, Germany, 3–5 September 2008. [Google Scholar]
- Degenhardt, R.; Rolfes, R.; Zimmermann, R.; Rohwer, K. COCOMAT—Improved material exploitation of composite airframe structures by accurate simulation of postbuckling and collapse. Compos. Struct. 2006, 73, 175–178. [Google Scholar] [CrossRef]
- Van Rijn, J.C.F.N.; Wiggenraad, J.F.M. A Seven-Point Bending Test to Determine the Strength of the Skin-Stiffener Interface in Composite Aircraft Panels; Technical Publication NLR-TP-2000-044; National Aerospace Laboratory NLR: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Bonanni, R.; Abrate, S. The behavior of damage-tolerant hat-stiffened composite panels loaded in uniaxial compression. Compos. Part A Appl. Sci. Manuf. 2001, 32, 1255–1262. [Google Scholar] [CrossRef]
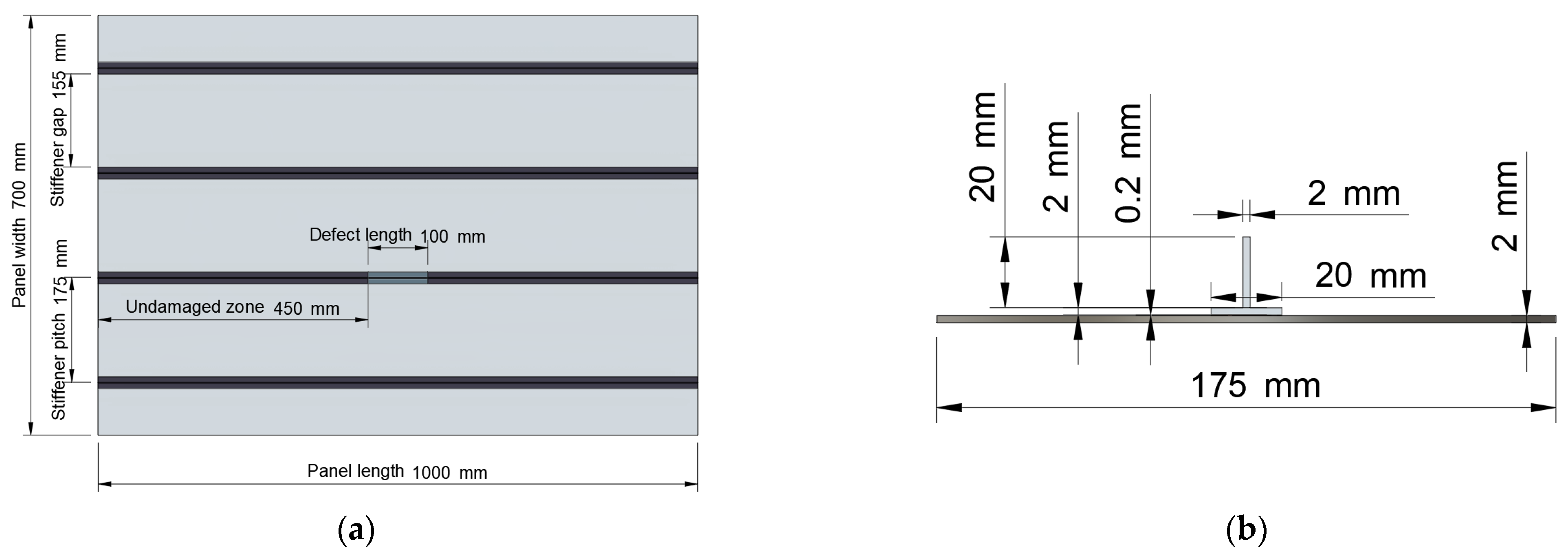






| Component | Material | E (MPa) | Poisson’s Ratio | Density (kg/m3) | Stress at Yield (MPa) | Hardening Slope (MPa) |
|---|---|---|---|---|---|---|
| Plate/Stiffeners | Al Alloy | 73,000 | 0.33 | 2700 | - | - |
| Adhesive | Epoxy Resin | 2750 | 0.35 | 1140 | 30 | 275 |
| Type of Stress | % of Load | HS 1 = 275 MPa (MPa) | HS 1 = 85 MPa (MPa) |
|---|---|---|---|
| 60% | 49.86 | 47.19 | |
| 80% | 60.56 | 54.75 | |
| 60% | −10.89 | −8.67 | |
| 80% | −13.37 | −9.18 | |
| Von Mises stress | 60% | 38.12 | 34.17 |
| 80% | 43.70 | 36.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frulla, G.; Parente, G. Design Considerations for a Compressed Stiffened Plate with Skin–Stringer Debonding Based on the Evaluation of Adhesive Layer Stress Distribution. Appl. Mech. 2025, 6, 7. https://doi.org/10.3390/applmech6010007
Frulla G, Parente G. Design Considerations for a Compressed Stiffened Plate with Skin–Stringer Debonding Based on the Evaluation of Adhesive Layer Stress Distribution. Applied Mechanics. 2025; 6(1):7. https://doi.org/10.3390/applmech6010007
Chicago/Turabian StyleFrulla, Giacomo, and Giovanni Parente. 2025. "Design Considerations for a Compressed Stiffened Plate with Skin–Stringer Debonding Based on the Evaluation of Adhesive Layer Stress Distribution" Applied Mechanics 6, no. 1: 7. https://doi.org/10.3390/applmech6010007
APA StyleFrulla, G., & Parente, G. (2025). Design Considerations for a Compressed Stiffened Plate with Skin–Stringer Debonding Based on the Evaluation of Adhesive Layer Stress Distribution. Applied Mechanics, 6(1), 7. https://doi.org/10.3390/applmech6010007





