Abstract
In this work, an analytical solution for the natural frequencies of elastically supported stepped beams with rigid segments is presented. The elastic end boundary conditions are modeled with a translational stiffness element, a rotational stiffness element, and an end-concentrated mass. This model is of great significance in machine construction studies. Under the assumption of Euler–Bernoulli beam theory, the non-dimensional equations of the motion and main equations that can give all of the boundary conditions were obtained by using Hamilton’s principle. After deriving the transverse displacement functions by means of using the separation-of-variables technique, the frequency equation was found by setting the determinant of the coefficient matrix to zero. The natural frequencies of the transverse vibrations were found according to physical and geometric parameters. The method was validated by using FEM results and findings from the literature. This study indicates that the physical and geometric parameters of the elastic supports and rigid segments affect the natural frequencies of the beam. The revealed analytical method can be used to calculate the natural frequencies and mode shapes of all beam types, such as elastically supported uniform beams and single-step beams with or without concentrated mass and/or rigid segments.
1. Introduction
The elements frequently used in engineering applications are called “typical elements”. Stepped beams, stepped shafts, and stepped pipes, some segments of which must be rigid because of the components on them, are typical elements that are used in structures, machines, and mechanical systems. The investigation of these beams in terms of free vibrations is of significance in machine construction studies.
Examples of stepped continuum applications include electric motor rotors, shafts with gears or pulleys, beam and pulleys with bearings in cable machinery, one-piece stepped shafts, long-stepped shafts in textile machinery, and hammer stone crusher rotors. Piping used to transport fluids with a rigid connecting segment in the middle region is an example of a stepped pipe application. Beams with rigid segment at the end or in the middle region are examples of stepped continuum applications with rigid segments.
A structure to which the beam with a rigid segment is attached may bend elastically under working conditions. Therefore, it is necessary to model the ends as an element with translational stiffness, an element with rotational stiffness, and a concentrated mass.
In practical applications, there are generally assembly parts that need to be modeled as concentrated mass. For example, the crane bridge with the concentrated mass at the ends is elastically supported by elastic beams at both ends in overhead cranes. Thus, by taking into consideration concentrated masses, a more realistic model is developed. A similar situation is often observed in the context of shafts. Roller bearings, which are generally placed on an elastic beam by means of assembly parts, should be modeled as an elastic support and concentrated mass. The bearings, fixing rings, and shaft bearings mounted on the shafts also correspond to the end-concentrated masses.
Elastic segments sometimes have different values in terms of their cross-sectional area sizes and Young’s modulus. The nomenclature employed for similar structures includes terms such as “two-part beam mass system” [] and “stepped beam with rigid segment”. Natural frequency analyses of such structures with rigid segments are performed similarly to continuum analyses.
The natural frequencies for the transverse free vibrations of a single-step beam were found [,,,]. The natural frequencies of a single-step Bernoulli–Euler beam with a circular cross-section were calculated as an exact solution by Jang and Bert [] for six different support options. Özkaya et al. [] investigated the natural frequencies of the constant cross-section beam-concentrated mass system.
The natural frequencies for the stepped beams with rigid segments are presented in [,,,,]. The natural frequencies and mode shapes of the beam systems consisting of one or more rigid parts, where these rigid parts are elastically supported, were derived []. The natural frequencies and mode shapes are given for a beam system whose rigid and elastic parts can be joined at any angle []. The natural frequencies and mode shapes were found for Timoshenko and Euler–Bernoulli beams with single-step rigid segments in the middle regions under classical and elastic boundary conditions []. In order to find the mode shape and natural frequencies of the Timoshenko beam without a rigid segment and with an elastic part instead, a set of solution equations written with respect to the axis origin is obtained []. The natural frequencies of elastically supported, tapered Rayleigh beams with end-concentrated masses and one axial force were determined using a new numerical method [].
The natural frequencies of beams with a rigid segment with and without elastic support were calculated using the transfer matrix method for multi-body systems []. The full dynamic stiffness method was used for the free vibration analysis of the system consisting of more than one rod and more than one rigid segment []. Here, the rods may be connected to the rigid bodies at any angle and from any position. For the free vibrations of Euler–Bernoulli and Timoshenko beams supported in a discrete manner by arbitrary numbers of intermediate elastic supports, the eigenvalue relations and mode shapes were derived [].
Elastic segments, rigid segments, point masses, and the ground can be connected to each other by elastic or rigid point-type connections to form a non-planar beam system []. An approach for the vibration analysis of three-dimensional motion has been formulated. A method has been developed for the calculation of natural frequencies and mode shapes by using a series of orthogonal transformations. Natural frequencies and mode shapes are given for two examples. The dimensionless general case solution form has been formulated by Tekin and Özkaya [] for the free vibrations of multi-step beams with built-in supports at both ends. Frequency equations giving exact solutions for three different types of single-step beams are presented. In the study, the first three natural frequency values were found for three different rectangular prismatic cross-section types of beams with one, two, and three steps.
The exact frequency equation of the thin beam with unequal masses at both ends was studied under the assumption of harmonic vibration []. An approximate formula for the fundamental frequency was derived using the Fredholm integral equation approach [].
The effect of the mass moment of inertia of the end masses on the natural frequency of the transverse vibration of the free thin beam with two unequal end masses was investigated theoretically and experimentally []. The effects of the misalignment and the mass ratios between the connection point and the center of mass on the first five natural frequencies were studied. The first three eigenfrequencies of the simply supported uniform beam with elastic boundary conditions and a concentrated mass were calculated using the Laplace transform method []. The eigenfrequencies of the simply supported constant cross-section beam with a concentrated mass and on an elastic foundation were obtained []. A solution for the beam’s natural frequencies was developed using the transfer matrix method for a beam with multiple supports, multiple concentrated masses, and multiple elastic supports []. An optimization method for the natural frequencies of the elastically supported beam with a concentrated mass is presented []. The first three natural frequencies of a simply supported beam with a variable and T-shaped cross-section with rotational elastic elements were determined using the Rayleigh–Ritz method []. The first three natural frequencies of a uniform beam placed on five translational and rotational elastic supports were obtained []. The natural frequencies were calculated for the beam to be three-step under the same conditions []. The natural frequencies of multi-span elastically supported Timoshenko beams with elastic intermediate supports and span junctions with elastic elements were derived analytically []. The pipe with a retaining clip in the middle without fluid was modeled by Dou, Ding, Mao, Feng, and Chen []. Another study shows that the elasticity coefficient of the foundation can be set to zero for a Rayleigh beam on an elastic Winkler foundation []. The results from the presented study can then be used for verification.
To the author’s knowledge, there is no analytical solution in the literature for the natural frequencies and mode shapes of the elastically supported rigid stepped beam with end-concentrated masses.
In the present work, an analytical solution for the natural frequencies and the corresponding mode shapes of the transverse vibrations of the elastically supported rigid stepped beam with end-concentrated masses are obtained. In summary, the derivation of an original solution form by using a single Cartesian coordinate system, the inclusion of concentrated masses in the modeling the end boundary conditions, the derivation of all equations with the Hamilton principle, and the presentation of natural frequency changes in a graphical form depending on the parameters for elastic boundary conditions are the original aspects of the research. In addition, the developed model and frequency equation can be used for the types of uniform, two-part, or single-step beams with or without concentrated masses or rigid segments. For example, for a cantilever beam, the natural frequencies can be evaluated without establishing another frequency equation, whether the rigid segment is at the end or in the middle region. By setting the length of the elastic segment on the right side of the rigid segment to zero, a clamped-free beam with a rigid segment at its end can be obtained.
2. Materials and Methods
A general equation was obtained using Hamilton’s principle to find an analytical solution for the natural frequency values of the slender beams with a rigid segment. From this general equation, the equations of motion and boundary conditions were derived.
2.1. Formulation of the Problem
Figure 1 illustrates the mechanical model of the two-step continuum (stepped beam, shaft, or pipe). The symbol is used in the figure to indicate each of the three different segments. While the other two segments are elastic, the segment denoted by is considered rigid.
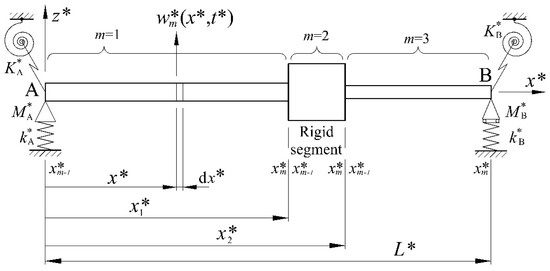
Figure 1.
Elastically supported stepped beam with a rigid segment.
In all figures and equations, the sign indicates dimensional quantities. Symbols that do not contain this sign are dimensionless. For example, the symbol is used for dimensional time. In the Cartesian coordinate system , shows the vertical coordinate. The coordinate in the axial direction is denoted as .
The distance between the endpoints is . The lateral displacement of the segment is . In Figure 1, the abscissa coordinates of the start and end of each segment are written as and next to the start and end lines of the steps. These variables, located at the two end points as and , take the value and , respectively. It is assumed that the support at the right end is also free to move axially.
In Figure 1, the ends were modeled using an element with translational stiffness, an element with rotational stiffness, and a concentrated mass. The symbols , , , and indicate the translational stiffness coefficient and the rotational stiffness coefficient for the end points A and B, respectively. The symbols and are used for the concentrated masses.
Hamilton’s principle was used in order to obtain the equations of motion of the beam and the boundary conditions. According to this principle, shown in Equation (1), the variation in the Lagrangian’s integral over time becomes zero [].
where the signs and mean the variation and the Lagrangian, respectively.
The difference in kinetic and potential energy, known as the Lagrangian, of the system is represented in the equation . Potential energy consists of strain energies and elastic potential energies.
Unlike the valuable studies carried out by Kopmaz and Telli [], Banerjee and Sobey [], Ilanko [], Naguleswaran [], and Dou, Ding, Mao, Feng, and Chen [], in this study, the equations of motion and the boundary conditions were obtained using Hamilton’s principle.
The limitations of the proposed model are as follows. This model is valid for beams with a uniform rigid segment, assuming that the center of mass of the rigid segment is located at its midpoint and also on its axis. This model is not valid for beams with more than one rigid segment. In addition, only beams supported at both ends are considered in this model. The mass moment of inertia of the concentrated masses located at the end points is also not considered.
By considering the motion and strain, shown in Figure 2, of an infinitesimal element of the beam, presented in Figure 1, the strain energy of the elastic segments of the beam were derived (Equations (2) and (3)). The sign , seen in Figure 2, represents the concentrated masses.
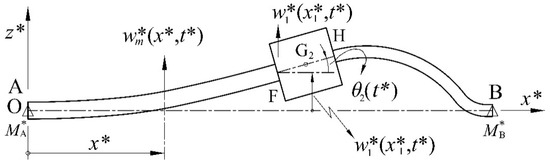
Figure 2.
The kinematic variables corresponding to the movement of the rigid segment.
The kinetic energy terms of the masses and the elastic potential energy terms of the elastic elements at the ends were added to the derived energy equation. In Figure 2, the angle of the rigid segment, , is shown. In order to write the kinetic energy expressions of the rigid segment, the kinematic terms in Figure 2 were used. In the figure, points F and H are the step junctions.
where with being the mass per unit length. The mass density and the cross-sectional area for each segment are written with corresponding subscript. and denote the mass of the rigid segment and the mass moment of inertia of the rigid segment about the point , which is the center of mass, respectively. The overdot and the prime signs indicate the partial derivatives with respect to the time and spatial coordinate , respectively.
where the symbols and are used for the Young’s modulus and the moment of the cross-section inertia in the axis of the elastic segments, respectively.
Hamilton’s principle was applied. For transverse vibration, the dimensional equations of motion, given as Equation (4), the continuity conditions as geometric and mechanic continuity at the junctions, and the main equations required to derive the end boundary conditions were found.
The geometric continuity in terms of deflection and slope due to the rigid segment must be ensured throughout the structure. Geometric continuity equations and the mechanic continuity condition equations can be obtained as follows:
By means of Hamilton’s principle, the equations from which any boundary condition equations can be derived as follows:
where indicates the variational operator.
2.1.1. Dimensionless Equations of Motion
In this study, as a result of the operations by means of the dimensional Lagrangian, the time non-dimensionalization [] and other non-dimensionalization parameters were found and are given as follows:
The ratios of masses per unit length and the bending rigidities can be written as follows:
Also, these symbols defined for short representations are used in dimensionless operations. By using non-dimensionalization parameters, the free vibration dimensionless equations of motion can be expressed as follows:
In these equations, the overdot sign is used to indicate the partial derivative with respect to the time variable . The prime sign indicates the partial derivative with respect to the non-dimensional spatial variable
2.1.2. Separation of Variables and Resulting Equations
As a result of operations performed, the separation of variables was achieved. The equations of motions and their solutions are given as follows:
where the symbol defined by
First, the dimensionless continuity equations were derived by applying the non-dimensionalization process. The equations obtained by means of Equation (22) are written as follows:
The equations corresponding to the end boundary conditions of the problem are obtained using Equations (9)–(12). The end boundary condition equations derived by applying the separation of variables method are given by
These equations are used as follows: If a free boundary condition exists at an endpoint, then displacement and sloping are present. Therefore, in the relationships given in Equations (30)–(33), any other term that does not contain the displacement and slope terms must be zero. Therefore, in these relationships, the second parentheses without a variation sign must be set equal to zero. It can be seen that all of the terms in this study are inside the second parenthesis. The use of such a boundary condition would be useful in verification studies to confirm the correctness of the procedures.
2.2. Solution Method for Eigenvalues
The coefficient matrix for the beam shown in Figure 1 was derived by means of the boundary and continuity conditions and is given in Appendix A.
In order to have a non-triviality condition, the determinant of the matrix has to be zero. Thus, the frequency equation, which is a function of , was found.
A computer program in Mathematica 10 has been developed for the derivation of the frequency equation and eigenvalues.
The exact values of corresponding to each mode of the structure can be evaluated. First, the graph of the frequency equation was drawn. The frequency equation will intersect the horizontal axis at same number of points as the number of natural frequencies. These intersection points show the eigenvalues. The approximate values of the intersection points can be obtained from the graph. These rough values were used with the FindRoot[charEqn,{, }] command in Mathematica 10 to evaluate the exact values.
It is more appropriate to represent the dimensionless value as for each mode. Thus, for , Equation (34) can be written as follows:
The unit of the dimensionless natural frequency shown in Equation (35) is rad/1-unit time. Some of the numerical values given in tabular form are written in dimensional form by means of Equation (35).
2.3. Verifications
It is necessary to verify the correctness of the derived analytical formulas and the computer implementations. In this section, the tables show the agreement of the natural frequencies obtained in this study with the values obtained in the literature.
The geometric, physical, and boundary condition values have been chosen both because they are based on previous experimental studies and because they have been used in the literature.
In applications, the component on beams and pipes is modeled as a rigid segment or a concentrated mass. The models corresponding to these situations are shown in Figure 3a,b. These beams were used in the verification.
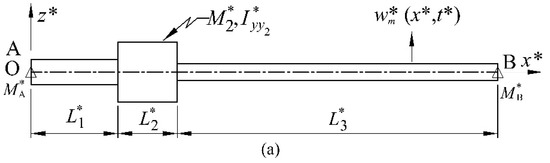

Figure 3.
The beam modeled as (a) a beam with a rigid segment; (b) a beam with concentrated mass.
The boundary conditions are not shown in Figure 3 in order to draw attention to the distances indicated with , , and . The dimensionless ones obtained from , , and have practical equivalents in applications, as they show the real distances.
2.3.1. Verification 1
The beam model corresponding to a two-step beam with a rigid segment has free boundary conditions at both ends [,]. The free boundary conditions can be obtained from the elastically supported model shown in Figure 1. By removing the terms of the support elements and the end-concentrated masses from Equations (30)–(33), free boundary condition equations are obtained.
The step lengths and the beam length of the circular cross-section beam system are given as , , , and [,]. The section diameters and physical properties were taken as , ,, , respectively. The dimensionless quantities were found to be , , , . The dimensional natural frequency was obtained from Equation (35) and formula, evaluated by means of converting it to Hz. The evaluated exact natural frequency values, the values obtained using FEM in the present work, and the results found in the referenced works [,] are showed in Hz in Table 1. The beam was modeled using the commercial software ANSYS Workbench 2024 R2. The Solid272 mesh element was used to mesh the model.

Table 1.
Values corresponding to the natural frequencies of the beam with a rigid segment under free boundary conditions.
The closeness of the present values in Table 1 and the mode shapes for the first three modes shown in Figure 4a–c confirms the validity of the work for the stepped beam under free boundary conditions. Red and blue indicate the regions of maximum and minimum displacement, respectively.
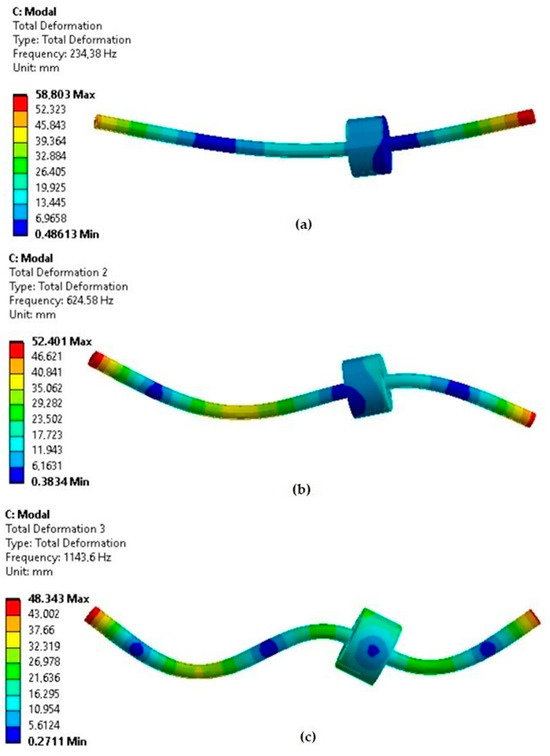
Figure 4.
The first (a), second (b), and third (c) mode shape of the stepped beam with a rigid segment under free boundary conditions.
2.3.2. Verification 2
In the case where there was a concentrated mass at both ends of the beam to be examined, a verification was performed. There was a free boundary condition at these ends []. The free boundary conditions were applied to the single-segment beam, given in Figure 3b, with the constant cross-section. Unlike the beam shown in Figure 3b, there was no concentrated mass in the middle regionof the beam []. The terms of the support elements were removed from Equations (30)–(33). Thus, free boundary condition equations were found for the end points.
The values corresponding to a completely constant cross-section beam were taken as be , , , . For the various values of the tip-concentrated masses and , the dimensionless values of the calculated eigenvalue parameter and the results found in the referenced work [] are presented in Table 2. The mass moment of inertia for the concentrated masses at the ends of the beam was assumed to be .

Table 2.
Values corresponding to the natural frequencies for the first five modes of the constant cross-section beam with concentrated mass at the ends with free boundary condition (,).
The values in Table 2 are identical. Thus, it is proven that the terms due to concentrated masses evaluated analytically are correct. This also shows that the terms have been used correctly in the written computer program.
2.3.3. Verification 3
If the concentrated mass at the end point of the beam supported, as shown in Figure 1, is assumed to be infinite, then this end point will not be able to move vertically. Therefore, a simple supported end case will occur for this end point []. This boundary condition has been created for the constant cross-section and one-piece beam given in Figure 3b. There is no concentrated mass in the middle region of the beam [].
The values corresponding to such a beam are taken as , , , , and . The dimensionless values of the calculated eigenvalue parameters and the results found in the referenced work [] are shown in Table 3 for various values of the tip-concentrated masses and . The infinitely large value of the concentrated mass has been used instead, [].

Table 3.
Numerical results corresponding to the natural frequencies of the constant cross-section beam with concentrated mass at the ends with free boundary condition (,).
The fact that the numerical values found in Table 3 for such an equivalent situation are the same confirms the accuracy of the analytical expressions and the written computer programs.
3. Results
3.1. Numerical Applications
In this section, the natural frequency values obtained using the frequency equation are listed in tables.
3.1.1. Case 1
It is assumed that there is a guided (vertically moving) end condition at both ends of the rigid stepped beam. The guided end of the beam is elastically connected to the ground. The values of the rotational stiffness coefficient are taken as to realize the guided end condition case. There are concentrated masses at the ends of the beam.
Dimensionless parameters have been chosen as , , , , , , , and . Here, was taken to examine the effect of only the mass. Dimensionless natural frequencies obtained for various values of the stiffness coefficients and have been presented in Table 4.

Table 4.
Natural frequencies for the first five modes of a guided-guided two-step beam supported by a spring element with translational stiffness and at both ends.
3.1.2. Case 2
For the beam with a rigid segment shown in Figure 1, the stiffness coefficients , and , of the elastic support elements have been taken as zero. Thus, free boundary conditions were created. Other parameters were chosen as , , , , , and . The natural frequency values corresponding to the first five modes have been obtained and are listed in Table 5 for the various values of the end-concentrated masses and .

Table 5.
Natural frequencies of a two-step beam with a rigid segment under free boundary conditions with concentrated mass at the ends.
3.1.3. Case 3
In this section, the natural frequencies and mode shapes of the elastically supported stepped beam with a rigid segment shown in Figure 1 have been presented.
In the calculations, the step lengths, beam length, section diameters, and other values of the circular cross-section beam system are designated as (), (), (), (), , ,, , and , respectively. Depending on these values, the dimensionless quantities have been found to be , , , and . The end-concentrated masses are designated as ().
The natural frequencies in Hz computed for the six values of the stiffness coefficients , , and , are given in Table 6. The first three in-plane mode shapes of the beam for the values in the third row of Table 6 are presented in Figure 5.

Table 6.
Natural frequencies in Hz for the first four modes of the two-step beam, which has the ends with elastic elements, with a rigid segment.
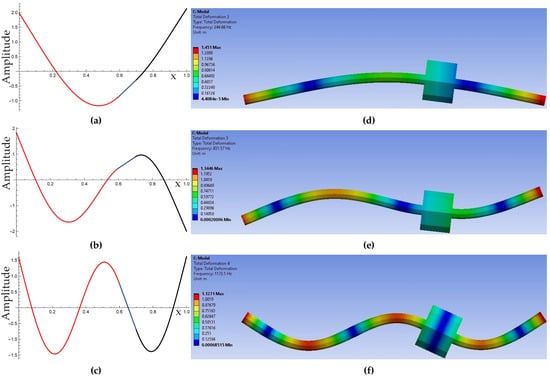
Figure 5.
The first, second, and third mode shapes obtained by the analytical method (a–c) and ANSYS (d–f) of the elastically supported stepped beam with a rigid segment. The blue line and the curves in red and black show the rigid and elastic segments in figures (a–c), respectively.
The shapes shown in Figure 5a–c represent the first three mode shapes found using the analytical solution. The eigenfunctions () are normalized so that . The shapes given in Figure 5d–f show the first three mode shapes found using ANSYS Workbench 2024 R2. The Beam188 is used to mesh the model. The first two in-plane rigid modes of this beam have not been considered for Table 6 and Figure 5. The blue straight lines and the others in Figure 5a–c represent the shape of the rigid segment and elastic segments, respectively, for each mode. The regions of maximum and minimum displacement are shown in red and blue, respectively.
3.2. Graphical Presentations and Discussions
The natural frequency values of the elastically supported stepped beam, shown in Figure 1, with a rigid segment are presented as graphs. Each figure consists of three separate graphs designated as a, b, and c. The purpose of presenting these graphs is to reveal the variations in the first three natural frequencies evaluated via the analytical method depending on their geometric and physical parameters. Explanations of the figures shown in the following sections are given in Table 7.

Table 7.
Explanations of the figures shown in the presentation sections.
3.2.1. Presentation 1
In Figure 6, the abscissa shows the distance of the mass center point , which is the midpoint of the rigid segment, from the origin. The parameters were chosen as , , , , , , and . Due to the assumption , the rotational kinetic energy of the rigid segment is neglected. The end-concentrated masses are assumed to be equal. For the values of the end-concentrated masses, 0, 0.001, 0.1, 0.2, 0.5, 1, 2, and 4 were used. Thus, the natural frequency curves were derived for the eight different cases.
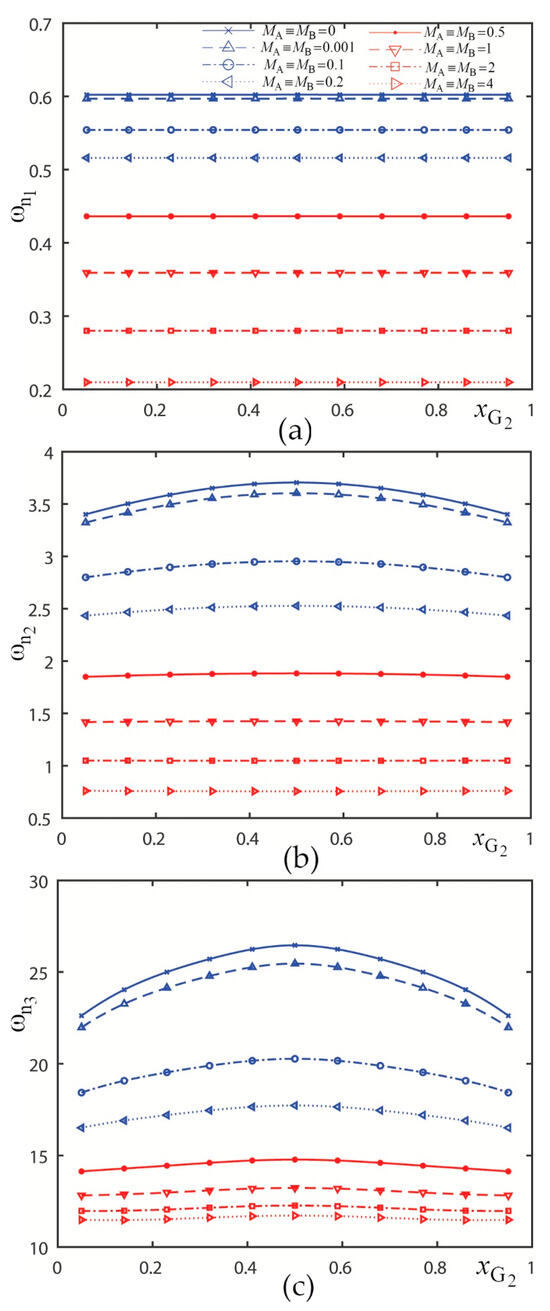
Figure 6.
The natural frequency values of the first (a), second (b), and third mode (c) versus the location of the mass center of the rigid segment.
There are two variable parameters in these plots: the value of the end-concentrated masses and the distance from the origin of the center of mass of the rigid segment. As the end-concentrated mass values increase, the natural frequency values decrease (Figure 6a–c) due to the increase in the kinetic energy value in the denominator of the Rayleigh’s Quotient []. The arrangement of the curves of the final concentrated mass values from the smallest to the largest proves this situation.
When the shape of the curves of the second and third natural frequencies is examined, some curves become an evident arc. It can be seen that the arc occurs in some curves and not in others. The arc occurs for small end-concentrated mass values. When the end-concentrated mass values are small, the strain energy portion of the total potential energy term in Rayleigh’s Quotient [] increases as the rigid segment approaches the middle. Accordingly, this situation occurs. The arc shape, especially for small values of the tip-concentrated masses, supports this fact. As the rigid segment approaches the edges, the natural frequency values decrease as the amount of shape change decreases.
It is observed that there is no arc in the natural frequency curve of the first mode. The total potential energy divided by the total reference kinetic energy is the Rayleigh’s Quotient. As the rigid segment approaches the middle section, the strain increases. The growing strain increases the potential energy. However, this increase is not large enough to create arcs for the first mode.
3.2.2. Presentation 2
The graphs shown in Figure 7 were created in order to investigate the effect of the end-concentrated masses, indicated by and , on the natural frequency values. In the graphs, the abscissa shows the values of the end-concentrated masses. The ordinate refers to the first three natural frequencies. The physical values of the beam were chosen as , , , , , , and . As mentioned above, the length of the left elastic segment was chosen to be , as given in []. Thus, there is no conflict with the node points of the first three modes. For the mass of the rigid segment indicated by , the values 0.01, 0.2, 0.5, 1, 2, 3, 4, and 5 were used. Separate curves were generated for each value. There are two variable parameters in the graphs: the value of the end-concentrated masses and the mass value of the rigid segment. Since the other parameters are constant, these three graphs show only the results of the increases in the end-concentrated mass values.
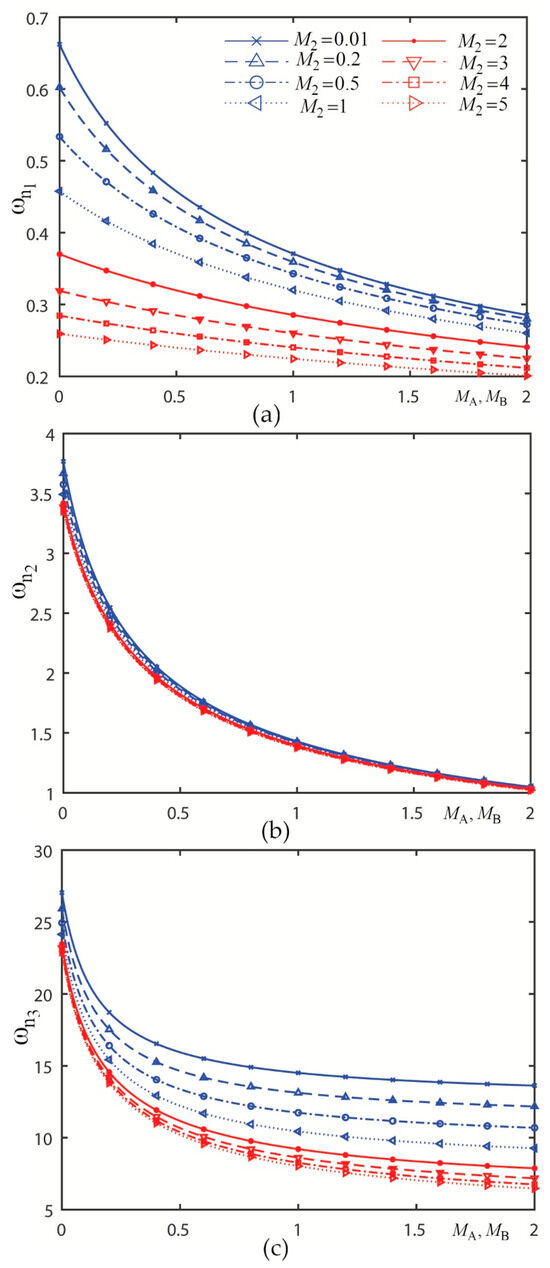
Figure 7.
The natural frequencies of the first (a), second (b), and third mode (c) versus the end masses and for the beam with ends elastically restrained against rotation and translation.
As the end-concentrated mass values shown on the abscissa increase, the natural frequency values decrease. This situation occurs due to the increase in kinetic energy specified in the Rayleigh’s Quotient. The change in the natural frequency curves of the third mode is shown in Figure 7c. As the value of the end-concentrated masses increases, the tendency to be small in the natural frequency values decreases. The increase in the potential energy values will decrease. As the value of the tip-concentrated masses increases, the increase in the kinetic energy value will decrease as the speed decreases. Thus, as the end-concentrated mass values increase, the reduction in natural frequencies will also decrease. This situation is most clearly seen in the change in the natural frequencies of the third mode. As the mass value of the rigid segment increases, the natural frequency values decrease. The reason for this is that the kinetic energy value in the denominator in the Rayleigh’s Quotient increases. The fact that the curves are listed one under the other, from small to large masses, proves this. Since the node point of the second mode is close to the rigid segment, the natural frequency curves of this mode are close to each other.
3.2.3. Presentation 3
The effect of the translational stiffness coefficient on the natural frequency is examined using the graphs shown in Figure 8. The value of the end-concentrated masses, indicated by the abscissa in the graphs, varies from 0 to 2. Depending on this value, the graph of the natural frequency values of the first three modes of the beam were plotted. In each graph, there are eight curves for the eight different values of the translational stiffness coefficient. The physical and geometric parameters of the beam were chosen as , , , , , and . was chosen to examine the effect of the mass only. Values of 0.01, 0.05, 0.1, 0.2, 0.5, 0.6, 0.8, and 1 were used as the coefficient of the element, with translational stiffness indicated by and .

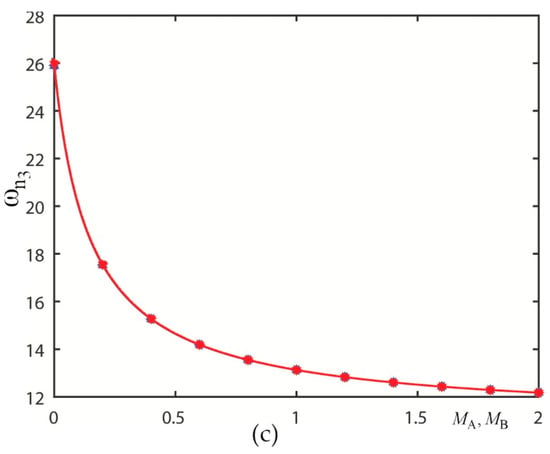
Figure 8.
The natural frequencies of the first (a), second (b), and third mode (c) versus the concentrated end-mass values and for the beam.
Figure 8 shows that, as the end-concentrated mass values increase, there is a decrease in the natural frequency values. This situation arises as a result of the increase in the kinetic energy value, which is the denominator term of the Rayleigh’s Quotient. It can be seen from Figure 8a,b that the natural frequency values grow as a result of the increase in the translational stiffness coefficients. This is caused by the growth of the potential energy value in the numerator of the Rayleigh’s Quotient. In Figure 8a,b, the curve showing the natural frequency value for the smallest translational stiffness coefficient is at the bottom. The curves with large stiffness coefficient values are located on this curve in order of size.
In Figure 8c, which shows the natural frequency values of the third mode, eight curves appear to overlap approximately. A conflict occurs when the values are completely close to each other. The numerical values that cause this situation were examined. It is shown in Table 8 that the values for the third natural frequency are close to each other.

Table 8.
The first three natural frequency values for the elastically supported beam with end-concentrated masses, .
The natural frequency values given in Table 8 were evaluated according to the stiffness coefficients of the eight separate curves seen in Figure 8c. The frequency values given in the first line of the table were taken as a reference value, and the differences were found as percentages according to the formula . As seen in the eighth row of Table 8, although the reference value stiffness coefficient increased by 100 times, the increase in frequency was 0.5%. It is seen that the increases in the first and second natural frequencies are 894.83 and 20.37%, respectively.
The fact that the values are close to each other can be partially seen in Figure 8b and the fourth column of Table 8, which show the natural frequencies of the second mode. The values presented in Table 8 demonstrate the effect of the stiffness coefficient of the translation stiffness element on the first three natural frequencies. The first natural frequency is most affected by the change in the value of the stiffness coefficient, while the third natural frequency is least affected.
When the natural frequency values of the third mode given in Table 8 are compared with each other, it is seen that the natural frequency values of the third mode are large for the greater values of the translational stiffness coefficient. Since it is a conservative system, it is understood that the changes in kinetic and potential energy are close to each other for small and values.
3.2.4. Presentation 4
The effect of the rotational stiffness coefficients on the first three natural frequencies is shown in the graphs given in Figure 9. The physical and geometric parameters of the beam, whose boundary conditions and shape are shown in Figure 1, were chosen as , , , , , , and . In the graphs, the horizontal axis indicates the value of the end-concentrated masses between 0 and 2. The coefficient of rotational stiffness is indicated by and , and the values 0.1, 0.2, 0.4, 0.6, 0.8, 1, 2, and 4 were used. As the end-concentrated mass values increase, the natural frequency values decrease.
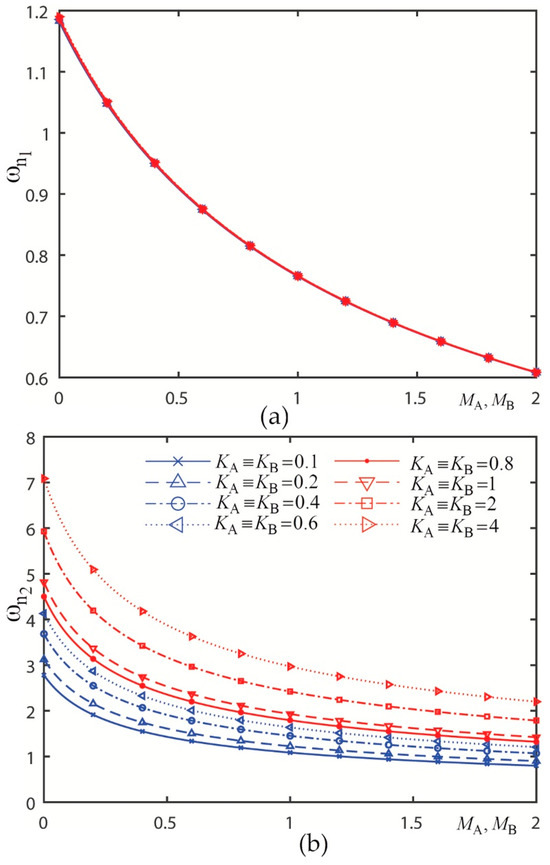
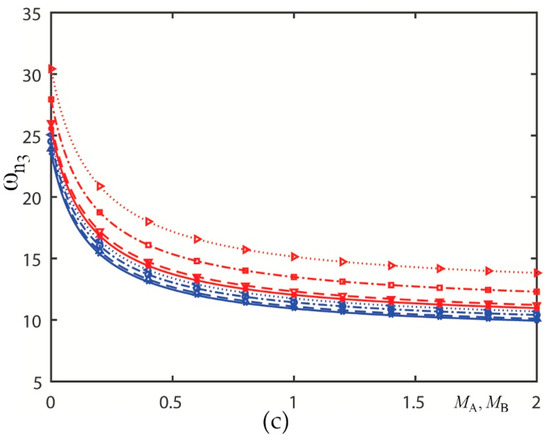
Figure 9.
The natural frequencies of the first (a), second (b), and third mode (c) versus concentrated end-mass values for the beam with ends elastically restrained against rotation and translation, and an end-concentrated mass, in this situation.
It can be seen in Figure 9b, c that there is an increase in the natural frequency values alongside the increase in rotational stiffness coefficients. This is caused by the increase in the elastic potential energy value.
In Figure 9a, which shows the variations in the natural frequencies of the first modes, the eight curves are approximately coincident. It is shown in Table 9 that the values for the first frequencies are close to each other. The same concentrated mass values were used in the calculations of all the values in Table 9. The natural frequency values given in Table 9 were calculated according to the stiffness coefficients of the eight separate curves seen in Figure 9a.

Table 9.
Natural frequencies for of the curves that appear to overlap in the graph given in Figure 9a which belong to the other two modes, but do not overlap.
As seen in Table 9, there is no significant change in the natural frequency values due to the increase in the rotational stiffness coefficient value. There is the potential energy term in the numerator of the Rayleigh’s Quotient. Since the increase in the first mode potential energy terms is not large enough, the values are close to each other.
3.2.5. Presentation 5
The influence of the unit length -mass ratio on the first three natural frequencies is shown in Figure 10. Dimensionless values were chosen as , , , , , , and . By taking , , , the entire effect of the rigid segment is neglected. The values 0, 0.01, 0.1, 0.2, 0.5, 1, 2, and 4 were used as the end-concentrated mass values. Natural frequency curves were obtained for each end-concentrated mass. The symbol is defined in Equation (19a) and shows the ratio of the unit–length masses. In the graphs, the ratio varies in value from 0.5 to 1. When the mass density of the two elastic segments is assumed to be approximately equal, the variable parameter for the value represents the ratio of the cross-sectional areas.
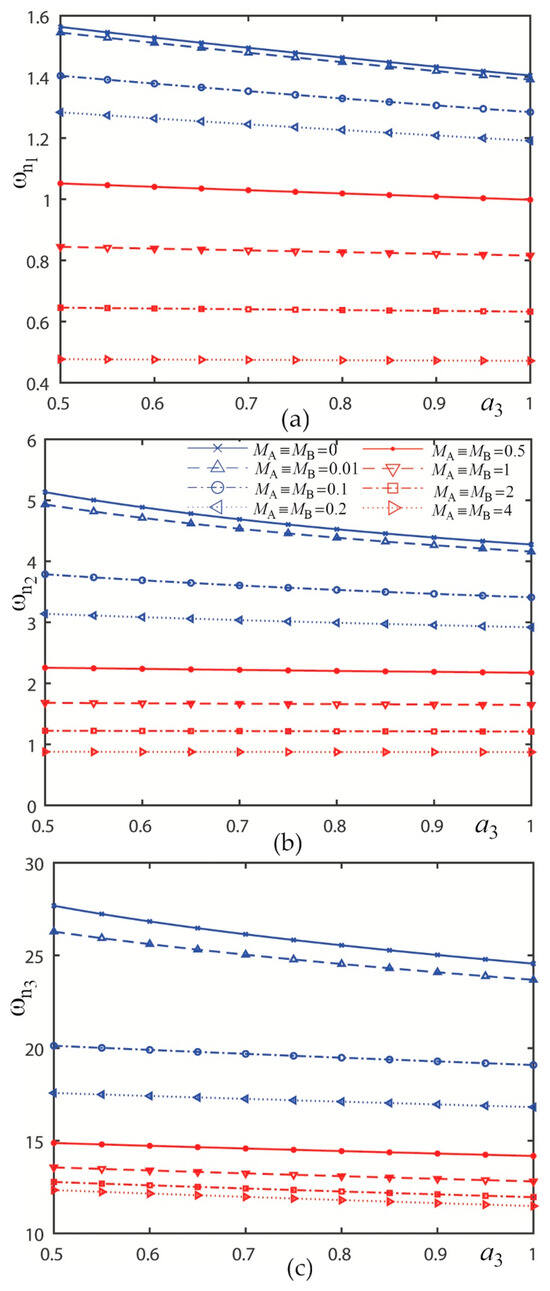
Figure 10.
The natural frequencies of the first (a), second (b), and third mode (c) versus the ratio of the cross-sectional areas for the beam.
As can be seen from the graph in Figure 10a–c, as the cross-sectional area of the elastic segment on the right side increases, the values of the natural frequency decrease. This situation occurs because that kinetic energy takes on greater values as the mass increases. According to the Rayleigh’s Quotient, as the kinetic energy increases, the natural frequency values decreases. When the concentrated masses equal zero, , the effect of the parameter is more clearly evident in the curves. When the end-concentrated mass values increase, the natural frequency values decrease. In addition, as the end-concentrated mass values increase, the effect of the parameter on the natural frequency gradually decreases.
3.2.6. Presentation 6
The effect of the mass moment of the inertia of the rigid segment on the first three natural frequencies is examined. In Equation (16b), the mass moment of the inertia relative to the center of the mass was indicated by the symbol . The variations in the natural frequency values of the beam are given in Figure 11. In the examinations, parameters depending on physical and geometric values were taken as , , , , , and . The mass moment of the inertia was chosen as 0, 0.005, 0.007, 0.0096, 0.0122, 0.0148, 0.0174 and 0.02 in the graphs.

Figure 11.
The variation in the natural frequency values of the first (a), second (b), and third (c) modes for the location of the center of mass and the moment of inertia of the rigid segment for the rigid stepped beam that is elastically supported and has an end-concentrated mass at both ends.
As the mass moment of inertia increases from 0 to 0.02, the kinetic energy of the system also increases. Therefore, the natural frequency values become smaller. The curves of the second and third modes, arranged one under the other, show this decrease. Apart from this, as the position of the center of mass of the rigid segment, indicated by , approaches 0.5, the rotation angle of the rigid segment decreases. As the rigid segment is at the midpoint, the angle of rotation is zero. Therefore, the rotational kinetic energy approaches zero, depending on the rotation angle. As the position of the rigid segment approaches to the right, the rotational kinetic energy of the rigid segment begins to be effective for the second and third modes. The curves given for in Figure 11b,c prove this situation. As a result, there is a decrease in the natural frequency values. If we look at the figure given in Figure 11a, it is seen that the frequency values are between 0.907 and 0.910. Due to the elastic boundary conditions, the small natural frequency values seen in Figure 11a occur. Since the components of this frequency do not affect the frequency sufficiently, the eight curves appear to coincide. When the rigid segment is moved from the end to the middle, the transverse displacement of the beam will increase. Therefore, the strain energy will increase. Thus, in all three graphs, the natural frequency values in the curves increase.
4. Discussion
In order to investigate the free undamped lateral vibrations of an elastically supported stepped beam with a rigid segment, an analytical solution was devised. Under the definitions made according to one Cartesian Axis Set, the frequency equation was derived. The natural frequencies and mode shapes of the beam were evaluated according to its physical and geometric parameters. Frequency value variations are presented in tables and graphs.
Unlike the other valuable studies [,,,], in this study, one Cartesian Axis Set was used like [,,,,] in the model. There are advantageous aspects of using only a single Cartesian coordinate system. The frequency equation found is valid for all values of the segment lengths of the beam with a rigid segment. For example, for a cantilever beam, the natural frequencies can be found with the same frequency equation whether the rigid segment is at the end or in the middle region. By setting the length of the elastic segment at the right of the rigid segment to zero, a clamped beam with a rigid segment at its end can be obtained.
The results of the numerical applications illustrated the following useful reductions. The increase in the end-concentrated masses causes a decrease in the natural frequency values, as shown Figure 6, Figure 7, Figure 8 and Figure 9. As the rigid section approaches the middle point, the strain and the natural frequency values increase accordingly. This situation can be seen in Figure 6b,c. By examining the plots in Figure 7a–c, it is found that the increase in the rigid segment mass causes the natural frequency values to decrease. Figure 8 and Figure 9 show that, as the stiffness coefficient of the translational stiffness elements or the stiffness coefficient of the rotational stiffness elements located at the end points or increases, the natural frequency values increase. Figure 10 shows the effect of changing the unit length mass ratio of one of the beams formed by the union of two beams on the natural frequency. It shows that when the unit length–mass ratio of one of the beams is increased, the natural frequency values become small. In Figure 11, it is indicated that the increase in the mass moment of inertia of the rigid segment causes the natural frequencies to decrease.
In this study, a linear case is considered. The nonlinear behavior of the material is not taken into account. As a continuation of this study, the effect of some nonlinear parameters, such as the stretching in the neutral axis according to Timoshenko’s beam theory, can be investigated.
Funding
This research received no external funding.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
The original contributions presented in this study are included in the article.
Acknowledgments
The author would like to thank Mert Uğur for his valuable support on Ansys.
Conflicts of Interest
The author declares no conflicts of interest.
Appendix A
The matrix of the coefficients for the beam with general boundary conditions at its end-points, as shown in Figure 1, is given row-by-row in Equation (A1). The first and second rows of the matrix are divided by , and the third and fourth rows are divided by . The rows of the coefficient matrix are written by using the short notation symbols given in Equation (A2). The frequency equation can be obtained by using the given coefficient matrix.
where the following abbreviations are used for clarity:
References
- Kopmaz, O.; Telli, S. On the Eigenfrequencies of a Two-Part Beam–Mass System. J. Sound Vib. 2002, 252, 370–384. [Google Scholar] [CrossRef]
- Taleb, N.J.; Suppiger, E.W. Vibration of Stepped Beams. J. Aerosp. Eng. 1961, 28, 295–298. [Google Scholar] [CrossRef]
- Balasubramanian, T.S.; Subramanian, G. On the performance of a four-degree-of-freedom per node element for stepped beam analysis and higher frequency estimation. J. Sound Vib. 1985, 99, 563–567. [Google Scholar] [CrossRef]
- Subramanian, G.; Balasubramanian, T.S. Beneficial effects of steps on the free vibration characteristics of beams. J. Sound Vib. 1987, 118, 555–560. [Google Scholar] [CrossRef]
- Sarıgül, A.S.; Aksu, G. A finite difference method for the free vibration analysis of stepped Timoshenko beams and shafts. Mech. Mach. Theory 1986, 21, 1–12. [Google Scholar] [CrossRef]
- Jang, S.K.; Bert, C.W. Free Vibration of Stepped Beams: Exact and Numerical Solutions. J. Sound Vib. 1989, 130, 342–346. [Google Scholar] [CrossRef]
- Özkaya, E.; Pakdemirli, M.; Öz, H.R. Non-Liner Vibrations of a Beam–Mass System Under Different Boundary Conditions. J. Sound Vib. 1997, 199, 679–696. [Google Scholar] [CrossRef]
- Magrab, E.B. Vibrations of Elastic Systems with Applications to MEMS and NEMS; Springer: Dordrecht/Heidelberg, Germany, 2012. [Google Scholar]
- Kopmaz, O.; Telli, S. Authors’ reply. J. Sound Vib. 2003, 265, 911–916. [Google Scholar] [CrossRef]
- Naguleswaran, S. Vibration of an Euler–Bernoulli stepped beam carrying a non-symmetrical rigid body at the step. J. Sound Vib. 2004, 271, 1121–1132. [Google Scholar] [CrossRef]
- Ilanko, S. Transcendental Dynamic Stability Functions for Beams Carrying Rigid Bodies. J. Sound Vib. 2005, 279, 1195–1202. [Google Scholar] [CrossRef]
- Lin, H.-Y.; Wang, C.-Y. Free Vibration Analysis of a Hybrid Beam Composed of Multiple Elastic Beam Segments and Elastic-Supported Rigid Bodies. J. Mar. Sci. Technol. 2012, 20, 525–533. [Google Scholar] [CrossRef]
- Obradovic, A.; Salinic, S.; Trifkovic, D.R.; Zoric, N.; Stokic, Z. Free vibration of structures composed of rigid bodies and elastic beam segments. J. Sound Vib. 2015, 347, 126–138. [Google Scholar] [CrossRef]
- Farghaly, S.H.; El-Sayed, T.A. Exact free vibration analysis for mechanical system composed of Timoshenko beams with intermediate eccentric rigid body on elastic supports: An experimental and analytical investigation. Mech. Syst. Signal Process. 2017, 82, 376–393. [Google Scholar] [CrossRef]
- El-Sayed, T.A.; Farghaly, S.H. Frequency Equation Using New Set of Fundamental Solutions with Application on the Free Vibration of Timoshenko Beams with Intermediate Rigid or Elastic Span. J. Vib. Control 2017, 24, 4764–4780. [Google Scholar] [CrossRef]
- Lee, J.W. Free Vibration Analysis of Elastically Restrained Tapered Beams with Concentrated Mass and Axial Force. Appl. Sci. 2023, 13, 10742. [Google Scholar] [CrossRef]
- Chen, D.; Gu, C.; Li, M.; Sun, B.; Li, X. Natural Vibration Characteristics Determination of Elastic Beam with Attachments Based on a Transfer Matrix Method. J. Vib. Control 2021, 28, 637–651. [Google Scholar] [CrossRef]
- Liu, X.; Sun, C.; Banerjee, J.R.; Dan, H.-C.; Chang, L. An exact dynamic stiffness method for multibody systems consisting of beams and rigid-bodies. Mech. Syst. Signal Process. 2021, 150, 107264. [Google Scholar] [CrossRef]
- Luo, J.; Zhu, S.; Zhai, W. Exact Closed-Form Solution for Free Vibration of Euler-Bernoulli and Timoshenko Beams with Intermediate Elastic Supports. Int. J. Mech. Sci. 2022, 213, 106842. [Google Scholar] [CrossRef]
- Flashner, H. An Approach to Modeling Vibrations of Systems Composed of Beams, Rigid Bodies, and Point Masses. J. Sound Vib. 2023, 551, 117609. [Google Scholar] [CrossRef]
- Tekin, A.; Özkaya, E. Ankastre Mesnetli Çok Kademeli Kirişlerin Serbest Titreşimleri. C.B.Ü. Fen Bilim. Derg. 2007, 3, 143–152. [Google Scholar]
- Shi, W.; Li, X.-F.; Lee, K.Y. Transverse Vibration of Free–Free Beams Carrying Two Unequal End Masses. Int. J. Mech. Sci. 2015, 90, 251–257. [Google Scholar] [CrossRef]
- Bilge, H.; Morgül, Ö.K. Analytical and Experimental Investigation of the Rotary Inertia Effects of Unequal End Masses on Transverse Vibration of Beams. Appl. Sci. 2023, 13, 2518. [Google Scholar] [CrossRef]
- Goel, R.P. Free Vibrations of a Beam-Mass System with Elastically Restrained Ends. J. Sound Vib. 1976, 47, 9–14. [Google Scholar] [CrossRef]
- Lau, J.H. Fundamental Frequency of a Constrained Beam. J. Sound Vib. 1981, 78, 154–157. [Google Scholar] [CrossRef]
- Bapat, C.N.; Bapat, C. Natural Frequencies of a Beam with Non-Classical Boundary Conditions and Concentrated Masses. J. Sound Vib. 1987, 112, 177–182. [Google Scholar] [CrossRef]
- Laura, P.A.A.; Filipich, C.P.; Cortinez, V.H. Vibrations of Beams and Plates Carrying Concentrated Masses. J. Sound Vib. 1987, 117, 459–465. [Google Scholar] [CrossRef]
- Liu, W.H.; Yeh, F.H. Free Vibration of a Restrained Non-Uniform Beam with Intermediate Masses. J. Sound Vib. 1987, 117, 555–570. [Google Scholar] [CrossRef]
- Naguleswaran, S. Transverse vibration of an Euler–Bernoulli uniform beam on up to five resilient supports including ends. J. Sound Vib. 2003, 261, 372–384. [Google Scholar] [CrossRef]
- Naguleswaran, S. Vibration of an Euler–Bernoulli beam on elastic end supports and with up to three step changes in cross-section. Int. J. Mech. Sci. 2002, 44, 2541–2555. [Google Scholar] [CrossRef]
- Jin, Y.; Lu, Y.; Yang, D.; Zhao, F.; Luo, X.; Zhang, P. An Analytical Method for Vibration Analysis of Multi-Span Timoshenko Beams under Arbitrary Boundary Conditions. Arch. Appl. Mech. 2023, 94, 529–553. [Google Scholar] [CrossRef]
- Dou, B.; Ding, H.; Mao, X.-Y.; Feng, H.-R.; Chen, L.-Q. Modeling and parametric studies of retaining clips on pipes. Mech. Syst. Signal Process. 2023, 186, 109912. [Google Scholar] [CrossRef]
- Kanwal, G.; Nawaz, R.; Ahmed, N. Analyzing the Effect of Rotary Inertia and Elastic Constraints on a Beam Supported by a Wrinkle Elastic Foundation: A Numerical Investigation. Buildings 2023, 13, 1457. [Google Scholar] [CrossRef]
- Pakdemirli, M.; Nayfeh, A.H. Nonlinear Vibrations of a Beam-Spring-Mass System. J. Vib. Acoust. 1994, 116, 433–439. [Google Scholar] [CrossRef]
- Banerjee, J.R.; Sobey, A.J. Further investigation into eigenfrequencies of a two-part beam–mass system. J. Sound Vib. 2003, 265, 899–908. [Google Scholar] [CrossRef]
- Ilanko, S. Comments On “On the Eigenfrequencies of a Two-Part Beam-Mass System”. J. Sound Vib. 2003, 265, 909–910. [Google Scholar] [CrossRef]
- Nayfeh, A.H. Introduction to Perturbation Techniques; Wiley: Hoboken, NJ, USA, 1993. [Google Scholar]
- Chen, G.; Zeng, X.; Liu, X.; Rui, X. Transfer Matrix Method for the Free and Forced Vibration Analyses of Multi-step Timoshenko Beams Coupled with Rigid Bodies on Springs. Appl. Math. Model. 2020, 87, 152–170. [Google Scholar] [CrossRef]
- Gurgoze, M. Study of Vibrations by Analytical Methods; Istanbul Technical University: Istanbul, Turkey, 1984. (In Turkish) [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).