Computational Analysis of Concrete Flow in a Reinforced Bored Pile Using the Porous Medium Approach
Abstract
:1. Introduction
2. Materials and Methods
2.1. Functional Principles of CFD
2.2. Porous Medium Representation of Reinforcements
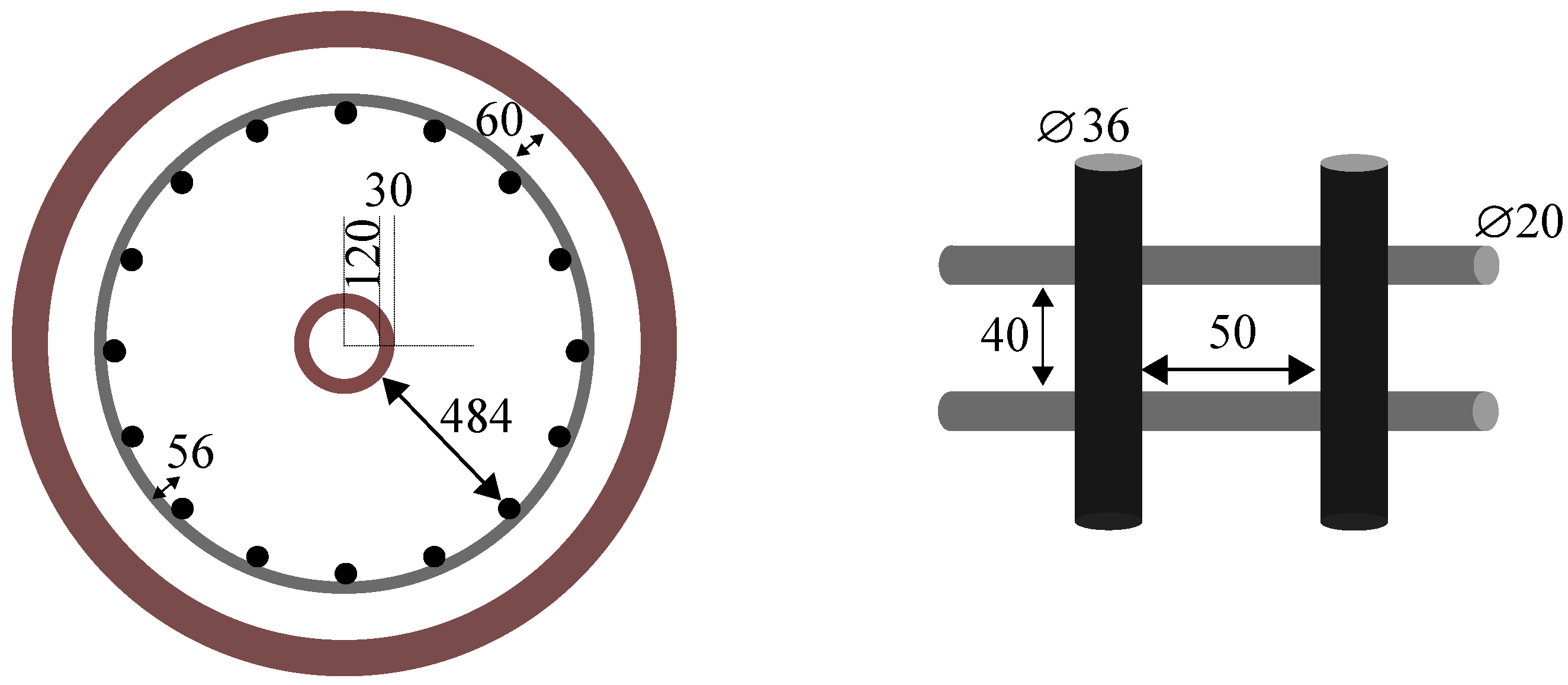
2.3. Reinforcement
2.4. Specification of the Material Properties
3. Computational Analysis
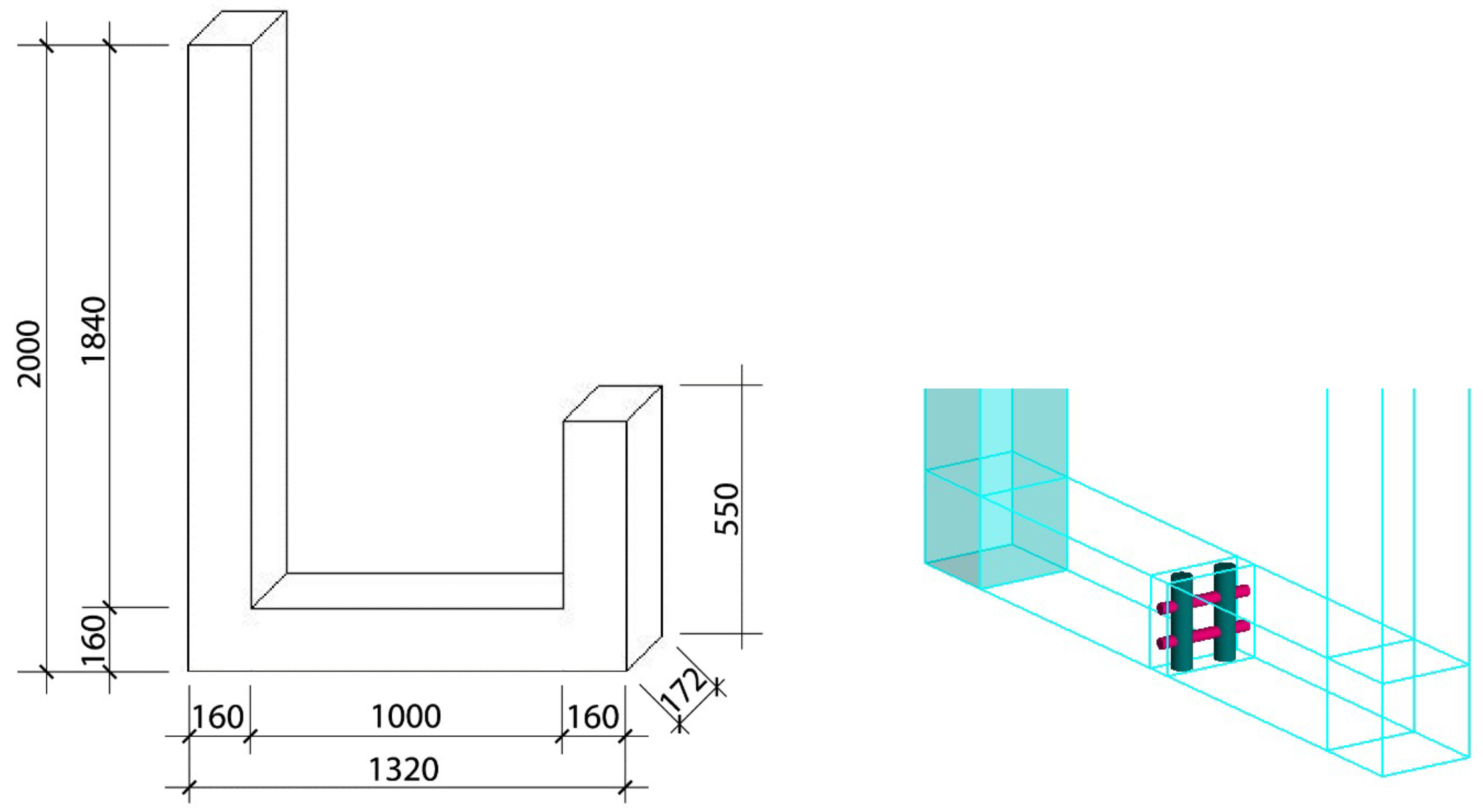
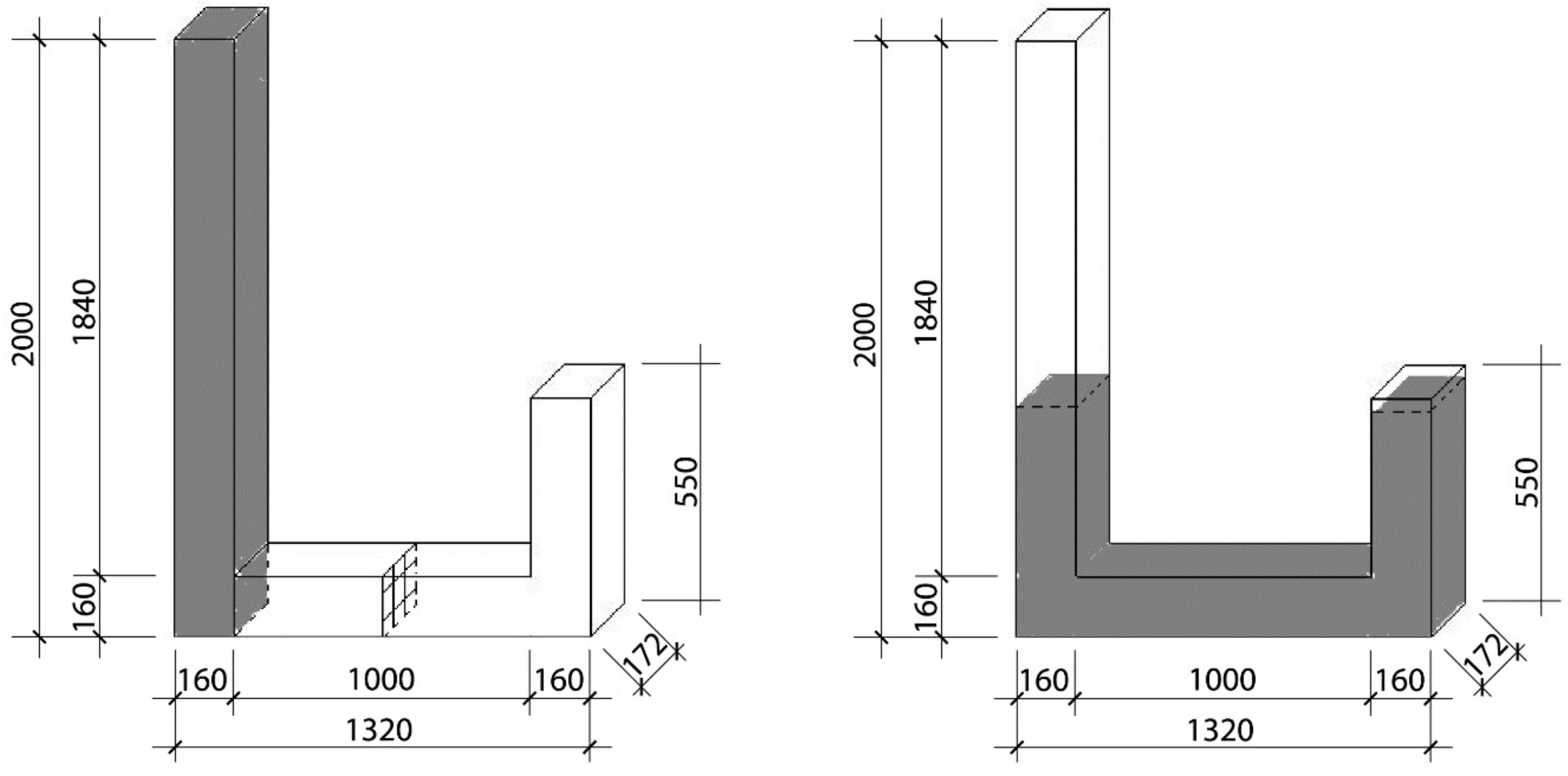
3.1. Simulation and Analysis of Concrete Flow in a U-Box
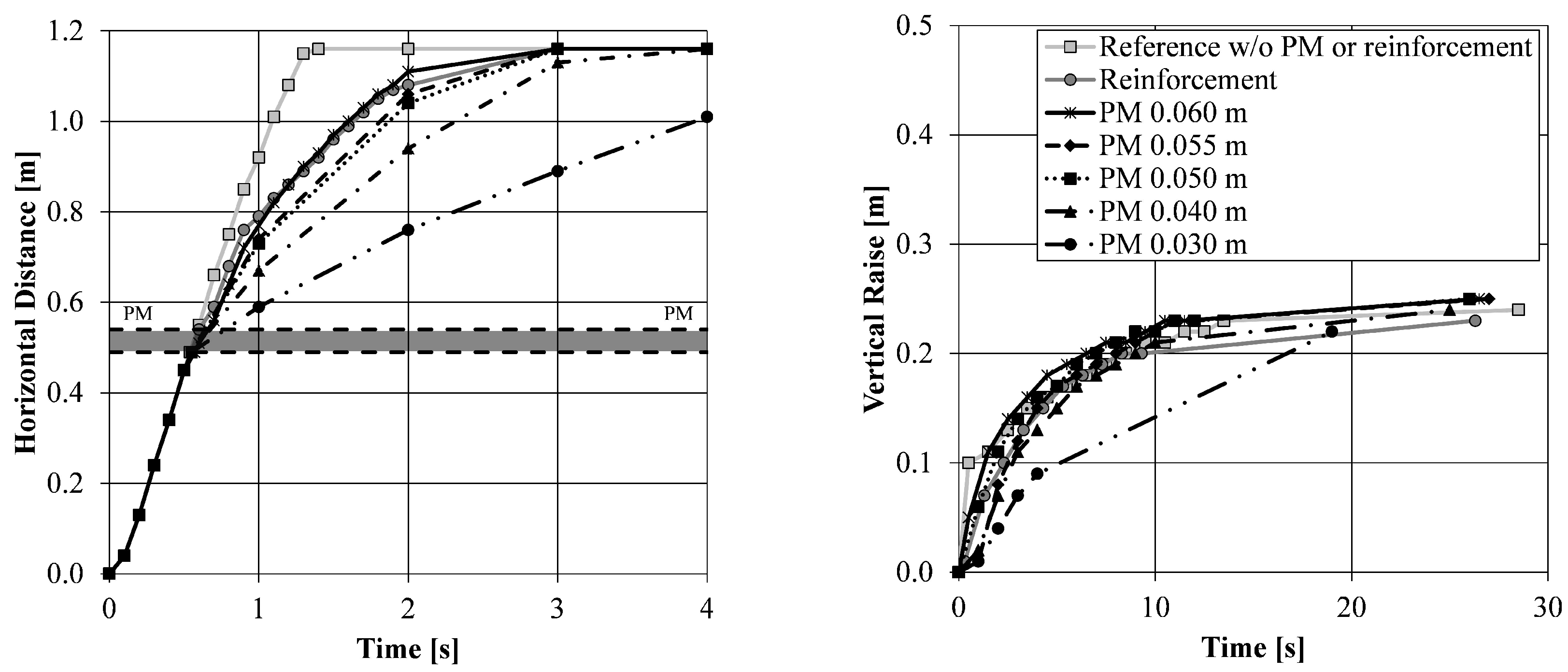
3.1.1. Influence of the Thickness of the Porous Medium
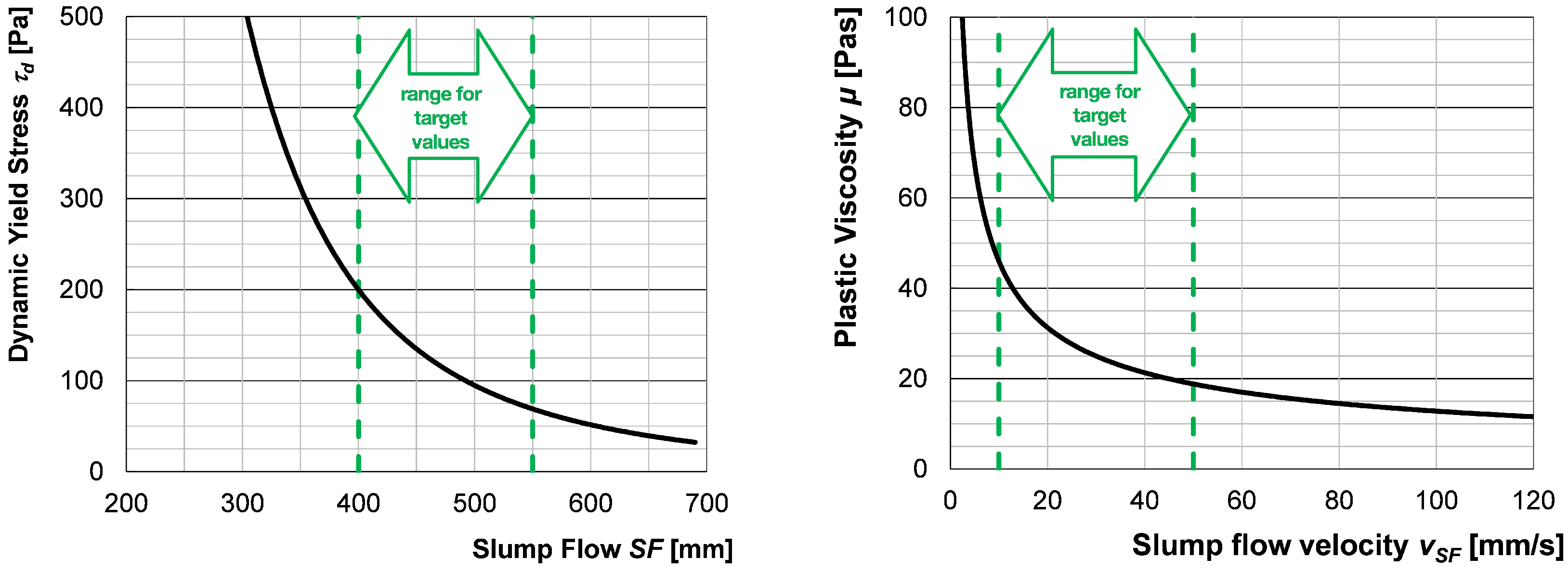
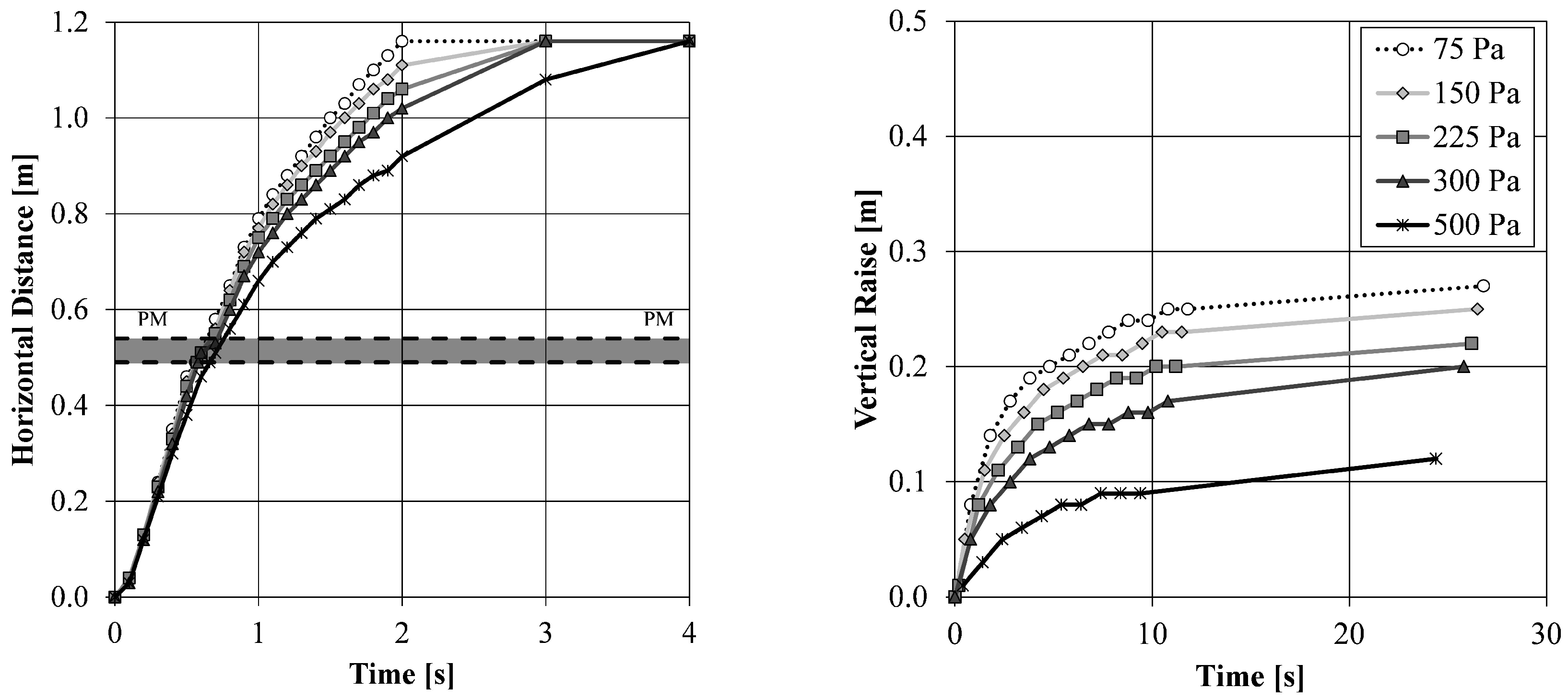
3.1.2. Influence of the Yield Stress
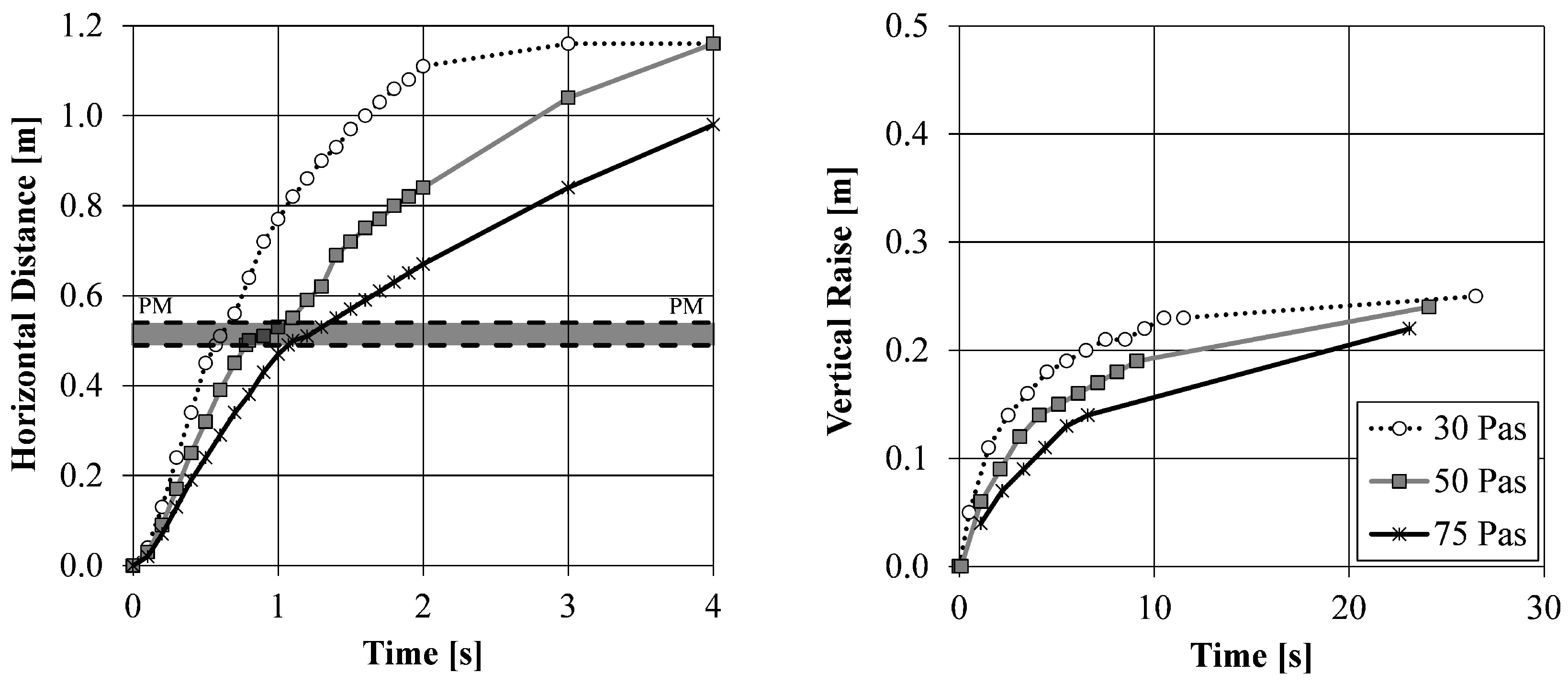
3.1.3. Influence of the Viscosity
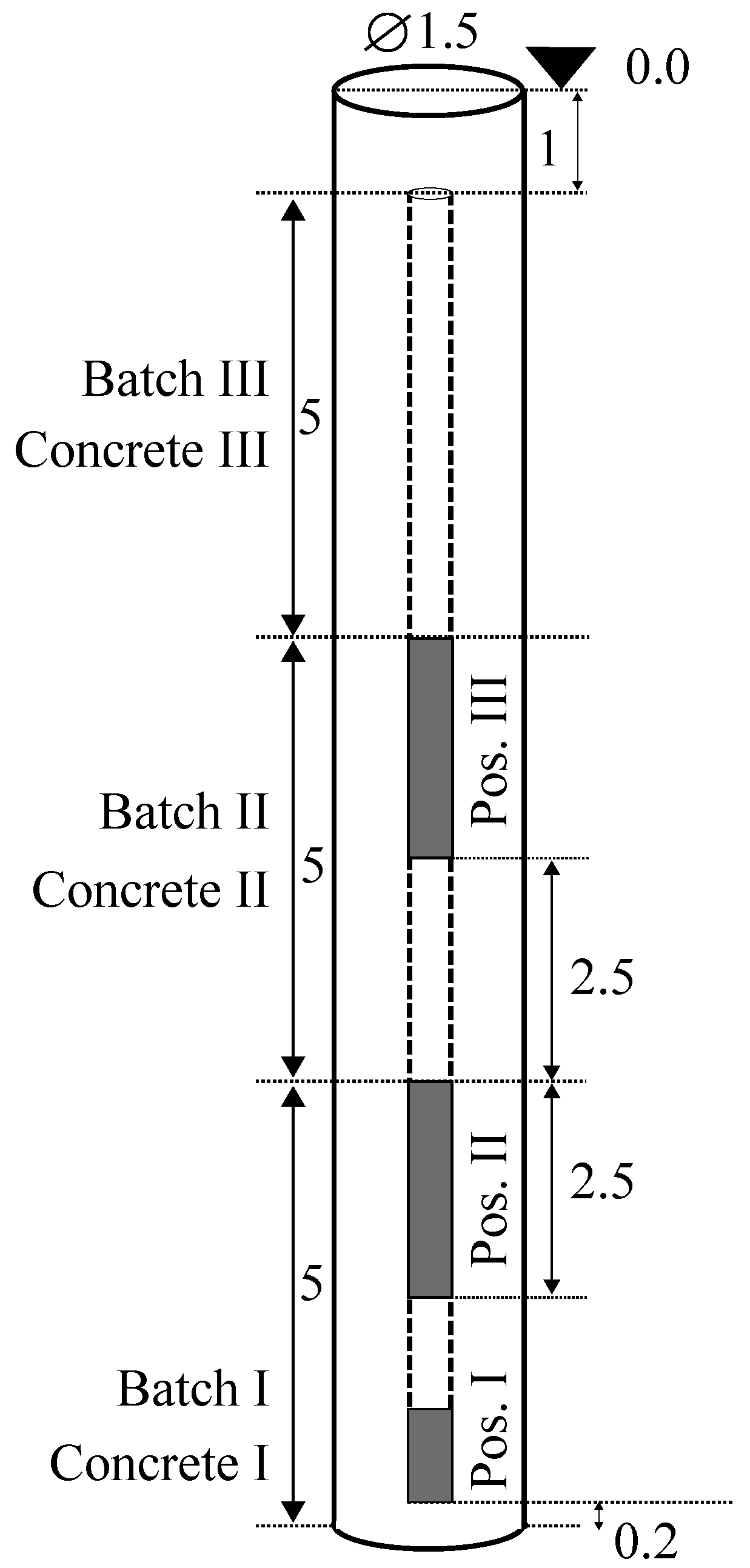
4. Simulation and Analysis of Concrete Flow in a Bored Pile
4.1. Effect of Pouring a Concrete with Low/Medium Yield Stress and Medium Viscosity in a Concrete with Same Properties (Concrete A)
4.2. Effect of Pouring a Concrete with Medium Yield Stress and High Viscosity in a Concrete with Same Properties (Concrete B)
4.3. Effect of Pouring a Concrete A in Concrete B
4.4. Comparison of the Calculation Time of Reinforced Pile and Pile with Porous Medium
5. Conclusions
- Using the porous medium approach to model reinforcements, the simulation of concrete flow is 10 times faster. Hence a drastic saving of computational time is achieved;
- The porous medium approach is accurate and this has been validated and the influence of the rheological parameters have been analysed using the U-Box simulations;
- Computational simulations of casting of a reinforced pile using the porous media approach showed that after a first batch of casting, subsequent batches of concrete cannot penetrate the reinforcements;
- After the first batch has been poured, subsequent batches of concrete prefer to rather move vertically against gravity rather than penetrate the reinforcement in the horizontal direction. As a consequence, if the first batch of concrete is less workable (i.e., high viscosity and yield stress), then the risk of the formation of defects in the cover zone is highly likely due to the flow resistance induced by the reinforcements;
- To improve the accuracy of the simulation, the thixotropy of concrete, i.e., the time-dependent evolution of the rheological properties of concrete must be considered.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| CFD | Computational Fluid Dynamics |
| PM | Porous Medium |
References
- Larish, M. Fundamental Mechanisms of Concrete Bleeding in Bored Piles. In Proceedings of the Concrete 2019 Conference, Sydney, Australia, 8–11 September 2019; pp. 8–11. [Google Scholar]
- EFFC/DFI-Concrete-Task-Group. Guide to Tremie Concrete for Deep Foundations. 2018. Available online: https://www.effc.org/content/uploads/2018/08/EFFC_DFI_Tremie_Concrete_Guide_2nd-Edition_2018_Final_rev2_28-08-18.pdf (accessed on 1 September 2018).
- Larisch, M. Concrete defects in bored piles as a result of insufficient applications of chemical admixtures. In Proceedings of The NZ Concrete Industry Conference, Dunedin, New Zealand, 10–12 October 2019. [Google Scholar]
- Liu, H.; Liu, W.; Sui, S.; Xu, H.; Wang, J. Dynamic monitoring technique of bored pile pouring process based on multi-frequency ultrasound. J. Civ. Struct. Health Monit. 2022, 12, 411–425. [Google Scholar] [CrossRef]
- Mechtcherine, V.; Gram, A.; Krenzer, K.; Schwabe, J.H.; Shyshko, S.; Roussel, N. Simulation of fresh concrete flow using Discrete Element Method (DEM): Theory and applications. Mater. Struct. 2014, 47, 615–630. [Google Scholar] [CrossRef]
- Cui, W.; Ji, T.; Li, M.; Wu, X. Simulating the workability of fresh self-compacting concrete with random polyhedron aggregate based on DEM. Mater. Struct. 2017, 50, 92. [Google Scholar] [CrossRef]
- Ramyar, E.; Cusatis, G. Discrete Fresh Concrete Model for Simulation of Ordinary, Self-Consolidating, and Printable Concrete Flow. J. Eng. Mech. 2022, 148, 04021142. [Google Scholar] [CrossRef]
- Xu, Z.; Li, Z.; Jiang, F. The applicability of SPH and MPS methods to numerical flow simulation of fresh cementitious materials. Constr. Build. Mater. 2021, 274, 121736. [Google Scholar] [CrossRef]
- Roussel, N.; Gram, A. Simulation of fresh concrete flow. In RILEM State-of-the-Art Reports; Springer: Dordrecht, The Netherlands, 2014; Volume 15. [Google Scholar]
- De Schryver, R.; El Cheikh, K.; Lesage, K.; Yardimci, M.Y.; De Schutter, G. Numerical Reliability Study Based on Rheological Input for Bingham Paste Pumping Using a Finite Volume Approach in OpenFOAM. Materials 2021, 14, 5011. [Google Scholar] [CrossRef] [PubMed]
- Roussel, N.; Geiker, M.R.; Dufour, F.; Thrane, L.N.; Szabo, P. Computational modeling of concrete flow: General overview. Cem. Concr. Res. 2007, 37, 1298–1307. [Google Scholar] [CrossRef] [Green Version]
- Vasilic, K.; Gram, A.; Wallevik, J.E. Numerical simulation of fresh concrete flow: Insight and challenges. RILEM Tech. Lett. 2019, 4, 57–66. [Google Scholar] [CrossRef]
- Larisch, M.; Qin, Z.; Alehossein, H. Performance control tests and numerical simulations for concrete in deep foundations. Concr. Aust. 2013, 39, 26–34. [Google Scholar]
- Grandstrand, O. New findings concerning the spreading of fresh concrete in bored piles. In Insights and Innovations in Structural Engineering, Mechanics and Computation; Zingoni, A., Ed.; CRC PRess: London, UK, 2016; Chapter 349; pp. 2116–2121. [Google Scholar]
- Wilkes, C. Modelling Tremie Concrete Placement in Deep Foundations. Ph.D. Thesis, University of Cambridge, Cambridge, UK, 2021. [Google Scholar]
- Vasilic, K.; Meng, B.; Kühne, H.C.; Roussel, N. Flow of fresh concrete through steel bars: A porous medium analogy. Cem. Concr. Res. 2011, 41, 496–503. [Google Scholar] [CrossRef]
- Vasilic, K.; Schmidt, W.; Kühne, H.C.; Haamkens, F.; Mechtcherine, V.; Roussel, N. Flow of fresh concrete through reinforced elements: Experimental validation of the porous analogy numerical method. Cem. Concr. Res. 2016, 88, 1–6. [Google Scholar] [CrossRef]
- Roussel, N. Correlation between yield stress and slump: Comparison between numerical simulations and concrete rheometers results. Mater. Struct. 2006, 39, 501–509. [Google Scholar] [CrossRef]
- Roussel, N.; Coussot, P. ’Fifty-cent rheometer’ for yield stress measurements: From slump to spreading flow. J. Rheol. 2005, 49, 705–718. [Google Scholar] [CrossRef]
- Roussel, N.; Staquet, S.; Schwarzentruber, L.A.; Le Roy, R.; Toutlemonde, F. SCC casting prediction for the realization of prototype VHPC-precambered composite beams. Mater. Struct. 2007, 40, 877–887. [Google Scholar] [CrossRef]
- De Larrard, F.; Ferraris, C.; Sedran, T. Fresh concrete: A Herschel-Bulkley material. Mater. Struct. 1998, 31, 494–498. [Google Scholar] [CrossRef]
- Tattersall, G.H.; Banfill, P.F. The Rheology of Fresh Concrete; Number Monograph; Pitman Advanced Pub. Program: MI, USA, 1983. [Google Scholar]
- Roussel, N. Understanding the Rheology of Concrete; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Vasilić, K. A Numerical Model for Self-Compacting Concrete Flow through Reinforced Sections: A Porous Medium Analogy. Ph.D. Thesis, Bundesanstalt für Materialforschung und-prüfung (BAM), Berlin, Germany, 2016. [Google Scholar]
- Manual, F.U. Flow3D User Manual, v9.3; Flow Science Inc.: Santa Fe, NM, USA, 2011; pp. 97–99. [Google Scholar]
- Tamayol, A.; Bahrami, M. Transverse permeability of fibrous porous media. Phys. Rev. E 2011, 83, 046314. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tamayol, A.; Bahrami, M. Analytical determination of viscous permeability of fibrous porous media. Int. J. Heat Mass Transf. 2009, 52, 2407–2414. [Google Scholar] [CrossRef]
- Kränkel, T. Rheology and Workability Testing of Deep Foundation Concrete in Europe and the US. 2018. Available online: https://www.effc.org/content/uploads/2018/08/R_D_Report_draft-2018.pdf (accessed on 1 September 2019).

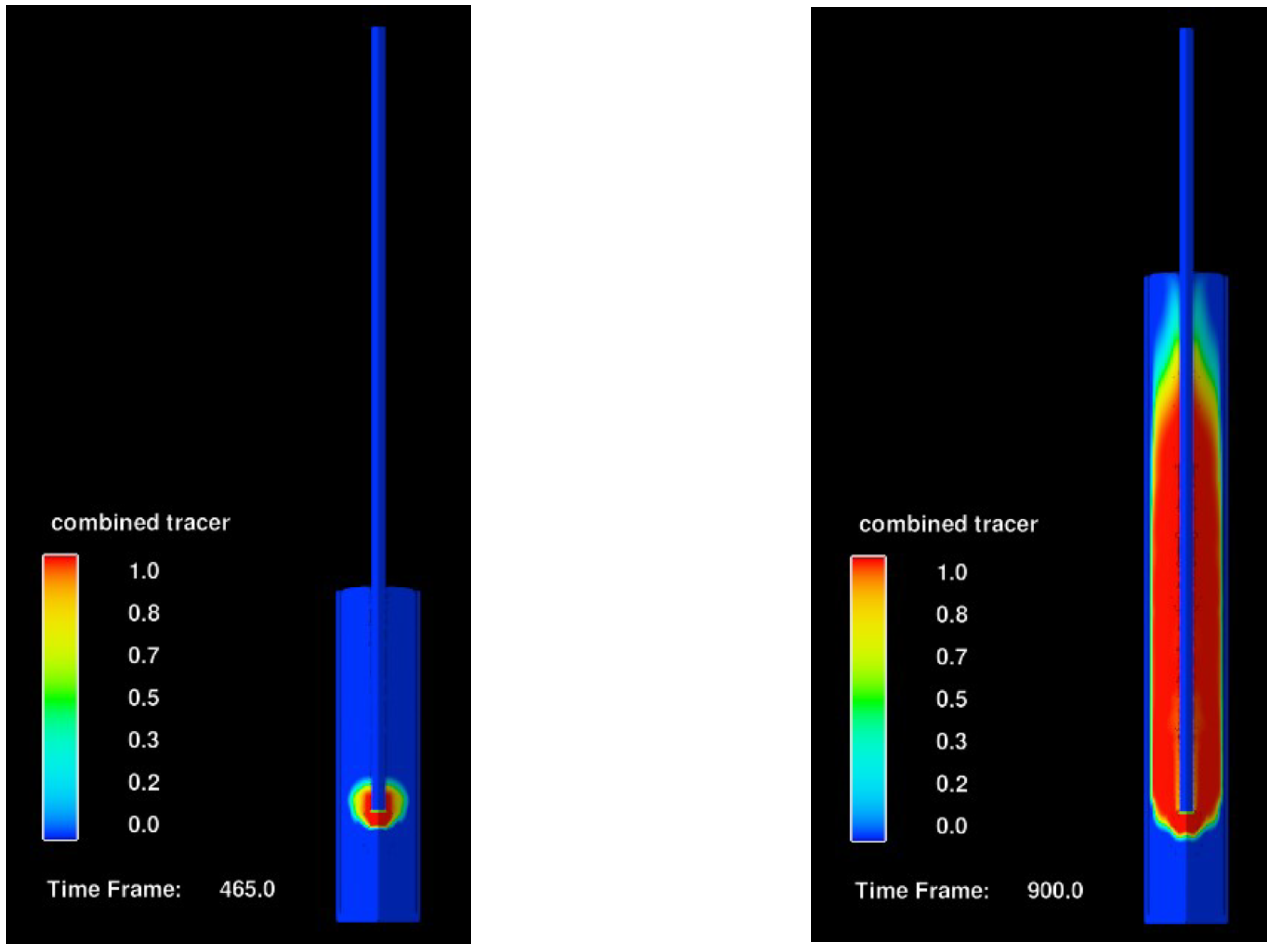
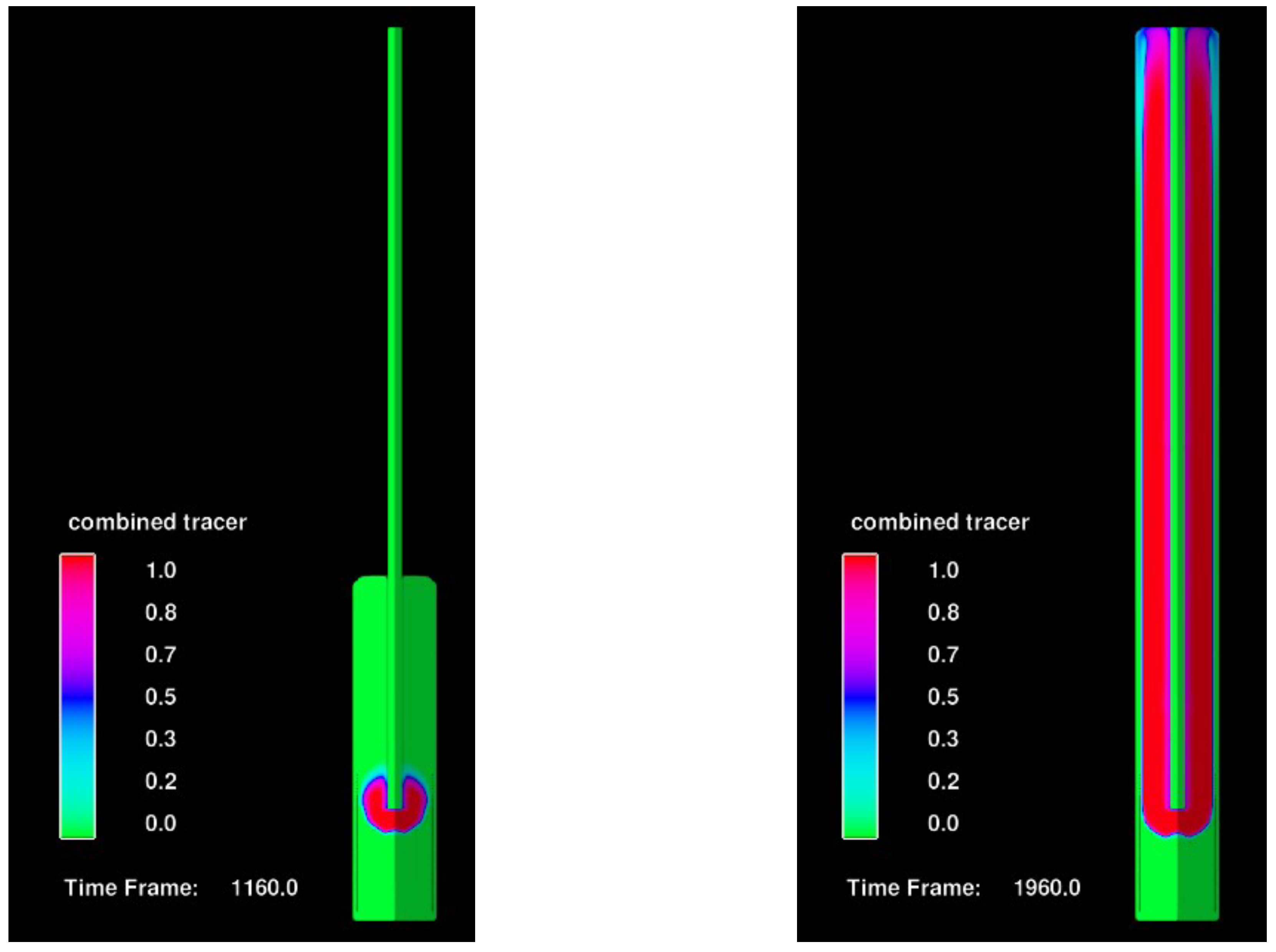
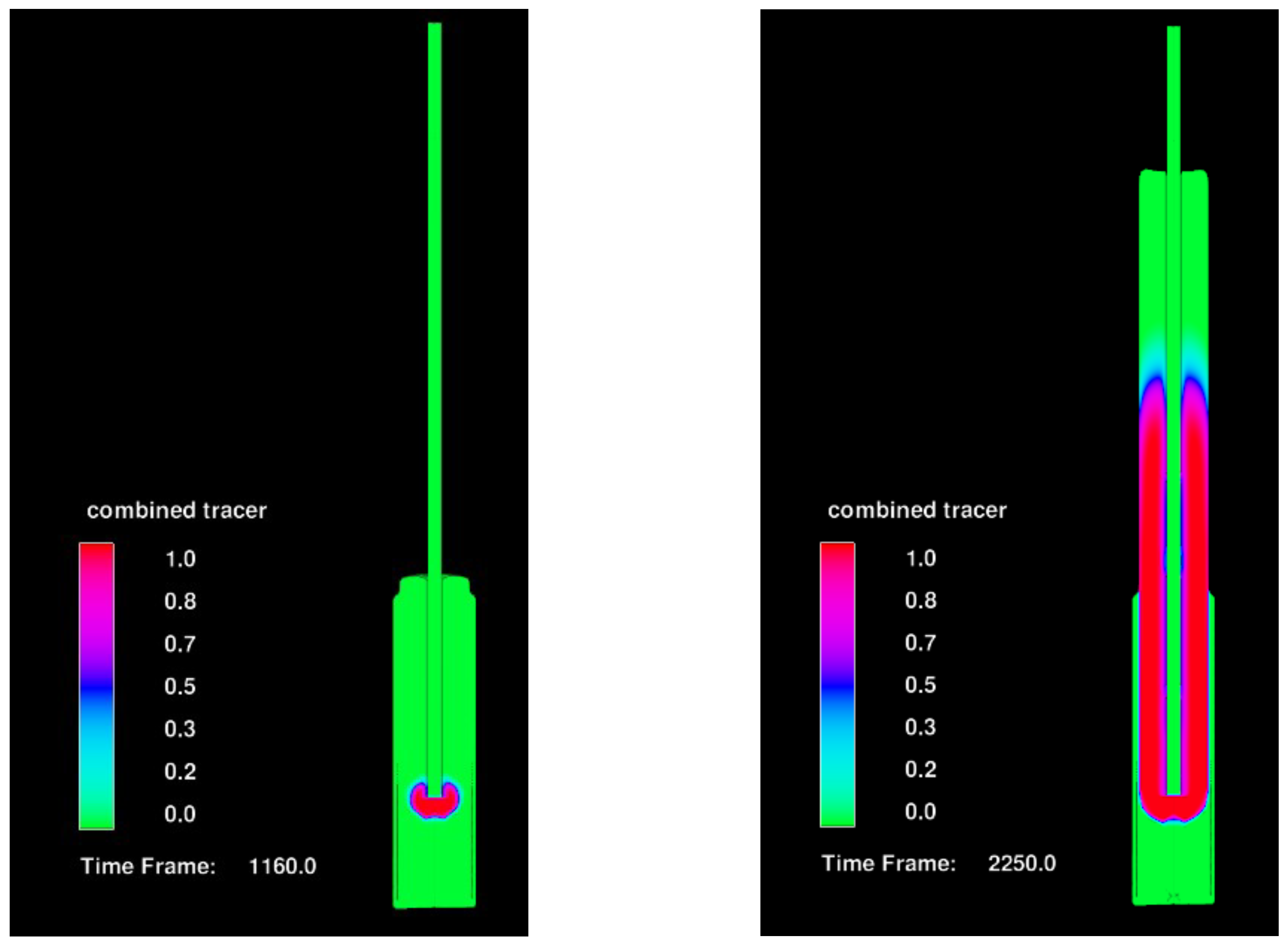
| Variation | Yield Stress [Pa] | Viscosity [Pa s] | Concrete Used for Bored Pile Simulations |
|---|---|---|---|
| 1 | 75 | 30 | - |
| 2 | 75 | 50 | - |
| 3 | 150 | 30 | Concrete A |
| 4 | 150 | 50 | - |
| 5 | 150 | 75 | - |
| 6 | 225 | 30 | - |
| 7 | 225 | 50 | - |
| 8 | 300 | 30 | - |
| 9 | 300 | 50 | - |
| 10 | 500 | 30 | - |
| 11 | 500 | 50 | Concrete B |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kränkel, T.; Weger, D.; Beckhaus, K.; Geppert, F.; Gehlen, C.; Timothy, J.J. Computational Analysis of Concrete Flow in a Reinforced Bored Pile Using the Porous Medium Approach. Appl. Mech. 2022, 3, 481-495. https://doi.org/10.3390/applmech3020028
Kränkel T, Weger D, Beckhaus K, Geppert F, Gehlen C, Timothy JJ. Computational Analysis of Concrete Flow in a Reinforced Bored Pile Using the Porous Medium Approach. Applied Mechanics. 2022; 3(2):481-495. https://doi.org/10.3390/applmech3020028
Chicago/Turabian StyleKränkel, Thomas, Daniel Weger, Karsten Beckhaus, Fabian Geppert, Christoph Gehlen, and Jithender J. Timothy. 2022. "Computational Analysis of Concrete Flow in a Reinforced Bored Pile Using the Porous Medium Approach" Applied Mechanics 3, no. 2: 481-495. https://doi.org/10.3390/applmech3020028
APA StyleKränkel, T., Weger, D., Beckhaus, K., Geppert, F., Gehlen, C., & Timothy, J. J. (2022). Computational Analysis of Concrete Flow in a Reinforced Bored Pile Using the Porous Medium Approach. Applied Mechanics, 3(2), 481-495. https://doi.org/10.3390/applmech3020028






