Spherical Indentation of a Micropolar Solid: A Numerical Investigation Using the Local Point Interpolation–Boundary Element Method
Abstract
:1. Introduction
2. Governing Equations
3. Solution Method
- 1.
- Set k = 0,, ;
- 2.
- Solve ;
- 3.
- Solve ;
- 4.
- If the > (a given tolerance) go to 2.
4. Numerical Results
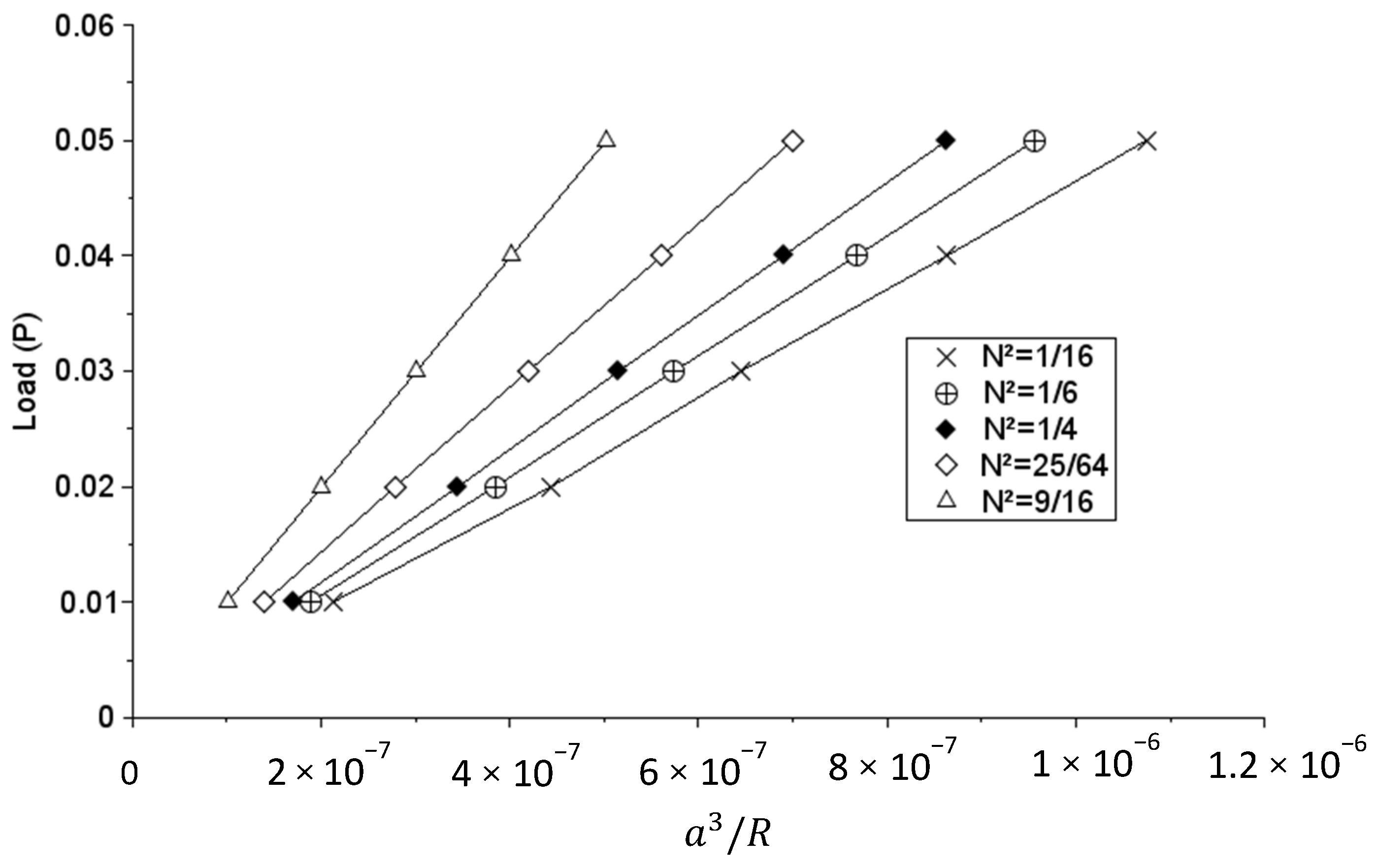
4.1. Influence of the Coupling Number
4.2. Influence of the Characteristic Length in Torsion and the Polar Ratio
4.3. Influence of the Characteristic Length in Bending
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Non symmetric macro stress tensor | |
| Couple stress tensor | |
| Macro displacement vector | |
| Micro rotation vector | |
| Displacement gradient tensor | |
| Small strain tensor | |
| Micro rotation gradient tensor | |
| Symmetric part of | |
| Anti-symmetric part of | |
| Traction vector | |
| Micro torque vector | |
| Lamé coefficient | |
| Lamé coefficient | |
| Cosserat couple modulus | |
| Rotation gradient moduli | |
| Material depression | |
| Radius of the projected contact area | |
| Radius of the indenter | |
| Applied load | |
| Young modulus | |
| Shear modulus | |
| Poisson ratio | |
| Characteristic length in torsion | |
| Characteristic length in bending | |
| Polar ratio | |
| Coupling number | |
| Contact stiffness |
References
- Sneddon, I.N. Fourier Transforms; McGraw-Hill: New York, NY, USA, 1951. [Google Scholar]
- Johnson, K.L. Contact mechanics, 9th ed.; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Oumarou, N.; Jehl, J.P.; Kouitat, R.; Stempfle, P. On the variation of mechanical parameters obtained from spherical depth sensing indentation. Int. J. Surf. Sci. Eng. 2010, 4, 416. [Google Scholar] [CrossRef]
- Eringen, A.C.; Suhubi, E.S. Nonlinear theory of simple micro-elastic solids—I. Int. J. Eng. Sci. 1964, 2, 189–203. [Google Scholar] [CrossRef]
- Cosserat, E.; Cosserat, F. Théorie Des Corps Déformables; Herman et Fils: Paris, France, 1909. [Google Scholar]
- Lakes, R.S. Experimental microelasticity of two porous solids. Int. J. Solids Struct. 1986, 22, 55–63. [Google Scholar] [CrossRef]
- Diebels, S. Micropolar mixture models on the basis of the Theory of Porous Media. In Porous Media; Ehlers, P.D.-I.W., Bluhm, P.D.D.-I.J., Eds.; Springer: Berlin/Heidelberg, Germany, 2002; pp. 121–145. [Google Scholar] [CrossRef]
- Goddard, J.D. From Granular Matter to Generalized Continuum. In Mathematical Models of Granular Matter; Capriz, G., Mariano, P.M., Giovine, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 1–22. [Google Scholar] [CrossRef] [Green Version]
- Park, H.C.; Lakes, R.S. Cosserat micromechanics of human bone: Strain redistribution by a hydration sensitive constituent. J. Biomech. 1986, 19, 385–397. [Google Scholar] [CrossRef]
- Cowin, S.C. Bone poroelasticity. J. Biomech. 1999, 32, 217–238. [Google Scholar] [CrossRef]
- Rosenberg, J.; Cimrman, R. Microcontinuum approach in biomechanical modelling. Math. Comput. Simul. 2003, 61, 249–260. [Google Scholar] [CrossRef]
- Ramtani, S. Electro-mechanics of bone remodelling. Int. J. Eng. Sci. 2008, 46, 1173–1182. [Google Scholar] [CrossRef]
- Eringen, A.C. Electromagnetic theory of microstretch elasticity and bone modelling. Int. J. Eng. Sci. 2004, 42, 231–242. [Google Scholar] [CrossRef]
- Diebels, S.; Geringer, A. Micromechanical and macromechanical modelling of foams: Identification of Cosserat parameters: Micromechanical and macromechanical modelling of foams. ZAMM-Journal of Applied Mathematics and Mechanics/Zeitschrift für Angewandte Mathematik und Mechanik 2014, 94, 414–420. [Google Scholar] [CrossRef]
- Neff, P.; Jeong, J.; Fischle, A. Stable identification of linear isotropic Cosserat parameters: Bounded stiffness in bending and torsion implies conformal invariance of curvature. Acta Mech. 2010, 211, 237–249. [Google Scholar] [CrossRef]
- Njiwa, R.K. Isotropic-BEM coupled with a local point interpolation method for the solution of 3D-anisotropic elasticity problems. Eng. Anal. Bound. Elem. 2011, 35, 611–615. [Google Scholar] [CrossRef]
- Pierson, G.; Bravetti, P.; Njiwa, R.K. Interaction implant-bone as a micropolar elastic medium: Porosity impact of the hard living media. Int. J. Theor. Appl. Multiscale Mech. 2020, 3, 229. [Google Scholar] [CrossRef]
- Njiwa, R.K.; Pierson, G.; Voignier, A. Coupling BEM and the Local Point Interpolation for the Solution of Anisotropic Elastic Nonlinear, Multi-Physics and Multi-Fields Problems. Int. J. Comput. Methods 2019, 17, 1950067. [Google Scholar] [CrossRef]
- Pierson, G.; Kouitat-Njiwa, R.; Bravetti, P. A boundary elements only solution method for 3D micropolar elasticity. Eng. Anal. Bound. Elem. 2021, 123, 84–92. [Google Scholar] [CrossRef]
- Eringen, A.C. Microcontinuum Field Theories; Springer: New York, NY, USA, 1999. [Google Scholar] [CrossRef]
- Iesan, D.; Pompei, A. On the equilibrium theory of microstretch elastic solids. Int. J. Eng. Sci. 1995, 33, 399–410. [Google Scholar] [CrossRef]
- Sládek, V.; Sládek, J. Boundary integral equation method in micropolar elasticity. Appl. Math. Model. 1983, 7, 433–440. [Google Scholar] [CrossRef] [Green Version]
- Nardini, D.; Brebbia, C.A. A new approach to free vibration analysis using boundary elements. Appl. Math. Model. 1983, 7, 157–162. [Google Scholar] [CrossRef]
- Gao, X.-W. The radial integration method for evaluation of domain integrals with boundary-only discretization. Eng. Anal. Bound. Elem. 2002, 26, 905–916. [Google Scholar] [CrossRef]
- Liu, G.R.; Gu, Y.T. A local radial point interpolation method (LRPIM) for free vibration analyses of 2-D solids. J. Sound Vib. 2001, 246, 29–46. [Google Scholar] [CrossRef] [Green Version]
- Christensen, P.W. A semi-smooth Newton method for elasto-plastic contact problems. Int. J. Solids Struct. 2002, 39, 2323–2341. [Google Scholar] [CrossRef]
| (MPa) | (MPa) | (MPa) | (N) | (N) | (N) | |
|---|---|---|---|---|---|---|
| 157,500 | 8166.67 | 1166.67 | 0 | 0 | 210 | 1/16 |
| 157,500 | 7000 | 3500 | 0 | 0 | 210 | 1/6 |
| 157,500 | 5833.33 | 5833.33 | 0 | 0 | 210 | 1/4 |
| 157,500 | 3141.026 | 11,217.95 | 0 | 0 | 210 | 25/64 |
| 157,500 | −2500 | 22,500 | 0 | 0 | 210 | 9/16 |
| Equation | |
|---|---|
| 9/16 |
| 1/16 | 1/6 | 1/4 | 25/64 | 9/16 | |
|---|---|---|---|---|---|
| 1.046 | 1.179 | 1.303 | 1.609 | 2.246 | |
| 1.07 | 1.2 | 1.33 | 1.64 | 2.285 |
| (MPa) | (MPa) | (MPa) | (N) | (N) | (N) | |
|---|---|---|---|---|---|---|
| 157,500 | 8166.67 | 1166.67 | −105 | 105 | 210 | 1/16 |
| 157,500 | 7000 | 3500 | −105 | 105 | 210 | 1/6 |
| 157,500 | 5833.33 | 5833.33 | −105 | 105 | 210 | 1/4 |
| 157,500 | 3141.026 | 11,217.95 | −105 | 105 | 210 | 25/64 |
| 157,500 | −2500 | 22,500 | −105 | 105 | 210 | 9/16 |
| (MPa) | (MPa) | (MPa) | (N) | (N) | (N) | |
|---|---|---|---|---|---|---|
| 157,500 | 8166.67 | 1166.67 | 105 | −105 | 420 | 1/16 |
| 157,500 | 7000 | 3500 | 105 | −105 | 420 | 1/6 |
| 157,500 | 5833.33 | 5833.33 | 105 | −105 | 420 | 1/4 |
| 157,500 | 3141.026 | 11,217.95 | 105 | −105 | 420 | 25/64 |
| 157,500 | −2500 | 22,500 | 105 | −105 | 420 | 9/16 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pierson, G.; Taghite, M.; Bravetti, P.; Njiwa, R.K. Spherical Indentation of a Micropolar Solid: A Numerical Investigation Using the Local Point Interpolation–Boundary Element Method. Appl. Mech. 2021, 2, 581-590. https://doi.org/10.3390/applmech2030033
Pierson G, Taghite M, Bravetti P, Njiwa RK. Spherical Indentation of a Micropolar Solid: A Numerical Investigation Using the Local Point Interpolation–Boundary Element Method. Applied Mechanics. 2021; 2(3):581-590. https://doi.org/10.3390/applmech2030033
Chicago/Turabian StylePierson, Gaël, M’Barek Taghite, Pierre Bravetti, and Richard Kouitat Njiwa. 2021. "Spherical Indentation of a Micropolar Solid: A Numerical Investigation Using the Local Point Interpolation–Boundary Element Method" Applied Mechanics 2, no. 3: 581-590. https://doi.org/10.3390/applmech2030033
APA StylePierson, G., Taghite, M., Bravetti, P., & Njiwa, R. K. (2021). Spherical Indentation of a Micropolar Solid: A Numerical Investigation Using the Local Point Interpolation–Boundary Element Method. Applied Mechanics, 2(3), 581-590. https://doi.org/10.3390/applmech2030033




