Quantized Approach to Damped Transversal Mechanical Waves
Abstract
1. Introduction
2. The Canonical Description of a Damped Harmonic Oscillator
3. The Damped Oscillator Quantization Procedure
4. Quantization of Damped Transversal Mechanical Waves
4.1. Commutation Rules
4.2. Calculating the Terms of the Hamiltonian
4.3. State Equation of Damped Traveling Quantum Waves
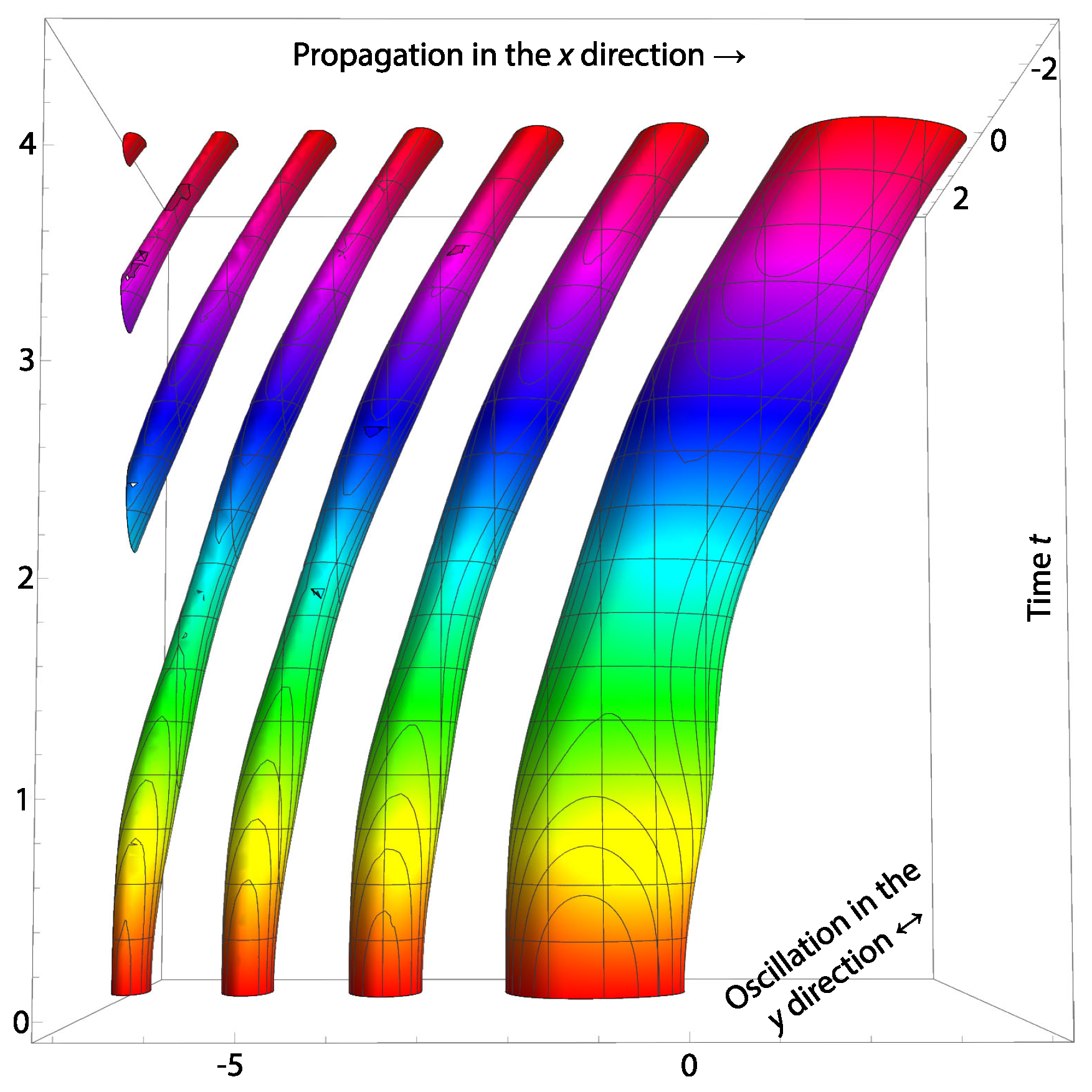
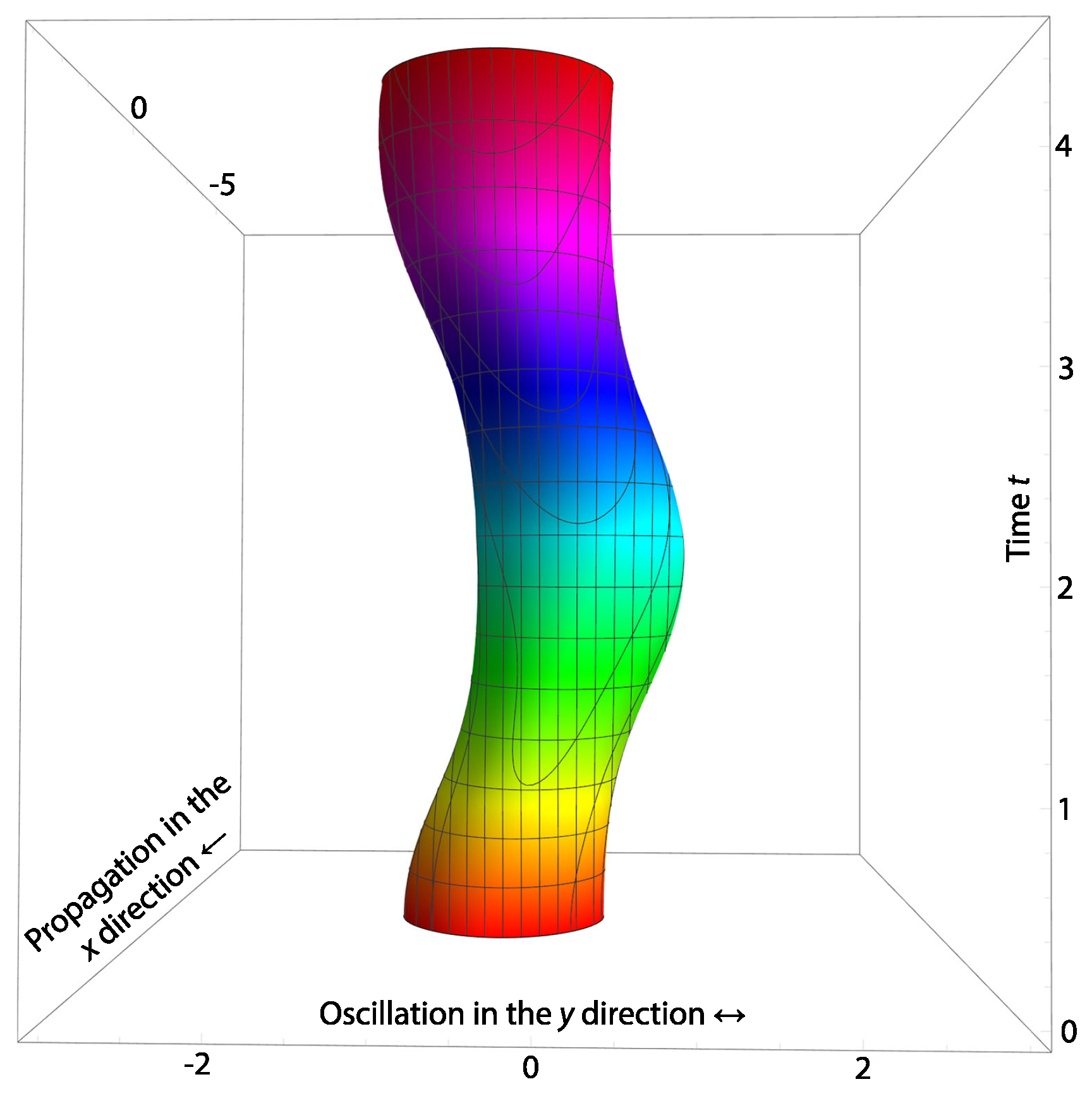
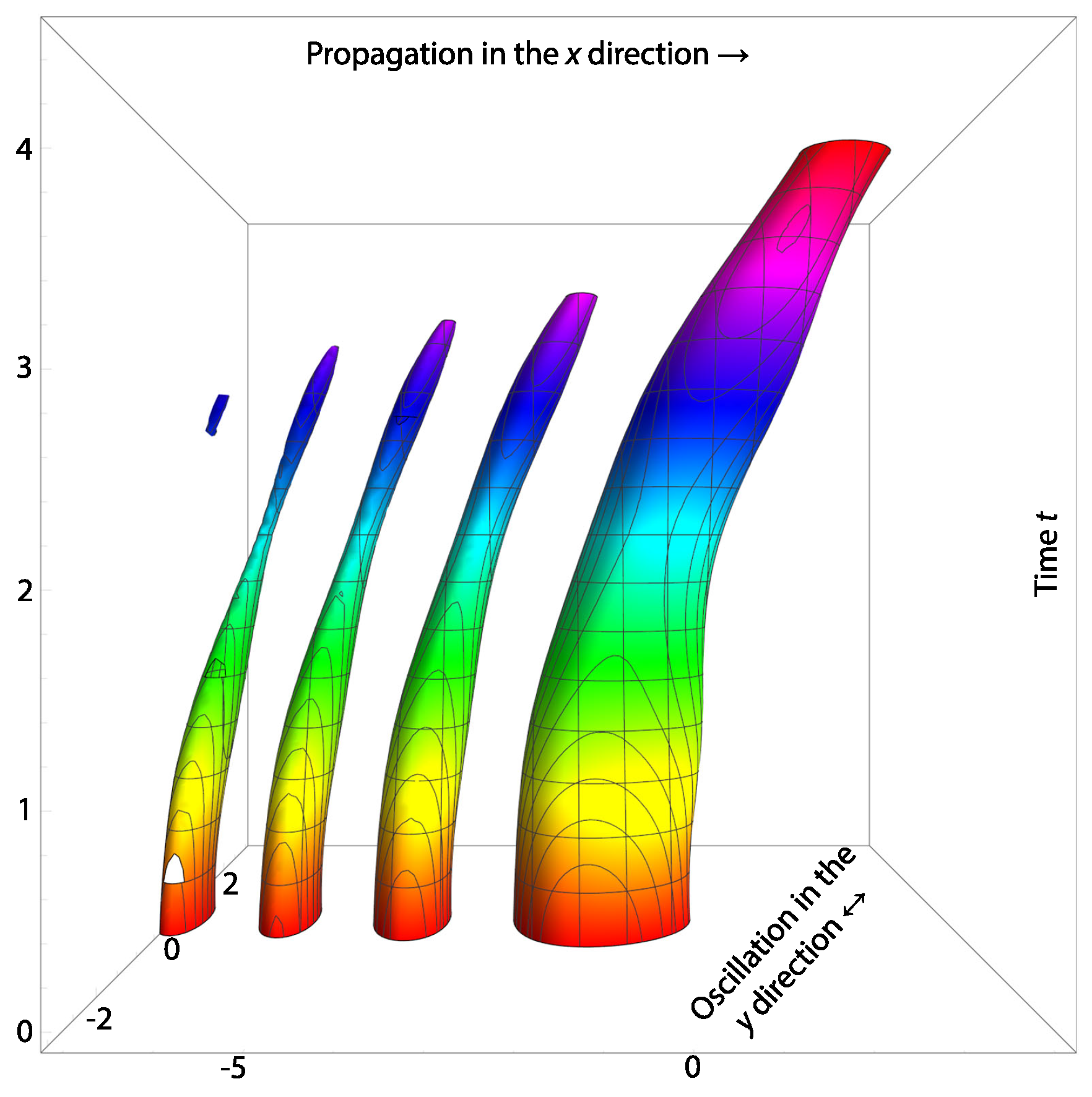
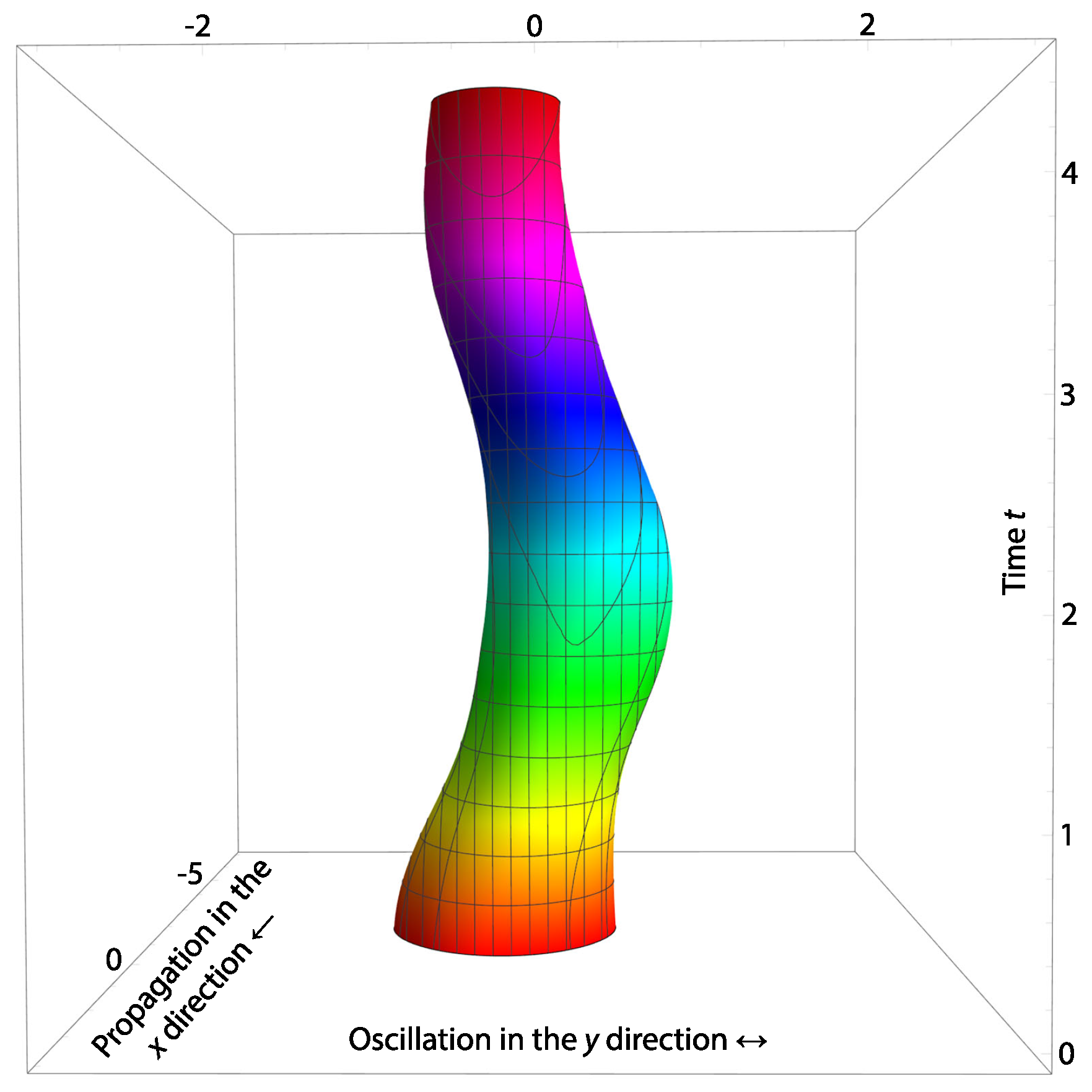
5. Oscillating–Traveling Damped Wave Packet
5.1. The Damped–Oscillating Part
5.2. The Gaussian Spreading Solution for the Free-Propagation Part
5.3. Airy Non-Spreading Solution for the Free-Propagation Part
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Márkus, F.; Gambár, K. Minimum Entropy Production Effect on a Quantum Scale. Entropy 2021, 23, 1350. [Google Scholar] [CrossRef] [PubMed]
- Ithier, G.; Collin, E.; Joyez, P.; Meeson, P.J.; Vion, D.; Esteve, D.; Chiarello, F.; Shnirman, A.; Makhlin, Y.; Schriefl, J.; et al. Decoherence in a Superconducting Quantum Bit Circuit. Phys. Rev. B 2005, 72, 134519. [Google Scholar] [CrossRef]
- Schoelkopf, R.J.; Girvin, S.M. Wiring up Quantum Systems. Nature 2008, 451, 664–669. [Google Scholar] [CrossRef] [PubMed]
- Márkus, B.G.; Szirmai, P.; Edelthalhammer, K.F.; Eckerlein, P.; Hirsch, A.; Hauke, F.; Nemes, N.M.; Chacón-Torres, J.C.; Náfrádi, B.; Forró, L.; et al. Ultralong Spin Lifetime in Light Alkali Atom Doped Graphene. ACS Nano 2020, 14, 7492–7501. [Google Scholar] [CrossRef] [PubMed]
- Márkus, B.G.; Gmitra, M.; Dóra, B.; Csősz, G.; Fehér, T.; Szirmai, P.; Náfrádi, B.; Zólyomi, V.; Forró, L.; Fabian, J.; et al. Ultralong 100 ns Spin Relaxation Time in Graphite at Room Temperature. Nat. Commun. 2023, 14, 2831. [Google Scholar] [CrossRef]
- Csősz, G.; Dóra, B.; Simon, F. Entropy in Spin Relaxation, Spintronics, and Magnetic Resonance. Phys. Status Solidi B 2020, 257, 2000301. [Google Scholar] [CrossRef]
- Csősz, G.; Szolnoki, L.; Kiss, A.; Dóra, B.; Simon, F. Generic Phase Diagram of Spin Relaxation in Solids and the Loschmidt Echo. Phys. Rev. Res. 2020, 2, 033058. [Google Scholar] [CrossRef]
- Mahmoud, A.; Ciubotaru, F.; Vanderveken, F.; Chumak, A.V.; Hamdioui, S.; Adelmann, C.; Cotofana, S. Introduction to Spin Wave Computing. J. Appl. Phys. 2020, 128, 161101. [Google Scholar] [CrossRef]
- Palma, G.M.; Suominen, K.-A.; Ekert, A.K. Quantum Computers and Dissipation. Proc. R. Soc. A 1996, 452, 567–584. [Google Scholar]
- Beke, D.; Valenta, J.; Károlyházy, G.; Lenk, S.; Czigány, Z.; Márkus, B.G.; Kamarás, K.; Simon, F.; Gali, G. Room-Temperature Defect Qubits in Ultrasmall Nanocrystals. J. Phys. Chem. Lett. 2020, 11, 1675–1681. [Google Scholar] [CrossRef]
- Zhou, X.; Koolstra, G.; Zhang, X.; Yang, G.; Han, X.; Dizdar, B.; Li, X.; Divan, R.; Guo, W.; Murch, K.W.; et al. Single Electrons on Solid Neon as a Solid-State Qubit Platform. Nature 2022, 605, 47–50. [Google Scholar] [CrossRef]
- Kollarics, S.; Simon, F.; Bojtor, A.; Koltai, K.; Klujber, G.; Szieberth, M.; Márkus, B.G.; Beke, D.; Kamarás, K.; Gali, A.; et al. Ultrahigh Nitrogen-Vacancy Center Concentration in Diamond. Carbon 2022, 188, 393–400. [Google Scholar] [CrossRef]
- Haken, H. Laser Theory, Encyclopedia of Physics; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1970; Volume XXV/2c. [Google Scholar]
- Haake, F. Springer Tracts Modern Physics; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1973; Volume 66, pp. 98–168. [Google Scholar]
- Caldirola, P. Forze non Conservative Nella Meccanica Quantistica. Il Nuovo C. 1941, 18, 393–400. [Google Scholar] [CrossRef]
- Kanai, E. On the Quantization of the Dissipative Systems. Prog. Theor. Phys. 1948, 3, 440–442. [Google Scholar] [CrossRef]
- Dekker, H. Classical and Quantum Mechanics of the Damped Harmonic Oscillator. Phys. Rep. 1981, 80, 1–110. [Google Scholar] [CrossRef]
- Dittrich, W.; Reuter, M. Classical and Quantum Dynamics; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Weiss, U. Quantum Dissipative Systems; World Scientific: Singapore, 2012. [Google Scholar]
- Leggett, A.J. Quantum Tunneling in the Presence of an Arbitrary Linear Dissipation Mechanism. Phys. Rev. B 1984, 30, 1208. [Google Scholar] [CrossRef]
- Caldeira, A.O.; Neto, A.H.C.; de Carvalho, T.O. Dissipative Quantum Systems Modeled by a Two-level-reservoir Coupling. Phys. Rev. B 1993, 48, 13974. [Google Scholar] [CrossRef] [PubMed]
- da Costa, M.R.; Caldeira, A.O.; Dutra, S.M.; Westfahl, H. Exact Diagonalization of Two Quantum Models for the Damped Harmonic Oscillator. Phys. Rev. A 2000, 61, 022107. [Google Scholar] [CrossRef]
- Choi, J.R. Analysis of Quantum Energy for Caldirola–Kanai Hamiltonian Systems in Coherent States. Results Phys. 2013, 3, 115–121. [Google Scholar] [CrossRef]
- Bagarello, F.; Gargano, F.; Roccati, F. A No-Go Result for the Quantum Damped Harmonic Oscillator. Phys. Lett. A 2019, 383, 2836–2838. [Google Scholar] [CrossRef]
- Bateman, H. On Dissipative Systems and Related Variational Principles. Phys. Rev. 1931, 38, 815. [Google Scholar] [CrossRef]
- Risken, H. The Fokker-Planck Equation: Methods of Solution and Applications, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1989; Appendix A.4; pp. 425–428. [Google Scholar]
- Serhan, M.; Abusini, M.; Al-Jamel, A.; El-Nasser, H.; Rabei, E.M. Quantization of the Damped Harmonic Oscillator. J. Math. Phys. 2018, 59, 082105. [Google Scholar] [CrossRef]
- Serhan, M.; Abusini, M.; Al-Jamel, A.; El-Nasser, H.; Rabei, E.M. Response to Comment on ‘Quantization of the Damped Harmonic Oscillator’. J. Math. Phys. 2019, 60, 094101. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. Path Integral Method for Quantum Dissipative Systems with Dynamical Friction: Applications to Quantum Dots/Zero-dimensional Nanocrystals. Superlattices Microstruct. 2020, 144, 106581. [Google Scholar] [CrossRef]
- Szegleti, A.; Márkus, F. Dissipation in Lagrangian Formalism. Entropy 2020, 22, 930. [Google Scholar] [CrossRef]
- Márkus, F.; Gambár, K. A Potential-Based Quantization Procedure of the Damped Oscillator. Quantum Rep. 2022, 4, 390–400. [Google Scholar] [CrossRef]
- Abers, E.S. Quantum Mechanics; Pearson Education: Upper Saddle River, NJ, USA, 2004. [Google Scholar]
- Airy, G.B. Tides and Waves. In Encyclopaedia Metropolitana (1817–1845), Mixed Sciences. Vol. 3; Rose, H.J., Rose, H.J., Smedley, E., Eds.; B. Fellowes: London, UK, 1841; pp. 241–396. [Google Scholar]
- Siviloglou, G.A.; Broky, J.; Dogariu, A.; Christodoulides, D.N. Observation of Accelerating Airy Beams. Phys. Rev. Lett. 2007, 99, 213901. [Google Scholar] [CrossRef]
- Siviloglou, G.A.; Christodoulides, D.N. Accelerating Finite Energy Airy Beams. Opt. Lett. 2007, 32, 979–981. [Google Scholar] [CrossRef]
- Kondakci, H.E.; Abouraddy, A.F. Diffraction-free Space–Time Light Sheets. Nat. Photonics 2017, 11, 733–740. [Google Scholar] [CrossRef]
- Kondakci, H.E.; Abouraddy, A.F. Airy Wave Packets Accelerating in Space-Time. Phys. Rev. Lett. 2018, 120, 163901. [Google Scholar] [CrossRef]
- Rozenman, G.G.; Zimmermann, M.; Efremov, M.A.; Schleich, W.P.; Shemer, L.; Arie, A. Amplitude and Phase of Wave Packets in a Linear Potential. Phys. Rev. Lett. 2019, 122, 124302. [Google Scholar] [CrossRef]
- Hall, L.A.; Yessenov, M.; Abouraddy, A.F. Arbitrarily Accelerating Space-Time Wave Packets. Opt. Lett. 2022, 47, 694–697. [Google Scholar] [CrossRef]
- Grosman, D.; Sheremet, N.; Pavlov, I.; Karlovets, D. Elastic Scattering of Airy Electron Packets on Atoms. Phys. Rev. A 2023, 107, 062819. [Google Scholar] [CrossRef]
- Rozenman, G.G.; Bondar, D.I.; Schleich, W.P.; Shemer, L.; Arie, A. Observation of Bohm Trajectories and Quantum Potentials of Classical Waves. Phys. Scr. 2023, 98, 044004. [Google Scholar] [CrossRef]
- Ostrogradski, M. Mémoires sur les équations différentielles, relatives au problème des isopérimètres. Mem. Ac. St. Petersbourg 1850, 6, 385. [Google Scholar]
- Dysthe, K.B. Note on a Modification to the Nonlinear Schrödinger Equation for Application to Deep Water Waves. Proc. R. Soc. Lond. A 1979, 369, 105–114. [Google Scholar]
- Craig, W.; Guyenne, P.; Sulem, C. A Hamiltonian Approach to Nonlinear Modulation of Surface Water Waves. Wave Motion 2010, 47, 552–563. [Google Scholar] [CrossRef]
- Guyenne, P.; Kairzhan, A.; Sulem, C.; Xu, B. Spatial Form of a Hamiltonian Dysthe Equation for Deep-Water Gravity Waves. Fluids 2021, 6, 103. [Google Scholar] [CrossRef]
- Márkus, F.; Gambár, K. Derivation of the Upper Limit of Temperature from the Field Theory of Thermodynamics. Phys. Rev. E 2004, 70, 055102(R). [Google Scholar] [CrossRef] [PubMed]
- Márkus, F.; Gambár, K. Symmetry Breaking and Dynamic Transition in the Negative Mass Term Klein–Gordon Equation. Symmetry 2024, 16, 144. [Google Scholar] [CrossRef]
- Gambár, K.; Márkus, F. Hamilton-Lagrange Formalism of Nonequilibrium Thermodynamics. Phys. Rev. E 1994, 50, 1227. [Google Scholar] [CrossRef]
- Gambár, K.; Rocca, M.C.; Márkus, F. A Repulsive Interaction in Classical Electrodynamics. Acta Polytechn. Hung. 2020, 17, 175–189. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics; John Wiley & Sons.: Hoboken, NJ, USA, 1999. [Google Scholar]
- Courant, R.; Hilbert, D. Methods of Mathematical Physics; Interscience: New York, NY, USA, 1966. [Google Scholar]
- Smilga, A.V. Comments on the Dynamics of the Pais–Uhlenbeck Oscillator. SIGMA 2009, 5, 017. [Google Scholar] [CrossRef]
- Motohashi, H.; Suyama, T. Third Order Equations of Motion and the Ostrogradsky Instability. Phys. Rev. D 2015, 91, 085009. [Google Scholar] [CrossRef]
- Razavy, M. Classical and Quantum Dissipative Systems; Imperial College Press: London, UK, 2005; p. 129. [Google Scholar]
- Halász, G.J.; Vibók, Á. Comparison of the Imaginary and Complex Absorbing Potentials Using Multistep Potential Method. Int. J. Quantum Chem. 2003, 92, 168–173. [Google Scholar] [CrossRef]
- Muga, J.G.; Palao, J.P.; Navarro, B.; Egusquiza, I.L. Complex Absorbing Potentials. Phys. Rep. 2004, 395, 357–426. [Google Scholar] [CrossRef]
- Márkus, B.G.; Márkus, F. Quantum Particle Motion in Absorbing Harmonic Trap. Indian J. Phys. 2016, 90, 441–446. [Google Scholar] [CrossRef][Green Version]
- Naqvi, K.R.; Waldenstrøm, S. Revival, Mirror Revival and Collapse may Occur even in a Harmonic Oscillator Wave Packet. Phys. Scr. 2000, 62, 12. [Google Scholar] [CrossRef]
- Berry, M.V.; Balazs, N.L. Nonspreading Wave Packets. Am. J. Phys. 1979, 47, 264–267. [Google Scholar] [CrossRef]
- Chen, K.C.; Dhara, P.; Heuck, M.; Lee, Y.; Dai, W.; Guha, S.; Englund, D. Zero-Added-Loss Entangled-Photon Multiplexing for Ground- and Space-Based Quantum Networks. Phys. Rev. Appl. 2023, 19, 054029. [Google Scholar] [CrossRef]
- Bersin, E.; Sutula, M.; Huan, Y.Q.; Suleymanzade, A.; Assumpcao, D.R.; Wei, Y.-C.; Stas, P.-J.; Knaut, C.M.; Knall, E.N.; Langrock, C.; et al. Telecom Networking with a Diamond Quantum Memory. PRX Quantum 2024, 5, 010303. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Márkus, F.; Gambár, K. Quantized Approach to Damped Transversal Mechanical Waves. Quantum Rep. 2024, 6, 120-133. https://doi.org/10.3390/quantum6010009
Márkus F, Gambár K. Quantized Approach to Damped Transversal Mechanical Waves. Quantum Reports. 2024; 6(1):120-133. https://doi.org/10.3390/quantum6010009
Chicago/Turabian StyleMárkus, Ferenc, and Katalin Gambár. 2024. "Quantized Approach to Damped Transversal Mechanical Waves" Quantum Reports 6, no. 1: 120-133. https://doi.org/10.3390/quantum6010009
APA StyleMárkus, F., & Gambár, K. (2024). Quantized Approach to Damped Transversal Mechanical Waves. Quantum Reports, 6(1), 120-133. https://doi.org/10.3390/quantum6010009




