Systematic Investigation on Surface Diradicals Using Theoretical Models: 2M/MgO and 2M/BaO (M = Cu, Ag, and Au)

Abstract

Diradical character is one of the characteristic quantities of functional open-shell molecules. Prof. Nakano devotedly studied the relationship between diradical character and material properties of open-shell molecules; now, we can use the diradical character as a powerful tool for molecular material designs. It is still unclear how the open-shell molecules are affected by the interaction with the surface although the molecules have been immobilised for device applications. In the present study, the adsorptions of model diradical molecules with s-electrons on the MgO (001) and BaO (001) surfaces are investigated using approximate spin projected density functional theory with plane-wave basis (AP-DFT/plane-wave) to provide a systematic discussion of surface–diradical interactions. The accuracy of AP-DFT/plane-wave was verified by comparisons with the calculated results by NEVPT2. The computational error introduced by DFT calculations on the diradical state (spin contamination error) is reduced by the surface–diradical interaction. In addition, it is shown that (1) the diradical character is amplified by the orbital polarisation effects of oxide ions, and (2) the character decreases when the magnetic orbitals become electron-rich due to electron donation from the surfaces. The two effects are competing; the former is pronounced in Au systems, whereas the latter is pronounced in Ag systems.

1. Introduction

Biradical molecules with two unpaired electrons in degenerate (or quasi-degenerate) orbitals are called diradical molecules. The electrons on the singly occupied molecular orbitals (SOMO) of diradical molecules are affected by strong electronic correlations, and they lead to various material functions (magnetism, non-linear optical properties, electronic conductivity, etc.) [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17]. Furthermore, diradical states often arise around the transition state structures in the dissociation of covalent bonds [1,2,3,4,5,6,7]. However, it is difficult to describe states containing strong electron correlations with single configuration; hence, approximations have been developed and proposed by many researchers to predict the unique functions of diradical molecules [1,7,8,9,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34].

The characteristic quantities of diradical molecules (i.e., diradical character) were defined by the tremendous efforts of quantum chemists [1,6,7,12,13,14,15,30]. Diradical character is the contribution of the two-electron excitation configuration in the ground electronic state. The character has been correlated with the magnetism, optical properties, reactivity and electronic conductivity of diradical molecules [1,6,7,12,13,14,15,30]. Equation (1) is the relation between optical properties and diradical character (y), which is derived by Prof. Nakano and co-workers, and it has been used for experimental measuring the y value [12].

$$y=1-\sqrt{1-{\left({\displaystyle \frac{E_{{\mathrm{S}}_{\mathrm{u}}}-E_{{\mathrm{T}}_{\mathrm{u}}}}{E_{{\mathrm{S}}_{\mathrm{g}}}}}\right)}^2}$$

(1)

$E_{{\mathrm{S}}_{\mathrm{g}}}$ is the energy difference between the singlet excited state and the singlet ground state with g symmetry, and $E_{{\mathrm{S}}_{\mathrm{u}}}$ is the energy difference between the singlet excited state and the singlet ground state with u symmetry. $E_{{\mathrm{S}}_{\mathrm{g}}}$ and $E_{{\mathrm{S}}_{\mathrm{u}}}$ are equivalent to the lowest energy peaks in the two- and single-photon absorption spectrum, respectively. $E_{{\mathrm{T}}_{\mathrm{u}}}$ is the energy difference between the triplet excited state and the singlet ground state, which can be measured by fluorescence or electron spin resonance spectroscopy. The Nakano’s equation transformed the concept in cyberspace into a measurable quantity in physical space; molecular designs by open-shell nature have been dramatically activated.

Attempts have been made to use individual molecules as devices (molecular devices). In molecular devices, functions that originate from the open-shell nature of molecules play an important role [6,7,8,9,10,11,12,13,14,15,16,17,35,36,37,38,39]. To use functional molecules as devices, the molecules should be immobilised in some way; for example, the molecules are adsorbed and aligned on stable substrates [35,36,37,38,39]. The electronic states of atoms and diradicals adsorbed on high-precision surfaces have been observed using scanning tunnelling microscopy [40,41,42,43]. However, the diradical character with surface adsorption has not been measured experimentally yet. Therefore, knowledge of how the diradical state varies in interaction with the surface is insufficient, and an approach from computational chemistry is required.

Density functional theory calculation with plane-wave basis (DFT/plane-wave calculation) is a typical first-principles method that allows high-throughput calculations of solid materials and surface reactions [44,45]. However, single referenced methods such as the Kohn–Sham (KS) DFT and Hartree–Fock (HF) are insufficient for treating molecules with large quantum multiplicity due to static correlations [1,7,8,9,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,45,46]. To calculate the diradical molecules with the single configuration, spin-polarised calculations are performed to obtain broken symmetric (BS) states, and spin contamination errors (SCEs) in the BS states are corrected via spin projections. The SCE correction scheme for DFT/plane-wave calculations has been developed recently (AP-DFT/plane-wave method) [7,32,33,34]. This technological development has made it possible to investigate the influence of surface adsorption on the diradical character of open-shell molecules.

Systematic investigation for surface–diradical interactions will be a promising way to provide new design guidelines for surface-anchored molecular devices. Theoretical investigations on s-electron model diradicals (diatomic Au–Au and H–H) and p-electron diradical molecules (p-benzyne and bisphenarenyl) were performed [47,48,49,50]. At the equilibrium internuclear distance, the Au atoms form a σ-bond using the 6s orbitals and becomes a closed shell singlet molecule. When the Au–Au bond is dissociating, the overlap between 6s orbitals is decay and quasi-degenerate orbitals derived from the 6s orbital are formed; the electronic state is not a closed shell but is a diradical state (open-shell singlet). The diradical state resulting from the dissociation (or formation) of the σ-bond has been investigated in detail using molecular hydrogen (H₂). Nevertheless, discussion under the effects of surface adsorption was not conducted. This is because the interaction of H with solid surfaces is generally large and H on the surfaces is often not radical. On the other hand, it has been confirmed both computationally and experimentally that unpaired electrons of Au atoms remain in the adsorbed state on MgO(001) [51,52,53,54]. Therefore, diradical states will arise during the Au–Au bond dissociation (or formation) process even at the adsorption states, and these diradical states should be influenced by the surface. In a previous study [47], the SCE in the energy curves of Au–Au dissociation on MgO (001) was discussed using the AP-DFT/plane-wave method. However, the diradical character, y value, could not be estimated, as no method had been developed at that time to estimate y values from the results of the DFT/plane-wave method with spin projection. After that, we developed the estimation scheme of y values using the calculated results by DFT/plane-wave [7,34]. In the previous study [48], the Au–Au and H–H models with interatomic distances where their s-orbitals become quasi-degenerate have been discussed to clarify the variations in y and SCE values on reactions of diradical state (in gas) to closed-shell state (on surface), closed-shell state (in gas) to diradical state (on surface), and diradical state (in gas) to diradical state (on surface). Thus, the dependence on the distance between the diradicals and surfaces [48] has been discussed systematically. However, the dependence on the elements has not been investigated yet.

In the present study, surface adsorption effects are investigated for the diatomic Au–Au (2Au), diatomic Ag–Ag (2Ag) and diatomic Cu–Cu (2Cu) models, where the atoms are separated to a distance where they have quasi-degenerate orbitals. The models allow elemental dependence to be elucidated for the surface adsorption s-electron diradicals. The calculated results show that 2Ag and 2Cu models behave differently from the 2Au model, and the difference in elements are discussed to deepen the physics of the interaction between open-shell molecules and solid surfaces.

2. Computational Procedure

2.1. Spin Contamination Error and Diradical Character

In this section, a brief description of SCE and diradical character is given, which is a theoretical basis for open-shell systems. For simplicity, a M–M bond dissociation (or creation) with two s-electrons is used that is the simplest model where SCE occur. Suppose that, under the mean-field approximation, the coefficients of basis sets for the one-electron wave functions ${\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}$ and ${\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}$ corresponding to the highest occupied molecular orbital (HOMO) and lowest unoccupied molecular orbital (LUMO) of the M₂ molecule at equilibrium interatomic distances are found. The spin polarised (spin unrestricted) all-electron wave function ${\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}$ for two M atoms at any interatomic distance is written by the ${\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}$, ${\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}$ and mixing parameter $\mathsf{\theta }$:

$$|{\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}⟩={\displaystyle \frac{1}{\sqrt{2}}}\left|\begin{array}{cc}\mathsf{\alpha }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\mathsf{\alpha }}\left({\mathbf{r}}_1\right)& \mathsf{\beta }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\mathsf{\beta }}\left({\mathbf{r}}_1\right)\\ \mathsf{\alpha }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\mathsf{\alpha }}\left({\mathbf{r}}_2\right)& \mathsf{\beta }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\mathsf{\beta }}\left({\mathbf{r}}_2\right)\end{array}\right|,$$

(2a)

$${\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\mathsf{\alpha }}\left(\mathbf{r}\right)=\cos \mathsf{\theta }{\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left(\mathbf{r}\right)+\sin \mathsf{\theta }{\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left(\mathbf{r}\right),$$

(2b)

$${\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\mathsf{\beta }}\left(\mathbf{r}\right)=\cos \mathsf{\theta }{\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left(\mathbf{r}\right)-\sin \mathsf{\theta }{\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left(\mathbf{r}\right).$$

(2c)

$\mathsf{\alpha }\left({\mathsf{\omega }}_i\right)$ and $\mathsf{\beta }\left({\mathsf{\omega }}_i\right)$ are spin functions and ${\mathsf{\omega }}_i$ is spin coordinates. Subscript i is index for electrons. Then, from Equation (2), we obtained Equation (3):

$$\begin{gathered} |{\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}⟩={\displaystyle \frac{{\mathrm{c}\mathrm{o}\mathrm{s}}^2\mathsf{\theta }}{\sqrt{2}}}\left|\begin{array}{cc}\mathsf{\alpha }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)& \mathsf{\beta }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)\\ \mathsf{\alpha }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)& \mathsf{\beta }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)\end{array}\right|-{\displaystyle \frac{{\mathrm{s}\mathrm{i}\mathrm{n}}^2\mathsf{\theta }}{\sqrt{2}}}\left|\begin{array}{cc}\mathsf{\alpha }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)& \mathsf{\beta }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)\\ \mathsf{\alpha }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)& \mathsf{\beta }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)\end{array}\right| \\ -{\displaystyle \frac{\mathrm{c}\mathrm{o}\mathrm{s}\mathsf{\theta }\mathrm{s}\mathrm{i}\mathrm{n}\mathsf{\theta }}{\sqrt{2}}}\left\{\left|\begin{array}{cc}\mathsf{\alpha }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)& \mathsf{\beta }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)\\ \mathsf{\alpha }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)& \mathsf{\beta }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)\end{array}\right|-\left|\begin{array}{cc}\mathsf{\alpha }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)& \mathsf{\beta }\left({\mathsf{\omega }}_1\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_1\right)\\ \mathsf{\alpha }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{L}\mathrm{U}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)& \mathsf{\beta }\left({\mathsf{\omega }}_2\right){\psi }_{\mathrm{H}\mathrm{O}\mathrm{M}\mathrm{O}}\left({\mathbf{r}}_2\right)\end{array}\right|\right\} \end{gathered}$$

(3)

The defining range of $\mathsf{\theta }$ is $0\le \mathsf{\theta }\le \pi /4$, where $\mathsf{\theta }=0$ corresponds to the equilibrium bond distance and $\mathsf{\theta }=\pi /4$ to the dissociation limit.

The first and second terms in Equation (3) are singlet states, while the third term is a triplet state; hence, the spin polarised Slater determinant ${|\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}⟩$ for M–M dissociation is not an eigenfunction of the spin operator. In general, ${|\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}⟩$ is not an eigenfunction of the spin operator due to the contamination of higher-order spin states although the ‘exact’ all-electron wavefunction of singlet state must be the eigenfunction [46]. The contamination of higher-order spin states is called spin contamination error (SCE), and several correction formulas have been proposed [20,21,22,23,24,25,26,27,28,29,32,33]. The AP-DFT/plane-wave method [32,33] used in this study is explained in Section 2.2.

The diradical character (y) is defined as the contribution of the two-electron excited configuration in the ground electronic state [1,12]. The y values analytically calculated by using the configuration interaction method (CI method), i.e., by adding the contribution of the two-electron excitation configuration to the HF ground state (CID method). It is also clear from Equation (3) that the contribution of the two-electron excitation configuration is included in ${|\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}⟩$ (the second term is the wavefunction of the two-electron excitation configuration). However, the ${\mathsf{\Psi }}_{\mathrm{S}\mathrm{P}}$ contains higher-order spin states (i.e., SCE) and this contribution should be removed; the contribution of triplet states in the biradical system can be removed by spin projection [12,30,55], then,

$$y=1-{\displaystyle \frac{2⟨{\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\alpha \right)}|{\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\beta \right)}⟩}{1+{⟨{\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\alpha \right)}|{\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\beta \right)}⟩}^2}}=1-{\displaystyle \frac{2T}{1+T^2}}.$$

(4)

${\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\alpha \right)}$ and ${\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\beta \right)}$ are the wavefunction corresponding to (SOMOs) for α and β electrons, respectively. The overlap, $⟨{\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\alpha \right)}|{\psi }_{\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\beta \right)}⟩$, is called the effective bond order, T [30]. In this study, spin projected T values are estimated using the electron density calculated by DFT/plane-wave (see Section 2.2).

2.2. Approximate Spin Projection (AP) Method for DFT

When open-shell singlet states are calculated using single referenced methods such as KS–DFT and HF methods, higher-order spin states, which should be orthogonal but not, are contaminated into the wavefunction. In the present study, the correction based on the approximate spin projection (AP) proposed by Yamaguchi et al. [18,19] is used. The AP method corrects the SCE by projecting onto the Heisenberg Hamiltonian of two-site spin system (Equation (5)).

$${\widehat{\mathbf{H}}}_{\mathrm{H}\mathrm{B}}=-2J_{\mathrm{a}\mathrm{b}}{\widehat{\mathbf{S}}}_{\mathrm{a}}{\widehat{\mathbf{S}}}_{\mathrm{b}}.$$

(5)

${\widehat{\mathbf{S}}}_{\mathrm{x}}$ is the spin operator at site x, and $J_{\mathrm{a}\mathrm{b}}$ is the effective integral between sites a and b. The $J_{\mathrm{a}\mathrm{b}}$ value, corrected for SCE by the projection, is given by Equation (6) (the derivation can be found in the previous works by Yamaguchi et al. [19,33]).

$$J_{\mathrm{a}\mathrm{b}}={\displaystyle \frac{E_{\mathrm{S}\mathrm{P}}^{\mathrm{L}\mathrm{S}}-E_{\mathrm{S}\mathrm{P}}^{\mathrm{H}\mathrm{S}}}{{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}^{\mathrm{H}\mathrm{S}}-{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}^{\mathrm{L}\mathrm{S}}}}$$

(6)

Equation (6) is known as the Yamaguchi formula. The subscript “SP” indicates that the value is the spin-polarised state obtained from single referenced methods such as HF and DFT. E is the total energy (eigenvalue for the total electron wavefunction) and $〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉$ is the expectation value of the square of total spin operator (${\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2$). The superscripts “LS” and “HS” refer to the low-spin and high-spin states, respectively. In the calculated models of this study, the LS state corresponds to the open-shell singlet and the HS state to the triplet. Although there are several methods for estimating J_ab values, the Yamaguchi formula is the equation that connects the classical and quantum limits of magnetism and can be widely applied to molecular magnetic calculations [30,31,32,33].

From Equation (6), the total energy of the open-shell singlet state corrected for SCE can be obtained (Equation (7)); Equation (7) is the Yamaguchi formula for total energy [19].

$$E_{\mathrm{A}\mathrm{P}}\left(\mathrm{L}\mathrm{S}\right)=\mathsf{\alpha }{E}_{\mathrm{S}\mathrm{P}}\left(\mathrm{L}\mathrm{S}\right)+(1-\mathsf{\alpha })E_{\mathrm{S}\mathrm{P}}\left(\mathrm{H}\mathrm{S}\right),$$

(7a)

$$\alpha ={\displaystyle \frac{{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}\left(\mathrm{H}\mathrm{S}\right)-{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}\left(\mathrm{L}\mathrm{S}\right)}{{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}\left(\mathrm{H}\mathrm{S}\right)-{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}\left(\mathrm{L}\mathrm{S}\right)}}.$$

(7b)

${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}$ is the eigenvalue of ${\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2$ for the spin-adapted wavefunction, specifically, ${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}\left(\mathrm{H}\mathrm{S}\right)=2$ and ${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}\left(\mathrm{L}\mathrm{S}\right)=0$, in this study. Ways to estimate ${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}$ for the KS–DFT method are still under debate [26,27,28,29]; nevertheless, it has been confirmed that the results obtained from the AP scheme are almost independent of the estimation scheme of ${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}$ [27]. By estimating ${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}$ using the formula proposed by Wang et al. (Equation (8)) [26], the AP method can be applied to calculated results of DFT/plane-wave [32,33].

$${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}=S\left(S+1\right)-{\rho }_{-},$$

(8a)

$$S={\displaystyle \frac{1}{2}}\left(\int {\rho }_{\alpha }\left(\mathbf{r}\right)dr-\int {\rho }_{\beta }\left(\mathbf{r}\right)\mathrm{d}\mathbf{r}\right),$$

(8b)

$${\rho }_{-}=\int {\rho }_{-}\left(\mathbf{r}\right)\mathrm{d}\mathbf{r},$$

(8c)

$${\rho }_{-}\left(\mathbf{r}\right)=\left\{\begin{array}{c}{\rho }_{\alpha }\left(\mathbf{r}\right)-{\rho }_{\beta }\left(\mathbf{r}\right)when{\rho }_{\alpha }\left(\mathbf{r}\right)-{\rho }_{\beta }\left(\mathbf{r}\right)<0\\ 0when{\rho }_{\alpha }\left(\mathbf{r}\right)-{\rho }_{\beta }\left(\mathbf{r}\right)\ge 0\end{array}\right..$$

(8d)

${\rho }_{\alpha }\left(\mathbf{r}\right)$ is the density of electrons with major spin (α electrons) and ${\rho }_{\beta }\left(r\right)$ is that with minor spin (β electrons). We then obtained AP-DFT/plane-wave formula for SCE on biradical system (Equation (9)).

$$SCE=\left(1-{\displaystyle \frac{2}{2+{\rho }_{-}\left(\mathbf{r};\mathrm{L}\mathrm{S}\right)-{\rho }_{-}\left(\mathbf{r};\mathrm{H}\mathrm{S}\right)}}\right)\left(E_{\mathrm{S}\mathrm{P}}\left(\mathrm{L}\mathrm{S}\right)-E_{\mathrm{S}\mathrm{P}}\left(\mathrm{H}\mathrm{S}\right)\right).$$

(9)

${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}$ values of DFT are often calculated by Equation (10), which is an equation derived for HF method [45].

$${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}={〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}+N_{\beta }-\sum _{i,j}^{occ.}{⟨{\psi }_i^{\left(\alpha \right)}|{\psi }_j^{\left(\beta \right)}⟩}^2.$$

(10)

According to Wang et al., Equation (10) is the noninteraction limit of DFT [26]. Here, the difference between the values by Equations (8) and (10) are determined as C_int, and solving the simultaneous equations of ${〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}$ for LS and HS states, we obtained Equation (11):

$$\begin{gathered} T^2=1+\int {\rho }_{-}\left(\mathbf{r};\mathrm{L}\mathrm{S}\right)\mathrm{d}\mathbf{r}+{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{S}\mathrm{P}}(\mathrm{H}\mathrm{S}:\mathrm{c}\mathrm{a}\mathrm{l}\mathrm{c}\mathrm{u}\mathrm{l}\mathrm{a}\mathrm{t}\mathrm{e}\mathrm{d}\mathrm{b}\mathrm{y}\mathrm{Equation}(8\left)\right)-{〈{\widehat{\mathbf{S}}}_{\mathbf{t}\mathbf{o}\mathbf{t}}^2〉}_{\mathrm{e}\mathrm{x}\mathrm{a}\mathrm{c}\mathrm{t}}\left(\mathrm{H}\mathrm{S}\right)- \\ {\sum }_{i,\mathrm{e}\mathrm{x}\mathrm{c}\mathrm{l}\mathrm{u}\mathrm{d}\mathrm{i}\mathrm{n}\mathrm{g}\mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{occ.}\left({⟨{\psi }_i^{\left(\alpha \right)}\left(\mathrm{L}\mathrm{S}\right)|{\psi }_i^{\left(\beta \right)}\left(\mathrm{L}\mathrm{S}\right)⟩}^2-{⟨{\psi }_i^{\left(\alpha \right)}\left(\mathrm{H}\mathrm{S}\right)|{\psi }_i^{\left(\beta \right)}\left(\mathrm{H}\mathrm{S}\right)⟩}^2\right)-{\sum }_{i\ne j}^{occ.}\left({⟨{\psi }_i^{\left(\alpha \right)}\left(\mathrm{L}\mathrm{S}\right)|{\psi }_j^{\left(\beta \right)}\left(\mathrm{L}\mathrm{S}\right)⟩}^2- \\ {⟨{\psi }_i^{\left(\alpha \right)}\left(\mathrm{H}\mathrm{S}\right)|{\psi }_j^{\left(\beta \right)}\left(\mathrm{H}\mathrm{S}\right)⟩}^2\right)+\left({\mathrm{C}}_{\mathrm{i}\mathrm{n}\mathrm{t}}\left(\mathrm{L}\mathrm{S}\right)-{\mathrm{C}}_{\mathrm{i}\mathrm{n}\mathrm{t}}\left(\mathrm{H}\mathrm{S}\right)\right). \end{gathered}$$

(11)

For biradical systems, the T² values are estimated using Equation (11) without any approximations. To estimate the T value using only electron density distribution, we applied following approximation [34]:

$${⟨{\psi }_{i\ne \mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\alpha \right)}\left(\mathrm{L}\mathrm{S}\right)|{\psi }_{i\ne \mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\beta \right)}\left(\mathrm{L}\mathrm{S}\right)⟩}^2\approx {⟨{\psi }_{i\ne \mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\alpha \right)}\left(\mathrm{H}\mathrm{S}\right)|{\psi }_{i\ne \mathrm{S}\mathrm{O}\mathrm{M}\mathrm{O}}^{\left(\beta \right)}\left(\mathrm{H}\mathrm{S}\right)⟩}^2,$$

(12)

$${⟨{\psi }_i^{\left(\alpha \right)}\left(\mathrm{L}\mathrm{S}\right)|{\psi }_j^{\left(\beta \right)}\left(\mathrm{L}\mathrm{S}\right)⟩}^2\approx {⟨{\psi }_i^{\left(\alpha \right)}\left(\mathrm{H}\mathrm{S}\right)|{\psi }_j^{\left(\beta \right)}\left(\mathrm{H}\mathrm{S}\right)⟩}^2,$$

(13)

$${\mathrm{C}}_{\mathrm{i}\mathrm{n}\mathrm{t}}\left(\mathrm{L}\mathrm{S}\right)\approx {\mathrm{C}}_{\mathrm{i}\mathrm{n}\mathrm{t}}\left(\mathrm{H}\mathrm{S}\right).$$

(14)

The T values were approximately estimated using the electron density (Equation (15)), and the y value is calculated from Equation (16) using the electron density obtained from the DFT/plane-wave calculation [7,34].

$$T=\sqrt{1+\int {\rho }_{\mathrm{L}\mathrm{S}}^{-}\left(r\right)dr-\int {\rho }_{\mathrm{H}\mathrm{S}}^{-}\left(r\right)dr}.$$

(15)

$$y=1-{\displaystyle \frac{2\sqrt{1+\int {\rho }_{LS}^{-}\left(r\right)dr-\int {\rho }_{HS}^{-}\left(r\right)dr}}{2+\int {\rho }_{\mathrm{L}\mathrm{S}}^{-}\left(r\right)dr-\int {\rho }_{\mathrm{H}\mathrm{S}}^{-}\left(r\right)dr}}.$$

(16)

In KS–DFT, artificial interactions such as self-interaction errors are included [56]. The HS state is taken as the reference to approximately reduce the effects of the errors (Equation (14)), and fifth and more order spin contaminations are approximately cancelled by Equations (12) and (13). The approximation accuracy has been discussed in previous studies with comparing the conventional scheme confirmed in molecular orbital theory [7,34]. For the s-electron systems, the y values calculated by Equation (16) is in high quantitative agreement [7].

2.3. Method

The AP-DFT/plane-wave calculations were performed using Vienna Ab initio Simulation Package (VASP, version 5.4.4) code [56,57,58,59,60]. The GGA-PBE exchange–correlation functional [61] was used. The core regions of the atoms were treated by the projector augmented plane-wave method [62,63], where the valence electrons were 11 (Au), 11 (Ag), 11 (Cu), 10 (Mg), 10 (Ba) and 6 (O). The cut-off energies for wavefunction and augmented charge were 500 and 2400 eV, respectively. The convergence threshold for the self-consistent field calculation of the electronic state was set at 10^–7 eV, and any symmetries were not applied. The LS and HS states in the present study were fixed at S = 0 and 1, respectively.

In this study, the y values and SCEs in total energies are estimated using approximation (see Section 2.2). Therefore, it is necessary to validate the accuracy. The accuracy of AP-DFT/plane-wave method was discussed using organic radical crystals and one-dimensional complexes for which experimental values of J_ab have been reported [11,33]. The J_ab values calculated by molecular orbital calculations and the experimental values were compared with the results by AP-DFT/plane-wave; it turned out that the J_ab values of AP-DFT/plane-wave method show good agreement with the experimental values and the approximation accuracy of AP-DFT/plane-wave is higher than molecular orbital calculations with AP method [11,33]. This is because the electronic structures are optimised under periodic boundary conditions. Although cluster models could be adopted to calculate the local open-shell electron nature in solids, the cluster models require technical approaches to reduce the effect of artificial edge sites, which makes structural optimisation difficult [64,65,66]. In this respect, the combination of the DFT/plane-wave and AP method, which allows structural optimisation under periodic boundary conditions, has advantages [11,33]. For trioxotriangulen, which is an organic radical with relatively large radical localisation, the calculated values by AP-DFT/plane-wave with GGA-PBE functional agreed with the experimental values to an extremely high accuracy (difference of 10 cm^–1) [11]. Similarly, for p-benzyne, the diradical character can be discussed at the GGA-PBE level [50]. On the other hand, for other organic radicals (bisphenalenyl group), their open singlet states could not be correctly estimated by the GGA-PBE method due to the self-interaction errors [67]. It has been reported that the energy and electronic states of the bisphenarenyls can be corrected by introducing on-site Coulomb parameters and performing spin projection [67]. The J_ab values calculated by the full-CI and AP-DFT/plane-wave methods were compared in a previous study using H–H and H–He–H biradical models [68]. The results of AP-DFT/plane-wave showed good agreement with the full-CI method, even when GGA-PBE was used as the exchange correlation functional; the plane-wave basis calculation with the PBE functional reproduced the results of the full-CI method, second to PBE0. Thus, for s- and p-electron systems, the GGA-PBE functional is sufficient for qualitative discussion of diradicals if the spins are well localised; the calculated values after spin projection are close to the results of the multi referenced wavefunction and experimental values.

For further proof of accuracy of AP-DFT/plane-wave calculations, in the present study, we performed complete active space (CAS) calculations. Multi reference perturbation for a CAS wavefunction with n-electron valence states, NEVPT2, calculations were performed [69,70]. The NEVPT2 calculations were performed by the ORCA programme [71,72]. The basis set used was SDD with corresponding effective core potentials [73,74,75]. The investigated active spaces are 2 orbitals with 2 electrons and 12 orbitals with 22 electrons. Initial guesses are summarised in Figure S1 (Supporting Information).

2.4. Calculated Models

The slabs used in a previous study [7] were adopted as the surface models for MgO (001) and BaO (001). The lattice constants were optimised in bulk, and the slab size was 3 × 3. The specific values of the supercell are summarised in Table S1. The layer thickness of the slab was four atomic layers, and the atoms in the bottom (fourth) layer were fixed during the structural optimisation to represent the bulk structure. The vacuum region (between the first and fourth atomic layers) was 19–20 Å.

The adsorption positions of M atoms (M = Au, Ag and Cu) were on-top of the O anion, the most stable adsorption site for these atoms [47,51,52]. The magnetism of mono-atomically adsorbed Au atoms on the O of MgO (001) was investigated experimentally [53,54], and the magnetic orbital was well investigated by theoretical calculations [7,47,51,52,76]. In this study, in addition to the 2Au/MgO (001), the systems including BaO (001), 2Ag and 2Cu, were also calculated for systematic investigation. The calculated models were visualised by the VESTA programme [77] and are shown in Figure 1. The ground states of these models were open singlet. In Model A, M atoms were adsorbed onto the MgO (001), and in Models B and C, M atoms were adsorbed onto the BaO (001). In this paper, Model X–2M represents that the M (=Au, Ag or Cu) atoms are adsorbed with the structure of model X (=A, B or C). The M–M distance for each model is summarised in

Table 1. Detail of calculated models.

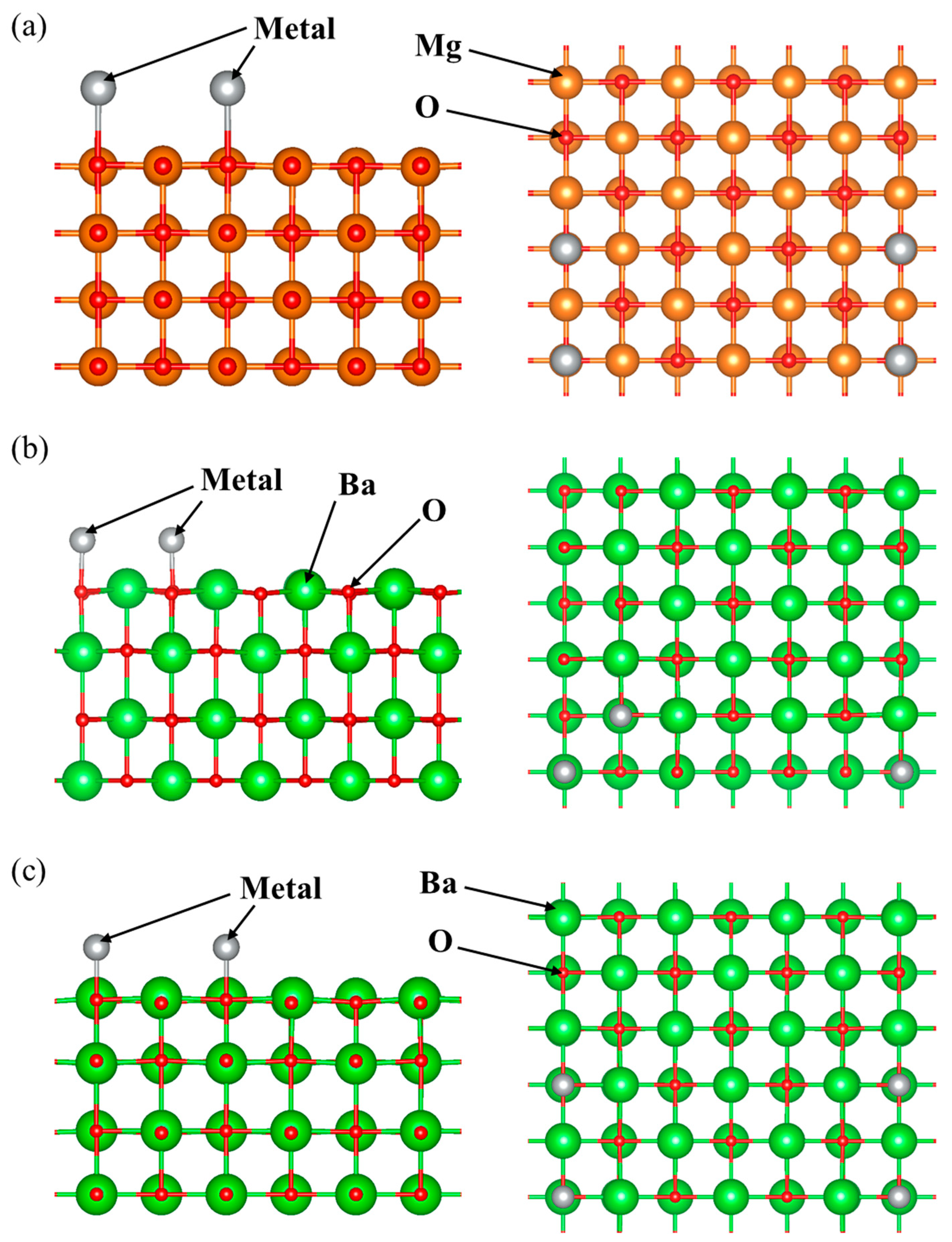
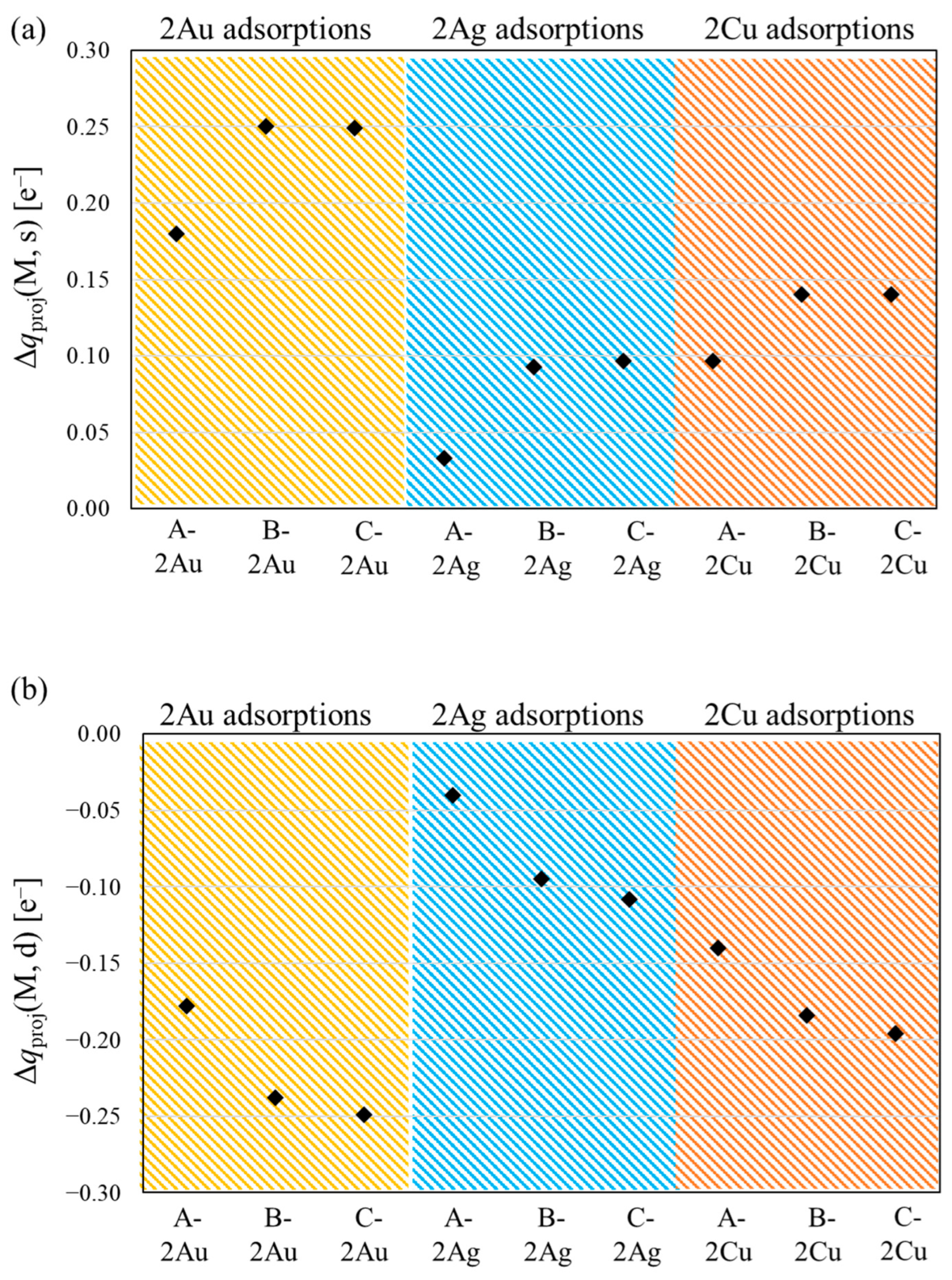
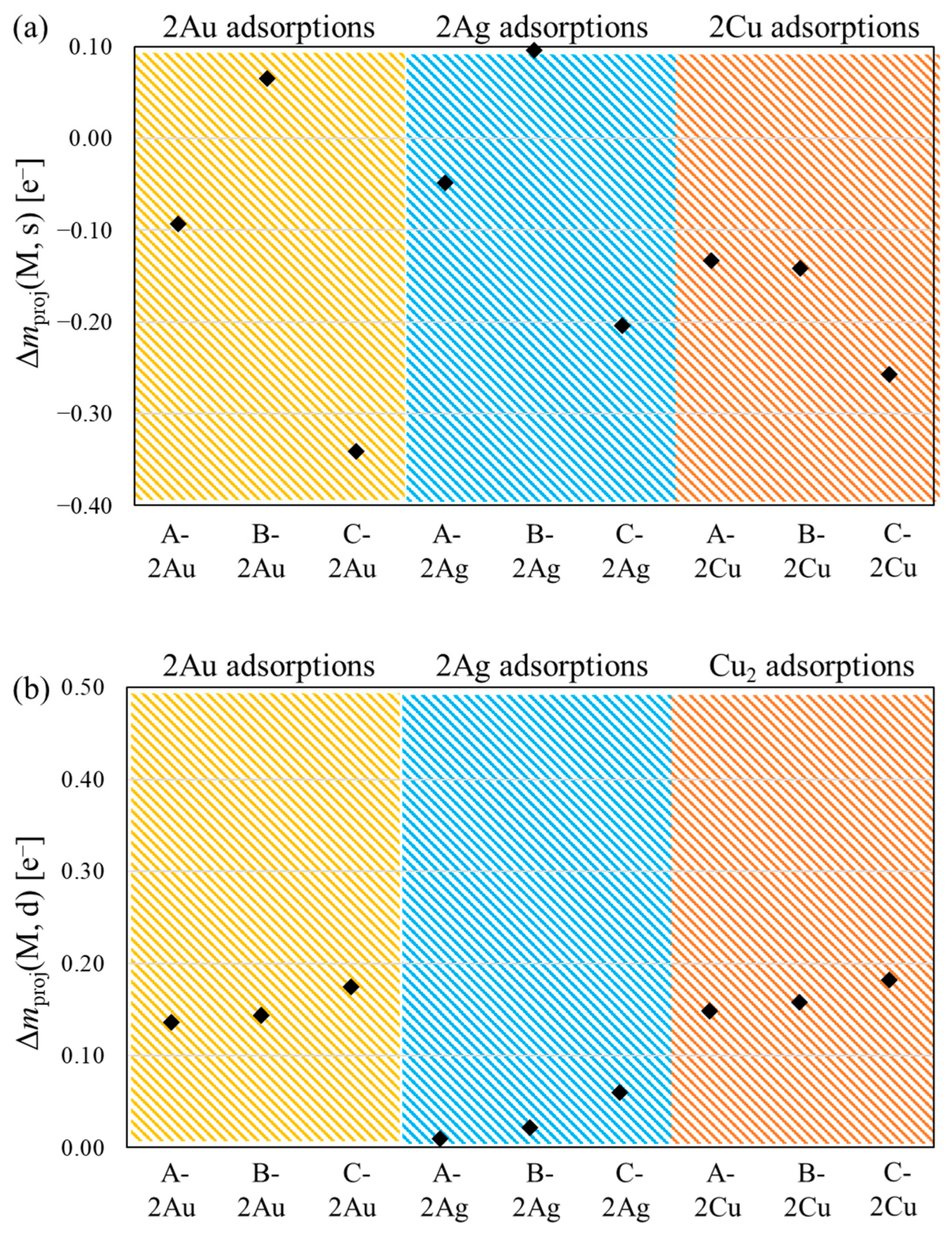
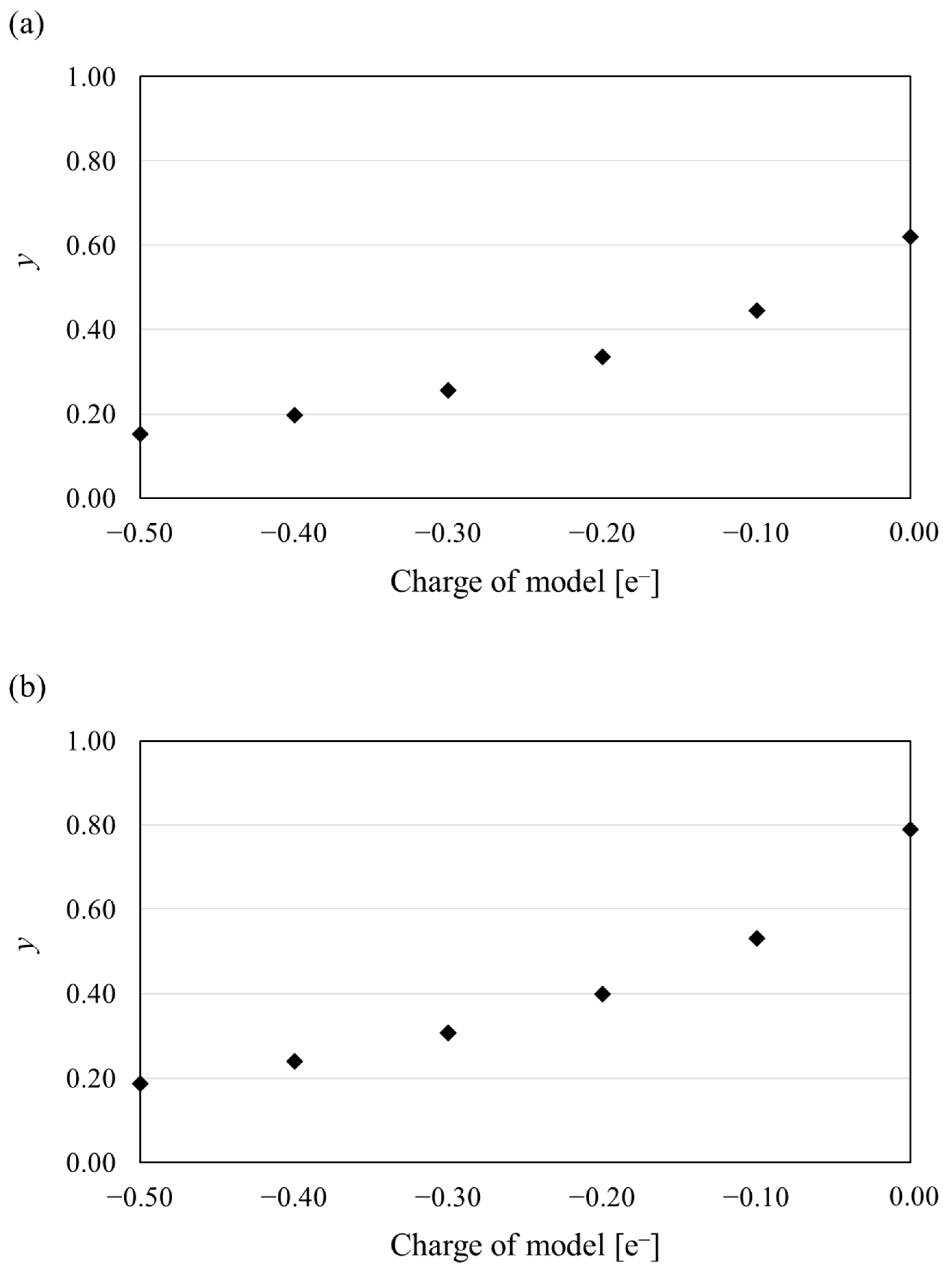
	Model A	Model B	Model C
Surface	MgO (001)	BaO (001)	BaO (001)
M–M distance	4.177	3.929	5.562
Au–O distance	2.301	2.209	2.205
Ag–O distance	2.479	2.257	2.234
Cu–O distance	1.997	1.883	1.881

. The M–M distances in Models A and B are close to each other; hence, we can investigate the differences between the surface nature of MgO and BaO. Conversely, Models B and C have the same surface but different M–M distances. The comparisons between the AP-DFT/plane-wave and NEVPT2 calculations were performed by removing the MgO and BaO slabs from the models in Figure 1. The models without these slabs are denoted X_gas-2M to distinguish them from the models shown in Figure 1. The subscript ‘gas’ indicates that the calculations are in the gas phase, which does not include interactions with the surfaces.

The calculated models in this study are not at the equilibrium of inter-nuclear distances. Therefore, to avoid misunderstandings, we use the term 2M instead of dimer (M₂). In M₂ (the dimer at the equilibrium bond length), stabilisation is obtained by the formation of a bonding orbital originating from the s-electron orbitals; Equation (3) shows the wavefunction focusing on this s-electron orbital interaction. From this equation, it is clear that as the interatomic distance increases from the equilibrium bond length, spin polarisation occurs, and the mixing of triplet states increases. In addition, as the interatomic distance increases, the HOMO–LUMO gap becomes smaller (due to the decrease in stabilisation by the orbital overlap) and the energy difference between singlet and triplet states also decreases. Therefore, in the effect of SCE on energy curves for M–M dissociation (or creation), the increase in M–M distance causes competing effects: increase in triplet contamination and decrease in singlet–triplet energy gap (the former increase the SCE in total energy, but the latter decrease it). For Models A and B, the effects of the former and the latter will be comparable, while for Model C, the latter effect will be more pronounced. Hence, the discussions based on the models will provide fundamental insights on covalent bond dissociation and creation on surfaces.

For M atoms, only the z-coordinate (perpendicular to the surface) is optimised, whereas for surface models, all atomic coordinates except for the atoms in the bottom layer are optimised. The optimised M–O distances are shown in Table 1, and the specific coordinates after geometry optimisation are shown in Tables S2–S10. For sampling of the k-points, Γ-centred 3 × 3 × 1 meshes were used unless otherwise noted.

For estimating adsorption energy (E_ads), M atoms in the gas phase were calculated using a 30 × 30 × 30 ų supercell.

$$E_{\mathrm{a}\mathrm{d}\mathrm{s}}=E\left(2\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)-2E\left(\mathrm{M}\right)-E\left(\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right).$$

(17)

E(M) is the total energy of the isolated atom, and this calculation was performed at the Γ point. E(2M/surf) is the total energy of the 2M adsorbed model shown in Figure 1, and E(surf) is the total energy of the geometry-optimised slab model. E_ads includes the surface distortion energy (E_dis), the 2M-surface interaction energy ($E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}}$), and the M–M interaction energy ($E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}$). E_dis was calculated by Equation (18):

$$E_{\mathrm{d}\mathrm{i}\mathrm{s}}={E\left(\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}-E\left(\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right).$$

(18)

The subscript “fix” indicates that the calculation was performed with the structure fixed after the 2M adsorption, i.e., ${E\left(\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}$, is the total energy obtained by removing only the adsorbed molecule (2M) from the structure of Figure 1 and performing the DFT calculation. Similarly, the total energy obtained by removing only the slab model from the structure of Figure 1, which is identical to X_gas-2M models, and performing the AP-DFT calculation is E(2M)_fix. The M-surface interaction ($E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}}$) can be estimated using the values (Equation (19)):

$$E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}}=E\left(2\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)-E{\left(2\mathrm{M}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}-E{\left(\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}$$

(19)

$E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}$ is calculated by Equation (20) using the total energy obtained by removing only one M atom from the structure in Figure 1 ($E{\left(\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}$).

$$E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}=E\left(2\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)-2E{\left(\mathrm{M}/\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}+E{\left(\mathrm{s}\mathrm{u}\mathrm{r}\mathrm{f}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}$$

(20)

For comparison, the M–M interaction in the gas phase ($E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M},\mathrm{g}\mathrm{a}\mathrm{s}}$) was calculated using Equation (21):

$$E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M},\mathrm{g}\mathrm{a}\mathrm{s}}=E{\left(2\mathrm{M}\right)}_{\mathrm{f}\mathrm{i}\mathrm{x}}-2E\left(\mathrm{M}\right)$$

(21)

3. Results and Discussion

3.1. Comparison with CAS Calculation

Table 2. Energy differences between singlet and triplet states of X_gas-M models in eV. Active space for NEVPTS is 2 orbitals with 2 electrons.

	AP-DFT/Plane-Wave	NEVPT2 (2o, 2e)
Model Agas-2Au	−0.31	−0.12
Model Bgas-2Au	−0.36	−0.27
Model Cgas-2Au	−0.02	0.00
Model Agas-2Ag	−0.39	−0.20
Model Bgas-2Ag	−0.46	−0.31
Model Cgas-2Ag	−0.04	−0.01
Model Agas-2Cu	−0.29	−0.16
Model Bgas-2Cu	−0.40	−0.25
Model Cgas-2Cu	−0.02	−0.01

summarises the energy difference between the singlet and triplet states (E_gap) in the X_gas-2M models, defined as E_gap = E(LS) − E(HS), where the singlet is stable when it is negatively large. The results of AP-DFT/plane-wave and NEVPT2 methods showed complete qualitative agreement. As shown Table 2, the E_gap calculated by the AP-DFT/plane-wave method has larger absolute values compared to those of NEVPT2. This is because the DFT/plane-wave method overestimates the orbital overlap between metal atoms due to self-interaction errors. Excessive orbital overlap in the singlet state leads to an overestimation of the stabilisation from orbital correlations between metal atoms. Therefore, the singlet state is over-stabilised and the absolute value of E_gap is larger in the DFT/plane-wave method. The over-stabilisation due to self-interaction errors is different from the singlet state destabilisation caused by spin contamination: the former is issued on exchange terms and the latter on correlation terms. We will correct the over-stabilisation using the hybrid-DFT or DFT+U methods. Therefore, the combination of the AP method and hybrid-DFT or DFT+U methods will result in a more accurate discussion on magnetism [67]. However, quantitative calculation of magnetism is not the aim of this study. Qualitative agreement is the most important for the research objective of the present study: elucidating the dependence of the open-shell nature of s-electron diradical systems on elementals.

The calculated y values are presented in

Table 3. Diradical characters of X_gas-M models. Two active spaces (2 orbitals with 2 electrons: 2o, 2e; 12 orbitals with 22 electrons: 12o, 22e) were applied to CASSCF calculations.

	AP-DFT/Plane-Wave	CASSCF (2o, 2e)	CASSCF (12o, 22e)
Model Agas-2Au	0.0964	0.6126	0.6100
Model Bgas-2Au	0.0002	0.5127	0.5077
Model Cgas-2Au	0.7428	0.9106	0.9100
Model Agas-2Ag	0.0307	0.4771	0.4745
Model Bgas-2Ag	0.0000	0.3880	0.3861
Model Cgas-2Ag	0.6218	0.8337	0.8323
Model Agas-2Cu	0.2013	0.5173	0.5167
Model Bgas-2Cu	0.0906	0.4347	0.4338
Model Cgas-2Cu	0.7309	0.8415	0.8415

; as with E_gap, the results of the AP-DFT/plane-wave and CASSCF methods are in qualitative agreement. Diradical character is defined as the contribution of the two-electron excitation configuration in the ground electronic state and is obtained by diagonalising the density matrix obtained from the CAS calculation. Therefore, in CAS calculations, the diradical character is related to the occupation number of the highest occupied orbital, n_b: namely, y = 1 − n_b. The n_b value is equivalent to the overlap between the highest spatial orbitals of the α and β electrons; hence, an increase in diradical character indicates that the orbital overlap between metal atoms becomes small [7,30]. Comparing the AP-DFT/plane-wave and CASSCF methods confirms that the diradical character of the AP-DFT/plane-wave method always has smaller values. By applying the AP method, the effect of self-interaction errors in the overestimation of orbital overlaps between metal atoms of DFT/plane-wave has been quantified. Therefore, it is possible to determine from the results in Table 3 the systems that are largely influenced by self-interaction errors: namely, Model B_gas-2Au and B_gas-2Ag. Sometimes, even if symmetry is not used, DFT calculations of highly symmetric systems such as diatomic dissociation may converge to a symmetric closed-shell solution. We performed AP-DFT/plane-wave calculations in addition to the interatomic distances shown in Table 1 to produce plots of orbital overlaps (T; Equation (15)) versus interatomic distances (Figure S2). There is no inflexion point in the plots, and the T values of Au and Ag are approximately 1 at the interatomic distance of Model B (3.9 Å); these results indicate that the DFT method yields almost closed-shell states due to the self-interaction errors in the B_gas-2Au and B_gas-2Ag. In contrast, the open-shell natures of the systems can be confirmed by accurately calculating the electron exchange term and performing multi-configuration calculations. For d-elements, there is a term called double-d-shell effect [78]; to investigate the effect of d-orbitals on diradical character, we performed CASSCF calculation with a large active space (12 orbitals with 22 electrons). As shown the results in Table 3, the effects of d-orbitals are negligible, and the contribution of s-orbitals are large in the calculated models. Thus, the influence of self-interaction errors should be carefully discussed when investigating adsorption effects. Although self-interaction error decreases quantitatively, the results by the AP-DFT/plane-wave method (exchange-correlation functional: GGA-PBE) are qualitatively in agreement with the results by NEVPT2.

3.2. Spin Contamination Error

The calculated energies are summarised in

Table 4. Calculated energies after SCE correction. The values in parenthesis are the SCEs on the calculated energies.

	${\mathit{E}}_{\mathbf{a}\mathbf{d}\mathbf{s}}$ [eV]	${\mathit{E}}_{\mathbf{i}\mathbf{n}\mathbf{t}}^{\mathbf{M}/\mathbf{s}\mathbf{u}\mathbf{r}\mathbf{f}}$ [eV]	${\mathit{E}}_{\mathbf{i}\mathbf{n}\mathbf{t}}^{\mathbf{M}/\mathbf{M}}$ [eV]	${\mathit{E}}_{\mathbf{i}\mathbf{n}\mathbf{t}}^{\mathbf{M}/\mathbf{M},\mathbf{g}\mathbf{a}\mathbf{s}}$ [eV]	${\mathit{E}}_{\mathbf{d}\mathbf{i}\mathbf{s}}$ [eV]
Model A-2Au	−1.831 (0.045)	−1.628 (−0.058)	−0.050 (0.046)	−0.349 (0.104)	0.145 (0.000)
Model B-2Au	−3.671 (0.050)	−3.606 (0.030)	0.020 (0.051)	−0.434 (0.019)	0.369 (0.000)
Model C-2Au	−3.559 (0.004)	−3.855 (−0.005)	0.086 (0.004)	−0.018 (0.008)	0.315 (0.000)
Model A-2Ag	−1.146 (0.073)	−0.769 (−0.021)	−0.317 (0.072)	−0.412 (0.094)	0.035 (0.000)
Model B-2Ag	−2.294 (0.092)	−2.102 (0.092)	−0.248 (0.092)	−0.488 (0.000)	0.296 (0.000)
Model C-2Ag	−1.992 (0.021)	−2.230 (0.001)	0.040 (0.021)	−0.043 (0.020)	0.281 (0.000)
Model A-2Cu	−1.968 (0.068)	−1.797 (−0.056)	−0.127 (0.069)	−0.326 (0.125)	0.155 (0.000)
Model B-2Cu	−3.683 (0.080)	−3.664 (−0.056)	−0.081 (0.079)	−0.457 (0.135)	0.439 (0.000)
Model C-2Cu	−3.480 (0.013)	−3.833 (0.002)	0.074 (0.013)	−0.024 (0.011)	0.378 (0.000)

, consisting of energies after correction for SCE using the AP method. The J_ab values with correction on SCE are summarised in Supporting Information (Table S11). All calculated values of $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M},\mathrm{g}\mathrm{a}\mathrm{s}}$ are negative; this indicates that orbital interactions occur in the calculated M–M distances (although the stabilisation is below 0.05 eV in Model C), and the calculated models are not in the classical magnetic limit. As shown by the calculated values of $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}$, positive values can be confirmed for several models. This is due to the slightly ionic nature of the M atoms on the surface, which results in electrostatic repulsion between the M–M atoms. The effects of the charge transfer are discussed in Section 3.3.

The numbers in parenthesis in Table 4 are the specific values of SCE, and positive values indicate that the estimated energy is artificially unstable due to the SCE. E_dis does not include the energy of the open-shell singlet; hence, the effects of SCE are zero. The other four energies ($E_{\mathrm{a}\mathrm{d}\mathrm{s}}$, $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{a}\mathrm{d}\mathrm{s}}$, $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}$, and $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M},\mathrm{g}\mathrm{a}\mathrm{s}}$) were affected by SCE. Among these four energies, the former three energies ($E_{\mathrm{a}\mathrm{d}\mathrm{s}}$, $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{a}\mathrm{d}\mathrm{s}}$, and $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}$) are related to the surface adsorption state and are investigated at first. Comparing the absolute values of the SCE among the elements, the SCE of the model containing 2Ag is relatively large. The largest SCE was 0.092 eV, which was identified in Model B-2Ag. However, the SCEs at these three energies are small, with absolute values below 0.1 eV. In contrast, for the gas phase (i.e., the SCEs on $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M},\mathrm{g}\mathrm{a}\mathrm{s}}$), some values are above 0.1 eV. Except for two models (Model B-2Au and Model B-2Ag), the absolute value of the SCE for the surface adsorption system did not exceed the absolute value of the SCE for the gas phase system.

The decrease in SCE by surface adsorption has been confirmed in previous studies, and the mechanism has been discussed in detail [32,47,48,76]. Concisely, the molecular-surface interactions decrease the energy difference between the open-shell singlet and triplet states, resulting in smaller SCE. Table 4 shows that the absolute values of $E_{\mathrm{a}\mathrm{d}\mathrm{s}}$ and $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{a}\mathrm{d}\mathrm{s}}$ are relatively small in systems containing 2Ag, and the differences between $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M}}$ and $E_{\mathrm{i}\mathrm{n}\mathrm{t}}^{\mathrm{M}/\mathrm{M},\mathrm{g}\mathrm{a}\mathrm{s}}$ of these systems are also small. This indicates that the interactions between 2Ag and the surfaces are smaller than in other systems (2Au and 2Cu) and is the reason behind the relatively large value of SCE in the Model X-2Ag system.

In Model B-2Au and B-2Ag, the orbital overlap in the gas phase is large due to the close distance between the metal atoms (Figure S2). Therefore, the contribution of the closed-shell singlet is significant, and in Model B-2Ag, the ground state in the gas phase is a closed-shell singlet (Figure S2). Closed-shell singlet states are not affected by SCE, as they have no open-shell electrons. Hence, in these two systems, the SCE in the surface-adsorbed state is larger than in the gas-phase system because of the change from closed-shell singlet (no SCE) to open-shell singlet (including SCE) due to surface adsorption. Because this closed-shell structure is artificial due to the self-interaction error (see Section 3.1), when the self-interaction errors are corrected by another approach such as hybrid-DFT, we will confirm the SCE reduction caused by surface interaction even in Models B-2Au and B-2Ag.

3.3. Diradical Character

In the SCE, no qualitative differences were detected between 2Au, 2Ag and 2Cu systems. Therefore, the discussions and results reported in previous studies [47,48] will be a theoretical basis for the relationship between surface–diradical interactions and SCE. However, apparent differences in the y value were detected; Figure 2 shows the difference in diradical character before and after adsorption (Δy). Δy > 0 indicates that the y value of the 2M molecule is amplified by the adsorption, while Δy < 0 indicates the y value is reduced. When s-electron diradicals are adsorbed on oxides, the polarisation effect due to the p orbital of O weakens the M–M bond, amplifying the y value [7,48]. However, in some models (specifically, Model A-2Ag, C-2Ag and C-2Cu), a significant amplification of the y value (Δy > 0) was not observed; hence, these three systems will have different mechanisms from one derived in the previous studies.

In diradical amplification by orbital polarisation, the d-electrons are promoted to the s-orbital, which was the original magnetic orbital. This indicates that the electronic state becomes closer to d⁹s² instead of d¹⁰s¹ by the interaction with the oxide ion [32,47,51,52,53,54,76]. When comparing the s- and d-electron orbitals, the d-electron orbital has a higher orbital localisation, which amplifies the y value. To confirm this d¹⁰s¹→d⁹s² change, the projected charge and local magnetic moment for the azimuthal quantum number l of atom i were estimated by Equations (22) and (23) [79].

$$q_{\mathrm{p}\mathrm{r}\mathrm{o}\mathrm{j}}\left(i,l\right)={\displaystyle \frac{1}{N_{\alpha ,ik}}}{\sum }_{nk}^{\mathrm{o}\mathrm{c}\mathrm{c}.}{f}_{\alpha ,nk}{\sum }_{m=-l}^l{\left|⟨Y_{\alpha ,ilm}|{\phi }_{\alpha ,ink}⟩\right|}^2+{\displaystyle \frac{1}{N_{\alpha ,ik}}}{\sum }_{nk}^{\mathrm{o}\mathrm{c}\mathrm{c}.}{f}_{\alpha ,nk}{\sum }_{m=-l}^l{\left|⟨Y_{\alpha ,ilm}|{\phi }_{\alpha ,ink}⟩\right|}^2.$$

(22)

$$m_{\mathrm{p}\mathrm{r}\mathrm{o}\mathrm{j}}\left(i,l\right)={\displaystyle \frac{1}{N_{\alpha ,ik}}}{\sum }_{nk}^{\mathrm{o}\mathrm{c}\mathrm{c}.}{f}_{\alpha ,nk}{\sum }_{m=-l}^l{\left|⟨Y_{\alpha ,ilm}|{\phi }_{\alpha ,ink}⟩\right|}^2-{\displaystyle \frac{1}{N_{\alpha ,ik}}}{\sum }_{nk}^{\mathrm{o}\mathrm{c}\mathrm{c}.}{f}_{\alpha ,nk}{\sum }_{m=-l}^l{\left|⟨Y_{\alpha ,ilm}|{\phi }_{\alpha ,ink}⟩\right|}^2$$

(23)

N_k and f_k represent the number of bands and occupancies at wavenumber k, respectively. Y is the spherical harmonic function. The subscripts n, l and m represent the principal quantum number, azimuthal quantum number and magnetic quantum number, respectively. In this definition, q_proj corresponds to the approximation of the number of valence electrons, where a more positive q_proj indicates an electron-rich state (the valence charge of the atom is more negative). m_proj > 0 indicates the atom has major spin, while atoms with m_proj < 0 have minor spin. Differences in local projected charges of M atom (M = Au, Ag and Cu) before and after their 2M adsorptions (Δq_proj) are shown in Figure 3, and differences in local projected magnetic moments of M atom (M = Au, Ag and Cu) before and after their 2M adsorptions (Δm_proj) are shown in Figure 4.

The value of Δq_proj(d) was negative for all M (Figure 3b). This indicates that the number of d-electrons in the 2M molecule decreased due to adsorption. The value of Δq_proj(s) confirmed that the number of s-electrons increased in correspondence with the decrease in d-electrons. Therefore, the change from d¹⁰s¹ to d⁹s² was detected for all M. However, there is a difference in the amount of change: the change is more pronounced for 2Au, whereas the change is minimal for 2Ag; for 2Cu, the change is intermediate between 2Au and 2Ag.

Due to the shift in the number of d-electrons from 10, the value of Δm_proj(d) is positive for all M, whereas Δm_proj(s) is negative except for two models (Model B-2Au, and B-2Ag). These changes of Δm_proj(d) and Δm_proj(s) correspond to the changes of Δq_proj(d) and Δq_proj(s); the reason why Δm_proj(s) is not negative in Model B-2Au and B-2Ag is the same as the reason for the increasing SCE as discussed in Section 3.2. In other words, because the contribution of closed-shell is large in the gas phase models, the isolated 2Au and 2Ag in the models have a minimal localised spin on the s-orbitals, and the value of m_proj(s) before adsorption is close to zero. Therefore, the positive Δm_proj(s) identified for Models B-2Au and B-2Ag is a GGA-PBE artefact, and this artefact does not affect the results for Models A-2Ag, C-2Ag and C-2Cu. The mechanism by which the diradical increase does not occur in these models is not an artefact derived from self-interaction errors.

The results shown in Figure 3 and Figure 4 confirm the electron localisation to the d-orbitals with the change from d¹⁰s¹ to d⁹s² in all 2M molecules. However, the polarisation effect by oxide ions on the 2Ag is small, which prevented a significant amplification of the y value. The reason for the weak polarisation of Ag is due to the weak orbital correlation between Ag–O, which is confirmed by the values of E_ads and E_int in Table 4; these absolute values are ca. 1 eV smaller than those of Au and Cu. The small orbital polarisation of Ag explains why the variation in y value in Model A-2Ag is close to zero; however, the decrease in y values by the adsorptions that were confirmed in the Model C-2Ag and Model C-2Cu cannot be explained.

The previous study argued that when the Au atoms in the 2Au interact with the cation and anion on the surface, the Au–Au interaction is affected by the ion term, whose contribution is negligible in the gas phase [47]. The contribution of the ion term is a factor that reduces the y value because the Au ions (Au⁺ and Au^–) has no unpaired electrons in the electronic structure. When the Au, Ag and Cu atoms are adsorbed on oxide ions, the atoms become slightly negative [32,47,51,52,53,54,76]. If the contribution of the counterion term is significant, a difference in the number of electrons on the atoms will be detected when comparing the results of monoatomic adsorption (the result of removing one adsorbed atom from the diatomic (2M) adsorption structure, fixing the geometry and optimising the electronic state) with the diatomic (2M) adsorption results. Specifically, the number of electrons on M of the diatomic (2M) adsorption is smaller than that of monomer adsorption due to the contribution of a cation term. Then, the number of electrons on the M atom was estimated by Bader charge analysis [80,81,82,83] and summarised in

Table 5. The number of electrons on the adsorbed M atom estimated Bader algorithm.

	Diatomic Adsorption	Mono Atom Adsorption	Difference *
Model A-2Au	11.254	11.290	–0.036
Model B-2Au	11.352	11.383	–0.031
Model C-2Au	11.358	11.373	–0.015
Model A-2Ag	11.083	11.103	–0.020
Model B-2Ag	11.175	11.211	–0.030
Model C-2Ag	11.189	11.206	–0.016
Model A-2Cu	11.091	11.105	–0.013
Model B-2Cu	11.141	11.167	–0.026
Model C-2Cu	11.174	11.189	–0.015

* Negative value indicates the diatomic adsorption is electron-deficient than the mono atom adsorption.

. However, the difference is minimal, with less than 0.03 electrons in Model C-2Ag and Model C-2Cu. This implies that the ion term affects the electronic states of the diatomic (2M) adsorptions but is not the main reason behind the decrease in the y value.

As shown in Figure 4, the Δm_proj(s) is significantly reduced in Models C. In 2Ag, despite the small change in d¹⁰s¹→d⁹s², the reduction in the Δm_proj(s) in Model C is significant. It is assumed from these results that the localised electrons on the s-electrons become delocalised, which has reduced their diradical character. The delocalisation factor is that the metal atoms are slightly negatively charged when adsorbed. The M atoms interact with the valence band (O p-band), which indicates that the localised electrons on the M atoms are partially delocalised to the oxide bands. In addition, the electron density between the M atoms will increase as the magnetic orbitals become electron rich. This increase in electrons on the adsorbed M atom is confirmed by the results shown in Table 5; the estimated values exceed the valence electron number of the M atoms, 11. Hence, the electron richness of the 2M will be the reason for the decrease in the y value. To verify this hypothesis, the charge dependence of the y values of the Model C_gas-2Ag, which is the model removed BaO from C-2Ag, was calculated by varying the valence charge from 0 to –0.5. The valence charge was varied by adding a background charge [84]. The y value decreases as the 2Ag valence charge becomes more negative (Figure 5a). The electron delocalisation is therefore the main factor that causes diradical decrease in the Model C-2Ag and C-2Cu.

It was confirmed that the y value of the diradical molecule decreases as it becomes electron rich. This decrease in the y value caused by the increase in electron richness is a competing effect with the increase in the y value caused by the orbital polarisation. The former effect is more pronounced in the 2Ag system, whereas the latter is more pronounced in the 2Au system. This result suggests that the y value could be adjusted by artificially upsetting their balance. In other words, the y value could be adjusted by artificially varying the number of electrons for systems with amplificated y value by the surface adsorption (e.g., Model C-2Au). Figure 5b shows the validation results: before changing the number of electrons, surface adsorption amplified the y value, whereas as the system became electron rich, the y value started to decrease. Therefore, it can be concluded that the open-shell electronic structure of the diradical molecule can be tuned by combining the diradical amplification by the orbital polarisation, which is caused by molecule–surface interaction, with the diradical reduction by variation in the number of electrons, which will be obtained from the application of an electric field or the formation of heterointerfaces with different Fermi energies.

4. Conclusions

The electronic states of s-electron diradical molecules were systematically investigated using the AP-DFT/plane-wave method to determine whether the interaction with stable ionic crystal surfaces varies the diradical states. The investigated models were the adsorptions of 2Au, 2Ag and 2Cu onto MgO (001) and BaO (001). The elemental dependence of SCE is explained by the mechanism proposed in our previous studies [47,48]. The mechanism shows that surface adsorption decreases the absolute value of SCE, but the region of interatomic distances where the SCE occurs become wider; the results of the present study revealed this rule holds, in general, for s-electron diradicals. In contrast, with regard to diradical character, a conclusion was obtained that was not reached in the discussion in the previous studies.

The AP-DFT/plane-wave calculations revealed the following: (1) the diradical character increases when the proportion of d-electrons in the magnetic orbitals increases due to orbital polarisation effects caused by oxide ions, and (2) the diradical character decreases when the magnetic orbitals become electron rich due to electron donation from oxide ions. The diradical amplifying (1) and reducing (2) effects compete. The effect (1) is more pronounced in 2Au, whereas the effect (2) is more pronounced in 2Ag; 2Cu exhibits a behaviour intermediate between 2Au and 2Ag. In this study, the correction for the exchange term is not performed. The balance of effects (1) and (2) will depend on the exchange term of DFT. For quantitative investigation, the correction scheme for the exchange term should be optimised. Comparison with the experimental values is best for the optimisation, but the quantitative investigation on the surface–diradical state from experimental is still difficult.

The presented study is just a model calculation, and it would be difficult to prepare the same system. However, the orbital polarisation effects which can be identified in the s-electron model system have a significant contribution to the interaction between real diradical molecules and surfaces [50], and the model calculations in this study have fundamental physicochemical significance towards the systematisation of surface–diradicals. The present conclusion, “the diradical enhancing effect by orbital polarisation competes with the diradical reducing effect by electron donation”, will contribute to the control of surface adsorbed diradical states. For example, it would be possible that, after amplifying the diradical character by orbital polarisation from the surface, the diradical character would be adjusted to the desired values using the latter effect (e.g., applying an electric field). The development of a technique for combining the electric field application method with the AP-DFT/plane-wave method and application as a candidate for molecular devices is currently underway. The development of this technique will establish a new guideline for the design of molecular devices: surface-induced diradical tuning.