On the 3D → 2D Isomerization of Hexaborane(12)
Abstract
:1. Introduction
2. Computational Methods
3. Results
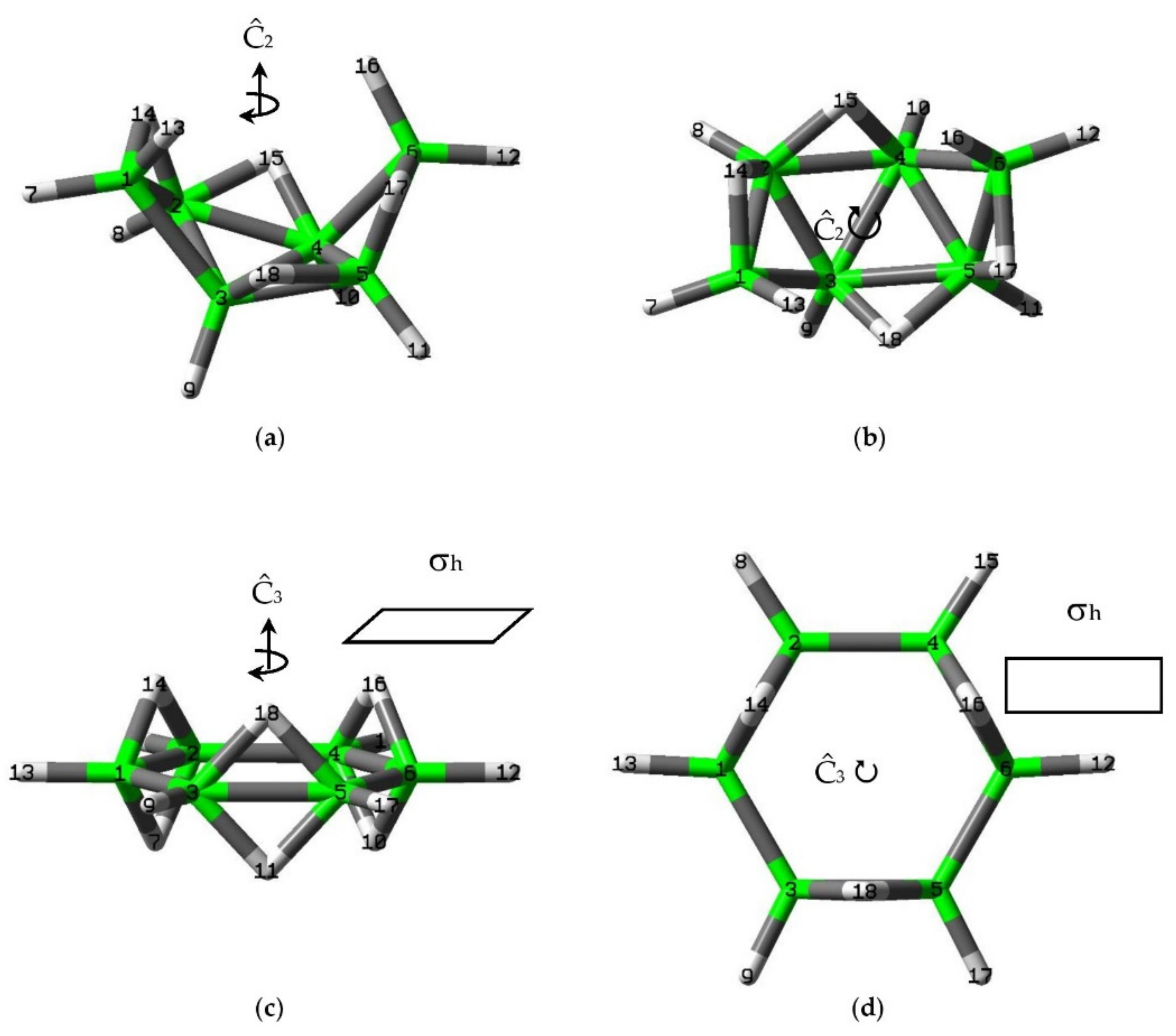
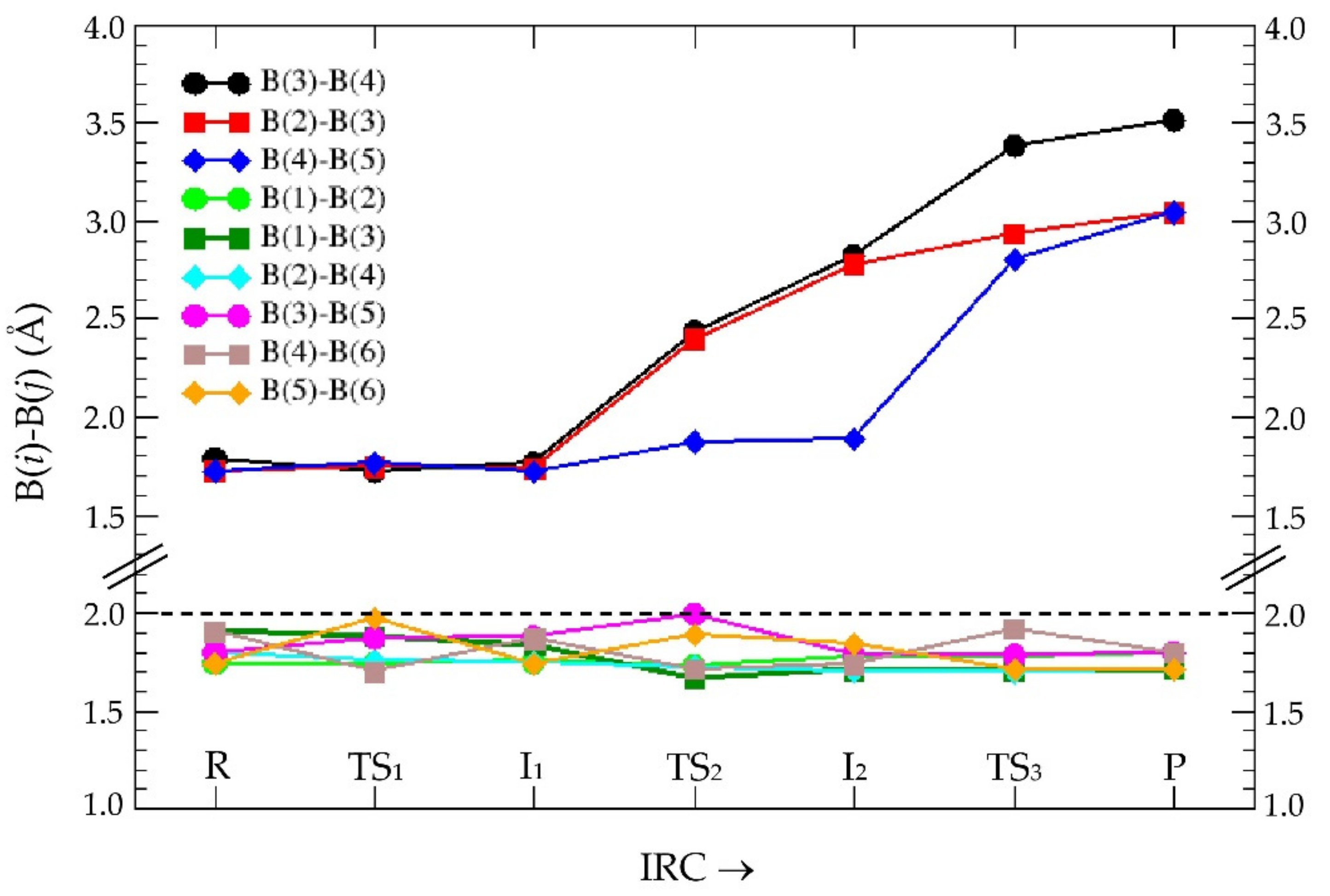
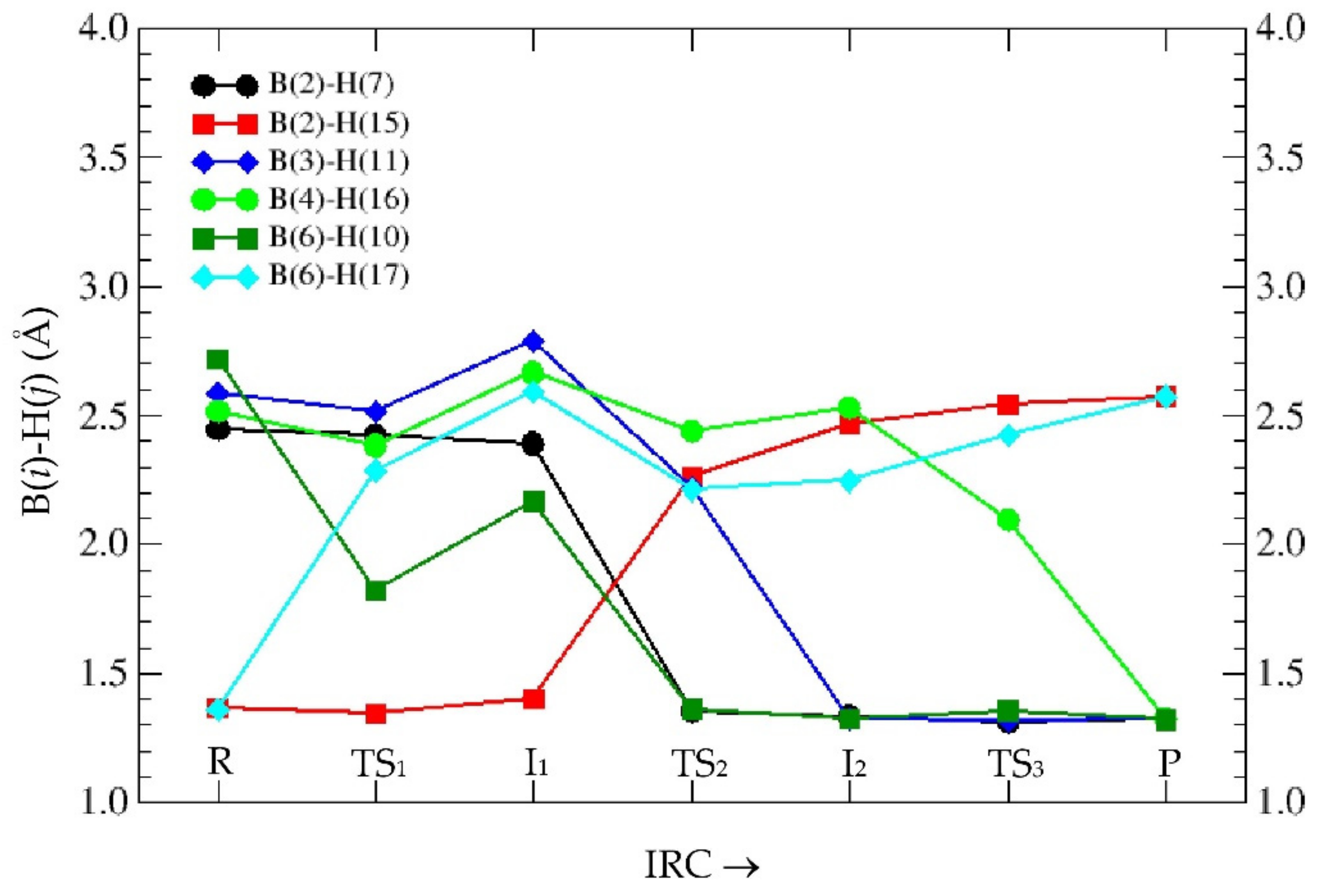
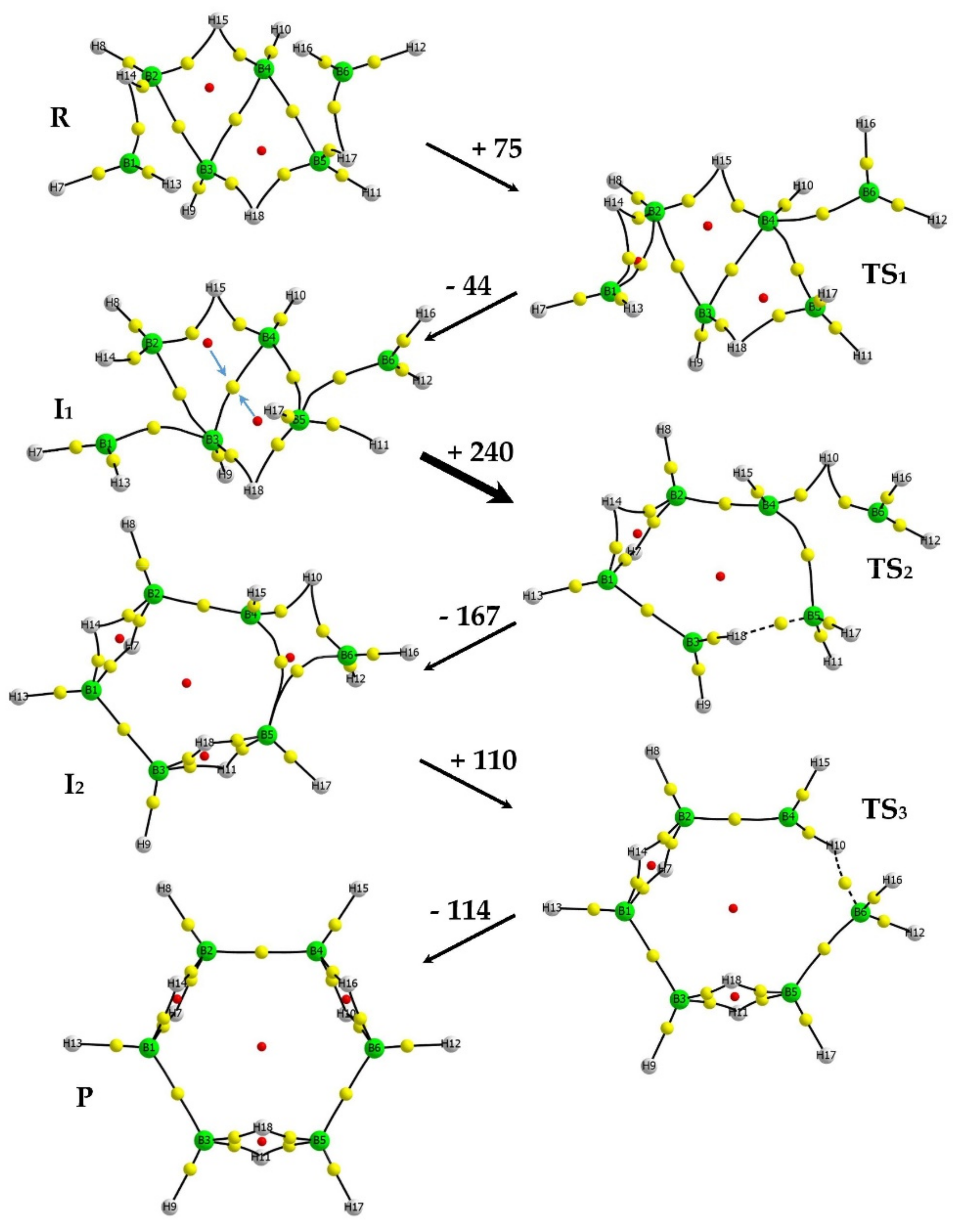
3.1. Intrinsic-Reaction-Coordinate (IRC) and Stationary Points in the 3D → 2D Isomerisation of Hexaborane(12)
3.2. QTAIM Analysis of the Stationary Points in the 3D → 2D Isomerisation of Hexaborane(12)
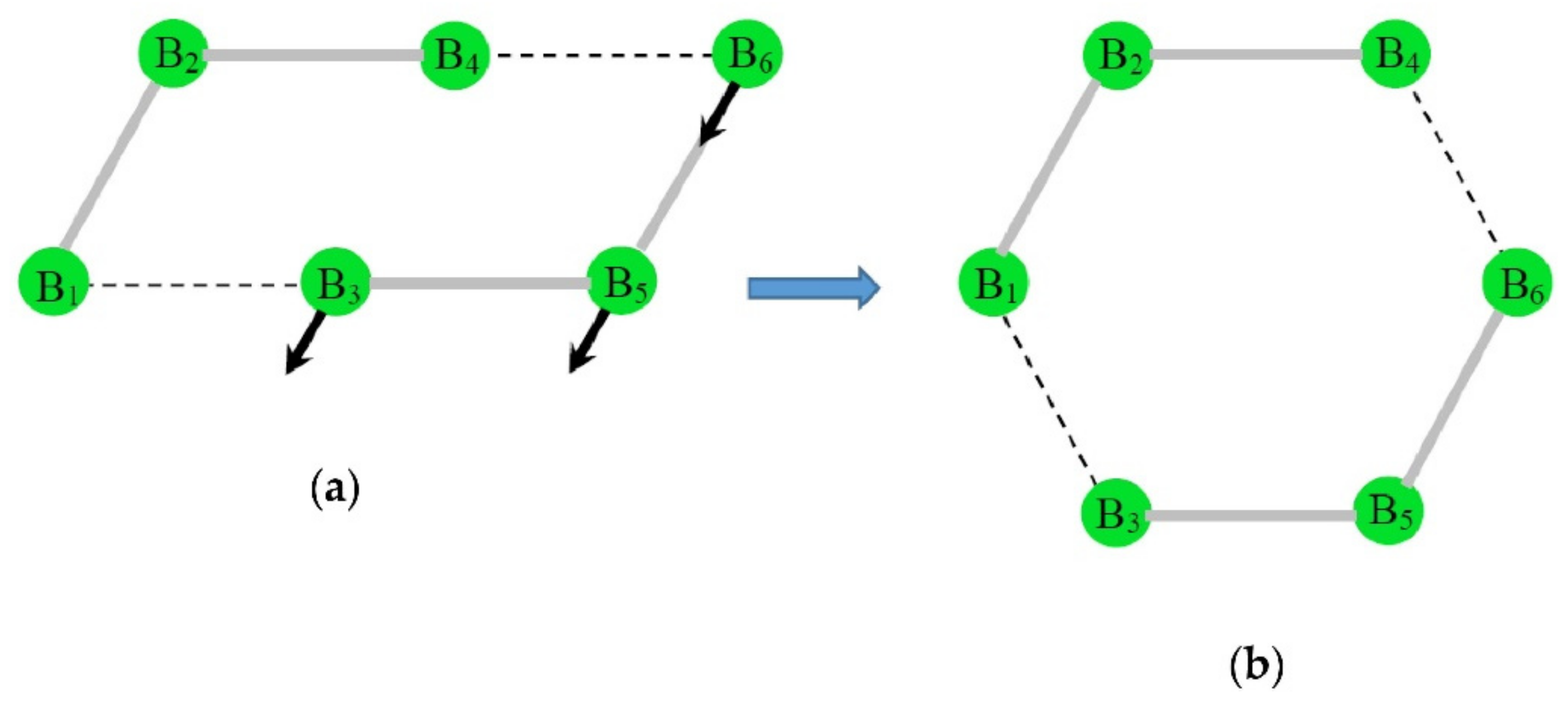
4. Discussion

5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Greenwood, N.N. Boron; Pergamon Press: Oxford, UK, 1975. [Google Scholar]
- Stock, A. Hydrides of Boron and Silicon; Cornell University Press: Ithaca, NY, USA, 1933. [Google Scholar]
- Muetterties, E.L. (Ed.) Boron Hydride Chemistry; Academic Press: New York, NY, USA, 1975. [Google Scholar]
- Bregadze, V.I.; Xie, Z. Boron Chemistry—A Rapidly Expanding Research Field. Eur. J. Inorg. Chem. 2017, 38–39, 4344–4692. [Google Scholar] [CrossRef] [Green Version]
- Lipscomb, W.N. Boron Hydrides; Benjamin: New York, NY, USA, 1963. [Google Scholar]
- Nishino, H.; Fujita, T.; Cuong, N.T.; Tominaka, S.; Miyauchi, M.; Iimura, S.; Hirata, A.; Umezawa, N.; Okada, S.; Nishibori, E.; et al. Formation and characterization of hydrogen boride sheets derived from MgB2 by cation exchange. J. Am. Chem. Soc. 2017, 139, 13761–13769. [Google Scholar] [CrossRef] [PubMed]
- Oliva-Enrich, J.M.; Kondo, T.; Alkorta, I.; Elguero, J.; Klein, D.J. Concatenation of diborane leads to new planar boron chemistry. Chem. Phys. Chem. 2020, 21, 2460–2467. [Google Scholar] [CrossRef] [PubMed]
- Poater, J.; Solà, M.; Viñas, C.; Teixidor, F. A Simple link between hydrocarbon and borohydride chemistries. Chem. Eur. J. 2013, 19, 4169–4175. [Google Scholar] [CrossRef] [PubMed]
- Greenwood, N.N.; Earnshaw, A. Chemistry of the Elements, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 1997. [Google Scholar]
- Amero, B.A.; Schram, E.P. Preparation and characterization of 1,2,3,4,5,6-hexakis(dimethylamino)-closo-hexaborane(6)-hexakis(dimethylaminodimethylaluminum), a novel boron cluster compound. Inorg. Chem. 1976, 15, 2842–2846. [Google Scholar] [CrossRef]
- Anju, R.S.; Roy, D.K.; Mondal, B.; Ramkumar, V.; Ghosh, S. An early-late transition metal hybrid analogue of hexaborane(12). Organometallics 2013, 16, 4618–4623. [Google Scholar] [CrossRef]
- Rao, C.E.; Yuvaraj, K.; Ghosh, S. Diruthenium analogues of hexaborane(12) and pentaborane(9): Synthesis and structural characterization of [(1,2-Cp*Ru)2B2H6S2] and [(2,3-Cp*Ru)2B3H6(µ-η1-EPh)], (E = S, Se and Te) (Cp* = η5-C5Me5). J. Organomet. Chem. 2015, 776, 123–128. [Google Scholar] [CrossRef]
- Greatrex, R.; Greenwood, N.N.; Millikan, M.B.; Rankin, D.W.H.; Robertson, H.E. The molecular-structure of hexaborane(12) in the gas-phase as determined by electron-diffraction. J. Chem. Soc. Dalton Trans. 1988, 9, 2335–2339. [Google Scholar] [CrossRef]
- Brain, P.T.; Hnyk, D.; Rankin, D.W.H.; Buhl, M.; Schleyer, P.V.R. The molecular-structures of pentaborane(11), B5H11, and hexaborane(12), B6H12, in the gas-phase as determined by electron-diffraction and ab-initio calculations. Polyhedron 1994, 13, 1453–1466. [Google Scholar] [CrossRef]
- Lipscomb, W.N. Topologies of B6 and B7 hydrides. J. Phys. Chem. 1961, 65, 1064–1066. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16; Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar]
- Dunning, T.H., Jr. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 1989, 90, 1007–1023. [Google Scholar] [CrossRef]
- Kendall, R.A.; Dunning, T.H., Jr.; Harrison, R.J. Electron affinities of the first-row atoms revisited. Systematic basis sets and wave functions. J. Chem. Phys. 1992, 96, 6796–6806. [Google Scholar] [CrossRef] [Green Version]
- Peng, C.; Schlegel, H.B. Combining synchronous transit and quasi-newton methods for finding transition states. Isr. J. Chem. 1993, 33, 449–454. [Google Scholar] [CrossRef]
- Peng, C.; Ayala, P.Y.; Schlegel, H.B.; Frisch, M.J. Using redundant internal coordinates to optimize equilibrium geometries and transition states. J. Comp. Chem. 1996, 17, 49–56. [Google Scholar] [CrossRef]
- Fukui, K. The path of chemical-reactions—The IRC approach. Acc. Chem. Res. 1981, 14, 363–368. [Google Scholar] [CrossRef]
- Hratchian, H.P.; Schlegel, H.B. Theory and Applications of Computational Chemistry: The First 40 Years; Dykstra, C.E., Frenking, G., Kim, K.S., Scuseria, G., Eds.; Elsevier: Amsterdam, The Netherlands, 2005; pp. 195–249. [Google Scholar]
- Bader, R.F.W. Atoms in Molecules: A Quantum Theory; Clarendon Press: Oxford, UK, 1990. [Google Scholar]
- Popelier, P.L.A. Atoms in Molecules. An Introduction; Prentice Hall: Harlow, UK, 2000. [Google Scholar]
- Keith, T.A. AIMAll; Version 19.10.12; TK Gristmill Software: Overland Park, KS, USA, 2017. [Google Scholar]
- Alkorta, I.; Thacker, J.C.R.; Popelier, P.L.A. An interacting quantum atom study of model SN2 reactions (X−⋯CH3X, X = F, Cl, Br, and I). J. Comput. Chem. 2018, 39, 546–556. [Google Scholar] [CrossRef] [Green Version]
- IUPAC. Compendium of Chemical Terminology, 2nd ed.; IUPAC: Research Triangle Park, NC, USA, 1997. [Google Scholar]
- Scott, L.T.; Jones, M., Jr. Rearrangements and interconversions of compounds of the formula (CH)n. Chem. Rev. 1972, 72, 181–202. [Google Scholar] [CrossRef]
- Katz, T.J.; Acton, N. Synthesis of prismane. J. Am. Chem. Soc. 1973, 95, 2736–2739. [Google Scholar] [CrossRef]
- Bolesov, I.G. Valence Isomers of Benzene. Russ. Chem. Rev. 1968, 37, 666–670. [Google Scholar] [CrossRef]
- Katz, T.J.; Roth, R.J.; Acton, N.; Carnahan, E.J. Synthesis of Benzvalene. J. Org. Chem. 1999, 64, 7663. [Google Scholar] [CrossRef]
- Krasowska, M.; Bettinger, H.F. Computational Study of the Isomerization Reactions of Borirane. J. Org. Chem. 2018, 83, 1804–1809. [Google Scholar] [CrossRef] [PubMed]
- Cotton, F.A.; Wilkinson, G.; Murillo, C.A.; Bochmann, M. Advanced Inorganic Chemistry, 6th ed.; Wiley-Interscience: New York, NY, USA, 1999. [Google Scholar]
- Francés-Monerris, A.; Holub, J.; Roca-Sanjuán, D.; Hnyk, D.; Lang, K.; Oliva-Enrich, J.M. A photochromic system among boron hydrides: The Hawthorne rearrangement. J. Phys. Chem. Lett. 2019, 10, 6202–6207. [Google Scholar] [CrossRef] [PubMed]
- Lipscomb, W.N. Framework Rearrangement in Boranes and Carboranes. Science 1966, 153, 373–378. [Google Scholar] [CrossRef] [PubMed]
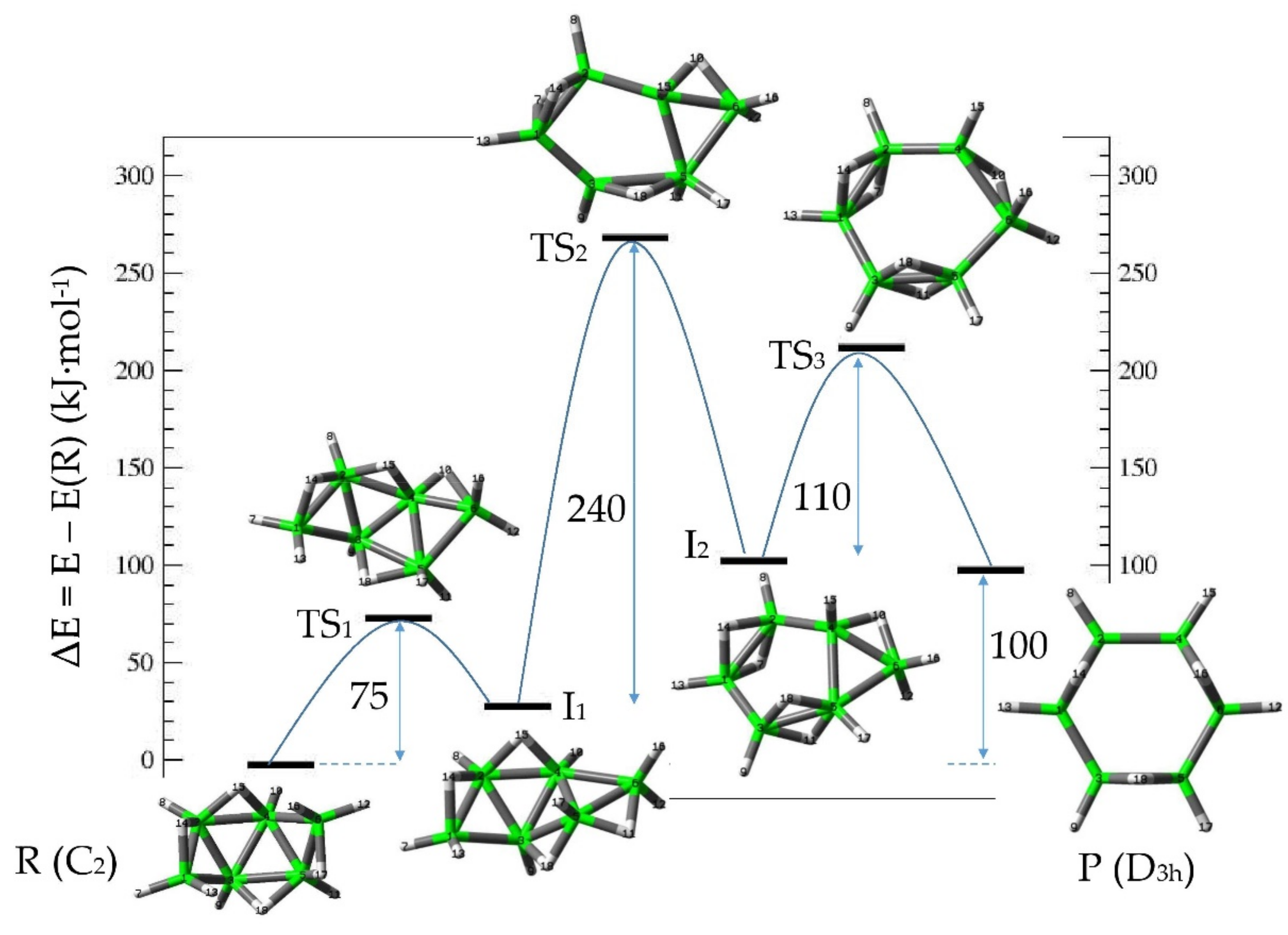
| SP | E | ΔER |
|---|---|---|
| R | −156.20882555 | 0.0 |
| TS1 | −156.18016535 | 75.2 |
| I1 | −156.19687483 | 31.4 |
| TS2 | −156.10584340 | 270.4 |
| I2 | −156.16931585 | 103.7 |
| TS3 | −156.12728434 | 214.1 |
| P | −156.17070415 | 100.1 |
| SP | Volume | B(3)-B(4) |
|---|---|---|
| R | 146.7 | 1.788 |
| TS1 | 160.5 | 1.720 |
| I1 | 152.5 | 1.763 |
| TS2 | 145.2 | 2.434 |
| I2 | 161.5 | 2.822 |
| TS3 | 168.6 | 3.385 |
| P | 173.3 | 3.512 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oliva-Enrich, J.M.; Alkorta, I.; Elguero, J.; Ferrer, M.; Burgos, J.I. On the 3D → 2D Isomerization of Hexaborane(12). Chemistry 2021, 3, 28-38. https://doi.org/10.3390/chemistry3010003
Oliva-Enrich JM, Alkorta I, Elguero J, Ferrer M, Burgos JI. On the 3D → 2D Isomerization of Hexaborane(12). Chemistry. 2021; 3(1):28-38. https://doi.org/10.3390/chemistry3010003
Chicago/Turabian StyleOliva-Enrich, Josep M., Ibon Alkorta, José Elguero, Maxime Ferrer, and José I. Burgos. 2021. "On the 3D → 2D Isomerization of Hexaborane(12)" Chemistry 3, no. 1: 28-38. https://doi.org/10.3390/chemistry3010003
APA StyleOliva-Enrich, J. M., Alkorta, I., Elguero, J., Ferrer, M., & Burgos, J. I. (2021). On the 3D → 2D Isomerization of Hexaborane(12). Chemistry, 3(1), 28-38. https://doi.org/10.3390/chemistry3010003








