Do Successful Researchers Reach the Self-Organized Critical Point?

Abstract

The index of success of the researchers is now mostly measured using the Hirsch index (h). Our recent precise demonstration, that statistically $h\sim \sqrt{N_c}\sim \sqrt{N_p}$, where $N_p$ and $N_c$ denote, respectively, the total number of publications and total citations for the researcher, suggests that average number of citations per paper ($N_c/N_p$), and hence h, are statistical numbers (Dunbar numbers) depending on the community or network to which the researcher belongs. We show here, extending our earlier observations, that the indications of success are not reflected by the total citations $N_c$, rather by the inequalities among citations from publications to publications. Specifically, we show that for highly successful authors, the yearly variations in the Gini index (g, giving the average inequality of citations for the publications) and the Kolkata index (k, giving the fraction of total citations received by the top $(1-k)$ fraction of publications; $k=0.80$ corresponds to Pareto’s 80/20 law) approach each other to $g=k\simeq 0.82$, signaling a precursor for the arrival of (or departure from) the self-organized critical (SOC) state of his/her publication statistics. Analyzing the citation statistics (from Google Scholar) of thirty successful scientists throughout their recorded publication history, we find that the g and k for the highly successful among them (mostly Nobel laureates, highest rank Stanford cite-scorers, and a few others) reach and hover just above (and then) below that $g=k\simeq 0.82$ mark, while for others they remain below that mark. We also find that all the lower (than the SOC mark 0.82) values of k and g fit a linear relationship, $k=1/2+cg$, with $c=0.39$, as suggested by an approximate Landau-type expansion of the Lorenz function, and this also indicates $k=g\simeq 0.82$ for the (extrapolated) SOC precursor mark.

1. Introduction

Inspiring research in sociophysics (see, e.g., [1,2,3,4,5,6]) has, in years, led to intense research activities in several statistical and statistical physical models and analysis of socio-dynamical problems. For example, the social opinion formation models of Galam (see e.g., [7,8]), of Biswas-Chatterjee-Sen (see e.g., [9,10]), of Minority Games (see e.g., [11]), of Kolkata Paise Restaurant games (see, e.g., [12,13]), and others. In view of the automatically encoded wide range of the citation data of the publications by scientists and their straightforward availability on the internet, we have studied the inequality statistics from Google Scholar data. The presence of ubiquitous inequalities allowed recently the studies of various scaling, etc., properties in their statistics (see e.g., [14,15]) of the Hirsch index [16], or the universal (or limiting) self-organized critical (SOC) behavior (see e.g., [17,18,19]) and their citation inequality like the century-old Gini (g) [20] and the recently introduced Kolkata (k) [21,22] indices. It may be noted at this stage that while g values measure the overall inequality in the distributions and k gives the fraction of “mass” or of total citations coming from the $(1-k)$ fraction of avalanches or publications, these studies [17,18,19] indicated that the inequalities in the avalanche size distributions, measured by g and k, just prior to the arrival of the SOC point in several standard physical models (like the sand-pile models of Bak–Tang–Wiesenfeld (BTW) [23], Manna [24], and others), and in social contexts of citations from publications [18,19] becomes equal: $g=k=0.84\pm 0.04$. It may also be noted that $k=0.80$ corresponds to Pareto’s 80/20 law (see e.g., [21,22]). This Pareto principle asserts that 20% of the causes are responsible for 80% of the outcomes. In other words, the principle suggests that a small fraction of the factors contribute in causing a large fraction of major events, from economics to quality management and even in personal development. In business, it is often used to identify the most important areas for improvement. It may be mentioned here that our earlier studies of inequality indices g and k [17,18,19,20,21,22] corresponded to the cumulative dynamics (as the sand-pile dynamics progress and cluster distributions grow or the publications by the authors or from the institutions progress over time and the citation size distributions grow since the start of the dynamics) as the system approaches the respective SOC states. Our study here is for the same inequality indices, but for small time intervals along growth dynamical paths of individual researchers.

We study here the inequality dynamics measured by the g and k indices of several successful researchers (mostly winners of international prizes, medals, or awards like Nobel, Fields, Boltzmann, Breakthrough, highest level Stanford c-score achievers, etc.), some distinguished sociophysics researchers, along with those of a few high level (but not so high Stanford c-score, though within “top 2%”) researchers, for data up to 2022, since their recorded first publication year. We collected the citation data of the publications (from online free Google Scholar, if an individual Google Scholar page exists). We calculate the g and k indices for each year, starting their first publication, by taking the citation statistics today (collected and analyzed in July–August 2023). We extracted the values for g and k for all the recorded publications of the scientist in each overlapping five-year window (since the first publication), where the windows continuously shift by one year till the year 2022 (corresponding to the last central year 2020 of the researcher) in the following figures for each researcher. The choice of five-year window size is found to give optimal stability in statistics (a smaller three-year window size did not give stability to the citation statistics for quite a few of the scientists.)

We find that the majority of the chosen scientists crossed the $g=k\simeq 0.82$ mark (which we interpret here as the precursor level of the SOC point [17]) early in their life and often they hover just above or below but around that level of inequality mark. Some others just touched the precursor mark ($g=k$) once or even multiple times and a few remained below that mark. For other known researchers considered here, the $g=k$ mark occurs marginally but does not cross ever. It is to be noted that this mark of reaching the SOC state (beyond the $g=k\simeq$ 0.82) level of inequality is for yearly statistics (within a 5-year window which slides yearly) and not for the overall success measuring indices (in their cumulative citation statistics) studied earlier for the citation statistics of some distinguished researchers (see e.g., [14]), where the SOC mark is observed to be a little higher ($g=k\simeq 0.86)$.

As mentioned earlier, the Hirsch index (h) [16], which gives the highest number of publications by a researcher, each of which has received equal or more than that number of citations, does not seem give a most suitable measure [15,25] of the success of individual researchers. It has now been well demonstrated [15] (using the kinetic theoretical exchange model ideas), analyzing the Scopus citation data for the top 120,000 (within the “top 2%”) Stanford cites score achievers that statistically $h\sim \sqrt{N_c}\sim \sqrt{N_p}$, where $N_c$ and $N_p$ denote, respectively, the total number of citations and total number of publications by the researcher. This suggests convincingly that the average number of citations per paper ($N_c/N_p$), and hence h, are statistical numbers (given by the effective Dunbar number [26,27]) depending on the community or network in which the researcher belongs [15,18]. We show here, extending our earlier observations (see, e.g., [14,18]), that the indications of success are not reflected by the total citations $N_c$, or for that matter by the Hirsch index h, rather than by the inequalities among the citations from publication to publication. Specifically, we show that for highly successful authors, the yearly variations (given by the statistics with overlapping 5-year windows) in the g index (given by the average inequality of the citations for the publications, $0\le g\le 1$) and the k index (giving the fraction of total citations received by the top $(1-k)$ fraction of publications, $0.5\le k\le 1$). In particular, achieving $g=k\simeq 0.82$ signals a precursor to the SOC state in the publication statistics. Analyzing the citation statistics (from the open-access Google Scholar) of thirty successful scientists throughout their recorded publication history, starting from their first recorded publication that the very successful among them (mostly Nobel laureates, higher-ranking Stanford c-scorers, and a few others) reach and hover just above and below that $g=k\simeq 0.82$ mark, characteristic of the SOC state ($k=0.82$ means 82% of citations come from 18% of publications). Others remain below the (SOC) level of extreme inequality in publication statistics.

2. Socio-Statistical Inequality and Its Measures

In 1905, American economist Lorenz [2,3] developed the Lorenz curve, a graphical representation of the distribution of wealth in a society. To construct this curve (illustrated by the red curve in Figure 1), one organizes the society’s population in ascending order of their wealth and then plots the cumulative fraction of wealth, denoted as $L\left(p\right)$, held by the poorest p fraction of individuals. One can similarly plot the cumulative fraction of citations against the fraction of papers that attracted those many citations. As indicated in Figure 1, the Gini index is calculated from the area between the equality line and the Lorenz curve, divided by the area (1/2) below the equality line for normalization. As such, $g=0$ signifies perfect equality and $g=1$ corresponds to extreme inequality. The index k is given by the fixed point of the complementary Lorentz function $L_c\left(p\right)\equiv 1-L\left(p\right)$. As such, k gives the fraction of citations attracted by the top cited k fraction of papers and $k=0.5$ means perfect equality, while extreme inequality corresponds to $k=1$.

Landau-Like Expansion of $L\left(p\right)$ and $g,k$ Approximate Relation

A minimal expansion [28] of the Lorenz function $L\left(p\right)$, employing a Landau-like expansion of free energy, suggests $L\left(p\right)=Ap+B{p}^2,A>0,B>0,A+B=1$. This gives $L\left(0\right)=0$ and $L\left(1\right)=1$ (with B = 0, the Lorenz function can represent only the equality line in Figure 1).

One can then calculate $g=1-2{\int }_0^{1}L\left(p\right)dp$, giving $A=1-3g$ and $B=3g$. Since $L\left(k\right)=1-k=Ak+B{k}^2$, one can obtain a quadratic equation involving g and k. An approximate solution of it, in the $g\to 0$ limit gives

$$k=1/2+C\times g,$$

(1)

where $C=3/8$ [28] suggesting that $g=k$ will occur at the Pareto value $k=0.80$. We see here a little deviation in the value of the constant C in the relation (1), for all the reported observations.

3. Inequality Data Analysis from Google Scholar

We collect the citation data for all the recorded publications in each year since the first entry in the record for thirty successful researchers having individual Google Scholar page and having minimum and maximum number of total publications $N_p$ = 127 and 2954, minimum and maximum number of total citations $N_c$ = 5769 and 463,382, minimum and maximum values of h = 22 and 328, respectively, for all those selected researchers. We considered three Nobel prize winners in each of the science subjects: physics (H. Amano, B. Josephson, A. B. McDonald), chemistry (R. Henderson, J. Frank, J.-P. Sauvage), physiology and medicine (M. Houghton, G. L. Semanza, S. Yamanka), and economics (A. Banerjee, W. Nordhaus, J. Stiglitz). Two Fields medalists (mathematics; S. Smalle, E. Witten), two Boltzmann award winners (statistical physics; D. Dhar, H. E. Stanley), two Breakthrough prize winners (physics; C. Kane, A. Sen), three of the top-most cite-scorers in the Stanford Scopus c-score list (M. Graetzel, R. C. Kessler, and Z. L. Wang; considered for h-index statistics in Ref. [15]), and six known contributors in econophysics and sociophysics: W. Brian Arthur (known for “El Farol Bar Problem” of minority choice, see, e.g., [11]), B. K. Chakrabarti (one of the “fathers of econophysics” [29,30]), R. I. M. Dunbar (known for Dunbar’s number of social connectivity, see, e.g., [31]), S. Galam (considered a pioneer of sociophysics), R. Mantegna (one of the “fathers of econophysics” [29,30]), V. M. Yakovenko (pioneer of kinetic exchange models of income/wealth distributions; see, e.g., [32]). We considered three of the highest-ranked Stanford cite-scorers for 2022 (M. Graetzel, R. C. Kessler, and Z. L. Wang [33]), and, for comparison, we also considered three lower-rank holders of the same “top 2% Stanford cite-scores” (I. Fofana, U. Sennur, and N. Tomoyuki [33]).

For studying the growth of inequality in the citation statistics of each of these researchers, we select a 5-year window, starting earliest publication, and note the present-day citations of each of these publications. We then construct the Lorenz function (see Figure 1) and extract the g and k indices as described in Section 4. We associate the g and k values with the middle year of the respective 5-year window and shift the window by one year and get the values of the inequality indices for each of the successive years up to 2020 (considering data up to 2022). These are shown in the following Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6.

One can see from Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6, for all the above-mentioned thirty scientists that for many of them (mostly Nobel prize winners and highest rank c-scorers), the g-index value goes over the k-index value in one (or multiple years) by crossing the $k=g\simeq 0.82$ line (see the corresponding insets). These crossings of the indices (at values above 0.80 value) preciously indicates large inequalities and entry into the SOC state [17] of the citation statistics of these scientists (see

Table 1. Consolidated inequality index ($g,k$) results for the citation statistics (from Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6) of the thirty chosen scientists (including twelve Nobel prize winners, two Fields medalists, two Boltzmann award winners, two Breakthrough prize winners, six distinguished sociophysics and econophysics researchers, three from the top and three from the bottom of the “top 2% Stanford cite-score scientists” (2022 list). NP(P) stands for Nobel Prize in physics, NP(C) stands for Nobel Prize in chemistry, NP(M) stands for Nobel Prize in physiology or medicine and NP(E) stands for Nobel Prize in economics, FM stands for Fields Medal in mathematics, BA stands for Boltzmann Award in statistical physics, BP(P) stands for Breakthrough Prize in physics, FEP stands for “fathers of econoophysics” [29,30], EFBP stands for “El Farol Bar problem” (see, e.g., [11]), DN stands for “Dunbar Number” (see, e.g., [31]), FSP stands for “father of sociophysics”, PKEM stands for pioneer in kinetic exchange modeling of Wealth distribution [32], SCS-x stands for Stanford Cite Score rank (‘x’ denoting the rank) among the “top 2%” scientists in 2022 [33], and “yearly-av.” stands for yearly-averaged publication in 5-year window; see text for more details.

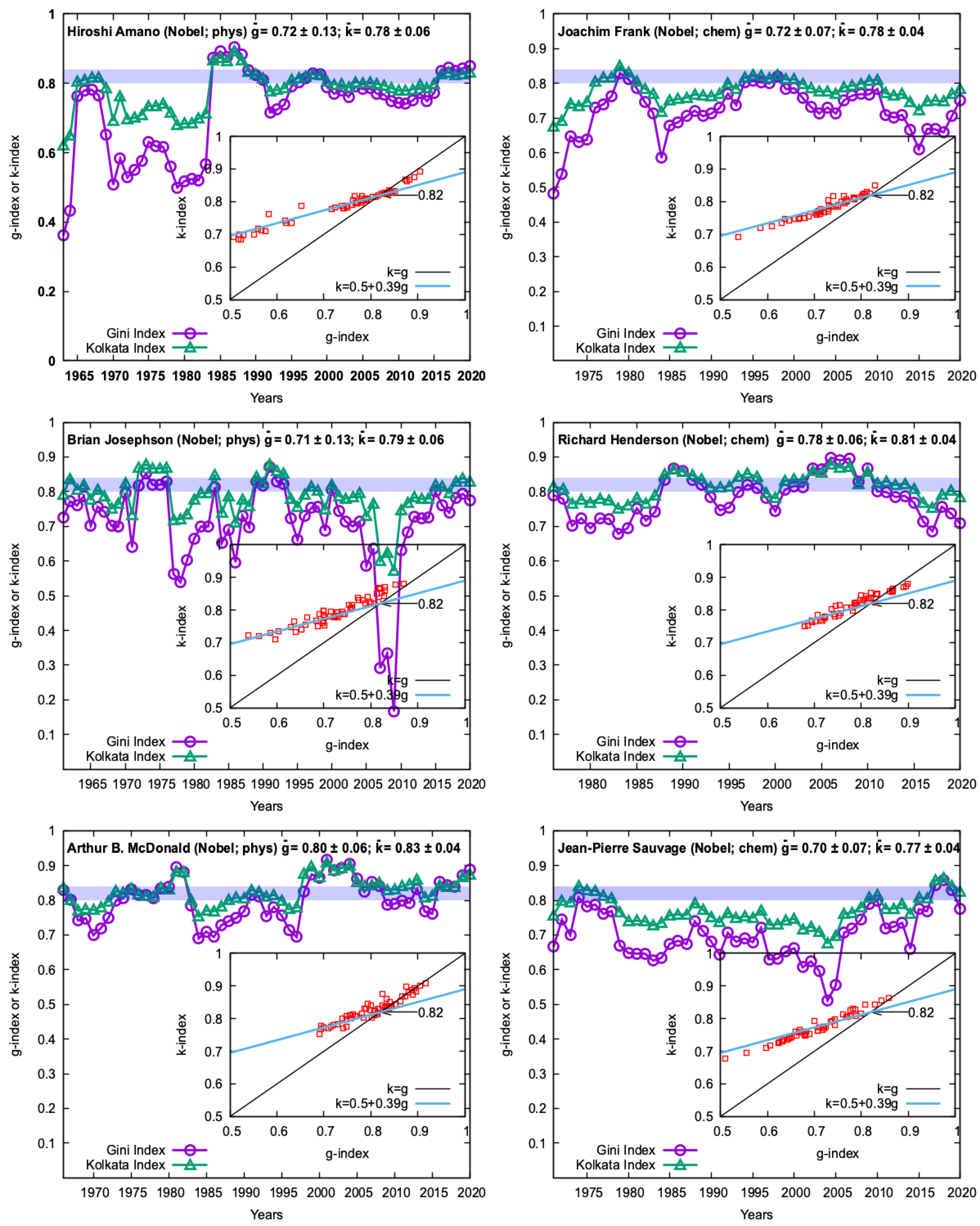
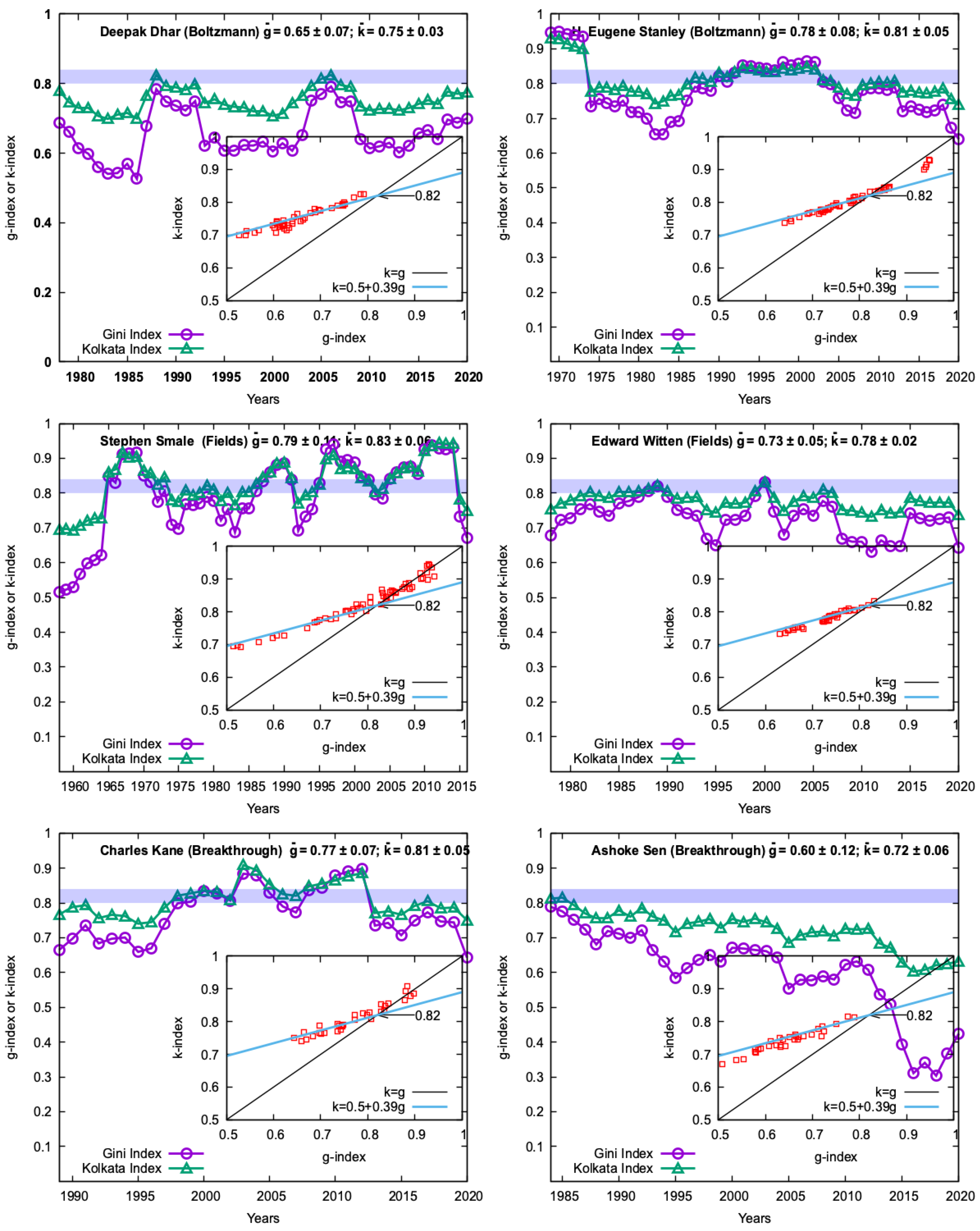
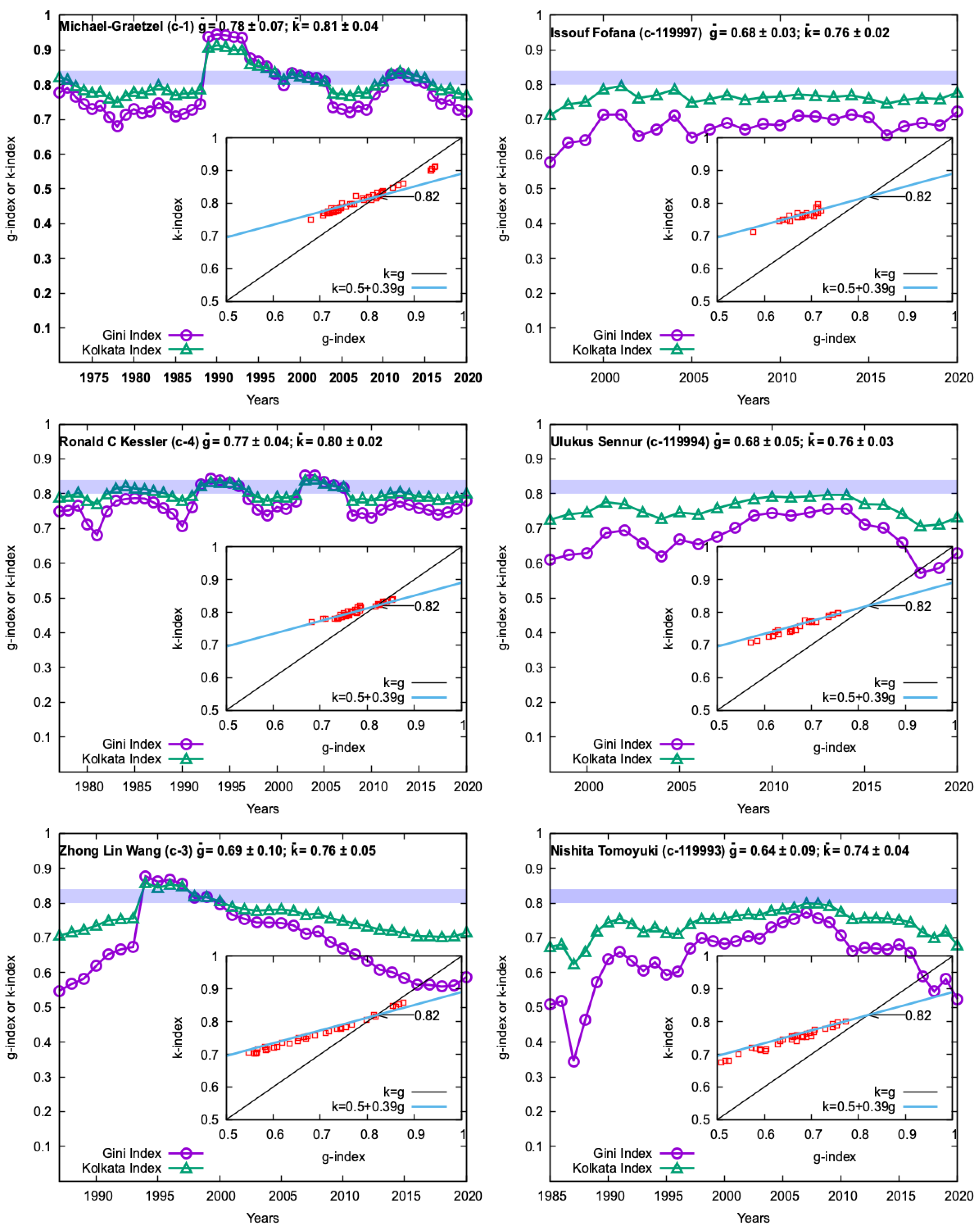
				Inequality Indices: Hirsch (h), Gini (g), Kolkata (k)					$\mathit{g}=\mathit{k}$ Line
Researcher Name	Award/Prize/ Known for	${\mathit{N}}_{\mathit{p}}$	${\mathit{N}}_{\mathit{c}}$	$\mathit{h}$	$\mathit{g}$ (overall)	$\mathit{k}$ (overall)	$\mathit{g}$ (yearly-av.)	$\mathit{k}$ (yearly-av.)	Crossed Near 0.82?
H Amano	NP(P)	2161	57,281	106	0.84	0.83	0.72 ± 0.13	0.78 ± 0.06	Yes
B Josephson	NP(P)	127	11,685	22	0.94	0.92	0.71 ± 0.13	0.79 ± 0.06	No
AB McDonald	NP(P)	437	25,111	53	0.91	0.88	0.80 ± 0.06	0.83 ± 0.04	Yes
J Frank	NP(C)	686	50,518	116	0.77	0.80	0.72 ± 0.07	0.78 ± 0.04	No
R Henderson	NP(C)	267	31,822	65	0.85	0.84	0.78 ± 0.06	0.81 ± 0.04	Yes
JP Sauvage	NP(C)	655	61,572	114	0.70	0.76	0.70 ± 0.07	0.77 ± 0.04	Marginally
M Houghton	NP(M)	529	59,029	102	0.85	0.84	0.73 ± 0.11	0.78 ± 0.05	Yes
GL Semenza	NP(M)	682	192,246	196	0.80	0.81	0.73 ± 0.08	0.78 ± 0.04	Yes
S Yamanaka	NP(M)	345	124,106	125	0.85	0.84	0.71 ± 0.11	0.78 ± 0.06	Yes
A Banerjee	NP(E)	524	79,076	106	0.86	0.86	0.84 ± 0.05	0.85 ± 0.04	Yes
W Nordhaus	NP(E)	647	101,219	124	0.87	0.86	0.82 ± 0.06	0.83 ± 0.03	Yes
J Stiglitz	NP(E)	2408	364,237	235	0.89	0.87	0.85 ± 0.05	0.85 ± 0.03	Yes
D Dhar	BA	209	8299	44	0.76	0.80	0.65 ± 0.07	0.75 ± 0.03	No
HE Stanley	BA, FEP	2070	225,169	204	0.83	0.83	0.78 ± 0.08	0.81 ± 0.05	Yes
S Smale	FM	346	48,084	85	0.87	0.85	0.79 ± 0.11	0.83 ± 0.06	Yes
E Witten	FM, BP(P)	620	242,911	206	0.79	0.81	0.73 ± 0.05	0.78 ± 0.02	Marginally
C Kane	BP(P)	189	80,714	75	0.88	0.87	0.77 ± 0.07	0.81 ± 0.05	Yes
A Sen	BP(P)	401	37,065	103	0.69	0.76	0.60 ± 0.12	0.72 ± 0.06	No
WB Arthur	EFBP	196	52,545	56	0.91	0.89	0.82 ± 0.09	0.84 ± 0.06	Yes
BK Chakrabarti	FEP	390	12,596	47	0.81	0.82	0.72 ± 0.11	0.78 ± 0.06	Marginally
RIM Dunbar	DN	857	85,486	141	0.79	0.81	0.73 ± 0.10	0.79 ± 0.05	Yes
S Galam	FSP	252	8828	42	0.81	0.83	0.75 ± 0.08	0.80 ± 0.04	Yes
RN Mantegna	FEP	259	28,561	68	0.84	0.84	0.72 ± 0.11	0.78 ± 0.05	Yes
VM Yakovenko	PKEM	171	9076	44	0.73	0.78	0.65 ± 0.07	0.75 ± 0.04	No
M Graetzel	SCS-1	2282	463,382	295	0.82	0.82	0.78 ± 0.07	0.81 ± 0.04	Yes
RC Kessler	SCS-4	1829	523,835	328	0.83	0.83	0.77 ± 0.04	0.80 ± 0.02	Yes
ZL Wang	SCS-3	2954	394,080	299	0.71	0.77	0.69 ± 0.10	0.76 ± 0.05	Yes
I Fofana	SCS-119997	353	5759	39	0.73	0.78	0.68 ± 0.03	0.76 ± 0.02	No
N Tomoyuki	SCS-119993	304	9073	51	0.75	0.79	0.64 ± 0.09	0.74 ± 0.04	No
U Sennur	SCS-119994	455	18,987	63	0.73	0.78	0.68 ± 0.05	0.76 ± 0.03	No

for consolidated results of g and k).

Although the study of the time variations of the g and k indices (as shown in Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6) and checking if g value ever goes over the k value by crossing the k versus g line (as shown in the insets) is indispensable for detecting if the SOC state has arrived or not, one can also have a straightforward (but only approximate) indication of the SOC state by looking at the ratio R of the citation number $n_C^{\max }$ of the highest cited paper and the effective Dunbar number D given by the average citation $N_c/N_p$ of the researcher. In

Table 2. An approximate indicator, $R=n_C^{\max }/D$, where the effective Dunbar number $D=N_c/N_p$ ($N_c$ denotes the total number of citations for $N_p$ papers by the researcher) and $n_C^{\max }$ denotes the citation of the most-cited paper by the researcher, to check if the researcher has achieved the SOC level or not. We find, for $R\ge 40$, the corresponding researchers certainly belong to the SOC level ($94\%$ success rate).

Researcher Name	Award/ Known for	${\mathit{N}}_{\mathit{p}}$	${\mathit{N}}_{\mathit{c}}$	${\mathit{n}}_{\mathit{C}}^{\mathbf{max}}$	h	$\mathit{D}={\mathit{N}}_{\mathit{C}}/{\mathit{N}}_{\mathit{P}}$	$\mathit{R}={\mathit{n}}_{\mathit{C}}^{\mathbf{max}}/\mathit{D}$	SOC Level Achieved (Table 1)	Comments
H Amano	NP(P)	2161	57,281	3154	106	27	119	Yes	Out of the eighteen researchers with $R\ge 40$, one failed in achieving the SOC level (crossing the $k=g\simeq 0.82$ line in Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6; see Table 1). $R\ge 40$ therefore indicates SOC level for the researcher with more than 94% success rate.
B Josephson	NP(P)	127	11,685	6554	22	92	71	No
AB McDonald	NP(P)	437	25,111	5375	53	57	94	Yes
J Frank	NP(C)	686	50,518	2299	116	74	31	No
R Henderson	NP(C)	267	31,822	3681	65	119	31	Yes
JP Sauvage	NP(C)	655	61,572	1805	114	94	19	Marginally
M Houghton	NP(M)	529	59,029	9952	102	112	89	Yes
GL Semenza	NP(M)	682	192,246	12,229	196	282	43	Yes
S Yamanaka	NP(M)	345	124,106	30,735	125	360	85	Yes
A Banerjee	NP(E)	524	79,076	9254	106	151	61	Yes
W Nordhaus	NP(E)	647	101,219	19,605	124	156	125	Yes
J Stiglitz	NP(E)	2408	364,237	23,844	235	151	158	Yes
D Dhar	BA	209	8299	1182	44	40	30	No
HE Stanley	BA, FEP	2070	225,169	14,348	204	109	132	Yes
S Smale	FM	346	48,084	7912	85	139	57	Yes
E Witten	FM, BP(P)	620	242,911	14,380	206	392	37	Marginally
C Kane	BP(P)	189	80,714	19,504	75	427	46	Yes
A Sen	BP(P)	401	37,065	1443	103	92	16	No
WB Arthur	EFBP	196	52,545	15,227	56	268	57	Yes
BK Chakrabarti	FEP	390	12,596	730	47	32	23	Marginally
RIM Dunbar	DN	857	85,486	5312	141	100	53	Yes
S Galam	FSP	252	8828	653	42	35	19	Yes
RN Mantegna	FEP	259	28,561	5796	68	110	53	Yes
VM Yakovenko	PKEM	171	9076	920	44	53	17	No
M Graetzel	SCS-1	2282	463,382	35,789	295	203	176	Yes
RC Kessler	SCS-4	1829	523,835	35,079	328	286	122	Yes
ZL Wang	SCS-3	2954	394,080	8120	299	133	61	Yes
I Fofana	SCS-119997	353	5759	333	39	16	20	No
N Tomoyuki	SCS-119993	304	9073	467	51	30	16	No
U Sennur	SCS-119994	455	18,987	1198	63	42	29	No

, we compare these $R=n_C^{\max }/D$ values (where $D=N_c/N_p$) and see how its higher values compare with the observation of SOC (when k versus g line is crossed affirmatively). We find, for $R\ge 40$, more than $94\%$ of cases correspond to the SOC level.

4. Summary and Discussion

Our earlier analysis [15] of the Scopus citation data for the 120,000 top Stanford cite-score scientists showed that the Hirsch index $h\sim {\sqrt{N}}_c\sim {\sqrt{N}}_p$, where $N_c$ and $N_p$ denote, respectively, the total number of citations and the total number of publications by the researcher. This, in turn, says that the average number of citations per paper ($N_c/N_p)$, and hence h, are statistical numbers (determined by the effective Dunbar number [26,31]) of the community or network (coauthors and followers) in which the researcher belongs [15,18]. Indeed, the anticipated increase in research impacts through collaboration (by increasing the number of coauthors) has been studied in Ref. [34], by looking at the average value of the community Dunbar number or $N_c/N_p$. Also, a detailed study from Google Scholar data on the relation between the Hirsch index of individual scientists with their average number of co-authors per paper has been reported in Ref. [35]. Our study here shows that the Hirsch index can not be a suitable measure of success for the researchers (even in Table 1; the highest $h=328$ does not correspond to a Nobel Prize winner, while the least one with $h=22$ do).

In an earlier study [18], we proposed that the citation inequality indices Gini $(0\le g\le 1)$ and Kolkata $(0.5\le k\le 1)$ to give better measures of success of the scientist (not $N_c$ or h) and g and k both expected to approach equality at $g=k\simeq 0.86$ for successful researchers. It may be mentioned here that we used the entire citation data (over all the years) to get the Lorenz curve and the overall values of g and k of the researcher, and this gave a little higher value of $g=k\simeq 0.86$ point. Indeed, our numerical study [17] of the overall or cumulative inequality statistics of the avalanches or cluster sizes in some mostly studied and mstly established SOC models also suggested the arrival of the equality point of the avalanche size inequality indices ($g=k\simeq 0.86$) just appears as a precursor of the SOC point of the respective sand-pile or SOC models. In other words, as mentioned already, the SOC points in sandpile models (like BTW [23], Manna [24], and other models) of physics signifies a critical state where sand grain avalanches of all sizes occur following a power law distribution. As shown in Ref. [17], even in these physics SOC models, the inequality statistics (Gini and Kolkata indices) corresponding to the avalanche size statistics reach similar values for the inequality indices of the unequal citations (considered here equivalent to the sand mass avalanches in sand piles).

We analyzed the citation data for all the recorded publications in each year since the first entry in the record for the chosen thirtky successful scientists, each having an individual Google Scholar page. They have the minimum and maximum number of total publications $N_p$ = 127 and 2954, and minimum and maximum number of total citations, $N_c$ = 5769 and 463,382, respectively. For studying the growth of inequality in the citation statistics of each of these scientists, we select 5-year windows, where the central year of each window moves every year. We construct the Lorenz functions for each of these windows (see Figure 1) and extract the yearly values (corresponding to the central year of the window) of g and k indices. We have plotted these yearly g and k values for all the working years, starting the recorded first year and for the third year from there and continued for successive years up to 2022 (by considering data up to 2022) for each of these chosen thirty scientists. These are then shown in Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6. The insets in each Figure show the corresponding plot of k versus g (disregarding the yearly sequence). These plots in all thirty cases of the researchers show quite a good linear fit to $k=0.5+0.39g$ (cf. Equation (1)), as obtained approximately using a (Landau-like) minimal polynomial expansion of the Lorenz function (see Section 2). The insets also show the actual or extrapolated (precursor of sand-pile SOC) point at $k=g=0.82\pm 0.02$. As we can see from Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6, for ten of the twelve Nobel prize winners, several of the other international prize winners are considered here, known sociophysicists, econophysicists, and all three of the highest rank Stanford cite-scorers, the crossing(s) of k versus g (often at multiple years), do take place convincingly. The same is also true (often marginally), for several others. The three lower rank (yet from the “top 2%”) Stanford cite-scorers did not come up to $g=k$ point. There are certainly a few notable anomalies in this analysis of the data set; e.g., B. Josephson, J. Frank (both Nobel laureates), D. Dhar (Boltzmann award winner), and A. Sen (Breakthrough prize winner) do not fit this picture of indeed reaching the SOC point. These anomalies may indicate some shortcomings of this kind of analysis. On the other hand, noting that out of twenty seven of the researchers have chosen here (neglecting the three lower rank, though from the “top 2%” Stanford cite-scorers), the visible evidence of SOC are seen for nineteen (neglecting the “no” and “marginal” entries in the last column of Table 1 for these twenty seven researchers), indicating a success rate more than 70% for identifying the outstanding researchers. In Table 2, we give quite a simple (though approximate) indicator $R=n_C^{\max }/D$ (where $n_C^{\max }$ denotes the maximum citation of any paper and D the effective Dunbar number of the researcher) to check if the researcher has achieved the SOC level or not. We see that the SOC level is achieved for $R\ge 40$, with a more than 94% coincidence rate.

In summary, as the Hirsch index h of a prolific researcher grows with the total citations $N_c$ as $h=0.5\sqrt{N_c}$ [15] and $N_c$ grows linearly with the total number $N_p$ of publications by the researcher, $N_c=D{N}_p$ (see [15,18]), where the effective Dunbar number D (∼75 [15]) of the network community in which the scientist belongs, h and $N_c$ can only give some average measures of success. In fact, most appreciated members of the community can in principle have uniformly high citations of order D for each of their publications and hence $h\ge D\simeq$ 75. Though such uniformly appreciated or cited scientists will have significantly low values of Gini and Kolkata indices, $g\simeq$ 0 and $k\simeq 0.5$. Our study here shows, notwithstanding some anomalies, most successful researchers have large fluctuations in the citations of one or more of their publications (presumably due to uneven but accurate appreciations from the usual Dunbar network or community and also possibly from outside the usual Dunbar community), which do not increase directly the D or h values, but lead to larger values of their inequality indices g and k, which may then hover around the SOC level value $g=k\simeq 0.82$, a little above the Pareto value (k = 0.80).