Probability Distribution Functions of Solar and Stellar Flares
Abstract
1. Introduction
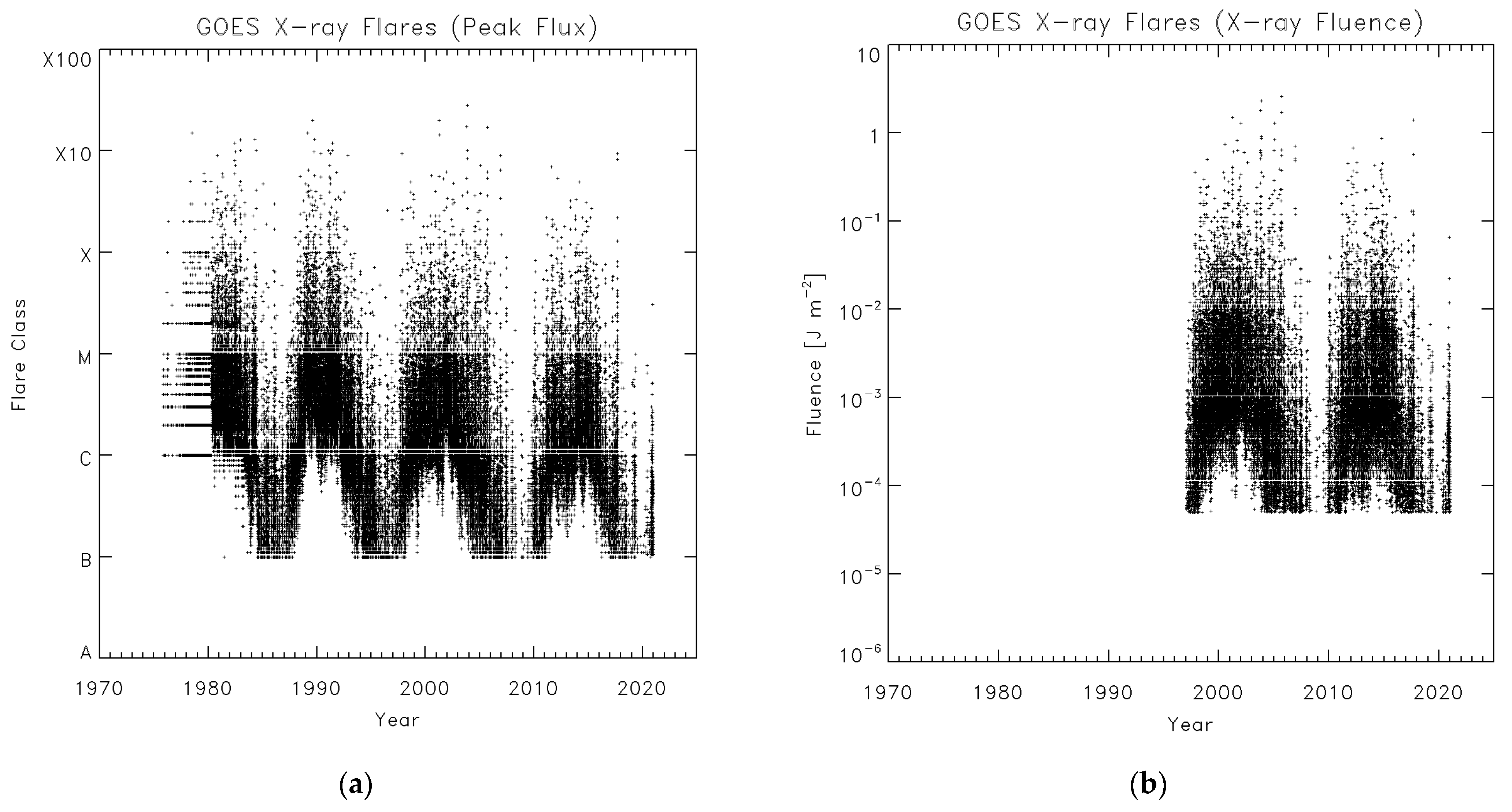
2. Data and Methods
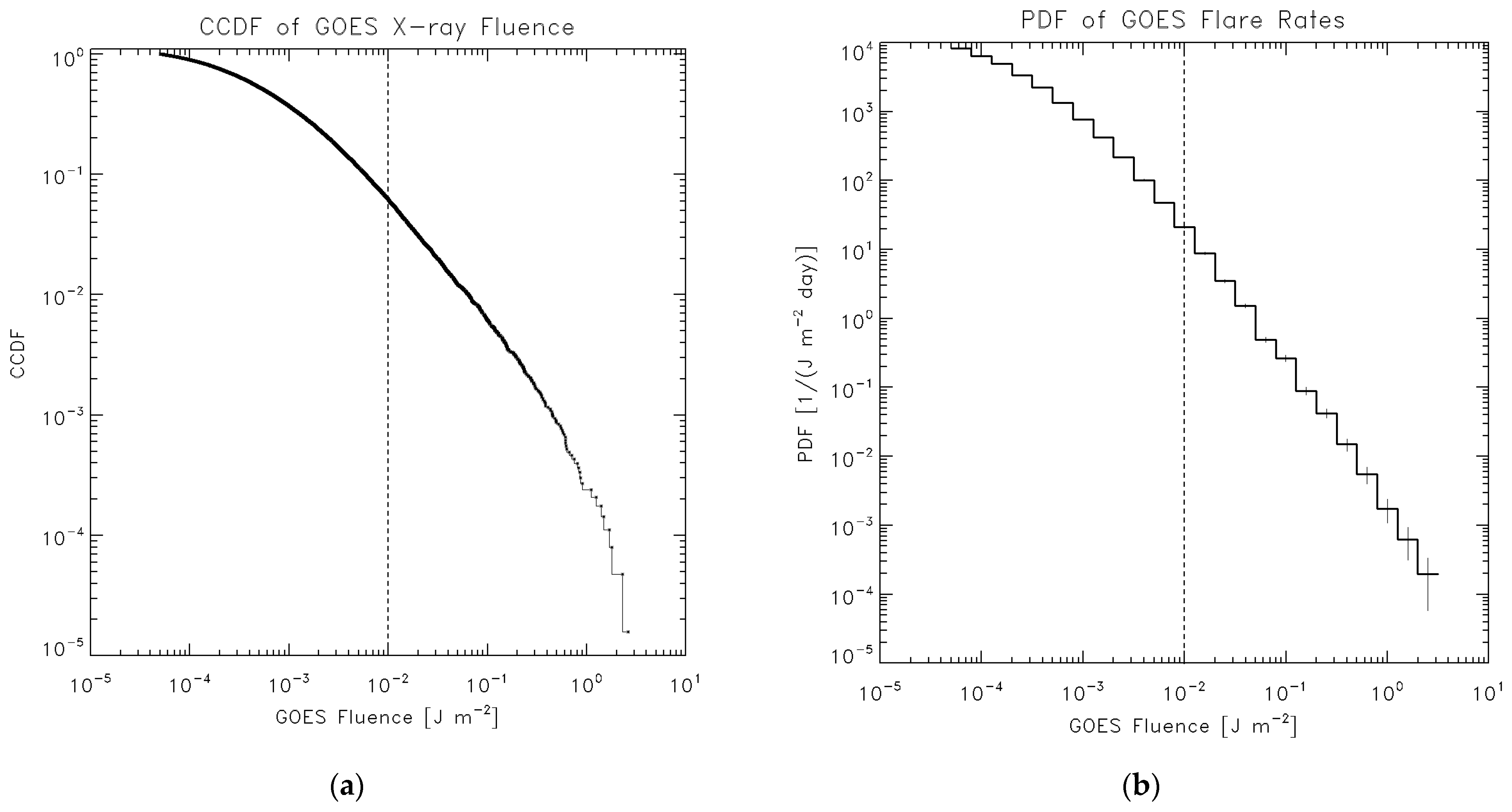
3. Results
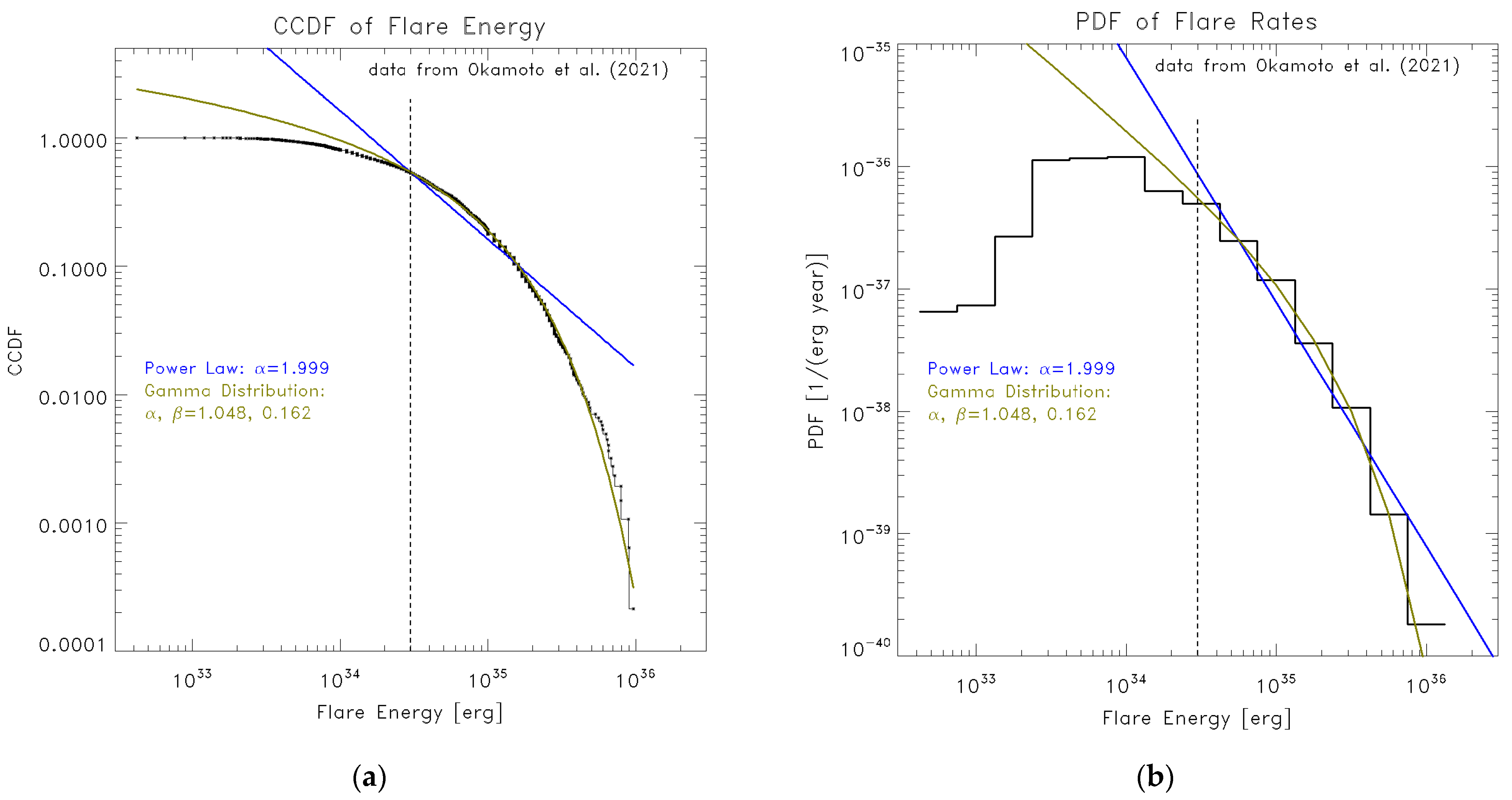
3.1. Tapered Power Law and Gamma Function Distributions
3.2. Weibull Distribution
3.3. Comparison with Published Results
3.4. Prediction of Extreme Events
4. Discussion
5. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Švestka, Ž. Solar Flares; D. Reidel Publishing Company: Dordrecht, The Netherlands, 1976. [Google Scholar] [CrossRef]
- Benz, A.O. Flare observations. Living Rev. Sol. Phys. 2017, 14, 2. [Google Scholar] [CrossRef]
- Carrington, R.C. Description of a singular appearance seen in the Sun on September 1, 1859. Mon. Not. R. Astron. Soc. 1859, 20, 13–15. [Google Scholar] [CrossRef]
- Cliver, E.W.; Schrijver, C.J.; Shibata, K.; Usoskin, I.G. Extreme solar events. Living Rev. Sol. Phys. 2022, 19, 2. [Google Scholar] [CrossRef]
- Priest, E.R.; Forbes, T.G. The magnetic nature of solar flares. Astron. Astrophys. Rev. 2002, 10, 313–377. [Google Scholar] [CrossRef]
- Lin, R.P.; Schwartz, R.A.; Kane, S.R.; Pelling, R.M.; Hurley, K.C. Solar hard X-ray microflares. Astrophys. J. 1984, 283, 421–425. [Google Scholar] [CrossRef]
- Crosby, N.; Aschwanden, M.; Dennis, B. Frequency distributions and correlations of solar X-ray flare parameters. Sol. Phys. 1993, 143, 275–299. [Google Scholar] [CrossRef]
- Parker, E.N. Nanoflares and the solar X-ray corona. Astrophys. J. 1988, 330, 474–479. [Google Scholar] [CrossRef]
- Sakurai, T. Heating mechanisms of the solar corona. Proc. Jpn. Acad. Ser. B 2017, 93, 87–97. [Google Scholar] [CrossRef]
- McIntosh, S.W.; de Pontieu, B.; Carlsson, M.; Hansteen, V.; Boerner, P.; Goossens, M. Alfvénic waves with sufficient energy to power the quiet solar corona and fast solar wind. Nature 2011, 475, 477–480. [Google Scholar] [CrossRef]
- Hinode Review Team. Achievements of Hinode in the first eleven years. Publ. Astron. Soc. Jpn. 2019, 71, R1. [Google Scholar] [CrossRef]
- Hudson, H.S. Solar flares, microflares, nanoflares, and coronal heating. Sol. Phys. 1991, 133, 357–369. [Google Scholar] [CrossRef]
- Schaefer, B.E.; King, J.R.; Deliyannis, C.P. Superflares on ordinary solar-type stars. Astrophys. J. 2000, 529, 1026–1030. [Google Scholar] [CrossRef]
- Maehara, H.; Shibayama, T.; Notsu, S.; Notsu, Y.; Nagao, T.; Kusaba, S.; Honda, S.; Nogami, D.; Shibata, K. Superflares on solar-type stars. Nature 2012, 485, 478–481. [Google Scholar] [CrossRef] [PubMed]
- Machol, J.; Viereck, R.; Peck, C.; Mothersbaugh, J., III. GOES X-ray Sensor (XRS) Operational Data; NOAA National Centers for Environmental Information (NCEI): Boulder, CO, USA, 2022. Available online: https://www.ngdc.noaa.gov/stp/satellite/goes/doc/GOES_XRS_readme.pdf (accessed on 10 December 2022).
- Bornmann, P.L.; Speich, D.; Hirman, J.; Matheson, L.; Grubb, R.; Garcia, H.A.; Viereck, R. GOES X-ray sensor and its use in predicting solar-terrestrial disturbances. In GOES-8 and Beyond. Proceedings of SPIE, Vol. 2812; Washwell, E.R., Ed.; Society of Photo-Optical Instrumentation Engineers (SPIE): Bellingham, WA, USA, 1996; pp. 291–298. [Google Scholar] [CrossRef]
- Veronig, A.; Temmer, M.; Hanslmeier, A.; Oruba, W.; Messerotti, M. Temporal aspects and frequency distributions of solar soft X-ray flares. Astron. Astrophys. 2002, 382, 1070–1080. [Google Scholar] [CrossRef]
- Donnelly, R.F. Solar flare X-ray and EUV emission: A terrestrial viewpoint. In Physics of Solar Planetary Environments; Williams, D.J., Ed.; American Geophysical Union: Washington, DC, USA, 1976; Volume 1, pp. 178–192. [Google Scholar] [CrossRef]
- Clauset, A.; Shalizi, C.R.; Newman, M.E.J. Power-law distributions in empirical data. SIAM Rev. 2009, 51, 661–703. [Google Scholar] [CrossRef]
- Kagan, Y.Y. Seismic moment distribution revisited: I. Statistical results. Geophys. J. Int. 2002, 148, 520–541. [Google Scholar] [CrossRef]
- Kagan, Y.Y. Seismic moment distributions. Geophys. J. Int. 1991, 106, 123–134. [Google Scholar] [CrossRef]
- Gutenberg, B.; Richter, C.F. Earthquake magnitude, intensity, energy, and acceleration. Bull. Seismol. Soc. Am. 1942, 32, 163–191. [Google Scholar] [CrossRef]
- Utsu, T. Representation and analysis of the earthquake size distribution: A historical review and some new approaches. Pure Appl. Geophys. 1999, 155, 509–535. [Google Scholar] [CrossRef]
- Weibull, W. The Phenomenon of Rupture in Solids. R. Swed. Inst. Engineer. Res. Proceed. 1939, 153, 1–55. Available online: https://pdfcoffee.com//the-phenomenon-of-rupture-in-solids-weibull-1939-pdf-free.html (accessed on 10 December 2022).
- Vere-Jones, D.; Robinson, R.; Yang, W. Remarks on the accelerated moment release model: Problems of model formulation, simulation and estimation. Geophys. J. Int. 2001, 144, 517–531. [Google Scholar] [CrossRef]
- Wingo, D.R. The left-truncated Weibull distribution: Theory and computation. Stat. Pap. 1989, 30, 39–48. [Google Scholar] [CrossRef]
- Stephens, M.A. EDF statistics for goodness of fit and some comparisons. J. Am. Stat. Assoc. 1974, 69, 730–737. [Google Scholar] [CrossRef]
- Akaike, H. A new look at the statistical model identification. IEEE Trans. Automat. Control 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Burnham, K.P.; Anderson, D.R.; Huyvaert, K.P. AIC model selection and multimodel inference in behavioral ecology: Some background, observations, and comparisons. Behav. Ecol. Sociobiol. 2011, 65, 23–35. [Google Scholar] [CrossRef]
- Sakurai, T.; Toriumi, S. Probability distribution functions of sunspot magnetic flux. arXiv 2022, arXiv:2211.13957. [Google Scholar] [CrossRef]
- Efron, B.; Tobshirani, R.J. An Introduction to the Bootstrap; Springer Science+Business Media: Dordrecht, The Netherlands, 1993; pp. 53–56. [Google Scholar]
- Simard, R.; L’Ecuyer, P. Computing the Two-Sided Kolmogorov-Smirnov Distribution. J. Stat. Softw. 2011, 39, 1–18. [Google Scholar] [CrossRef]
- Gopalswamy, N. Extreme solar eruptions and their space weather consequences. In Extreme Events in Geospace; Buzulukova, N., Ed.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 37–63. [Google Scholar] [CrossRef]
- Cliver, E.W.; Dietrich, W.F. The 1859 space weather event revisited: Limits of extreme activity. J. Space Weather Space Clim. 2013, 3, A31. [Google Scholar] [CrossRef]
- Emslie, A.G.; Dennis, B.R.; Shih, A.Y.; Chamberlin, P.C.; Mewaldt, R.A.; Moore, C.S.; Share, H.; Vourlidas, A.; Welsch, B.T. Global energetics of thirty-eight large solar eruptive events. Astrophys. J. 2012, 759, 71. [Google Scholar] [CrossRef]
- Koch, D.G.; Borucki, W.J.; Basri, G.; Batalha, N.M.; Brown, T.M.; Caldwell, D.; Christensen-Dalsgaard, J.; Cochran, W.D.; DeVore, E.; Dunham, E.W.; et al. Kepler mission—Design, realized photometric performance, and early science. Astrophys. J. Lett. 2010, 713, L79–L86. [Google Scholar] [CrossRef]
- Shibayama, T.; Maehara, H.; Notsu, S.; Notsu, Y.; Nagao, T.; Honda, S.; Ishii, T.; Nogami, D.; Shibata, K. Superflares on solar-type stars observed with Kepler. I. Statistical properties of superflares. Astrophys. J. Suppl. 2013, 209, 5. [Google Scholar] [CrossRef]
- Maehara, H.; Shibayama, T.; Notsu, Y.; Notsu, S.; Honda, S.; Nogami, D.; Shibata, K. Statistical properties of superflares on solar-type stars based on 1-min cadence data. Earth Planets Space 2015, 67, 59. [Google Scholar] [CrossRef]
- Okamoto, S.; Notsu, Y.; Maehara, H.; Namekata, K.; Honda, S.; Ikuta, K.; Nogami, D.; Shibata, K. Statistical properties of superflares on solar-type stars: Results using all of the Kepler primary mission data. Astrophys. J. 2021, 906, 72. [Google Scholar] [CrossRef]
- Deluca, A.; Corral, Á. Fitting and goodness-of-fit test of non-truncated and truncated power-law distributions. Acta Geophys. 2013, 61, 1351–1394. [Google Scholar] [CrossRef]
- Corral, Á.; Gonzalez, Á. Power law size distributions in geoscience revisited. Earth Space Sci. 2019, 6, 673–697. [Google Scholar] [CrossRef]

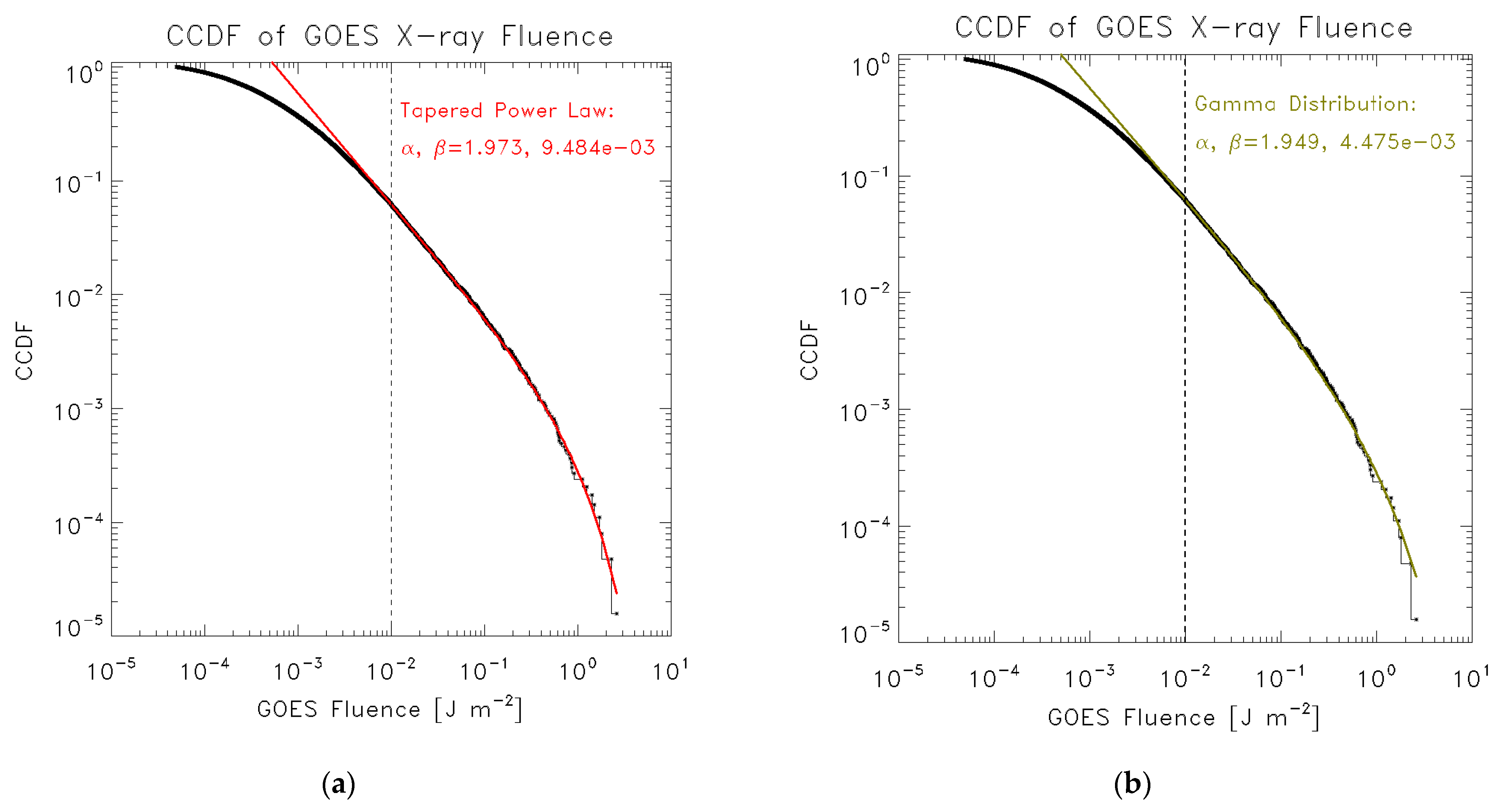

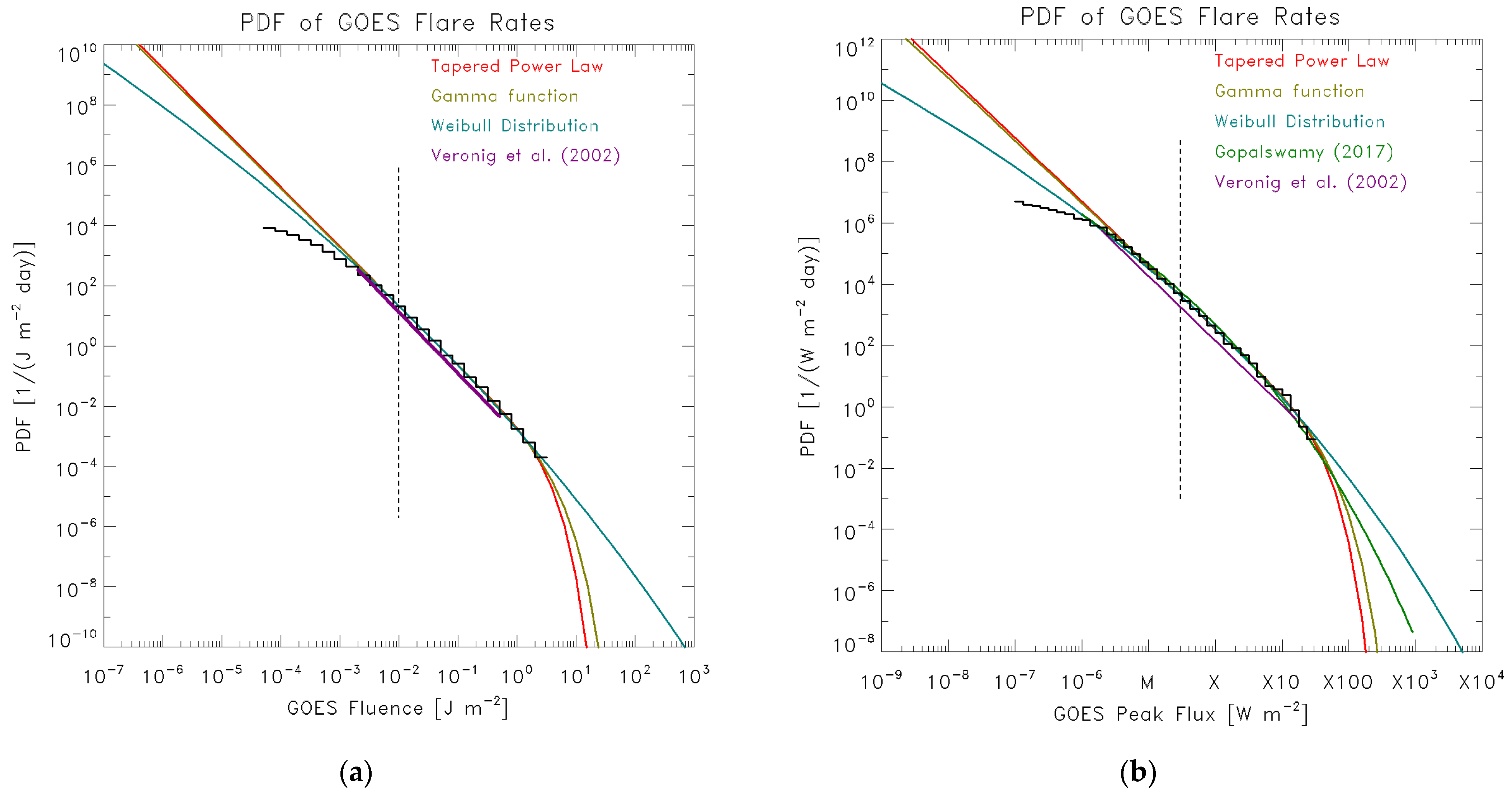

| Model | α | β | ΔAIC | √N × KS | K-S p-Value |
|---|---|---|---|---|---|
| Power law | 2.015 ± 0.023 | 11.6 | 0.69 | 0.72 | |
| Tapered power law | 1.973 ± 0.021 | 0.00948 ± 0.0031 | 0.00 | 0.47 | 0.98 |
| Gamma function | 1.949 ± 0.032 | 0.00448 ± 0.0020 | 1.10 | 0.57 | 0.90 |
| Weibull | k = 0.0648 ± 0.0242 | 14.7 ± 5.9 | 7.20 | 0.69 | 0.73 |
| Model | α | β | ΔAIC | √N × KS | K-S p-Value |
|---|---|---|---|---|---|
| Power law | 2.162 ± 0.028 | 15.7 | 0.89 | 0.41 | |
| Tapered power law | 2.077 ± 0.032 | 0.026 ± 0.007 | 0.00 | 0.40 | 0.99 |
| Gamma function | 2.040 ± 0.045 | 0.014 ± 0.005 | 1.48 | 0.44 | 0.99 |
| Weibull | k = 0.104 ± 0.030 | 10.2 ± 3.3 | 7.00 | 0.57 | 0.90 |
| X-ray Fluence (J m−2) | Approx. GOES Flux | Total Energy (erg) | Interval (Years) |
|---|---|---|---|
| 1.0 × 10−2 | M1.0 | 1.5 × 1031 | 1.3 × 10−2/1.3 × 10−2 |
| 1.0 × 10−1 | M9.0 | 8.8 × 1031 | 1.4 × 10−1/1.4 × 10−1 |
| 1.0 × 100 | X7 | 5.1 × 1032 | 3.0 × 100/2.3 × 100 |
| 2.0 × 100 | X15 | 8.7 × 1032 | 1.2 × 101/7.3 × 100 |
| 5.0 × 100 | X36 | 1.8 × 1033 | 1.8 × 102/5.6 × 101 |
| 6.3 × 100 | X45 † | 2.1 × 1033 | 4.6 × 102/1.1 × 102 |
| X-ray Fluence (J m−2) | Approx. GOES Flux | Total Energy (erg) | Interval (Years) |
|---|---|---|---|
| 6.5 × 100/9.9 × 100 | X46/X69 | 2.2 × 1033/3.0 × 1033 | 1.0 × 103 |
| 1.0 × 101/1.6 × 101 | X70/X108 | 3.0 × 1033/4.3 × 1033 | 1.0 × 104 |
| 1.4 × 101/2.3 × 101 | X95/X152 | 3.8 × 1033/5.6 × 1033 | 1.0 × 105 |
| 1.8 × 101/3.0 × 101 | X122/X197 | 4.7 × 1033/6.9 × 1033 | 1.0 × 106 |
| 2.7 × 101/4.6 × 101 | X177/X293 | 6.3 × 1033/9.5 × 1033 | 1.0 × 108 |
| 3.4 × 101/5.9 × 101 | X224/X374 | 7.7 × 1033/1.2 × 1034 | 4.6 × 109 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sakurai, T. Probability Distribution Functions of Solar and Stellar Flares. Physics 2023, 5, 11-23. https://doi.org/10.3390/physics5010002
Sakurai T. Probability Distribution Functions of Solar and Stellar Flares. Physics. 2023; 5(1):11-23. https://doi.org/10.3390/physics5010002
Chicago/Turabian StyleSakurai, Takashi. 2023. "Probability Distribution Functions of Solar and Stellar Flares" Physics 5, no. 1: 11-23. https://doi.org/10.3390/physics5010002
APA StyleSakurai, T. (2023). Probability Distribution Functions of Solar and Stellar Flares. Physics, 5(1), 11-23. https://doi.org/10.3390/physics5010002





