BFSS Matrix Model Cosmology: Progress and Challenges
Abstract
:1. Introduction
2. BFSS Matrix Model
3. Emergent Metric Space-Time
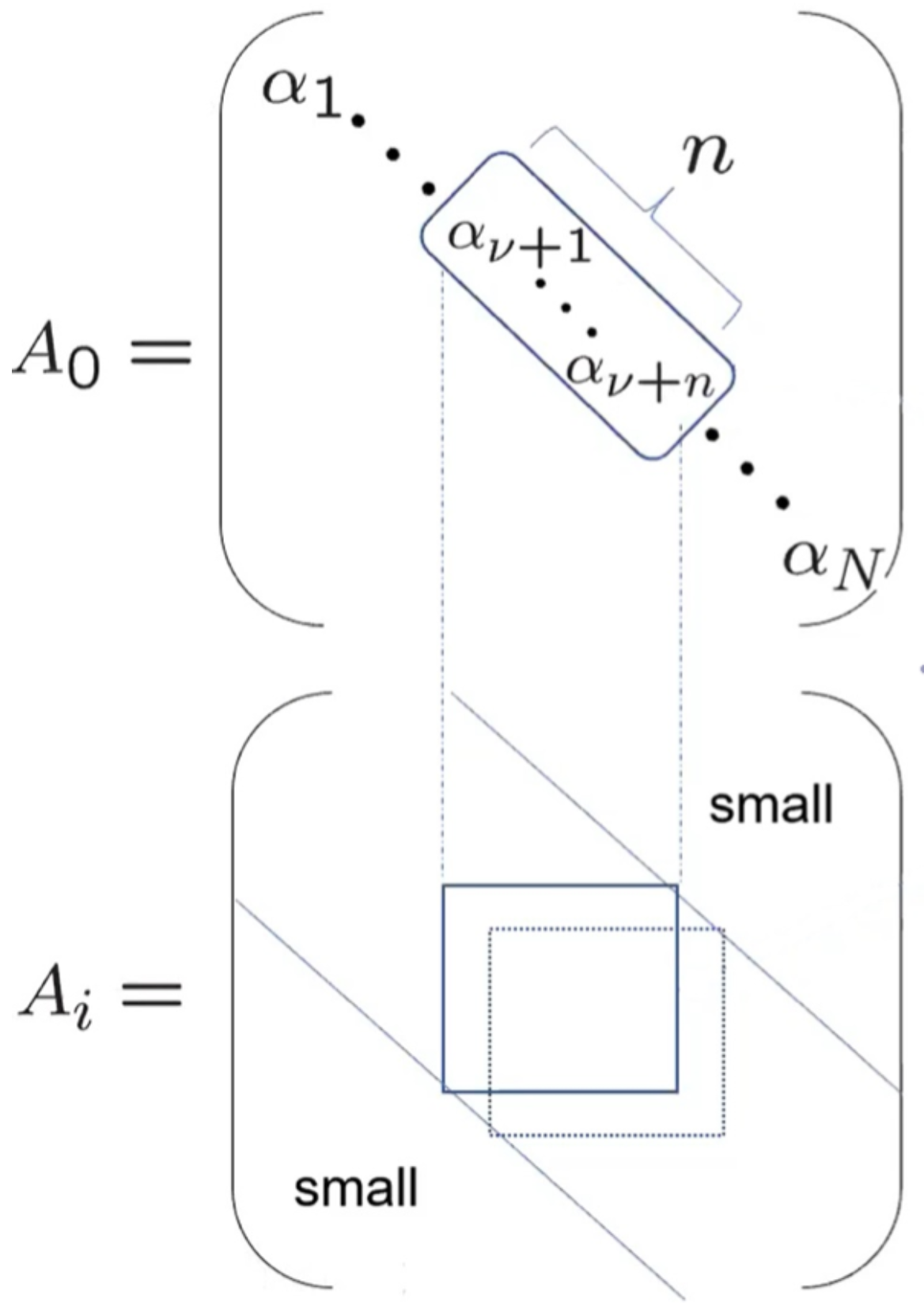
4. Emergent Early Universe Cosmology
5. Challenges and Future Directions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guth, A.H. The inflationary universe: A possible solution to the horizon and flatness problems. Phys. Rev. D 1981, 23, 347–356, Reprinted: Adv. Ser. Astrophys. Cosmol. 1987, 3, 139–148. [Google Scholar] [CrossRef] [Green Version]
- Brout, R.; Englert, F.; Gunzig, E. The creation of the universe as a quantum phenomenon. Ann. Phys. 1978, 115, 78–106. [Google Scholar] [CrossRef]
- Starobinsky, A.A. A New type of isotropic cosmological models without singularity. Phys. Lett. B 1980, 91, 99–102. [Google Scholar] [CrossRef]
- Sato, K. First order phase transition of a vacuum and expansion of the universe. Mon. Not. R. Astron. Soc. 1981, 195, 467–479. [Google Scholar] [CrossRef] [Green Version]
- Brandenberger, R.H. Alternatives to the inflationary paradigm of structure formation. Int. J. Mod. Phys. Conf. Ser. 2011, 1, 67–79. [Google Scholar] [CrossRef] [Green Version]
- Brandenberger, R.H. Cosmology of the very early universe. AIP Conf. Proc. 2010, 1268, 3–70. [Google Scholar]
- Brandenberger, R.; Peter, P. Bouncing cosmologies: Progress and problems. Found. Phys. 2017, 47, 797–850. [Google Scholar] [CrossRef] [Green Version]
- Ooguri, H.; Vafa, C. On the geometry of the string landscape and the swampland. Nucl. Phys. B 2007, 766, 21–33. [Google Scholar] [CrossRef] [Green Version]
- Obied, G.; Ooguri, H.; Spodyneiko, L.; Vafa, C. De Sitter space and the swampland. arXiv 2018, arXiv:1806.08362. [Google Scholar] [CrossRef]
- Bedroya, A.; Vafa, C. Trans-Planckian censorship and the swampland. J. High Energy Phys. 2020, 2009, 123. [Google Scholar] [CrossRef]
- Bedroya, A.; Brandenberger, R.; Loverde, M.; Vafa, C. Trans-Planckian censorship and inflationary cosmology. Phys. Rev. D 2020, 101, 103502. [Google Scholar] [CrossRef]
- Brandenberger, R. String cosmology and the breakdown of local effective field theory. arXiv 2021, arXiv:2112.04082. [Google Scholar] [CrossRef]
- Brandenberger, R.; Kamali, V. Unitarity problems for an effective field theory description of early universe cosmology. Eur. Phys. J. C 2022, 82, 818. [Google Scholar] [CrossRef] [PubMed]
- Dvali, G.; Gomez, C.; Zell, S. Quantum break-time of de Sitter. J. Cosmol. Astrpart. Phys. 2017, 6, 028. [Google Scholar] [CrossRef] [Green Version]
- Dvali, G.; Gomez, C. On exclusion of positive cosmological constant. Fortsch. Phys. 2019, 67, 1800092. [Google Scholar] [CrossRef]
- Dvali, G.; Gomez, C.; Zell, S. Quantum breaking bound on de Sitter and swampland. Fortsch. Phys. 2019, 67, 1800094. [Google Scholar] [CrossRef] [Green Version]
- Brahma, S.; Dasgupta, K.; Tatar, R. Four-dimensional de Sitter space is a Glauber-Sudarshan state in string theory. J. High Energy Phys. 2021, 2021, 114. [Google Scholar] [CrossRef]
- Brahma, S.; Dasgupta, K.; Tatar, R. de Sitter space as a Glauber-Sudarshan state. J. High Energy Phys. 2021, 2021, 104. [Google Scholar] [CrossRef]
- Bernardo, H.; Brahma, S.; Dasgupta, K.; Tatar, R. Crisis on infinite earths: Short-lived de Sitter vacua in the string theory landscape. J. High Energy Phys. 2021, 2021, 37. [Google Scholar] [CrossRef]
- Brahma, S.; Brandenberger, R.; Laliberte, S. Emergent cosmology from matrix theory. J. High Energy Phys. 2022, 3, 67. [Google Scholar] [CrossRef]
- Brahma, S.; Brandenberger, R.; Laliberte, S. Emergent metric space-time from matrix theory. J. High Energy Phys. 2022, 9, 31. [Google Scholar] [CrossRef]
- Banks, T.; Fischler, W.; Shenker, S.H.; Susskind, L. M theory as a matrix model: A Conjecture. Phys. Rev. D 1997, 55, 5112–5128. [Google Scholar] [CrossRef] [Green Version]
- Hoppe, J. Quantum Theory of a Massless Relativistic Surface. Ph.D. Thesis, Massachusetts Institute of Technology (MIT), Cambridge, MA, USA, 1982. Available online: http://dspace.mit.edu/handle/1721.1/15717 (accessed on 5 December 2022).
- de Witt, B.; Hoppe, J.; Nicolai, H. On the quantum mechanics of supermembranes. Nucl. Phys. B 1988, 305, 545–581. [Google Scholar] [CrossRef] [Green Version]
- Das, S.R.; Jevicki, A. String field theory and physical interpretation of D=1 strings. Mod. Phys. Lett. A 1990, 5, 1639–1650. [Google Scholar] [CrossRef]
- Dijkgraaf, R.; Verlinde, E.P.; Verlinde, H.L. Matrix string theory. Nucl. Phys. B 1997, 500, 43–61. [Google Scholar] [CrossRef] [Green Version]
- Craps, B.; Sethi, S.; Verlinde, E.P. A matrix big bang. J. High Energy Phys. 2005, 10, 5. [Google Scholar] [CrossRef] [Green Version]
- Blau, M.; O’Loughlin, M. DLCQ and plane wave matrix big bang models. J. High Energy Phys. 2008, 9, 97. [Google Scholar] [CrossRef] [Green Version]
- Berenstein, D.E.; Maldacena, J.M.; Nastase, H.S. Strings in flat space and pp waves from N=4 superYang-Mills. J. High Energy Phys. 2002, 4, 13. [Google Scholar] [CrossRef] [Green Version]
- Maldacena, J.M.; Nunez, C. Towards the large N limit of pure N=1 superYang-Mills. Phys. Rev. Lett. 2001, 86, 588–591. [Google Scholar] [CrossRef] [Green Version]
- Craps, B.; Evnin, O. Light-like big bang singularities in string and matrix theories. Class. Quant. Grav. 2011, 28, 204006. [Google Scholar] [CrossRef] [Green Version]
- Smolin, L. M theory as a matrix extension of Chern-Simons theory. Nucl. Phys. B 2000, 591, 227–242. [Google Scholar] [CrossRef]
- Yargic, Y.; Lanier, J.; Smolin, L.; Wecker, D. A cubic matrix action for the Standard Model and beyond. arXiv 2022, arXiv:2201.04183. [Google Scholar] [CrossRef]
- Brandenberger, R.H.; Vafa, C. Superstrings in the early universe. Nucl. Phys. B 1989, 316, 391–410. [Google Scholar] [CrossRef]
- Kripfganz, J.; Perlt, H. Cosmological impact of winding strings. Class. Quant. Grav. 1988, 5, 453. [Google Scholar] [CrossRef] [Green Version]
- Brandenberger, R.H. String gas cosmology. In String Cosmology; Erdmenger, J., Ed.; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2009; pp. 193–230. Available online: https://www.lehmanns.ch/shop/technik/24731252-9783527628070-string-cosmology (accessed on 5 December 2022).
- Hagedorn, R. Statistical thermodynamics of strong interactions at high-energies. Nuovo Cim. Suppl. 1965, 3, 147–186, Reprinted: Quark–Gluon Plasma: Theoretical Foundations; Kapusta, J.I., Müller, B., Rafelski, J., Eds.; North-Holland: Amsterdam, The Netherlands; 2003; pp. 24–63. Available online: http://cds.cern.ch/record/346206 (accessed on 5 December 2022).
- Vilenkin, A. Gravitational field of vacuum domain walls and strings. Phys. Rev. D 1981, 23, 852–857. [Google Scholar] [CrossRef]
- Nayeri, A.; Brandenberger, R.H.; Vafa, C. Producing a scale-invariant spectrum of perturbations in a Hagedorn phase of string cosmology. Phys. Rev. Lett. 2006, 97, 021302. [Google Scholar] [CrossRef] [Green Version]
- Brandenberger, R.H.; Nayeri, A.; Patil, S.P.; Vafa, C. Tensor modes from a primordial Hagedorn phase of string cosmology. Phys. Rev. Lett. 2007, 98, 231302. [Google Scholar] [CrossRef] [Green Version]
- Agrawal, P.; Gukov, S.; Obied, G.; Vafa, C. Topological gravity as the early phase of our universe. arXiv 2020, arXiv:2009.10077. [Google Scholar] [CrossRef]
- Ydri, B. Review of M(atrix)-theory, type IIB matrix model and Matrix String Theory. arXiv 2017, arXiv:1708.00734. [Google Scholar] [CrossRef]
- Taylor, W. M(atrix) theory: Matrix quantum mechanics as a fundamental theory. Rev. Mod. Phys. 2001, 73, 419–462. [Google Scholar] [CrossRef] [Green Version]
- Ishibashi, N.; Kawai, H.; Kitazawa, Y.; Tsuchiya, A. A large-N reduced model as superstring. Nucl. Phys. B 1997, 498, 467–491. [Google Scholar] [CrossRef]
- Aoki, H.; Iso, S.; Kawai, H.; Kitazawa, Y.; Tada, T. Space-time structures from IIB matrix model. Prog. Theor. Phys. 1998, 99, 713–746. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.W.; Nishimura, J.; Tsuchiya, A. Expanding (3+1)-dimensional universe from a Lorentzian matrix model for superstring theory in (9+1)-dimensions. Phys. Rev. Lett. 2012, 108, 011601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nishimura, J.; Vernizzi, G. Spontaneous breakdown of Lorentz invariance in IIB matrix model. J. High Energy Phys. 2000, 4, 15. [Google Scholar] [CrossRef] [Green Version]
- Ambjorn, J.; Anagnostopoulos, K.N.; Bietenholz, W.; Hotta, T.; Nishimura, J. Monte Carlo studies of the IIB matrix model at large N. J. High Energy Phys. 2000, 7, 11. [Google Scholar] [CrossRef]
- Nishimura, J.; Sugino, F. Dynamical generation of four-dimensional space-time in the IIB matrix model. J. High Energy Phys. 2002, 5, 1. [Google Scholar] [CrossRef]
- Kawai, H.; Kawamoto, S.; Kuroki, T.; Matsuo, T.; Shinohara, S. Mean field approximation of IIB matrix model and emergence of four-dimensional space-time. Nucl. Phys. B 2002, 647, 153–189. [Google Scholar] [CrossRef] [Green Version]
- Aoyama, T.; Nishimura, J.; Okubo, T. Spontaneous breaking of the rotational symmetry in dimensionally reduced super Yang-Mills models. Prog. Theor. Phys. 2011, 125, 537–563. [Google Scholar] [CrossRef] [Green Version]
- Nishimura, J.; Okubo, T.; Sugino, F. Systematic study of the SO(10) symmetry breaking vacua in the matrix model for type IIB superstrings. J. High Energy Phys. 2011, 10, 135. [Google Scholar] [CrossRef] [Green Version]
- Kabat, D.N.; Lifschytz, G.; Lowe, D.A. Black hole thermodynamics from calculations in strongly coupled gauge theory. Int. J. Mod. Phys. A 2001, 16, 856–865. [Google Scholar] [CrossRef]
- Kabat, D.N.; Lifschytz, G.; Lowe, D.A. Black hole entropy from nonperturbative gauge theory. Phys. Rev. D 2001, 64, 124015. [Google Scholar] [CrossRef]
- Watson, S.; Brandenberger, R. Stabilization of extra dimensions at tree level. J. Cosmol. Astrpart. Phys. 2003, 11, 8. [Google Scholar] [CrossRef]
- Patil, S.P.; Brandenberger, R.H. The cosmology of massless string modes. J. Cosmol. Astrpart. Phys. 2006, 1, 5. [Google Scholar] [CrossRef] [Green Version]
- Brahma, S.; Brandenberger, R.; Laliberte, S. Spontaneous symmetry breaking in the BFSS model: Analytical results using the Gaussian expansion method. arXiv 2022, arXiv:2209.01255. [Google Scholar] [CrossRef]
- Nishimura, J. New perspectives on the emergence of (3+1)D expanding space-time in the Lorentzian type IIB matrix model. PoS 2020, CORFU2019, 178. [Google Scholar] [CrossRef]
- Hirasawa, M.; Anagnostopoulos, K.; Azuma, T.; Hatakeyama, K.; Ito, Y.; Nishimura, J.; Papadoudis, S.; Tsuchiya, A. A new phase in the Lorentzian type IIB matrix model and the emergence of continuous space-time. PoS 2022, LATTICE2021, 428. [Google Scholar] [CrossRef]
- Hatakeyama, K.; Anagnostopoulos, K.; Azuma, T.; Hirasawa, M.; Ito, Y.; Nishimura, J.; Papadoudis, S.; Tsuchiya, A. Relationship between the Euclidean and Lorentzian versions of the type IIB matrix model. PoS 2022, LATTICE2021, 341. [Google Scholar] [CrossRef]
- Hatakeyama, K.; Anagnostopoulos, K.; Azuma, T.; Hirasawa, M.; Ito, Y.; Nishimura, J.; Papadoudis, S.; Tsuchiya, A. Complex Langevin studies of the emergent space-time in the type IIB matrix model. In Proceedings of the East Asia Joint Symposium on Fields and Strings 2021; Iso, S., Itoyama, H., Maruyoshi, K., Nishinaka, T., Oota, T., Sakai, K., Tsuchiya, A., Eds.; World Scientific Co. Ltd.: Singapore, 2022; pp. 9–18. [Google Scholar] [CrossRef]
- Khoury, J.; Ovrut, B.A.; Steinhardt, P.J.; Turok, N. The ekpyrotic universe: Colliding branes and the origin of the hot big bang. Phys. Rev. D 2001, 64, 123522. [Google Scholar] [CrossRef] [Green Version]
- Khoury, J.; Ovrut, B.A.; Seiberg, N.; Steinhardt, P.J.; Turok, N. From big crunch to big bang. Phys. Rev. D 2002, 65, 086007. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.W.; Nishimura, J.; Tsuchiya, A. Late time behaviors of the expanding universe in the IIB matrix model. J. High Energy Phys. 2012, 10, 147. [Google Scholar] [CrossRef]
- Ito, Y.; Nishimura, J.; Tsuchiya, A. Power-law expansion of the Universe from the bosonic Lorentzian type IIB matrix model. J. High Energy Phys. 2015, 11, 70. [Google Scholar] [CrossRef] [Green Version]
- Hatakeyama, K.; Matsumoto, A.; Nishimura, J.; Tsuchiya, A.; Yosprakob, A. The emergence of expanding space–time and intersecting D-branes from classical solutions in the Lorentzian type IIB matrix model. Prog. Theor. Exp. Phys. 2020, 2020, 43B10. [Google Scholar] [CrossRef]
- Wetterich, C. Cosmology and the fate of dilatation symmetry. Nucl. Phys. B 1988, 302, 668–696. [Google Scholar] [CrossRef] [Green Version]
- Peebles, P.J.E.; Ratra, B. Cosmology with a time variable cosmological constant. Astrophys. J. 1988, 325, L17–L20. Available online: https://articles.adsabs.harvard.edu/full/1988ApJ...325L..17P (accessed on 5 December 2022). [CrossRef]
- Ratra, B.; Peebles, P.J.E. Cosmological consequences of a rolling homogeneous scalar field. Phys. Rev. D 1988, 37, 3406–3427. [Google Scholar] [CrossRef]
- Bernardo, H.; Brandenberger, R.; Fröhlich, J. Towards a dark sector model from string theory. J. Cosmol. Astrpart. Phys. 2022, 9, 40. [Google Scholar] [CrossRef]
- Mukhanov, V.F.; Feldman, H.A.; Brandenberger, R.H. Theory of cosmological perturbations. Phys. Rep. 1992, 215, 203–333. [Google Scholar] [CrossRef] [Green Version]
- Brandenberger, R. Lectures on the theory of cosmological perturbations. In The Early Universe and Observational Cosmology; Bretón, N., Cervantes-Cota, J.L., Salgado, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 127–167. [Google Scholar] [CrossRef] [Green Version]
- Kawahara, N.; Nishimura, J.; Takeuchi, S. High temperature expansion in supersymmetric matrix quantum mechanics. J. High Energy Phys. 2007, 12, 103. [Google Scholar] [CrossRef]
- Brandenberger, R.; Wang, Z. Nonsingular ekpyrotic cosmology with a nearly scale-invariant spectrum of cosmological perturbations and gravitational waves. Phys. Rev. D 2020, 101, 063522. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brahma, S.; Brandenberger, R.; Laliberte, S. BFSS Matrix Model Cosmology: Progress and Challenges. Physics 2023, 5, 1-10. https://doi.org/10.3390/physics5010001
Brahma S, Brandenberger R, Laliberte S. BFSS Matrix Model Cosmology: Progress and Challenges. Physics. 2023; 5(1):1-10. https://doi.org/10.3390/physics5010001
Chicago/Turabian StyleBrahma, Suddhasattwa, Robert Brandenberger, and Samuel Laliberte. 2023. "BFSS Matrix Model Cosmology: Progress and Challenges" Physics 5, no. 1: 1-10. https://doi.org/10.3390/physics5010001
APA StyleBrahma, S., Brandenberger, R., & Laliberte, S. (2023). BFSS Matrix Model Cosmology: Progress and Challenges. Physics, 5(1), 1-10. https://doi.org/10.3390/physics5010001




