Generalized Extended Uncertainty Principle Black Holes: Shadow and Lensing in the Macro- and Microscopic Realms
Abstract
:1. Introduction
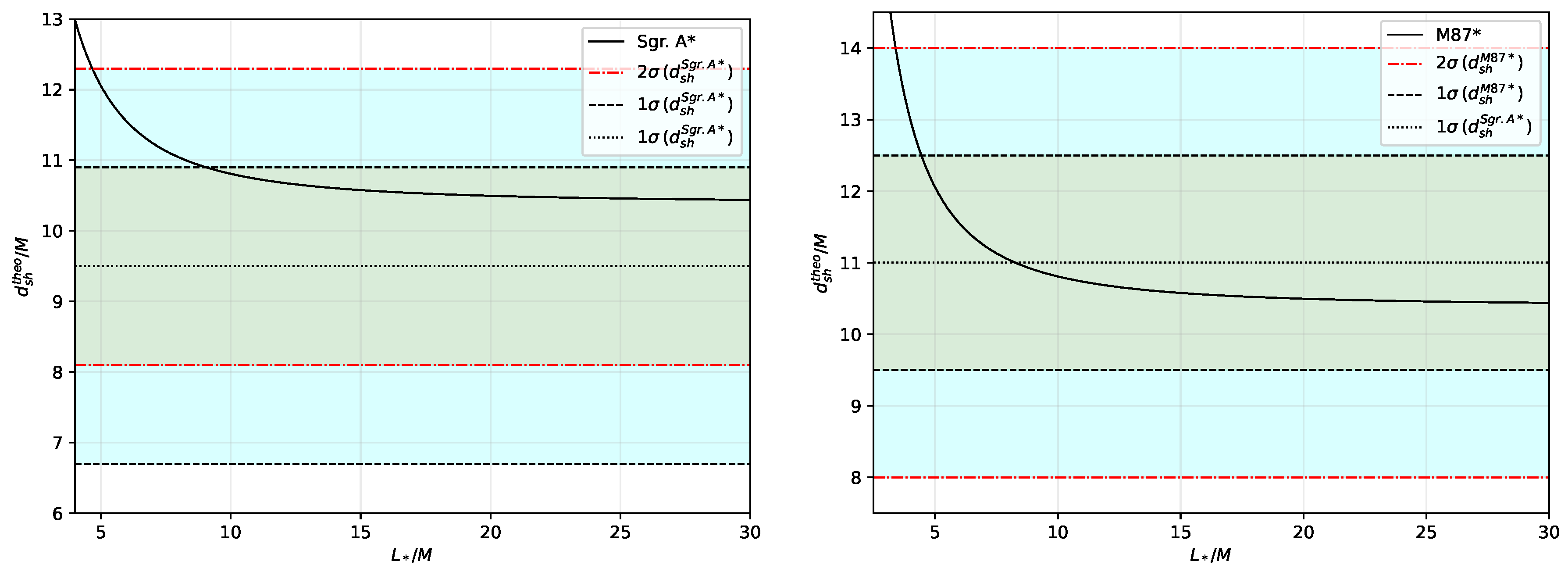
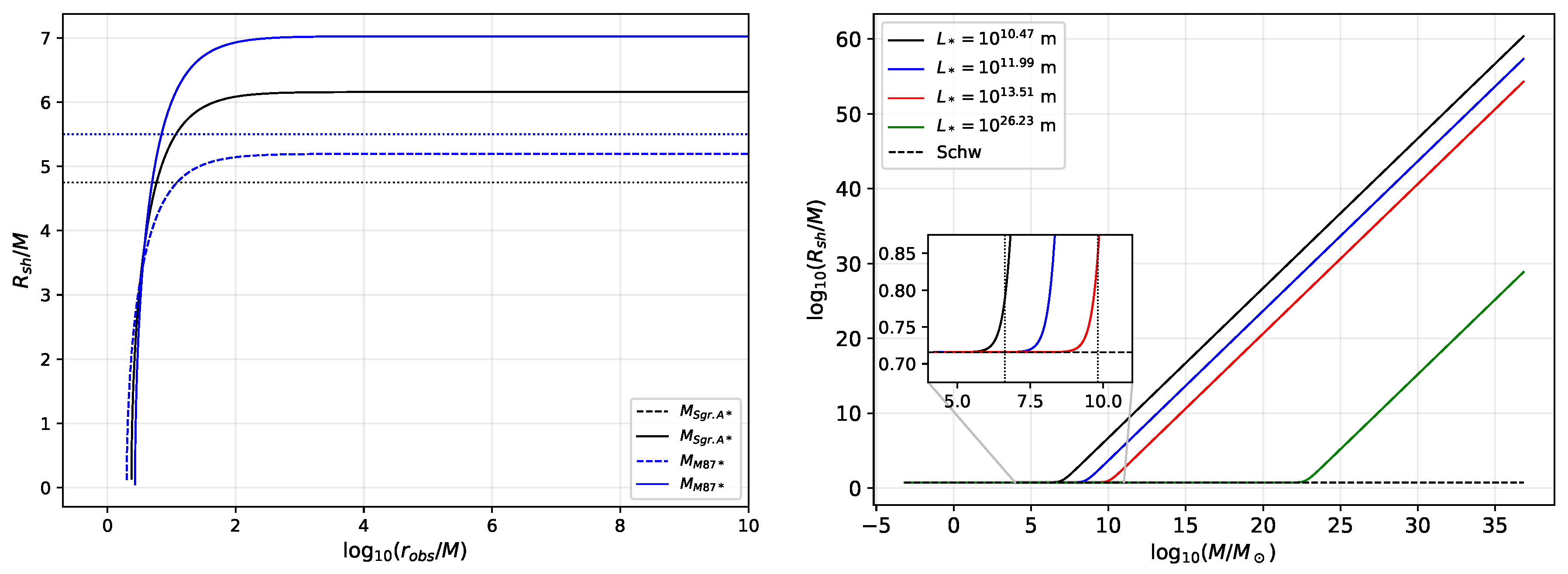
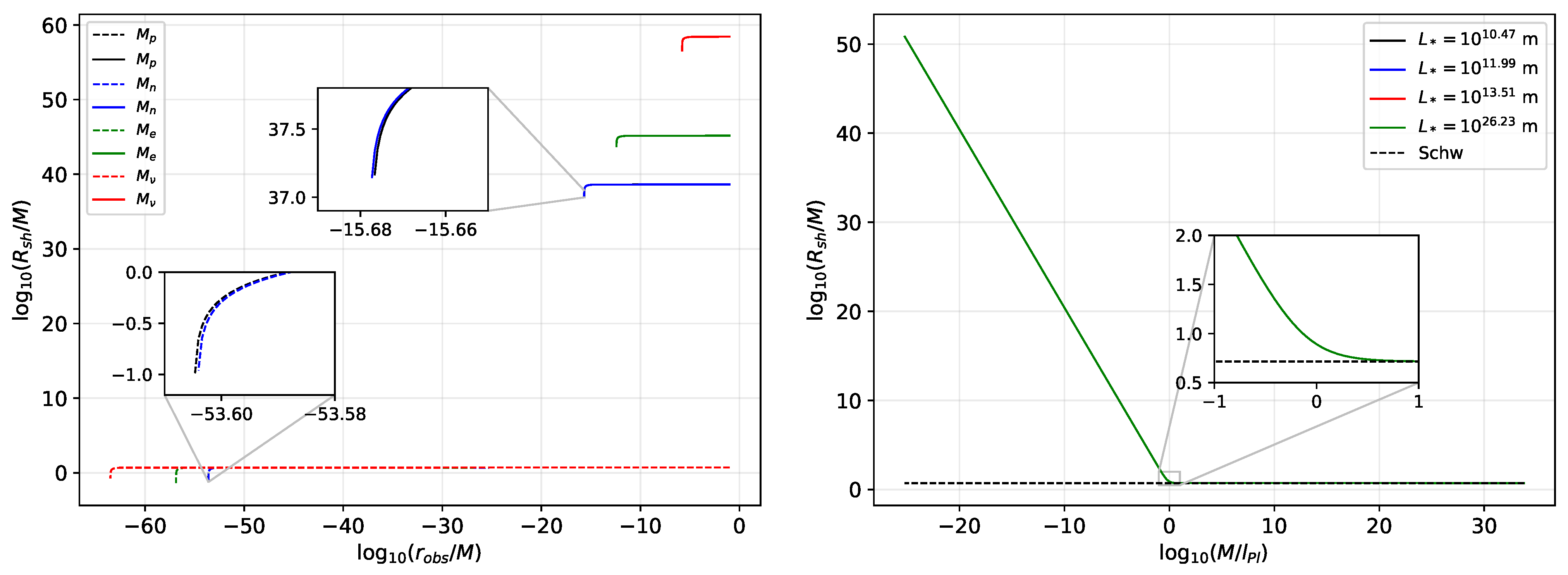
2. Shadow and Constraints to the Large Fundamental Length Scale
3. Weak Deflection Angle
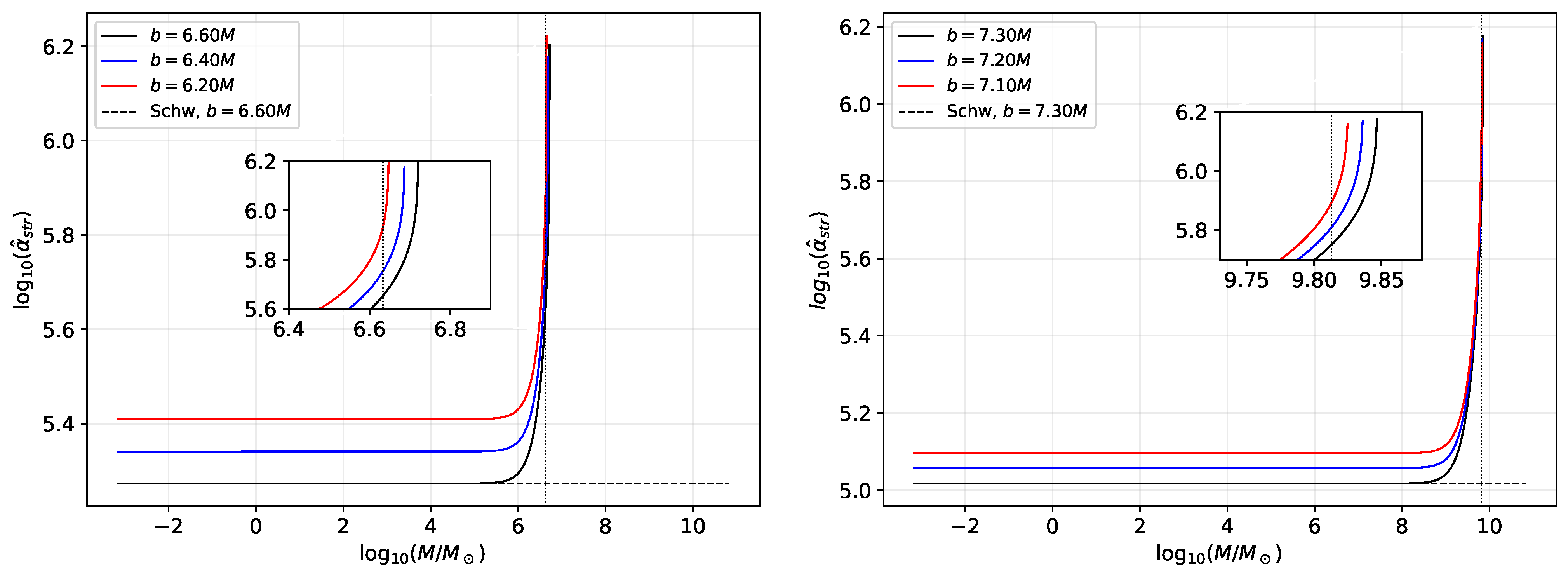
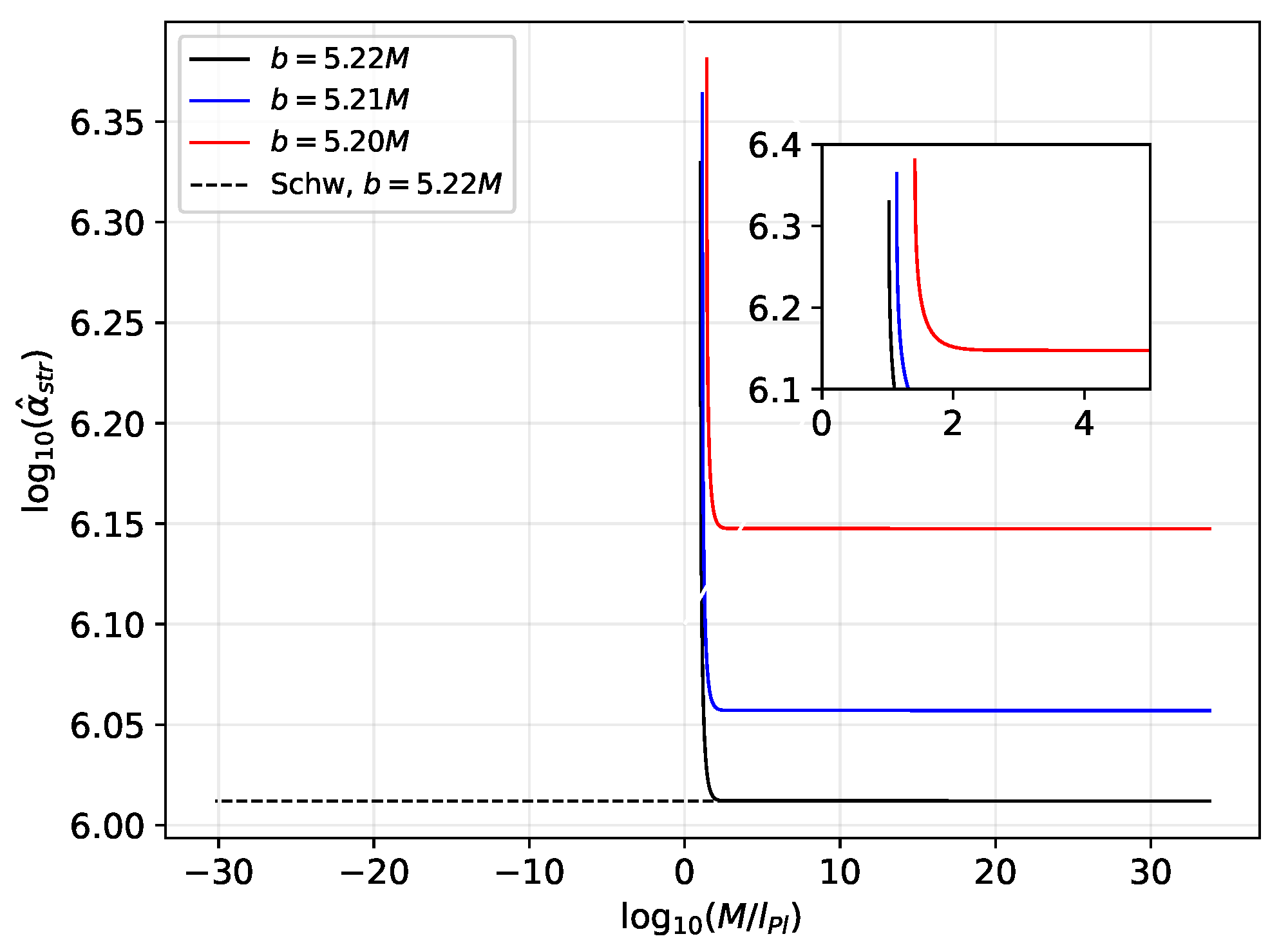
4. Strong Deflection Angle
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Akiyama, K. et al. [Event Horizon Telescope Collaboration] First M87 Event Horizon Telescope results. I. The shadow of the supermassive black hole. Astrophys. J. Lett. 2019, 875, L1. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope Collaboration] First Sagittarius A* event Horizon Telescope results. I. The shadow of the supermassive black hole in the center of the Milky Way. Astrophys. J. Lett. 2022, 930, L12. [Google Scholar] [CrossRef]
- Schwarzschild, K. Über das Gravitationsfeld eines Massenpunktes nach der Einsteinschen Theorie. Sitzungsber. Preuss. Akad. Wiss. Berl. Math. Phys. 1916, 1916, 189–196. Available online: https://www.jp-petit.org/Schwarzschild-1916-exterior-de.pdf (accessed on 1 October 2022).
- Schwarzschild, K. “Golden Oldie”: On the gravitational field of a mass point according to Einstein’s theory. Gen. Relat. Gravit. 2003, 35, 951–959. [Google Scholar] [CrossRef]
- Kerr, R.P. Gravitational field of a spinning mass as an example of algebraically special metrics. Phys. Rev. Lett. 1963, 11, 237–238. [Google Scholar] [CrossRef]
- Rovelli, C. Quantum Gravity; Cambridge University Press: Cambridge, MA, USA, 2004. [Google Scholar] [CrossRef]
- Wheeler, J.A. Geons. Phys. Rev. 1955, 97, 511–536. [Google Scholar] [CrossRef]
- Maggiore, M. Quantum groups, gravity, and the generalized uncertainty principle. Phys. Rev. D 1994, 49, 5182–5187. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Maggiore, M. A generalized uncertainty principle in quantum gravity. Phys. Lett. B 1993, 304, 65–69. [Google Scholar] [CrossRef] [Green Version]
- Maggiore, M. The algebraic structure of the generalized uncertainty principle. Phys. Lett. B 1993, 319, 83–86. [Google Scholar] [CrossRef] [Green Version]
- Amati, D.; Ciafaloni, M.; Veneziano, G. Can spacetime be probed below the string size? Phys. Lett. B 1989, 216, 41–47. [Google Scholar] [CrossRef]
- Bambi, C.; Urban, F.R. Natural extension of the generalized uncertainty principle. Class. Quant. Grav. 2008, 25, 095006. [Google Scholar] [CrossRef]
- Costa Filho, R.N.; Braga, J.P.M.; Lira, J.H.S.; Andrade, J.S., Jr. Extended uncertainty from first principles. Phys. Lett. B 2016, 755, 367–370. [Google Scholar] [CrossRef]
- Tawfik, A.; Diab, A. Generalized uncertainty principle: Approaches and applications. Int. J. Mod. Phys. D 2014, 23, 1430025. [Google Scholar] [CrossRef] [Green Version]
- Zhu, T.; Ren, J.-R.; Li, M.-F. Influence of generalized and extended uncertainty principle on thermodynamics of FRW universe. Phys. Lett. B 2009, 674, 204–209. [Google Scholar] [CrossRef] [Green Version]
- Mignemi, S. Extended uncertainty principle and the geometry of (anti)-de-sitter space. Mod. Phys. Lett. A 2010, 25, 1697–1703. [Google Scholar] [CrossRef]
- Dabrowski, M.P.; Wagner, F. Extended uncertainty principle for Rindler and cosmological horizons. Eur. Phys. J. C 2019, 79, 716. [Google Scholar] [CrossRef] [Green Version]
- Hamil, B.; Merad, M.; Birkandan, T. Effects of extended uncertainty principle on the relativistic Coulomb potential. Int. J. Mod. Phys. A 2021, 36, 2150018. [Google Scholar] [CrossRef]
- Hamil, B.; Merad, M.; Birkandan, T. Bound-state solutions of the two-dimensional Dirac equation with Aharonov–Bohm-Coulomb interaction in the presence of extended uncertainty principle. Phys. Scr. 2020, 95, 105307. [Google Scholar] [CrossRef]
- Moradpour, H.; Aghababaei, S.; Ziaie, A.H. A Note on effects of generalized and extended uncertainty principles on Jüttner gas. Symmetry 2021, 13, 213. [Google Scholar] [CrossRef]
- Aghababaei, S.; Moradpour, H.; Vagenas, E.C. Hubble tension bounds the GUP and EUP parameters. Eur. Phys. J. Plus 2021, 136, 997. [Google Scholar] [CrossRef]
- Mureika, J.R. Extended Uncertainty Principle black holes. Phys. Lett. B 2019, 789, 88–92. [Google Scholar] [CrossRef]
- Lu, X.; Xie, Y. Probing an Extended Uncertainty Principle black hole with gravitational lensings. Mod. Phys. Lett. A 2019, 34, 1950152. [Google Scholar] [CrossRef]
- Kumaran, Y.; Övgün, A. Weak deflection angle of extended uncertainty principle black holes. Chin. Phys. C 2020, 44, 025101. [Google Scholar] [CrossRef] [Green Version]
- Cheng, H.; Zhong, Y. Instability of a black hole with f (R) global monopole under extended uncertainty principle. Chin. Phys. C 2021, 45, 105102. [Google Scholar] [CrossRef]
- Hassanabadi, H.; Chung, W.S.; Lütfüoğlu, B.C.; Maghsoodi, E. Effects of a new extended uncertainty principle on Schwarzschild and Reissner–Nordström black holes thermodynamics. Int. J. Mod. Phys. A 2021, 36, 2150036. [Google Scholar] [CrossRef]
- Hamil, B.; Lütfüoğlu, B.C. The effect of higher-order extended uncertainty principle on the black hole thermodynamics. EPL (Europhys. Lett.) 2021, 134, 50007. [Google Scholar] [CrossRef]
- Ökcü, O.; Aydiner, E. Investigating bounds on the extended uncertainty principle metric through astrophysical tests. EPL (Europhys. Lett.) 2022, 138, 39002. [Google Scholar] [CrossRef]
- Pantig, R.C.; Yu, P.K.; Rodulfo, E.T.; Övgün, A. Shadow and weak deflection angle of extended uncertainty principle black hole surrounded with dark matter. Ann. Phys. 2022, 436, 168722. [Google Scholar] [CrossRef]
- Hamil, B.; Lütfüoğlu, B.C.; Dahbi, L. EUP-corrected thermodynamics of BTZ black hole. Int. J. Mod. Phys. A 2022, 37, 2250130. [Google Scholar] [CrossRef]
- Chen, H.; Hassanabadi, H.; Lütfüoğlu, B.C.; Long, Z.W. Quantum corrections to the quasinormal modes of the Schwarzschild black hole. arXiv 2022, arXiv:2203.03464. [Google Scholar] [CrossRef]
- Kempf, A.; Mangano, G.; Mann, R.B. Hilbert space representation of the minimal length uncertainty relation. Phys. Rev. D 1995, 52, 1108–1118. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Calmet, X.; Carr, B.; Winstanley, E. Quantum Black Holes; Springer Briefs in Physics; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar] [CrossRef]
- Hawking, S. Gravitationally collapsed objects of very low mass. Mon. Not. R. Astron. Soc. 1971, 152, 75–78. [Google Scholar] [CrossRef] [Green Version]
- Synge, J.L. The escape of photons from gravitationally intense stars. Mon. Not. R. Astron. Soc. 1966, 131, 463–466. [Google Scholar] [CrossRef]
- Luminet, J.P. Image of a spherical black hole with thin accretion disk. Astron. Astrophys. 1979, 75, 228–235. Available online: https://adsabs.harvard.edu/full/1979A%26A....75..228L (accessed on 1 October 2022).
- Konoplya, R.A. Quantum corrected black holes: Quasinormal modes, scattering, shadows. Phys. Lett. B 2020, 804, 135363. [Google Scholar] [CrossRef]
- Hu, Z.; Zhong, Z.; Li, P.C.; Guo, M.; Chen, B. QED effect on a black hole shadow. Phys. Rev. D 2021, 103, 044057. [Google Scholar] [CrossRef]
- Tamburini, F.; Feleppa, F.; Thidé, B. Constraining the Generalized Uncertainty Principle with the light twisted by rotating black holes and M87*. Phys. Lett. B 2022, 826, 136894. [Google Scholar] [CrossRef]
- Devi, S.; S, A.N.; Chakrabarti, S.; Majhi, B.R. Shadow of quantum extended Kruskal black hole and its super-radiance property. arXiv 2021, arXiv:2105.11847. [Google Scholar] [CrossRef]
- Anacleto, M.A.; Campos, J.A.V.; Brito, F.A.; Passos, E. Quasinormal modes and shadow of a Schwarzschild black hole with GUP. Ann. Phys. 2021, 434, 168662. [Google Scholar] [CrossRef]
- Xu, Z.; Tang, M. Testing the quantum effects near the event horizon with respect to the black hole shadow. Chin. Phys. C 2022, 46, 085101. [Google Scholar] [CrossRef]
- Karmakar, R.; Gogoi, D.J.; Goswami, U.D. Quasinormal modes and thermodynamic properties of GUP-corrected Schwarzschild black hole surrounded by quintessence. arXiv 2022, arXiv:2206.09081. [Google Scholar] [CrossRef]
- Rayimbaev, J.; Pantig, R.C.; Övgün, A.; Abdujabbarov, A.; Demir, D. Quasiperiodic oscillations, weak field lensing and shadow cast around black holes in symmergent gravity. arXiv 2022, arXiv:2206.06599. [Google Scholar] [CrossRef]
- Dyson, F.W.; Eddington, A.S.; Davidson, C. A Determination of the deflection of light by the Sun’s gravitational field, from observations made at the total eclipse of May 29, 1919. Philos. Trans. R. Soc. Lond. A 1920, 220, 291–333. [Google Scholar] [CrossRef]
- Virbhadra, K.S.; Ellis, G.F.R. Schwarzschild black hole lensing. Phys. Rev. D 2000, 62, 084003. [Google Scholar] [CrossRef] [Green Version]
- Bozza, V.; Capozziello, S.; Iovane, G.; Scarpetta, G. Strong field limit of black hole gravitational lensing. Gen. Rel. Grav. 2001, 33, 1535–1548. [Google Scholar] [CrossRef]
- Bozza, V. Gravitational lensing in the strong field limit. Phys. Rev. D 2002, 66, 103001. [Google Scholar] [CrossRef] [Green Version]
- Gibbons, G.W.; Werner, M.C. Applications of the Gauss-Bonnet theorem to gravitational lensing. Class. Quantum Gravity 2008, 25, 235009. [Google Scholar] [CrossRef]
- Werner, M.C. Gravitational lensing in the Kerr-Randers optical geometry. Gen. Rel. Grav. 2012, 44, 3047–3057. [Google Scholar] [CrossRef] [Green Version]
- Ishihara, A.; Suzuki, Y.; Ono, T.; Kitamura, T.; Asada, H. Gravitational bending angle of light for finite distance and the Gauss-Bonnet theorem. Phys. Rev. D 2016, 94, 084015. [Google Scholar] [CrossRef] [Green Version]
- Ishihara, A.; Suzuki, Y.; Ono, T.; Asada, H. Finite-distance corrections to the gravitational bending angle of light in the strong deflection limit. Phys. Rev. D 2017, 95, 044017. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, G.; Övgün, A. Circular orbit of a particle and weak gravitational lensing. Phys. Rev. D 2020, 101, 124058. [Google Scholar] [CrossRef]
- Xu, C.; Yang, Y. Determination of bending angle of light deflection subject to possible weak and strong quantum gravity effects. Int. J. Mod. Phys. A 2020, 35, 2050188. [Google Scholar] [CrossRef]
- Zhang, R.; Lin, J.; Huang, Q. Strong gravitational lensing for the quantum-modified Schwarzschild black hole. Int. J. Theor. Phys. 2021, 60, 387–396. [Google Scholar] [CrossRef]
- Fu, Q.M.; Zhang, X. Gravitational lensing by a black hole in effective loop quantum gravity. Phys. Rev. D 2022, 105, 064020. [Google Scholar] [CrossRef]
- Lu, X.; Xie, Y. Gravitational lensing by a quantum deformed Schwarzschild black hole. Eur. Phys. J. C 2021, 81, 627. [Google Scholar] [CrossRef]
- Jusufi, K.; Övgün, A.; Banerjee, A.; Sakallı, t.I. Gravitational lensing by wormholes supported by electromagnetic, scalar, and quantum effects. Eur. Phys. J. Plus 2019, 134, 428. [Google Scholar] [CrossRef] [Green Version]
- Perlick, V.; Tsupko, O.Y.; Bisnovatyi-Kogan, G.S. Influence of a plasma on the shadow of a spherically symmetric black hole. Phys. Rev. D 2015, 92, 104031. [Google Scholar] [CrossRef] [Green Version]
- Perlick, V.; Tsupko, O.Y.; Bisnovatyi-Kogan, G.S. Black hole shadow in an expanding universe with a cosmological constant. Phys. Rev. D 2018, 97, 104062. [Google Scholar] [CrossRef] [Green Version]
- Pantig, R.C.; Övgün, A. Testing dynamical torsion effects on the charged black hole’s shadow, deflection angle and greybody with M87* and Sgr A* from EHT. arXiv 2022, arXiv:2206.02161. [Google Scholar] [CrossRef]
- Nandi, K.K.; Izmailov, R.N.; Yanbekov, A.A.; Shayakhmetov, A.A. Ring-down gravitational waves and lensing observables: How far can a wormhole mimic those of a black hole? Phys. Rev. D 2017, 95, 104011. [Google Scholar] [CrossRef]
- Eitel, K. Direct neutrino mass experiments. Nucl. Phys. B Proc. Suppl. 2005, 143, 197–204. [Google Scholar] [CrossRef]
- do Carmo, M. Riemannian Geometry; Birkhäuser: Boston, MA, USA, 1992. [Google Scholar]
- Tsukamoto, N. Deflection angle in the strong deflection limit in a general asymptotically flat, static, spherically symmetric spacetime. Phys. Rev. D 2017, 95, 064035. [Google Scholar] [CrossRef] [Green Version]
- Zhao, S.S.; Xie, Y. Strong deflection gravitational lensing by a modified Hayward black hole. Eur. Phys. J. C 2017, 77, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Bisnovatyi-Kogan, G.S.; Tsupko, O.Y. Strong gravitational lensing by Schwarzschild black holes. Astrophysics 2008, 51, 99–111. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lobos, N.J.L.S.; Pantig, R.C. Generalized Extended Uncertainty Principle Black Holes: Shadow and Lensing in the Macro- and Microscopic Realms. Physics 2022, 4, 1318-1330. https://doi.org/10.3390/physics4040084
Lobos NJLS, Pantig RC. Generalized Extended Uncertainty Principle Black Holes: Shadow and Lensing in the Macro- and Microscopic Realms. Physics. 2022; 4(4):1318-1330. https://doi.org/10.3390/physics4040084
Chicago/Turabian StyleLobos, Nikko John Leo S., and Reggie C. Pantig. 2022. "Generalized Extended Uncertainty Principle Black Holes: Shadow and Lensing in the Macro- and Microscopic Realms" Physics 4, no. 4: 1318-1330. https://doi.org/10.3390/physics4040084
APA StyleLobos, N. J. L. S., & Pantig, R. C. (2022). Generalized Extended Uncertainty Principle Black Holes: Shadow and Lensing in the Macro- and Microscopic Realms. Physics, 4(4), 1318-1330. https://doi.org/10.3390/physics4040084





